Performance Improvement of a Liquid Molten Salt Pump: Geometry Optimization and Experimental Verification
Abstract
:1. Introduction
2. Numerical and Experimental Method of Geometry Optimization
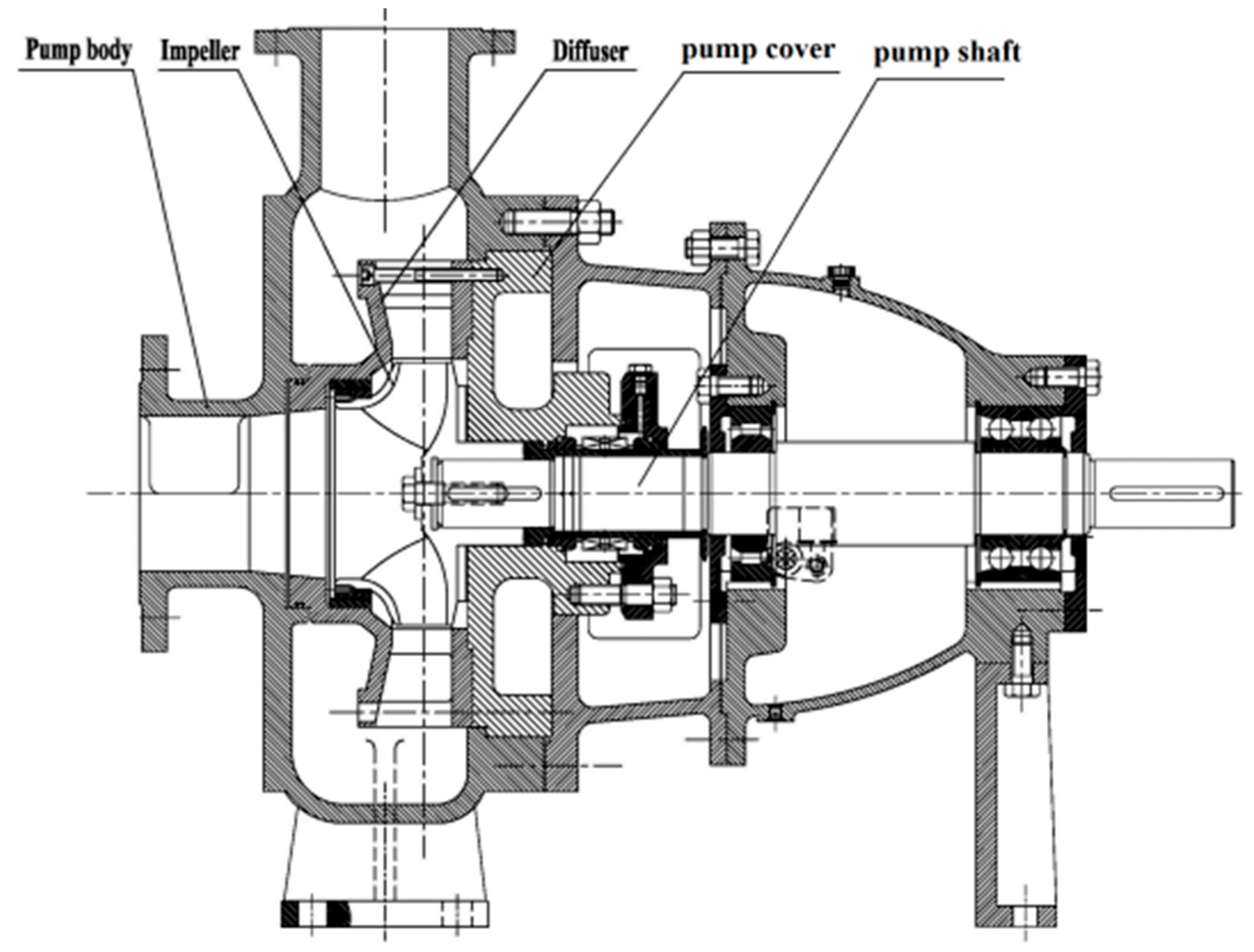
2.1. Prototype Liquid Molten Salt Pump
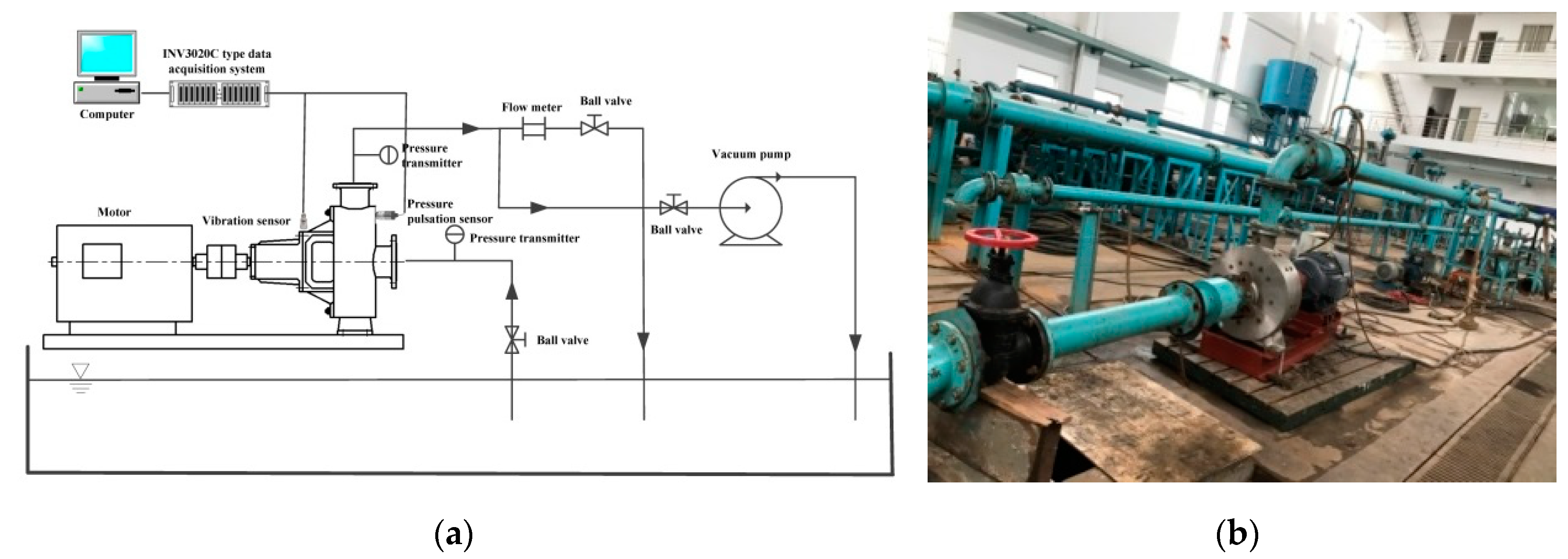
2.2. Performance Experiment
2.3. Numerical Simulation Method
2.3.1. Grid independence Analysis
2.3.2. Boundary Conditions
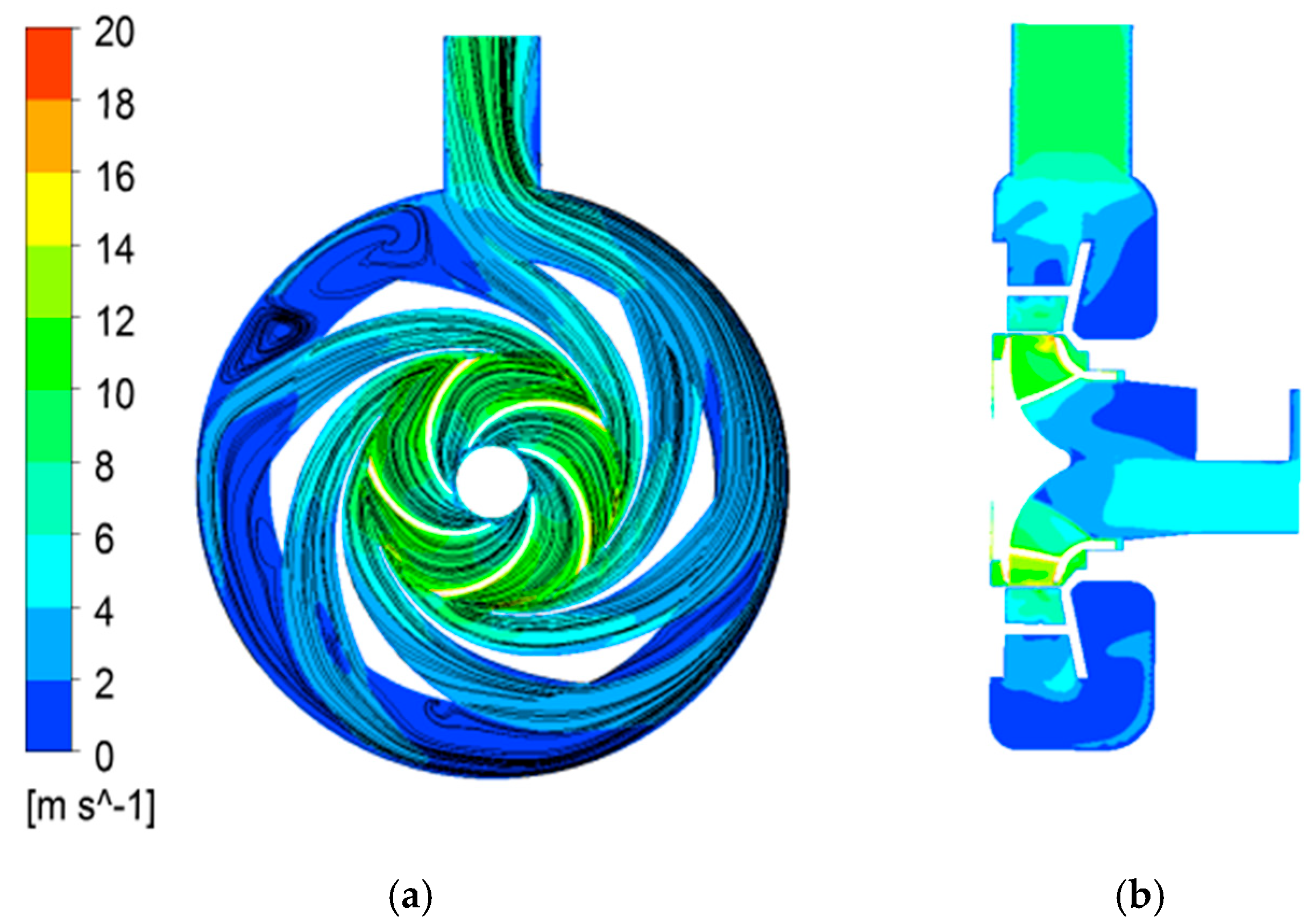
2.4. Analysis of Internal Flow Field of the Prototype Pump
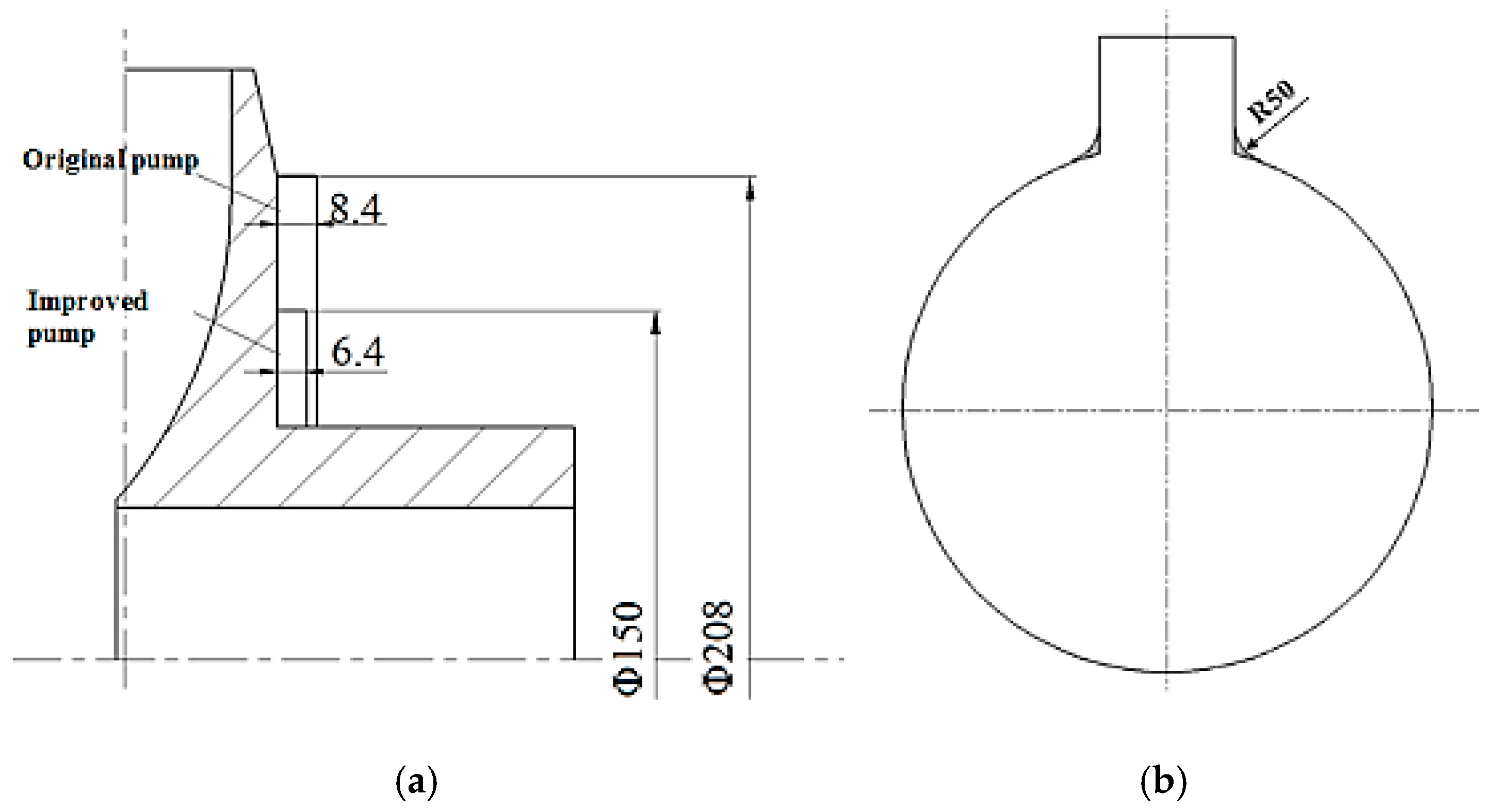
2.5. Geometric Modification
3. Comparison and Analysis of Experiment Results
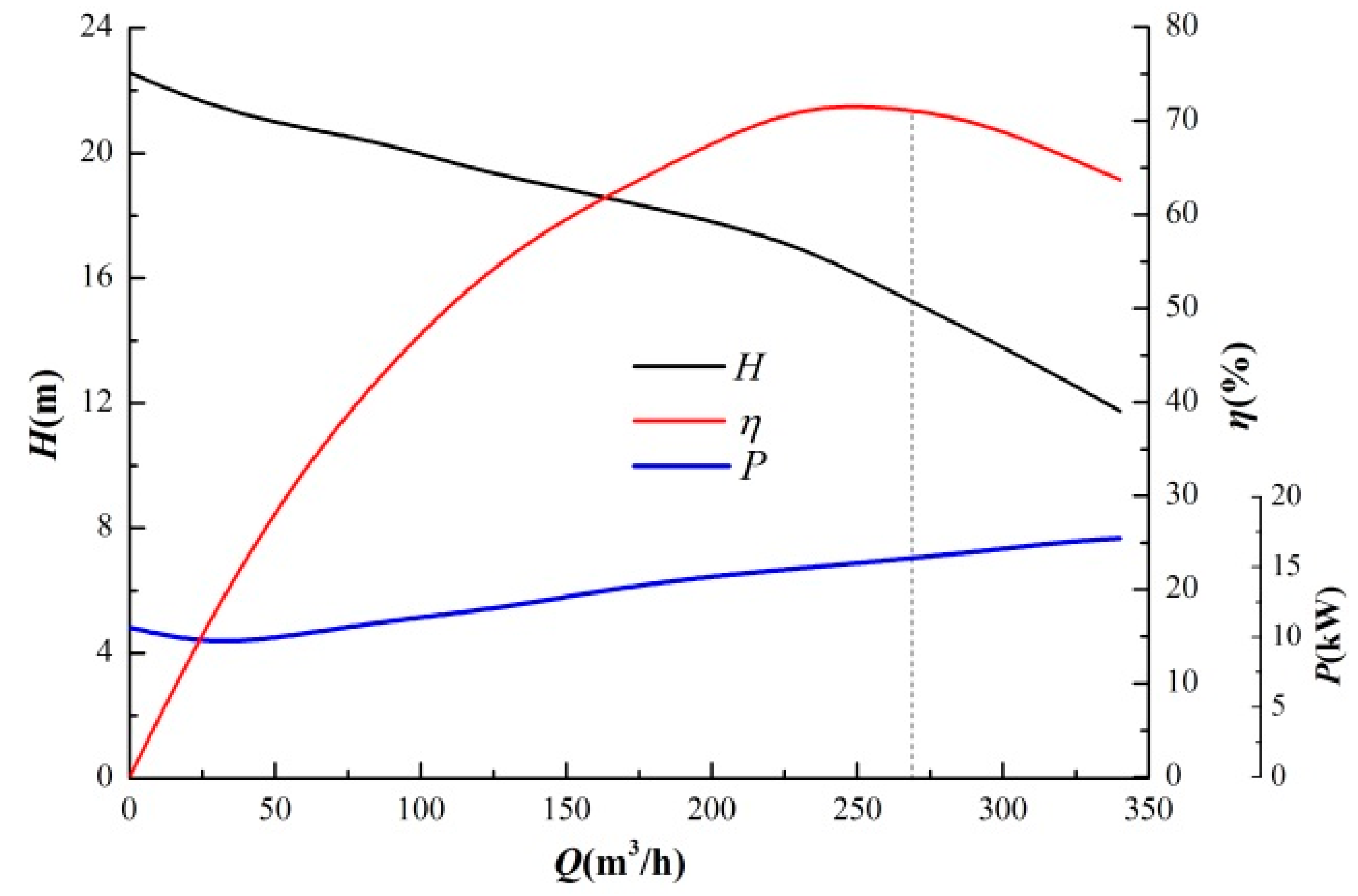
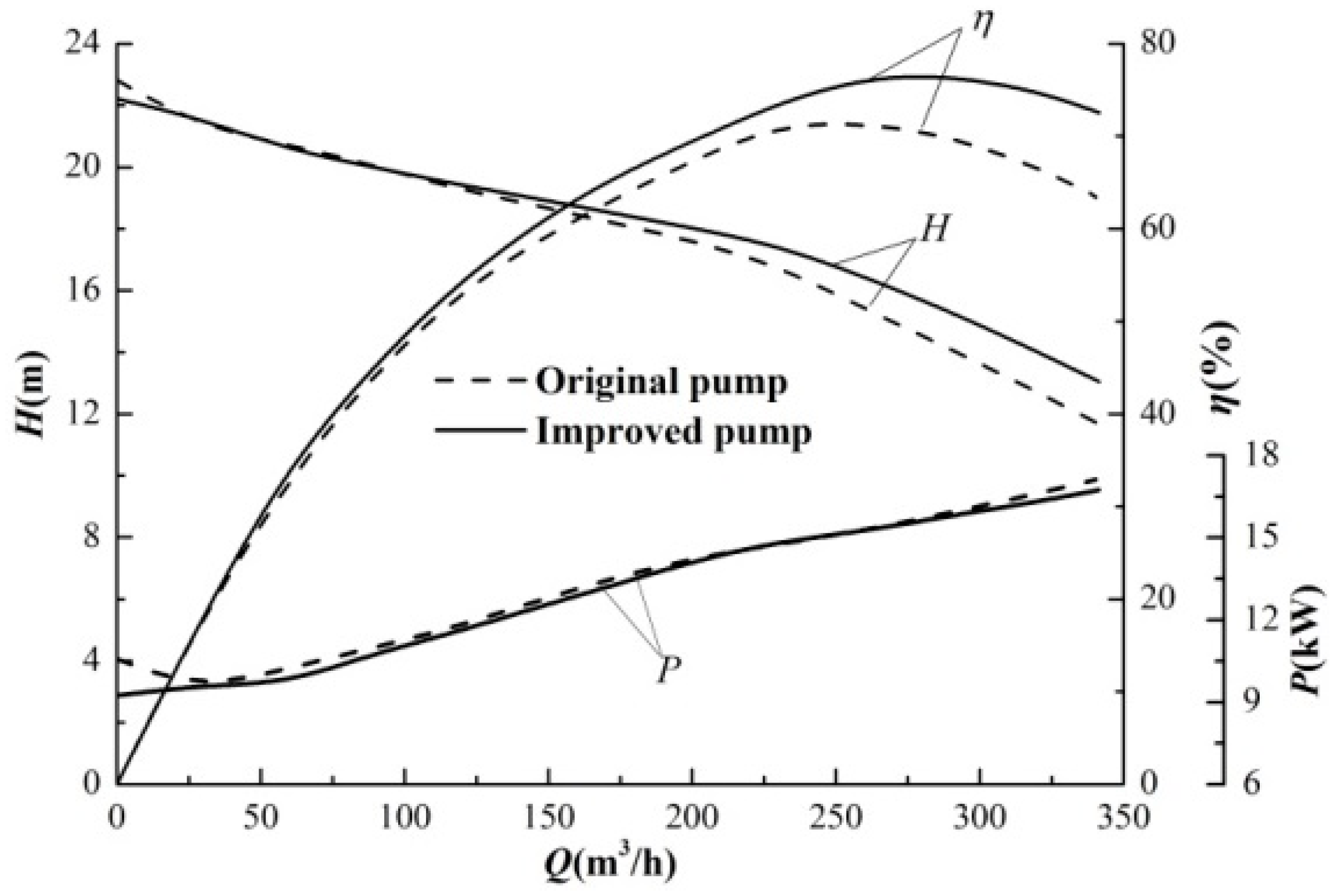
3.1. Energy Performance
3.2. Pressure Pulsation Characteristics
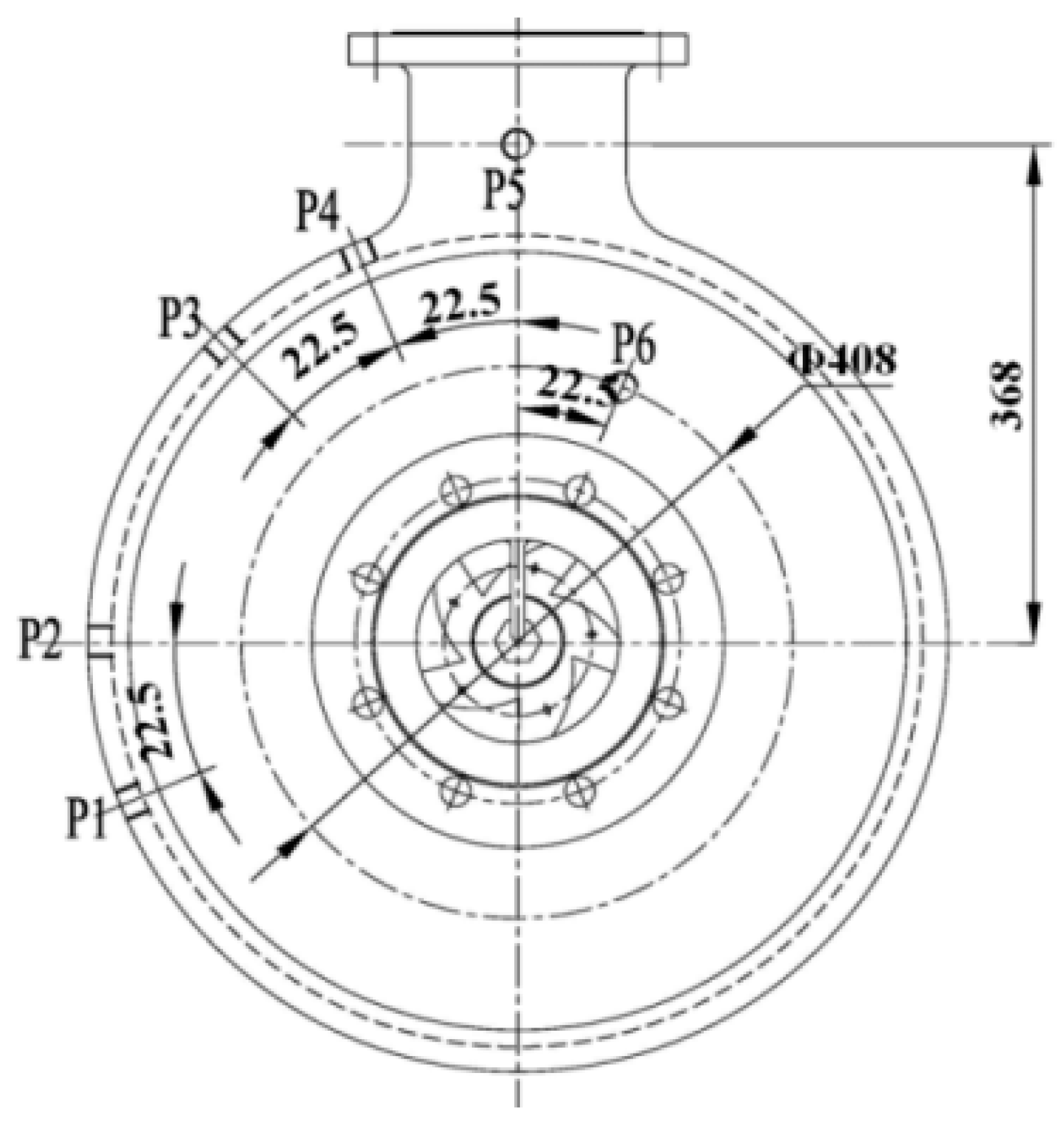
3.2.1. Arrangement of Monitoring Points of Pressure Pulsation
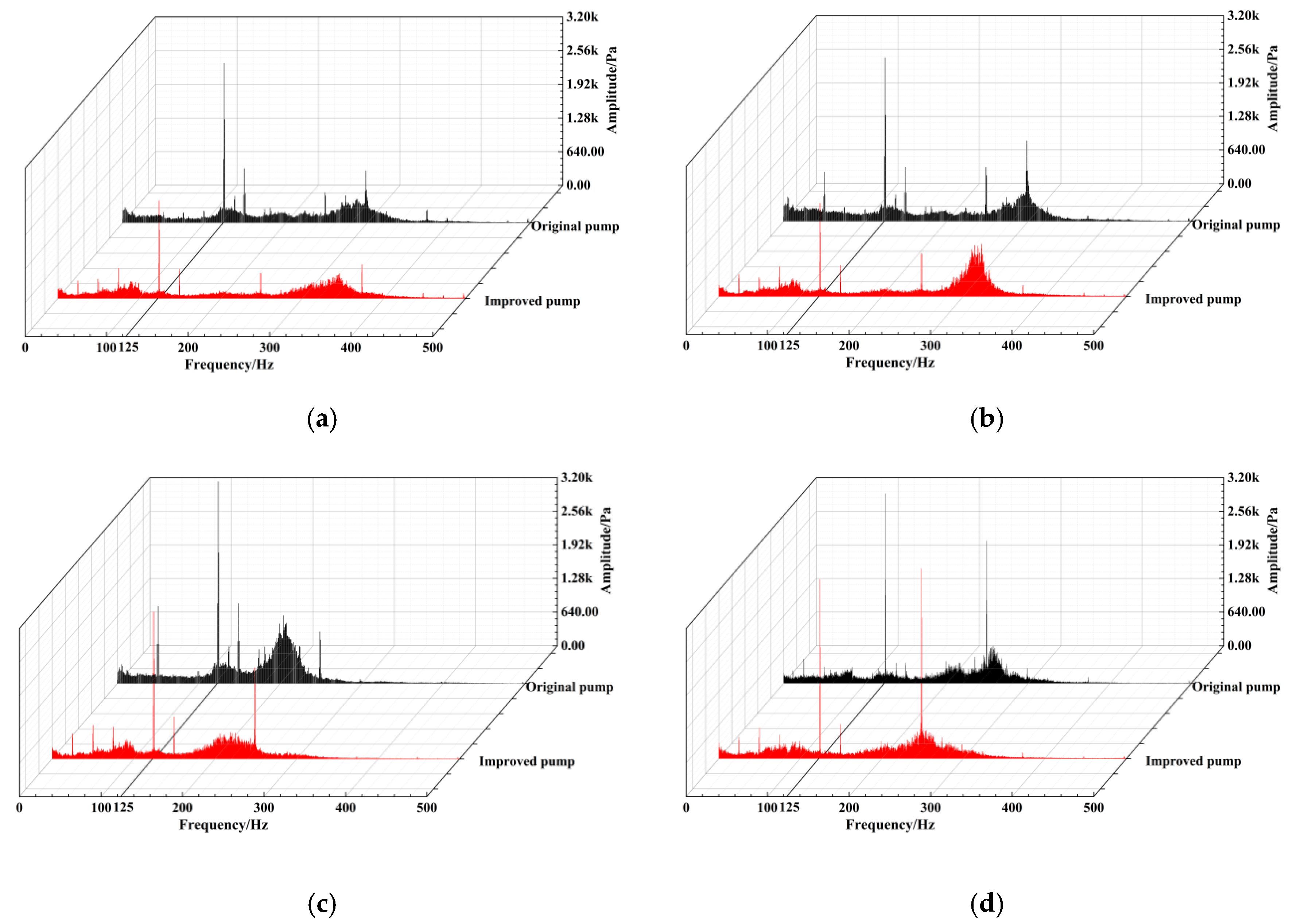
3.2.2. Pressure Pulsation Results at P1–P4
3.2.3. Pressure Pulsation Results at P5
3.2.4. Pressure Pulsation Results at P6
3.3. Vibration Characteristics
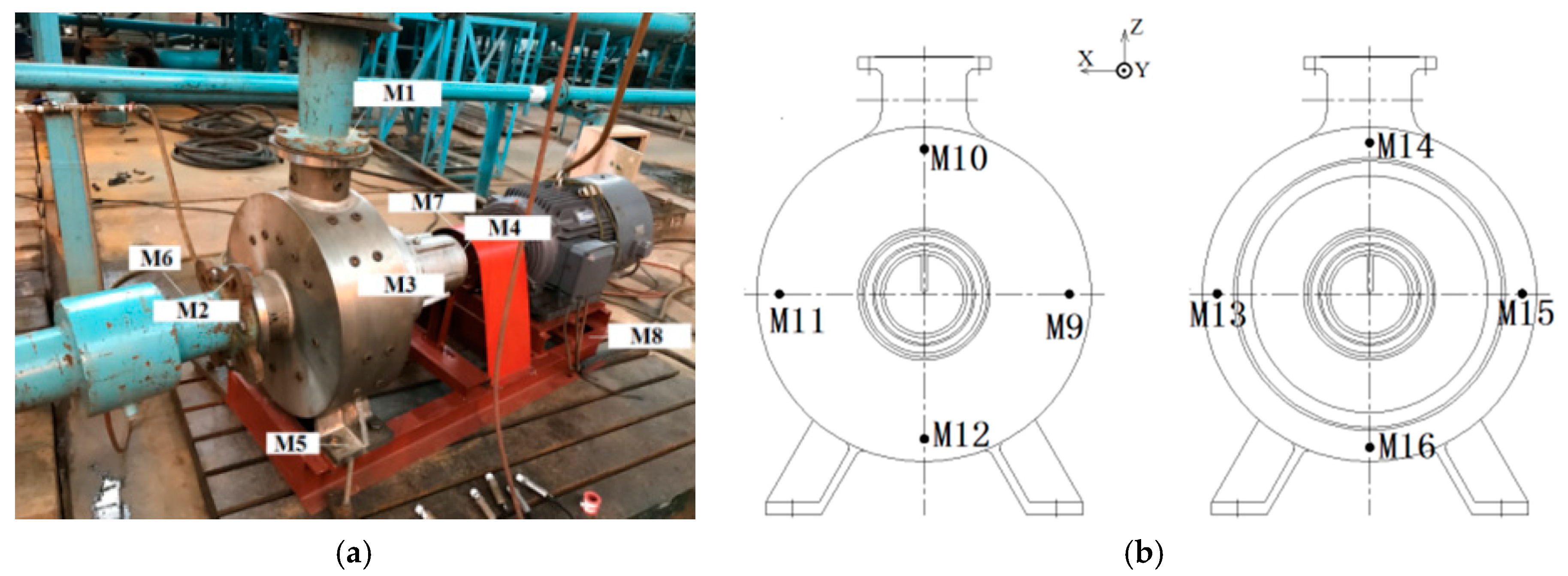
3.3.1. Arrangement of Monitoring Points of Vibration
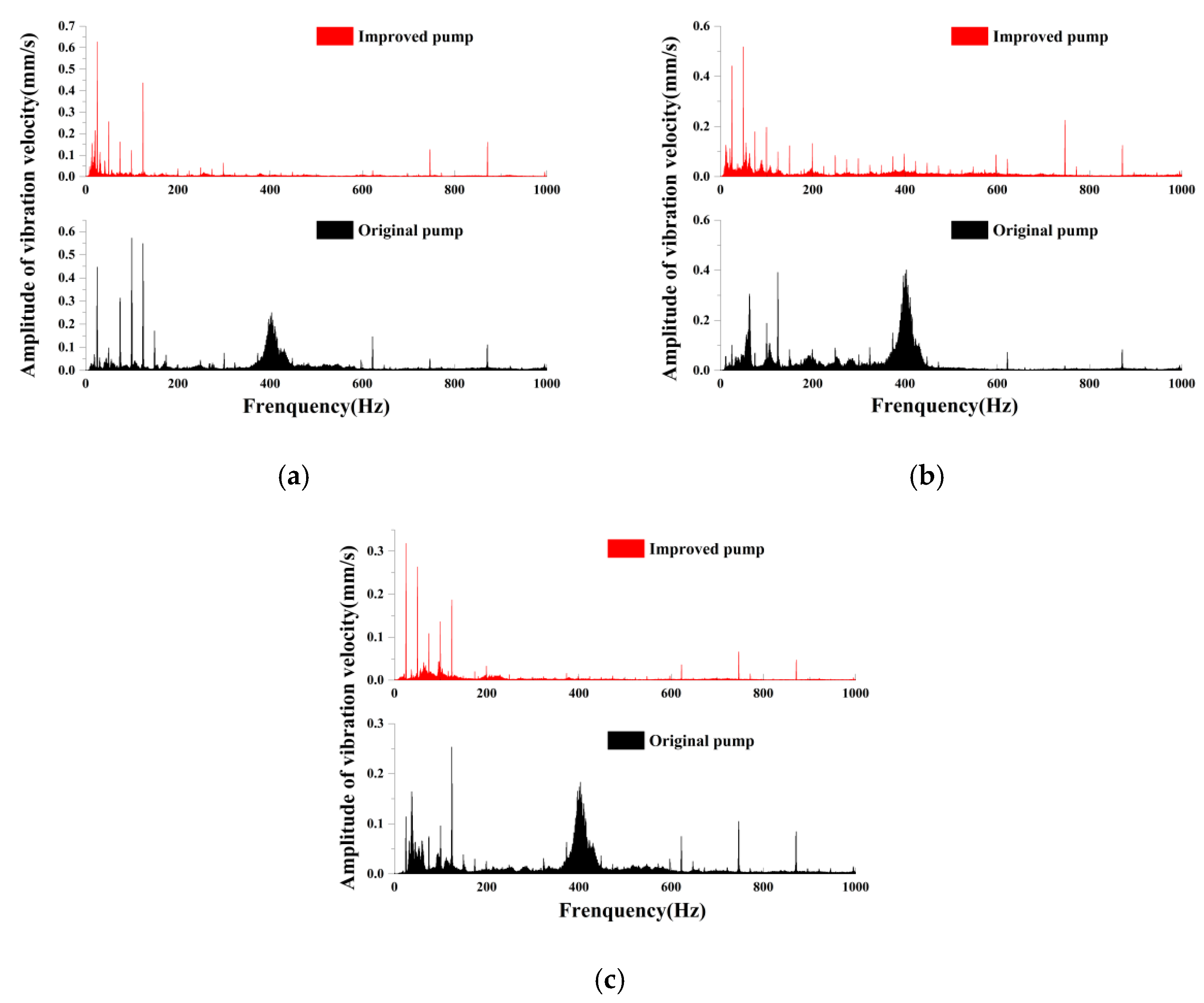
3.3.2. Vibration Results at M1
- (1)
- In the X, Y, and Z directions, the main excitation frequencies of the improved pump at M1 are the axial frequency (25 Hz), twice the axial frequency (50 Hz), 4 times the axial frequency (100 Hz), and the blade-passing frequency (125 Hz), and the maximum frequency of vibration amplitude is the axial frequency.
- (2)
- Vibration of both the original and improved pumps is mainly in the X and Y directions, while the vibration amplitude in the Z direction is the smallest.
- (3)
- Vibration amplitudes of the improved pump in the X, Y, and Z directions increased by 8.7%, 12%, and 8.7%, respectively, from those of the original pump.
- (4)
- Both the original and improved pumps have high-frequency vibration because the outlet flange is connected with the outlet pipe, and high-frequency vibration will be produced by pipeline vibration transmitted to the outlet flange.
- (5)
- The original pump has an evident wide-frequency band between 300–500 Hz. There is no wide-frequency band in the vibration spectrum of the optimized model, because the pressure pulsation (300–500 Hz) at the outlet of the optimized pump is significantly lower than that of the original model.
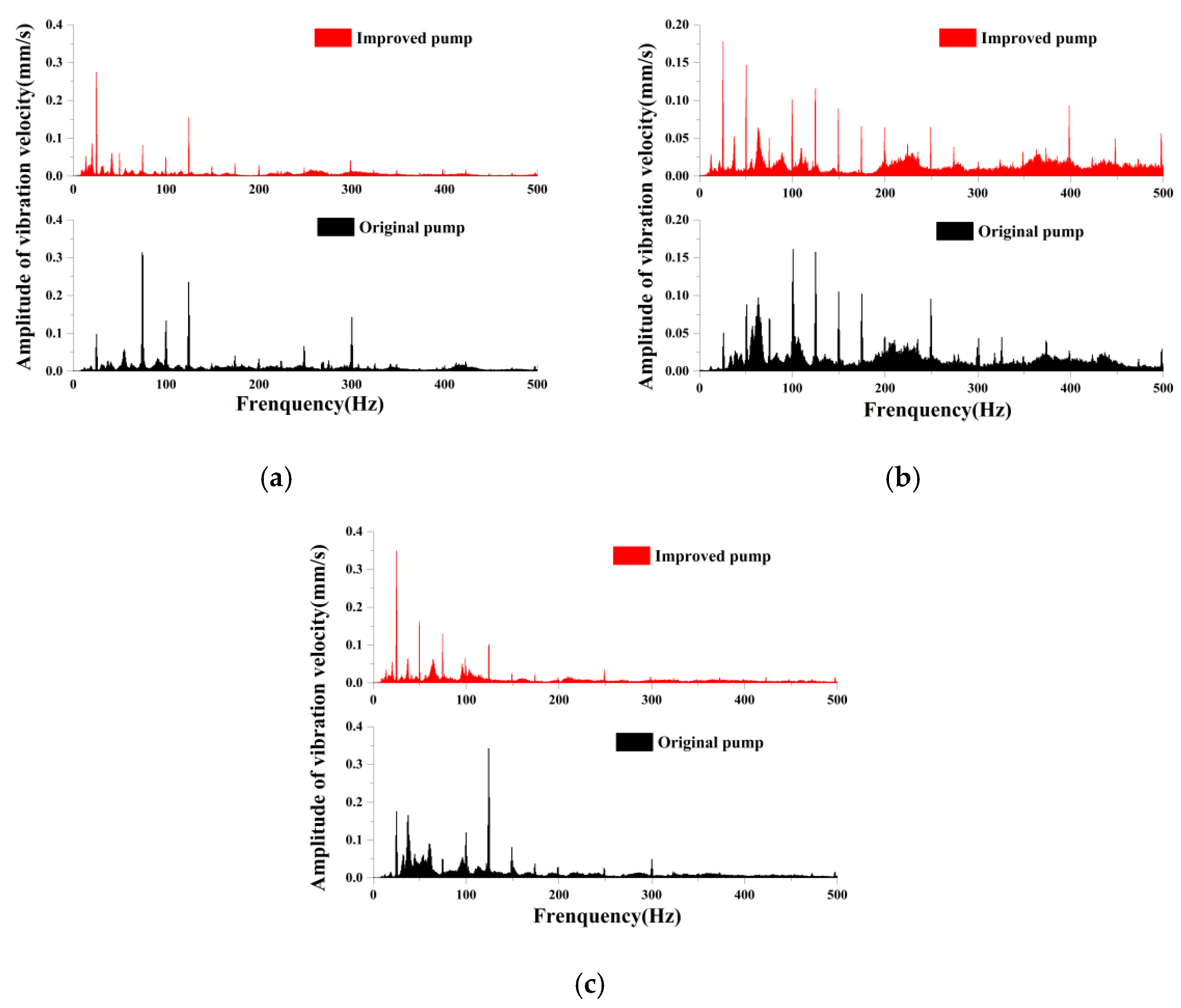
3.3.3. Vibration at M5
- (1)
- In the X, Y, and Z directions, the main excitation frequencies of the improved pump at M5 are the axial frequency (25 Hz), twice the axial frequency (50 Hz), and 4 times the axial frequency (100 Hz). The maximum frequency of vibration amplitude is the axial frequency (25 Hz).
- (2)
- Vibration frequency of both the original and improved pump is mainly in the X and Z directions, while the vibration amplitude in the Y direction is the smallest. But there are wide frequency bands with 50 Hz, 220 Hz, and 400 Hz in the Y direction, which are syntony frequencies.
- (3)
- Vibration amplitudes of the improved pump in the X, Y, and Z directions increased by 29.6%, 1.3%, and 3.1%, respectively, from those of the original pump.
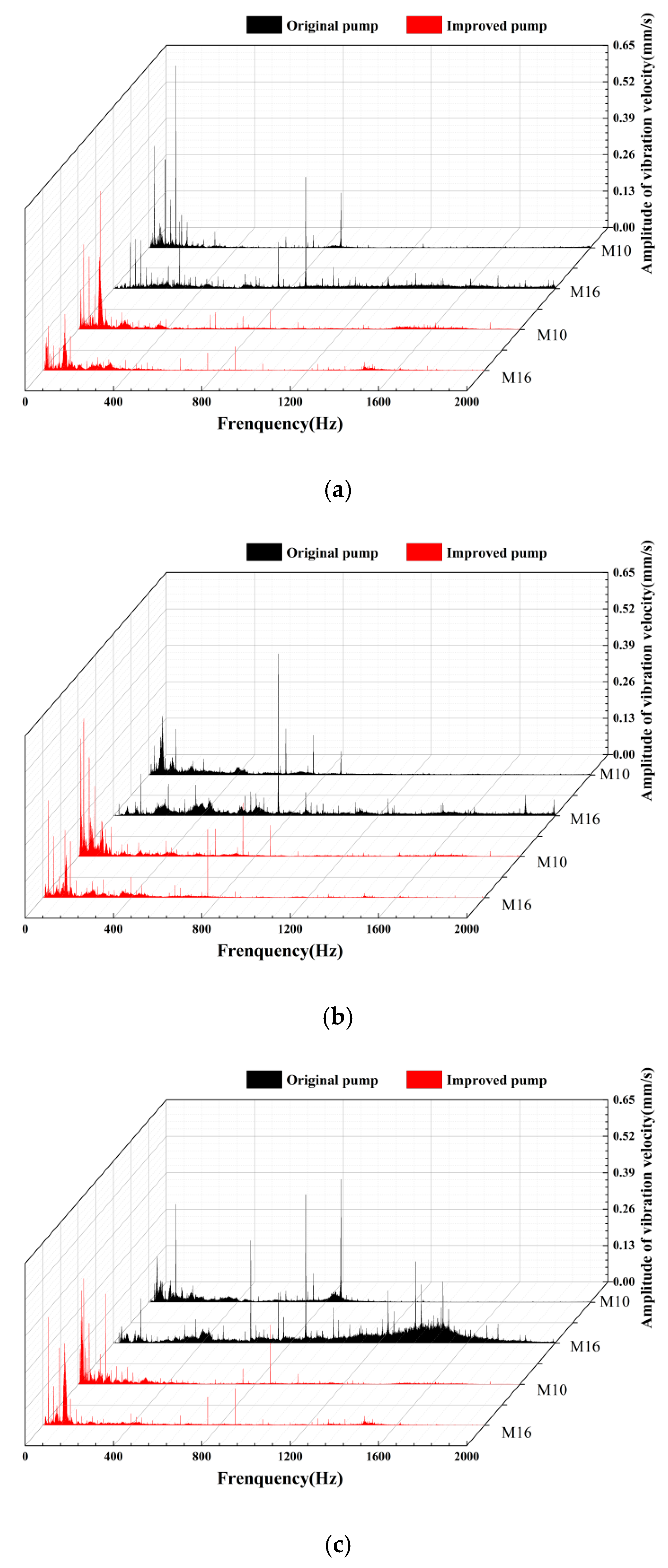
3.3.4. Vibration at M10 and M16
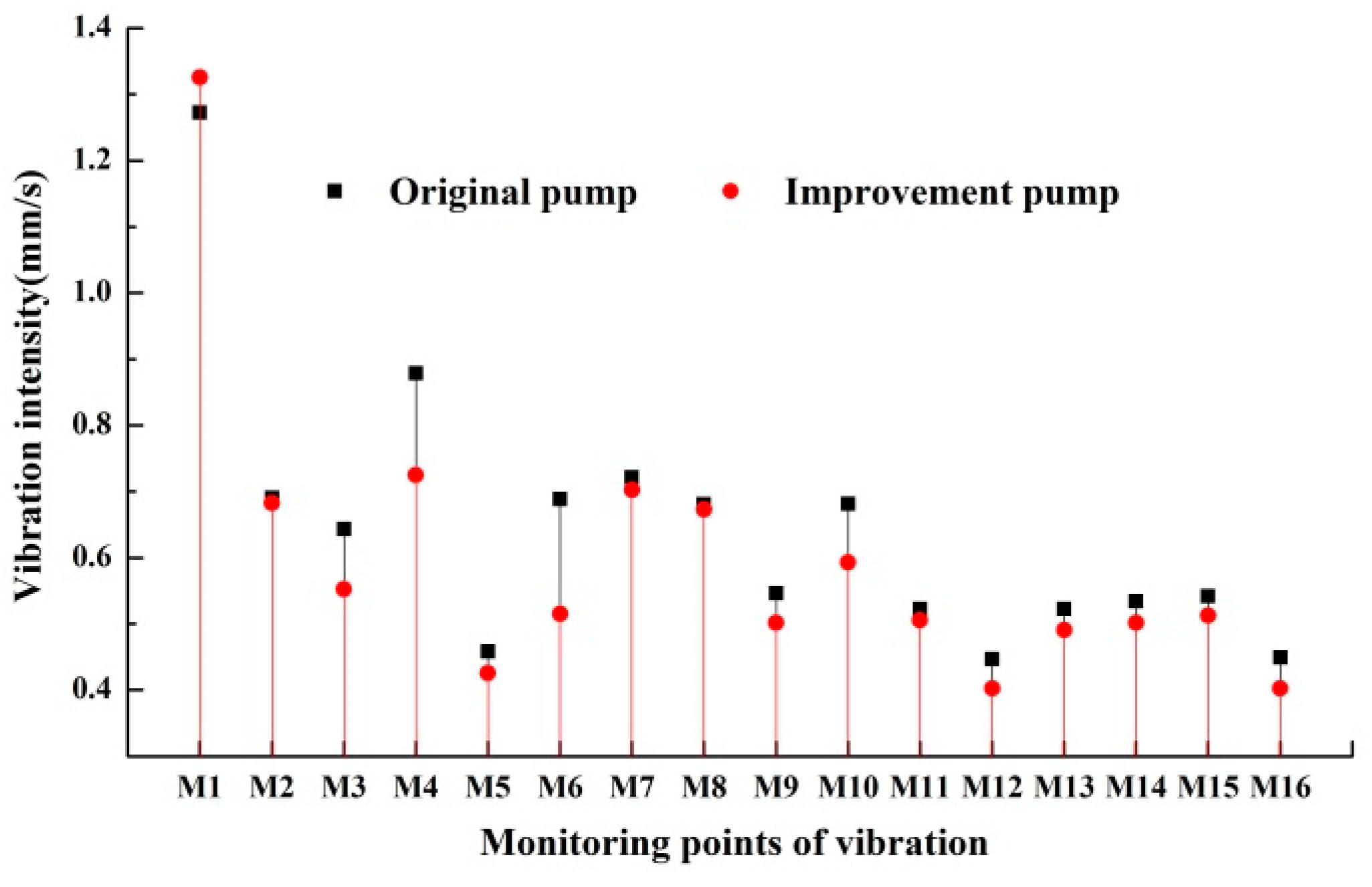
3.3.5. Vibration Intensity at Each Measuring Point
4. Conclusions
- (1)
- Under the design flow rate, the efficiency of the improved pump is 76.7%, which is 5.26 percentage points greater than that of the original pump.
- (2)
- Compared with the original pump, the amplitude of pressure pulsation at points P1, P2, P3, and P4 in the improved pump decrease by 38.9%, 42.9%, 27.1%, and 5.4%, respectively. At P6, the peak-to-peak value of the time domain of pressure pulsation in the improved pump is 71.4% less than in the original pump, and the amplitude of the frequency domain in the improved pump decreases by 32.5%.
- (3)
- The wide frequency band at M1 in the improved pump disappears, and wide frequency bands at other vibration monitoring points also decrease or disappear. The maximum vibration intensity at M4 in the improved pump is 1.04 mm/s, which is 18.1% less than 1.27 mm/s in the original pump.
Author Contributions
Funding
Conflicts of Interest
References
- Shao, C.L.; Zhao, Y. Numerical Study of the Dimensionless Characteristics and Modeling Experiment of a Molten Salt Pump that Transports Viscous Fluids. Int. J. Numer. Methods Heat Fluid Flow 2017, 27, 2131–2153. [Google Scholar] [CrossRef]
- Li, H. Unsteady Numerical Simulation of 3D Turbulent Flow in Molten Salt Pump. Master’s Thesis, Jiangsu University, Zhenjiang, China, 2008. (In Chinese). [Google Scholar]
- Cheng, W.J.; Gu, B.Q.; Shao, C.L.; Wang, Y. Hydraulic Characteristics of Molten Salt Pump Transporting Solid-liquid Two-phase Medium. Nucl. Eng. Des. 2017, 324, 220–230. [Google Scholar] [CrossRef]
- Cheng, W.J.; Gu, B.Q.; Shao, C.L. A Numerical Study on the Steady Flow in Molten Salt Pump under Various Conditions for Improved Hydraulic Performance. Int. J. Numer. Method Heat Fluid Flow 2017, 27, 1870–1886. [Google Scholar] [CrossRef]
- Zhu, Y.; Kang, C.; Li, Q. Investigation of Structural Dynamic Characteristics of Molten-Salt Pump Rotor Based on Fluid-Thermal-Structure Coupling. Chin. J. Process Eng. 2018, 18, 957–964. [Google Scholar]
- Shao, C.L.; Zhou, J.F.; Cheng, W.J. Effect of Viscosity on the External Characteristics and Flow Field of a Molten Salt Pump in the View of Energy Loss. Heat Mass Transf. 2018. [Google Scholar] [CrossRef]
- Wang, L.; Hou, Y.; Shi, L.; Wu, Y.W.; Tian, W.X.; Song, D.K.; Qiu, S.; Su, G.H. Experimental Study and Optimized Design on Electromagnetic Pump for Liquid Sodium. Ann. Nucl. Energy 2019, 124, 426–440. [Google Scholar] [CrossRef]
- Hammitt, F.G. Discussion: Cavitation Damage to Centrifugal Pump Impellers during Operation with Liquid Metals and Molten Salt at 1050-1400F. J. Basic Eng. 1963, 85, 335–336. [Google Scholar] [CrossRef]
- Guo, B. Design and Reliability Analysis of Molten Salt Pump at High Temperature. Master’s Thesis, Jiangsu University, Zhenjiang, China, 2016. (In Chinese). [Google Scholar]
- Yang, M.G.; Shao, T.; Gao, B.; Li, Z.; Wang, D. Interior Flow and Unsteady Performance of Molten Salt Pump with Splitter Space Guide Vane. J. Drain. Irrig. Mach. Eng. 2015, 33, 306–310. [Google Scholar]
- Wang, Y.F.; Zhang, J.F.; Yuan, S.Q.; Huang, X.; Zhang, X. Influence of Splitter Blades in the Structural Dynamic Characteristics on a High-temperature Molten Salt Pump. Fluid Mach. 2016, 44, 38–44. [Google Scholar]
- Shao, C.L.; Zhou, J.F.; Gu, B.Q.; Cheng, W.J. Experimental Investigation of the Full Flow Field in a Molten Salt Pump by Particle Image Velocimetry. J. Fluids Eng. 2015, 137, 104501. [Google Scholar] [CrossRef]
- Kang, C.; Zhou, L.; Wang, W.F.; Yang, M.G. Influence of Axial Vane on Inner Flow and Performance of a Molten-salt Pump. In Proceedings of the ASME-JSME-KSME 2011 Joint Fluids Engineering Conference, Hamamatsu, Japan, 24–29 July 2011; pp. 249–255. [Google Scholar]
- Shao, C.L.; Zhou, J.F.; Cheng, W.J. Experimental and Numerical Study of External Performance and Internal Flow of a Molten Salt Pump that Transports Fluids with Different Viscosities. Int. J. Heat Mass Transf. 2015, 89, 627–640. [Google Scholar] [CrossRef]
- Yang, M.G.; Gao, B.; Jia, W.D.; Wang, C.L.; Wu, N.X. Analysis of Cooling and Lubricating System of Bearings in Pumps for Melted Salts. J. Jiangsu Univ. (Nat. Sci. Ed.) 2005, 26, 149–152. [Google Scholar]
- Wang, Z.; Naterer, G.F.; Gabriel, K.S.; Secnik, E.; Gravelsins, R.; Daggupati, V. Thermal Design of a Solar Hydrogen Plant with a Copper–Chlorine Cycle and Molten Salt energy Storage. Int. J. Hydrog. Energy 2011, 36, 11258–11272. [Google Scholar] [CrossRef]





| The pressure Pulsation Sensor | The Vibration Sensor | ||
|---|---|---|---|
| Category | Parameter | Category | Parameter |
| Scope of measurement | 0–1 Mpa | Scope of measurement | 0.5–8 kHz |
| Precision | 0.25% FS | Sensitivity | 10 mV/ms−2 |
| Scope of measurement | 0–2 kHz | Installation resonance frequency | 25 kHz |
| Operating temperature | −10–80 °C | Verification conditions | Temperature 20 °C Humidity 50% |
| Parameter | Flow Rate | Head | Speed | Vibration |
|---|---|---|---|---|
| Random uncertainty | ±0.02 | ±0.03 | ±0.01 | ±0.19 |
| Systematic uncertainty | ±0.22 | ±0.24 | ±0.08 | ±0.11 |
| Uncertainty | ±0.22 | ±0.24 | ±0.08 | ±0.22 |
| Standard | ±2.00 | ±1.50 | ±0.40 | ±3.60 |
| No. | Grid Number | Head (m) | Efficiency (%) |
|---|---|---|---|
| 1 | 15241854 | 16.05 | 72.98 |
| 2 | 48210231 | 16.08 | 73.06 |
| 3 | 50143163 | 16.10 | 73.18 |
| 4 | 52473702 | 16.11 | 73.22 |
| 5 | 61016470 | 16.11 | 73.23 |
| Direction | Flow Rate | Original Pump | Improved Pump | ||
|---|---|---|---|---|---|
| M3 | M4 | M3 | M4 | ||
| X | 0.8Qd | 0.63 | 1.27 | 0.61 | 1.04 |
| 1.0Qd | 0.56 | 0.85 | 0.53 | 0.79 | |
| 1.2Qd | 0.53 | 0.74 | 0.48 | 0.67 | |
| Y | 0.8Qd | 0.59 | 0.62 | 0.55 | 0.53 |
| 1.0Qd | 0.52 | 0.53 | 0.49 | 0.45 | |
| 1.2Qd | 0.41 | 0.45 | 0.35 | 0.40 | |
| Z | 0.8Qd | 0.63 | 0.93 | 0.60 | 0.88 |
| 1.0Qd | 0.64 | 0.88 | 0.62 | 0.81 | |
| 1.2Qd | 0.59 | 0.83 | 0.56 | 0.75 | |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, K.; Lu, X.; Li, Y.; He, X.; Liu, H.; Kim, H.-B. Performance Improvement of a Liquid Molten Salt Pump: Geometry Optimization and Experimental Verification. Symmetry 2019, 11, 423. https://doi.org/10.3390/sym11030423
Wang K, Lu X, Li Y, He X, Liu H, Kim H-B. Performance Improvement of a Liquid Molten Salt Pump: Geometry Optimization and Experimental Verification. Symmetry. 2019; 11(3):423. https://doi.org/10.3390/sym11030423
Chicago/Turabian StyleWang, Kai, Xin Lu, Yu Li, Xianghui He, Houlin Liu, and Hyoung-Bum Kim. 2019. "Performance Improvement of a Liquid Molten Salt Pump: Geometry Optimization and Experimental Verification" Symmetry 11, no. 3: 423. https://doi.org/10.3390/sym11030423
APA StyleWang, K., Lu, X., Li, Y., He, X., Liu, H., & Kim, H.-B. (2019). Performance Improvement of a Liquid Molten Salt Pump: Geometry Optimization and Experimental Verification. Symmetry, 11(3), 423. https://doi.org/10.3390/sym11030423





