Questions of Mirror Symmetry at the Photoexcited and Ground States of Non-Rigid Luminophores Raised by Circularly Polarized Luminescence and Circular Dichroism Spectroscopy. Part 2: Perylenes, BODIPYs, Molecular Scintillators, Coumarins, Rhodamine B, and DCM
Abstract
1. Introduction
2. Results
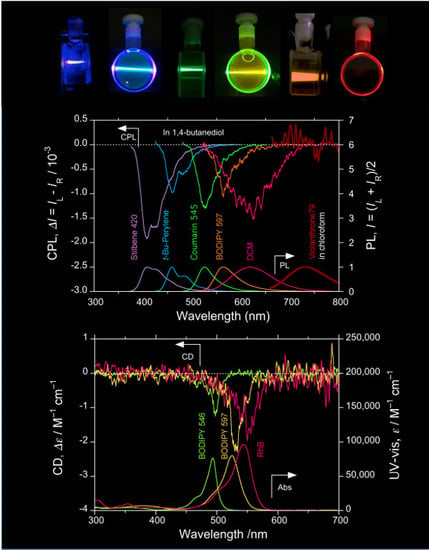
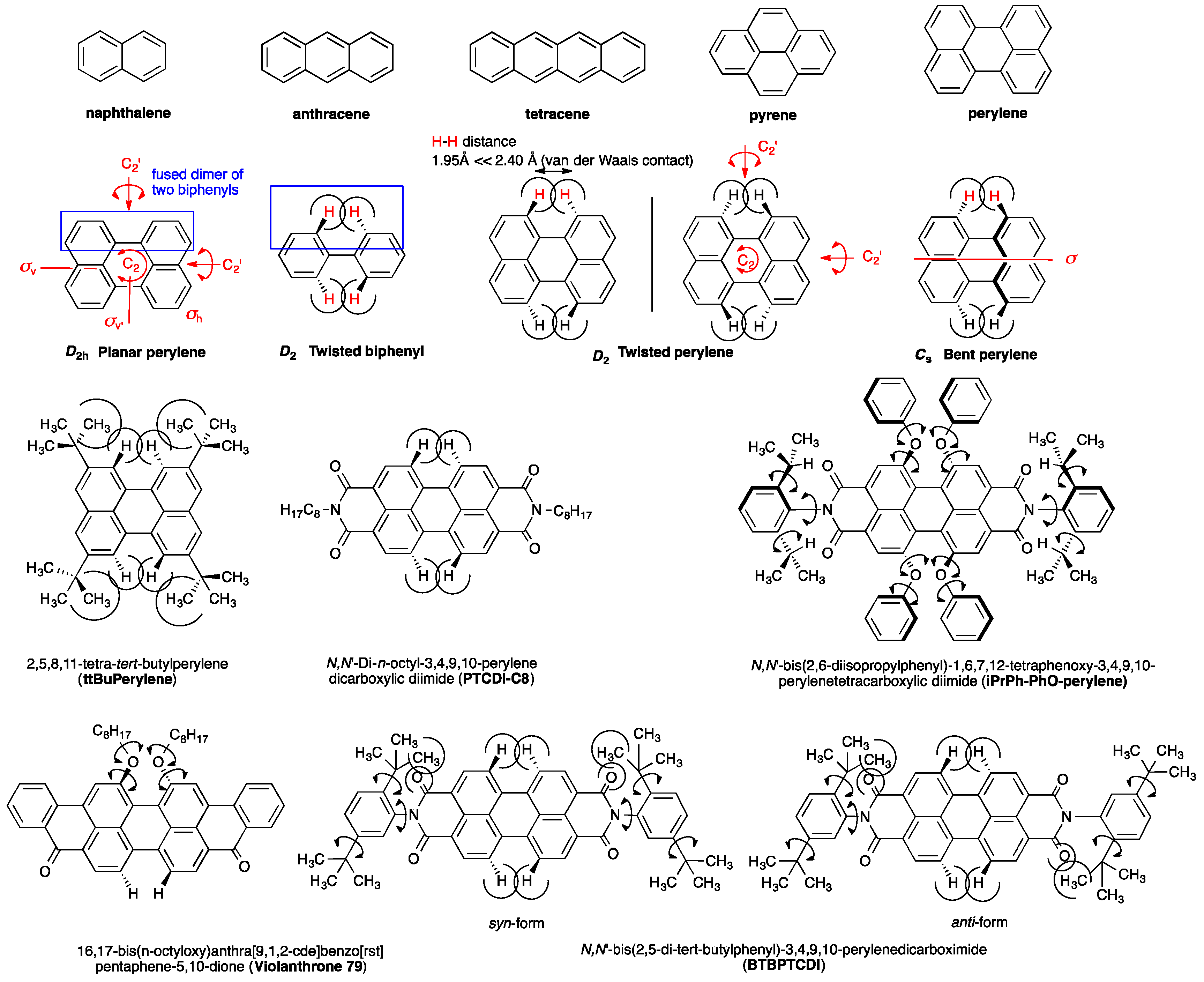
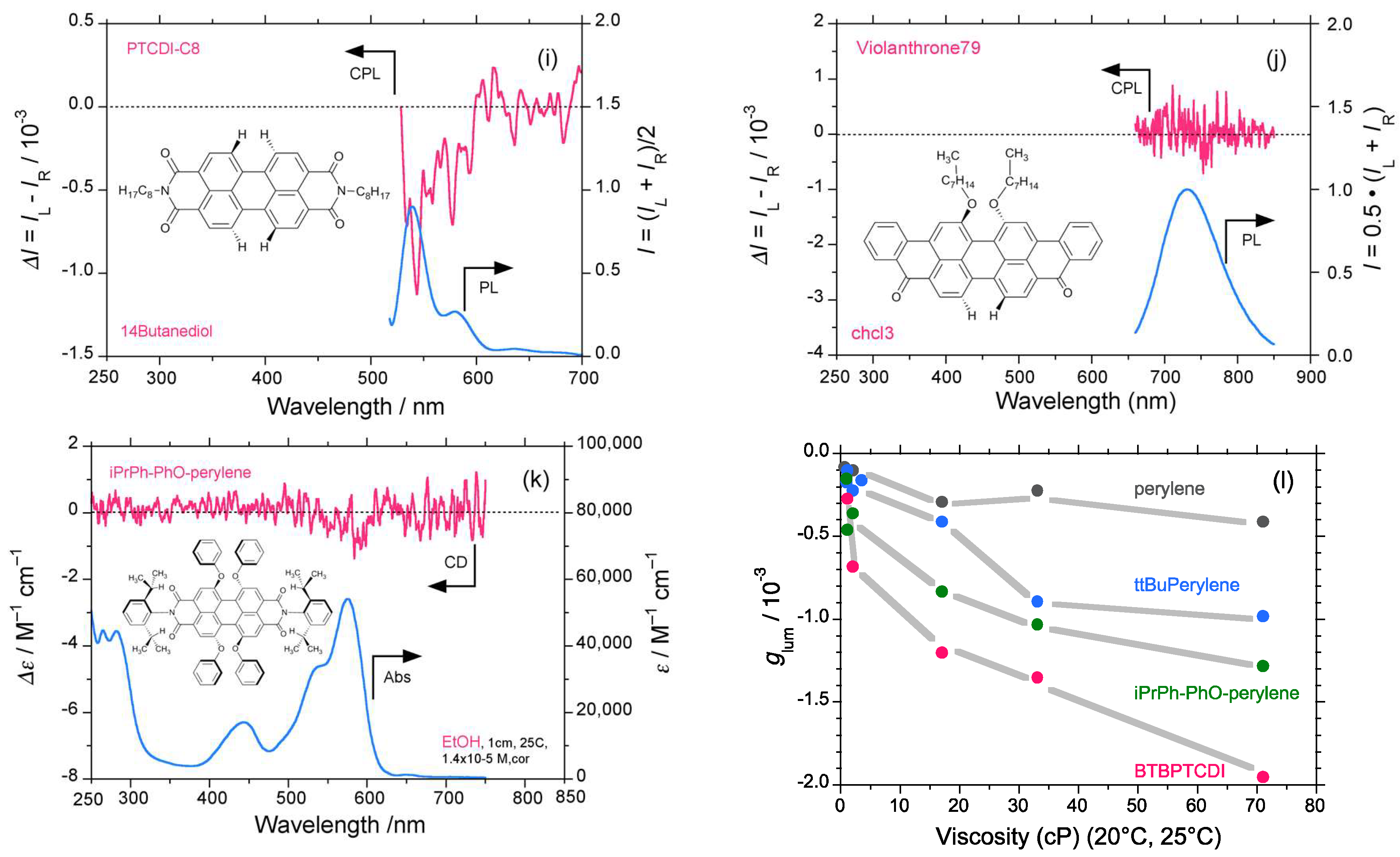
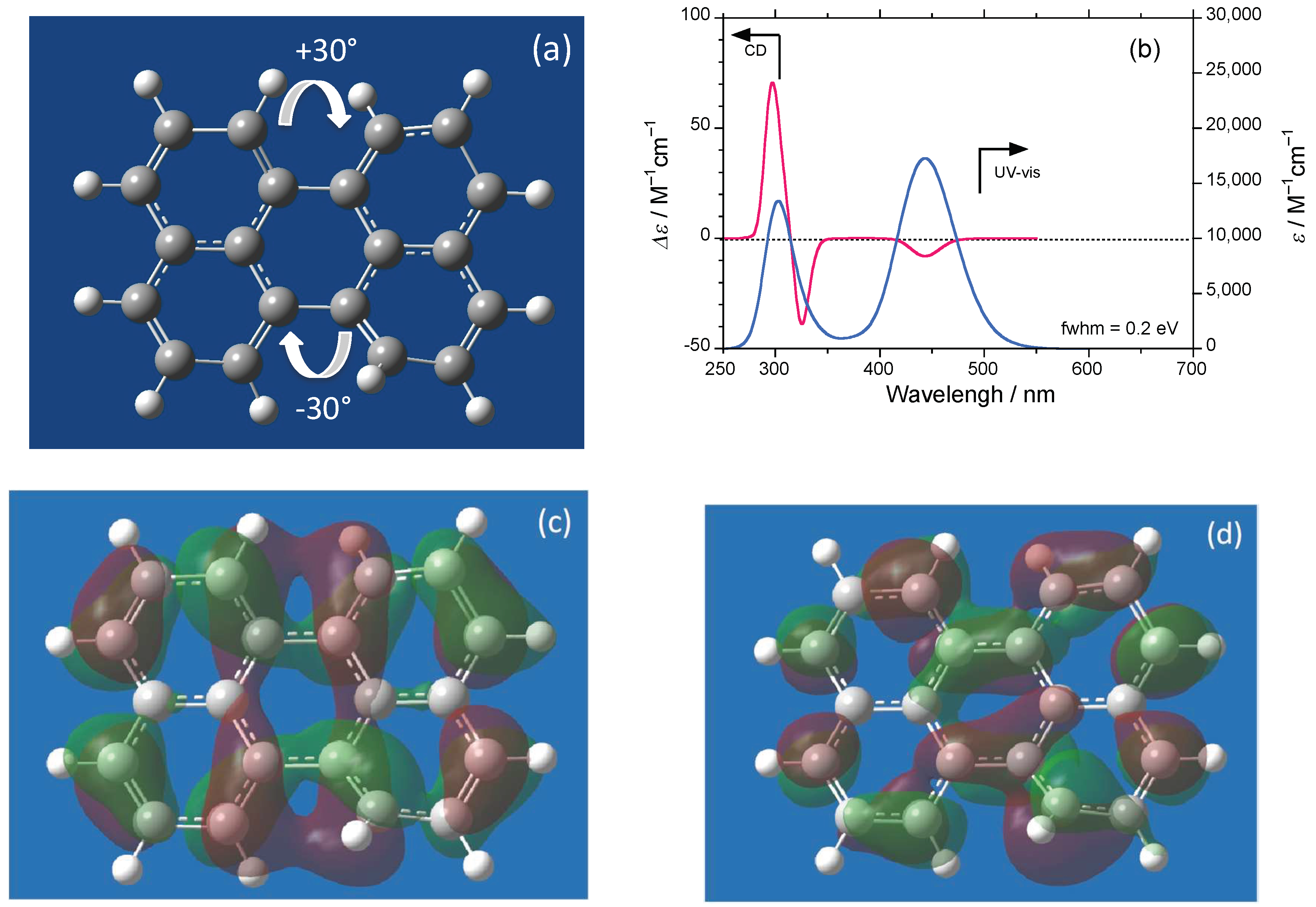
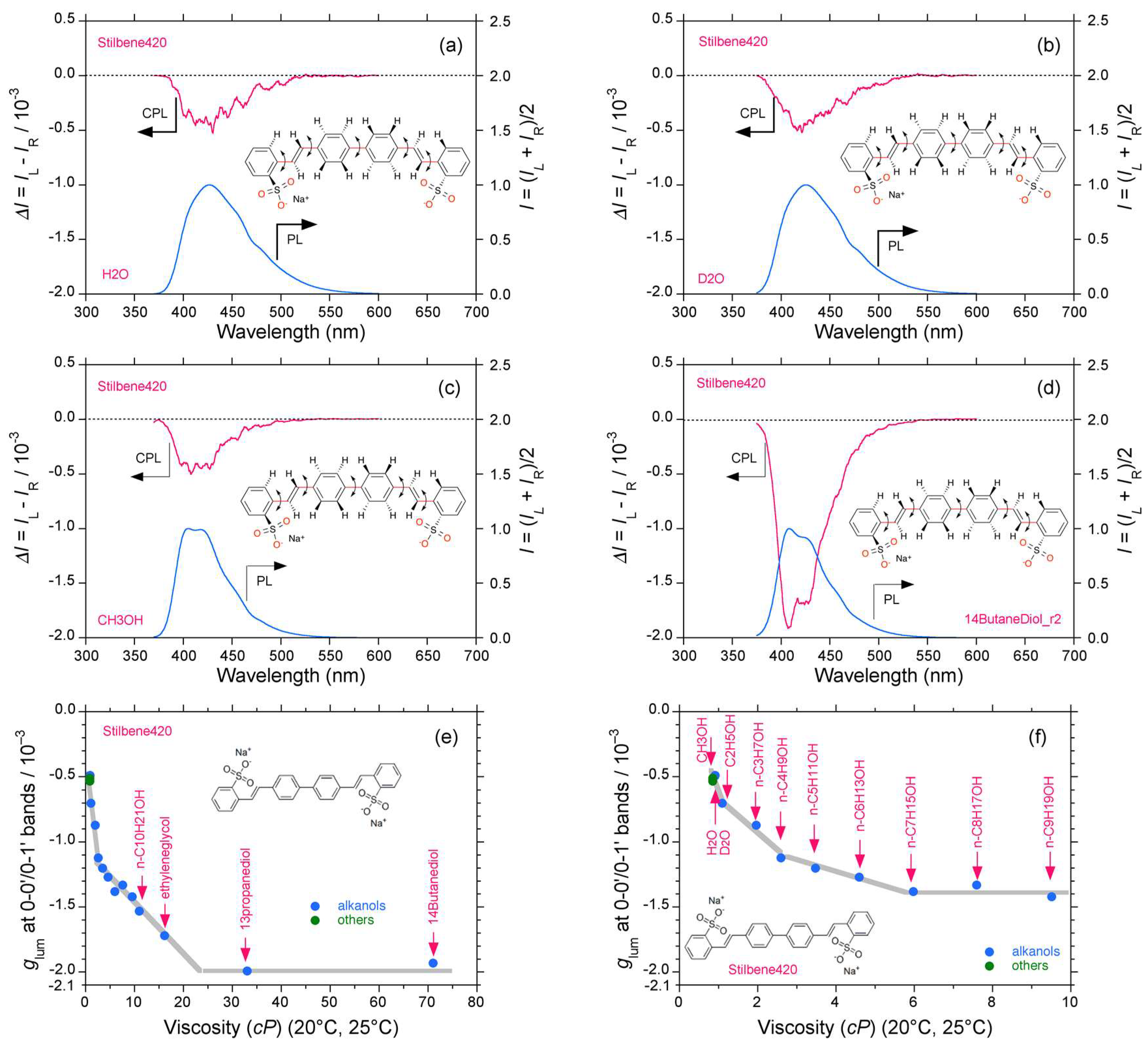
2.1. D2h/D2 Symmetrical Perylene and C2/C1 Symmetrical Derivatives
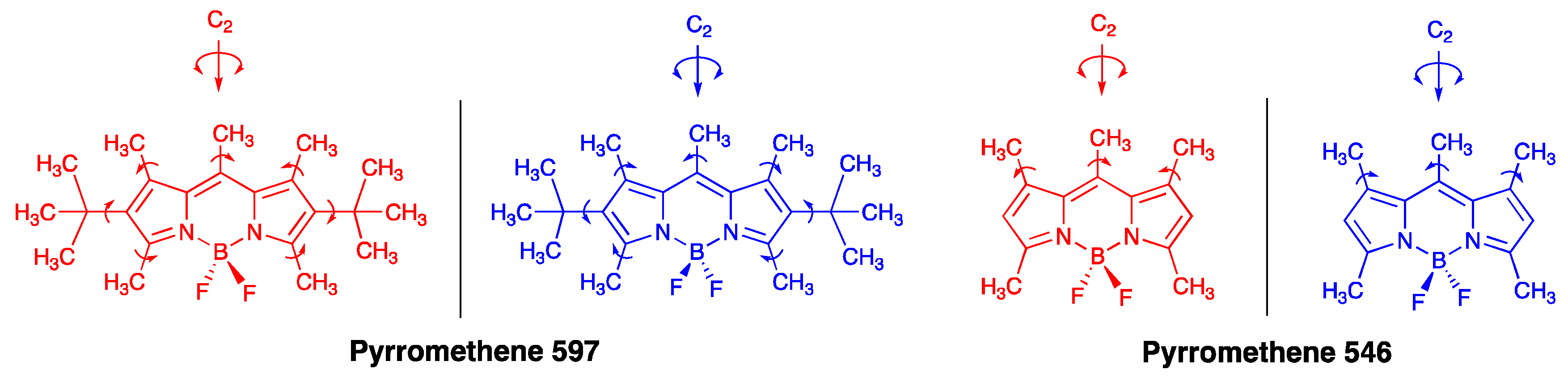
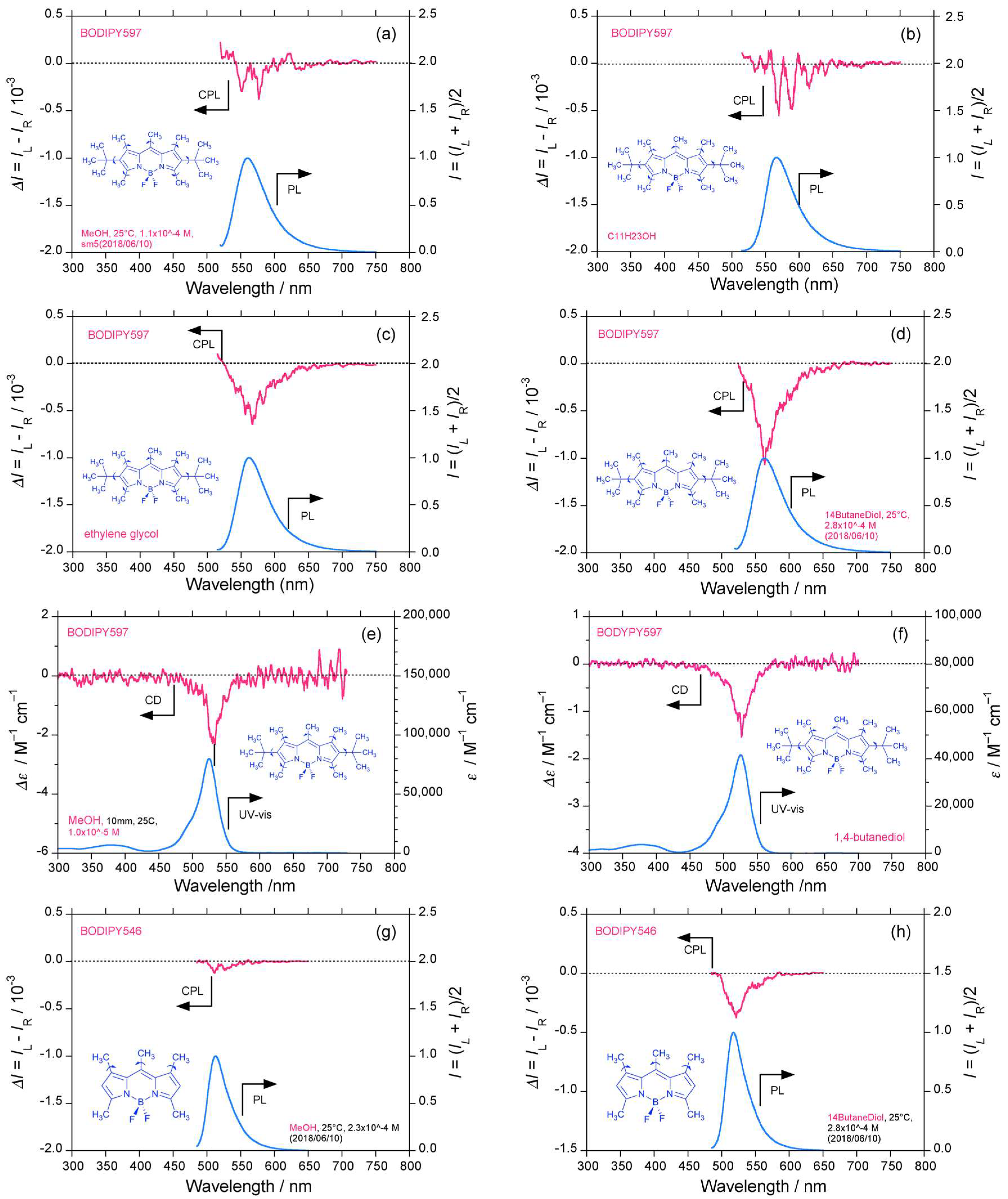
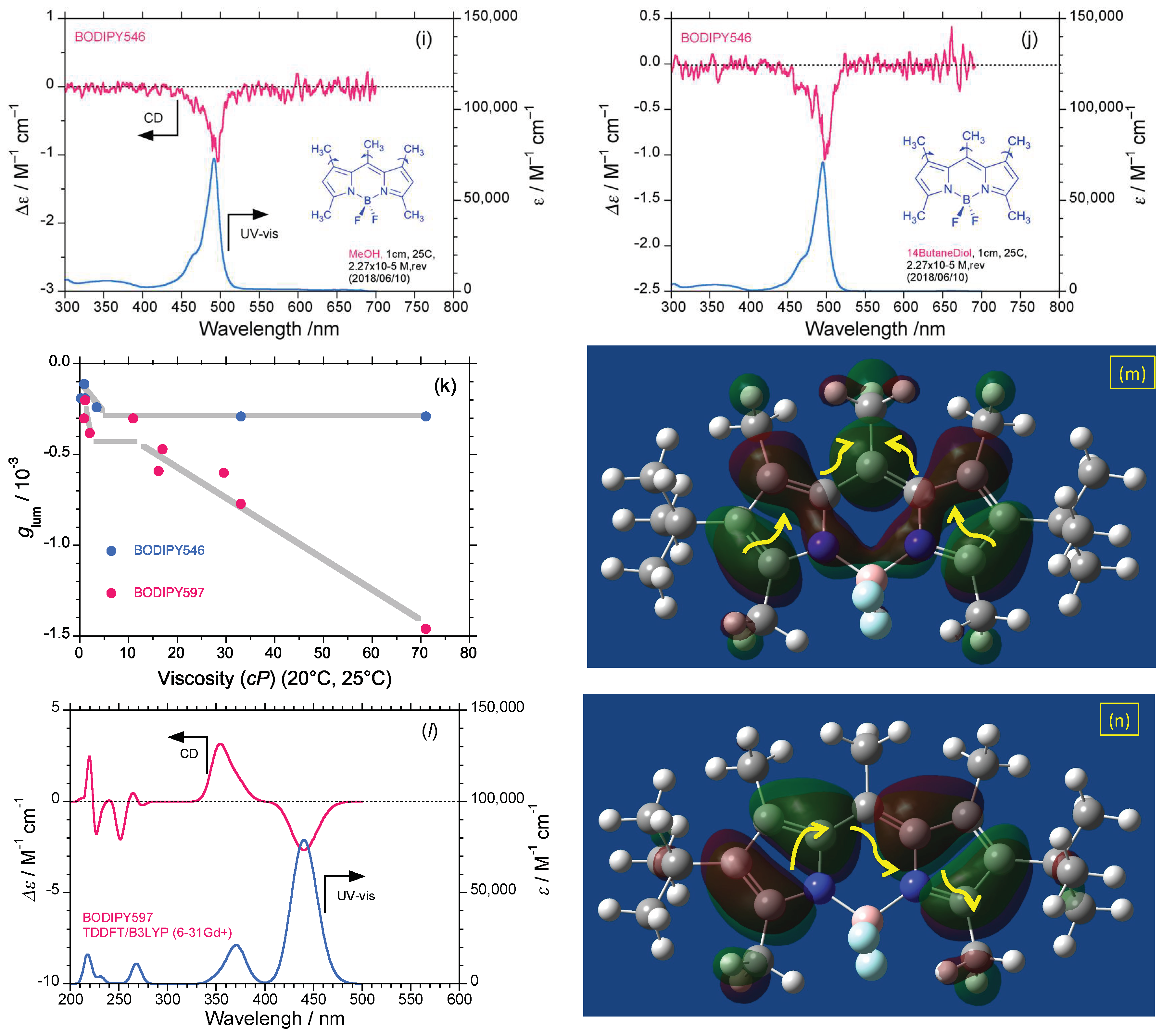
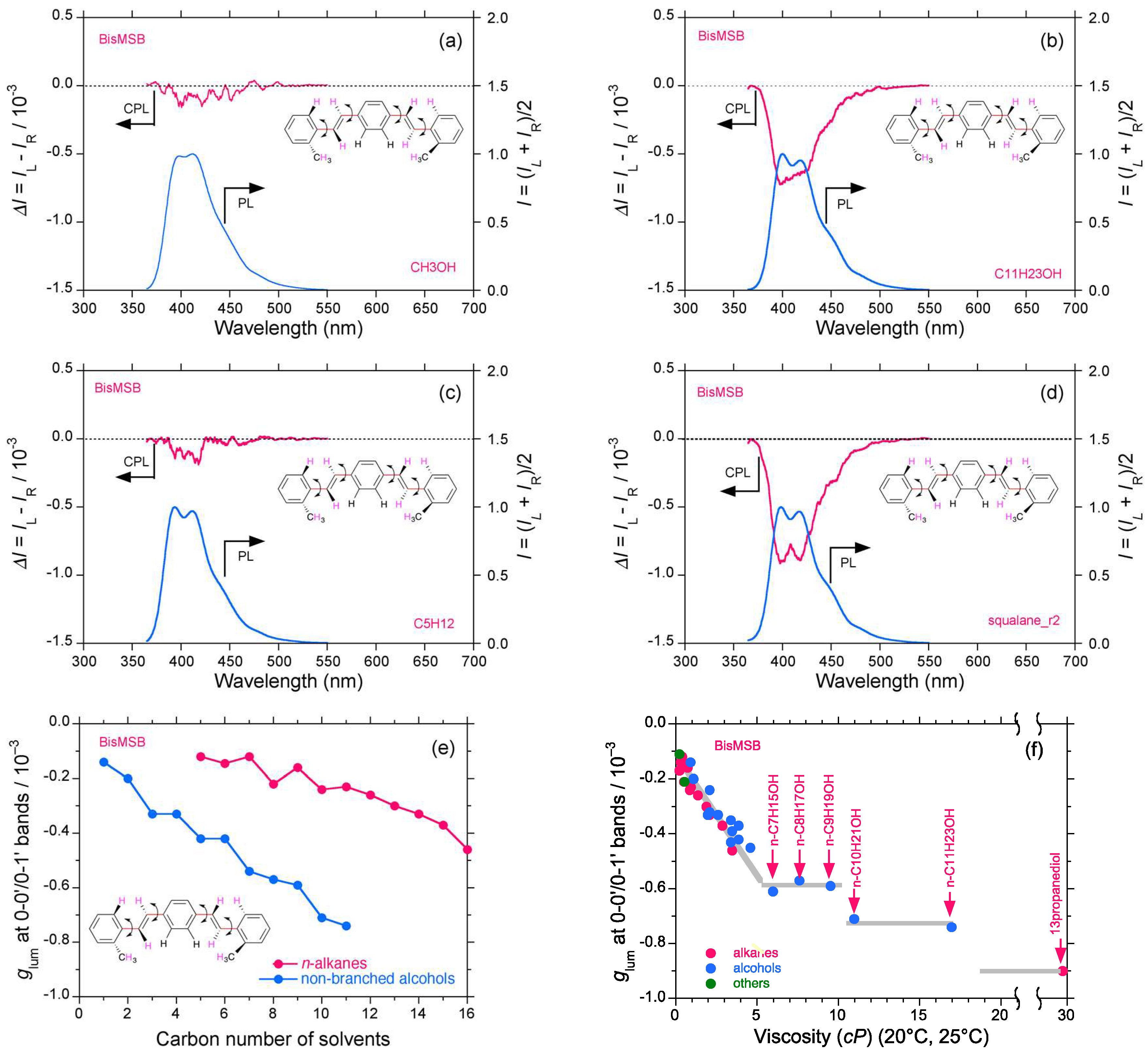
2.2. Rigid Luminophores Bearing Multiple Three-Fold Symmetrical Alkyl Substituents
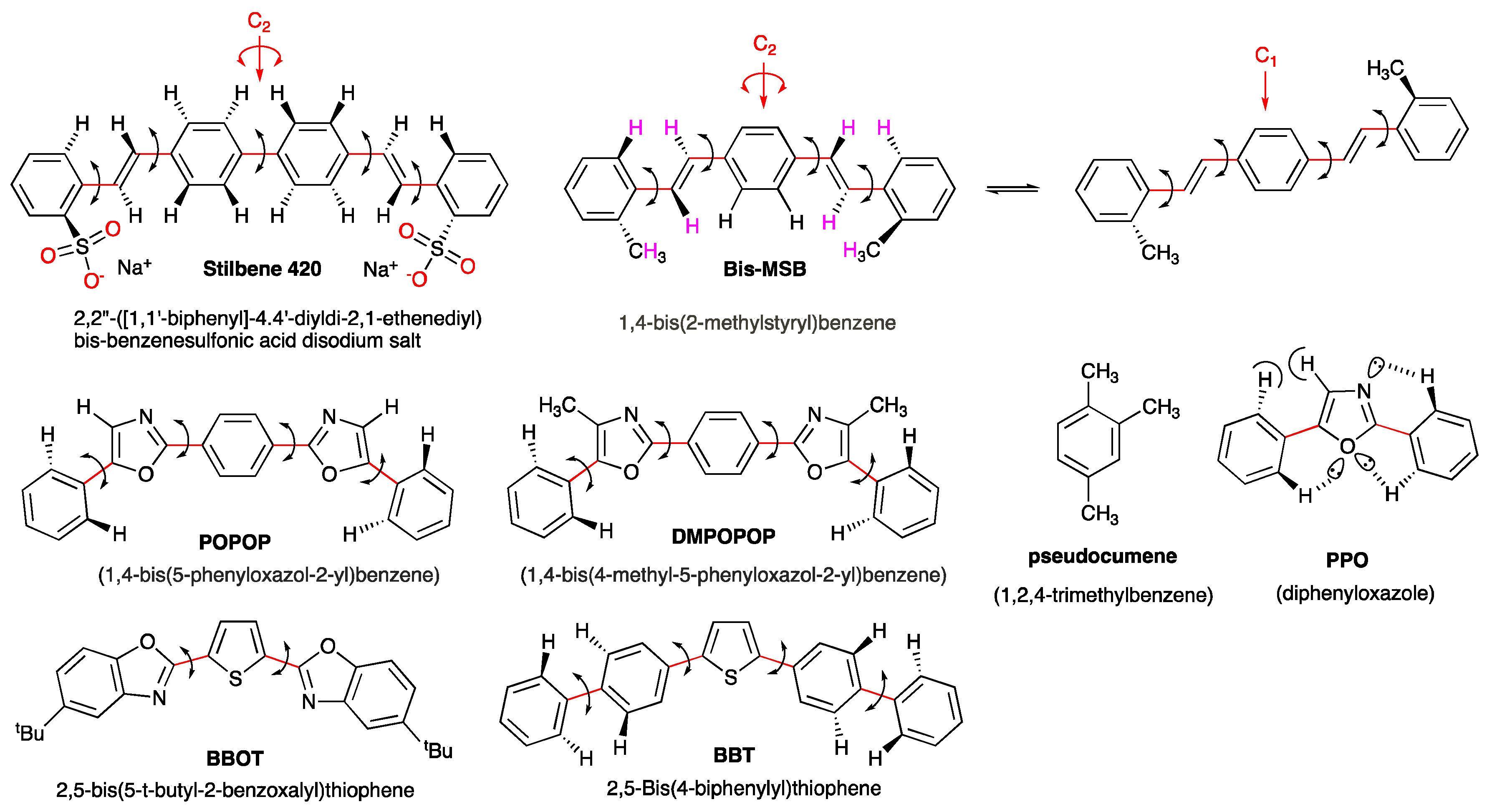
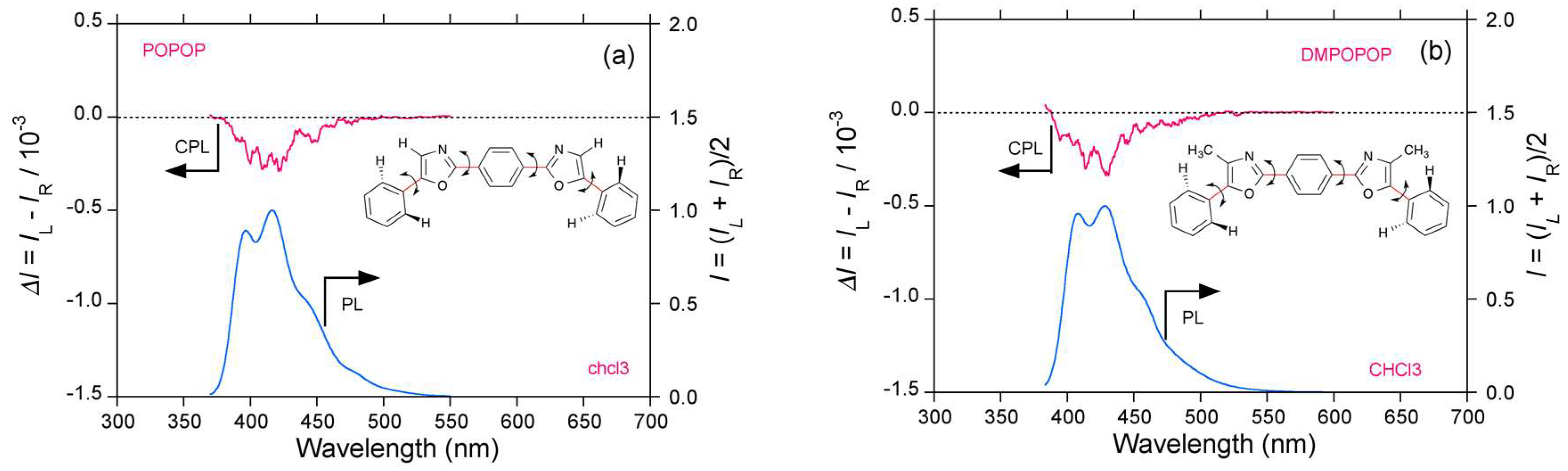
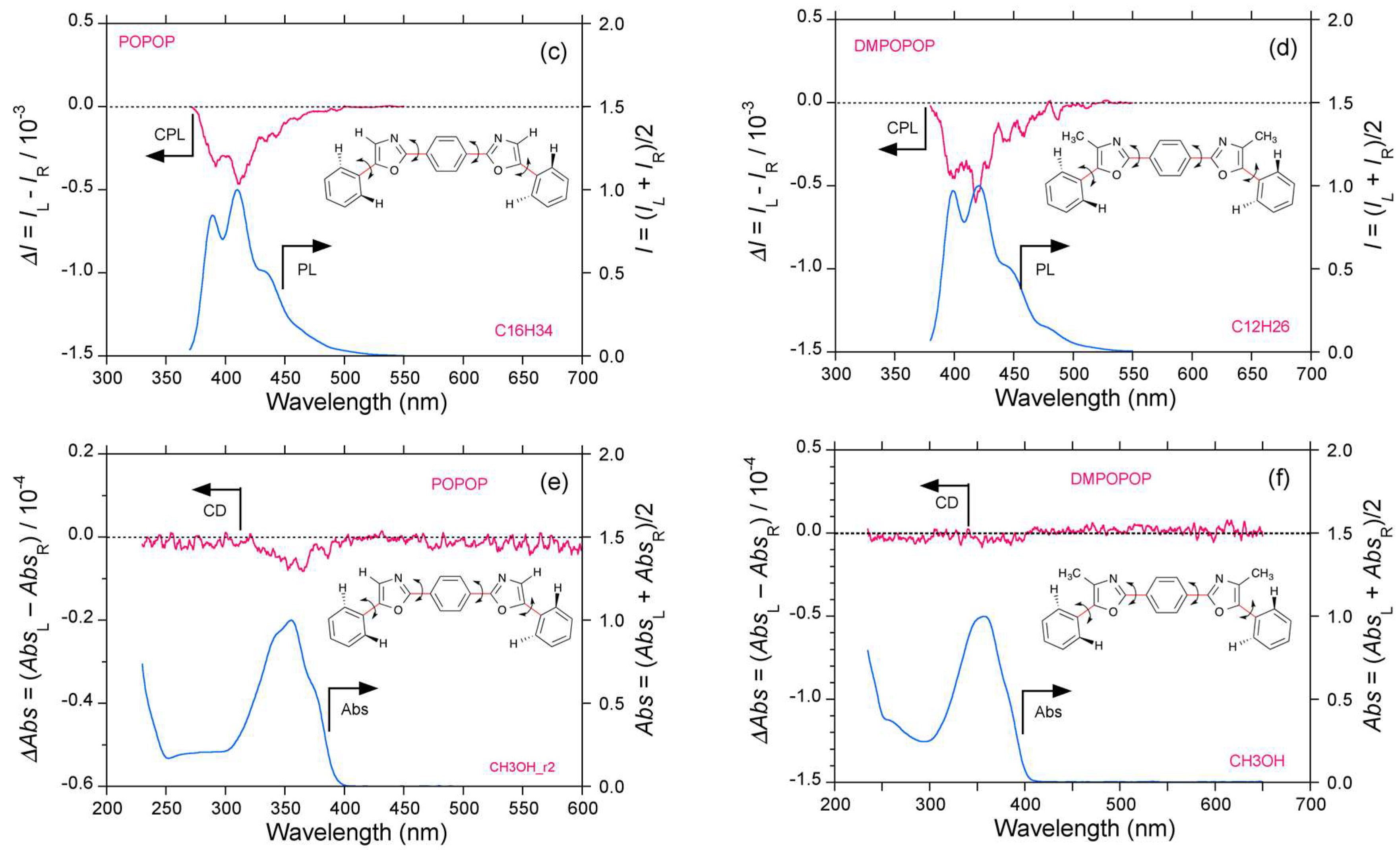
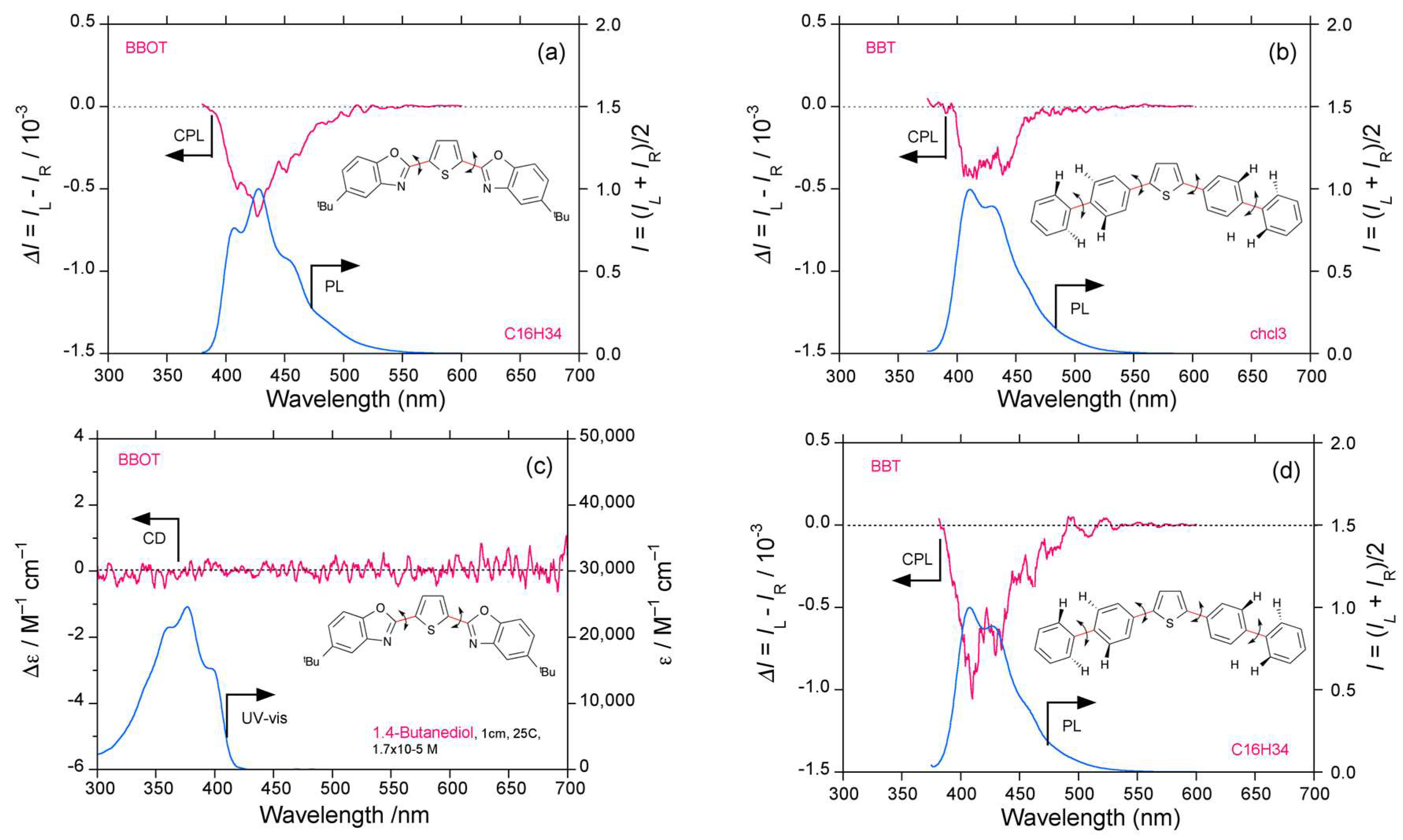
2.3. Organic Scintillators
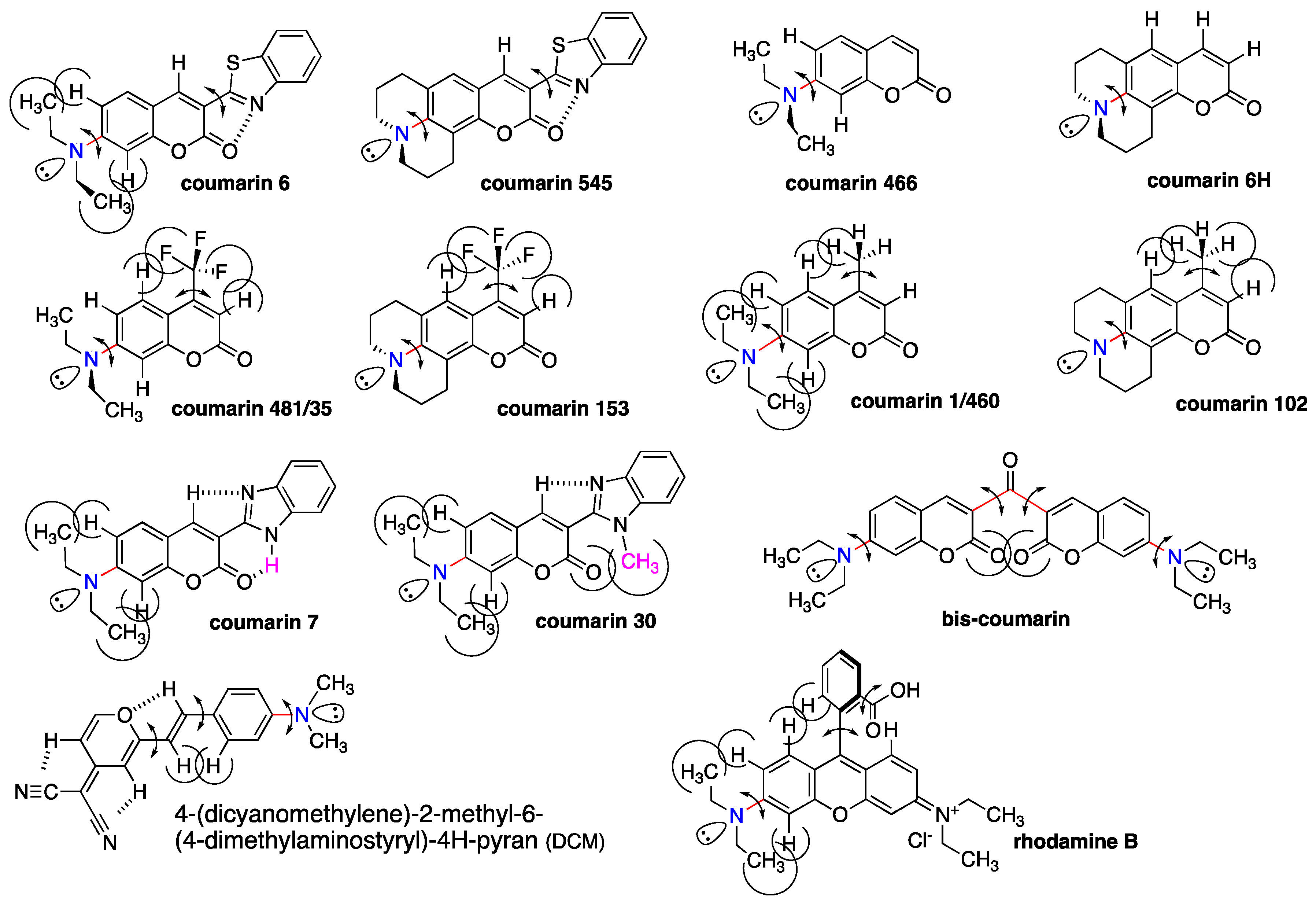
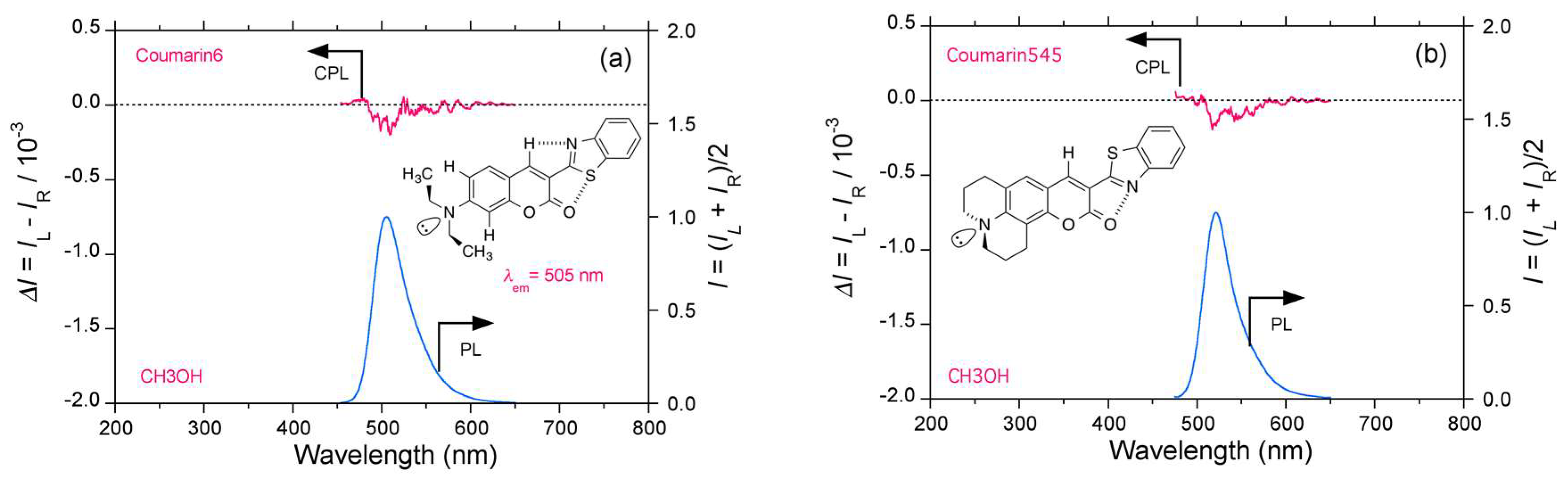
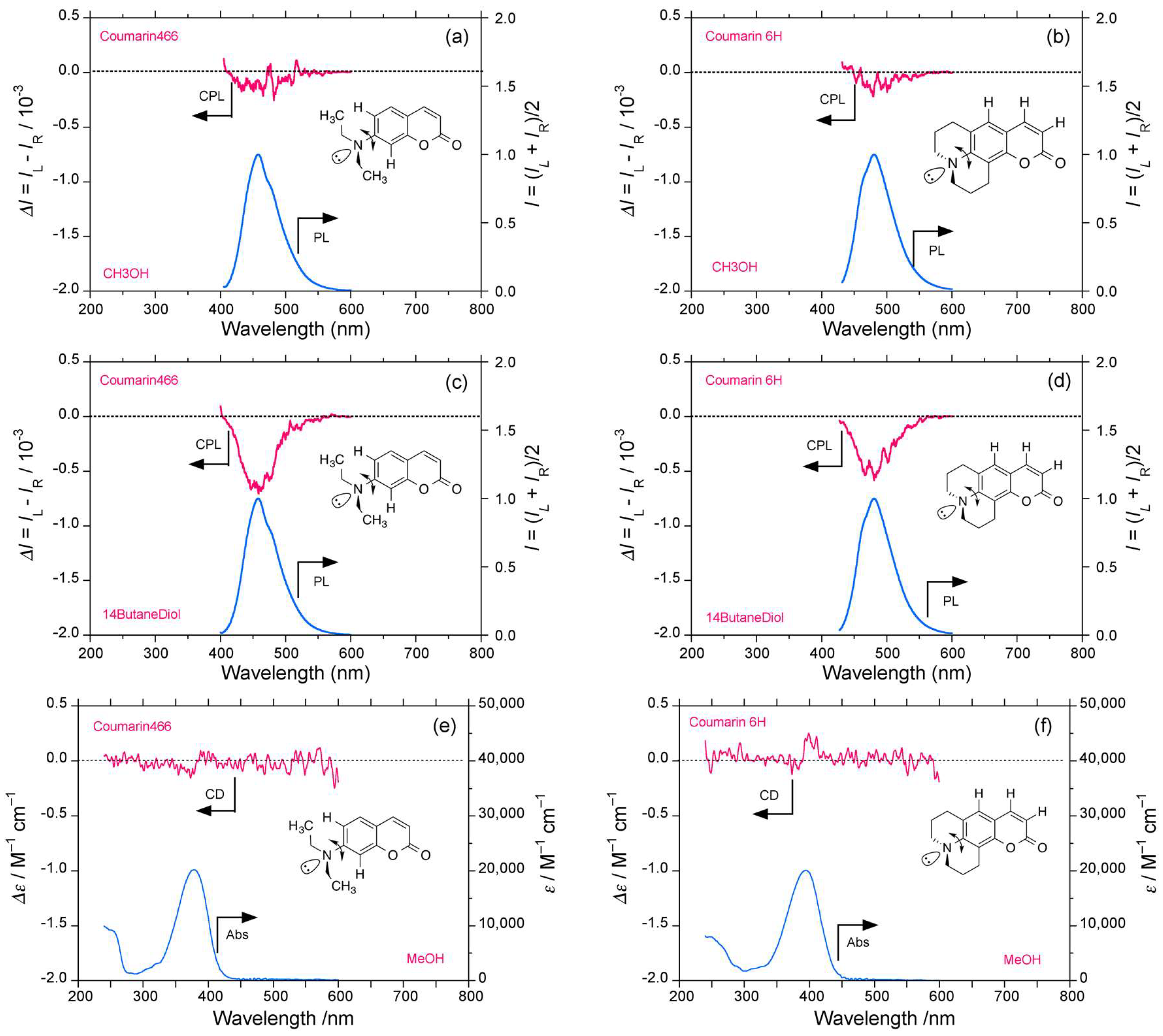
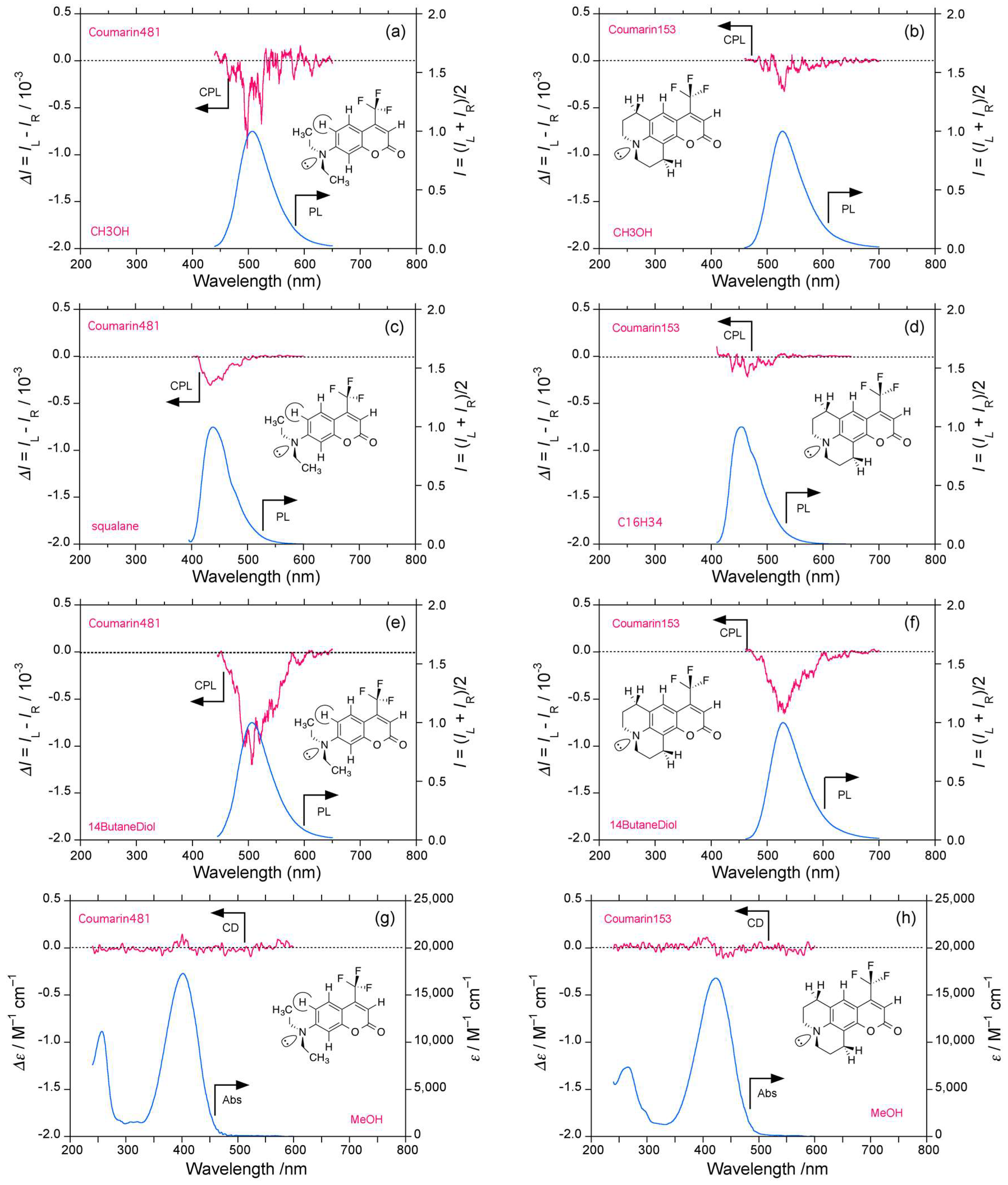
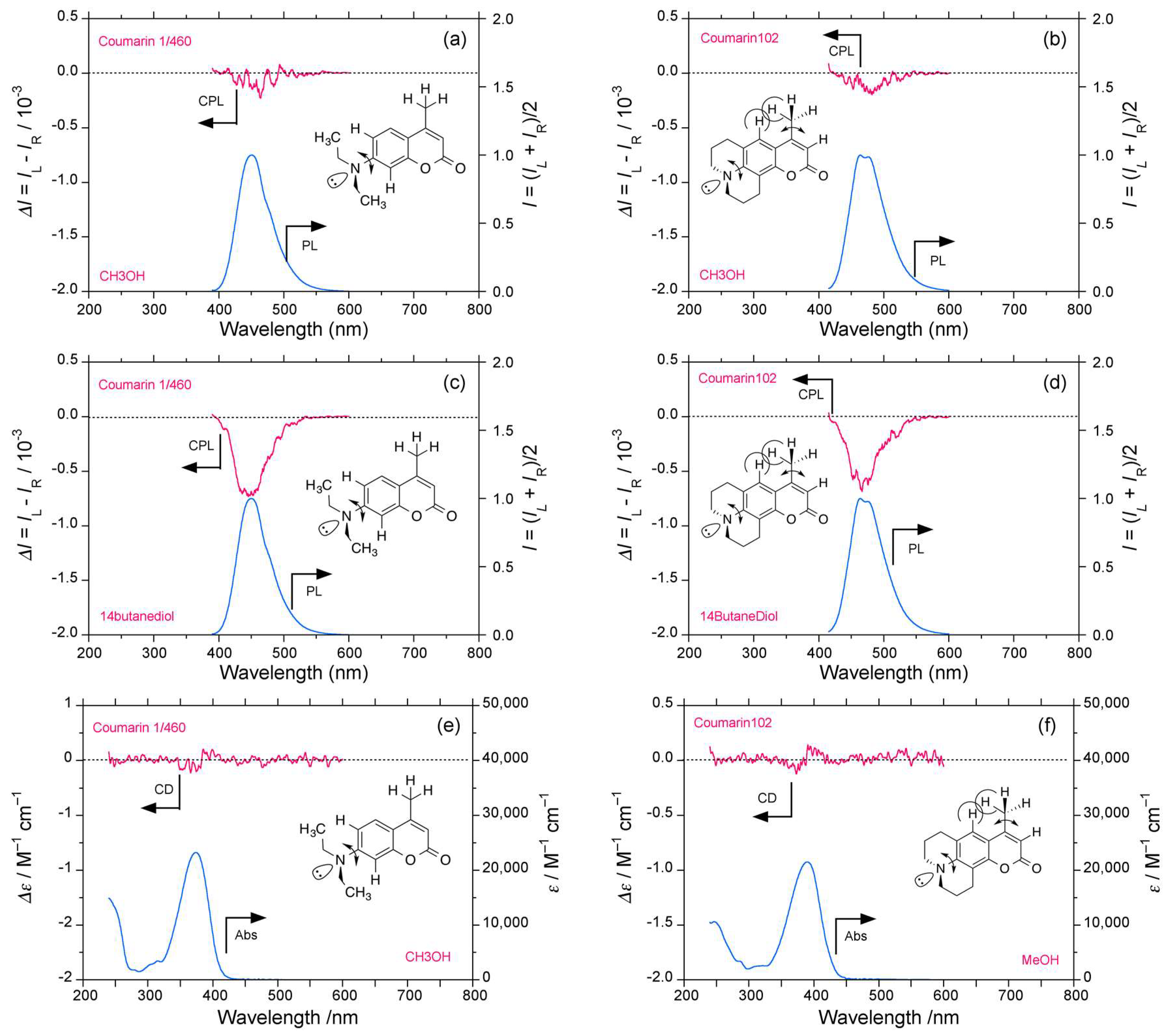
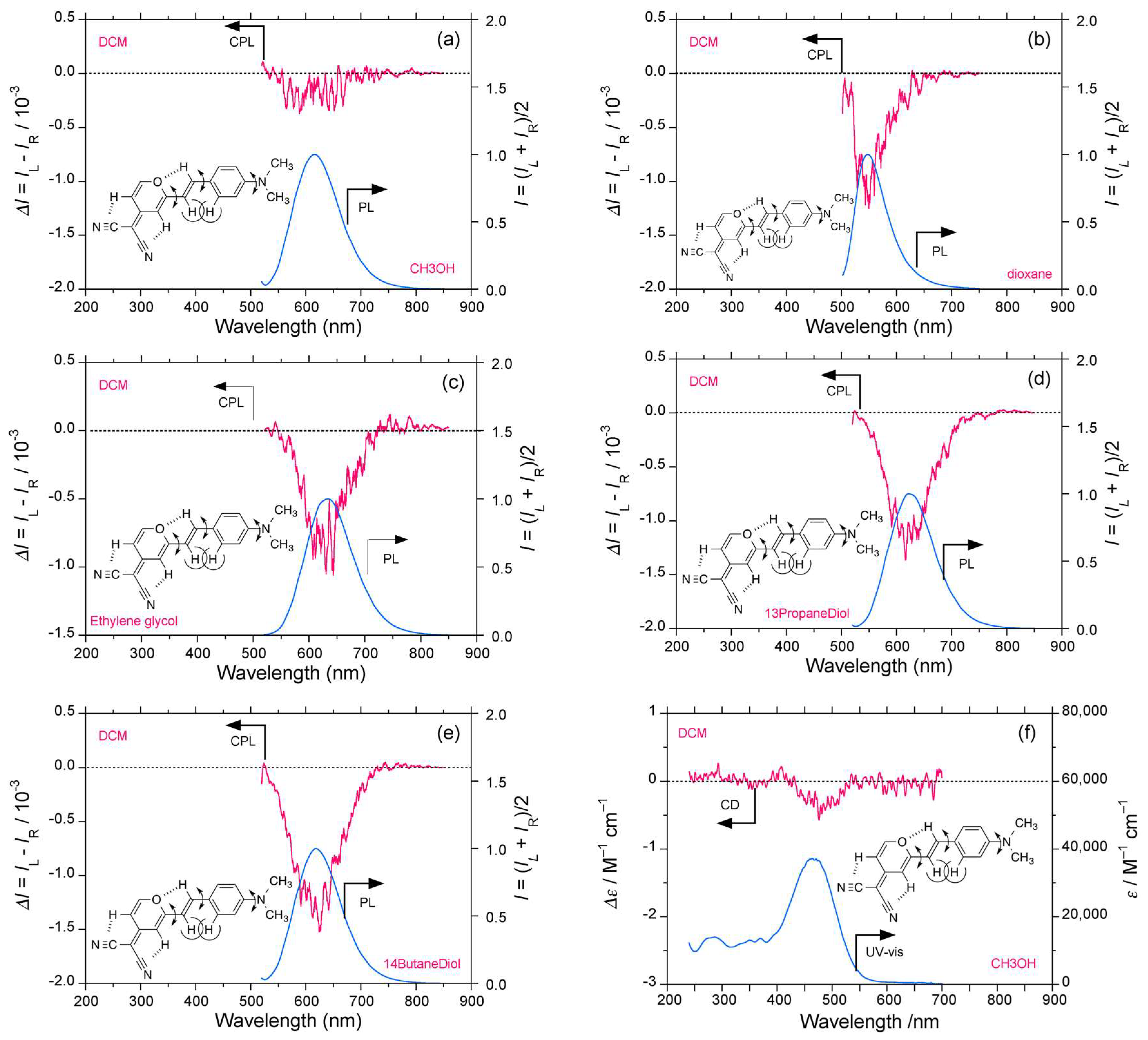
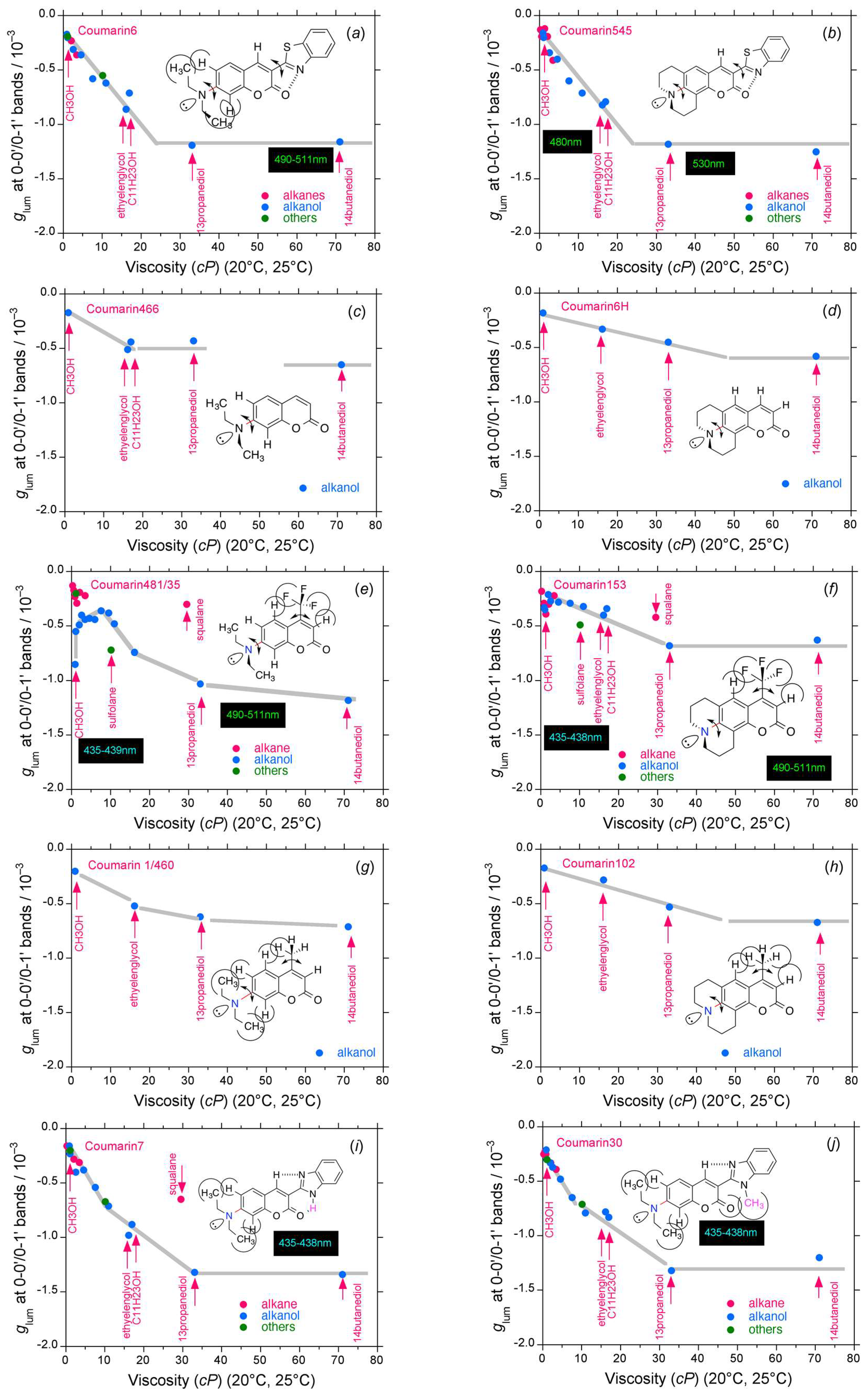
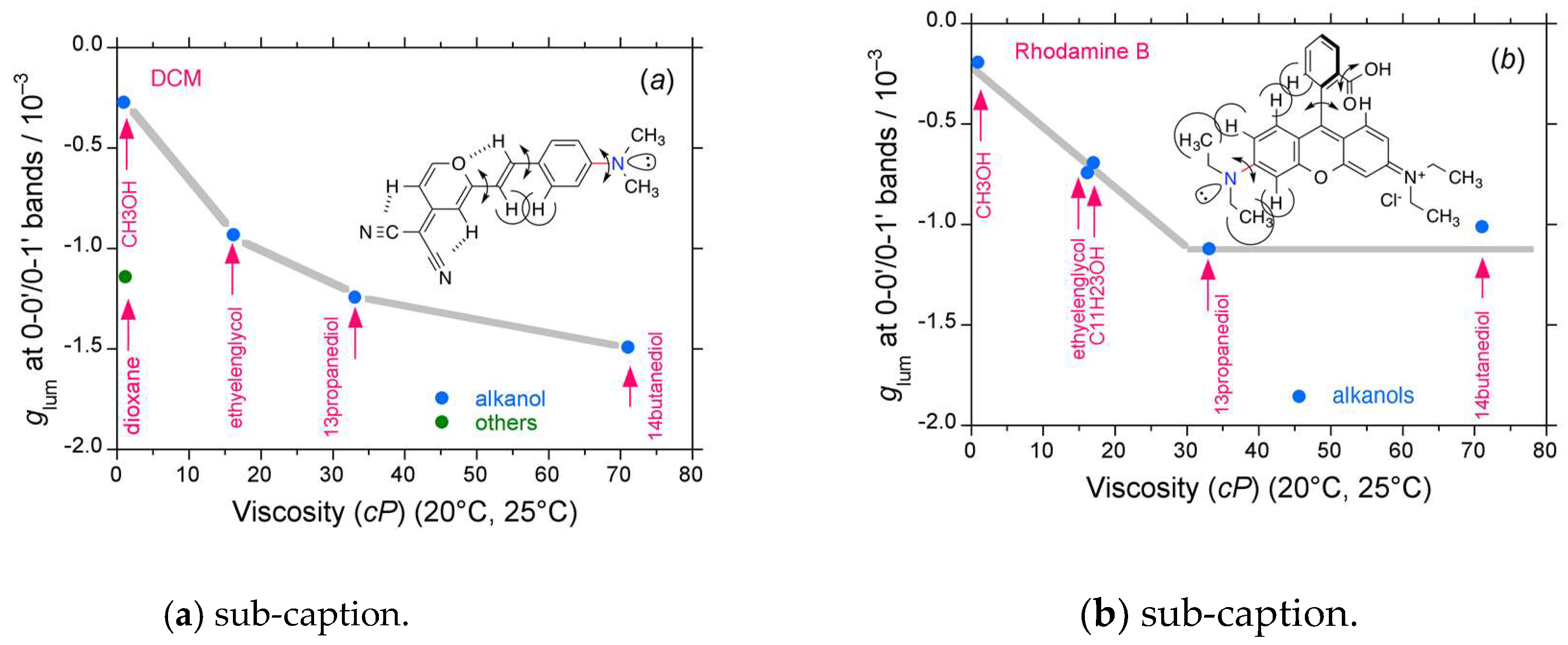
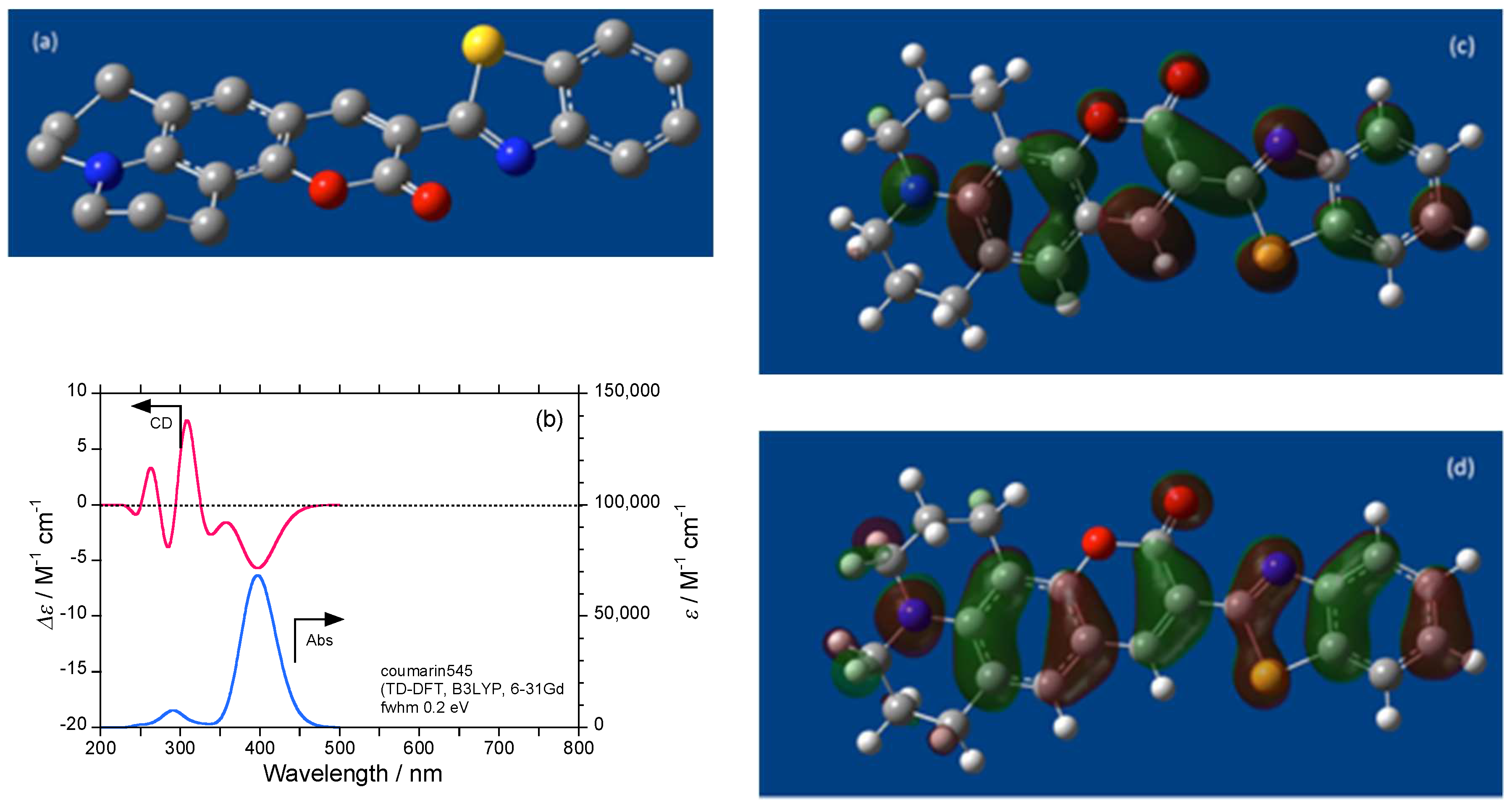
2.4. Luminophores Carrying Dialkylamino Group with Flip-Flop and/or Rotatable Motions
3. Discussion
4. Materials and Methods
4.1. Instrumentation
4.2. Materials
4.2.1. Luminophores (vendor)
4.2.2. Solvents
4.3. Preparation of Sample Solutions
4.4. Chiroptical Analysis
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Gardner, M. The New Ambidextrous Universe—Symmetry and Asymmetry from Mirror Reflections to Superstrings; 3rd ed.; Freeman: New York, NY, USA, 1990; ISBN 9780486442440. [Google Scholar]
- Miller, S.L. A Production of Amino Acids under Possible Primitive Earth Conditions. Science 1953, 117, 528–529. [Google Scholar] [CrossRef] [PubMed]
- Hanafusa, H.; Akabori, S. Polymerization of Aminoacetonitrile. Bull. Chem. Soc. Jpn. 1959, 32, 626–630. [Google Scholar] [CrossRef]
- Harada, K.; Fox, S.W. Thermal Synthesis of Natural Amino-Acids from a Postulated Primitive Terrestrial Atmosphere. Nature 1964, 201, 335–336. [Google Scholar] [CrossRef] [PubMed]
- Seckbach, J.; Chela-Flores, J.; Owen, T.; Raulin, F. (Eds.) Life in the Universe: From the Miller Experiment to the Search for Life on other Worlds; Kluwer: Dordrecht, Germany, 2004; ISBN 1-4020-2371-5. [Google Scholar]
- Breslow, R. A Likely Possible Origin of Homochirality in Amino Acids and Sugars on Prebiotic Earth. Tetrahedron Lett. 2011, 52, 2028–2032. [Google Scholar] [CrossRef]
- Mason, S.F. Chemical Evolution: Origin of the Elements, Molecules, and Living Systems; Oxford University Press: New York, NY, USA, 1991; ISBN 0-19-855272-6. [Google Scholar]
- Wagnière, G.H. On Chirality and the Universal Asymmetry: Reflections on Image and Mirror Image; Wiley-VCH: Zülich, Switzerland, 2007; ISBN 978-3-90639-038-3. [Google Scholar]
- Guijarro, A.; Yus, M. Origin of Chirality in the Molecules of Life: A Revision from Awareness to the Current Theories and Perspectives of this Unsolved Problem; RSC Publishing: Cambridge, UK, 2008; ISBN 978-0-85404-156-5. [Google Scholar]
- Rauchfuss, H. Chemical Evolution and the Origin of Life; Mitchell, T.N., Translator; Springer: Berlin, Germany, 2008; ISBN 978-3-540-78822-5. [Google Scholar]
- Soai, K. (Ed.) Amplification of Chirality; Springer: Berlin, Germany, 2008; ISBN 978-3-540-77868-4. [Google Scholar]
- Meierhenrich, U. Amino Acids and the Asymmetry of Life; Springer: Berlin, Germany, 2010; ISBN 978-3-540-76885-2. [Google Scholar]
- Boyd, R. Stardust, Supernovae and the Molecules of Life: Might We All Be Aliens? Springer: New York, NY, USA, 2012; ISBN 978-1-4614-1331-8. [Google Scholar]
- MacDermott, A.J. The Ascent of Parity-Violation: Exochirality in the Solar System and Beyond. Enantiomer 2000, 5, 153–168. [Google Scholar] [PubMed]
- Fujiki, M.; Yoshida, K.; Suzuki, N.; Rahim, N.A.A.; Jalil, J.A. Tempo-Spatial Chirogenesis. Limonene-Induced Mirror Symmetry Breaking of Si–Si Bond Polymers During Aggregation in Chiral Fluidic Media. J. Photochem. Photobiol. A Chem. 2016, 331, 120–129. [Google Scholar] [CrossRef]
- Schwieterman, E.W.; Kiang, N.Y.; Parenteau, M.N.; Harman, C.E.; DasSarma, S.; Fisher, T.M.; Arney, G.N.; Hartnett, H.E.; Reinhard, C.T.; Olson, S.L.; et al. Exoplanet Biosignatures: A Review of Remotely Detectable Signs of Life. Astrobiology 2018, 18, 663–708. [Google Scholar] [CrossRef] [PubMed]
- Schrodinger, E. What Is Life? With Mind and Matter and Autobiographical Sketches; Reprint Version; Cambridge University Press: Cambridge, UK, 2012; ISBN 1107683653. [Google Scholar]
- Freedman, R.; Geller, R.; Kaufmann, W.J. Universe, 10th ed.; Freeman, W.H., Ed.; W.H. Freeman and Company, Now an Imprint of Macmillan Higher Education, a Division of Macmillan Publishers: London, UK, 2015; ISBN 1319042384. [Google Scholar]
- Accelerating Expansion of the Universe. Available online: https://en.wikipedia.org/wiki/Accelerating_expansion_of_the_universe (accessed on 11 November 2018).
- Forbes, N.; Mahon, B. Faraday, Maxwell, and the Electromagnetic Field: How Two Men Revolutionized Physics; Prometheus Books: Amherst, NY, USA, 2014; ISBN 9781616149420. [Google Scholar]
- Lennartson, A. Appendix. Absolute Asymmetric Synthesis 1874–2009. In Absolute Asymmetric Synthesis; University of Gothenburg: Gothenburg, Sweden, 2011; pp. 59–74. ISBN 978-91-628-7836-8. [Google Scholar]
- Beth, R.A. Mechanical Detection and Measurement of the Angular Momentum of Light. Phys. Rev. 1936, 50, 115–125. [Google Scholar] [CrossRef]
- Inoue, Y. Asymmetric Photochemical Reactions in Solution. Chem. Rev. 1992, 92, 741–770. [Google Scholar] [CrossRef]
- Laur, P. The First Decades After the Discovery of CD and ORD by Aimé Cotton in 1895. In Comprehensive Chiroptical Spectroscopy: Applications in Stereochemical Analysis of Synthetic Compounds, Natural Products, and Biomolecules; Berova, N., Polavarapu, P.L., Nakanishi, K., Woody, R.W., Eds.; Wiley: Hoboken, NJ, USA, 2000; Volume 2, Chapter 1; pp. 1–35. [Google Scholar] [CrossRef]
- Rikken, G.L.J.A.; Raupach, E. Enantioselective Magnetochiral Photochemistry. Nature 2000, 405, 932–935. [Google Scholar] [CrossRef] [PubMed]
- Ribó, J.M.; Blanco, C.; Crusats, J.; El-Hachemi, Z.; Hochberg, D.; Moyano, A. Absolute Asymmetric Synthesis in Enantioselective Autocatalytic Reaction Networks: Theoretical Games, Speculations on Chemical Evolution and Perhaps A Synthetic Option. Chem. Eur. J. 2014, 20, 17250–17271. [Google Scholar] [CrossRef]
- Okano, K.; Taguchi, M.; Fujiki, M.; Yamashita, T. Circularly Polarized Luminescence of Rhodamine B in a Supramolecular Chiral Medium Formed by a Vortex Flow. Angew. Chem. Int. Ed. 2011, 50, 12474–12477. [Google Scholar] [CrossRef] [PubMed]
- Sun, J.; Li, Y.; Yan, F.; Liu, C.; Sang, Y.; Tian, F.; Feng, Q.; Duan, P.; Zhang, L.; Shi, X.; et al. Control Over the Emerging Chirality in Supramolecular Gels and Solutions by Chiral Microvortices in Milliseconds. Nat. Commun. 2018, 9, 2599. [Google Scholar] [CrossRef]
- Mineo, P.; Villari, V.; Scamporrino, E.; Micali, N. New Evidence about the Spontaneous Symmetry Breaking: Action of an Asymmetric Weak Heat Source. J. Phys. Chem. B 2015, 119, 12345–12353. [Google Scholar] [CrossRef] [PubMed]
- Eugene Wigner. Available online: https://en.wikipedia.org/wiki/Eugene_Wigner (accessed on 17 November 2018).
- Wigner, E.P. Violation of Symmetry in Physics. Sci. Am. 1965, 213, 28–36. [Google Scholar] [CrossRef]
- Gross, D.J. Symmetry in Physics: Wigner’s Legacy. Phys. Today 1995, 48, 46–50. [Google Scholar] [CrossRef]
- Lee, T.D.; Yang, C.N. Question of Parity Conservation in Weak Interactions. Phys. Rev. 1956, 104, 254–258. [Google Scholar] [CrossRef]
- Wu, C.S.; Ambler, E.; Hayword, R.W.; Hoppes, D.D.; Hudson, R.P. Experimental Test of Parity Conservation on Beta Decay. Phys. Rev. 1957, 105, 1413–1415. [Google Scholar] [CrossRef]
- Schopper, H. Circular Polarization of γ-Rays: Further Proof for Parity Failure in β Decay. Philos. Mag. 1957, 2, 710–713. [Google Scholar] [CrossRef]
- Goldhaber, M.; Grodzins, L.; Sunyar, A. Helicity of Neutrinos. Phys. Rev. 1958, 109, 1015–1017. [Google Scholar] [CrossRef]
- Fagg, L.W.; Hanna, S.S. Polarization Measurements on Nuclear Gamma Rays. Rev. Mod. Phys. 1959, 31, 711–758. [Google Scholar] [CrossRef]
- Wu, C.S. Parity Experiments in Beta Decay. Rev. Mod. Phys. 1959, 31, 783–790. [Google Scholar] [CrossRef]
- Christenson, J.H.; Cronin, J.W.; Fitch, V.L.; Turlay, R. Evidence for the 2π Decay of the K20 Meson. Phys. Rev. Lett. 1964, 13, 138–140. [Google Scholar] [CrossRef]
- Aubert, B.; et al. [BABAR Collaboration] Observation of CP Violation in the B0 Meson System. Phys. Rev. Lett. 2001, 87, 091801. [Google Scholar] [CrossRef] [PubMed]
- Abe, K.; et al. [Belle Collaboration] Observation of Large CP Violation in the Neutral B Meson System. Phys. Rev. Lett. 2001, 87, 091802. [Google Scholar] [CrossRef] [PubMed]
- Abe, K.; et al. [T2K Collaboration] Combined Analysis of Neutrino and Antineutrino Oscillations at T2K. Phys. Rev. Lett. 2017, 118, 151801. [Google Scholar] [CrossRef] [PubMed]
- Calvert, J.G.; Pitts, J.N., Jr. Photochemistry, 2nd ed.; John Wiley & Sons, Inc.: New York, NY, USA, 1966; Chapter 4-2D Selection rule; pp. 258–260. ISBN 0471130907. [Google Scholar]
- Turro, N.J. Modern Molecular Photochemistry; University Science Books: Sausalito, CA, USA, 1991; Chapter 5.6 State Mixing: Breakdown of the Single Orbital Configuration and Pure Multiplicity Approximations; pp. 96–103. ISBN 0935702717. [Google Scholar]
- Görling, C.; Jalviste, E.; Ohta, N.; Ottinger, C. Lifetime Measurements of the Collision-Free Slow Fluorescence from Glyoxal S1/T1 Gateway Levels in a Beam. J. Phys. Chem. A 1998, 102, 10620–10629. [Google Scholar] [CrossRef]
- Yamazaki, I.; Aratani, N.; Akimoto, S.; Yamazaki, T.; Osuka, A. Observation of Quantum Coherence for Recurrence Motion of Exciton in Anthracene Dimers in Solution. J. Am. Chem. Soc. 2003, 125, 7192–7193. [Google Scholar] [CrossRef]
- Latal, H. Parity Violation in Atomic Physics. In Chirality—From Weak Bosons to the α-Helix; Janoschek, R., Ed.; Springer: Berlin, Germany, 1991; pp. 1–17. ISBN 978-3-642-76569. [Google Scholar]
- Van der Meer, S. Stochastic Cooling and the Accumulation of Antiprotons. Rev. Mod. Phys. 1985, 57, 689–698. [Google Scholar] [CrossRef]
- Rubbia, C. Experimental Observation of the Intermediate Vector Bosons W+, W– and Z0. Rev. Mod. Phys. 1985, 57, 699–722. [Google Scholar] [CrossRef]
- Walgate, R. What Will Come After the Z0? Nature 1983, 303, 473. [Google Scholar] [CrossRef]
- Bouchiat, M.A.; Bouchiat, C.C. Weak Neutral Currents in Atomic Physics. Phys. Lett. B 1974, 48, 111–114. [Google Scholar] [CrossRef]
- Baied, P.E.G.; Brimcombe, M.W.S.M.; Roberts, G.J.; Sandars, P.G.H.; Soreide, D.C.; Fortson, E.N.; Lewis, L.L.; Lindahl, E.G.; Soreide, D.C. Search for Parity Non-Conserving Optical Rotation in Atomic Bismuth. Nature 1976, 264, 528–529. [Google Scholar] [CrossRef]
- Forte, M.; Heckel, B.R.; Ramsey, N.F.; Green, K.; Greene, G.L.; Byrne, J.; Pendlebury, J.M. First measurement of parity-nonconserving neutron-spin rotation: The tin isotopes. Phys. Rev. Lett. 1980, 45, 2088–2091. [Google Scholar] [CrossRef]
- Bucksbaum, P.H.; Commins, E.D.; Hunter, L.R. Observations of Parity Non-Conservation in Atomic Thallium. Phys. Rev. D 1981, 24, 1134–1148. [Google Scholar] [CrossRef]
- Emmons, T.P.; Reeves, J.M.; Fortson, E.N. Parity-Non-Conserving Optical Rotation in Atomic Lead. Phys. Rev. Lett. 1983, 51, 2089–2091. [Google Scholar] [CrossRef]
- Bouchiat, M.-A.; Pottier, L. Optical Experiments and Weak Interactions. Science 1986, 234, 1203–1210. [Google Scholar] [CrossRef]
- Bouchiat, M.A.; Bouchiat, C.C. Parity Violation in Atoms. Rep. Prog. Phys. 1997, 60, 1351–1396. [Google Scholar] [CrossRef]
- Wood, C.S.; Bennett, S.C.; Cho, D.; Masterson, B.P.; Roberts, J.L.; Tanner, C.E.; Wieman, C.E. Measurement of Parity Nonconservation and an Anapole Moment in Cesium. Science 1997, 275, 1759–1763. [Google Scholar] [CrossRef]
- Mitchell, G.E.; Bowman, J.D.; Penttilä, S.I.; Sharapov, E.I. Parity Violation in Compound Nuclei: Experimental Methods and Recent Results. Phys. Rep. 2001, 354, 157–241. [Google Scholar] [CrossRef]
- Guéna, J.; Lintz, M.; Bouchiat, M.-A. Atomic Parity Violation: Principles, Recent Results, Present Motivations. Mod. Phys. Lett. A 2005, 20, 375–390. [Google Scholar] [CrossRef]
- Yamagata, Y. A Hypothesis for the Asymmetric Appearance of Biomolecules on Earth. J. Theor. Biol. 1966, 11, 495–498. [Google Scholar] [CrossRef]
- Rein, D.W. Some Remarks on Parity Violating Effects of Intramolecular Interactions. J. Mol. Evol. 1974, 4, 15–22. [Google Scholar] [CrossRef]
- Letokhov, V.S. On Difference of Energy Levels of Left and Right Molecules Due to Weak Interactions. Phys. Lett. A 1975, 53, 275–276. [Google Scholar] [CrossRef]
- Zel’Dovich, Ya. B.; Saakyan, D.B.; Sobel’Man, I.I. Energy Difference between Right-Hand and Left-Hand Molecules due to Parity Nonconservation in Weak Interactions of Electrons with Nuclei. JETP Lett. 1977, 25, 94–97. [Google Scholar]
- Keszthelyi, L. Origin of the Asymmetry of Biomolecules and Weak Interaction. Orig. Life 1977, 8, 299–340. [Google Scholar] [CrossRef]
- Harris, R.A.; Stodolsky, L. Quantum Beats in Optical Activity and Weak Interactions. Phys. Lett. B 1978, 78, 313–317. [Google Scholar] [CrossRef]
- Hegstrom, R.A.; Rein, D.W.; Sandars, P.G.H. Calculation of the Parity Nonconserving Energy Difference between Mirror-Image Molecules. J. Chem. Phys. 1980, 73, 2329–2341. [Google Scholar] [CrossRef]
- Mason, S.F.; Tranter, G.E. Energy Inequivalence of Peptide Enantiomers from Parity Non-Conservation. Chem. Commun. 1983, 117–119. [Google Scholar] [CrossRef]
- Mason, S.F.; Tranter, G.E. The Parity-Violating Energy Difference between Enantiomeric Molecules. Mol. Phys. 1984, 53, 1091–1111. [Google Scholar] [CrossRef]
- Barron, L.D. Symmetry and molecular chirality. Chem. Soc. Rev. 1986, 15, 189–223. [Google Scholar] [CrossRef]
- Kondepudi, D. Parity Violations and the Origin of Bimolecular Handedness. In Entropy, Information and Evolution: New Perspective on Physical and Biological Evolution; Weber, B.H., Depew, D.J., Smith, J.D., Eds.; MIT Press: Cambridge, MA, USA, 1988; ISBN 0262731681. [Google Scholar]
- Quack, M. Structure and Dynamics of Chiral Molecules. Angew. Chem. Int. Ed. 1989, 28, 571–586. [Google Scholar] [CrossRef]
- Hegstrom, R.A.; Kondepudi, D.K. The Handedness of the Universe. Sci. Am. 1990, 262, 108–115. [Google Scholar] [CrossRef]
- Salam, A. The Role of Chirality in the Origin of Life. J. Mol. Evol. 1991, 33, 105–113. [Google Scholar] [CrossRef]
- Macdermott, A.J. Electroweak Enantioselection and the Origin of Life. Orig. Life Evol. Biosph. 1995, 25, 191–199. [Google Scholar] [CrossRef]
- Kikuchi, O.; Kiyonaga, H. Parity-Energy Shift of Helical n-Alkanes. J. Mol. Struct. (Theochem.) 1994, 312, 271–274. [Google Scholar] [CrossRef]
- Avetisov, V.; Goldanskii, V. Mirror Symmetry-Breaking at the Molecular Level. Proc. Natl. Acad. Sci. USA 1996, 93, 11435–11442. [Google Scholar] [CrossRef]
- Bonner, W.A. Enantioselective Autocatalysis. IV. Implications for Parity Violation Effects. Orig. Life Evol. Biosph. 1996, 26, 27–45. [Google Scholar] [CrossRef]
- Szabó-Nagy, A.; Keszthelyi, L. Demonstration of the Parity-Violating Energy Difference between Enantiomers. Proc. Natl. Acad. Sci. USA 1999, 96, 4252–4255. [Google Scholar] [CrossRef]
- Gottselig, M.; Luckhaus, D.; Quack, M.; Stohner, J.; Willeke, M. Mode Selective Stereomutation and Parity Violation in Disulfane Isotopomers H2S2, D2S2, T2S2. Helv. Chim. Acta 2001, 84, 1846–1861. [Google Scholar] [CrossRef]
- Compton, R.N.; Pagni, R.M. The Chirality of Biomolecules. Adv. At. Mol. Opt. Phys. 2002, 48, 219–261. [Google Scholar] [CrossRef]
- Schwerdtfeger, P.; Gierlich, J.; Bollwein, T. Large Parity-Violation Effects in Heavy-Metal-Containing Chiral Compounds. Angew. Chem. Int. Ed. 2003, 42, 1293–1296. [Google Scholar] [CrossRef]
- MacDermott, A.J.; Hegstrom, R.A. A Proposed Experiment to Measure the Parity-Violating Energy Difference between Enantiomers from the Optical Rotation of Chiral Ammonia-Like “Cat” Molecules. Chem. Phys. 2004, 305, 55–68. [Google Scholar] [CrossRef]
- Quack, M.; Stohner, J.; Willeke, M. High-Resolution Spectroscopic Studies and Theory of Parity Violation in Chiral Molecules. Annu. Rev. Phys. Chem. 2008, 59, 741–769. [Google Scholar] [CrossRef]
- Bargueño, P.; Gonzalo, I.; de Tudela, R.P. Detection of Parity Violation in Chiral Molecules by External Tuning of Electroweak Optical Activity. Phys. Rev. A 2009, 80, 012110. [Google Scholar] [CrossRef]
- Dorta-Urra, A.; Peñate-Rodríguez, H.C.; Bargueño, P.; Rojas-Lorenzo, G.; Miret-Artés, S. Dissipative Geometric Phase and Decoherence in Parity-Violating Chiral Molecules. J. Chem. Phys. 2012, 136, 174505. [Google Scholar] [CrossRef]
- Famiano, M.A.; Boyd, R.N.; Kajino, T.; Onaka, T.; Mo, Y. Amino Acid Chiral Selection via Weak Interactions in Stellar Environments: Implications for the Origin of Life. Sci. Rep. 2018, 8, 8833. [Google Scholar] [CrossRef]
- Daussy, Ch.; Marrel, T.; Amy-Klein, A.; Nguyen, C.T.; Bordé, C.J.; Chardonnet, C. Limit on the Parity Nonconserving Energy Difference between the Enantiomers of a Chiral Molecule by Laser Spectroscopy. Phys. Rev. Lett. 1999, 83, 1554–1557. [Google Scholar] [CrossRef]
- Wang, W.; Yi, F.; Ni, Y.; Zhao, Z.; Jin, X.; Tang, Y. Parity Violation of Electroweak Force in Phase Transitions of Single Crystals of D- and L-Alanine and Valine. J. Biol. Phys. 2000, 26, 51–65. [Google Scholar] [CrossRef]
- Fujiki, M. Experimental Tests of Parity Violation at Helical Polysilylene Level. Macromol. Rapid Commun. 2001, 22, 669–674. [Google Scholar] [CrossRef]
- Pagni, R.M.; Compton, R.N. Asymmetric Synthesis of Optically Active Sodium Chlorate and Bromate Crystals. Cryst. Growth Des. 2002, 2, 249–253. [Google Scholar] [CrossRef]
- Scolnik, T.; Portnaya, I.; Cogan, U.; Tal, S.; Haimovitz, R.; Fridkin, M.; Elitzur, A.C.; Deamer, D.W.; Shinitzky, M. Subtle Differences in Structural Transitions between Poly-L- and Poly-D-Amino Acids of Equal Length in Water. Phys. Chem. Chem. Phys. 2006, 8, 333–339. [Google Scholar] [CrossRef]
- Kodona, E.K.; Alexopoulos, C.; Panou-Pomonis, E.; Pomonis, P.J. Chirality and Helix Stability of Polyglutamic Acid Enantiomers. J. Colloid Interface Sci. 2008, 319, 72–80. [Google Scholar] [CrossRef]
- Darquié, B.; Stoeffler, C.; Shelkovnikov, A.; Daussy, C.; Amy-Klein, A.; Chardonnet, C.; Zrig, S.; Guy, L.; Crassous, J.; Soulard, P. Progress Toward the First Observation of Parity Violation in Chiral Molecules by High-Resolution Laser Spectroscopy. Chirality 2010, 22, 870–884. [Google Scholar] [CrossRef]
- Fujiki, M. Mirror Symmetry Breaking in Helical Polysilanes: Preference between Left and Right of Chemical and Physical Origin. Symmetry 2010, 2, 1625–1652. [Google Scholar] [CrossRef]
- Albert, S.; Arn, F.; Bolotova, I.; Chen, Z.; Fábri, C.; Grassi, G.; Lerch, P.; Quack, M.; Seyfang, G.; Wokaun, A.; et al. Synchrotron-Based Highest Resolution Terahertz Spectroscopy of the ν24 Band System of 1,2-Dithiine (C4H4S2): A Candidate for Measuring the Parity Violating Energy Difference between Enantiomers of Chiral Molecules. J. Phys. Chem. Lett. 2016, 7, 3847–3853. [Google Scholar] [CrossRef]
- Kozlova, S.G.; Gabuda, S.P. Thermal Properties of Zn2(C8H4O4)2·C6H12N2 Metal-Organic Framework Compound and Mirror Symmetry Violation of Dabco Molecules. Sci. Rep. 2017, 7, 11505. [Google Scholar] [CrossRef]
- Lightner, D.A.; Gurst, J.E. Organic Conformational Analysis and Stereochemistry from Circular Dichroism Spectroscopy; Wiley-VCH: Weinheim, Germany, 2000; ISBN 978-0-471-35405-5. [Google Scholar]
- Eliel, E.L.; Wilen, S.H. Stereochemistry of Organic Compounds, 1st ed.; Wiley-Interscience: Hoboken, NJ, USA, 1994; ISBN 9780471016700. [Google Scholar]
- Anslyn, E.V.; Dougherty, D.A. Modern Physical Organic Chemistry; University Science: Mill Valley, CA, USA, 2005; ISBN 9781891389313. [Google Scholar]
- Hund, F. Symmetriecharaktere yon Termen bei Systemen mit Gleichen Partikeln in der Quantenmechanik. Z. Phys. 1927, 43, 788–803. [Google Scholar] [CrossRef]
- Bell, R.P. The Tunnel Effect in Chemistry; Chapman and Hall: London, UK, 1980; ISBN 0-412-21340-0. [Google Scholar]
- Laane, J. Vibrational Potential Energy Surfaces in Electronic Excited States. In Frontiers of Molecular Spectroscopy; Laane, J., Ed.; Elsevier: New York, NY, USA, 2009; Chapter 4; pp. 63–132. ISBN 978-0-444-53175-9. [Google Scholar]
- Fujiki, M.; Koe, J.R.; Mori, T.; Kimura, Y. Questions of Mirror Symmetry at the Photoexcited and Ground States of Non-Rigid Luminophores Raised by Circularly Polarized Luminescence and Circular Dichroism Spectroscopy: Part 1. Oligofluorenes, Oligophenylenes, Binaphthyls and Fused Aromatics. Molecules 2018, 23, 2606. [Google Scholar] [CrossRef]
- Shindo, Y.; Nakagawa, M. On the Artifacts in Circularly Polarized Emission Spectroscopy. Appl. Spectrosc. 1985, 39, 32–38. [Google Scholar] [CrossRef]
- Blok, P.M.L.; Dekkers, H.P.J.M. Measurement of the Circular Polarization of the Luminescence of Photoselected Samples under Artifact-free Conditions. Appl. Spectrosc. 1990, 44, 305–309. [Google Scholar] [CrossRef]
- Kaur, S. A Review on Electronic Spectroscopy of Perylene. Master’s Thesis, San Jose State University, San Jose, CA, USA, 1999. Available online: http://scholarworks.sjsu.edu/etd_theses/1879 (accessed on 1 February 2019).
- Allamandola, L.J.; Tielens, A.G.G.M.; Barker, J.R. Interstellar Polycyclic Aromatic Hydrocarbons—The Infrared Emission Bands, the Excitation/Emission Mechanism, and the Astrophysical Implications. Astrophys. J. Suppl. Ser. 1989, 71, 733–775. [Google Scholar] [CrossRef]
- Tielens, A.G.G.M. Interstellar Polycyclic Aromatic Hydrocarbon Molecules. Annu. Rev. Astron. Astrophys. 2008, 46, 289–337. [Google Scholar] [CrossRef]
- Walter, E.R.H.; Williams, J.A.G.; Parker, D. Solvent polarity and oxygen sensitivity, rather than viscosity, determine lifetimes of biaryl-sensitised terbium luminescence. Chem. Commun. 2017, 53, 13344–133347. [Google Scholar] [CrossRef]
- Arbeloa, F.L.; Bañuelos, J.; Martínez, V.; Arbeloa, T.; López Arbeloa, I. Structural, Photophysical and Lasing Properties of Pyrromethene Dyes. Int. Rev. Phys. Chem. 2005, 24, 339–374. [Google Scholar] [CrossRef]
- Cerdán, L.; García-Moreno, S.; Costela, A.; García-Moreno, I.; de la Moya, A.S. Circularly Polarized Laser Emission Induced in Isotropic and Achiral Dye Systems. Sci. Rep. 2016, 6, 28740. [Google Scholar] [CrossRef]
- Ebata, K.; Inada, T.; Kabuto, C.; Sakurai, H. Hexakis(fluorodimethylsilyl)benzene, Hexakis(methoxy-dimethylsilyl)benzene, and Related Compounds. Novel Neutral Pentacoordinate Structures for Silicon and Merry-Go-Round Degenerate Fluorine Migration. J. Am. Chem. Soc. 1994, 116, 3595–3596. [Google Scholar] [CrossRef]
- Organic Scintillators Energy. Available online: https://en.wikipedia.org/wiki/Scintillator#Organic_scintillators (accessed on 13 October 2018).
- Araki, T.; Enomoto, S.; Furuno, K.; Gando, Y.; Ichimura, K.; Ikeda, H.; Inoue, K.; Kishimoto, Y.; Koga, M.; Koseki, Y.; et al. Experimental Investigation of Geologically Produced Antineutrinos with KamLAND. Nature 2005, 436, 499–503. [Google Scholar] [CrossRef]
- KamLAND. Available online: https://en.wikipedia.org/wiki/Kamioka_Liquid_Scintillator_Antineutrino_Detector (accessed on 7 November 2018).
- Nucleosynthesis Reactions. Available online: https://en.wikipedia.org/wiki/Nucleosynthesis (accessed on 7 November 2018).
- Jones, G., II; Jackson, W.R.; Choi, C.Y.; Bergmark, W.R. Solvent Effects on Emission Yield and Lifetime for Coumarin Laser Dyes. Requirements for a Rotatory Decay Mechanism. J. Phys. Chem. 1985, 89, 294–300. [Google Scholar] [CrossRef]
- Weber, M.J. Handbook of Laser Wavelengths; CRC Press: Boca Raton, FL, USA, 1998; ISBN 0849335086. [Google Scholar]
- Birks, J.B. The Theory and Practice of Scintillation Counting; Elsevier: Amsterdam, The Netherlands, 1964; ISBN 978-0-08-010472-0. [Google Scholar]
- Duarte, F.J. (Ed.) Tunable Lasers Handbook (Optics and Photonics); Academic Press: Cambridge, MA, USA, 1995; ISBN 012222695X. [Google Scholar]
- Fujiki, M.; Jalilah, A.J.; Suzuki, N.; Taguchi, M.; Zhang, W.; Abdellatif, M.M.; Nomura, K. Chiral Optofluidics: Gigantic Circularly Polarized Light Enhancement of All-trans-poly(9,9-di-n-octylfluorene-2,7-vinylene) during Mirror-symmetry-breaking Aggregation by Optically Tuning Fluidic Media. RSC Adv. 2012, 2, 6663–6671. [Google Scholar] [CrossRef]
- Weinberg Angle. Available online: https://en.wikipedia.org/wiki/Weinberg_angle (accessed on 2 October 2018).
- Electroweak Interaction. Available online: https://en.wikipedia.org/wiki/Electroweak_interaction (accessed on 30 October 2018).
- Nakashima, H.; Koe, J.R.; Torimitsu, K.; Fujiki, M. Transfer and Amplification of Chiral Molecular Information to Polysilylene Aggregates. J. Am. Chem. Soc. 2001, 123, 4847–4848. [Google Scholar] [CrossRef] [PubMed]
- Fujiki, M. Mirror Symmetry Breaking of Silicon Polymers—From Weak Bosons to Artificial Helix. Chem. Rec. 2009, 9, 271–298. [Google Scholar] [CrossRef] [PubMed]
- Nakano, Y.; Fujiki, M. Circularly Polarized Light Enhancement by Helical Polysilane Aggregates Suspension in Organic Optofluids. Macromolecules 2011, 44, 7511–7519. [Google Scholar] [CrossRef]
- Fujiki, M.; Yoshida, K.; Suzuki, N.; Zhang, J.; Zhang, W.; Zhu, X. Mirror Symmetry Breaking and Restoration within μm-sized Polymer Particles in Optofluidic Media by Pumping Circularly Polarised Light. RSC Adv. 2013, 3, 5213–5219. [Google Scholar] [CrossRef]
- Fujiki, M.; Kawagoe, Y.; Nakano, Y.; Nakao, A. Mirror-Symmetry-Breaking in Poly[(9,9-di-n-octylfluorenyl-2,7-diyl)-alt-biphenyl] (PF8P2) is Susceptible to Terpene Chirality, Achiral Solvents and Mechanical Stirring. Molecules 2013, 18, 7035–7057. [Google Scholar] [CrossRef] [PubMed]
- Wang, L.; Suzuki, N.; Liu, J.; Matsuda, T.; Rahim, N.A.A.; Zhang, W.; Fujiki, M.; Zhang, Z.; Zhou, N.; Zhu, X. Limonene Induced Chiroptical Generation and Inversion during Aggregation of Achiral Polyfluorene Analogs: Structure-Dependence and Mechanism. Polym. Chem. 2014, 5, 5920–5927. [Google Scholar] [CrossRef]
- Fujiki, M.; Donguri, Y.; Zhao, Y.; Nakao, A.; Suzuki, N.; Yoshida, K.; Zhang, W. Photon Magic: Chiroptical Polarisation, Depolarisation, Inversion, Retention and Switching of Non-Photochromic Light-Emitting Polymers in Optofluidic Medium. Polym. Chem. 2015, 6, 1627–1638. [Google Scholar] [CrossRef]
- Nakano, Y.; Ichiyanagi, F.; Naito, M.; Yang, Y.; Fujiki, M. Chiroptical Generation and Inversion During the Mirror-Symmetry-Breaking Aggregation of Dialkylpolysilanes due to Limonene Chirality. Chem. Commun. 2012, 48, 6636–6638. [Google Scholar] [CrossRef]
- Duong, T.S.; Fujiki, M. The Origin of Bisignate Circularly Polarized Luminescence (CPL) Spectra from Chiral Polymer Aggregates and Molecular Camphor: Anti-kasha’s Rule Revealed by CPL Excitation (CPLE) Spectra. Polym. Chem. 2017, 8, 4673–4679. [Google Scholar] [CrossRef]
- Jalilah, A.J.; Asanoma, F.; Fujiki, M. Unveiling Controlled Breaking of the Mirror Symmetry of Eu(fod)3 with α-/β-Pinene and BINAP by Circularly Polarised Luminescence (CPL), CPL Excitation, and 19F-/31P{1H}-NMR Spectra and Mulliken Charges. Inorg. Chem. Front. 2018, 5, 2718–2733. [Google Scholar] [CrossRef]
- Mislow, K. Absolute Asymmetric Synthesis: A Commentary. Collect. Czech. Chem. Commun. 2003, 68, 849–864. [Google Scholar] [CrossRef]
- Kawasaki, T.; Tanaka, H.; Tsutsumi, T.; Kasahara, T.; Sato, I.; Soai, K.J. Chiral discrimination of cryptochiral saturated quaternary and tertiary hydrocarbons by asymmetric autocatalysis. Am. Chem. Soc. 2006, 128, 6032–6033. [Google Scholar] [CrossRef]
- Kawasaki, T.; Hohberger, C.; Araki, Y.; Hatase, K.; Beckerle, K.; Okuda, J.; Soai, K. Discrimination of Cryptochirality in Chiral Isotactic Polystyrene by Asymmetric Autocatalysis. Chem. Commun. 2009, 5621–5623. [Google Scholar] [CrossRef][Green Version]
- Amako, T.; Nakabayashi, K.; Suzuki, N.; Guo, S.; Rahim, N.A.A.; Harada, T.; Fujiki, M.; Imai, Y. Pyrene Magic: Chiroptical Enciphering and Deciphering 1,3-Dioxolane Bearing Two Wirepullings to Drive Two Remote Pyrenes. Chem. Commun. 2015, 51, 8237–8240. [Google Scholar] [CrossRef]
- Nakanishi, S.; Nakabayashi, K.; Mizusawa, T.; Suzuki, N.; Guo, S.; Fujiki, M.; Imai, Y. Cryptochiral Binaphthyl–Bipyrene Luminophores Linked with Alkylene Esters: Intense Circularly Polarised Luminescence, But Ultraweak Circular Dichroism. RSC Adv. 2016, 6, 99172–99176. [Google Scholar] [CrossRef]
- Nakabayashi, K.; Kitamura, S.; Suzuki, N.; Guo, S.; Fujiki, M.; Imai, Y. Non-Classically Controlled Signs in a Circularly Polarised Luminescent Molecular Puppet: The Importance of the Wire Structure Connecting Binaphthyl and Two Pyrenes. Eur. J. Org. Chem. 2016, 64–69. [Google Scholar] [CrossRef]
- Hara, N.; Yanai, M.; Kaji, D.; Shizuma, M.; Tajima, N.; Fujiki, M.; Imai, Y. A Pivotal Biaryl Rotamer Bearing Two Floppy Pyrenes that Exhibits Cryptochiral Characteristics in the Ground State. ChemistrySelect 2018, 3, 9970–9973. [Google Scholar] [CrossRef]
- Maeda, K.; Hirose, D.; Okoshi, N.; Shimomura, K.; Wada, Y.; Ikai, T.; Kanoh, S.; Yashima, E. Direct Detection of Hardly Detectable Hidden Chirality of Hydrocarbons and Deuterated Isotopomers by a Helical Polyacetylene through Chiral Amplification and Memory. J. Am. Chem. Soc. 2018, 140, 3270–3276. [Google Scholar] [CrossRef]
- Bakasov, A.; Ha, T.-K.; Quack, M. Ab Initio Calculation of Molecular Energies including Parity Violating Interactions. J. Chem. Phys. 1998, 109, 7263–7285. [Google Scholar] [CrossRef]
- Soai, K.; Kawasaki, T. Asymmetric Autocatalysis with Amplification of Chirality. Top. Curr. Chem. 2008, 284, 1–33. [Google Scholar] [CrossRef]
- Green, M.M.; Jain, V. Homochirality in Life: Two Equal Runners, One Tripped. Orig. Life Evol. Biosph. 2010, 40, 111–118. [Google Scholar] [CrossRef] [PubMed]
- Sandars, P.G.H. A Toy Model for the Generation of Homochirality During Polymerization. Orig. Life Evol. Biosph. 2003, 33, 575–587. [Google Scholar] [CrossRef] [PubMed]
- Viedma, C. Selective Chiral Symmetry Breaking during Crystallization: Parity Violation or Cryptochiral Environment in Control? Cryst. Growth Des. 2007, 7, 553–556. [Google Scholar] [CrossRef]
- McLaughlin, D.T.; Nguyen, T.P.T.; Mengnjo, L.; Bian, C.; Leung, Y.H.; Goodfellow, E.; Ramrup, P.; Woo, S.; Cuccia, L.A. Viedma Ripening of Conglomerate Crystals of Achiral Molecules Monitored Using Solid-State Circular Dichroism. Cryst. Growth Des. 2014, 14, 1067–1076. [Google Scholar] [CrossRef]
- Liu, M.; Zhang, L.; Wang, T. Supramolecular Chirality in Self-Assembled Systems. Chem. Rev. 2015, 115, 7304–7397. [Google Scholar] [CrossRef]
- Yashima, E.; Ousaka, N.; Taura, D.; Shimomura, K.; Ikai, T.; Maeda, K. Supramolecular Helical Systems: Helical Assemblies of Small Molecules, Foldamers, and Polymers with Chiral Amplification and Their Functions. Chem. Rev. 2016, 22, 13752–13990. [Google Scholar] [CrossRef]
- Enoto, T.; Wada, Y.; Furuta, Y.; Nakazawa, K.; Yuasa, T.; Okuda, K.; Makishima, K.; Sato, M.; Sato, Y.; Nakano, T.; et al. Photonuclear Reactions Triggered by Lightning Discharge. Nature 2017, 551, 481–484. [Google Scholar] [CrossRef]
- Bargueño, P.; de Tudela, R.P. The Role of Supernova Neutrinos on Molecular Homochirality. Orig. Life Evol. Biosph. 2007, 37, 253–257. [Google Scholar] [CrossRef]
- Alexander, S.; Marcianò, A.; Smolin, L. Gravitational Origin of the Weak Interaction’s Chirality. Phys. Rev. D 2014, 89, 065017. [Google Scholar] [CrossRef]
- Bargueño, P. Gravitational Origin Parity Violation. Chirality 2015, 27, 375–381. [Google Scholar] [CrossRef]
- Ribó, J.M.; Crusats, J.; Sagúes, F.; Claret, J.; Rubires, R. Chiral Sign Induction by Vortices During the Formation of Mesophases in Stirred Solutions. Science 2001, 292, 2063–2066. [Google Scholar] [CrossRef]
- Tamburini, F.; Thidé, B.; Molina-Terriza, G.; Gabriele Anzolin, G. Twisting of Light Around Rotating Black Holes. Nat. Phys. 2011, 7, 195–197. [Google Scholar] [CrossRef]
- Higurashi, E.; Ohguchi, O.; Tamamura, T.; Ukita, H.; Sawada, R. Optically Induced Rotation of Dissymmetrically Shaped Fluorinated Polyimide Micro-Objects in Optical Traps. J. Appl. Phys. 1997, 82, 2773–2779. [Google Scholar] [CrossRef]
- Simpson, N.B.; Dholakia, K.; Allen, L.; Padgett, M.J. Mechanical Equivalence of Spin and Orbital Angular Momentum of Light: An Optical Spanner. Opt. Lett. 1997, 22, 52–54. [Google Scholar] [CrossRef]
- Friese, M.E.J.; Rubinsztein-Dunlop, H.; Gold, J.; Hagberg, P.; Hanstorp, D. Optically Driven Micromachine Elements. Appl. Phys. Lett. 2001, 78, 547–549. [Google Scholar] [CrossRef]
- Curtis, J.E.; Koss, B.A.; Grier, D.G. Dynamic Holographic Optical Tweezers. Opt. Commun. 2002, 207, 169–175. [Google Scholar] [CrossRef]
- Brasselet, E.; Murazawa, N.; Misawa, H.; Juodkazis, S. Optical Vortices from Liquid Crystal Droplets. Phys. Rev. Lett. 2009, 103, 103903. [Google Scholar] [CrossRef]
- Ambrosio, A.; Marrucci, L.; Borbone, F.; Roviello, A.; Maddalena, P. Light-Induced Spiral Mass Transport in Azo-Polymer Films Under Vortex-Beam Illumination. Nat. Commun. 2012, 3, 989. [Google Scholar] [CrossRef]
- Watabe, M.; Juman, G.; Miyamoto, K.; Omatsu, T. Light Induced Conch-Shaped Relief in An Azo-Polymer Film. Sci. Rep. 2014, 4, 4281. [Google Scholar] [CrossRef]
- Brullot, W.; Vanbel, M.K.; Swusten, T.; Verbiest, T. Resolving Enantiomers Using the Optical Angular Momentum of Twisted Light. Sci. Adv. 2016, 2, e1501349. [Google Scholar] [CrossRef]
- Shen, Z.; Su, L.; Yuan, X.-C.; Shen, Y.-C. Trapping and Rotating of a Metallic Particle Trimer with Optical Vortex. Appl. Phys. Lett. 2016, 109, 241901. [Google Scholar] [CrossRef]
- Katoh, M.; Fujimoto, M.; Mirian, N.S.; Konomi, T.; Taira, Y.; Kaneyasu, T.; Kuroda, K.; Miyamoto, A.; Miyamoto, K.; Sasaki, S. Helical Phase Structure of Radiation from an Electron in Circular Motion. Sci Rep. 2017, 7, 6130. [Google Scholar] [CrossRef]
- Taira, Y.; Masahiro Katoh, M. Gamma-Ray Vortices Emitted from Nonlinear Inverse Thomson Scattering of a Two-Wavelength Laser Beam. Phys. Rev. A 2018, 98, 052130. [Google Scholar] [CrossRef]
- Chen, Y.; Gao, J.; Jiao, X.-Q.; Sun, K.; Shen, W.-G.; Qiao, L.-F.; Tang, H.; Lin, X.-F.; Jin, X.-M. Mapping Twisted Light into and Out of a Photonic Chip. Phys. Rev. Lett. 2018, 121, 233602. [Google Scholar] [CrossRef]
- Samlan, C.T.; Suna, R.R.; Naik, D.N.; Viswanathan, N.K. Spin-orbit Beams for Optical Chirality Measurement. Appl. Phys. Lett. 2018, 112, 031101. [Google Scholar] [CrossRef]
- Torrres, J.P.; Torner, L. (Eds.) Twisted photons: Applications of Light with Orbital Angular Momentum; Wiley-VCH: Weinheim, Germany, 2011; ISBN 3527635378. [Google Scholar]
- Inoue, Y.; Ramamurthy, V. (Eds.) Chiral Photochemistry: Molecular and Supramolecular Photochemistry; CRC Press: Tokyo, Japan, 2004; ISBN 9780824757106. [Google Scholar]
- Fujiki, M. Creation and Controlling Asymmetric Small Molecules, Polymers, Colloids, and Small Objects Endowed with Polarized Light and Spin Polarized Particles. Kobunshi Ronbunshu 2017, 74, 114–133. [Google Scholar] [CrossRef]
- Elsila, J.E.; Glavin, D.P.; Dworkin, J.P. Cometary Glycine Detected in Samples Returned by Stardust. Meteorit. Planet. Sci. 2009, 44, 1323–1330. [Google Scholar] [CrossRef]
- Altwegg, K.; Balsiger, H.; Bar-Nun, A.; Berthelier, Je.; Bieler, A.; Bochsler, P.; Briois, C.; Calmonte, U.; Combi, M.R.; Cottin, H.; et al. Prebiotic Chemicals—Amino Acid and Phosphorus—In the Coma of Comet 67P/Churyumov-Gerasimenko. Sci. Adv. 2016, 2, e1600285. [Google Scholar] [CrossRef]
- McGuire, B.A.; Carroll, B.P.; Loomis, R.A.; Finneran, I.A.; Jewell, R.P.; Remijan, A.J.; Blake, G.A. Discovery of the Interstellar Chiral Molecule Propylene Oxide (CH3CHCH2O). Science 2016, 352, 1449–1452. [Google Scholar] [CrossRef]
- Urata, H.; Shinohara, K.; Ogura, E.; Uweda, Y.; Akagi, M. Mirror-image DNA. J. Am. Chem. Soc. 1991, 113, 8174–8175. [Google Scholar] [CrossRef]
- Zawadzke, L.E.; Berg, J.M. A Racemic Protein. J. Am. Chem. Soc. 1992, 114, 4002–4003. [Google Scholar] [CrossRef]
- Riddick, J.A.; Bunger, W.B.; Sakano, T.K. Organic Solvents: Physical Properties and Methods of Purification, 4th ed.; John Wiley & Sons: New York, NY, USA, 1986; ISBN 0-471-08467-0. [Google Scholar]
- Lide, D.R. Handbook of Organic Solvents; CRC Press: Boca Raton, FL, USA, 1994; ISBN 0849389305. [Google Scholar]
- Viswanath, D.S.; Ghosh, T.; Prasad, D.H.L.; Dutt, N.V.K.; Rani, K.Y. Viscosity of Liquids; Theory, Estimation, Experiment and Data; Springer: Berlin, Germany, 2007; ISBN 9048173787. [Google Scholar]
- Properties of Organic Solvents. Available online: http://murov.info/orgsolvents.htm (accessed on 12 June 2018).
- Hardy, R.C.; Cottington, R.L. Viscosity of Deuterium Oxide and Water in the Range 5°C to 125 °C. J. Res. Natl. Bureau Stand. 1949, 42, 573–578. [Google Scholar] [CrossRef]
- Cho, C.H.; Urquidi, J.; Singh, S.; Robinson, G.W. Thermal Offset Viscosities of Liquid H2O, D2O, and T2O. J. Phys. Chem. B 1999, 103, 1991–1994. [Google Scholar] [CrossRef]





© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fujiki, M.; Koe, J.R.; Amazumi, S. Questions of Mirror Symmetry at the Photoexcited and Ground States of Non-Rigid Luminophores Raised by Circularly Polarized Luminescence and Circular Dichroism Spectroscopy. Part 2: Perylenes, BODIPYs, Molecular Scintillators, Coumarins, Rhodamine B, and DCM. Symmetry 2019, 11, 363. https://doi.org/10.3390/sym11030363
Fujiki M, Koe JR, Amazumi S. Questions of Mirror Symmetry at the Photoexcited and Ground States of Non-Rigid Luminophores Raised by Circularly Polarized Luminescence and Circular Dichroism Spectroscopy. Part 2: Perylenes, BODIPYs, Molecular Scintillators, Coumarins, Rhodamine B, and DCM. Symmetry. 2019; 11(3):363. https://doi.org/10.3390/sym11030363
Chicago/Turabian StyleFujiki, Michiya, Julian R. Koe, and Seiko Amazumi. 2019. "Questions of Mirror Symmetry at the Photoexcited and Ground States of Non-Rigid Luminophores Raised by Circularly Polarized Luminescence and Circular Dichroism Spectroscopy. Part 2: Perylenes, BODIPYs, Molecular Scintillators, Coumarins, Rhodamine B, and DCM" Symmetry 11, no. 3: 363. https://doi.org/10.3390/sym11030363
APA StyleFujiki, M., Koe, J. R., & Amazumi, S. (2019). Questions of Mirror Symmetry at the Photoexcited and Ground States of Non-Rigid Luminophores Raised by Circularly Polarized Luminescence and Circular Dichroism Spectroscopy. Part 2: Perylenes, BODIPYs, Molecular Scintillators, Coumarins, Rhodamine B, and DCM. Symmetry, 11(3), 363. https://doi.org/10.3390/sym11030363