Balanced Truncation Model Order Reduction in Limited Frequency and Time Intervals for Discrete-Time Commensurate Fractional-Order Systems
Abstract
:1. Introduction
2. System Representation
3. Model Order Reduction
4. Controllability and Observability Gramians for Discrete-Time Fractional-Order Systems
4.1. Gramians in the Time Domain
4.2. Gramians in the Frequency Domain
4.3. Frequency Weighted Gramians
5. Simulation Examples
6. Conclusions
Supplementary Materials
Author Contributions
Funding
Conflicts of Interest
References
- Ferdi, Y. Computation of Fractional Order Derivative and Integral via Power Series Expansion and Signal Modelling. Nonlinear Dyn. 2006, 46, 1–15. [Google Scholar] [CrossRef]
- Dhabale, A.S.; Dive, R.; Aware, M.V.; Das, S. A New Method for Getting Rational Approximation for Fractional Order Differintegrals. Asian J. Control 2015, 17, 2143–2152. [Google Scholar] [CrossRef]
- Vinagre, B.M.; Podlubny, I.; Hernandez, A.; Feliu, V. Some approximations of fractional order operators used in control theory and applications. Fractional Calculus Appl. Anal. 2000, 3, 231–248. [Google Scholar]
- Xue, D.; Zhao, C.; Chen, Y. A Modified Approximation Method of Fractional Order System. In Proceedings of the 2006 International Conference on Mechatronics and Automation, Luoyang, China, 25–28 June 2006; pp. 1043–1048. [Google Scholar]
- Dzieliński, A.; Sierociuk, D. Stability of Discrete Fractional Order State-space Systems. J. Vib. Control 2008, 14, 1543–1556. [Google Scholar] [CrossRef]
- Stanisławski, R.; Latawiec, K.J. Fractional-order discrete-time Laguerre filters—A new tool for modeling and stability analysis of fractional-order LTI SISO systems. Discrete Dyn. Nat. Soc. 2016, 2016, 1–9. [Google Scholar] [CrossRef]
- Du, B.; Wei, Y.; Liang, S.; Wang, Y. Rational approximation of fractional order systems by vector fitting method. Int. J. Control Autom. Syst. 2017, 15, 186–195. [Google Scholar] [CrossRef]
- Stanisławski, R.; Rydel, M.; Latawiec, K.J. Modeling of discrete-time fractional-order state space systems using the balanced truncation method. J. Franklin Inst. 2017, 354, 3008–3020. [Google Scholar] [CrossRef]
- Krajewski, W.; Viaro, U. A method for the integer-order approximation of fractional-order systems. J. Franklin Inst. 2014, 351, 555–564. [Google Scholar] [CrossRef]
- Rydel, M. New integer-order approximations of discrete-time non-commensurate fractional-order systems using the cross Gramian. Adv. Comput. Math. 2018. [Google Scholar] [CrossRef]
- Tavakoli-Kakhki, M.; Haeri, M. Model reduction in commensurate fractional-order linear systems. Proc. Inst. Mech. Eng. Part I J. Syst. Control Eng. 2009, 223, 493–505. [Google Scholar] [CrossRef]
- Garrappa, R.; Maione, G. Model order reduction on Krylov subspaces for fractional linear systems. IFAC Proc. Volumes 2013, 46, 143–148. [Google Scholar] [CrossRef]
- Shen, J.; Lam, J. H∞ Model Reduction for Positive Fractional Order Systems. Asian J. Control 2014, 16, 441–450. [Google Scholar] [CrossRef]
- Jiang, Y.L.; Xiao, Z.H. Arnoldi-based model reduction for fractional order linear systems. Int. J. Syst. Sci. 2015, 46, 1411–1420. [Google Scholar] [CrossRef]
- Rydel, M.; Stanisławski, R.; Latawiec, K.J.; Gałek, M. Model order reduction of commensurate linear discrete-time fractional-order systems. IFAC PapersOnLine 2018, 51, 536–541. [Google Scholar] [CrossRef]
- Gawronski, W.; Juang, J. Model reduction in limited time and frequency intervals. Int. J. Syst. Sci. 1990, 21, 349–376. [Google Scholar] [CrossRef]
- Benner, P.; Kürschner, P.; Saak, J. Frequency-Limited Balanced Truncation with Low-Rank Approximations. SIAM J. Sci. Comput. 2016, 38, A471–A499. [Google Scholar] [CrossRef]
- Zulfiqar, U.; Imran, M.; Ghafoor, A.; Liaquat, M. A New Frequency-Limited Interval Gramians-Based Model Order Reduction Technique. IEEE Trans. Circuits Syst. Express Briefs 2017, 64, 680–684. [Google Scholar] [CrossRef]
- Kürschner, P. Balanced truncation model order reduction in limited time intervals for large systems. Adv. Comput. Math. 2018, 44, 1821–1844. [Google Scholar] [CrossRef]
- Enns, D. Model reduction with balanced realizations: An error bound and frequency weighted generalization. In Proceedings of the 23rd IEEE Conference on Decision and Control, Las Vegas, NV, USA, 12–14 December 1984; pp. 127–132. [Google Scholar]
- Lin, C.; Chiu, T. Model reduction via frequency weighted balanced realization. Theory Adv. Technol. 1992, 8, 341–451. [Google Scholar]
- Wang, G.; Sreeram, V.; Liu, W.Q. A new frequency-weighted balanced truncation method and an error bound. IEEE Trans. Autom. Control 1999, 44, 1734–1737. [Google Scholar] [CrossRef]
- Varga, A.; Anderson, B. Accuracy-enhancing methods for balancing-related frequency-weighted model and controller reduction. Automatica 2003, 39, 919–927. [Google Scholar] [CrossRef]
- Sreeram, V.; Sahlan, S. Improved results on frequency-weighted balanced truncation and error bounds. Int. J. Robust Nonlin. 2012, 22, 1195–1211. [Google Scholar] [CrossRef]
- Imran, M.; Ghafoor, A.; Sreeram, V. A frequency weighted model order reduction technique and error bounds. Automatica 2014, 50, 3304–3309. [Google Scholar] [CrossRef]
- Rydel, M.; Stanisławski, R. A new frequency weighted Fourier-based method for model order reduction. Automatica 2018, 88, 107–112. [Google Scholar] [CrossRef]
- Monje, C.; Chen, Y.; Vinagre, B.; Xue, D.; Feliu-Batlle, V. Fractional-order Systems and Controls: Fundamentals and Applications; Series on Advances in Industrial Control; Springer: London, UK, 2010. [Google Scholar]
- Podlubny, I. Fractional Differential Equations; Academic Press: Orlando, FL, USA, 1999. [Google Scholar]
- Moore, B. Principal component analysis in linear systems: controllability, observability and model reduction. IEEE Trans. Autom. Control 1981, AC–26, 17–32. [Google Scholar] [CrossRef]
- Safonov, M.G.; Chiang, R.Y. A Schur Method for Balanced-Truncation Model Reduction. IEEE Trans. Autom. Control 1989, 34, 729–733. [Google Scholar] [CrossRef]
- Antoulas, A. Approximation of Large-Scale Dynamical System; SIAM: Philadelphia, PA, USA, 2005. [Google Scholar]
- Laub, A.; Heath, M.; Paige, C.; Ward, R. Computation of system balancing transformations and other applications of simultaneous diagonalization algorithms. IEEE Trans. Autom. Control 1987, 32, 115–122. [Google Scholar] [CrossRef]
- Rydel, M.; Stanisławski, W. Selection of reduction parameters for complex plant MIMO LTI models using the evolutionary algorithm. Math. Comput. Simul 2017, 140, 94–106. [Google Scholar] [CrossRef]
- Penzl, T. Eigenvalue decay bounds for solutions of Lyapunov equations: The symmetric case. Syst. Control Lett. 2000, 40, 139–144. [Google Scholar] [CrossRef]
- Sadkane, M. A low-rank Krylov squared Smith method for large-scale discrete-time Lyapunov equations. Linear Algebra Appl. 2012, 436, 2807–2827. [Google Scholar] [CrossRef]
- Penzl, T. Algorithms for Model Reduction of Large Dynamical Systems. Linear Algebra Appl. 2006, 415, 322–343. [Google Scholar] [CrossRef]
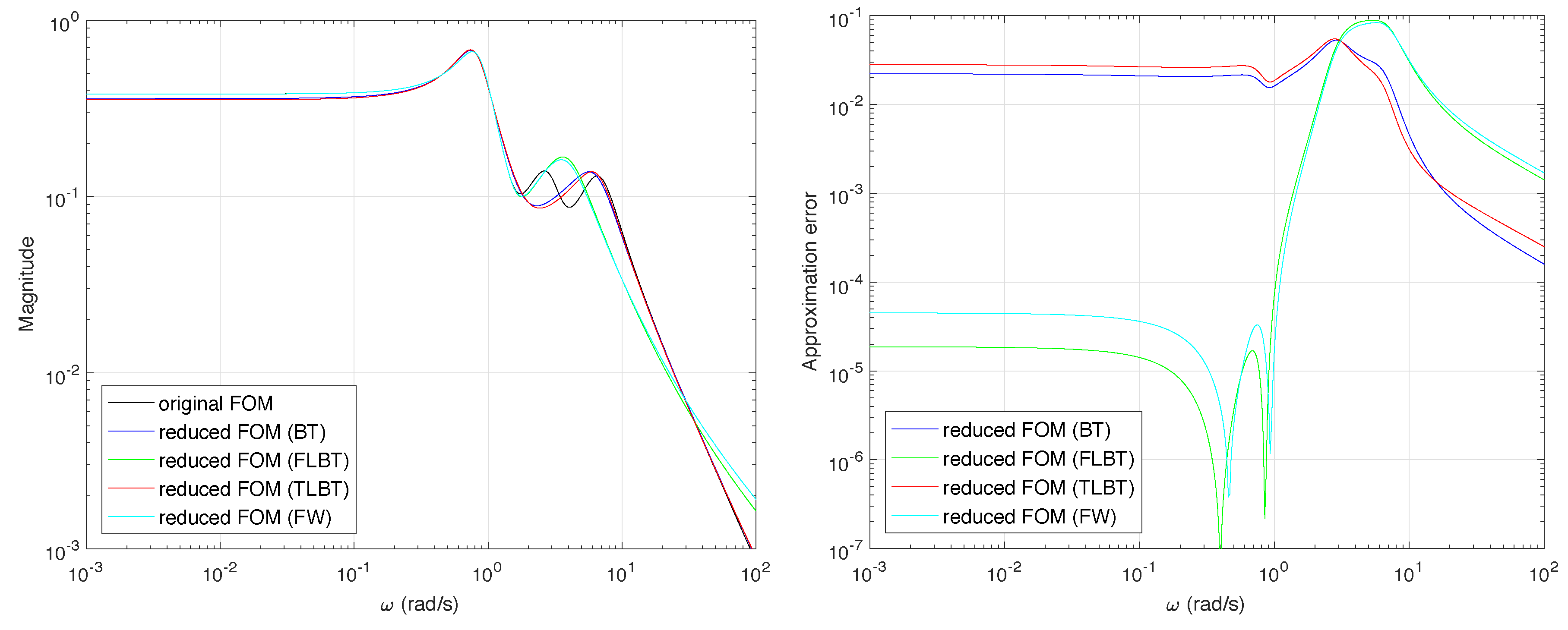
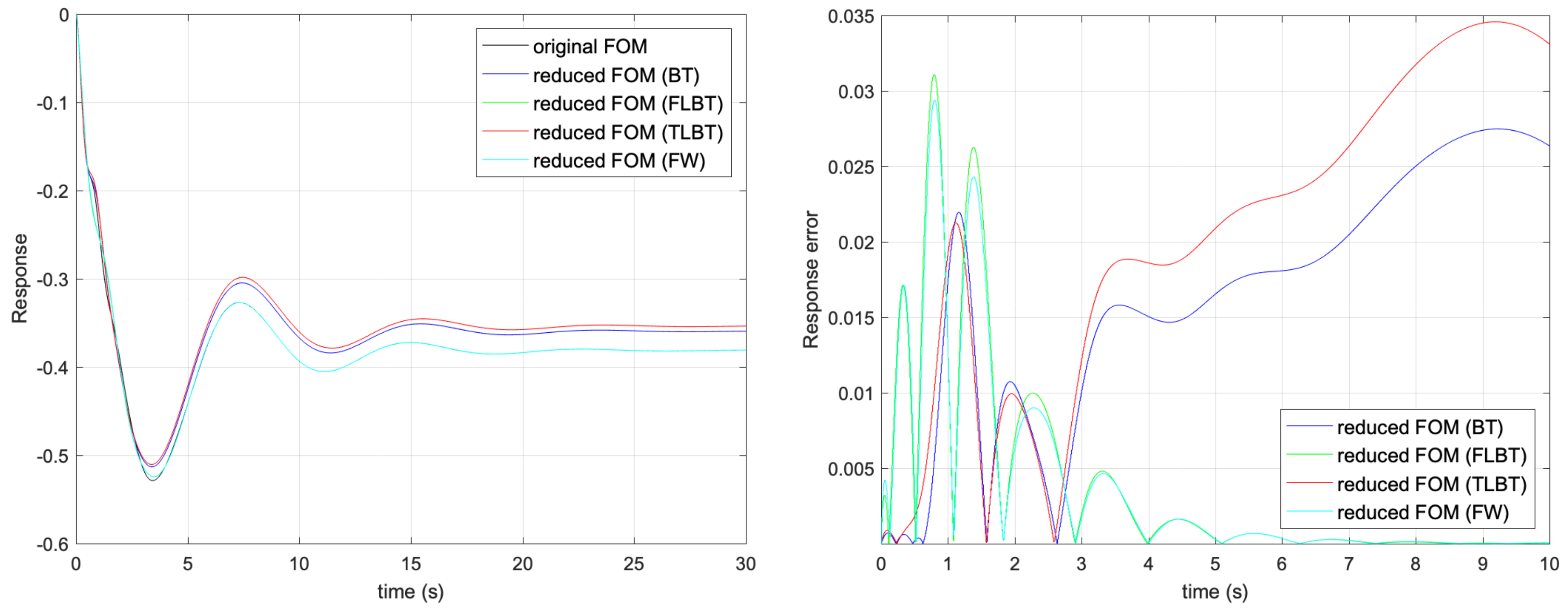
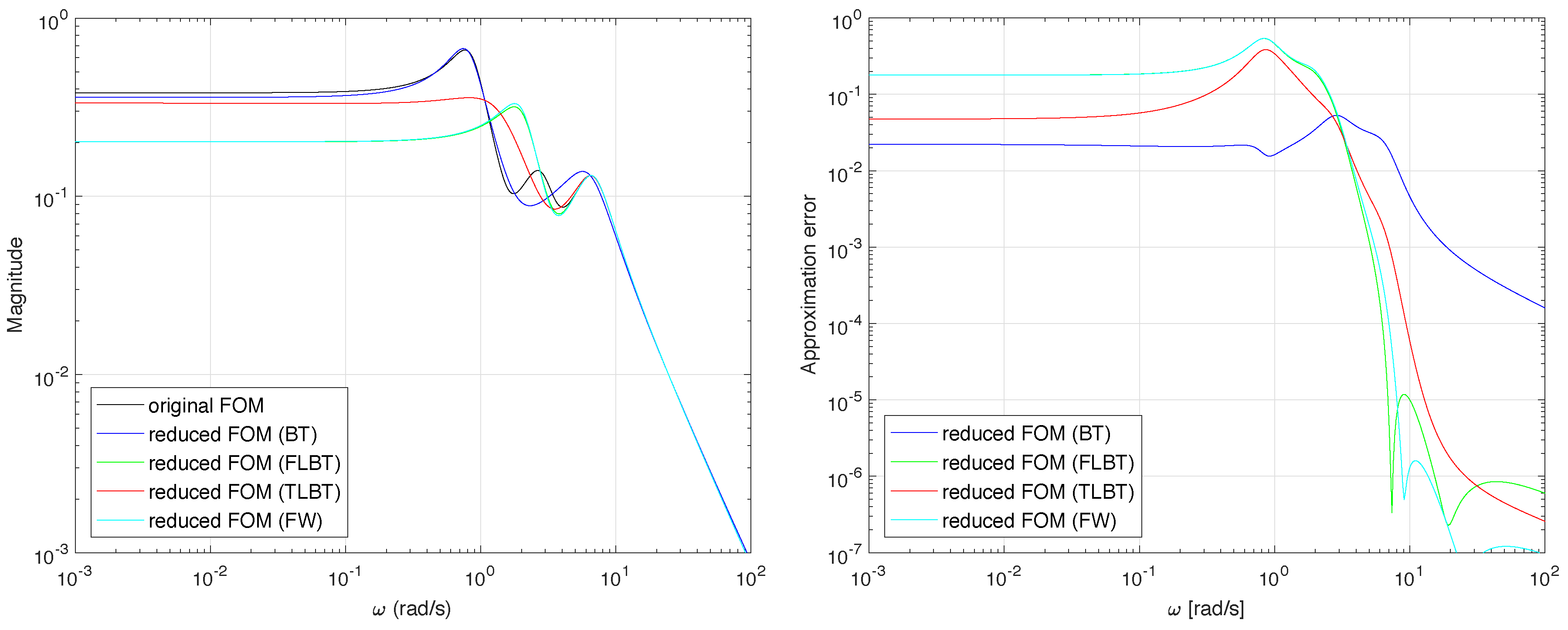
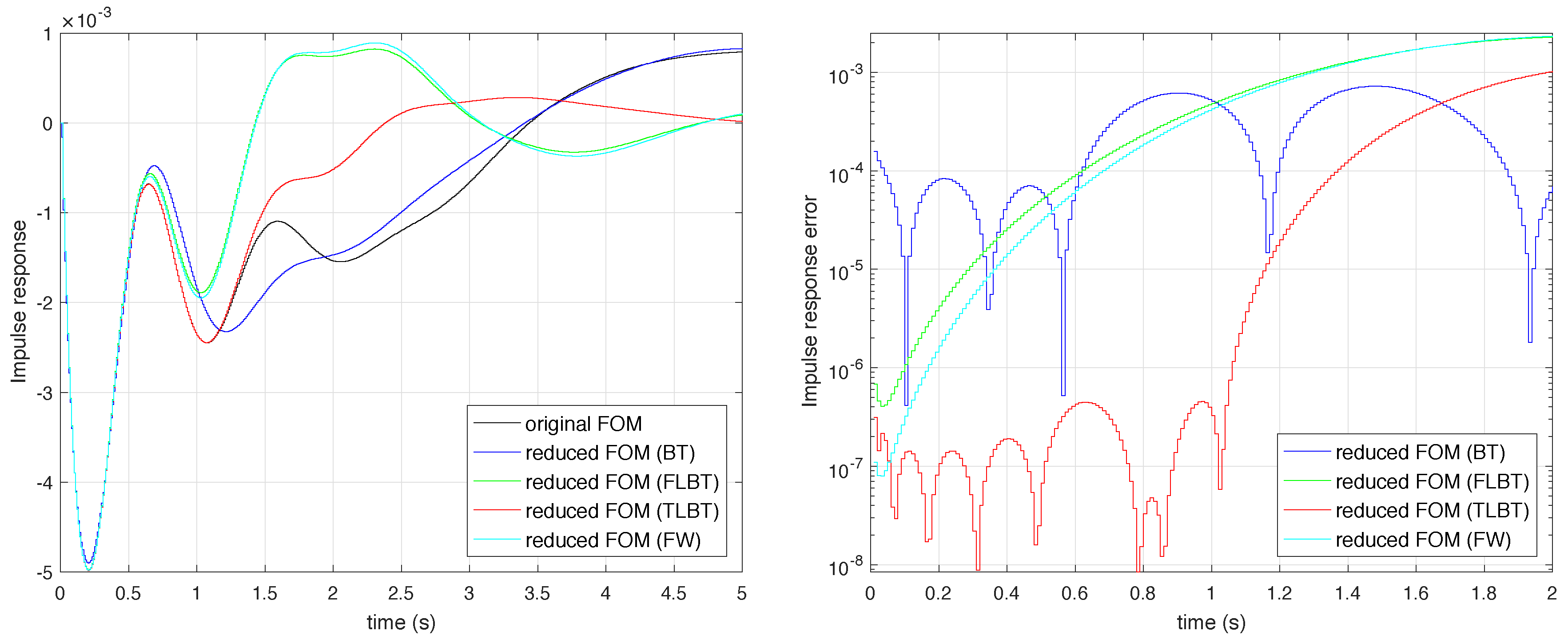
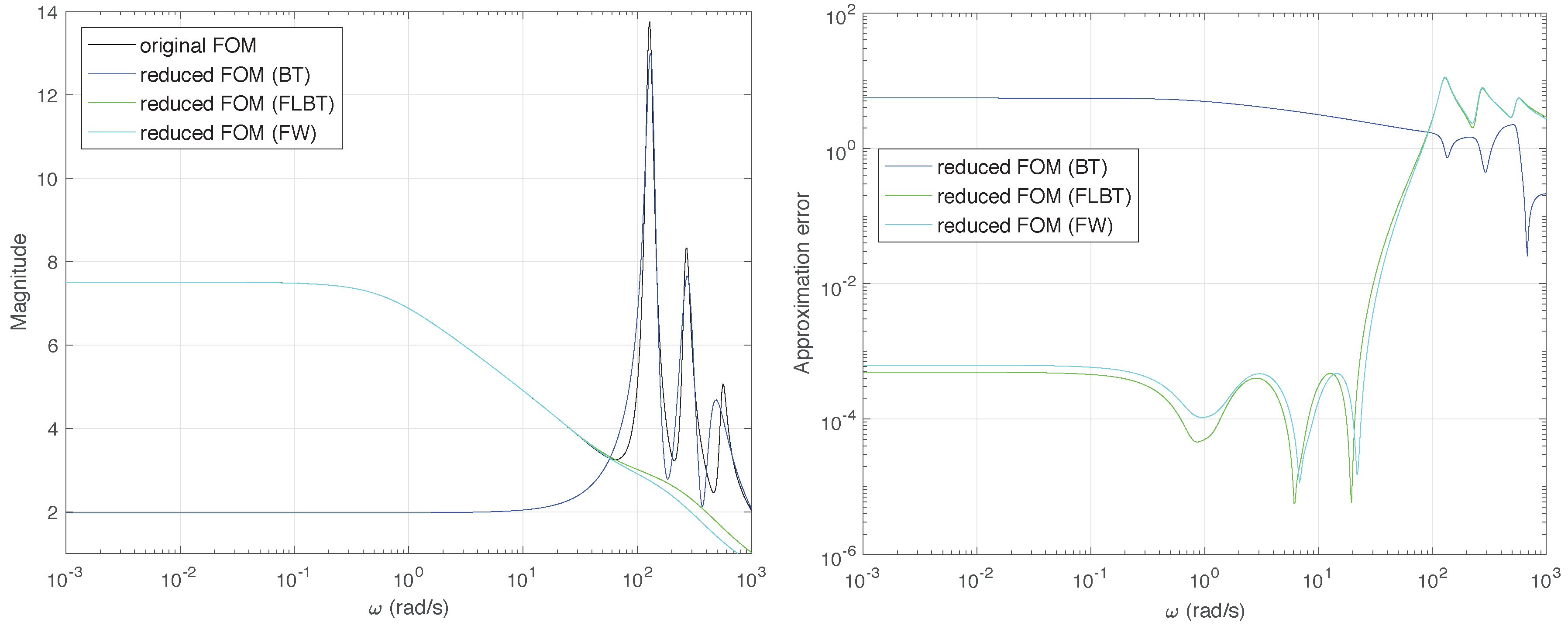
| BT | ||||
| FLBT | ||||
| TLBT | ||||
| FW |
| BT | |||
| FLBT | |||
| TLBT | |||
| FW |
| BT | |||
| FLBT | |||
| FW |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rydel, M.; Stanisławski, R.; Latawiec, K.J. Balanced Truncation Model Order Reduction in Limited Frequency and Time Intervals for Discrete-Time Commensurate Fractional-Order Systems. Symmetry 2019, 11, 258. https://doi.org/10.3390/sym11020258
Rydel M, Stanisławski R, Latawiec KJ. Balanced Truncation Model Order Reduction in Limited Frequency and Time Intervals for Discrete-Time Commensurate Fractional-Order Systems. Symmetry. 2019; 11(2):258. https://doi.org/10.3390/sym11020258
Chicago/Turabian StyleRydel, Marek, Rafał Stanisławski, and Krzysztof J. Latawiec. 2019. "Balanced Truncation Model Order Reduction in Limited Frequency and Time Intervals for Discrete-Time Commensurate Fractional-Order Systems" Symmetry 11, no. 2: 258. https://doi.org/10.3390/sym11020258
APA StyleRydel, M., Stanisławski, R., & Latawiec, K. J. (2019). Balanced Truncation Model Order Reduction in Limited Frequency and Time Intervals for Discrete-Time Commensurate Fractional-Order Systems. Symmetry, 11(2), 258. https://doi.org/10.3390/sym11020258





