A MAGDM Algorithm with Multi-Granular Probabilistic Linguistic Information
Abstract
:1. Introduction
2. Preliminaries
3. Main Results
3.1. Definitions of Multi-Granular Probabilistic Linguistic Term Sets
- (1)
- If , then by Equation (2), we calculate
- (2)
- If , then by Definition 4, we add some elements to the one with the smaller number of elements.
3.2. Distance Measures between Multi-Granular PLTSs
- (1)
- 0 1;
- (2)
- 0, if and only if ; and
- (3)
- .
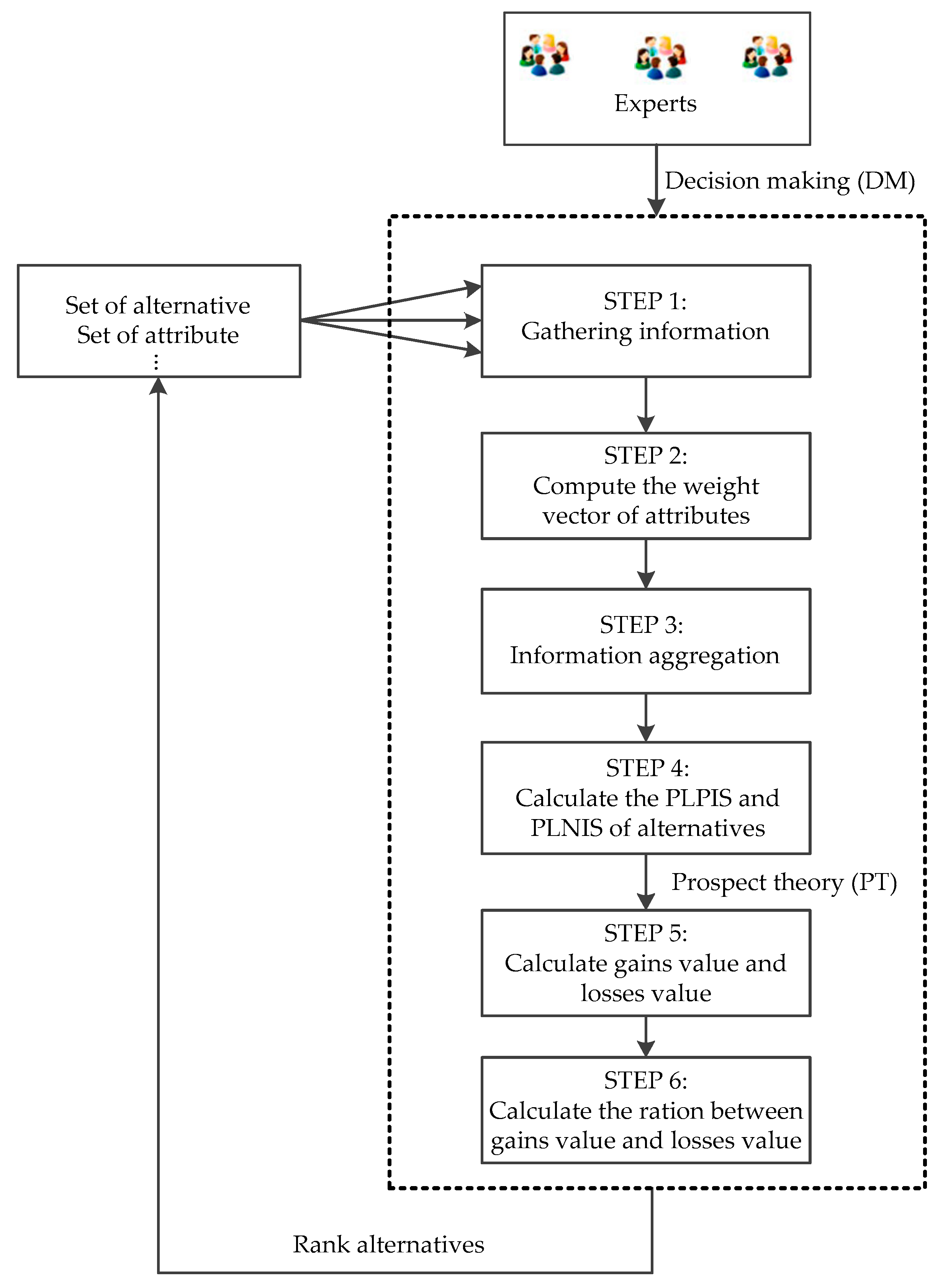
3.3. A MAGDM Algorithm Based on PT
4. Case Studies
4.1. The Applications of the Algorithm
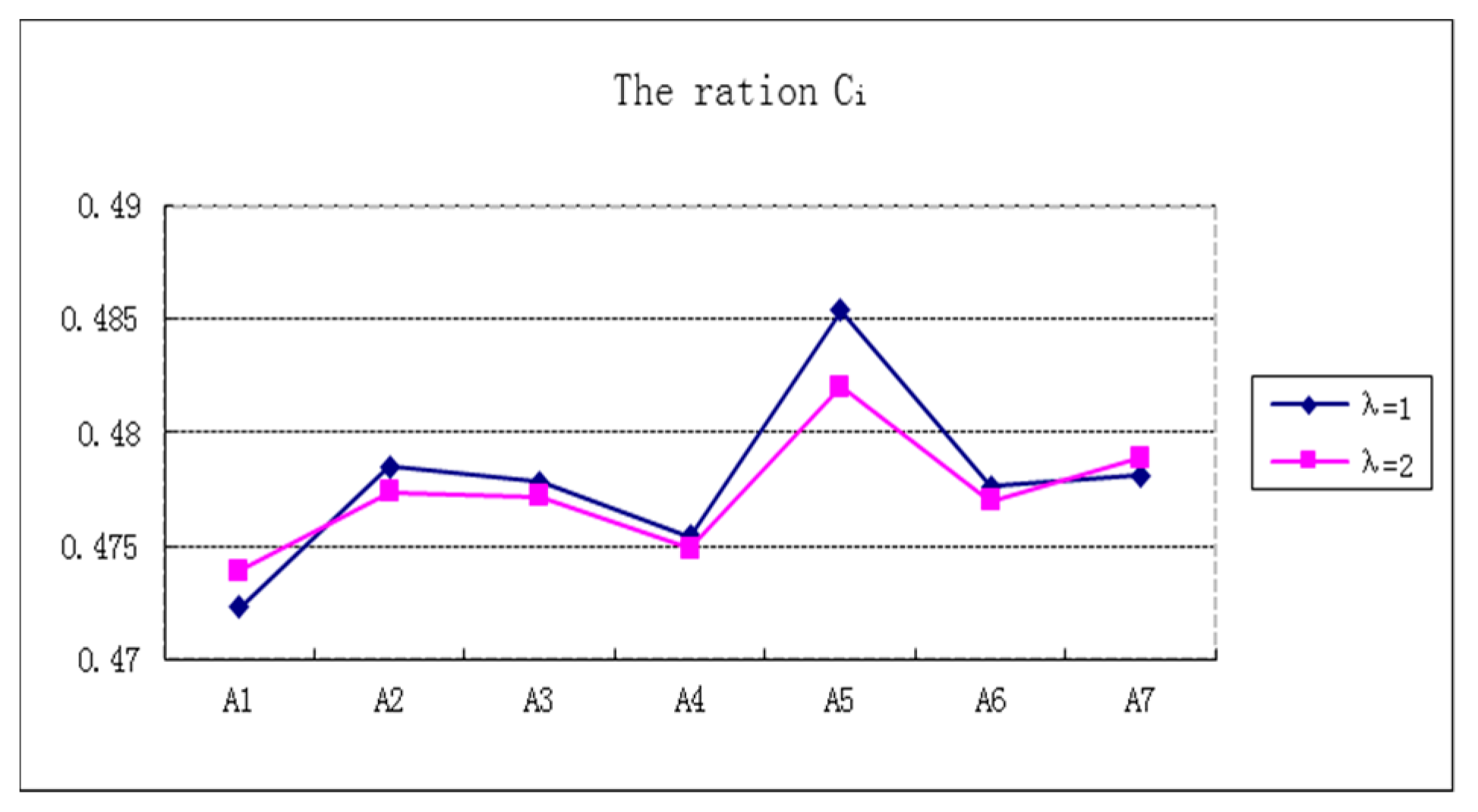
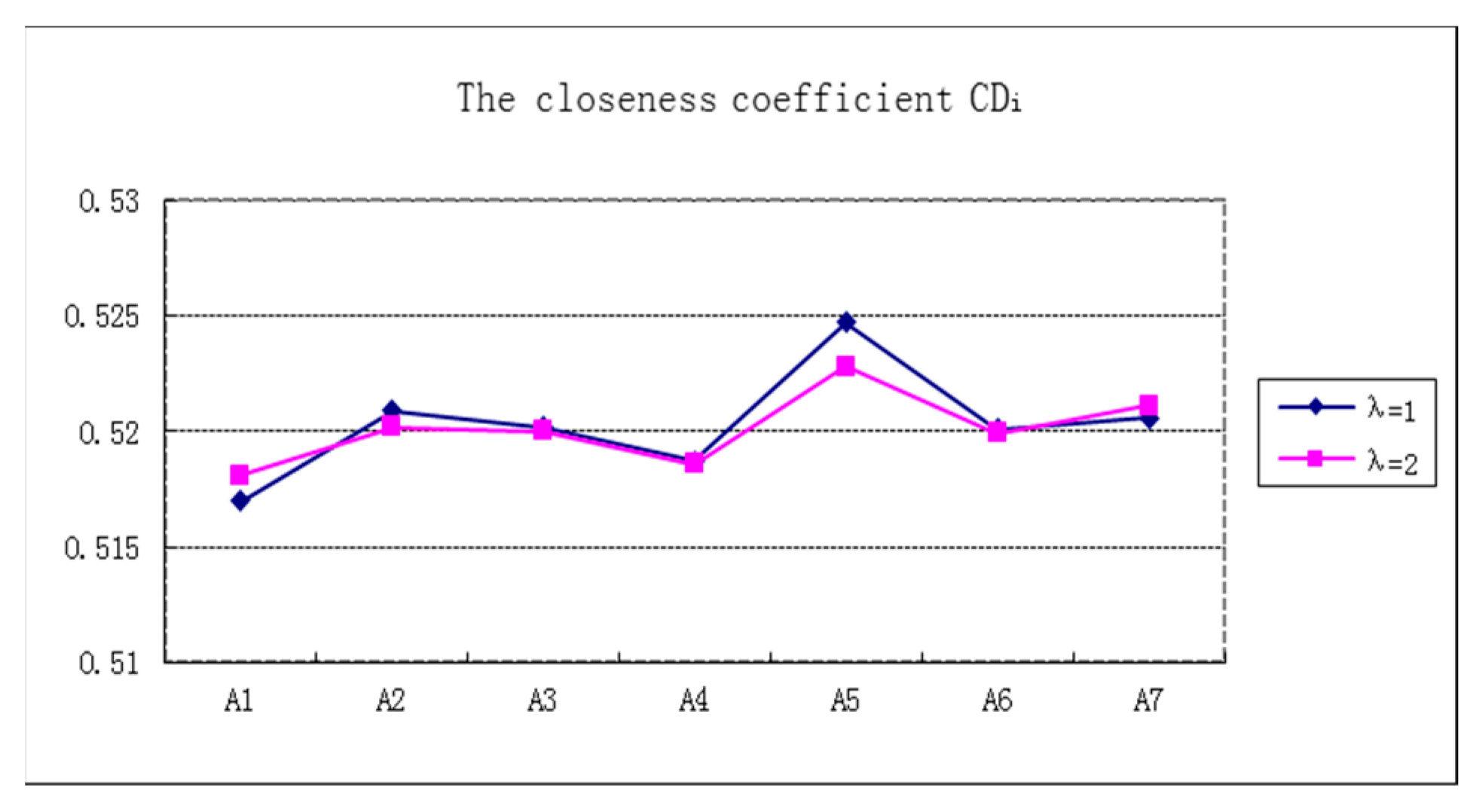
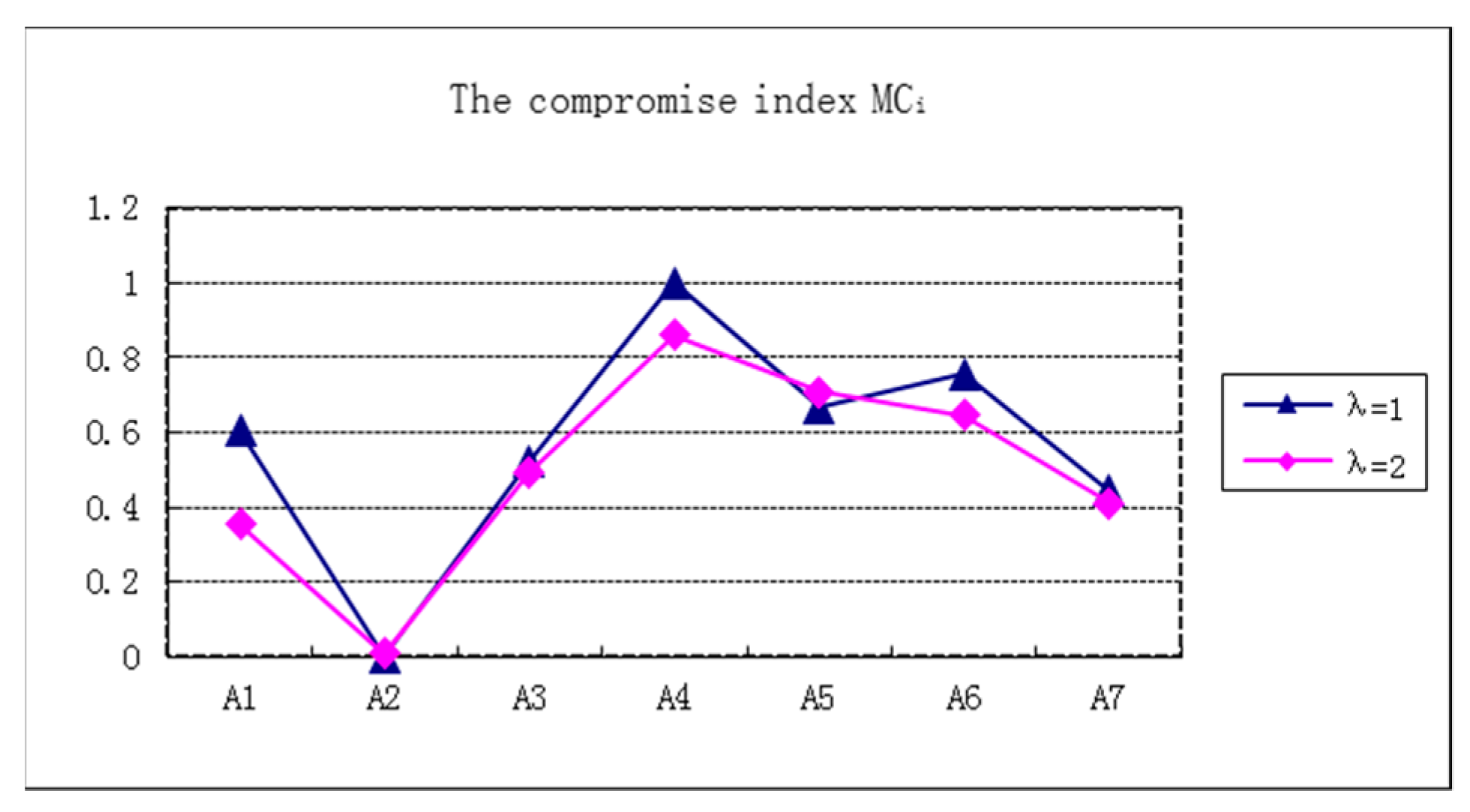
4.2. Sensitivity Analysis
4.3. Comparative Analysis
4.4. The Second Case Study
Funding
Conflicts of Interest
References
- Zhou, L.G.; Chen, H.Y. Continous ordered linguistic distance measure and its application to multiple attribute group decision making. Group Decis. Negot. 2013, 22, 739–758. [Google Scholar] [CrossRef]
- Parreiras, R.O.; Ekel, P.Y.; Martni, J.S.C.; Palhares, R.M. A flexible consensus scheme for multicriteria group decision making under linguistiic assessments. Inf. Sci. 2010, 180, 1075–1089. [Google Scholar] [CrossRef]
- Grossglauser, M.; Saner, H. Data–driven healthcare: From patterns to actions. Eur. J. Prev. Cardiol. 2014, 21, 14–17. [Google Scholar] [CrossRef]
- Celotto, A.; Loia, V.; Senatore, S. Fuzzy linguistic approach to quality assessment model for electricity network infrastructure. Inf. Sci. 2015, 304, 1–15. [Google Scholar] [CrossRef]
- Tao, Z.F.; Chen, H.Y.; Zhou, L.G.; Liu, J.P. 2-Tuple linguistic soft set and its application to group decision making. Soft Comput. 2015, 19, 1201–1213. [Google Scholar] [CrossRef]
- Sengupta, A.T.; Pal, K.; Zhou, L.G.; Chen, H.Y. Fuzzy Preference Ordering of Interval Numbers in Decision Problems; Springer: Heidelberg, Germany, 2009; Volume 238, pp. 140–143. [Google Scholar]
- Zadeh, L.A. Fuzzy sets. Inf. Control 1965, 3, 338–353. [Google Scholar] [CrossRef]
- Xu, Z.S. Linguistic Decision Making: Theory and Methods; Springer: Berlin/Heidelberg, Germany, 2012. [Google Scholar]
- Herrera, F.; Verdegay, J.L. Linguistic assessments in group decision. In Proceedings of the First European Congress on Fuzzy and Intelligent Technologies, Aachen, Germany, 7–10 September 1993; pp. 941–948. [Google Scholar]
- Herrera, F.; Herrera-Viedma, E.; Verdegay, J.L. A model of consensus in group decision making under linguistic assessments. Fuzzy Sets Syst. 1996, 78, 73–87. [Google Scholar] [CrossRef]
- Herrera, F.; Herrera-Viedma, E.; Verdegay, J.L. A rational consensus model in group decision making using linguistic assessments. Fuzzy Sets Syst. 1997, 88, 31–49. [Google Scholar] [CrossRef]
- Xu, Z.S. A method for multiple attribute decision making with incomplete weight information in linguistic setting. Knowl.-Based Syst. 2007, 20, 719–725. [Google Scholar] [CrossRef]
- Arieh, D.B.; Chen, Z. Linguistic group decision-making: Opinion aggregation and measures of consensus. Fuzzy Opt. Decis. Mak. 2006, 5, 371–386. [Google Scholar] [CrossRef]
- Xu, Z.S. An approach based on the uncertain LOWG and induced uncertain LOWG operators to group decision making with uncertain multiplicative linguistic preference relations. Decis. Support Syst. 2006, 41, 488–499. [Google Scholar] [CrossRef]
- Liu, P.D.; Mahmood, T.; Khan, Q. Multi-Attribute Decision-Making Based on Prioritized Aggregation Operator under Hesitant. Symmetry 2017, 9, 11. [Google Scholar] [CrossRef]
- Liao, H.C.; Zhang, C.; Luo, L. A multiple attribute group decision making method based on two novel intuitionistic multiplicative distance measures. Inf. Sci. 2018, 467, 766–783. [Google Scholar] [CrossRef]
- Rodríguez, R.M.; Martínez, L.; Herrera, F. Hesitant fuzzy linguistic term sets for decision making. IEEE Trans. Fuzzy Syst. 2012, 20, 109–119. [Google Scholar] [CrossRef]
- Torra, V. Hesitant fuzzy sets. Int. J. Intell. Syst. 2010, 25, 529–539. [Google Scholar] [CrossRef]
- Zadeh, L.A. The concept of a linguistic variable and its application to approximate reasoning—I. Inf. Sci. 1975, 8, 199–249. [Google Scholar] [CrossRef]
- Dong, Y.C.; Wu, Y.Z.; Zhang, H.J.; Zhang, G.Q. Multi-granular unbalanced linguistic distribution assessments with interval symbolic proportions. Knowl.-Based Syst. 2015, 82, 139–151. [Google Scholar] [CrossRef]
- Wu, Z.B.; Xu, J.P. Possibility distribution-based approach for MAGDM with hesitant fuzzy linguistic information. IEEE Trans. Cybern. 2016, 46, 694–705. [Google Scholar] [CrossRef]
- Zhang, G.Q.; Dong, Y.C.; Xu, Y.F. Consistency and consensus measures for linguistic preference relations based on distribution assessments. Inf. Fusion 2014, 17, 46–55. [Google Scholar] [CrossRef]
- Liu, H.B.; Rodriguez, R.M. A fuzzy envelope for hesitant fuzzy linguistic term set and its application to multi-criteria decision making. Inf. Sci. 2014, 258, 220–238. [Google Scholar] [CrossRef]
- Yang, J.B. Rule and utility based evidential reasoning approach for multi-attribute decision analysis under uncertainties. Eur. J. Oper. Res. 2001, 131, 31–61. [Google Scholar] [CrossRef]
- Yang, J.B.; Xu, D.L. On the evidential reasoning algorithm for multiple attribute decision analysis under uncertainty. IEEE Trans. Syst. Man Cybern. Part A Syst. Hum. 2002, 32, 289–304. [Google Scholar] [CrossRef]
- Wang, J.H.; Hao, J.Y. A new version of 2-tuple fuzzy linguistic representation model for computing with words. IEEE Trans. Fuzzy Syst. 2006, 14, 435–445. [Google Scholar] [CrossRef]
- Pang, Q.; Wang, H.; Xu, Z.S. Probabilistic linguistic term sets in multi-attribute group decision making. Inf. Sci. 2016, 369, 128–143. [Google Scholar] [CrossRef]
- Lin, M.W.; Xu, Z.S.; Zhai, Y.L.; Yao, Z.Q. Multi-attribute group decision-making under probabilistic uncertain linguistic environment. J. Oper. Res. Soc. 2017, 1–15. [Google Scholar] [CrossRef]
- Xu, Z.S.; Zhou, W. Consensus building with a group of decision makers under the hesitant probabilistic fuzzy environment. Fuzzy Opt. Decis. Mak. 2017, 16, 481–503. [Google Scholar] [CrossRef]
- Lin, M.W.; Xu, Z.S. Probabilistic Linguistic Distance Measures and Their Applications in Multi-criteria Group Decision Making. In Soft Computing Applications for Group Decision-Making and Consensus Modeling; Springer: Cham, Switzerland, 2018; Volume 357, pp. 411–440. [Google Scholar]
- Kobina, A.; Liang, D.C. Probabilistic linguistic power aggregation operators for multi-criteria group decision making. Symmetry 2017, 9, 12. [Google Scholar] [CrossRef]
- Xu, Z.S.; Wang, H. Managing multi-granularity linguistic information in qualitative group decision making: An overview. Granul. Comput. 2016, 1, 21–35. [Google Scholar] [CrossRef]
- Wang, H.; Xu, Z.S.; Zeng, X.J. Hesitant fuzzy linguistic term sets for linguistic decision making: Current developments, issues and challenges. Inf. Fusion 2018, 43, 1–12. [Google Scholar] [CrossRef]
- Gorzalczany, M.B. An interval-valued fuzzy inference method-some basic properties. Fuzzy Sets Syst. 1989, 31, 243–251. [Google Scholar] [CrossRef]
- Atanassov, K.T. Intuitionistic fuzzy sets. Fuzzy Sets Syst. 1986, 20, 87–96. [Google Scholar] [CrossRef]
- Atanassov, K.T.; Gargov, G. Interval–valued intuitionistic fuzzy sets. Fuzzy Sets Syst. 1989, 31, 343–349. [Google Scholar] [CrossRef]
- Chen, N.; Xu, Z.S.; Xia, M.M. Interval-valued hesitant preference relations and their applications to group decision making. Knowl-Based Syst. 2013, 37, 528–540. [Google Scholar] [CrossRef]
- W, L.; W, Y.M.; Martinez, L. A group decision method based on prospect theory for emergency situations. Inf. Sci. 2017, 418, 119–135. [Google Scholar]
- Yao, S.; Yu, D.; Song, Y.; Yao, H.; Hu, Y.; Guo, B. Dry bulk carrier investment selection through a dual group decision fusing mechanism in the green supply chain. Sustainability 2018, 10, 4528. [Google Scholar] [CrossRef]
- Herrera, F.; Herrera-Viedma, E.; Verdegay, J.L. A sequential selection process in group decision making with a linguistic assessment approach. Inf. Sci. 1995, 85, 223–239. [Google Scholar] [CrossRef]
- Rodríguez, R.M.; Martínez, L.; Herrera, F. A group decision making model dealing with comparative linguistic expressions based on hesitant fuzzy linguistic term sets. Inf. Sci. 2013, 241, 28–42. [Google Scholar] [CrossRef]
- Yager, R.R. Generalized OWA aggregation operators. Fuzzy Opt. Decis. Mak. 2004, 3, 93–107. [Google Scholar] [CrossRef]
- Ma, J.; Fan, Z.P.; Huang, L.H. A subjective and objective integrated approach to determine attribute weights. Eur. J. Oper. Res. 1999, 112, 397–404. [Google Scholar] [CrossRef]
- Kahneman, D.; Tversky, A. Prospect theory: An analysis of decision under risk. Econometrica 1979, 47, 263–292. [Google Scholar] [CrossRef]
- Xu, Z.S.; Xia, M.M. Hesitant Fuzzy entropy and cross-entropy and their use in multi-attribute decision-making. Int. J. Intell. Syst. 2012, 27, 799–822. [Google Scholar] [CrossRef]
- Wang, L.Z.; Zhang, X.; Wang, Y.M. A prospect theory-based interval dynamic reference point method for emergency decision making. Expert Syst. Appl. 2015, 42, 9379–9388. [Google Scholar] [CrossRef]
- Zhang, X.L.; Xu, Z.S. The TODIM analysis approach based on novel measured functions under hesitant fuzzy environment. Knowl.-Based Syst. 2014, 61, 48–58. [Google Scholar] [CrossRef]
| Alternative | ||||||||
|---|---|---|---|---|---|---|---|---|
| Alternative | ||||||||
|---|---|---|---|---|---|---|---|---|
| Alternative | ||||||||
|---|---|---|---|---|---|---|---|---|
| Alternative | ||||
| Alternative | ||||
| . | ||||
| Alternative | ||||
| Alternative | ||||
| . |
| Attribute | ||||||||
| Weight | 0.0922 | 0.1481 | 0.1022 | 0.1023 | 0.1815 | 0.0832 | 0.1948 | 0.0955 |
| Alternative | ||||
| (0.0307,0.0273,0.0263) | (0.0494,0.0329,0.0212) | (0.0511,0.0397,0.0000) | (0.0303,0.0273,0.0244) | |
| (0.0294,0.0263,0.0239) | (0.0470,0.0384,0.0212) | (0.0341,0.0326,0.0265) | (0.0336,0.0303,0.0244) | |
| (0.0307,0.0282,0.0220) | (0.0428,0.0329,0.0212) | (0.0511,0.0449,0.0000) | (0.0334,0.0303,0.0244) | |
| (0.0615,0.0297,0.0000) | (0.0483,0.0219,0.0212) | (0.0281,0.0243,0.0151) | (0.0341,0.0327,0.0244) | |
| (0.0283,0.0273,0.0220) | (0.0399,0.0282,0.0274) | (0.0292,0.0277,0.0114) | (0.0283,0.0244,0.0114) | |
| (0.0263,0.0261,0.0137) | (0.0375,0.0329,0.0212) | (0.0681,0.0298,0.0000) | (0.0341,0.0298,0.0292) | |
| (0.0220,0.0218,0.0205) | (0.0355,0.0353,0.0274) | (0.0303,0.0298,0.0195) | (0.0438,0.0284,0.0000) | |
| Alternative | ||||
| (0.0605,0.0269,0.0173) | (0.0555,0.0247,0.0000) | (0.0519,0.0505,0.0186) | (0.0318,0.0248,0.0182) | |
| (0.0538,0.0403,0.0346) | (0.0277,0.0270,0.0216) | (0.0539,0.0433,0.0186) | (0.0318,0.0311,0.0227) | |
| (0.0489,0.0346,0.0269) | (0.0241,0.0238,0.0123) | (0.0478,0.0371,0.0072) | (0.0277,0.0273,0.0248) | |
| (0.0413,0.0202,0.0173) | (0.0277,0.0257,0.0185) | (0.0693,0.0108,0.0000) | (0.0281,0.0227,0.0212) | |
| (0.0445,0.0269,0.0259) | (0.0277,0.0269,0.0216) | (0.0492,0.0289,0.0186) | (0.0286,0.0273,0.0248) | |
| (0.0439,0.0432,0.0134) | (0.0257,0.0247,0.0238) | (0.0526,0.0289,0.0278) | (0.0283,0.0283,0.0182) | |
| (0.0427,0.0403,0.0259) | (0.0256,0.0247,0.0198) | (0.0577,0.0434,0.0278) | (0.0318,0.0280,0.0227) |
| Attribute | ||||
| PLPIS | (0.0615,0.0297,0.0263) | (0.0494,0.0384,0.0274) | (0.0681,0.0449,0.0265) | (0.0438,0.0327,0.0292) |
| Attribute | ||||
| PLPIS | (0.0605,0.0432,0.0346) | (0.0555,0.0270,0.0238) | (0.0693,0.0505,0.0278) | (0.0318,0.0311,0.0248) |
| Attribute | ||||
| PLNIS | (0.0220,0.0218,0.0000) | (0.0355,0.0219,0.0212) | (0.0281,0.0243,0.0000) | (0.0283,0.0244,0.0000) |
| Attribute | ||||
| PLNIS | (0.0413,0.0202,0.0134) | (0.0241,0.0238,0.0000) | (0.0478,0.0108,0.0000) | (0.0277,0.0227,0.0182) |
| Attribute | ||||||||
|---|---|---|---|---|---|---|---|---|
| Distance Parameter | ||||||||
| 2.2775 | 2.3581 | 2.1791 | 2.0516 | 2.0394 | 2.1872 | 2.1285 | ||
| 2.4984 | 2.3883 | 2.2884 | 2.2535 | 2.0766 | 2.3228 | 2.2020 | ||
| Attribute | ||||||||
|---|---|---|---|---|---|---|---|---|
| Distance Parameter | ||||||||
| 4.8225 | 4.9277 | 4.5605 | 4.3157 | 4.2010 | 4.5976 | 4.4524 | ||
| 5.2726 | 5.0031 | 4.7956 | 4.7448 | 4.3088 | 4.8694 | 4.5975 | ||
| Attribute | ||||||||
|---|---|---|---|---|---|---|---|---|
| Distance Parameter | ||||||||
| 0.4723 | 0.4785 | 0.4778 | 0.4754 | 0.4854 | 0.4776 | 0.4781 | ||
| 0.4739 | 0.4774 | 0.4772 | 0.4779 | 0.4820 | 0.4770 | 0.4789 | ||
| Distance Parameter | Rank |
|---|---|
| Distance Parameter | Parameters | |||||||||
| 0.2587 | 0.2620 | 0.2616 | 0.2604 | 0.2657 | 0.2615 | 0.2617 | ||||
| 0.5097 | 0.5152 | 0.5146 | 0.5123 | 0.5210 | 0.5143 | 0.5142 | ||||
| 0.4939 | 0.5003 | 0.5005 | 0.4985 | 0.5101 | 0.5003 | 0.5016 | ||||
| Distance Parameter | Parameters | |||||||||
| 0.2595 | 0.2614 | 0.2613 | 0.2601 | 0.2638 | 0.2612 | 0.2622 | ||||
| 0.5114 | 0.5141 | 0.5140 | 0.5121 | 0.5178 | 0.5139 | 0.5152 | ||||
| 0.4939 | 0.4988 | 0.4991 | 0.4965 | 0.5060 | 0.4985 | 0.5019 | ||||
| Distance Parameter | Parameters | Rank | ||
|---|---|---|---|---|
| Attribute | ||||||||
|---|---|---|---|---|---|---|---|---|
| Distance Parameter | ||||||||
| 0.5170 | 0.5209 | 0.5202 | 0.5187 | 0.5247 | 0.5201 | 0.5206 | ||
| 0.5181 | 0.5202 | 0.5200 | 0.5186 | 0.5228 | 0.5199 | 0.5211 | ||
| Distance Parameter | Rank |
|---|---|
| Attribute | ||||||||
|---|---|---|---|---|---|---|---|---|
| Distance Parameter | ||||||||
| 0.6045 | 0.0000 | 0.5221 | 1.0000 | 0.6663 | 0.7571 | 0.4424 | ||
| 0.3547 | 0.0088 | 0.4947 | 0.8607 | 0.7075 | 0.6459 | 0.4109 | ||
| Distance Parameter | Rank |
|---|---|
| Attribute | ||||||||
|---|---|---|---|---|---|---|---|---|
| Distance Parameter | ||||||||
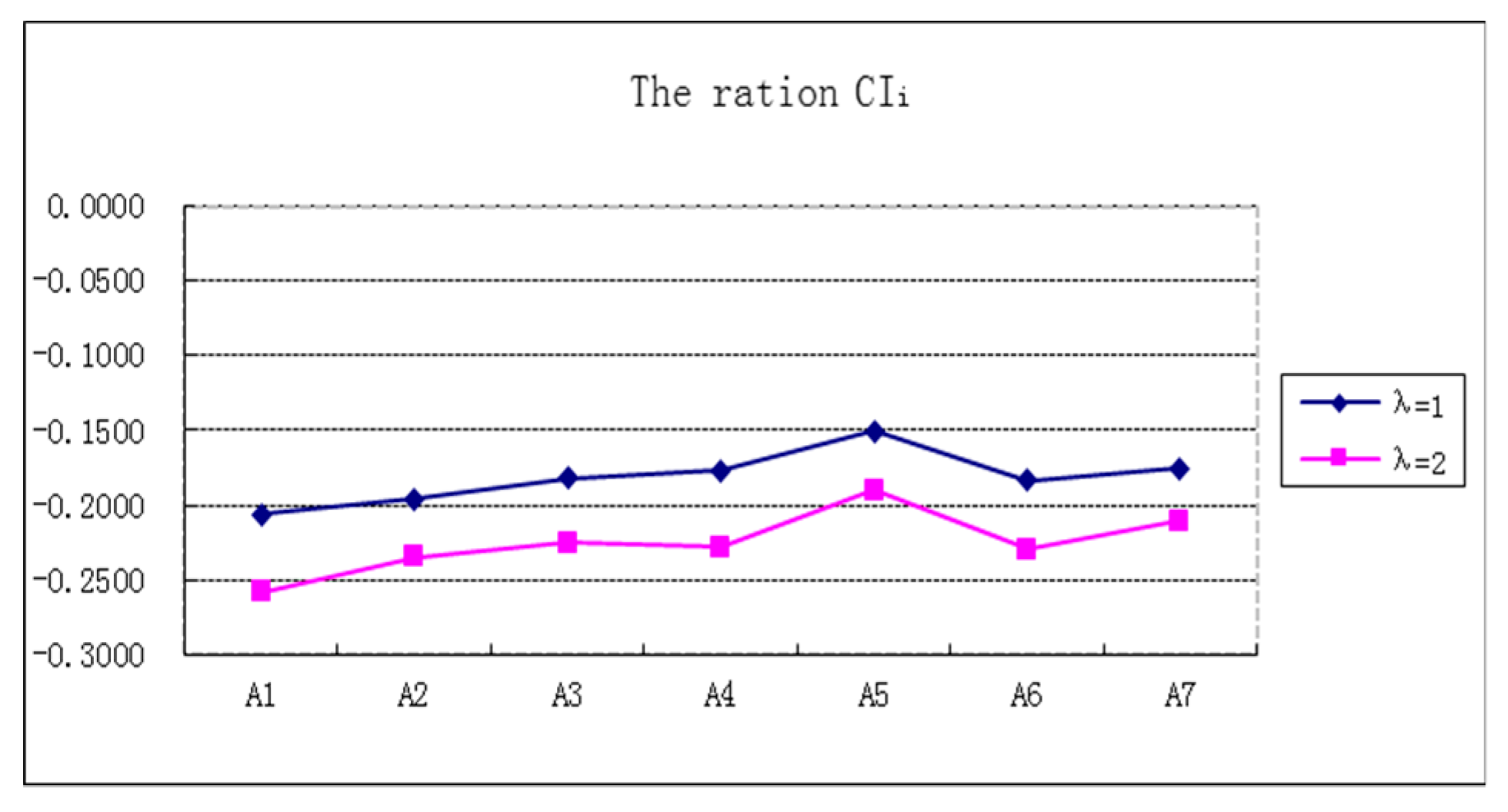
| −0.2062 | −0.1958 | −0.1821 | −0.1767 | −0.1506 | −0.1832 | −0.1754 | ||
| −0.2585 | −0.2349 | −0.2251 | −0.2280 | −0.1903 | −0.2297 | −0.2103 | ||
| Distance Parameter | Rank |
|---|---|
| Alternative | ||||||||
|---|---|---|---|---|---|---|---|---|
| Alternative | ||||||||
|---|---|---|---|---|---|---|---|---|
| Alternative | ||||||||
|---|---|---|---|---|---|---|---|---|
| Attribute | ||||||||
| Weight | 0.1258 | 0.1774 | 0.1025 | 0.1222 | 0.1137 | 0.0953 | 0.1409 | 0.1222 |
| Alternative | ||||
| (0.0373 0.0326 0.0300) | (0.0855,0.0690,0.0000) | (0.0342,0.0337,0.0304) | (0.0385,0.0349,0.0181) | |
| (0.0419,0.0390,0.0326) | (0.0536,0.0263,0.0169) | (0.0321,0.0266,0.0146) | (0.0387,0.0349,0.0272) | |
| (0.0419,0.0409,0.0373) | (0.0591,0.0591,0.0557) | (0.0315,0.0228,0.0195) | (0.0375,0.0291,0.0136) | |
| (0.0332,0.0180,0.0093) | (0.0591,0.0564,0.0197) | (0.0489,0.0456,0.0000) | (0.0377,0.0362,0.0349) | |
| (0.0380,0.0373,0.0300) | (0.0522,0.0263,0.0169) | (0.0318,0.0304,0.0244) | (0.0360,0.0349,0.0136) | |
| (0.0394,0.0326,0.0240) | (0.0468,0.0131,0.0084) | (0.0342,0.0342,0.0298) | (0.0349,0.0344,0.0317) | |
| (0.0419,0.0379,0.0047) | (0.0430,0.0329,0.0084) | (0.0456,0.0451,0.0000) | (0.0519,0.0475,0.0000) | |
| Alternative | ||||
| (0.0379,0.0313,0.0168) | (0.0318,0.0313,0.0227) | (0.0425,0.0157,0.0134) | (0.0389,0.0233,0.0181) | |
| (0.0379,0.0349,0.0325) | (0.0310,0.0272,0.0247) | (0.0470,0.0429,0.0365) | (0.0371,0.0317,0.0291) | |
| (0.0325,0.0323,0.0295) | (0.0308,0.0282,0.0182) | (0.0413,0.0335,0.0313) | (0.0370,0.0349,0.0317) | |
| (0.0379,0.0329,0.0217) | (0.0318,0.0318,0.0212) | (0.0417,0.0379,0.0335) | (0.0349,0.0348,0.0226) | |
| (0.0323,0.0271,0.0084) | (0.0318,0.0295,0.0227) | (0.0470,0.0470,0.0411) | (0.0407,0.0361,0.0226) | |
| (0.0379,0.0325,0.0311) | (0.0318,0.0309,0.0106) | (0.0356,0.0261,0.0067) | (0.0334,0.0291,0.0181) | |
| (0.0312,0.0295,0.0271) | (0.0302,0.0272,0.0247) | (0.0548,0.0538,0.0000) | (0.0475,0.0325,0.0233) |
| Alternative | Algorithm | |||||||
| PT () | 0.4370 | 0.5545 | 0.6555 | 0.4802 | 0.4167 | 0.2644 | 0.3808 | |
| TOPSIS () | 0.4966 | 0.5492 | 0.6070 | 0.5217 | 0.4790 | 0.3521 | 0.4482 | |
| VIKOR () | 0.4458 | 0.9999 | 0.8332 | 0.3952 | 0.2124 | 0.1100 | 0.5794 | |
| Pang Qi et al.’s method () | −0.4627 | −0.2415 | −0.0000 | −0.3566 | −0.5358 | −1.0676 | −0.6659 | |
| Alternative | Algorithm | |||||||
| PT () | 0.4387 | 0.6077 | 0.6942 | 0.4768 | 0.4441 | 0.3054 | 0.3709 | |
| TOPSIS () | 0.4967 | 0.5755 | 0.6213 | 0.5205 | 0.4974 | 0.3916 | 0.4427 | |
| VIKOR () | 0.3811 | 0.9999 | 0.9048 | 0.3483 | 0.2452 | 0.0824 | 0.3601 | |
| Pang Qi et al.’s method () | −0.5607 | −0.1859 | −0.0000 | −0.4418 | −0.5030 | −0.9665 | −0.8629 |
| Alternative | Algorithm | Ranking |
| PT () | ||
| TOPSIS () | ||
| VIKOR () | ||
| Pang Qi et al.’s method () | ||
| Alternative | Algorithm | Ranking |
| PT () | ||
| TOPSIS () | ||
| VIKOR () | ||
| Pang Qi et al.’s method () |
© 2019 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, J.-X. A MAGDM Algorithm with Multi-Granular Probabilistic Linguistic Information. Symmetry 2019, 11, 127. https://doi.org/10.3390/sym11020127
Wang J-X. A MAGDM Algorithm with Multi-Granular Probabilistic Linguistic Information. Symmetry. 2019; 11(2):127. https://doi.org/10.3390/sym11020127
Chicago/Turabian StyleWang, Ju-Xiang. 2019. "A MAGDM Algorithm with Multi-Granular Probabilistic Linguistic Information" Symmetry 11, no. 2: 127. https://doi.org/10.3390/sym11020127
APA StyleWang, J.-X. (2019). A MAGDM Algorithm with Multi-Granular Probabilistic Linguistic Information. Symmetry, 11(2), 127. https://doi.org/10.3390/sym11020127





