Signed Domination Number of the Directed Cylinder

Abstract

In a digraph $D=\left(V\right(D),A(D\left)\right)$, a two-valued function $f:V\left(D\right)\to \{-1,1\}$ defined on the vertices of D is called a signed dominating function if $f\left(N^{-}\left[v\right]\right)\ge 1$ for every v in D. The weight of a signed dominating function is $f\left(V\left(D\right)\right)={\sum }_{v\in V\left(D\right)}f\left(v\right)$. The signed domination number ${\gamma }_s\left(D\right)$ is the minimum weight among all signed dominating functions of D. Let $P_m\times C_n$ be the Cartesian product of directed path $P_m$ and directed cycle $C_n$. In this paper, the exact value of ${\gamma }_s(P_m\times C_n)$ is determined for any positive integers m and n.

1. Introduction

All digraphs considered in this paper are finite without loops and multiple arcs, and we refer to [1] for notation and graph-theoretical terminology not defined here. In a digraph D with vertex set $V\left(D\right)$ and arc set $A\left(D\right)$, u is said to be an in-neighbor of v if $uv\in A\left(D\right)$. For a vertex $v\in V\left(D\right)$, denote $N_D^{-}\left(v\right)=\{u:uv\in A\left(D\right)\}$ be the open in-neighborhood of v. The closed in-neighborhood of v is $N_D^{-}\left[v\right]=N_D^{-}\left(v\right)\cup \left\{v\right\}$. In all cases above, we omit the subscript D when the digraph D is clear from the context. For $S\subseteq V\left(D\right)$, $D\left[S\right]$ denotes the subdigraph induced by S.

For two digraphs $D_1=(V_1,A_1)$ and $D_2=(V_2,A_2)$, the Cartesian product $D_1\times D_2$ is the digraph with vertex set $V_1\times V_2$ and $(x_1,x_2)(y_1,y_2)\in A(D_1\times D_2)$ if and only if $x_1=y_1$ and $x_2{y}_2\in A_2$ or $x_2=y_2$ and $x_1{y}_1\in A_1$, where $x_i,y_i\in V_i$ for $i=1,2$. We use $D_1\cong D_2$ to present that $D_1$ and $D_2$ are isomorphic. 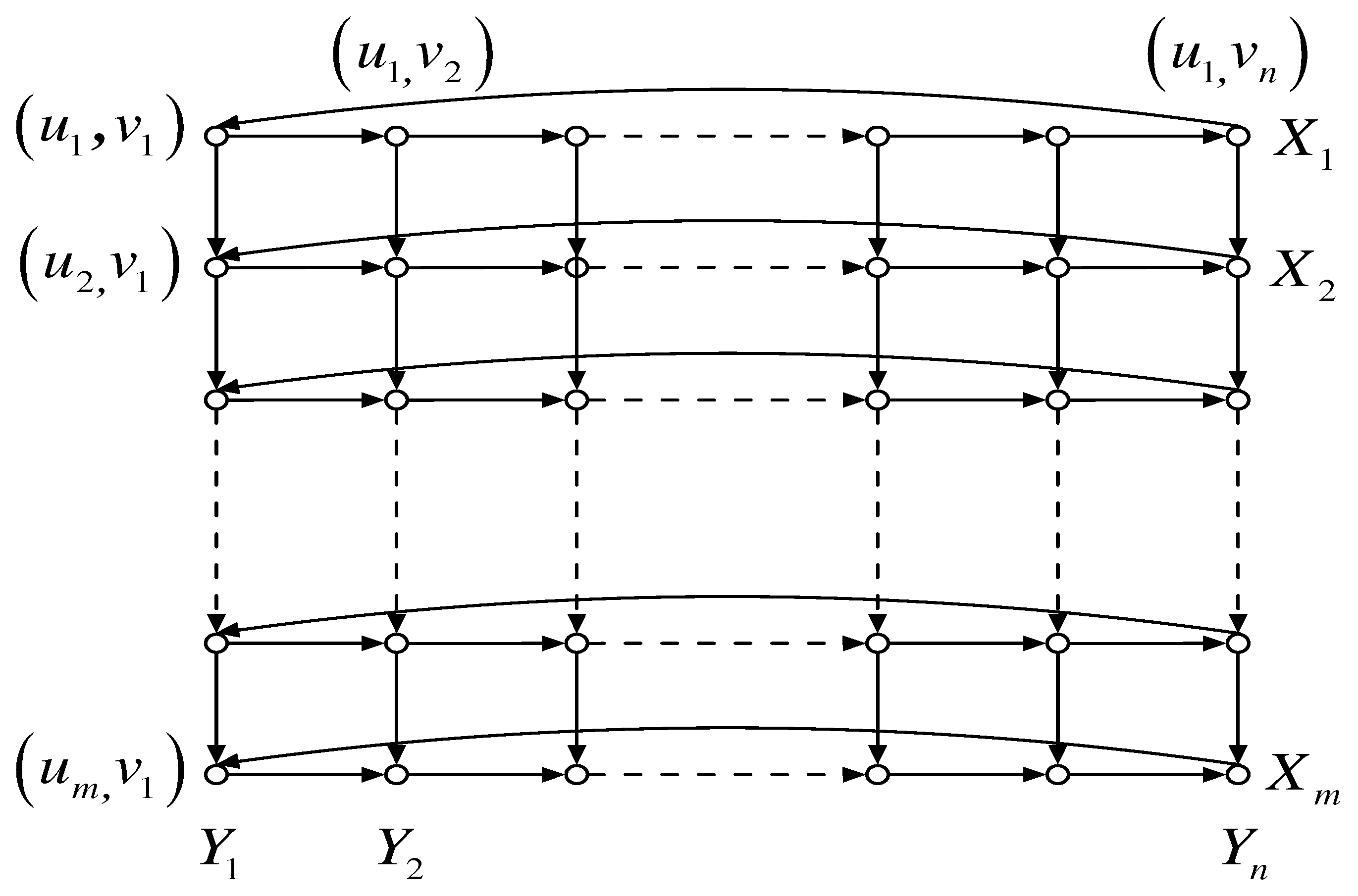The sets of vertices of the directed path $P_m$ and the directed cycle $C_n$ are denoted by $\{u_1,u_2,\dots ,u_m\}$ and $\{v_1,v_2,\dots ,v_n\}$, respectively. Furthermore, in the Cartesian product $P_m\times C_n$ (see Figure 1), let $X_j={\cup }_{i=1}^n\left\{(u_j,v_i)\right\}$ for $1\le j\le m$ and let $Y_i={\cup }_{j=1}^m\left\{(u_j,v_i)\right\}$ for $1\le i\le n$. In fact, $D\left[X_j\right]$ and $D\left[Y_i\right]$ are isomorphic to the directed cycle $C_n$ and the directed path $P_m$, respectively. Throughout this paper, for each vertex $(u_j,v_i)$ in $P_m\times C_n$, the subscript i is taken modulo n. Hence, if $i\le 0$ and k is the smallest positive integer such that $0<kn+i\le n$, then $(u_j,v_i)=(u_j,v_{kn+i})$.

Domination and its variations in graphs have been widely investigated as they have many applications in the real word and other disciplines such as computer networks and location theory, see [2,3,4,5]. The signed domination is one variation of domination and is now well studied in the literature. The study of signed domination of undirected graphs was initiated by Dunbar et al. in [6]. Then, many authors paid much attention to signed domination of graphs, see [7,8,9,10,11,12,13,14,15] and elsewhere. The signed domination of graph has some applications, for example, this concept can also serve as a model of social networks in which global decisions must be made similar to that exhibited in [10]. In [16], Zelinka generalizes this concept to digraphs. For a two-valued function $f:V\left(D\right)\to \{-1,1\}$, the weight of f is $w\left(f\right)={\sum }_{v\in V\left(D\right)}f\left(v\right)$. Formally, a two-valued function $f:V\left(D\right)\to \{-1,1\}$ is said to be a signed dominating function (SDF) if $f\left(N^{-}\left[v\right]\right)\ge 1$ for each vertex $v\in V\left(D\right)$. The signed domination number of D, denoted by ${\gamma }_s\left(D\right)$, is the minimum weight among all signed dominating functions of D. Signed domination of digraphs was studied by several authors including [16,17,18]. Throughout this paper, if f is a signed dominating function of D, then we let P and M denote the sets of those vertices in D which are assigned under f the value 1 and $-1$, respectively. Hence $\left|V\right(D\left)\right|=\left|P\right|+\left|M\right|$ and ${\gamma }_s\left(D\right)=\left|P\right|-\left|M\right|=\left|V\left(D\right)\right|-2\left|M\right|$.

Product graphs are considered in order to gain global information from the factor graphs [19]. Many interesting wireless networks are based on product graphs with simple factors, such as paths and cycles. In particular, any square grid (resp., torus) is the Cartesian product of two paths (resp., cycles) [20]. The domination numbers of Cartesian product of two directed paths (resp., cycles) have been recently determined by several authors [21,22,23,24,25,26,27]. Even more recently, Zhang and Shaheen [28] determined the exact value of signed domination number ${\gamma }_s(P_m\times P_n)$ on Cartesian product $P_m$ and $P_n$ for any $1\le m\le 6$ and $n\ge 1$, and Wang et al. [29] solved the remaining cases.

In this paper, we continue to study the signed domination number of $P_m\times C_n$ and obtain its exact value for any positive integers m and n.

2. Signed Domination Number of $P_m\times C_n$

In this section we determine the exact values of signed domination number of Cartesian product $P_m\times C_n$ for any $m\ge 1$ and $n\ge 2$. Notice that $P_1\times C_n\cong C_n$. In [16], Zelinka obtained that ${\gamma }_s\left(C_n\right)=n$. Thus, in what follows, we may assume that $m\ge 2$ in the Cartesian product $P_m\times C_n$. We begin with one lemma that will be useful in the proof of our main results.

Lemma 1.

Let f be a SDF of$P_m\times C_n$$(m\ge 2)$. The following statements are true:

(1) 

$X_1\subseteq P$.

(2) 

For$2\le j\le m$,$|X_j\cap M|\le \lfloor n/2\rfloor$.

(3) 

For$2\le j\le m-1$,$|(X_j\cup X_{j+1})\cap M|\le n-\lceil n/3\rceil$.

(4) 

For$2\le j\le m-2$,$\left|\right(X_j\cup X_{j+1}\cup X_{j+2})\cap M|\le n$.

Proof. 

The statements (1) and (2) are trivial by the definition of SDF.

(3) If $n\equiv 0\left(mod3\right)$, then $n=3k$ for some integer $k\ge 1$. Suppose on the contrary that there exists some $2\le j\le m-1$ such that $|(X_j\cup X_{j+1})\cap M|\ge n-\lceil n/3\rceil +1=2k+1$. Then at least one of $|X_j\cap M|\ge k+1$ and $|X_{j+1}\cap M|\ge k+1$ holds. Without loss of generality, assume that $|X_j\cap M|=k+s$, where $1\le s\le \lfloor 3k/2\rfloor -k$. By the definition of SDF, $|X_{j+1}\cap M|\le 3k-2(k+s)=k-2s$. Thus $|(X_j\cup X_{j+1})\cap M|\le (k+s)+(k-2s)=2k-s\le 2k-1$, a contradiction. Therefore, $|(X_j\cup X_{j+1})\cap M|\le 2k=n-\lceil n/3\rceil$. For the cases that $n\equiv 1\left(mod3\right)$ or $n\equiv 2\left(mod3\right)$, one can reach the same contradictions by applying the argument similar to that above. Consequently, the statement (3) is true.

(4) If $n\equiv 0\left(mod3\right)$, then $n=3k$ for some integer $k\ge 1$. Assume that $\left|\right(X_j\cup X_{j+1}\cup X_{j+2})\cap M|\le n=3k$ is false for $2\le j\le m-2$. Then there exists some $2\le j\le m-2$ such that $\left|\right(X_j\cup X_{j+1}\cup X_{j+2})\cap M|\ge 3k+1$. Hence it follows that at least one of $|X_j\cap M|\ge k+1$, $|X_{j+1}\cap M|\ge k+1$ and $|X_{j+2}\cap M|\ge k+1$ is true. Suppose that $|X_{j+1}\cap M|=k+s$, where $1\le s\le \lfloor 3k/2\rfloor -k$. Then $|X_j\cap M|\le 3k-2(k+s)=k-2s$ and $|X_{j+2}\cap M|\le 3k-2(k+s)=k-2s$ by the definition of SDF. Thus $\left|\right(X_j\cup X_{j+1}\cup X_{j+2})\cap M|\le 3k-3s\le 3k-3$, which is a contradiction. This implies that either $|X_j\cap M|\ge k+1$ or $|X_{j+2}\cap M|\ge k+1$. Without loss of generality, assume that $|X_j\cap M|=k+s$, where $1\le s\le \lfloor 3k/2\rfloor -k$. Let $|X_{j+1}\cap M|=l$, where $0\le l\le k-2s$. If $l=0$, then $2s\le k$. By the statement (2), $|(X_j\cup X_{j+1}\cup X_{j+2})\cap M|\le (k+s)+3k/2\le 3k$, a contradiction again. So $l\ge 1$. Let $X_{j+1}\cap M={\cup }_{a=1}^l=\left\{(u_{j+1},v_{i_a})\right\}$, $X_{j+2,i_a}^{*}=\{(u_{j+2},v_{i_a-1}),(u_{j+2},v_{i_a})\}$, and $X_{j+2}^{*}={\cup }_{a=1}^l\left\{X_{j+2,i_a}^{*}\right\}$, where $1\le i_1<i_2<\dots <i_l\le n$. By the definition of SDF, $X_{j+2}^{*}\subseteq P$. Since $3k-2l\ge 3k-2(k-2s)\ge k+4$, it follows that $D[X_{j+2}\setminus X_{j+2}^{*}]$ would be divided into some directed segments (that is, these directed segments are all isomorphic to directed paths) by l pairs of vertices $X_{j+2,i_1}^{*}$, $X_{j+2,i_2}^{*}$,…,$X_{j+2,i_l}^{*}$. 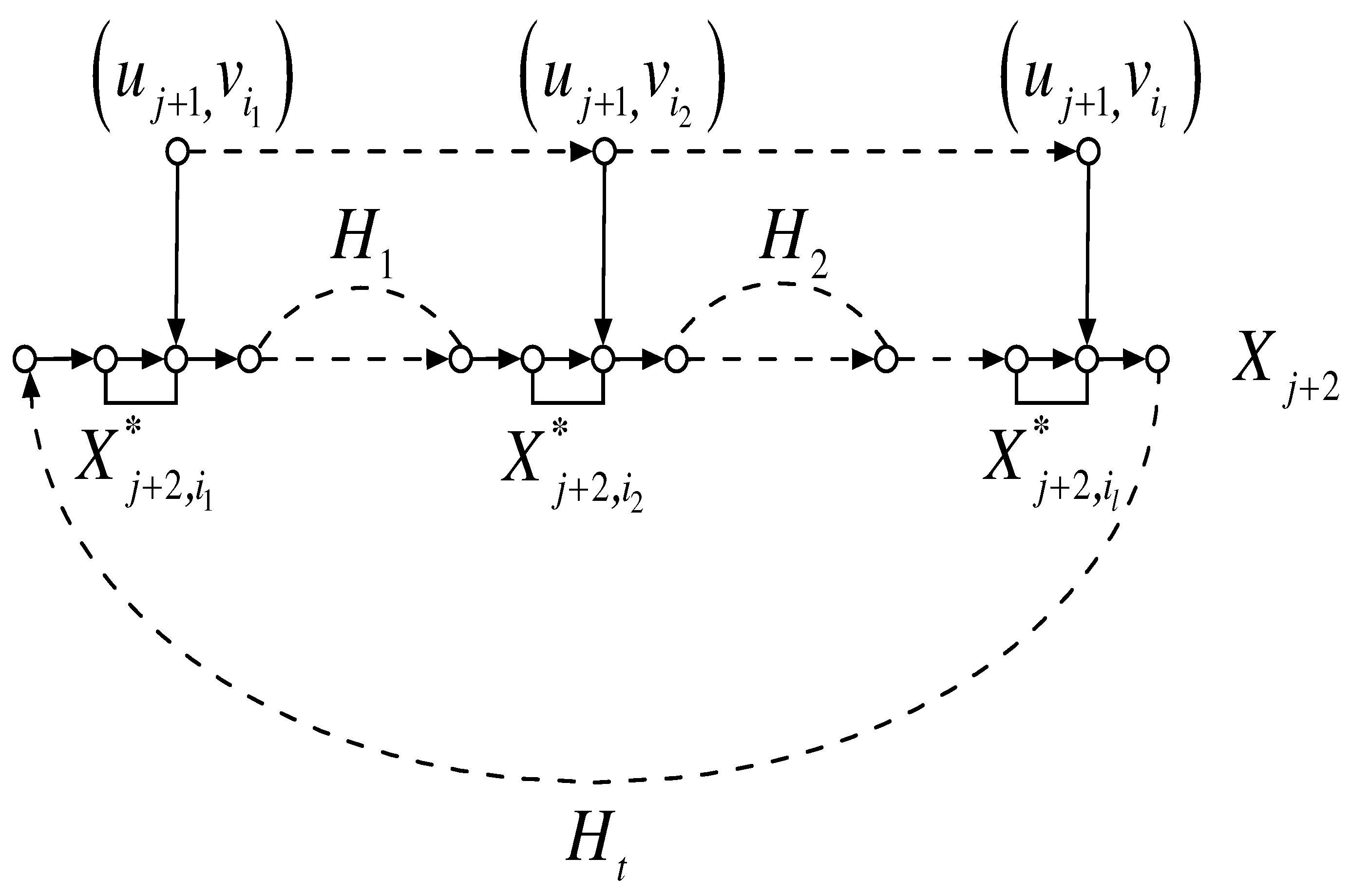Without loss of generality, assume that there are t such directed segments, denoted by $H_b$, where $1\le b\le t\le l$ (The directed segments $H_b$’s of $D[X_{j+2}\setminus X_{j+2}^{*}]$ are shown in Figure 2). Then each segment $H_b$ contains at most $\left(\right|V\left(H_b\right)|+1)/2$ vertices which are assigned under f the value $-1$ by the definition of SDF, where $1\le b\le t$. Thus $|X_{j+2}\cap M|\le (3k-2l+t)/2\le (3k-l)/2$ as ${\sum }_{b=1}^t\left|V\left(H_b\right)\right|=3k-2l$. Notice that $l\le k-2s$, that is, $2s\le k-l$. Then $|(X_j\cup X_{j+1}\cup X_{j+2})\cap M|\le (k+s)+l+(3k-l)/2\le 3k$, which is a contradiction. Consequently, the statement (4) is true for the case when $n\equiv 0\left(mod3\right)$. By a similar argument above, one can obtain the same contradictions for the cases when $n\equiv 1\left(mod3\right)$ or $n\equiv 2\left(mod3\right)$. Hence the statement (4) holds. This completes the proof of Lemma 1. □

Next we shall give our main results in this paper. The methods to prove the following theorems are similar. First we present the upper bound on ${\gamma }_s(P_m\times C_n)$ by constructing signed dominating function for $P_m\times C_n$ with weight attaining the upper bound, then we establish the lower bound on ${\gamma }_s(P_m\times C_n)$ by Lemma 1, moreover, the lower bound coincides with the upper bound. Thus the exact value of ${\gamma }_s(P_m\times C_n)$ is determined.

Theorem 1.

For any integer$n\ge 2$,

$${\gamma }_s(P_2\times C_n)=\left\{\begin{array}{cc}{\displaystyle n}\hfill & ifn\equiv 0\left(mod2\right),\hfill \\ {\displaystyle n+1}\hfill & ifn\equiv 1\left(mod2\right).\hfill \end{array}\right.$$

Proof. 

Let $X_2^{*}={\cup }_{i=1}^{\lfloor n/2\rfloor }\left\{(u_2,v_{2i})\right\}$. Define $f:V(P_2\times C_n)\to \{-1,1\}$ by assigning to each vertex of $X_2^{*}$ the value $-1$ while to each vertex of $V(P_2\times C_n)\setminus X_2^{*}$ the value 1. It is easy to check that f is a SDF of $P_2\times C_n$ with weight $w\left(f\right)=2\lceil n/2\rceil$. If $n\equiv 0\left(mod2\right)$ ($n\equiv 1\left(mod2\right)$, respectively), then ${\gamma }_s(P_2\times C_n)\le w\left(f\right)=n$ ($n+1$, respectively), and ${\gamma }_s(P_2\times C_n)=2n-2\left|M\right|\ge n$ ($n+1$, respectively) by Lemma 1 (1) and (2). This implies that ${\gamma }_s(P_2\times C_n)=n$ ($n+1$, respectively) when $n\equiv 0\left(mod2\right)$ ($n\equiv 1\left(mod2\right)$, respectively). This completes the proof of Theorem 1. □

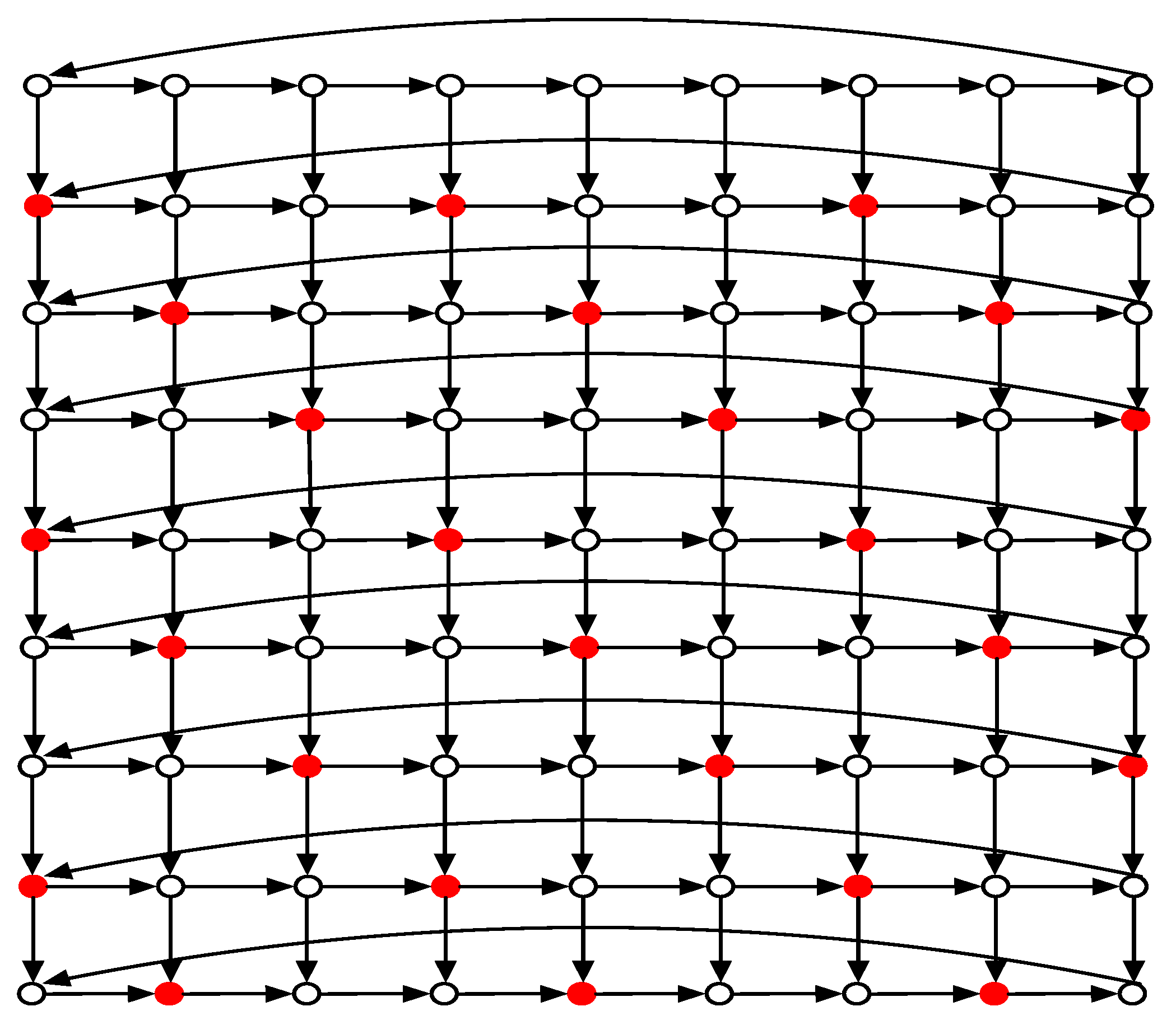
Theorem 2.

For any integers$n,m\ge 3$such that$n\equiv 0\left(mod3\right)$,

$${\gamma }_s(P_m\times C_n)=\frac{n(m+2)}{3}.$$

Proof. 

Suppose that $n=3k$ for some integer $k\ge 1$. We next consider the following cases to complete the proof of Theorem 2.

Case 1.$m\equiv 0\left(mod3\right)$. Then $m=3r$ for some integer $r\ge 1$. We first show that ${\gamma }_s(P_m\times C_n)\le n(m+2)/3$ by constructing a SDF of $P_m\times C_n$ with weight $n(m+2)/3$. Let $X_{3j-1}^{*}={\cup }_{t=0}^{k-1}\left\{(u_{3j-1},v_{3t+1})\right\}$, $X_{3j}^{*}={\cup }_{t=0}^{k-1}\left\{(u_{3j},v_{3t+2})\right\}$ for $j=1,2,\dots ,r$, and $X_{3j+1}^{*}={\cup }_{t=0}^{k-1}\left\{(u_{3j+1},v_{3t+3})\right\}$ for $j=1,2,\dots ,r-1$. Define $f:V(P_{3r}\times C_{3k})\to \{-1,1\}$ by assigning to each vertex of $\left({\cup }_{j=1}^{r-1}\{X_{3j-1}^{*},X_{3j}^{*},X_{3j+1}^{*}\}\right)\cup \{X_{3r-1}^{*},X_{3r}^{*}\}$ the value $-1$ while to each vertex of $V(P_{3r}\times C_{3k})\setminus \left({\cup }_{j=1}^{r-1}\{X_{3j-1}^{*},X_{3j}^{*},X_{3j+1}^{*}\}\right)\cup \{X_{3r-1}^{*},X_{3r}^{*}\}$ the value 1. It is easy to verify that f is a SDF of $P_{3r}\times C_{3k}$ with weight $w\left(f\right)=k(3r+2)=n(m+2)/3$ (The SDF f of $P_9\times C_9$ is shown in Figure 3). Thus ${\gamma }_s(P_{3r}\times C_{3k})\le w\left(f\right)=n(m+2)/3$. By Lemma 1 (3) and (4), we have $\left|\right(X_2\cup X_3)\cap M|\le 2k$ and $\left|\right(X_{3j-2}\cup X_{3j-1}\cup X_{3j})\cap M|\le 3k$ for every $2\le j\le r$, and so $\left|M\right|\le 2k+3k(r-1)=k(3r-1)$ from Lemma 1 (1). Then ${\gamma }_s(P_{3r}\times C_{3k})=9rk-2\left|M\right|\ge 3rk+2k=n(m+2)/3$, which implies that ${\gamma }_s(P_{3r}\times C_{3k})=n(m+2)/3$.

Case 2.$m\equiv 1\left(mod3\right)$. Then $m=3r+1$ for some integer $r\ge 1$. We first prove that ${\gamma }_s(P_m\times C_n)\le n(m+2)/3$ by establishing a SDF of $P_m\times C_n$ with weight $n(m+2)/3$. Set $X_{3j-1}^{*}={\cup }_{t=0}^{k-1}\left\{(u_{3j-1},v_{3t+1})\right\}$, $X_{3j}^{*}={\cup }_{t=0}^{k-1}\left\{(u_{3j},v_{3t+2})\right\}$ and $X_{3j+1}^{*}={\cup }_{t=0}^{k-1}\left\{(u_{3j+1},v_{3t+3})\right\}$ for $j=1,2,\dots ,r$. Define $g:V(P_{3r+1}\times C_{3k})\to \{-1,1\}$ as follows: each vertex of ${\cup }_{j=1}^r\{X_{3j-1}^{*},X_{3j}^{*},X_{3j+1}^{*}\}$ is assigned the value $-1$ while each vertex of $V(P_{3r+1}\times C_{3k})\setminus {\cup }_{j=1}^r\{X_{3j-1}^{*},X_{3j}^{*},X_{3j+1}^{*}\}$ is assigned the value 1. It is not hard to see that g is a SDF of $P_{3r+1}\times C_{3k}$ with weight $w\left(g\right)=3k(r+1)=n(m+2)/3$ (The SDF g of $P_7\times C_9$ can be obtained by restricting f defined in Case 1 on $P_7\times C_9$, which is depicted in Figure 3). Thus ${\gamma }_s(P_{3r+1}\times C_{3k})\le w\left(g\right)=n(m+2)/3$. For each $1\le j\le r$, it follows from Lemma 1 (4) that $\left|\right(X_{3j-1}\cup X_{3j}\cup X_{3j+1})\cap M|\le 3k$. According to Lemma 1 (1), we have $\left|M\right|\le 3rk$. Hence ${\gamma }_s(P_{3r+1}\times C_{3k})=3k(3r+1)-2\left|M\right|\ge 3rk+3k=n(m+2)/3$. This implies that ${\gamma }_s(P_{3r+1}\times C_{3k})=n(m+2)/3$.

Case 3.$m\equiv 2\left(mod3\right)$. Then $m=3r+2$ for some integer $r\ge 1$. We first demonstrate that ${\gamma }_s(P_m\times C_n)\le n(m+2)/3$ by giving a SDF of $P_m\times C_n$ with weight $n(m+2)/3$. We write $X_{3j-1}^{*}={\cup }_{t=0}^{k-1}\left\{(u_{3j-1},v_{3t+1})\right\}$ for $j=1,2,\dots ,r+1$, $X_{3j}^{*}={\cup }_{t=0}^{k-1}\left\{(u_{3j},v_{3t+2})\right\}$ and $X_{3j+1}^{*}={\cup }_{t=0}^{k-1}\left\{(u_{3j+1},v_{3t+3})\right\}$ for $j=1,2,\dots ,r$. Assigning to all vertices of $\left({\cup }_{j=1}^r\{X_{3j-1}^{*},X_{3j}^{*},X_{3j+1}^{*}\}\right)\cup \left\{X_{3r+2}^{*}\right\}$ the value $-1$ and to all other vertices the value 1, we produce a SDF h of $P_{3r+2}\times C_{3k}$ with weight $w\left(f\right)=k(3r+4)=n(m+2)/3$ (The SDF h of $P_8\times C_9$ can be obtained by restricting f defined in Case 1 (see Figure 3) to $P_8\times C_9$). So ${\gamma }_s(P_{3r+2}\times C_{3k})\le n(m+2)/3$. According to Lemma 1 (3) and (4), we obtain $\left|\right(X_2\cup X_3)\cap M|\le 2k$, $\left|\right(X_{3r+1}\cup X_{3r+2})\cap M|\le 2k$ and $\left|\right(X_{3j-2}\cup X_{3j-1}\cup X_{3j})\cap M|\le 3k$ for every $2\le j\le r$. This means that $\left|M\right|\le 4k+3k(r-1)=k(3r+1)$ by Lemma 1 (1). Therefore, ${\gamma }_s(P_{3r+2}\times C_{3k})=3k(3r+2)-2\left|M\right|\ge 3rk+4k=n(m+2)/3$, implying that ${\gamma }_s(P_{3r+2}\times C_{3k})=n(m+2)/3$. This completes the proof of Theorem 2. □

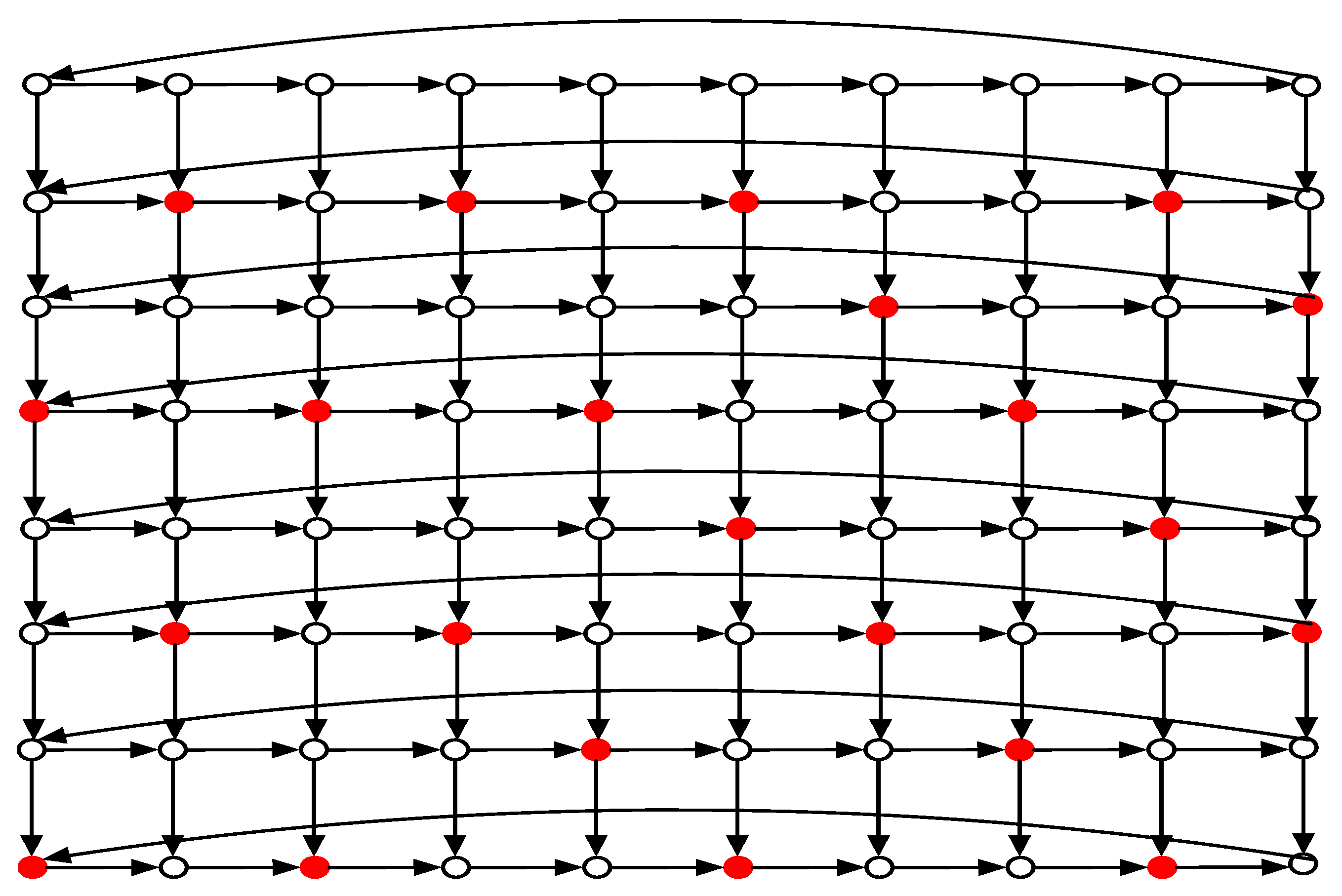
Theorem 3.

For any positive integers$n\ge 4,m\ge 3$such that$n\equiv 1\left(mod3\right)$,

$${\gamma }_s(P_m\times C_n)=\left\{\begin{array}{cc}{\displaystyle \frac{m(n+2)+2n-8}{3}}\hfill & ifm\equiv 0\left(mod2\right),\hfill \\ {\displaystyle \frac{m(n+2)+2n-2}{3}}\hfill & ifm\equiv 1\left(mod2\right).\hfill \end{array}\right.$$

Proof. 

Suppose that $n=3k+1$ for some integer $k\ge 1$. We first prove that Theorem 3 is true when $k=1$. Let $X_{2j}^{*}={\cup }_{i=1}^2\left\{(u_{2j},v_{2i})\right\}$ for $1\le j\le \lfloor m/2\rfloor$. Define $f:V(P_m\times C_4)\to \{-1,1\}$ by assigning to each vertex of ${\cup }_{j=1}^{\lfloor m/2\rfloor }{X}_{2j}^{*}$ the value $-1$ while to each vertex of $V(P_m\times C_4)\setminus {\cup }_{j=1}^{\lfloor m/2\rfloor }{X}_{2j}^{*}$ the value 1. It is easy to check that f is a SDF of $P_m\times C_4$ with weight $w\left(f\right)=4\lceil m/2\rceil$. Then ${\gamma }_s(P_m\times C_4)\le w\left(f\right)=2m$ or $2m+2$ when $m\equiv 0\left(mod2\right)$ or $m\equiv 1\left(mod2\right)$. By Lemma 1 (1) and (3), if $m\equiv 0\left(mod2\right)$ ($m\equiv 1\left(mod2\right)$, respectively), then we have $\left|M\right|\le m$ ($m-1$, respectively), and so ${\gamma }_s(P_m\times C_4)=4m-2\left|M\right|\ge 2m$ ($2m+2$, respectively). This implies that ${\gamma }_s(P_m\times C_4)=2m$ or $2m+2$ when $m\equiv 0\left(mod2\right)$ or $m\equiv 1\left(mod2\right)$. We next assume that $k\ge 2$ and proceed the proof of Theorem 3 by considering two cases.

Case 1.$m\equiv 0\left(mod2\right)$. Then $m=2r$ for some integer $r\ge 2$. We first show that ${\gamma }_s(P_m\times C_n)\le (m(n+2)+2n-8)/3$ by constructing a SDF of $P_m\times C_n$ with weight $\left(m\right(n+2)+2n-8)/3$. Set $X_{2j-1}^{*}={\cup }_{t=0}^{k-2}\left\{(u_{2j-1},v_{n+2-j-3t})\right\}$ for $j=2,3,\dots ,r$ and $X_{2j}^{*}={\cup }_{t=0}^{k-1}\left\{(u_{2j},v_{n+3-j-3t})\right\}\cup \left\{(u_{2j},v_{5-j})\right\}$ for $j=1,2,\dots ,r$. Define $g:V(P_{2r}\times C_{3k+1})\to \{-1,1\}$ as follow: each vertex of ${\cup }_{j=2}^r\{X_{2j-1}^{*},X_{2j}^{*}\}\cup ({\cup }_{t=0}^{k-1}\left\{(u_2,v_{n+2-3t})\right\}\cup \left\{(u_2,v_4)\right\})$ is assigned the value $-1$ while each vertex of $V(P_{2r}\times C_{3k+1})\setminus ({\cup }_{j=2}^r\{X_{2j-1}^{*},X_{2j}^{*}\}\cup ({\cup }_{t=0}^{k-1}\left\{(u_2,v_{n+2-3t})\right\}\cup \left\{(u_2,v_4)\right\}))$ is assigned the value 1. It is not hard to verify that g is a SDF of $P_{2r}\times C_{3k+1}$ with weight $w\left(g\right)=2r(k+1)+2k-2=\left(m\right(n+2)+2n-8)/3$ (The SDF g of $P_8\times C_{10}$ is shown in Figure 4). Thus ${\gamma }_s(P_{2r}\times C_{3k+1})\le w\left(g\right)=(m(n+2)+2n-8)/3$. By Lemma 1 (3) and (4), we have $\left|\right(X_2\cup X_3\cup X_4)\cap M|\le 3k+1$ and $\left|\right(X_{2j-1}\cup X_{2j})\cap M|\le 2k$ for every $3\le j\le r$, and so $\left|M\right|\le (3k+1)+2k(r-2)=k(2r-1)+1$ from Lemma 1 (1). Then ${\gamma }_s(P_{2r}\times C_{3k+1})=2r(3k+1)-2\left|M\right|\ge 2r(k+1)+2k-2=(m(n+2)+2n-8)/3$, which implies that ${\gamma }_s(P_{2r}\times C_{3k+1})=(m(n+2)+2n-8)/3$.

Case 2.$m\equiv 1\left(mod2\right)$. Then $m=2r+1$ for some integer $r\ge 1$. We first demonstrate that ${\gamma }_s(P_m\times C_n)\le (m(n+2)+2n-2)/3$ by establishing a SDF of $P_m\times C_n$ with weight $\left(m\right(n+2)+2n-2)/3$. We write $X_{2j-1}^{*}={\cup }_{t=0}^{k-2}\left\{(u_{2j-1},v_{n+2-j-3t})\right\}$ for $j=2,3,\dots ,r+1$ and $X_{2j}^{*}={\cup }_{t=0}^{k-1}\left\{(u_{2j},v_{n+3-j-3t})\right\}\cup \left\{(u_{2j},v_{5-j})\right\}$ for $j=1,2,\dots ,r$. Assigning to all vertices of ${\cup }_{j=2}^r\{X_{2j-1}^{*},X_{2j}^{*}\}\cup ({\cup }_{t=0}^{k-1}\left\{(u_2,v_{n+2-3t})\right\}\cup \left\{(u_2,v_4)\right\})\cup \left({\cup }_{t=0}^{k-2}\left\{(u_{2r+1},v_{n+1-r-3t})\right\}\right)$ the value $-1$, and to all other vertices the value 1, we produce a SDF h of $P_{2r+1}\times C_{3k+1}$ with weight $w\left(h\right)=2r(k+1)+3k+1=\left(m\right(n+2)+2n-2)/3$ (The SDF h of $P_7\times C_{10}$ can be obtained by restricting g defined in Case 1 (see Figure 4) to $P_7\times C_{10}$). So ${\gamma }_s(P_{2r+1}\times C_{3k+1})\le w\left(h\right)=(m(n+2)+2n-2)/3$. For every $1\le j\le r$, it follows from Lemma 1 (3) that $\left|\right(X_{2j}\cup X_{2j+1})\cap M|\le 2k$. This derives that $\left|M\right|\le 2kr$ by Lemma 1 (1). Therefore, ${\gamma }_s(P_{2r+1}\times C_{3k+1})=(2r+1)(3k+1)-2\left|M\right|\ge 2r(k+1)+3k+1=(m(n+2)+2n-2)/3$, implying that ${\gamma }_s(P_{2r+1}\times C_{3k+1})=(m(n+2)+2n-2)/3$. This completes the proof of Theorem 3. □

Theorem 4.

For any positive integers$n\ge 2,m\ge 3$such that$n\equiv 2\left(mod3\right)$,

$${\gamma }_s(P_m\times C_n)=\left\{\begin{array}{cc}{\displaystyle \frac{m(n+1)+2n-4}{3}}\hfill & ifm\equiv 0\left(mod2\right),\hfill \\ {\displaystyle \frac{m(n+1)+2n-1}{3}}\hfill & ifm\equiv 1\left(mod2\right).\hfill \end{array}\right.$$

Proof. 

Suppose that $n=3k+2$ for some integer $k\ge 0$. We first show that Theorem 4 is true when $k=0$. Define $f:V(P_m\times C_2)\to \{-1,1\}$ by assigning to each vertex of ${\cup }_{j=1}^{\lfloor m/2\rfloor }\left\{(u_{2j},v_2)\right\}$ the value $-1$ while to each vertex of $V(P_m\times C_2)\setminus {\cup }_{j=1}^{\lfloor m/2\rfloor }\left\{(u_{2j},v_2)\right\}$ the value 1. It is easy to check that f is a SDF of $P_m\times C_2$ with weight $w\left(f\right)=2\lceil m/2\rceil$. Then ${\gamma }_s(P_m\times C_2)\le w\left(f\right)=m$ or $m+1$ when $m\equiv 0\left(mod2\right)$ or $m\equiv 1\left(mod2\right)$. By Lemma 1 (1) and (3), if $m\equiv 0\left(mod2\right)$ ($m\equiv 1\left(mod2\right)$, respectively), then we have $\left|M\right|\le m/2$ ($(m-1)/2$, respectively), and so ${\gamma }_s(P_m\times C_4)=2m-2\left|M\right|\ge m$ ($m+1$, respectively). This implies that ${\gamma }_s(P_m\times C_2)=m$ or $m+1$ when $m\equiv 0\left(mod2\right)$ or $m\equiv 1\left(mod2\right)$. We next assume that $k\ge 1$ and consider two cases to complete the proof of Theorem 4.

Case 1.$m\equiv 0\left(mod2\right)$. Then $m=2r$ for some integer $r\ge 2$. We first prove that ${\gamma }_s(P_m\times C_n)\le (m(n+1)+2n-4)/3$ by constructing a SDF of $P_m\times C_n$ with weight $\left(m\right(n+1)+2n-4)/3$. Let $X_{2j-1}^{*}={\cup }_{t=0}^{k-1}\left\{(u_{2j-1},v_{n+2-j-3t})\right\}$ for $j=2,3,\dots ,r$ and $X_{2j}^{*}={\cup }_{t=0}^k\left\{(u_{2j},v_{n+3-j-3t})\right\}$ for $j=1,2,\dots ,r$. Define $g:V(P_{2r}\times C_{3k+2})\to \{-1,1\}$ as follow: each vertex of ${\cup }_{j=2}^r\{X_{2j-1}^{*},X_{2j}^{*}\}\cup \left({\cup }_{t=0}^k\left\{(u_2,v_{n+2-3t})\right\}\right)$ is assigned the value $-1$ while each vertex of $V(P_{2r}\times C_{3k+2})\setminus {\cup }_{j=2}^r\{X_{2j-1}^{*},X_{2j}^{*}\}\cup \left({\cup }_{t=0}^k\left\{(u_2,v_{n+2-3t})\right\}\right)$ is assigned the value 1. It is not hard to verify that g is a SDF of $P_{2r}\times C_{3k+2}$ with weight $w\left(g\right)=2r(k+1)+2k=\left(m\right(n+1)+2n-4)/3$ (The SDF g of $P_8\times C_{11}$ is shown in Figure 5). Thus ${\gamma }_s(P_{2r}\times C_{3k+2})\le w\left(g\right)=(m(n+1)+2n-4)/3$. By Lemma 1 (3) and (4), we have $\left|\right(X_2\cup X_3\cup X_4)\cap M|\le 3k+2$ and $\left|\right(X_{2j-1}\cup X_{2j})\cap M|\le 2k+1$ for every $3\le j\le r$. So $\left|M\right|\le (3k+2)+(2k+1)(r-2)=k(2r-1)+r$ according to Lemma 1 (1). Then ${\gamma }_s(P_{2r}\times C_{3k+2})=2r(3k+2)-2\left|M\right|\ge 2r(k+1)+2k=(m(n+1)+2n-4)/3$, which implies that ${\gamma }_s(P_{2r}\times C_{3k+2})=(m(n+1)+2n-4)/3$.

Case 2.$m\equiv 1\left(mod2\right)$. Then $m=2r+1$ for some integer $r\ge 1$. We first demonstrate that ${\gamma }_s(P_m\times C_n)\le (m(n+1)+2n-1)/3$ by establishing a SDF of $P_m\times C_n$ with weight $\left(m\right(n+1)+2n-1)/3$. We write $X_{2j-1}^{*}={\cup }_{t=0}^{k-1}\left\{(u_{2j-1},v_{n+2-j-3t})\right\}$ for $j=2,3,\dots ,r+1$ and $X_{2j}^{*}={\cup }_{t=0}^k\left\{(u_{2j},v_{n+3-j-3t})\right\}$ for $j=1,2,\dots ,r$. Assigning to all vertices of ${\cup }_{j=2}^r\{X_{2j-1}^{*},X_{2j}^{*}\}\cup \left({\cup }_{t=0}^{k-1}\left\{(u_2,v_{n+2-3t})\right\}\right)\cup \left({\cup }_{t=0}^{k-1}\left\{(u_{2r+1},v_{n+1-r-3t})\right\}\right)$ the value $-1$, and to all other vertices the value 1, we produce a SDF h of $P_{2r+1}\times C_{3k+2}$ with weight $w\left(h\right)=2r(k+1)+3k+2=\left(m\right(n+1)+2n-1)/3$ (The SDF h of $P_7\times C_{11}$ can be obtained by restricting g defined in Case 1 (see Figure 5) to $P_7\times C_{11}$). So ${\gamma }_s(P_{2r+1}\times C_{3k+2})\le w\left(h\right)=(m(n+1)+2n-1)/3$. For every $1\le j\le r$, it follows from Lemma 1 (3) that $\left|\right(X_{2j}\cup X_{2j+1})\cap M|\le 2k+1$. This means that $\left|M\right|\le r(2k+1)$ by Lemma 1 (1). Therefore, ${\gamma }_s(P_{2r+1}\times C_{3k+2})=(2r+1)(3k+2)-2\left|M\right|\ge 2r(k+1)+3k+2=(m(n+1)+2n-1)/3$, implying that ${\gamma }_s(P_{2r+1}\times C_{3k+2})=(m(n+1)+2n-1)/3$. This completes the proof of Theorem 4. □

3. Conclusions and Future Work

This paper is a contribution to the theory of signed domination of graphs. In particular, we determine the exact value of signed domination number of the Cartesian product $P_m\times C_n$ for any positive integers m and n. It is also interesting to study the signed domination number of the Cartesian product of directed cycles in future.