Numerical Investigation of Heat Conduction with Multiple Moving Heat Sources
Abstract
1. Introduction
- Firstly, the adaptive mesh is optimal in the sense of equidistribution on each subdomain, and can be generated very efficiently, since the local equidistributed mesh on each subdomain can be obtained in parallel.
- Secondly, it is found that the jump always vanishes on the specially designed adaptive mesh. Consequently, the discretization of the underlying model equation is further simplified while maintaining a second-order convergence rate in space. Moreover, the derived discretization is reinterpreted from a novel perspective, which shows some inspiration to extend the proposed method to a multi-dimensional case.
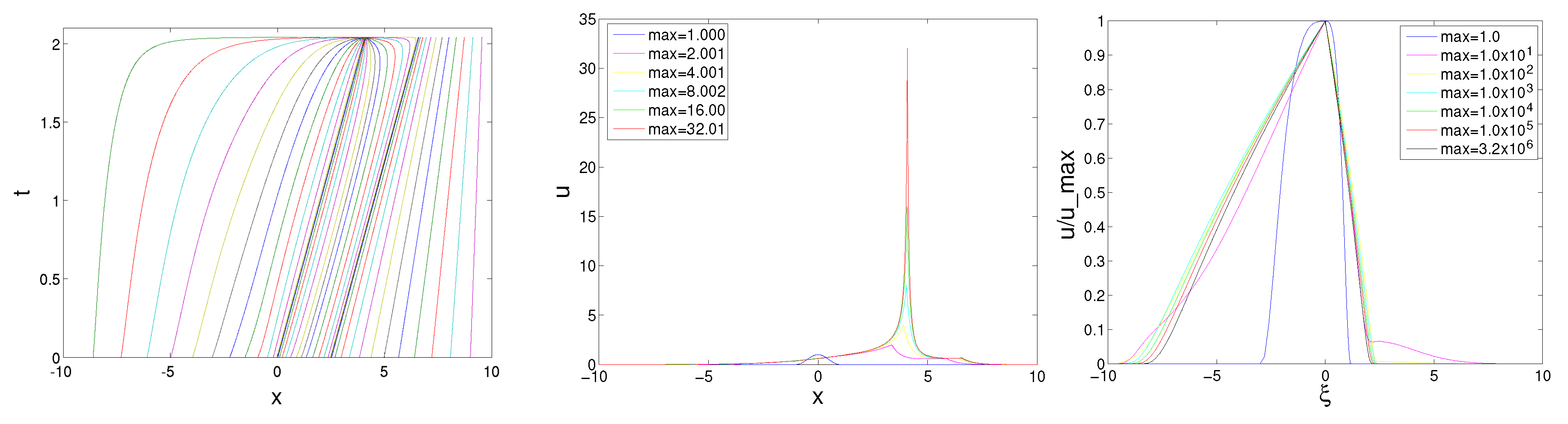
- Thirdly, with the help of exact tracing of each heat source by a fixed mesh point, the blow-up phenomenon that occurs at multiple locations simultaneously has been successfully observed by the present numerical experiment of symmetrically moving sources.
- Finally, various numerical experiments are carried out to investigate the solution behavior in terms of the motions of heat sources, such as the types of motion and the distance between the sources. The blow-up phenomena in terms of the distance of two moving sources are numerically discussed. It is found as expected that, if the sources are moving with high speeds, there may exist a critical distance, such that blow-up must occur if the distance of the sources is less than the critical distance, while blow-up is avoided when the distance is larger than this critical distance.
2. Model Equation
3. Numerical Method
3.1. Domain-Decomposed Adaptive Mesh Generation
3.2. Discretization on the Moving Mesh
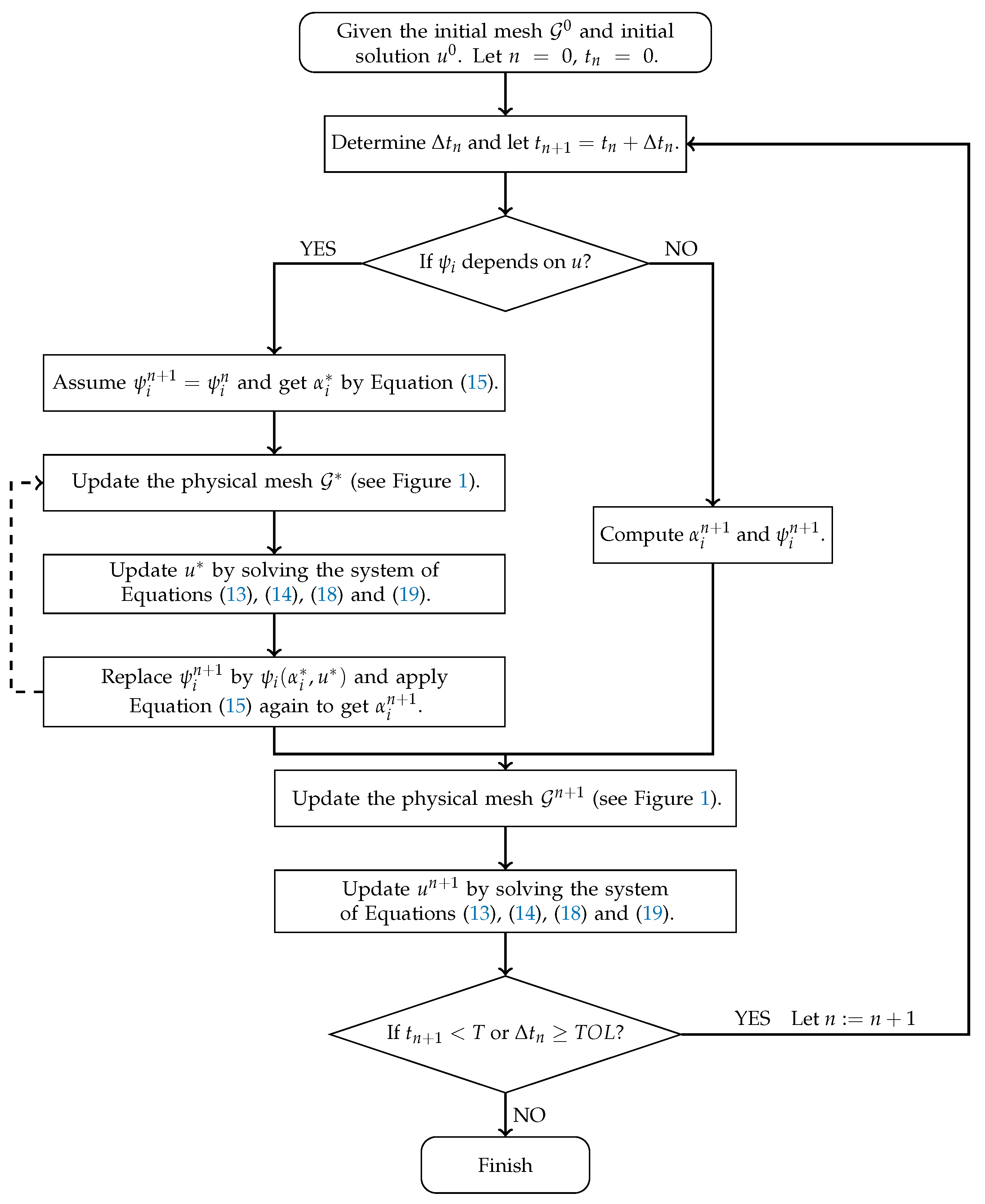
3.3. Numerical Algorithm
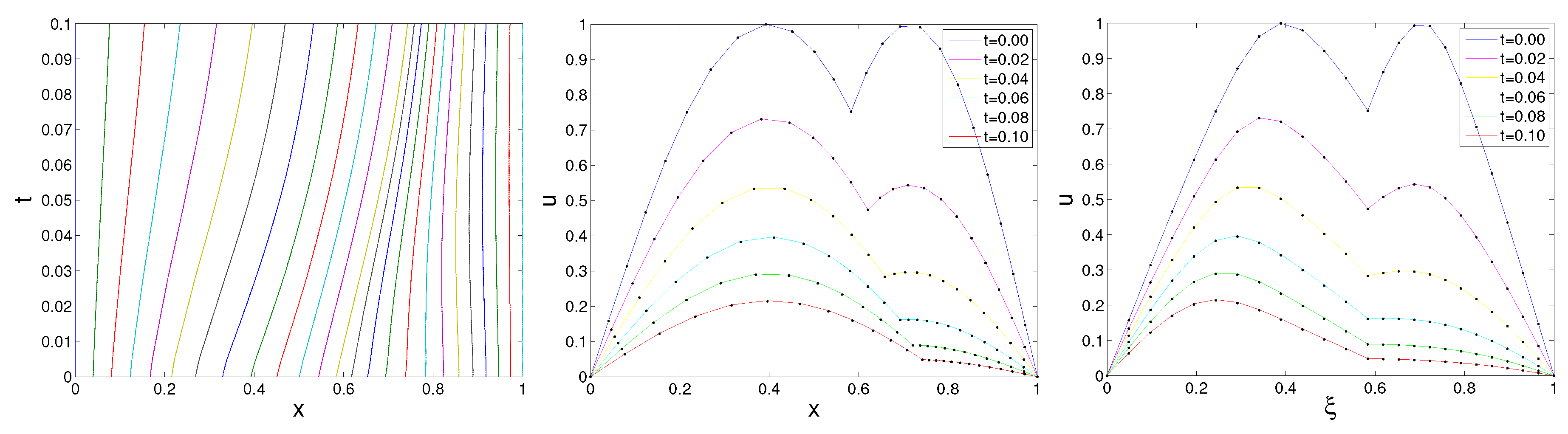
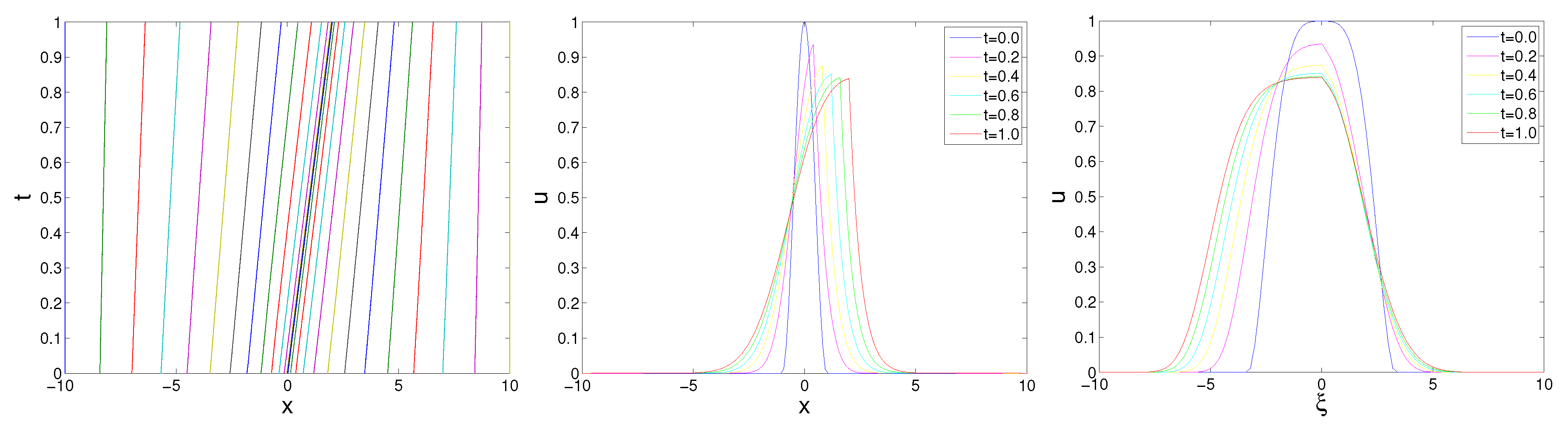
4. Numerical Examples and Discussion
5. Conclusions
Funding
Acknowledgments
Conflicts of Interest
References
- Beyer, R.P.; LeVeque, R.J. Analysis of a one-dimensional model for the immersed boundary method. SIAM J. Numer. Anal. 1992, 29, 332–364. [Google Scholar] [CrossRef]
- Mirkoohi, E.; Ning, J.; Bocchini, P.; Fergani, O.; Chiang, K.N.; Liang, S.Y. Thermal modeling of temperature distribution in metal additive manufacturing considering effects of build layers, latent heat, and temperature-sensitivity of material properties. J. Manuf. Mater. Process. 2018, 2, 63. [Google Scholar] [CrossRef]
- Ma, J.; Sun, Y.; Yang, J. Analytical solution of dual-phase-lag heat conduction in a finite medium subjected to a moving heat source. Int. J. Therm. Sci. 2018, 125, 34–43. [Google Scholar] [CrossRef]
- Sun, Y.; Liu, S.; Rao, Z.; Li, Y.; Yang, J. Thermodynamic response of beams on Winkler foundation irradiated by moving laser pulses. Symmetry 2018, 10, 328. [Google Scholar] [CrossRef]
- Reséndiz-Flores, E.O.; Saucedo-Zendejo, F.R. Two-dimensional numerical simulation of heat transfer with moving heat source in welding using the Finite Pointset Method. Int. J. Heat Mass Transf. 2015, 90, 239–245. [Google Scholar] [CrossRef]
- Kirk, C.M.; Olmstead, W.E. The influence of two moving heat sources on blow-up in a reactive-diffusive medium. Zeitschrift für Angewandte Mathematik und Physik 2000, 51, 1–16. [Google Scholar] [CrossRef]
- Kirk, C.M.; Olmstead, W.E. Blow-up in a reactive-diffusive medium with a moving heat source. Zeitschrift für angewandte Mathematik und Physik 2002, 53, 147–159. [Google Scholar] [CrossRef]
- Li, Z. Immersed interface methods for moving interface problems. Numer. Algorithms 1997, 14, 269–293. [Google Scholar] [CrossRef]
- Budd, C.J.; Huang, W.; Russell, R.D. Moving mesh methods for problems with blow-up. SIAM J. Sci. Comput. 1996, 17, 305–327. [Google Scholar] [CrossRef]
- Huang, W.; Ma, J.; Russell, R.D. A study of moving mesh PDE methods for numerical simulation of blow-up in reaction diffusion equations. J. Comput. Phys. 2008, 227, 6532–6552. [Google Scholar] [CrossRef]
- Hu, Z.; Wang, H. A moving mesh method for kinetic/hydrodynamic coupling. Adv. Appl. Math. Mech. 2012, 4, 685–702. [Google Scholar] [CrossRef]
- Cheng, J.B.; Huang, W.; Jiang, S.; Tian, B. A third-order moving mesh cell-centered scheme for one-dimensional elastic-plastic flows. J. Comput. Phys. 2017, 349, 137–153. [Google Scholar] [CrossRef]
- Lu, C.; Huang, W.; Qiu, J. An adaptive moving mesh finite element solution of the regularized long wave equation. J. Sci. Comput. 2018, 74, 122–144. [Google Scholar] [CrossRef]
- Zhang, F.; Huang, W.; Li, X.; Zhang, S. Moving mesh finite element simulation for phase-field modeling of brittle fracture and convergence of Newton’s iteration. J. Comput. Phys. 2018, 356, 127–149. [Google Scholar] [CrossRef]
- Huang, W.; Ren, Y.; Russell, R.D. Moving mesh partial differential equations (MMPDES) based on the equidistribution principle. SIAM J. Numer. Anal. 1994, 31, 709–730. [Google Scholar] [CrossRef]
- Huang, W.Z.; Russell, R.D. Adaptive Moving Mesh Methods; Springer Science & Business Media: Berlin, Germany, 2011. [Google Scholar]
- Haynes, R.D.; Russell, R.D. A Schwarz waveform moving mesh method. SIAM J. Sci. Comput. 2007, 29, 656–673. [Google Scholar] [CrossRef]
- Gander, M.J.; Haynes, R.D. Domain decomposition approaches for mesh generation via the equidistribution principle. SIAM J. Numer. Anal. 2012, 50, 2111–2135. [Google Scholar] [CrossRef]
- Haynes, R.D.; Kwok, F. Discrete analysis of domain decomposition approaches for mesh generation via the equidistribution principle. Math. Comput. 2017, 86, 233–273. [Google Scholar] [CrossRef]
- Ma, J.; Jiang, Y. Moving mesh methods for blow-up in reaction-diffusion equations with traveling heat source. J. Comput. Phys. 2009, 228, 6977–6990. [Google Scholar] [CrossRef]
- Zhu, H.; Liang, K.; Cheng, X. A numerical investigation of blow-up in reaction-diffusion problems with traveling heat sources. J. Comput. Appl. Math. 2010, 234, 3332–3343. [Google Scholar] [CrossRef]
- Hu, Z.; Wang, H. A moving mesh method for heat equation with traveling singular sources. Appl. Math. J. Chin. Univ. Ser. A 2013, 28, 115–126. (In Chinese) [Google Scholar]
- Toselli, A.; Widlund, O.B. Domain Decomposition Methods—Algorithms and Theory; Spinger: Berlin, Germany, 2005. [Google Scholar]
- Peskin, C.S. Numerical analysis of blood flow in the heart. J. Comput. Phys. 1977, 25, 220–252. [Google Scholar] [CrossRef]
- Hu, Z. Moving Mesh Method and Its Applicatios Coupled with Dynamic Domain Decomposition. Ph.D. Thesis, Zhejiang University, Hangzhou, China, 2012. (In Chinese). [Google Scholar]
- Brunner, H.; Wu, X.; Zhang, J. Computational solution of blow-up problems for semilinear parabolic PDEs on unbounded domains. SIAM J. Sci. Comput. 2010, 31, 4478–4496. [Google Scholar] [CrossRef]
- Huang, W.; Ren, Y.; Russell, R.D. Moving mesh methods based on moving mesh partial differential equations. J. Comput. Phys. 1994, 113, 279–290. [Google Scholar] [CrossRef]
- Budd, C.J.; Leimkuhler, B.; Piggott, M.D. Scaling invariance and adaptivity. Appl. Numer. Math. 2001, 39, 261–288. [Google Scholar] [CrossRef]

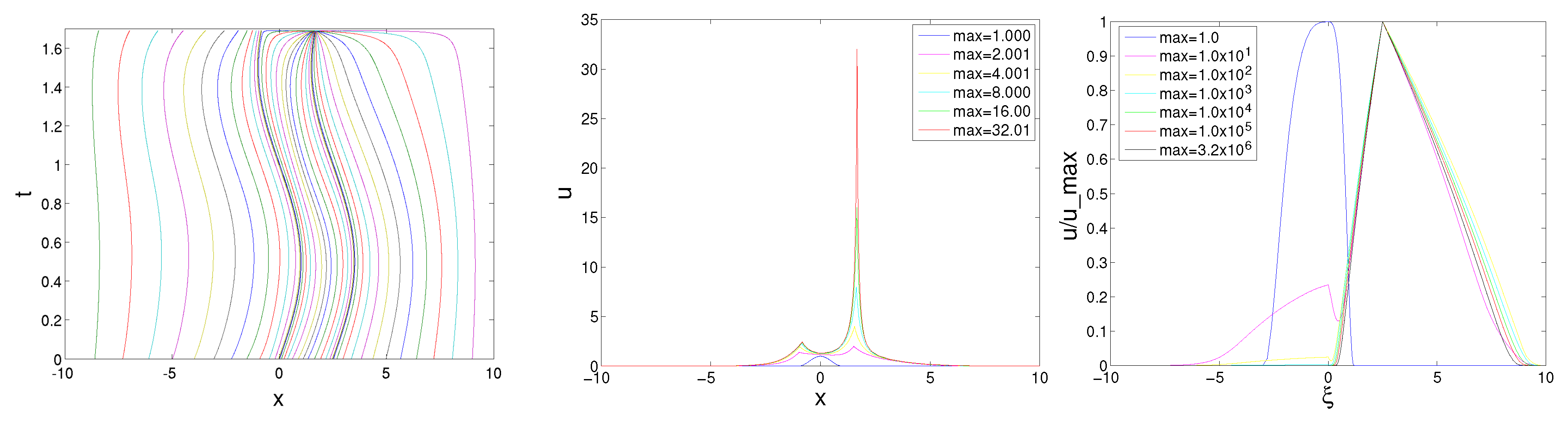

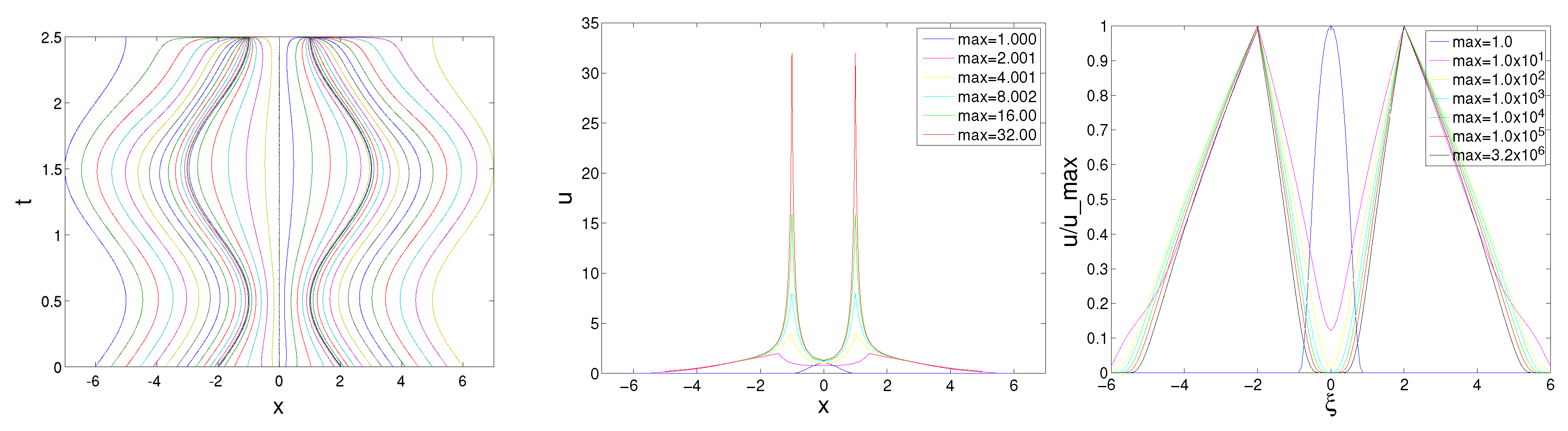
| N, L | Ratio | Ratio | Ratio | Ratio | ||||
|---|---|---|---|---|---|---|---|---|
| 40, 40 | 8.2720 × | - | 1.0931 × | - | 1.3721 × | - | 6.5460 × | - |
| 80, 160 | 2.0540 × | 4.0274 | 2.6996 × | 4.0491 | 3.3908 × | 4.0465 | 6.0108 × | 1.0890 |
| 160, 640 | 5.1038 × | 4.0245 | 6.6945 × | 4.0326 | 8.4144 × | 4.0297 | 1.1557 × | 5.2009 |
| 320, 2560 | 1.2732 × | 4.0085 | 1.6687 × | 4.0117 | 2.0981 × | 4.0104 | 2.9347 × | 3.9381 |
| 640, 10,240 | 3.1807 × | 4.0029 | 4.1678 × | 4.0038 | 5.2408 × | 4.0035 | 7.3175 × | 4.0106 |
| 1280, 40,960 | 7.9500 × | 4.0010 | 1.0416 × | 4.0013 | 1.3098 × | 4.0011 | 1.8237 × | 4.0124 |
| 2560, 163,840 | 1.9874 × | 4.0003 | 2.6038 × | 4.0003 | 3.2743 × | 4.0003 | 4.5462 × | 4.0115 |
| 2.03881627 | 1.14555847 | 0.48168300 | ||||||
| 5 | 3.10495654 | 3.49923032 | 0.61947967 | |||||
| 10 | 5.21961249 | 10.60946467 | 0.88168884 | |||||
| 25 | 11.64283736 | 16.52257933 | - | 0.78709834 | ||||
| 50 | 22.56509042 | - | 1.40807506 | - | 0.76986615 | |||
| 100 | 44.58805080 | - | 1.26522313 | - | 0.71490192 | |||
© 2018 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hu, Z. Numerical Investigation of Heat Conduction with Multiple Moving Heat Sources. Symmetry 2018, 10, 673. https://doi.org/10.3390/sym10120673
Hu Z. Numerical Investigation of Heat Conduction with Multiple Moving Heat Sources. Symmetry. 2018; 10(12):673. https://doi.org/10.3390/sym10120673
Chicago/Turabian StyleHu, Zhicheng. 2018. "Numerical Investigation of Heat Conduction with Multiple Moving Heat Sources" Symmetry 10, no. 12: 673. https://doi.org/10.3390/sym10120673
APA StyleHu, Z. (2018). Numerical Investigation of Heat Conduction with Multiple Moving Heat Sources. Symmetry, 10(12), 673. https://doi.org/10.3390/sym10120673