Cyclicity in EL–Hypergroups
Abstract
1. Introduction, Preliminaries and Historical Context
1.1. The Origins by Wall
1.2. The Original Approach of De Salvo and Freni
1.3. The Approach of Vougiouklis
1.4. An Alternative Approach of the Italian School
1.5. Corsini’s Book: A Synthesis of Approaches
1.6. Current State of the Art
1.7. A Remark Concluding the Introduction
- There exist two main approaches towards cyclicity in the hyperstructure theory: through the fact that we get a hypergroup by repeatedly applying the hyperoperation on a generator, or through the canonical projection. Both were introduced by the Italian school. The former was independently introduced by Vougiouklis and is related to the original Wall’s definition. The latter is related to the notion of completeness and the results of Koskas.
- Vougiouklis defined numerous cases of cyclicity and periods such as single power (one generator only) and finite/infinite period. The purpose of his definition was to study a specific class of cyclic hypergroups, those based on P–hyperoperations.
- The original definition of Wall, the definition of Vougiouklis and the definition using canonical projection were provided for hypergroups. The original definition of De Salvo and Freni was provided for semihypergroups.
- Cyclicity of a (semi)hypergroup (defined by the Italian schools) and period of a hypergroup (defined by Vougiouklis) are, in a general case, different.
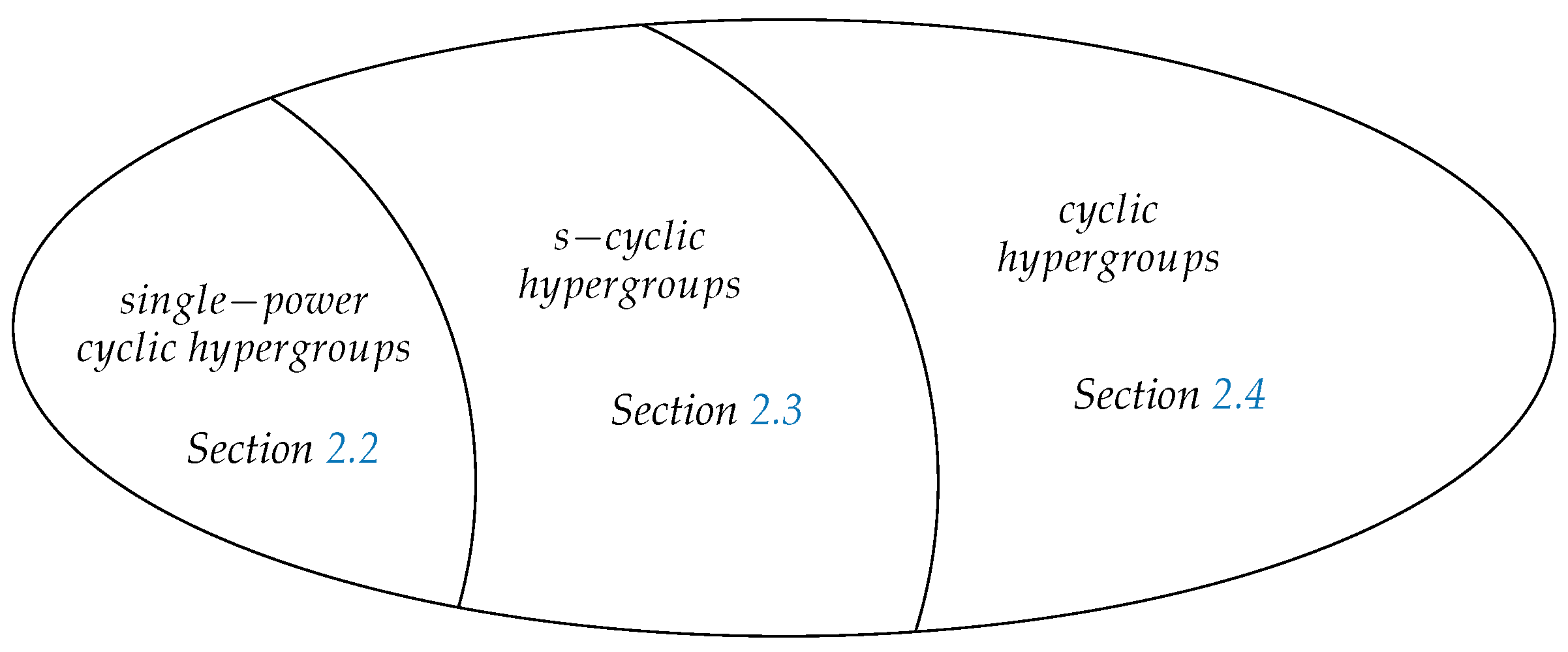
2. Examples
2.1. Theoretical Background
- For any pair , there exists a pair such that and .
- The semi-hypergroup defined by Formula (6) is a hypergroup.
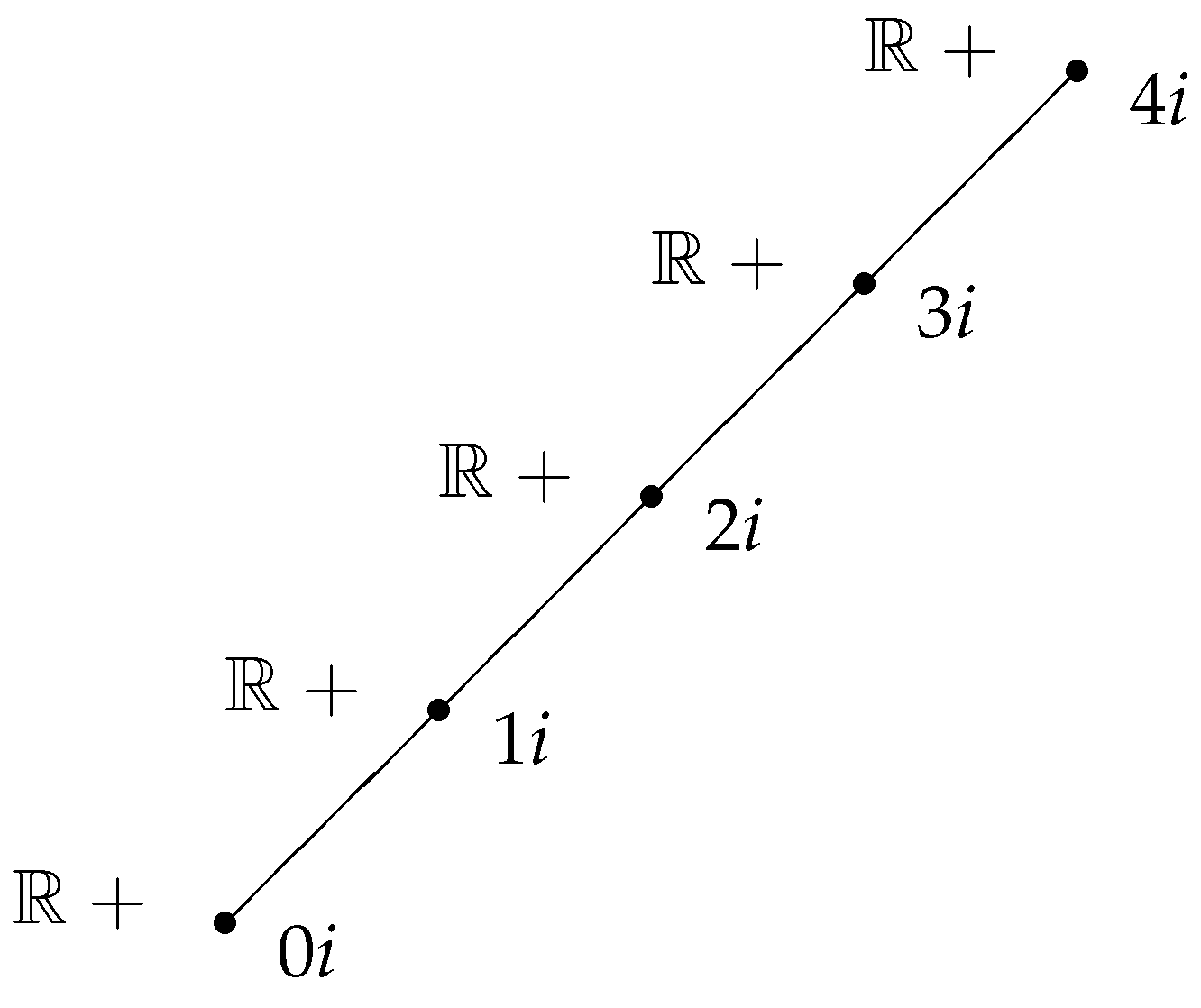
2.2. Single Power Cyclic Hypergroups
2.3. s–Cyclic Hypergroups Which Are Not Single Power Cyclic
| * | e | |||||
| e | + | − | − | − | + | + |
| + | + | + | − | − | ||
| + | + | − | − | |||
| + | − | − | ||||
| + | + | |||||
| + |
2.4. Cyclic Hypergroups Which Are Not s–Cyclic
| * | 1 | 2 | 3 | 4 |
| 1 | ||||
| 2 | ||||
| 3 | ||||
| 4 |
3. New Results
- If , then , i.e., .
- If are such that exists, then , i.e., .
4. Conclusions and Future Work
- operationally equivalent if and , for all ,
- inseparable if for we have if and only if ,
- essentially indistinguishable if they are operationally equivalent and inseparable.
Author Contributions
Funding
Conflicts of Interest
References
- Marty, F. Sur une généralisation de la notion de groupe. IV Congrès des Mathématiciens Scandinaves 1934, 45–49. [Google Scholar]
- Wall, H.S. Hypergroups. Am. J. Math. 1934, 59, 77–98. [Google Scholar] [CrossRef]
- De Salvo, M.; Freni, D. Sugli ipergruppi ciclici e completi. Matematiche (Catania) 1980, 35, 211–226. [Google Scholar]
- Corsini, P. Sur les semi-hypergroupes complètes et les groupoides. Atti Soc. Pel. Sci. Fis. Mat. Nat. 1980, 26, 391–398. [Google Scholar]
- Corsini, P.; Romeo, G. Hypergroupes complètes et T–groupoids; Atti Convegno su “Sistemi binari e loro applicazioni”: Taormina, Italy, 1978; pp. 129–146. [Google Scholar]
- De Salvo, M. Sugli ipergruppi completi finiti. Riv. Mat. Univ. Parma 1982, 8, 269–280, (submitted 1980). [Google Scholar]
- Freni, D. Ipergruppi ciclici e torsione negli ipergruppi. Matematiche (Catania) 1980, 35, 270–286. [Google Scholar]
- De Salvo, M.; Freni, D. Semi-ipergruppi e ipergruppi ciclici. Atti Sem. Mat. Fis. Univ. Modena 1981, 30, 44–59. [Google Scholar]
- Freni, D. Una nota su gli ipergruppoidi ciclici. Ratio Mathematica 1995, 9, 101–111. [Google Scholar]
- Vougiouklis, T. Cyclicity in a special class of hypergroups. Acta Univ. Carolinae Math. Phys. 1981, 22, 3–6. [Google Scholar]
- Konguetsof, L.; Vougiouklis, T.; Kessoglides, M.; Spartalis, S. On cyclic hypergroups with period. Acta Univ. Carolinae Math. Phys. 1987, 28, 3–7. [Google Scholar]
- Vougioulis, T. Isomorphisms on P–hypergroups and cyclicity. Ars Combinatoria 1990, 29A, 241–245. [Google Scholar]
- De Salvo, M.; Freni, D. Ipergruppi finitamente generati. Riv. Mat. Univ. Parma 1986, 12, 177–186. [Google Scholar]
- De Salvo, M. Su le potenze ad esponente intero in un ipergruppo e gli r–ipergruppi. Riv. Mat. Univ. Parma 1985, 4, 409–421. [Google Scholar]
- Antampoufis, N.; Hošková-Mayerová, Š. A brief survey on the two different approaches of fundamental equivalence relations on hyperstructures. Ratio Math. 2017, 33, 47–60. [Google Scholar]
- Koskas, M. Groupoids, demi-hypergroupes et hypergroupes. J. Math. Pure Appl. 1970, 48, 155–192. [Google Scholar]
- Freni, D. A note on the core of a hypergroup and the transitive closure β⋆ of β. Riv. Mat. Pura Appl. 1991, 8, 153–156. [Google Scholar]
- Corsini, P. Prolegomena of Hypergroup Theory; Aviani Editore: Tricesimo, Italy, 1993. [Google Scholar]
- Corsini, P.; Leoreanu, V. Applications of Hyperstructure Theory; Kluwer Academic Publishers: Dodrecht, The Netherlands; Boston, MA, USA; London, UK, 2003. [Google Scholar]
- Al Tahan, M.; Davvaz, B. On some properties of single power cyclic hypergroups and regular relations. J. Algebra Appl. 2017, 16, 1750214. [Google Scholar] [CrossRef]
- Al Tahan, M.; Davvaz, B. On a special single-power cyclic hypergroup and its automorphisms. Discrete Math. Algorithm. Appl. 2016, 8, 1650059. [Google Scholar] [CrossRef]
- Karimian, M.; Davvaz, B. On the γ-cyclic hypergroups. Commun. Algebra 2006, 34, 4579–4589. [Google Scholar] [CrossRef]
- Freni, D. A new characterization of the derived hypergroup via strongly regular equivalencies. Commun. Algebra 2002, 30, 3977–3989. [Google Scholar] [CrossRef]
- Chvalina, J. Commutative hypergroups in the sense of Marty and ordered sets. In General Algebra and Ordered Sets, Proceedings of the International Conference Olomouc; Verlag Johannes Heyn: Olomouc, Czech Republic, 1994; pp. 19–30. [Google Scholar]
- Chvalina, J. Functional Graphs, Quasi-Ordered Sets and Commutative Hypergroups; Masaryk University: Brno, Czech Republic, 1995. (In Czech) [Google Scholar]
- Massouros, C.G. On path hypercompositions in graphs and automata. MATEC Web Conf. 2016, 41, 05003. [Google Scholar] [CrossRef]
- Novák, M. On EL–semihypergroups. Eur. J. Combin. 2015, 44 Pt B, 274–286. [Google Scholar] [CrossRef]
- Novák, M. Some basic properties of EL–hyperstructures. Eur. J. Combin. 2013, 34, 446–459. [Google Scholar] [CrossRef]
- Novák, M.; Cristea, I. Composition in EL–hyperstructures. Hacet. J. Math. Stat. 2018, in press. [Google Scholar]
- Křehlík, Š.; Novák, M. From lattices to Hv–matrices. An. Şt. Univ. Ovidius Constanţa 2016, 24, 209–222. [Google Scholar] [CrossRef]
- Novák, M.; Křehlík, Š. EL–hyperstructures revisited. Soft Comput. 2018, 22, 7269–7280. [Google Scholar] [CrossRef]
- Chvalina, J.; Křehlík, Š.; Novák, M. Cartesian composition and the problem of generalising the MAC condition to quasi-multiautomata. An. Şt. Univ. Ovidius Constanţa 2016, 24, 79–100. [Google Scholar]
- Pickett, H.E. Homomorphisms and subalgebras of multialgebras. Pac. J. Math. 1967, 21, 327–342. [Google Scholar] [CrossRef]
- Novák, M. EL–semihypergroups in which the quasi-ordering is not antisymmetric. In Mathematics, Information Technologies and Applied Sciences 2017: Post-Conference Proceedings of Extended Versions of Selected Papers; University of Defence: Brno, Czech Republic, 2017; pp. 183–192. [Google Scholar]
- Jantosciak, J. Reduced hypergroups. In Algebraic Hyperstructures and Applications, Proceedings of the Fourth International Congress, Xanthi, Greece, 1990; Vougiouklis, T., Ed.; World Scientific: Singapore, 1991; pp. 119–122. [Google Scholar]
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Novák, M.; Křehlík, Š.; Cristea, I. Cyclicity in EL–Hypergroups. Symmetry 2018, 10, 611. https://doi.org/10.3390/sym10110611
Novák M, Křehlík Š, Cristea I. Cyclicity in EL–Hypergroups. Symmetry. 2018; 10(11):611. https://doi.org/10.3390/sym10110611
Chicago/Turabian StyleNovák, Michal, Štepán Křehlík, and Irina Cristea. 2018. "Cyclicity in EL–Hypergroups" Symmetry 10, no. 11: 611. https://doi.org/10.3390/sym10110611
APA StyleNovák, M., Křehlík, Š., & Cristea, I. (2018). Cyclicity in EL–Hypergroups. Symmetry, 10(11), 611. https://doi.org/10.3390/sym10110611





