The Variety of 7-Dimensional 2-Step Nilpotent Lie Algebras
Abstract
:1. Introduction
2. The Variety
3. Degenerations of Lie Algebras
- (a)
- ,
- (b)
- ,
- (c)
- , where is the center of ,
- (d)
- for , where is the k-th trivial cohomology group for ,
- (e)
- , where is the maximal abelian subalgebra of .
The Classification of Complex 7-Dimensional 2-Step Nilpotent Lie Algebras
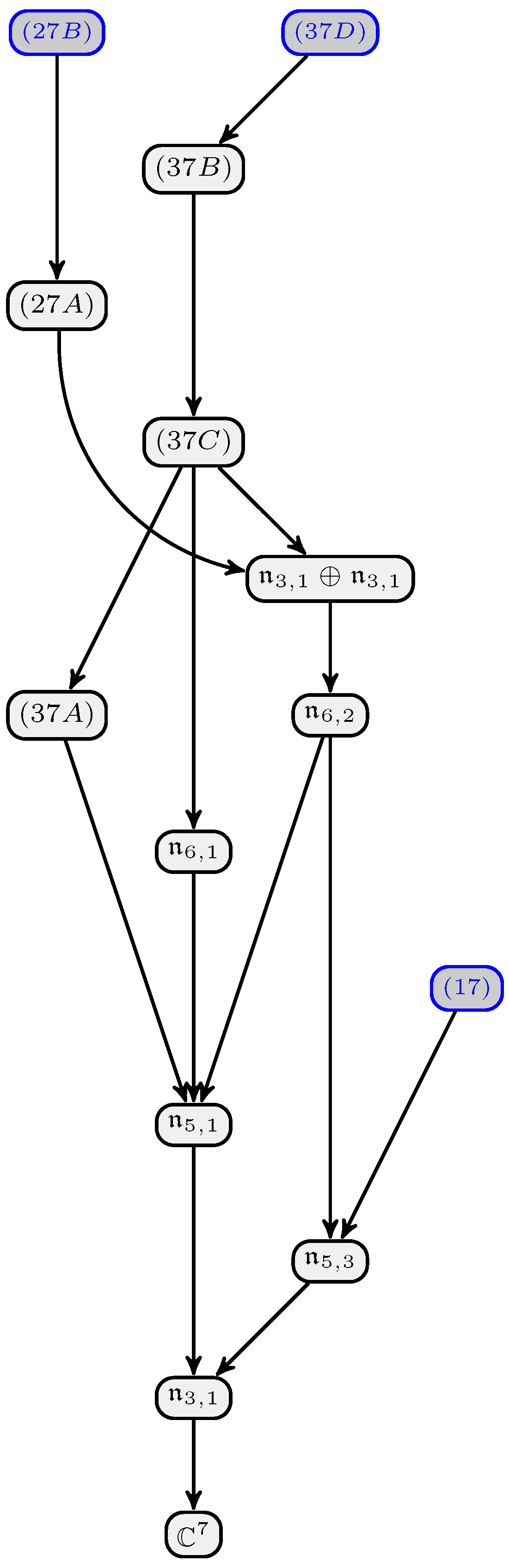
4. The Irreducible Components
- 1.
- ,
- 2.
- ,
- 3.
- .
Acknowledgments
Conflicts of Interest
References
- Burde, D. Degenerations of nilpotent Lie algebras. J. Lie Theory 1999, 9, 193–202. [Google Scholar]
- Burde, D. Degenerations of 7-Dimensional Nilpotent Lie Algebras. Commun. Algebra 2005, 33, 1259–1277. [Google Scholar] [CrossRef]
- Burde, D.; Steinhoff, C. Classification of Orbit Closures of 4-Dimensional Complex Lie Algebras. J. Algebra 1999, 214, 729–739. [Google Scholar] [CrossRef]
- Carles, R.; Diakité, Y. Sur les variétés d’algèbres de Lie de dimension ≤7. J. Algebra 1984, 91, 53–63. [Google Scholar] [CrossRef]
- Grunewald, F.; O’Hallloran, J. Varieties of Nilpotent Lie Algebras of Dimension Less Than Six. J. Algebra 1988, 112, 315–325. [Google Scholar] [CrossRef]
- Kirillov, A.A.; Neretin, Y.A. The Variety An of n-Dimensional Lie Algebra Structures. Am. Math. Soc. Transl. 1987, 137, 21–30. [Google Scholar]
- Lauret, J. Degenerations of Lie algebras and geometry of Lie groups. Differ. Geom. Appl. 2003, 18, 177–194. [Google Scholar] [CrossRef]
- Nesterenko, M.; Popovych, R. Contractions of low-dimensional Lie algebras. J. Math. Phys. 2006, 47, 123515. [Google Scholar] [CrossRef]
- Seeley, C. Degenerations of 6-dimensional nilpotent lie algebras over . Commun. Algebra 1990, 18, 3493–3505. [Google Scholar]
- Weimar-Woods, E. The three-dimensional real Lie algebras and their contractions. J. Math. Phys. 1991, 32, 2028–2033. [Google Scholar] [CrossRef]
- Ancochea Bermúdez, J.M.; Fresán, J.; Margalef Bentabol, J. Contractions of low-dimensional nilpotent Jordan algebras. Commun. Algebra 2011, 39, 1139–1151. [Google Scholar] [CrossRef]
- Gorshkov, I.; Kaygorodov, I.; Popov, Y. Degenerations of Jordan Algebras. arXiv, 2017; arXiv:1707.08836. [Google Scholar]
- Kashuba, I.; Martin, M.E. Deformations of Jordan algebras of dimension four. J. Algebra 2014, 399, 277–289. [Google Scholar] [CrossRef]
- Kashuba, I.; Patera, J. Graded contractions of Jordan algebras and of their representations. J. Phys. A-Math. Gen. 2003, 36, 12453. [Google Scholar]
- Casas, J.M.; Khudoyberdiyev, A.K.; Ladra, M.; Omirov, B.A. On the degenerations of solvable Leibniz algebras. Linear Algebra Appl. 2013, 439, 315–325. [Google Scholar] [CrossRef]
- Ismailov, N.; Kaygorodov, I.; Volkov, Y. The geometric classification of Leibniz algebras. arXiv, 2017; arXiv:1705.04346. [Google Scholar]
- Kaygorodov, I.; Popov, Y.; Pozhidaev, A.; Volkov, Y. Degenerations of nilpotent Leibniz and Zinbiel algebras. Linear Multilinear Algebra, 2018; arXiv:1611.06454. [Google Scholar]
- Beneš, T.; Burde, D. Degenerations of pre-Lie algebras. J. Math. Phys. 2009, 50, 112102. [Google Scholar] [CrossRef]
- Beneš, T.; Burde, D. Classification of orbit closures in the variety of three dimensional Novikov algebras. J. Algebra Appl. 2014, 13, 1350081. [Google Scholar] [CrossRef]
- De Azcarraga, J.; Izquierdo, J.; Picon, M. Contractions of Filippov algebras. J. Math. Phys. 2011, 52, 013516. [Google Scholar] [CrossRef]
- Kaygorodov, I.; Popov, Y.; Volkov, Y. Degenerations of binary Lie and nilpotent Malcev algebras. arXiv, 2016; arXiv:1609.07392. [Google Scholar]
- Armour, A.; Zhang, Y. Geometric Classification of 4-Dimensional Superalgebras, Chapter Algebra, Geometry and Mathematical Physics. Springer Proc. Math. Stat. 2014, 85, 291–323. [Google Scholar]
- Alvarez, M.A.; Hernández, I.; Universidad de Antofagasta, Antofagasta, Chile. Unpublished work. 2017.
- Alvarez, M.A.; Hernández, I.; Kaygorodov, I. Degenerations of Jordan superalgebras. arXiv, 2017; arXiv:1708.07758. [Google Scholar]
- Goze, M.; Remm, E. k-step nilpotent Lie algebras. Georgian Math. J. 2015, 22, 219–234. [Google Scholar] [CrossRef]
- Brega, A.; Cagliero, L.; Chaves-Ochoa, A. The Nash—Moser theorem of Hamilton and rigidity of finite dimensional nilpotent Lie algebras. J. Pure Appl. Algebra 2017, 221, 2250–2265. [Google Scholar] [CrossRef]
- Alvarez, M.A. On rigid 2-step nilpotent Lie algebras. Algebra Colloq. (In Press).
- Mumford, D. The red book of varieties and schemes. In Lecture Notes in Mathematics; Springer: Berlin, Germany, 1988. [Google Scholar]
- Borel, A. Linear Algebraic Groups. In Graduate Texts in Mathematics, 2nd ed.; Springer: Berlin, Germany, 1991. [Google Scholar]
- Crawley-Boevey, W. Geometry of Representations of Algebras; Lecture Notes; Oxford University: Oxford, UK, 1993. [Google Scholar]
- Gong, M.P. Classification of Nilpotent Lie Algebras of Dimension 7 (over Algebraically Closed Fields and ). Ph.D. Thesis, University of Waterloo, Waterloo, ON, Canada, 1998. [Google Scholar]
- Šnobl, L.; Winternitz, P. Classification and Identification of Lie Algebras; CRM Monograph Series; American Mathematical Society: Providence, RI, USA, 2014. [Google Scholar]
| Lie Product | |||||
|---|---|---|---|---|---|
| , | , | 21 | |||
| , | , | 28 | |||
| , | , | , | 30 | ||
| , | , | 24 | |||
| , | , | 29 | |||
| , | , | , | 27 | ||
| , | , | , | 30 | ||
| , | , | 24 | |||
| , | , | 25 | |||
| , | 26 | ||||
| , | 22 | ||||
| , | 20 | ||||
| 15 | |||||
| 0 | |||||
| Reason Lemma 2 | |
|---|---|
| Parametrized Basis | |||||||
|---|---|---|---|---|---|---|---|
| , | , | , | , | , | , | ||
| , | , | , | , | , | , | ||
| , | , | , | , | , | , | ||
| , | , | , | , | , | , | ||
| , | , | , | , | , | , | ||
| , | , | , | , | , | , | ||
| , | , | , | , | , | |||
| , | , | , | , | , | , | ||
| , | , | , | , | , | , | ||
| , | , | , | , | , | , | ||
| , | , | , | , | , | , | ||
| , | , | , | , | , | , | ||
| , | , | , | , | , | , | ||
| , | , | , | , | , | |||
| , | , | , | , | , | , | ||
© 2018 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Alvarez, M.A. The Variety of 7-Dimensional 2-Step Nilpotent Lie Algebras. Symmetry 2018, 10, 26. https://doi.org/10.3390/sym10010026
Alvarez MA. The Variety of 7-Dimensional 2-Step Nilpotent Lie Algebras. Symmetry. 2018; 10(1):26. https://doi.org/10.3390/sym10010026
Chicago/Turabian StyleAlvarez, María Alejandra. 2018. "The Variety of 7-Dimensional 2-Step Nilpotent Lie Algebras" Symmetry 10, no. 1: 26. https://doi.org/10.3390/sym10010026
APA StyleAlvarez, M. A. (2018). The Variety of 7-Dimensional 2-Step Nilpotent Lie Algebras. Symmetry, 10(1), 26. https://doi.org/10.3390/sym10010026




