Evaluation of Soil Hydraulic Properties in Northern and Central Tunisian Soils for Improvement of Hydrological Modelling
Abstract
1. Introduction
2. Materials and Methods
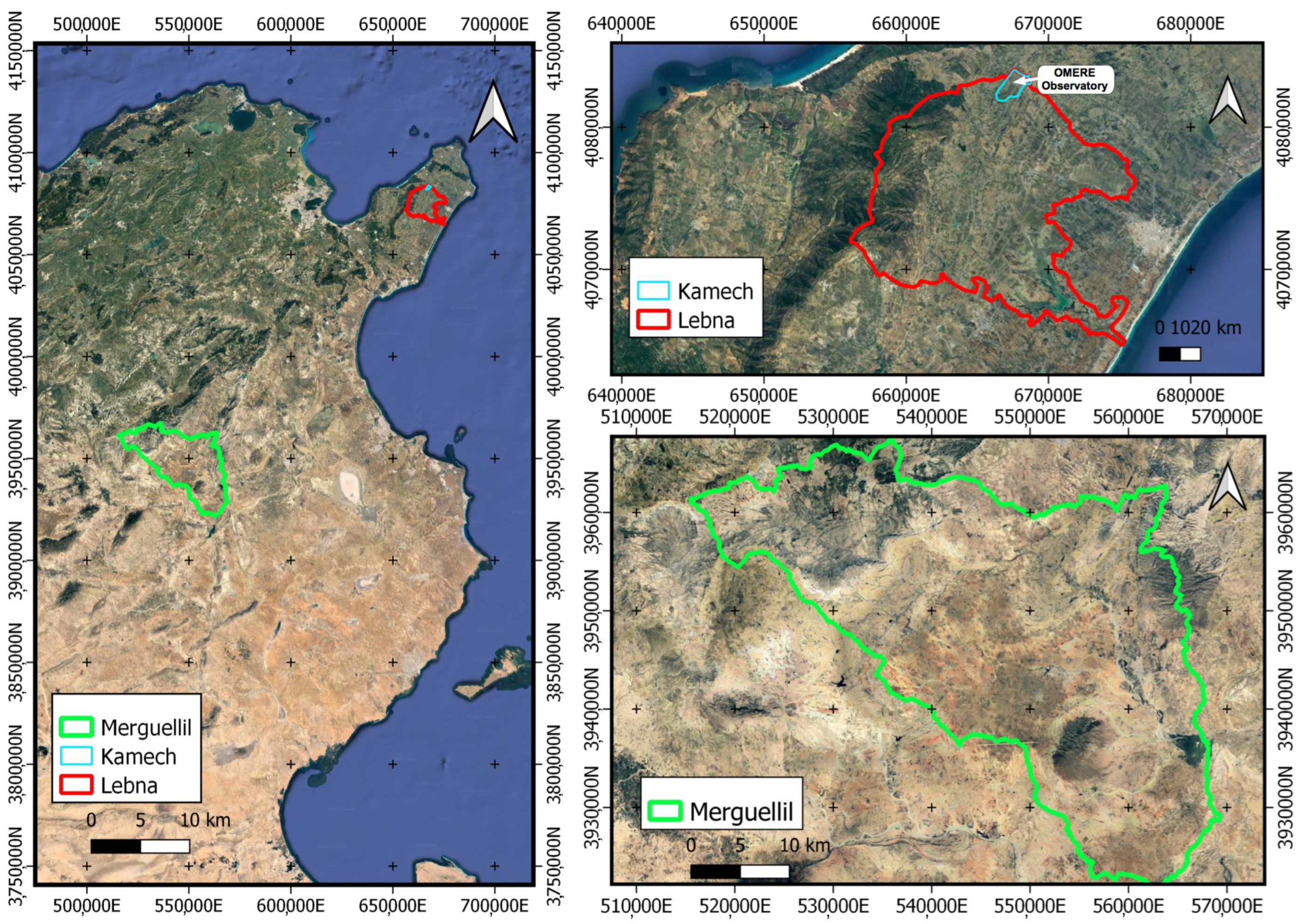
2.1. Study Area
2.2. Experimental Design
2.3. Estimation of Soil Hydraulic Properties
2.4. The Empirical Method: ROSETTA
2.5. The Arya and Paris (AP) Model
2.6. The BEST Method
2.7. Water Flow Modelling
2.8. Data Analysis
3. Results
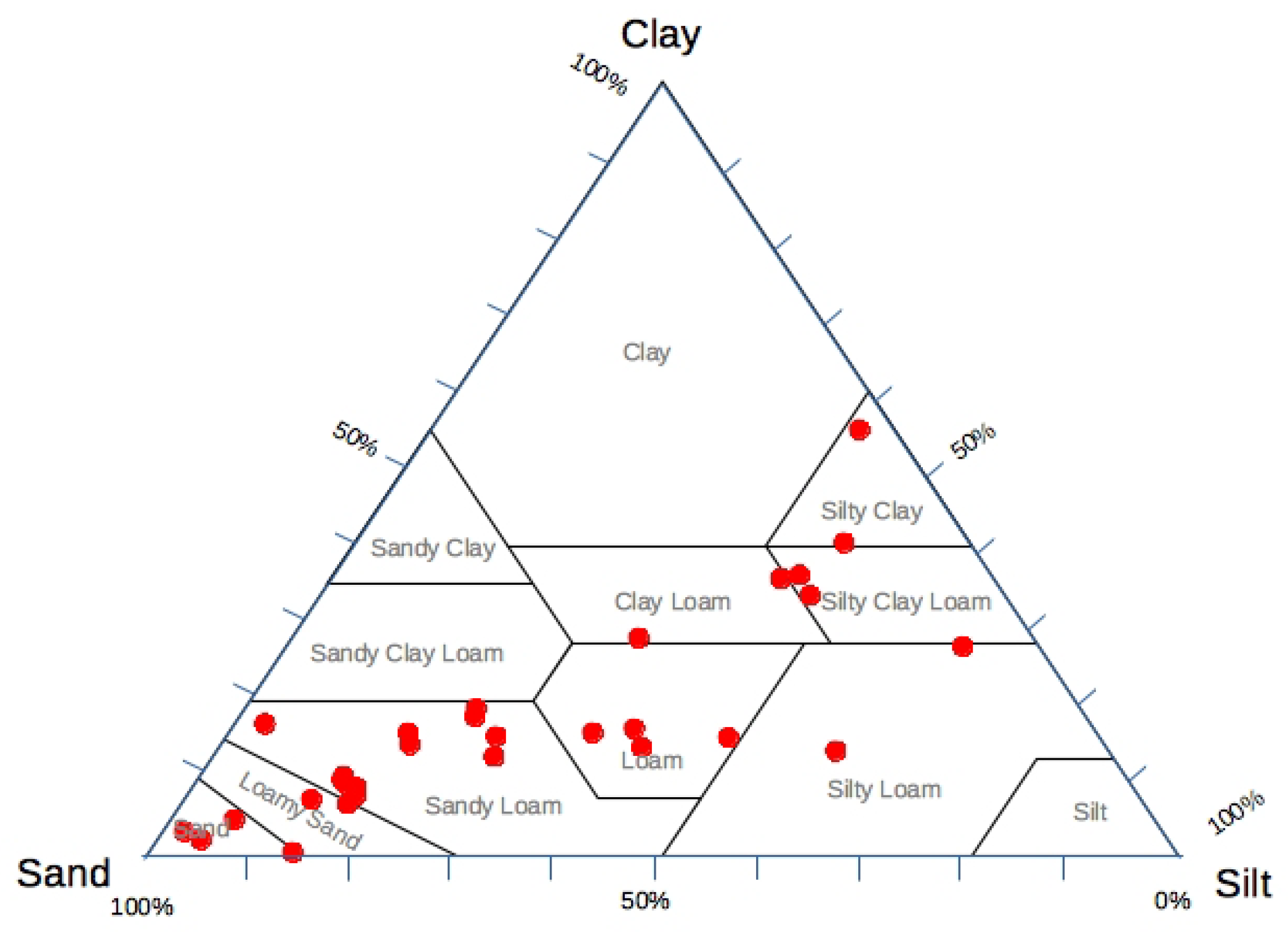
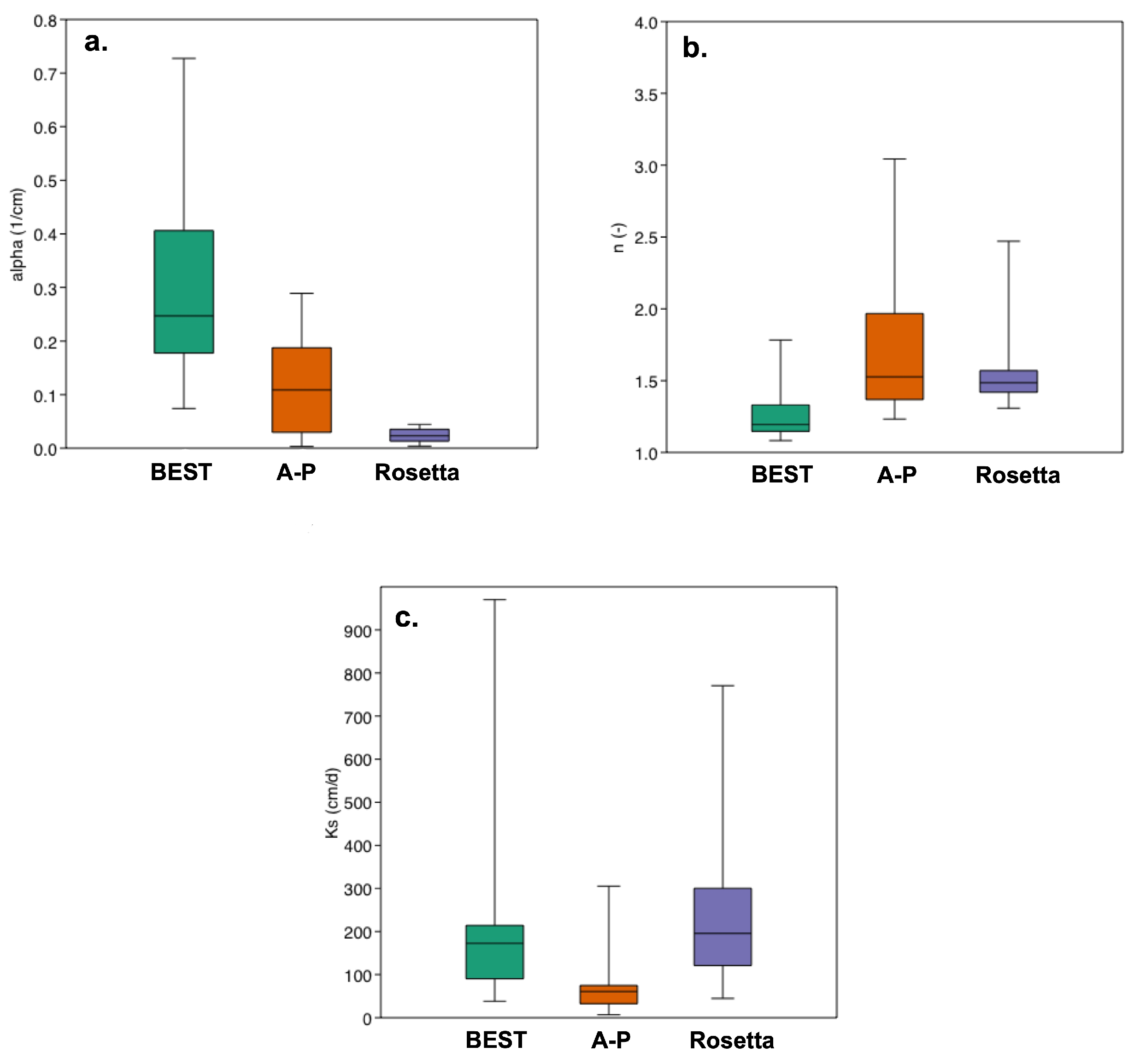
3.1. The Soil Hydraulic Parameters
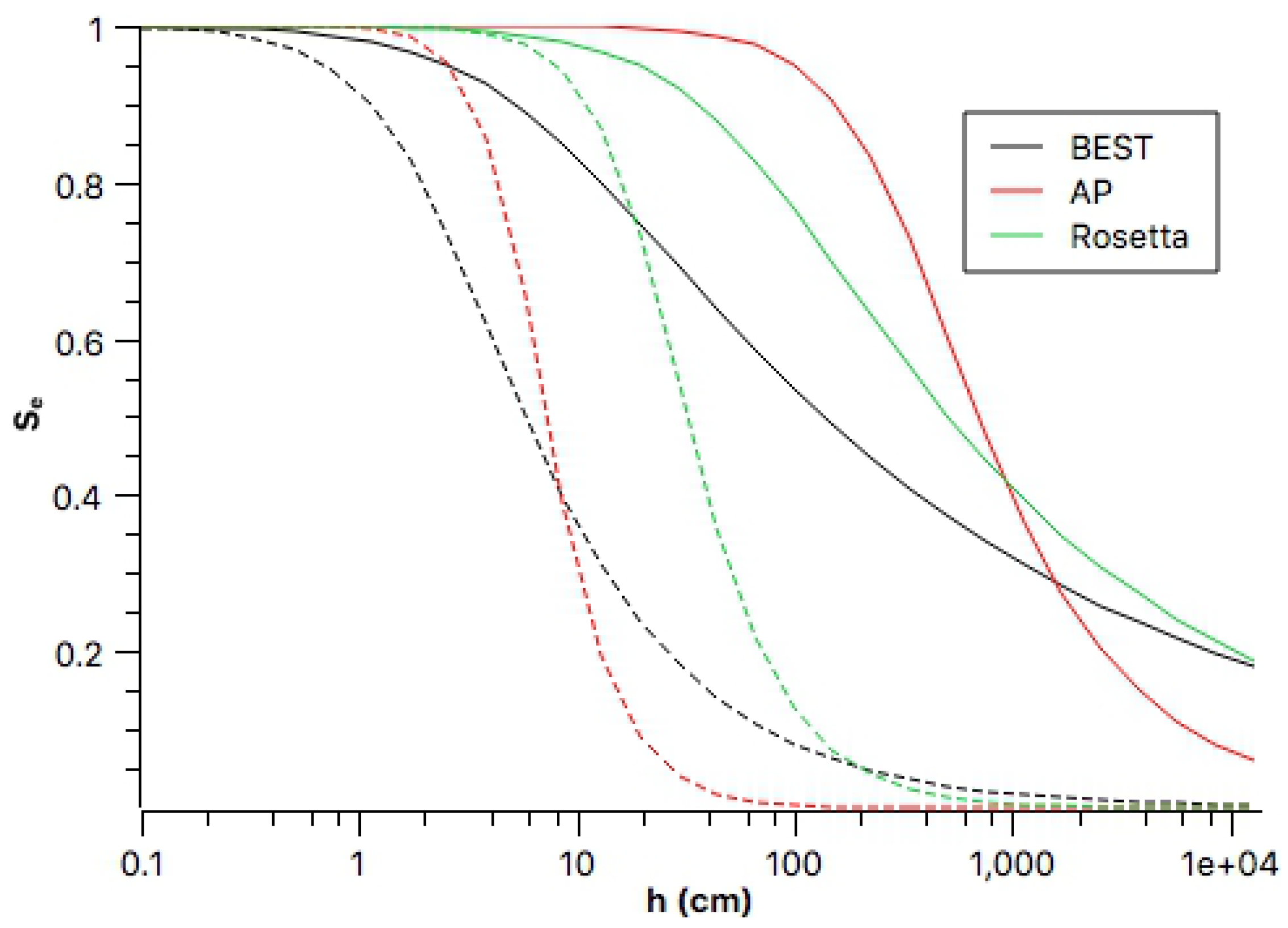
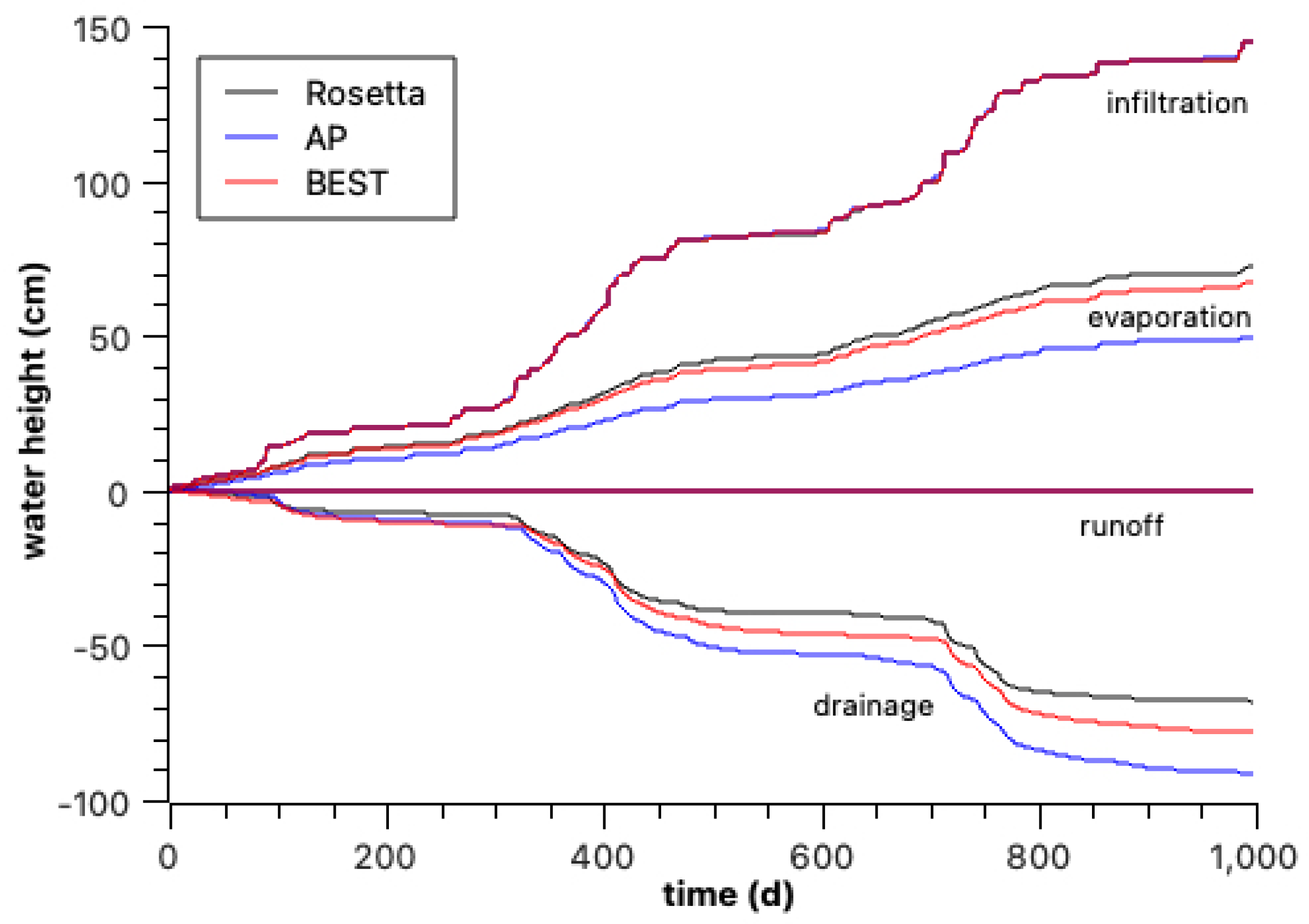
Modelling Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A
Appendix A.1
References
- Maier, F.; Van Meerveld, I.; Greinwald, K.; Gebauer, T.; Lustenberger, F.; Hartmann, A.; Musso, A. Effects of Soil and Vegetation Development on Surface Hydrological Properties of Moraines in the Swiss Alps. Catena 2020, 187, 104353. [Google Scholar] [CrossRef]
- Archer, N.A.L.; Bonell, M.; Coles, N.; MacDonald, A.M.; Auton, C.A.; Stevenson, R. Soil Characteristics and Landcover Relationships on Soil Hydraulic Conductivity at a Hillslope Scale: A View towards Local Flood Management. J. Hydrol. 2013, 497, 208–222. [Google Scholar] [CrossRef]
- Zimmermann, A.; Schinn, D.S.; Francke, T.; Elsenbeer, H.; Zimmermann, B. Uncovering Patterns of Near-Surface Saturated Hydraulic Conductivity in an Overland Flow-Controlled Landscape. Geoderma 2013, 195–196, 1–11. [Google Scholar] [CrossRef]
- Lassabatere, L.; Peyneau, P.-E.; Yilmaz, D.; Pollacco, J.; Fernández-Gálvez, J.; Latorre, B.; Moret-Fernández, D.; Di Prima, S.; Rahmati, M.; Stewart, R.D.; et al. Scaling procedure for straightforward computation of sorptivity. Hydrol. Earth Syst. Sci. Discuss. 2021, 25, 5083–5102. [Google Scholar] [CrossRef]
- Lassabatere, L.; Peyneau, P.-E.; Yilmaz, D.; Pollacco, J.; Fernández-Gálvez, J.; Latorre, B.; Moret-Fernández, D.; Di Prima, S.; Rahmati, M.; Stewart, R.D.; et al. Mixed formulation for an easy and robust numerical computation of sorptivity. Hydrol. Earth Syst. Sci. 2023, 27, 895–915. [Google Scholar] [CrossRef]
- Simonson, R.W. Outline of a generalized theory of soil genesis1. Soil Sci. Soc. Am. J. 1959, 23, 152–156. [Google Scholar] [CrossRef]
- Lin, H. Temporal stability of soil moisture spatial pattern and subsurface preferential flow pathways in the Shale Hills catchment. Vadose Zone J. 2006, 5, 317–340. [Google Scholar] [CrossRef]
- Wang, Z.; Shi, W. Robust variogram estimation combined with isometric log-ratio transformation for improved accuracy of soil particle-size fraction mapping. Geoderma 2018, 324, 56–66. [Google Scholar] [CrossRef]
- She, D.; Qian, C.; Timm, L.C.; Beskow, S.; Wei, H.; Caldeira, T.L.; Oliveira, L.M. Multi-scale correlations between soil hydraulic properties and associated factors along a Brazilian watershed transect. Geoderma 2017, 286, 15–24. [Google Scholar]
- Wang, Y.; Shao, M.; Liu, Z.; Horton, R. Regional-scale variation and distribution patterns of soil saturated hydraulic conductivities in surface and subsurface layers in the loessial soils of China. J. Hydrol. 2013, 487, 13–23. [Google Scholar] [CrossRef]
- Deb, S.K.; Shukla, M.K. Variability of hydraulic conductivity due to multiple factors. Am. J. Environ. Sci. 2012, 8, 489–502. [Google Scholar] [CrossRef]
- Papanicolaou, A.N.; Elhakeem, M.; Wilson, C.G.; Burras, C.L.; West, L.T.; Lin, H.; Clark, B.; Oneal, B.E. Spatial variability of saturated hydraulic conductivity at the hillslope scale: Understanding the role of land management and erosional effect. Geoderma 2015, 243–244, 58–68. [Google Scholar] [CrossRef]
- Kanso, T.; Tedoldi, D.; Gromaire, M.C.; Ramier, D.; Saad, M.; Chebbo, G. Horizontal and Vertical Variability of Soil Hydraulic Properties in Roadside Sustainable Drainage Systems (SuDS)—Nature and Implications for Hydrological Performance Evaluation. Water 2018, 10, 987. [Google Scholar] [CrossRef]
- Das Gupta, S.; Mohanty, B.P.; Köhne, J.M. Soil Hydraulic Conductivities and their Spatial and Temporal Variations in a Vertisol. Soil Sci. Soc. Am. J. 2006, 70, 1872–1881. [Google Scholar] [CrossRef]
- Rienzner, M.; Gandolfi, C. Investigation of spatial and temporal variability of saturated soil hydraulic conductivity at the field-scale. Soil Tillage Res. 2014, 135, 28–40. [Google Scholar] [CrossRef]
- Yang, G.; Xu, Y.; Huo, L.; Wang, H. Analysis of Temperature Effect on Saturated Hydraulic Conductivity of the Chinese Loess. Water 2022, 14, 1327. [Google Scholar] [CrossRef]
- Sobieraj, J.A.; Elsenbeer, H.; Coelho, R.M.; Newton, B. Spatial Variability of Soil Hydraulic Conductivity along a Tropical Rainforest Catena. Geoderma 2002, 108, 79–90. [Google Scholar] [CrossRef]
- Santra, P.; Chopra, U.; Chakraborty, D. Spatial variability of soil properties and its application in predicting surface map of hydraulic parameters in an agricultural farm. Curr. Sci. 2008, 95, 937–945. [Google Scholar]
- Usowicz, B.; Lipiec, J. Spatial variability of saturated hydraulic conductivity and its links with other soil properties at the regional scale. Sci. Rep. 2021, 11, 8293. [Google Scholar] [CrossRef]
- Libohova, Z.; Schoeneberger, P.; Bowling, L.C.; Owens, P.R.; Wysocki, D.; Wills, S.; Williams, C.O.; Seybold, C. Soil systems for upscaling saturated hydraulic conductivity for hydrological modeling in the critical zone. Vadose Zone J. 2018, 17, 170051. [Google Scholar] [CrossRef]
- Qiao, J.; Zhu, Y.; Jia, X.; Huang, L.; Shao, M. Estimating the Spatial Relationships between Soil Hydraulic Properties and Soil Physical Properties in the Critical Zone (0–100 m) on the Loess Plateau, China: A State-Space Modeling Approach. Catena 2018, 160, 385–393. [Google Scholar] [CrossRef]
- Becker, R.; Gebremichael, M.; Märker, M. Impact of soil surface and subsurface properties on soil saturated hydraulic conductivity in the semi-arid Walnut Gulch Experimental Watershed, Arizona, USA. Geoderma 2018, 322, 112–120. [Google Scholar] [CrossRef]
- Baiamonte, G.; Bagarello, V.; D’Asaro, F.; Palmeri, V. Factors influencing point measurement of near-surface saturated soil hydraulic conductivity in a small Sicilian basin. Land Degrad. Dev. 2017, 28, 970–982. [Google Scholar] [CrossRef]
- Price, K.; Jackson, C.R.; Parker, A.J. Variation of surficial soil hydraulic properties across land uses in the southern Blue Ridge Mountains, North Carolina, USA. J. Hydrol. 2010, 383, 256–268. [Google Scholar] [CrossRef]
- Salemi, L.F.; Groppo, J.D.; Trevisan, R.; Moraes, J.M.; Ferraz, S.F.B.; Villani, J.P.; Duarte-Neto, P.J.; Martinelli, L.A. Land-use change in the Atlantic rainforest region: Consequences for the hydrology of small catchments. J. Hydrol. 2013, 499, 100–109. [Google Scholar] [CrossRef]
- Pinto, L.C.; Mello, C.R.; Norton, L.D.; Curi, N. Land-use influence on the soil hydrology: An approach in upper Grande River basin, Southeast Brazil. Ciênc. Agrotec. 2019, 43, e015619. [Google Scholar] [CrossRef]
- Picciafuoco, T.; Morbidelli, R.; Flammini, A.; Saltalippi, C.; Corradini, C.; Strauss, P.; Blöschl, G. A pedotransfer function for field-scale saturated hydraulic conductivity of a small watershed. Vadose Zone J. 2019, 12, 1–15. [Google Scholar] [CrossRef]
- Jabro, J. Estimation of saturated conductivity of solis from particle size distribution and bulk density date. Trans. Am. Soc. Agric. Eng. 1992, 35, 557–560. [Google Scholar] [CrossRef]
- Reichardt, K.; Timm, L.C. Soil, Plant and Atmosphere: Concepts, Processes and Applications; Springer: Basel, Switzerland, 2020. [Google Scholar]
- Tietje, O.; Hennings, V. Accuracy of the saturated hydraulic conductivity prediction by pedo-transfer functions compared to the variability within FAO textural classes. Geoderma 1996, 69, 71–84. [Google Scholar] [CrossRef]
- Zhang, Y.; Schaap, M.G. Estimation of saturated hydraulic conductivity with pedotransfer functions: A review. J. Hydrol. 2019, 575, 1011–1030. [Google Scholar] [CrossRef]
- Szabó, B.; Weynants, M.; Weber, T.K.D. Updated European Hydraulic Pedotransfer Functions with Communicated Uncertainties in the Predicted Variables (Euptfv2). Geosci. Model Dev. 2021, 14, 151–175. [Google Scholar] [CrossRef]
- Nasta, P.; Szabó, B.; Romano, N. Evaluation of Pedotransfer Functions for Predicting Soil Hydraulic Properties: A Voyage from Regional to Field Scales across Europe. J. Hydrol. Reg. Stud. 2021, 37, 100903. [Google Scholar] [CrossRef]
- Beniaich, A.; Otten, W.; Shin, H.-C.; Cooper, H.V.; Rickson, J.; Soulaimani, A.; El Gharous, M. Evaluation of Pedotransfer Functions to Estimate Some of Soil Hydraulic Characteristics in North Africa: A Case Study from Morocco. Front. Environ. Sci. 2023, 11, 1090688. [Google Scholar] [CrossRef]
- Patil, N.G.; Singh, S.K. Pedotransfer functions for estimating soil hydraulic properties: A review. Pedosphere 2016, 26, 417–430. [Google Scholar] [CrossRef]
- Van Looy, K.; Bouma, J.; Herbst, M.; Koestel, J.; Minasny, B.; Mishra, U.; Montzka, C.; Nemes, A.; Pachepsky, Y.A.; Padarian, J.; et al. Pedotransfer functions in Earth system science: Challenges and perspectives. Rev. Geophys. 2017, 55, 1199–1256. [Google Scholar] [CrossRef]
- Abdelbaki, A.M. Selecting the most suitable pedotransfer functions for estimating saturated hydraulic conductivity according to the available soil inputs. Ain Eng. J. 2021, 12, 2603–2615. [Google Scholar] [CrossRef]
- Haverkamp, R.; Ross, P.J.; Smettem, K.R.J.; Parlange, J.Y. Three-dimensional analysis of infiltration from the disc infiltrometer: 2. Physically based infiltration equation. Water Resour. Res. 1994, 30, 2931–2935. [Google Scholar] [CrossRef]
- Braud, I.; De Condappa, D.; Soria, J.M.; Haverkamp, R.; Angulo-Jaramillo, R.; Galle, S.; Vauclin, M. Use of scaled forms of the infiltration equation for the estimation of unsaturated soil hydraulic properties (the Beerkan method). Eur. J. Soil Sci. 2005, 56, 361–374. [Google Scholar] [CrossRef]
- Lassabatere, L.; Angulo-Jaramillo, R.; Soria Ugalde, J.M.; Cuenca, R.; Braud, I.; Haverkamp, R. Beerkan Estimation of Soil Transfer Parameters through Infiltration Experiments–BEST. Soil. Sci. Soc. Am. J. 2006, 70, 521–532. [Google Scholar] [CrossRef]
- Nemes, A.; Schaap, M.; Leij, F.; Wösten, J. Description of the unsaturated soil hydraulic database UNSODA version 2.0. J. Hydrol. 2001, 251, 151–162. [Google Scholar] [CrossRef]
- Zhang, Y.; Schaap, M.G. Weighted recalibration of the rosetta pedotransfer model with improved estimates of hydraulic parameter distributions and summary statistics (Rosetta3). J. Hydrol. 2017, 547, 39–53. [Google Scholar] [CrossRef]
- Odong, J. Evaluation of empirical formulae for determination of hydraulic conductivity based on grain-size analysis. J. Am. Sci. 2007, 3, 54–60. [Google Scholar]
- Salarashayeri, A.F.; Siosemarde, M. Prediction of Soil Hydraulic Conductivity from Particle Size Distribution Analysis. World Acad. Sci. Eng. Technol. 2012, 6, 16–20. [Google Scholar]
- Bilardi, S.; Ielo, D.; Moraci, N. Predicting the Saturated Hydraulic Conductivity of Clayey Soil sand Clayey or Silty Sands. Geosciences 2020, 10, 393. [Google Scholar] [CrossRef]
- Arya, L.M.; Paris, J.F. A physicoempirical model to predict the soil moisture characteristics from particle-size distribution and bulk density data. Soil Sci. Soc. Am. J. 1981, 45, 1023–1030. [Google Scholar] [CrossRef]
- Arya, L.M.; Leij, F.J.; Shouse, P.J.; van Genuchten, M.T. Relationship between the Hydraulic Conductivity Function and the Particle-Size Distribution. Soil Sci. Soc. Am. J. 1999, 63, 1063–1070. [Google Scholar] [CrossRef]
- You, T.; Li, S.; Guo, Y.; Wang, C.; Liu, X.; Zhao, J.; Wang, D. A superior soil–water characteristic curve for correcting the Arya–Paris model based on particle size distribution. J. Hydrol. 2022, 613, 128393. [Google Scholar] [CrossRef]
- Araya, S.N.; Ghezzehei, T.A. Using Machine Learning for Prediction of Saturated Hydraulic Conductivity and Its Sensitivity to Soil Structural Perturbations. Water Resour. Res. 2019, 55, 5715–5737. [Google Scholar] [CrossRef]
- Siltecho, S.; Hammecker, C.; Sriboonlue, V.; Clermont Dauphin, C.; Trelo-Ges, V.; Antonino, A.C.D.; Angulo-Jaramillo, R. Use of field and laboratory methods for estimating unsaturated hydraulic properties under different land uses. Hydrol. Earth Syst. Sci. 2015, 19, 1193–1207. [Google Scholar] [CrossRef]
- Weihermüller, L.; Lehmann, P.; Herbst, M.; Rahmati, M.; Verhoef, A.; Or, D.; Jacques, D.; Vereecken, H. Choice of Pedotransfer Functions Matters when Simulating Soil Water Balance Fluxes. J. Adv. Model. Earth Syst. 2021, 13, 1–30. [Google Scholar] [CrossRef]
- Šimůnek, J.; van Genuchten, M.T.; Šejna, M. Recent developments and applications of the HYDRUS computer software packages. Vadose Zone J. 2016, 15, 25. [Google Scholar] [CrossRef]
- Beck, H.; Zimmermann, N.; McVicar, T.; Vergopolan, N.; Berg, A.; Wood, E.F. Present and future Köppen-Geiger climate classification maps at 1-km resolution. Sci. Data 2018, 5, 180214. [Google Scholar] [CrossRef]
- Molénat, J.; Raclot, D.; Zitouna, R.; Andrieux, P.; Coulouma, G.; Feurer, D.; Grunberger, O.; Lamachère, J.; Bailly, J.; Belotti, J.; et al. OMERE: A Long-Term Observatory of Soil and Water Resources, in Interaction with Agricultural and Land Management in Mediterranean Hilly Catchments. Vadose Zone J. 2018, 17, 180086. [Google Scholar] [CrossRef]
- Hmaied, A. The Spatial Distribution of the Saturated Hydraulic Conductivity in the Upstream Part of the Merguellil Watershed for the Improvement of the Hydrological Modeling. Master’s Thesis, National Agronomic Institute of Tunisia (INAT), Tunis, Tunisia, 2022. [Google Scholar]
- Richards, L.A. Capillary conduction of liquids through porous media. J. Appl. Phys. 1931, 1, 318–333. [Google Scholar]
- van Genuchten, M. A closed-form equation for predicting the hydraulic conductivity of unsaturated soils. Soil Sci. Soc. Am. J. 1980, 44, 892–898. [Google Scholar] [CrossRef]
- Mualem, Y. A new model for predicting the hydraulic conductivity of unsaturated porous media. Water Resour. Res. 1976, 12, 513–522. [Google Scholar] [CrossRef]
- Burdine, N.T. Relative permeability calculation from pore size distribution data. Pet. Trans. Am Inst. Min. Metall. Eng. 1953, 198, 71–77. [Google Scholar] [CrossRef]
- Brooks, R.H.; Corey, C.T. Hydraulic properties of porous media. Hydrol. Paper 1964, 3, 1–27. [Google Scholar]
- Schaap, M.G.; Leij, F.J.; van Genuchten, M.T. Rosetta: A computer program for estimating soil hydraulic parameters with hierarchical pedotransfer functions. J. Hydrol. 2001, 251, 163–176. [Google Scholar] [CrossRef]
- Haverkamp, R.; Bouraoui, C.; Zammit, R.; Angulo-Jaramillo, R.; Delleur, J.W. Soil properties and moisture movement in the unsaturated zone. In The Handbook of Groundwater Engineering; Delleur, J.W., Ed.; CRC Press: Boca Raton, FL, USA, 1998; pp. 2931–2935. [Google Scholar]
- Fox, J.; Marquez, M.M.; Bouchet-Valat, M. Rcmdr: R Commander, R package version 2.9-1; 2023. Available online: https://socialsciences.mcmaster.ca/jfox/Misc/Rcmdr/ (accessed on 5 February 2024).
- Fang, S.; Shen, P.; Qi, X.; Zhao, F.; Gu, Y.; Huang, J.; Li, Y. The distribution of Van Genuchten model parameters on soil-water characteristic curves in Chinese Loess Plateau and new predicting method on unsaturated permeability coefficient of loess. PLoS ONE 2023, 18, e0278307. [Google Scholar] [CrossRef]
- Tóth, B.; Weynants, M.; Nemes, A.; Makó, A.; Bilas, G.; Tóth, G. New generation of hydraulic pedotransfer functions for Europe. Eur. J. Soil Sci. 2014, 66, 226–238. [Google Scholar] [CrossRef] [PubMed]



| Parameter | Methods | p-Value | |
|---|---|---|---|
| AP–B | 9.32 | 2.26 | |
| B–R | 31 | 2.58 | |
| AP–R | 20.16 | 7.12 | |
| AP–B | 20.16 | 7.12 | |
| B–R | 2.61 | 0.11 | |
| AP–R | 27.13 | 1.90 | |
| n | AP–B | 31 | 2.58 |
| n | B–R | 23.52 | 1.24 |
| n | AP–R | 2.61 | 0.11 |
| Q1 | Q2 | Q3 | Q4 | |||||
|---|---|---|---|---|---|---|---|---|
| Drainage | ||||||||
| B–R | 20.16 | 7.1 | 7.26 | 0.01 | 7.26 | 0.01 | 20.16 | 7.1 |
| B–AP | 0.29 | 0.59 | 0.29 | 0.59 | 0.032 | 0.86 | 0.29 | 0.59 |
| R–AP | 27.13 | 2 | 27.13 | 1.9 | 23.52 | 1.9 | 27.13 | 1.9 |
| Infiltration | ||||||||
| B–R | 0.2 | 0.66 | 6.4 | 0.01 | 5.44 | 0.02 | 0.2 | 0.66 |
| B–AP | 4.5 | 0.03 | 0.07 | 0.8 | 0.33 | 0.56 | 3.57 | 0.06 |
| R–AP | 4.5 | 0.03 | 11 | 9.1 | 7 | 0.01 | 7 | 8.15 |
| Runoff | ||||||||
| B–R | 0.33 | 0.56 | 0.5 | 0.48 | 0.5 | 0.48 | 0.143 | 0.705 |
| B–AP | 0 | 1 | 1 | 0.32 | 1 | 0.32 | 2 | 0.16 |
| R–AP | 0.33 | 0.56 | 0 | 1 | 0 | 1 | 0 | 1 |
| Evaporation | ||||||||
| B–R | 20.16 | 7.1 | 20.16 | 7.1 | 20.16 | 7.1 | 20.16 | 7.1 |
| B–AP | 0.29 | 0.59 | 0.29 | 0.59 | 0.29 | 0.59 | 0.29 | 0.59 |
| R–AP | 27.13 | 1.9 | 27.13 | 1.9 | 27.13 | 1.9 | 27.13 | 1.9 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hmaied, A.; Podwojewski, P.; Gharnouki, I.; Chaabane, H.; Hammecker, C. Evaluation of Soil Hydraulic Properties in Northern and Central Tunisian Soils for Improvement of Hydrological Modelling. Land 2024, 13, 385. https://doi.org/10.3390/land13030385
Hmaied A, Podwojewski P, Gharnouki I, Chaabane H, Hammecker C. Evaluation of Soil Hydraulic Properties in Northern and Central Tunisian Soils for Improvement of Hydrological Modelling. Land. 2024; 13(3):385. https://doi.org/10.3390/land13030385
Chicago/Turabian StyleHmaied, Asma, Pascal Podwojewski, Ines Gharnouki, Hanene Chaabane, and Claude Hammecker. 2024. "Evaluation of Soil Hydraulic Properties in Northern and Central Tunisian Soils for Improvement of Hydrological Modelling" Land 13, no. 3: 385. https://doi.org/10.3390/land13030385
APA StyleHmaied, A., Podwojewski, P., Gharnouki, I., Chaabane, H., & Hammecker, C. (2024). Evaluation of Soil Hydraulic Properties in Northern and Central Tunisian Soils for Improvement of Hydrological Modelling. Land, 13(3), 385. https://doi.org/10.3390/land13030385







