Influence of Anionic Surfactant on Saturated Hydraulic Conductivity of Loamy Sand and Sandy Loam Soils
Abstract
:1. Introduction
2. Material and Method
2.1. Soil Preparation
2.2. Surfactant
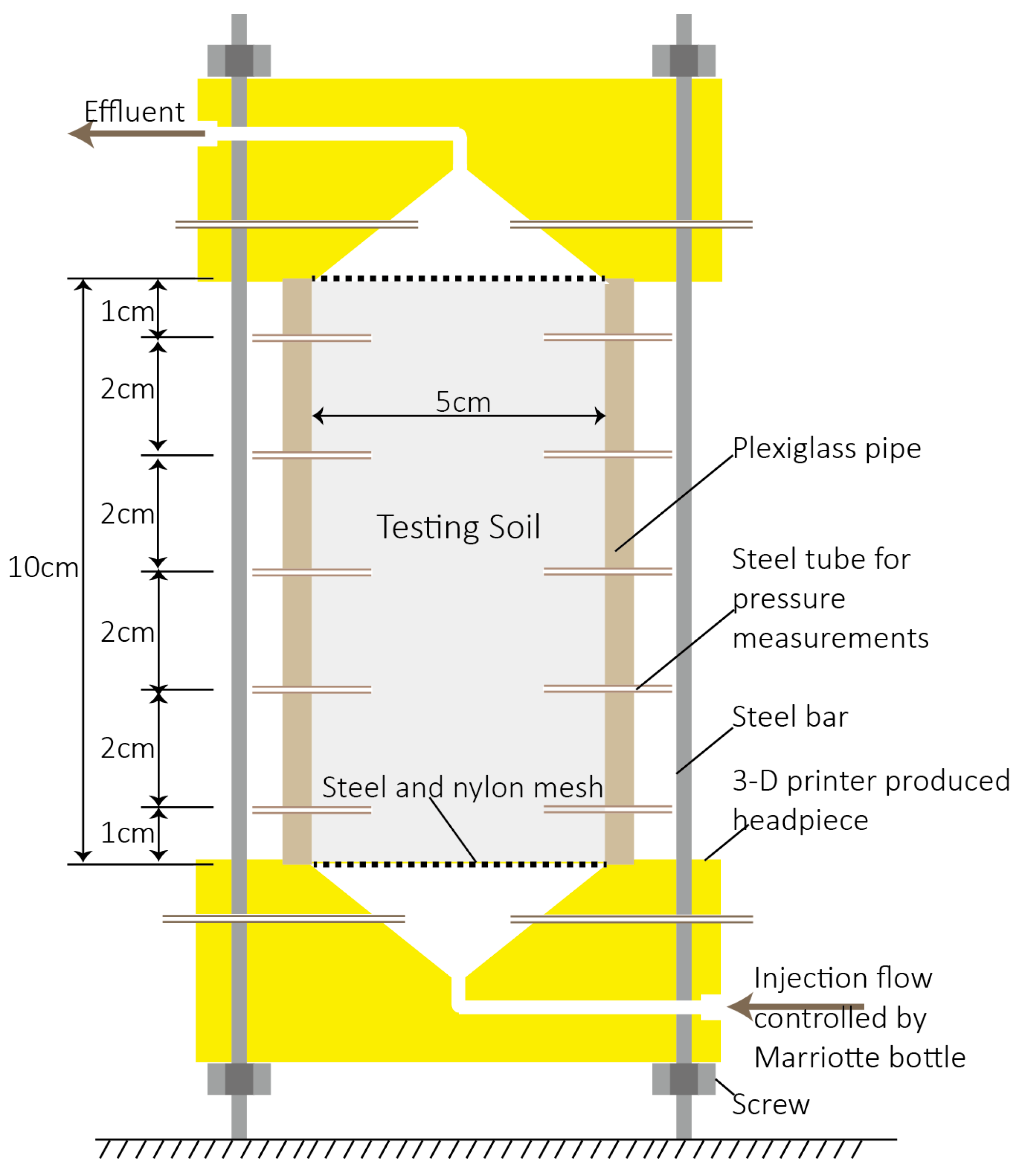
2.3. Apparatus and Procedure
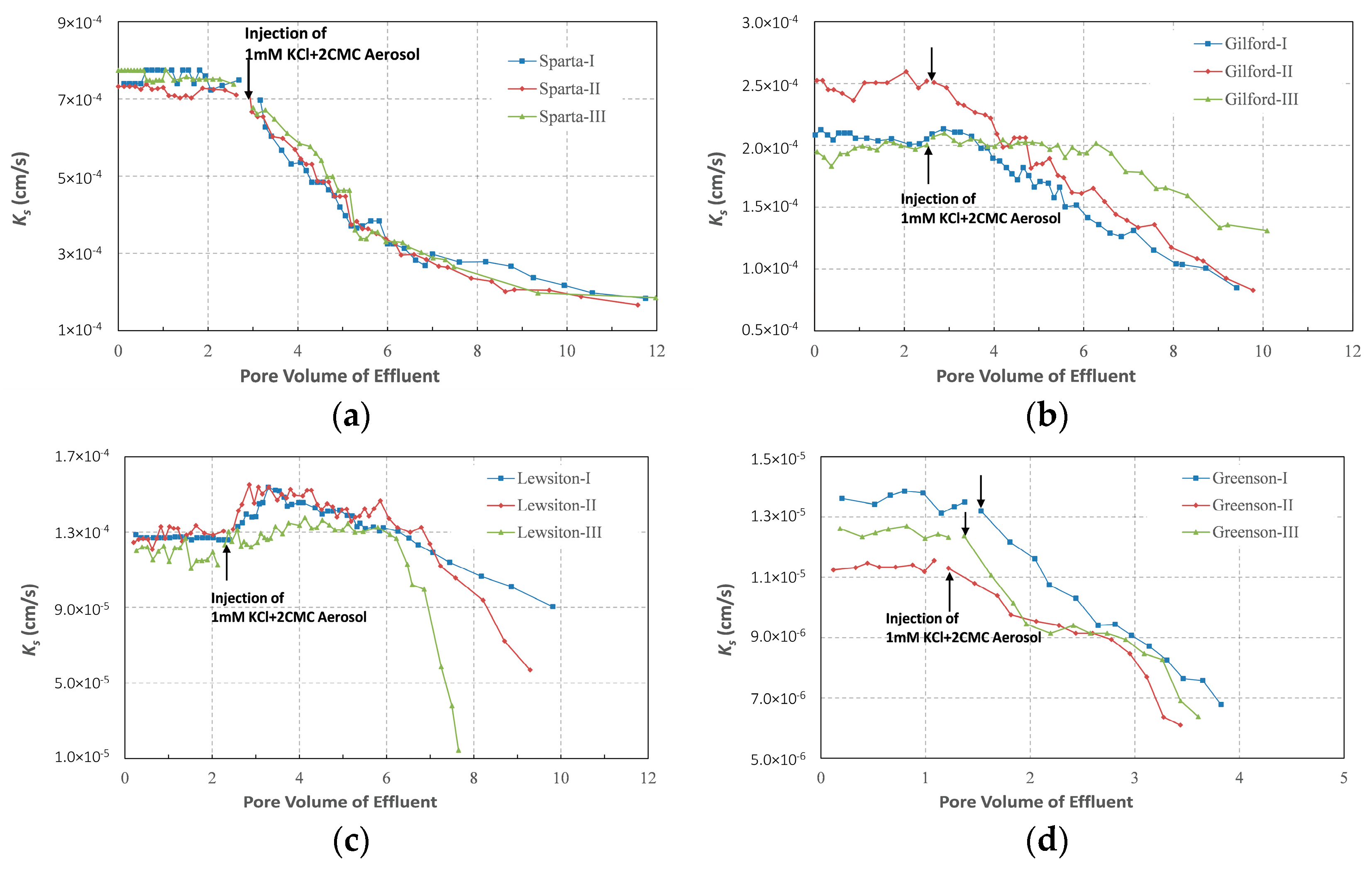
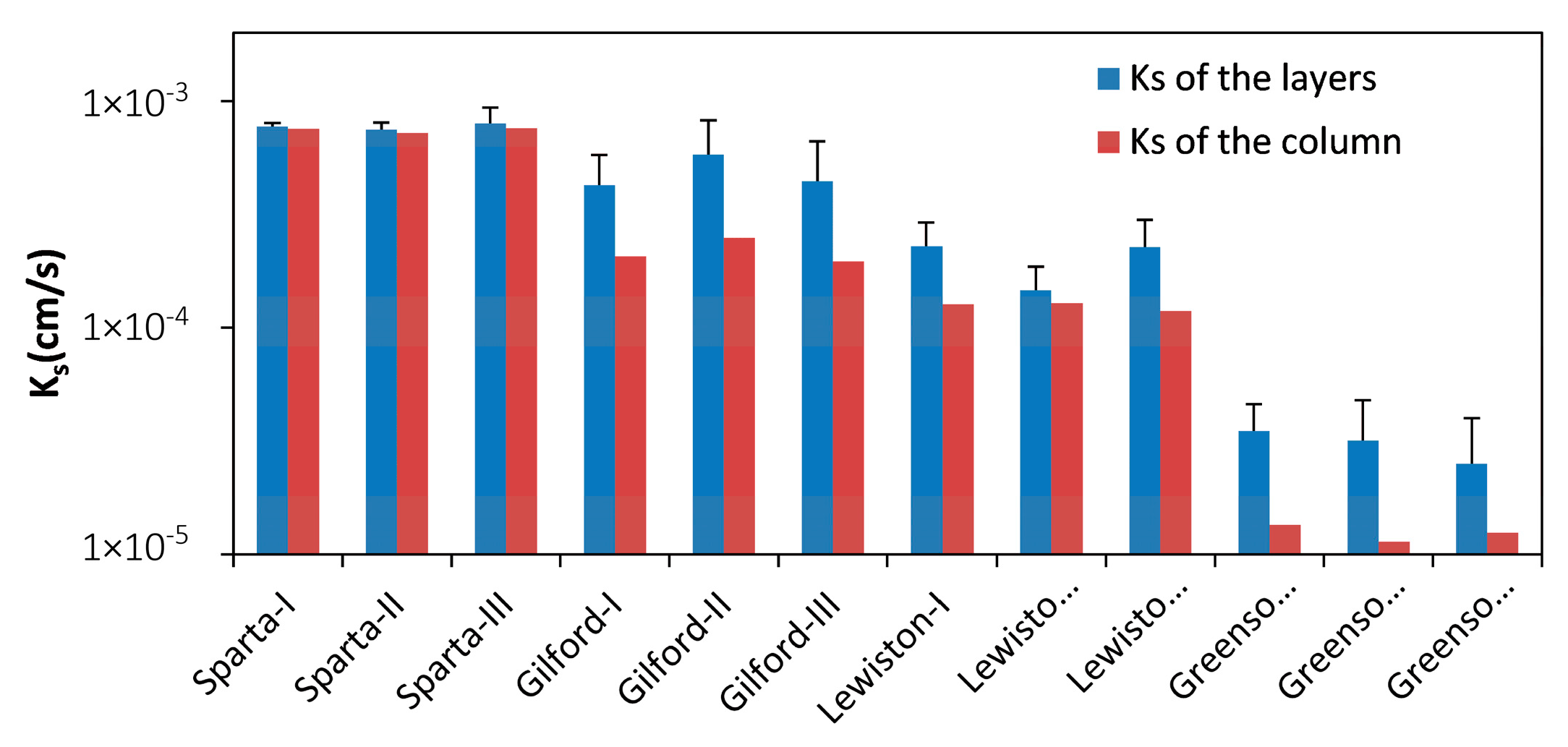
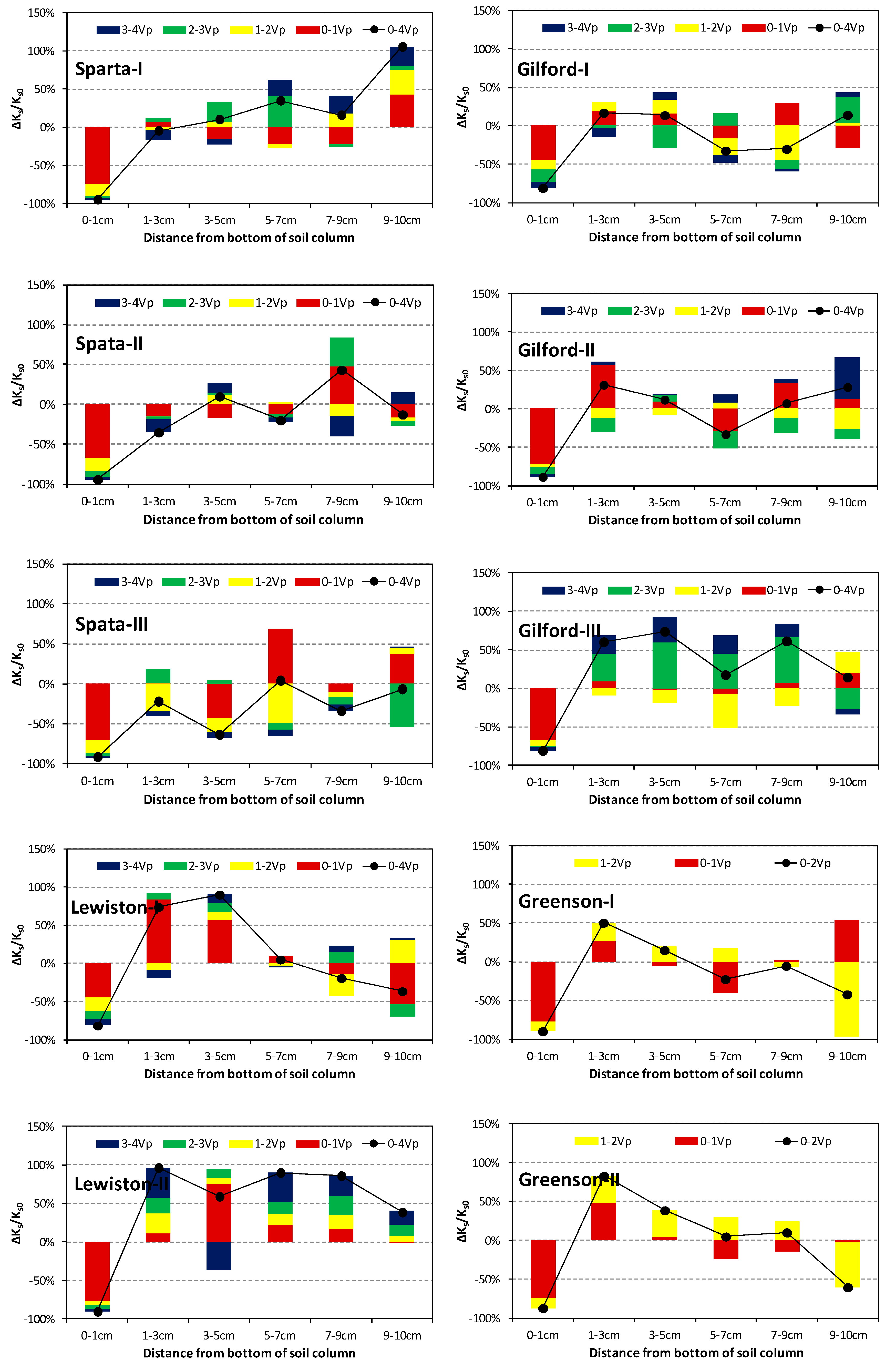
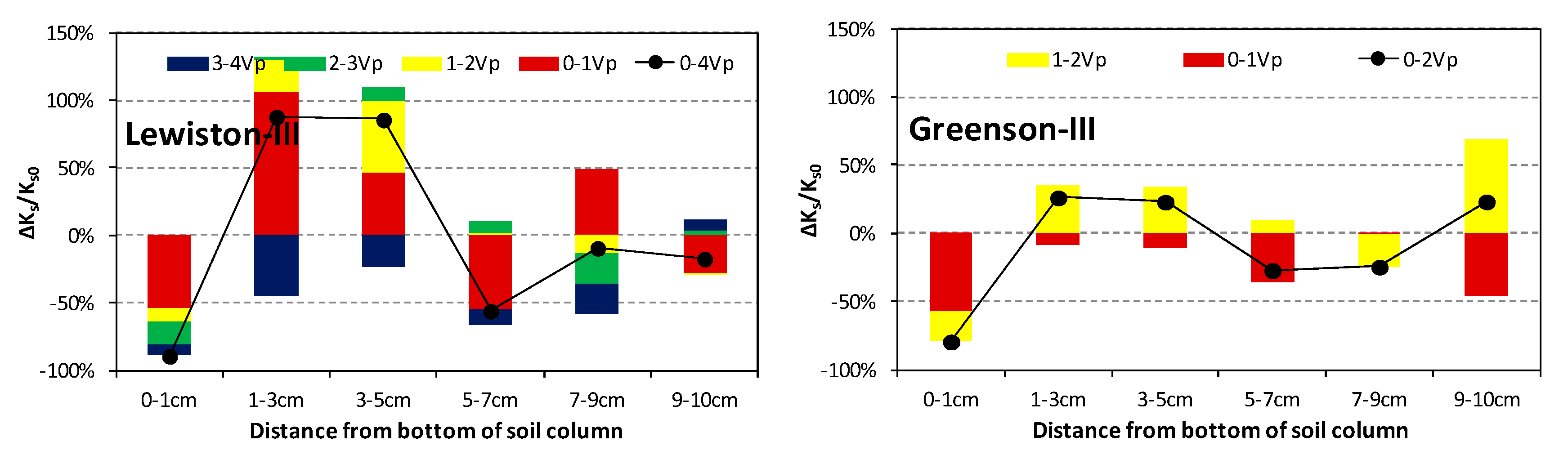
3. Results
3.1. Saturated Hydraulic Conductivities of the Columns
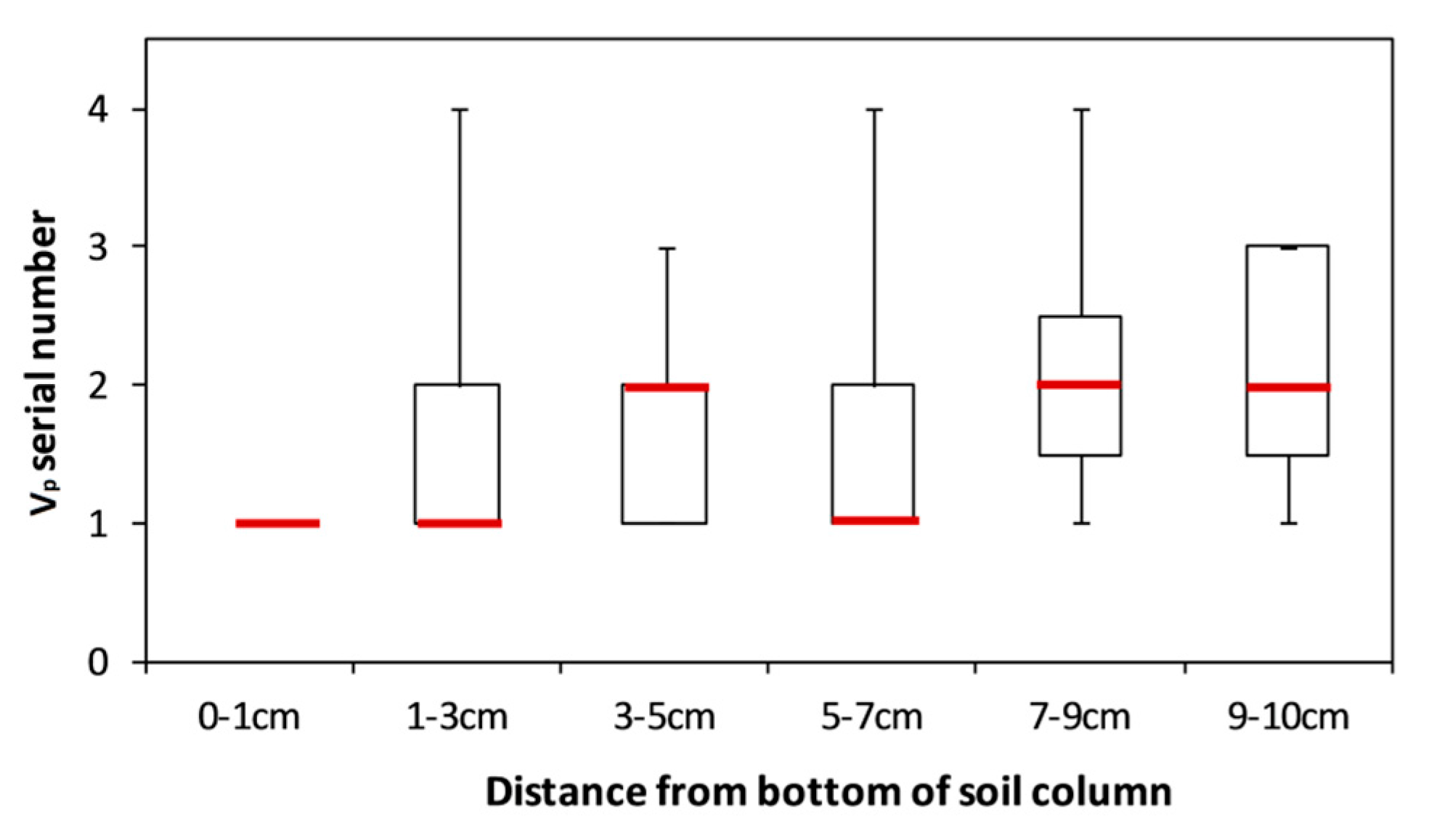
3.2. Saturated Hydraulic Conductivities of Layers
4. Discussion
5. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Al-Jayyousi, O.R. Greywater reuse: Towards sustainable water management. Desalination 2003, 156, 181–192. [Google Scholar] [CrossRef]
- Hamilton, A.J.; Stagnitti, F.; Xiong, X.; Kreidl, S.L.; Benke, K.K.; Maher, P. Wastewater irrigation: The state of play. Vadose Zone J. 2007, 6, 823–840. [Google Scholar] [CrossRef]
- Abu-Zreig, M.; Rudra, R.P.; Dickinson, W.T. Effect of application of surfactants on hydraulic properties of soils. Biosyst. Eng. 2003, 84, 363–372. [Google Scholar] [CrossRef]
- Misra, R.K.; Sivongxay, A. Reuse of laundry greywater as affected by its interaction with saturated soil. J. Hydrol. 2009, 366, 55–61. [Google Scholar] [CrossRef]
- Eriksson, E.; Auffarth, K.; Eilersen, A.M.; Henze, M.; Ledin, A. Household chemicals and personal care products as sources for xenobiotic organic compounds in grey wastewater. Water SA 2003, 29, 135–146. [Google Scholar] [CrossRef]
- Brunner, P.H.; Capri, S.; Marcomini, A.; Giger, W. Occurrence and behavior of linear alkylbenzenesulfonates, nonylphenol, nonylphenol monophenol and nonylphenol diethoxylates in sewage and sewage-sludge treatment. Water Res. 1988, 22, 1465–1472. [Google Scholar] [CrossRef]
- Berna, J.L.; Moreno, A.; Ferrer, J. The behavior of las in the environment. J. Chem. Technol. Biotechnol. 1991, 50, 387–398. [Google Scholar] [CrossRef]
- Bruno, F.; Curini, R.; Di, C.A.; Fochi, I.; Nazzari, M. Determination of surfactants and some of their metabolites in untreated and anaerobically digested sewage sludge by subcritical water extraction followed by liquid chromatography-mass spectrometry. Environ. Sci. Technol. 2002, 36, 4156–4161. [Google Scholar] [CrossRef] [PubMed]
- Cirelli, A.F.; Ojeda, C.; Castro, M.J.L.; Salgot, M. Surfactants in sludge-amended agricultural soils: A review. Environ. Chem. Lett. 2008, 6, 135–148. [Google Scholar] [CrossRef]
- Fernandez, P.; Fernandez, P.; Suter, M.J.F.; Giger, W. Determination of the quaternary ammonium surfactant ditallowdimethylammonium in digested sludges and marine sediments by supercritical fluid extraction and liquid chromatography with postcolumn ion-pair formation. Anal. Chem. 1996, 68, 921–929. [Google Scholar] [CrossRef] [PubMed]
- Chatterjee, R. Fresh produce from wastewater. Environ. Sci. Technol. 2008, 42, 7732. [Google Scholar] [CrossRef] [PubMed]
- Liwarska-Bizukojc, E.; Miksch, K.; Malachowskajutsz, A.; Kalka, J. Acute toxicity and genotoxicity of five selected anionic and nonionic surfactants. Chemosphere 2005, 58, 1249–1253. [Google Scholar] [CrossRef] [PubMed]
- Greek, B.F. Sales of detergents growing despite recession. Chem. Eng. News 1991, 69, 25. [Google Scholar] [CrossRef]
- Ying, G.G. Fate, behavior and effects of surfactants and their degradation products in the environment. Environ. Int. 2006, 32, 417–431. [Google Scholar] [CrossRef] [PubMed]
- Kuhnt, G. Behavior and fate of surfactants in soil. Environ. Toxicol. Chem. 1993, 12, 1813–1820. [Google Scholar] [CrossRef]
- Wiel-Shafran, A.; Ronen, Z.; Weisbrod, N.; Adar, E.; Gross, A. Potential changes in soil properties following irrigation with surfactant-rich greywater. Ecol. Eng. 2006, 26, 348–354. [Google Scholar] [CrossRef]
- Pinto, U.; Maheshwari, B.L.; Grewal, H.S. Effects of greywater irrigation on plant growth, water use and soil properties. Res. Conserv. Recycl. 2010, 54, 429–435. [Google Scholar] [CrossRef]
- Travis, M.J.; Wielshafran, A.; Weisbrod, N.; Adar, E.; Gross, A. Greywater reuse for irrigation: Effect on soil properties. Sci. Total Environ. 2010, 408, 2501–2508. [Google Scholar] [CrossRef] [PubMed]
- Laha, S.; Tansel, B.; Ussawarujikulchai, A. Surfactant-soil interactions during surfactant-amended remediation of contaminated soils by hydrophobic organic compounds: A review. J. Environ. Manag. 2008, 90, 95–100. [Google Scholar] [CrossRef] [PubMed]
- Liu, M.W.; Roy, D. Surfactant-induced interactions and hydraulic conductivity changes in soil. Waste Manag. 1995, 15, 463–470. [Google Scholar] [CrossRef]
- Mulligan, C.N.; Yong, R.N.; Gibbs, B.F. Surfactant-enhanced remediation of contaminated soil: A review. Eng. Geol. 2001, 60, 371–380. [Google Scholar] [CrossRef]
- Travis, M.J.; Weisbrod, N.; Gross, A. Accumulation of oil and grease in soils irrigated with greywater and their potential role in soil water repellency. Sci. Total Environ. 2008, 394, 68–74. [Google Scholar] [CrossRef] [PubMed]
- Law, J.P., Jr.; Kunze, G.W. Reaction of surfactants with montmorillonite: Adsorption mechanisms. Soil Sci. Soc. Am. Proc. 1965, 30, 321–332. [Google Scholar] [CrossRef]
- Lehrsch, G.A.; Sojka, R.E.; Reed, J.L.; Henderson, R.A.; Kostka, S.J. Surfactant and irrigation effects on wettable soils: Runoff, erosion, and water retention responses. Hydrol. Process. 2011, 25, 766–777. [Google Scholar] [CrossRef]
- Mingorance, M.D.; Gálvez, J.F.; Peña, A.; Barahona, E. Laboratory methodology to approach soil water transport in the presence of surfactants. Colloids Surf. A-Physicochem. Eng. Asp. 2007, 306, 75–82. [Google Scholar] [CrossRef]
- Eltaif, N.I.; Gharaibeh, M.A. Effects of single and mixed ion solutions on hydraulic and physical properties of a clay soil. Water Air Soil Pollut. 2007, 181, 297–302. [Google Scholar] [CrossRef]
- Abusharar, T.M.; Salameh, A.S. Reductions in hydraulic conductivity and infiltration rate in relation to aggregate stability and irrigation water turbidity. Agric. Water Manag. 1995, 29, 53–62. [Google Scholar] [CrossRef]
- Chu, W.; So, W.S. Modeling the two stages of surfactant-aided soil washing. Water Res. 2001, 35, 761–767. [Google Scholar] [CrossRef]
- Grasso, D.; Subramaniam, K.; Pignatello, J.J.; Yang, Y.; Ratté, D. Micellar desorption of polynuclear aromatic hydrocarbons from contaminated soil. Colloids Surf. A-Physicochem. Eng. Asp. 2001, 194, 65–74. [Google Scholar] [CrossRef]
- Celik, M.; Goyal, A.; Maney, E.; Somasundaran, P. Role of surfactant precipitation and redissolution in the adsorption of sulfonate on minerals. Soc. Pet. Eng. J. 1978, 24, 233–239. [Google Scholar]
- Stellner, K.L.; Scamehorn, J.F. Surfactant precipitation in aqueous-solutions containing mixtures of anionic and nonionic surfactants. J. Am. Oil Chem. Soc. 1986, 63, 566–574. [Google Scholar] [CrossRef]
- Stellner, K.L.; Scamehorn, J.F. Hardness tolerance of anionic surfactant solutions. 1. anionic surfactant with added mono-valent electrolyte. Langmuir 1989, 5, 70–77. [Google Scholar] [CrossRef]
- Nikpay, M.; Lazik, D.; Krebs, P. Water displacement by surfactant solution: An experimental study to represent wastewater loss from sewers to saturated soil. Int. J. Environ. Sci. Technol. 2015, 12, 2447–2454. [Google Scholar] [CrossRef]
- Gee, G.W.; Bauder, J.W. Particle size analysis. In Methods of Soil Analysis, Part 1. Physical and Mineralogical Methods; Klute, A., Ed.; American Society of Agronomy and Soil Science Societry of America: Madison, WI, USA, 1986; pp. 383–411. [Google Scholar]
- Rhoades, J.D. Salinity: Electrical conductivity and total dissolved solids. In Methods of Soil Analysis. Part 3 Chemical Methods; Sparks, D.L., Ed.; Anmerican Society of Agronomy and Soil Science Society of America: Madison, WI, USA, 1996; pp. 417–435. [Google Scholar]
- Rangel, D.P.; Superak, C.; Bielschowsky, M.; Farris, K.; Falconer, R.E.; Baveye, P.C. Rapid Prototyping and 3-D Printing of Experimental Equipment in Soil Science Research. Soil Sci. Soc. Am. J. 2013, 77, 54–59. [Google Scholar] [CrossRef]
- Gardner, K.H.; Arias, M.S. Clay Swelling and Formation Permeability Reductions Induced by a Nonionic Surfactant. Environ. Sci. Technol. 2000, 34, 160–166. [Google Scholar] [CrossRef]
- Baveye, P.; Vandevivere, P.; Hoyle, B.L.; Deleo, P.C.; de Lozasa, D.S. Environmental impact and mechanisms of the biological clogging of saturated soils and aquifer materials. Crit. Rev. Environ. Sci. Technol. 1998, 28, 123–191. [Google Scholar] [CrossRef]
- Hallett, P.D.; Young, I.M. Changes to water repellence of soil aggregates caused by substrate-induced microbial activity. Eur. J. Soil Sci. 1999, 50, 35–40. [Google Scholar] [CrossRef]
- Vandevivere, P.; Baveye, P. Saturated hydraulic conductivity reduction caused by aerobic bacteria in sand columns. Soil Sci. Soc. Am. J. 1992, 56, 1–13. [Google Scholar] [CrossRef]
- Faybishenko, B.A. Hydraulic Behavior of Quasi-Saturated Soils in the Presence of Entrapped Air: Laboratory Investigations. Water Resour. Res. 1995, 31, 2421–2435. [Google Scholar] [CrossRef]
- Rauch, W.; Stegner, T. The colmation of leaks in sewer systems during dry weather flow. Water Sci. Technol. 1994, 30, 205–210. [Google Scholar]
- Vollertsen, J.; Hvitved-Jacobsen, T. Exfiltration from gravity sewers: A pilot scale study. Water Sci. Technol. 2003, 47, 69–76. [Google Scholar] [PubMed]
- Blackwood, D.J.; Gilmour, D.J.; Ellis, J.B.; Revitt, D.M.; Stainer, A. Exfiltration from sewers; is it a serious problem? In Proceedings of the 10th International Conference on Urban Drainage, Copenhagen, Denmark, 21–26 August 2005. [Google Scholar]
- Rutscha, M.; Rieckermannb, J.; Cullmannc, J.; Ellisd, J.B.; Vollertsene, J.; Krebsa, P. Towards a better understanding of sewer exfiltration. Water Res. 2008, 42, 2385–2394. [Google Scholar] [CrossRef] [PubMed]
- Nikpay, M.; Lazik, D.; Krebs, P. Permeability changes by surfactant solution: An experimental study to represent wastewater loss from sewers to saturated soil. Environ. Earth Sci. 2015, 73, 8443–8450. [Google Scholar] [CrossRef]
- Giles, C.H.; Macewan, T.; Nakhwa, S.; Smith, D. Studies in adsorption. Part XI. A system of classification of solution adsorption isotherms, and its use in diagnosis of adsorption mechanisms and in measurement of specific surface areas of solids. J. Chem. Soc. 1960, 786, 3973–3993. [Google Scholar] [CrossRef]

| Properties | Soil Series * | ||||
|---|---|---|---|---|---|
| Sparta | Gilford | Lewiston | Greenson | ||
| Composition and texture | Sand (%) | 82 | 84.4 | 79.7 | 66.9 |
| Silt (%) | 8.4 | 7.7 | 7.7 | 13.6 | |
| Clay (%) | 9.5 | 7.9 | 12.5 | 19.5 | |
| Organic matter (%) | 3.4 | 4.2 | - ** | 3.8 | |
| USDA texture | Loamy sand | Loamy sand | Sandy loam | Sandy loam | |
| Chemical properties | pH | 6.9 | 5.2 | 7.5 | 7.4 |
| EC(dS/m) | 0.36 | 0.68 | 1.01 | 0.75 | |
| CEC (meq/100g) | 8.6 | 8.4 | 11.5 | 17.5 | |
| Sodium (mg/kg) | 3.27 | 1.87 | 11.29 | 13.55 | |
| Magnesium (mg/kg) | 4.84 | 5.71 | 14.91 | 12 | |
| Calcium (mg/kg) | 9.36 | 14.64 | 37.1 | 42.9 | |
| SAR | 0.22 | 0.10 | 0.39 | 0.47 | |
| Test | Specification | Molecule Formula |
|---|---|---|
| Appearance | Liquid |  |
| Molar weight (g) | 742 | |
| CMC (%/wt) | 0.04 | |
| Ca tolerance (ppm, CaCO3 at 0.5%) | 200 | |
| pH (As is) | 7.0–8.0 | |
| Surface tension (min·mN/m) | 36 |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Peng, Z.; Darnault, C.J.G.; Tian, F.; Baveye, P.C.; Hu, H. Influence of Anionic Surfactant on Saturated Hydraulic Conductivity of Loamy Sand and Sandy Loam Soils. Water 2017, 9, 433. https://doi.org/10.3390/w9060433
Peng Z, Darnault CJG, Tian F, Baveye PC, Hu H. Influence of Anionic Surfactant on Saturated Hydraulic Conductivity of Loamy Sand and Sandy Loam Soils. Water. 2017; 9(6):433. https://doi.org/10.3390/w9060433
Chicago/Turabian StylePeng, Zhenyang, Christophe J. G. Darnault, Fuqiang Tian, Philippe C. Baveye, and Hongchang Hu. 2017. "Influence of Anionic Surfactant on Saturated Hydraulic Conductivity of Loamy Sand and Sandy Loam Soils" Water 9, no. 6: 433. https://doi.org/10.3390/w9060433
APA StylePeng, Z., Darnault, C. J. G., Tian, F., Baveye, P. C., & Hu, H. (2017). Influence of Anionic Surfactant on Saturated Hydraulic Conductivity of Loamy Sand and Sandy Loam Soils. Water, 9(6), 433. https://doi.org/10.3390/w9060433





