Variability of Darcian Flux in the Hyporheic Zone at a Natural Channel Bend
Abstract
:1. Introduction
2. Study Site and Methods
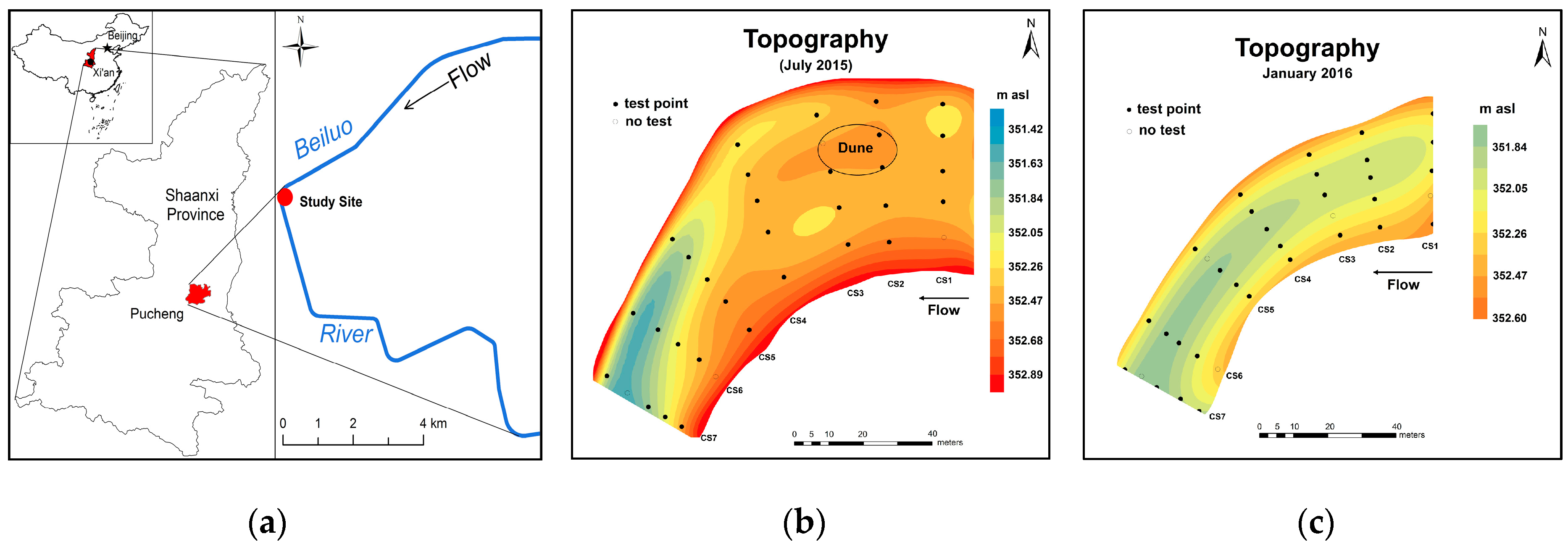
2.1. Study Site
2.2. Study Methods
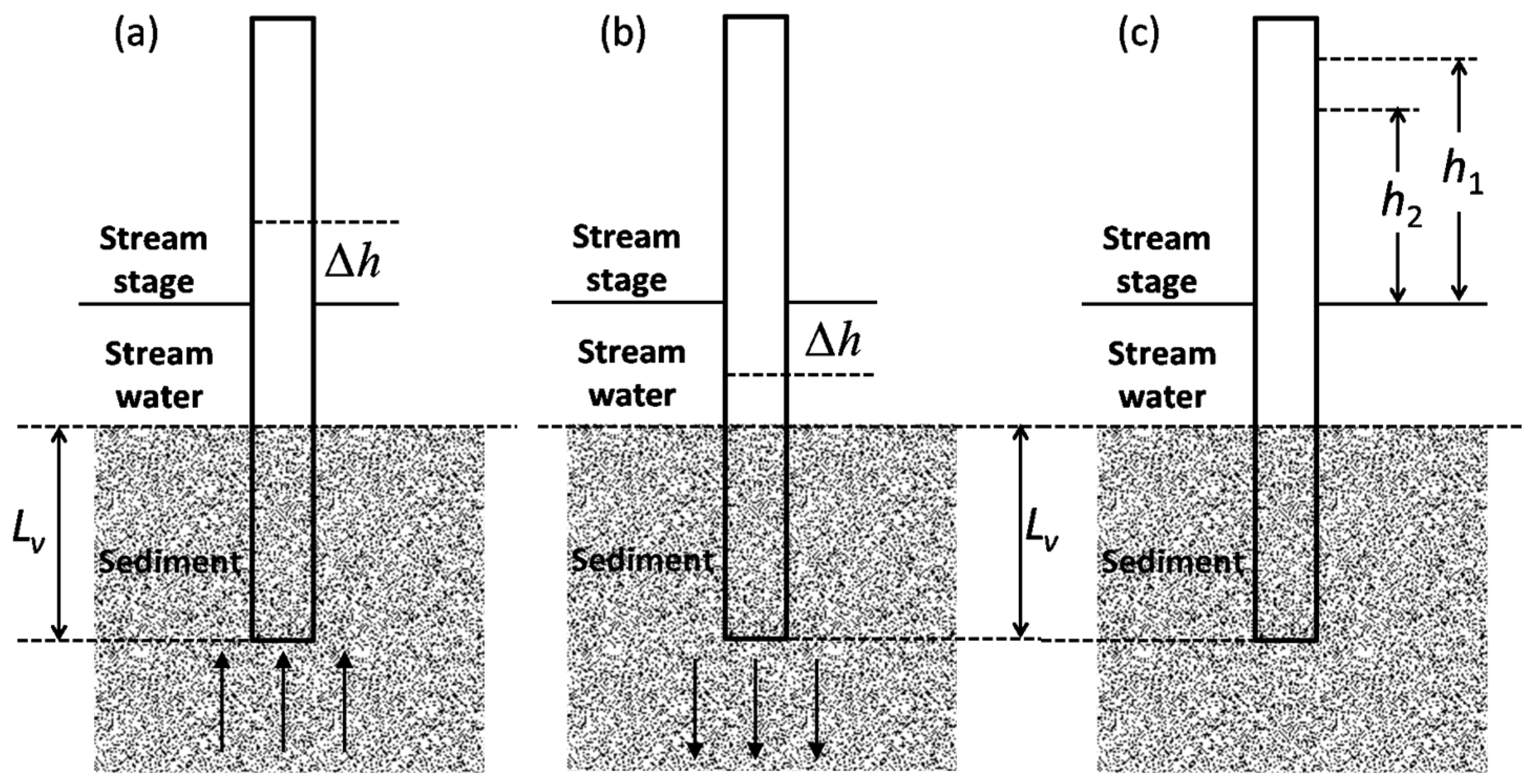
2.2.1. Determination of Vertical Head Gradient
2.2.2. Determination of Vertical Hydraulic Conductivity
2.2.3. Determination of Vertical Darcian Flux
2.2.4. Estimation of Porosity of Streambed Sediments
3. Results
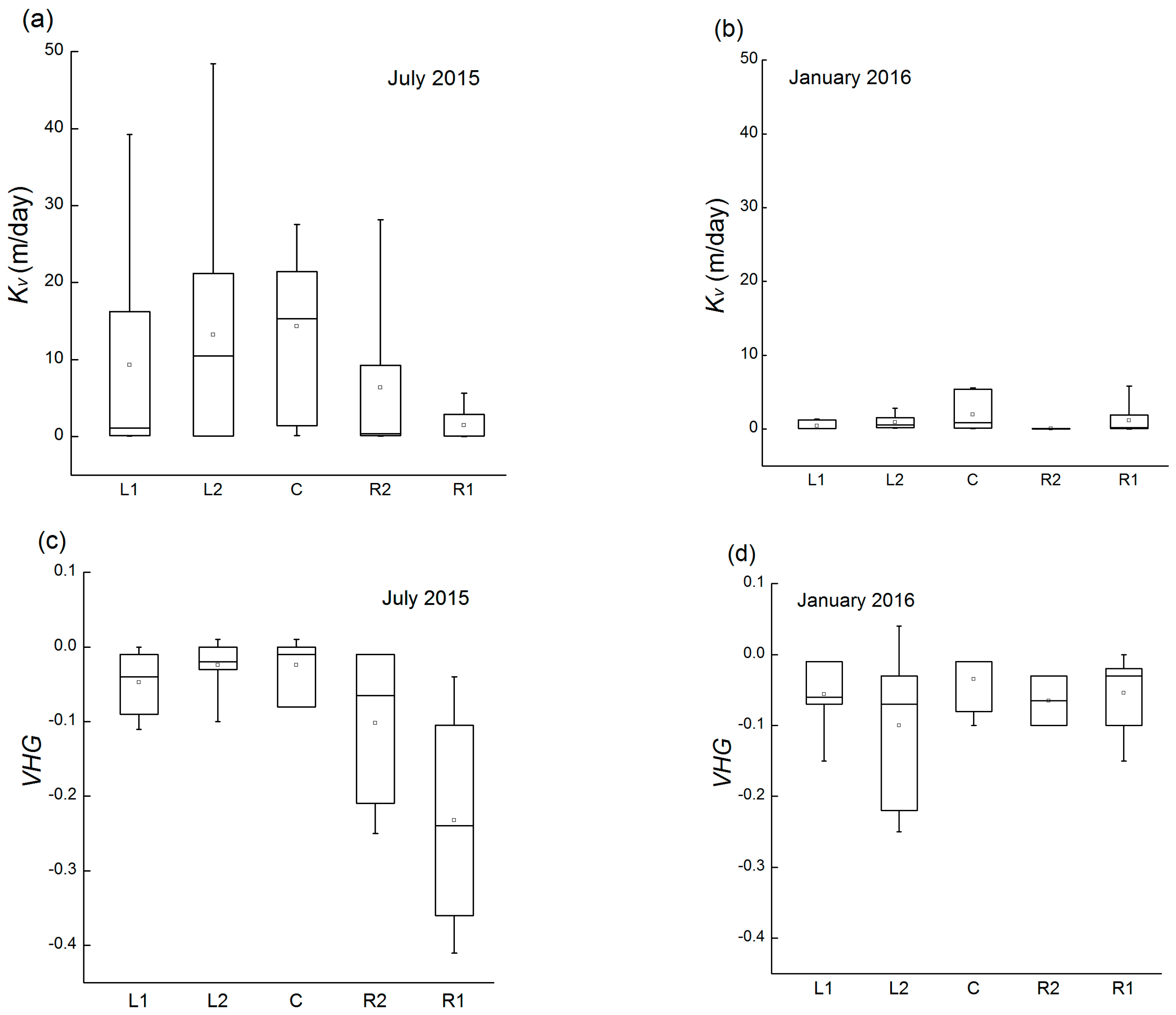
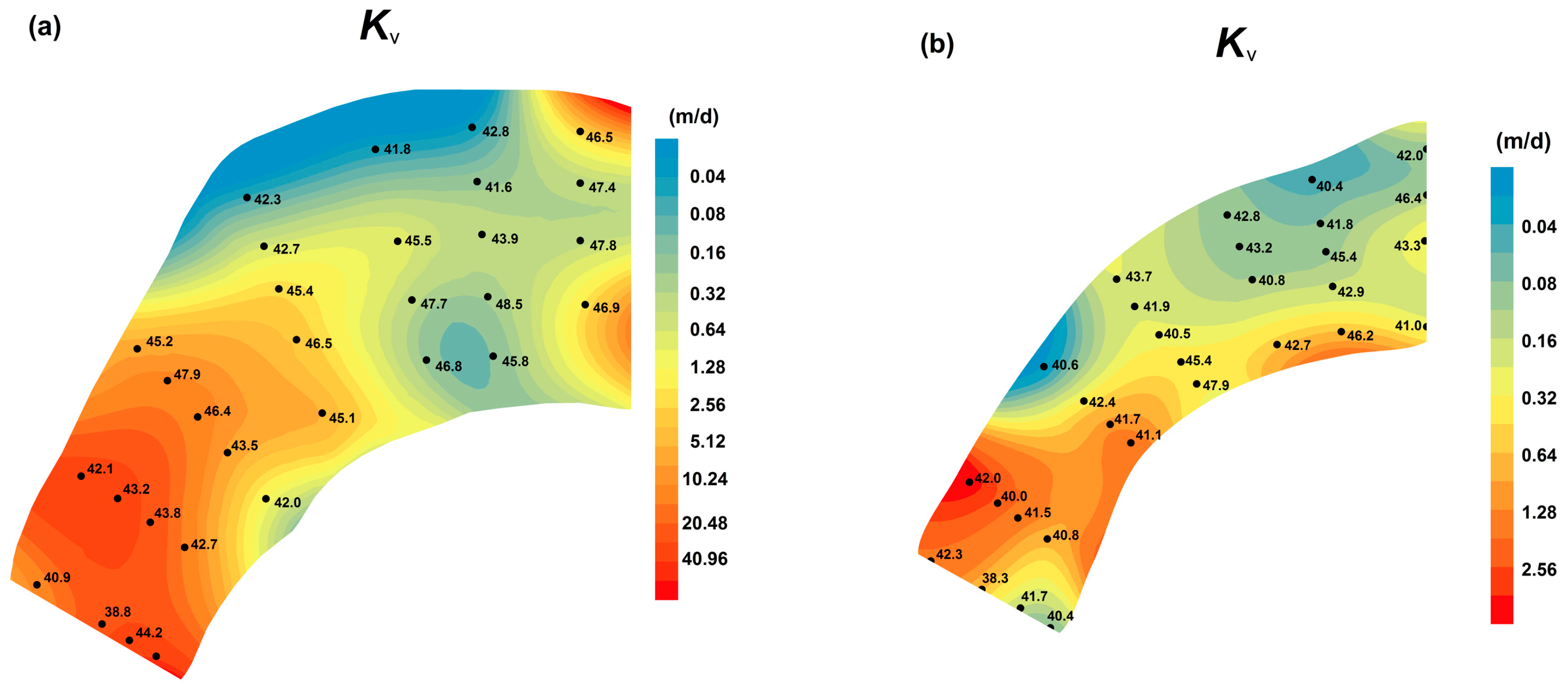
3.1. Vertical Hydraulic Conductivity
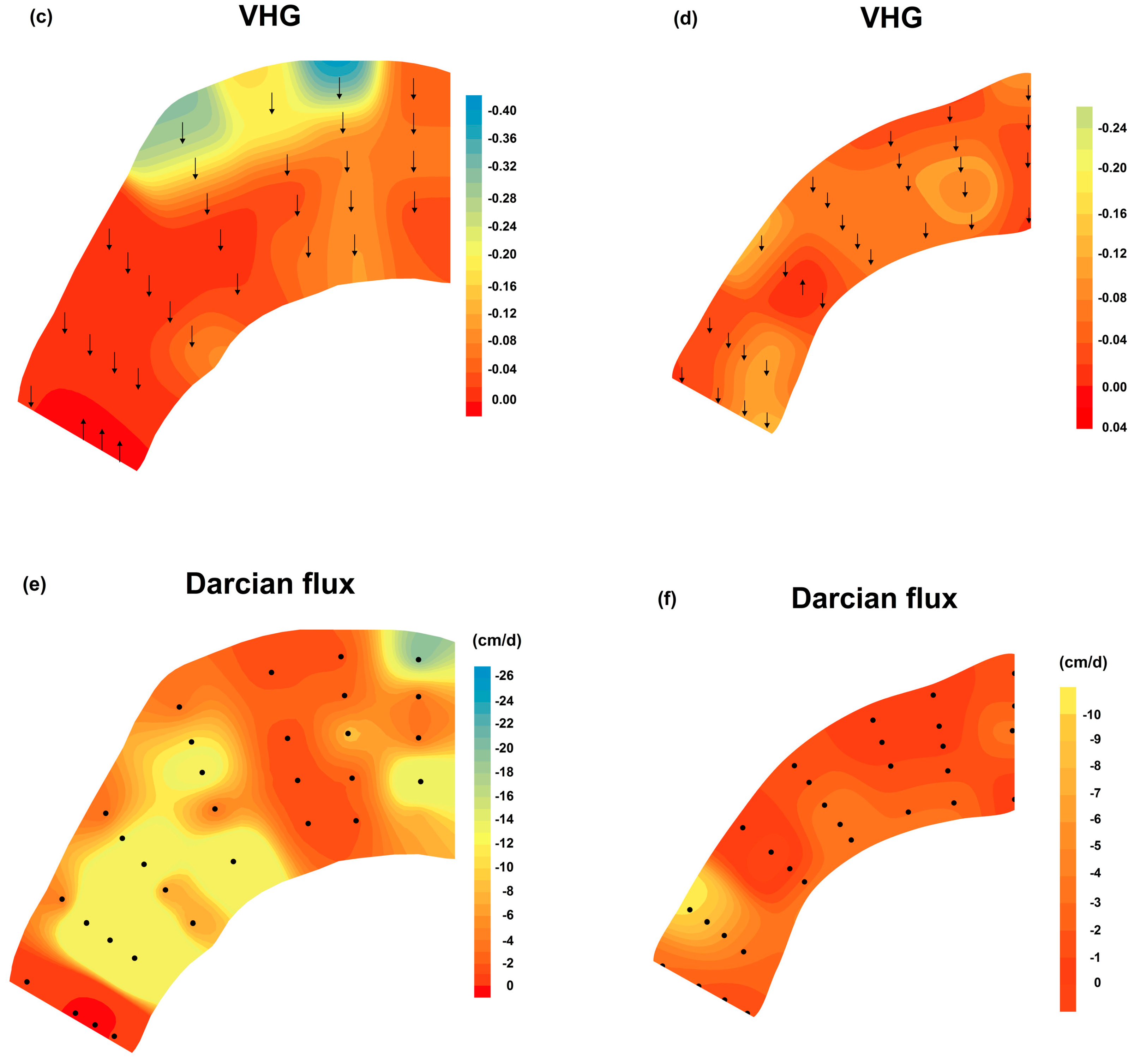
3.2. Vertical Head Gradient
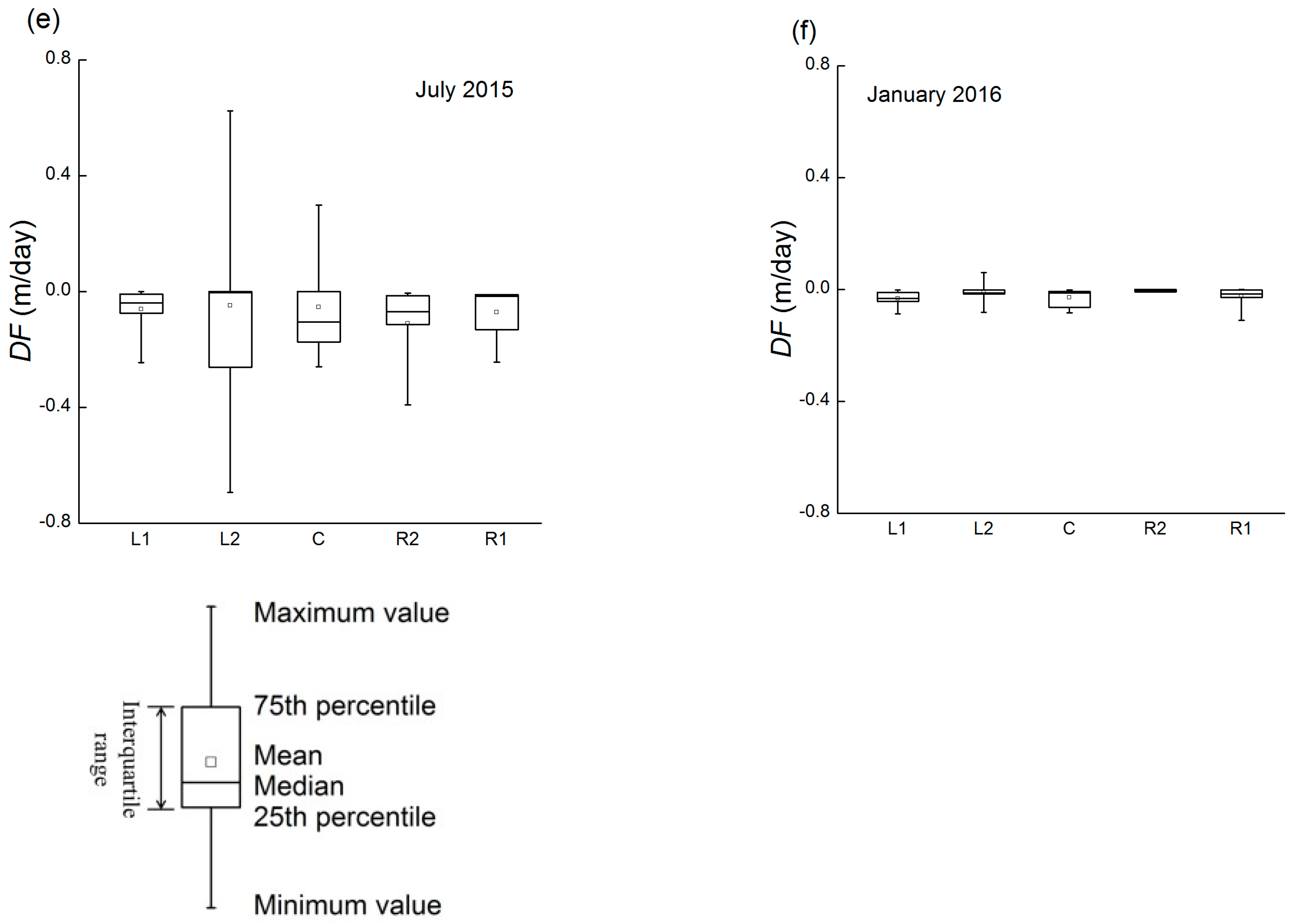
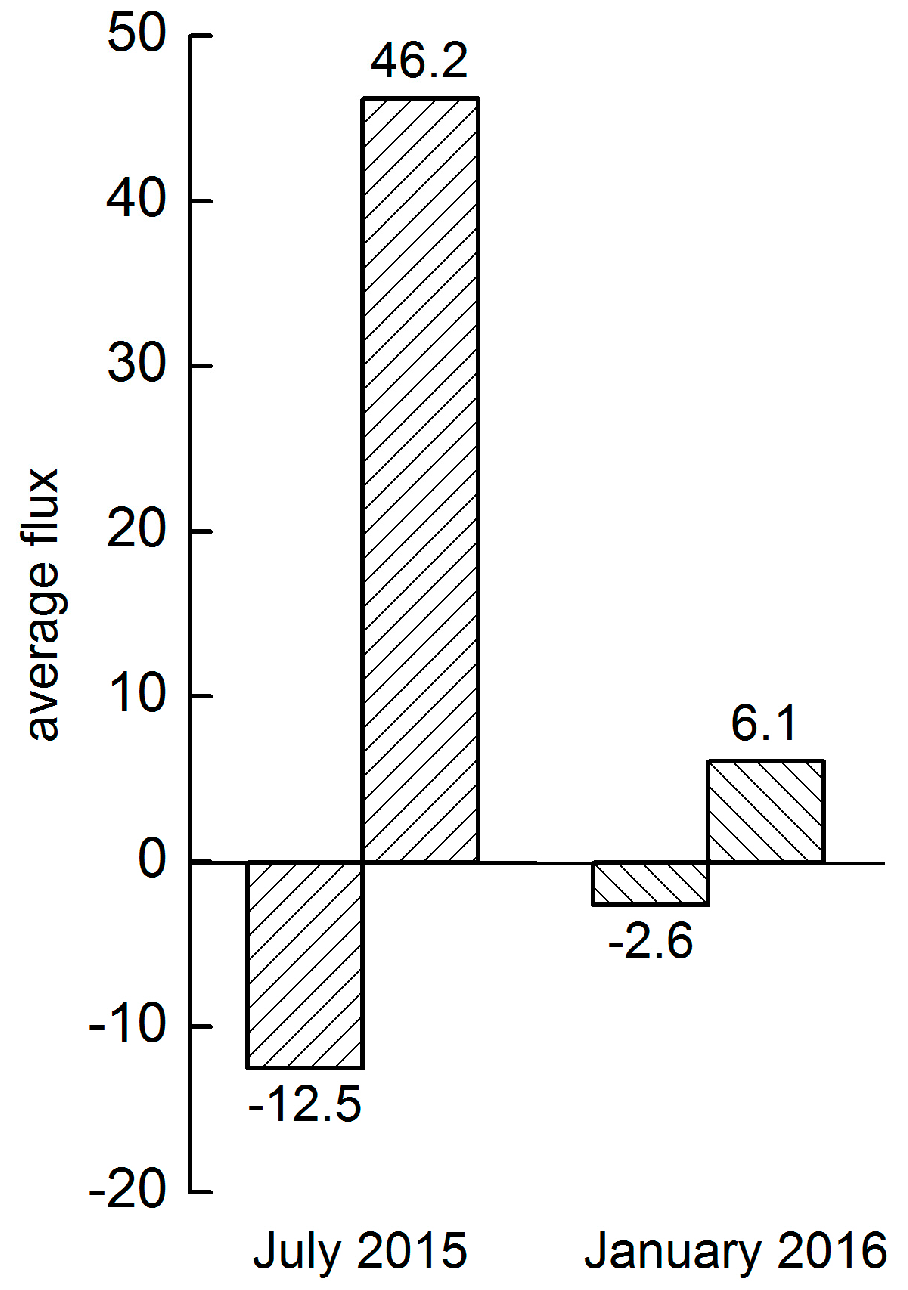
3.3. Vertical Darcian flux
3.4. Sediment Grain Size
4. Discussion
4.1. Porosity
4.2. Stream Topography
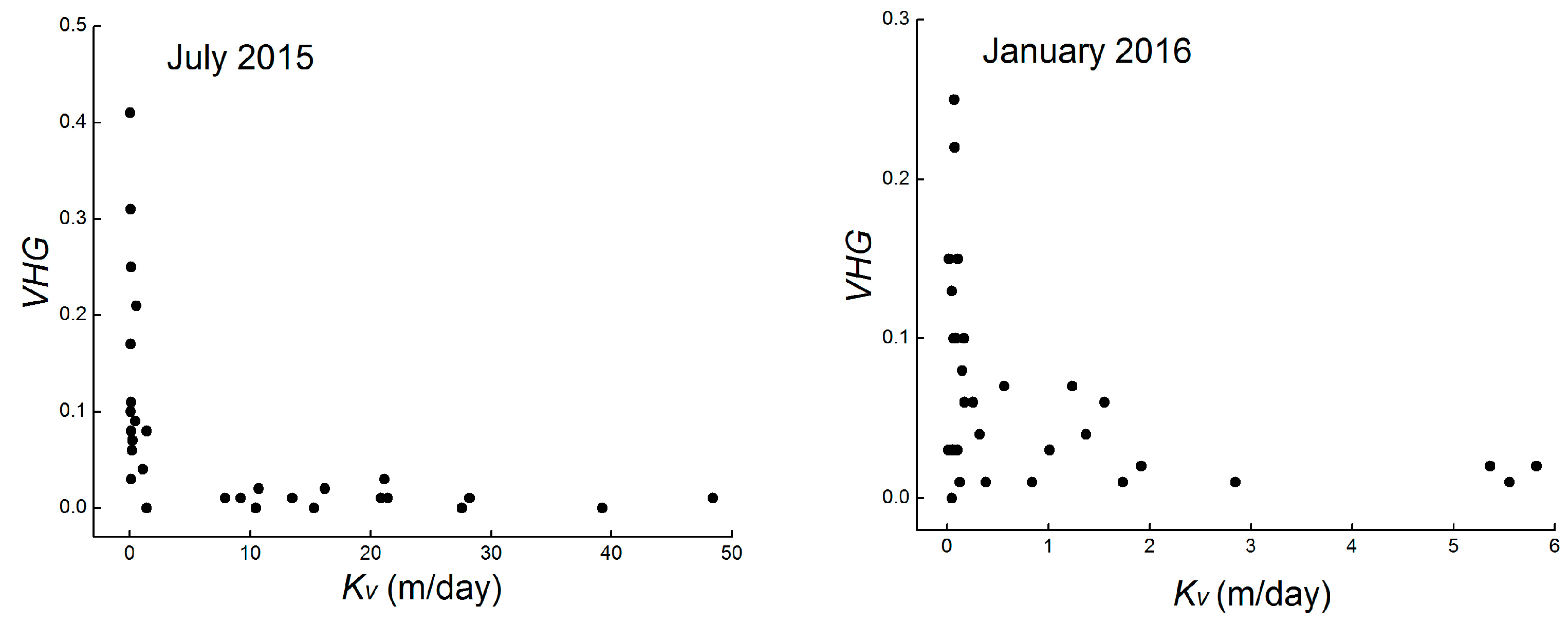
4.3. Correlation of Measured Streambed Attributes
5. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Hancock, P.J. Human impacts on the stream-groundwater exchange zone. Environ. Manag. 2002, 29, 763–781. [Google Scholar] [CrossRef] [PubMed]
- Boulton, A.J.; Findlay, S.; Marmonier, P.; Stanley, E.H.; Valett, H.M. The functional significance of the hyporheic zone in streams and rivers. Annu. Rev. Ecol. Syst. 1998, 29, 59–81. [Google Scholar] [CrossRef]
- Hancock, P.J.; Boulton, A.J.; Humphreys, W.F. Aquifers and hyporheic zones: Towards an ecological understanding of groundwater. Hydrogeol. J. 2005, 13, 98–111. [Google Scholar] [CrossRef]
- Tonina, D.; Marzadri, A.; Bellin, A. Benthic uptake rate due to hyporheic exchange: The effects of streambed morphology for constant and sinusoidally varying nutrient loads. Water 2015, 7, 398–419. [Google Scholar] [CrossRef]
- Sophocleous, M. Interactions between groundwater and surface water: The state of the science. Hydrol. J. 2002, 10, 52–67. [Google Scholar]
- Stonedahl, S.H.; Harvey, J.W.; Packman, A.I. Interactions between hyporheic flow produced by stream meanders, bars, and dunes. Water Resour. Res. 2013, 49, 5450–5461. [Google Scholar] [CrossRef]
- Hassan, M.A.; Tonina, D.; Beckie, R.D.; Kinnear, M. The effects of discharge and slope on hyporheic flow in step-pool morphologies. Hydrol. Process. 2015, 29, 419–433. [Google Scholar] [CrossRef]
- Hester, E.T.; Doyle, M.W. In-stream geomorphic structures as drivers of hyporheic exchange. Water Resour. Res. 2008, 44, 893–897. [Google Scholar] [CrossRef]
- Allen, D.J.; Darling, W.G.; Gooddy, D.C.; Lapworth, D.J.; Newell, A.J.; Williams, A.T. Interaction between groundwater, the hyporheic zone and a Chalk stream: A case study from the River Lambourn, UK. Hydrol. J. 2010, 18, 1125–1141. [Google Scholar]
- Cardenas, M.B.; Wilson, J.L. Exchange across a sediment–water interface with ambient groundwater discharge. J. Hydrol. 2007, 346, 69–80. [Google Scholar] [CrossRef]
- Cardenas, M.B. Stream-aquifer interactions and hyporheic exchange in gaining and losing sinuous streams. Water Resour. Res. 2009, 45, 267–272. [Google Scholar] [CrossRef]
- Cardenas, M.B. A model for lateral hyporheic flow based on valley slope and channel sinuosity. Water Resour. Res. 2009, 45, 206–216. [Google Scholar] [CrossRef]
- Kalbus, E.; Reinstorf, F.; Schirmer, M. Measuring methods for groundwater-surface water interactions: A review. Hydrol. Earth Syst. 2006, 10, 873–887. [Google Scholar] [CrossRef]
- Jiang, W.W.; Song, J.X.; Zhang, J.L.; Wang, Y.Y.; Zhang, N.; Zhang, X.H.; Long, Y.Q.; Li, J.X.; Yang, X.G. Spatial variability of streambed vertical hydraulic conductivity and its relation to distinctive stream morphologies in the Beiluo River, Shaanxi Province, China. Hydrol. J. 2015, 23, 1617–1626. [Google Scholar] [CrossRef]
- Min, L.L.; Yu, J.J.; Liu, C.M.; Zhu, J.T.; Wang, P. The spatial variability of streambed vertical hydraulic conductivity in an intermittent river, northwestern China. Environ. Earth. Sci. 2013, 69, 873–883. [Google Scholar] [CrossRef]
- Packman, A.I.; Salehin, M. Relative roles of stream flow and sedimentary conditions in controlling hyporheic exchange. Hydrobiologia 2003, 494, 291–297. [Google Scholar] [CrossRef]
- MacDonald, M.J.; Chu, C.F.; Guilloit, P.P.; Ng, K.M. A generalized Blake-Kozeny equation for multisized spherical particles. AIChE J. 1991, 37, 1583–1588. [Google Scholar] [CrossRef]
- Edwardson, K.J.; Bowden, W.B.; Dahm, C.; Morrice, J. The hydraulic characteristics and geochemistry of hyporheic and parafluvial zones in Arctic tundra streams, North Slope, Alaska. Adv. Water Resour. 2003, 26, 907–923. [Google Scholar] [CrossRef]
- Chen, X.H.; Chen, X. Stream water infiltration, bank storage, and storage zone changes due to stream-stage fluctuations. J. Hydrol. 2003, 280, 246–264. [Google Scholar] [CrossRef]
- Chen, X.H.; Song, J.X.; Cheng, C.; Wang, D.M.; Lackey, S.O. A new method for mapping variability in vertical seepage flux in streambeds. Hydrol. J. 2009, 17, 519–525. [Google Scholar] [CrossRef]
- Storey, R.G.; Howard, K.W.F.; Williams, D.D. Factors controlling riffle-scale hyporheic exchange flows and their seasonal changes in a gaining stream: A three-dimensional groundwater flow model. Water Resour. Res. 2003, 39, 180–189. [Google Scholar] [CrossRef]
- Ran, D.C. Water and sediment variation and ecological protection measures in the middle reach of the Yellow River. Resour. Sci. 2006, 28, 93–100. [Google Scholar]
- Käser, D.H.; Binley, A.; Heathwaite, A.L.; Krause, S. Spatio-temporal variations of hyporheic flow in a riffle-step-pool sequence. Hydrol. Process. 2009, 23, 2138–2149. [Google Scholar] [CrossRef]
- Genereux, D.P.; Leahy, S.; Mitasova, H.; Kennedy, C.D.; Corbett, D.R. Spatial and temporal variability of streambed hydraulic conductivity in West Bear Creek, North Carolina, USA. J. Hydrol. 2008, 358, 332–353. [Google Scholar] [CrossRef]
- Hvorslev, M.J. Time Lag and Soil Permeability in Ground-Water Observations; U.S. Army Bulletin: Vicksburg, MS, USA, 1951. [Google Scholar]
- Chen, X. Streambed hydraulic conductivity for rivers in south-central Nebraska. J. Am. Water Resour. Assoc. 2004, 40, 561–573. [Google Scholar] [CrossRef]
- Chen, X. Statistical and geostatistical features of streambed hydraulic conductivities in the Platte River, Nebraska. Environ. Geol. 2005, 48, 693–701. [Google Scholar] [CrossRef]
- Omojola, A.D.; Akinpelu, S.J.; Adesegun, A.M.; Akinyemi, O.D. A Micro Study to Determine Porosity, Hydraulic Conductivity, Permeability and the Discharge Rate of Groundwater in Ondo State Riverbeds, Southwestern Nigeria. Int. J. Geosci. 2014, 5, 1254–1262. [Google Scholar] [CrossRef]
- Stephens, D.B.; Hsu, K.C.; Prieksat, M.A.; Ankeny, M.D.; Blandford, N.; Roth, T.L.; Kesley, J.A.; Whitworth, J.R. A comparison of estimated and calculated effective porosity. Hydrol. J. 1998, 6, 156–165. [Google Scholar] [CrossRef]
- Datry, T.; Lamouroux, N.; Thivin, G.; Descloux, S.; Baudoin, J.M. Estimation of sediment hydraulic conductivity in river reaches and its potential use to evaluate streambed clogging. River Res. Appl. 2015, 31, 880–891. [Google Scholar] [CrossRef]
- Sebok, E.; Duque, C.; Engesgaard, P.; Boegh, E. Spatial variability in streambed hydraulic conductivity of contrasting stream morphologies: Channel bend and straight channel. Hydrol. Process. 2015, 29, 458–472. [Google Scholar] [CrossRef]
- Mugnai, R.; Sattamini, A.; Ja, A.D.S.; Reguamangia, A.H. A survey of Escherichia coli and Salmonella in the Hyporheic Zone of a subtropical stream: Their bacteriological, physicochemical and environmental relationships. PLoS ONE 2015, 10, 883. [Google Scholar] [CrossRef] [PubMed]
- Gamage, K.; Screaton, E.; Bekins, B.; Aiello, I. Permeability-porosity relationships of subduction zone sediments. Mar. Geol. 2011, 279, 19–36. [Google Scholar] [CrossRef]
- Sperry, J.M.; Peirce, J.J. A model for estimating the hydraulic conductivity of granular material based on grain shape, grain size, and porosity. Groundwater 1995, 33, 892–898. [Google Scholar] [CrossRef]
- Dong, W.H.; Chen, X.H.; Wang, Z.W.; Ou, G.X.; Liu, C. Comparison of vertical hydraulic conductivity in a streambed-point bar system of a gaining stream. J. Hydrol. 2012, 450–451, 9–16. [Google Scholar] [CrossRef]
- Miller, R.B.; Heeren, D.M.; Fox, G.A.; Halihan, T.; Storm, D.E.; Mittelstet, A.R. The hydraulic conductivity structure of gravel-dominated vadose zones within alluvial floodplains. J. Hydrol. 2014, 513, 229–240. [Google Scholar] [CrossRef]
- Marion, D.; Nur, A.; Yin, H.; Han, D. Compressional velocity and porosity in sand-clay mixtures. Geophysics 1992, 57, 554–563. [Google Scholar] [CrossRef]
- Morin, R.H. Negative correlation between porosity and hydraulic conductivity in sand-and-gravel aquifers at Cape Cod, Massachusetts, USA. J. Hydrol. 2006, 316, 43–52. [Google Scholar] [CrossRef]
- Valett, H.M.; Fisher, S.G.; Grimm, N.B.; Camill, P. Vertical hydrologic exchange and ecological stability of a desert stream ecosystem. Ecology 1994, 75, 548–560. [Google Scholar] [CrossRef]
- Kennedy, C.D.; Murdoch, L.C.; Genereux, D.P.; Corbett, D.R.; Stone, K.; Pham, P.; Mitasova, H. Comparison of Darcian flux calculations and seepage meter measurements in a sandy streambed in North Carolina, United States. Water Resour. Res. 2010, 46, 5109–5115. [Google Scholar] [CrossRef]
- Shope, C.L.; Constantz, J.E.; Cooper, C.A.; Reeves, D.M.; Pohll, G.; Mckay, W.A. Influence of a large fluvial island, streambed, and stream bank on surface water-groundwater fluxes and water table dynamics. Water Resour. Res. 2012, 48, 109–119. [Google Scholar] [CrossRef]



| Flow Condition | July 2015 | January 2016 |
|---|---|---|
| Number of measurements | 31 | 30 |
| Average channel width (m) | 29 | 25 |
| Average water depth (cm) | 81 | 59 |
| Max. water depth (cm) | 136 | 98 |
| Sinuosity | 1.40 | 1.32 |
| Average water velocity (m/s) | 0.54 | 0.36 |
| Mean water velocity of (L1/L2/C/R2/R1) 1 (m/s) | 0.47/0.50/0.59/0.61/0.52 | 0.25/0.36/0.45/0.39/0.35 |
| Statistic | Range | Median Value | Average Value | Coefficient of Variation | ||||
|---|---|---|---|---|---|---|---|---|
| July 2015 | January 2016 | July 2015 | January 2016 | July 2015 | January 2016 | July 2015 | January 2016 | |
| Kv (m/day) | 0.03~48.43 | 0.01~5.82 | 1.40 | 0.17 | 9.20 | 0.89 | 1.29 | 1.62 |
| K20 1 (m/day) | 0.02~38.23 | 0.02~8.55 | 1.13 | 0.25 | 7.45 | 1.31 | 1.29 | 1.62 |
| VHG | −0.41~0.01 | −0.25~0.04 | −0.03 | −0.04 | −0.07 | −0.06 | 1.31 | 1.06 |
| DF (cm/day) | −69.37~62.4 | −11.08~6.05 | −1.98 | −1.41 | −6.76 | −2.28 | 1.38 | 1.46 |
| φ | 0.39~0.46 | 0.39~0.49 | 0.41 | 0.41 | 0.41 | 0.43 | 0.04 | 0.07 |
| Sediment | July 2015 | January 2016 | ||||||
|---|---|---|---|---|---|---|---|---|
| L and L1 | C | R and R1 | L and L1 | C | R and R1 | |||
| Particle size | Average percentage (%) | 0.075–2 mm (sand) | 91.0 | 91.7 | 73.1 | 72.6 | 78.6 | 56.2 |
| <0.075 mm (silt + clay) | 3.2 | 2.4 | 26.3 | 25.7 | 18.3 | 41.7 | ||
| Average median grain size | d50 (mm) | 0.18 | 0.20 | 0.14 | 0.15 | 0.17 | 0.07 | |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license ( http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xu, S.; Song, J.; Jiang, W.; Zhang, G.; Wen, M.; Zhang, J.; Xue, Y. Variability of Darcian Flux in the Hyporheic Zone at a Natural Channel Bend. Water 2017, 9, 170. https://doi.org/10.3390/w9030170
Xu S, Song J, Jiang W, Zhang G, Wen M, Zhang J, Xue Y. Variability of Darcian Flux in the Hyporheic Zone at a Natural Channel Bend. Water. 2017; 9(3):170. https://doi.org/10.3390/w9030170
Chicago/Turabian StyleXu, Shaofeng, Jinxi Song, Weiwei Jiang, Guotao Zhang, Ming Wen, Junlong Zhang, and Ying Xue. 2017. "Variability of Darcian Flux in the Hyporheic Zone at a Natural Channel Bend" Water 9, no. 3: 170. https://doi.org/10.3390/w9030170
APA StyleXu, S., Song, J., Jiang, W., Zhang, G., Wen, M., Zhang, J., & Xue, Y. (2017). Variability of Darcian Flux in the Hyporheic Zone at a Natural Channel Bend. Water, 9(3), 170. https://doi.org/10.3390/w9030170






