Effect of Twist Angle Regulation via Flexible Variable-Twist Blades on External Characteristics of Axial-Flow Pumps

Abstract

In the field of marine resource development, conventional axial-flow adjustable-blade pumps rely on the monolithic rotation of rigid blades for operational condition regulation, a mechanism constrained by simplistic angular adjustments that inadequately adapt to the dynamic and complex marine operational environment. To address this limitation, this study proposes a novel angle-adjustment scheme utilizing flexible variable-twist blades, where operational condition regulation is achieved through active blade twisting, enabling refined and adaptive angle modulation. Four typical blade profiles were selected for the variable-twist blades at distinct angular positions (−1°, +1°, −2°, and +2°), corresponding to the four conventional angle-adjustment positions of axial-flow adjustable-blade pumps. Numerical simulations were conducted to investigate the hydraulic performance impacts of the proposed flexible variable-twist blades compared to traditional rigid blades under identical angular configurations. The results demonstrate that under high-flow conditions (1.2 Q), the torsion-based angle-adjustment strategy exhibits superior efficiency across all four angular positions: −1° configuration: 11.1% efficiency improvement; +1° configuration: comparable efficiency; −2° configuration: 78% efficiency improvement; and +2° configuration: 3.2% efficiency improvement. Moreover, at equivalent angular settings, the variable-twist blades significantly enhance hydraulic performance and expand the high-efficiency operating range of the pump compared to conventional rigid blades. The implementation of flexible variable-twist blade technology not only advances the performance of axial-flow pumps in marine engineering applications but also provides a new approach for high-efficiency research on axial-flow pumps.

1. Introduction

Axial-flow pumps represent a category of vane-type hydraulic machinery characterized by a relatively high specific speed and simple structural configuration. Owing to their high-flow, low-head characteristics; exceptional efficiency; rapid start-up capability; and operational versatility, these pumps are widely employed across diverse sectors in marine science [1]. However, during actual operations, parameters, such as the flow rate and head, are often dynamically variable, leading to significant deviations between the actual operating point and the designed optimum [2]. A prolonged operation under off-design conditions results in reduced hydraulic efficiency, accompanied by detrimental vibrations and cavitation phenomena. Consequently, operational adjustments are imperative to enhance pump efficiency under variable working conditions when system requirements deviate from nominal design specifications [3]. High-efficiency axial-flow pumps play a critical role in marine applications by reducing energy consumption and enhancing desalination performance to alleviate freshwater scarcity, minimizing pollutant discharge into marine ecosystems through an enhanced wastewater treatment capacity and boosting hydraulic circulation efficiency to increase power generation outputs in tidal and wave energy systems. Research on high-efficiency axial-flow pumps profoundly impacts marine science by advancing resource exploitation, environmental protection, and technological innovation, thereby delivering substantial scientific and economic value [4].

Common operational adjustment strategies for hydraulic systems include speed regulations, guide-vane inlet adjustments, blade-angle modifications, and varying the number of operational axial-flow pumps [5]. Among these, blade-angle adjustments have been widely adopted as a conventional method for condition regulations [6]. By altering the blade setting angle, this approach enables a flexible modulation of the pump flow rate and head without requiring rotational speed variations. Owing to its capacity to decouple flow-head control from speed constraints, substantial technical documentation exists on blade-angle adjustments, supported by extensive theoretical and experimental investigations conducted by domestic and international researchers [7].

Wu Z. J. et al. [8] conducted a three-dimensional numerical investigation of the full flow passage in an axial-flow pump, proposing four blade-setting-angle configurations under design conditions. In contrast, Chen H. X. et al. [9] performed multi-condition numerical simulations and external characteristic calculations on the same pump model under varying blade angles, considering both steady and unsteady operating states. Their computational results were validated against experimental data, revealing vortex blockage phenomena near the hub region under low-flow conditions. Yan T. X. et al. [10] systematically investigated the influence of guide-vane inlet setting angles on pump performance through numerical modeling, focusing on hydraulic characteristics and internal flow patterns. Their findings indicate that guide-vane angle adjustments predominantly affect the guide-vane assembly and discharge components. While moderately increasing the inlet angle enhances efficiency under high-flow conditions, excessively small angles were shown to narrow the pump’s high-efficiency operational range. Al-Obaidi A. R. et al. [11] analyzed temporal and frequency-domain behaviors of flow fields and pressure fluctuations in axial-flow pumps through blade-angle variations. Their results demonstrate significant correlations between blade angles and distinct flow regimes, with both angular settings and unsteady flow effects substantially influencing pressure fluctuation amplitudes. Yune Sung Kim et al. [12] evaluated the hydrodynamic impacts of propeller hub sweep angles, blade pitch angles, and inlet angles on submersible axial-flow pumps. A parametric assessment quantified the effects of rotor blade geometric parameters on the overall pump efficiency and total head characteristics. Furthermore, Yun Jeong-Eui et al. [13] employed numerical simulations to optimize inlet guide-vane geometries and installation angles. By integrating response surface methodology with design exploration techniques, they developed an analytical framework for obtaining optimal guide-vane configurations under specified operational constraints. Sun Aoran et al. [14] numerically investigated the effects of five inlet angles of guide vanes on axial-flow pump performance. Results demonstrate that under off-design conditions, adjusting the inlet angle of guide vanes effectively reduced incidence angles and improved internal flow patterns. Clockwise adjustments enhanced efficiency in high-flow conditions, while counterclockwise adjustments optimized performance in low-flow regimes. Shi Lijian et al. [15] conducted numerical simulations to analyze the impact of incidence angles on axial-flow pump impeller performance. Key findings reveal that (1) increased incidence angles elevated the head and shifted the high-efficiency zone toward larger flow rates; (2) enlarging mid-span incidence angles improved both the head and efficiency under design conditions; (3) reducing hub-side incidence angles enhanced off-design performance; and (4) for constant specific speed conditions, larger incidence angles decreased the slope of head-flow curves while expanding the high-efficiency range. The aforementioned studies provide an in-depth investigation into how variable-angle adjustments affect the hydraulic performance of axial-flow pumps and the internal flow patterns within the impeller, offering valuable insights that bridge variable-angle adjustments with flow pattern analyses for the current research. However, all existing variable-angle-adjustment methods involve rotating the rigid blades of the impeller. Building upon previous research and referencing the blade placement angles discussed in these studies, this study selects appropriate variable-twist angles for the blades to examine the impact of variable-twist blades on the hydraulic performance of axial-flow pumps.

Conventional angle-adjustment strategies in axial-flow pumps rely on globally rotating rigid impeller blades to modify the blade setting angle for operational condition regulation [16]. However, due to the inherent disparity in flow patterns between the hub and tip regions of impellers, such monolithic angular adjustments exhibit limited adaptability to diverse operating conditions and lack the capability for localized flow-field optimization. To address these constraints, this study proposes an innovative torsion-based angle-adjustment methodology utilizing variable-twist blades. By dynamically modulating the blade chord setting angle along the span-wise direction (from the hub to the tip) in response to operational demands, this approach enables graded angular reconfigurations of the blade profile. The resultant localized angle modulation allows for targeted adaptations to region-specific flow characteristics, thereby achieving enhanced hydraulic performance across varying operational conditions.

To investigate the performance enhancement capabilities of flexible variable-twist blades in axial-flow pumps, four distinct angular configurations (−1°, +1°, −2°, and +2°) were selected for the morphing blades, and a three-dimensional hydraulic model of the pump was constructed using UG NX. Numerical simulations were subsequently performed to analyze the external characteristic curves and internal flow patterns (pressure distribution, streamline topology, and turbulent kinetic energy dissipation). A comparative study was conducted to evaluate the impacts of both torsion-based and conventional rigid-blade angle-adjustment strategies on hydraulic performance and internal flow dynamics under identical angular displacements across multiple operating conditions.

2. Computational Model and Numerical Simulations

2.1. Blade Twist Deformation Method and Angle Selection

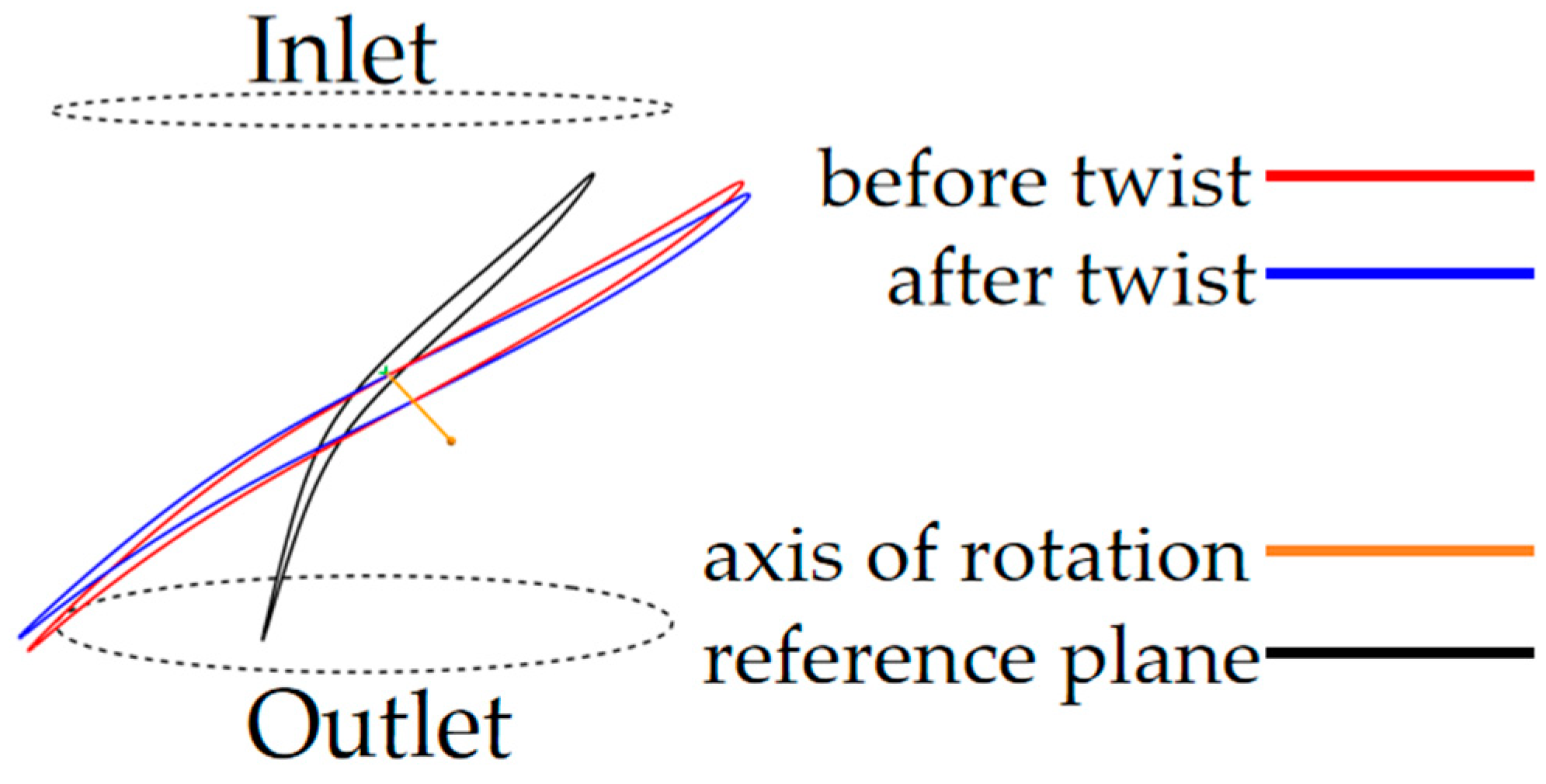
The pump blade employed in the numerical simulations incorporated 11 span-wise sections along the blade height. As illustrated in Figure 1, these span-wise sections were sequentially numbered from 01 to 11 along the hub-to-tip direction. Span-wise section 01, which intersects with the hub, was defined as the reference plane for geometric parameterization and subsequent flow analysis.

The torsional deformation of the morphing blade was realized by rotating span-wise sections 02 to 11. In the original configuration of the pump blade, all 11 span-wise sections were initialized at a 0° blade setting angle. A rotation axis was defined by constructing a line perpendicular to the span-wise sections through the midpoint of reference plane 01, based on the baseline blade geometry. The counterclockwise direction was designated as the positive angular displacement, whereas a clockwise rotation corresponds to negative values. Figure 2 provides a schematic representation of the −2° angular displacement applied to span-wise section 05, demonstrating the graded torsional reconfiguration strategy.

Span-wise sections 02 to 11 were sequentially rotated clockwise by 1° to generate the −1° blade configuration, designated as Group Y₁. Following this methodology, three additional configurations were constructed: +1° (Group Y₂), −2° (Group Y₃), and +2° (Group Y₄).

Table 1. Table of blade span-wise-section angle variations.

Group	Number
	01	02	03	04	05	06	07	08	09	10	11
Y1	0°	−1°	−1°	−1°	−1°	−1°	−1°	−1°	−1°	−1°	−1°
Y2	0°	+1°	+1°	+1°	+1°	+1°	+1°	+1°	+1°	+1°	+1°
Y3	0°	−2°	−2°	−2°	−2°	−2°	−2°	−2°	−2°	−2°	−2°
Y4	0°	+2°	+2°	+2°	+2°	+2°	+2°	+2°	+2°	+2°	+2°

systematically summarizes the angular displacement profiles across all span-wise sections for each blade configuration group.

2.2. Blade-Angle Adjustments and Angle Selections for Axial-Flow Adjustable-Blade Pumps

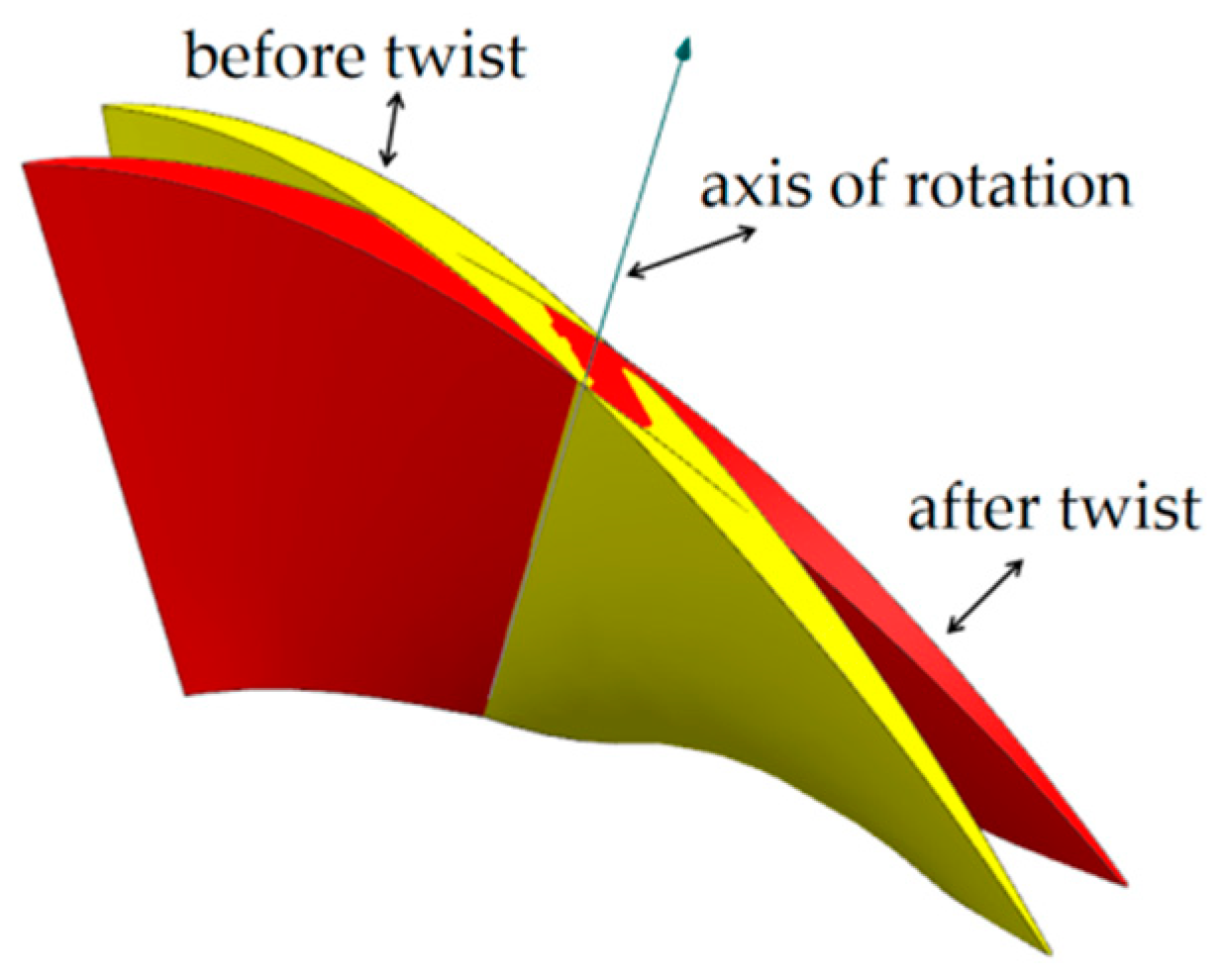
The angle-adjustment mechanism of conventional axial-flow adjustable-blade pumps fundamentally differs from the torsion-based approach. In traditional rigid-blade systems, angular modulation is achieved through an integral rotation of the entire blade assembly about a defined axis. A rotation axis is established at the midpoint of the root span-wise section (blade base), aligning with the torsional reference frame of variable-twist blades. Consistent with the established convention, a counterclockwise rotation corresponds to a positive angular displacement, while a clockwise rotation corresponds to negative values. Figure 3 schematically illustrates this monolithic angular adjustment strategy, with

Table 2. Table of blade-angle adjustments for axial-flow adjustable-blade pumps.

	Number	Angle
1	V1	−1°
2	V2	+1°
3	V3	−2°
4	V4	+2°

providing the blade-angle adjustments for axial-flow adjustable-blade pumps.

2.3. Selection of Comparison Groups

To systematically evaluate the performance-enhancement capabilities of variable-twist blades versus conventional axial-flow adjustable-blade pumps under varying operational conditions, comparative groups were established based on the four angles for both blade configurations.

Table 3. Comparison grouping table.

	Group
1	V1:Y1
2	V2:Y2
3	V3:Y3
4	V4:Y4

comprehensively outlines the experimental grouping scheme. This study meticulously investigates the performance-enhancement of variable-twist blades compared to conventional axial-flow propeller pumps under four distinct angle-adjustment conditions (−1°, +1°, −2°, and +2°). All angle modifications were systematically implemented using a standard blade designed with 0° as the reference configuration, while maintaining consistent geometric properties for the blade length, cross-sectional chord length, and thickness throughout the design process.

2.4. Modeling and Mesh Generation of the Axial-Flow Pump

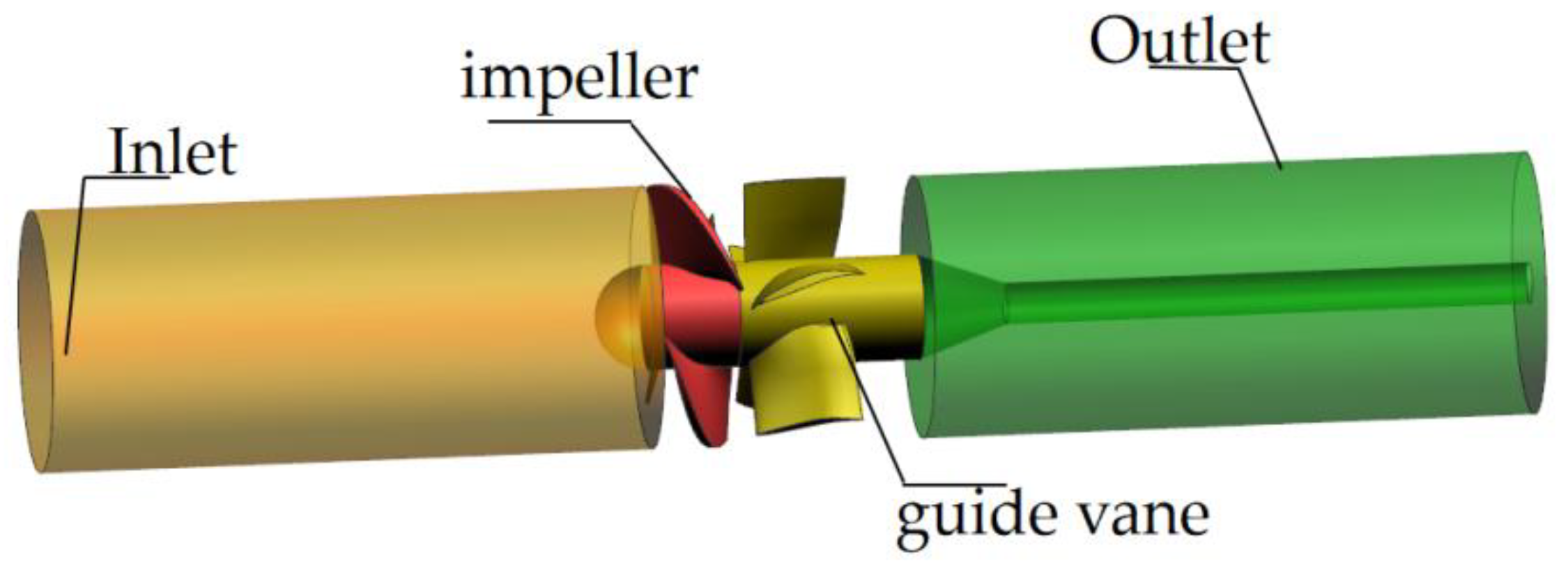
The 350ZLB-100 axial-flow pump was selected as the research prototype (manufactured by Jinan Taida Pump Industry Co., Ltd., Jinan City, Shandong Province, China). A three-dimensional model of the pump unit was developed using the UG NX software (version 12.0). Key design specifications include a rated flow capacity of 401.64 L/S, a rated head of 4.80 m, a rotational speed of 1450 rpm, an impeller diameter of 0.3 m, 3 impeller blades, and 5 guide vanes (Figure 4).

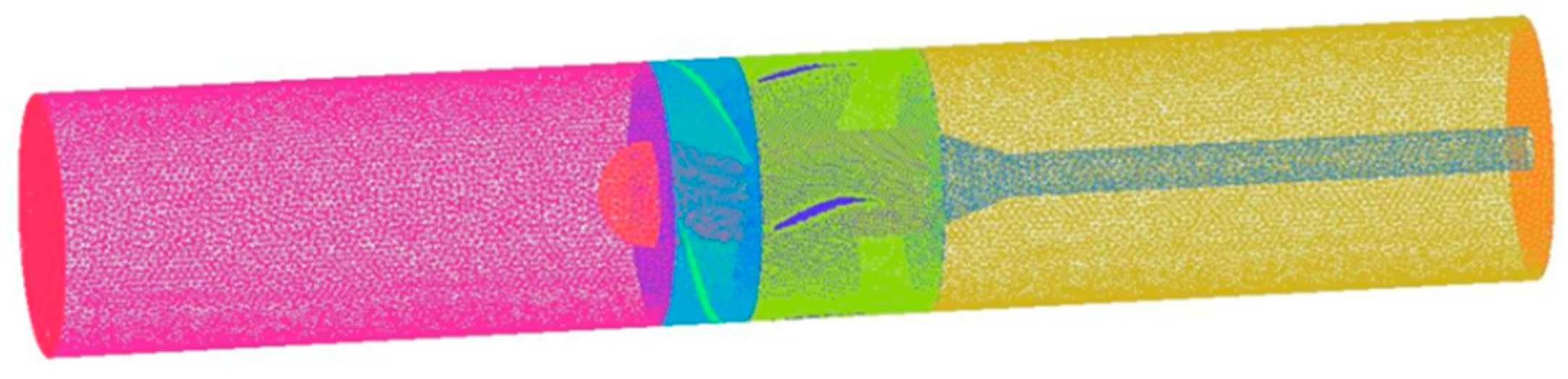
Given the requirement for variable blade-twist angles in this study and the structural complexity of the impeller blades, structured grids prove inadequate for accommodating the torsional deformation of the blades, presenting significant grid-generation challenges and yielding unsatisfactory grid quality that compromises computational accuracy. Consequently, unstructured grids with superior geometric adaptability were employed for the impeller domain in the numerical simulations. Local grid refinements were implemented in the blade regions to increase grid density, thereby ensuring enhanced computational precision. Figure 5 shows a mesh diagram of the axial-flow pump section.

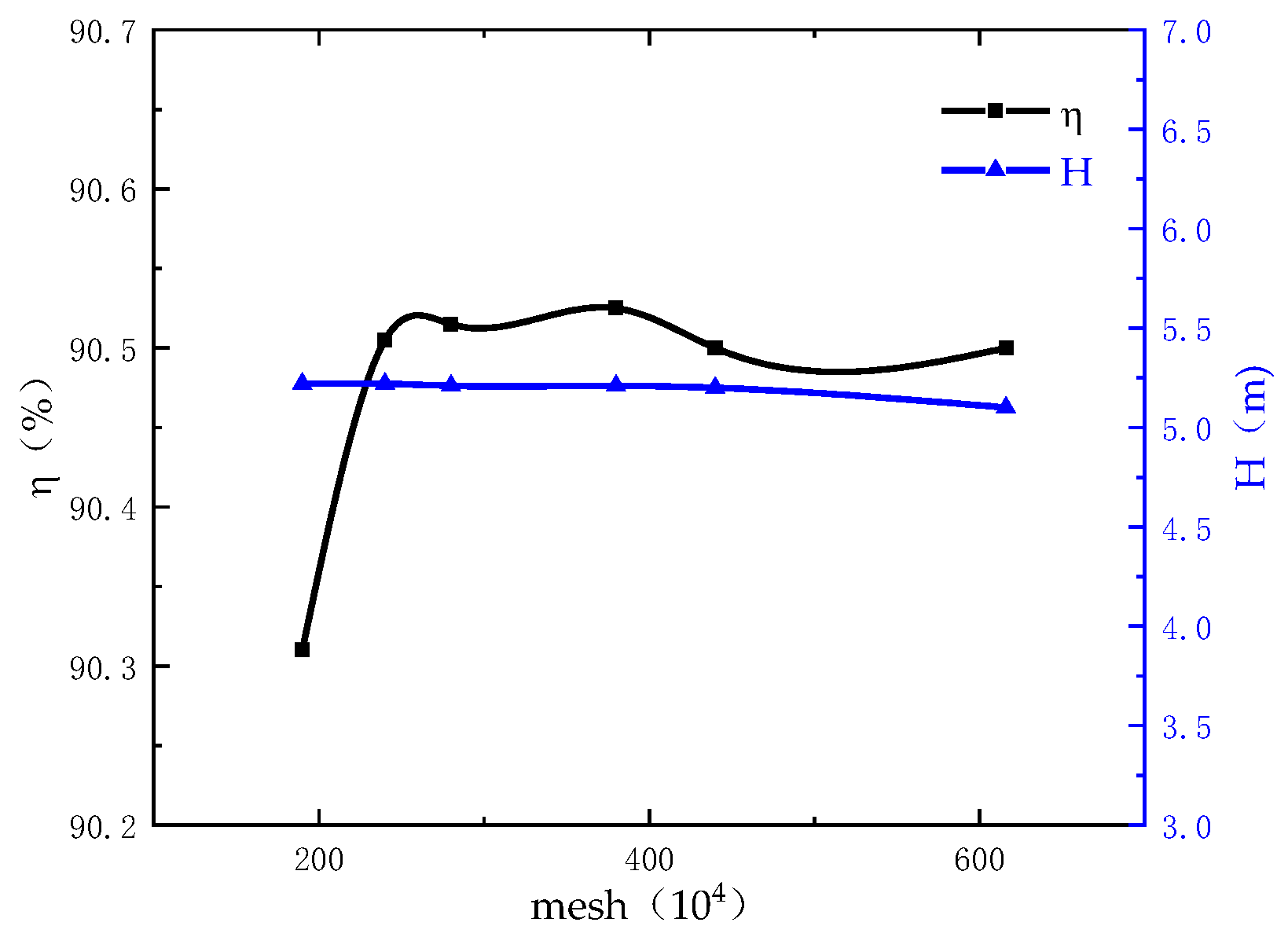
The computational domain was discretized using unstructured mesh, which demonstrates superior adaptability to the geometric complexity of the pump assembly. Localized mesh refinements were strategically implemented in regions with high velocity/pressure gradients to ensure solution accuracy while maintaining computational efficiency [17]. Five distinct mesh configurations with progressive resolution levels (1.9 million, 2.4 million, 2.8 million, 3.8 million, and 4.4 million cells) were generated for mesh independence verification. A systematic mesh convergence analysis was performed under design operating conditions [18], with the quantitative results of key performance metrics presented in

Table 4. Mesh convergence verification.

	Number (×104)	Efficiency (%)	Head (m)
1	190	90.31	5.22
2	240	90.50	5.21
3	280	90.51	5.21
4	380	90.52	5.21
5	440	90.50	5.21
6	660	90.50	5.20

and Figure 6.

The computational-domain meshing process revealed that an increased mesh density improves resolution accuracy in resolving internal flow-field characteristics within the pump [19]. As shown in Figure 6, hydraulic performance parameters (efficiency) reached peak values and stabilized at 3.8 million elements, with the corresponding mesh configuration achieving minimum orthogonal quality exceeding 0.2. Based on this asymptotic convergence behavior, it was determined that further mesh refinements beyond 3.8 million elements exerted negligible influence on the computational results. Consequently, a mesh configuration of 3.8 million elements was adopted for subsequent numerical simulations.

2.5. Numerical Simulations and Boundary Condition Setup

The flow of water inside the pump can be treated as a three-dimensional, incompressible, viscous turbulent flow. For numerical simulations, the governing equations of fluid mechanics were used to formulate and solve the system of equations [20]. These fundamental equations include the continuity equation, energy equation, and momentum equation [21]. Since heat exchange is not considered, the governing equations were simplified to the following two:

• Continuity Equation

The mass conservation equation for fluids, also known as the continuity equation [22], is expressed as follows:

$${\displaystyle \frac{\partial \rho }{\partial t}}+{\displaystyle \frac{\partial \rho u_i}{\partial x_i}}=0$$

(1)

In this equation, $\rho$ represents the density, $t$ represents time, and $u$ represents the velocity vector.

2.

Momentum Equation:

For viscous incompressible fluids, the momentum equation, also known as the N-S equation, is expressed as follows [23]:

$${\displaystyle \frac{\partial u_i}{\partial t}}+{\displaystyle \frac{\partial \left(u_i{u}_j\right)}{\partial x_j}}=-{\displaystyle \frac{1}{\rho }}{\displaystyle \frac{\partial p}{\partial x_i}}+{\displaystyle \frac{1}{\rho }}{\displaystyle \frac{\partial }{\partial x_j}}\left(\mu \left({\displaystyle \frac{\partial u_i}{\partial x_j}}+{\displaystyle \frac{\partial u_j}{\partial x_i}}\right)\right)+f_i$$

(2)

In this equation, $\rho$ represents the density, $t$ represents time, $\mu$ represents the dynamic viscosity, $u$ represents the velocity vector, and $f_i$ represents the source term.

The Reynolds number was calculated as follows:

$$\mathrm{Re}={\displaystyle \frac{\rho u{D}_h}{\mu }}$$

(3)

In this equation, $\rho$ represents the density, $u$ represents the velocity vector, $\mu$ represents the dynamic viscosity, and $D_h$ represents the hydraulic diameter.

The numerical simulations in this study were conducted at 25 °C, with the water density set to 1000 kg/m³ and with a hydraulic diameter of 1.7 m. Under the design operating conditions, the inlet flow velocity of the pump was 5.685 m/s, yielding a Reynolds number (Re) of 1.08 × 10⁷.

This numerical simulation was conducted under high-Reynolds-number conditions and employed a steady-state approach. Given these parameters, the standard $k-\epsilon$ turbulence model is well-suited for rotating machinery simulations due to its robustness and adaptability in capturing turbulent flow characteristics within axial-flow pumps. Consequently, the standard $k-\epsilon$ turbulence model was adopted for the current study.

The standard $k-\epsilon$ turbulence model is capable of meeting the requirements for numerical simulations of rotating machinery [24]. It exhibits strong adaptability and can effectively capture the turbulent flow characteristics inside axial-flow pumps. Therefore, the standard $k-\epsilon$ turbulence model was adopted for this numerical simulation [25].

$${\displaystyle \frac{\partial \left(\rho k\right)}{\partial t}}+{\displaystyle \frac{\partial \left(\partial k{u}_i\right)}{\partial x_i}}={\displaystyle \frac{\partial }{\partial x_j}}\left[\left(\mu +{\displaystyle \frac{{\mu }_t}{{\sigma }_k}}\right){\displaystyle \frac{\partial k}{\partial x_j}}\right]+{\mu }_t\left({\displaystyle \frac{\partial u_i}{\partial x_j}}+{\displaystyle \frac{\partial u_j}{\partial x_i}}\right){\displaystyle \frac{\partial u_i}{\partial x_j}}-\rho \epsilon$$

(4)

$${\displaystyle \frac{\partial \left(\rho k\right)}{\partial t}}+{\displaystyle \frac{\partial \left(\rho \epsilon u_i\right)}{\partial x_i}}={\displaystyle \frac{\partial }{\partial x_j}}\left[\left(\mu +{\displaystyle \frac{{\mu }_t}{{\sigma }_{\epsilon }}}\right){\displaystyle \frac{\partial \epsilon }{\partial x_j}}\right]+{\displaystyle \frac{C_{\epsilon 1}}{k}}{\mu }_t\left({\displaystyle \frac{\partial u_i}{\partial x_j}}+{\displaystyle \frac{\partial u_j}{\partial x_i}}\right){\displaystyle \frac{\partial u_i}{\partial x_j}}-C_{\epsilon 2}\rho {\displaystyle \frac{{\epsilon }^2}{k}}$$

(5)

In this equation, $C_{\epsilon 1}$ = 1.44, $C_{\epsilon 2}$ = 1.92, ${\sigma }_k$ = 1.0, and ${\sigma }_{\epsilon }$ = 1.3.

3. Analysis of Numerical Simulation Results

3.1. External Characteristic Curves of the Pump

As evidenced by the external characteristic data (

Table 5. (a) Comparison table of efficiency between V₁ and Y₁. (b) Comparison table of head between V₁ and Y₁.

(a)
Q (m/s)	V1-η (%)	Y1-η (%)	Difference
4.548	82.56	83.49	−0.93
5.117	87.18	87.25	−0.07
5.685	90.29	90.02	0.27
6.254	83.53	85.34	−1.81
6.822	57.54	63.93	−6.39
(b)
Q (m/s)	V1-H (m)	Y1-H (m)	Difference
4.548	6.12	6.10	0.02
5.117	5.41	5.52	−0.11
5.685	4.68	4.98	−0.30
6.254	3.13	3.49	−0.36
6.822	1.32	1.69	−0.37

,

Table 6. (a) Comparison table of efficiency between V₂ and Y₂. (b) Comparison table of head between V₂ and Y₂.

(a)
Q (m/s)	V2-η (%)	Y2-η (%)	Difference
4.548	73.60	74.20	−0.60
5.117	82.52	82.49	0.03
5.685	87.50	87.53	−0.03
6.254	89.83	90.28	−0.45
6.822	84.35	84.97	−0.62
(b)
Q (m/s)	V2-H (m)	Y2-H (m)	Difference
4.548	7.03	7.03	0
5.117	6.38	6.38	0
5.685	5.75	5.82	−0.07
6.254	4.98	5.13	−0.15
6.822	3.53	3.67	−0.14

,

Table 7. (a) Comparison table of efficiency between V₃ and Y₃. (b) Comparison table of head between V₃ and Y₃.

(a)
Q (m/s)	V3-η (%)	Y3-η (%)	Difference
4.548	84.43	84.80	−0.37
5.117	89.02	88.68	0.43
5.685	89.21	90.36	−1.15
6.254	78.06	82.52	−4.46
6.822	28.07	50.01	−21.94
(b)
Q (m/s)	V3-H (m)	Y3-H (m)	Difference
4.548	5.78	5.98	−0.20
5.117	5.20	5.44	−0.24
5.685	4.13	4.61	−0.48
6.254	2.46	3.01	−0.55
6.822	0.47	1.10	−0.63

and

Table 8. (a) Comparison table of efficiency between V₄ and Y₄. (b) Comparison table of head between V₄ and Y₄.

(a)
Q (m/s)	V4-η (%)	Y4-η (%)	Difference
4.548	73.52	72.13	1.39
5.117	82.79	80.01	2.78
5.685	87.3	85.98	1.32
6.254	89.84	90.05	−0.21
6.822	84.47	87.16	−2.69
(b)
Q (m/s)	V4-H (m)	Y4-H (m)	Difference
4.548	7.07	7.44	−0.37
5.117	6.47	6.70	−0.23
5.685	5.73	6.06	−0.33
6.254	4.99	5.54	−0.55
6.822	3.54	4.24	−0.70

) and curve distribution plots (Figure 7, Figure 8, Figure 9 and Figure 10), the efficiency and head curves of the two variable-angle-adjustment methods for the pump exhibit nearly identical trends under the same blade angle across varying operating conditions, thereby indirectly validating the feasibility of angle modulation via variable-twist blade control. Specifically, the Y₁ group consistently outperformed the V₁ group in efficiency under all conditions, with a marked 11.1% improvement at 1.2 Q (57.54% for V₁ vs. 63.93% for Y₁). While the Y₂ and V₂ groups demonstrated comparable efficiency across all flow rates, the Y₃ group surpassed V₃ under high-flow conditions, achieving a 78% efficiency increase at 1.2 Q (28.07% for V₃ vs. 50.01% for Y₃). Notably, the Y₄ and V₄ groups exhibited significant efficiency divergence: V₄ outperformed Y₄ below 1.1 Q (e.g., 73.52% vs. 72.13% at 0.8 Q), whereas Y₄ excelled above 1.1 Q, attaining an 87.16% efficiency compared to V₄’s 84.47% at 1.2 Q (3.2% improvement). These distinct efficiency patterns conclusively demonstrate that variable-twist blade modulation enhances hydraulic performance, particularly under high-flow operating conditions.

3.2. Flow Pattern Analysis in the Impeller Region

Taking Groups Y₁ and V₁ as examples, the flow patterns in the impeller region under 0.8 Q and 1.2 Q operating conditions were analyzed to investigate the influence of variable-twist blades on internal flow characteristics.

3.2.1. A Flow Pattern Analysis in the Impeller Region of Group Y₁ and Group V₁ Pumps Under the 0.8 Q Operating Condition

Figure 11 presents the pressure distribution diagrams of the impeller blades for the Y₁ and V₁ groups under the 0.8 Q operating condition. From the pressure contour plots on the front side of the blade, both the Y₁ and V₁ groups exhibit similar pressure distribution patterns, with minimum pressure observed at the leading edge and maximum pressure at the trailing edge. Specifically, the Y₁ group shows a maximum pressure of 117.02 kPa and a minimum pressure of −324.8 kPa, while the V₁ group reaches a maximum pressure of 128.05 kPa and a minimum pressure of −322.497 kPa. Notably, the area of minimum pressure at the leading edge in the Y₁ group is significantly smaller than that in the V₁ group, and the region of maximum pressure at the trailing edge in Y₁ is also reduced compared to V₁. In contrast, the pressure distribution on the blade backside shows negligible differences between the two groups. These observations suggest that the Y₁ blade configuration achieves more stable flow conditions at the leading edge, with reduced flow impingement, and promotes uniform flow distribution at the trailing edge, indicating effective suppression of flow separation.

Figure 12 presents the streamline distributions of Y₁ and V₁ impeller blades under the 0.8 Q condition, revealing nearly identical flow patterns at Span 09 with stable hydrodynamic behavior and negligible flow separation or turbulence. However, at Span 03, both configurations exhibit flow separation near the trailing edge, with Y₁ demonstrating more pronounced flow separation. A detailed analysis of Spans 03 and 05 identified significant flow impingement at the leading edge of the V₁ blades, forming a low-velocity zone indicative of flow detachment and hydrodynamic instability, whereas the Y₁ blades eliminated this low-velocity zone through optimized hub-region inflow alignment, effectively mitigating impingement losses and enhancing energy transfer efficiency.

To evaluate the turbulent kinetic energy (TKE) distribution in the impeller domain under high-flow conditions, two critical cross-sections were defined, P1 (impeller inlet) and P2 (impeller outlet), as schematically represented in Figure 13, enabling a systematic analysis of blade-angle modulation strategies on impeller TKE dissipation patterns.

Figure 14 compares the TKE distributions of the Y₁ and V₁ impellers, showing consistent TKE concentration patterns dominated by tip-leakage vortices and blade boundary layers. In the P1 section, Y₁ exhibits reduction in TKE dissipation along blade surfaces compared to V₁, confirming optimized inflow attachment and energy loss mitigation, while in the P2 section, Y₁ demonstrates a decrease in tip-region TKE dissipation, evidencing enhanced outflow uniformity and suppressed turbulence-induced flow separation at the discharge.

3.2.2. Flow Pattern Analysis in the Impeller Region of Group Y₁ and Group V₁ Pumps Under the 1.2 Q Operating Condition

Figure 15 displays the pressure distribution diagrams of the impeller blades for the Y₁ and V₁ groups under the 1.2 Q operating condition. From the pressure contour plots on the front side of the blade, both groups exhibit consistent pressure distribution patterns: maximum pressure occurs at the leading edge, while minimum pressure is observed in the mid-section of the blade. Specifically, the Y₁ group shows a maximum pressure of 86.4 kPa and a minimum pressure of −128.39 kPa, whereas the V₁ group achieves a maximum of 89.2 kPa and a minimum of −141.6 kPa. Notably, the area of the minimum pressure region in the mid-section of the Y₁ group is significantly larger than that of the V₁ group. This indicates higher local flow velocities within the Y₁ configuration, which enhance the kinetic energy of the fluid, thereby improving the pump’s head and efficiency. The distinct pressure distribution characteristics highlight the Y₁ group’s superior ability to optimize flow dynamics under high-flow (1.2 Q) conditions.

Figure 16 illustrates the streamline distributions of the Y₁ and V₁ impellers under the 1.2 Q condition, showing stable flow patterns at Span 09 without flow separation or turbulence. However, at Spans 03 and 05, V₁ exhibits flow impingement at the leading edge (evidenced by a low-velocity zone), whereas Y₁ eliminates this zone through optimized hub-side and mid-span inflow alignment, confirming enhanced hydrodynamic stability.

Figure 17 presents the turbulent kinetic energy distributions of the Y₁ and V₁ impellers, demonstrating similar TKE concentration patterns dominated by tip-leakage vortices and blade boundary layers; while the P1 section shows negligible differences, Y₁ reduces tip-region TKE dissipation in the P2 section compared to V₁, demonstrating improved outflow dynamics and suppressed turbulence-induced energy losses.

4. Conclusions

Diverging from conventional axial-flow adjustable-blade pump angle-adjustment strategies, this study proposes a torsion-based angle modulation scheme through blade deformation, selecting four angular configurations ( −1°, +1°, −2°, and +2°) to investigate the hydraulic performance impacts of variable-twist versus rigid-blade systems under identical angular displacements across operating conditions. Comprehensive analyses were conducted on external characteristic curves, pressure distributions, streamline patterns at fixed span-wise sections, and turbulent kinetic energy profiles at defined impeller cross-sections, elucidating the hydrodynamic improvements of variable-twist blades in internal flow regulation.

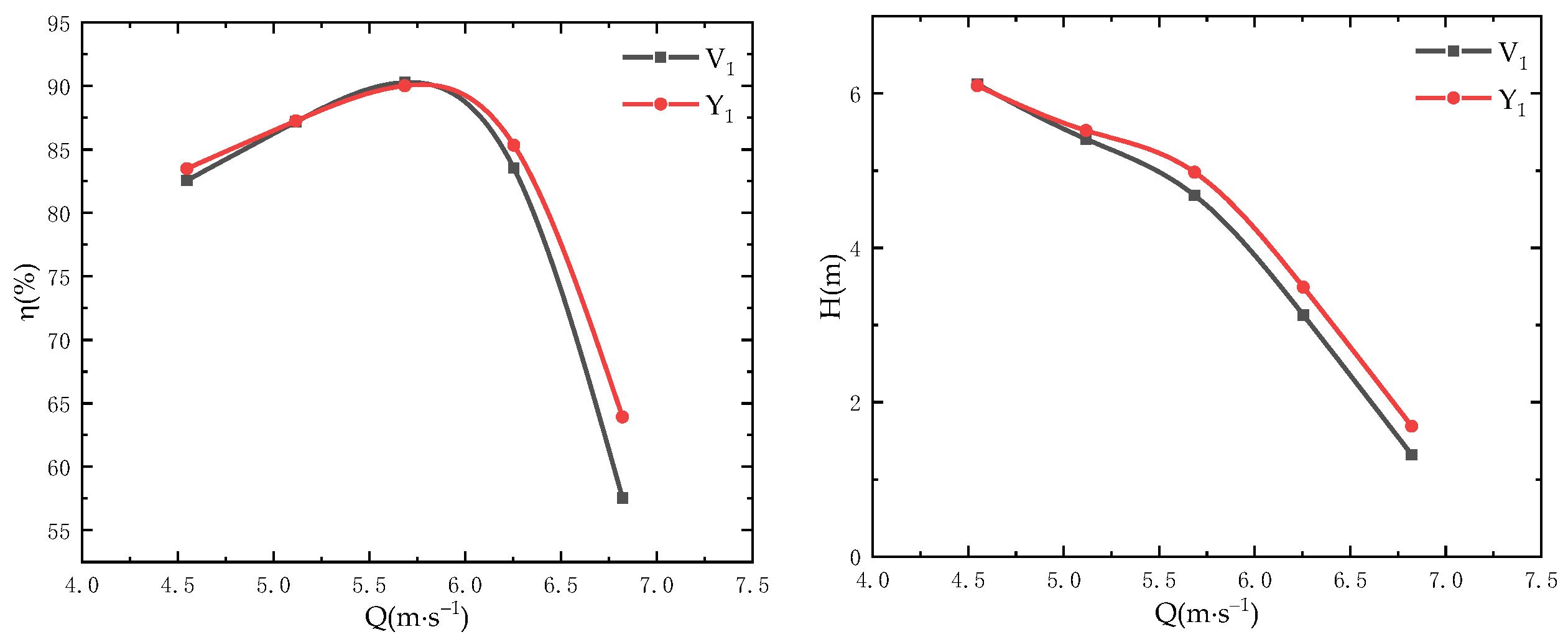
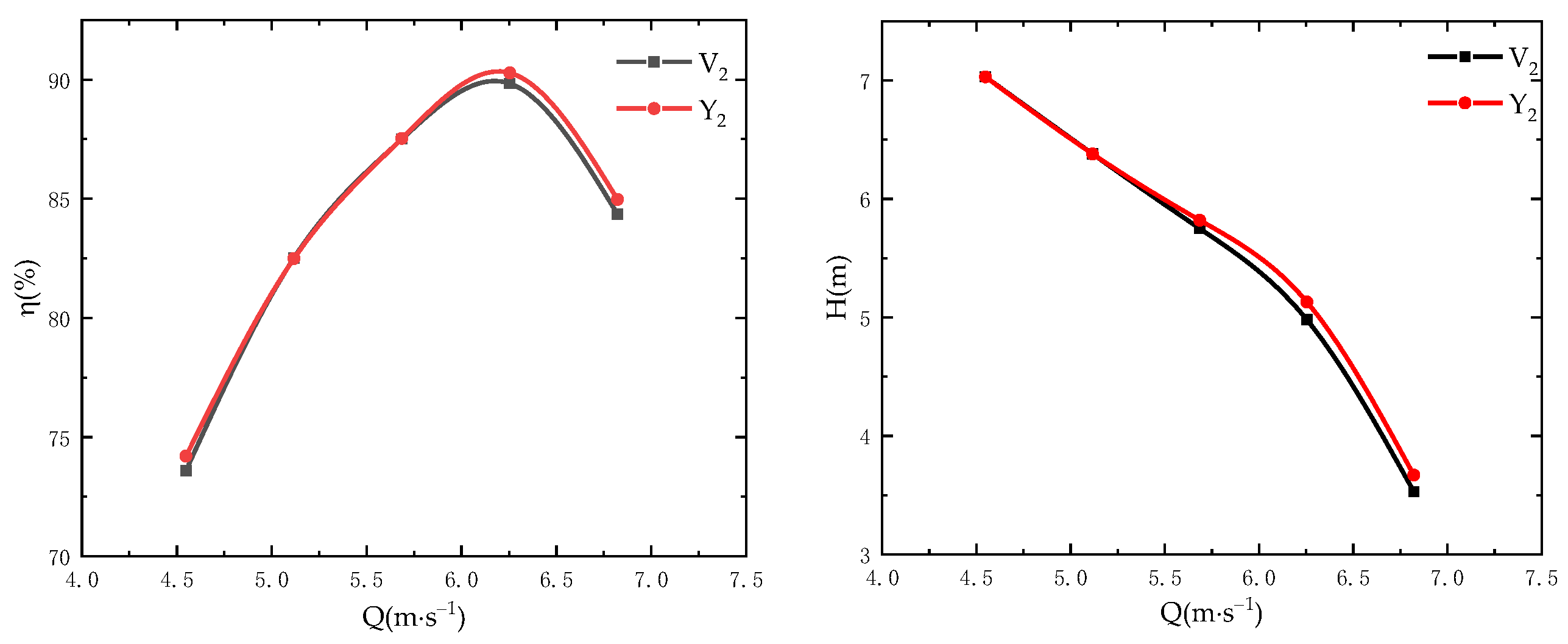
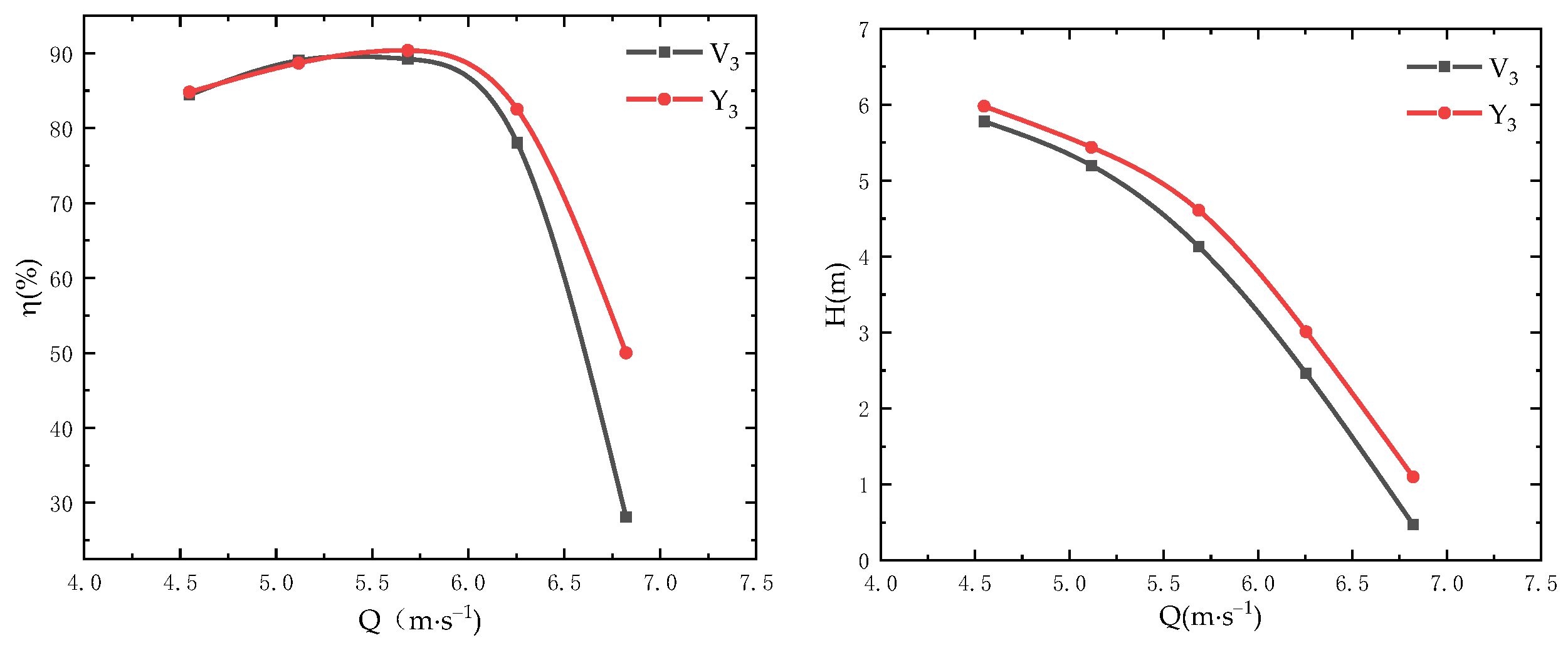
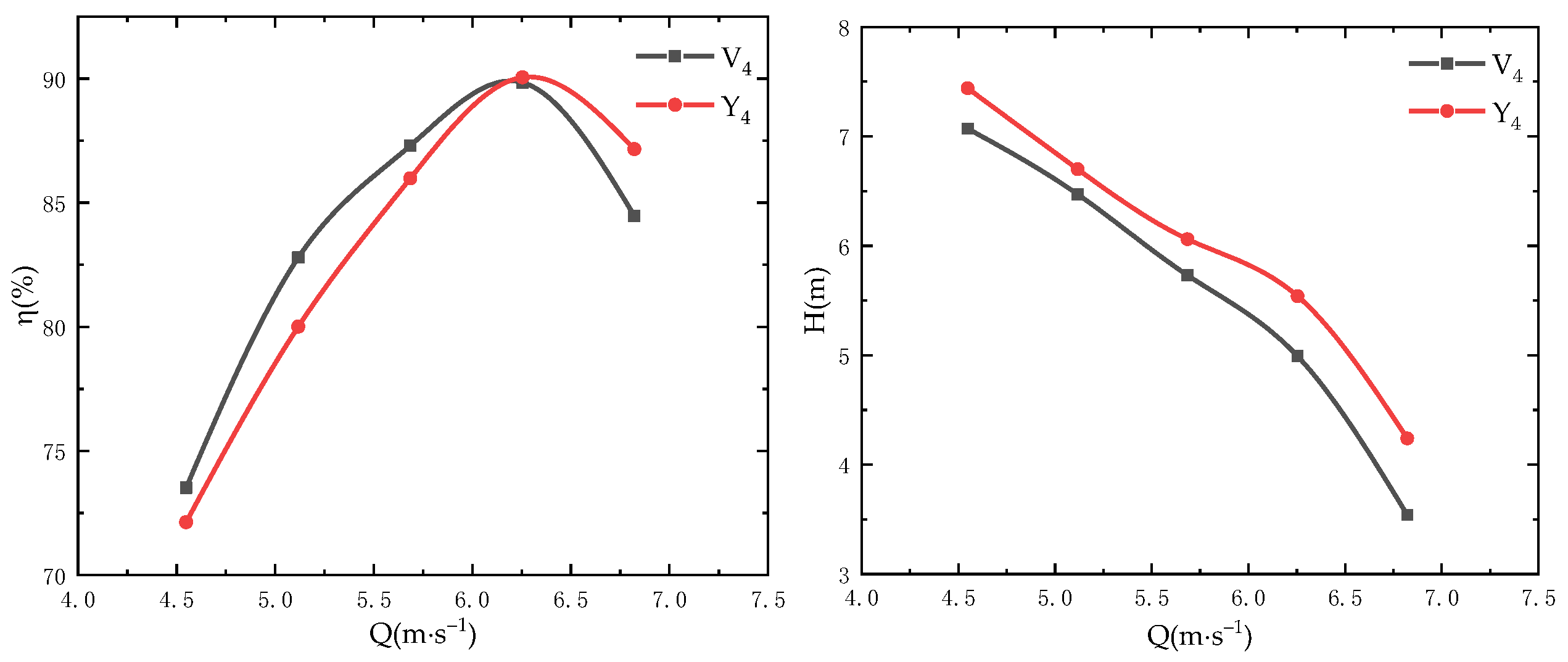
An analysis of external characteristic curves demonstrated consistent efficiency and head trends between both angle-adjustment strategies under identical angular configurations across flow regimes, validating the feasibility of torsion-based modulation. The results demonstrate that compared with rigid blades, the efficiency of variable torsion blades is not significantly improved under low-flow conditions. Under high-flow conditions (1.2 Q), the torsion-based angle-adjustment strategy exhibits superior efficiency across all four angular positions: −1° configuration: 11.1% efficiency improvement; +1° configuration: comparable efficiency; −2° configuration: 7.8% efficiency improvement; and +2° configuration: 3.2% efficiency improvement. The differential efficiency enhancements across the four angular groups confirm the superior hydraulic performance of variable-twist blades, particularly under high-flow conditions.

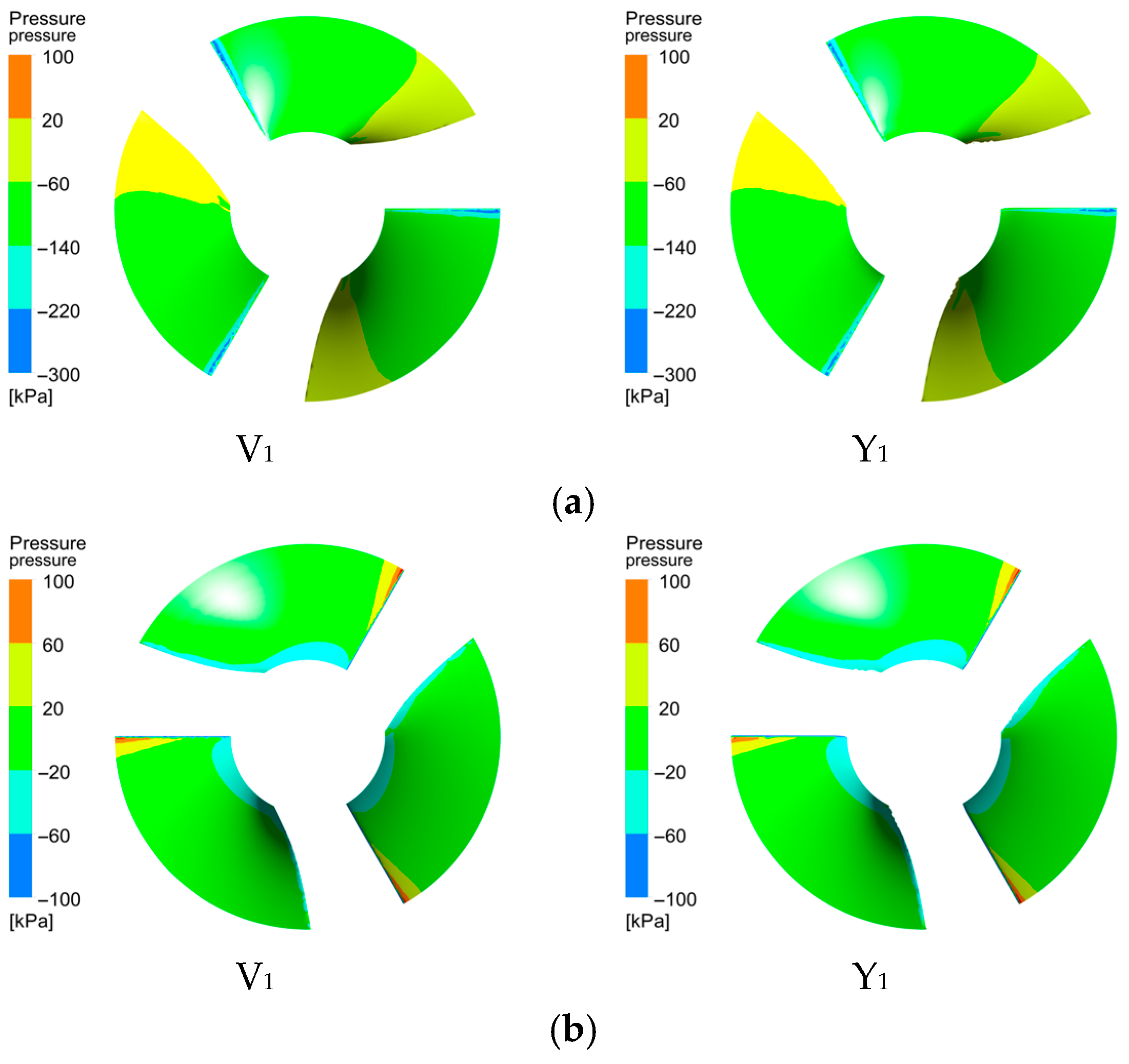
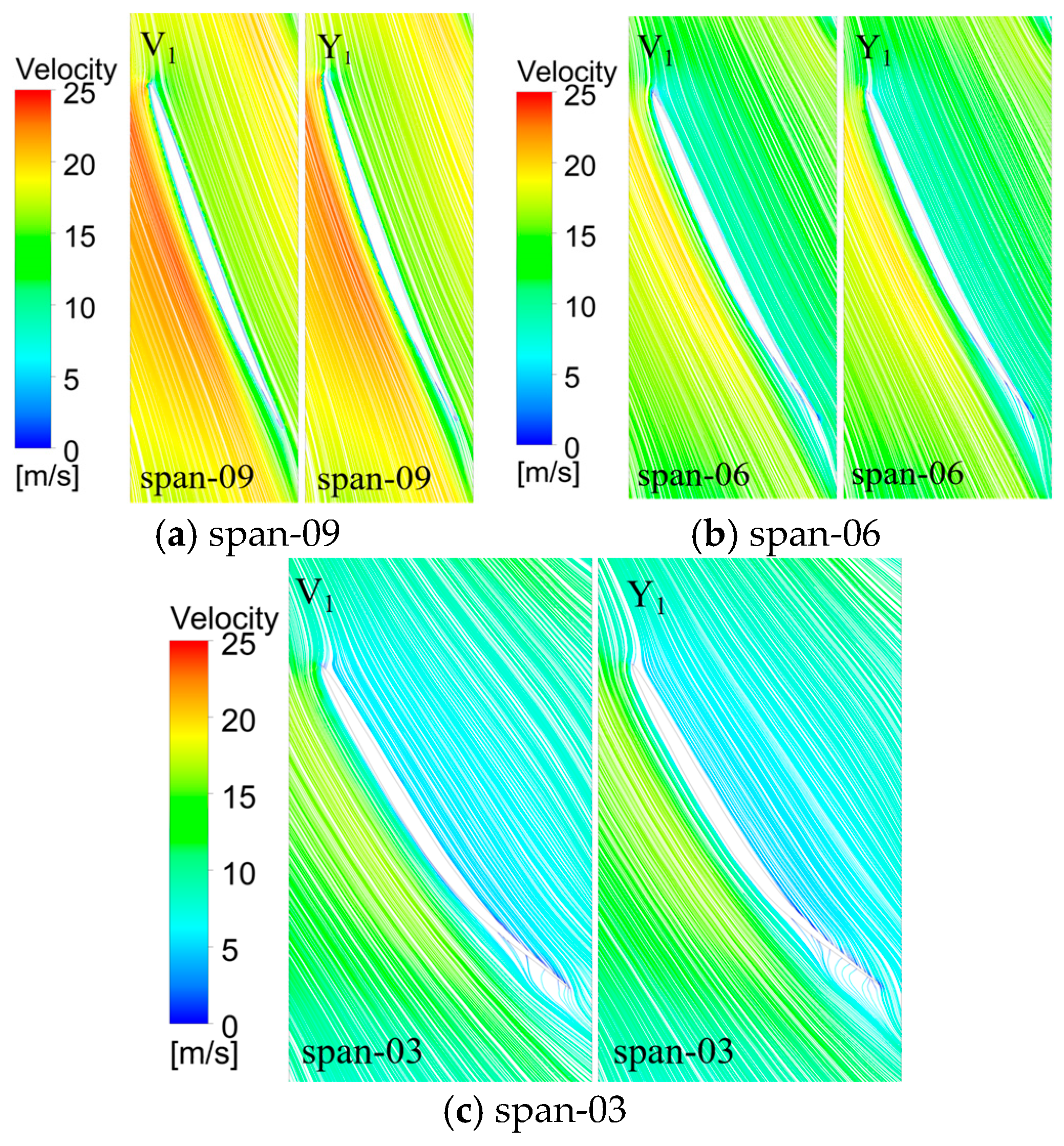
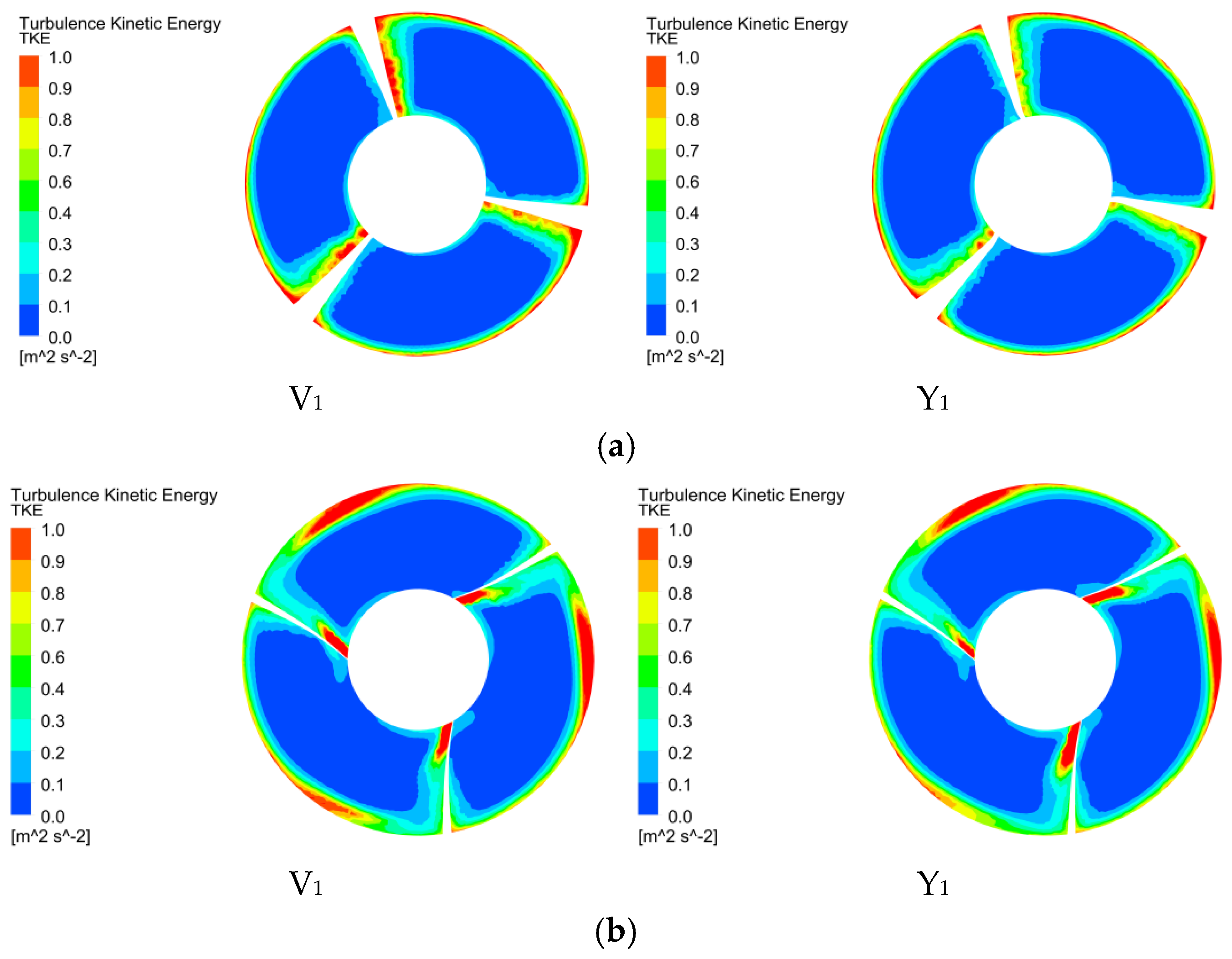
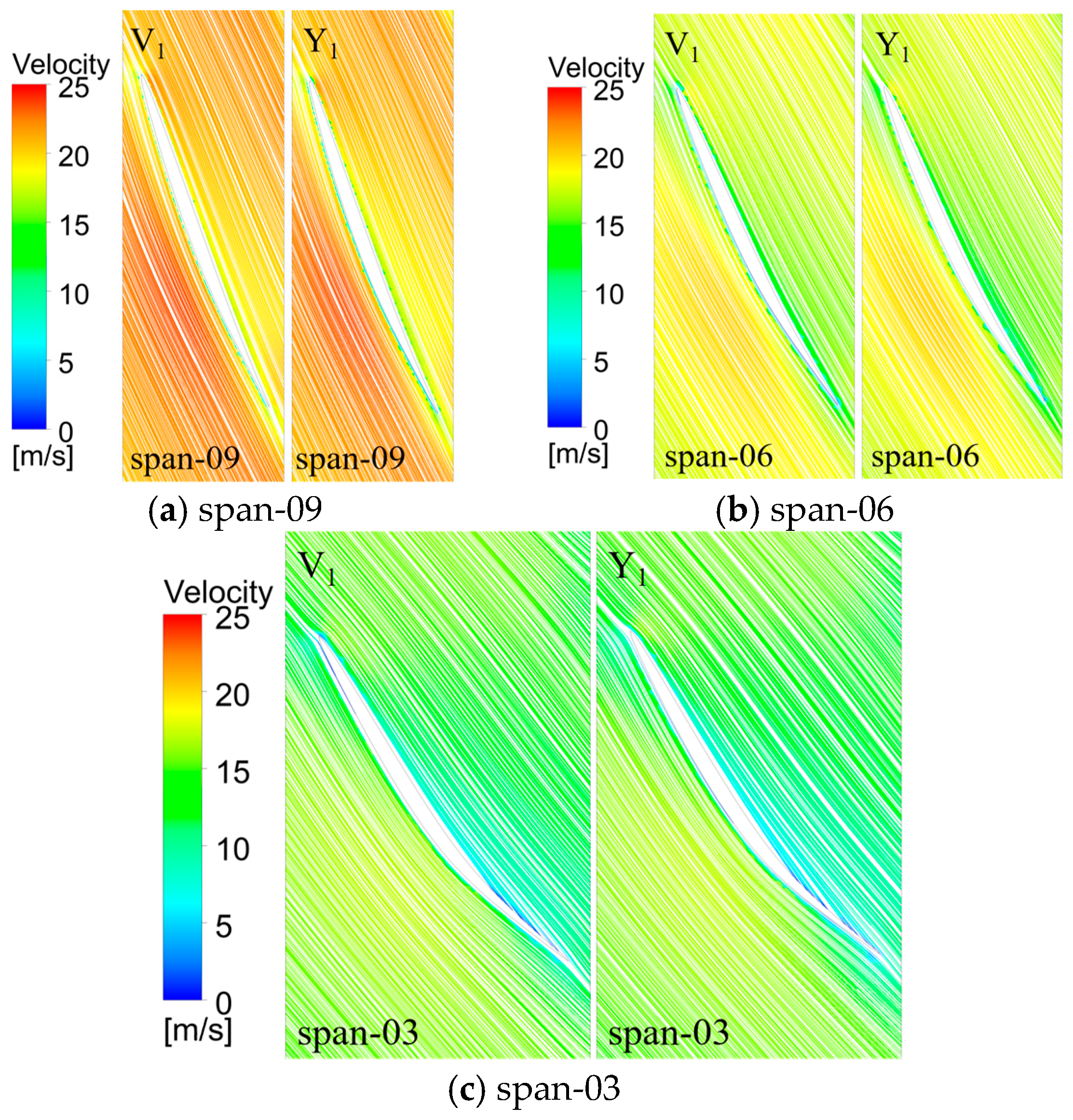
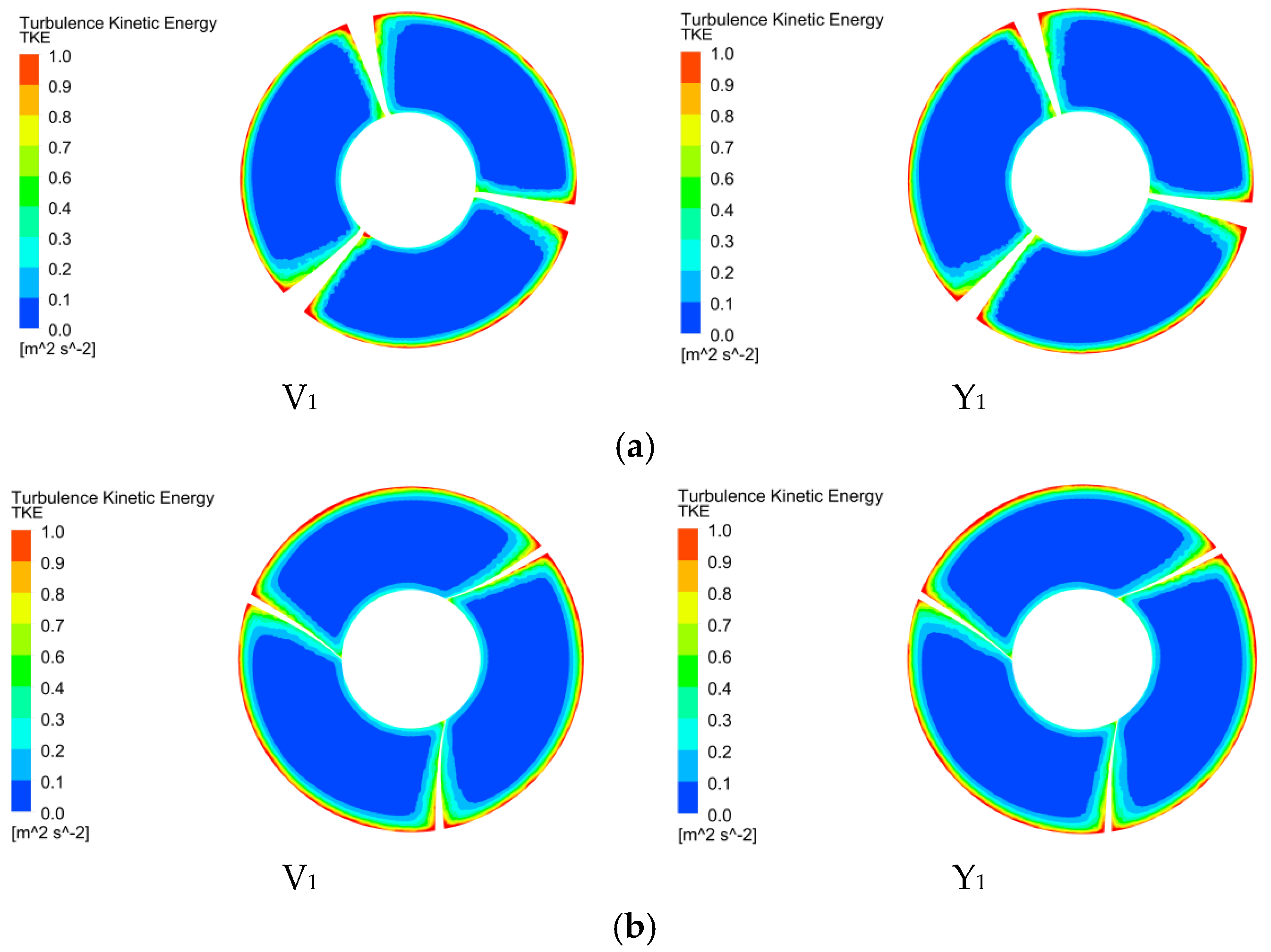
An internal flow analysis demonstrated that under the 0.8 Q condition, the variable-twist blade configuration optimizes inflow alignment at the impeller inlet and outflow uniformity in the tip region compared to rigid-blade counterparts, reducing turbulent losses at the impeller’s inlet/outlet zones. Meanwhile, under the 1.2 Q condition, it enhances hub-side and mid-span inflow attachment while suppressing turbulent kinetic energy dissipation at the discharge, thus proving that torsion-based blade modulation effectively improves hydrodynamic stability and energy transfer efficiency within the impeller domain.

Building upon the present research framework, future investigations should examine the effects of variable-twist blades on cavitation characteristics and pressure pulsation behavior within the impeller domain under diverse operating conditions. Additionally, expanding the range of torsional deformation angles for variable-twist blades would enable a comprehensive evaluation of broader angular variations on overall hydraulic performance.