Ionic Speciation of Ecotoxic Lead (2+), Cadmium (2+), and Naturally Occurring Ions with Dissolved Organic Matter in Seawater from the Bay of Bengal by Differential Pulse Anodic Stripping Voltammetry, Continuous Binding Model, and Computational Chemical Equilibria: Effect of Global Warming
Abstract
1. Introduction
2. Continuous Binding Model for Metal Speciation with Dissolved Organic Matter (DOM)
3. Material and Methods
3.1. Methodology and Instrumentation
3.2. Electrode Preparation
3.3. Reagents
3.4. Seawater Collection and Storage
3.5. Analytical Procedures
3.6. Computational Ionic Speciation Calculation by Minimization of Total Equilibrium Activity (MINTEQA) Model
4. Results and Discussion
4.1. DPASV Analysis of Cd2+ and Pb2+ in BoB Water
| Location | Metal Ion (M2+) | Free Metal Ion [Mn+] | Metal Binding Capacity (nM) | Conditional Stability Constant, (log K, M−1) | Method |
|---|---|---|---|---|---|
| Central North Pacific [8] | Cd | 20 fM (surface) 22 pM (600 m) | 0.1 nM (surface to 175 m) | 12.0 | DPASV |
| North Pacific and Southern Atlantic Ocean [30] | Cd | 0.864 pM | L2: 0.147 L1: ND | L1: 11.5 ± 0.7 L2: 10.2 ± 0.2 | AdCSV |
| Southern Yellow and Bohai Seas, China [9] | Cd | 0.8–4.0 pM | CdL: 0.38–0.58 | 10.8–12.4 | ICP-MS and ASV (HMDE) |
| Narragansett Bay Estuary, USA [31] | Pb | 0.4–1.0 pM | 0.60–1.0 | 9.6–10.4 | ASV |
| San Francisco Bay [32] | Pb | 0·3 pM | L1 = 0·89 ± 0·35 L2 = 12·8 ± 2 | PbL1: 10.5 ± 3 PbL2: 10.6 ± 4 | DPASV (TMF-RGCDE) |
| Southern Yellow and Bohai Seas, China [8] | Pb | 0.2–2 pM | 0.052–10 | 9.6–10.4 | ICP-MS DPASV (HMDE) |
| Eastern North Pacific [33] | Pb | ~0.4 pM | 0.2 and 0.5 | 9.7 | DPASV |
| Bay of Bengal, Bangladesh (This work) | Pb | 22.5 μg L−1 | L1 = 130 ± 2 L2 = 319 ± 4 | PbL1: 8.7 ± 0.4 PbL2: 7.2 | DPASV- GCTMF |
| Bay of Bengal, Bangladesh (This work) | Cd | <1 μg L−1 | L1 = 63 ± 4 L2 = 3.4 ± 0.6 | CdL1: 7.3 ± 0.4 CdL2: 7.3 | DPASV- GCTMF |
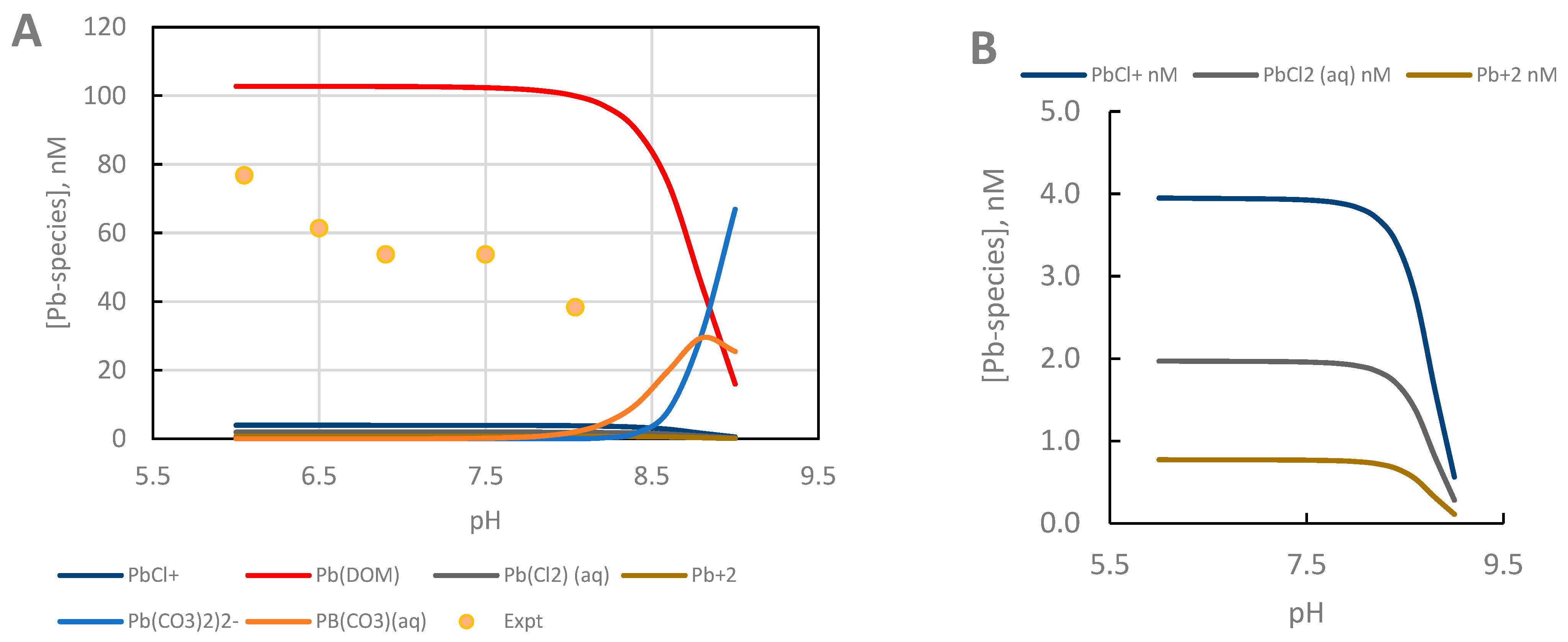
4.2. Speciation of Metal Ions in BoB Water and Bioavailable Fraction of PTE
4.3. General Input Parameters for MINTEQA
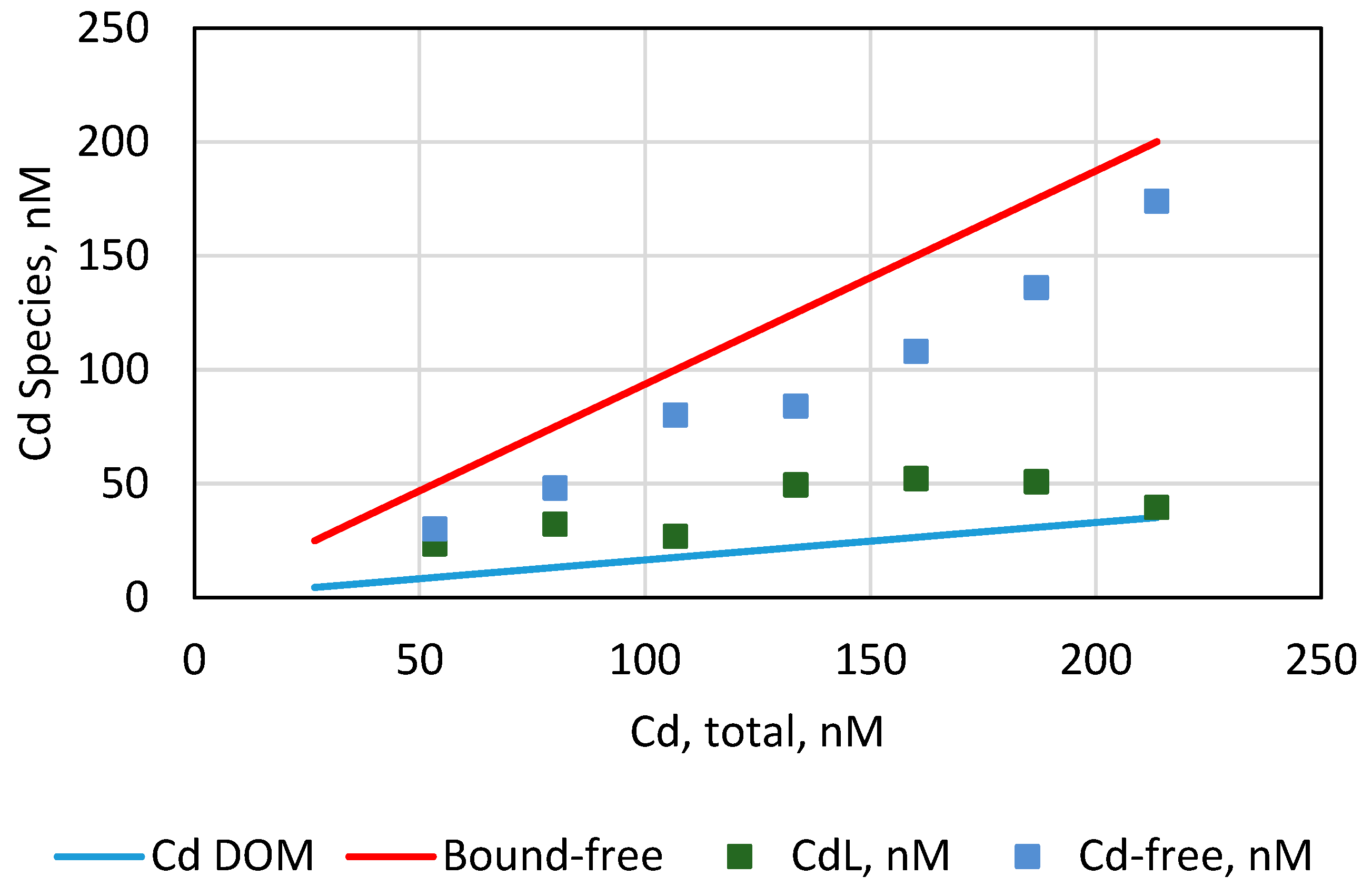
4.4. Cd2+ Speciation
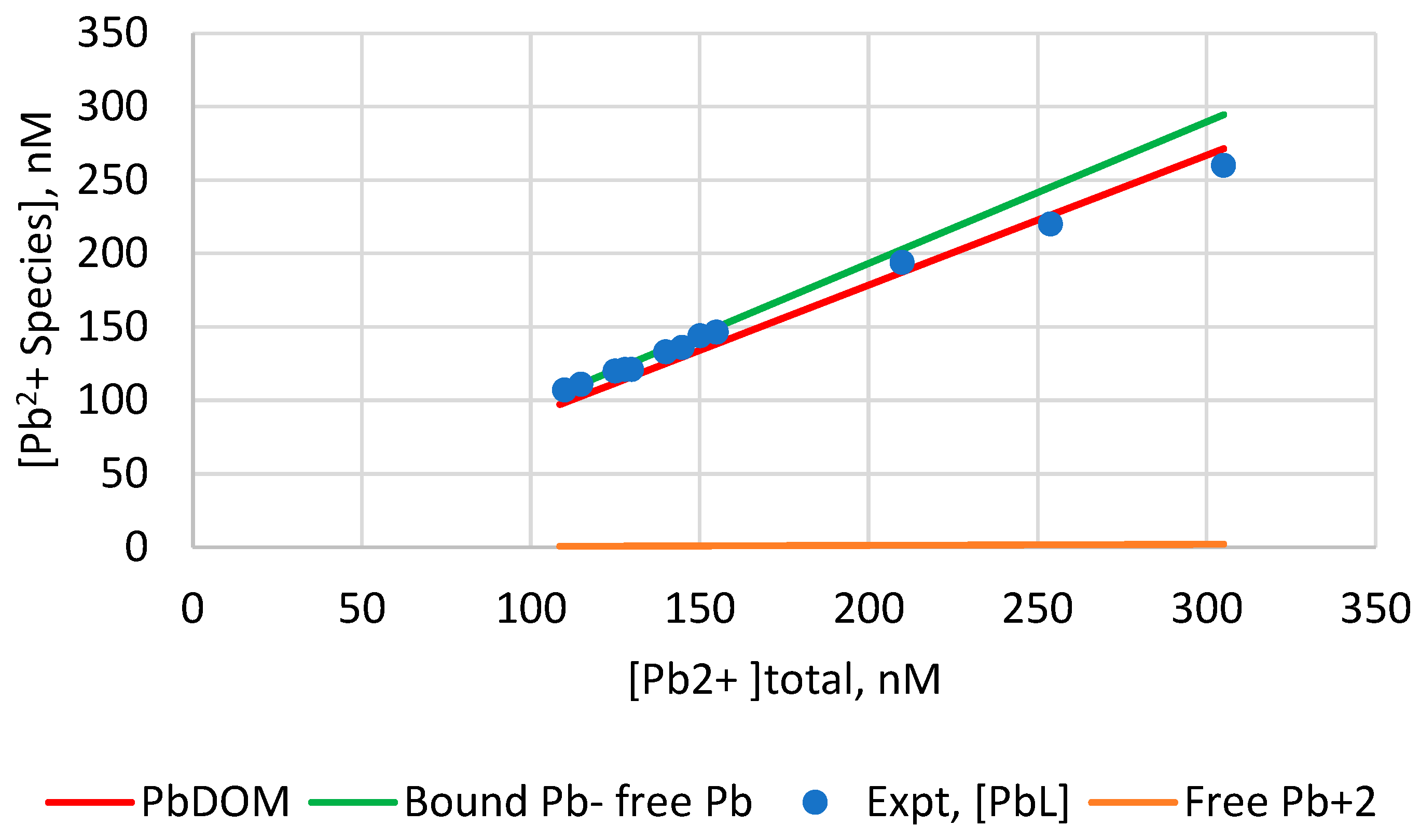
4.5. Pb2+ Speciation
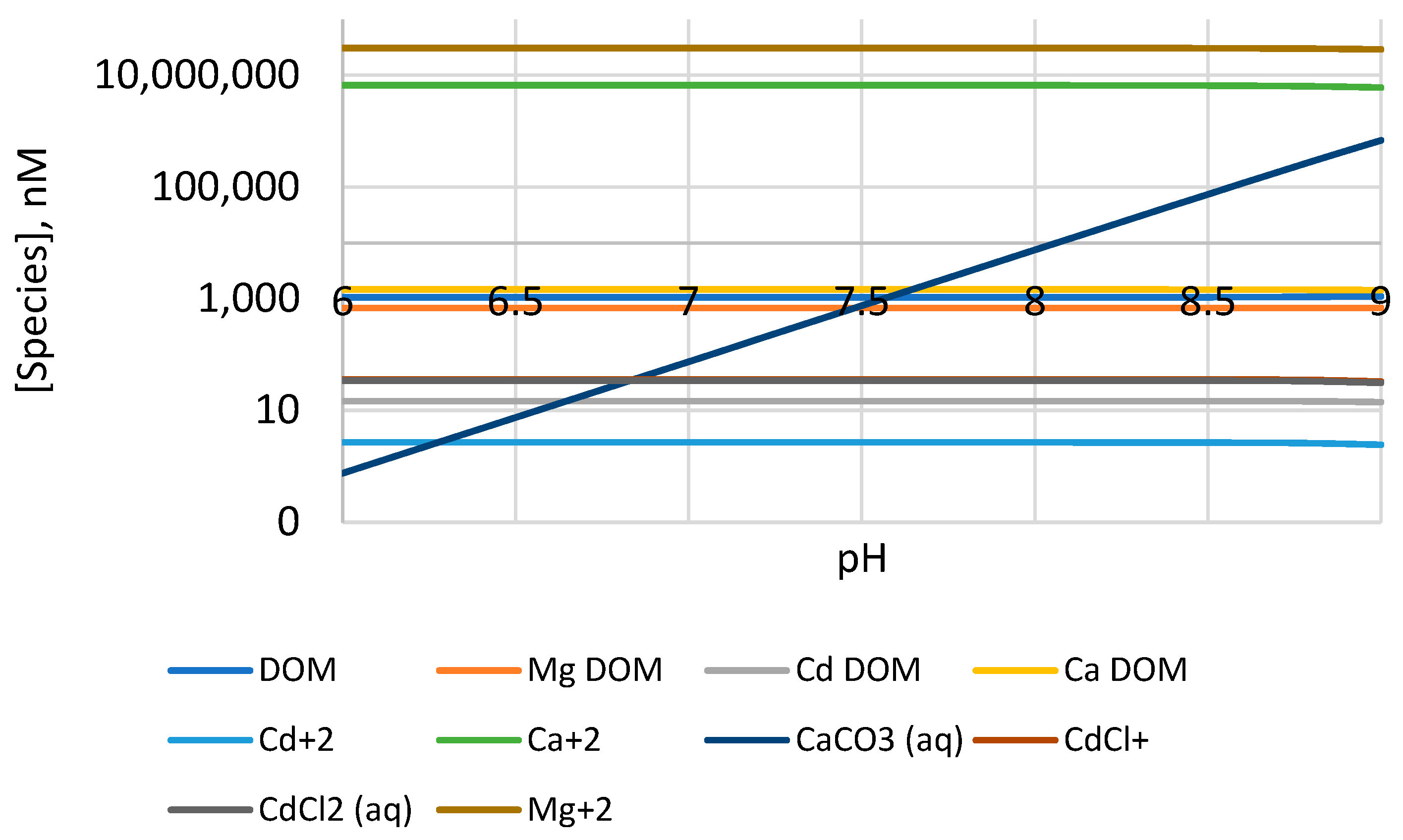
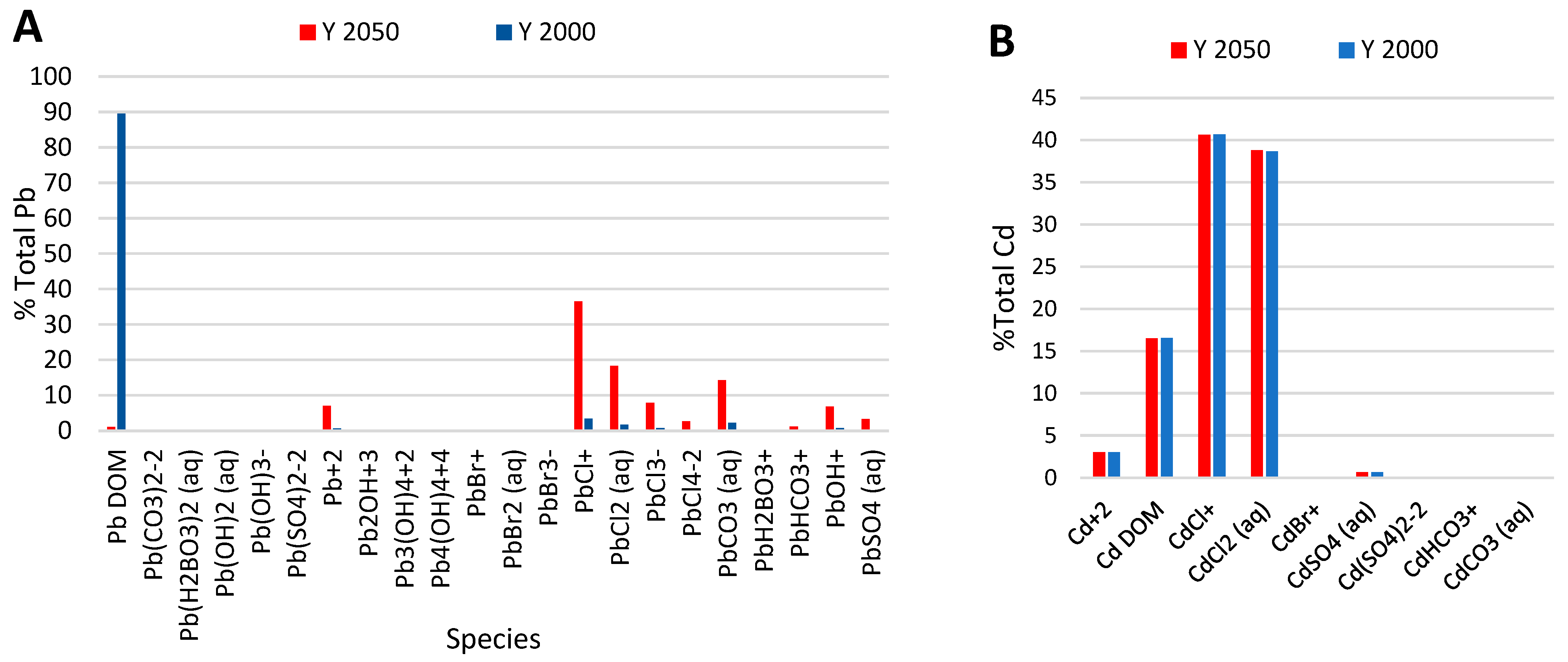
4.6. Effect of Global Warming on the Speciation of Pb2+ and Cd2+ in BoB
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Stockdale, A.; Tipping, E.; Lofts, S.; Mortimer, R.J.G. Effect of Ocean Acidification on Organic and Inorganic Speciation of Trace Metals. Environ. Sci. Technol. 2016, 50, 1906–1913. [Google Scholar] [CrossRef] [PubMed]
- Batley, G.E.; Apte, S.C.; Stauber, J.L. A Speciation and Bioavailability of Trace Metals in Water: Progress Since 1982. Aust. J. Chem. 2004, 57, 903–919. [Google Scholar] [CrossRef]
- Allen, H.E.; Hansen, D.J. The Importance of Trace Metal Speciation to Water Quality Criteria. Environ. Res. 1996, 68, 42–54. [Google Scholar] [CrossRef]
- Morel, F.M.M. Trace Metals–Phytoplankton Interactions: An Overview. Oceanogr. Ser. 1986, 43, 177–189. [Google Scholar] [CrossRef]
- Mahowald, N.M.; Hamilton, D.S.; Katherine, R.M.; Mackey, J.; Moore, K.; Baker, A.R.; Scanza, R.A.; Zhang, Y. Aerosol trace metal leaching and impacts on marine microorganisms. Nat. Commun. 2018, 9, 2614. [Google Scholar] [CrossRef]
- Aiken, G.R.; Hsu-Kim, H.; Ryan, J.N. Influence of Dissolved Organic Matter on the Environmental Fate of Metals, Nanoparticles, and Colloids. Environ. Sci. Technol. 2011, 45, 3196–3201. [Google Scholar] [CrossRef]
- Van Den Berg, C.M.G. Determination of The Complexing Capacity and Conditional Stability Constants of Complexes of Copper (II) with Natural Organic Ligands in Seawater by Cathodic Stripping Voltammetry of Copper—Catechol Complex Ions. Mar. Chem. 1984, 15, 1–18. [Google Scholar] [CrossRef]
- Bruland, K.W. Complexation of cadmium by natural organic ligands in the central North Pacific. Limnol. Oceanogr. 1992, 37, 1008–1017. [Google Scholar] [CrossRef]
- Ren, Y.; Wang, X.; Zhang, C.; Liu, J.; Shi, X.; Li, L. The distribution and speciation of dissolved Cd and Pb in the Bohai Sea and Yellow Sea, China. Mar. Pollut. Bull. 2023, 186, 114437. [Google Scholar] [CrossRef]
- Velasquez, I.B.; Jacinto, G.S.; Valera, F.S. The speciation of dissolved copper, cadmium and zinc in Manila Bay, Philippines. Mar. Pollut. Bull. 2002, 45, 210–217. [Google Scholar] [CrossRef]
- Srichandan, S.; Panigrahy, R.C.; Baliarsingh, S.K.; Rao, S.B.; Pati, P.; Sahu, B.K.; Sahu, K.C. Distribution of trace metals in surface seawater and zooplankton of the Bay of Bengal, off Rushikulya estuary, East Coast of India. Mar. Pollut. Bull. 2016, 111, 468–475. [Google Scholar] [CrossRef]
- Khan, M.Z.H.; Hasan, M.R.; Khan, M.; Aktar, S.; Fatema, K. Distribution of Heavy Metals in Surface Sediments of the Bay of Bengal Coast. J. Toxicol. 2017, 2017, 9235764. [Google Scholar] [CrossRef] [PubMed]
- Ikhsani, I.U.; Wong, K.H.; Kim, T.; Mashio, A.; Norisuye, K.; Obata, H. Biogeochemistry of dissolved trace metals in the Bay of Bengal. Mar. Chem. 2024, 262, 104394. [Google Scholar] [CrossRef]
- Kibria, G.; Hossain, M.M.M.; Mallick, D.; Lau, T.C.; Wu, R. Trace/Heavy Metal Pollution Monitoring in Estuary and Coastal Area of the Bay of Bengal, Bangladesh and Implicated Impacts. Mar. Pollut. Bull. 2016, 105, 2016. [Google Scholar] [CrossRef] [PubMed]
- Dash, D.R.; Dash, S.; Patro, S.K.; Tripathy, K.S.; Sahu, B.K. Speciation of Zinc in Surface Waters of The Rushikulya Estuary (Bay of Bengal). J. Mar. Biol. Ass. India 1997, 39, 33–39. [Google Scholar]
- Nabi, M. Interaction of Lead (Pb) and Cadmium (Cd) with Hydroxide and Bicarbonate Anions and Dissolved Organics in Seawater: An Electrochemical Approach. Master’s Dissertation, Department of Chemistry, University of Dhaka, Dhaka, Bangladesh, 2000. [Google Scholar]
- Milliman, J.D.; Meade, R.H. World-wide delivery of river sediment to the oceans. J. Geol. 1983, 91, 1–21. [Google Scholar] [CrossRef]
- Chakraborty, P.; Babu, P.V.R.; Sarma, V.V. A study of lead and cadmium speciation in some estuarine and coastal sediments. Chem. Geol. 2012, 294, 217–225. [Google Scholar] [CrossRef]
- BOBLME: Country Report on Pollution in the BOBLME-Bangladesh. BOBLME Ecology-01, 2011. Available online: http://www.boblme.org/documentRepository/BOBLME-2011-Ecology-01.pdf (accessed on 17 July 2024).
- Zhang, H.; Davison, W. Direct in situ measurements of labile inorganic and organically bound metal species in synthetic solutions and natural waters using diffusive gradients in thin films. Anal. Chem. 2000, 72, 4447–4457. [Google Scholar] [CrossRef]
- Buffle, J. Complexation Reactions in Aquatic Systems; An Analytical Approach; Ellis Horwood: Chichester, UK, 1988; pp. 203–205, 221. [Google Scholar]
- Bard, A.J.; Faulkner, L.R. Electrochemical Methods: Fundamentals and Applications, 2nd ed.; Wiley & Sons: Hoboken, NJ, USA, 2000; pp. 286–458. [Google Scholar]
- Chapman, K. The Toxic Tide of Ship Breaking. Chemistry World, 21 February 2022. Available online: https://www.chemistryworld.com/features/the-toxic-tide-of-ship-breaking/4015158.article (accessed on 17 July 2024).
- Mota, A.M.; Pinheiro, J.P.; Gonçalves, M.L.S. Electrochemical Methods for Speciation of Trace Elements in Marine Waters: Dynamic Aspects. J. Phys. Chem. A 2012, 116, 6433–6442. [Google Scholar] [CrossRef][Green Version]
- Felmy, A.R.; Girvin, D.C.; Jenne, E.A. MINTEQ—A Computer Program for Calculating Aqueous Geochemical Equilibria, EPA-600/3-84-031; U.S. Environmental Protection Agency: Athens, GA, USA, 1984. [Google Scholar]
- Capodaglio, G.; Coale, K.H.; Bruland, K.W. Lead speciation in surface waters of the eastern North Pacific. Mar. Chem. 1990, 29, 221–233. [Google Scholar] [CrossRef]
- Stumm, W.; Morgan, J.J. Aquatic Chemistry; Jon Wiley and Sons Inc.: Hoboken, NJ, USA, 1981; p. 508. [Google Scholar]
- Hansell, D.A.; Orellana, M.V. Dissolved Organic Matter in the Global Ocean: A Primer. Gels 2021, 7, 128. [Google Scholar] [CrossRef] [PubMed]
- Shah, C.; Sudheer, A.K.; Bhushan, R. Distribution of dissolved organic carbon in the Bay of Bengal: Influence of sediment discharge, fresh water flux, and productivity. Mar. Chem. 2018, 203, 91–101. [Google Scholar] [CrossRef]
- Carrasco, G.G. Concentrations, Distributions and Chemical Speciation of Zinc and Cadmium in the Equatorial and South Atlantic Ocean. Ph.D. Thesis, Ocean & Earth Sciences, Old Dominion University, Virginia Beach, VA, USA, 2010. [Google Scholar] [CrossRef]
- Buckley, P.J.M.; Van Den Berg, C.M.G. Copper Complexation Profiles in The Atlantic Ocean: A comparative study using electrochemical and ion exchange techniques. Mar. Chem. 1986, 19, 281–296. [Google Scholar] [CrossRef]
- Kozelka, P.B.; Bruland, K.W. Chemical speciation of dissolved Cu, Zn, Cd, Pb in Narragansett Bay, Rhode Island. Mar. Chem. 1998, 60, 267–282. [Google Scholar] [CrossRef]
- Kozelka, P.B.; Sañudo-Wilhelmy, S.; Flegal, A.R.; Bruland, K.W. Physicochemical speciation of lead in South SanFrancisco Bay. Estuar. Coast. Shelf Sci. 2023, 44, 649–658. [Google Scholar] [CrossRef]
- Diviš, P.; Dočekalová, H.; Brulík, L.; Pavliš, M.; Hekera, P. Use of the Diffusive Gradients in Thin Films Technique to Evaluate (Bio)available Trace Metal Concentrations in River Water. Anal. Bioanal. Chem. 2007, 387, 2239–2244. [Google Scholar] [CrossRef]
- Sasamal, S.K. High saline waters in Bay of Bengal. Proc. Indian Acad. Sci. (Earth Planet. Sci.) 1990, 99, 367–381. [Google Scholar] [CrossRef]
- Available online: http://www.seafriends.org.nz/oceano/seawater.htm (accessed on 17 July 2024).
- Grimm, D.M.; Azarraga, L.V.; Carreira, L.A.; Susetyo, W. Continuous mltilagand distribution model used to predict the stability constant of copper (II) metal complexation with humic materials from fluorescence quenching data. Environ. Sci. Technol. 1991, 25, 1427–1431. [Google Scholar] [CrossRef]
- Tuschall, J.R., Jr.; Brezonik, P.L. Evaluation of the Copper Anodic Stripping Voltammetry Complexometric Titration for Complexing Capacities and Conditional Stability Constants. Anal. Chem. 1981, 53, 1986–1989. [Google Scholar] [CrossRef]
- Sarma, V.V.S.S.; Krishna, M.S.; Srinivas, T.N.R.; Kumari, V.R.; Yadav, K.; Kumar, M.D. Elevated acidification rates due to deposition of atmospheric pollutants in the coastal Bay of Bengal. Geophys. Res. Lett. 2021, 48, e2021GL095159. [Google Scholar] [CrossRef]
- Stocker, T.F.; Qin, D.; Plattner, G.K.; Tignor, M.; Allen, S.K.; Boschung, J.; Nauels, A.; Xia, Y.; Bex, V.; Midgley, P.M. (Eds.) The Physical Science Basis. Contribution of Working Group I to the Fifth Assessment Report of the Intergovernmental Panel on Climate Change; Cambridge University Press: Cambridge, UK; New York, NY, USA, 2013; Available online: https://www.ipcc.ch/report/ar5/syr/ (accessed on 17 July 2024).
- Panchang, N.; Ambokar, M. Ocean acidification in the Northern Indian ocean: A review. J. Asian Earth Sci. 2021, 219, 104904. [Google Scholar] [CrossRef]
- Millero, F.J.; Woosley, R.; Ditrolio, B.; Waters, J. Effect of ocean acidification on the speciation of metals in seawater. Oceanography 2009, 22, 72–84. [Google Scholar] [CrossRef]
- Sarker, S.; Panassa, E.; Hossain, M.S.; Chowdhury, S.R.; Yadav, A.K.; Sharifuzzaman, S.M. A bio-physicochemical perspective of the Bay of Bengal. J. Mar. Biol. Assoc. UK 2020, 100, 517–528. [Google Scholar] [CrossRef]


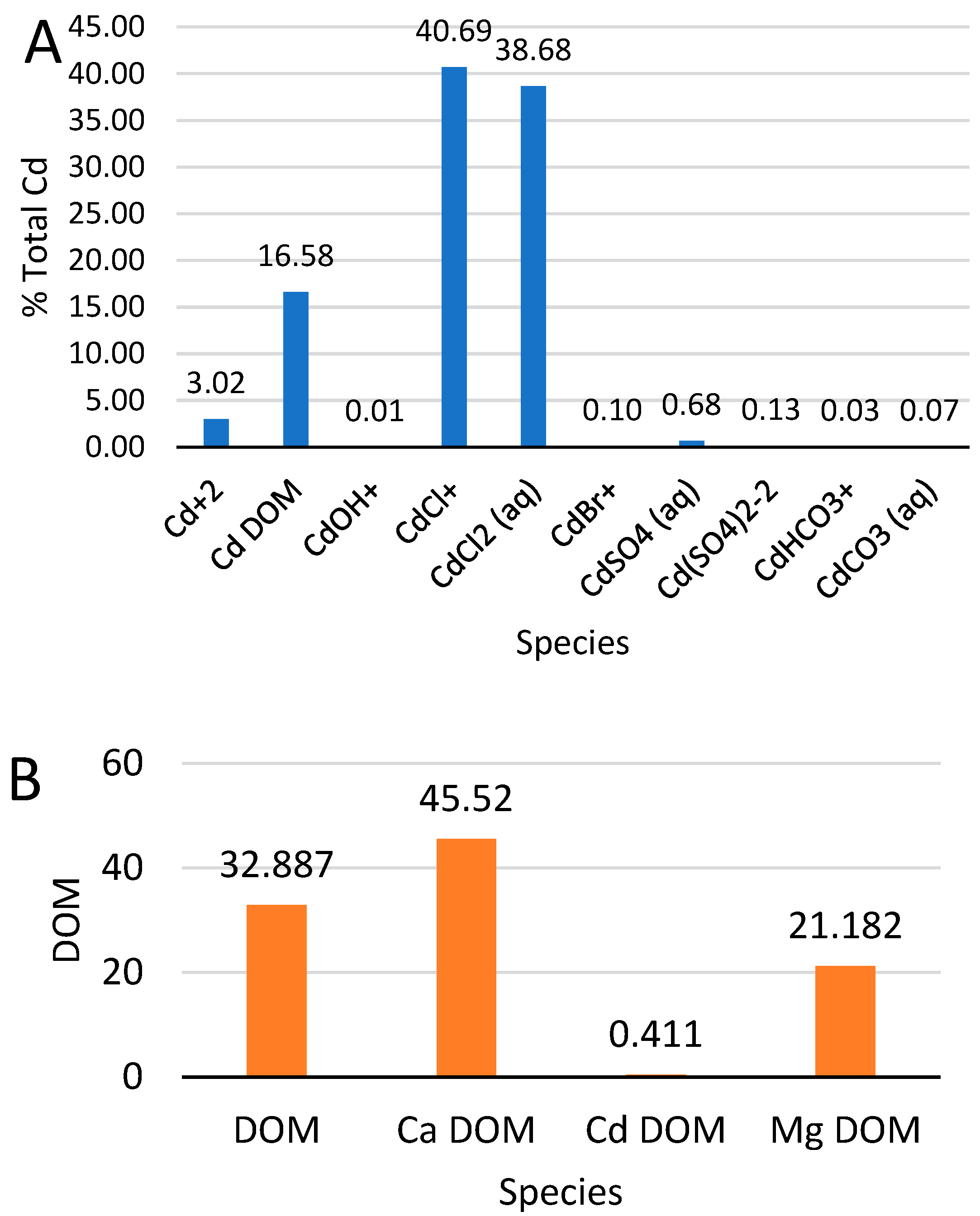

| Model | Cd2+ | Pb2+ |
|---|---|---|
| One site (n = 34) | K1 = (1.94 ± 0.2) × 107 M−1 log K1 = 7.28 L1 = 66.4 ± 4.0 nM R2 = 0.92 | K1 = (1.1 ± 0.06) × 108 M−1 log K1 = 8.04 L1 = 293 ± 7 nM R2 = 0.97 |
| Two sites (n = 33) | K1 = (1.94 ± 0.2) × 107 M−1 log K1 = 7.28 L1 = 63.0 ± 4 nM K2 = (1.95 ± 0.6) × 107 M−1 log K2 = 7.29 L2 = 3.4 ± 0.6 nM R2 = 0.98 | K1 = (4.89 ± 0.1) × 108 M−1 log K1 = 8.69 L1 = 130 ± 2 nM K2 = (1.56 ± 0.04) × 107 M−1 log K2 = 7.19 L2 = 319 ± 4 nM R2 = 0.99 |
| Components and Physical Parameters | Values | Units |
|---|---|---|
| Cl− | 546 | mmolal |
| Na+ | 468 | mmolal |
| SO42− | 28.1 | mmolal |
| Mg2+ | 53.3 | mmolal |
| Ca2+ | 10.4 | mmolal |
| K+ | 10 | mmolal |
| Br− | 0.83 | mmolal |
| H3BO3 | 0.46 | mmolal |
| Sr2+ | 0.09 | mmolal |
| Pb2+ | 23 | µg/L |
| Cd2+ | 9 | µg/L |
| pH | 8.08 | |
| Temperature | 25 | °C |
| Density | 1.033 | g/cm3 |
| Input Cd2+, | CdCl+ | CdCl2 (aq) | Cd-DOM | CdCO3 (aq) | CdHCO3+ | CdSO4 (aq) | Cd2+ | MINTEQA (Bound-Free) | Expt. | Expt. Cd2+ |
|---|---|---|---|---|---|---|---|---|---|---|
| Total, nM | Cd-DOM | |||||||||
| 26.7 | 10.9 | 10.3 | 4.4 | 0.02 | 0.01 | 0.18 | 0.8 | 25.0 | ||
| 53.4 | 21.7 | 20.6 | 8.9 | 0.04 | 0.01 | 0.36 | 1.6 | 50.0 | 23.4 | 30 |
| 80.1 | 32.6 | 31.0 | 13.3 | 0.06 | 0.02 | 0.55 | 2.4 | 75.0 | 32.1 | 48 |
| 106.8 | 43.5 | 41.3 | 17.7 | 0.07 | 0.03 | 0.73 | 3.2 | 100.0 | 26.8 | 80 |
| 133.5 | 54.3 | 51.7 | 22.1 | 0.09 | 0.03 | 0.91 | 4.0 | 125.1 | 49.4 | 84 |
| 160.2 | 65.2 | 62.0 | 26.5 | 0.11 | 0.04 | 1.09 | 4.8 | 150.1 | 52.1 | 108 |
| 186.8 | 76.1 | 72.3 | 30.8 | 0.13 | 0.05 | 1.28 | 5.7 | 175.1 | 50.8 | 136 |
| 213.5 | 87.0 | 82.7 | 35.2 | 0.15 | 0.06 | 1.46 | 6.5 | 200.1 | 39.5 | 174 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nabi, M.; Hussam, A.; Khan, A.H. Ionic Speciation of Ecotoxic Lead (2+), Cadmium (2+), and Naturally Occurring Ions with Dissolved Organic Matter in Seawater from the Bay of Bengal by Differential Pulse Anodic Stripping Voltammetry, Continuous Binding Model, and Computational Chemical Equilibria: Effect of Global Warming. Water 2025, 17, 1470. https://doi.org/10.3390/w17101470
Nabi M, Hussam A, Khan AH. Ionic Speciation of Ecotoxic Lead (2+), Cadmium (2+), and Naturally Occurring Ions with Dissolved Organic Matter in Seawater from the Bay of Bengal by Differential Pulse Anodic Stripping Voltammetry, Continuous Binding Model, and Computational Chemical Equilibria: Effect of Global Warming. Water. 2025; 17(10):1470. https://doi.org/10.3390/w17101470
Chicago/Turabian StyleNabi, Mahmudun, Abul Hussam, and Amir H. Khan. 2025. "Ionic Speciation of Ecotoxic Lead (2+), Cadmium (2+), and Naturally Occurring Ions with Dissolved Organic Matter in Seawater from the Bay of Bengal by Differential Pulse Anodic Stripping Voltammetry, Continuous Binding Model, and Computational Chemical Equilibria: Effect of Global Warming" Water 17, no. 10: 1470. https://doi.org/10.3390/w17101470
APA StyleNabi, M., Hussam, A., & Khan, A. H. (2025). Ionic Speciation of Ecotoxic Lead (2+), Cadmium (2+), and Naturally Occurring Ions with Dissolved Organic Matter in Seawater from the Bay of Bengal by Differential Pulse Anodic Stripping Voltammetry, Continuous Binding Model, and Computational Chemical Equilibria: Effect of Global Warming. Water, 17(10), 1470. https://doi.org/10.3390/w17101470








