Capillary Imbibition Laws of Fresh–Brackish Waters in Sandstone
Abstract
1. Introduction
2. Methodology
2.1. Sample Preparation
2.2. Experimental Design
- (1)
- Water imbibition mass and height of the water imbibition front measurements
- (2)
- NMR T2 spectra and stratified moisture distribution measurements
2.3. Test Theory of Nuclear Magnetic Resonance (NMR)
- (1)
- Measurement of T2 spectra
- (2)
- Measurement of the stratified moisture distribution
3. Observation of Capillary Imbibition
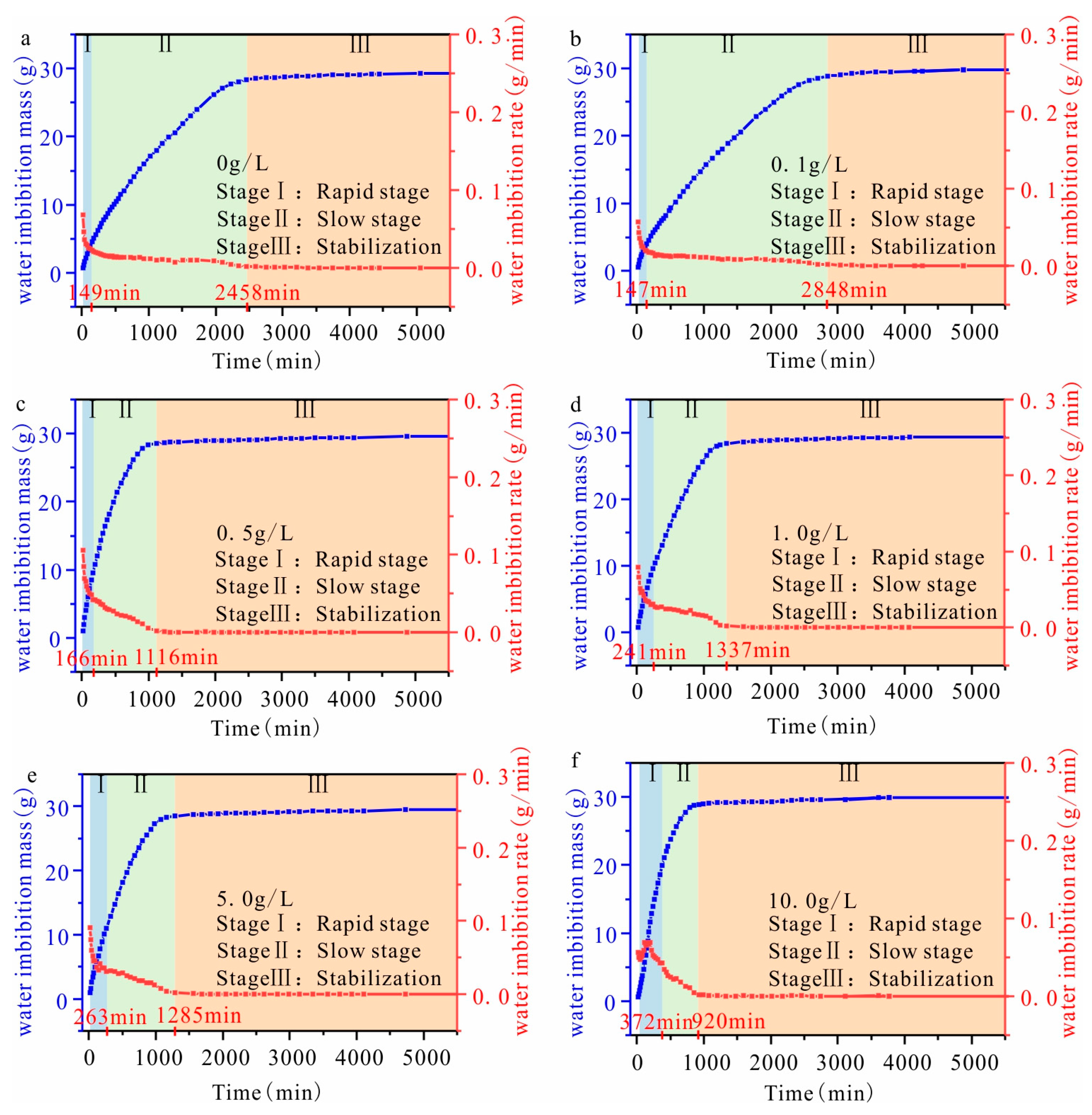
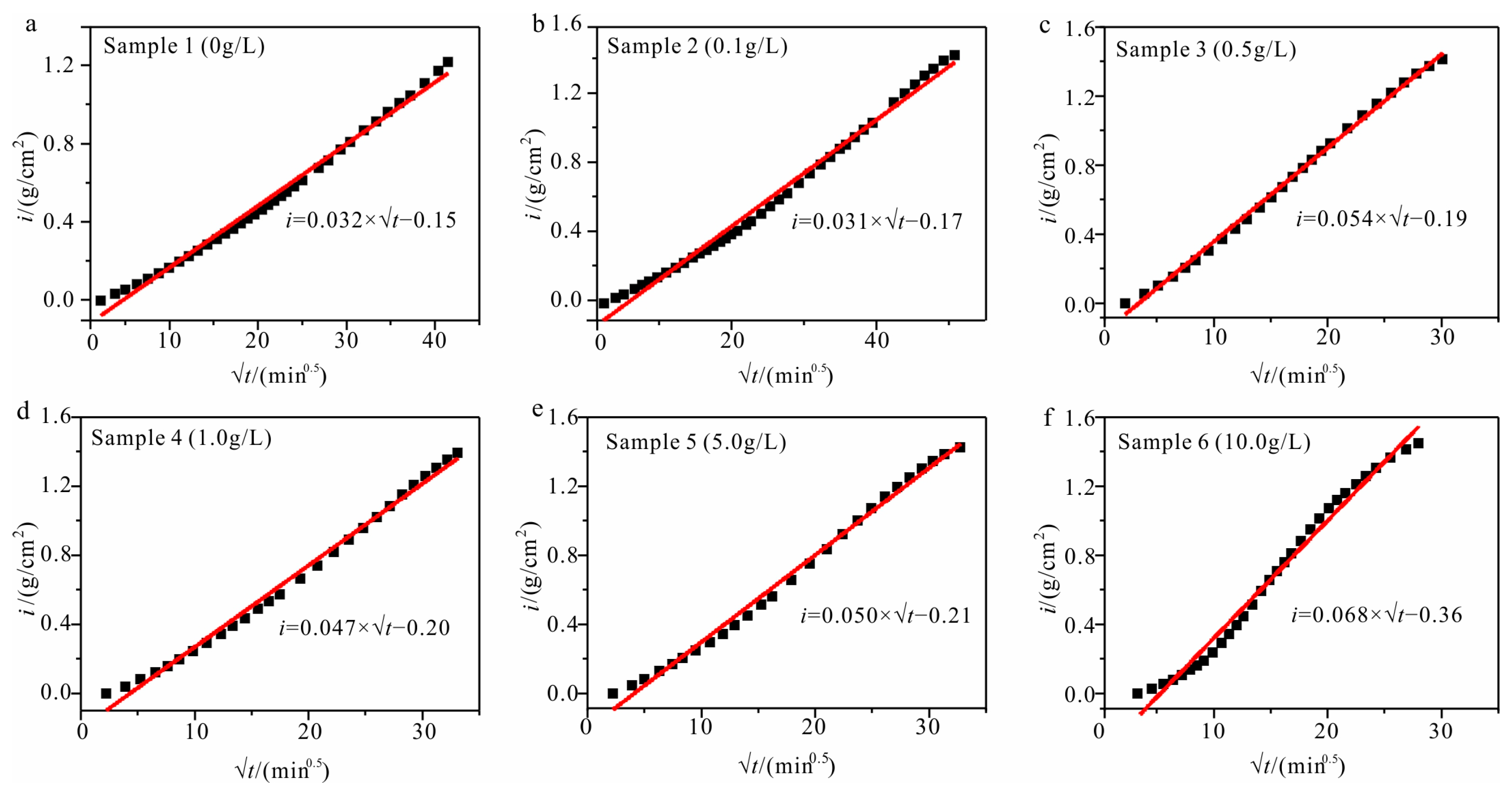
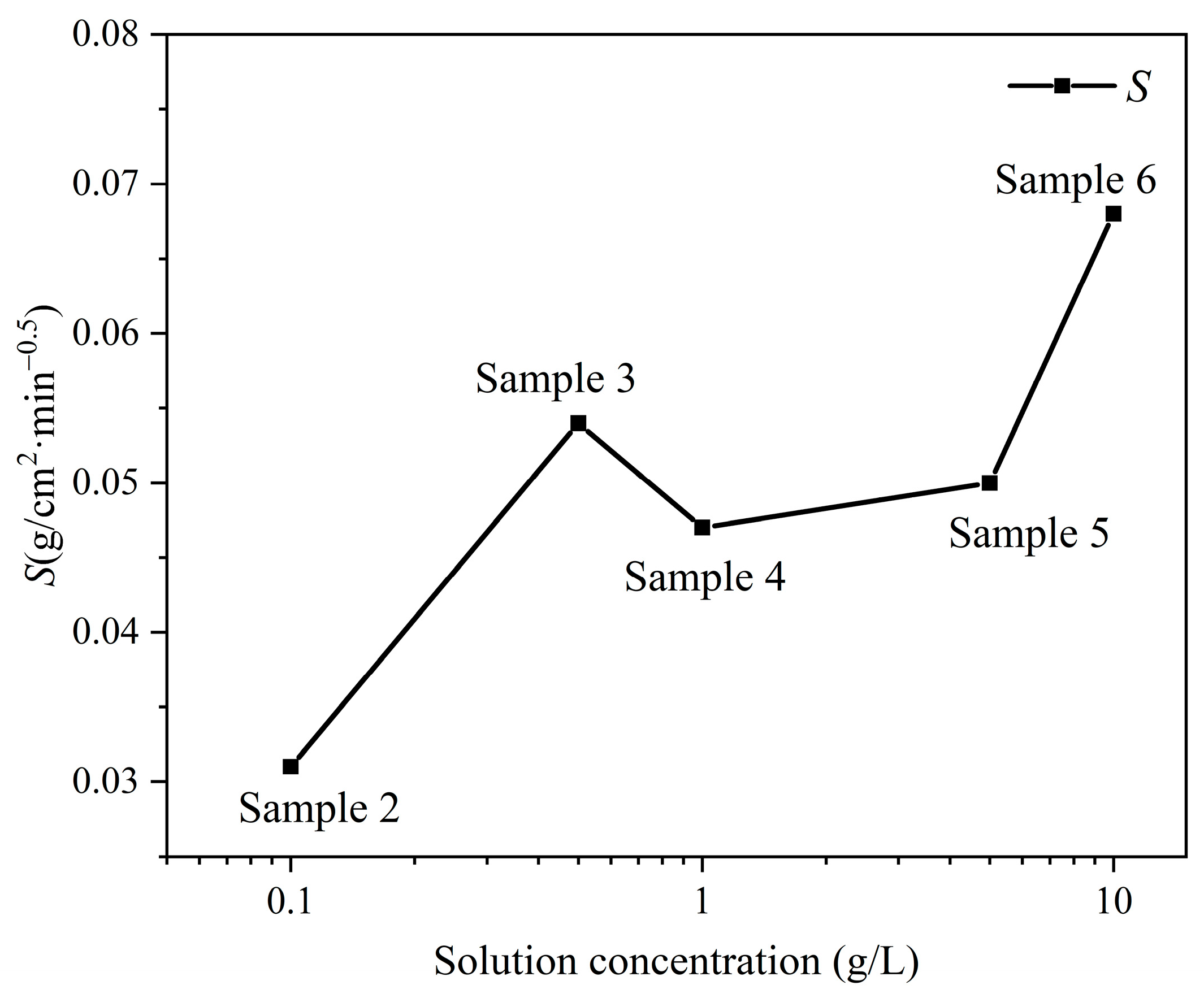
3.1. The Variation in Water Imbibition Mass and Sorptivity S
- (1)
- Water imbibition mass
- (2)
- Sorptivity S
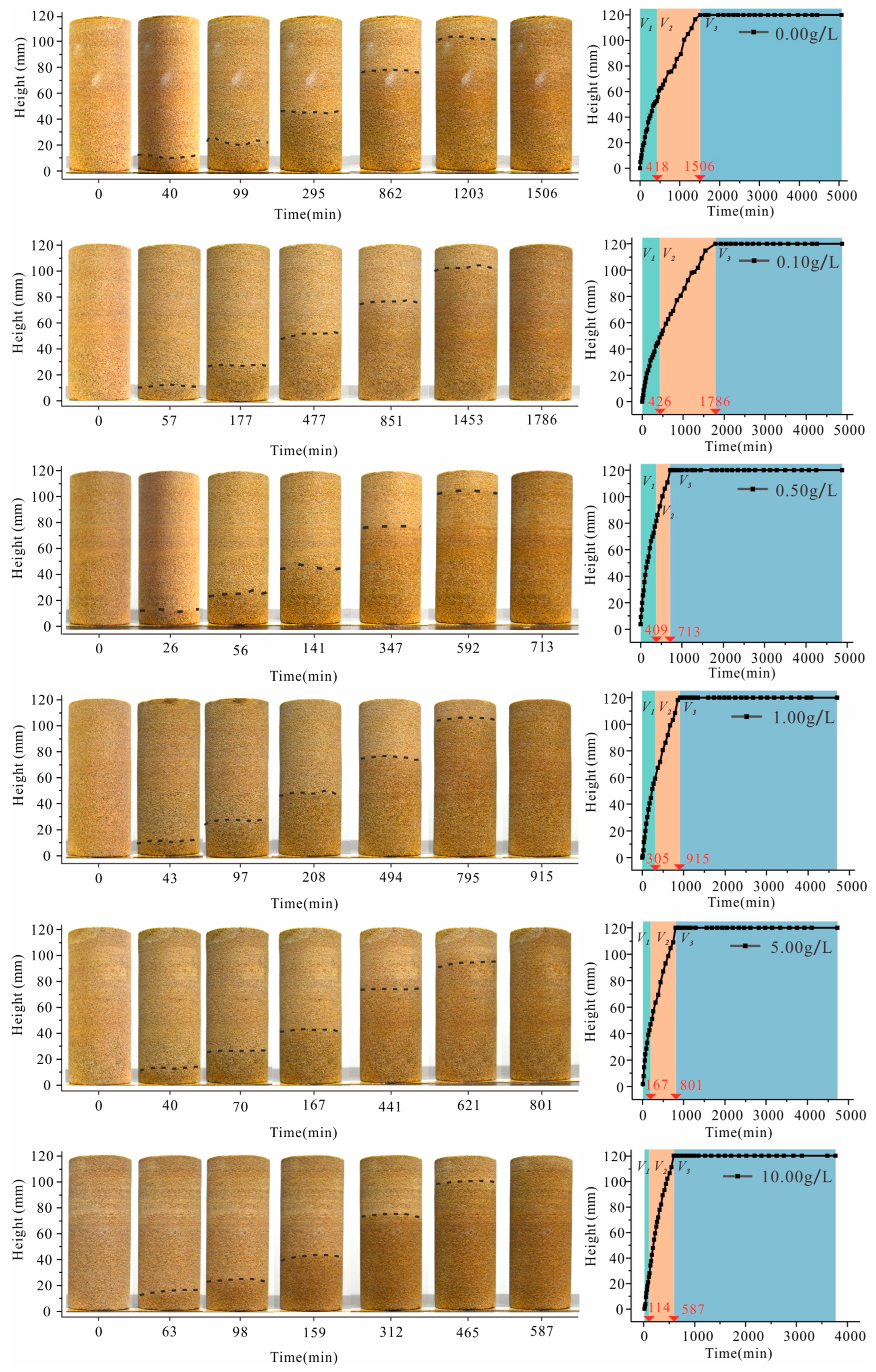
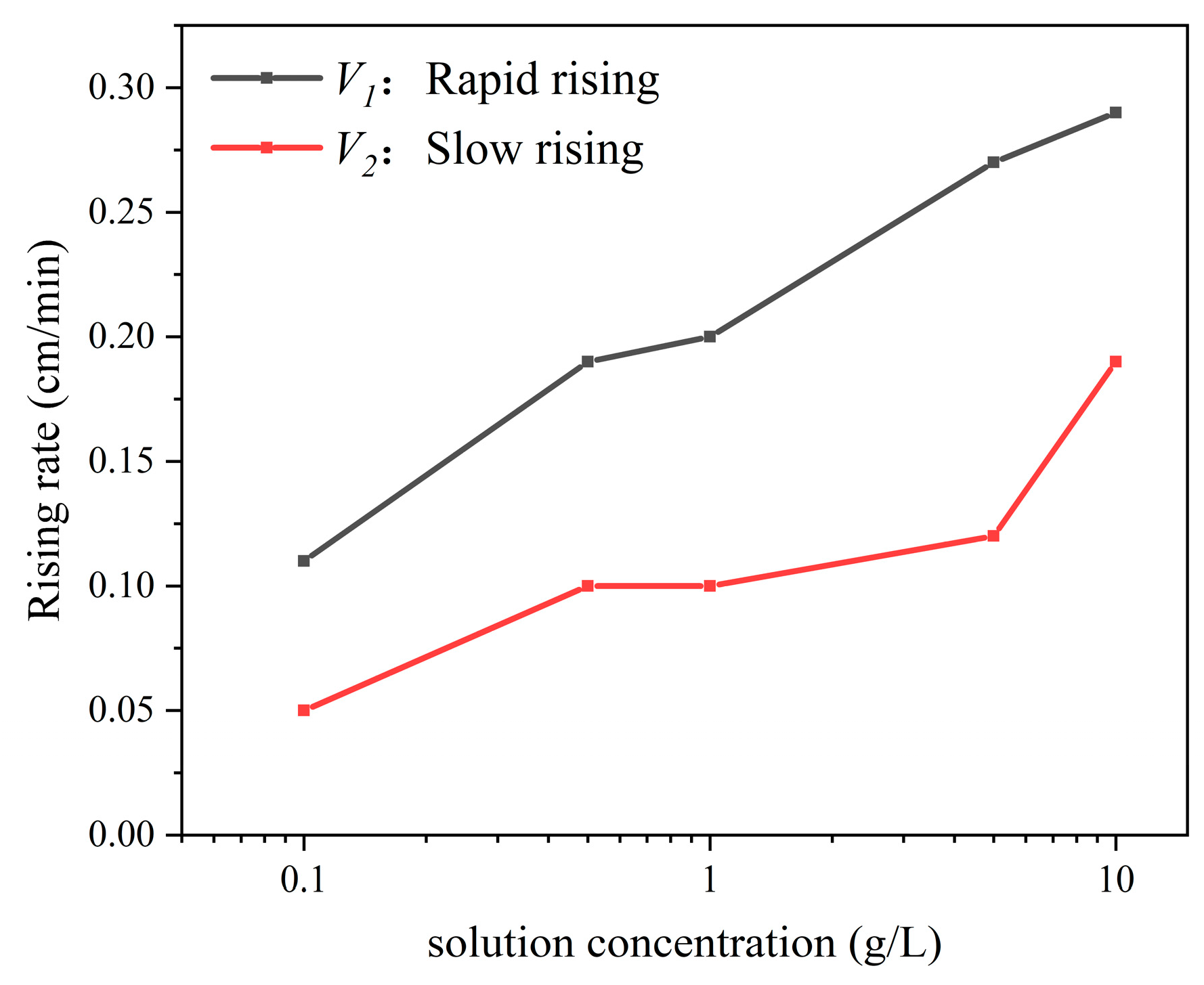
3.2. Variation in the Height of the Imbibition Front
4. Observation of Capillary Imbibition Using NMR
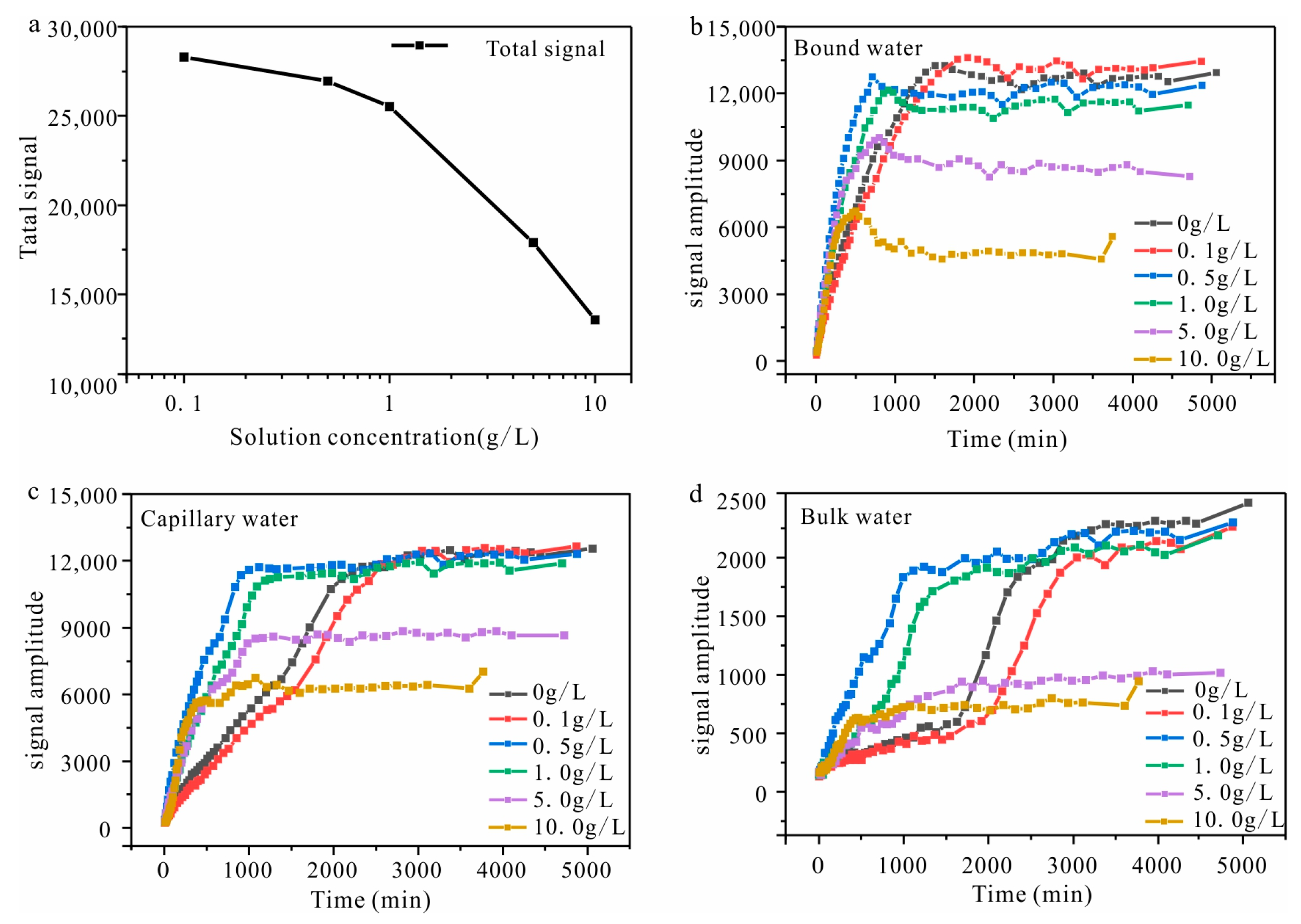
4.1. Variation in T2 Spectra
- (1)
- Variation in T2 spectra
- (2)
- Variations in pore water
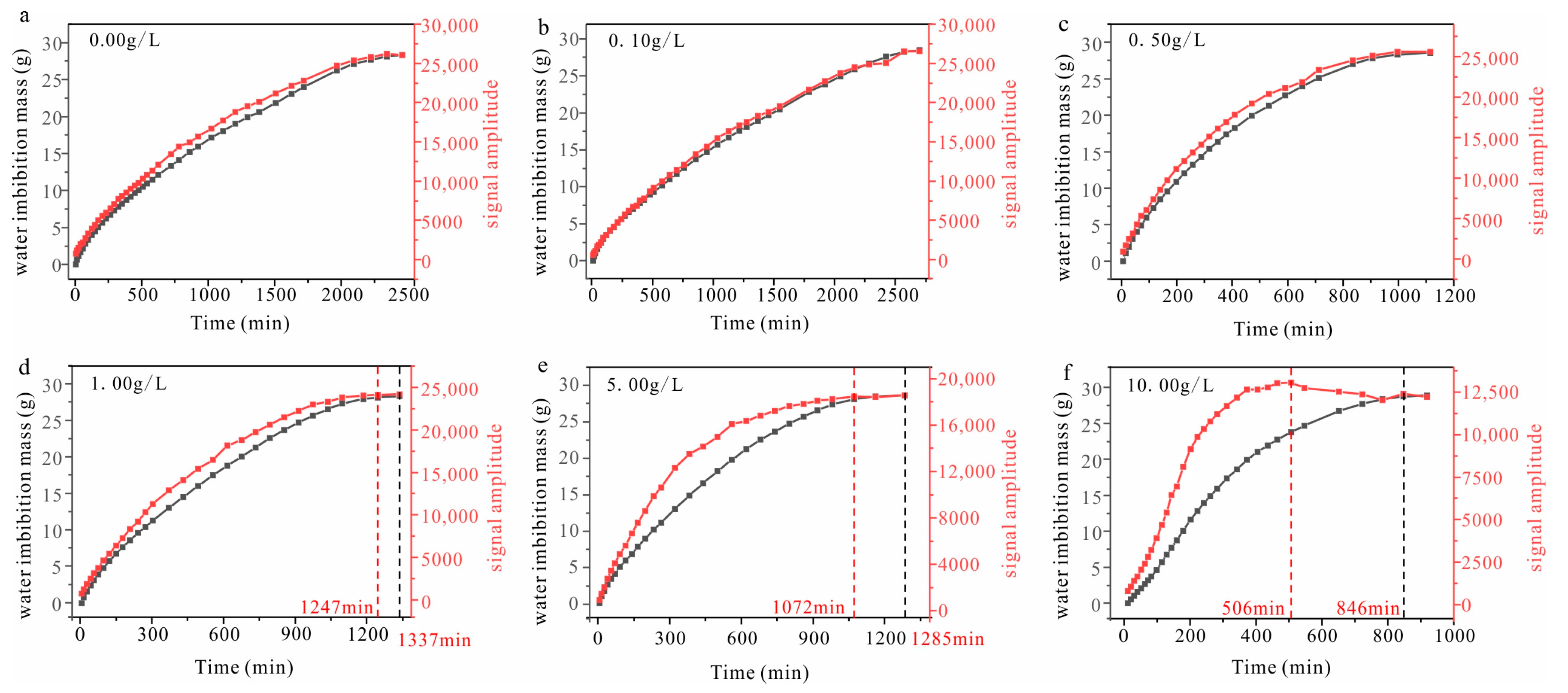
- (3)
- Comparison between the NMR signal amplitude and water imbibition mass
4.2. Variation in Stratified Moisture Distribution during Capillary Imbibition
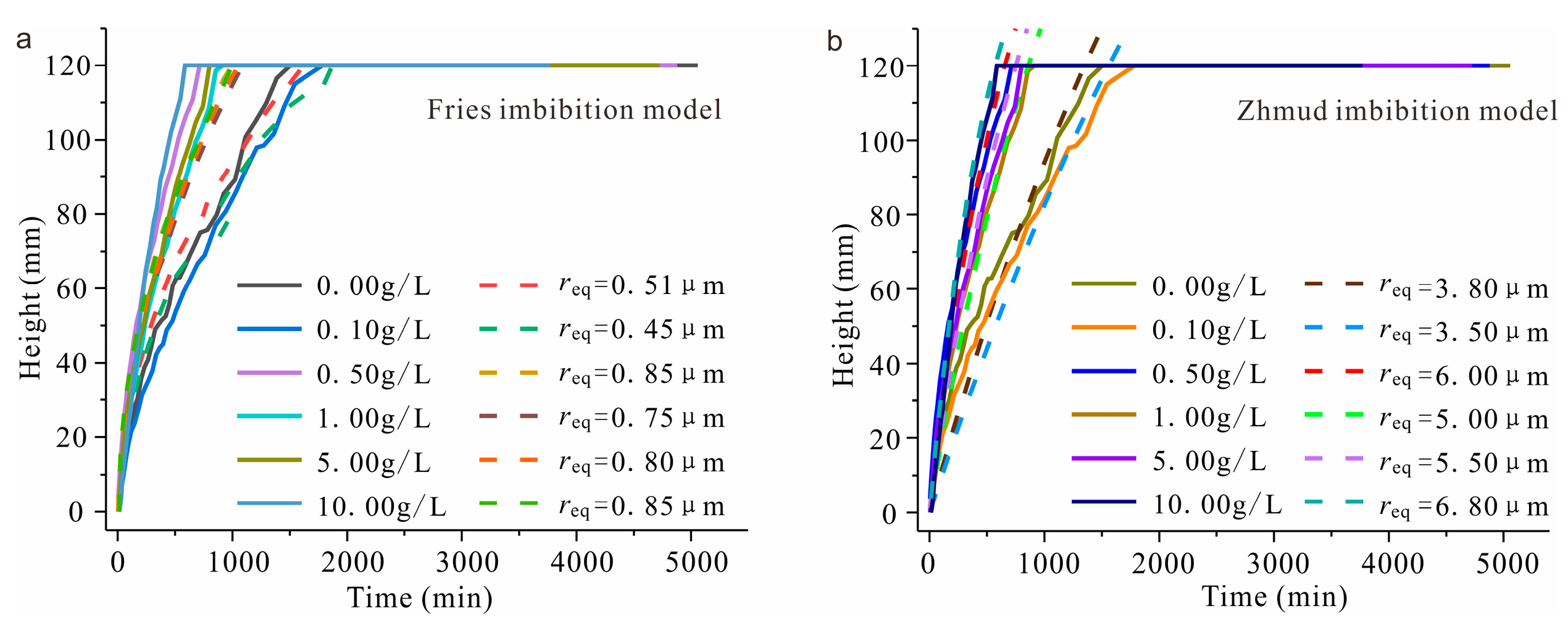
5. Discussion
- (1)
- The imbibition model of a single-tube cylindrical capillary
- (2)
- Impact of varying solution concentrations on the solution’s properties
- (3)
- The modification of sandstone’s pore structure during the brackish water imbibition process
6. Conclusions
- (1)
- The solution concentration alters the rate of water imbibition during capillary imbibition. An increase in solution concentration leads to an enhancement in the rate of water imbibition mass, sorptivity, and the rate at which the height of the imbibition front rises.
- (2)
- The total water absorption of sandstone decreases with increasing solution concentration. The total signal amplitude of the T2 spectra decreases with increasing solution concentration, and the boundary on both sides of the relaxation time gradually shifts toward the center.
- (3)
- When the solution concentration exceeds 0.50 g/L, the NMR signal amplitude of the sample does not exhibit synchronized variations with the water imbibition mass over time. It may even demonstrate an inverse trend at certain stages. For instance, the signal amplitude of the sample with a concentration of 10.00 g/L exhibits a subsequent decline after reaching its maximum. At the same time, the mass continues to improve as the water imbibition time increases.
- (4)
- The solution concentration significantly influences the water imbibition process of sandstone for two potential reasons: Firstly, the presence of salinity alters the liquid properties. The calculation shows that variations in solution properties at varying concentrations do not significantly impact the water absorption rate. Furthermore, the imbibition process of brackish water modifies the pore structures of sandstone. In the water imbibition process of a sample perpendicular to the bedding plane, the surface of the sample is regarded as the evaporation front, which leads to the generation of crystallization close to the sample surface. As a result, crystallization clogs the parallel water migration pathway along the bedding surface, which decreases the water migration time and increases the water imbibition rate. The law that the equivalent capillary radius is higher in the sample with a higher solution concentration was demonstrated by comparing the classical capillary dynamics model and test results.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Nishiyama, N.; Yokoyama, T.; Takeuchi, S. Size distributions of pore water and entrapped air during drying infiltra-tion processes of sandstone characterized by water expulsion porosimetry. Water Resour. Res. 2012, 48, W09556. [Google Scholar] [CrossRef]
- Jiang, Q.; Deng, H.; Li, J.; Luo, Z.; Assefa, E.; Fang, J.; Xiao, Y. The degradation effect and mechanism by water-rock interaction in the layered sandstone in the Three Gorges reservoir area. Arab. J. Geosci. 2019, 12, 722. [Google Scholar] [CrossRef]
- Shi, Q.; Cui, S.; Wang, S.; Mi, Y.; Sun, Q.; Wang, S.; Yu, J. Experiment study on CO2 adsorption performance of thermal treated coal: Inspiration for CO2 storage after underground coal thermal treatment. Energy 2022, 254, 124392. [Google Scholar] [CrossRef]
- Šimůnek, J.; Jarvis, N.J.; Van Genuchten, M.T.; Gärdenäs, A. Review and comparison of models for describing non-equilibrium and preferential flow and transport in the vadose zone. J. Hydrol. 2003, 272, 14–35. [Google Scholar] [CrossRef]
- Kong, J.; Xin, P.; Hua, G.F.; Luo, Z.Y.; Shen, C.J.; Chen, D.; Li, L. Effects of vadose zone on groundwater table fluctuations in unconfined aquifers. J. Hydrol. 2015, 528, 397–407. [Google Scholar] [CrossRef]
- Zaitsev, G.A.; Dubrovina, O.A.; Shainurov, R.I. Iron and manganese migration in “soil–plant” system in Scots pine stands in conditions of contamination by the steel plant’s emissions. Sci. Rep. 2020, 10, 11025. [Google Scholar] [CrossRef] [PubMed]
- Huang, B.; Yuan, Z.; Li, D.; Zheng, M.; Nie, X.; Liao, Y. Effects of soil particle size on the adsorption, distribution, and migration behaviors of heavy metal (loid) s in soil: A review. Environ. Sci. Process. Impacts 2020, 22, 1596–1615. [Google Scholar] [CrossRef]
- Siver, P.A.; Canavan, R.W.; Field, C.K.; Marsicano, L.J.; Lott, A. Historical Changes in Connecticut Lakes over a 55-Year Perio; American Society of Agronomy, Crop Science Society of America, and Soil Science Society of America: Madison, WI, USA, 1996. [Google Scholar] [CrossRef]
- Yin, X.; Feng, Q.; Zheng, X.; Zhu, M.; Wu, X.; Guo, Y.; Wu, M.; Li, Y. Spatio-temporal dynamics and eco-hydrological controls of water and salt migration within and among different land uses in an oasis-desert system. Sci. Total Environ. 2021, 772, 145572. [Google Scholar] [CrossRef] [PubMed]
- Cunningham, M.A.; Snyder, E.; Yonkin, D.; Ross, M.; Elsen, T. Accumulation of deicing salts in soils in an urban environmen. Urban Ecosyst. 2008, 11, 17–31. [Google Scholar] [CrossRef]
- Lucas, R. Ueber das Zeitgesetz des kapillaren Aufstiegs von Flüssigkeite. Kolloid-Zeitschrift 1918, 23, 15–22. [Google Scholar] [CrossRef]
- Washburn, E.W. The dynamics of capillary flow. Phys. Rev. 1921, 17, 273. [Google Scholar] [CrossRef]
- Benavente, D.; Lock, P.; Ángeles García Del Cura, M.; Ordóñez, S. Predicting the capillary imbibition of porous rocks from microstructure. Transp. Porous Media 2002, 49, 59–76. [Google Scholar] [CrossRef]
- Shen, A.; Xu, Y.; Liu, Y.; Cai, B.; Liang, S.; Wang, F. A model for capillary rise in micro-tube restrained by a sticky layer. Results Phys. 2018, 9, 86–90. [Google Scholar] [CrossRef]
- Wang, P.; Zhang, Q.; Wang, M.; Yin, B.; Hou, D.; Zhang, Y. Atomistic insights into cesium chloride solution transport through the ultra-confined calcium–silicate–hydrate channel. Phys. Chem. Chem. Phys. 2019, 21, 11892–11902. [Google Scholar] [CrossRef] [PubMed]
- Kim, E.; Whitesides, G.M. Imbibition and flow of wetting liquids in noncircular capillaries. J. Phys. Chem. B 1997, 101, 855–863. [Google Scholar] [CrossRef]
- Lundblad, A.; Bergman, B. Determination of contact angle in porous molten-carbonate fuel-cell electrodes. J. Electrochem. Soc. 1997, 144, 984–987. [Google Scholar] [CrossRef]
- Fries, N.; Dreyer, M. An analytic solution of capillary rise restrained by gravity. J. Colloid Interface Sci. 2008, 320, 259–263. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Y.; Zhang, Y.; Huang, J. Experimental study on capillary water absorption of sandstones from different grotto heritage sites in China. Herit. Sci. 2022, 10, 25. [Google Scholar] [CrossRef]
- Scherer, G.W. Stress from crystallization of salt. Cem. Concr. Res. 2004, 34, 1613–1624. [Google Scholar] [CrossRef]
- Lavalle, J. Recherches sur la formation lente des cristaux à la température ordinair. Compte Rend. Acad. Sci. 1853, 36, 493–495. [Google Scholar]
- Becker, G.F.; Day, A.L. The Linear Force of Growing Crystals. Proc. Wash. Acad. Sci. 1905, 7, 283–288. [Google Scholar] [CrossRef]
- Correns, C.W.; Steinborn, W. Experimente zur Messung und Erklärung der sogenannten Kristallisationskraf. Z. Krist.-Cryst. Mater. 1939, 101, 117–133. [Google Scholar] [CrossRef]
- Everett, D.H. The thermodynamics of frost damage to porous solids. Trans. Faraday Soc. 1961, 57, 1541–1551. [Google Scholar] [CrossRef]
- Lubelli, B.; Cnudde, V.; Diaz-Goncalves, T.; Franzoni, E.; van Hees, R.P.; Ioannou, I.; Menendez, B.; Nunes, C.; Siedel, H.; Stefanidou, M.; et al. Towards a more effective and reliable salt crystallization test for porous building materials: State of the art. Mater. Struct. 2018, 51, 1–21. [Google Scholar] [CrossRef]
- Steiger, M.; Asmussen, S. Crystallization of sodium sulfate phases in porous materials: The phase diagram Na2SO4–H2O and the generation of stress. Geochim. Cosmochim. Acta 2008, 72, 4291–4306. [Google Scholar] [CrossRef]
- Yang, H.; Chen, C.; Zhao, G.; Zhou, J. Electrical resistivity analysis for the internal capillary water migration mechanism of porous stone. Acta Geophys. 2024, 72, 213–231. [Google Scholar] [CrossRef]
- Çelik, M.Y.; Sert, M. An assessment of capillary water absorption changes related to the different salt solutions and their concentrations ratios in the Döğer tuff (Afyonkarahisar-Turkey) used as building stone of cultural heritages. J. Build. Eng. 2021, 35, 102102. [Google Scholar] [CrossRef]
- Al-Naddaf, M. Quantifying the influence of halite and sylvite crystallization on capillary water absorption coefficient of sandstone. J. Am. Inst. Conserv. 2011, 50, 1–13. [Google Scholar] [CrossRef]
- Wang, T.; Jia, H.; Sun, Q.; Tan, X.; Tang, L. Effects of thawing-induced softening on fracture behaviors of frozen rock. J. Rock Mech. Geotech. Eng. 2024, 16, 979–989. [Google Scholar] [CrossRef]
- Matteson, A.; Tomanic, J.P.; Herron, M.M.; Allen, D.F.; Kenyon, W.E. NMR relaxation of clay/brine mixtures. SPE Reserv. Eval. Eng. 2000, 3, 408–413. [Google Scholar] [CrossRef]
- Jia, H.; Ding, S.; Wang, Y.; Zi, F.; Sun, Q.; Yang, G. An NMR-based investigation of pore water freezing process in sandstone. Cold Reg. Sci. Technol. 2019, 168, 102893. [Google Scholar] [CrossRef]
- Jia, H.; Ding, S.; Zi, F.; Dong, Y.; Shen, Y. Evolution in sandstone pore structures with freeze-thaw cycling and interpretation of damage mechanisms in saturated porous rocks. Catena 2020, 195, 104915. [Google Scholar] [CrossRef]
- Christopher, H.; Thomas, K.T. Water movement in porous building materials-VII. The sorptivity of mortar. Build. Environ. 1986, 21, 113–118. [Google Scholar] [CrossRef]
- Siegesmund, S.; Dürrast, H. Physical and Mechanical Properties of Rocks. In Stone in Architecture: Properties, Durability; Springer: Berlin/Heidelberg, Germany, 2014; pp. 97–224. [Google Scholar]
- Jia, H.; Dong, B.; Wu, D.; Shi, Q.; Wei, Y. Capillary Imbibition in Layered Sandston. Water 2023, 15, 737. [Google Scholar] [CrossRef]
- Zhmud, B.V.; Tiberg, F.; Hallstensson, K. Dynamics of capillary rise. J. Colloid Interface Sci. 2000, 228, 263–269. [Google Scholar] [CrossRef] [PubMed]
- Laliberte, M. Model for calculating the viscosity of aqueous solutions. J. Chem. Eng. Data 2007, 52, 321–335. [Google Scholar] [CrossRef]
- Ge, Y.; Chang, C.; Yang, W.; Zhang, B.; Yuan, J.; Yu, J. Effect of inorganic salts on surface tension of solutions and properties of concrete. Concrete 2007, 6, 7–9. [Google Scholar]
- Sghaier, N.; Prat, M.; Nasrallah, S.B. On the influence of sodium chloride concentration on equilibrium contact angle. Chem. Eng. J. 2006, 122, 47–53. [Google Scholar] [CrossRef]
- Englert, A.H.; Rodrigues, R.T.; Rubio, J. Dissolved air flotation (DAF) of fine quartz particles using an amine as collector. Int. J. Miner. Process. 2009, 90, 27–34. [Google Scholar] [CrossRef]
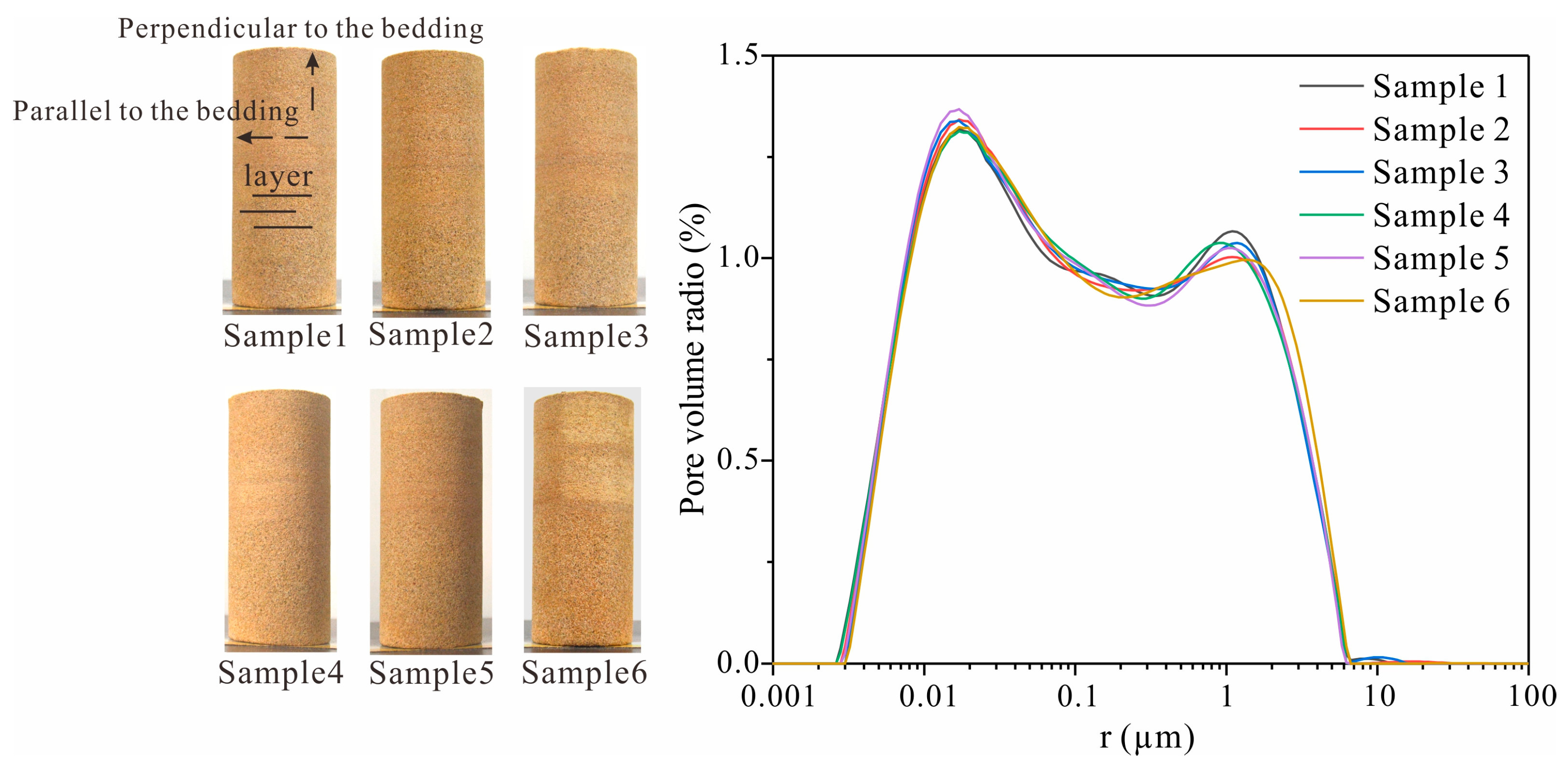


| Sample | Solution Concentration (g/L) | Porosity (%) | P-Wave Velocity (km·s−1) |
|---|---|---|---|
| 1 | 0.00 | 19.40 | 2.09 |
| 2 | 0.10 | 19.41 | 2.08 |
| 3 | 0.50 | 19.45 | 2.06 |
| 4 | 1.00 | 19.38 | 2.03 |
| 5 | 5.00 | 19.11 | 2.04 |
| 6 | 10.00 | 19.47 | 2.06 |
| Solution Concentration (g/L) | Parameter | Stage I | Stage II |
|---|---|---|---|
| 0.00 g/L | T (min) | 149 | 2458 |
| M (g) | 4.51 | 23.81 | |
| R (g/min) | 0.035 | 0.40 | |
| 0.10 g/L | T (min) | 147 | 2848 |
| M (g) | 4.04 | 24.77 | |
| R (g/min) | 0.26 | 0.011 | |
| 0.50 g/L | T (min) | 166 | 1116 |
| M (g) | 9.53 | 19.04 | |
| R (g/min) | 0.065 | 0.025 | |
| 1.00 g/L | T (min) | 241 | 1337 |
| M (g) | 9.6 | 18.82 | |
| R (g/min) | 0.045 | 0.018 | |
| 5.00 g/L | T (min) | 263 | 1285 |
| M (g) | 11.03 | 21.78 | |
| R (g/min) | 0.048 | 0.019 | |
| 10.00 g/L | T (min) | 372 | 920 |
| M (g) | 19.92 | 25.16 | |
| R (g/min) | 0.056 | 0.019 |
| Solution Concentration (g/L) | Bound Water | Capillary Water | Bulk Water |
|---|---|---|---|
| 0 g/L | 1506 min | 2342 min | 2876 min |
| 0.1 g/L | 1904 min | 2696 min | 3048 min |
| 0.5 g/L | 713 min | 996 min | 1116 min |
| 1.0 g/L | 915 min | 1072 min | 1594 min |
| 5.0 g/L | 801 min | 1162 min | 1285 min |
| 10.0 g/L | 506 min | 506 min | 546 min |
| Solution Concentrations (g/L) | Density (kg/m3) | Viscosity (Pa·s) | Surface Tension (N/m) | Contact Angle (°) |
|---|---|---|---|---|
| 0.00 | 1000.00 | 9.55 × 10−4 | 7.16 × 10−2 | 33.00 |
| 0.10 | 1000.07 | 9.56 × 10−4 | 7.16 × 10−2 | 33.00 |
| 0.50 | 1000.35 | 9.57 × 10−4 | 7.16 × 10−2 | 33.01 |
| 1.00 | 1000.70 | 9.58 × 10−4 | 7.16 × 10−2 | 33.01 |
| 5.00 | 1003.50 | 9.70 × 10−4 | 7.16 × 10−2 | 33.07 |
| 10.00 | 1007.00 | 9.84 × 10−4 | 7.17 × 10−2 | 33.13 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jia, H.; Yang, X.; Wei, Y.; Sun, Q.; Tang, L. Capillary Imbibition Laws of Fresh–Brackish Waters in Sandstone. Water 2024, 16, 1180. https://doi.org/10.3390/w16081180
Jia H, Yang X, Wei Y, Sun Q, Tang L. Capillary Imbibition Laws of Fresh–Brackish Waters in Sandstone. Water. 2024; 16(8):1180. https://doi.org/10.3390/w16081180
Chicago/Turabian StyleJia, Hailiang, Xiaoyu Yang, Yao Wei, Qiang Sun, and Liyun Tang. 2024. "Capillary Imbibition Laws of Fresh–Brackish Waters in Sandstone" Water 16, no. 8: 1180. https://doi.org/10.3390/w16081180
APA StyleJia, H., Yang, X., Wei, Y., Sun, Q., & Tang, L. (2024). Capillary Imbibition Laws of Fresh–Brackish Waters in Sandstone. Water, 16(8), 1180. https://doi.org/10.3390/w16081180







