Angle of Attack Characteristics of Full-Active and Semi-Active Flapping Foil Propulsors

Abstract

As a propulsor with a good application prospect, the flapping foil has been a hot research topic in the past decade. Although the research results of flapping foils have been very abundant, the performance-influencing mechanism of flapping foils is still not perfect, and the research considering three-dimensional (3D) effects for engineering applications is still very limited. Based on the above considerations, a systematic and parametric analysis of a small aspect ratio flapping foil is conducted to correlate the influencing factors including angle of attack (AoA) characteristics and wake vortex on the propulsive efficiency. Three-dimensional numerical analyses of full-active and semi-active flapping foils are carried out in this paper, in which the former focuses on different heave amplitudes and pitch amplitudes, and the latter concentrates on different spring stiffnesses. The analysis covers the full range of advance coefficient, which starts around 0 and ends at a thrust drop of 0. Firstly, the influence of the maximum AoA (α_max) on the efficiency and thrust coefficient of these two kinds of flapping foils is analyzed. The results show that for the small aspect ratio flapping foil in this paper, regardless of the full-active or semi-active form, the peak efficiency as high as 75% for both generally appears around α_max = 0.2 rad, while the peak thrust coefficient of 0.5 occurs near α_max = 0.3 rad. Then, by analyzing the wake flow field, it is found that the lower efficiency of larger α_max working points is mainly due to the larger vortex dissipation loss, while the lower efficiency of smaller α_max working points is mainly due to the larger friction loss of the foil surface. Furthermore, the plumpness of different AoA curves is compared and analyzed. It was found that, unlike the results of full-active flapping foils, the shape of the AoA curve of semi-active flapping foils with different spring stiffnesses is similar, and the relationship with efficiency is not strictly corresponding. This study is expected to provide guidance on both academics and industries in relevant fields.

1. Introduction

Since the discovery that many natural swimmers such as fish and aquatic mammals are able to utilize the physical principles of unsteady hydrodynamics to achieve both high maneuverability and high propulsive efficiency, much research has been carried out on the bionic hydrodynamics and design of bionic propulsion. Among the many existing bionic propulsors, flapping hydrofoil propulsors occupy a special place because so many living species have adapted to them. Furthermore, the efficiency of a flapping foil can reach as high as 80% as evidenced in experimental tests [1]. Therefore, growing attention has been devoted to research on flapping foil applications for waterborne vehicles, including natural observation, theoretical analysis, experimental studies, and computational investigations. Up to now, many forms of flapping hydrofoil propulsion have been developed, such as classic fully imposed flapping foil propulsion (full-active flapping foil propulsion) [1,2,3,4], semi-active flapping foil propulsion [5,6,7], flexible flapping foils [8,9,10,11], and tandem flapping foils [12,13,14,15]. In the research on the gradual application of flapping foils, the focus has gradually shifted from 2D flapping foils to 3D ones [7,16,17,18]. These flapping foil propulsion systems can be applied not only for ships and underwater vehicles, but also for aircraft and ocean energy devices [14,19]. Therefore, they have a wide range of applications and great prospects, and have always been a hot research topic in the field of biomimetic propulsion.

In order to explore the mechanism of high efficiency of flapping foil propulsion, researchers have conducted extensive and continuous research. These works have been mostly conducted on the influence of St number and angle of attack (AoA) on the performance. For example, Anderson et al. [1] measured the thrust and efficiency of multiple sets of 2D flapping foils at different St numbers, maximum AoA (α_max), and phase differences, and achieved preliminary optimization of the optimal working conditions of flapping foil propulsion. The highest efficiency reached 87%, and the corresponding optimal α_max is believed to lie within the range of 15°~25°. Schouveiler et al. [20] also experimentally measured the performance of flapping foils under a large number of different St numbers and α_max, and concluded that the highest efficiency occurs at an α_max of 15°. At the same time, they also preliminarily explored the influence of asymmetric pitch motion on the AoA curve and flapping foils performance. Build upon the method employed by Read et al. [2], Hover et al. [21] directly compared the performance obtained with four specific AoA profiles in experiments. Esfahani et al. [22] also studied the influence of different AoA profiles, but their method was to make the heave motion an elliptical trajectory. Subsequently, Xiao et al. [23] and Karbasian et al. [24] further improved the motion trajectory of the flapping foils, thereby controlling the shape of the AoA curve and the development of the wake vortex, playing a role in increasing thrust. Most of them found the same conclusion that explicit control of the AoA in flapping foil propulsion can broadly increase thrust and efficiency, compared to a baseline case in which the heave and pitch motions are sinusoidal. In addition to St number and AoA, some scholars have also conducted research on the geometric characteristic parameters of flapping foils. Floc’H et al. [25] compared the propulsion performance of flapping foils and propellers by using the advance coefficient J instead of the St number to describe the performance of flapping foils, and discussed the performance over a very large range of heave and pitch amplitudes. The authors also conducted similar work in the early stage [4]. Floryan et al. [3] studied the performance of flapping foils with small heave and pitch amplitudes, and pointed out that lower-speed flapping foils can achieve higher efficiency because of the smaller viscosity loss.

With the increasing demand for engineering applications, researchers have conducted research on different forms of flapping foils (especially semi-active and flexible forms) in order to better adapt to different working conditions. Zhang et al. [8] used numerical methods to observe the wake vortex structure of an elastic constrained flapping plate (a kind of semi-active flapping plate) with sinusoidal heave motion, and discussed the effects of frequency ratio and spring stiffness on the performance of the flapping plate. Bøckmann et al. [9] experimentally measured a high aspect ratio 3D semi-active flapping foil constructed with a NACA 0015 airfoil, which initially achieved high propulsion efficiency and was compared with a full-active flapping foil. Xiao et al. [10] simulated a small aspect ratio semi-active flapping foil with an elliptical cross-section and discussed the effects of parameters such as frequency ratio and density ratio on performance and flow field. Thaweewat et al. [11] comprehensively analyzed the influencing parameters of semi-active flapping foils and compared them with full-active flapping wings. In addition, Yang et al. [13] applied semi-active flapping wings to wave energy gliders and improved their performance by optimizing the tandem hydrofoils. In the study of flexible foils, Dewey et al. [5] analyzed the propulsion performance of a flapping flexible flat plate and discussed the effects of plate stiffness and frequency ratio on thrust and efficiency. Cleaver et al. [6] also conducted similar work at small heave amplitudes, exploring the effects of different stiffnesses and aspect ratios. Mannam et al. [16] and Sanmiguel et al. [26] also conducted similar research on flexible flapping foils and flexible flapping plates. For ease of implementation, Lee et al. [7] used a purely flapping flexible foil to generate thrust, and the results showed that this approach is feasible.

Understanding the complex fluid–structure interaction is crucial to the high-efficiency design of the flapping foil propulsion system; therefore, many researchers also studied the wake vortex structure characteristics of the system. Anderson et al. [1] and Schouveiler et al. [20] have preliminarily observed the flow field of flapping foils under high St numbers and confirmed the presence of obvious wake vortex structures after the oscillating foils. For clearer observation, Buchholz et al. [27] used flow visualization to investigate the wake structure produced by a rigid flat panel of aspect ratio 0.54 at low Strouhal numbers, and observed a 3D von Kármán vortex street. At the same time, Dong et al. [28] employed an immersed boundary method to investigate the effect of aspect ratio on the wake topology and hydrodynamic performance of thin ellipsoidal flapping foils. The simulations showed that the wake of thrust-producing finite aspect ratio flapping foils was dominated by two sets of interconnected vortex loops. Then, Schnipper et al. [29] observed the wake vortex structure of pure pitch flapping foils with different St numbers and pitch amplitudes on a thin soap film. Experimental results showed diverse wake structures for various amplitudes and oscillation frequencies. The wake signatures show the existence of a von Kármán vortex street, a reverse von Kármán vortex street, “2P” wake, “2P + 2S” wake, “4P” wake, “4P + 2S” wake, and other wakes varying from “4P” to “8P” structures. Subsequently, Andersen et al. [30] further compared these experimental results with numerical results and established a correlation between vortex structure, thrust, and efficiency. In 2021, our group also analyzed the relationship between the efficiency of full-active flapping foils and the wake vortex structure. [4]. Under the highest efficiency condition, the wake vortex usually appears in a strip shape, while the efficiency is lower when obvious vortex streets appear. In Zurman-Nasution’s work [31], they examined the similarities and differences between 2D and 3D simulations through a case study in order to evaluate the efficacy and limitations of using 2D simulations to describe a 3D system. Recently, Li’s team directly measured the lift, drag, and moment of a NACA 0012 hydrofoil in experiments, where wake flow fields were visualized by phase-locked particle image velocimetry [32], and further compared with prediction results by an unsteady RANS simulation [33]. In addition to rigid flapping foils, Cleaver et al. [6], Mannam et al. [16], Sanmiguel et al. [26], Clark et al. [34], and Shao [35] conducted numerical observations and experiments on the flow field vortex structure of a flexible oscillating flat plate and flexible flapping foils successively.

As reviewed above, it can be seen that there is a close relationship between the efficiency and thrust coefficient of flapping foils and the AoA and wake vortex. However, firstly, the corresponding relationships are still uncertain, and the influencing mechanism is not completely clear. Secondly, although there have been abundant research results on oscillating foil thrusters, virtually all studies have been limited to low Reynolds numbers or small amplitudes, which are far from those encountered in practical applications. In addition, research considering 3D effects for engineering applications is still very limited. Based on the above considerations, distinguishing from previous 2D research and considering the influence of flow at the end of the foil, a sufficiently small aspect ratio foil (c = 0.1 m, h = 0.2 m) is selected, and a systematical and relatively comprehensive 3D study is conducted on the AoA characteristics of full-active and semi-active flapping foils in this paper. The analysis in this paper covers the full range of advance coefficient, which starts around 0 and ends at a thrust drop of 0. The full-active propulsors are focused on different heave and pitch amplitudes, and the semi-active ones pay attention to different spring stiffnesses. We want to correlate the AoA characteristics to the propulsive performance of both full-active and semi-active flapping foils in this research, which aims to provide essential implications and guidance for flapping foil propulsors in marine applications.

We begin by describing the geometric structure, motion, and kinematic and dynamics parameters of full-active and semi-active flapping hydrofoils in Section 2. Then, the computational method is described and fully verified in Section 3. In Section 4, a systematic presentation on the simulating results is included. Our particular interest is centered on the impact of AoA characteristics on the performance of flapping foils, mainly on efficiency and thrust coefficient. Considering that the AoA of a flapping foil changes at any time during its operation, the influence of the α_max and the shape of the AoA time-history curve have been analyzed, and the relationship between the wake structure and efficiency has also been discussed.

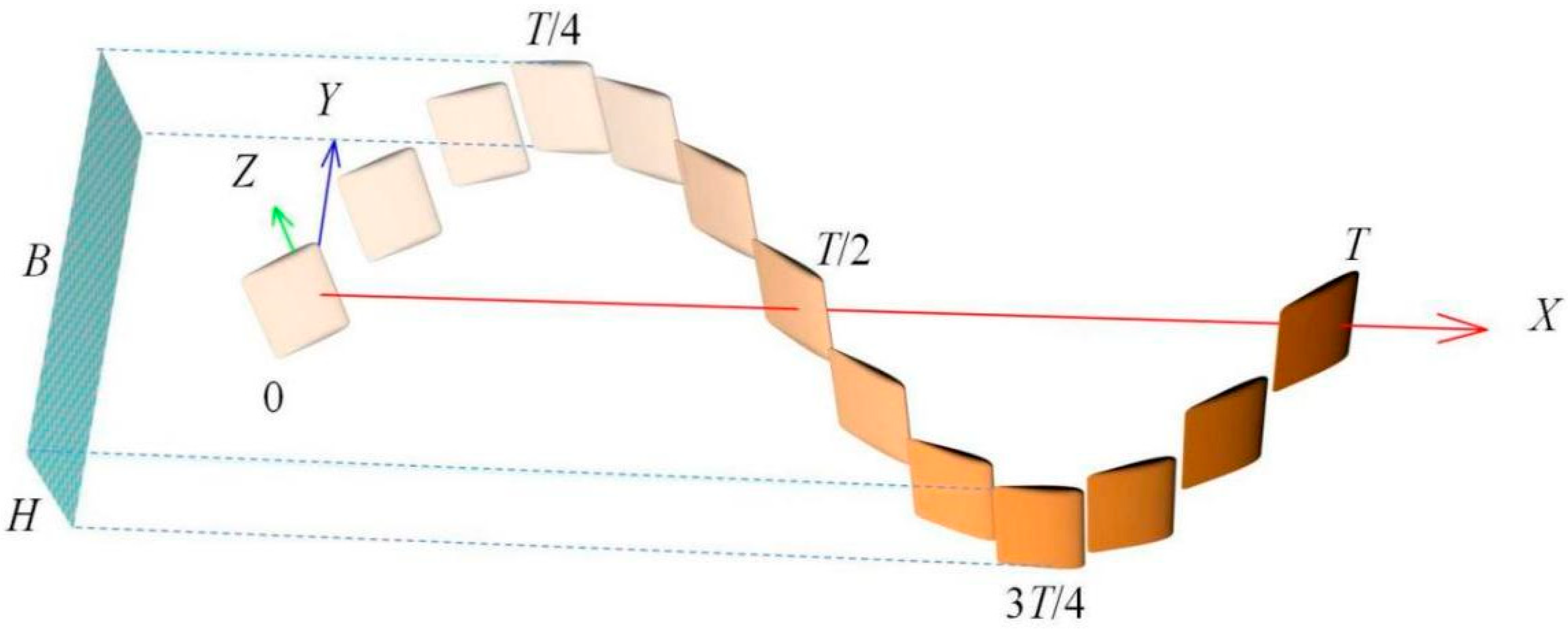
2. Description and Definition of Flapping Foil Propulsion

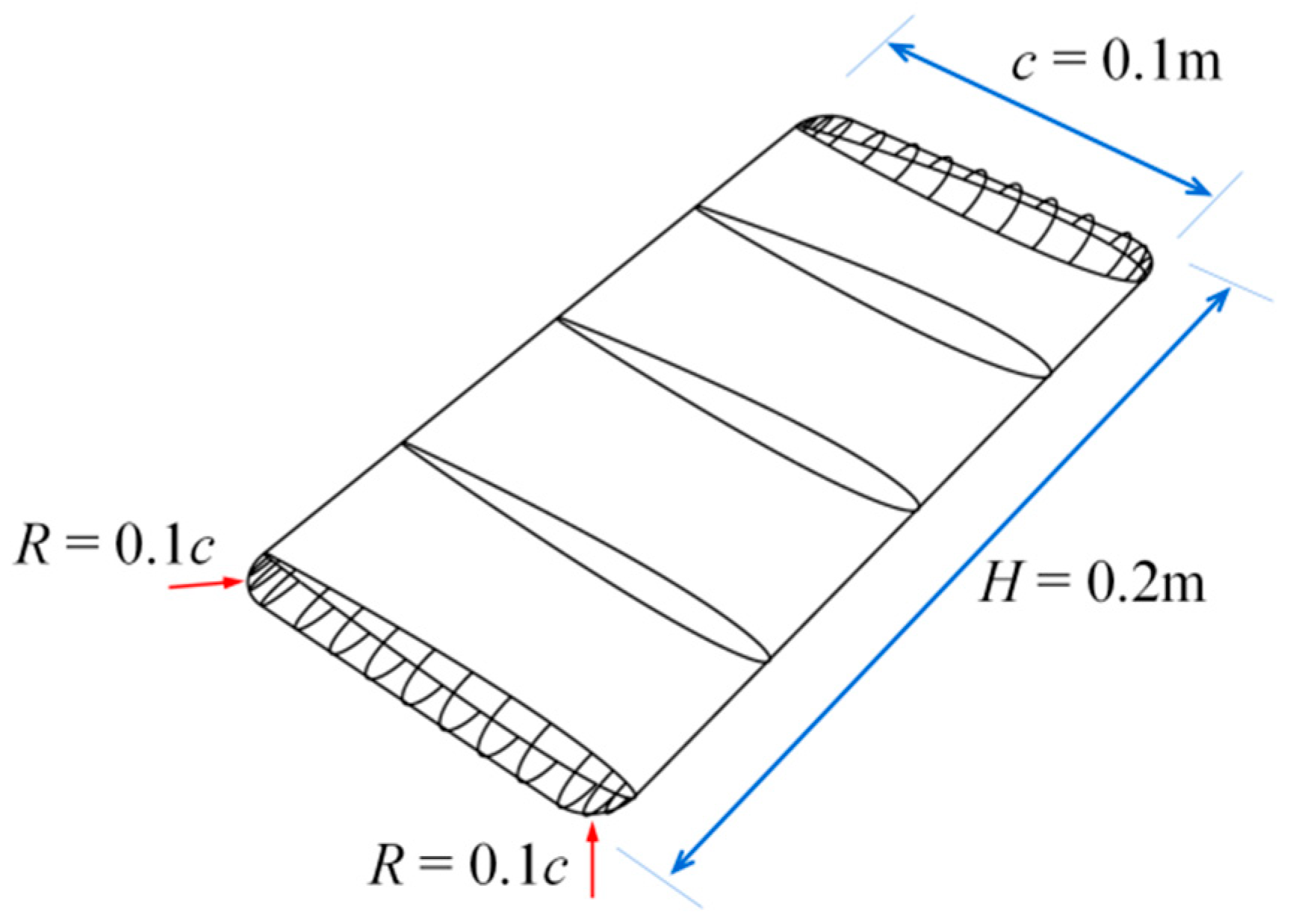
2.1. Geometric Structure and Motion Definition

In this paper, a rigid 3D airfoil is used, with the chord length c = 0.1 m and the span-wise dimension H = 0.2 m, i.e., aspect ratio AR = 2.0. The NACA 0012 airfoil is used, and the 3D flapping foil is designed with equal chord length. Round corners are designed at both ends of the span direction of the flapping foil, with a radius of R = 0.1c, and connected with an elliptic curve to construct the end shape, as shown in Figure 1. The pitch axis position of the full-active flapping foil is set at the 0.3c distance from the leading edge, which is similar to the setting of Anderson et al. [1]. In order to obtain a larger hydrodynamic moment, the pitch axis position of the semi-active flapping foil is set at the leading edge of the airfoil, which was adopted in the studies of Zhang et al. [5] and Thaweewat et al. [8]. Simplification is made in the numerical simulation, so the actual pitch axis and its connecting components are not reflected in the model.

Considering the research on the flow mechanism of full-active flapping foils and the engineering application requirements of semi-active flapping foil propulsion, both these two kinds of flapping foils have been analyzed in this paper. Unlike the traditional propeller, the movement of rigid flapping foil propulsion is more complex, which is generally composed of a combination of uniform linear motion (along the X direction), periodic heave motion (along the Y direction), and pitch motion (rotation around the Z axis). Their motion trajectories at different times are roughly shown in Figure 2, with an advancing velocity V_A (m/s), a period T (s), a heave height B = 2y₀ (m), and a sweeping area B × H.

Usually, both full-active and semi-active flapping foils are subjected to heave motion vertically with a simple harmonic function at their pivot point, which is expressed in Equation (1). For pitch motion, the full-active flapping foils usually follow a sinusoidal curve, but pitching angle θ is ahead of the heave y by a phase difference of ϕ, and its pitching angle can be expressed as Equation (2). Unlike the full-active flapping foil with given heave and pitch motion, the pitching motion of semi-active flapping foils is controlled by a torsion spring attached to the foil as illustrated in Figure 3. Consequently, the sinusoidal heave motion together with the advance velocity creates an oscillating hydrodynamic force and moment causing the foil to work at an AoA (α), but the torsion spring is used to restore the foil towards the equilibrium position. In addition, it does not have any other degrees of freedom. Under the combined action of this hydrodynamic moment and torsion spring, the flapping foil produces a pitching angle θ, determined by the pitch motion equation, which can be written as Equation (3).

$$\mathrm{Heave}\mathrm{motion}:y=y_0\sin (2\pi ft)$$

(1)

$$\mathrm{Pitch}\mathrm{motion}(\mathrm{full}-\mathrm{active}):\theta ={\theta }_0\sin (2\pi ft+\phi )$$

(2)

$$\mathrm{P}\mathrm{i}\mathrm{t}\mathrm{c}\mathrm{h}\mathrm{m}\mathrm{o}\mathrm{t}\mathrm{i}\mathrm{o}\mathrm{n}(\mathrm{s}\mathrm{e}\mathrm{m}\mathrm{i}-\mathrm{a}\mathrm{c}\mathrm{t}\mathrm{i}\mathrm{v}\mathrm{e}):I\ddot{\theta }+K\theta =M_z$$

(3)

where: y₀ is the heave amplitude, m; f is the flapping frequency, Hz; θ₀ is the pitching amplitude, radians; φ is the phase angle difference between the heaving and pitching motions, radians; I denotes inertia moment of the foil, $\mathrm{K}\mathrm{g}·{\mathrm{m}}^2$; K denotes torsion spring stiffness, $\mathrm{N}·\mathrm{m}/\mathrm{r}\mathrm{a}\mathrm{d}$; θ is the pitching angle, radians; M_z denotes the fluid moment imposed on foil, $\mathrm{N}·\mathrm{m}$, and t is the time, s.

In order to measure the amplitude of the heave motion of the flapping foils in this paper, the heave amplitude ratio y₀/c is defined, where c is the chord length. In this paper, different heave amplitude ratios y₀/c are realized by adjusting the heave amplitude in the following simulation. According to Floc’H et al. [25], the range of the heave amplitude ratio used in this study is y₀/c = 0.5, 1.0, 2.5, 4.0.

For pitch motion of full-active flapping foil, the fixed flapping frequency f = 1.0 Hz is used and viscosity coefficient ν = 10⁻⁶ m²/s is used. In the full-active flapping foils analysis, a set of pitch amplitudes ${\theta }_0(0.2,0.3,0.4,0.5,0.6\mathrm{r}\mathrm{a}\mathrm{d})$ are used in this paper to cover a more comprehensive range, and phase difference $\phi$ is 90°. For semi-active flapping foils, the pitch motion is solved based on Equation (3) with different spring stiffnesses and moments of inertia, and the advancing velocity V_A is also adjusted to achieve different working conditions.

2.2. Nondimensional Propulsive Indicators

To study flapping foil propulsion, some non-dimensional numbers have been used in this paper. As mentioned above, the flapping frequency f is determined in this study, and different working conditions are obtained by adjusting the advancing velocity V_A. St number is expressed as the following Equation (4):

$$\mathrm{St}={\displaystyle \frac{fB}{V_A}}$$

(4)

where B = 2y₀ is heave height, m; fB is the characteristic velocity of heave motion.

For the convenience of comparing with marine propellers, Floc’H [25] introduced the advance coefficient, J, as shown in Equation (5), which is inherited in this paper.

$$J={\displaystyle \frac{V_A}{fB}}={\displaystyle \frac{1}{\mathrm{St}}}$$

(5)

In order to measure the torsional spring stiffness K and moment of inertia I of semi-active flapping foils, the spring stiffness ratio K′ and frequency ratio r are defined, respectively. With reference to the work in Thaweewat et al. [8], the dimensionless parameter spring stiffness ratio K′ is defined to describe the spring stiffness. Taking fB as the reference speed and the swept area BH as the reference area (as shown in Figure 2), the spring stiffness ratio K′ is redefined in this paper as the following Equation (6):

$$K^{\prime }={\displaystyle \frac{K}{\rho {\left(fB\right)}^2\left(BH\right)c}}$$

(6)

where ρ is the density of water, Kg/m³; H is the span-wise size of the flapping foil, m.

The definition of frequency ratio r in this paper is the same as that in Thaweewat [8]. It is the forced-to-natural frequency ratio, i.e., the ratio between the flapping and natural frequencies. It can be expressed as the following Equation (7):

$$r={\displaystyle \frac{f}{f_N}}=2\pi f\sqrt{{\displaystyle \frac{I}{K}}}$$

(7)

where f_N is the natural frequency of the semi-active flapping foil in air, Hz; I is the inertia moment of the semi-active flapping foil, kg·m².

It is common knowledge that the AoA of the flapping foil is one of the important parameters affecting the performance. This paper will focus on the AoA characteristics of full-active and semi-active flapping foils. As shown in Figure 3, the maximum angle of attack ${\alpha }_{max}=\mathrm{m}\mathrm{a}\mathrm{x}\left[\left|\alpha \left(t\right)\right|\right]$, and AoA can be expressed as Equation (8):

$$\alpha (t)=\arctan {\displaystyle \frac{V_y(t)}{V_x}}-\theta (t)$$

(8)

The main focus of this study is on the relationship between the efficiency $\eta$ and thrust coefficient K_T and the AoA. According to Anderson et al. [1], the efficiency of the flapping motion is defined as the ratio of the average output and the average input power in one cycle after full convergence, which is defined as Equation (9). The thrust coefficient is defined as Equation (10).

$$\eta ={\displaystyle \frac{P_{out}}{P_{in}}}$$

(9)

$$K_T={\displaystyle \frac{{\int }_{t_0}^{t_0+T}{F}_{x}dt}{\rho {\left(fB\right)}^2\left(BH\right)}}$$

(10)

where F_x is the resulting hydrodynamic thrust in the forward direction.

The definitions of output power P_out and efficiency $\eta$ are similar for semi-active and full-active flapping foils. The resulting hydrodynamic force is the thrust F_x in the forward direction. Because the force could not remain constant, the output power is expressed as the time average of instantaneous power, which is defined as Equation (11). Similarly, the input power of the flapping foil also fluctuates periodically, so the input power is also expressed as the time average of instantaneous power. The difference is that the input power of a full-active flapping foil includes F_y and M_Z, two parts, while the input forces of a semi-active flapping foils consist of only the heave force F_y; and the pitch motion of semi-active flapping foils is constrained by springs. The input powers of both are expressed in Equation (12) and Equation (13), respectively.

$$P_{out}={\displaystyle \frac{1}{T}}{\int }_{t_0}^{t_0+T}{F}_x{V}_{A}dt$$

(11)

$$\mathrm{full}-\mathrm{active}:P_{in}={\displaystyle \frac{1}{T}}{\int }_{t_0}^{t_0+T}\left(F_y{V}_y+M_z{\omega }_z\right)dt$$

(12)

$$\mathrm{semi}-\mathrm{active}:P_{in}={\displaystyle \frac{1}{T}}{\int }_{t_0}^{t_0+T}{F}_y{V}_{y}dt$$

(13)

where V_y is the velocity component of the pivot center in the Y direction, and ω_z is the angular velocity of the pivot center, M_z is the torque on the pitching center of flapping foil, t₀ is a certain moment after stable performance.

On the basis of our previous research [36], if a small frequency ratio is selected (below 0.5), its impact on the performance is negligible, and the semi-active flapping foil can work normally in a very large range of spring stiffness ratios. Therefore, in this paper, the frequency ratio remains unchanged and is set to r = 0.2. A set of spring stiffness ratios is used to analyze semi-active flapping foils, $K^{\prime }=0.8,1.6,4.0$. With the fixed geometric parameters and the frequency of the flapping foils, the spring stiffness $K$ can be calculated using Formula (6) based on the sweep height $B$ and spring stiffness ratio $K^{\prime }$. Subsequently, the inertia moment of the flapping foils $I$ can be calculated using Equation (7) based on the frequency ratio r.

3. Computational Method and Validation

3.1. Governing Equations

An integral-type Reynolds-averaged incompressible viscous fluid dynamics equation is adopted, and the continuity equation and momentum equation can be written as the following Equations (14) and (15). The turbulence viscosity coefficient is confirmed by Menter’s k-ω shear-stress transport turbulence model [37].

$$\underset{S}{\oint }\stackrel{⃑}{v}\cdot d\stackrel{⃑}{S}=0$$

(14)

$${\displaystyle \frac{\partial }{\partial t}}\underset{\Omega }{\int }{v}_{i}d\mathsf{\Omega }+\underset{S}{\oint }{v}_i\stackrel{⃑}{v}\cdot d\stackrel{⃑}{S}={\displaystyle \frac{1}{\rho }}\underset{S}{\oint }{\tau }_{ij}d{S}_j-\underset{S}{\oint }{\displaystyle \frac{p}{\rho }}d{S}_i$$

(15)

where Ω is the element volume; $\overrightarrow{v}$ is the flow velocity; $v_i$ is velocity component; ${\tau }_{ij}$ is the sum of ${\tau }_{ij}$ is the sum of laminar viscous stress and turbulent viscous stress; p is the pressure; $\rho$ is the density of water; S_j is the components of the area vector and $\overrightarrow{S}$ is the area vector of the element surface.

In this paper, the CFD software FINE/Marine 8.2 (a software package of the NUMECA company; ISIS-CFD solver is developed by the European Space Agency, Paris, France) is used to investigate the hydrodynamic performance of flapping foil propulsion. This solver adopts internal implicit iteration within a time-step iteration to ensure a strong and accurate flow/motion coupling. The hydrodynamic performance of semi-active flapping foils should be obtained by solving the fluid hydrodynamic equation and the rigid body dynamics equation simultaneously. The dynamic equation of semi-active flapping foils has been introduced above in Equation (3).

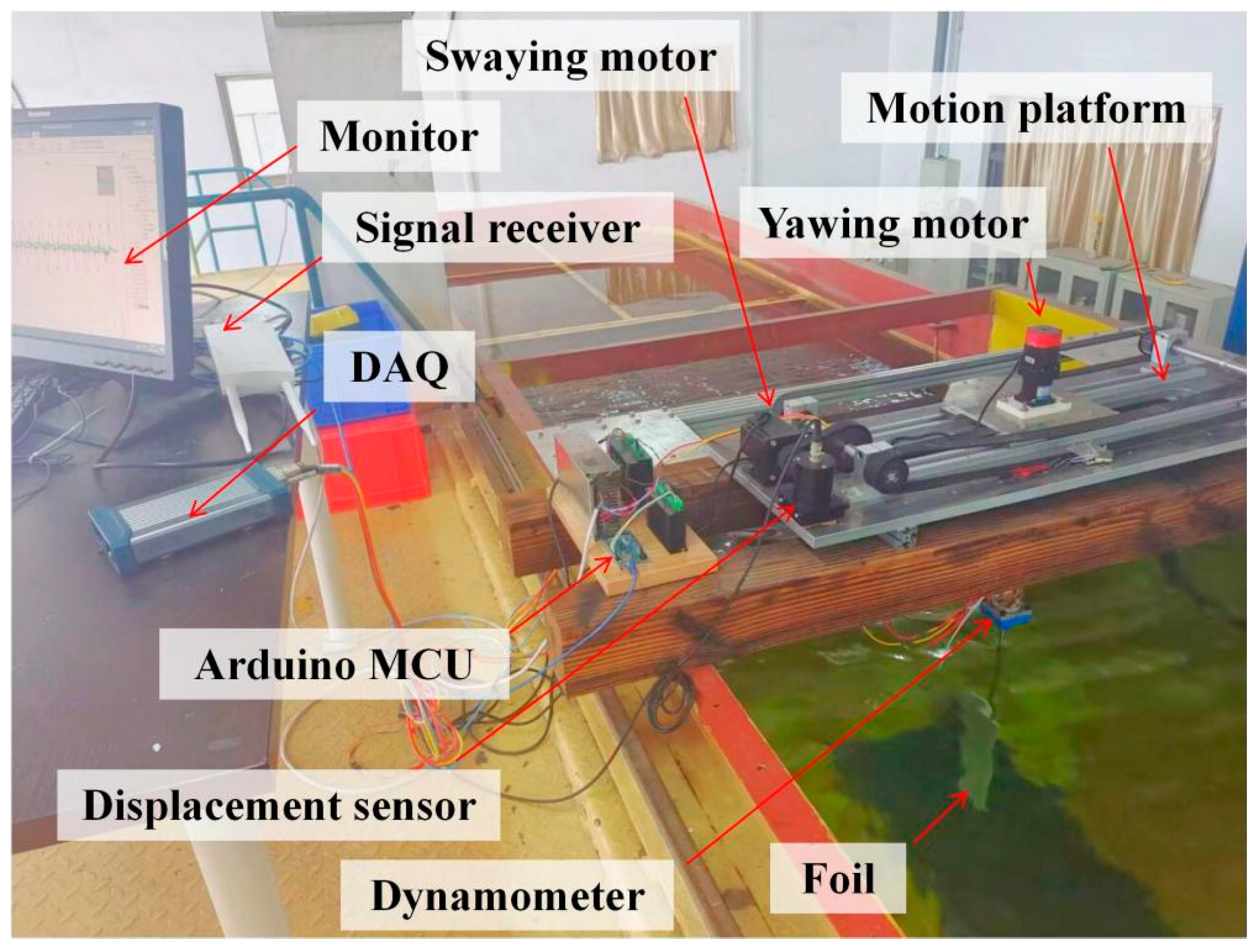
3.2. Mesh and Method

The computational domain simulates a water tunnel with 4 m width, 4 m height, and 8 m length test section, as shown in Figure 4. The viscous effect of the water tunnel wall is ignored, and the four outside boundary conditions are set as slip walls. The right boundary gives the velocity inlet and the left boundary the extrapolated pressure outlet. The setting methods for the boundary conditions are shown in Figure 4.

In order to accurately simulate the pressure and velocity gradient on the foil surface, as well as the separated vortex in the trail, a gradually subdivided hexahedral mesh is used in the computational domain. To accurately simulate the pressure and velocity gradient at the leading and trailing edges of the foil, additional subdivisions are carried out in all three directions of the flapping foil. The refinement method is shown in

Table 1. Grid size and refinement scheme.

Direction	Hydrofoil Surface Region	Region I	Region II
spanwise/Z	$H/128$	$c/64$	$c/32$
chordwise/X	$c/128$
normal direction/Y	$c/128$

, and the enlarged detail of the grid near the foil surface is shown in Figure 4. In order to adapt to the large-scale compound motion of the foil, the elastic twist grid technology is also adopted in the calculation. The deformation of the grid during the operation of the flapping foil can be roughly seen in the enlarged detail of Figure 4. Due to our extensive grid validation work on flapping hydrofoils in the early stages [4,18,36], we will not repeat the introduction here.

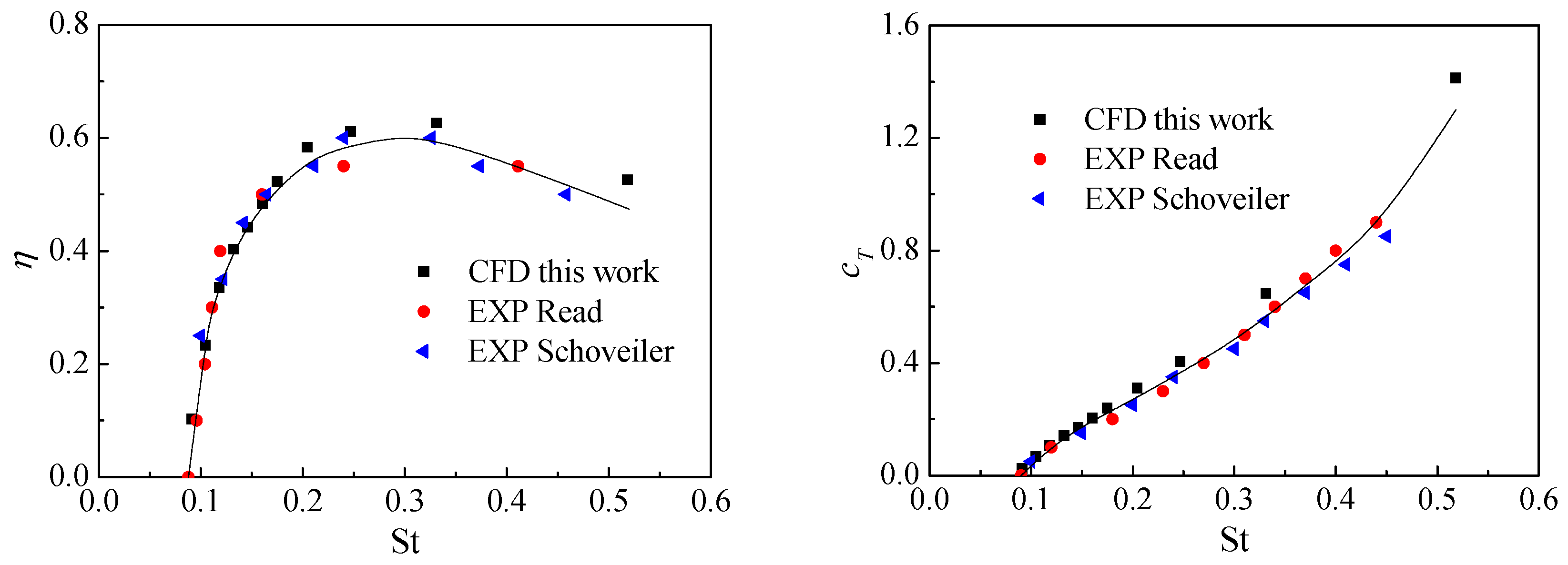
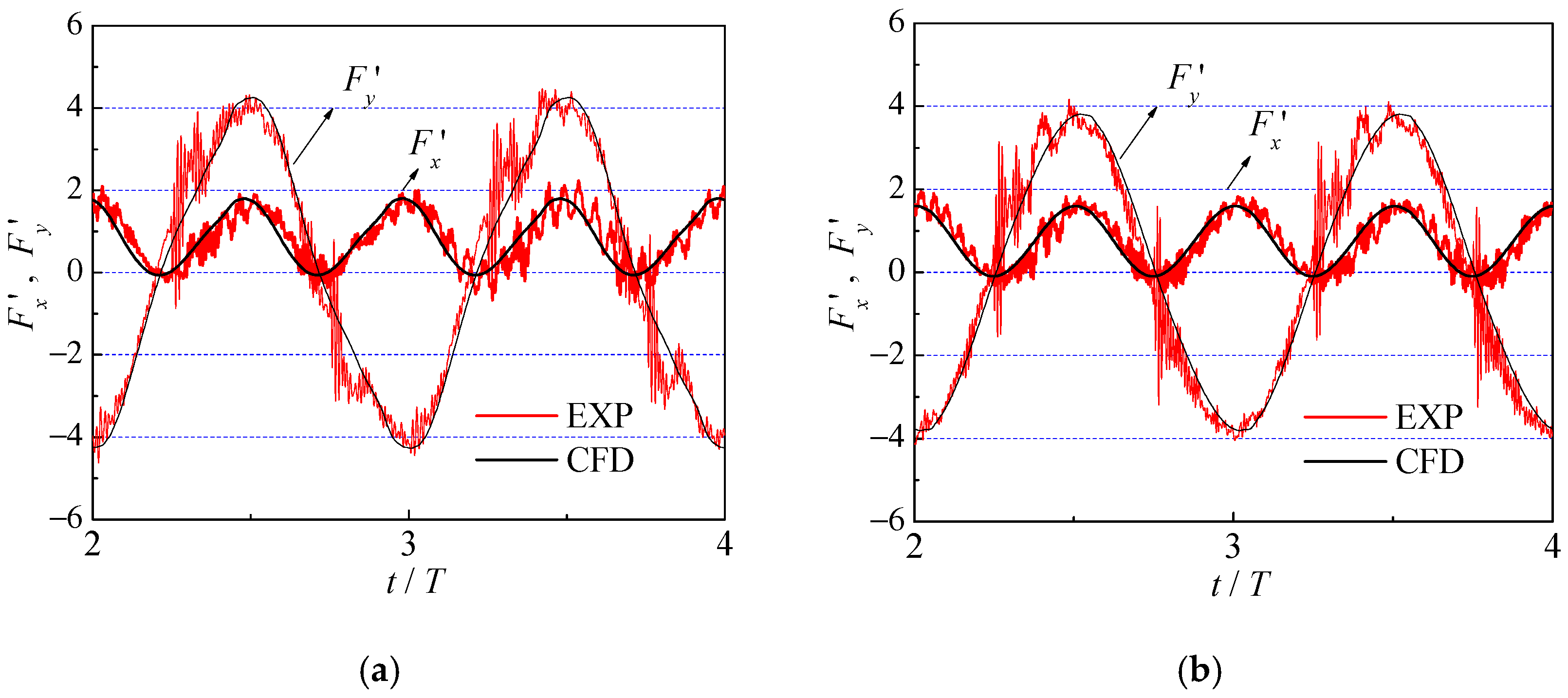
3.3. Validation

The flapping foil system studied in this paper is a 3D problem, while there are few experimental results on 3D flapping foil. In order to validate the numerical method used in the current work, two types of validation are carried out in this part: simulation verification of selected benchmark conditions in Read’s [2] and Schoveiler’s [20] quasi-2D experiments, and an independently designed experimental verification for the 3D simulation methods.

3.3.1. Simulation Verification of 2D Experimental Results

This part verifies the quasi-2D experiment results of Read [2] and Schoveiler [20], hoping to prove the feasibility of the 2D numerical method in this paper firstly. In their experiments, endplates were used on each strut to prevent flow around the ends of the foil and maintain approximately 2D flow [2]. In the future, the accuracy of the 3D method may be further discussed through 3D experiments or mutual verification with other 3D simulation results.

A rigid 2D NACA 0012 airfoil is used, and the motion is composed of active heave and pitch motions. The heave amplitude-to-chord ratio (y₀/c) is 0.75, and the pitching axis is 1/3 chord from the leading edge of the foil. The experimental and the calculated results for f = 1.2 Hz, α_max = 20° are compared and shown in Figure 5. The comparison presents an excellent agreement between the two results in terms of the propulsive efficiency η and the thrust coefficient c_T. The definition of thrust coefficient given by Read et al. [2] and Schouveiler et al. [20] is slightly different from that in this paper, and the specific definition can be referred to the description in the literature.

Subsequently, in order to verify the accuracy of the flow field simulation in this method, the pure pitching flapping foil measured by Schnipper et al. [29] was further simulated by a 2D method. The experiment was carried out with a very thin soap film, and the chord length of the flapping foil was also very small, only 6 mm. The soap film is a classical 2D flow because of the Re number. The experimental and the calculated results are compared and shown in Figure 6. The shaded graph in Figure 6 shows the experimental results in the literature, and the contour graph is the 2D CFD results in this paper. It can be seen that, on the whole, the simulation results of the wake core position are in good agreement with the experimental results, except some vortex core positions show slight deviation.

In the verification of 2D numerical methods, much work has been carried out for grid independence, turbulence models, time steps, and experimental results with Read, which is detailed in our previous work [36]. Therefore, this article will not elaborate further.

3.3.2. Experimental Verification of 3D Simulation Methods

The above two-part verification focused on the numerical methods for 2D flapping foils. In the verification work of 3D numerical methods, time-step and turbulence model validation and grid independence validation were also carried out in our previous work [18]. In order to further verify the reliability of the numerical method for the 3D flapping foil simulation, a hydrodynamic performance experiment of the active flapping foil was designed and completed independently in this paper.

A motion platform with two degrees of freedom is designed and built on a circulating water tank, and a three-component force strain gauge is used to measure the hydrodynamic performance of the active flapping foil in this paper at twice the size, with a chord length of 0.2 m and a span length of 0.4 m. Increasing the size of the flapping foil is to increase the measured force value and improve the testing accuracy. The circulating tank of the Harbin Institute of Technology is 2 m wide and 1.4 m deep, and the initial position of the flapping foil is placed in the center. The selected experimental flow velocity, that is V_A, is approximately 0.1~0.5 m/s, with a heave period of 4~8 s, and a heave amplitude of 0.25 m, to achieve different advance coefficients. The initial pitching amplitude is 0.32 rad, and other dimensionless quantities are set the same as the numerical calculation in this paper. The motion of the two degrees of freedom of the flapping wing is controlled by an Arduino microcontroller using a scanning pulse method, with a theoretical pulse time control error of less than 10 microseconds. The experimental site and efficiency measurement results are shown in Figure 7 and Figure 8. It can be seen that the efficiency measured in the experiment is in good agreement with the calculated results.

In Figure 9, the force-history curves of two operating points are further compared, in which $F_x^{\prime }$ and $F_y^{\prime }$ are the dimensionless quantities of two forces, expressed as:

$$F_x^{\prime }={\displaystyle \frac{F_x}{\rho {\left(fB\right)}^2\left(BH\right)}},F_y^{\prime }={\displaystyle \frac{F_y}{\rho {\left(fB\right)}^2\left(BH\right)}}$$

(16)

Because the real watershed of the circulating water tank experiment is much larger than the computational domain of the example in Figure 4, we have also conducted a comparison between the two numerical simulation results of the computational domain described in Figure 4 and the actual watershed of the water tank, and the two were almost identical. Considering the compactness of this paper and the fact that the size of the water tank basin is significantly larger than the flapping foil working area, the results of the latter are not presented. It can be seen from the above result comparisons that the experimental results and the numerical results are also well consistent. The specific experimental details and more detailed results will be presented in another experimental research paper.

4. Results and Analysis

The purpose of this paper is to explore the impact of AoA characteristics on the performance of flapping foils, mainly on efficiency and thrust coefficient. Considering that the AoA of a flapping foil changes at any time during its operation, the influence of the ${\alpha }_{max}$ and the shape of the AoA time-history curve will be analyzed, and the relationship between the wake structure and efficiency is also be discussed.

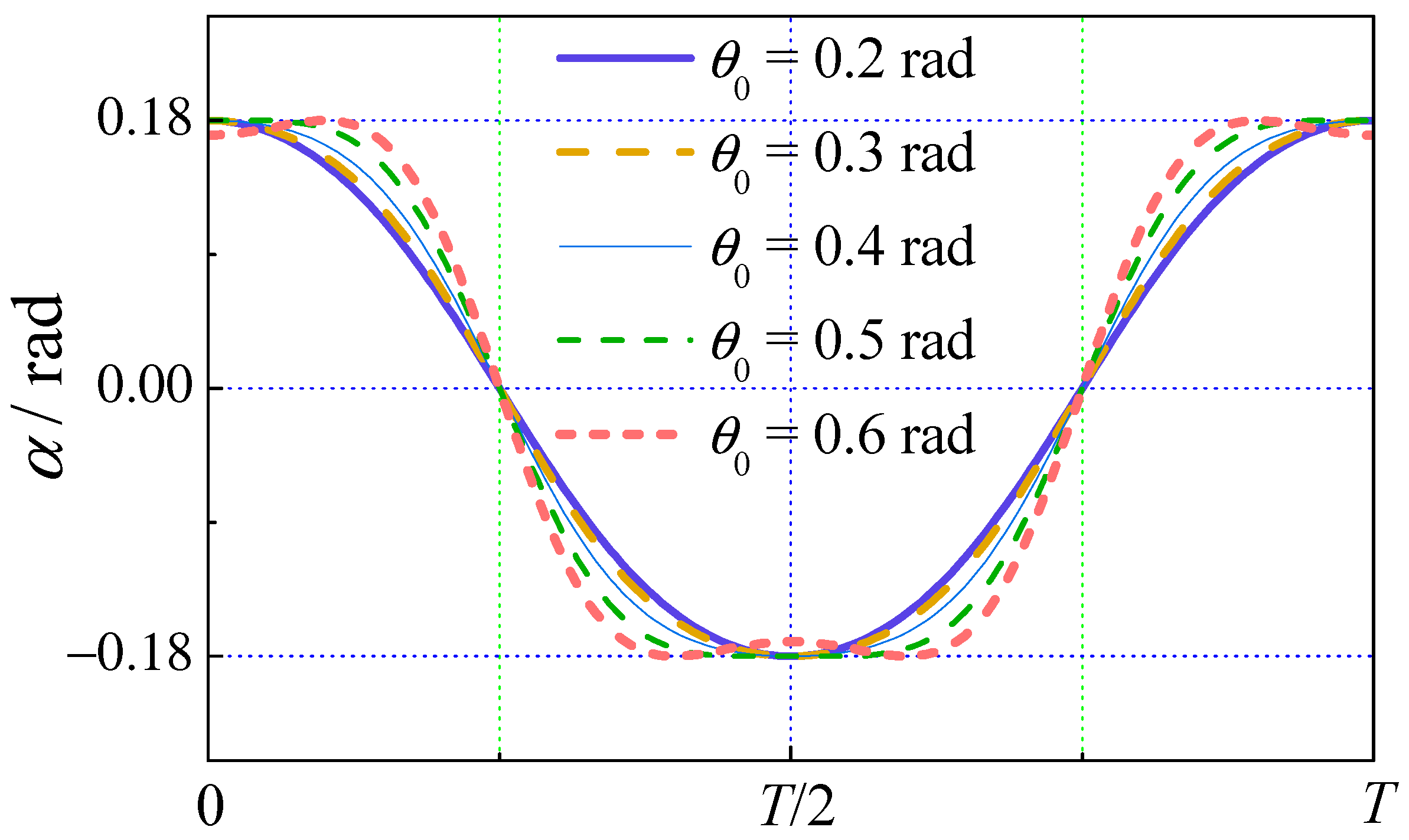
4.1. Maximum AoA Analysis

As AoA is one of the main parameters that affects flapping foil efficiency [1], Schouveiler et al. [20] measured flapping foil performance at certain heave amplitudes, with different St numbers and different ${\alpha }_{max}$ values, through experiments, and concluded that the highest efficiency of flapping foils occurs at ${\alpha }_{max}=15°$. Based on the numerical results and analysis on 2D flapping foils we obtained before [4], it is found that at different pitch and heave amplitudes, the high efficiency of flapping foils almost always occurs in the range of ${\alpha }_{max}=0.06~0.25\mathrm{r}\mathrm{a}\mathrm{d}$. The highest efficiency can be reached when ${\alpha }_{max}=0.16\mathrm{r}\mathrm{a}\mathrm{d}\approx 9°$. Although there is a certain deviation in the results of Schouveiler’s experimental and that of our simulation, both results indicate an important correlation between the ${\alpha }_{max}$ and peak efficiency. The deviation between the two may be caused by differences in other working parameters and 3D effects.

Hence, for definitive conclusions of ${\alpha }_{max}$ and further analysis of the influence on thrust coefficient, a more exhaustive and systematical investigation of ${\alpha }_{max}$ is performed in this paper. Taking small aspect ratio flapping foils as the object, a systematic and comprehensive 3D hydrodynamic analysis of full-active and semi-active flapping wings with different parameter combinations is conducted, including:

(1) Full-active flapping foils: fixed heave amplitude ($y_0/c=2.5$), with a series of different pitch amplitudes (θ₀ = 0.2 rad–0.6 rad); fixed pitch amplitude (θ₀ = 0.3 rad, θ₀ = 0.5 rad), with a series of different heave amplitudes ($y_0/c=0.5,1.0,2.5,4.0$);

(2) Semi-active flapping foils: fixed heave amplitude ($y_0/c=2.5$) and frequency ratio (r = 0.2), with different spring stiffness ratios (K′ = 0.8, 1.6, 4.0).

The series of curves showing the relationship between efficiency η, thrust coefficient K_T, and ${\alpha }_{max}$ are listed in Figure 10, Figure 11, Figure 12, Figure 13, Figure 14 and Figure 15. Among them, Figure 10 and Figure 11 list a series of full-active flapping foils with fixed heave amplitudes but different pitch amplitudes; Figure 12 and Figure 13 illustrate a series of full-active flapping foils with fixed pitch amplitudes but different heave amplitudes; finally, Figure 14 and Figure 15 show a series of results of semi-active flapping foils with different spring stiffnesses. In order to enhance the comparison, some graphs also show the efficiency and thrust coefficient of the oscillating wing as a function of the advance coefficient. In order to demonstrate the comparison between full-active and semi-active flapping foils, some data of full-active ones are also listed in Figure 14, which can be referred to in Figure 10 for specific correspondence.

4.1.1. Full-Active Flapping Foils

Firstly, Figure 10 and Figure 11 present a parametric study of the full-active flapping foil for the effect of ${\alpha }_{max}$ and advance coefficient J on the efficiency η and thrust coefficient K_T. Heave amplitude is fixed ($y_0/c=2.5$), with varying pitch amplitudes (θ₀ = 0.2 rad–0.6 rad). The heave and pitch motions in this session are all simple harmonics with a 90° phase difference and the pitching axis is set at the 0.3c distance from the leading edge.

From Figure 10a, it appears that the ${\alpha }_{max}$ corresponding to peak efficiency under different pitch amplitude conditions is basically within the same range, roughly in the range of ${\alpha }_{max}=0.12~0.25\mathrm{r}\mathrm{a}\mathrm{d}$. This is consistent with the results of the previous 2D simulation [4], and even closer to the results of Schouveiler’s experiment [20]. In Figure 10b, the ${\alpha }_{max}$ corresponding to the peak thrust coefficient under different pitch amplitude conditions remains almost unchanged, approximately around ${\alpha }_{max}=0.3\mathrm{r}\mathrm{a}\mathrm{d}$. This is because at a smaller ${\alpha }_{max}$, the lift coefficient of the airfoil decreases, leading to a decrease in thrust, whereas a too large ${\alpha }_{max}$ can cause separation of the airfoil flow, which cannot generate effective thrust. At the same time, it was also found that as the pitch amplitudes increased, although the peak of the thrust coefficient slightly decreased, the overall $K_T-{\alpha }_{max}$ curves tended to be smoother, and there are no obvious peak points of K_T at large pitch amplitude conditions (θ₀ = 0.5 and θ₀ = 0.6).

The $\eta -J$ curves and $K_T-J$ curves in Figure 11 show significant differences under different pitch amplitude conditions, but their overall shape is very similar to that of the propeller’s. As a propulsion device, analyzing the parameters of the flapping foils with different advance coefficients can make it easier to generalize and apply to thruster design. From Figure 11a, it can be seen that as the pitch amplitude increases, the peak efficiency improves from 0.6 to around 0.75, which will be analyzed in detail later. Furthermore, as the pitch amplitude increases, the curve gradually shifts to the left, and the advance coefficient J range of high-efficiency gradually decreases. The $K_T-J$ curve in Figure 11b also gradually shifts to the left with the increase in pitch amplitude, but in contrast to the peak efficiency situation, the peak of thrust coefficient K_T decreased from 0.5 to around 0.4, with the increase in pitch amplitude. Because the ship and vehicle usually work at a speed close to the propeller’s highest efficiency, a smaller pitching amplitude θ₀ will mean a larger working range.

Figure 10. Propulsive efficiency η and thrust coefficient K_T of a full-active flapping foil as function of ${\alpha }_{max}$, for different pitching angles. (a) $\eta -{\alpha }_{max}$, (b) $K_T-{\alpha }_{max}$.

Figure 10. Propulsive efficiency η and thrust coefficient K_T of a full-active flapping foil as function of ${\alpha }_{max}$, for different pitching angles. (a) $\eta -{\alpha }_{max}$, (b) $K_T-{\alpha }_{max}$.

Figure 11. Propulsive efficiency η and thrust coefficient K_T of a full-active flapping foil as function of advance coefficient J, for different pitching angles. (a) $\eta -J$, (b) $K_T-J$.

Figure 11. Propulsive efficiency η and thrust coefficient K_T of a full-active flapping foil as function of advance coefficient J, for different pitching angles. (a) $\eta -J$, (b) $K_T-J$.

Secondly, the relationships between the ${\alpha }_{max}$ and propulsive performance of full-active flapping foils with a series of heave amplitudes are shown in Figure 12 and Figure 13. The cases of pitch amplitudes of ${\theta }_0=0.3\mathrm{r}\mathrm{a}\mathrm{d}$ and ${\theta }_0=0.5\mathrm{r}\mathrm{a}\mathrm{d}$ are studied separately, and the values of the heave amplitudes are shown in the figures ($y_0/c$ = 0.5, 1.0, 2.5, 4.0).

From Figure 12, it appears that the ${\alpha }_{max}$ corresponding to peak efficiency is almost consistent at different heave amplitudes, indicating that heave amplitudes have little effect on the ${\alpha }_{max}$ corresponding to peak efficiency, which is consistent with the results of 2D flapping foils [4]. At the same time, it was found that the heave amplitude has a significant impact on the peak efficiency, and as the heave amplitude $y_0/c$ increases from 0.5 to 4.0, the peak efficiency improves significantly. In Figure 12, after the heave amplitude $y_0/c$ is greater than 2.5, the effect on peak efficiency becomes insensitive. In addition, comparing Figure 12a,b, it can be seen that the ${\alpha }_{\mathrm{m}\mathrm{a}\mathrm{x}}$ values corresponding to the efficiency peaks at the two pitch amplitudes are very close, which is consistent with the results in Figure 12a.

In Figure 13, under different heave amplitude conditions, the relationship between ${\alpha }_{max}$ of the flapping foils and K_T is not as obvious as in Figure 10b. However, it can still be observed that the effect of heave amplitude on the thrust coefficient is also significant. Smaller heave amplitudes (such as $y_0/c$ = 0.5) correspond to very large thrust coefficients, and there is no obvious peak point for the thrust coefficient, which continues to increase with the increase in the ${\alpha }_{max}$ value. Under conditions with larger heave amplitudes (such as the red and green curves in Figure 13), the ${\alpha }_{max}$ corresponding to the peak thrust coefficient still remains around 0.3 rad, which is close to the results in Figure 10b.

Figure 12. Propulsive efficiency η of a full-active flapping foil as function of ${\alpha }_{max}$, for a series of heaving amplitudes. (a) θ₀ = 0.3 rad, (b) θ₀ = 0.5 rad.

Figure 12. Propulsive efficiency η of a full-active flapping foil as function of ${\alpha }_{max}$, for a series of heaving amplitudes. (a) θ₀ = 0.3 rad, (b) θ₀ = 0.5 rad.

Figure 13. Thrust coefficient K_T of a full-active flapping foil as function of ${\alpha }_{max}$, for a series of heaving amplitudes. (a) θ₀ = 0.3 rad, (b) θ₀ = 0.5 rad.

Figure 13. Thrust coefficient K_T of a full-active flapping foil as function of ${\alpha }_{max}$, for a series of heaving amplitudes. (a) θ₀ = 0.3 rad, (b) θ₀ = 0.5 rad.

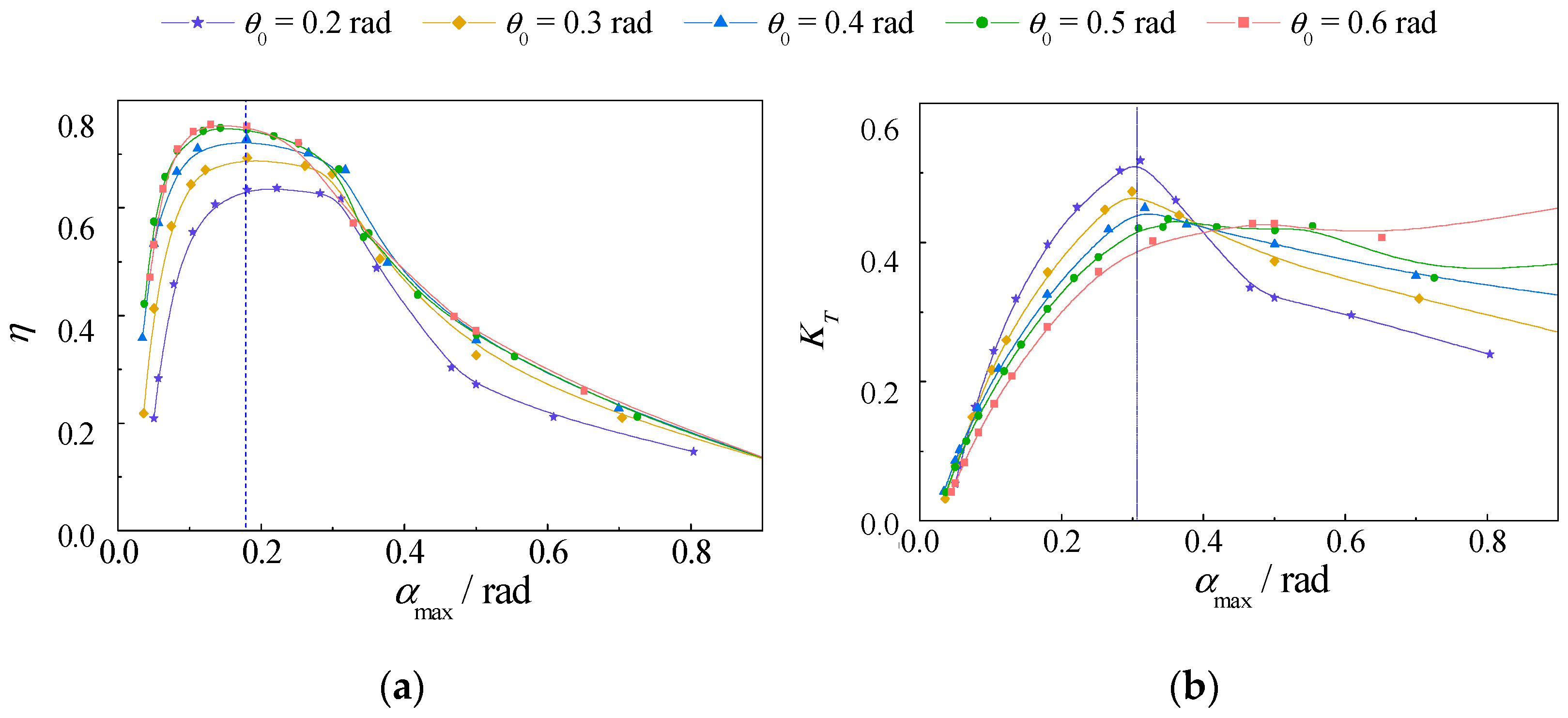
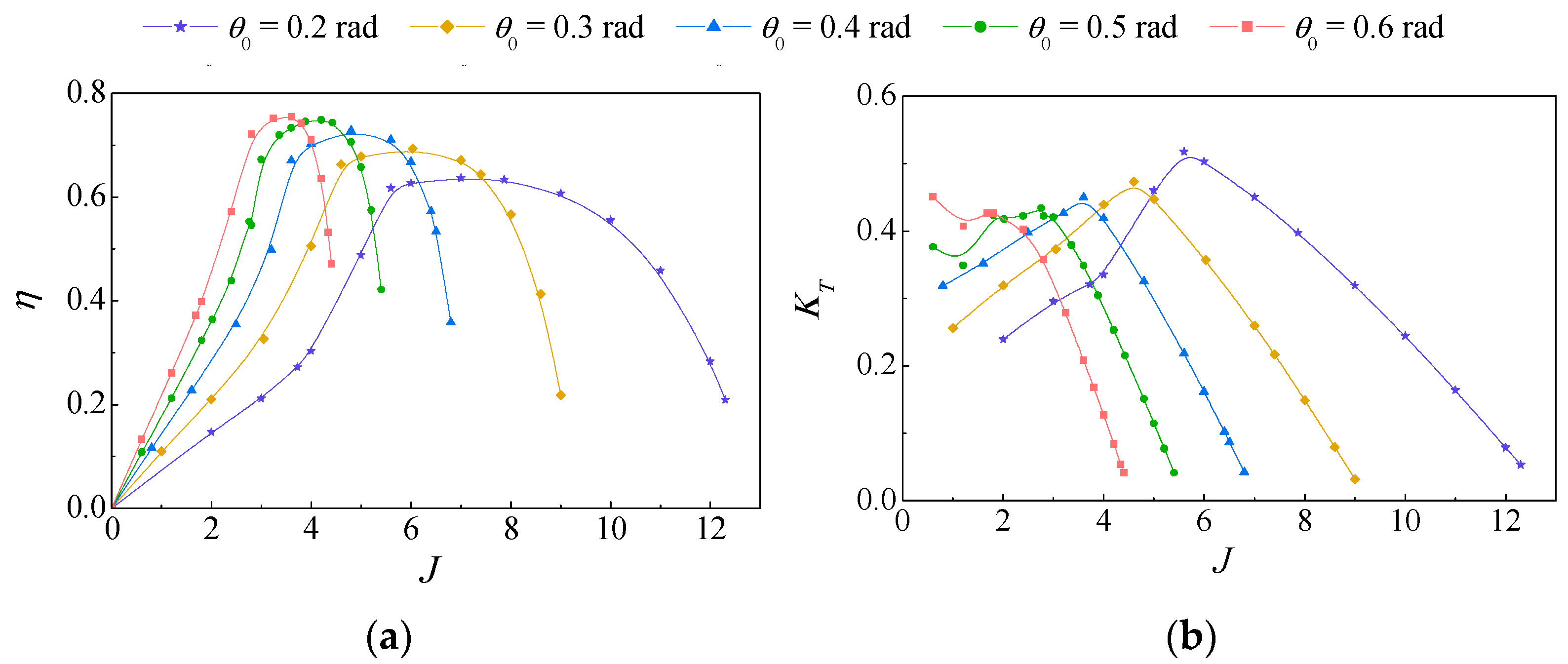
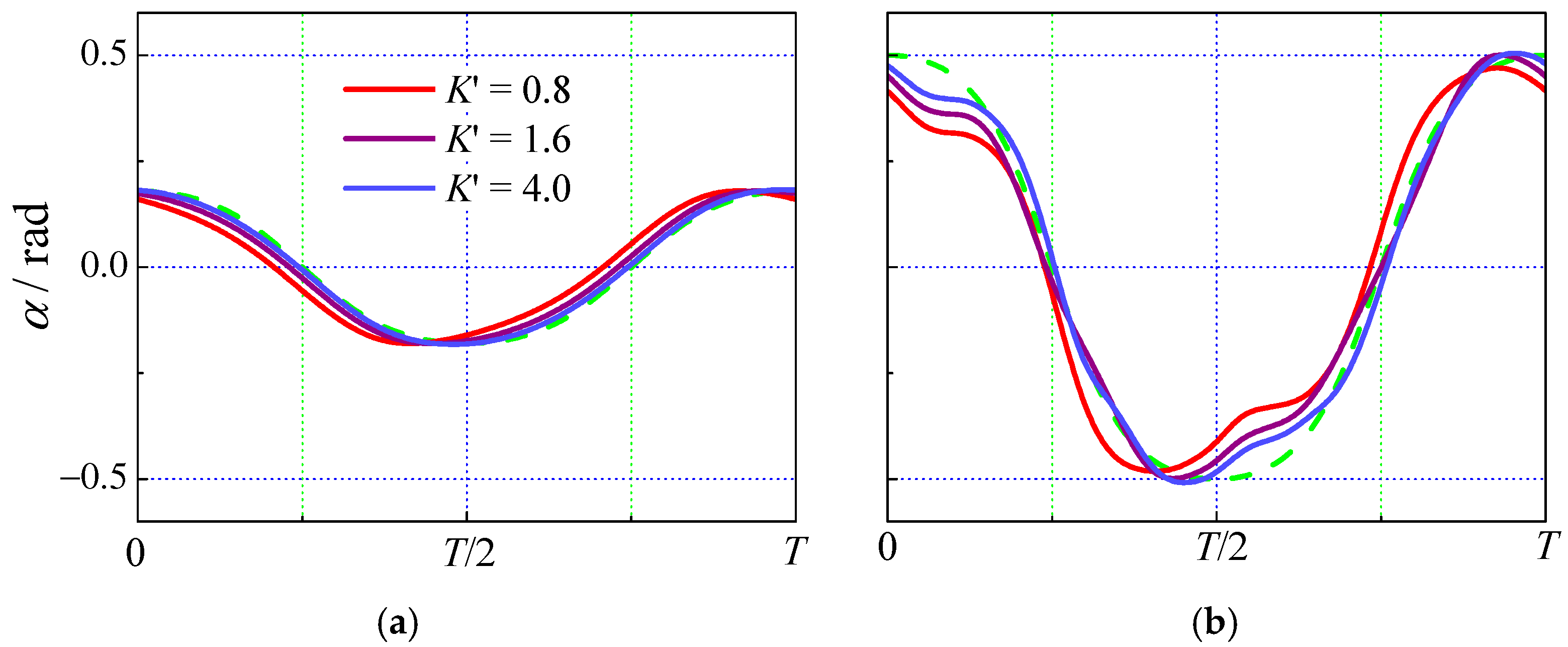
4.1.2. Semi-Active Flapping Foils

In the final part, we conducted a comparative analysis on the semi-active flapping foils and provided the curves of $\eta -{\alpha }_{max}$, $\eta -$ J, $K_T-{\alpha }_{max},\mathrm{a}\mathrm{n}\mathrm{d}{K}_T-$ J with different spring stiffness ratios (K′ = 0.8, 1.6, 4.0), which are shown in Figure 14 and Figure 15. For the purpose of comparison, some data of the $\eta -{\alpha }_{max}$ curves and $\eta -$ J curves of the full-active flapping foils with different pitch amplitudes are also presented in colored lines in Figure 14 and Figure 15 (the red line with hollow points represents the curve of the semi-active flapping foils, while the other colored lines with solid points describe the performance of the full-active ones with different pitch amplitudes). Thaweewat et al. [8] also made a similar comparison in their work. It can be seen from Figure 14 that there are many similarities between the $\eta$ curves of the full-active flapping foils with different pitch amplitudes and those of the semi-active flapping foils with different spring stiffness ratios.

Firstly, from Figure 14a, it can be seen that the envelope lines of the $\eta -$ J curves of the two kinds of flapping foils are almost identical, as shown by the black solid line in the figure, which is also mentioned in Thaweewat’s research [8]. They compared the performance of the semi-active flapping foils with a certain spring stiffness and full-active flapping foils with different pitch amplitudes, and found that semi-active swing wings can adapt well to different J numbers. They found that the efficiency curve of such semi-active propulsors tends to connect the peaks of each efficiency curve of the full-active flapping foils with different pitch amplitudes. Here, through further research on semi-active flapping foils with different spring stiffness ratios, it can be found that compared to full-active flapping foils, the working range of semi-active propulsors is generally wider. In addition, as the spring stiffness increases, the efficiency curve of semi-active propulsors shifts downwards to the right with the envelope line, resulting in a decrease in peak efficiency. That is, although semi-active propulsors have almost no effect on improving peak efficiency, they are beneficial for improving the high-efficiency working range of flapping foils. Comparing Figure 11b and Figure 15b, it can be seen that semi-active flapping foils and full-active ones have very similar thrust coefficient curves, and the peak thrust coefficient is also relatively close, so thrust will not be reduced due to the use of semi-active propulsors. Secondly, it can be clearly seen from Figure 14b that, similar to the case of active flapping foils, the position of the ${\alpha }_{max}$ corresponding to peak efficiency of semi-active propulsors is also relatively concentrated. Compared with the full-active propulsors, although the curve of the semi-active ones slightly shifts to the right, the ${\alpha }_{max}$ values corresponding to the peak efficiency points are still around 0.2 rad. It can be said that the ${\alpha }_{max}$ values corresponding to the peak efficiency of the semi-active and the full-active propulsors are basically the same. At the same time, it can be observed from Figure 14b that due to the tendency of the semi-active propulsors’ efficiency curve to shift to the right, the efficiency of semi-active propulsors is generally lower than that of full-active ones in smaller ${\alpha }_{max}$ region, while, on the right half of the efficiency curve, the efficiency of the semi-active ones is significantly higher than that of the full-active propulsors. Moreover, smaller spring stiffness is beneficial for achieving greater peak efficiency in semi-active propulsors.

Figure 14. Propulsive efficiency η of a semi-active flapping foil as function of ${\alpha }_{max}$and advance coefficient J for a series of spring stiffness ratios. (a) θ₀ = 0.3 rad, (b) θ₀ = 0.5 rad.

Figure 14. Propulsive efficiency η of a semi-active flapping foil as function of ${\alpha }_{max}$and advance coefficient J for a series of spring stiffness ratios. (a) θ₀ = 0.3 rad, (b) θ₀ = 0.5 rad.

Figure 15. Thrust coefficient K_T of a semi-active flapping foil as function of ${\alpha }_{max}$, for a series of heaving amplitudes. (a) $K_T-J$, (b) $K_T-{\alpha }_{max}$.

Figure 15. Thrust coefficient K_T of a semi-active flapping foil as function of ${\alpha }_{max}$, for a series of heaving amplitudes. (a) $K_T-J$, (b) $K_T-{\alpha }_{max}$.

Figure 15 further illustrates the analysis of the thrust coefficient for semi-active flapping foils with different spring stiffnesses. It is obvious that this curve is similar to the curve of full-active propulsors with different pitch amplitudes, and except for the k′ = 0.8 condition, the peak thrust coefficient or inflection point of the other two curves also appears around 0.3 rad. It is interesting that the k′ = 0.8 curve has not reached its peak point and continues to increase with the increase in the ${\alpha }_{max}$; we speculate that the small spring stiffness causes the pitching angle of the wing to be too large, resulting in an increase in the thrust direction component. This requires further analysis and exploration in the future. But the results in Figure 15b still could further prove the conclusion that the ${\alpha }_{max}$ not only plays a key role in propulsor efficiency but also has a significant impact on thrust. Similar to the analysis in Figure 10b and Figure 11b, the curve of the thrust coefficient changing with the advance coefficient will shift to the right with the increase in spring stiffness, and the peak value will gradually increase, indicating that the influence of spring stiffness on semi-active propulsors is similar to that of pitch amplitude on full-active ones. This has also been discussed in our previous research [36]. This is also easy to understand. Under the condition of a low advance coefficient, the ability of the spring with small stiffness (e.g., $K^{\prime }=0.8\mathrm{N}·\mathrm{m}/\mathrm{r}\mathrm{a}\mathrm{d}$) to resist hydrodynamic torque is low, which could avoid the flapping foils working at high AoA, resulting in higher thrust. At a higher advance coefficient, the relative flow velocity increases, and the hydrodynamic effect on the foil is enhanced. Therefore, the spring with higher stiffness (e.g., $K^{\prime }=4.0\mathrm{N}·\mathrm{m}/\mathrm{r}\mathrm{a}\mathrm{d}$) can resist the effect of hydrodynamic torque more strongly, so that the flapping foil can work at a larger AoA with higher thrust.

In summary, the existing research results show that the ${\alpha }_{max}$ has the great impact on flapping foil efficiency and thrust coefficient. Peak efficiency of full-active and semi-active propulsors usually occurs around ${\alpha }_{max}=0.2\mathrm{r}\mathrm{a}\mathrm{d}$, and within a certain range, they can both have high efficiency. Except for flapping foils with small heave amplitudes, the peak thrust coefficients of full-active and semi-active propulsors are approximately around ${\alpha }_{max}=0.3\mathrm{r}\mathrm{a}\mathrm{d}$. The working conditions with small heave amplitudes, due to their generally low efficiency, do not have much engineering application value, and will not be discussed here.

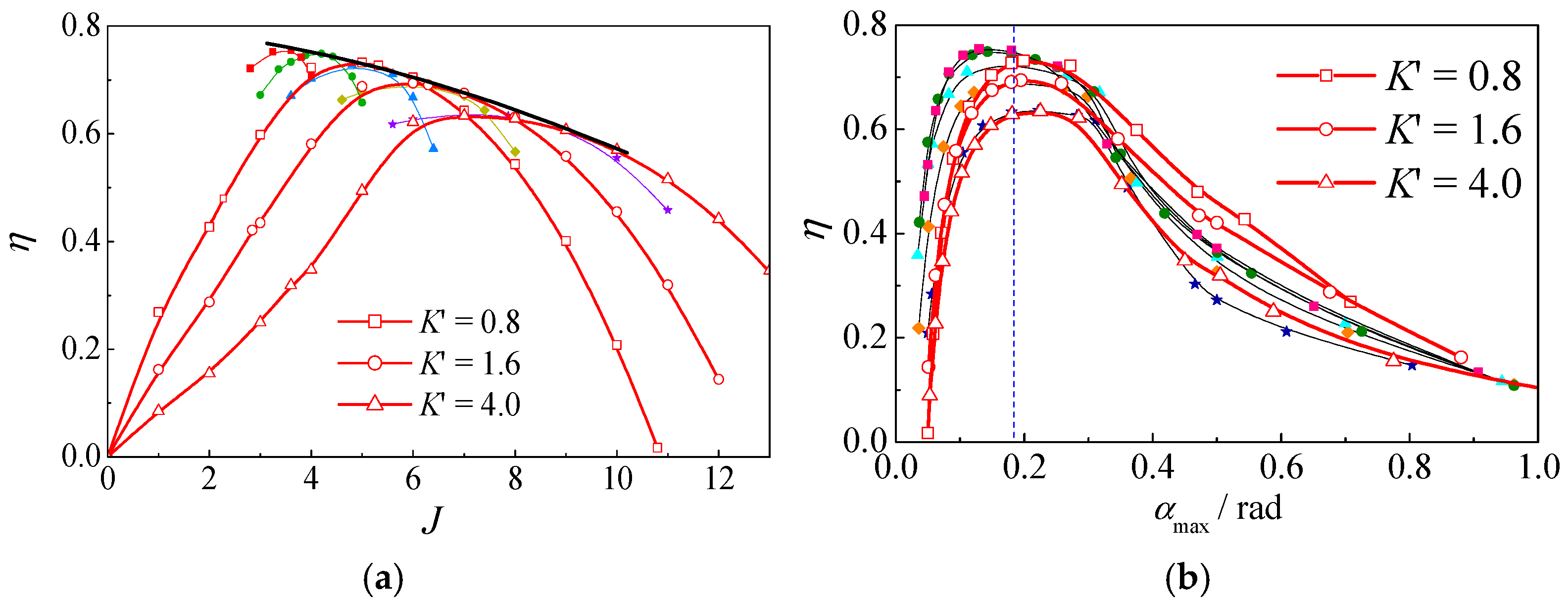
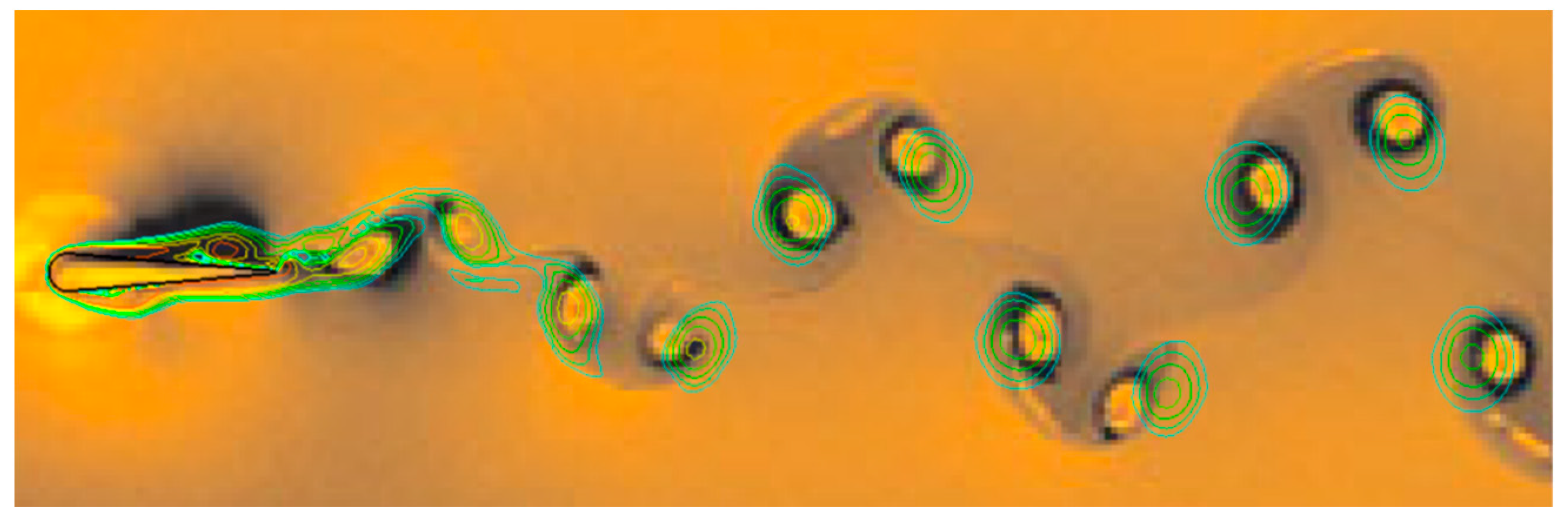
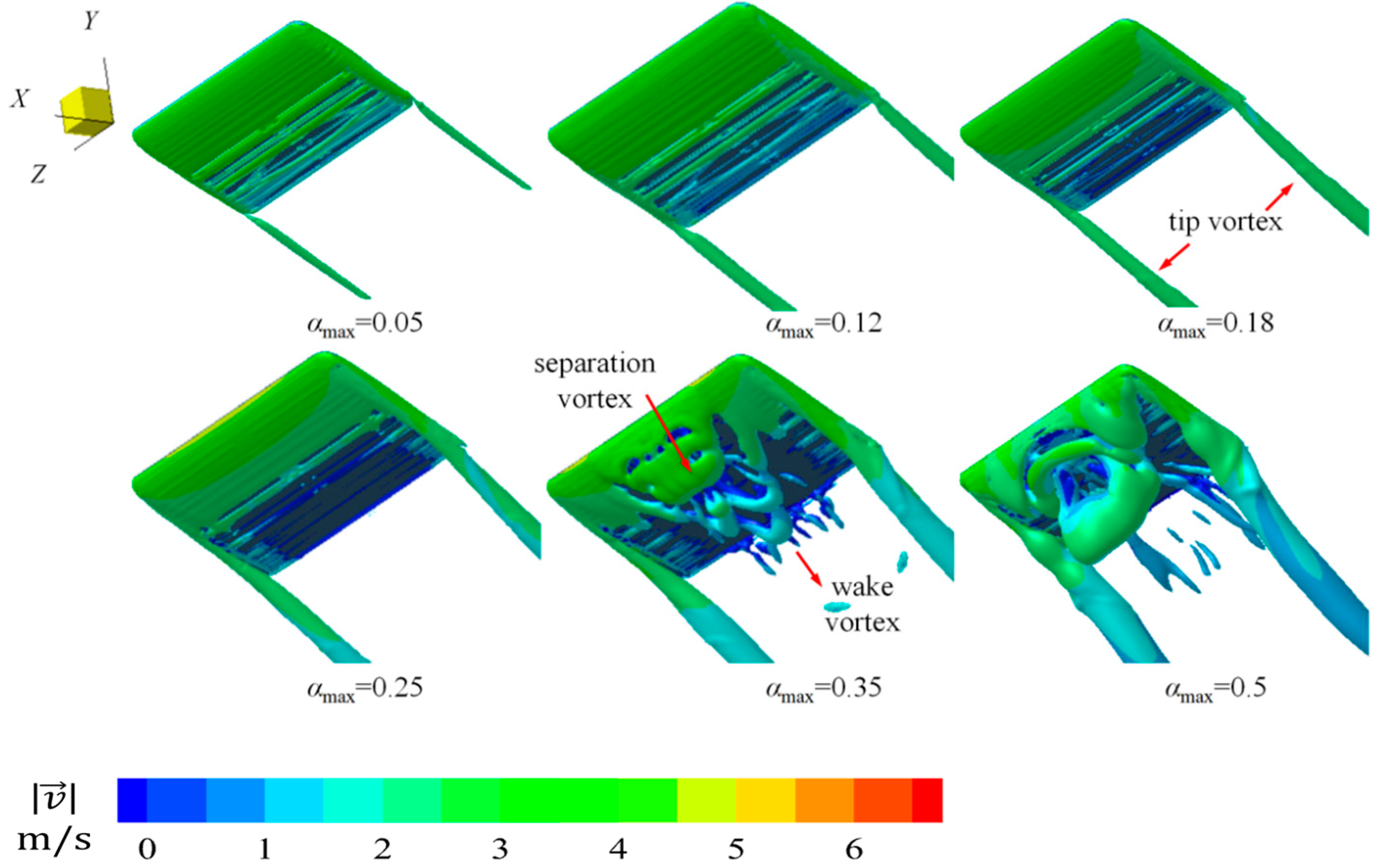
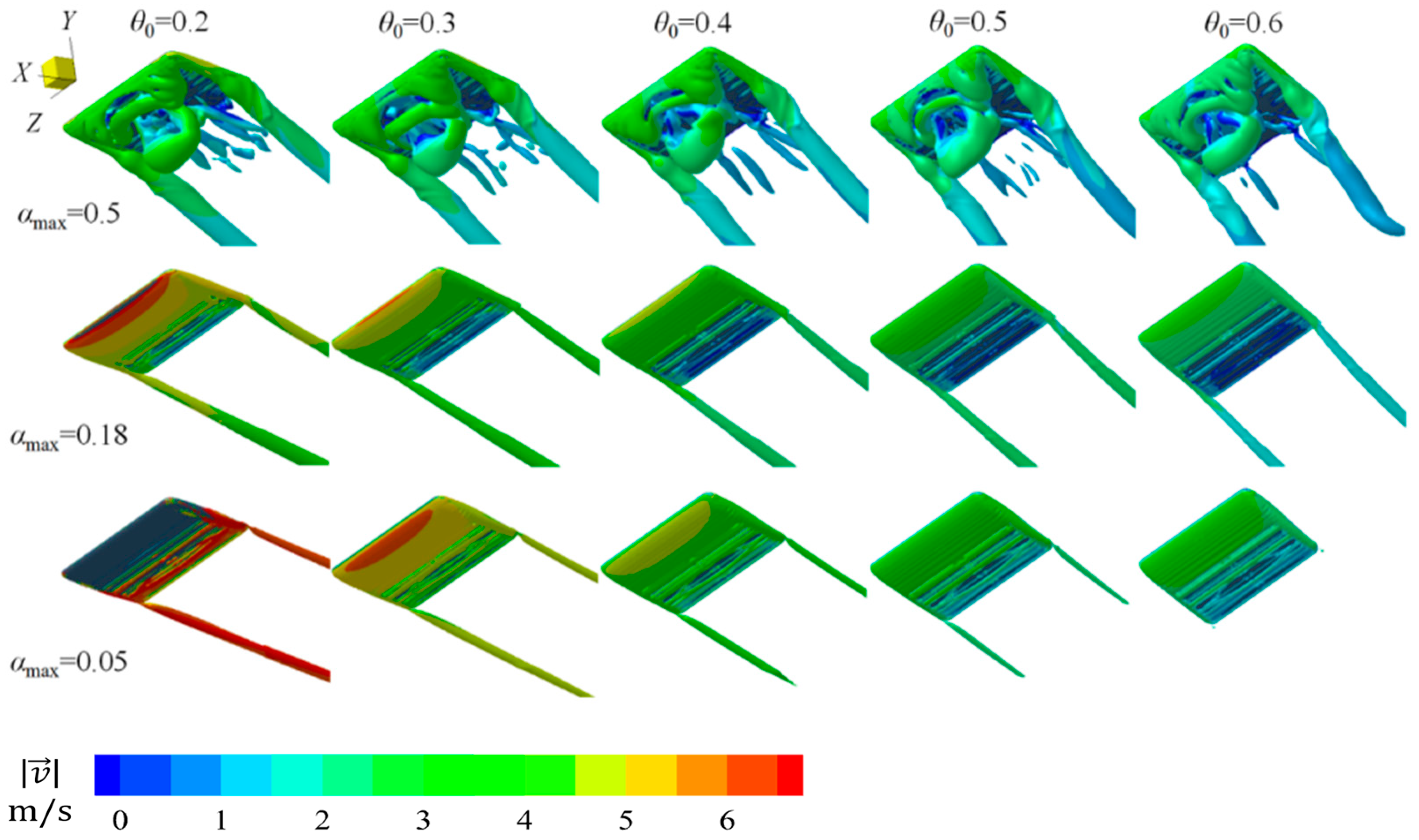
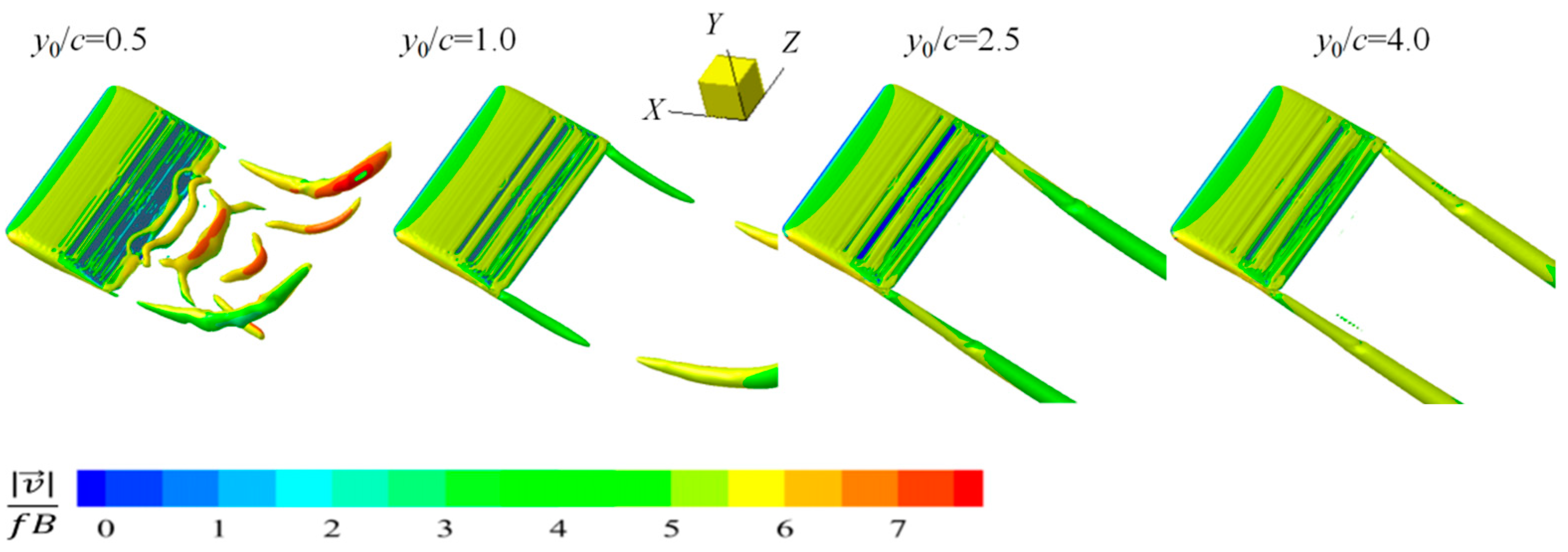
4.2. Analysis of the Vortex Structure

As the AoA deviates from the high efficiency ${\alpha }_{max}$ range, the efficiency of propulsors decreases rapidly. In order to analyze this phenomenon, the following sections will observe and analyze the flow field vortex structures of full-active and semi-active propulsors.

Firstly, the vortex structures of the full-active flapping foil are observed at different working conditions. The vortex structures at six different ${\alpha }_{max}$ values (0.05, 0.12, 0.18, 0.25, 0.35, and 0.5 rad) are listed under the conditions of $y_0/c=2.5$ and ${\theta }_0=0.5\mathrm{r}\mathrm{a}\mathrm{d}$, as shown in Figure 16. The variable of the scale is the relative velocity v, measured in m/s. The wakes in this figure are obtained using the contour surface of the Q-criterion variable [38]. All images used the same contour surface, and the contour surface was rendered using the same color value of relative velocity, in order to observe the magnitude of wake velocity. The figures show the moment when the flapping foil is at the full cycle time, at which point it is in the middle position of the heave motion, with the pitching angle reaching its maximum value and the AoA almost reaching its maximum value. From the graph, it can be seen that under smaller ${\alpha }_{max}$ conditions, as within the range of ${\alpha }_{max}=0.05~0.25\mathrm{r}\mathrm{a}\mathrm{d}$, the vortex structure of the flapping foils only has tip vortices at both ends. As the ${\alpha }_{max}$ increases, as within the range of ${\alpha }_{max}=0.25~0.5\mathrm{r}\mathrm{a}\mathrm{d}$, the vortex structure of the flow field becomes more complex. In addition to tip vortices, separation vortices on the suction surface and wake vortices begin to appear. Multi-vortex shedding from the leading edge, the trailing edge, and other parts of the foil’s surface (in the form of secondary vortices induced by leading-edge vortices) is the typical character of high AoA situations, which is consistent with previous research findings by Zhu [39].

From the above analysis, it can be seen that the vortex structure of the flapping foils mainly has two forms, namely form 1: only the tip vortex; form 2: simultaneous tip vortices, separation vortices, and wake vortices. Therefore, the following analysis mainly focuses on these two forms of vortex structures, selecting two typical working conditions with ${\alpha }_{max}$ of 0.05 and 0.5, respectively. At the same time, in order to observe the vortex structure of the peak efficiency point, the analysis of the working condition with ${\alpha }_{max}$ = 0.18 has been added. Figure 17 shows the vortex structure of the flow field of a full-active flapping foil with different pitch amplitudes at three maximum angles of attack, with a certain lift and sink amplitude. The variable of the scale is the relative velocity v, m/s. Overall, ${\alpha }_{max}$ has a much greater impact on the wake structure than the pitch amplitude. For the first row working condition, due to the large ${\alpha }_{max}$, the wake vortices at different pitch amplitudes are much more complex than the other two, with obvious tip vortices at both ends, separation vortices on the suction surface, and wake vortices behind the flapping foil. For the latter two row working conditions, only the tip vortex is present in the wake vortex, and their wake flow characteristics are relatively consistent, showing a relatively straight shape in the early stages of development. In the last row, under the working condition of ${\alpha }_{max}=0.05\mathrm{r}\mathrm{a}\mathrm{d}$, due to the ${\alpha }_{max}$ value being too small, the tip vortex is significantly weakened, manifested as a thinner tip vortex and insufficient development, especially in the case of ${\theta }_0=0.6\mathrm{r}\mathrm{a}\mathrm{d}$, as shown in the bottom right corner of Figure 17; the tip vortex almost cannot be observed or distinguished. It can be inferred from the graphs that a too large or too small ${\alpha }_{max}$ is not conducive to the generation of thrust. It can be understood that when the ${\alpha }_{max}$ is too large, there is obvious separation phenomenon on the flapping foil surface, which will not produce effective lift, thus affecting the generation of thrust. When the ${\alpha }_{\mathrm{m}\mathrm{a}\mathrm{x}}$ is too small, the AoA decreases, and the lift coefficient of the airfoil decreases, leading to reduced thrust.

From a horizontal comparison, although the advance coefficient J varies at different pitch amplitudes, the vortex structures around the flapping foils are almost the same due to the consistent ${\alpha }_{max}$, making it difficult to explain the significant differences in efficiency at different pitch amplitudes. According to the forces acting on the flapping foil, lift L and drag D, which are generated at relative inflow velocities, can be decomposed into thrust F_x and lateral forces F_y in terms of flapping foil performance, as shown in Figure 18. It can be seen from the force relationship that under a certain angle of attack α, when the pitching angle θ is larger, the larger the relative flow angle (α + θ), the smaller the impact of drag on the reduction in thrust, resulting in smaller power loss and higher efficiency. Because the relative flow angle (α + θ) is only related to velocity, and the velocity relationship can be represented by the advance coefficient J. And further analysis shows that the advance coefficient J of a flapping foil at the moment of ${\alpha }_{max}$ varies at different pitch amplitudes, as shown in

Table 2. Advance coefficient J of flapping foil at the moment of ${\alpha }_{max}$ at different pitch amplitudes.

J	$${\mathit{\theta }}_0=0.2 \mathbf{r}\mathbf{a}\mathbf{d}$$	$${\mathit{\theta }}_0=0.3 \mathbf{r}\mathbf{a}\mathbf{d}$$	$${\mathit{\theta }}_0=0.4 \mathbf{r}\mathbf{a}\mathbf{d}$$	$${\mathit{\theta }}_0=0.5 \mathbf{r}\mathbf{a}\mathbf{d}$$	$${\mathit{\theta }}_0=0.6 \mathbf{r}\mathbf{a}\mathbf{d}$$
${\alpha }_{max}$ = 0.05	3.73	3.05	2.49	2.02	1.6
${\alpha }_{max}$ = 0.18	7.87	6.03	4.8	3.87	3.2
${\alpha }_{max}$ = 0.5	12.3	8.6	6.51	5.21	4.34

. As the pitch amplitude increases, the advance coefficient J decreases significantly, which can also be seen from the magnitude of wake velocity in Figure 17. Therefore, it can also be said that the reason for the higher peak efficiency of the flapping foil with lower pitch amplitude is due to the lower inlet coefficient J at its peak efficiency point. Similar explanations have also been used in Floryan et al.’s [3] study.

$${\alpha }_{max}$$

$${\alpha }_{max}$$

Figure 19 shows the vortex structure of a full-active propeller with a pitch amplitude ${\theta }_0=0.5 \mathrm{r}\mathrm{a}\mathrm{d}$ and a series of different heave amplitudes. Due to the fact that at a constant frequency, a larger heave amplitude corresponds to a larger heave velocity, the velocity cloud map in Figure 19 is dimensionless. The figures show the moment when the flapping foils are at the full cycle time, at which point it is in the middle position of the heave motion. By comparison, it can be seen that at larger heave amplitudes, the vortex structure of the flapping foil only consists of tip vortices. As the heave amplitude decreases, the tip vortex gradually becomes curved. This is because due to the reduction in heave amplitude, under similar dimensionless velocity conditions, the advancing velocity V_A decreases, and the advance of the propeller within one cycle decreases, resulting in significant trajectory fluctuations of the tip vortex. At the same time, the vortex structure becomes complicated, in the case of y₀/c = 0.5, in the suction surface of the flapping foil, which should be an important reason for the low peak efficiency of the flapping foil with small heave amplitude.

Figure 20 shows the vortex structure of a semi-active propeller with different spring stiffness ratios ($K^{\prime }$ = 0.8, 1.6, 4.0 rad). It can be seen that at the working points corresponding to three different values of ${\alpha }_{max}$, their characteristics are consistent with those of full-active propellers. That is, at larger ${\alpha }_{\mathrm{m}\mathrm{a}\mathrm{x}}$, the flow field exhibits a more complex vortex structure, while at the peak efficiency point (${\alpha }_{max}$ = 0.18) and a smaller ${\alpha }_{max}$ point, only tip vortices exist in the flow field. Further comparison with Figure 17 reveals that, at the working point of ${\alpha }_{max}$ = 0.5, the vortex structures of semi-active propellers with different spring stiffnesses and full-active propellers with different pitch amplitudes have slightly different vortex structures, especially with spring stiffness coefficients of 0.8 and 1.6; their separation vortex sizes are much smaller. Meanwhile, by comparing the efficiency of these eight working points in Figure 14b, it can be observed that the semi-active propeller efficiency is significantly higher than full-active propellers at ${\alpha }_{max}$ = 0.5. We speculate that the difference in surface vortex size may be the reason for the difference in efficiency at positions with a large ${\alpha }_{max}$.

4.3. AoA Trajectory

In previous studies, many researchers have focused on the influence of the AoA characteristics on the performance of 2D flapping foils, and found that imposing a non-sinusoidal effective AoA profile could affect the performance of flapping foils, including efficiency and thrust coefficient [20,21,22,23,24]. In order to further explore the reasons for the pitch amplitude influence on peak efficiency, Figure 21 shows the AoA time-history curves of the full-active propeller at the maximum efficiency operating point (${\alpha }_{max}=0.18\mathrm{r}\mathrm{a}\mathrm{d}$) at five pitch amplitudes. It can be seen that as the pitch amplitude increases, the AoA curve gradually becomes fuller and tends from a sinusoidal waveform to a square-like wave. This phenomenon corresponds to the result in Figure 10, that is, as the pitch amplitude of the flapping foil increases, the peak efficiency of the full-active propeller gradually increases. It can indirectly explain the reasons why the peak efficiency achieved by the flapping foils varies at different pitch amplitudes.

From the results in Figure 14, it can be seen that semi-active propellors with different spring stiffnesses and full-active propellors with different pitch amplitudes have very similar $\eta -{\alpha }_{max}$ curves. Their peak thrust coefficient and efficiency are relatively close. At the same time, the ${\alpha }_{max}$ value for obtaining peak efficiency is also very close between the two. In order to further explore the relationship between the efficiency change of the semi-active propellers and the AoA trajectory, the AoA curves of the semi-active propellers with different spring stiffnesses are also compared in this part, as shown in Figure 22. For the convenience of comparison, the AoA time-history curve of the active propellor under the condition ${\theta }_0=0.3\mathrm{r}\mathrm{a}\mathrm{d}$ is also listed in this figure, as shown by the green dashed line. Figure 22a shows the highest efficiency working point (${\alpha }_{max}=0.18\mathrm{r}\mathrm{a}\mathrm{d})$, and Figure 22b shows the high angle of attack working point (${\alpha }_{max}=0.5\mathrm{r}\mathrm{a}\mathrm{d}$). As the spring stiffness decreases, there is no significant difference in the shape of the AoA trajectory of the semi-active propellers. It is only that the AoA trajectory gradually shifts to the left, which is consistent at the two working points in Figure 22. At the highest efficiency working point of ${\alpha }_{max}=0.18\mathrm{r}\mathrm{a}\mathrm{d}$, the AoA curves advance by approximately 31°, 19°, and 7°, when spring stiffness $K^{\prime }=0.8,1.6,4.0$, respectively. At the working point of ${\alpha }_{max}=0.5\mathrm{r}\mathrm{a}\mathrm{d}$, the AoA curve shifts more significantly in the three cases, leading by approximately 34°, 24°, and 18°, respectively.

Then, in order to analyze the reasons for the forward movement phenomenon in the AoA curve of the semi-active flapping foils, we observed the pitching angle θ and AoA time-history curve with different values of ${\alpha }_{max}$(${\alpha }_{max}=0.18,0.5\mathrm{r}\mathrm{a}\mathrm{d}$), as shown in Figure 23. The phase difference between the pitching angle curve and the heave motion curve of semi-active propellers in this paper is approximately 90 degrees, which is consistent with the phase difference usually set in the typical active flapping foil, while the phase of AoA curve changes with the different of ${\alpha }_{max}$. It can be seen that with spring stiffness $K^{\prime }=0.8$, the maximum pitching angle of both the semi-active propellers occurs at the moment when it is slightly to the right of 1/2T position (red and blue dashed lines in Figure 23). According to the definition of AoA, it is known that due to the right shift of the maximum pitching angle, the value of the AoA at that moment will be smaller, resulting in the position of the ${\alpha }_{max}$ being shifted to the left. The phenomenon of the maximum pitching angle of the semi-active propellers deviating to the right can be understood as a slight increase in pitching angle after the middle position due to the inertia effect and hydrodynamic hysteresis effect. This phenomenon that the pitching angle is not synchronized with the time-history curve of AoA was also reflected in the results of Zhang [5] and Thaweewat [8].

In summary, from the AoA curve of semi-active propellers, it can be seen that, unlike the AoA trajectory of full-active propellers at different pitch amplitudes in Figure 21, the shape of the AoA trajectory with different spring stiffnesses is similar, and the difference in fullness is small, but their efficiency difference is significant. Therefore, it is difficult to evaluate the efficiency of semi-active propellers based on the degree of fullness of the AoA trajectory. However, due to the application of springs and the influence of spring stiffness and other factors, the AoA trajectory of the semi-active propellers exhibits a phase difference from the pitching angle curve, and the AoA trajectory shows an asymmetric shape. In the future, it may be necessary to start from these aspects and further explore the correlation between the shape of the AoA trajectory and the efficiency of semi-active propellers, seeking the key factors and reasons for the differences in efficiency of semi-active propellers.

5. Conclusions

The AoA characteristics of active and semi-active small aspect ratio flapping foils are investigated systematically in this work. The three-dimensional viscous flow over the flapping foil is simulated by the CFD method, with a focus on the impact of AoA on flapping foil efficiency. The parameter analysis of the two kinds of oscillating foils with different motion parameters are discussed, including AoA, pitching angle, heaving amplitude, spring stiffness, and trajectory.

• The $\eta -{\alpha }_{max}$ and K_T $-{\alpha }_{max}$ curves of full-active and semi-active flapping foils were analyzed separately. The results show that for the small aspect ratio NACA 0012 flapping foils in this paper, regardless of the active or semi-active form, the peak efficiency generally appears around α_max = 0.2 rad, and can have high efficiency (75%) within a certain range. While the peak thrust coefficient (0.5) is concentrated around α_max = 0.3 rad, and after exceeding this point, the thrust coefficient slowly decreases;
• To analyze the rapid reduction in efficiency after deviation from the peak efficiency point, the flow field vortex structure of the full-active and semi-active flapping foils is observed. Three typical operating point positions (${\alpha }_{max}=0.05,0.18,0.5\mathrm{r}\mathrm{a}\mathrm{d}$) were compared under various conditions. It is evident that when the ${\alpha }_{max}$ is small the flow-field vortex structure of the flapping foil is relatively simple, only tip vortices at both ends; while when the ${\alpha }_{max}$ is larger, the flow-field vortex structure becomes complicated. Moreover, the dissipation of these complex vortex systems is one of the reasons for the lower efficiency at this operating point. In addition, at small heave amplitudes, even at peak efficient operating points (${\alpha }_{max}=0.18\mathrm{r}\mathrm{a}\mathrm{d}$), there still are obvious separation vortices, which is probably the reason for the lower efficiency of flapping foils with small heave amplitudes;
• In the analysis of the $\eta -{\alpha }_{max}$ and K_T $-{\alpha }_{max}$ curves of the flapping foil, it was also found that although the peak efficiency usually appears around ${\alpha }_{max}=0.2\mathrm{r}\mathrm{a}\mathrm{d}$, there are still significant differences in the peak efficiency at different pitch amplitudes, heave amplitudes, and spring stiffnesses. Flapping foils with larger pitch and heave amplitudes, as well as smaller spring stiffness, will have higher peak efficiency. It can be roughly inferred that, except for the difference in peak efficiency caused by complex vortex systems at different heave amplitudes, the difference in peak efficiency between the other two is caused by different values of the advance coefficient J.

In summary, different from previous studies on 2D problems with low Reynolds numbers or small amplitude ranges, this paper systematically and comprehensively investigates the AoA characteristics of commonly used full-active and semi-active flapping foils in the field of propulsion. The analysis in this article covers the full range of thrust coefficients, starting from around 0 and ending with a thrust drop of 0. The accurate relationship between AoA characteristics and the peak points of efficiency and thrust coefficient for both two kinds of flapping foils are obtained. This study will help us determine the AoA range of flapping foils with high propulsion efficiency and thrust coefficient, providing necessary theoretical guidance for the design of flapping foil propulsion in marine applications. The numerical results agree well with previous experimental measurements and, in addition, allow access to the velocity and vorticity fields as functions of space and time, which in turn allows us to identify the underlying thrust production mechanisms more easily. These findings are based on results obtained by varying one parameter while keeping the others fixed. Hence, further research and verification of the results will require exhaustive investigation by 3D experiments in the future.