Validation of an Enhanced Drinking Water Temperature Model during Distribution
Abstract
1. Introduction
2. Materials and Methods
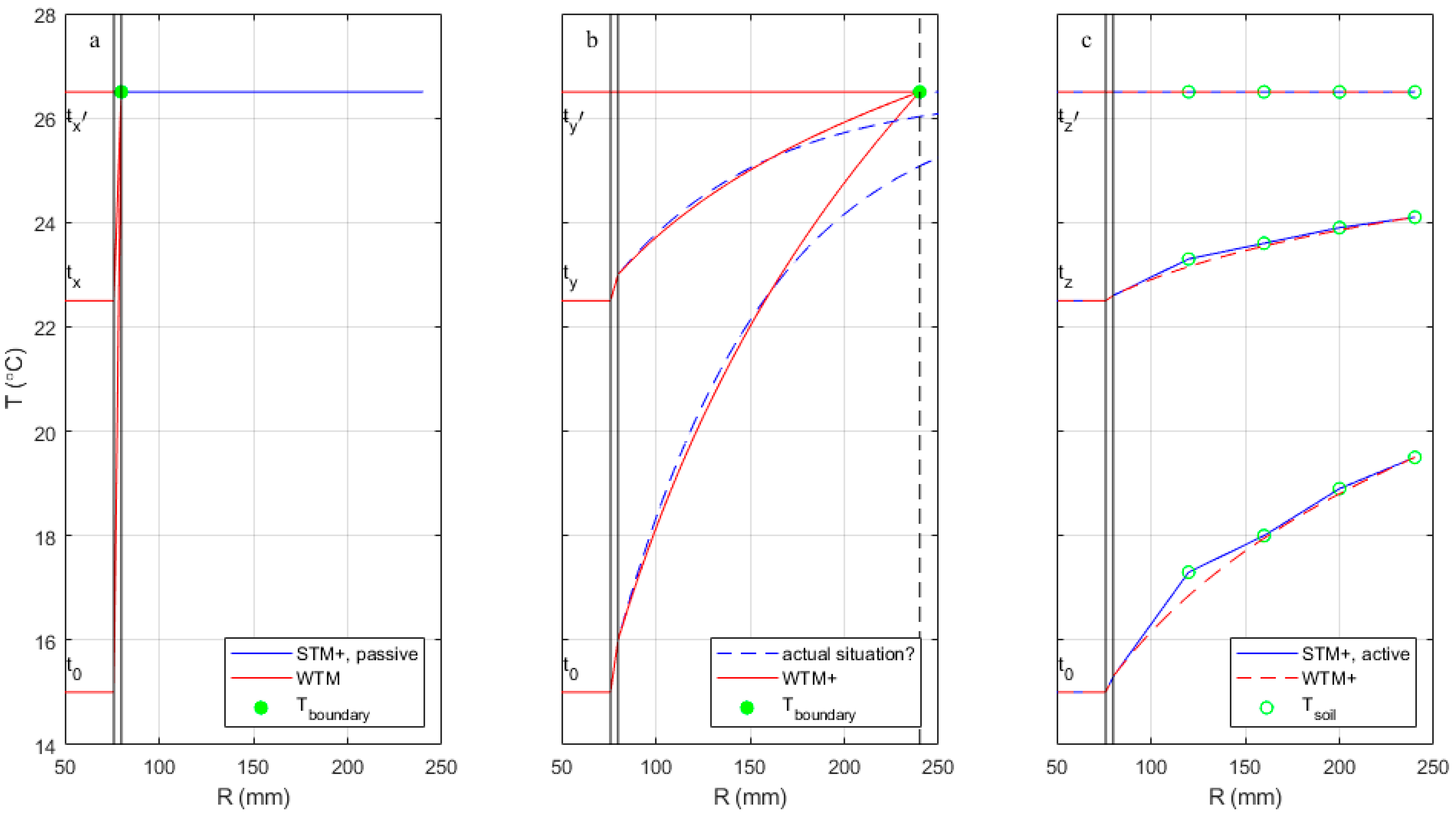
2.1. The Water Temperature Model (WTM+)
2.2. Tboundary from the Soil Temperature Model (STM+)
2.3. Model Normalization for Validation
2.4. Case Studies
2.5. Description Case Study 1—Single Pipe, Ø160 mm PVC
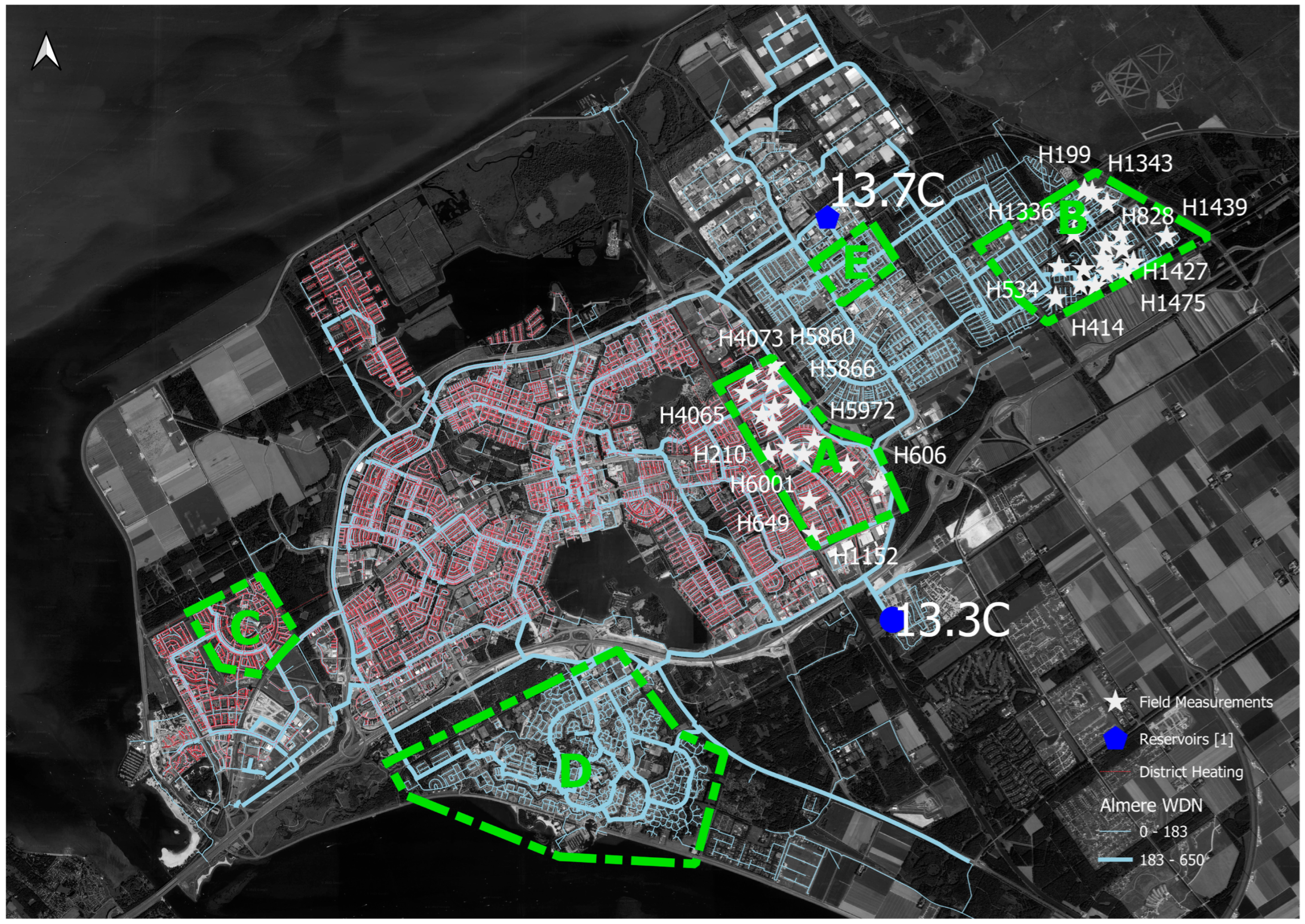
2.6. Description Case Study 2—Drinking Water Distribution Network
3. Results
3.1. Case Study 1—Single Pipe
3.2. Case Study 2—DWDN
4. Discussion
4.1. Reflection on the Model Approach
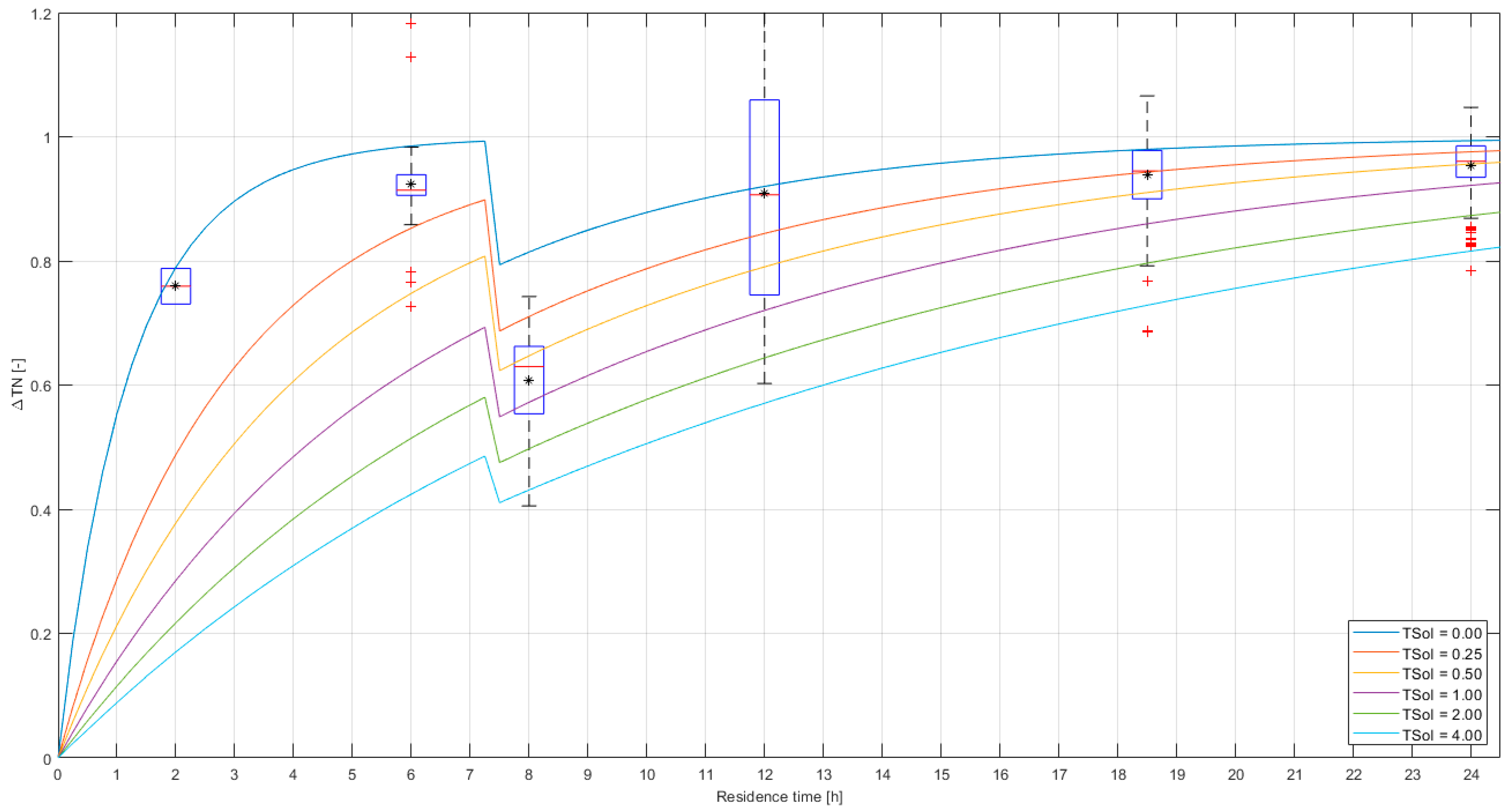
4.2. Estimation of TSoI
4.3. Recommendations for Validation Measurements
4.4. Practical Application
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Equations for Error Estimation
Appendix B. Case Study 1
Appendix B.1. Additional Information on Case Study 1
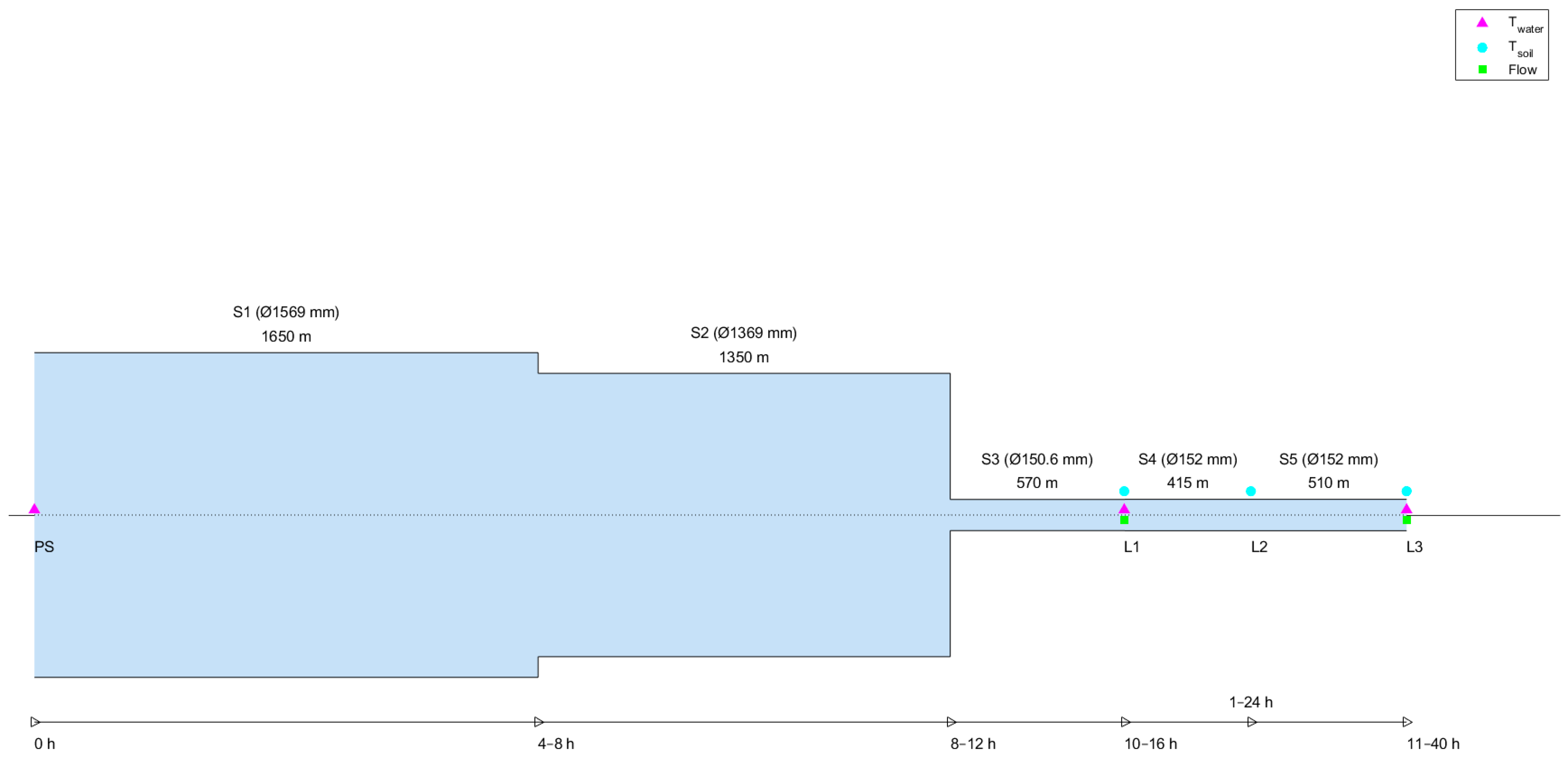
- Tsoil (temperature of the soil) was measured at L1, L2, and L3, at various distances from the pipe.
- The flow was measured at L1 and L3. During the measurements, there was limited demand from customers along the pipe, as there is only one residential customer, and some sports facilities that were closed during the COVID-19 pandemic. The demand at the customer location was measured, and it had a negligible effect on the flow at L1. The residence time between L1 and L3 was calculated from the pipe length, pipe diameter, and flow at L3.
- Note that the flow through stretches S1, S2, and S3 are not measured.
| ID | Start Time | End Time | Flow Rate [m3/h] | Residence Time [h] | Re [103] | Nu [-] |
|---|---|---|---|---|---|---|
| 1 | 19 May 6:30 (18 May 12:00) | 20 May 16:00 | 2.1 | 8.0 | 4.4 | 3.66 |
| 2 | 20 May 16:15 | 22 May 12:30 | 1.4 | 12.0 | 2.9 | 3.66 |
| 3 | 22 May 13:00 | 25 May 16:30 | 0.7 | 24.0 | 1.5 | 3.66 |
| 4 | 25 May 16:45 | 27 May 08:30 | 1.4 | 12.0 | 2.9 | 3.66 |
| 5 | 27 May 09:15 | 27 May 20:00 | 16.7 | 1.0 | 35.0 | 221.49 |
| 6 | 28 May 08:00 | 28 May 15:00 | 8.5 | 2.0 | 17.8 | 129.04 |
| 7 | 28 May 15:30 | 29 May 18:45 | 1.4 | 12.0 | 2.9 | 3.66 |
| 8 | 29 May 19:00 | 2 June 07:45 | 0.9 | 18.6 | 1.9 | 3.66 |
| 9 | 2 June 08:15 | 3 June 10:00 | 2.8 | 6.0 | 5.9 | 53.08 |
| Parameter | Unit | Value | Uncertainty | Reference |
|---|---|---|---|---|
| D1 | mm | 152 | - | Information from water utility Evides |
| D2 | mm | 160 | - | |
| Nu | - | Table A1 | Unclear beforehand where transition between turbulent and laminar flow is found | For turbulent flows: Nu = 0.027 Re0.8 Pr0.33 For laminar flows: Nu = 3.66 [4] |
| λpipe | Wm−1K−1 | 0.16 | - | PVC [4] |
| D3 | mm | D2 + TSoI × D1 | To be determined | |
| λsoil | Wm−1K−1 | 1.6 | ± 0.3 °C | Measured [17] |
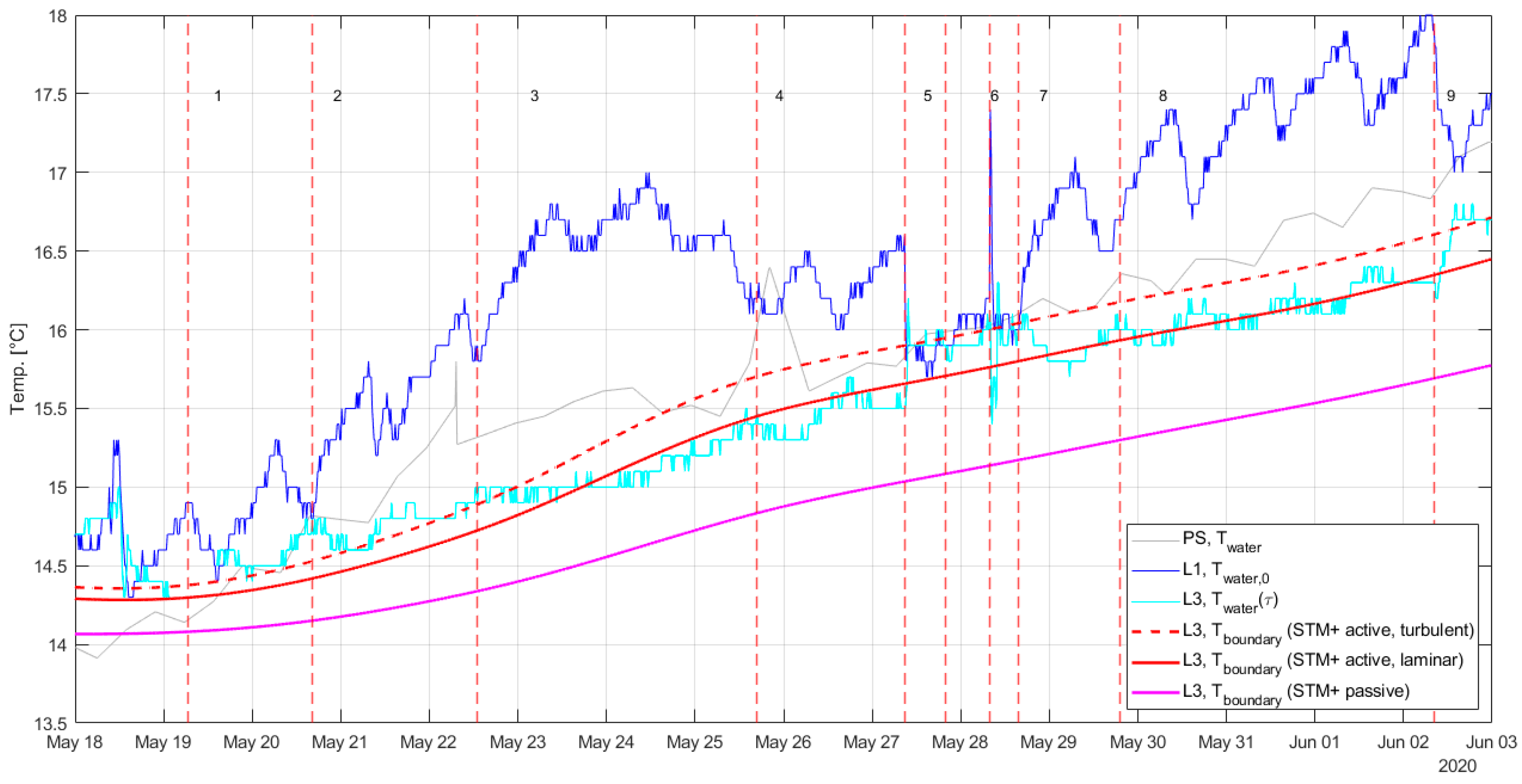
| Twater,0 | °C | Time series, Figure 4. | ± 0.1 °C | Measured (L1) |
| Twater | °C | ± 0.1 °C | Measured (L3) | |
| Tboundary | °C | ± 0.1 °C | For flowing water, from STM+ |
Appendix B.2. Measurement Data Processing
- : the residence time follows from the measured flows.
- : drinking water temperature measured at location L1.
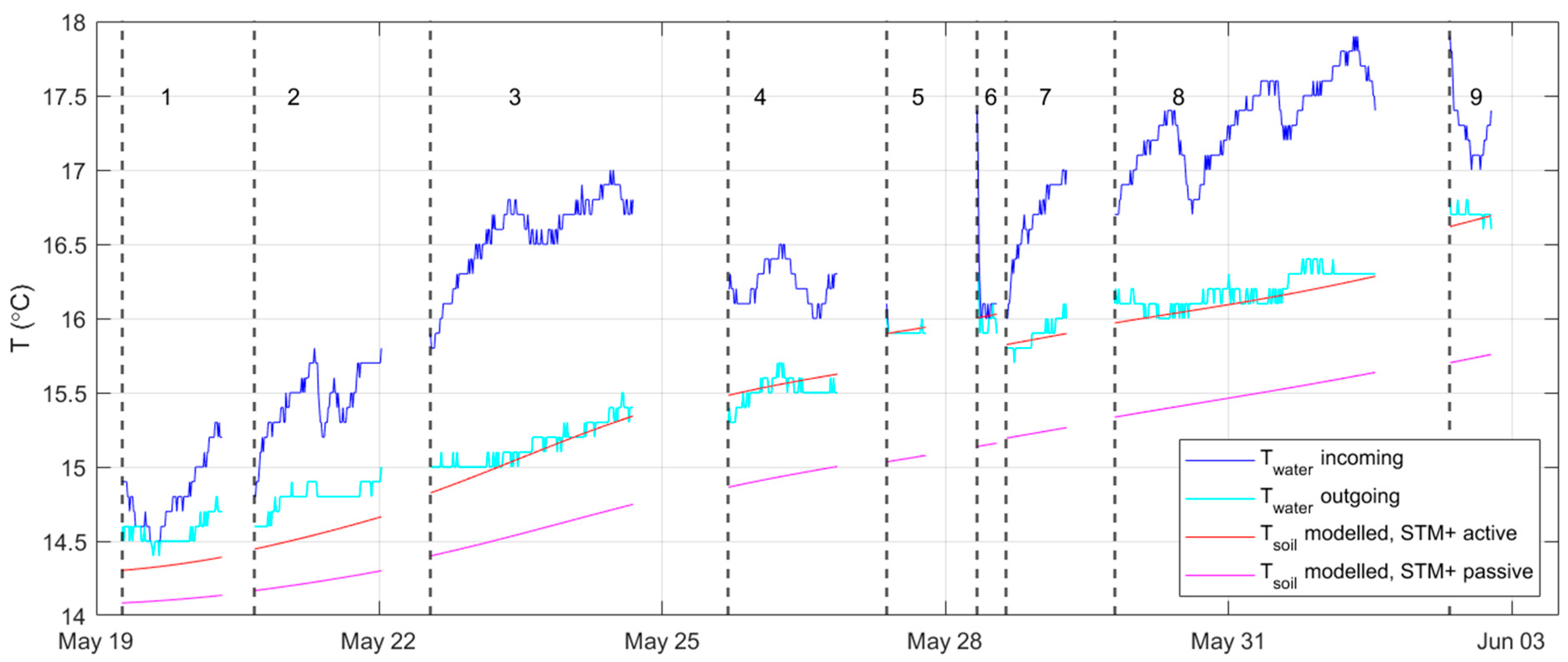
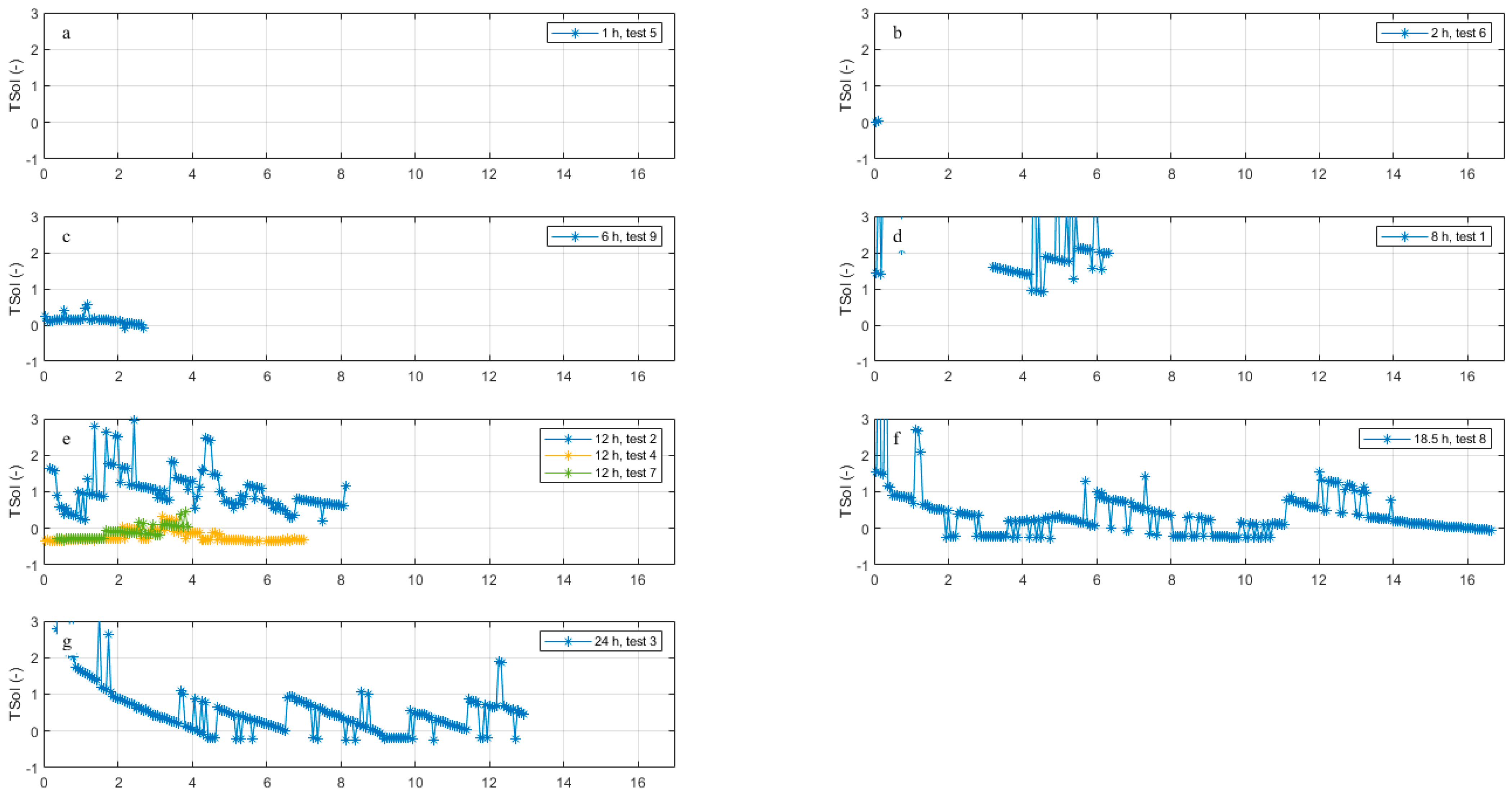
- : drinking water temperature measured at location L3, corrected for residence time τ (). For example, for test 4, Q = 1.4 m3/h and thus τ = 5 (L1 to L2) + 7 h (L2 to L3). Therefore, at L3,, where the data during the transition time of 12 h are discarded. Table A3 shows the residence times and the amount of data points left for the analysis after discarding the transition period (see also Figure A3).
- : Tboundary was estimated with the STM+ results for L3. We thus assume that the same boundary condition is valid for the entire pipe length between L1 and L3. For the tests, we assume that, during the residence time, the Tboundary that is experienced by the flowing water is the average of the modeled soil temperature during this residence time: (see also Figure A3). Note that Tboundary for flowing water was used for turbulent and laminar flows. In Figure A5, the test results with Tboundary for stagnant water are shown.
- : the accuracy can be estimated as . The accuracy of the flow meter is 0.004 m3/h (1 liter with a log frequency of 15 min). During the test phases, the flow is kept more or less constant, and the accuracy plus variability lead to a ΔQ equal to 0.012 m3/h. For the flow rates of Table A1, this results in less than 1 min for the short residence times of test 5 and 6 (τ = 1 and 2 h, respectively) and almost 30 min for the longest residence time of test 3 (τ = 24 h). This means an uncertainty in the residence time of less than 2%, and therefore, it is neglected.
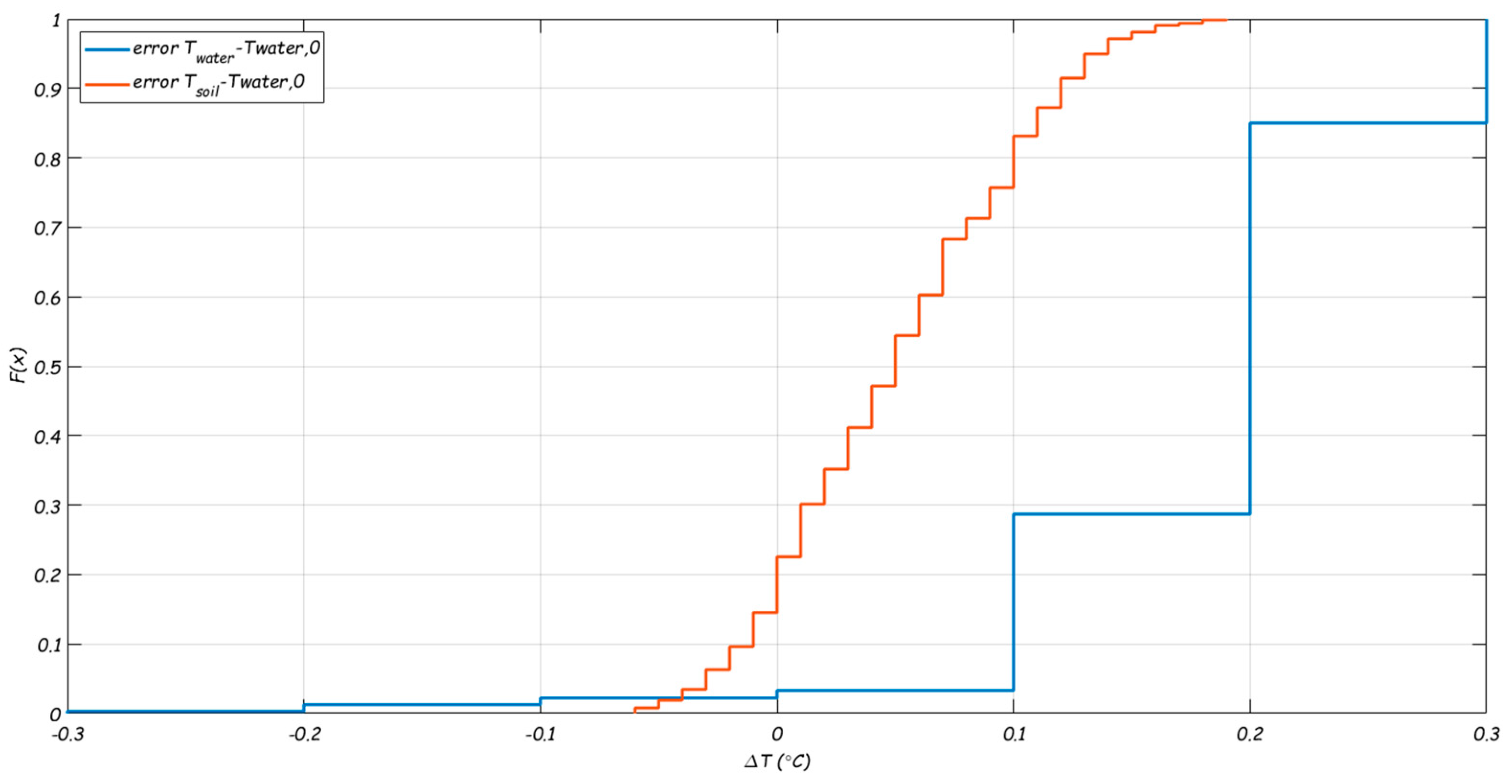
- : the accuracy is related to the accuracy of drinking water temperature measurements. The accuracy in and is ± 0.1 °C. The calibration that was carried out after the measurement period showed an average error between the two sensors at L1 and L3 (i.e., and ) of 0.2 °C (2 × εw = 0.2 °C, Figure A2). The uncertainty for the modeled is estimated as 0.1 °C [17]. When these errors have the opposite sign, the largest error will occur. With εw = −εs = 0.1 °C, the uncertainty in is determined as (see Appendix A) . This means that, when () is small, the uncertainty in is large. This uncertainty is not negligible. Figure A4 shows 985 data points with their corresponding normalized temperature difference ( and uncertainty bars).
- The drinking water temperature did not actually increase, but there is a measurement error. The measurement uncertainty (0.2 °C) is large compared to the measured temperature differences (0.1–0.2 °C). As so few data points show this effect, this explanation seems likely, and it demonstrates that the measurement uncertainty needs to be considered.
- The soil temperatures were actually higher at these points, indicating that the modeled is incorrect (Table A5 shows a sensitivity to ). However, as these points were clustered in time, this explanation seems unlikely.
- 3.
- This is a coincidence. The size of the uncertainty in ΔTN correlates with the driving force () and is expected to be independent of the residence time τ. It seems likely that it is just a coincidence that the driving force was relatively small during the tests with small residence times. Before the controlled residence times tests started and data were already recorded, there were some days where ), while and the temperature of the drinking water at the PS were different.
- 4.
- There is in fact a correlation between τ and . The soil temperatures that we considered as may not be correct for all test conditions, i.e., the boundary condition cannot be retrieved from the STM+. The high flow tests (short residence times) lasted relatively shortly (5 to 10 h) and the assumption of an (dynamic) equilibrium may not be valid, whereas it may be valid for the longer tests (at lower flows).
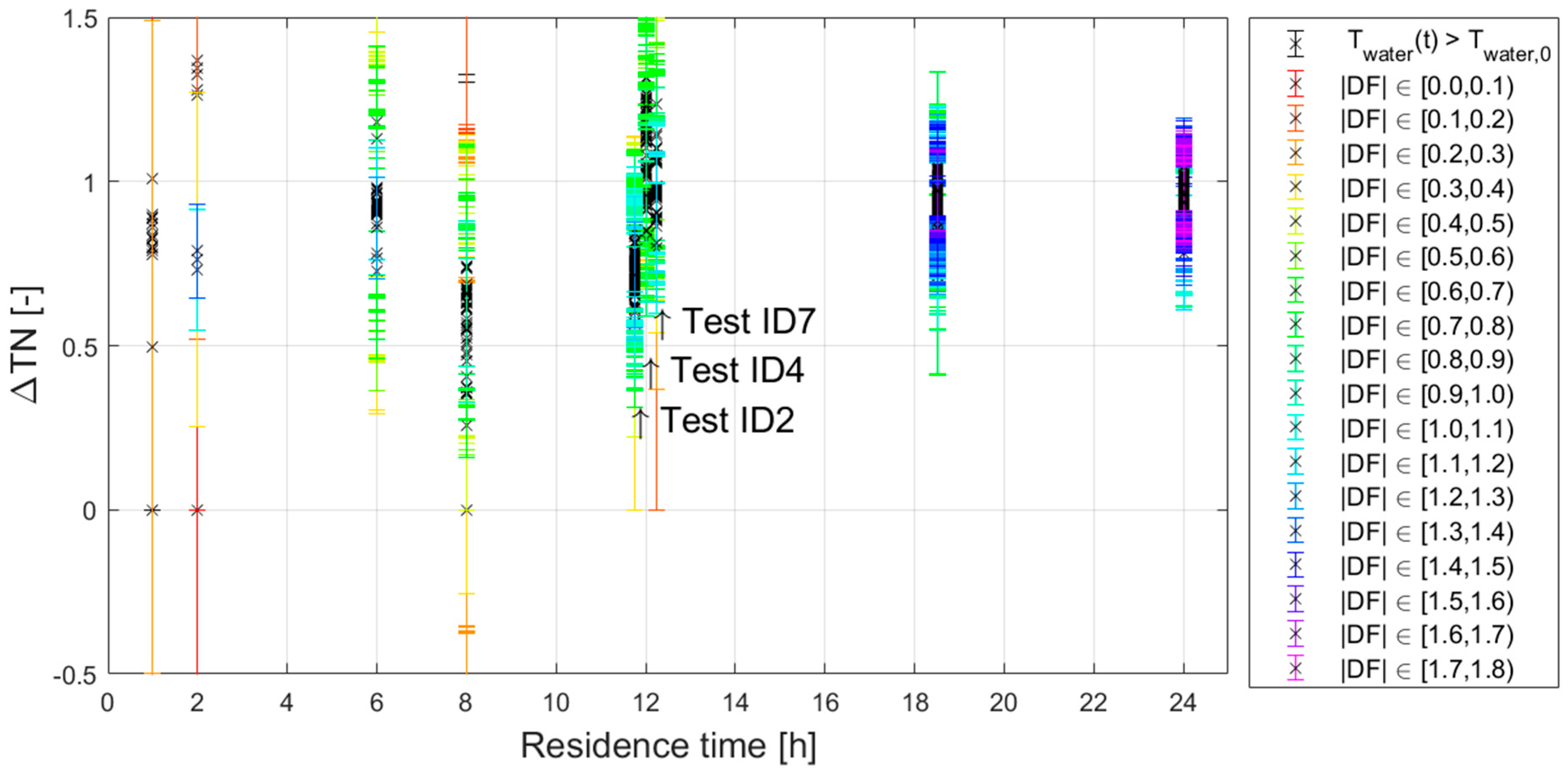
- The 22 data points with a negative driving force are excluded from the validation.
- is expected to be between 0 and 1. A data point with an uncertainty of > 0.5 is considered unreliable. These are data points for which the difference between Twater,0 and Tboundary are small and therefore the value of ΔTN loses its meaning. There are 93 data points with > 0.5 (indicated with red and yellow error bars in Figure A4). These are excluded from the validation.
| ID | Start Time | End Time | Residence Time [h] | Amount of Data Points after Adjusting for Residence Time (and Filtering) |
|---|---|---|---|---|
| 1 | 19 May 6:30 | 20 May 16:00 | 8.0 | 103 (60) |
| 2 | 20 May 16:15 | 22 May 12:30 | 12.0 | 130 (128) |
| 3 | 22 May 13:00 | 25 May 16:30 | 24.0 | 207 (207) |
| 4 | 25 May 16:45 | 27 May 08:30 | 12.0 | 112 (109) |
| 5 | 27 May 09:15 | 27 May 20:00 | 1.0 | 41 (0) |
| 6 | 28 May 08:00 | 28 May 15:00 | 2.0 | 21 (2) |
| 7 | 28 May 15:30 | 29 May 18:45 | 12.0 | 62 (57) |
| 8 | 29 May 19:00 | 2 Jun 07:45 | 18.6 | 266 (266) |
| 9 | 2 Jun 08:15 | 3 Jun 10:00 | 6.0 | 83 (41) 1 |
Appendix B.3. Sensitivity Analysis of WTM+, Applied to a Single Pipe
| Parameter | [Unit] | Default Value | Multiplication Factor | Resulting Multiplication Factor for | Resulting Multiplication Factor for τ at Which = 0.999 |
|---|---|---|---|---|---|
| Tboundary − Twater,0 | [K] | 5 | 2 | 2.00 | 1.00 |
| D1 | [mm] | 152 | 0.5 | 1.63 | 0.40 |
| λsoil | [Wm−1K−1] | 1.60 | 2 | 1.62 | 0.40 |
| t | [s] | 9000 | 2 | 1.58 | - |
| TSoI × D1 | [m] | 0.152 | 0.5 | 1.35 | 0.61 |
| λpipe | [Wm−1K−1] | 0.16 | 2 | 1.18 | 0.78 |
| dpipe | [mm] | 4 | 0.5 | 1.11 | 0.86 |
| Nu | [-] | 100 | 2 | 1.01 | 0.98 |
| Parameter | [Unit] | Default Value | Realistic Alternative | Explanation | Resulting Multiplication Factor for | Resulting Multiplication Factor for τ at Which = 0.999 |
|---|---|---|---|---|---|---|
| Nu | [-] | 100 | 3.66 | laminar flow (i.so. turbulent) | 0.61 (1/0.61 = 1.64) | 1.90 (1/1.90 = 0.52) |
| 400 | Q: 0.1 to 0.7 m/s | 1.02 | 0.97 | |||
| λsoil | [Wm−1K−1] | 1.60 | 1.9 | wet sand (i.so. dry sand) | 1.34 | 0.62 |
| D1 | [mm] | 152 | 125 | one step down in nominal diameter | 1.18 | 0.77 |
| λpipe | [Wm−1K−1] | 0.16 | 0.4 | PE (i.so. PVC) | 1.18 | 0.78 |
| dpipe | [mm] | 4 | 3 | one step down in pressure class | 1.05 | 0.93 |
| t | [s] | 9000 | 10,000 | measurement inaccuracy (ca. 15 min) | 1.12 | - |
| Tboundary − Twater,0 | [K] | 5 | 4.8 | measurement inaccuracy (0.2 K) | 1.04 | 1.00 |
Appendix B.4. Extra Results for Case Study 1
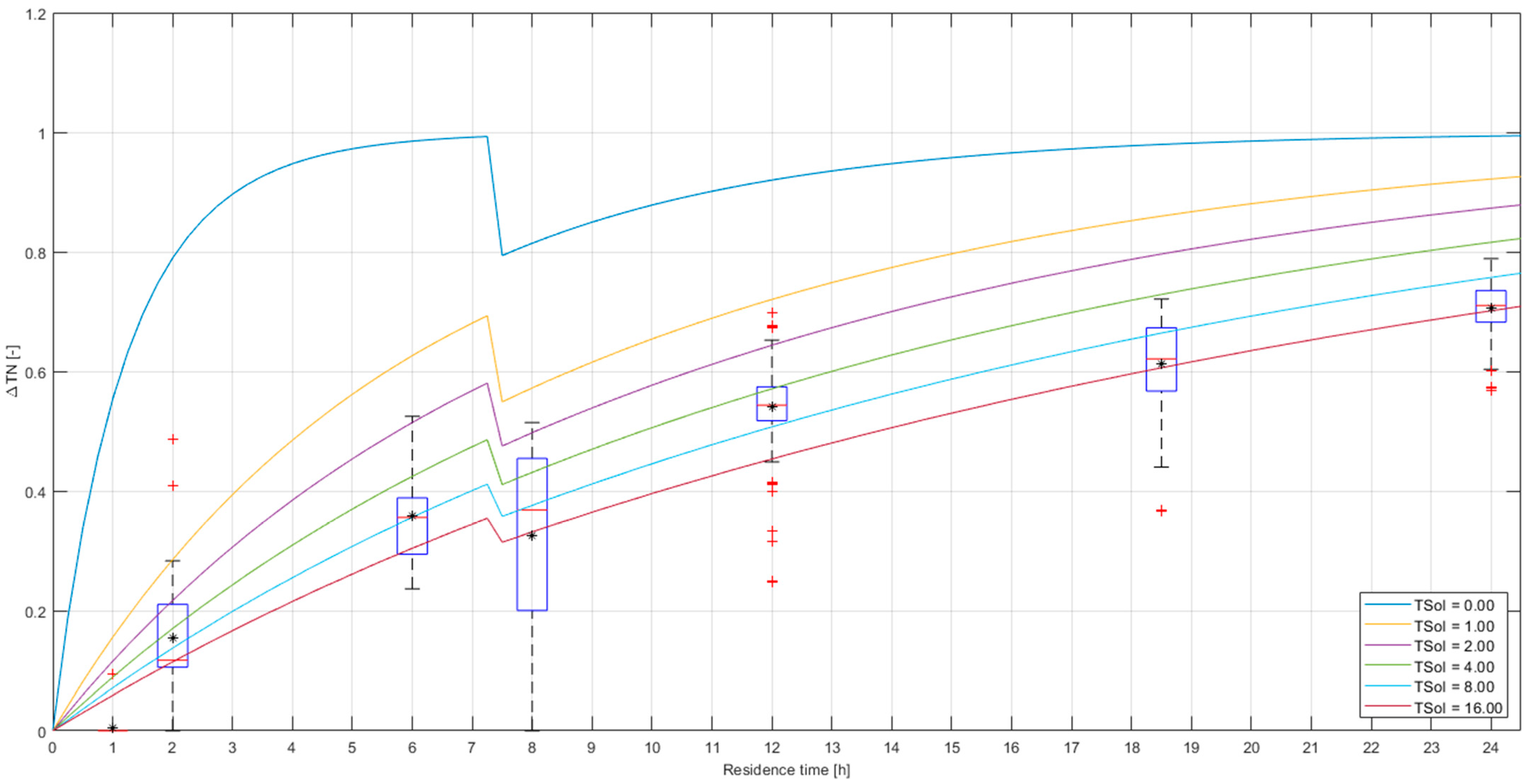
Appendix C. Case Study 2
Appendix C.1. Additional Information on Case Study 2: Soil Temperatures
- There is a variation in installation depth with the top of the distribution mains in the older parts (built between 1980 and 2000, roughly the center and southern part, amongst which areas A and D in Figure 3) at −1.3 m and in the newer parts (roughly the western and north-eastern part, amongst which areas B, E, and C in Figure 3) at −1.1 m (meaning that the center of the Ø110 and Ø160 mm pipes are installed at −1.35 to −1.38m and −1.15 to −1.18 m, respectively). The transport mains (>180 mm) are installed at ca. 1.5 m coverage (center of the Ø200 and Ø310 mm pipe at ca. −1.6 to −1.7 m).
- The soil type is influenced by the backfill that was used for pipe installation. The older parts have a mixture of sand and clay, and the newer parts have sand only.
- The variation in soil cover (asphalt, tiles, grass, or bushes) is very high and very distributed over the entire area. There is even soil cover variation over the length of a single pipe. This level of detail was not considered in this study.
- Above-ground anthropogenic heat sources were not considered either. It is not yet entirely clear which are important and how much influence they would have.
- Below-ground anthropogenic heat sources include electricity cables and the DHN, where the DHN most likely has a significant effect [17]. The northwestern part of Almere (amongst which areas A and C; see Figure 3) has a DHN. The effect of the DHN parallel to the DWN is not considered in this paper as the distance between the two is more than 2.5 m. The crossings of DWDN and DHN pipes are taken into consideration.
- : the residence time follows from the hydraulic network model. Here, the demand patterns of 31 August 2020 were applied, but the model was not calibrated for this particular day, so there may be some valve positions that are incorrect, which may lead to errors in residence time.
- : was estimated by using weather data, processed with the STM [4,18] and STM+ [17]. There were no soil temperature measurements available. Five different boundary conditions were suggested (Table A7):
- TTM is the soil temperature around transport mains (D ≥ 180 mm). TTM = 18.0 °C. This is the STM calculated temperature at −1.7 m (Figure A6) in peri-urban areas (clay/sand under grass).
- TTM_DHN is the soil temperature around transport mains with a DHN crossing. TTM_DHN = 20.1 °C. This is the STM calculated temperature at −1.7 m in peri-urban areas (clay/sand under grass) + 2.1 °C from the primary network DHN as from the STM+.
- TTM is the soil temperature around distribution mains in the older part of Almere. TDM = 18.7 °C. This is the STM calculated temperature at −1.35 m (Figure A6) in peri-urban areas (clay/sand under grass).
- TTM is the soil temperature around distribution mains with a DHN crossing in the older part of Almere. TDM_DHN = 19.5 °C. This is the STM calculated temperature at −1.35 m in peri-urban areas (clay/sand under grass) + 0.8 °C from the secondary network DHN as from the STM+.
- TTM_NE is the soil temperature around distribution mains in the newer (north-eastern) part of Almere. TDM_NE = 20.5 °C. This is the maximum drinking water temperature that was measured in area B (Figure 3) and is the average of the STM calculated temperatures at −1.15 m (Figure A6) for peri-urban (clay/sand under grass) and urban (sand, under tiles with various shade conditions) areas.
- : drinking water temperature measured at locations F1 and F2 (13.7 and 13.3 °C, respectively).
- : drinking water temperature measured at hydrants (Figure 3).

| Scenario | λsoil | ρsoil | Cp, soil | αsoil | Cover | Albedo | Global Radiation | QF | a3 |
|---|---|---|---|---|---|---|---|---|---|
| W·m·K−1 | kg·m−3 | J·kg−1·K−1 | 10−6 m2s−1 | - | W·m−2 | W·m−2 | W·m−2 | ||
| Peri-urban, under grass (clay) | 1.0 | 2.103 | 1.103 | 0.5 | Grass | 0.12 | Rg | 50 | −50 |
| Urban, under grass (sand) | 1.2 | 2.103 | 1.103 | 0.6 | Grass | 0.12 | Rg | 100 | −100 |
| Urban, under tiles, no extra shade (sand) | 1.2 | 2.103 | 1.103 | 0.6 | Tiles | 0.12 | Rg | 100 | −100 |
| Urban, under tiles, half shade (sand) | 50% × Rg | ||||||||
| Urban, under tiles, full shade (sand) | 0 |
| Tboundary | Value | Pipe Group Conditions | Where in Network | Where in Model |
|---|---|---|---|---|
| TTM | 18.0 °C. | −1.5 m, sand + clay, no DHN crossings | Most transport mains (>180 mm) | |
| TTM DHN | TTM + 2.1 = 20.1 °C. | −1.5 m, sand + clay, with DHN crossings | Some transport mains (>180 mm) with crossings of DHN | |
| TDM | 18.7 °C. | −1.3 m, sand + clay, no DHN crossings | Area A and D | distribution mains in whole network, except area B |
| TDM DHN | TDM + 0.8 = 19.5 °C. | −1.3 m, sand + clay, with DHN crossings | Part of Area A with crossings of DHN | distribution mains in whole network, except area B |
| TDM NE | 20.5 °C. | −1.1 m, sand, no DHN crossings | Area B, C, and E | distribution mains in area B |
Appendix C.2. Additional Information on Case Study 2: WTM+
| Parameter | Unit | Value | Uncertainty | Reference |
|---|---|---|---|---|
| D1 | mm | 50–300 | - | Hydraulic network model Almere, from Vitens |
| D2 | mm | 1.052 × D1 | - | Outside diameter of PVC estimated with multiplication factor to inside diameter. AC pipes treated as PVC. |
| λpipe | Wm−1K−1 | 0.16 | Some pipes are AC, but modeled as PVC | AC pipes treated as PVC [4] |
| Nu | - | Unclear where transition between turbulent and laminar flow is, transition at Re = 5000 from case study 1 | For Re > 5000: Nu = 0.027 Re0.8 Pr0.33 [4], Re from hydraulic network model Almere, Pr = 7. For Re <= 5000 Nu = 3.66 | |
| D3 | mm | D2 + TSoI × D1 | Unknown | To be determined |
| λsoil | Wm−1K−1 | 1.6 | Unknown | Dry sand [17] |
| Twater,0 | °C | = 13.7 = 13.3 | ± 0.05 °C | Vitens |
| Twater | °C | 13.0–21.0 | ± 0.05 °C | Measured at hydrants, Vitens |
| Tboundary | °C | TDM = 18.7 TDM_DHN = 19.5 TDM_NE = 20.5 TTM = 18 TTM_DHN = 20.1 | Unknown | For stagnant water, from STM (Table A7). |
Appendix C.3. Sensitivity Analysis of WTM+, Applied to a Drinking Water Distribution Network
| Residence Time (h) | Conditions for Tboundary | ||
|---|---|---|---|
| A | 13.3 | 5–15 | TDM, TDM_DHN |
| B | 13.7 | 10–15 | TDM_NE |
| C | 13.3 | >24 | TDM, TDM_DHN |
| D | 13.3 | >10 | TDM |
| E | 13.7 | 10–15 | TDM_NE |
| Scenario | Scale % | TSoI | TDM °C | TDM_DHN °C | TDM_NE °C | TTM °C | TTM_DHN °C | |
|---|---|---|---|---|---|---|---|---|
| Base | 1 | 1.6 | 18.7 | 19.5 | 20.5 | 18.0 | 20.1 | |
| TSoI | 0.1–10 | |||||||
| 14.7–23.1 | TDM + 0.8 | TDM–0.5 | TDM + 1.4 | |||||
| 15.0–23.0 | ||||||||
| 13.7–24.2 | ||||||||
| 13.7–24.2 | ||||||||
| 13–23 | ||||||||
| λsoil | 1.1–1.9 | |||||||
| D1 | −10 to +10 | |||||||
| Pipe length | −10 to +10 |
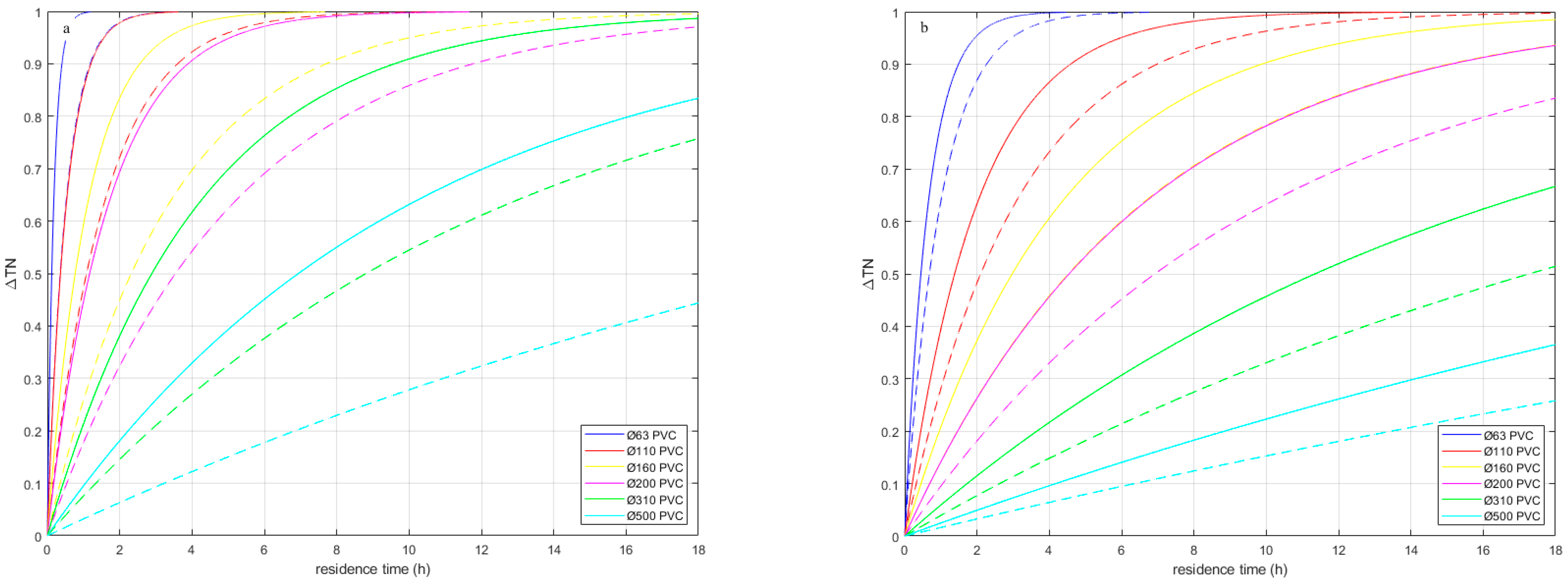
- TSoI: Figure A7a shows that ΔT ≤ 0.8 °C for a TSoI < 1. The larger the TSoI, the slower the heat exchange between soil and drinking water, and, in this case, it means that, at a larger TSoI, Twater will be lower. Figure A7a shows that the effect is similar for most areas but stronger in area B. This is most likely caused by a difference in residence times. The residence times toward area C, e.g., are large (>20 h) and thus most likely the drinking water temperature at most nodes has reached Tboundary, even with an extra delay due to the TSoI, leading to a maximum of ΔT = 0.8 °C. On the other hand, areas B and E are very close to a reservoir, and the effect of a larger TSoI is more noticeable (ΔT ≥ 1.2 °C).
- Tboundary: the model is very sensitive to Tboundary. Especially scenario shows a large effect; the other scenarios have a more local effect, and the effect is smaller (Figure A7b). The larger the area where this local Tboundary is imposed, the larger the number of customers nodes that are affected.
- The scenario shows that, when Tboundary decreases or increases at (almost) all locations (Figure A7c), the Twater changes with it. The effect is small (but not zero) in areas B and E, where Tboundary is the same as in the base case.
- The scenario shows the local effect of TDM_NE only in the areas that have this boundary condition (Area B and E); there is no effect in areas A, C, and D. A range of 8 °C (from 15 to 23 °C) in TDM_NE leads to a range of 5 °C (−2.5 to 1.5 °C) in ΔT and thus in Twater in areas B and E (Figure A7d).
- The scenario (Figure A7e) shows the effect when only Tboundary around transport mains is changed. An 8 °C change in Tboundary leads to a ca. 1.5 °C change in Twater.
- The scenario (Figure A7f) shows that a different Tboundary at DHN crossings with the distribution mains has a small effect, but larger than 0 °C. This effect is more apparent when we only look at the areas that have distribution mains with DHN crossings (areas A and C), where the effect is larger in area C (an 8 °C change in Tboundary leads to a ca. 5 °C change in Twater) with longer residence times than in area A. In area A, the effect is also noticeable and should not be neglected.
- The scenario (Figure A7b) only considers the crossings of the DHN with transport mains. The effect is almost 0 (<±0.2 °C). This means that the current number of DHN crossings with the transport mains does not lead to higher Twater.
- : the ΔT due to variations in is less than ±0.3 °C (Figure A7g), and this means that is not the most important parameter to estimate accurately for the WTM+ in a real DWDN. It should be noted that Tboundary, as estimated with the STM, is indeed sensitive to .
- Pipe length and diameter: the ΔT is less than ± 0.15 °C for pipe length and diameter changes (Figure A7h); the WTM+ is not very sensitive to these parameters. This means that, when the WTM+ is applied to the DWDN of case study 2, the results will be representative for a range of DWDNs throughout the Netherlands and the five areas are representative for the effects of differences in residence time, local Tboundary, and network layouts.

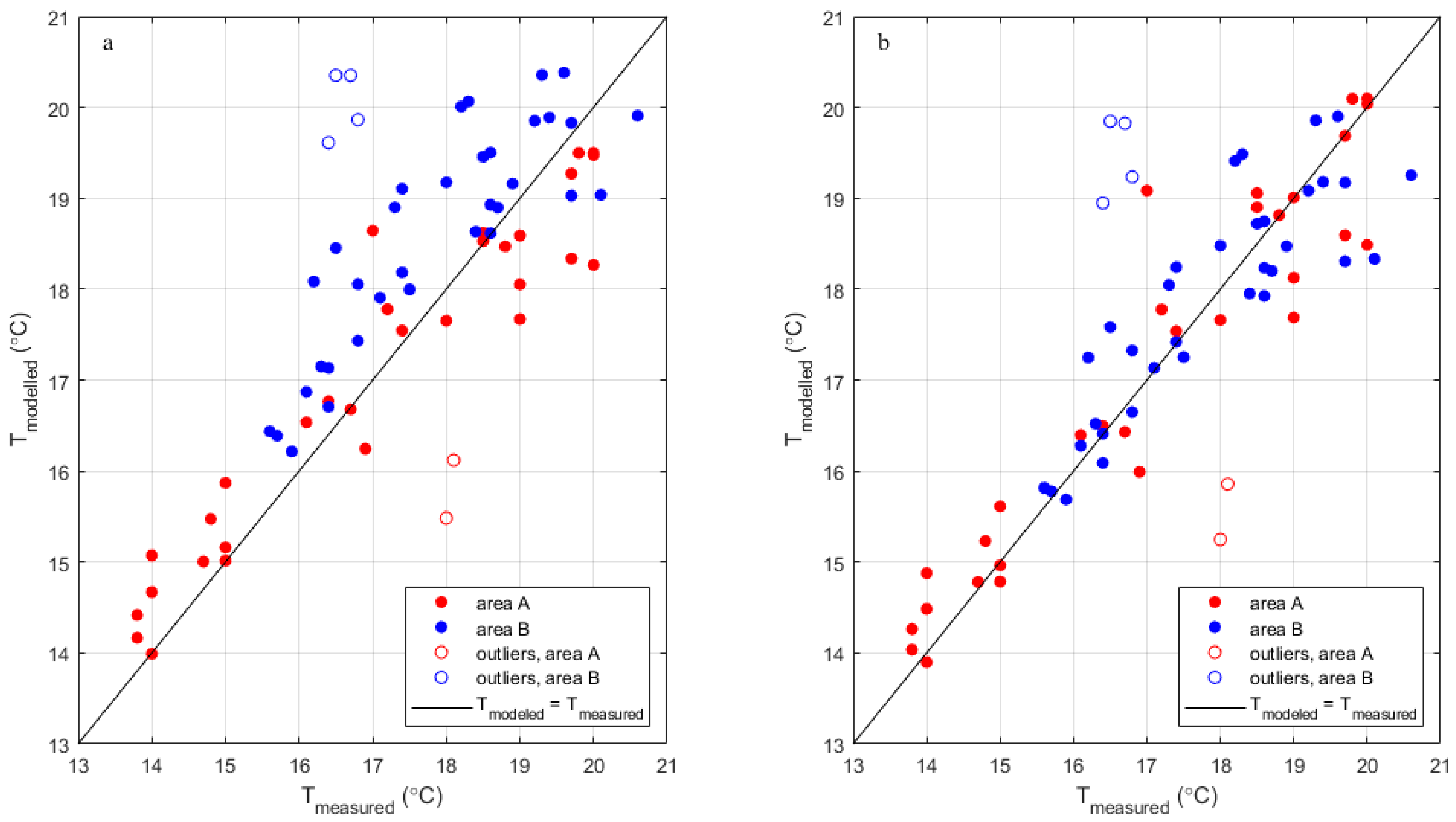
Appendix C.4. Extra Results for Case Study 2
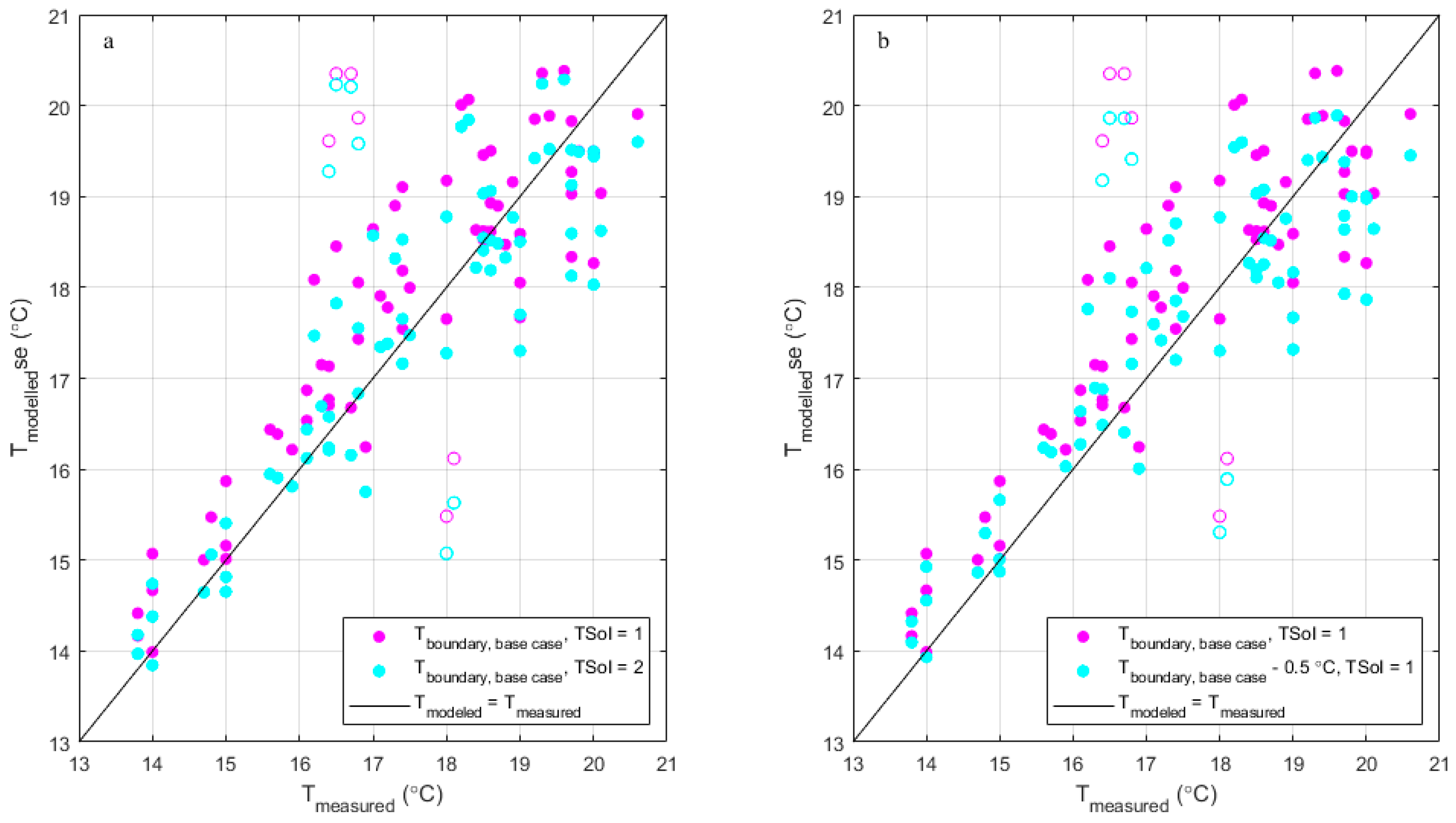

| Figure, Line Color | Area | Case | Tboundary (°C) | TSoI | Excluding Outliers | Including Outliers | ||
|---|---|---|---|---|---|---|---|---|
| RMSE (°C) | R2 | RMSE (°C) | R2 | |||||
| Figure 7a, red | A | Base | 19.5 | 1 | 0.75 | 0.82 | 0.92 | 0.72 |
| Figure 7a , blue | B | Base | 20.5 | 1 | 1.01 | 0.31 | 1.49 | 0 |
| Figure A8a,b, magenta | Total | Base | 19.5/20.5 | 1 | 0.92 | 0.72 | 1.25 | 0.48 |
| n.a. | A | Change in TSoI | 19.5 | 2 | 0.80 | 0.82 | 1.03 | 0.68 |
| n.a. | B | Change in TSoI | 20.5 | 2 | 0.76 | 0.66 | 1.29 | 0.08 |
| Figure A8a, cyan | Total | Change in TSoI | 19.5/20.5 | 2 | 0.77 | 0.80 | 1.17 | 0.57 |
| n.a. | A | Change in Tboundary | 19.0 | 1 | 0.88 | 0.71 | 1.06 | 0.56 |
| n.a. | B | Change in Tboundary | 20.0 | 1 | 0.80 | 0.50 | 1.24 | 0 |
| Figure A8b, cyan | Total | Change in Tboundary | 19.0/20.0 | 1 | 0.83 | 0.72 | 1.15 | 0.49 |
| n.a. | A | Best fit TSoI | 19.5 | 1 | 0.75 | 0.82 | 0.92 | 0.72 |
| n.a. | B | Best fit TSoI | 20.5 | 3 | 0.71 | 0.71 | 1.22 | 0.21 |
| Figure A8a, cyan | Total | Best fit TSoI | 19.5/20.5 | 2 | 0.77 | 0.80 | 1.17 | 0.57 |
| Figure 7b, red | A | Optimal, best fit (RMSE) Tboundary AND TSoI | 20.1 | 2 | 0.70 | 0.88 | ||
| n.a. | Best fit (R2) Tboundary AND TSoI | 20.5 | 3 | 0.74 | 0.89 | |||
| Figure 7b, blue | B | Optimal, best fit (RMSE) Tboundary AND TSoI | 20.1 | 2 | 0.70 | 0.67 | ||
| n.a. | best fit (R2) Tboundary AND TSoI | 21.4 | 7 | 0.78 | 0.74 | |||
| n.a | Total | best fit (RMSE) Tboundary AND TSoI | 20.1 | 2 | 0.70 | 0.83 | ||
| n.a. | best fit (R2) Tboundary AND TSoI | 20.5 | 3 | 0.72 | 0.84 | |||
References
- Calero Preciado, C.; Boxall, J.; Soria-Carrasco, V.; Martínez, S.; Douterelo, I. Implications of climate change: How does increased water temperature influence biofilm and water quality of chlorinated drinking water distribution systems? Front. Microbiol. 2021, 12, 658927. [Google Scholar] [CrossRef] [PubMed]
- Ahmad, J.I.; Dignum, M.; Liu, G.; Medema, G.; van der Hoek, J.P. Changes in biofilm composition and microbial water quality in drinking water distribution systems by temperature increase induced through thermal energy recovery. Environ. Res. 2021, 194, 110648. [Google Scholar] [CrossRef] [PubMed]
- Agudelo-Vera, C.; Avvedimento, S.; Boxall, J.; Creaco, E.; de Kater, H.; Di Nardo, A.; Djukic, A.; Douterelo, I.; Fish, K.E.; Iglesias Rey, P.L.; et al. Drinking Water Temperature around the Globe: Understanding, Policies, Challenges and Opportunities. Water 2020, 12, 1049. [Google Scholar] [CrossRef]
- Blokker, E.J.M.; Pieterse-Quirijns, E.J. Modeling temperature in the drinking water distribution system. J. Am. Water Work. Assoc. 2013, 105, E19–E29, Erratum in J. Am. Water Work. Assoc. 2018, 110, 98. [Google Scholar] [CrossRef]
- Agudelo-Vera, C.M.; Blokker, M.; de Kater, H.; Lafort, R. Identifying (subsurface) anthropogenic heat sources that influence temperature in the drinking water distribution system. Drink. Water Eng. Sci. 2017, 10, 83–91. [Google Scholar] [CrossRef]
- Agudelo-Vera, C.M.; Blokker, E.J.M.; Pieterse-Quirijns, E.J. Early warning system to forecast maximum temperature in drinking water distribution systems. J. Water Supply Res. Technol.—AQUA 2015, 64, 496–503. [Google Scholar] [CrossRef]
- De Pasquale, A.M.; Giostri, A.; Romano, M.C.; Chiesa, P.; Demeco, T.; Tani, S. District heating by drinking water heat pump: Modelling and energy analysis of a case study in the city of Milan. Energy 2017, 118, 246–263. [Google Scholar] [CrossRef]
- Hubeck-Graudal, H.; Kirstein, J.K.; Ommen, T.; Rygaard, M.; Elmegaard, B. Drinking water supply as low-temperature source in the district heating system: A case study for the city of Copenhagen. Energy 2020, 194, 116773. [Google Scholar] [CrossRef]
- Díaz, S.; Boxall, J.; Lamarche, L.; González, J. The impact of ground heat capacity on drinking water temperature. J. Water Resour. Plan. Manag. 2023, 149, 04023012. [Google Scholar] [CrossRef]
- Hypolite, G.; Ferrasse, J.H.; Boutin, O.; Del Sole, S.; Cloarec, J.F. Dynamic modeling of water temperature and flow in large water system. Appl. Therm. Eng. 2021, 196, 117261. [Google Scholar] [CrossRef]
- Jakubek, D.; Ocłoń, P.; Nowak-Ocłoń, M.; Sułowicz, M.; Varbanov, P.S.; Klemeš, J.J. Mathematical modelling and model validation of the heat losses in district heating networks. Energy 2023, 267, 126460. [Google Scholar] [CrossRef]
- Hussein, A.; Klein, A. Modelling and validation of district heating networks using an urban simulation platform. Appl. Therm. Eng. 2021, 187, 116529. [Google Scholar] [CrossRef]
- Danielewicz, J.; Śniechowska, B.; Sayegh, M.A.; Fidorów, N.; Jouhara, H. Three-dimensional numerical model of heat losses from district heating network pre-insulated pipes buried in the ground. Energy 2016, 108, 172–184. [Google Scholar] [CrossRef]
- Wang, H.; Meng, H.; Zhu, T. New model for onsite heat loss state estimation of general district heating network with hourly measurements. Energy Convers. Manag. 2018, 157, 71–85. [Google Scholar] [CrossRef]
- Kuntuarova, S.; Licklederer, T.; Huynh, T.; Zinsmeister, D.; Hamacher, T.; Perić, V. Design and Simulation of District Heating Networks: A Review of Modeling Approaches and Tools. Energy 2024, 305, 132189. [Google Scholar] [CrossRef]
- Hägg, R. Dynamic Simulation of District Heating Networks in Dymola. Master’s Thesis, Lund University, Lund, Sweden, 2016. [Google Scholar]
- van Esch, J. BTM+ Model and Expert Tool; Deltares: Delft, The Netherlands, 2022. [Google Scholar]
- Çengel, Y.A. Heat Transfer: A Practical Approach; McGraw-Hill Companies, Inc.: New York, NY, USA, 1998; 1006p. [Google Scholar]
- Blokker, E.J.M.; van Osch, A.M.; Hogeveen, R.; Mudde, C. Thermal energy from drinking water and cost benefit analysis for an entire city. J. Water Clim. Chang. 2013, 4, 11–16. [Google Scholar] [CrossRef]
- Shang, F.; Uber, J.G. EPANET Multi-Species Extension User’s Manual; EPA: Cincinnati, OH, USA, 2008. [Google Scholar]
- Trinh, K.T. On the critical Reynolds number for transition from laminar to turbulent flow. arXiv 2010, arXiv:1007.0810. [Google Scholar]
- Blokker, M.; van Summeren, J.; van Laarhoven, K. Measuring drinking water temperature changes in a distribution network. In Proceedings of the 2nd International Joint Conference WDSA/CCWI, Valencia, Spain, 18–22 July 2022. [Google Scholar]
- Vewin and Energie-Nederland. Intersectoral Agreement Drinking Water and District Heating Pipes: Agreements on Distances; Vewin and Energie-Nederland: Den Haag, The Netherlands, 2024. (In Dutch) [Google Scholar]
| Scenario | TSoI | TDM °C | TDM_DHN °C | TDM_NE °C | TTM °C | TTM_DHN °C |
|---|---|---|---|---|---|---|
| Base case | 1 | 18.7 | 19.5 | 20.5 | 18.0 | 20.1 |
| Optimal case | 2 | 20.1 * | 20.1 * | |||
| Extra cases | 0.1–10 | 18.0–21.0 * | 19.0–22.0 * | |||
| Case Study 1 | Case Study 2 | Recommendation for Measurements | |
|---|---|---|---|
| Trajectory | Single pipe | DWDN | Single pipe, measured at 3 or more locations over pipe length |
| D1 (mm) | 152 | 101 (51% of length) | Range of diameters |
| Length (km) | 0.9 | Ca. 700 | Result from Q and τ |
| τ (h) | 1, 2, 8, 12, 18, 24 | 5–24 | Use model to estimate the range of interest (depends on D1) and measure at three or more τ, plus at very large τ |
| Q (m3/h) | Controlled (1, 4 to 0.06) | Result of demands | Use flows both in laminar and turbulent regimes |
| Duration | 2 weeks (2 h to 2 days per test) | 1 day (morning, afternoon) | 2–5 days per test |
| # data points | 870 (41 to 266 per test) | 72 | >40 per test; duplicate each test |
| Water source | Surface water | Groundwater | Groundwater |
| Season | Spring | Summer | Summer, winter |
| Parameters that are known accurately | D1, D2, λsoil, λwater, λpipe, αwater, τ (Q, Re), Tboundary, Twater, Twater,0 | D1, D2, λwater, λpipe (but PVC was assumed for all pipes in the model) αwater, Twater, Twater,0 | D1, D2, λsoil, λwater, λpipe, αwater, τ, Nu, Tboundary, Twater, Twater,0 |
| Strength | Many parameters are known accurately. Parameters are constant over space | Absolute temperature differences are significant. Parameters are constant over time | Many parameters are known accurately. Absolute temperature differences are significant. Parameters are constant over space or time |
| Weakness | Absolute temperature differences are small. Flow is enforced, not natural, and there may not be a natural equilibrium Nusselt not known | Residence time and Tboundary are not accurately known Nusselt is not known |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Blokker, M.; Pan, Q.; van Laarhoven, K. Validation of an Enhanced Drinking Water Temperature Model during Distribution. Water 2024, 16, 2796. https://doi.org/10.3390/w16192796
Blokker M, Pan Q, van Laarhoven K. Validation of an Enhanced Drinking Water Temperature Model during Distribution. Water. 2024; 16(19):2796. https://doi.org/10.3390/w16192796
Chicago/Turabian StyleBlokker, Mirjam, Quan Pan, and Karel van Laarhoven. 2024. "Validation of an Enhanced Drinking Water Temperature Model during Distribution" Water 16, no. 19: 2796. https://doi.org/10.3390/w16192796
APA StyleBlokker, M., Pan, Q., & van Laarhoven, K. (2024). Validation of an Enhanced Drinking Water Temperature Model during Distribution. Water, 16(19), 2796. https://doi.org/10.3390/w16192796







