Experimental Investigation of Bridge Scour under Pressure Flow Conditions
Abstract
1. Introduction
2. Materials and Methods
2.1. Dimensional Analysis
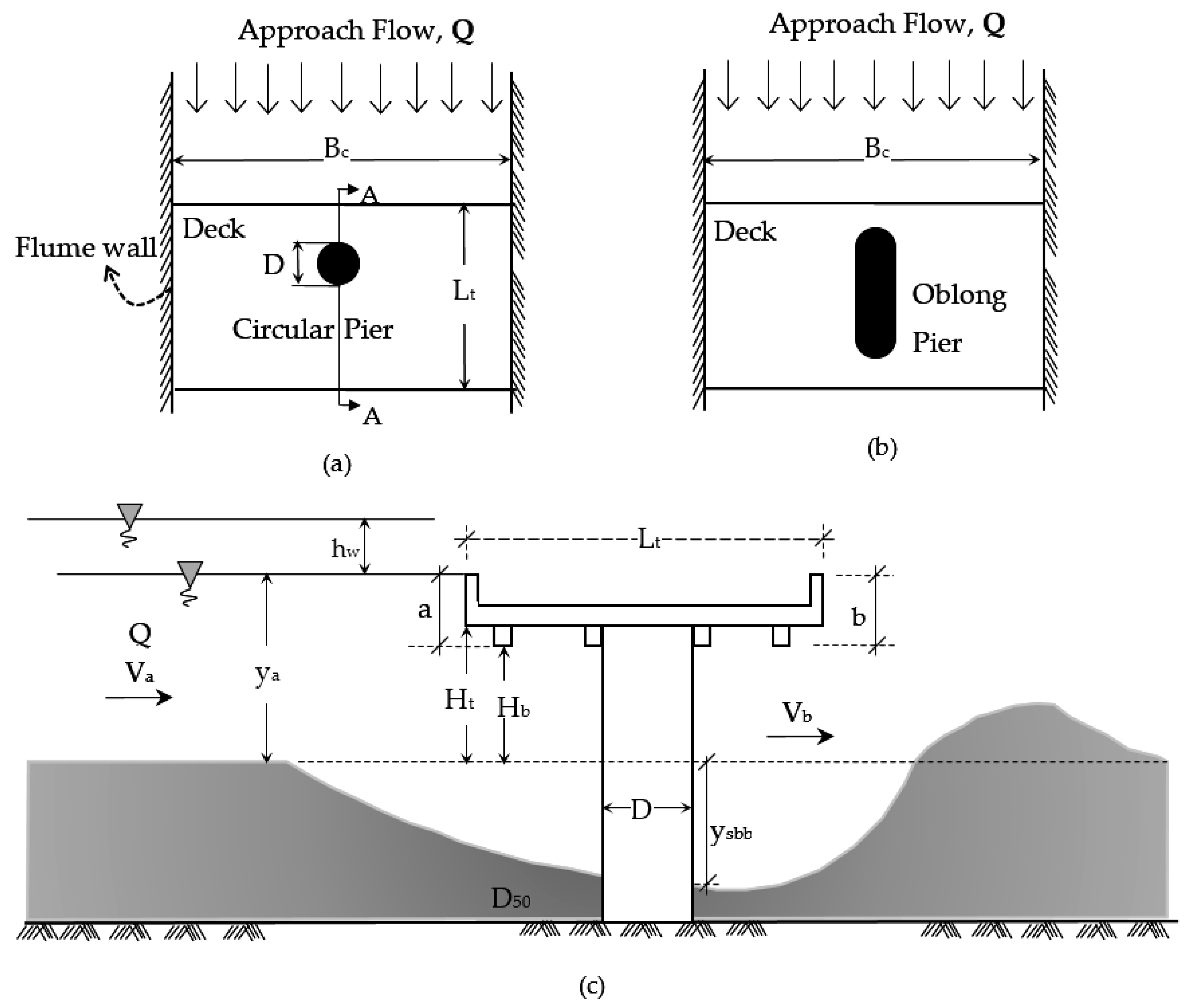
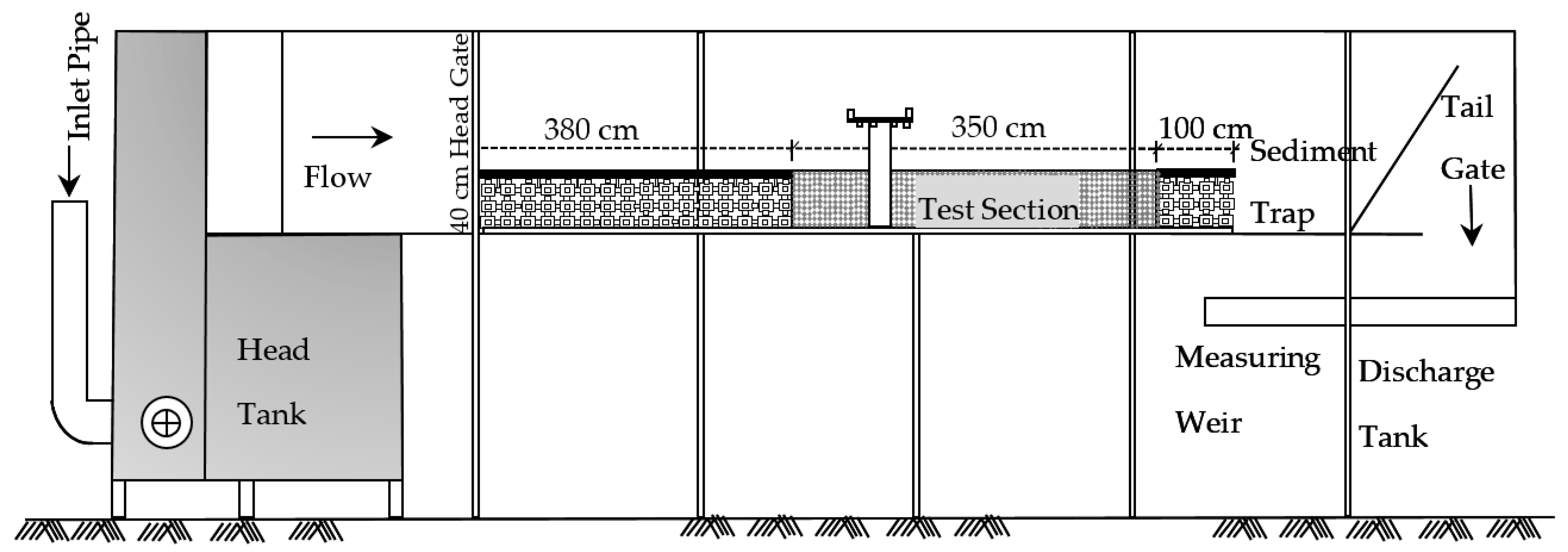
2.2. Experimental Setup
2.3. Experimental Program and Procedure
3. Results and Discussion
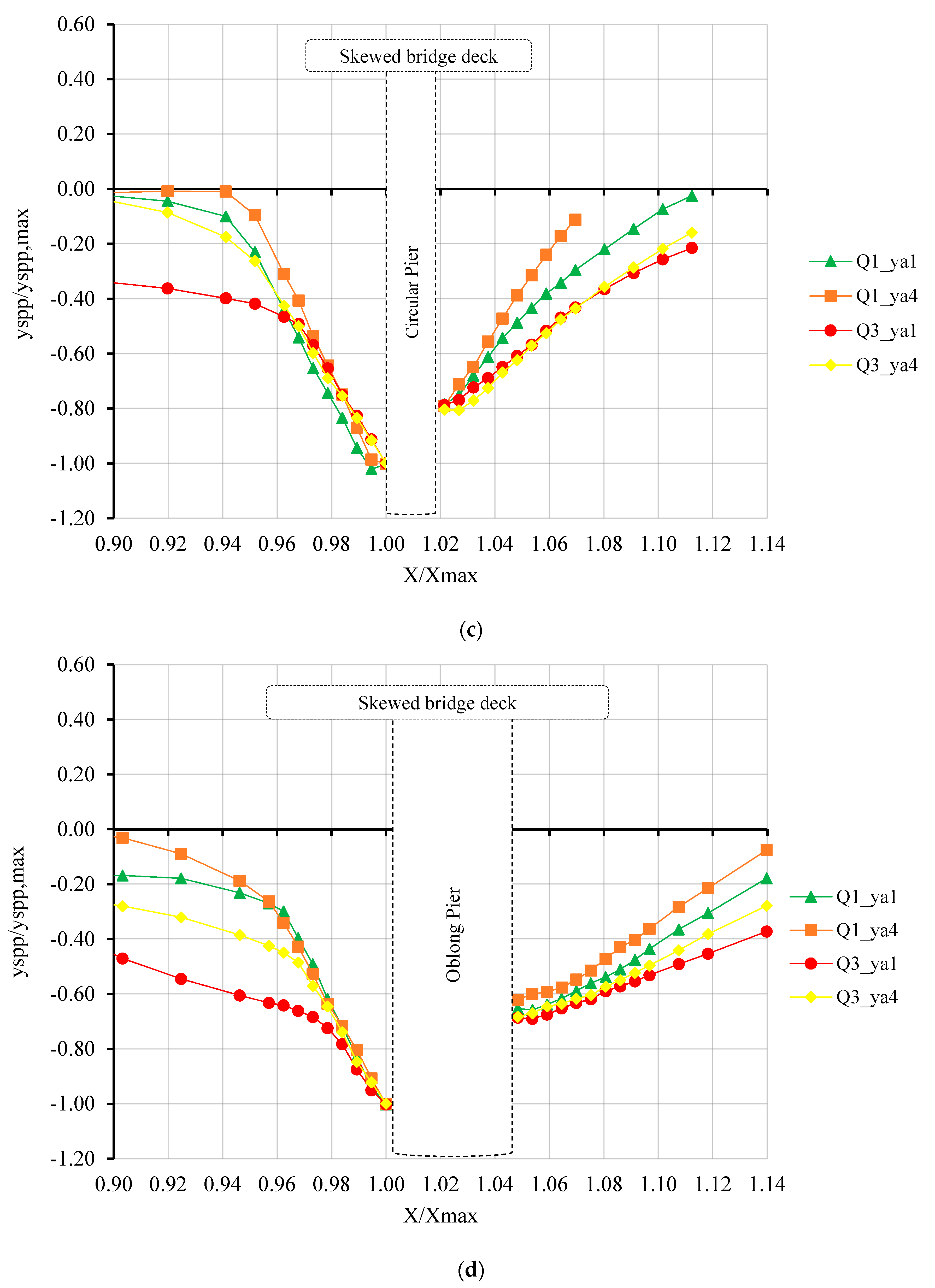
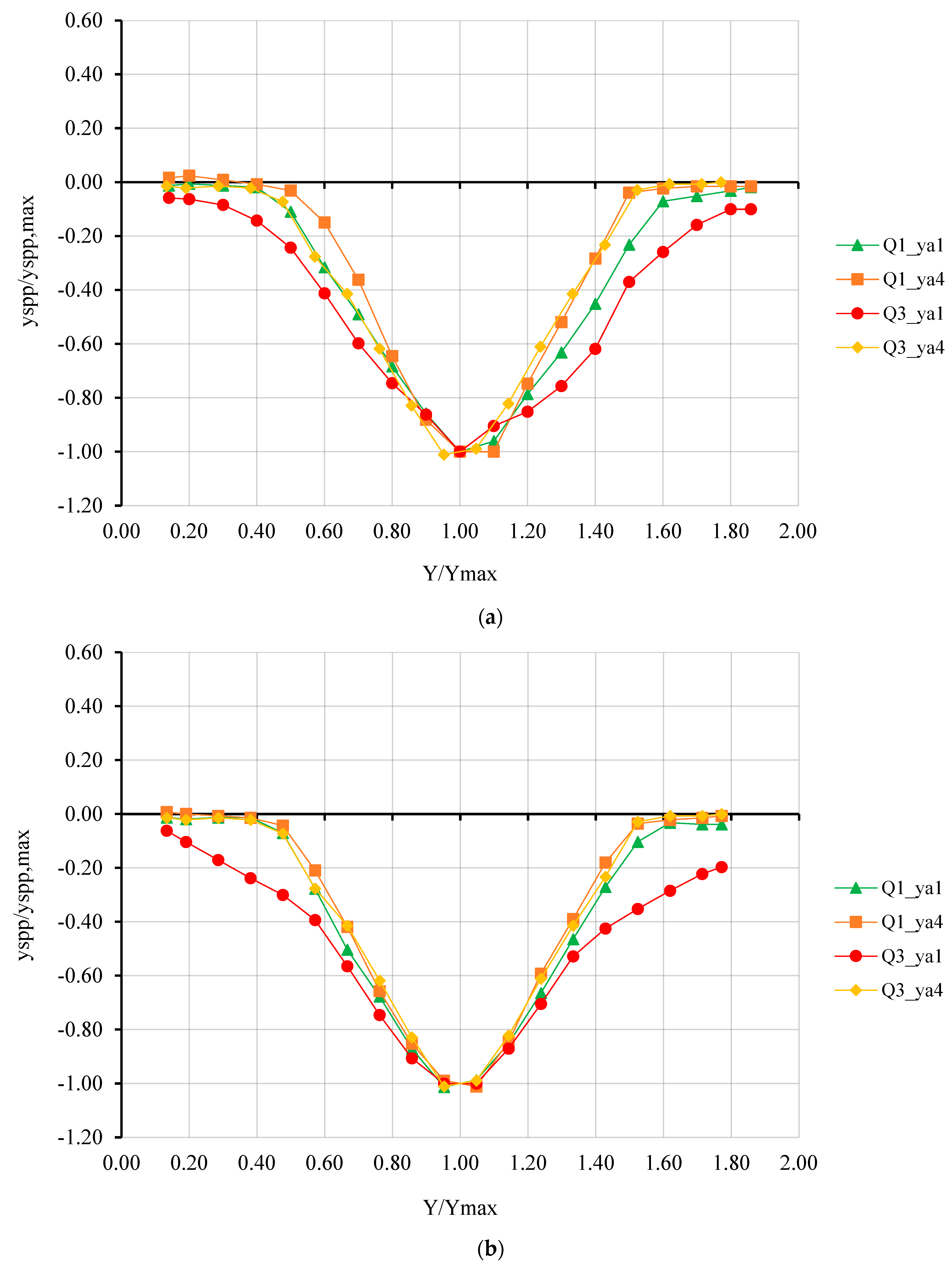
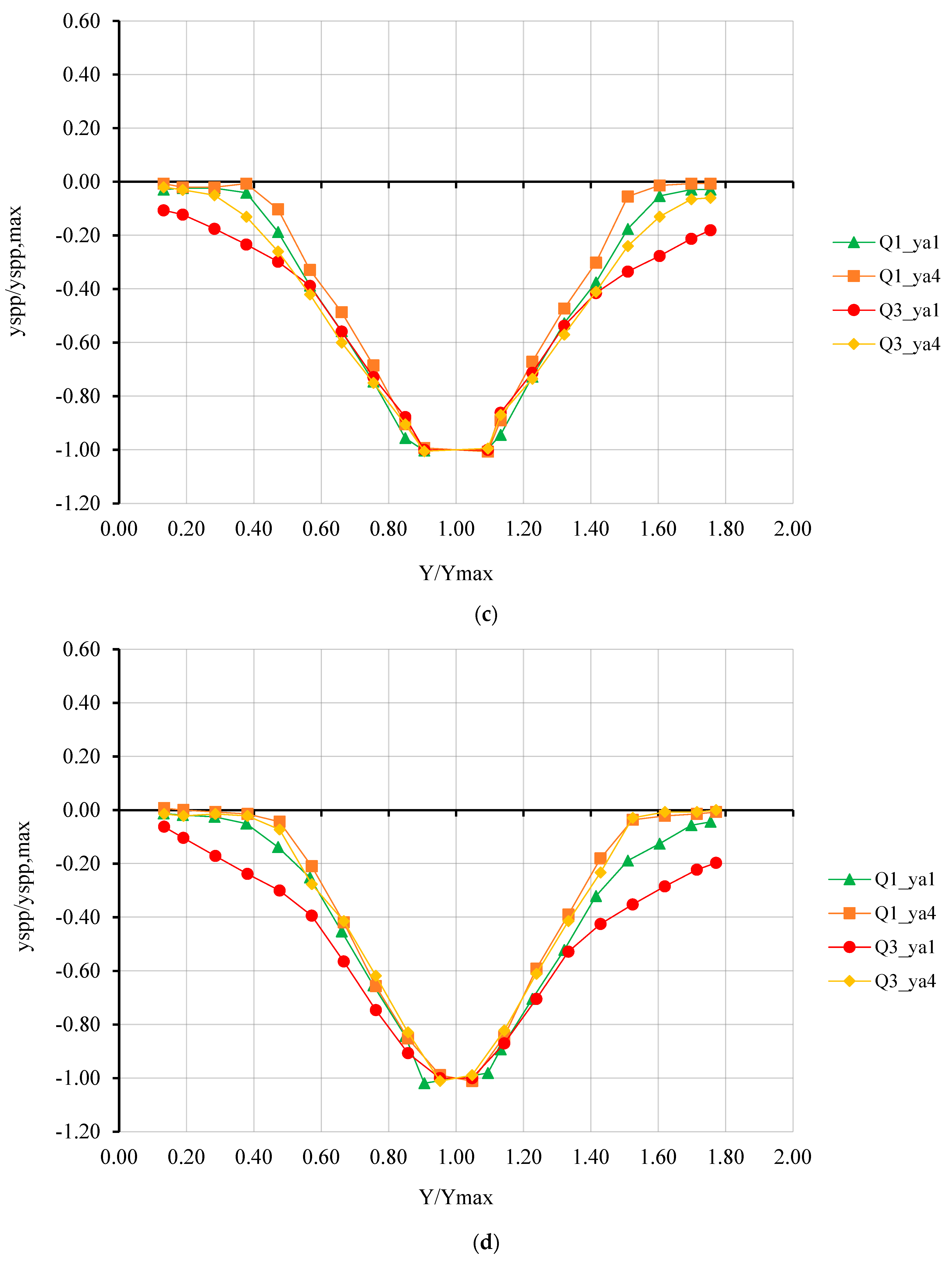
3.1. Observations of the Features of Pressure Flow Scour
3.2. Development of a Regression-Based Equation for Pressurized Bridge Pier Scour
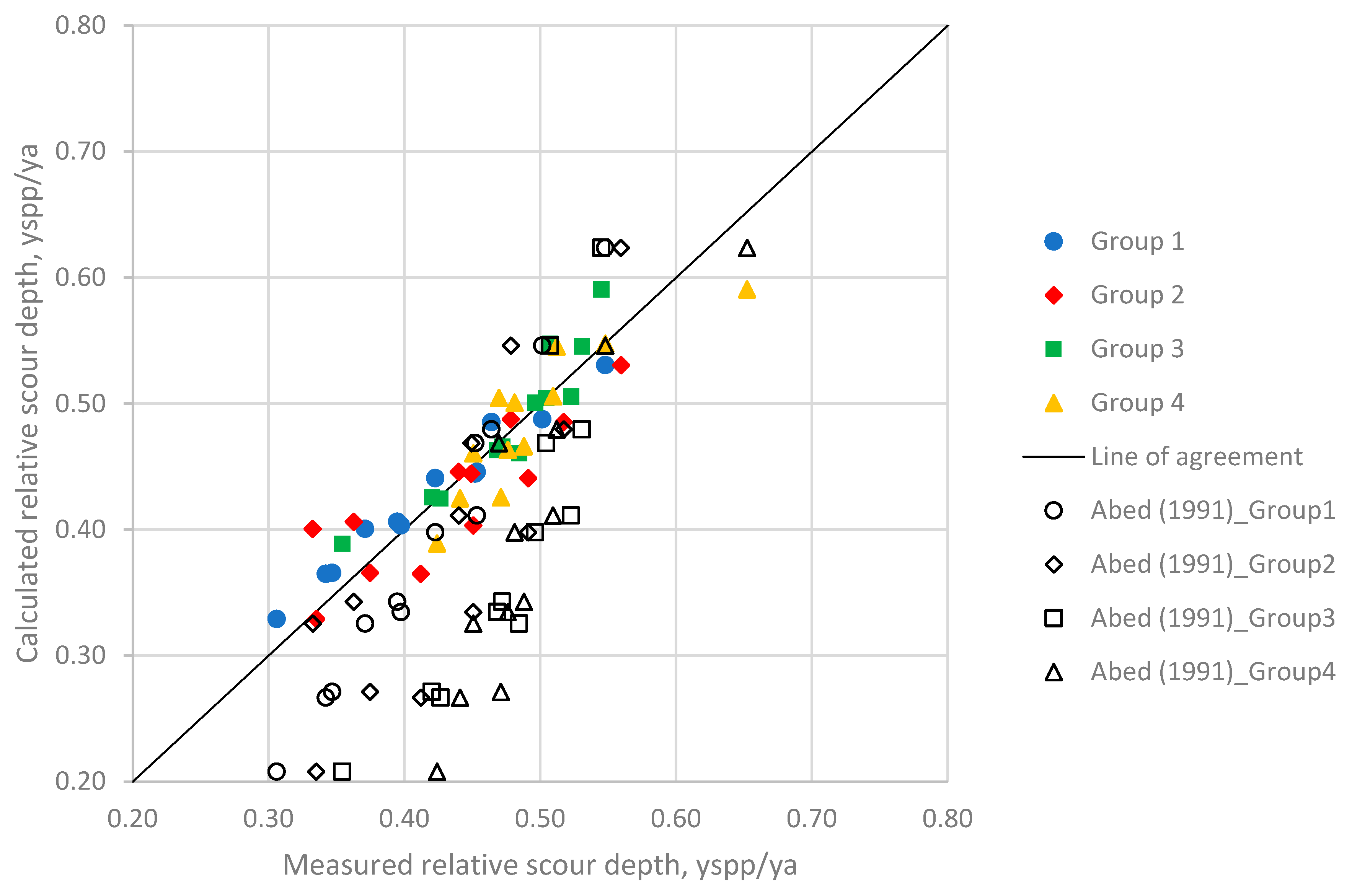
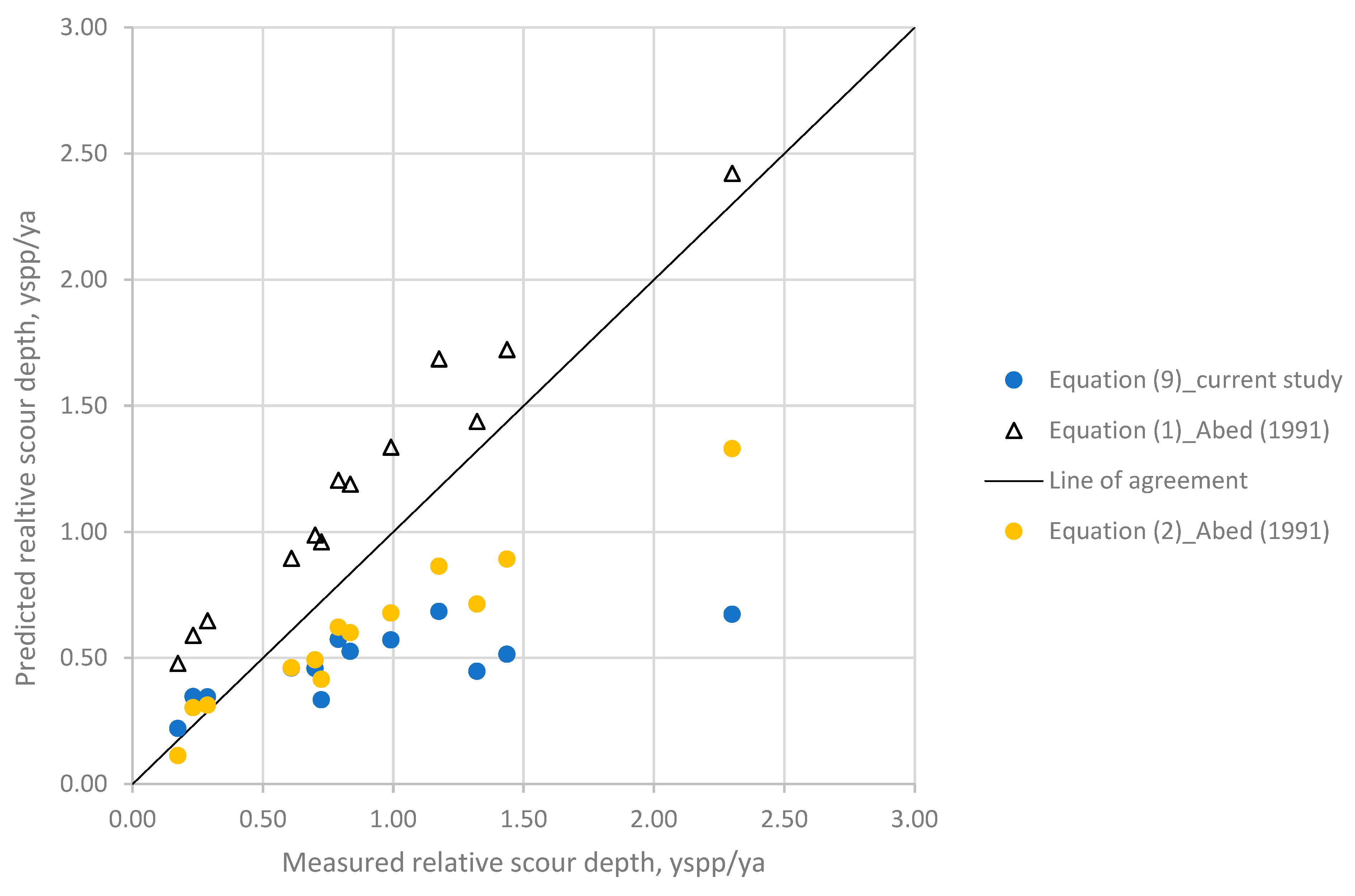
3.3. Comparison of the Results with Available Data in the Literature
3.4. Design Approach to Determine Foundation Depth of Bridges
4. Conclusions
- Observations during experiments and measurements of scour hole depths suggested that there was a strong interaction between the flow–pier–bridge that caused excessive scouring, not only around the piers but upstream of the bridge, generating serious bed level variations.
- Deeper and wider scour holes occurred under pressurized flow conditions, especially for small discharge values.
- Previous studies on fully pressurized flow conditions showed that as the weir overtopping depth increased, the maximum scour depth decreased. However, the results of the current study showed that the depth and width of the scour hole increased under fully pressured flow conditions. This was thought to be the result of vortices, and turbulence occurred due to the interaction with the deck. So, this explains the excessive scouring and failure of bridges when flood overtakes the structure.
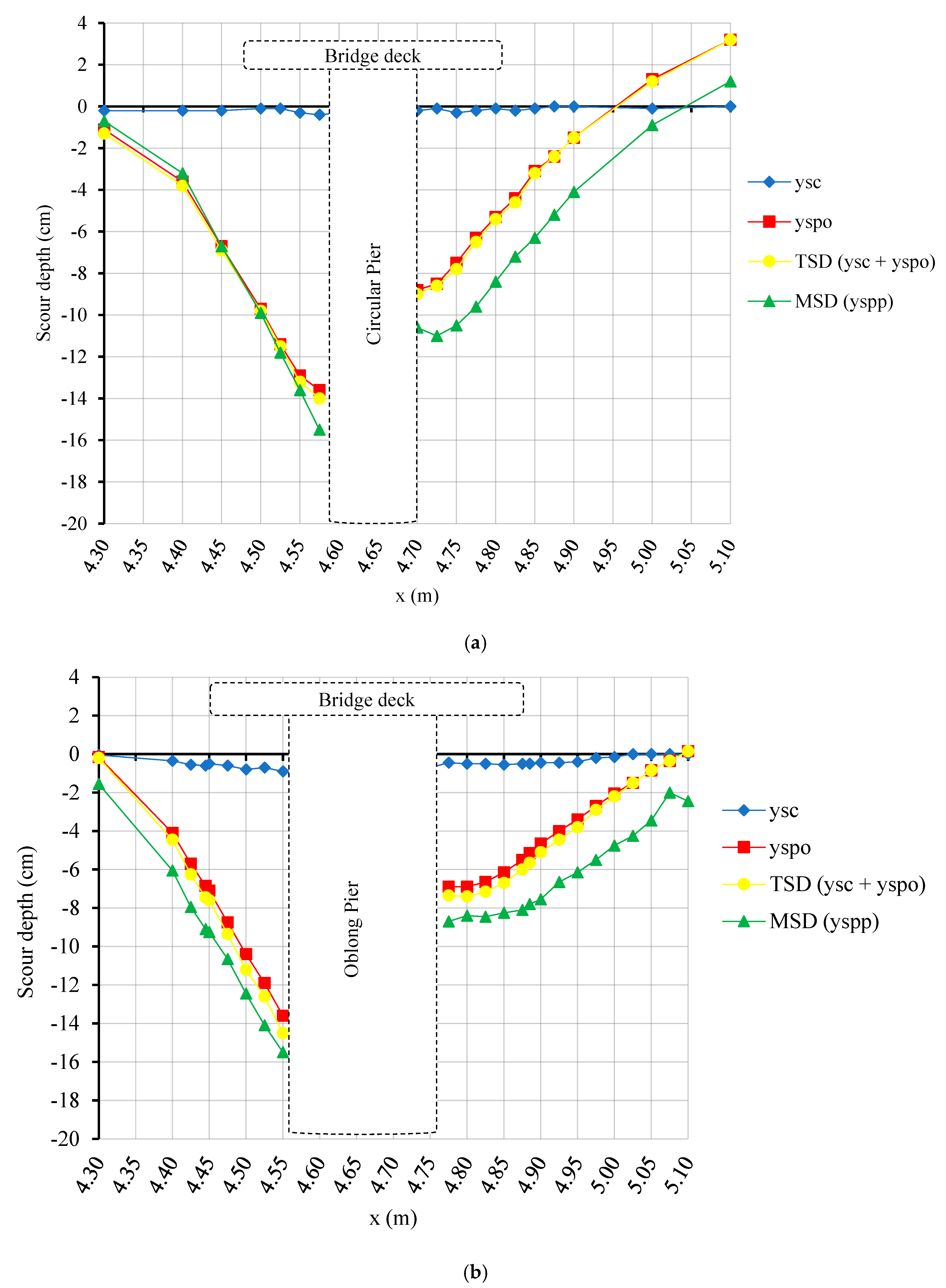
- The pressurized scour depth, MSD, value was smaller than the sum of the vertical contraction and local scour depths, TSD, under the same flow conditions for a small discharge value. It was found that the MSD values were all smaller than the TSD values, up to 24.2% for Group 1, 29.4% for Group 3, and 20.4% for Group 4 for the PPF condition, except for Group 2, for which the MSD value was 42.9% greater than the TSD value. For the FPF condition, the greatest difference between the MSD and TSD values was obtained for Group 4 at 49.4%. So, it can be seen that the pier shape significantly affected the maximum scour depth whether the deck was skewed or not. The difference between the MSD and TSD values was found to decrease as the discharge increased. As the discharge kept increasing, the TSD value was found to be greater than the MSD value for most of the experiments. It was found that the MSD value was greater for all test groups for 120 L/s with a maximum of 38.3% for Group 4 for the PPF condition. However, the MSD values were greater for Groups 1 and 3, with 16.5% and 19.3%, respectively, while the MSD values were 17.8% and 12.2% smaller than the TSD values for Groups 2 and 4, respectively. Thus, it is seen that the pier shape was the dominant parameter of bridge pier scour. Although the number of experiments in the current study is limited, it is encouraging that the current approach adopted in the guidelines seems to enable a safe design in predicting foundation depth at pier sites.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Wardhana, K.; Hadipriono, F.C. Analysis of recent bridge failures in the United States. J. Perform. Constr. Facil. 2003, 17, 144–150. [Google Scholar] [CrossRef]
- Maddison, B. Scour failure of bridges. Proc. Inst. Civ. Eng. Forensic Eng. 2012, 165, 39–52. [Google Scholar] [CrossRef]
- Ettema, R.; Melville, B.W.; Constantinescu, G. Evaluation of Bridge Scour Research: Pier Scour Processes and Predictions; Transportation Research Board of the National Academies: Washington, DC, USA, 2011. [Google Scholar]
- Gibson, A.H.; Matsumoto, T. Flood-induced Bridge Failures in Papua New Guinea. In MATEC Web of Conferences 2019; EDP Sciences: Cedex, France, 2019; Volume 258. [Google Scholar] [CrossRef][Green Version]
- Akay, H.; Baduna Kocyigit, M.; Yanmaz, A.M. Development of a Safety-Inspection Method for River Bridges in Turkey. Water 2019, 11, 1902. [Google Scholar] [CrossRef]
- Akay, H.; Baduna Kocyigit, M. Hydrologic Assessment Approach for River Bridges in Western Black Sea Basin, Turkey. J. Perform. Constr. Facil. 2019, 34, 04019090. [Google Scholar] [CrossRef]
- Boothroyd, R.J.; Williams, R.D.; Hoey, T.B.; Tolentino, P.L.; Yang, X. National-scale assessment of decadal river migration at critical bridge infrastructure in the Philippines. Sci. Total Environ. 2021, 768, 144460. [Google Scholar] [CrossRef]
- Garg, R.K.; Chandra, S.; Kumar, A. Analysis of bridge failures in India from 1977 to 2017. Struct. Infrastruct. Eng. 2022, 18, 295–312. [Google Scholar] [CrossRef]
- Coleman, S.E.; Melville, B.W. Case study: New Zealand Bridge scour experiences. J. Hydraul Eng. 2001, 127, 535–546. [Google Scholar] [CrossRef]
- Wang, C.; Yu, X.; Liang, F. A review of bridge scour: Mechanism. estimation. monitoring and countermeasures. Nat. Hazards 2017, 87, 1881–1906. [Google Scholar] [CrossRef]
- Shahriar, A.R.; Ortiz, A.C.; Montoya, B.M.; Gabr, M.A. Bridge Pier Scour: An overview of factors affecting the phenomenon and comparative evaluation of selected models. Transp. Geotech. 2021, 28, 100549. [Google Scholar] [CrossRef]
- Ciancimino, A.; Jones, L.; Sakellariadis, L.; Anastasopoulos, I.; Foti, S. Experimental assessment of the performance of a bridge pier subjected to flood-induced foundation scour. Géotechnique 2022, 72, 998–1015. [Google Scholar] [CrossRef]
- Baranwal, A.; Das, B.S.; Setia, B. A comparative study of scour around various shaped bridge pier. Eng. Res. Express 2023, 5, 015052. [Google Scholar] [CrossRef]
- Ettema, R.; Constantinescu, G.; Melville, B.W. Flow-field complexity and design estimation of pier-scour depth: Sixty years since Laursen and Toch. J. Hydraul. Eng. 2017, 143, 03117006. [Google Scholar] [CrossRef]
- Abed, L.M. Local Scour around Bridge Piers in Pressure Flow. Ph.D. Dissertation, Colorado State University, Fort Collins, CO, USA, 1991. [Google Scholar]
- Jones, J.S.; Bertoldi, D.A.; Umbrell, E.R. Preliminary studies of pressure flow scour. In Hydraulic Engineering; ASCE: Reston, Virginia, USA, 1993; pp. 916–921. [Google Scholar]
- Arneson, L.A.; Abt, S.R. Vertical contraction scour at bridges with water flowing under pressure conditions. Trans. Res. Rec. 1998, 1647, 10–17. [Google Scholar] [CrossRef]
- Umbrell, E.R.; Young, G.K.; Stein, S.M.; Jones, J.S. Clear-water contraction scour under bridges in pressure flow. J. Hydraul. Eng. 1998, 124, 236–240. [Google Scholar] [CrossRef]
- Lyn, D.A. Pressure-flow scour: A reexamination of the HEC-18 equation. J. Hydraul. Eng. 2008, 134, 1015–1020. [Google Scholar] [CrossRef]
- Guo, J.; Kerenyi, K.; Pagan-Ortiz, J.E. Bridge Pressure Flow Scour for Clear Water Conditions (No. FHWA-HRT-09-041). Turner-Fairbank Highway Research Center. 2009. Available online: https://rosap.ntl.bts.gov/view/dot/862 (accessed on 27 July 2024).
- Shan, H.; Xie, Z.; Bojanowski, C.; Suaznabar, O.; Lottes, S.; Shen, J.; Kerenyi, K. Submerged Flow Bridge Scour under Clear Water Conditions (No. FHWA-HRT-12-034). Turner-Fairbank Highway Research Center. 2012. Available online: https://rosap.ntl.bts.gov/view/dot/24983 (accessed on 25 July 2024).
- Farhangi, A.; Thamer, A.M.A.; Ghazali, A.H.; Yusuf, B. Maximum scour depth at submerged skewed bridge and pier. Malays. J. Civ. Eng. 2016, 28, 20–34. [Google Scholar] [CrossRef]
- Baduna Koçyiğit, M.; Karakurt, O. Pressure flow and weir scour beneath a bridge deck. Can. J. Civ. Eng. 2019, 46, 534–543. [Google Scholar] [CrossRef]
- Carnacina, I.; Pagliara, S.; Leonardi, N. Bridge pier scour under pressure flow conditions. River Res. Appl. 2019, 35, 844–854. [Google Scholar] [CrossRef]
- Baduna Koçyiğit, M.; Karakurt, O.; Akay, H. Effect of various flow, sediment and geometrical parameters on partially or fully submerged deck scour. SN Appl. Sci. 2021, 3, 308. [Google Scholar] [CrossRef]
- Baduna Koçyiğit, M.; Koçyiğit, Ö.; Akay, H.; Demir, G. Experimental investigation of the effect of skew angle on partially or fully submerged deck scour. Can. J. Civ. Eng. 2020, 47, 1027–1036. [Google Scholar] [CrossRef]
- Abdelhaleem, F.S.; Mohamed, I.M.; Shaaban, I.G.; Ardakanian, A.; Fahmy, W.; Ibrahim, A. Pressure-Flow Scour under Bridge Deck in Clear Water Conditions. Water 2023, 15, 404. [Google Scholar] [CrossRef]
- Koushki, M.; Chamani, M.R.; Moghim, M.N. Assessment of equilibrium pressure-flow scour depth using jet flow theory. Int. J. Sediment Res. 2023, 38, 141–151. [Google Scholar] [CrossRef]
- Majid, S.A.; Aamir; Tripathi, S. Pressure-flow scour due to vertical contraction: A review. J. Hydraul. Eng. 2021, 147, 03121002. [Google Scholar] [CrossRef]
- Fleit, G.; Baranya, S.; Ehlers, R.; Bihs, H. CFD modeling of flow and local scour around submerged bridge decks. J. Coast. Hydraul. Struct. 2023, 3, 1–19. [Google Scholar] [CrossRef]
- Bento, A.M.; Pêgo, J.P.; Viseu, T.; Couto, L. Scour Development Around an Oblong Bridge Pier: A Numerical and Experimental Study. Water 2023, 15, 2867. [Google Scholar] [CrossRef]
- Kadam, G.; Dawari, B. Experimental, Analytical, and Numerical Evaluation of Bridge Pier Scouring. Civ. Environ. Eng. 2024, 20, 411–425. [Google Scholar] [CrossRef]
- Richardson, E.V.; Davis, S.R. Evaluating Scour at Bridges (No. FHWA-NHI-01-001). Federal Highway Administration. Office of Bridge Technology. United States. 2001. Available online: https://rosap.ntl.bts.gov/view/dot/50281 (accessed on 28 July 2024).
- Holnbeck, S.R.; Parrett, C.; Tillinger, T.N. Bridge scour and change in contracted section. Razor Creek. In Proceedings of the National Conference on Hydraulic Engineering, San Francisco, CA, USA, 25–30 July 1993; pp. 2249–2255. [Google Scholar]
- Sturm, T.W.; Ettema, R.; Melville, B.M. Evaluation of Bridge-Scour Research: Abutment and Contraction Scour Processes and Prediction; National Co-operative Highway Research Program (NCHRP): Washington, DC, USA, 2011; pp. 1–27. [Google Scholar]
- Hong, S.H.; Abid, I. Physical model study of bridge contraction scour. KSCE J. Civ. Eng. 2016, 20, 2578–2585. [Google Scholar] [CrossRef]
- Saha, R.; Lee, S.O.; Hong, S.H. A comprehensive method of calculating maximum bridge scour depth. Water 2018, 10, 1572. [Google Scholar] [CrossRef]
- Hong, S.H.; Lee, S.O. Insight of bridge scour during extreme hydrologic events by laboratory model studies. KSCE J. Civ. Eng. 2018, 22, 2871–2879. [Google Scholar] [CrossRef]
- Yang, Y.; Xiong, X.; Melville, B.W.; Sturm, T.W. Flow redistribution at bridge contractions in compound channel for extreme hydrological events and implications for sediment scour. J. Hydraulic Eng. 2021, 147, 04021005. [Google Scholar] [CrossRef]
- Dankoo, A.; Yonesi, H.; Torabipoudeh, H.; Saneie, M. The effect of pressure flow conditions on bridge pier scour in compound open channels with vegetation. J. Hydraul. 2022, 17, 89–103. [Google Scholar]
- Yang, Y.; Melville, B.W.; Xiong, X.; Wang, L. Temporal evolution of scour at bridge abutments in compound channels. Int. J. Sediment Res. 2022, 37, 662–674. [Google Scholar] [CrossRef]
- Dankoo, A.; Yonesi, H.A.; Torabipoudeh, H.; Saneie, M.; Rahimi, H. Investigation approaching flow to bridge and prediction of bridge pier scour with floodplain vegetation in compound channels under pressurized flow conditions. SN Appl. Sci. 2023, 5, 185. [Google Scholar] [CrossRef]
- Eldho, T.I. Experimental study on Hydrodynamic Characteristics and Scour in Pressure Flow Conditions under a Bridge Deck. In Proceedings of the 10th International Symposium on Hydraulic Structures (ISHS) 2024, ETH Zurich, Switzerland, 17–19 June 2024. [Google Scholar] [CrossRef]
- Yang, Y.; Xiong, X.; Melville, B.W.; Sturm, T.W. Delineating Flood-Induced Sediment Scour in Bridge-Contracted Channels: Processes, Patterns, and Scour Depth under Various Scour Regimes. J. Hydraul. Eng. 2024, 150, 04024042. [Google Scholar] [CrossRef]
- Harasti, A.; Gilja, G.; Potočki, K.; Lacko, M. Scour at bridge piers protected by the riprap sloping structure: A review. Water 2021, 13, 3606. [Google Scholar] [CrossRef]
- Chauhan, V.; Padhi, E.; Chavan, R.; Singhal, G.D. A review of bridge scour mitigation measures using flow deflecting structures. ISH J. Hydraul. Eng. 2023, 29 (Supp. S1), 434–447. [Google Scholar] [CrossRef]
- Gupta, L.K.; Pandey, M.; Raj, P.A.; Pu, J.H. Scour Reduction around Bridge Pier Using the Airfoil-Shaped Collar. Hydrology 2023, 10, 77. [Google Scholar] [CrossRef]
- JIA, X.; Mou, X.; Ji, H. Experimental study on local scour protection and contact pressure of the facing water surface at the pier based on the guide pillar. Ocean Eng. 2024, 302, 117670. [Google Scholar] [CrossRef]
- Arneson, L.A.; Zevenbergen, L.W.; Lagasse, P.F.; Clopper, P.E. Evaluating Scour at Bridges (No. FHWA-HIF-12-003). National Highway Institute (US). 2012. Available online: https://ponce.sdsu.edu/hec-18-scour.pdf (accessed on 25 September 2024).
- Karakurt, O. Investigation of Scouring under Bridge Deck in Pressured and Weir Type of Flow. Ph.D. Thesis, Civil Engineering Department, Gazi University, Ankara, Turkey, 2016. (In Turkish). [Google Scholar]
- Oliveto, G.; Hager, W.H. Temporal evolution of clear-water pier and abutment scour. J. Hydraul. Eng. 2002, 128, 811–820. [Google Scholar] [CrossRef]
- Dey, S.; Raikar, R.V.; Roy, A. Scour at submerged cylindrical obstacles under steady flow. J. Hydraul. Eng. 2008, 134, 105–109. [Google Scholar] [CrossRef]
- ISO 16331-1:2017(E); Optics and Optical Instruments-Laboratory Procedures for Testing Surveying and Construction Instruments-Part 1: Performance of Handheld Laser Distance Meters. International Organization for Standardization: Geneva, Switzerland, 2017.












| Variable | Abed [15] | Farhangi et al. [22] | Carnacina et al. [24] | Present Study (2024) | |
|---|---|---|---|---|---|
| Flow condition | PPF | FPF, WF | PPF | FPF, WF | FPF, WF |
| Pier shape | OB | RC | CR | CR | OB |
| Pier width (m) | 0.15 | 0.04 | 0.03 | 0.01 | 0.01 |
| Pier length (m) | 1.83 | 0.40 | - | - | 0.02 |
| D50 (mm) | 3.2 | 0.23, 0.80 | 1.0 | 0.8 | 0.8 |
| ya (m) | 0.244–0.579 | 0.19–0.33 | 0.07–0.176 | 0.345 | 0.415 |
| Va (m/s) | 0.305–0.914 | 0.23–0.42 | NA | - | |
| Discharge (m3/s) | 0.181–1.104 | 0.078–0.097 | NA | 0.100–0.120 | 0.100–0.120 |
| Fra (-) | 0.128–0.590 | 0.128–0.300 | NA | - | - |
| Hb (m) | 0.14–0.47 | 0.13–0.24 | 0.038–0.165 | - | - |
| Deck width (m) | 2.44 | - | 0.6 | 1.00 | 1.00 |
| Deck length (m) | 1.83 | 0.04 | 1.8–3.6 | 0.30 | - |
| Skew angle (°) | - | 0–30 | - | 15 | 15 |
| Test duration (hr) | 3 | 9–12 | 6–70 | 9–17 | 9–17 |
| D10 (m) | D50 (m) | D60 (m) | D84.1 (m) | Cu | Cc | σg | |
|---|---|---|---|---|---|---|---|
| Sediment | 0.00084 | 0.00084 | 0.00084 | 0.001 | 1.00 | 1.00 | 1.19 |
| Main Experimental Group Name | Subgroup | Flow Condition | Skew Angle of Deck (°) | Pier Shape | The Angle of Attack of the Pier (°) | # of Experiments |
|---|---|---|---|---|---|---|
| Group 1 | Only deck | PPF, FPF | 0 | - | - | 12 |
| Only pier | FF | 0 | Circular | 0 | 12 | |
| Pier and deck | PPF, FPF | 0 | Circular | 0 | 12 | |
| Group 2 | Only deck | PPF, FPF | 0 | - | - | 12 |
| Only pier | FF | 0 | Oblong | 0 | 12 | |
| Pier and deck | PPF, FPF | 0 | Oblong | 0 | 12 | |
| Group 3 | Only deck | PPF, FPF | 15 | - | - | 12 |
| Only pier | FF | 15 | Circular | 0 | 12 | |
| Pier and deck | PPF, FPF | 15 | Circular | 0 | 12 | |
| Group 4 | Only deck | PPF, FPF | 15 | - | - | 12 |
| Only pier | FF | 15 | Oblong | 0 | 12 | |
| Pier and deck | PPF, FPF | 15 | Oblong | 0 | 12 |
| Q | ya | Effect of Approach Flow Depth (% Relative Difference) | Effect of Discharge (% Relative Difference) | Effect of Skew Angle (% Relative Difference) | Effect of Pier Shape (% Relative Difference) | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| (L/s) | (cm) | G1 | G2 | G3 | G4 | G1 | G2 | G3 | G4 | G1–G3 | G2–G4 | G1–G2 | G3–G4 |
| (1) | (2) | (3) | (4) | (5) | (6) | (7) | (8) | (9) | (10) | (11) | (11) | (12) | (13) |
| Q1–Q3 | Circular pier | Oblong pier | α = 0° | α = 15° | |||||||||
| Q1 100 | 34.5 | - | - | - | - | 21.2 | 26.1 | 8.0 | 38.9 | 11.5 | 5.9 | 1.9 | 6.9 |
| 37.5 | 5.1 | 11.1 | 1.7 | 13.0 | 17.6 | 42.6 | 12.4 | 4.9 | 19.6 | 34.6 | 8.1 | 3.4 | |
| 39.5 | 12.2 | 3.3 | 4.6 | 14.8 | 21.9 | 31.1 | 18.1 | 2.2 | 21.2 | 25.7 | 8.0 | 12.0 | |
| 41.5 | 18.6 | 9.2 | 15.5 | 8.6 | 21.3 | 20.9 | 36.7 | 6.3 | 15.7 | 26.6 | 9.4 | 19.7 | |
| Q3 120 | 34.5 | - | - | - | - | 0.5 | 16.6 | 2.1 | 19.7 | ||||
| 37.5 | 7.9 | 0.5 | 5.9 | 14.7 | 14.4 | 1.0 | 11.5 | 3.5 | |||||
| 39.5 | 11.6 | 0.5 | 4.3 | 15.6 | 17.4 | 2.1 | 16.2 | 3.1 | |||||
| 41.5 | 18.5 | 13.0 | 6.9 | 16.9 | 30.5 | 11.3 | 9.1 | 7.0 | |||||
| Equation No. | Group 1 | Group 2 | Group 3 | Group 4 | |
|---|---|---|---|---|---|
| RMSE | (8) (Current study) | 0.01 | 0.01 | 0.01 | 0.01 |
| (1) (Abed’s equation) | 0.02 | 0.03 | 0.05 | 0.05 | |
| R2 (%) | (1) (Abed’s equation) | 93 | 41 | 31 | 35 |
| Group No. | Q (L/s) | ya (cm) | Fra (-) | ysco (cm) | yspo (cm) | TSD (cm) | MSD yspp.max (cm) | Difference (%) |
|---|---|---|---|---|---|---|---|---|
| 1 | 100 | 34.5 | 0.158 | 0.004 | 0.136 | 0.140 | 0.155 | −9.7 |
| 37.5 | 0.139 | 0.003 | 0.11 | 0.113 | 0.149 | −24.2 | ||
| 39.5 | 0.129 | 0.002 | 0.099 | 0.101 | 0.137 | −26.3 | ||
| 41.5 | 0.119 | 0.001 | 0.084 | 0.085 | 0.127 | −33.1 | ||
| 110 | 34.5 | 0.158 | 0.009 | 0.147 | 0.156 | 0.175 | −10.9 | |
| 37.5 | 0.153 | 0.006 | 0.132 | 0.138 | 0.170 | −18.8 | ||
| 39.5 | 0.141 | 0.003 | 0.120 | 0.123 | 0.157 | −21.7 | ||
| 41.5 | 0.131 | 0.002 | 0.107 | 0.109 | 0.142 | −23.2 | ||
| 120 | 34.5 | 0.189 | 0.030 | 0.158 | 0.188 | 0.189 | −0.5 | |
| 37.5 | 0.167 | 0.025 | 0.145 | 0.170 | 0.158 | 7.6 | ||
| 39.5 | 0.154 | 0.014 | 0.135 | 0.149 | 0.167 | −10.8 | ||
| 41.5 | 0.143 | 0.009 | 0.128 | 0.137 | 0.164 | −16.5 | ||
| 2 | 100 | 34.5 | 0.158 | 0.085 | 0.137 | 0.222 | 0.155 | 42.9 |
| 37.5 | 0.139 | 0.050 | 0.117 | 0.167 | 0.136 | 22.8 | ||
| 39.5 | 0.129 | 0.030 | 0.105 | 0.135 | 0.148 | −9.1 | ||
| 41.5 | 0.119 | 0.010 | 0.090 | 0.100 | 0.139 | −28.2 | ||
| 110 | 34.5 | 0.158 | 0.019 | 0.153 | 0.172 | 0.165 | 4.6 | |
| 37.5 | 0.153 | 0.010 | 0.141 | 0.151 | 0.165 | −8.2 | ||
| 39.5 | 0.141 | 0.030 | 0.139 | 0.169 | 0.178 | −5.1 | ||
| 41.5 | 0.131 | 0.010 | 0.124 | 0.134 | 0.171 | −21.7 | ||
| 120 | 34.5 | 0.189 | 0.064 | 0.162 | 0.225 | 0.193 | 16.6 | |
| 37.5 | 0.167 | 0.046 | 0.143 | 0.188 | 0.194 | −3.1 | ||
| 39.5 | 0.154 | 0.028 | 0.122 | 0.150 | 0.194 | −22.7 | ||
| 41.5 | 0.143 | 0.024 | 0.138 | 0.162 | 0.138 | 17.8 | ||
| 3 | 100 | 34.5 | 0.158 | 0.001 | 0.148 | 0.149 | 0.171 | −12.6 |
| 37.5 | 0.139 | 0.001 | 0.120 | 0.121 | 0.172 | −29.4 | ||
| 39.5 | 0.129 | 0.001 | 0.119 | 0.120 | 0.166 | −27.5 | ||
| 41.5 | 0.119 | 0.001 | 0.107 | 0.108 | 0.146 | −26.4 | ||
| 110 | 34.5 | 0.158 | 0.010 | 0.147 | 0.157 | 0.174 | −10.1 | |
| 37.5 | 0.153 | 0.010 | 0.150 | 0.160 | 0.193 | −17.1 | ||
| 39.5 | 0.141 | 0.005 | 0.146 | 0.150 | 0.185 | −18.7 | ||
| 41.5 | 0.131 | 0.002 | 0.127 | 0.129 | 0.177 | −27.2 | ||
| 120 | 34.5 | 0.189 | 0.045 | 0.168 | 0.212 | 0.188 | 12.8 | |
| 37.5 | 0.167 | 0.035 | 0.149 | 0.184 | 0.199 | −7.3 | ||
| 39.5 | 0.154 | 0.025 | 0.154 | 0.179 | 0.190 | −6.1 | ||
| 41.5 | 0.143 | 0.014 | 0.148 | 0.162 | 0.200 | −19.3 | ||
| 4 | 100 | 34.5 | 0.158 | 0.043 | 0.136 | 0.179 | 0.159 | 12.3 |
| 37.5 | 0.139 | 0.033 | 0.108 | 0.141 | 0.177 | −20.4 | ||
| 39.5 | 0.129 | 0.023 | 0.105 | 0.128 | 0.184 | −30.2 | ||
| 41.5 | 0.119 | 0.016 | 0.073 | 0.089 | 0.175 | −49.4 | ||
| 110 | 34.5 | 0.158 | 0.087 | 0.133 | 0.220 | 0.188 | 17.1 | |
| 37.5 | 0.153 | 0.083 | 0.149 | 0.232 | 0.191 | 21.5 | ||
| 39.5 | 0.141 | 0.059 | 0.143 | 0.201 | 0.187 | 7.5 | ||
| 41.5 | 0.131 | 0.028 | 0.125 | 0.153 | 0.180 | −15.3 | ||
| 120 | 34.5 | 0.189 | 0.123 | 0.144 | 0.266 | 0.224 | 19.0 | |
| 37.5 | 0.167 | 0.103 | 0.163 | 0.266 | 0.192 | 38.3 | ||
| 39.5 | 0.154 | 0.076 | 0.136 | 0.212 | 0.189 | 12.2 | ||
| 41.5 | 0.143 | 0.058 | 0.149 | 0.207 | 0.186 | 11.6 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Baduna Koçyiğit, M.; Koçyiğit, Ö. Experimental Investigation of Bridge Scour under Pressure Flow Conditions. Water 2024, 16, 2773. https://doi.org/10.3390/w16192773
Baduna Koçyiğit M, Koçyiğit Ö. Experimental Investigation of Bridge Scour under Pressure Flow Conditions. Water. 2024; 16(19):2773. https://doi.org/10.3390/w16192773
Chicago/Turabian StyleBaduna Koçyiğit, Müsteyde, and Önder Koçyiğit. 2024. "Experimental Investigation of Bridge Scour under Pressure Flow Conditions" Water 16, no. 19: 2773. https://doi.org/10.3390/w16192773
APA StyleBaduna Koçyiğit, M., & Koçyiğit, Ö. (2024). Experimental Investigation of Bridge Scour under Pressure Flow Conditions. Water, 16(19), 2773. https://doi.org/10.3390/w16192773






