Research on Sediment Erosion and Anti-Wear Coating Materials for Water-Intake Components of Hydraulic Turbines in Sandy Rivers
Abstract
1. Introduction
2. Numerical Simulation of Sand and Water Flow
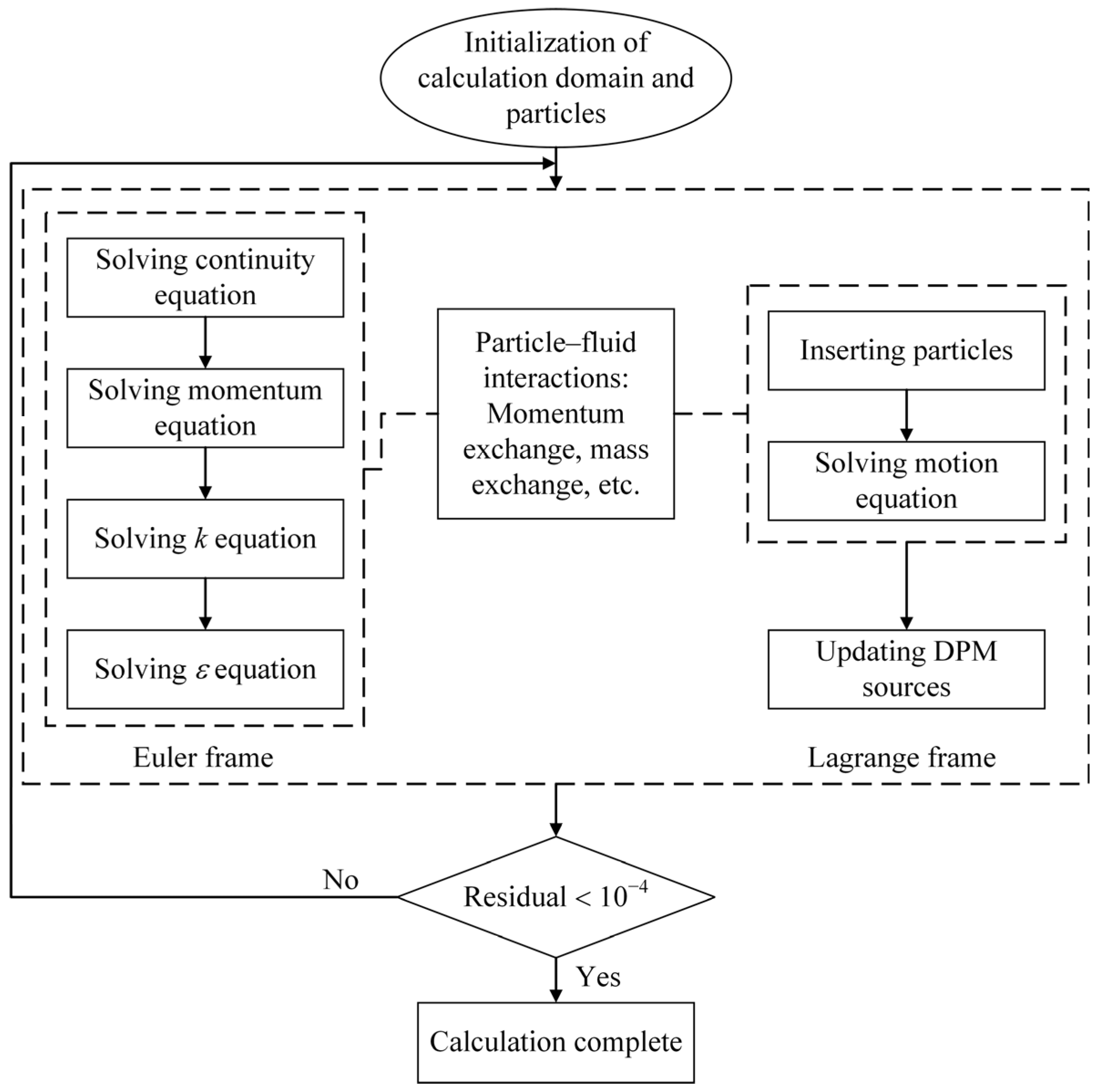
2.1. Mathematical Model
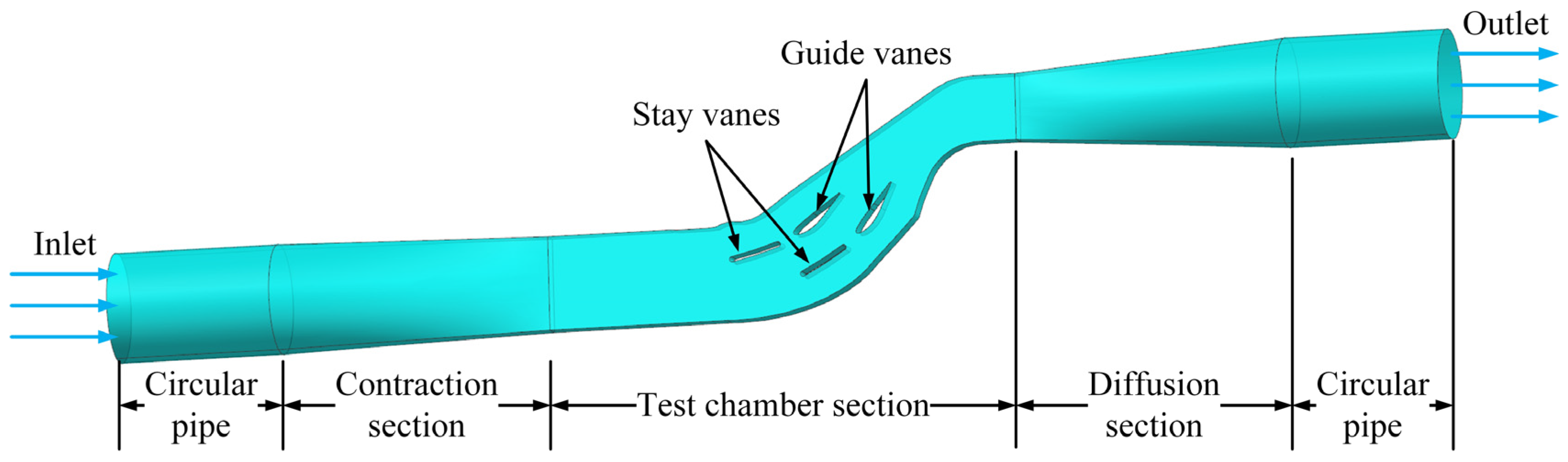
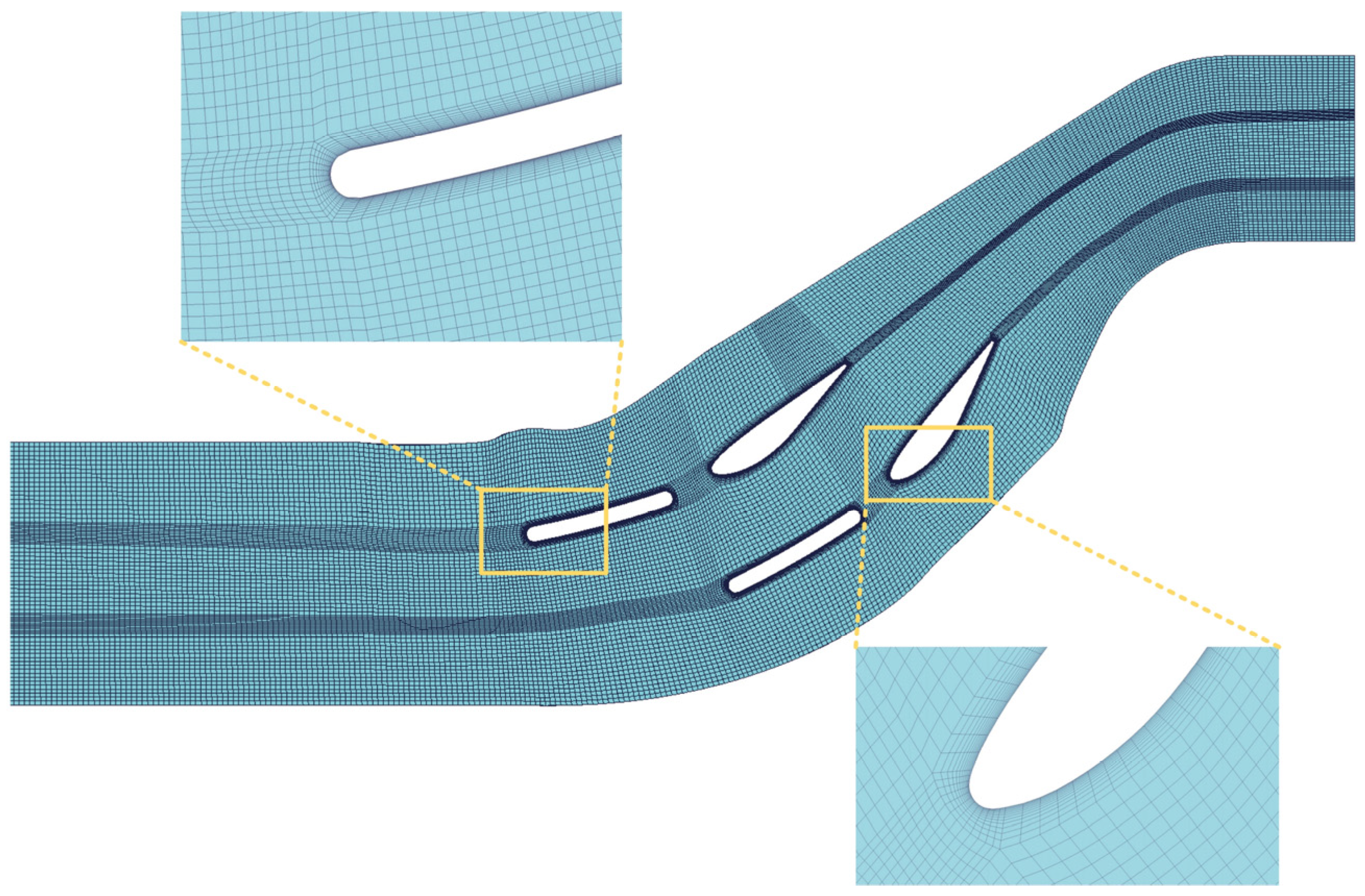
2.2. Geometric Model and Computational Mesh Discretization
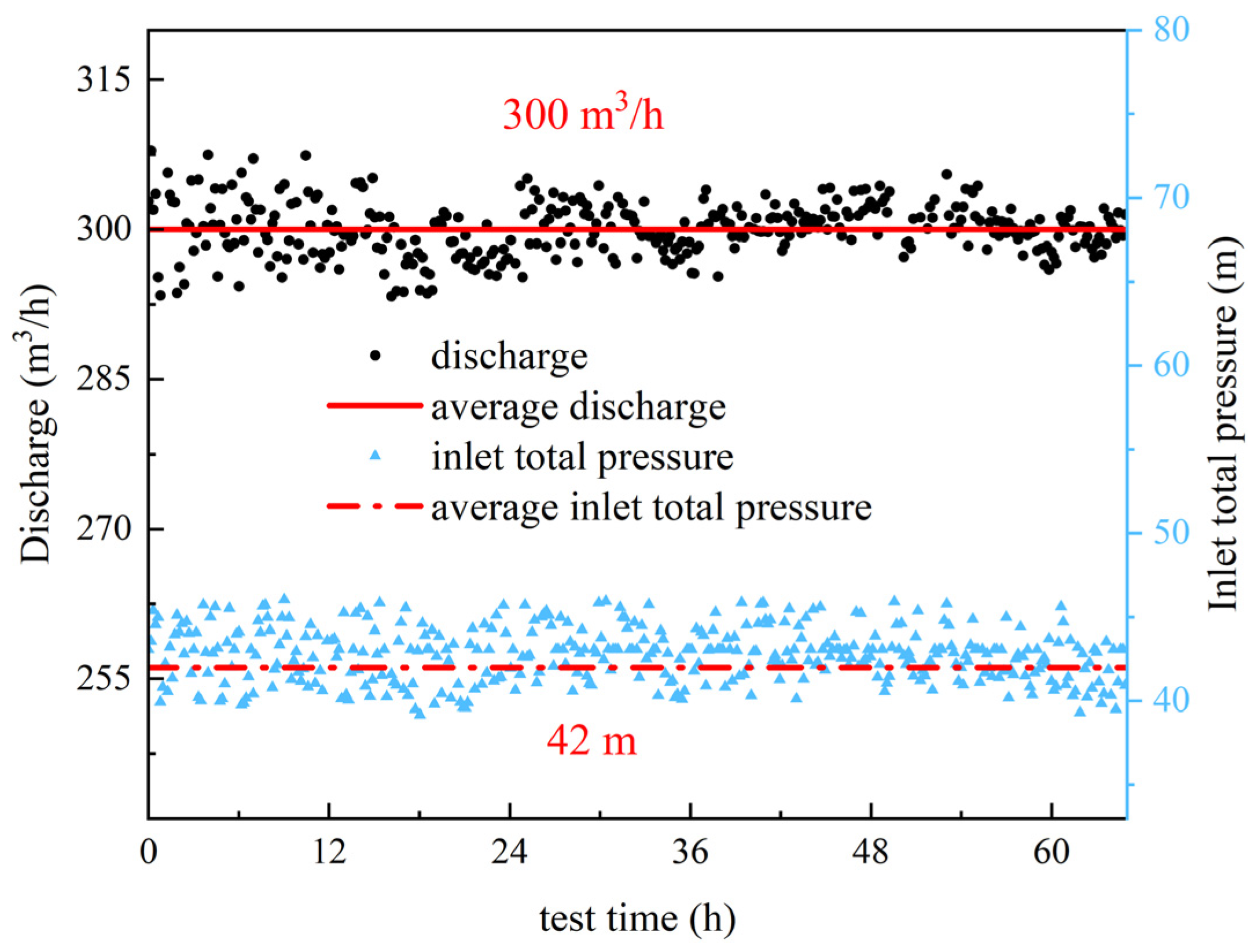
2.3. Boundary Condition
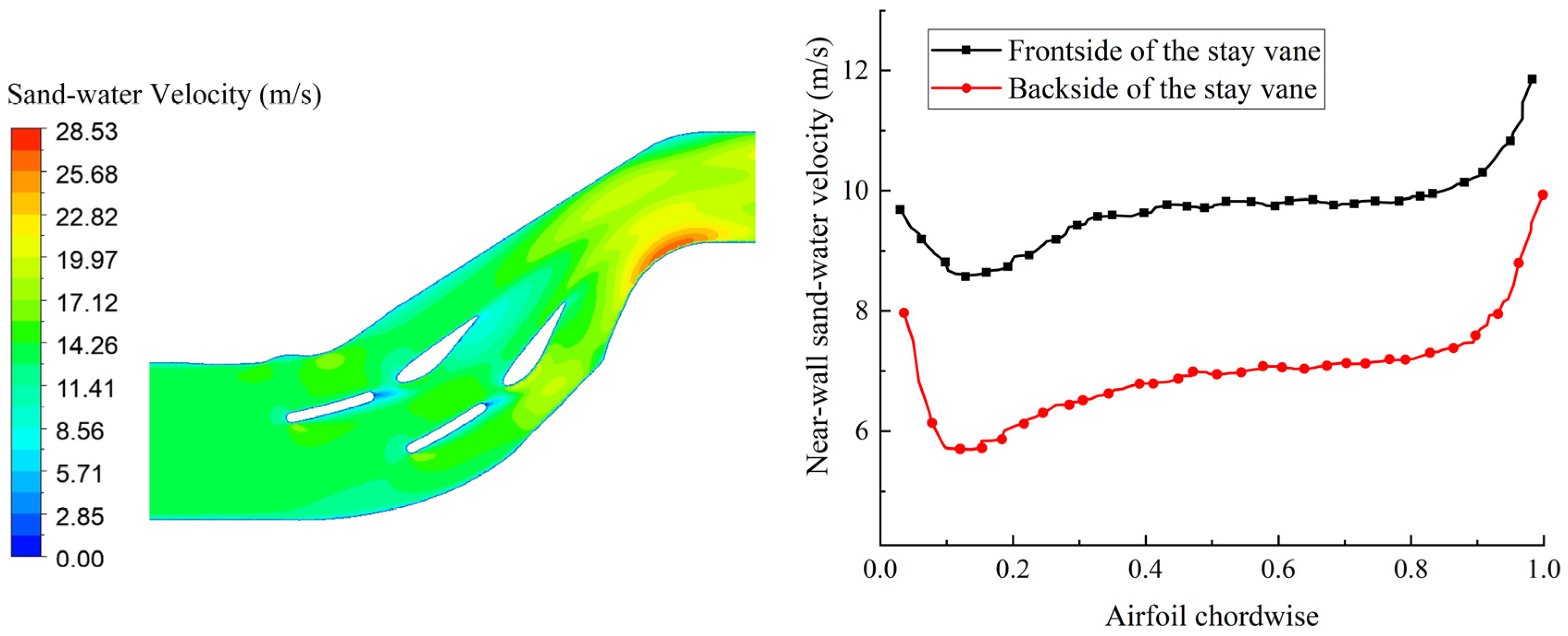
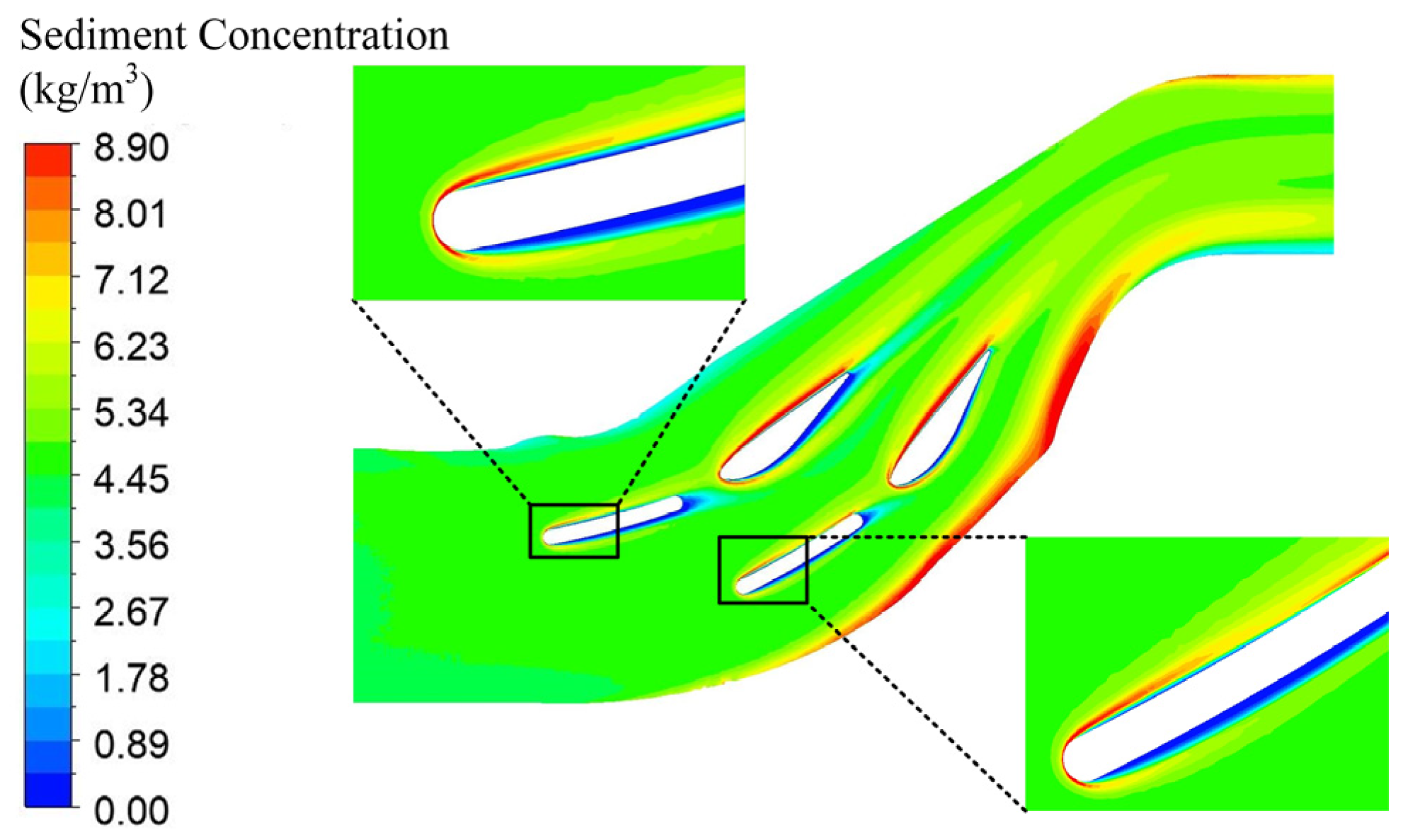
2.4. Numerical Simulation Results and Analysis
3. Test Study on Sediment Erosion of Coating Materials
3.1. Physical Properties of Coating Materials
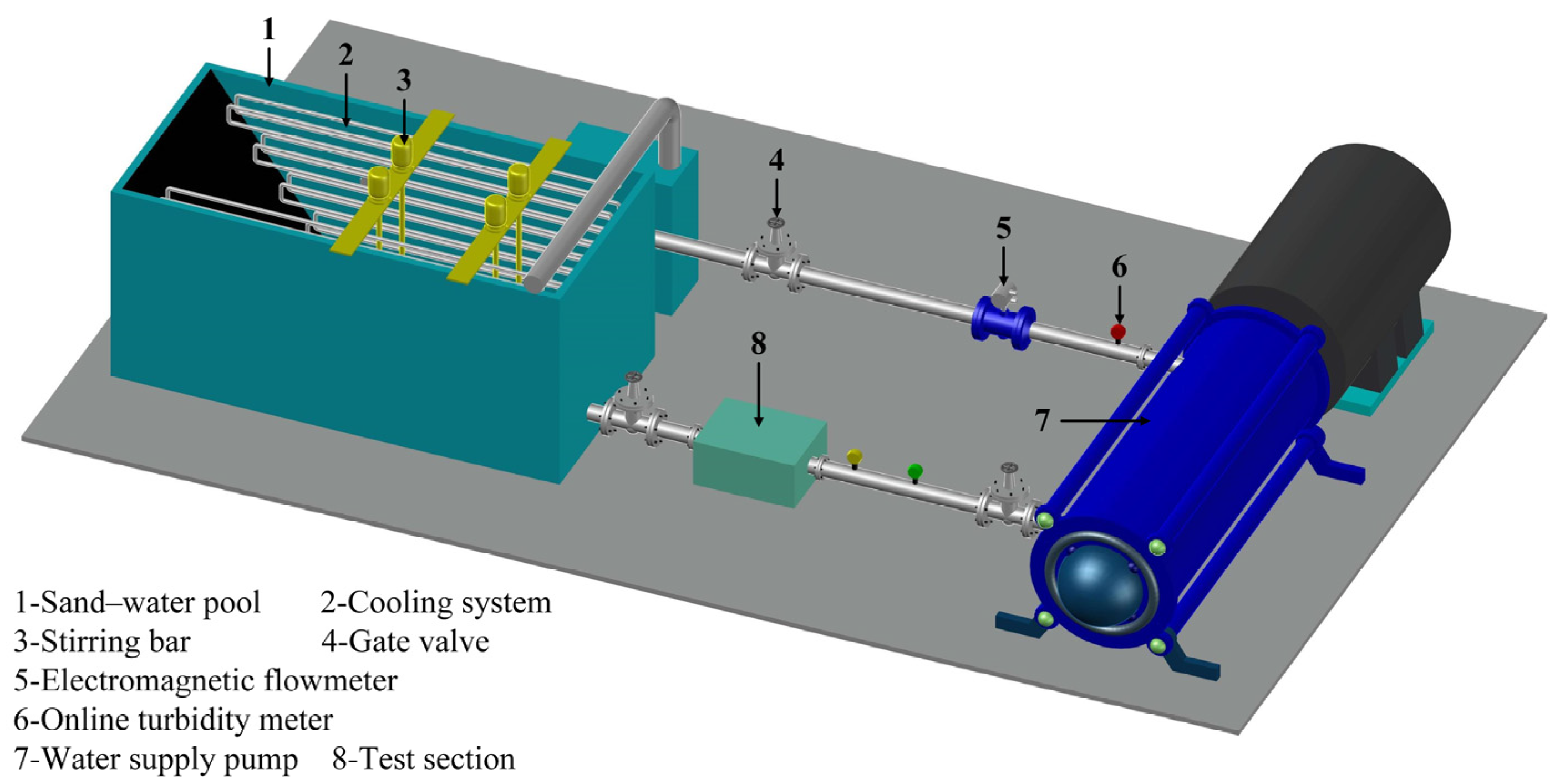
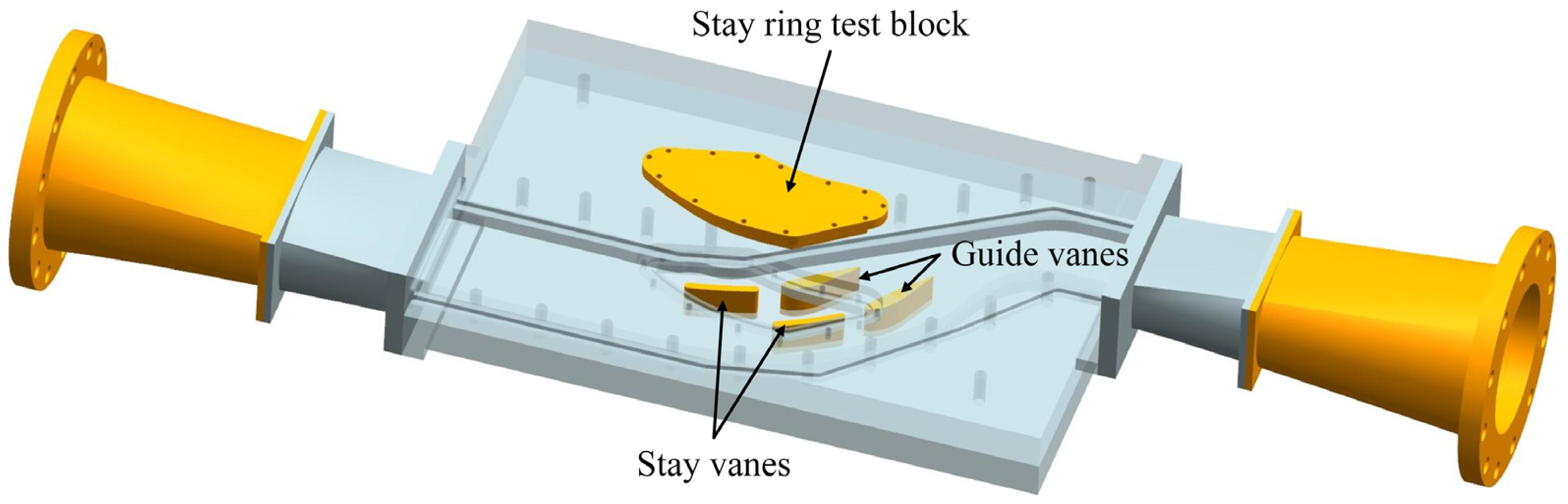
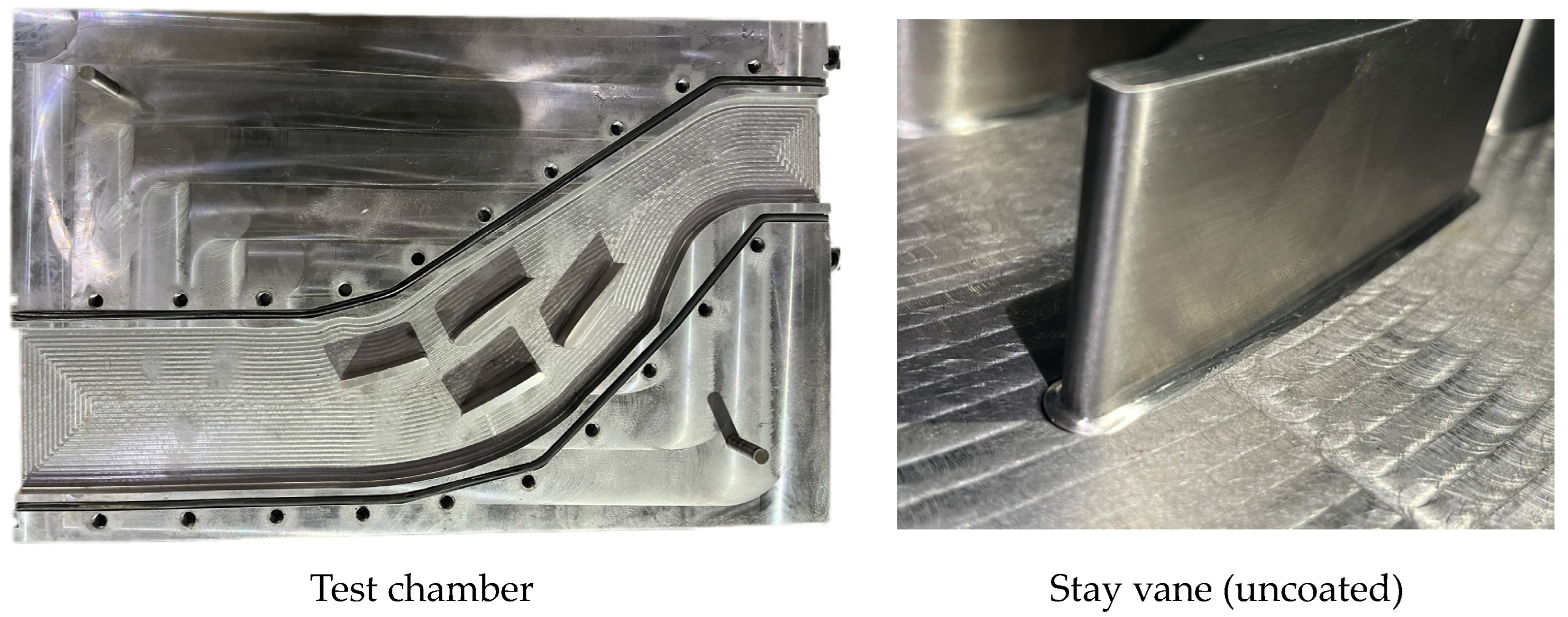
3.2. Test System
3.3. Measurement Method
3.4. Analysis of Test Results
4. Erosion Estimation Model for the Erosion-Resistant Coating Material
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Finnie, I. Erosion of surfaces by solid particle. Wear 1960, 3, 87–103. [Google Scholar] [CrossRef]
- Tabakoff, W.; Kotwal, R.; Hamed, A. Erosion study of different materials affected by coal ash particles. Wear 1979, 52, 161–173. [Google Scholar] [CrossRef]
- McLaury, B.S. A Model to Predict Solid Particle Erosion in Oilfeld Geometries. Ph.D. Thesis, University of Tulsa, Tulsa, OK, USA, 1993. [Google Scholar]
- Oka, Y.I.; Okamura, K.; Yoshida, T. Practical estimation of erosion damage caused by solid particle impact. Wear 2005, 259, 95–101. [Google Scholar] [CrossRef]
- Thapa, S.B.; Thapa, B.; Dahlhaug, G.O. Empirical modelling of sediment erosion in Francis turbines. Energy 2012, 41, 386–391. [Google Scholar] [CrossRef]
- Aslam Noon, A.; Kim, M.H. Erosion wear on Francis turbine components due to sediment flow. Wear 2017, 378–379, 126–135. [Google Scholar] [CrossRef]
- Koirala, R.; Thapa, B.; Neopane, H.P.; Zhu, B.; Chhetry, B. Sediment erosion in guide vanes of Francis turbine: A case study of Kaligandaki Hydropower Plant, Nepal. Wear 2016, 362–363, 53–60. [Google Scholar]
- Wei, X.Y.; Pei, J.; Wang, W.Q.; Yu, Z.F. Numerical study on sediment erosion characteristics of Francis turbine runner. Eng. Fail. Anal. 2024, 161, 108270. [Google Scholar] [CrossRef]
- Sharma, S.; Gandhi, B.K. Assessment of erosion wear in low specific speed Francis turbine due to particulate flow. Adv. Powder Technol. 2023, 34, 104065. [Google Scholar] [CrossRef]
- Zhang, R. Wear analysis of hydraulic turbine shell based on solid-liquid two-phase flow. Value Eng. 2022, 41, 83–85. [Google Scholar]
- Zhang, R. CFD-based study of hydraulic turbine guide vane sediment erosion. Yunnan Water Power 2023, 39, 224–227. [Google Scholar]
- Zhao, X.Y.; Liu, X.B.; Chen, J.R. Sediment wear of hydraulic turbine moving guide vane at Yingxiuwan hydropower station. Chin. J. Hydrodyn. 2021, 36, 728–734. [Google Scholar]
- Pang, J.Y.; Zhang, H.Z.; Yang, J.M.; Chen, Y.; Liu, X.B. Numerical and experimental study on sediment erosion of Francis turbine runner for hydropower stations. Chin. J. Hydrodyn. 2020, 35, 436–443. [Google Scholar]
- Pang, J.Y.; Liu, H.Z.; Liu, X.B.; Yang, H.; Peng, Y.; Zeng, Y.; Yu, Z. Study on sediment erosion of high head Francis turbine runner in Minjiang River basin. Renew. Energy 2022, 192, 849–858. [Google Scholar] [CrossRef]
- Peng, G.J.; Wang, Z.W.; Xiao, Y.X.; Yongyao, L. Abrasion predictions for Francis turbines based on liquid–solid two-phase fluid simulations. Eng. Fail. Anal. 2013, 33, 327–335. [Google Scholar]
- Pan, J.L.; Liu, Q.; Pang, J.Y.; Zhang, F.; Tao, R.; Xiao, R.; Zhou, L.; Liu, W. Comparative evaluation of sand erosion in reversible turbine at pump mode and turbine mode. J. Energy Storage 2024, 79, 110185. [Google Scholar] [CrossRef]
- Koirala, R.; Neopane, P.H.; Zhu, B.; Thapa, B. Effect of sediment erosion on flow around guide vanes of Francis turbine. Renew. Energy 2019, 136, 1022–1027. [Google Scholar] [CrossRef]
- Thapa, S.B.; Trivedi, C.; Dahlhaug, G.O. Design and development of guide vane cascade for a low speed number Francis turbine. J.Hydrodyn. Ser. B 2016, 28, 676–689. [Google Scholar] [CrossRef]
- Hu, L.W.; Liang, A.; Li, H.C.; Zhang, W.; Zhu, B. Impact of rotor-stator axial spacing on the gas-liquid-solid flow characteristics of a multiphase rotodynamic pump based on the Euler multi-fluid model. Phys. Fluids. 2024, 36, 1–17. [Google Scholar] [CrossRef]
- Tian, W.W.; Liu, X.B.; Li, J.N.; Yuan, S.; Lu, S.Y.; Li, Y.B. Experimental study on sediment wear of guide vanes of Francis turbine in high head hydropower station. J. Chin. Soc. Power Eng. 2020, 40, 686–692. [Google Scholar]
- Gandhi, B.K.; Borse, S.V. Effect of particle size and size distribution on estimating erosion wear of cast iron in sand-water slurries. Indian J. Eng. Mater. Sci. 2002, 9, 480–486. [Google Scholar]
- Nguyen, V.B.; Nguyen, Q.B.; Zhang, Y.W.; Lim, C.Y.; Khoo, B.C. Effect of particle size on erosion characteristics. Wear 2016, 348, 126–137. [Google Scholar] [CrossRef]
- Sharma, S.; Gandhi, B.K. Experimental investigation on rotating domain wear of hydrodynamic machine due to particulate flow. Powder Technol. 2022, 410, 117884. [Google Scholar] [CrossRef]
- Casteletti, L.C.; Arnoni, E.A.B.; Neto, A.L.; Fernandes, F.A.; Totten, G.E. Effect of binders and surface finish on wear resistance of HVOF coatings. Surf. Eng. 2010, 26, 440–446. [Google Scholar] [CrossRef]
- Bolelli, G.; Berger, L.M.; Börner, T.; Koivuluoto, H.; Lusvarghi, L.; Lyphout, C.; Markocsan, N.; Matikainen, V.; Nylén, P.; Sassatelli, P.; et al. Tribology of HVOF- and HVAF-sprayed WC–10Co4Cr hardmetal coatings: A comparative assessment. Surf. Coat. Technol. 2015, 265, 125–144. [Google Scholar] [CrossRef]
- Fu, L.; Chen, X.M.; Liu, W.; Wei, G.Y.; Mao, P.Z.; Zhao, J. Organizational structure and anti-wear properties of HVOF sprayed Cr3C2-25NiCr coatings and WC-12Co coatings. Corros. Prot. 2022, 43, 56–60. [Google Scholar]
- Zhao, X.Y.; Peng, Y.J.; Yang, J.X.; Chen, J.; Xu, L.; Tang, W.; Liu, X. Sediment wear of turbine guide vane before and after tungsten carbide treatment. Adv. Mech. Eng. 2022, 14, 16878132221089435. [Google Scholar] [CrossRef]
- Maharajan, S.; Ravindran, D.; Rajakarunakaran, S.; Khan, M.A. Analysis of surface properties of tungsten carbide (WC) coating over austenitic stainless steel (SS316) using plasma spray process. Mater. Today Proc. 2020, 27, 2463–2468. [Google Scholar] [CrossRef]
- Xing, Z.G.; Lv, Z.L.; Cui, Y. Erosion resistance of polyurethane toughened modified epoxy resin bonded SiC particles wear-resistant coatings. Mater. Mech. Eng. 2010, 34, 84–87+100. [Google Scholar]
- Zhang, L.; Chen, W.J.; Li, G.; Zhou, H.; Cui, T.F.; Shi, G.S. Research on Abrasion Resistance of Movable Guide Vane Protection Material for Hydraulic Turbine. Water Resour. Power 2023, 41, 211–214. [Google Scholar]
- Hu, S.K.; Deng, C.H.; Yu, J. Application of HTPB-modified epoxy resin composites on hydraulic turbine blades. Rubber Sci. Technol. 2012, 10, 26–27+30. [Google Scholar]
- Liang, A.; Li, H.C.; Zhang, W.W.; Yao, Z.; Zhu, B.; Wang, F. Study on pressure fluctuation and rotating stall characteristics in the vaneless space of a pump-turbine in pump mode. J. Energy Storage 2024, 94, 1–19. [Google Scholar] [CrossRef]
- Koirala, R.; Thapa, B.; Neopane, P.H.; Zhu, B. A review on flow and sediment erosion in guide vanes of Francis turbines. Renew. Sustain. Energy Rev. 2016, 75, 1054–1065. [Google Scholar] [CrossRef]
- ANSYS Inc. ANSYS Fluent Theory Guide; ANSYS Inc.: Canonsburg, PA, USA, 2022. [Google Scholar]
- Pang, J.Y.; Chang, X.; Gang, Y.Y.; Zhou, Z.; Xiang, W.; Zhou, L.; Liu, X.; Wang, Z. Design and Study of a Sediment Erosion Test Device for a Single-Flow Channel in the Guide Apparatus of a Reaction Hydraulic Turbine. J. Mar. Sci. Eng. 2024, 12, 777. [Google Scholar] [CrossRef]
- ASTM G73-10; Standard Test Method for Liquid Impingement Erosion Using Rotating Apparatus. ASTM International: West Conshohocken, PA, USA, 2010.
- ASTM G76-18; Standard Test Method for Conducting Erosion Tests by Solid Particle Impingement Using Gas Jets. ASTM International: West Conshohocken, PA, USA, 2018.





| Item | EP-512 Indicator | EP-530 Indicator |
|---|---|---|
| Color | aluminium red | white |
| Lustre | flat | flat |
| Standard film thickness | Wet film: 100 micron/channel Dry film: 50 micron/channel | Wet film: 90 micron/channel Dry film: 80 micron/channel |
| Theoretical coating rate | about 10 m2/L | about 8 m2/L |
| Flash point | 28 °C | 23 °C |
| Proportion | Approx. 1.5 (after mixing the two components) | Approx. 1.4 (after mixing the two components) |
| Curing agent content (%) | 70 | 95 |
| Test Specimen | Coating Material | Coating Thickness (mm) | Erosion Rate |
|---|---|---|---|
| 1 | Epoxy mortar | 2 | E1 |
| 2 | Epoxy mortar | 1 | E2 |
| 3 | Epoxy mortar + 30% curing agent | 2 | E3 |
| Stay ring test block | Epoxy mortar | 1 | E |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, Y.; Gang, Y.; Su, L.; Wang, T.; Cai, Y.; Li, X.; Liu, X.; Pang, J. Research on Sediment Erosion and Anti-Wear Coating Materials for Water-Intake Components of Hydraulic Turbines in Sandy Rivers. Water 2024, 16, 2764. https://doi.org/10.3390/w16192764
Wang Y, Gang Y, Su L, Wang T, Cai Y, Li X, Liu X, Pang J. Research on Sediment Erosion and Anti-Wear Coating Materials for Water-Intake Components of Hydraulic Turbines in Sandy Rivers. Water. 2024; 16(19):2764. https://doi.org/10.3390/w16192764
Chicago/Turabian StyleWang, Yongfei, Yuanyuan Gang, Lei Su, Tong Wang, Yinhui Cai, Xiaofei Li, Xiaobing Liu, and Jiayang Pang. 2024. "Research on Sediment Erosion and Anti-Wear Coating Materials for Water-Intake Components of Hydraulic Turbines in Sandy Rivers" Water 16, no. 19: 2764. https://doi.org/10.3390/w16192764
APA StyleWang, Y., Gang, Y., Su, L., Wang, T., Cai, Y., Li, X., Liu, X., & Pang, J. (2024). Research on Sediment Erosion and Anti-Wear Coating Materials for Water-Intake Components of Hydraulic Turbines in Sandy Rivers. Water, 16(19), 2764. https://doi.org/10.3390/w16192764





