Analysis and Identification of Eccentricity of Axial-Flow Impeller by Variational Mode Decomposition
Abstract
1. Introduction
2. Research Object
2.1. Axial-Flow Unit
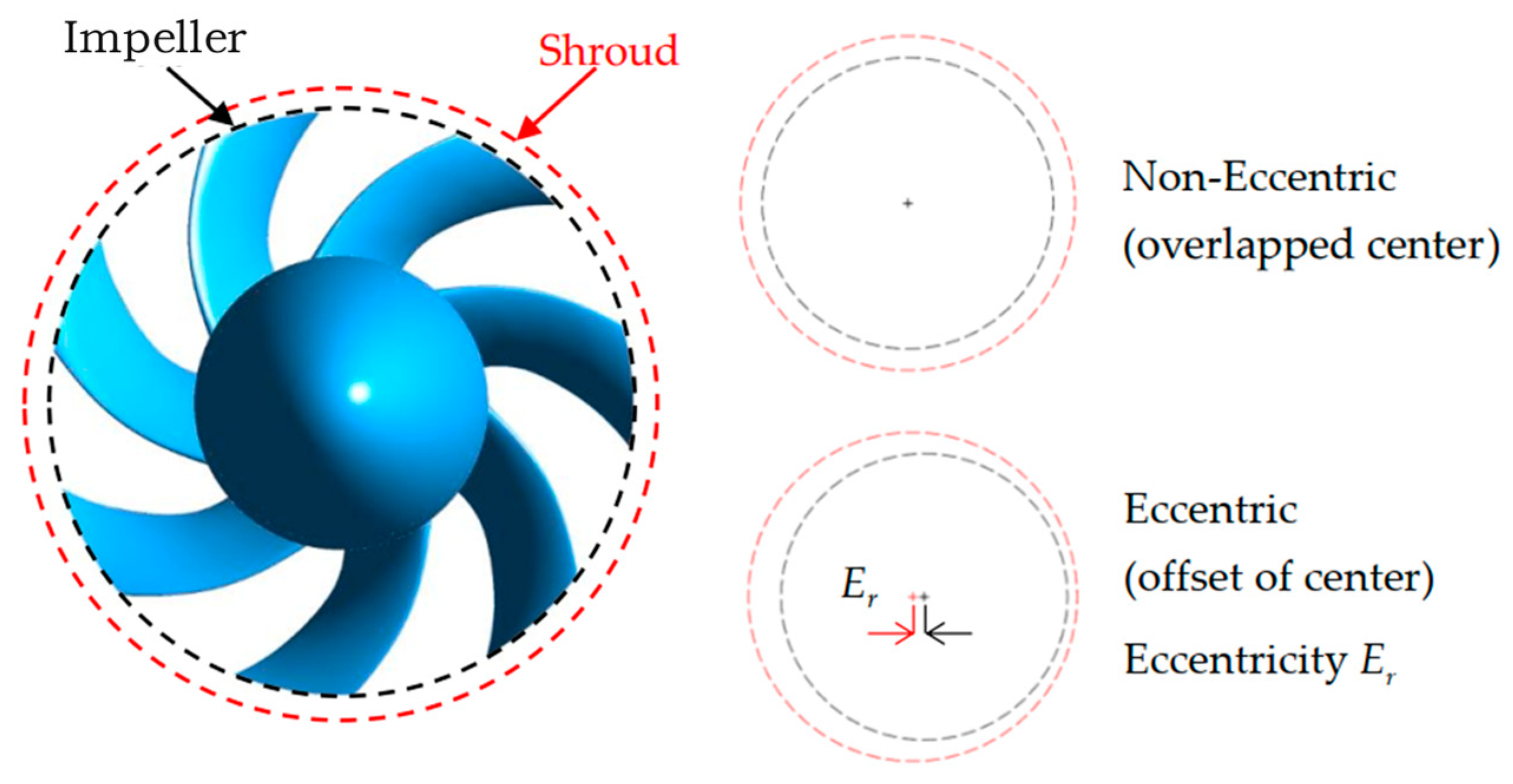
2.2. Impeller Eccentricity
3. Numerical and Mathematical Methods
3.1. Detached Eddy Simulation Model
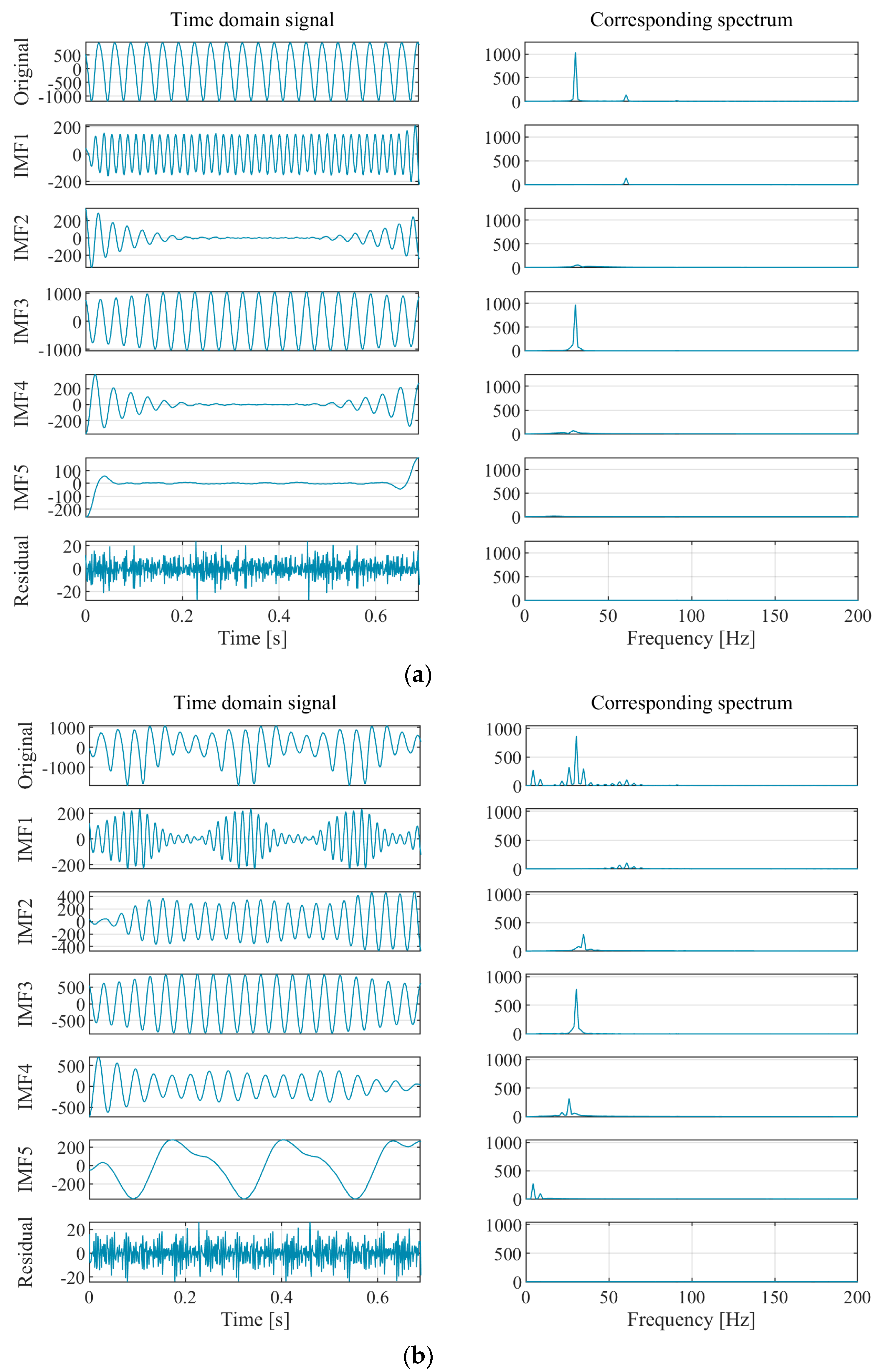
3.2. Variational Mode Decomposition
4. Computational Fluid Dynamics Settings
4.1. Setup of Simulation
- (1)
- The inflow of the inlet section is set as the computational domain inlet boundary; the condition is set as the velocity inlet type. Inlet conditions include Vin = 2.378 m/s and ∂p/∂x = 0. Additionally, turbulence intensity is 5%.
- (2)
- The outlet of the exit section is designated as the final computational domain, and the condition of the outlet is set as a pressure outlet. The equation for outlet conditions is p = 1 atm and ∂V/∂x = 0.
- (3)
- To exchange data among domain parts, several interfaces are adopted. The interfaces between the impeller and guide vane and between the impeller and outlet section are set as rotor–stator interfaces, while the other interfaces are set as stationary. Walls are set as no-slip type.
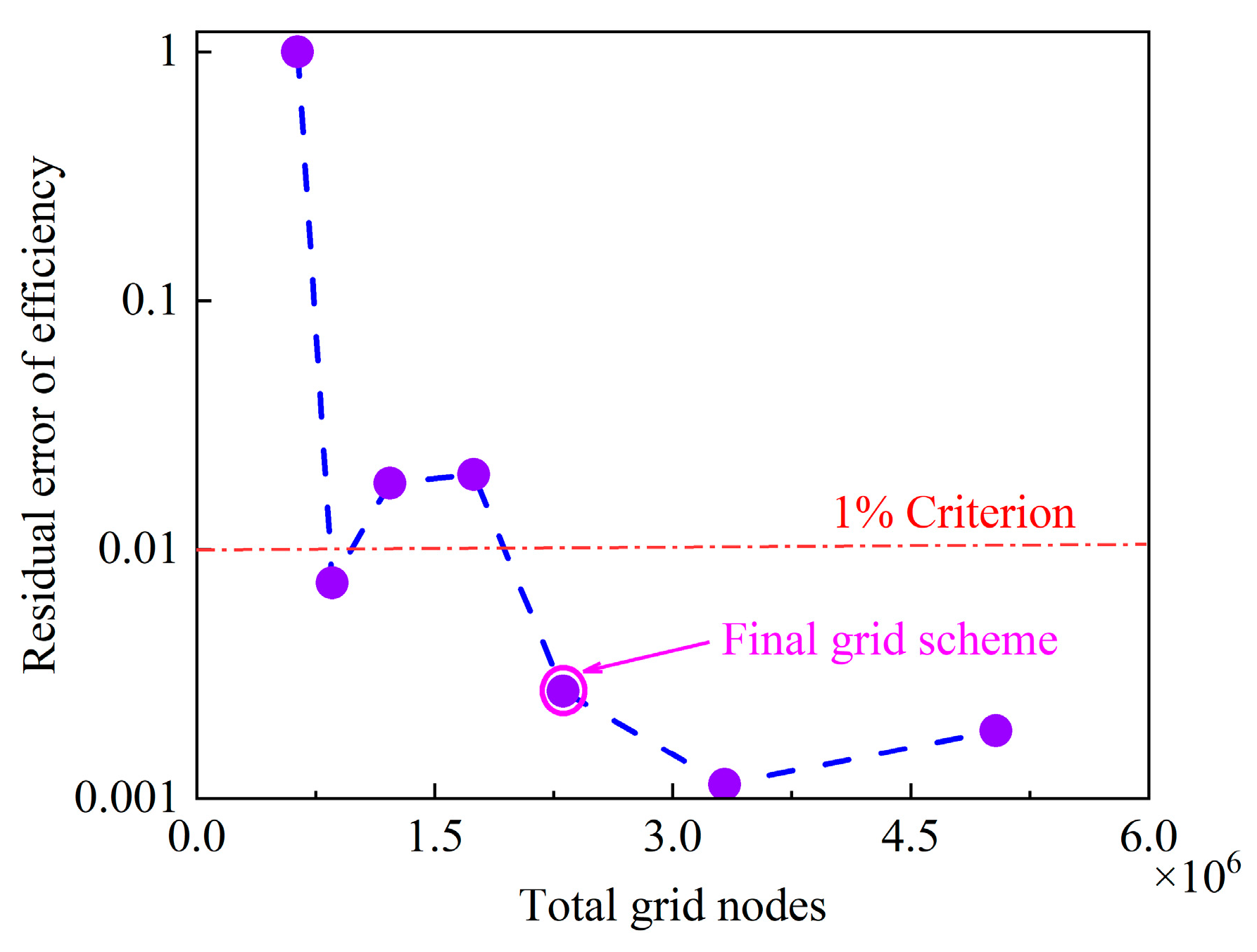
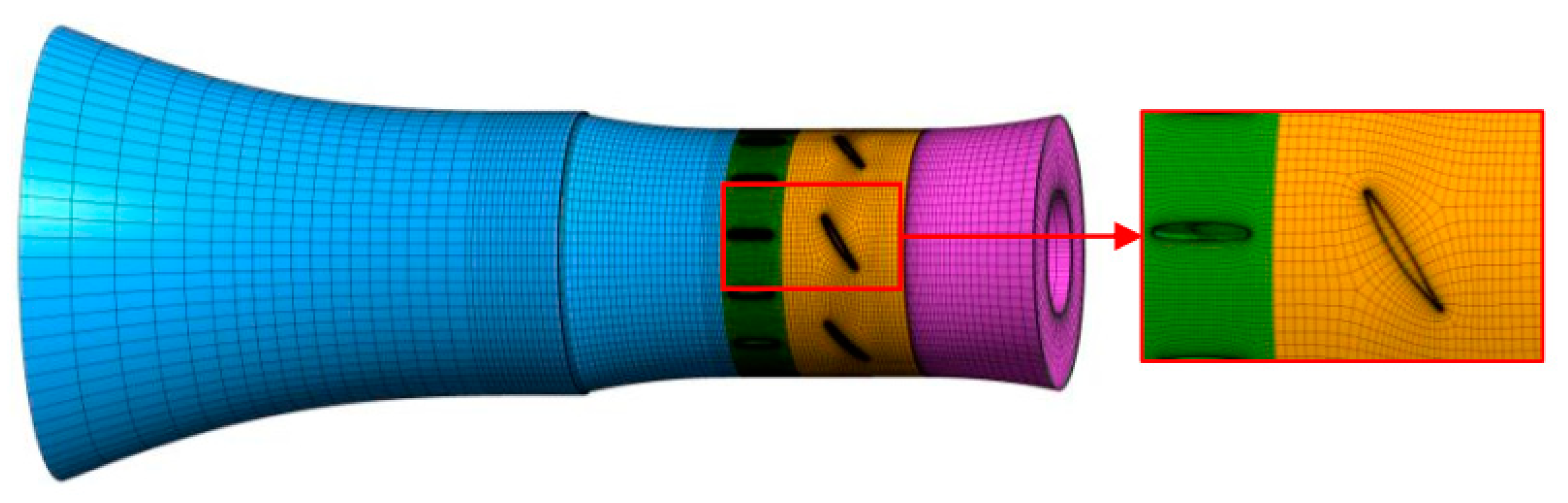
4.2. Grid Preparation and Check
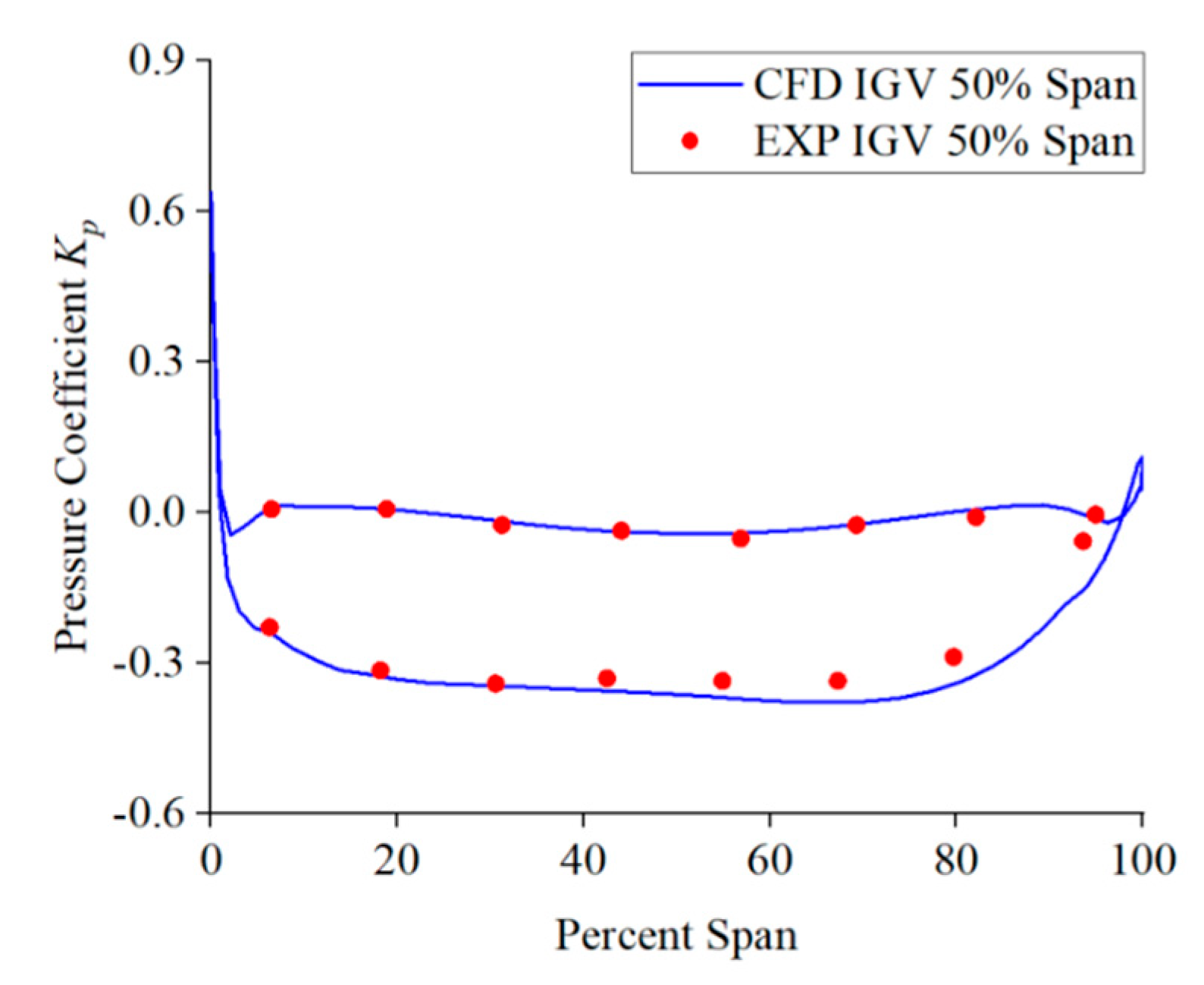
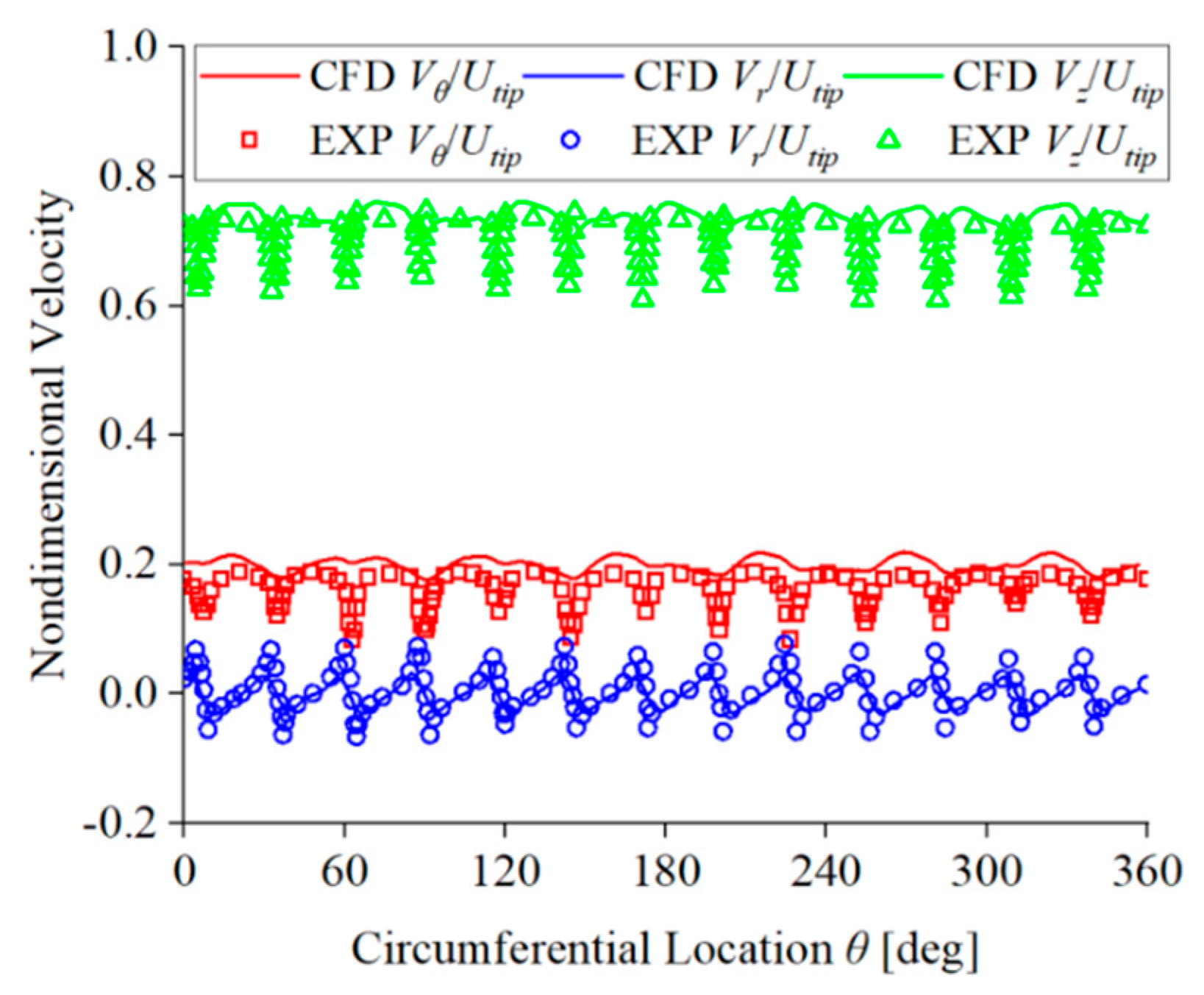
4.3. Experimental–Numerical Validation
5. Analysis of Simulation Results
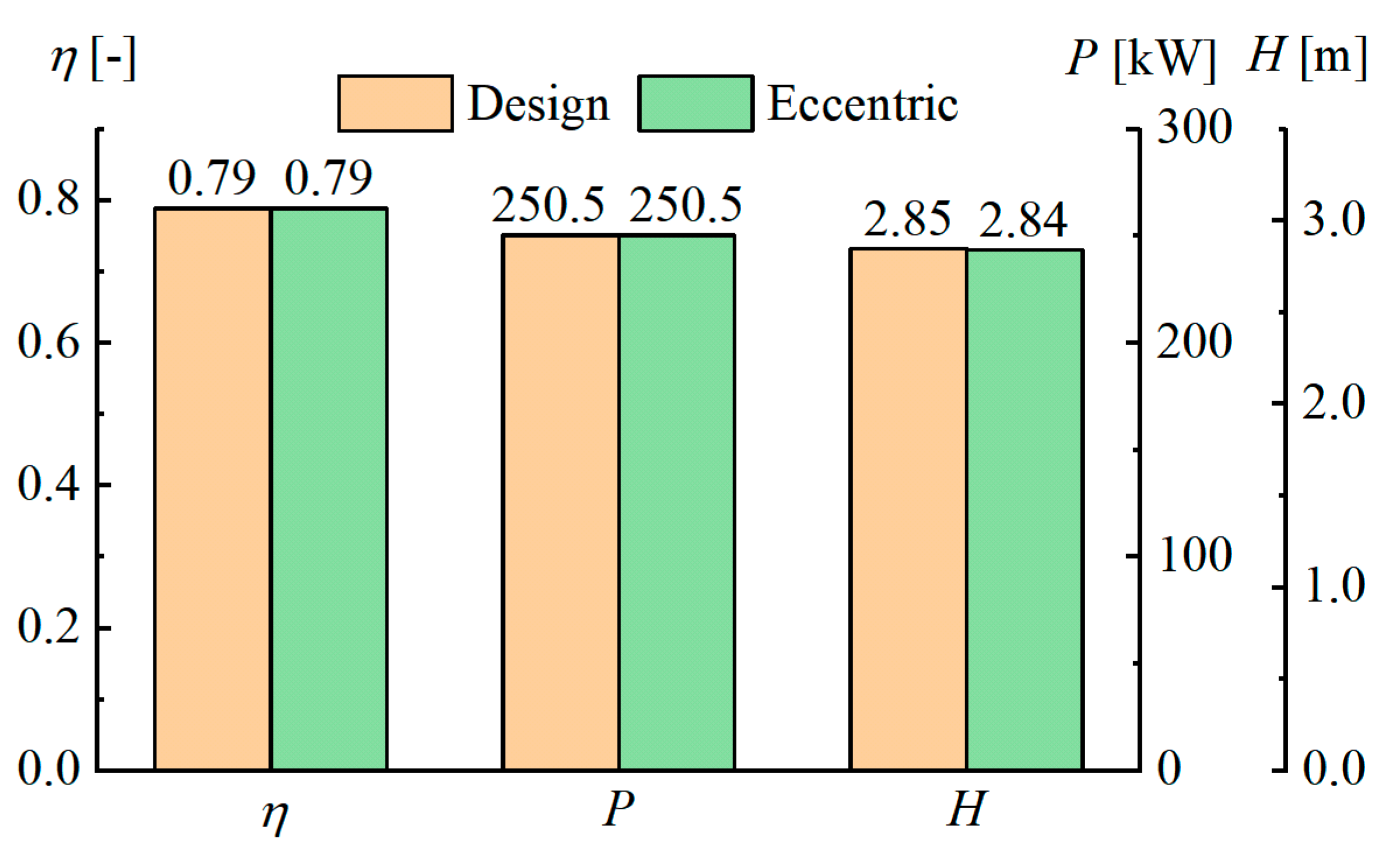
5.1. Performance Comparison
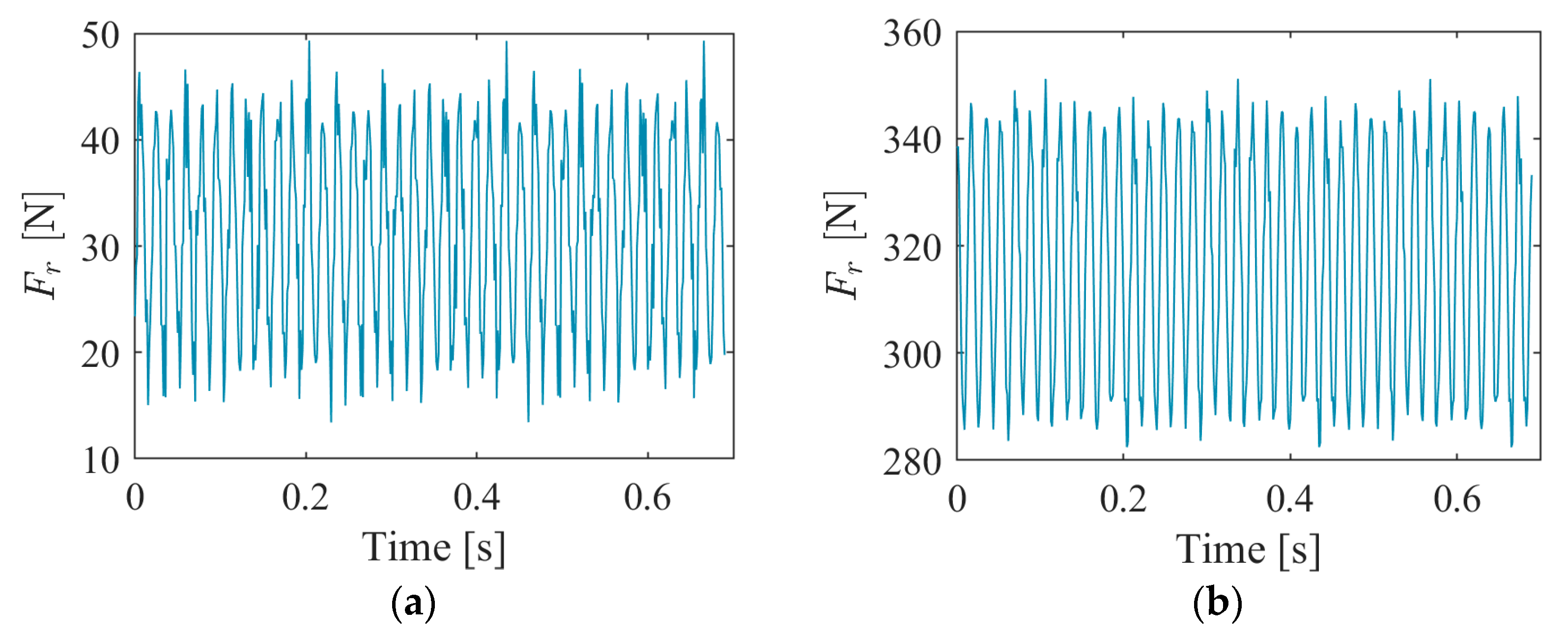
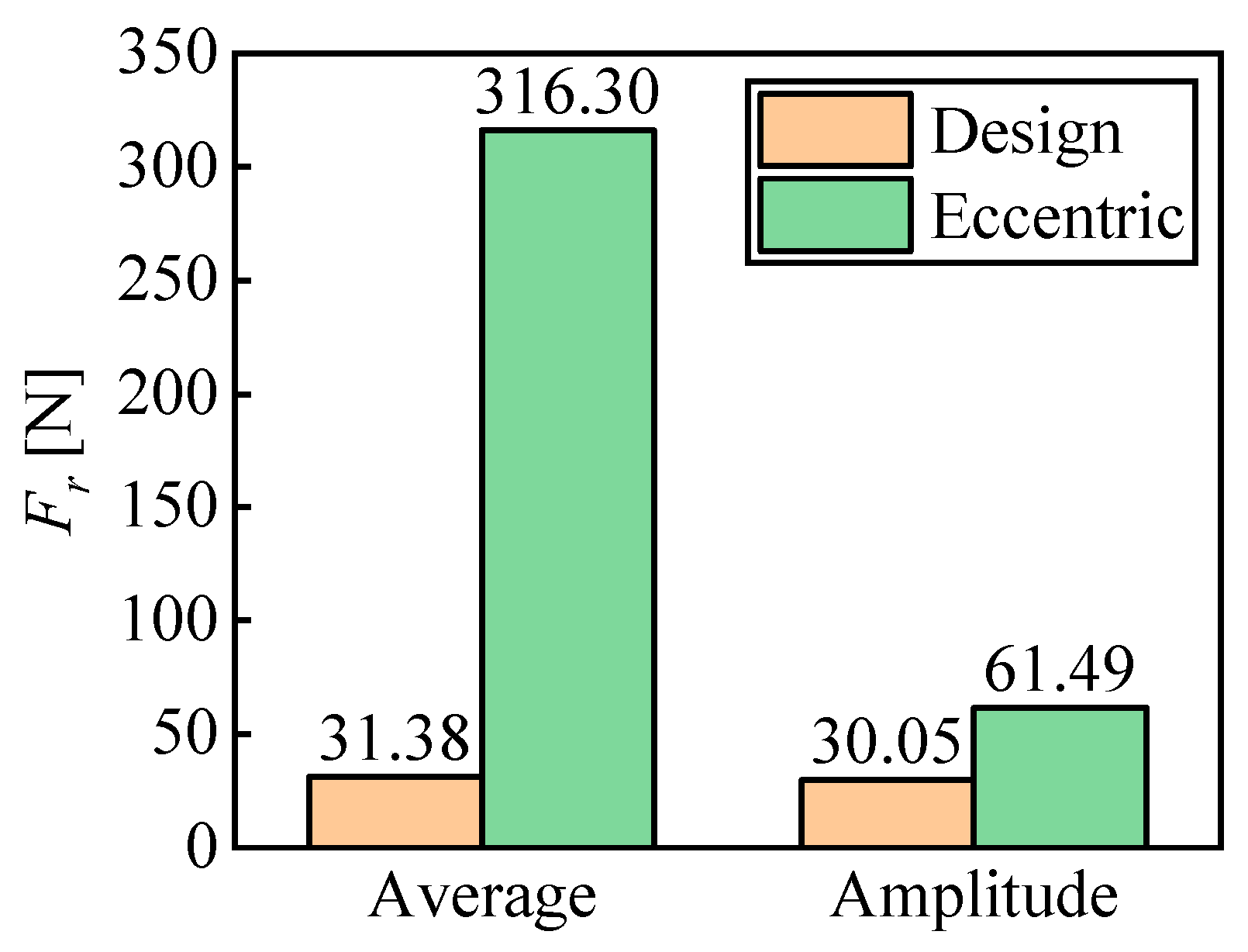
5.2. Comparison of Radial Force
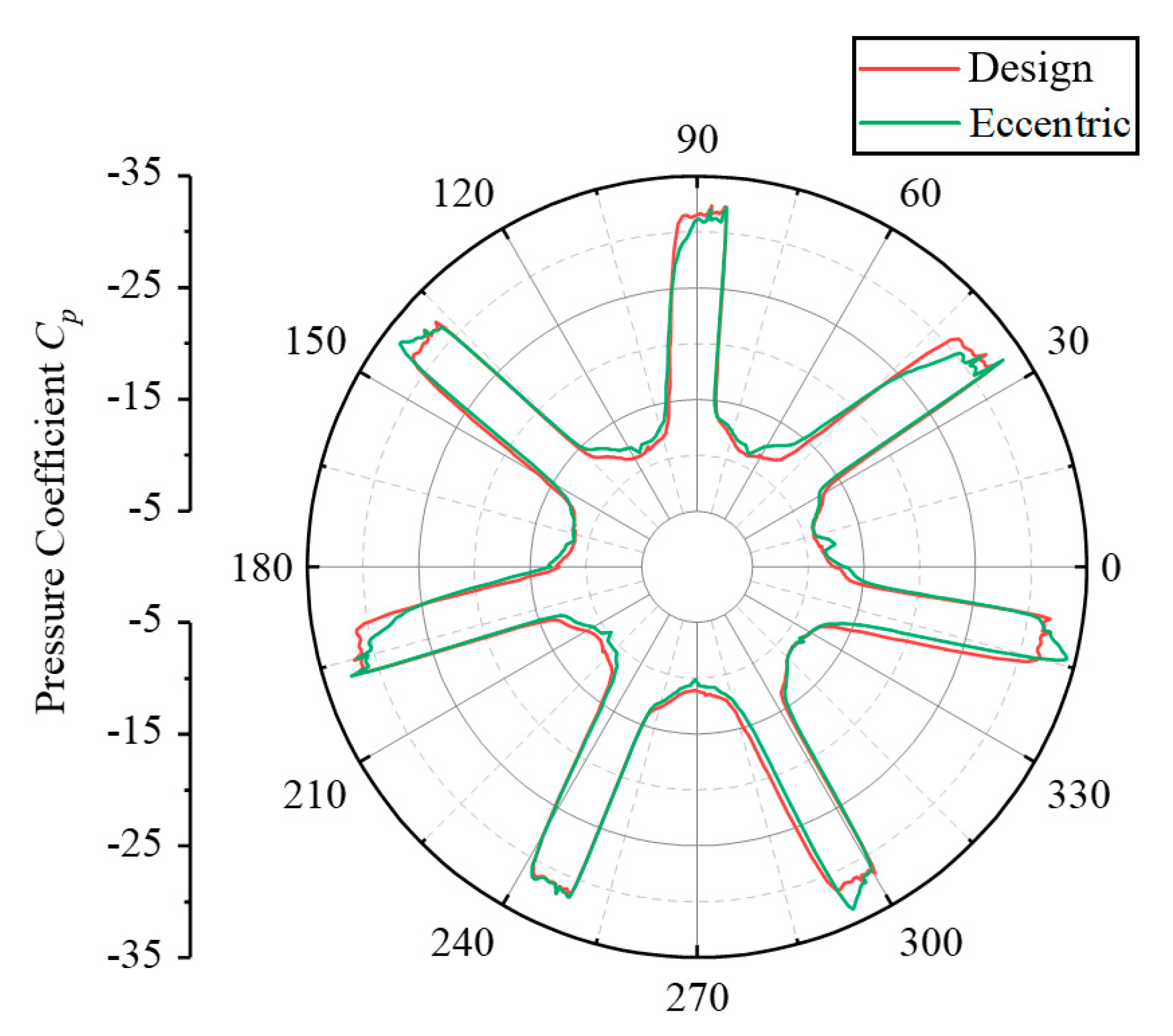
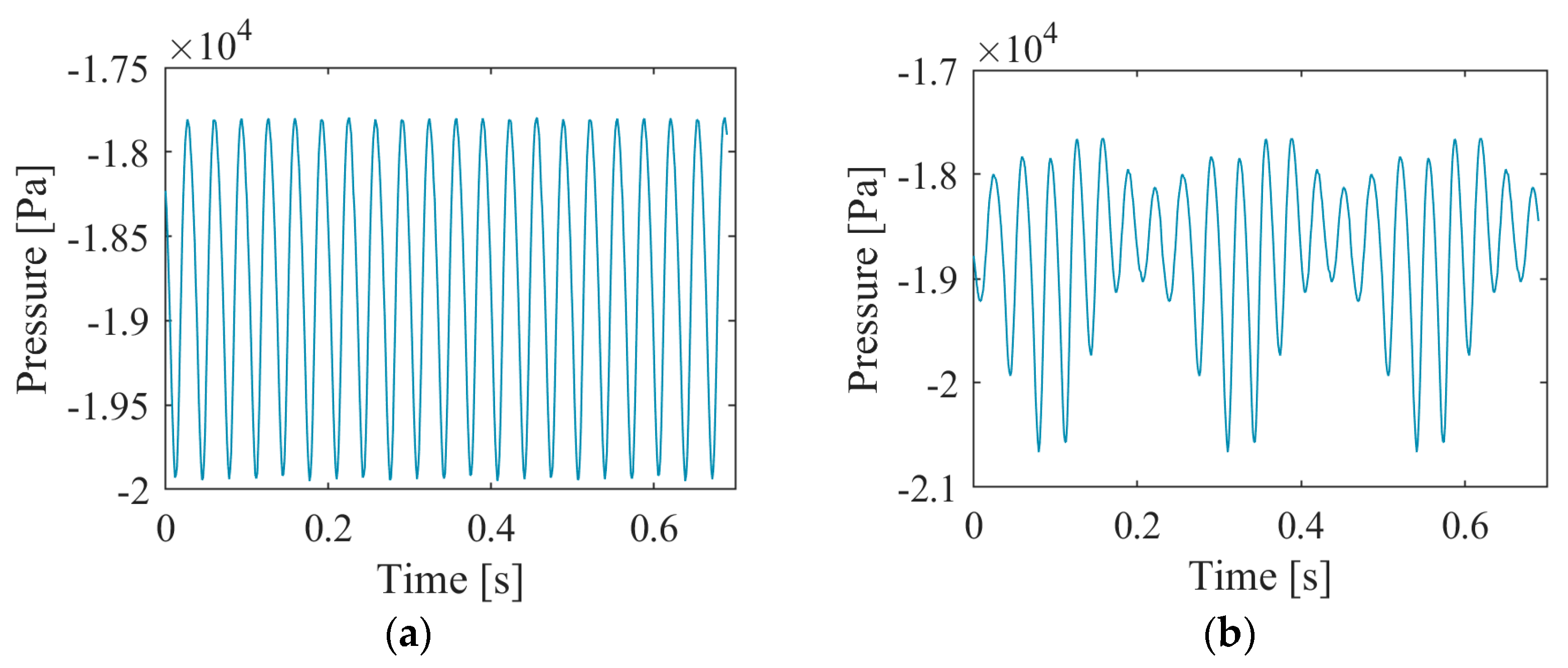
5.3. Pressure Pulsation Comparison
5.4. Comparison of Blade Tip Vortex
6. Conclusions and Discussion
- (a)
- Under eccentric operating conditions, the performance of the unit changes very little. Under design conditions, the efficiency of the unit is about 80%, the power is about 251 kW, and the head is about 2.85 m. When the impeller is eccentric, the efficiency, power, and head of the unit vary by less than 1%.
- (b)
- Eccentricity of the impeller can cause a sharp increase in radial force on the impeller. Under design conditions, the average radial force of the impeller is 31.38 N; under eccentric conditions, the average value of the impeller radial force increased by nine times, reaching 316.30 N.
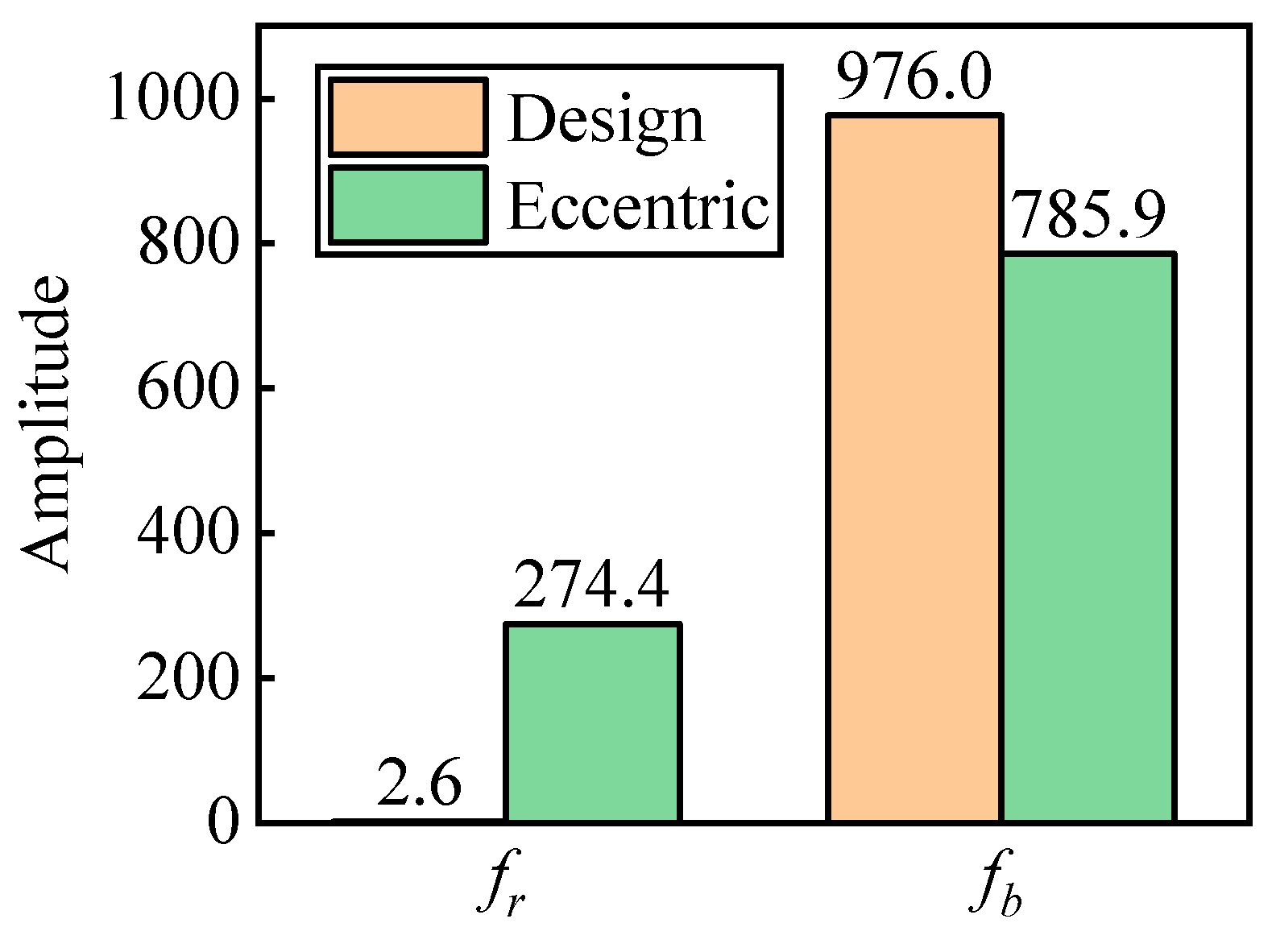
- (c)
- Impeller eccentricity will increase the influence of impeller frequency on downstream pressure pulsation. Under design conditions, fr has almost no effect on pressure pulsation, with a corresponding amplitude of 2.6. Under eccentric conditions, the amplitude corresponding to fr increased by more than 100 times, reaching 274.4.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Han, H.; Jia, Y.; Zhang, Y. Technology research and test analyses of shaft sealing reactor coolant pump full load test. Large Electr. Mach. Hydraul. Turbine 2017, 251, 21–23. [Google Scholar]
- Tao, R.; Xiao, R.; Liu, W. Investigation of the flow characteristics in a main nuclear power plant pump with eccentric impeller. Nucl. Eng. Des. 2018, 327, 70–81. [Google Scholar] [CrossRef]
- Furukawa, A.; Shigemitsu, T.; Watanabe, S. Performance test and flow measurement of contra-rotating axial flow pump. J. Therm. Sci. 2007, 16, 7–13. [Google Scholar] [CrossRef]
- Miller, G.; Corren, D.; Armstrong, P.; Franceschi, J. A study of an axial-flow turbine for kinetic hydro power generation. Energy 1987, 12, 155–162. [Google Scholar] [CrossRef]
- Zhang, Y.L.; Li, J.F.; Wang, T.; Xiao, J.J.; Jia, X.Q.; Zhang, L. Pressure distribution on the inner wall of the volute casing of a centrifugal pump. Sci. Technol. Nucl. Install. 2022, 2022, 3563459. [Google Scholar] [CrossRef]
- Zhu, H.; Bo, G.; Zhou, Y.; Zhang, R.; Cheng, J. Pump selection and performance prediction for the technical innovation of an axial-flow pump station. Math. Probl. Eng. 2018, 2018, 6543109. [Google Scholar] [CrossRef]
- Al-Obaidi, A.R.; Qubian, A. Effect of outlet impeller diameter on performance prediction of centrifugal pump under single-phase and cavitation flow conditions. Int. J. Nonlinear Sci. Numer. Simul. 2022, 23, 1203–1229. [Google Scholar] [CrossRef]
- Al-Obaidi, A.R.; Alhamid, J. Investigation of the main flow characteristics mechanism and flow dynamics within an axial flow pump based on different transient load conditions. Iran. J. Sci. Technol. Trans. Mech. Eng. 2023, 47, 1397–1415. [Google Scholar] [CrossRef]
- Al-Obaidi, A.R. Effect of Different Guide Vane Configurations on Flow Field Investigation and Performances of an Axial Pump Based on CFD Analysis and Vibration Investigation. Exp. Tech. 2023, 48, 69–88. [Google Scholar] [CrossRef]
- Al-Obaidi, A.R.; Khalaf, H.; Alhamid, J. Investigation of the Influence of Varying Operation Configurations on Flow Behaviors Characteristics and Hydraulic Axial-Flow Pump Performance. In Proceedings of the 4th International Conference on Science Education in The Industrial Revolution 4.0, ICONSEIR, Medan, Indonesia, 24 November 2022. [Google Scholar]
- Al-Obaidi, A.R. Investigation of effect of pump rotational speed on performance and detection of cavitation within a centrifugal pump using vibration analysis. Heliyon 2019, 5, e01910. [Google Scholar] [CrossRef]
- Xia, X.; Luo, H.; Li, S.; Wang, F.; Zhou, L.; Wang, Z. Characteristics and factors of mode families of axial turbine runner. Int. J. Mech. Sci. 2023, 251, 108356. [Google Scholar] [CrossRef]
- Wu, H.; Miorini, R.L.; Katz, J. Measurements of the tip leakage vortex structures and turbulence in the meridional plane of an axial water-jet pump. Exp. Fluids 2011, 50, 989–1003. [Google Scholar] [CrossRef]
- Zhang, D.; Shi, W.; Bin, C.; Guan, X. Unsteady flow analysis and experimental investigation of axial-flow pump. J. Hydrodyn. Ser. B 2010, 22, 35–43. [Google Scholar] [CrossRef]
- Lin, F.; Chen, J. Oscillatory tip leakage flows and stability enhancement in axial compressors. Int. J. Rotating Mach. 2018, 2018, 9076472. [Google Scholar] [CrossRef]
- Wang, J.; Cheng, H.; Xu, S.; Ji, B.; Long, X. Performance of cavitation flow and its induced noise of different jet pump cavitation reactors. Ultrason. Sonochem. 2019, 55, 322–331. [Google Scholar] [CrossRef]
- Kan, K.; Binama, M.; Chen, H.; Zheng, Y.; Zhou, D.; Su, W.; Muhirwa, A. Pump as turbine cavitation performance for both conventional and reverse operating modes: A review. Renew. Sustain. Energy Rev. 2022, 168, 112786. [Google Scholar] [CrossRef]
- Sentyabov, A.V.; Timoshevskiy, M.V.; Pervunin, K.S. Gap cavitation in the end clearance of a guide vane of a hydroturbine: Numerical and experimental investigation. J. Eng. Thermophys. 2019, 28, 67–83. [Google Scholar] [CrossRef]
- Kim, S.; Choi, C.; Kim, J.; Park, J.; Baek, J. Tip clearance effects on cavitation evolution and head breakdown in turbopump inducer. J. Propuls. Power 2013, 29, 1357–1366. [Google Scholar] [CrossRef]
- Tan, D.; Li, Y.; Wilkes, I.; Vagnoni, E.; Miorini, R.L.; Katz, J. Experimental investigation of the role of large scale cavitating vortical structures in performance breakdown of an axial waterjet pump. J. Fluids Eng. 2015, 137, 111301. [Google Scholar] [CrossRef]
- Al-Obaidi, A.R. Experimental Diagnostic of Cavitation Flow in the Centrifugal Pump under Various Impeller Speeds Based on Acoustic Analysis Method. Arch. Acoust. 2023, 48, 159–170. [Google Scholar]
- Zhao, M.; Zhao, W.; Wan, D. Numerical simulations of propeller cavitation flows based on OpenFOAM. J. Hydrodyn. 2020, 32, 1071–1079. [Google Scholar] [CrossRef]
- Al-Obaidi, A. Experimental and Numerical Investigations on the Cavitation Phenomenon in a Centrifugal Pump. Doctoral Dissertation, University of Huddersfield, Huddersfield, UK, 2018. [Google Scholar]
- Khalid, M.R.; Reza, K.; Ghorbani, S. Common Failures in Hydraulic Kaplan Turbine Blades and Practical Solutions. Materials 2023, 16, 3303. [Google Scholar] [CrossRef] [PubMed]
- Posa, A.; Lippolis, A. Effect of working conditions and diffuser setting angle on pressure fluctuations within a centrifugal pump. Int. J. Heat Fluid Flow 2019, 75, 44–60. [Google Scholar] [CrossRef]
- Li, J.; Zhang, Y.; Liu, K.; Xian, H.; Yu, J. Numerical simulation of hydraulic force on the impeller of reversible pump turbines in generating mode. J. Hydrodyn. Ser B 2017, 29, 603–609. [Google Scholar] [CrossRef]
- Thomas, H.J. Unstable natural vibration of turbine rotors induced by the clearance flow in glands and blading. Bull l’AIM 1958, 71, 1039–1063. [Google Scholar]
- Ehrich, F. Rotor whirl forces induced by the tip clearance effect in axial flow compressors. In Proceedings of the International Design Engineering Technical Conferences and Computers and Information in Engineering Conference, Albuquerque, NM, USA, 19–22 September 1993; American Society of Mechanical Engineers: New York, NY, USA, 2021; Volume 6395, pp. 7–17. [Google Scholar]
- Jung, Y.; Choi, M.; Oh, S.; Baek, J. Effects of a nonuniform tip clearance profile on the performance and flow field in a centrifugal compressor. Int. J. Rotating Mach. 2012, 2012, 340439. [Google Scholar] [CrossRef]
- Strelets, M. Detached eddy simulation of massively separated flows. In Proceedings of the 39th Aerospace Sciences Meeting and Exhibit, Reno, NV, USA, 8–11 January 2001; p. 879. [Google Scholar]
- Patankar, S. Numerical Heat Transfer and Fluid Flow; Taylor & Francis: Abingdon, UK, 2018. [Google Scholar]
- Piomelli, U. Large-eddy simulation-Present state and future perspectives. In Proceedings of the 36th AIAA Aerospace Sciences Meeting and Exhibit, Reno, NV, USA, 12–15 January 1998; p. 534. [Google Scholar]
- Durbin, P.A. Some recent developments in turbulence closure modeling. Annu. Rev. Fluid Mech. 2018, 50, 77–103. [Google Scholar] [CrossRef]
- Dragomiretskiy, K.; Zosso, D. Variational mode decomposition. IEEE Trans. Signal Process. 2013, 62, 531–544. [Google Scholar] [CrossRef]
- Huang, N.E.; Shen, Z.; Long, S.R.; Wu, M.C.; Shih, H.H.; Zheng, Q.; Yen, N.C.; Tung, C.C.; Liu, H.H. The empirical mode decomposition and the Hilbert spectrum for nonlinear and non-stationary time series analysis. Proc. R. Soc. Lond. Ser. A Math. Phys. Eng. Sci. 1998, 454, 903–995. [Google Scholar] [CrossRef]
- Yan, H.; Bai, H.; Zhan, X.; Wu, Z.; Wen, L.; Jia, X. Combination of VMD Mapping MFCC and LSTM: A New Acoustic Fault Diagnosis Method of Diesel Engine. Sensors 2022, 22, 8325. [Google Scholar] [CrossRef]
- Xiao, Q.; Li, J.; Sun, J.; Feng, H.; Jin, S. Natural-gas pipeline leak location using variational mode decomposition analysis and cross-time–frequency spectrum. Measurement 2018, 124, 163–172. [Google Scholar] [CrossRef]
- Kan, K.; Zhang, Q.; Xu, Z.; Zheng, Y.; Gao, Q.; Shen, L. Energy loss mechanism due to tip leakage flow of axial flow pump as turbine under various operating conditions. Energy 2022, 255, 124532. [Google Scholar] [CrossRef]
- Zierke, W.C.; Straka, W.A.; Taylor, P.D. An experimental investigation of the flow through an axial-flow pump. J. Fluids Eng. 1995, 117, 485–490. [Google Scholar] [CrossRef]
- Kundu, P.; Sarkar, A.; Nagarajan, V. Improvement of Performance of S1210 Hydrofoil with Vortex Generators and Modified Trailing Edge. Renew. Energy 2019, 142, 643–657. [Google Scholar] [CrossRef]
- Lu, Z.; Li, N.; Tao, R.; Yao, Z.; Liu, W.; Xiao, R. Influence of eccentric impeller on pressure pulsation of large-scale vaned-voluted centrifugal pump. Proc. Inst. Mech. Eng. Part A J. Power Energy 2023, 237, 591–601. [Google Scholar] [CrossRef]







| Parameter | Notation | Value |
|---|---|---|
| Rotation speed (r/min) | n | 260 |
| Impeller tip diameter (m) | Dtip | 1.058 |
| Tip clearance width (mm) | htc | 3.30 |
| Impeller blade number | Ni | 7 |
| Vane blade number | Nv | 13 |
| Rated flow rate (m3/s) | Qr | 7095 |
| Rated head | Hr | 2.39 |
| Rated efficiency | ηr | 0.758 |
| Component | Mesh Node Number |
|---|---|
| Inlet section | 250,760 |
| Vanes | 985,906 |
| Impeller | 919,472 |
| Outlet section | 173,600 |
| Total | 2,329,738 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, H.; Guan, Y.; Hu, Z.; Guang, W.; Zhu, D.; Tao, R.; Xiao, R. Analysis and Identification of Eccentricity of Axial-Flow Impeller by Variational Mode Decomposition. Water 2024, 16, 2605. https://doi.org/10.3390/w16182605
Zhang H, Guan Y, Hu Z, Guang W, Zhu D, Tao R, Xiao R. Analysis and Identification of Eccentricity of Axial-Flow Impeller by Variational Mode Decomposition. Water. 2024; 16(18):2605. https://doi.org/10.3390/w16182605
Chicago/Turabian StyleZhang, Houyu, Yingbo Guan, Zilong Hu, Weilong Guang, Di Zhu, Ran Tao, and Ruofu Xiao. 2024. "Analysis and Identification of Eccentricity of Axial-Flow Impeller by Variational Mode Decomposition" Water 16, no. 18: 2605. https://doi.org/10.3390/w16182605
APA StyleZhang, H., Guan, Y., Hu, Z., Guang, W., Zhu, D., Tao, R., & Xiao, R. (2024). Analysis and Identification of Eccentricity of Axial-Flow Impeller by Variational Mode Decomposition. Water, 16(18), 2605. https://doi.org/10.3390/w16182605








