Energy Loss and Noise Reduction of Centrifugal Pump Based on Bionic V-Groove Geometry
Abstract
1. Introduction
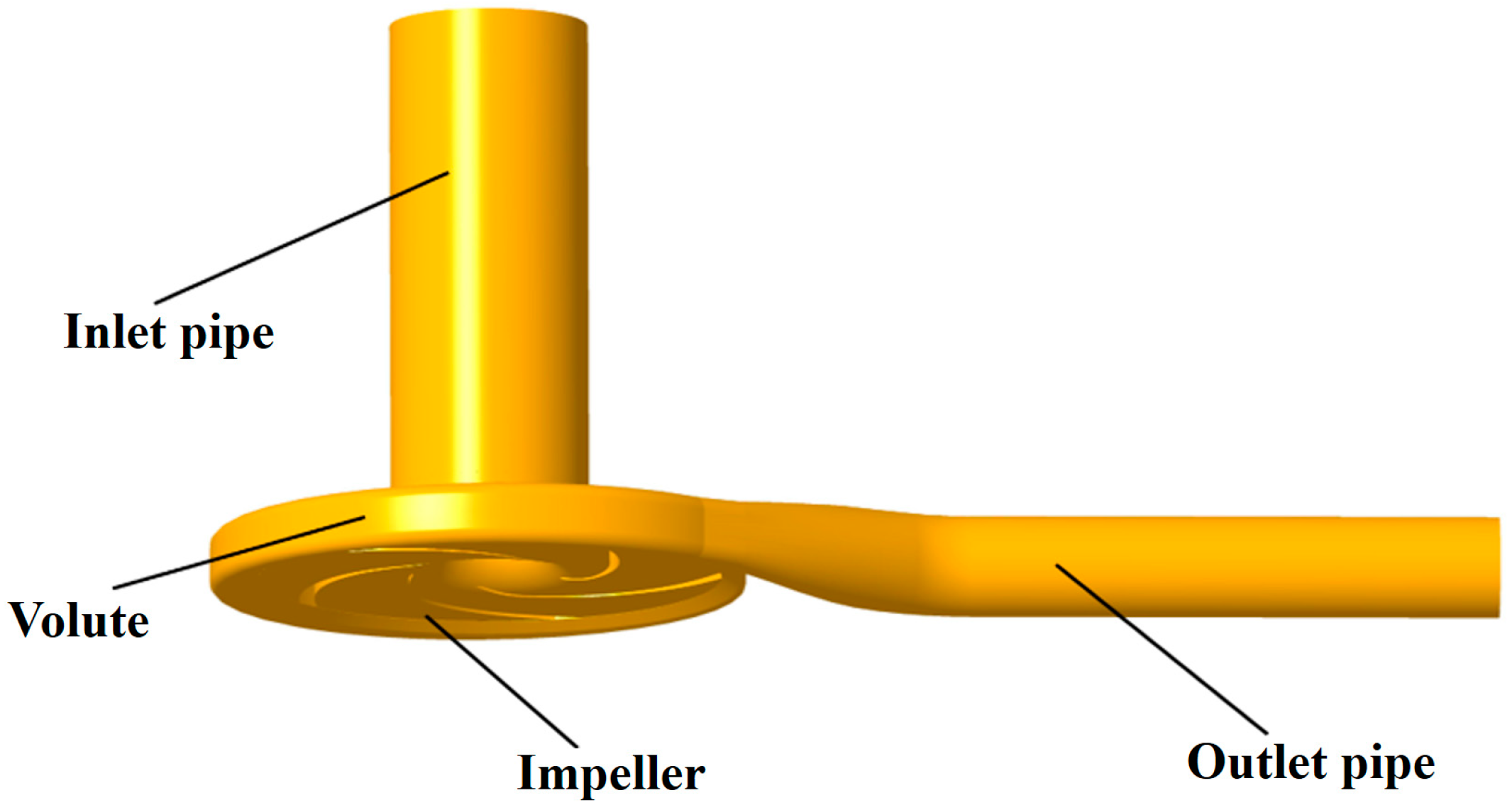
2. Research Model and Numerical Simulation Strategies
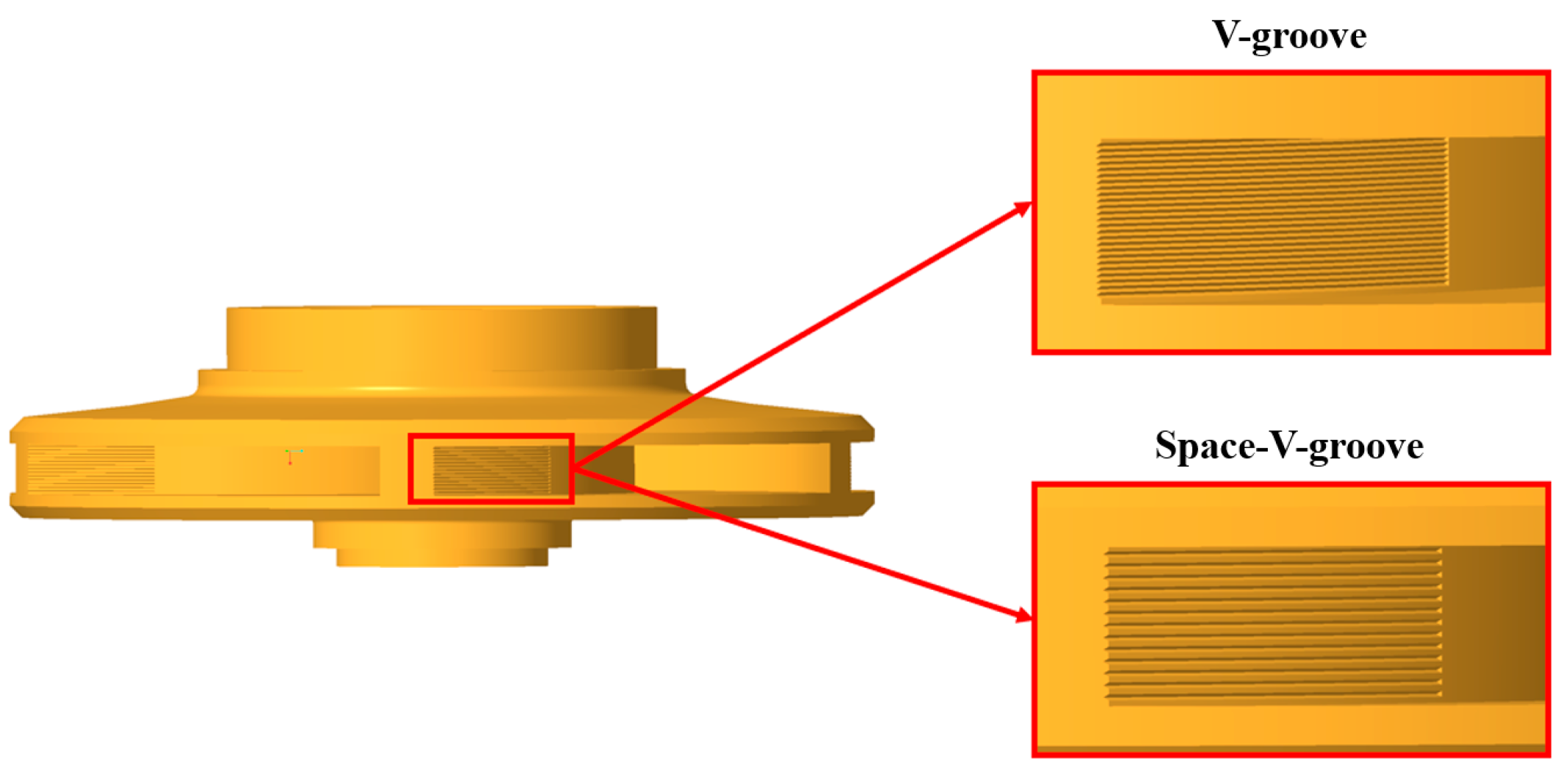
2.1. Bionic Groove Design
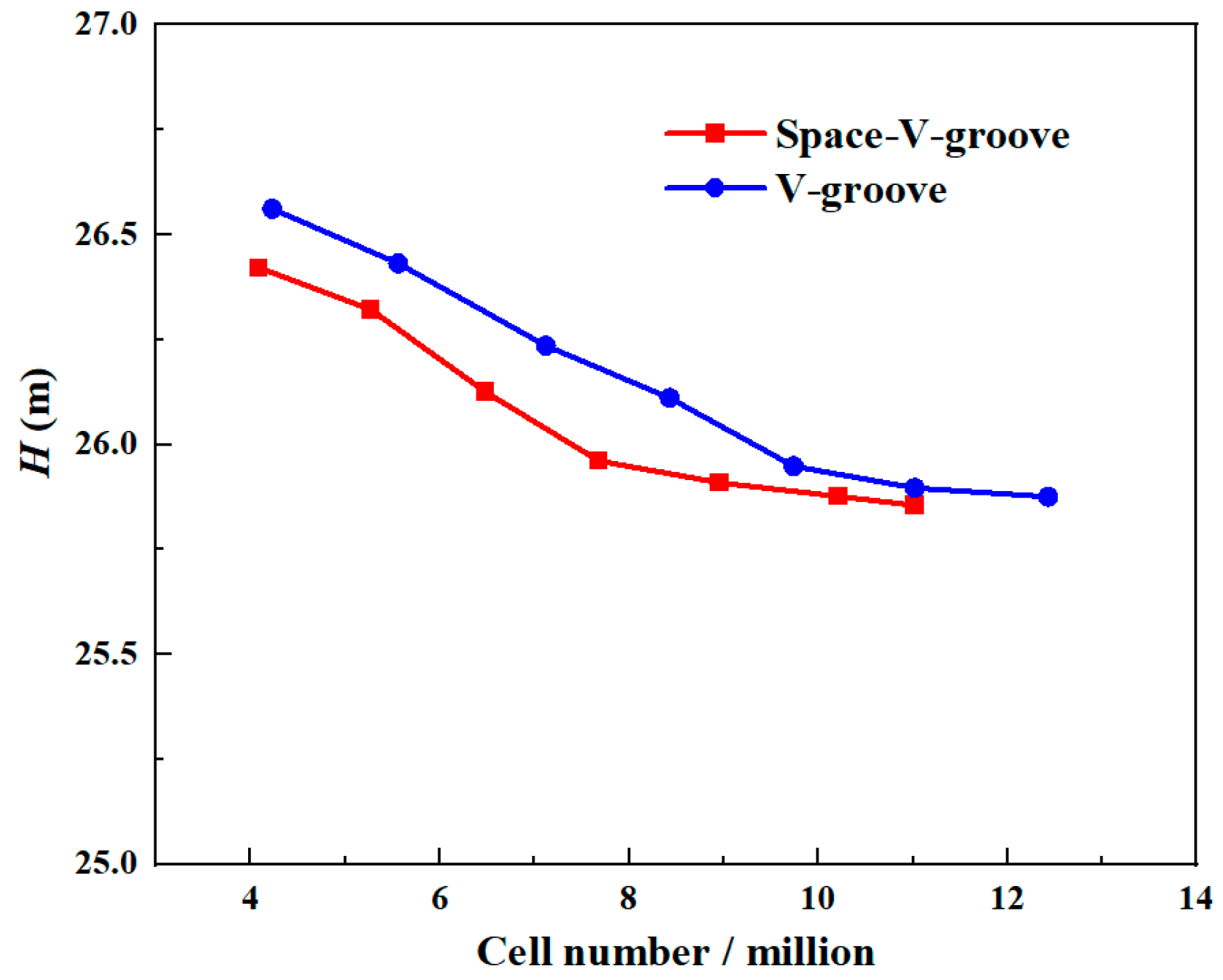
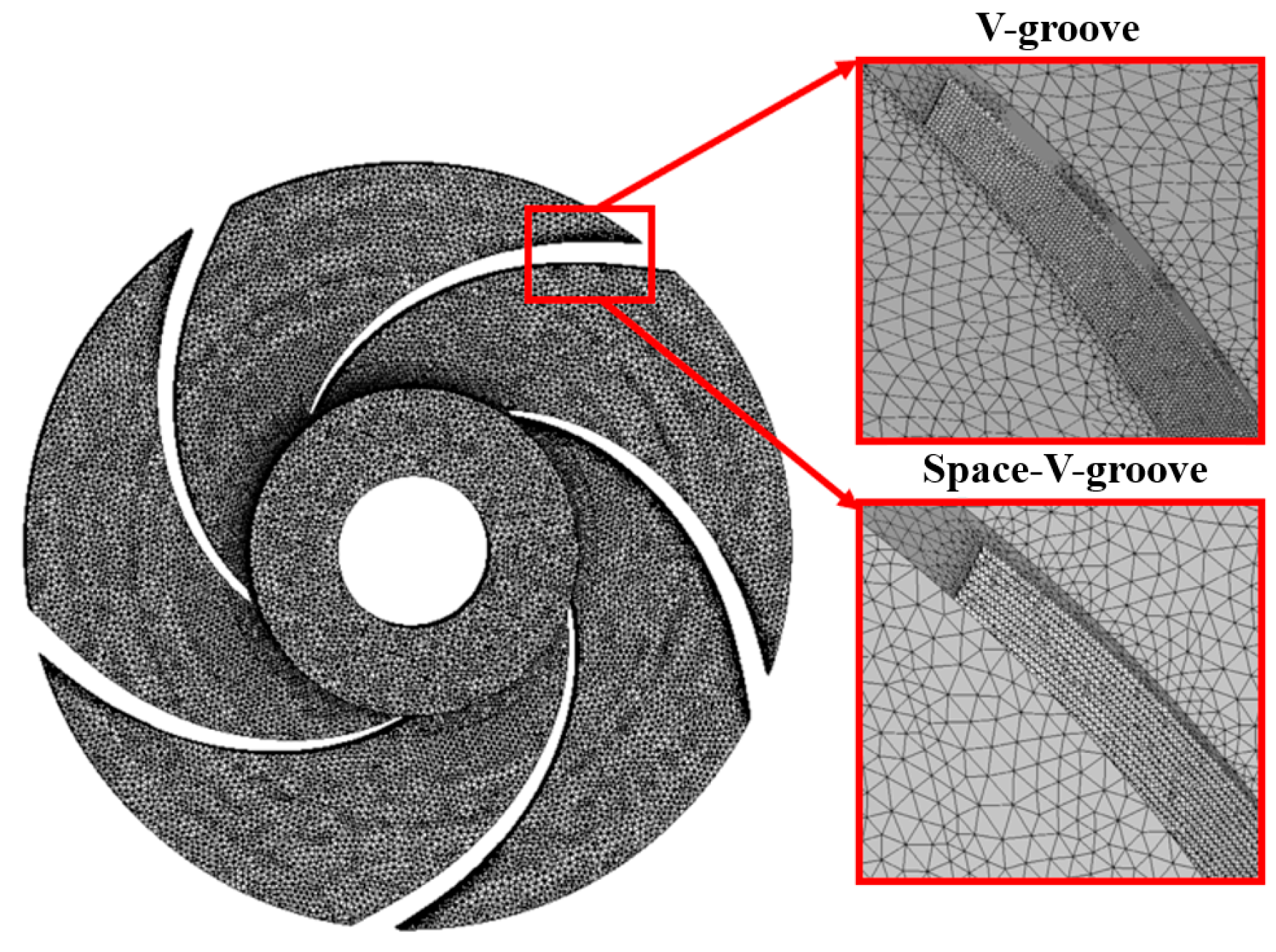
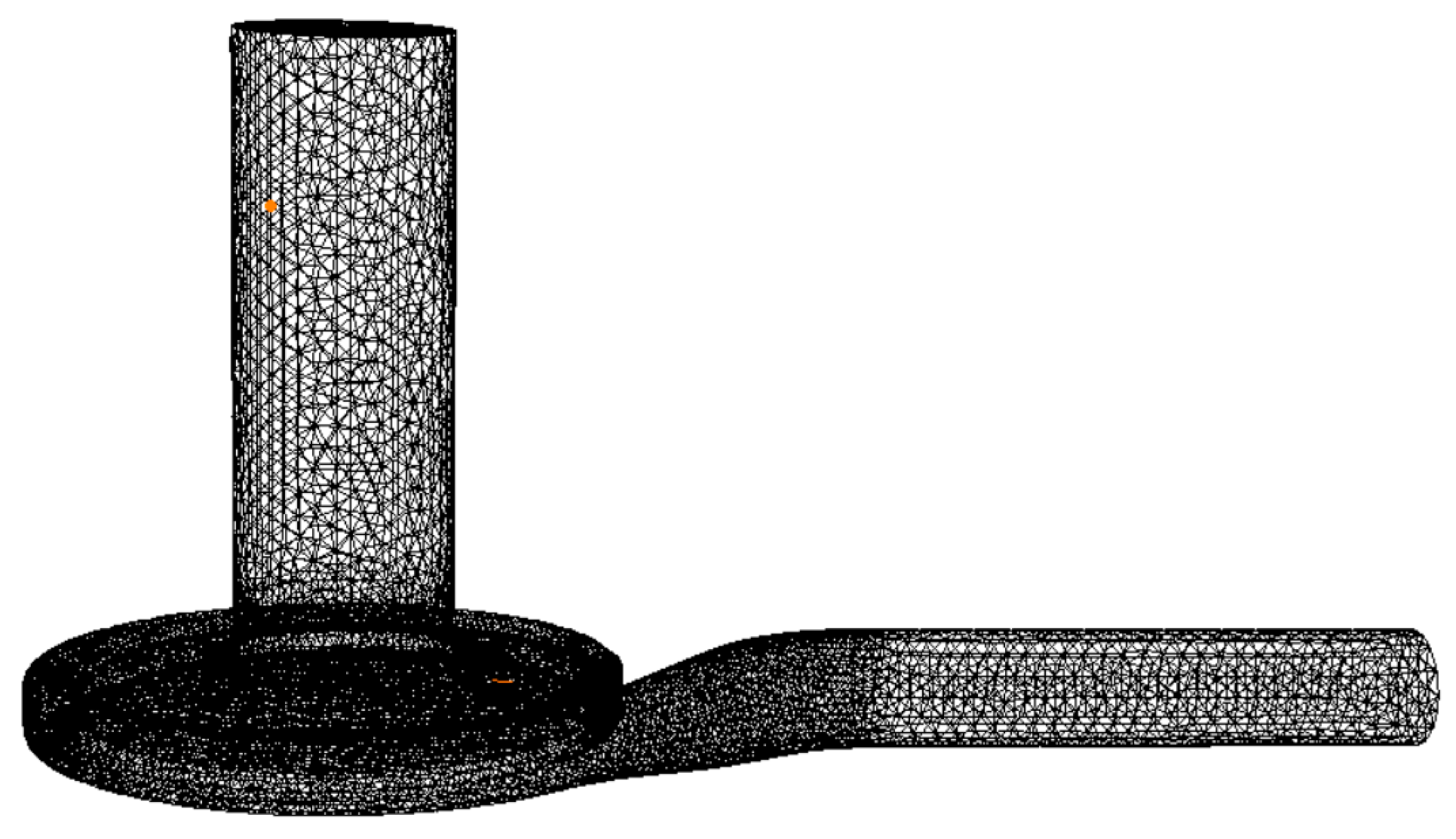
2.2. Grid Generation
2.2.1. Computational Fluid Dynamics Grid
2.2.2. Computational Aeroacoustics Grid
2.3. Boundary Conditions
2.3.1. Flow Field Boundary Conditions
2.3.2. Acoustic Boundary Conditions
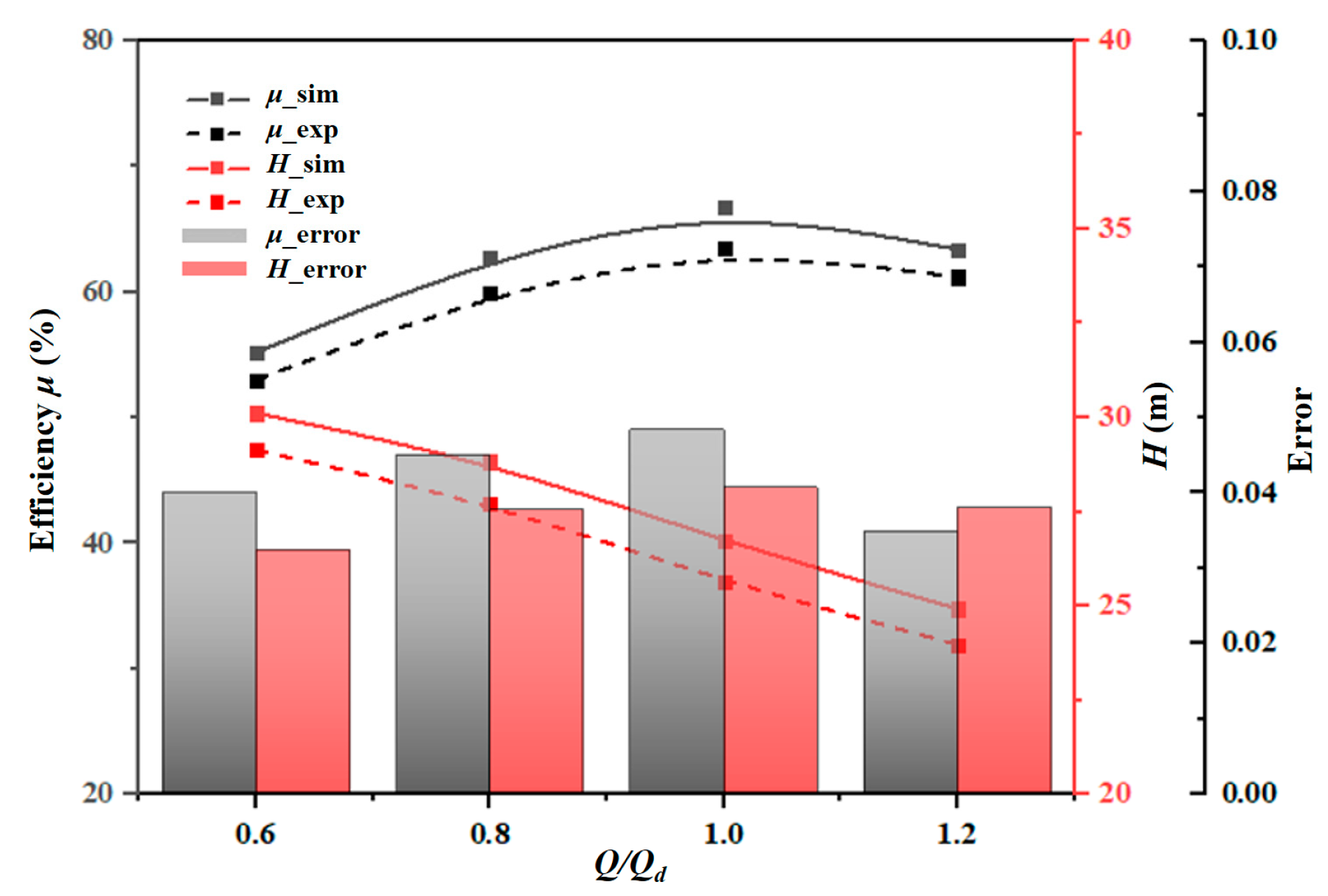
2.4. Test Verification
3. Results and Discussion
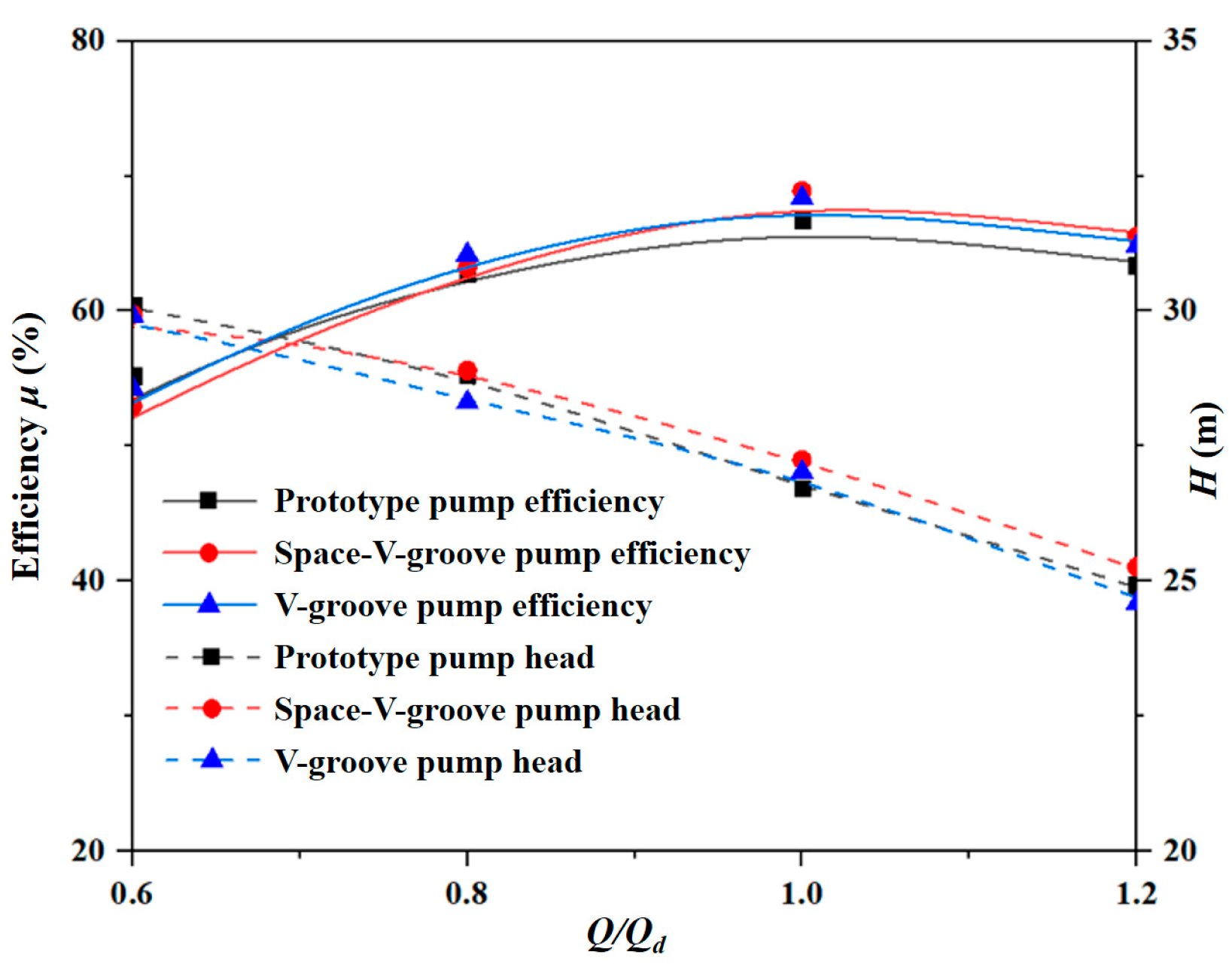
3.1. Impact of Bionic Grooved Blades on Hydraulic Performance
3.2. Analysis of Bionic Groove Blades on Drag Reduction
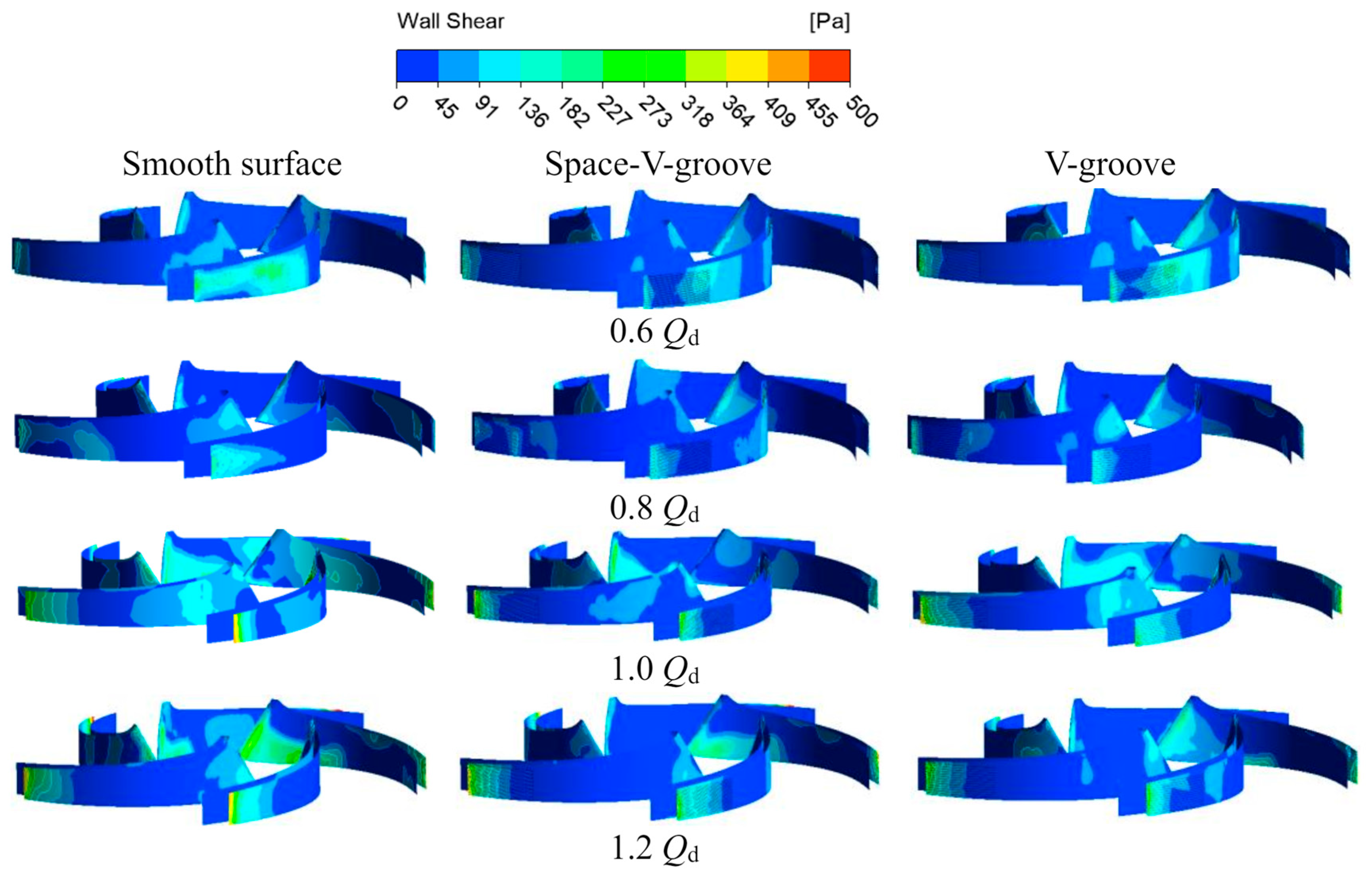
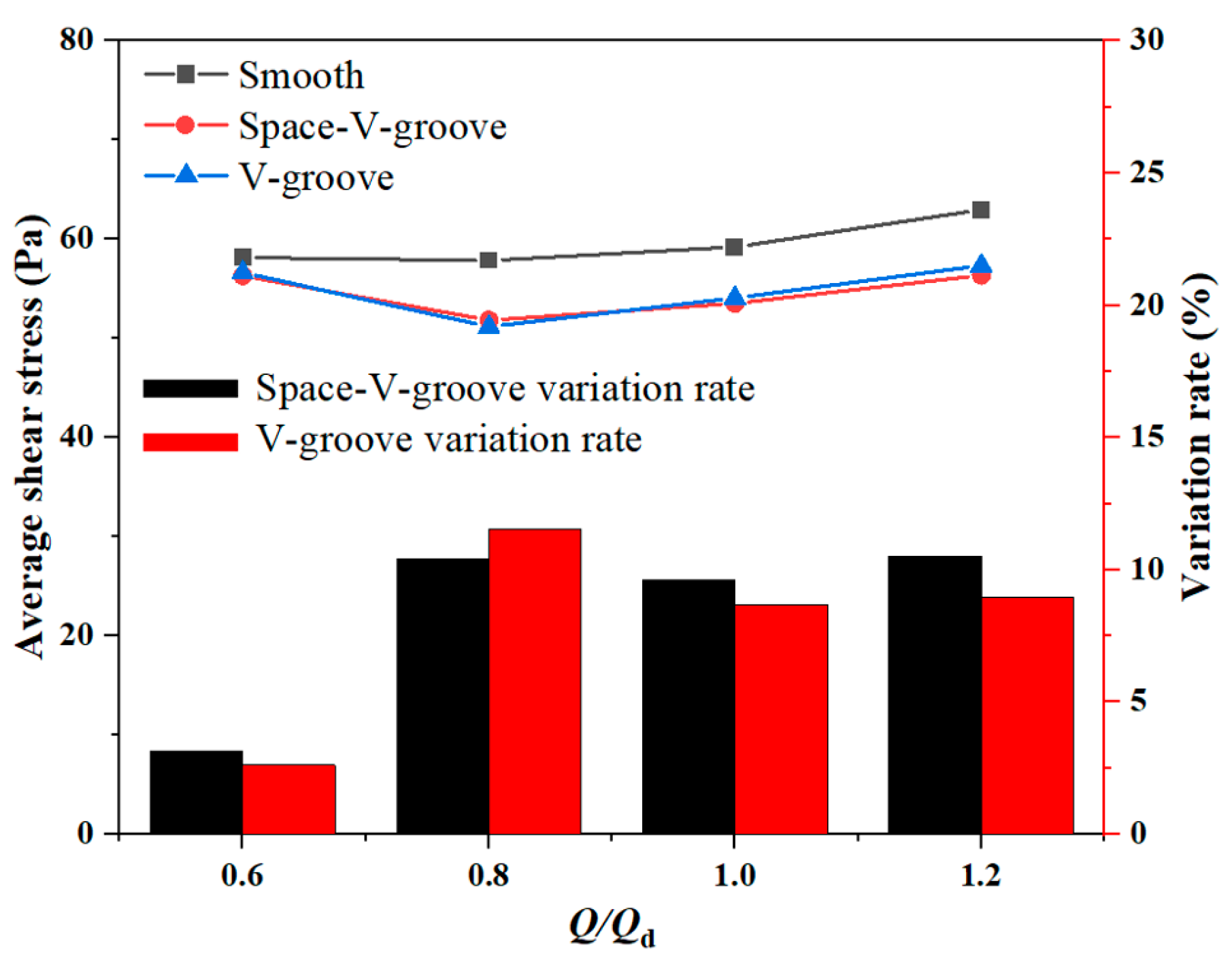
3.2.1. Wall Shear Stress Analysis
3.2.2. Blade Surface Velocity Analysis
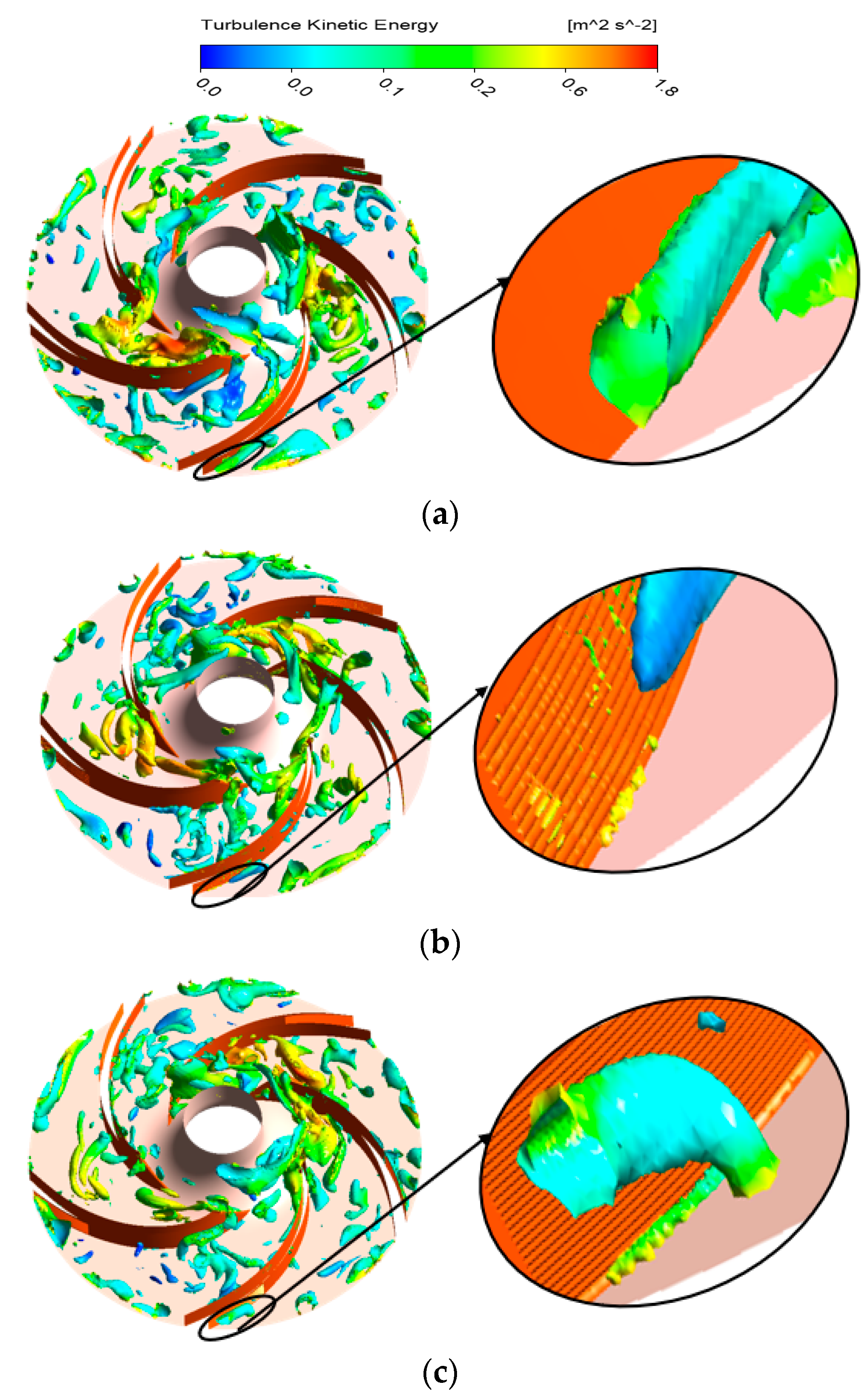
3.3. Vortex Core Analysis
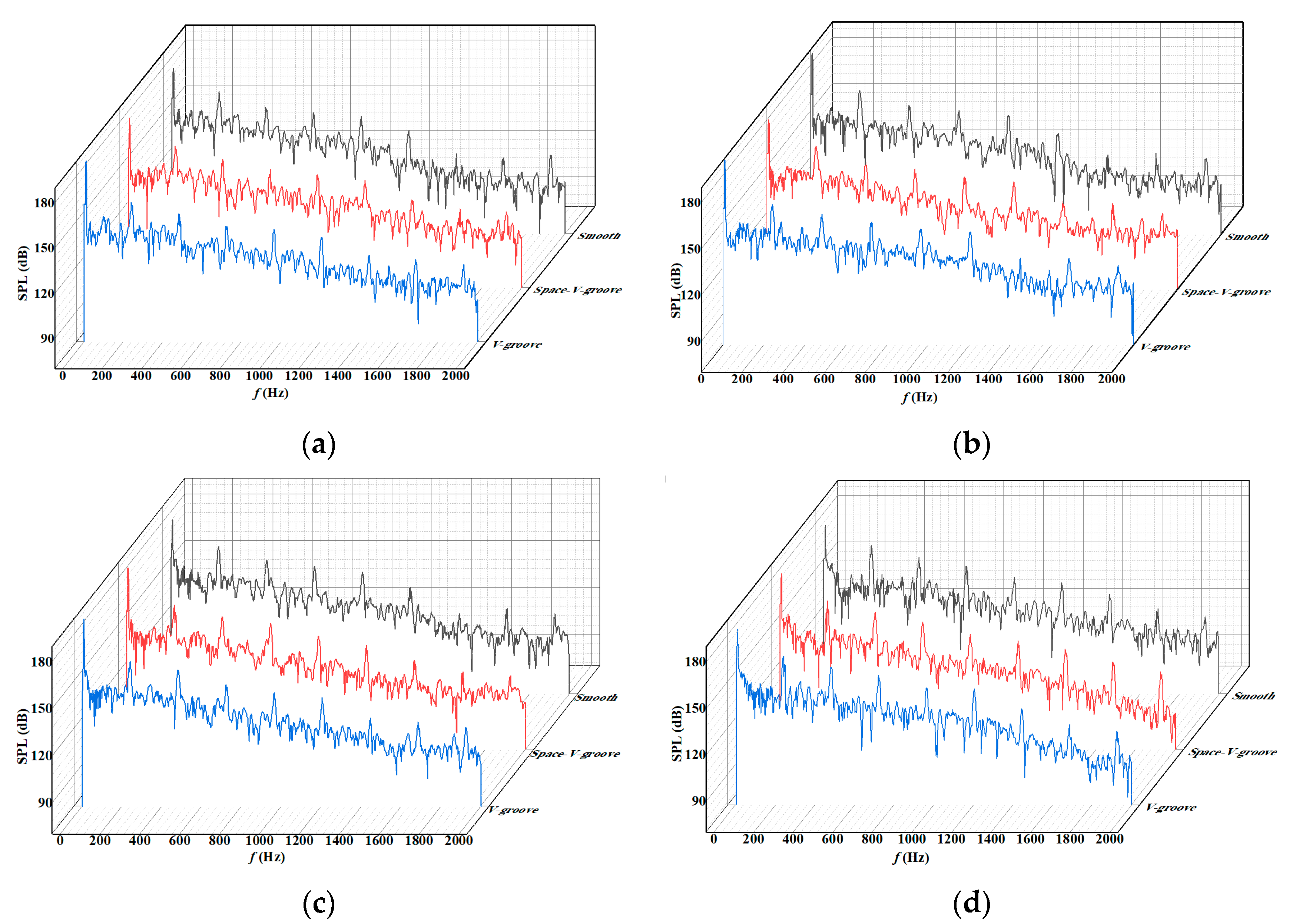
3.4. Flow Excitation Noise Analysis
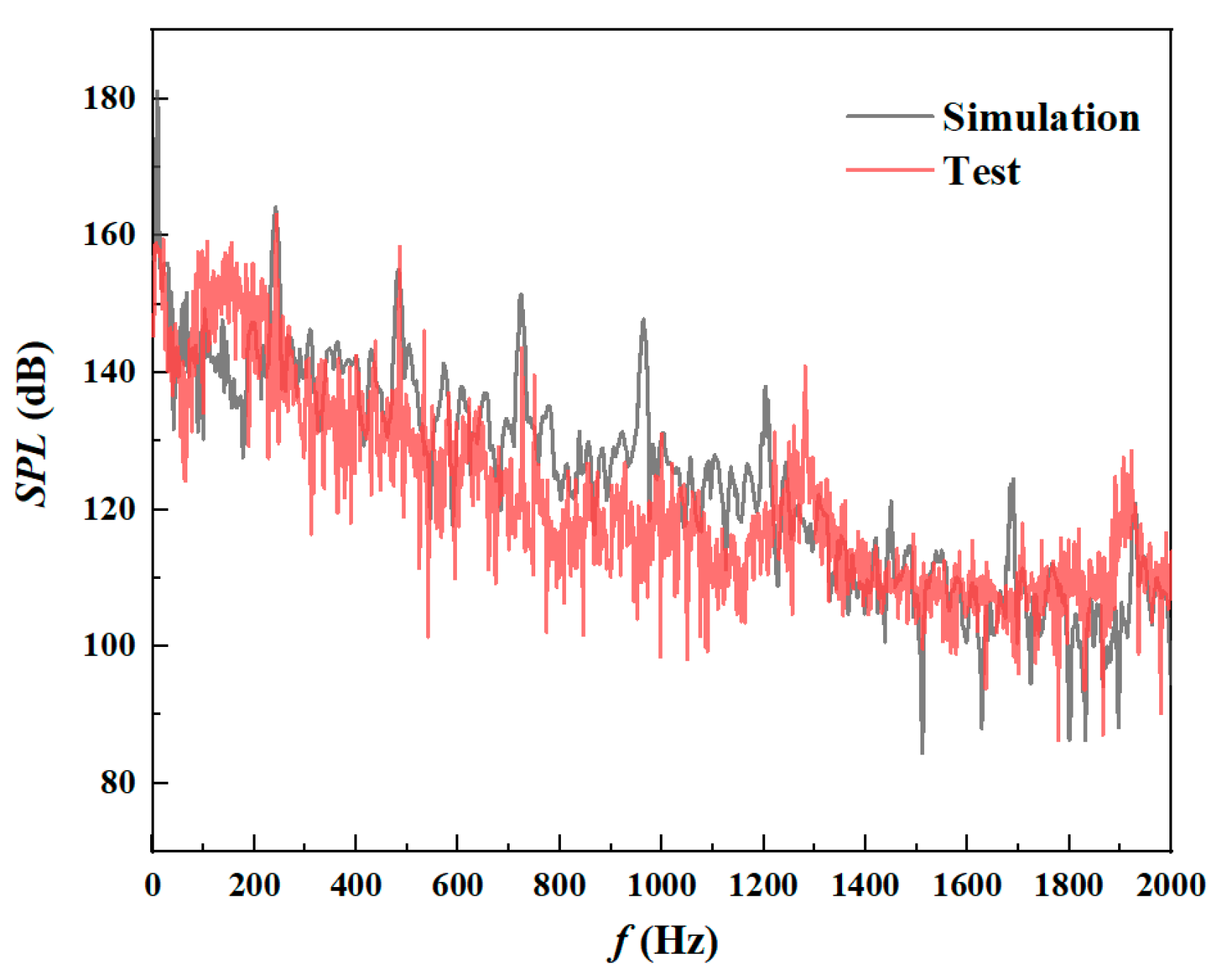
3.4.1. Acoustic Power Analysis
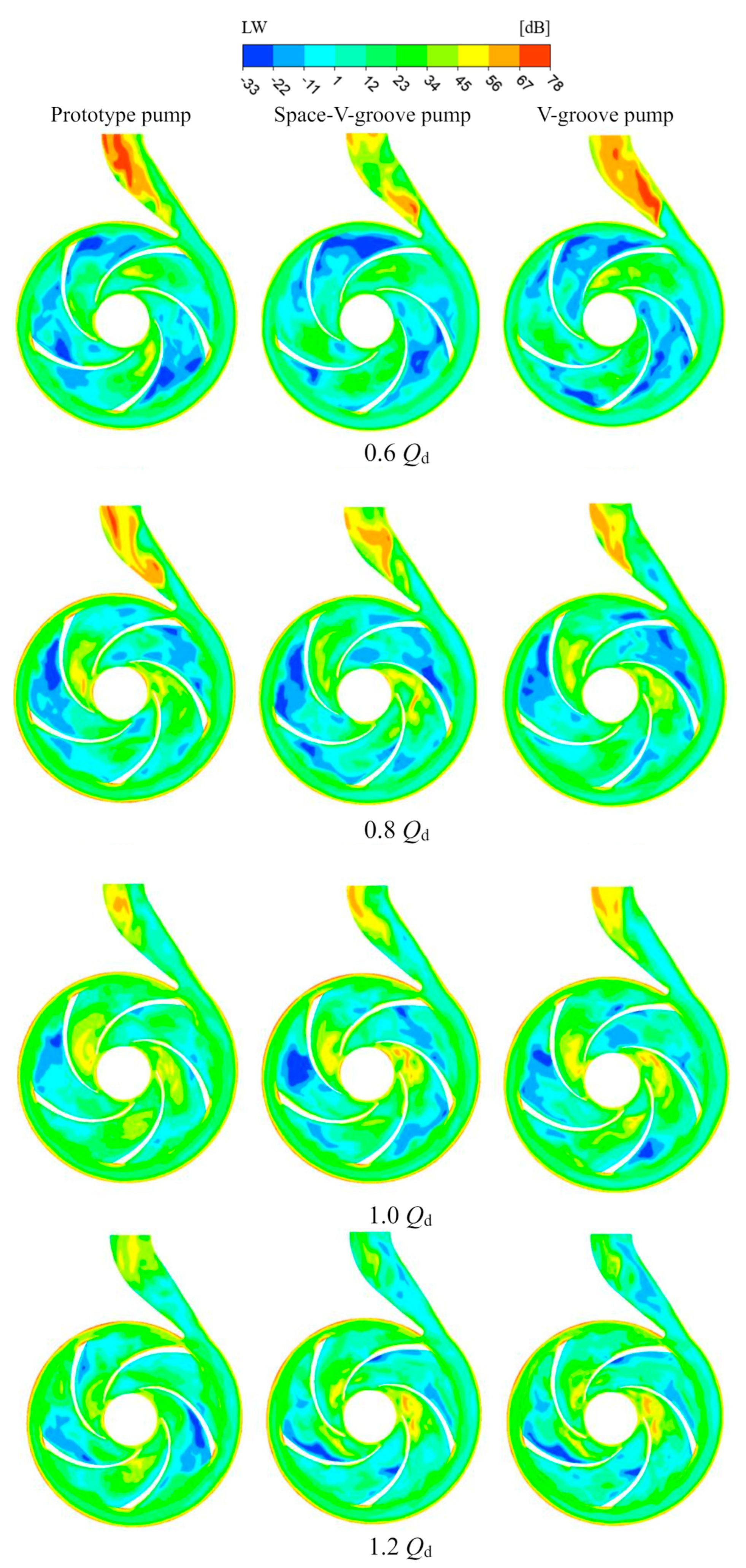
3.4.2. Internal Field Noise Analysis
4. Conclusions
- (1)
- The bionic groove geometry can effectively enhance hydraulic performance. At rated operating conditions, the two bionic groove pumps show the most significant improvement in hydraulic performance, with the Space-V-groove increasing head by 0.66 m and efficiency by 3.30%, and the V-groove improving head by 0.23 m and efficiency by 2.49%. Space-V-groove geometry is better than V-groove in improving hydraulic performance.
- (2)
- The Space-V-groove has better drag reduction than the V-groove. At rated operating conditions, the drag reduction was 2.86% for the Space-V-groove pump and 1.82% for the V-groove pump. The bionic groove geometry enables the obstruction of the high-speed flow near the wall and reduces the Reynolds stress at the blade outlet.
- (3)
- The bionic groove geometry can destroy the vortex shedding and trailing vortex, which can effectively control the turbulence and prevent the vortex core inside the impeller channel. Meanwhile, the bionic groove geometry can destroy the vortex core on the blade surface and decrease the energy inside the impeller, thereby reducing the acoustic power.
- (4)
- The bionic groove geometry can reduce the total sound pressure level by about 0.71% to 1.36% under different operating conditions. Particularly at rated operating conditions, the bionic groove geometry reduces the total sound pressure level the most, both by 1.36% and 1.2%, respectively. Meanwhile, Space-V-groove is more effective in reducing internal field noise.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Wang, C.; Shi, W.; Wang, X.; Jiang, X.; Yang, Y.; Li, W.; Zhou, L. Optimal design of multistage centrifugal pump based on the combined energy loss model and computational fluid dynamics. Appl. Energy 2017, 187, 10–26. [Google Scholar] [CrossRef]
- Lin, T.; Li, X.; Zhu, Z.; Xie, J.; Li, Y.; Yang, H. Application of enstrophy dissipation to analyze energy loss in a centrifugal pump as turbine. Renew. Energy 2021, 163, 41–55. [Google Scholar] [CrossRef]
- Choi, J.-S.; McLaughlin, D.K.; Thompson, D.E. Experiments on the unsteady flow field and noise generation in a centrifugal pump impeller. J. Sound Vib. 2003, 263, 493–514. [Google Scholar] [CrossRef]
- Langthjem, M.A.; Olhoff, N. A numerical study of flow-induced noise in a two-dimensional centrifugal pump. Part II. Hydroacoustics. J. Fluids Struct. 2004, 19, 369–386. [Google Scholar] [CrossRef]
- Wang, Y.; Ding, Z. Influence of blade number on flow-induced noise of centrifugal pump based on CFD/CA. Vacuum 2020, 172, 109058. [Google Scholar]
- Tian, L.M.; Ren, L.Q.; Liu, Q.P.; Han, Z.W.; Jiang, X. The mechanism of drag reduction around bodies of revolution using bionic non-smooth surfaces. J. Bionic Eng. 2007, 4, 109–116. [Google Scholar] [CrossRef]
- An, Y.; Wei, W.; Li, S.; Liu, C.; Meng, X.; Yan, Q. Research on the mechanism of drag reduction and efficiency improvement of hydraulic retarders with bionic non-smooth surface spoilers. Eng. Appl. Comput. Fluid Mech. 2020, 14, 447–461. [Google Scholar] [CrossRef]
- Lin, Y.; Li, X.; Zhu, Z.; Wang, X.; Lin, T.; Cao, H. An energy consumption improvement method for centrifugal pump based on bionic optimization of blade trailing edge. Energy 2022, 246, 123323. [Google Scholar] [CrossRef]
- Lin, Y.; Li, X.; Li, B.; Jia, X.-Q.; Zhu, Z. Influence of impeller sinusoidal tubercle trailing-edge on pressure pulsation in a centrifugal pump at nominal flow rate. J. Fluids Eng. 2021, 143, 091205. [Google Scholar] [CrossRef]
- Ma, L.; Gu, Y.; Xia, K.; Mou, J.; Wu, D.; Yan, M. Effect of bionic nonsmooth surface vane on the antiwear characteristics of double-vane pump. Appl. Bionics Biomech. 2022, 2022, 4442417. [Google Scholar] [CrossRef] [PubMed]
- Dai, C.; Guo, C.; Chen, Y.; Dong, L.; Liu, H. Analysis of the influence of different bionic structures on the noise reduction performance of the centrifugal pump. Sensors 2021, 21, 886. [Google Scholar] [CrossRef] [PubMed]
- Dai, C.; Guo, C.; Ge, Z.; Liu, H.; Dong, L. Study on drag and noise reduction of bionic blade of centrifugal pump and mechanism. J. Bionic Eng. 2021, 18, 428–440. [Google Scholar] [CrossRef]
- Tian, L.; Gao, Z.; Ren, L.; Han, Z.; Liao, G. The study of the efficiency enhancement of bionic coupling centrifugal pumps. J. Braz. Soc. Mech. Sci. Eng. 2013, 35, 517–524. [Google Scholar] [CrossRef]
- Tian, L.; Jin, E.; Li, Z.; Mei, H.; Wang, Y.; Shang, Y. The fluid control mechanism of bionic structural heterogeneous composite materials and its potential application in enhancing pump efficiency. Adv. Mech. Eng. 2015, 7, 1687814015619551. [Google Scholar] [CrossRef]
- Wang, B.; Chen, M.; Lu, Z.; Sun, J.; Wei, J. Optimized design of oil well pump plunger using bionic structure of decision system. Soft Comput. 2022, 26, 10827–10836. [Google Scholar] [CrossRef]
- Liu, H.; Wang, X.; Lu, Y.; Yan, Y.; Zhao, W.; Wu, X.; Zhang, Z. Application and optimal design of the bionic guide vane to improve the safety serve performances of the reactor coolant pump. Nucl. Eng. Technol. 2022, 54, 2491–2509. [Google Scholar] [CrossRef]
- Liu, H.; Lu, Y.; Wang, X.; Li, Y.; Yan, Y.; Lai, X. Investigation of the effects of the vane blades on the CAP1400 nuclear coolant pump’s performance based on a bionic strategy. Nucl. Eng. Des. 2021, 384, 111465. [Google Scholar] [CrossRef]
- Zhang, Z.; Dai, Y.; Gu, Y.; Shi, Z.; Mou, J. Effect of Bionic Groove Surface Blade on Cavitation Characteristics of Centrifugal Pump//Fluids Engineering Division Summer Meeting. Am. Soc. Mech. Eng. 2019, 59025, V001T01A041. [Google Scholar]
- Gu, Y.; Yu, L.; Mou, J.; Shi, Z.; Yan, M.; Wu, D. Influence of circular non-smooth structure on cavitation damage characteristics of centrifugal pump. J. Braz. Soc. Mech. Sci. Eng. 2022, 44, 155. [Google Scholar] [CrossRef]
- Walsh, M.J. Riblets as a viscous drag reduction technique. AIAA J. 1983, 21, 485–486. [Google Scholar] [CrossRef]
- Gu, Y.; Zhao, G.; Zheng, J.; Li, Z.; Liu, W.; Muhammad, F. Experimental and numerical investigation on drag reduction of non-smooth bionic jet surface. Ocean Eng. 2014, 81, 50–57. [Google Scholar] [CrossRef]
- Proudman, I. The generation of noise by isotropic turbulence. Proc. R. Soc. London. Ser. A Math. Phys. Sci. 1952, 214, 119–132. [Google Scholar]
- Sarkar, S.; Husseini, M.Y. Computation of the Sound Generated by Isotropic Turbulence; ICASE Technical Report; NASA Langley Research Center: Hampton, VA, USA, 1993; pp. 74–93. [Google Scholar]





| Components | Parameters | Symbols | Value |
|---|---|---|---|
| Impeller | Inlet diameter/mm | D1 | 60 |
| Outlet diameter/mm | D2 | 140 | |
| Outlet width/mm | b2 | 8 | |
| Blade wrap angle/° | φ | 100 | |
| Blade number | z | 5 | |
| Volute | Base circle diameter/mm | D3 | 150 |
| Inlet width/mm | b3 | 14 | |
| Outlet diameter/mm | Dd | 32 |
| Internal Field Noise | Frequency/Hz | |||
|---|---|---|---|---|
| APF (48.3) | BPF (241.7) | 2 BPF (483.4) | 3 BPF (725.1) | |
| Test/dB | 143.8 | 160.1 | 158.4 | 143.6 |
| Simulation/dB | 142.6 | 162.9 | 153.9 | 150.1 |
| Error | 0.83% | 1.10% | 2.84% | 4.53% |
| Q/Qd | Efficiency/% | Increased Efficiency/% | |||
|---|---|---|---|---|---|
| Prototype | Space-V | V | Space-V | V | |
| 0.6 | 55.23 | 54.25 | 54.32 | −1.72 | −1.65 |
| 0.8 | 62.82 | 63.45 | 64.22 | 1.04 | 2.23 |
| 1.0 | 66.74 | 68.91 | 68.40 | 3.30 | 2.49 |
| 1.2 | 63.42 | 65.54 | 64.81 | 3.38 | 2.19 |
| Q/Qd | Torque/N*m | DRR/% | |||
|---|---|---|---|---|---|
| Prototype | Space-V | V | Space-V | V | |
| 0.6 | 3.19 | 3.24 | 3.23 | −1.56 | −1.25 |
| 0.8 | 3.45 | 3.41 | 3.40 | 1.16 | 1.51 |
| 1.0 | 3.85 | 3.74 | 3.78 | 2.86 | 1.82 |
| 1.2 | 4.31 | 4.27 | 4.29 | 0.93 | 0.46 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, Y.; Dong, L.; Zhou, R.; Guo, C.; Dai, C. Energy Loss and Noise Reduction of Centrifugal Pump Based on Bionic V-Groove Geometry. Water 2024, 16, 2183. https://doi.org/10.3390/w16152183
Wang Y, Dong L, Zhou R, Guo C, Dai C. Energy Loss and Noise Reduction of Centrifugal Pump Based on Bionic V-Groove Geometry. Water. 2024; 16(15):2183. https://doi.org/10.3390/w16152183
Chicago/Turabian StyleWang, Yinchu, Liang Dong, Runze Zhou, Chao Guo, and Cui Dai. 2024. "Energy Loss and Noise Reduction of Centrifugal Pump Based on Bionic V-Groove Geometry" Water 16, no. 15: 2183. https://doi.org/10.3390/w16152183
APA StyleWang, Y., Dong, L., Zhou, R., Guo, C., & Dai, C. (2024). Energy Loss and Noise Reduction of Centrifugal Pump Based on Bionic V-Groove Geometry. Water, 16(15), 2183. https://doi.org/10.3390/w16152183






