Predicting Water Inflow in Tunnel Construction: A Fracture Network Model with Non-Darcy Flow Considerations
Abstract
1. Introduction
2. Governing Equation
2.1. Constitutive Model of Fracture
2.2. Mechanical Response Fractured Mass
2.3. Fluid Flow
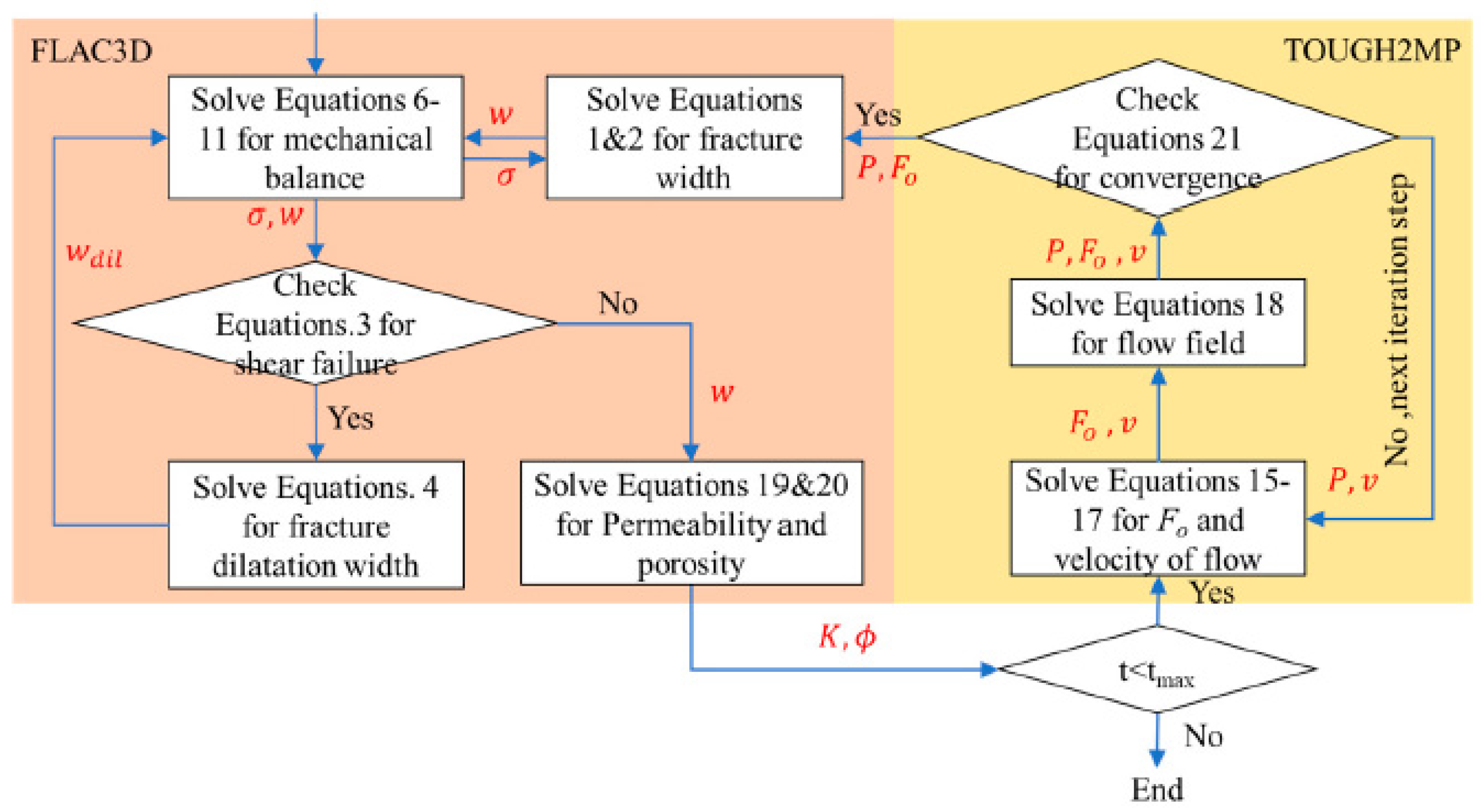
2.4. Hydro-Mechanical (HM) Coupling Framework
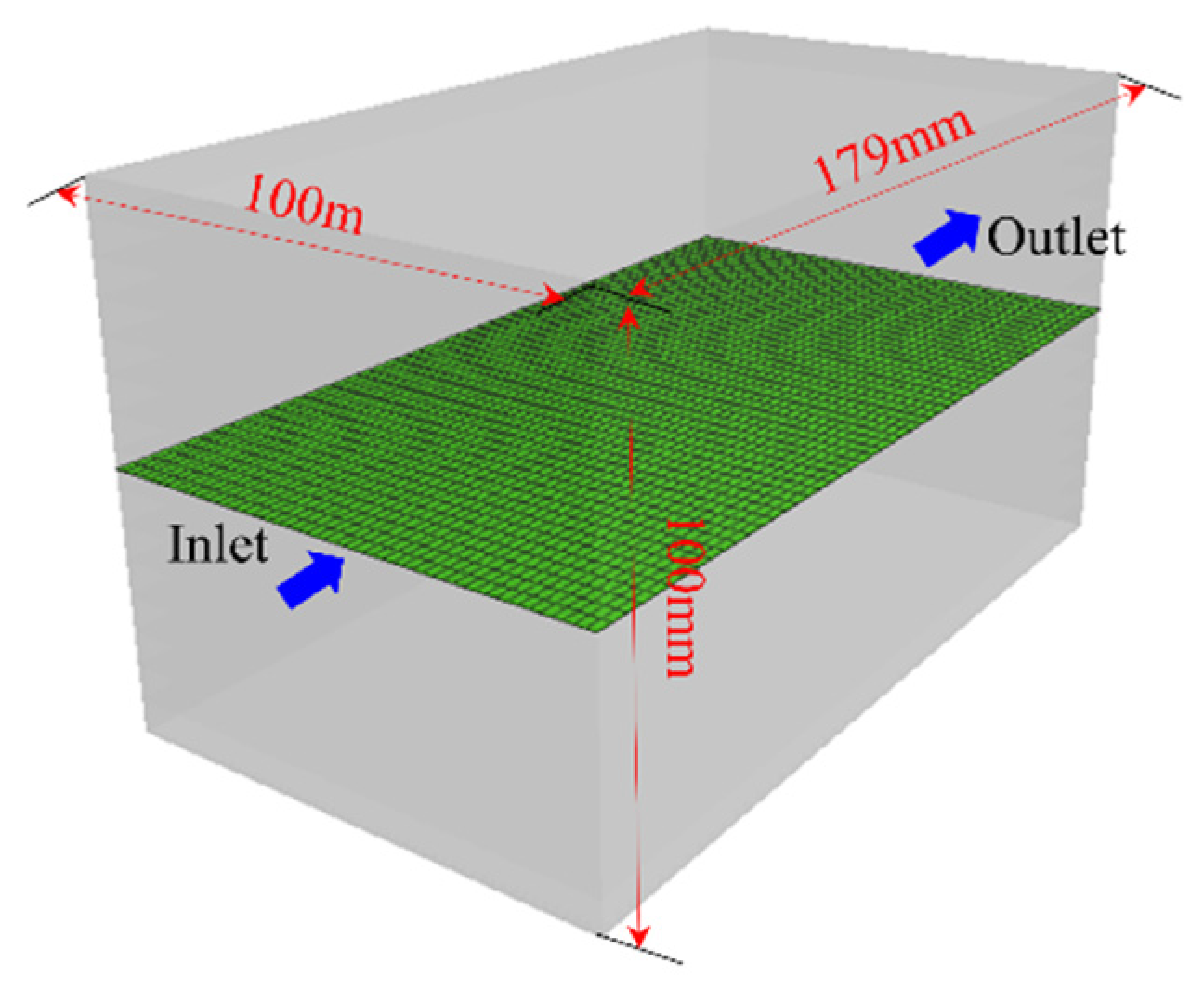
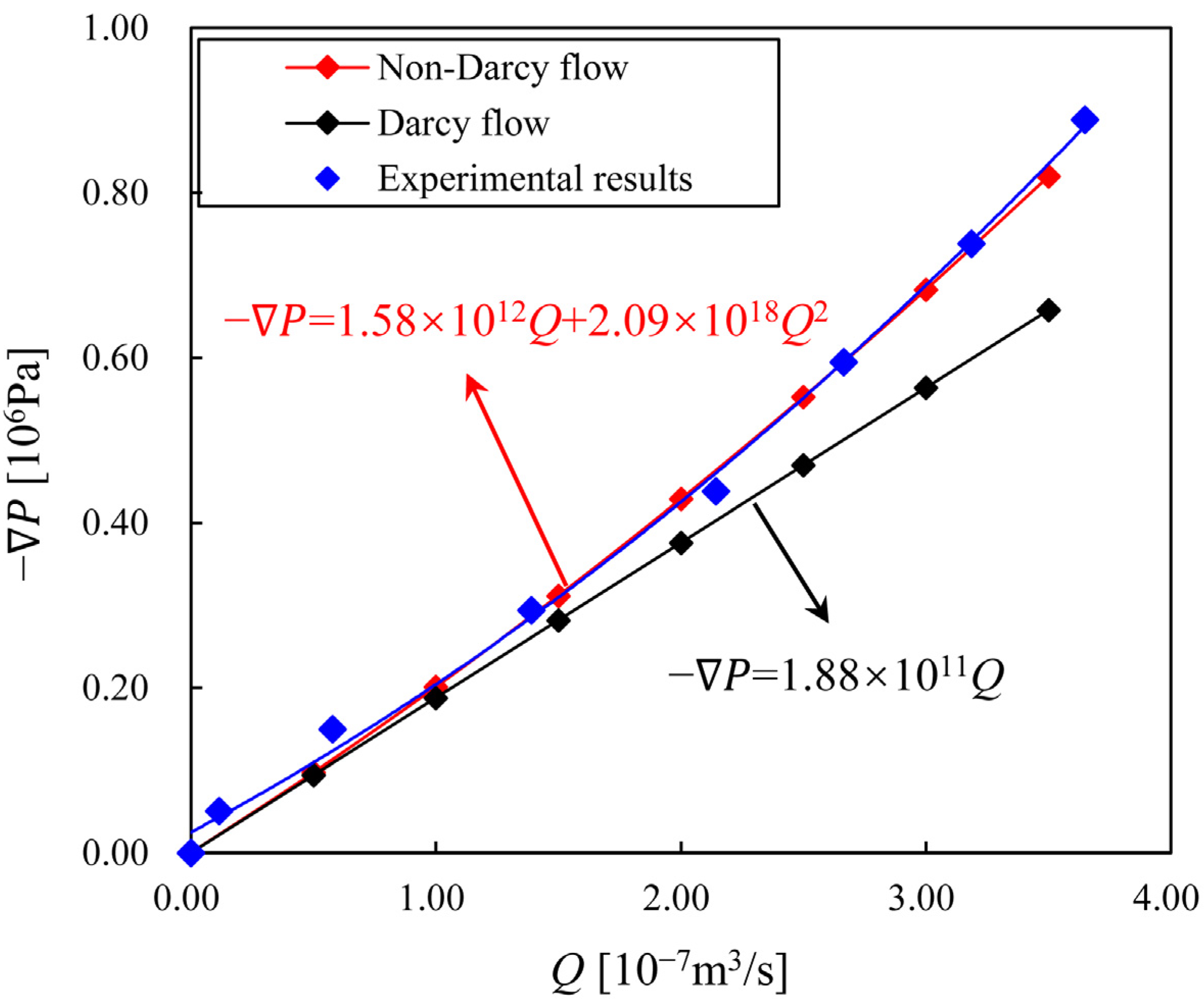
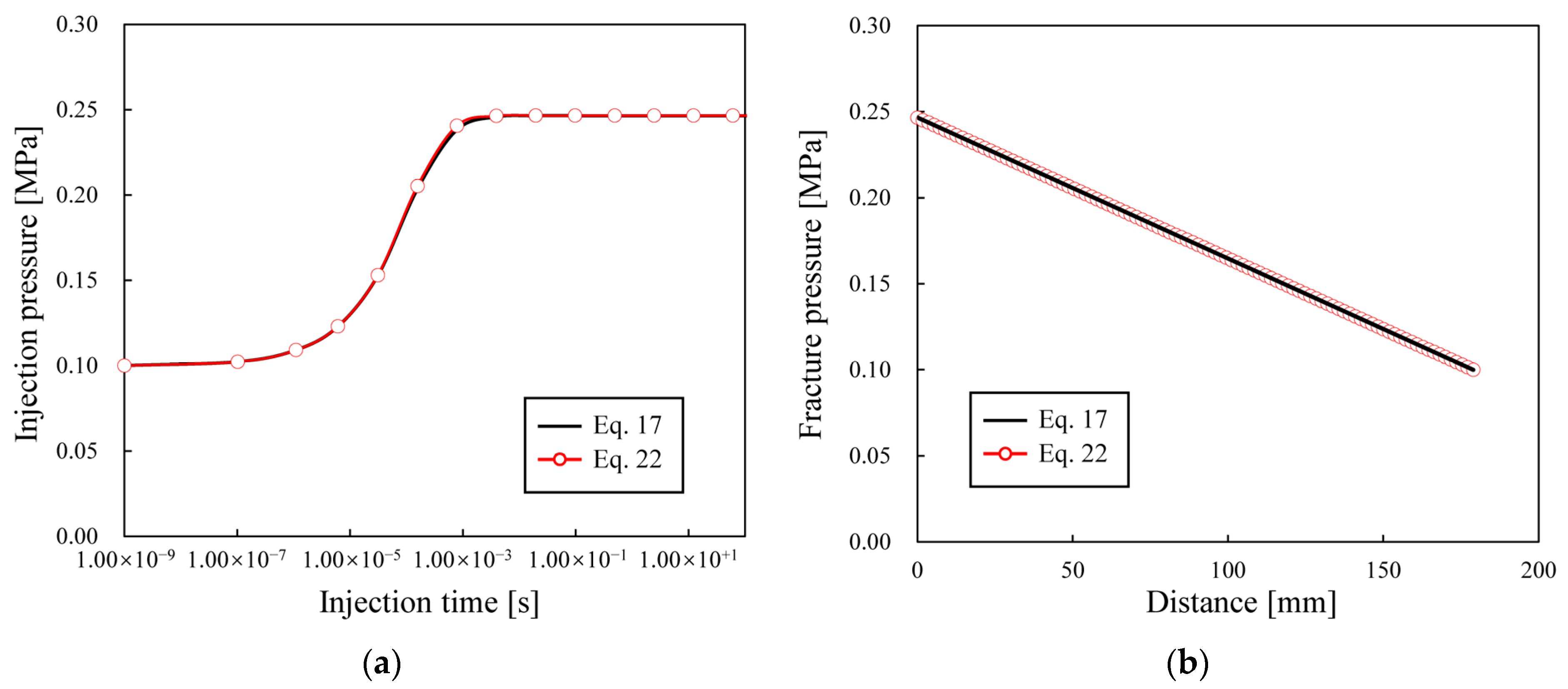
2.5. Model Validation
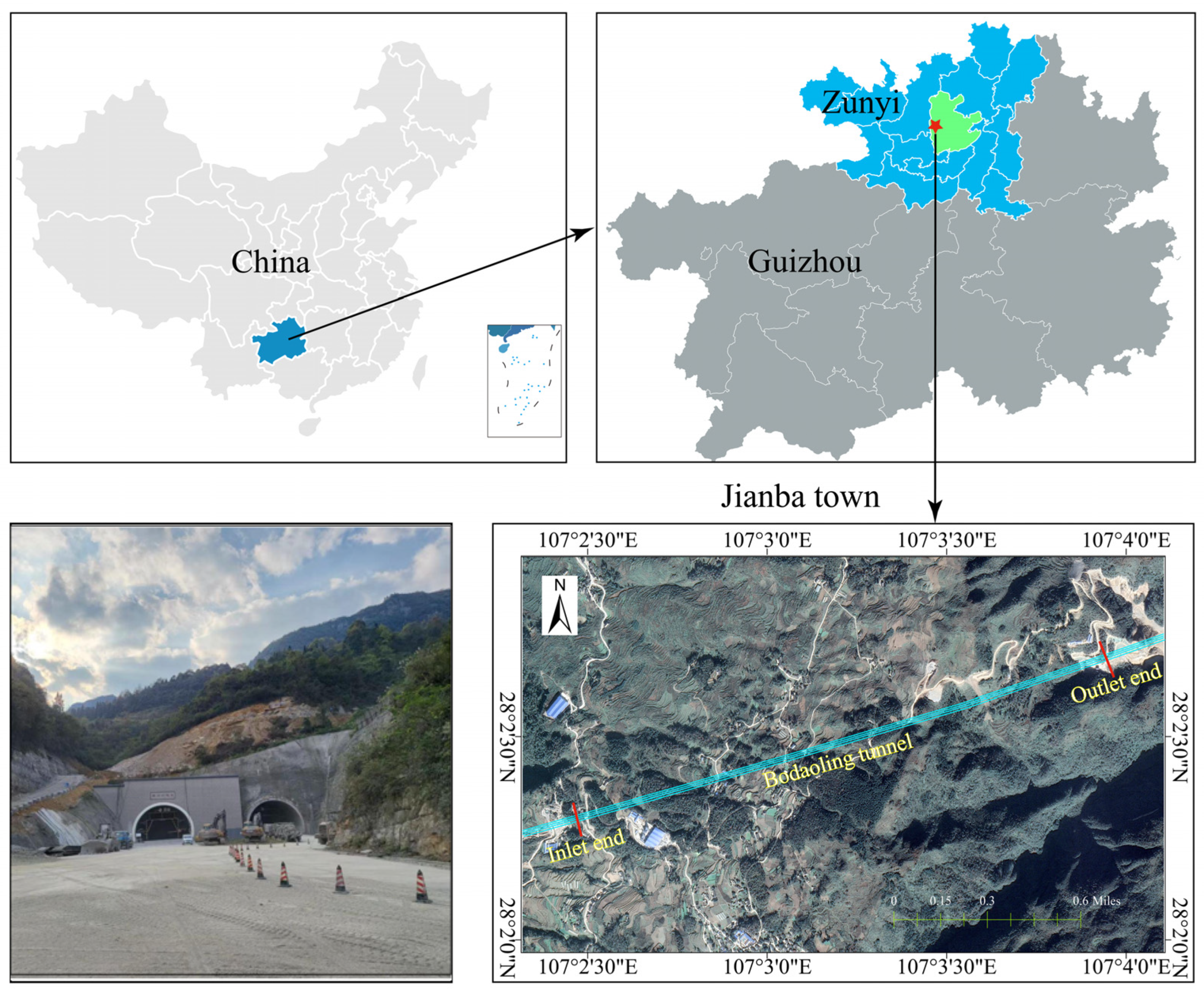
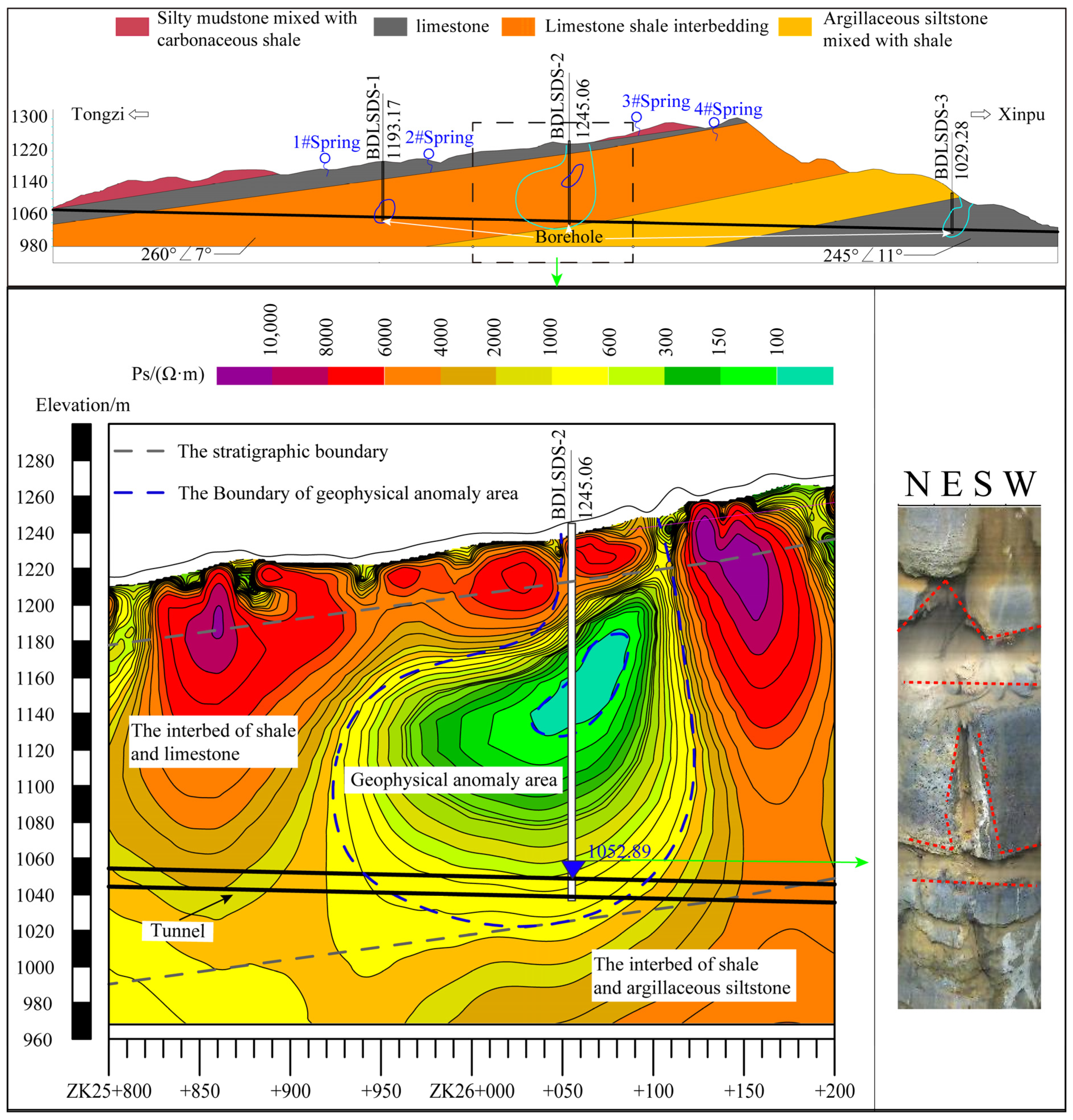
3. Engineering Geology
4. Numerical Model
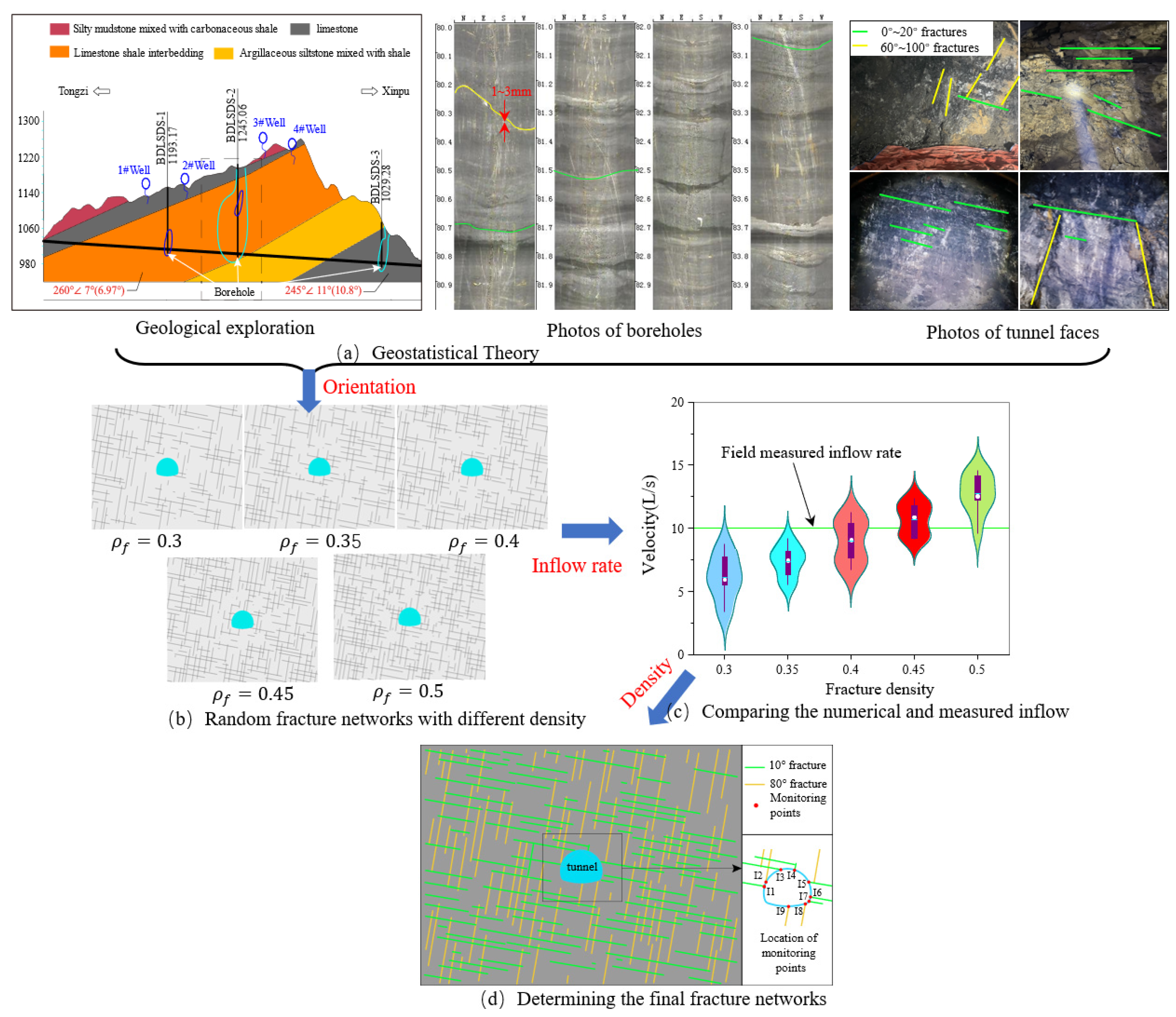
4.1. Determination of Fracture Networks
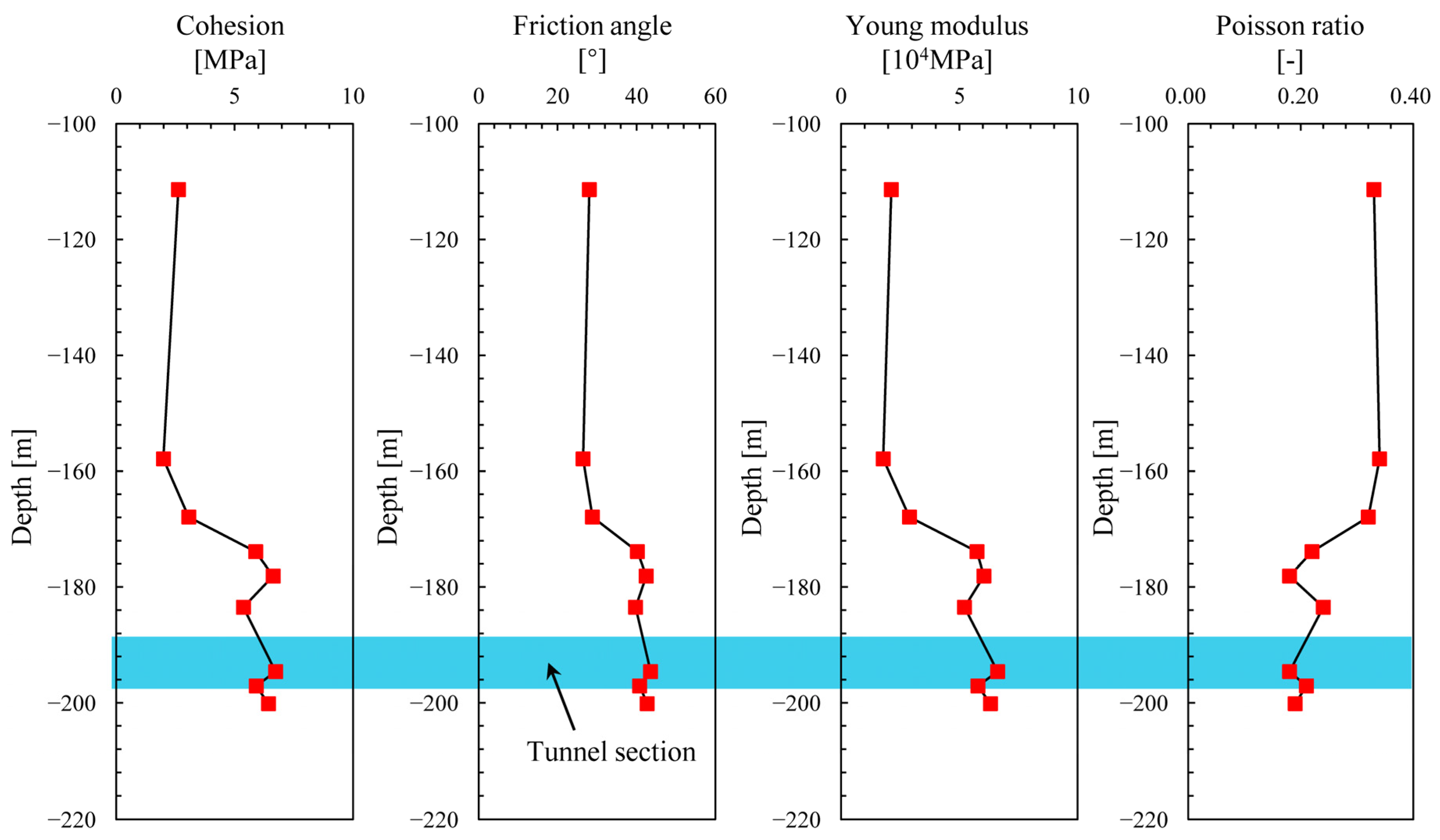
4.2. Parameter Determination
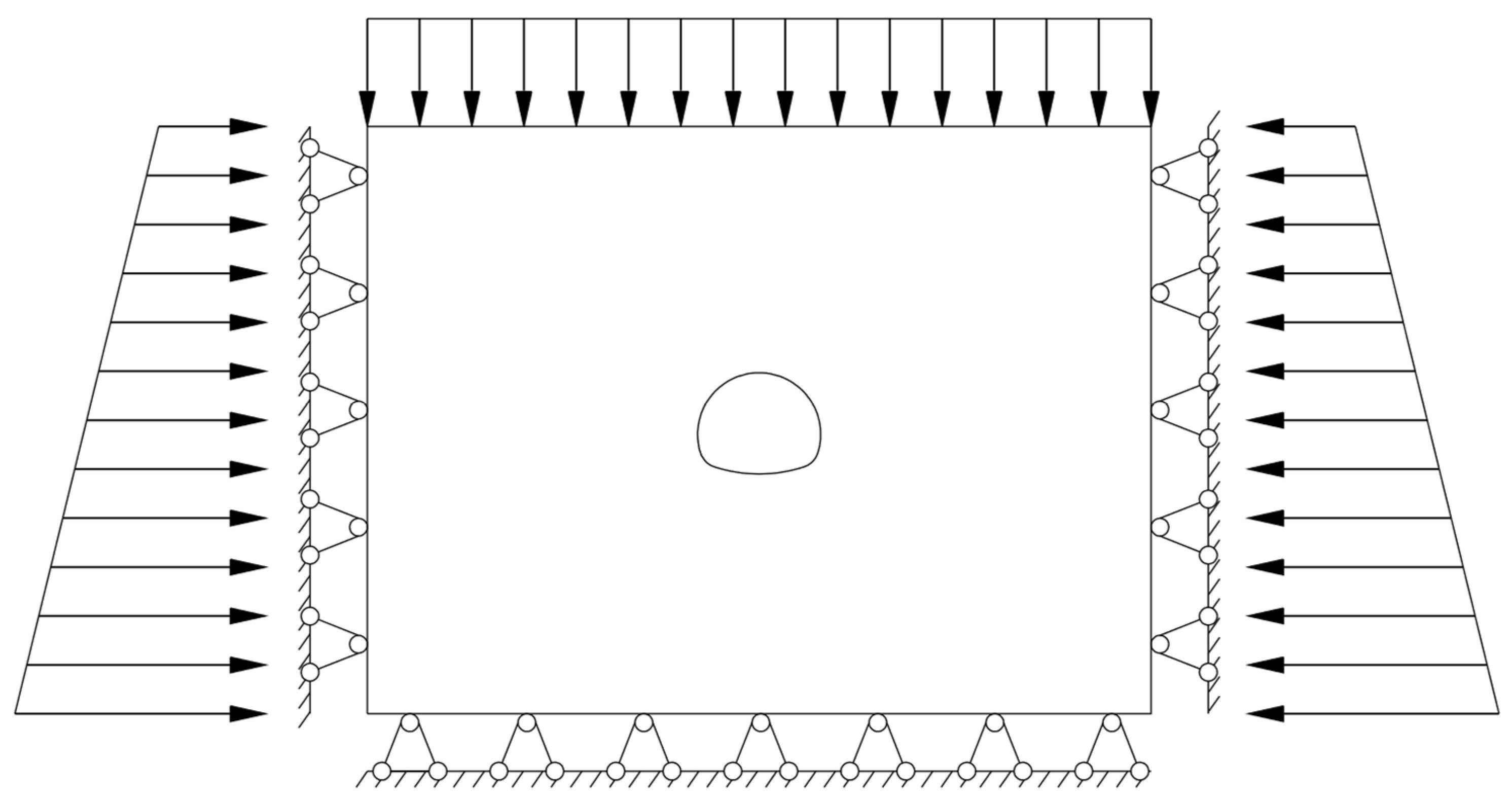
4.3. Initial and Boundary Conditions
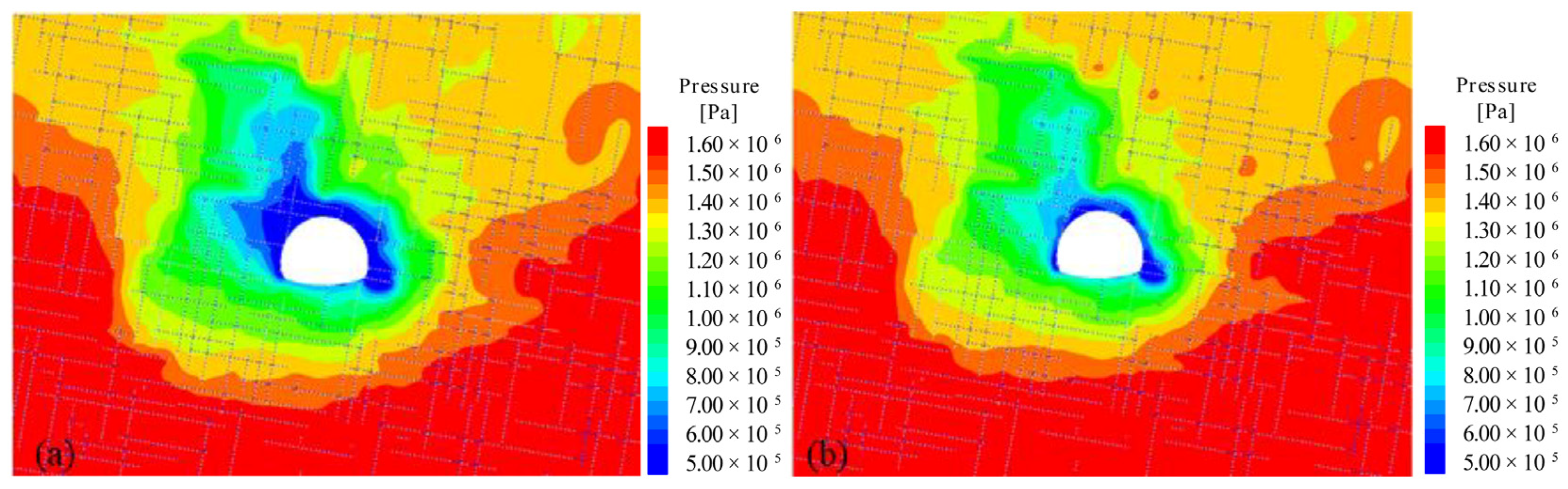
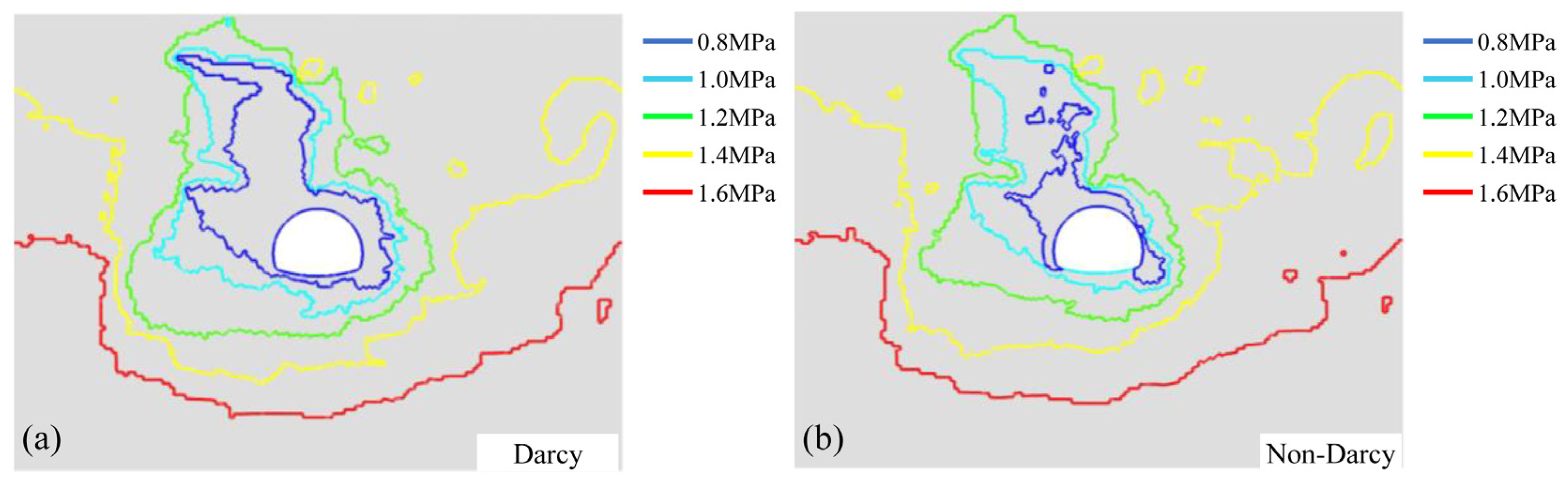
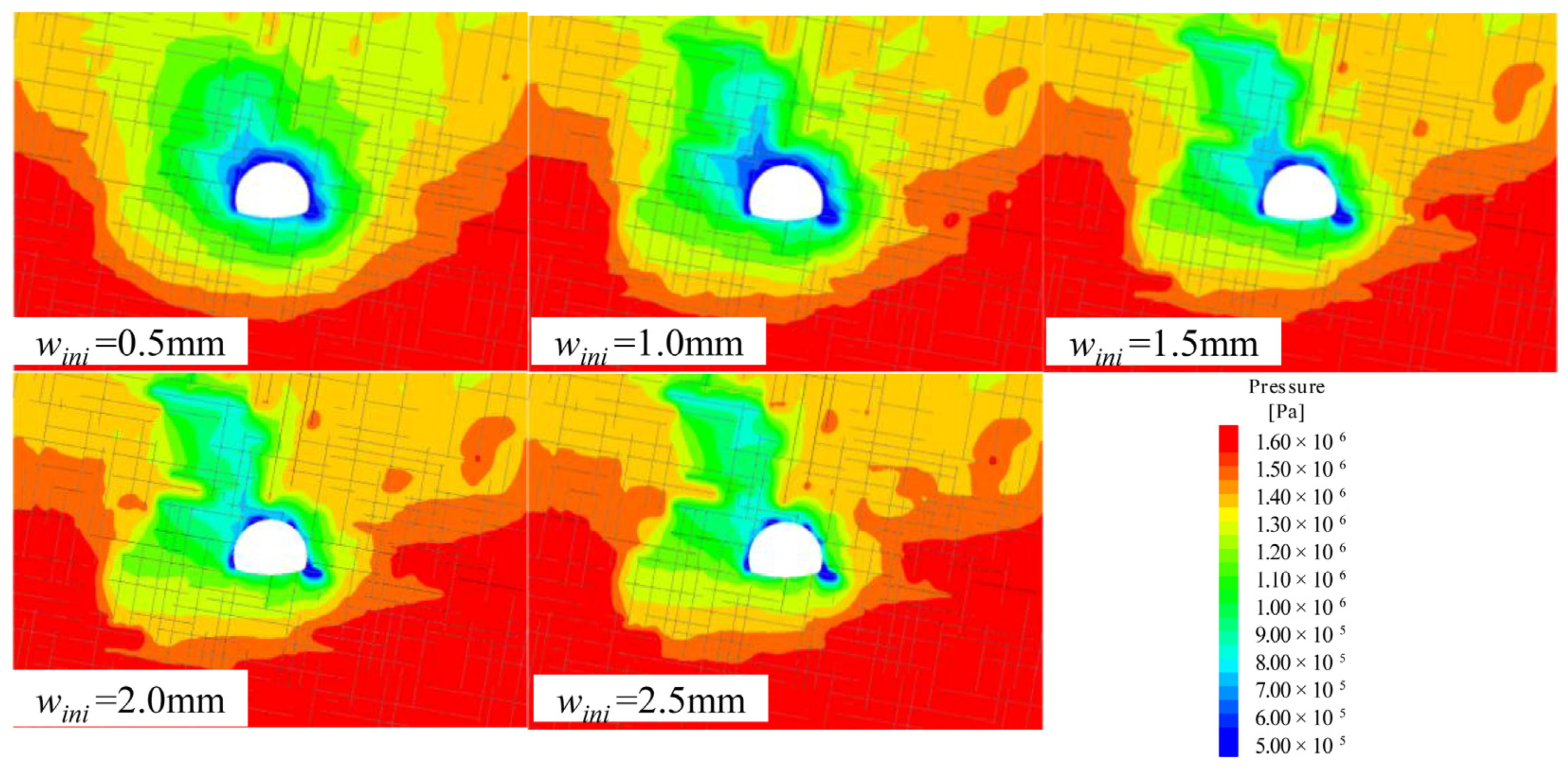
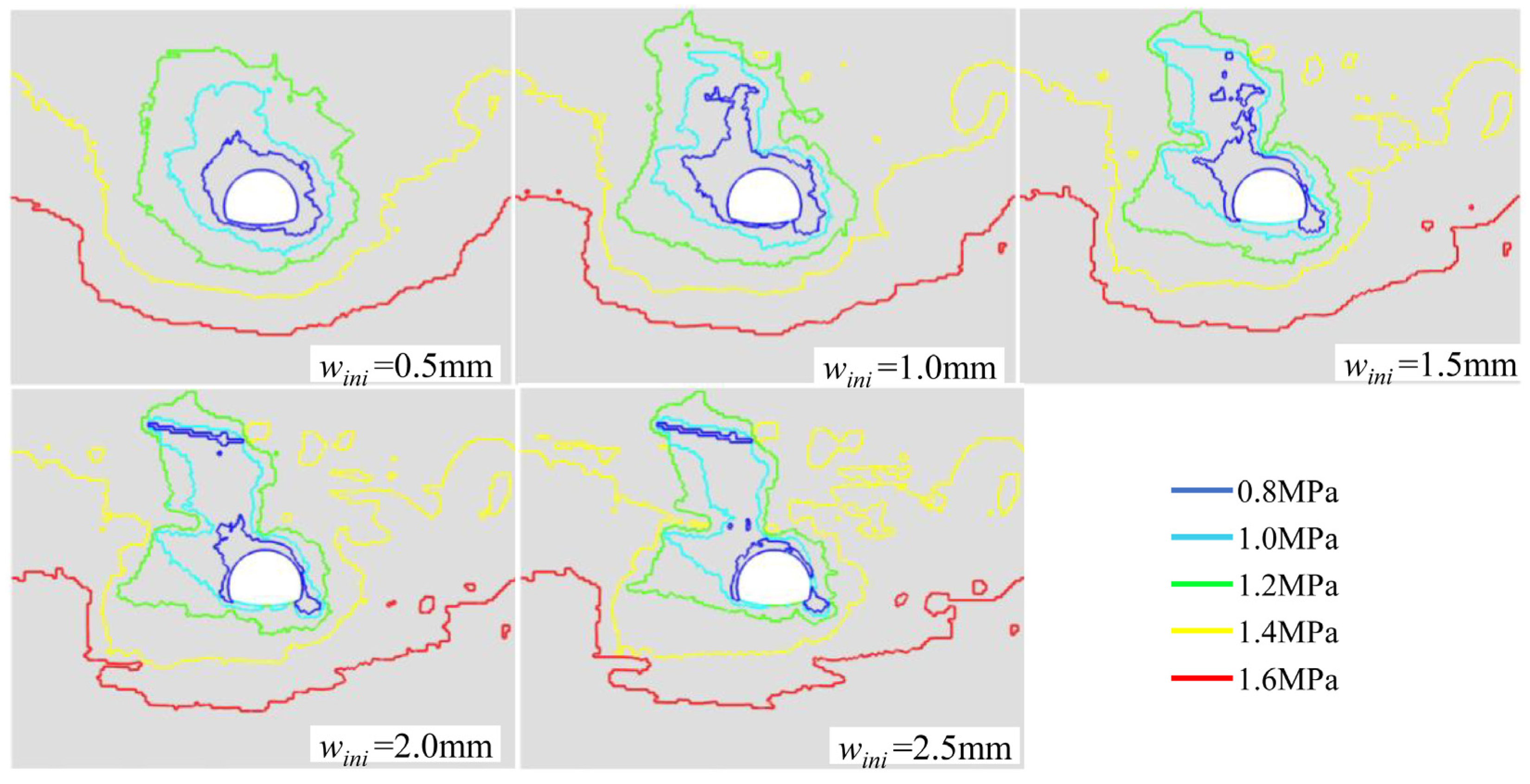
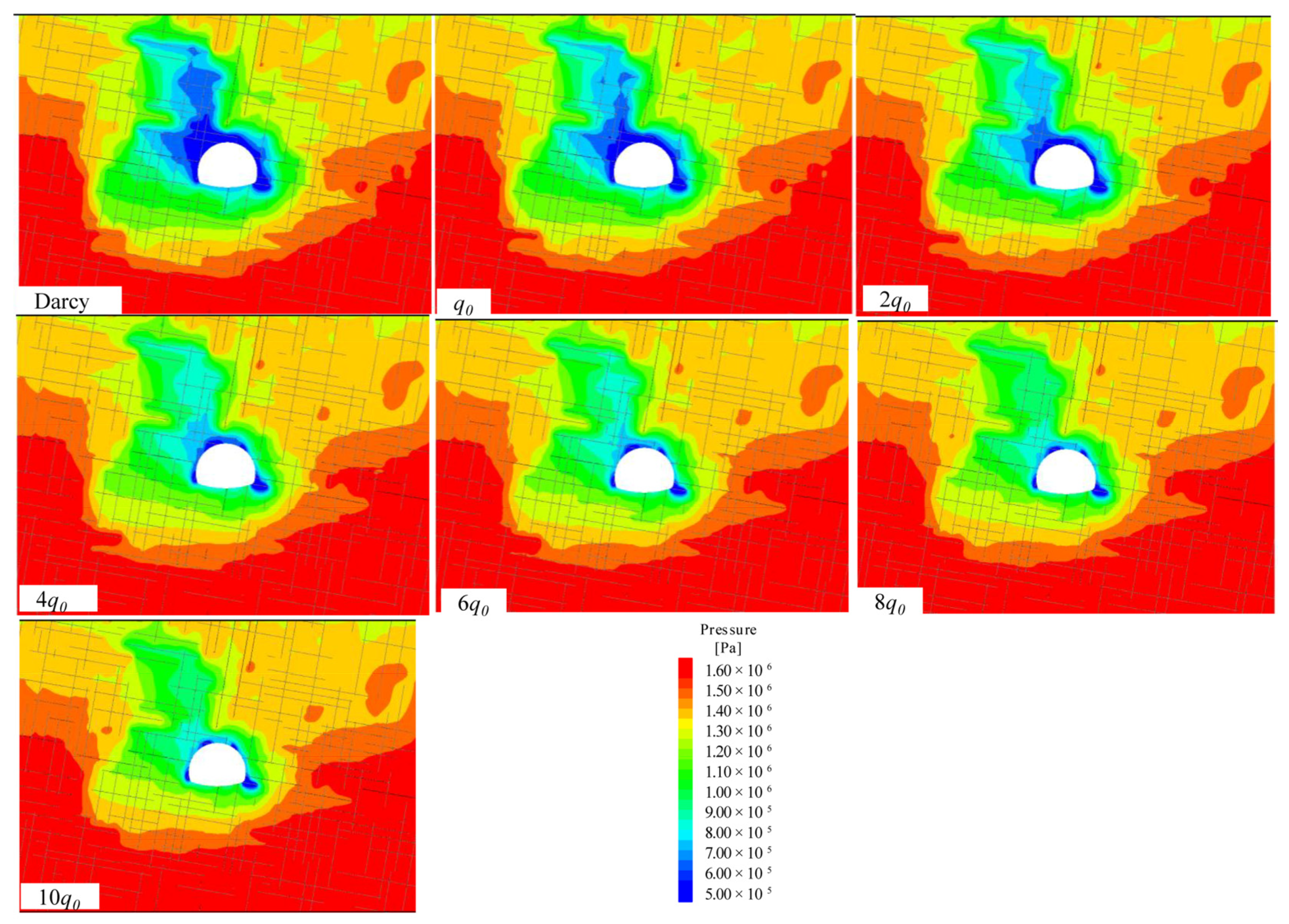
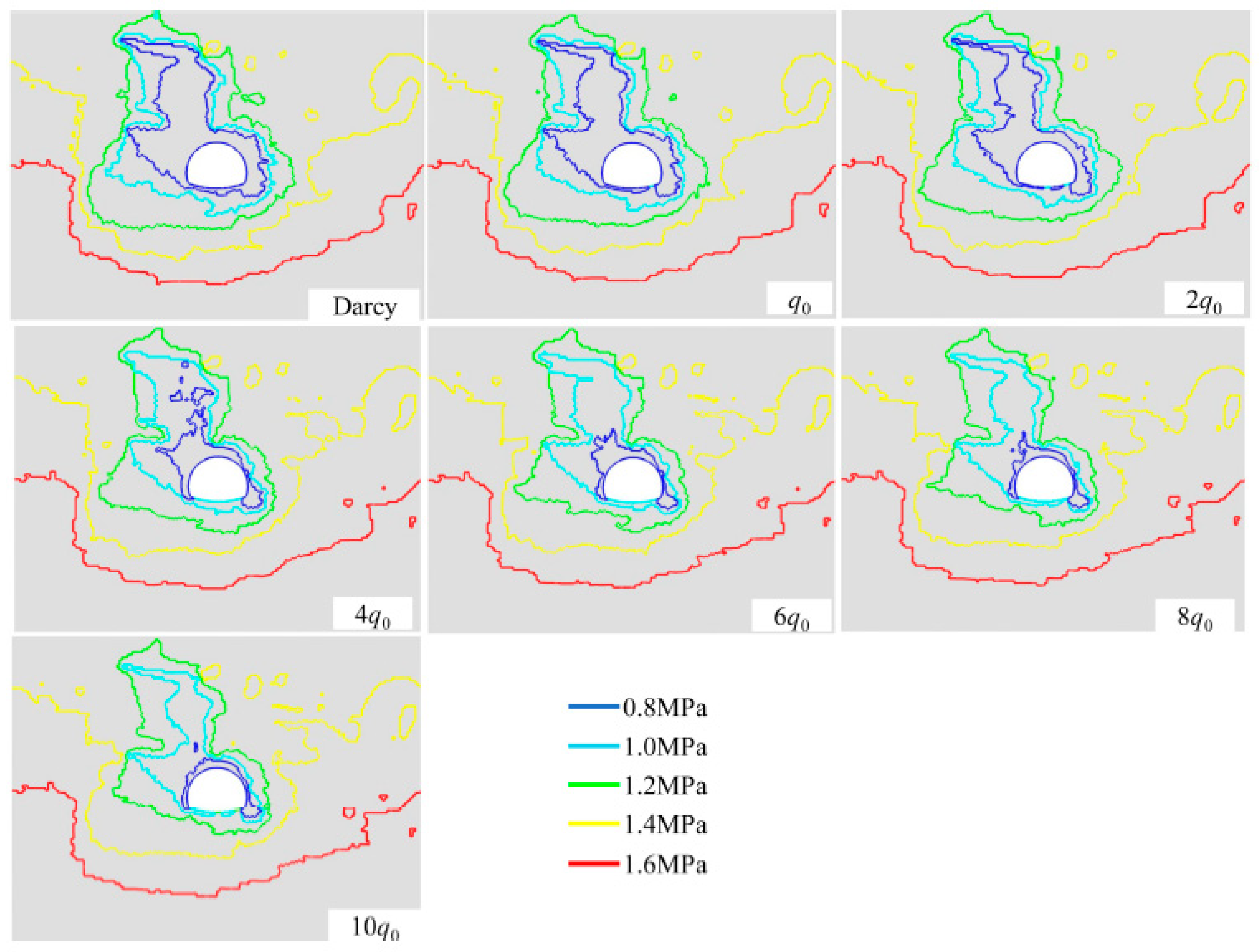
4.4. Numerical Results
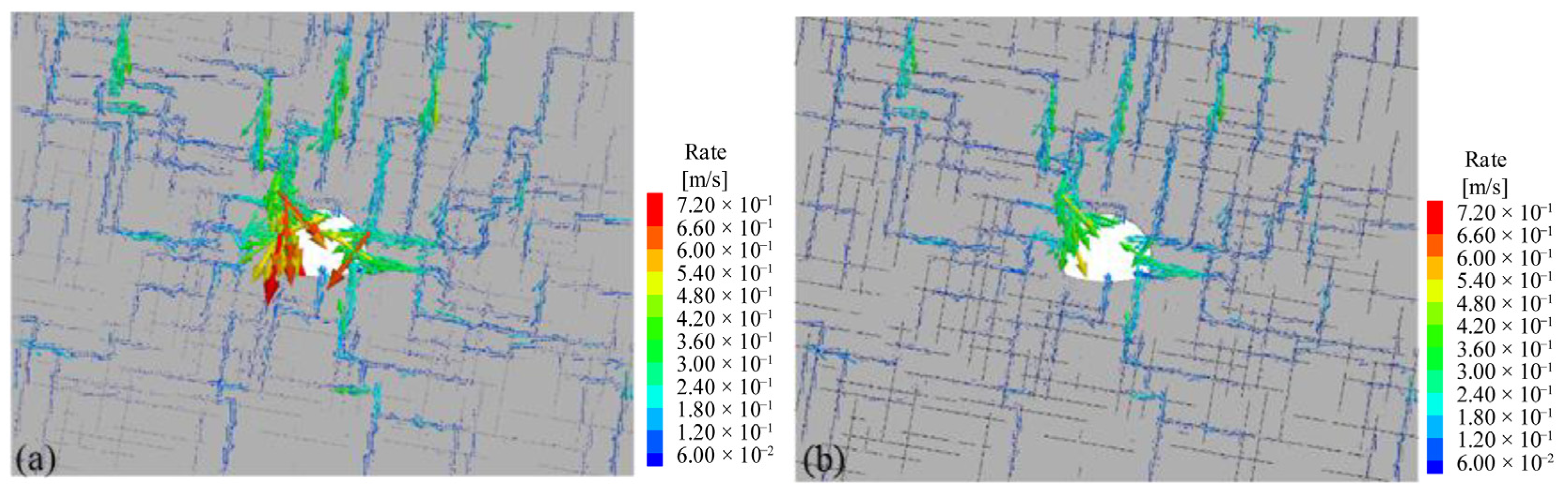
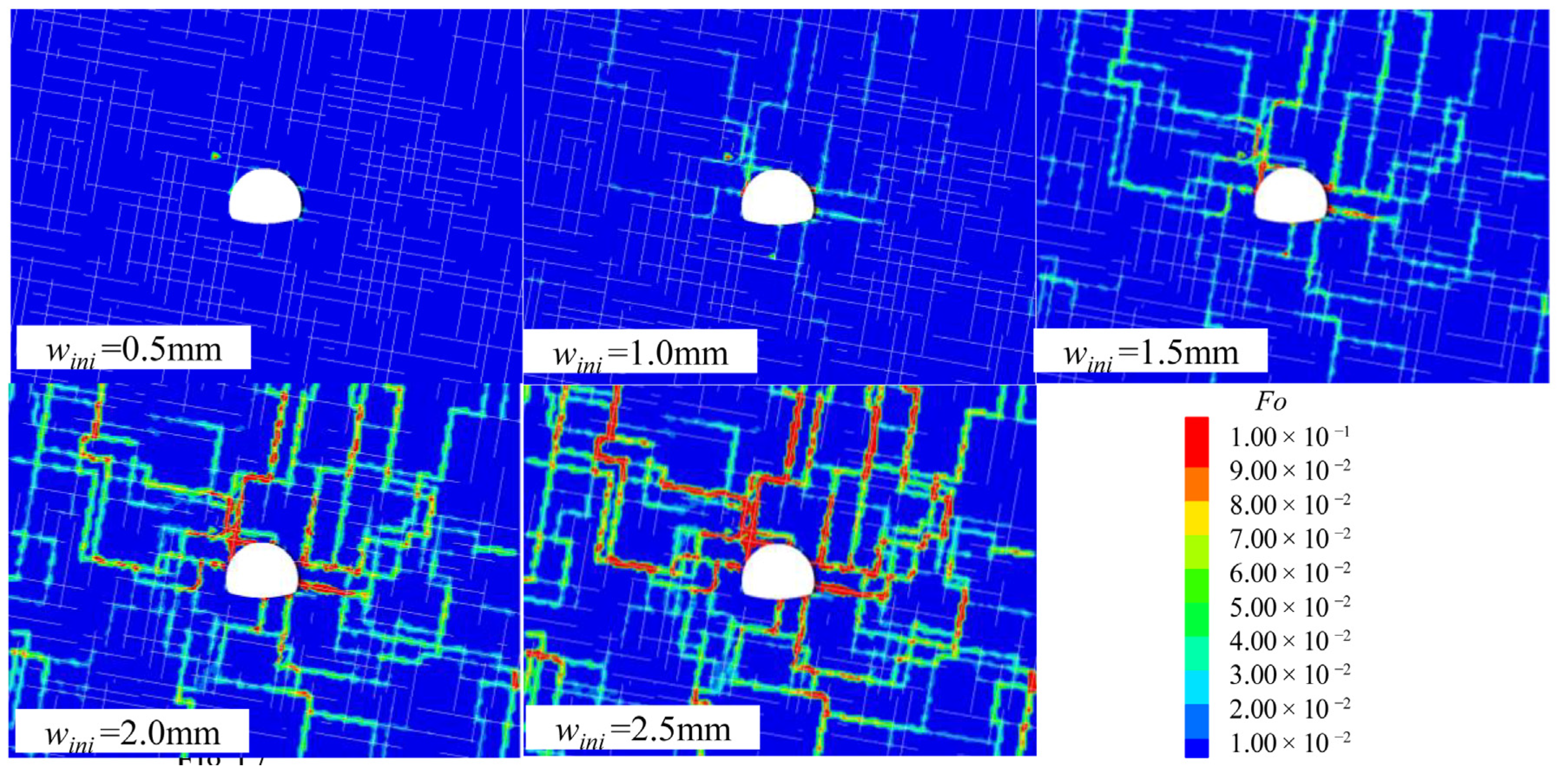
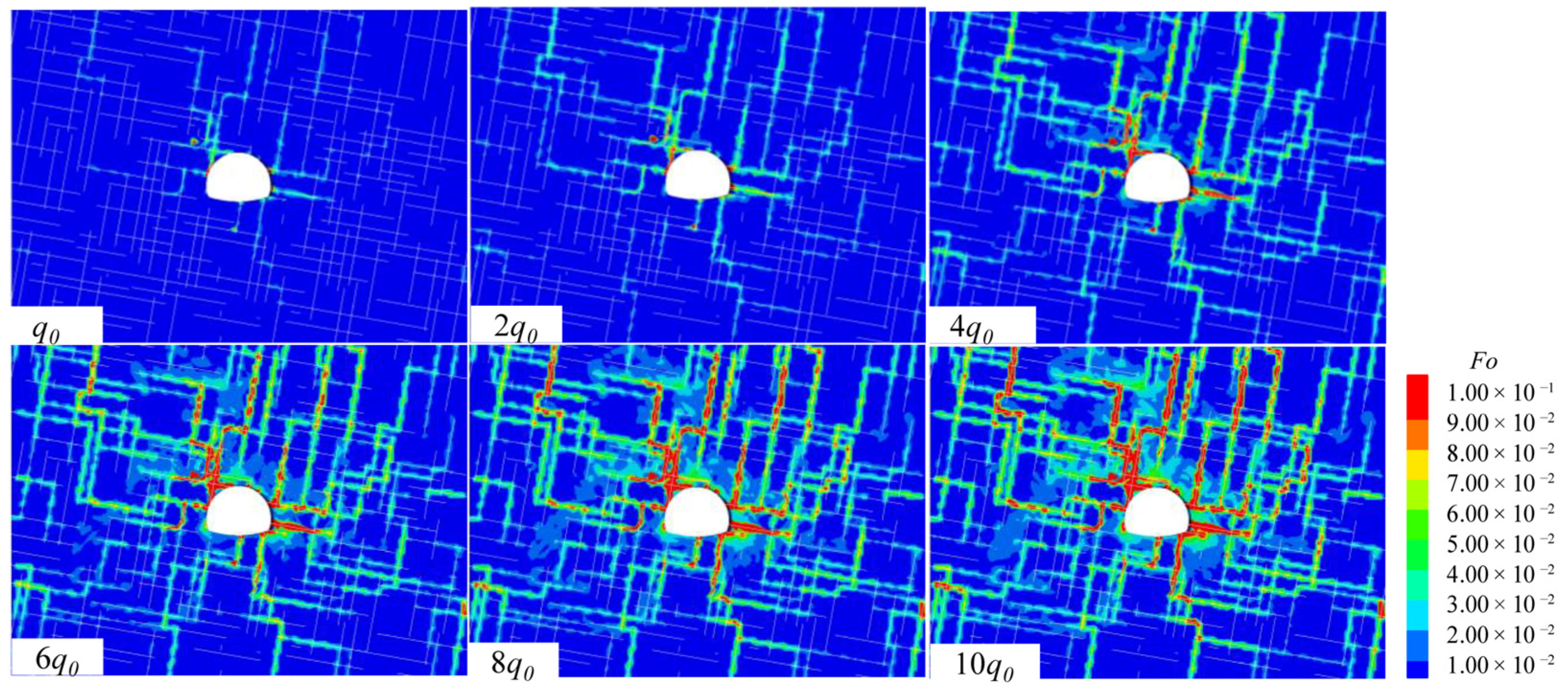
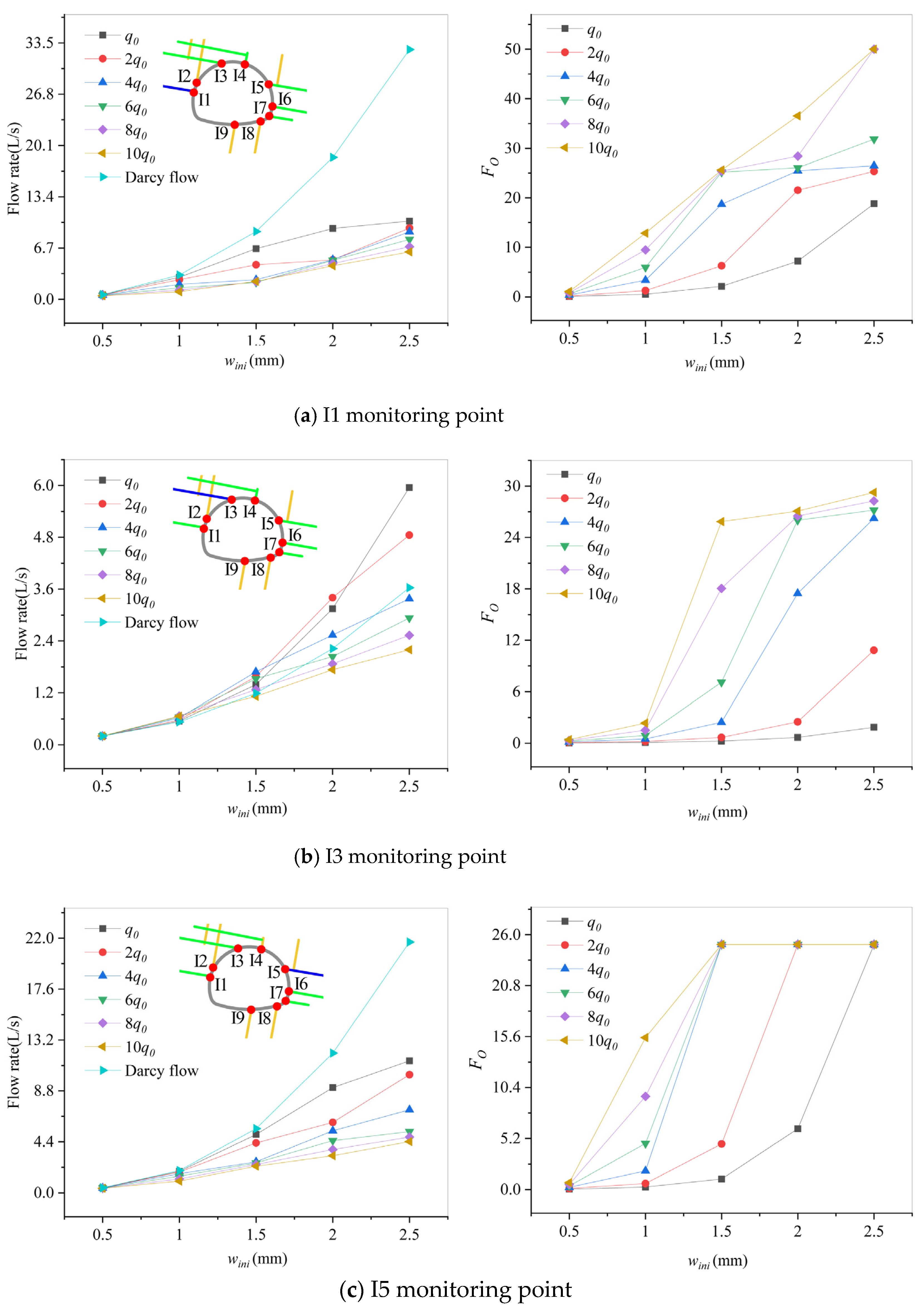
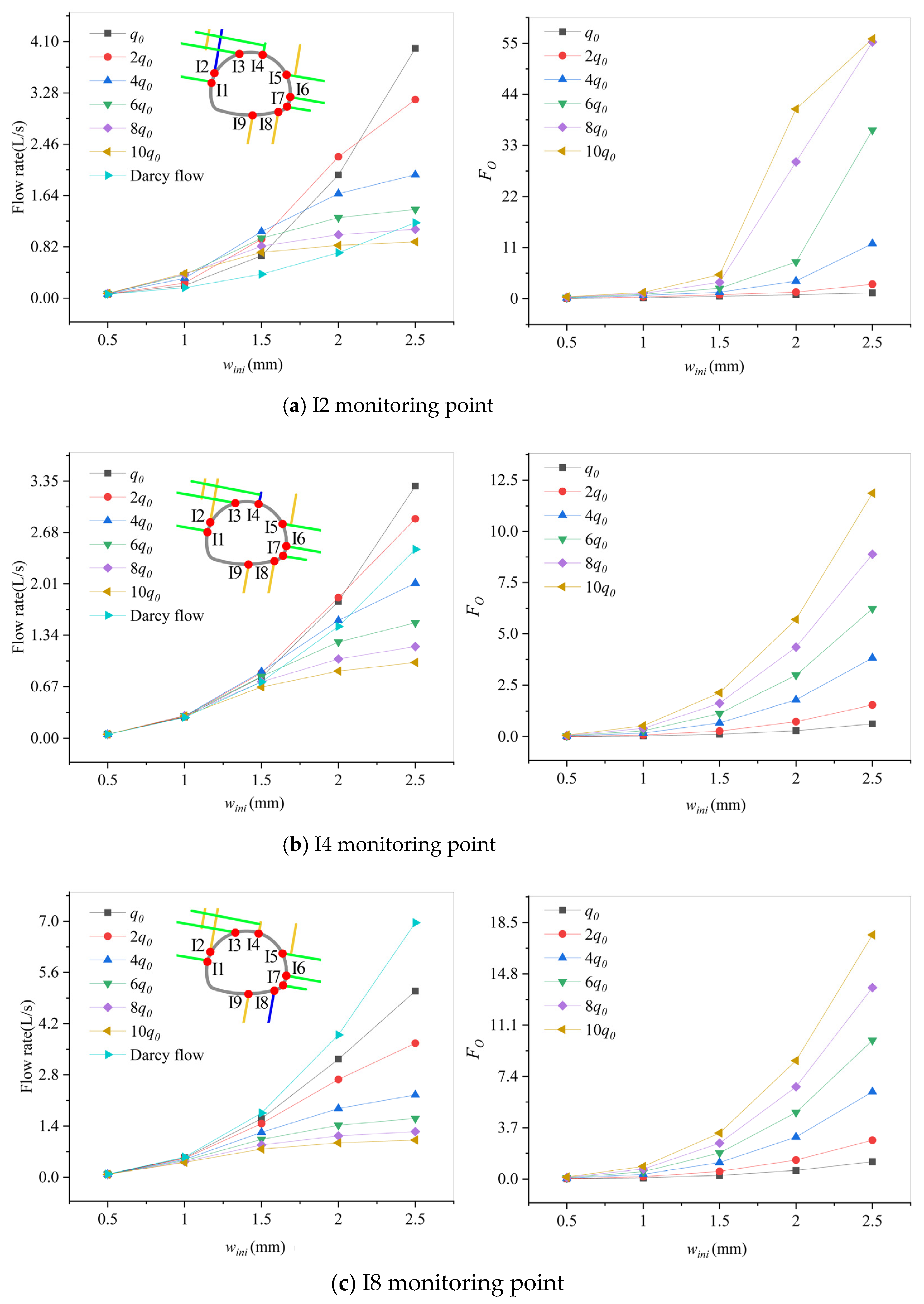
4.5. The Effect of Fracture Features and Non-Darcy Effect
5. Discussion
6. Conclusions
- (1)
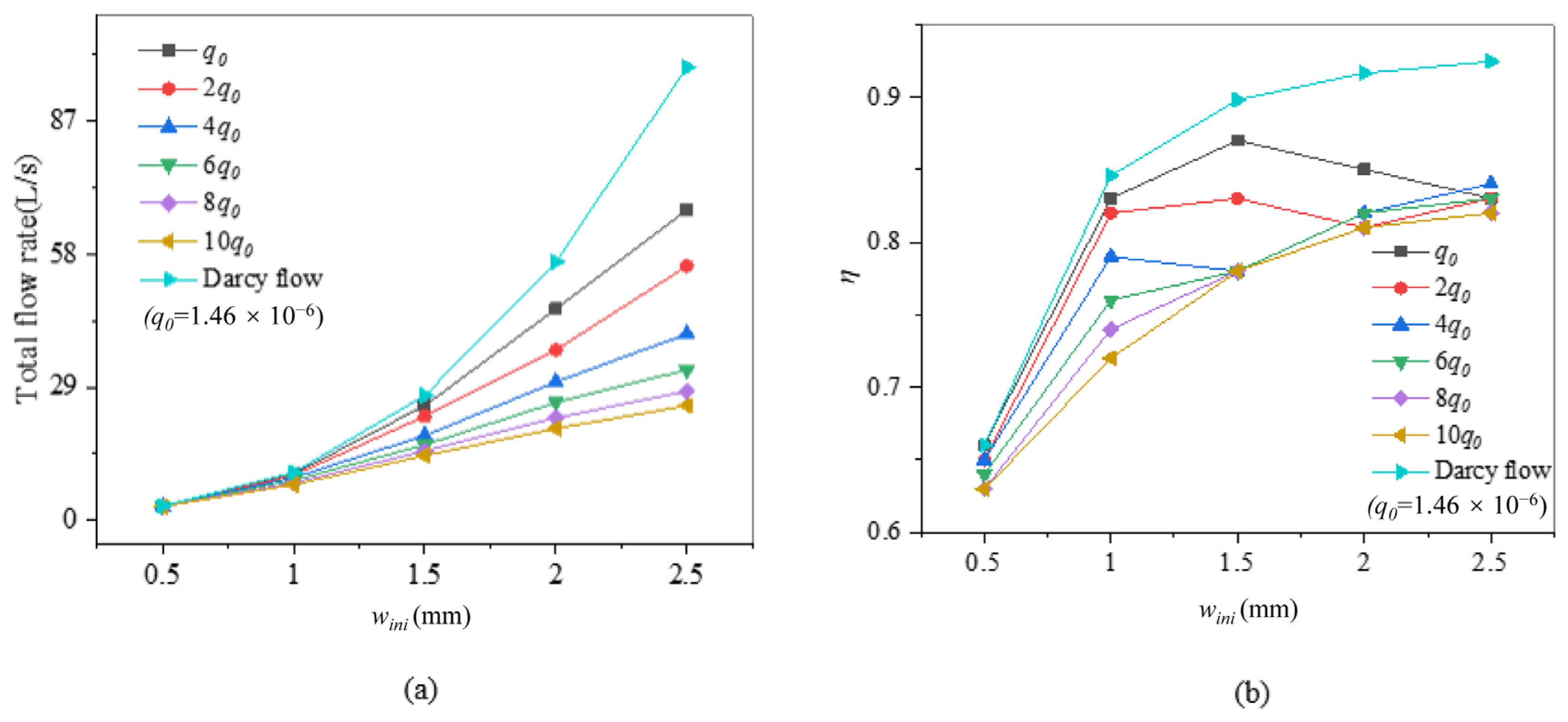
- Darcy flow tends to overestimate water inflow, especially when fracture widths range from 0 to 3 mm, with Darcy flow exceeding non-Darcy flow by up to 25%, consistent with previous research by Chen, Liao, Zhou, Hu, Yang, Zhao, Wu, and Yang [12] in karst tunnels of Guizhou.
- (2)
- Increasing initial fracture width results in higher flow rates and stronger non-Darcy effects. Additionally, raising the q value further intensifies the non-Darcy effects and reduces flow rates. However, there is a threshold value at which the impact of non-Darcy effects slows down, and this threshold value is approximately 8.77 × 10−6 for the Bodaoling Tunnel.
- (3)
- The interaction of non-Darcy flow and complex fracture networks results in intricate flow patterns. Non-Darcy flow obstruction can redirect flow through the fracture network to adjacent inflow points, causing non-Darcy flow to be faster than Darcy flow at specific locations. This underscores the necessity of considering the non-Darcy effect in predicting water inflow in complex fractured rock masses.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Li, G.; Li, C.; Liao, J.; Wang, H. A New Hydro-Mechanical Coupling Numerical Model for Predicting Water Inflow in Karst Tunnels Considering Deformable Fracture. Sustainability 2023, 15, 14703. [Google Scholar] [CrossRef]
- Qin, Y.; Lai, J.; Gao, G.; Yang, T.; Zan, W.; Feng, Z.; Liu, T. Failure analysis and countermeasures of a tunnel constructed in loose granular stratum by shallow tunnelling method. Eng. Fail. Anal. 2022, 141, 106667. [Google Scholar] [CrossRef]
- Shahriar, K.; Sharifzadeh, M.; Hamidi, J.K. Geotechnical risk assessment based approach for rock TBM selection in difficult ground conditions. Tunn. Undergr. Space Technol. 2008, 23, 318–325. [Google Scholar] [CrossRef]
- Li, L.; Lei, T.; Li, S.; Zhang, Q.; Xu, Z.; Shi, S.; Zhou, Z. Risk assessment of water inrush in karst tunnels and software development. Arab. J. Geosci. 2015, 8, 1843–1854. [Google Scholar] [CrossRef]
- Peng, L.J. Existing Operational Railway Tunnel Water Leakage Causes and Remediation Technologies. Adv. Mater. Res. 2014, 1004–1005, 1444–1449. [Google Scholar] [CrossRef]
- Zhang, Y.; Zhang, D.; Fang, Q.; Xiong, L.; Yu, L.; Zhou, M. Analytical solutions of non-Darcy seepage of grouted subsea tunnels. Tunn. Undergr. Space Technol. 2020, 96, 103182. [Google Scholar] [CrossRef]
- Wu, Q.; Wang, M.; Wu, X. Investigations of groundwater bursting into coal mine seam floors from fault zones. Int. J. Rock Mech. Min. Sci. 2004, 41, 557–571. [Google Scholar] [CrossRef]
- Jeannin, P.-Y.; Malard, A.; Rickerl, D.; Weber, E. Assessing karst-hydraulic hazards in tunneling—The Brunnmühle spring system—Bernese Jura, Switzerland. Environ. Earth Sci. 2015, 74, 7655–7670. [Google Scholar] [CrossRef]
- Apaydin, A.; Korkmaz, N.; Ciftci, D. Water inflow into tunnels: Assessment of the Gerede water transmission tunnel (Turkey) with complex hydrogeology. Q. J. Eng. Geol. Hydrogeol. 2018, 52, 346–359. [Google Scholar] [CrossRef]
- Li, S.-C.; Zhou, Z.-Q.; Li, L.-P.; Xu, Z.-H.; Zhang, Q.-Q.; Shi, S.-S. Risk assessment of water inrush in karst tunnels based on attribute synthetic evaluation system. Tunn. Undergr. Space Technol. 2013, 38, 50–58. [Google Scholar] [CrossRef]
- Chen, T.; Yin, H.; Zhai, Y.; Xu, L.; Zhao, C.; Zhang, L. Numerical Simulation of Mine Water Inflow with an Embedded Discrete Fracture Model: Application to the 16112 Working Face in the Binhu Coal Mine, China. Mine Water Environ. 2022, 41, 156–167. [Google Scholar] [CrossRef]
- Chen, Y.-F.; Liao, Z.; Zhou, J.-Q.; Hu, R.; Yang, Z.; Zhao, X.-J.; Wu, X.-L.; Yang, X.-L. Non-Darcian flow effect on discharge into a tunnel in karst aquifers. Int. J. Rock Mech. Min. Sci. 2020, 130, 104319. [Google Scholar] [CrossRef]
- Goodman, R.E. Ground Water Inflows during Tunnels Driving; College of Engineering, University of California: Berkeley, CA, USA, 1964. [Google Scholar]
- Moon, J.; Fernandez, G. Effect of Excavation-Induced Groundwater Level Drawdown on Tunnel Inflow in a Jointed Rock Mass. Eng. Geol. 2010, 110, 33–42. [Google Scholar] [CrossRef]
- Kolymbas, D.; Wagner, P. Groundwater ingress to tunnels—The exact analytical solution. Tunn. Undergr. Space Technol. 2007, 22, 23–27. [Google Scholar] [CrossRef]
- Perrochet, P. Confined Flow into a Tunnel during Progressive Drilling: An Analytical Solution. Groundwater 2005, 43, 943–946. [Google Scholar] [CrossRef]
- Park, K.-H.; Owatsiriwong, A.; Lee, J.-G. Analytical solution for steady-state groundwater inflow into a drained circular tunnel in a semi-infinite aquifer: A revisit. Tunn. Undergr. Space Technol. 2008, 23, 206–209. [Google Scholar] [CrossRef]
- Sato, K.; Iizawa, M. Fundamental study on unsteady flow around underground cavern in unconfined groundwater. Proc. Jpn. Soc. Civ. Eng. 1983, 1983, 213–221. [Google Scholar] [CrossRef][Green Version]
- Shi, W.; Yang, T.; Yu, S. Experimental Investigation on Non-Darcy Flow Behavior of Granular Limestone with Different Porosity. J. Hydrol. Eng. 2020, 25, 06020004. [Google Scholar] [CrossRef]
- Hassani, A.N.; Katibeh, H.; Farhadian, H. Numerical analysis of steady-state groundwater inflow into Tabriz line 2 metro tunnel, northwestern Iran, with special consideration of model dimensions. Bull. Eng. Geol. Environ. 2016, 75, 1617–1627. [Google Scholar] [CrossRef]
- Zhang, K.; Xue, Y.; Xu, Z.; Su, M.; Qiu, D.; Li, Z. Numerical study of water inflow into tunnels in stratified rock masses with a dual permeability model. Environ. Earth Sci. 2021, 80, 260. [Google Scholar] [CrossRef]
- Chen, Z.; Su, Z.; Li, M.; Shen, Q.; Fan, L.; Zhang, Y. Investigation of the Tunnel Water Inflow Prediction Method Based on the MODFLOW-DRAIN Module. Water 2024, 16, 1078. [Google Scholar] [CrossRef]
- Jin, X.; Li, Y.; Luo, Y.; Liu, H. Prediction of city tunnel water inflow and its influence on overlain lakes in karst valley. Environ. Earth Sci. 2016, 75, 1162. [Google Scholar] [CrossRef]
- Zhang, X.; Wang, M.; Feng, D.; Wang, J. Water Inflow Amount Prediction for Karst Tunnel with Steady Seepage Conditions. Sustainability 2023, 15, 10638. [Google Scholar] [CrossRef]
- Quinn, P.M.; Cherry, J.A.; Parker, B.L. Quantification of non-Darcian flow observed during packer testing in fractured sedimentary rock. Water Resour. Res. 2011, 47, W09533. [Google Scholar] [CrossRef]
- Lee, S.H.; Lee, K.; Yeo, I.W. Assessment of the validity of Stokes and Reynolds equations for fluid flow through a rough-walled fracture with flow imaging. Geophys. Res. Lett. 2014, 41, 4578–4585. [Google Scholar] [CrossRef]
- Chen, Y.-F.; Zhou, J.-Q.; Hu, S.-H.; Hu, R.; Zhou, C.-B. Evaluation of Forchheimer equation coefficients for non-Darcy flow in deformable rough-walled fractures. J. Hydrol. 2015, 529, 993–1006. [Google Scholar] [CrossRef]
- Zhou, J.-Q.; Liu, H.-B.; Li, C.; He, X.-L.; Tang, H.; Zhao, X.-J. A semi-empirical model for water inflow into a tunnel in fractured-rock aquifers considering non-Darcian flow. J. Hydrol. 2021, 597, 126149. [Google Scholar] [CrossRef]
- Liu, H.-B.; Zhou, J.-Q.; Li, C.; Tan, J.; Hou, D. Semi-empirical models for predicting stable water inflow and influence radius of a tunnel considering non-Darcian effect. J. Hydrol. 2023, 621, 129574. [Google Scholar] [CrossRef]
- Rui, X.; Yu, T. An efficient karst fracture seepage path construction algorithm based on a generalized disk model. Arab. J. Geosci. 2019, 12, 262. [Google Scholar] [CrossRef]
- Zhou, J.-Q.; Gan, F.-S.; Li, C.; Tang, H. A global inertial permeability for fluid flow in rock fractures: Criterion and significance. Eng. Geol. 2023, 322, 107167. [Google Scholar] [CrossRef]
- Huang, Z.; Zhao, K.; Li, X.; Zhong, W.; Wu, Y. Numerical characterization of groundwater flow and fracture-induced water inrush in tunnels. Tunn. Undergr. Space Technol. 2021, 116, 104119. [Google Scholar] [CrossRef]
- Farhadian, H.; Katibeh, H.; Huggenberger, P.; Butscher, C. Optimum model extent for numerical simulation of tunnel inflow in fractured rock. Tunn. Undergr. Space Technol. 2016, 60, 21–29. [Google Scholar] [CrossRef]
- Shi, S.; Guo, W.; Li, S.; Xie, X.; Li, X.; Zhao, R.; Xue, Y.; Lu, J. Prediction of tunnel water inflow based on stochastic deterministic three-dimensional fracture network. Tunn. Undergr. Space Technol. 2023, 135, 104997. [Google Scholar] [CrossRef]
- Zhang, R.-H.; Zhang, L.-H.; Luo, J.-X.; Yang, Z.-D.; Xu, M.-Y. Numerical simulation of water flooding in natural fractured reservoirs based on control volume finite element method. J. Pet. Sci. Eng. 2016, 146, 1211–1225. [Google Scholar] [CrossRef]
- Xie, Y.; Liao, J.; Zhao, P.; Xia, K.; Li, C. Effects of fracture evolution and non-Darcy flow on the thermal performance of enhanced geothermal system in 3D complex fractured rock. Int. J. Min. Sci. Technol. 2024, 34, 443–459. [Google Scholar] [CrossRef]
- Liao, J.; Xu, B.; Mehmood, F.; Hu, K.; Wang, H.; Hou, Z.; Xie, Y. Numerical study of the long-term performance of EGS based on discrete fracture network with consideration of fracture deformation. Renew. Energy 2023, 216, 119045. [Google Scholar] [CrossRef]
- Bandis, S.; Lumsden, A.; Barton, N. Fundamentals of rock joint deformation. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1983, 20, 249–268. [Google Scholar] [CrossRef]
- Itasca. Manual of FLAC3D; Itasca Consulting Group: Minneapolis, MN, USA, 2015. [Google Scholar]
- Ahmadi, A.; Arani, A.A.A.; Lasseux, D. Numerical Simulation of Two-Phase Inertial Flow in Heterogeneous Porous Media. Transp. Porous Media 2010, 84, 177–200. [Google Scholar] [CrossRef][Green Version]
- Muljadi, B.P.; Blunt, M.J.; Raeini, A.Q.; Bijeljic, B. The impact of porous media heterogeneity on non-Darcy flow behaviour from pore-scale simulation. Adv. Water Resour. 2016, 95, 329–340. [Google Scholar] [CrossRef]
- Ma, D.; Li, Q.; Cai, K.-C.; Zhang, J.-X.; Li, Z.-H.; Hou, W.-T.; Sun, Q.; Li, M.; Du, F. Understanding water inrush hazard of weak geological structure in deep mine engineering: A seepage-induced erosion model considering tortuosity. J. Central South Univ. 2023, 30, 517–529. [Google Scholar] [CrossRef]
- Zhang, K.; Yamamoto, H.; Pruess, K. TMVOC-MP: A Parallel Numerical Simulator for Three-PhaseNon-Isothermal Flows of Multicomponent Hydrocarbon Mixtures Inporous/Fractured Media; Lawrence Berkeley National Laboratory Report 2007, LBNL-63827; Lawrence Berkeley National Lab. (LBNL): Berkeley, CA, USA, 2008. [Google Scholar] [CrossRef]
- Zhou, L.; Su, X.; Lu, Y.; Ge, Z.; Zhang, Z.; Shen, Z. A New Three-Dimensional Numerical Model Based on the Equivalent Continuum Method to Simulate Hydraulic Fracture Propagation in an Underground Coal Mine. Rock Mech. Rock Eng. 2019, 52, 2871–2887. [Google Scholar] [CrossRef]
- Liao, J.; Wang, H.; Mehmood, F.; Cheng, C.; Hou, Z. An anisotropic damage-permeability model for hydraulic fracturing in hard rock. Acta Geotech. 2023, 18, 3661–3681. [Google Scholar] [CrossRef]
- Rong, G.; Yang, J.; Cheng, L.; Tan, J.; Peng, J.; Zhou, C. A Forchheimer equation-based flow model for fluid flow through rock fracture during shear. Rock Mech. Rock Eng. 2018, 51, 2777–2790. [Google Scholar] [CrossRef]
- Geertsma, J. Estimating the Coefficient of Inertial Resistance in Fluid Flow Through Porous Media. Soc. Pet. Eng. J. 1974, 14, 445–450. [Google Scholar] [CrossRef]
- de Castro, A.R.; Radilla, G. Non-Darcian flow of shear-thinning fluids through packed beads: Experiments and predictions using Forchheimer’s law and Ergun’s equation. Adv. Water Resour. 2017, 100, 35–47. [Google Scholar] [CrossRef]
- Huang, T.; Du, P.; Peng, X.; Wang, P.; Zou, G. Pressure drop and fractal non-Darcy coefficient model for fluid flow through porous media. J. Pet. Sci. Eng. 2020, 184, 106579. [Google Scholar] [CrossRef]
- Guo, J.; Qian, Y.; Chen, J.; Chen, F. The Minimum Safe Thickness and Catastrophe Process for Water Inrush of a Karst Tunnel Face with Multi Fractures. Processes 2019, 7, 686. [Google Scholar] [CrossRef]
- Javadi, M.; Sharifzadeh, M.; Shahriar, K.; Mitani, Y. Critical Reynolds number for nonlinear flow through rough-walled fractures: The role of shear processes. Water Resour. Res. 2014, 50, 1789–1804. [Google Scholar] [CrossRef]
- Zhou, J.-Q.; Hu, S.-H.; Fang, S.; Chen, Y.-F.; Zhou, C.-B. Nonlinear flow behavior at low Reynolds numbers through rough-walled fractures subjected to normal compressive loading. Int. J. Rock Mech. Min. Sci. 2015, 80, 202–218. [Google Scholar] [CrossRef]
- Zeng, Z.; Grigg, R. A Criterion for Non-Darcy Flow in Porous Media. Transp. Porous Media 2006, 63, 57–69. [Google Scholar] [CrossRef]
- Zhang, Z.; Nemcik, J. Fluid flow regimes and nonlinear flow characteristics in deformable rock fractures. J. Hydrol. 2013, 477, 139–151. [Google Scholar] [CrossRef]
- Zimmerman, R.W.; Al-Yaarubi, A.; Pain, C.C.; Grattoni, C.A. Non-linear regimes of fluid flow in rock fractures. Int. J. Rock Mech. Min. Sci. 2004, 41, 163–169. [Google Scholar] [CrossRef]
- Moutsopoulos, K.N.; Papaspyros, I.N.; Tsihrintzis, V.A. Experimental investigation of inertial flow processes in porous media. J. Hydrol. 2009, 374, 242–254. [Google Scholar] [CrossRef]
- Tzelepis, V.; Moutsopoulos, K.N.; Papaspyros, J.N.; Tsihrintzis, V.A. Experimental investigation of flow behavior in smooth and rough artificial fractures. J. Hydrol. 2015, 521, 108–118. [Google Scholar] [CrossRef]
- Yao, C.; Shao, Y.; Yang, J.; Huang, F.; He, C.; Jiang, Q.; Zhou, C. Effects of non-darcy flow on heat-flow coupling process in complex fractured rock masses. J. Nat. Gas Sci. Eng. 2020, 83, 103536. [Google Scholar] [CrossRef]
- Cao, Z.-Z.; Xue, Y.-F.; Wang, H.; Chen, J.-R.; Ren, Y.-L. The non-Darcy characteristics of fault water inrush in karst tunnel based on flow state conversion theory. Therm. Sci. 2021, 25, 4415–4421. [Google Scholar] [CrossRef]
- Xue, Y.; Teng, T.; Zhu, L.; He, M.; Ren, J.; Dong, X.; Liu, F. Evaluation of the Non-Darcy Effect of Water Inrush from Karst Collapse Columns by Means of a Nonlinear Flow Model. Water 2018, 10, 1234. [Google Scholar] [CrossRef]
- Cherubini, C.; Giasi, C.I.; Pastore, N. Bench scale laboratory tests to analyze non-linear flow in fractured media. Hydrol. Earth Syst. Sci. 2012, 16, 2511–2522. [Google Scholar] [CrossRef]
- Gattinoni, P.; Consonni, M.; Francani, V.; Leonelli, G.; Lorenzo, C. Tunnelling in landslide areas connected to deep seated gravitational deformations: An example in Central Alps (northern Italy). Tunn. Undergr. Space Technol. 2019, 93, 103100. [Google Scholar] [CrossRef]
- Nikakhtar, L.; Zare, S. Evaluation of Underground Water Flow into Tabriz Metro Tunnel First Line by Hydro-Mechanical Coupling Analysis. Int. J. Geotech. Eng. 2020, 157, 1–7. [Google Scholar] [CrossRef]
- Zhao, X.; Yang, B.; Niu, Y.; Yang, C. Seepage-Fractal Characteristics of Fractured Media Rock Materials Due to High-Velocity Non-Darcy Flow. Fractal Fract. 2022, 6, 685. [Google Scholar] [CrossRef]
- Geng, S.; He, X.; Zhu, R.; Li, C. A new permeability model for smooth fractures filled with spherical proppants. J. Hydrol. 2023, 626, 130220. [Google Scholar] [CrossRef]
- Jia, J.; Chen, Y.; Luo, H.; Ma, G. Seepage stability analysis of a deep-buried tunnel in fractured rocks based on a non-Darcy hydro-mechanical coupled method. Tunn. Undergr. Space Technol. 2023, 142, 105393. [Google Scholar] [CrossRef]





| Parameter | Unit | Value | Data Source and Note |
|---|---|---|---|
| Young’s modulus E | GPa | 60 | Testing report of BDSDS-2 |
| Poisson ratio v | - | 0.2 | Testing report of BDSDS-2 |
| Friction angle of intact rock fI | ° | 44 | Testing report of BDSDS-2 |
| Cohesion of intact rock cI | kPa | 6000 | Testing report of BDSDS-2 |
| Initial permeability of intact rock kI | m2 | 10−15 | From Huang et al. [49] |
| Initial porosity of intact rock φI | % | 2.5 | From Huang et al. [49] |
| Friction angle of fractured rock ff | ° | 30 | From Guo et al. [50] |
| Cohesion of fractured rock cf | kPa | 860 | From Guo et al. [50] |
| Initial fracture width wini | mm | 1 | Sensitive analysis |
| Tortuosity τ | - | 1.91 | From Ahmadi et al. [40] |
| Empirical constant q0 | - | 1.46 × 10−6 | From data matching |
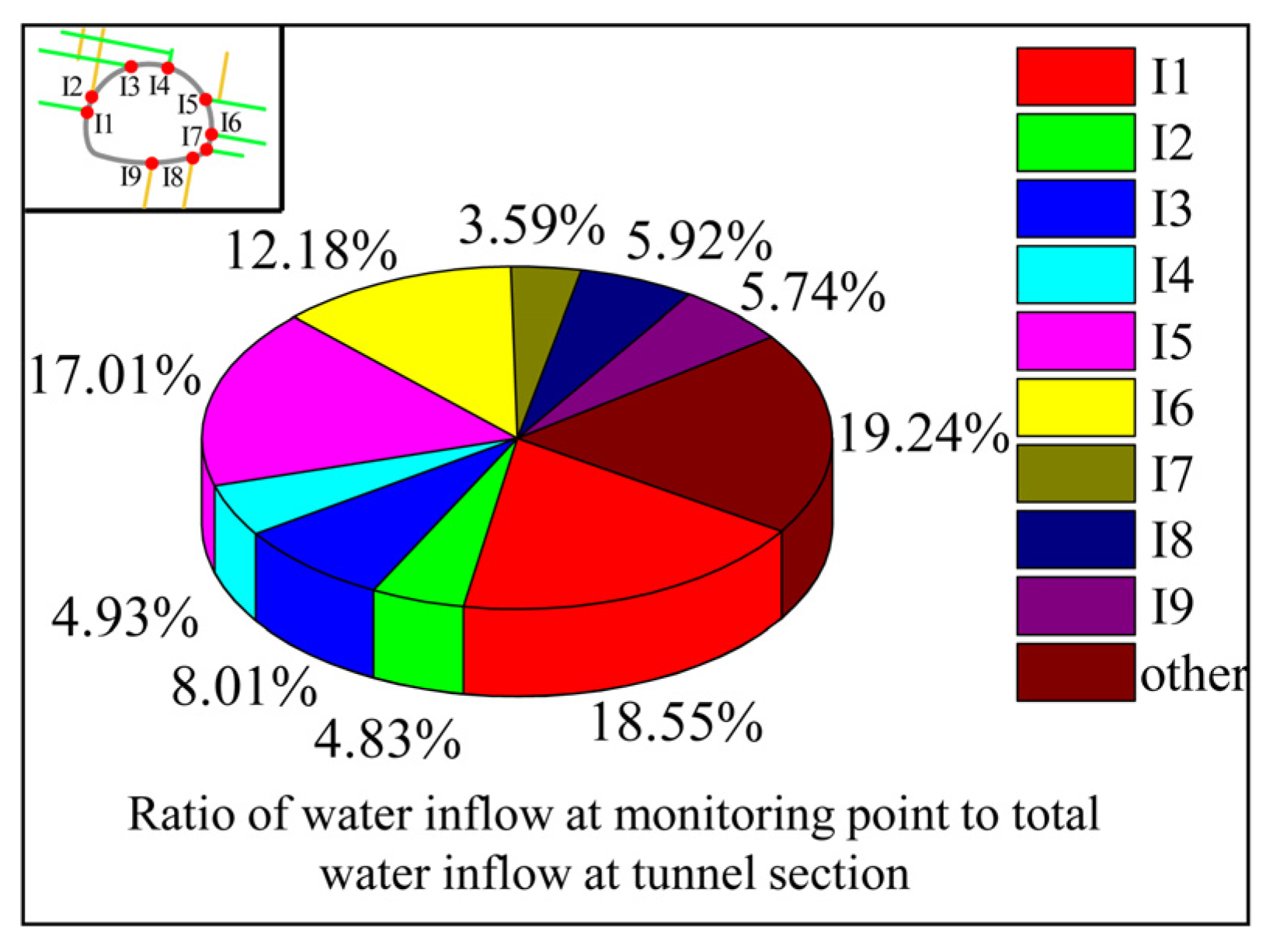
| Number | Velocity/(L/s) | Decrease Rate (%) | Fo | |
|---|---|---|---|---|
| Darcy Flow | Non-Darcy Flow | |||
| I2 | 0.17 | 0.39 | −137 | 1.37 |
| I4 | 0.27 | 0.29 | −7 | 0.53 |
| I7 | 0.49 | 0.57 | −16 | 1.67 |
| I3 | 0.53 | 0.66 | −25 | 2.35 |
| I8 | 0.55 | 0.41 | 26 | 0.91 |
| I9 | 0.56 | 0.42 | 25 | 0.92 |
| I6 | 1.19 | 0.87 | 27 | 5.33 |
| I5 | 1.92 | 1.01 | 47 | 15.45 |
| I1 | 3.17 | 0.99 | 69 | 12.87 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hu, K.; Yao, L.; Liao, J.; Wang, H.; Luo, J.; Xu, X. Predicting Water Inflow in Tunnel Construction: A Fracture Network Model with Non-Darcy Flow Considerations. Water 2024, 16, 1885. https://doi.org/10.3390/w16131885
Hu K, Yao L, Liao J, Wang H, Luo J, Xu X. Predicting Water Inflow in Tunnel Construction: A Fracture Network Model with Non-Darcy Flow Considerations. Water. 2024; 16(13):1885. https://doi.org/10.3390/w16131885
Chicago/Turabian StyleHu, Ke, Liang Yao, Jianxing Liao, Hong Wang, Jiashun Luo, and Xiangdong Xu. 2024. "Predicting Water Inflow in Tunnel Construction: A Fracture Network Model with Non-Darcy Flow Considerations" Water 16, no. 13: 1885. https://doi.org/10.3390/w16131885
APA StyleHu, K., Yao, L., Liao, J., Wang, H., Luo, J., & Xu, X. (2024). Predicting Water Inflow in Tunnel Construction: A Fracture Network Model with Non-Darcy Flow Considerations. Water, 16(13), 1885. https://doi.org/10.3390/w16131885









