An Efficient Seepage Element Containing Drainage Pipe
Abstract
1. Introduction
2. Theory Presentation and Model Evaluation
2.1. Finite Element Governing Equations of the Seepage Field
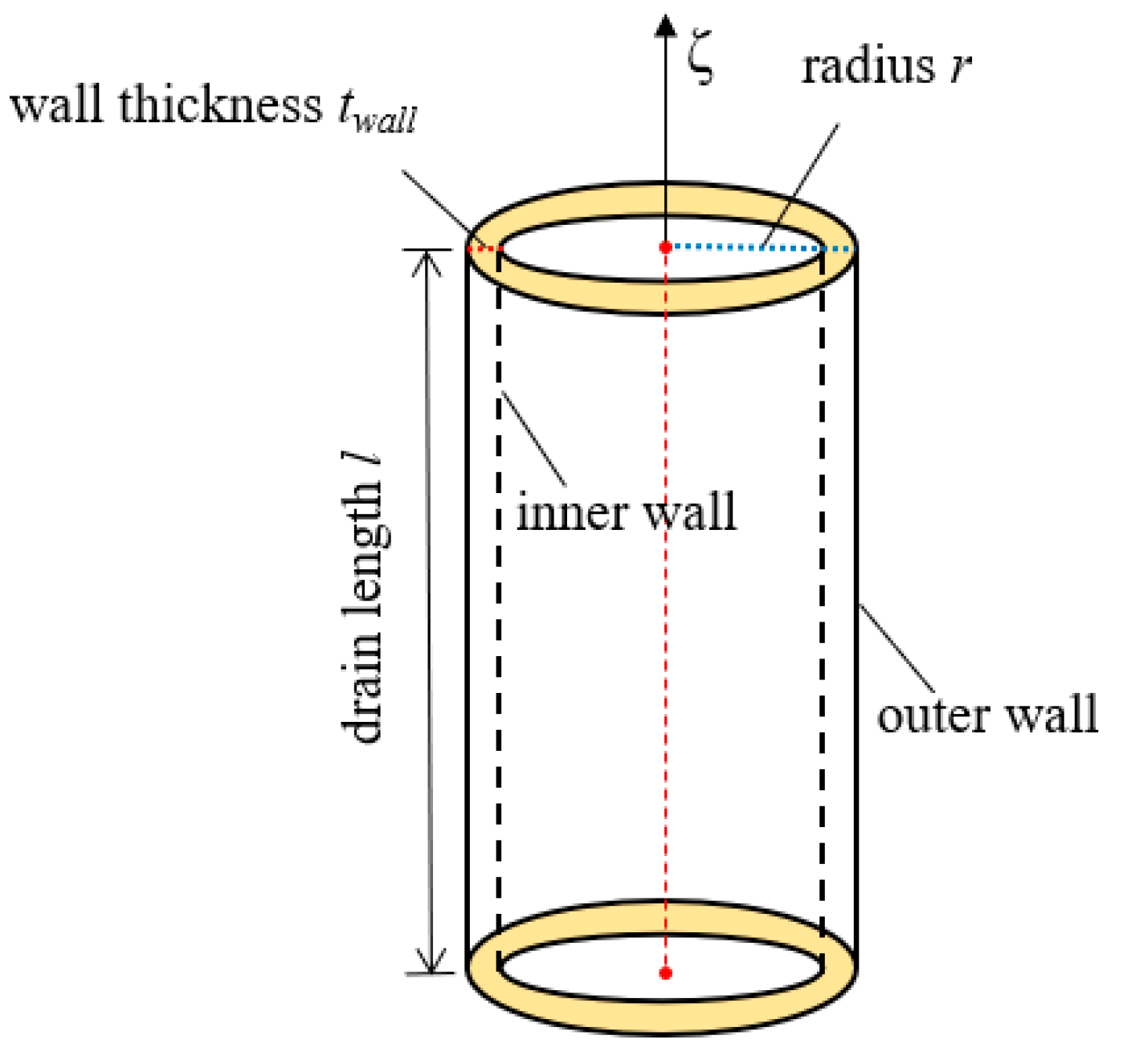
2.2. The Construction of the Seepage Element Containing Drainage Pipe
2.3. Transient Equation Solving Scheme
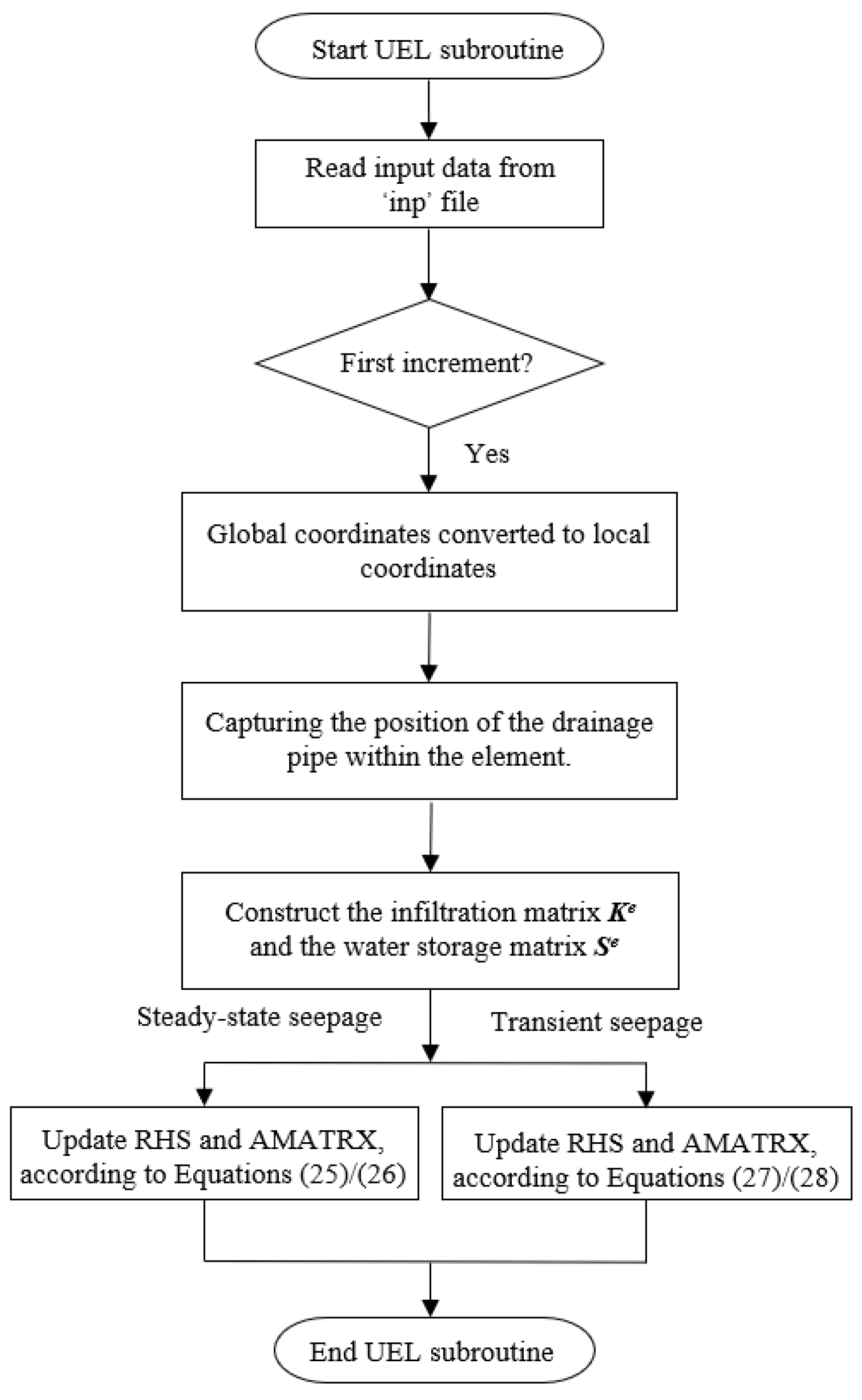
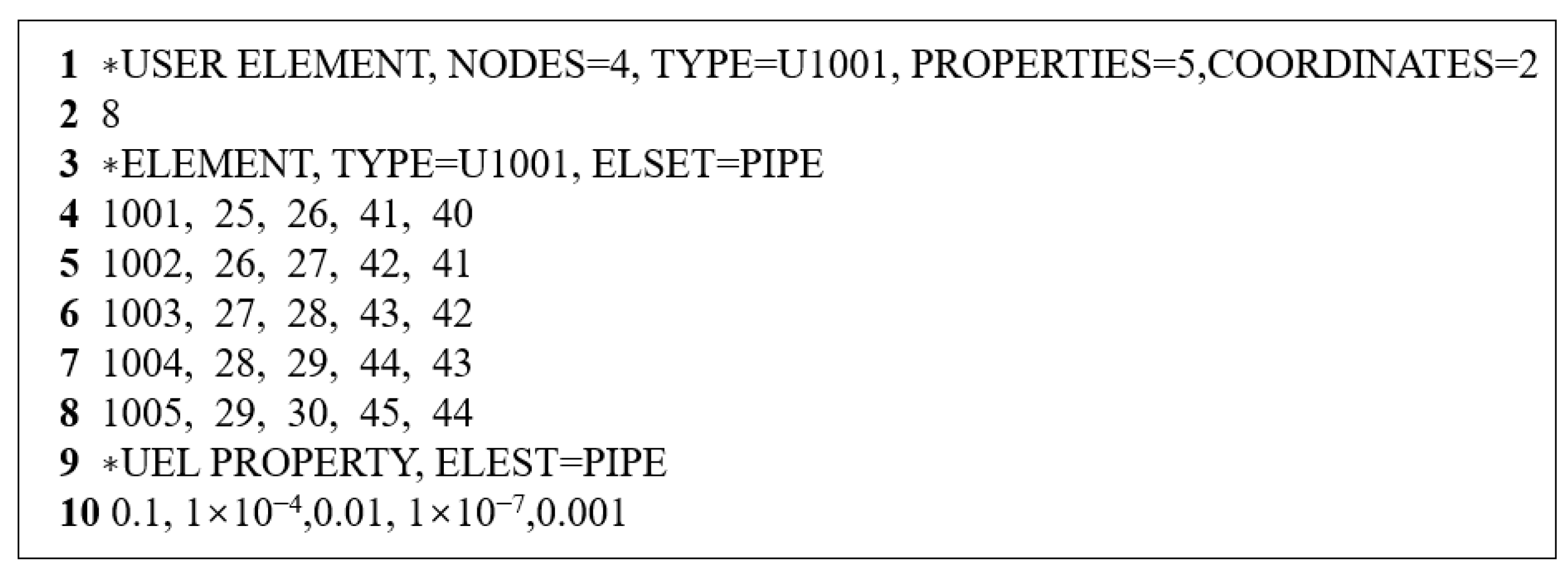
3. Implementation of the Seepage Element Containing Drainage Pipe in Abaqus
4. Validation and Analysis
4.1. Steady Seepage Simulation with a New Element Containing Drainage Pipe for a Dyke Dam
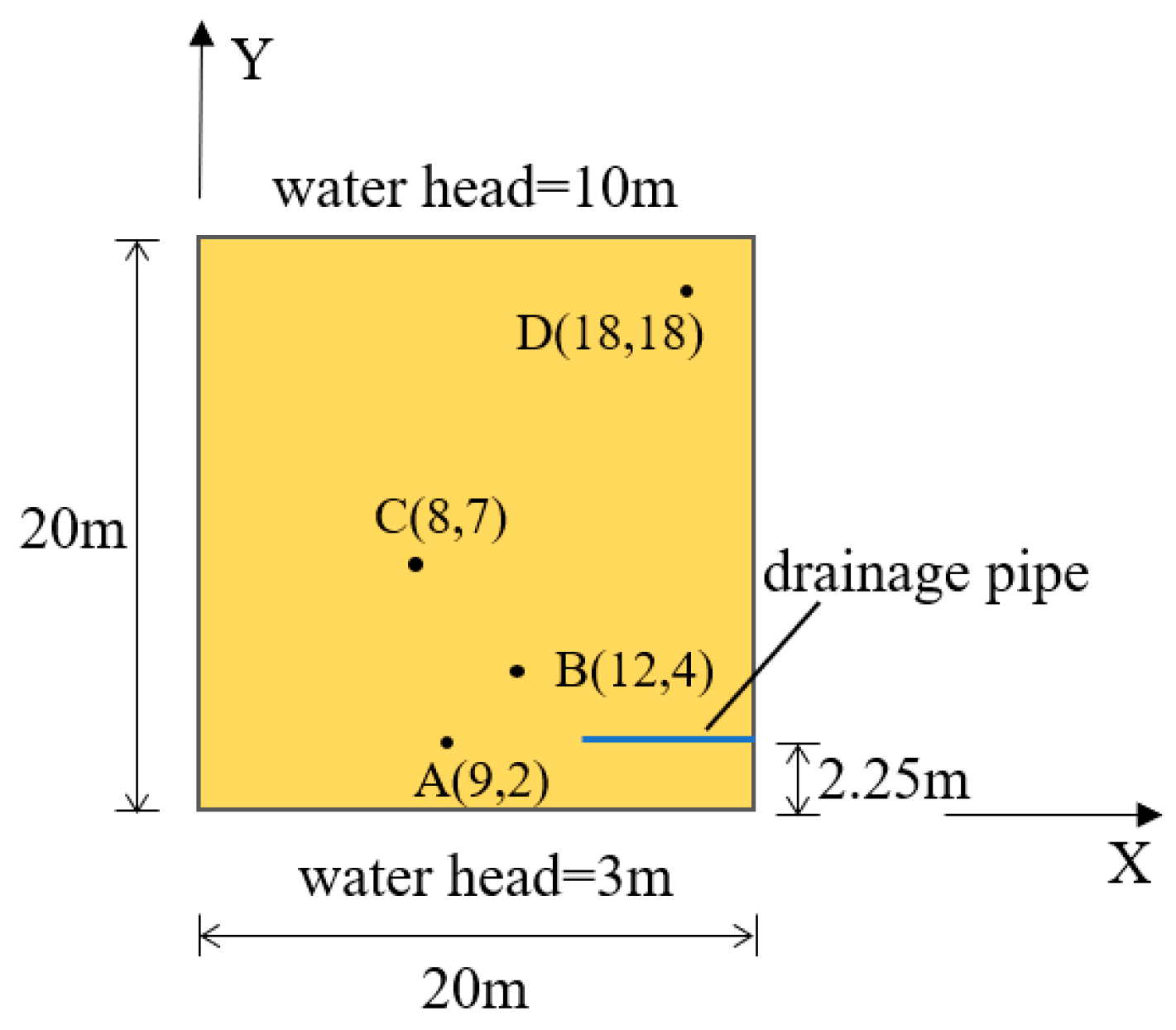
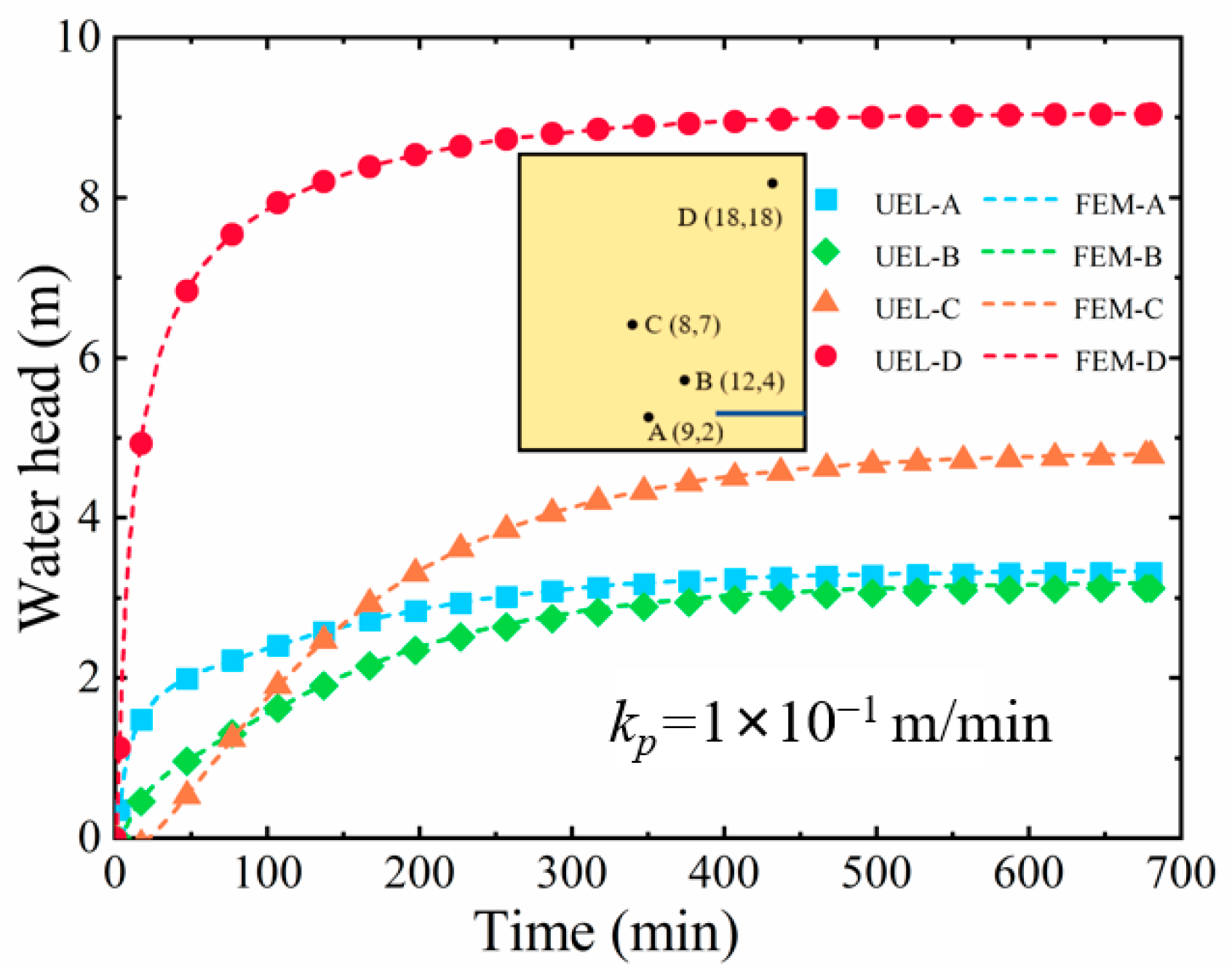
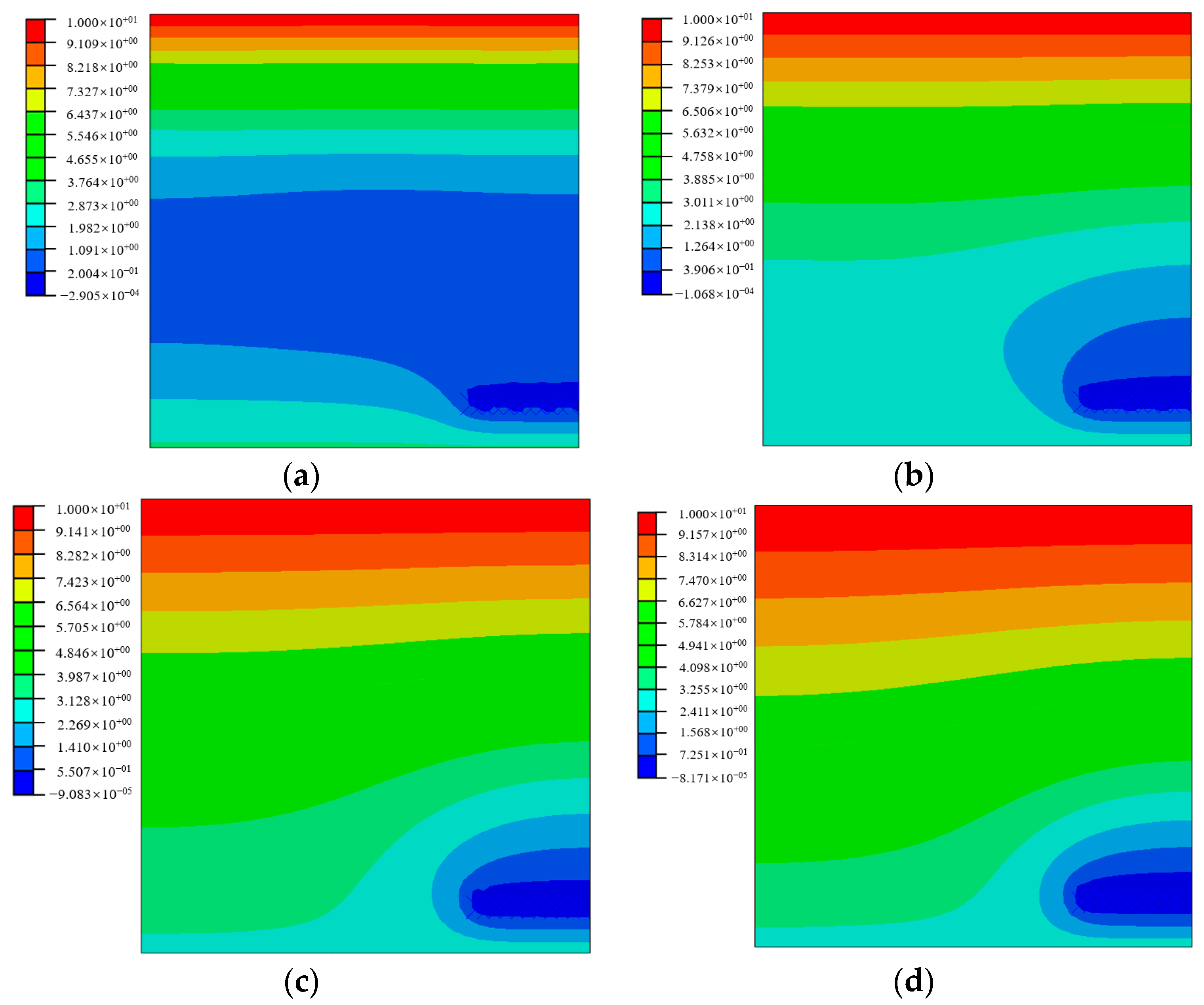
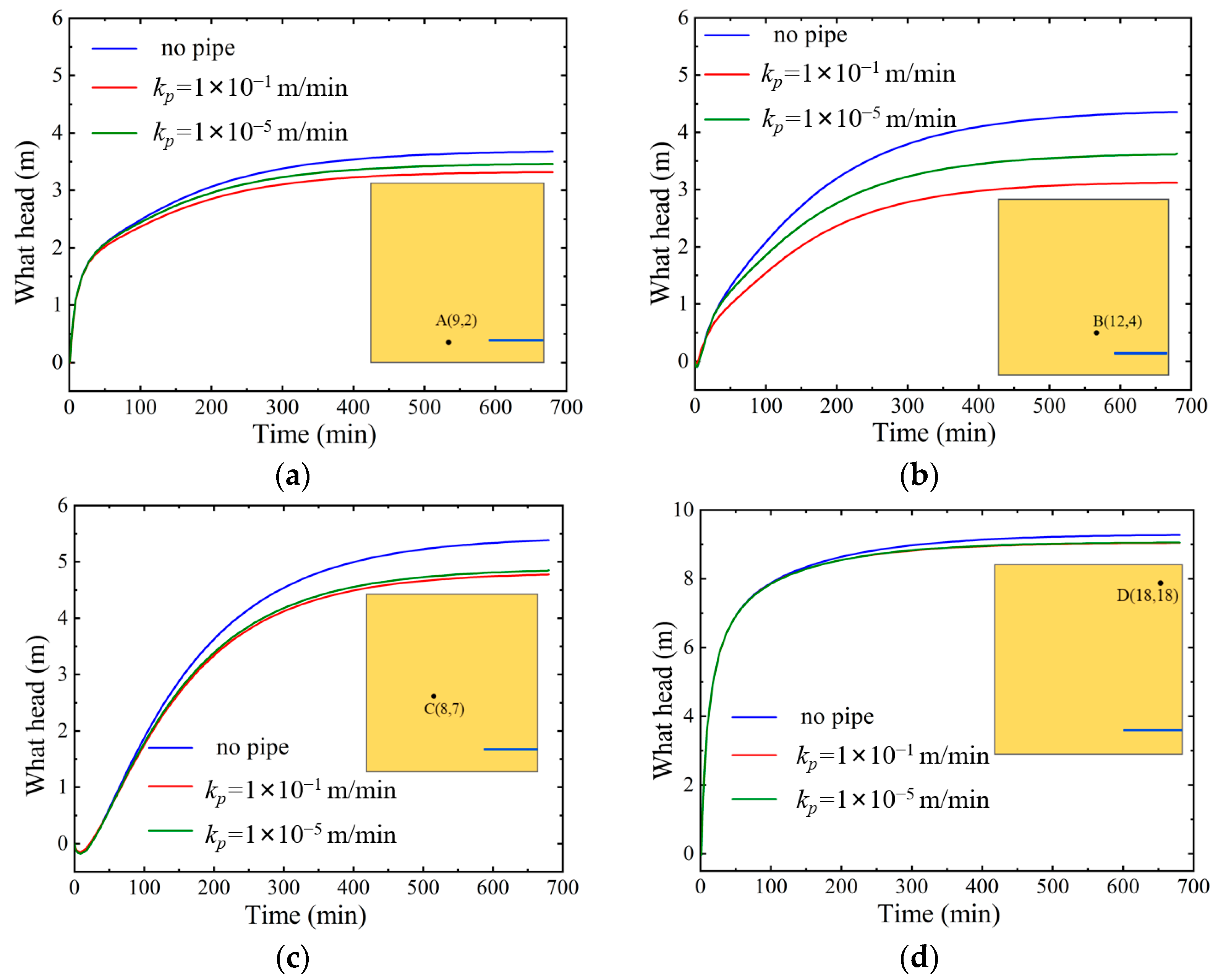
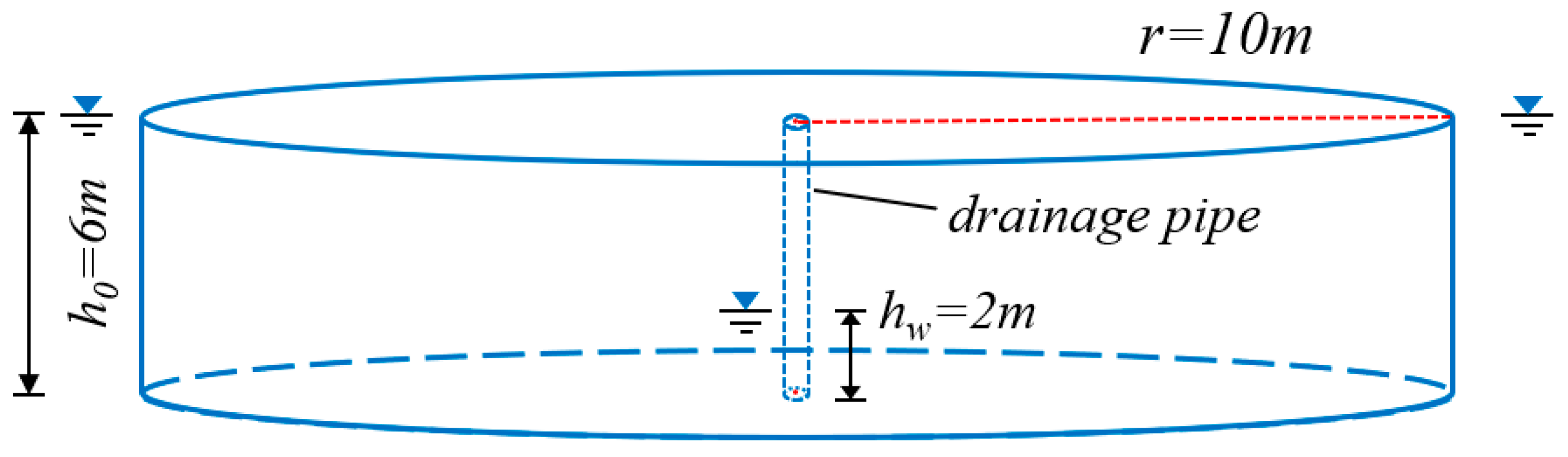
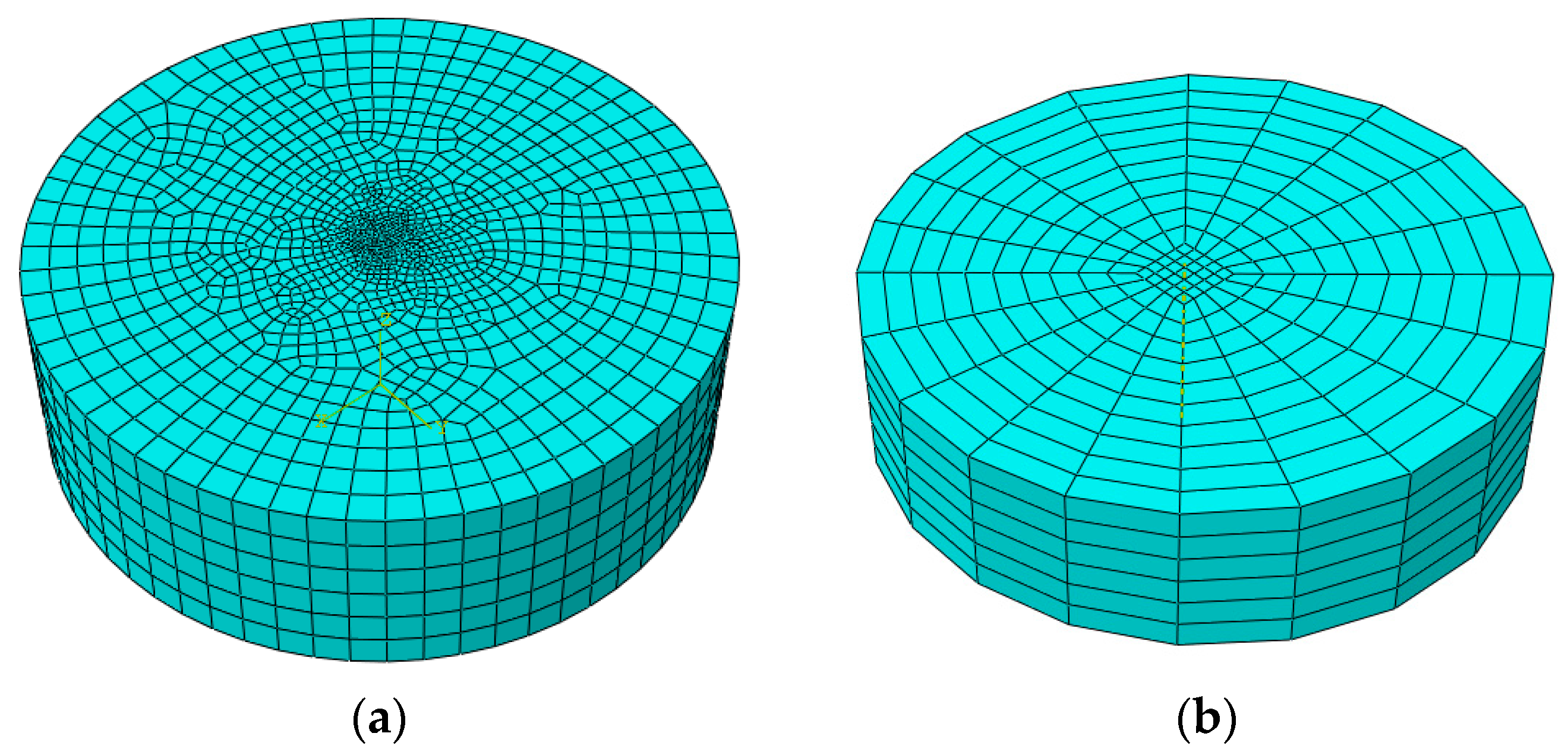
4.2. Transient State Seepage Simulation with a New Element Containing Drainage Pipe
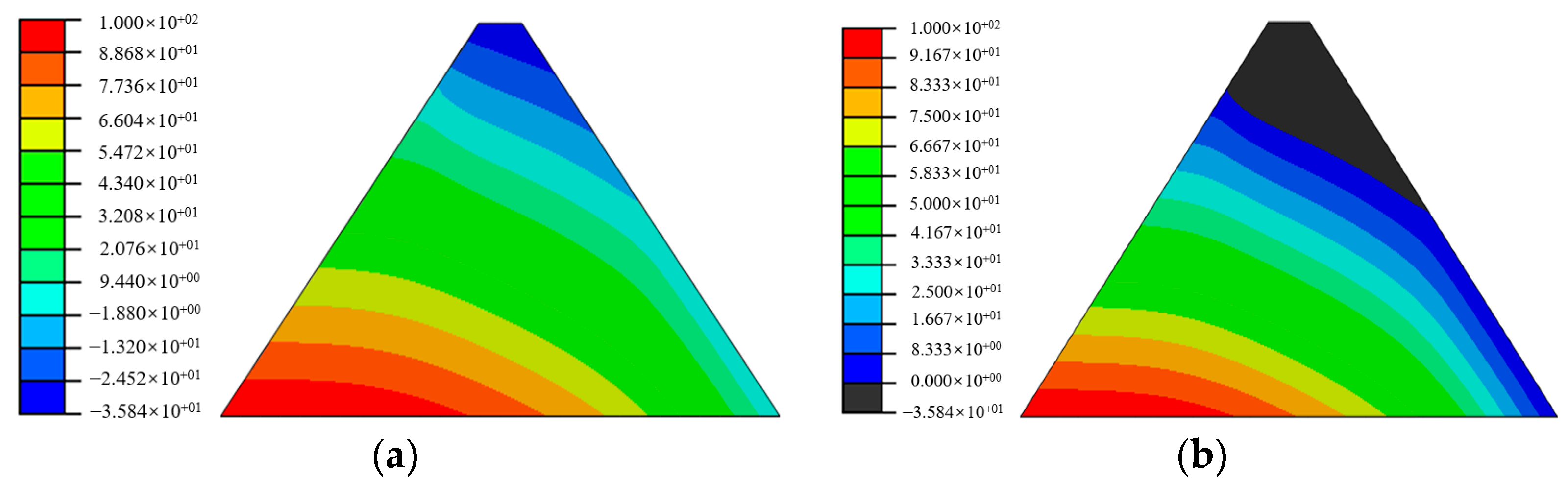
4.3. Comparison and Validation of the Element Containing Drainage Pipe in a Three-Dimensional Seepage Field
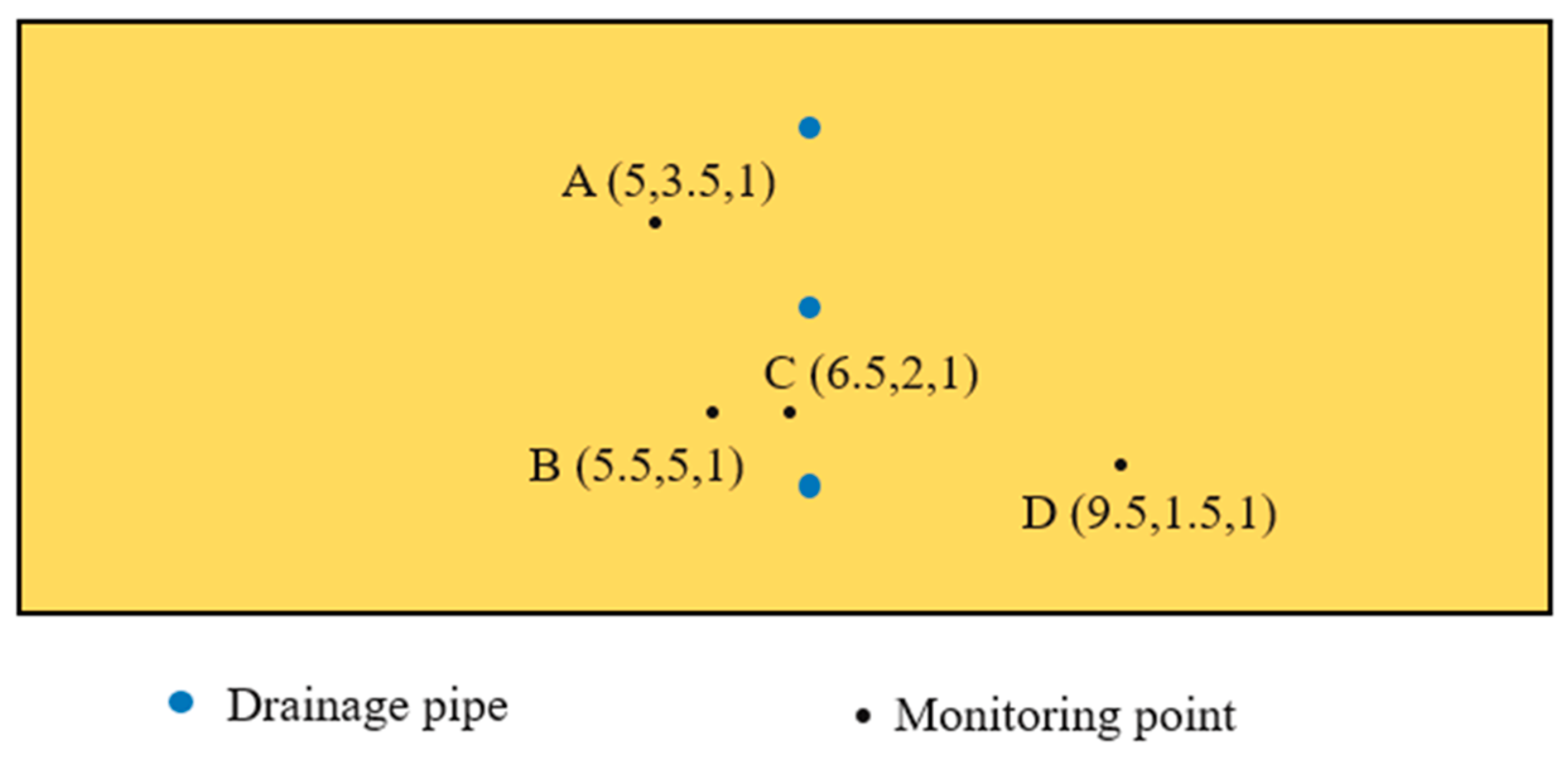
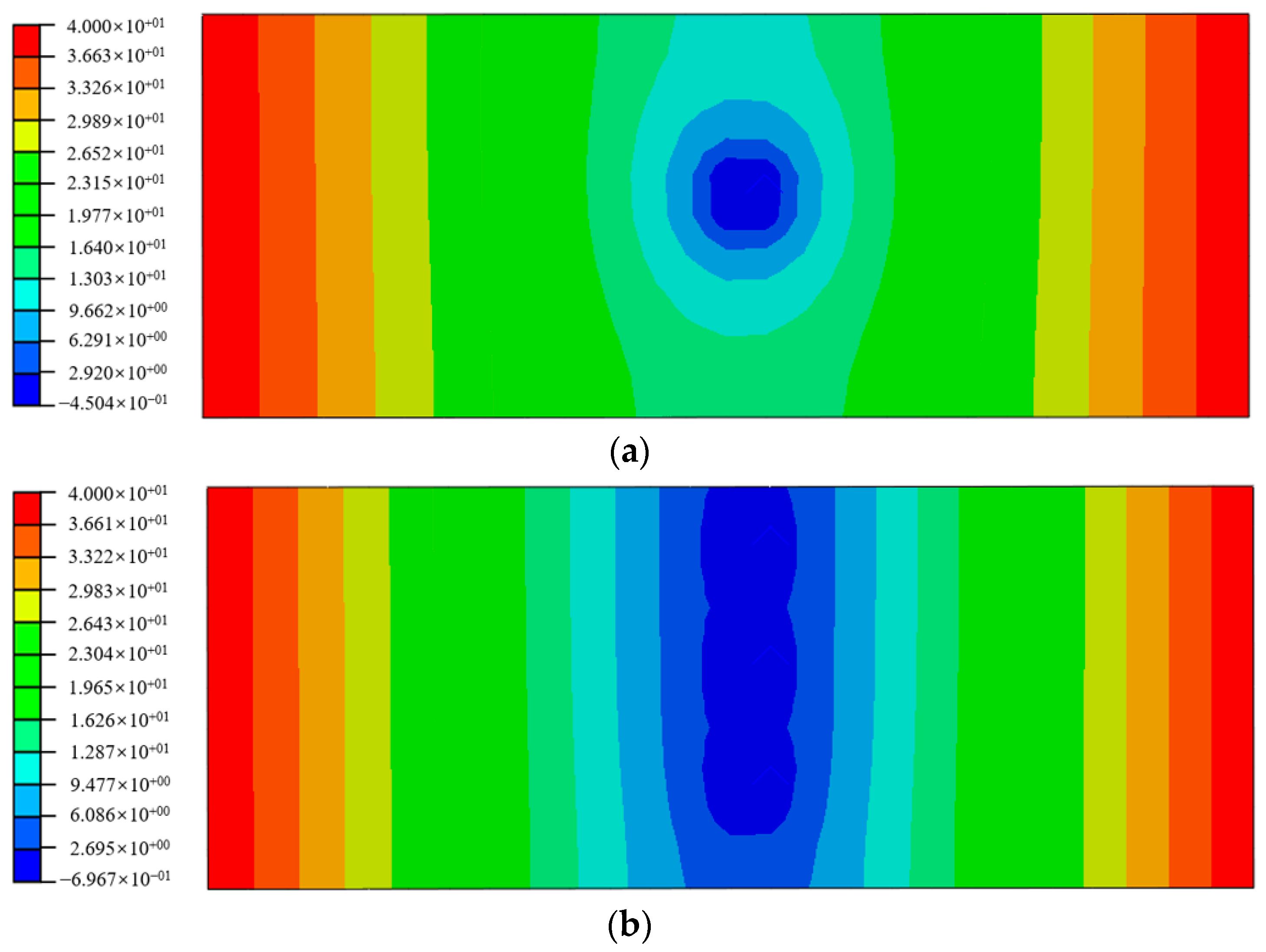
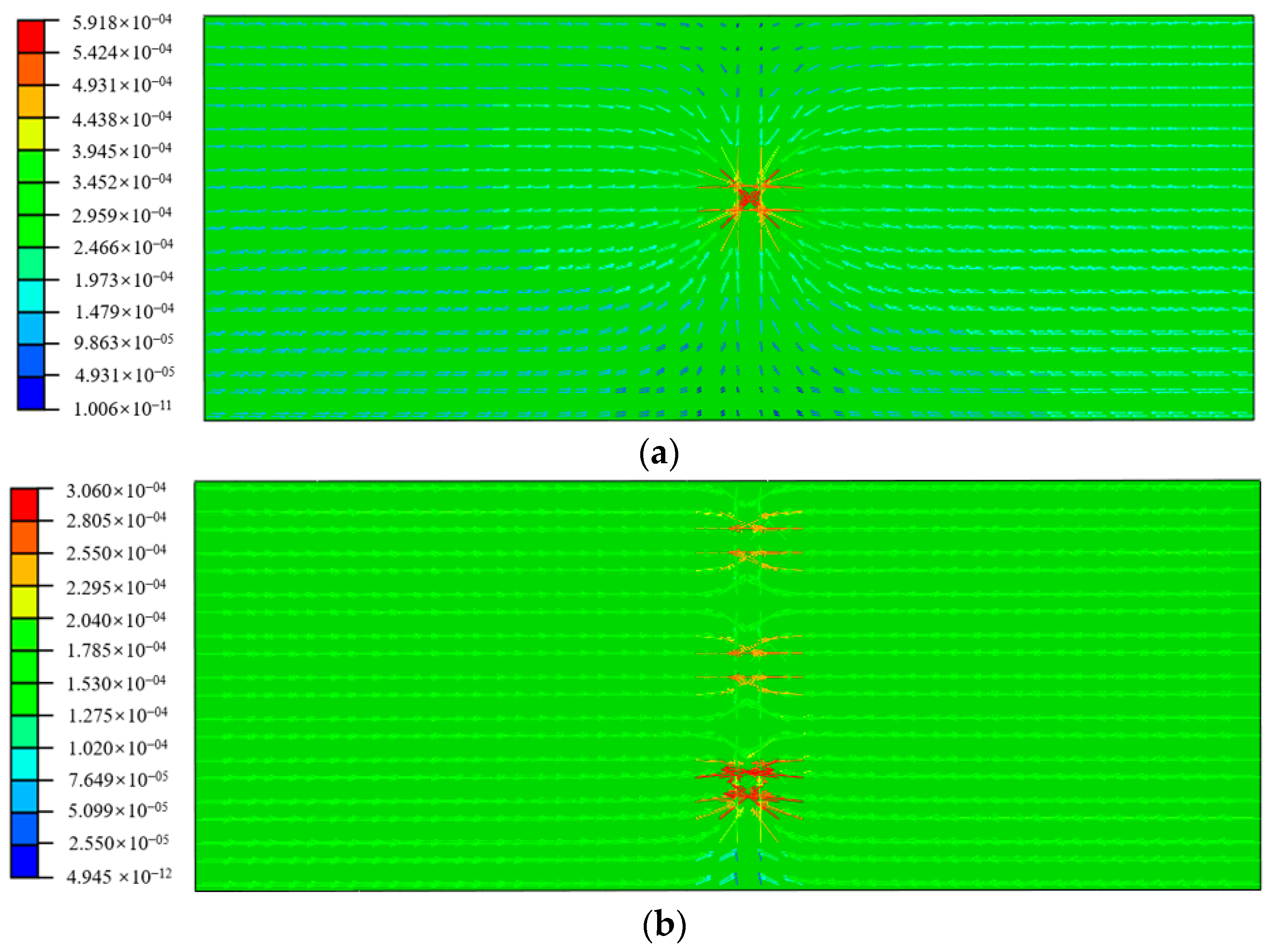
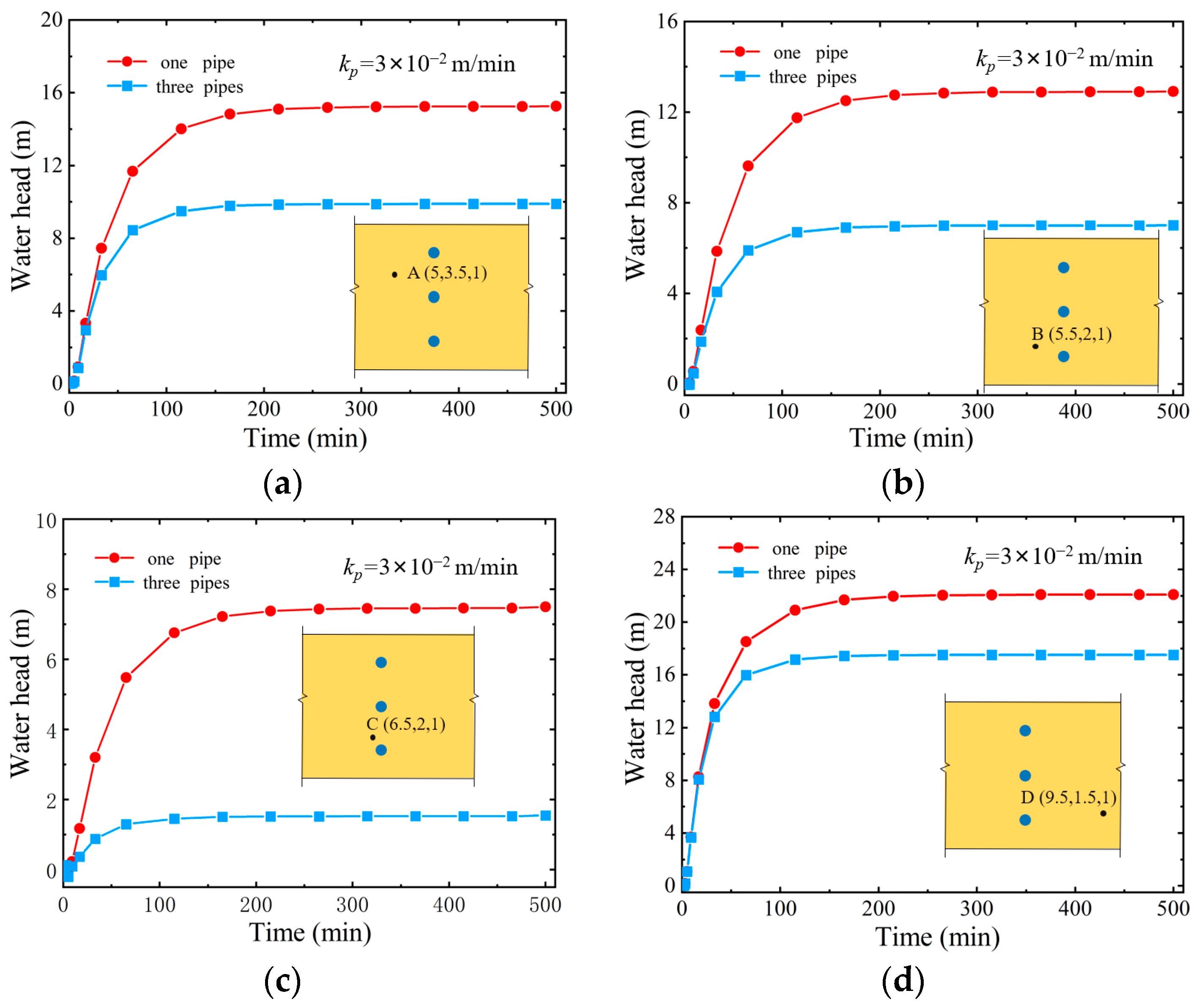
4.4. The Three-Dimensional Seepage Analysis of a Rock Mass Containing Drainage Pipes
5. Conclusions
- (1)
- In the proposed seepage element, the seepage matrix includes the drainage discharge contribution of the drainage pipe, and the element nodal seepage load array includes the permeable conductivity contribution of the drainage pipe. Therefore, in the newly proposed element model, it not only finely reflects the permeable conduction mechanism of the drainage pipe but also avoids the mesh meshing of the drainage pipe, ensuring calculation accuracy and improving calculation efficiency.
- (2)
- Taking the simulation of seepage in steady-state dyke dam, transient soil, and rock mass as examples, the reliability of the proposed element model and the correctness of the secondary development program UEL connecting to the Abaqus software are verified through comparative analysis with the calculation results of the traditional finite element model, providing convenience for the efficient use of the proposed seepage element containing drainage pipe.
- (3)
- By tracking the water head or pore pressure values of monitoring points near drainage pipes, it is found that the points closer to the drainage pipe are more affected by the drainage pipe, resulting in greater pressure release and smaller head values, while the points further away from the drainage pipe have a weaker impact, resulting in a higher water head. In addition, under the influence of gravity, the drainage pipe has a greater impact on the points above it.
- (4)
- The transient seepage of a three-dimensional rock mass with three drainage pipes under a fixed water head boundary is efficiently simulated using the proposed seepage element. By comparing the simulation results with those of a single drainage pipe, the combined pressure relief effect of multiple drainage pipes is explored. It is found that the pore pressure release at the position between the two pipes is more significant, and as the distance increases, this superimposed effect gradually weakens.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Cong, S.; Tang, L.; Ling, X.; Xing, W.; Geng, L.; Li, X.; Li, G.; Li, H. Three-Dimensional Numerical Investigation on the Seepage Field and Stability of Soil Slope Subjected to Snowmelt Infiltration. Water 2021, 13, 2729. [Google Scholar] [CrossRef]
- Liu, W.; Luo, X.; Huang, F.; Fu, M. Uncertainty of the Soil–Water Characteristic Curve and Its Effects on Slope Seepage and Stability Analysis under Conditions of Rainfall Using the Markov Chain Monte Carlo Method. Water 2017, 9, 758. [Google Scholar] [CrossRef]
- Chen, L.; Xi, B.; Dong, Y.; He, S.; Shi, Y.; Gao, Q.; Liu, K.; Zhao, N. Study on the Stability and Seepage Characteristics of Underwater Shield Tunnels under High Water Pressure Seepage. Sustainability 2023, 15, 15581. [Google Scholar] [CrossRef]
- Zhang, C.; Chai, J.; Cao, J.; Xu, Z.; Qin, Y.; Lv, Z. Numerical Simulation of Seepage and Stability of Tailings Dams: A Case Study in Lixi, China. Water 2020, 12, 742. [Google Scholar] [CrossRef]
- Yu, J.; Li, D.; Zheng, J.; Zhang, Z.; He, Z.; Fan, Y. Analytical Study on the Seepage Field of Different Drainage and Pressure Relief Options for Tunnels in High Water-Rich Areas. Tunn. Undergr. Space Technol. 2023, 134, 105018. [Google Scholar] [CrossRef]
- Li, X.; Li, D. A Numerical Procedure for Unsaturated Seepage Analysis in Rock Mass Containing Fracture Networks and Drainage Holes. J. Hydrol. 2019, 574, 23–34. [Google Scholar] [CrossRef]
- Aranda, J.Á.; Beneyto, C.; Sánchez-Juny, M.; Bladé, E. Efficient Design of Road Drainage Systems. Water 2021, 13, 1661. [Google Scholar] [CrossRef]
- Sharma, V.; Fujisawa, K.; Murakami, A. Space-Time Finite Element Method for Transient and Unconfined Seepage Flow Analysis. Finite Elem. Anal. Des. 2021, 197, 103632. [Google Scholar] [CrossRef]
- Sun, J.; Sun, H.; Lu, M.; Han, B. Finite-Difference Solution for Dissipation of Excess Pore Water Pressure within Liquefied Soil Stabilized by Stone Columns with Consideration of Coupled Radial-Vertical Seepage. Soil Dyn. Earthq. Eng. 2024, 176, 108328. [Google Scholar] [CrossRef]
- Zhang, Y.T. Solving the seepage field with drainage holes using boundary elements. J. Hydraul. Eng. 1982, 7, 36–40. (In Chinese) [Google Scholar]
- Fipps, G.; Skaggs, R.W.; Nieber, J.L. Drains as a Boundary Condition in Finite Elements. Water Resour. Res. 1986, 22, 1613–1621. [Google Scholar] [CrossRef]
- Du, Y.L.; Xu, G.-A.; Huang, Y.H. The study of three-dimensional seepage analysis for the complex rock foundation. J. Hydraul. Eng. 1984, 8, 1–9. (In Chinese) [Google Scholar]
- Wang, J.; Jiang, H.X. Simulating drainage holes with line sink drainage element. Chin. J. Geotech. Eng. 2008, 30, 677–684. (In Chinese) [Google Scholar]
- Wang, L.; Liu, Z.; Zhang, Y.T. Analysis of seepage field near a drainage holes curtain. J. Hydraul. Eng. 1992, 12, 15–20. (In Chinese) [Google Scholar]
- Chen, Y.F.; Zhou, C.B.; Zheng, H. A numerical solution to seepage problems with complex drainage systems. Comput. Geotech. 2008, 35, 383–393. [Google Scholar] [CrossRef]
- Xu, Z.G.; Cao, C.; Li, K.H.; Chai, J.R.; Xiong, W.; Zhao, J.Y.; Qin, R.G. Simulation of drainage hole arrays and seepage control analysis of the Qingyuan Pumped Storage Power Station in China: A case study. Bull. Eng. Geol. Environ. 2019, 78, 6335–6346. [Google Scholar] [CrossRef]
- Su, H.Z.; Li, J.Y.; Wen, Z.P.; Guo, Z.Y.; Zhou, R.L. Integrated certainty and uncertainty evaluation approach for seepage control effectiveness of a gravity dam. Appl. Math. Model. 2019, 65, 1–22. [Google Scholar] [CrossRef]
- Bai, C.S.; Chai, J.R.; Xu, Z.G.; Qin, Y. Numerical simulation of drainage holes and performance evaluation of the seepage control of gravity dam: A case study of Heihe reservoir in China. Arab. J. Sci. Eng. 2022, 47, 4801–4819. [Google Scholar] [CrossRef]
- Zhu, B.F.; Li, Y.; Xu, P. Substitution of the curtain of drainage holes by a seeping layer in the three dimensional finite element analysis of seepage field. Water Resour. Hydropower Eng. 2007, 38, 24–28. (In Chinese) [Google Scholar]
- Hu, J.; Chen, S.H. Air element method for modeling the drainage hole in seepage analysis. Rock Soil Mech. 2003, 24, 281–287. (In Chinese) [Google Scholar]
- Chen, S.H.; Shahrour, I. Composite Element Method for the Bolted Discontinuous Rock Masses and Its Application. Int. J. Rock Mech. Min. Sci. 2008, 45, 384–396. [Google Scholar] [CrossRef]
- Chen, S.H.; Feng, X.M.; Isam, S. Numerical Estimation of REV and Permeability Tensor for Fractured Rock Masses by Composite Element Method. Num. Anal. Methods Geomech. 2008, 32, 1459–1477. [Google Scholar] [CrossRef]
- Chen, S.H.; Qiang, S.; Shahrour, I.; Egger, P. Composite Element Analysis of Gravity Dam on a Complicated Rock Foundation. Int. J. Geomech. 2008, 8, 275–284. [Google Scholar] [CrossRef]
- Chen, S.H.; Xue, L.L.; Xu, G.S.; Shahrour, I. Composite element method for the seepage analysis of rock masses containing fractures and drainage holes. Int. J. Rock Mech. Min. Sci. 2010, 47, 762–770. [Google Scholar] [CrossRef]
- Chen, S.H.; Feng, X.M. Composite element model for rock mass seepage flow. J. Hydrodynam. (Ser. B) 2006, 18, 219–224. [Google Scholar]
- Vincent, K.K.; Muthama, M.N.; Muoki, S.N. Darcy’s law equation with application to underground seepage in earth dams in calculation of the amount of seepage. Am. J. Appl. Math. Stat. 2014, 2, 143–149. [Google Scholar] [CrossRef]
- Lai, Y.M.; Wu, Z.; Zhu, Y.; Zhu, L. Nonlinear analysis for the coupled problem of temperature and seepage fields in cold regions tunnels. Cold Reg. Sci. Technol. 1999, 29, 89–96. [Google Scholar] [CrossRef]
- Zhou, Z.; Ling, T.; Huang, F.; Zhang, M. The Face Stability Analysis of Shield Tunnels Subjected to Seepage Based on the Variational Principle. Sustainability 2022, 14, 16538. [Google Scholar] [CrossRef]
- Wise, J.; Hunt, S.; Al Dushaishi, M. Prediction of Earth Dam Seepage Using a Transient Thermal Finite Element Model. Water 2023, 15, 1423. [Google Scholar] [CrossRef]
- Fukuchi, T. Numerical Analyses of Steady-State Seepage Problems Using the Interpolation Finite Difference Method. Soils Found. 2016, 56, 608–626. [Google Scholar] [CrossRef]
- Lipnikov, K.; Manzini, G.; Svyatskiy, D. Analysis of the Monotonicity Conditions in the Mimetic Finite Difference Method for Elliptic Problems. J. Comput. Phys. 2011, 230, 2620–2642. [Google Scholar] [CrossRef]
- Xing, Y.; Yao, L.; Ji, Y. A Solution Strategy Combining the Mode Superposition Method and Time Integration Methods for Linear Dynamic Systems. Acta Mech. Sin. 2022, 38, 521433. [Google Scholar] [CrossRef]
- Yang, Y.; Zhang, Z.; Feng, Y.; Wang, K. A Novel Solution for Seepage Problems Implemented in the Abaqus UEL Based on the Polygonal Scaled Boundary Finite Element Method. Geofluids 2022, 2022, 5797014. [Google Scholar] [CrossRef]
- Kshirsagar, S.; Nguyen-Xuan, H.; Liu, G.R.; Natarajan, S. An SFEM Abaqus UEL for Nonlinear Analysis of Solids. Int. J. Comput. Methods 2023, 20, 2350003. [Google Scholar] [CrossRef]



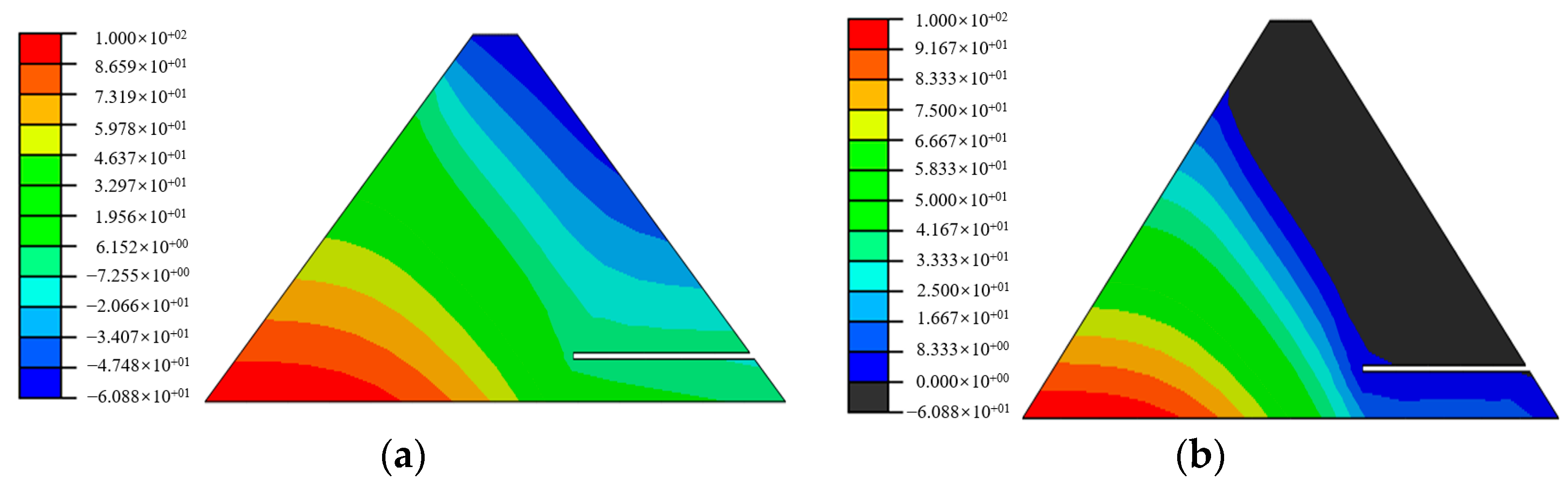
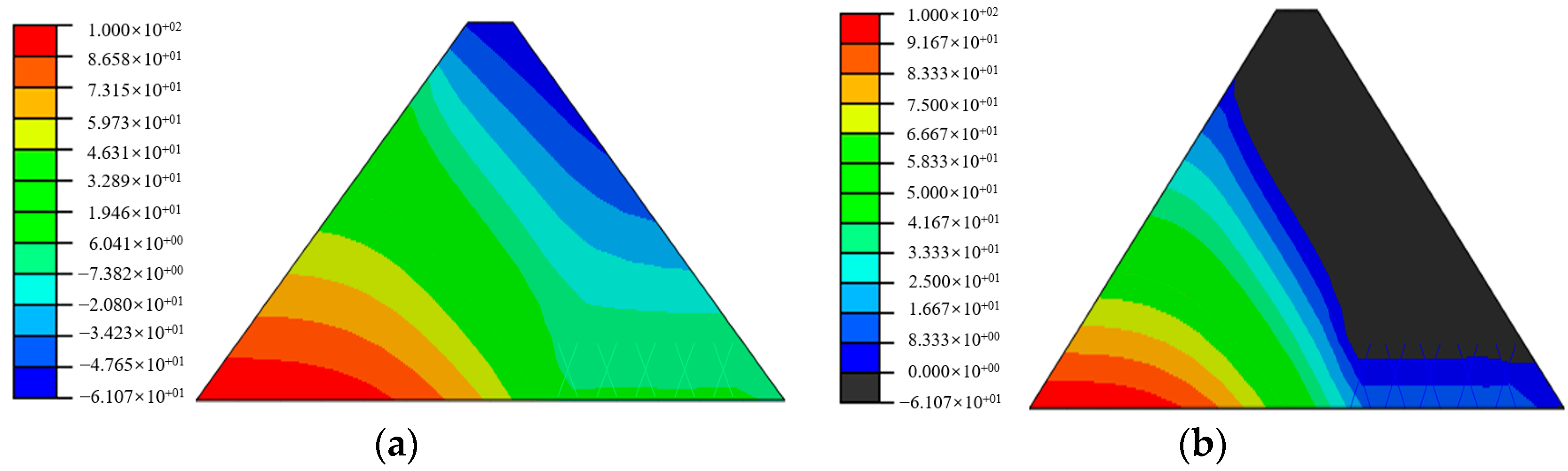



| Method | Case | Monitoring Points | ||||
|---|---|---|---|---|---|---|
| A | B | C | D | E | ||
| Traditional FEM | No drainage pipe layout | 38.5672 | 54.5833 | 27.2837 | 38.5764 | 7.9902 |
| With kp = 1 × 10−4 m/s | −8.5477 | 28.9266 | −9.0734 | 19.9254 | −11.1304 | |
| With kp = 1 × 10−6 m/s | −8.5313 | 28.9302 | −9.0672 | 19.9282 | −11.1271 | |
| UEL | With kp = 1 × 10−4 m/s | −9.0211 | 28.1991 | −9.5175 | 19.5152 | −11.4228 |
| With kp = 1 × 10−6 m/s | −9.0156 | 28.2763 | −9.4996 | 19.5584 | −11.4072 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xia, X.; Xu, X.; Gu, X.; Zhang, Q. An Efficient Seepage Element Containing Drainage Pipe. Water 2024, 16, 1440. https://doi.org/10.3390/w16101440
Xia X, Xu X, Gu X, Zhang Q. An Efficient Seepage Element Containing Drainage Pipe. Water. 2024; 16(10):1440. https://doi.org/10.3390/w16101440
Chicago/Turabian StyleXia, Xiaozhou, Xinxiang Xu, Xin Gu, and Qing Zhang. 2024. "An Efficient Seepage Element Containing Drainage Pipe" Water 16, no. 10: 1440. https://doi.org/10.3390/w16101440
APA StyleXia, X., Xu, X., Gu, X., & Zhang, Q. (2024). An Efficient Seepage Element Containing Drainage Pipe. Water, 16(10), 1440. https://doi.org/10.3390/w16101440






