Large Scale Experimental Study on Waves and Submerged Horizontal Cylinders
Abstract
:1. Introduction
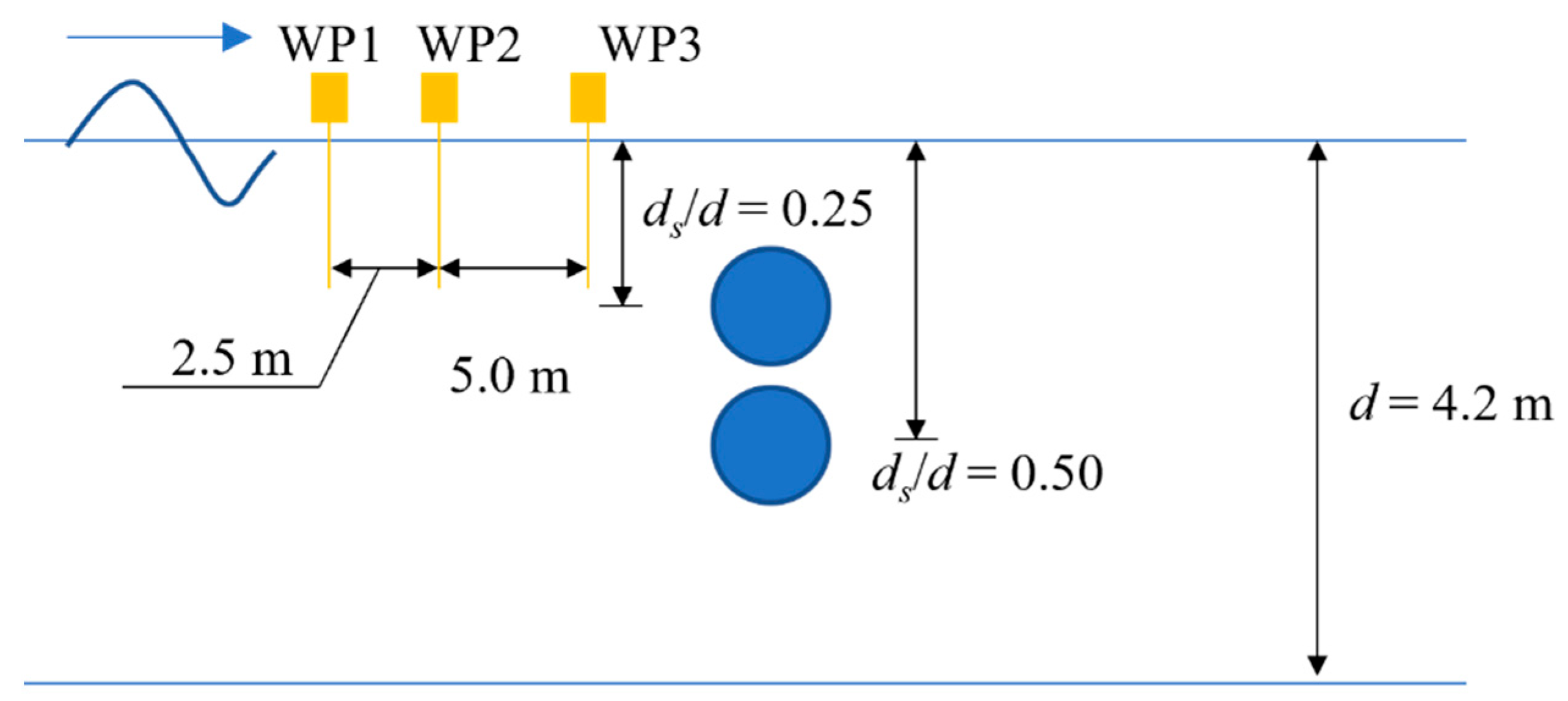
2. Physical Model Tests
3. Test Results and Analysis
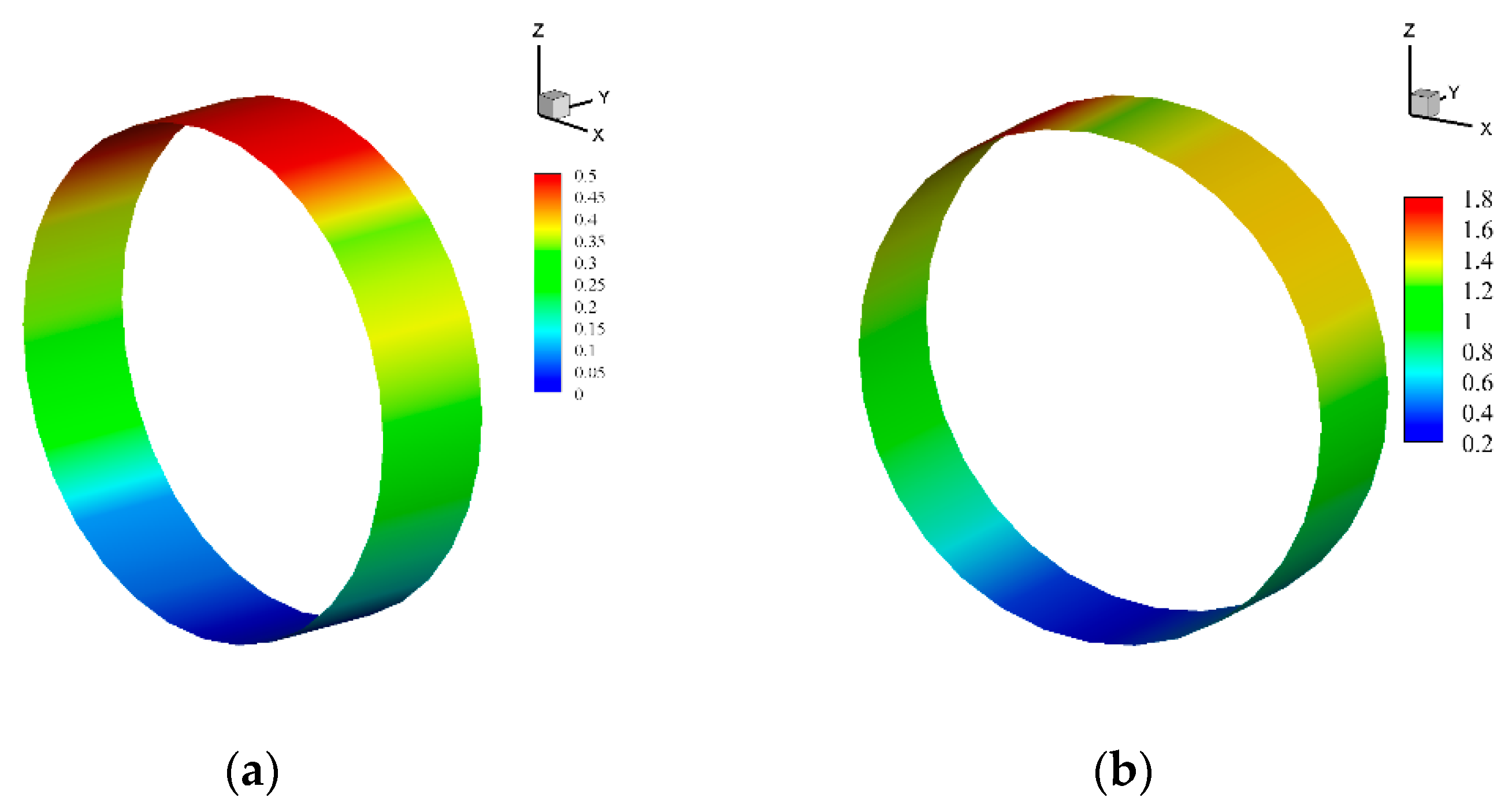
3.1. Dynamic Pressure on the Cylinder Surface
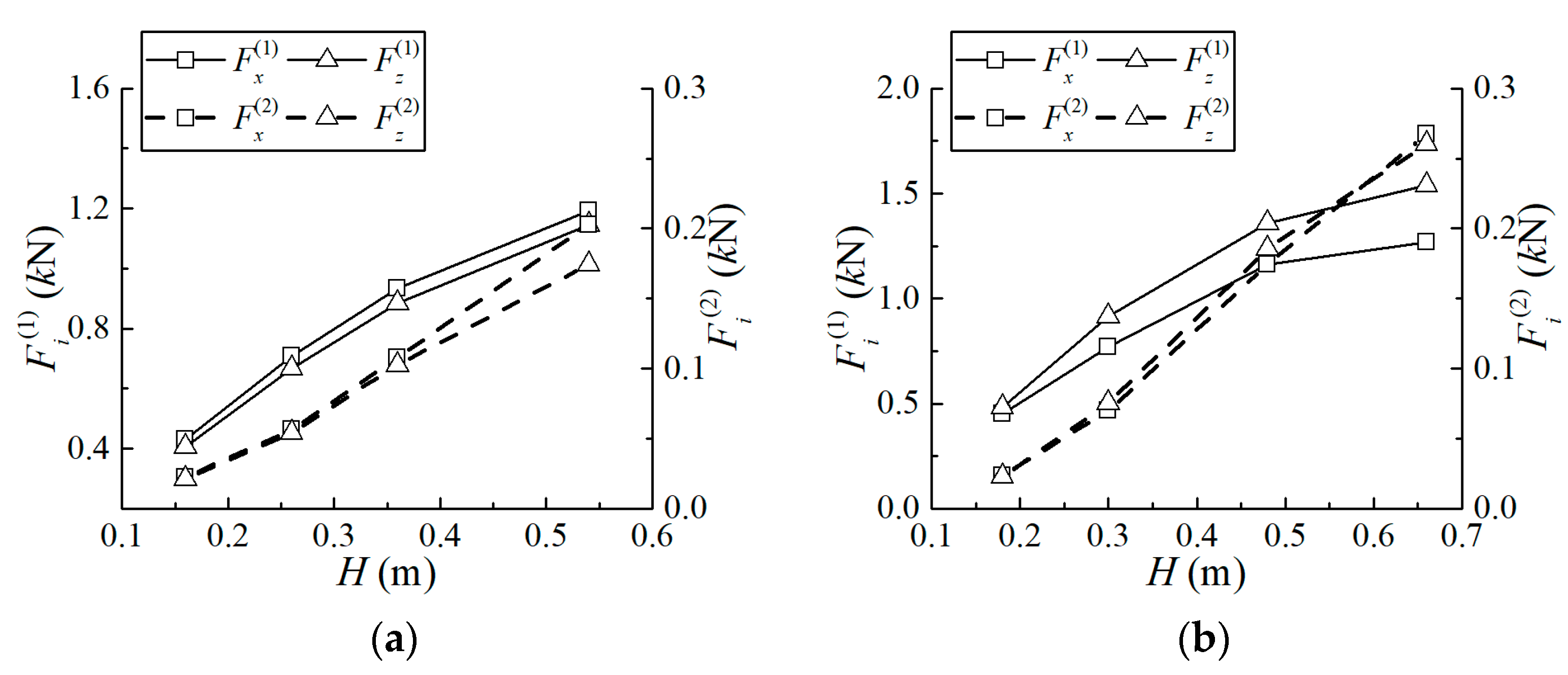
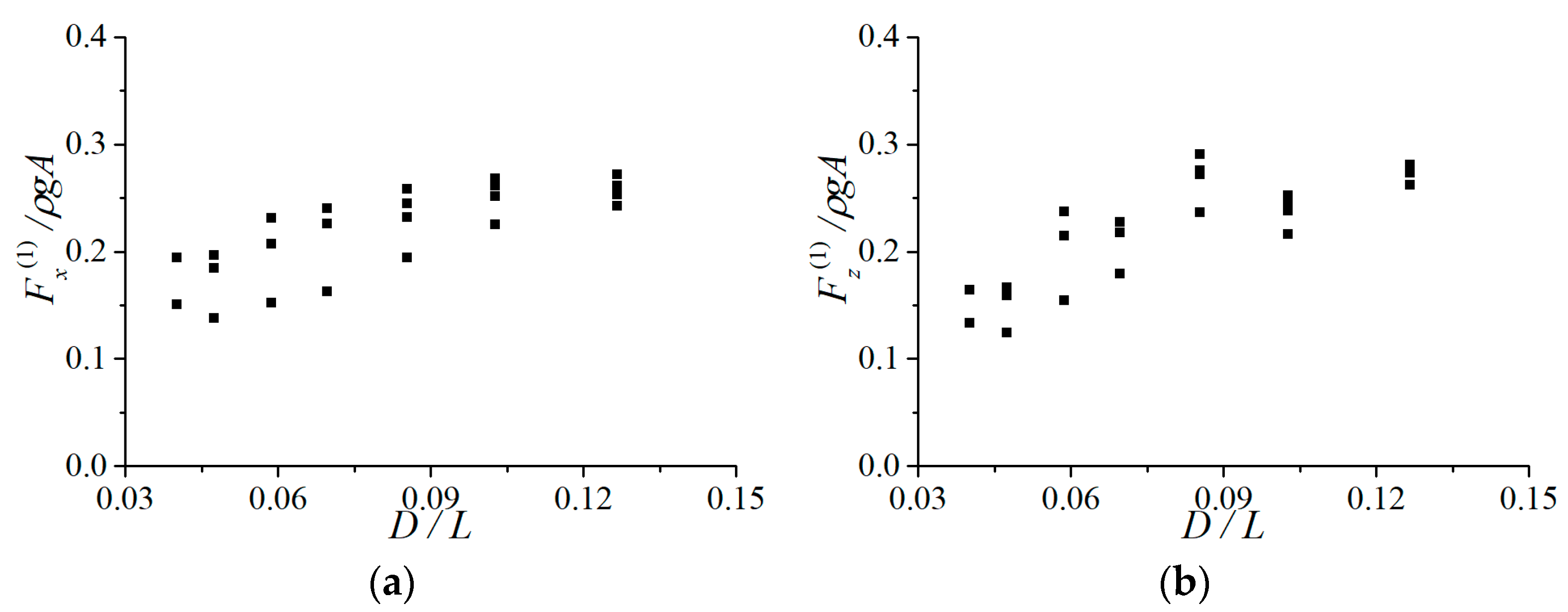
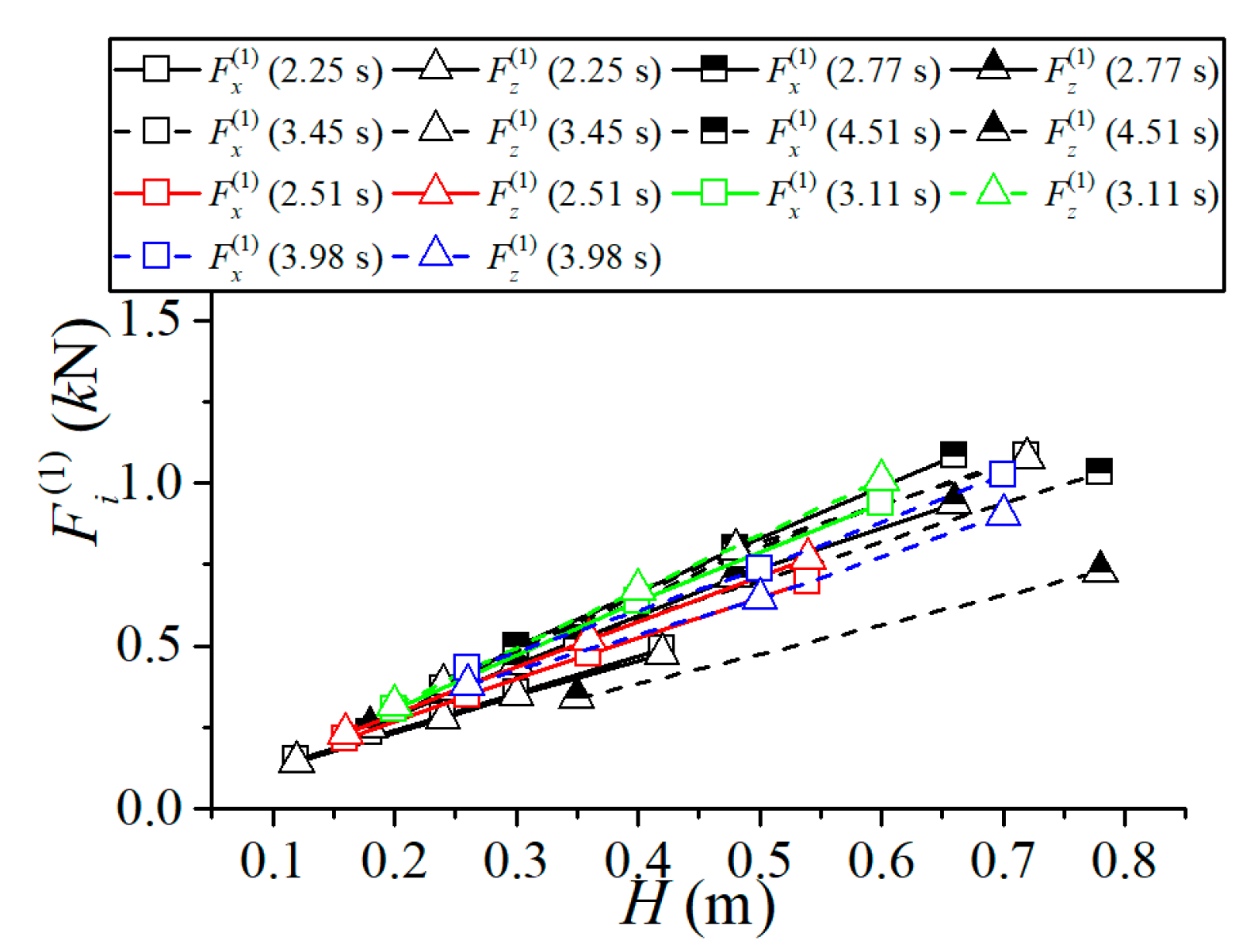
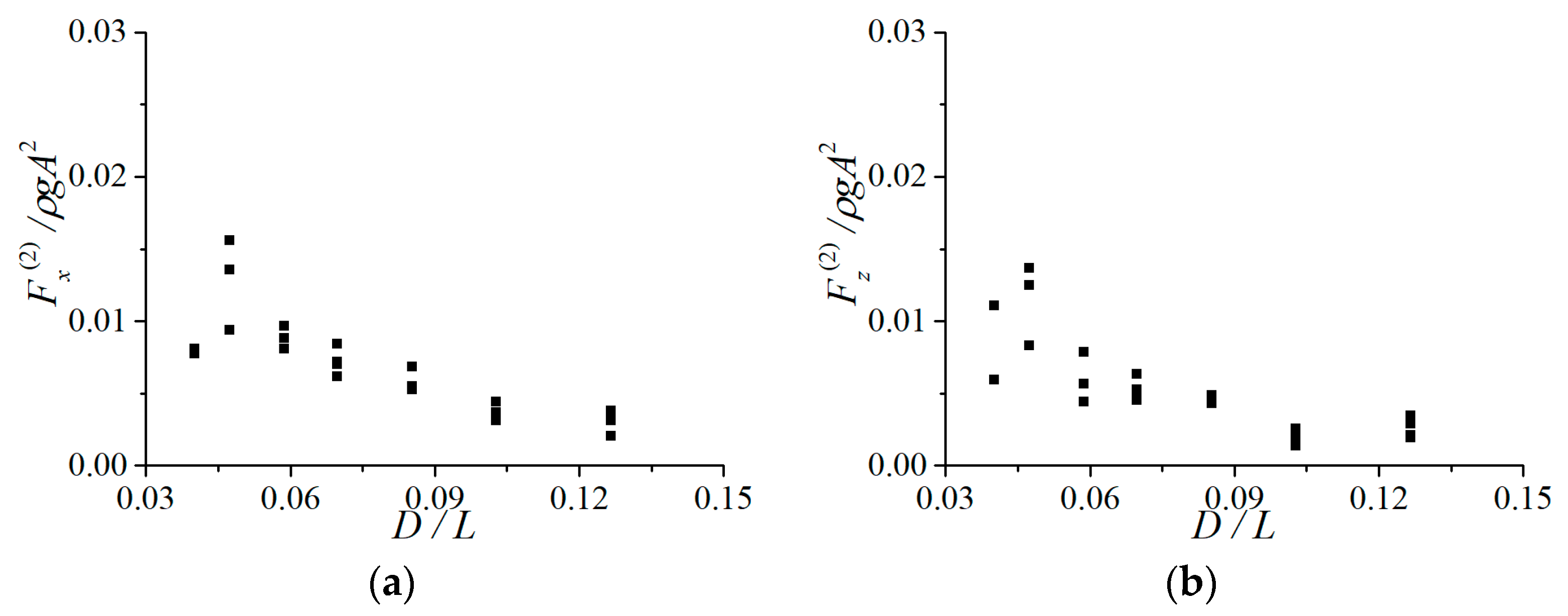
3.2. Wave Force Analysis of Submerged Cylinder
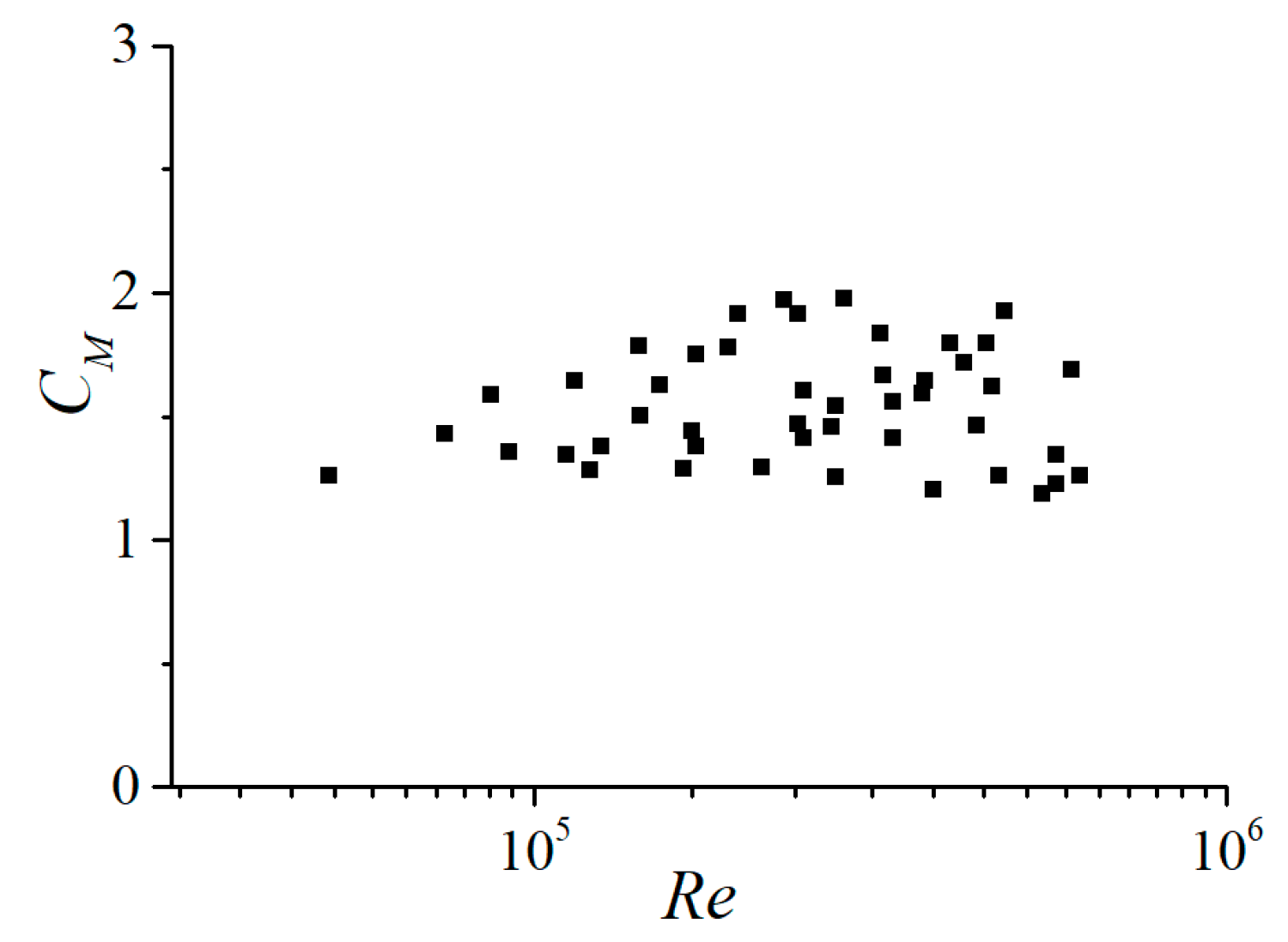
3.3. Morison Formula Inertia Value
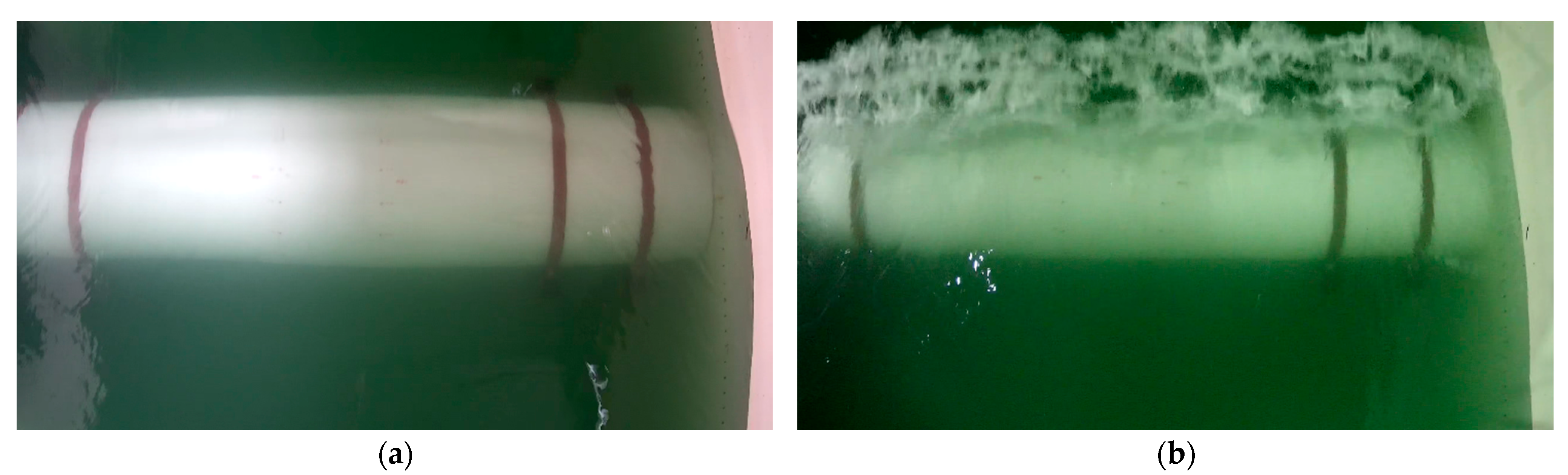
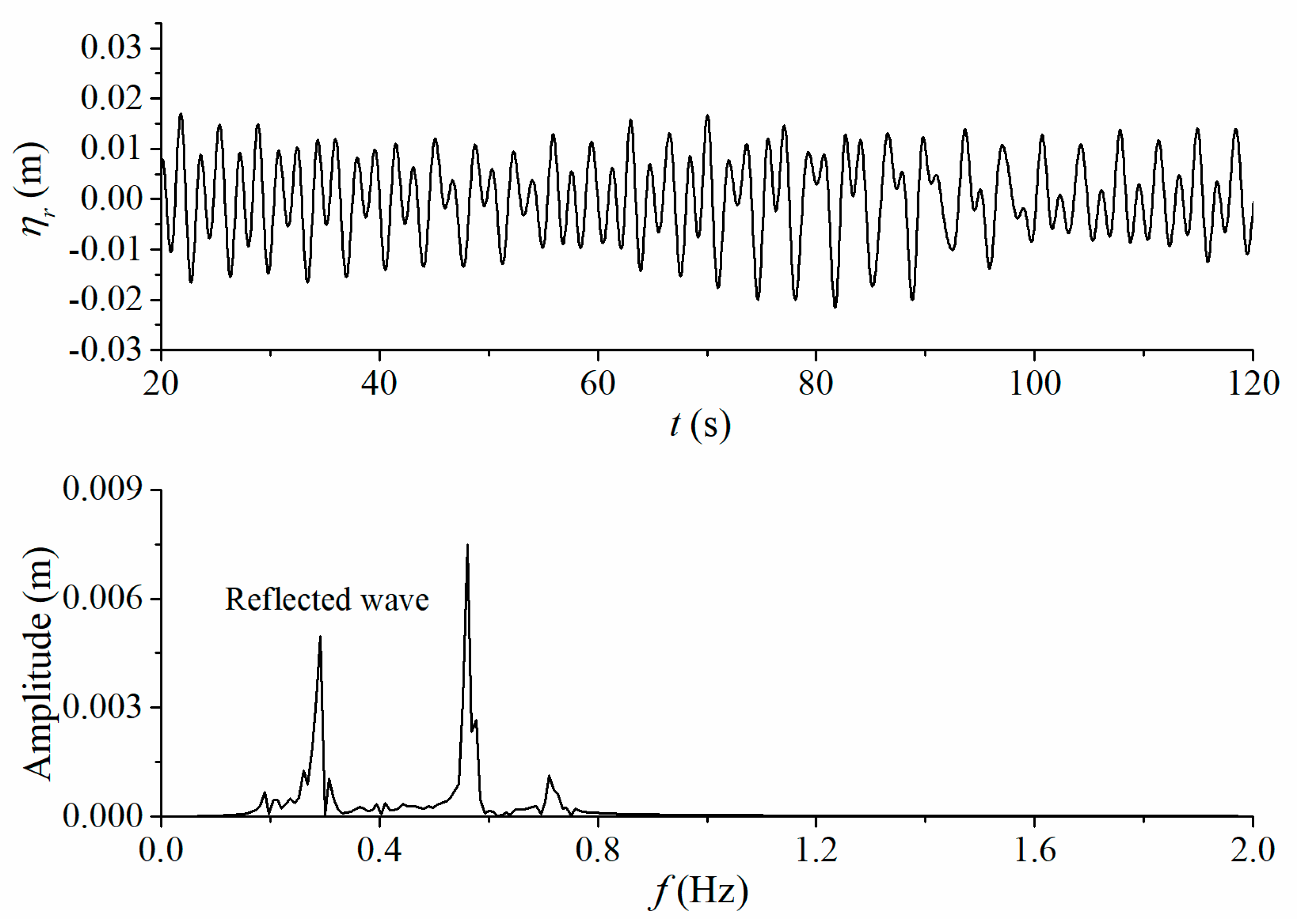
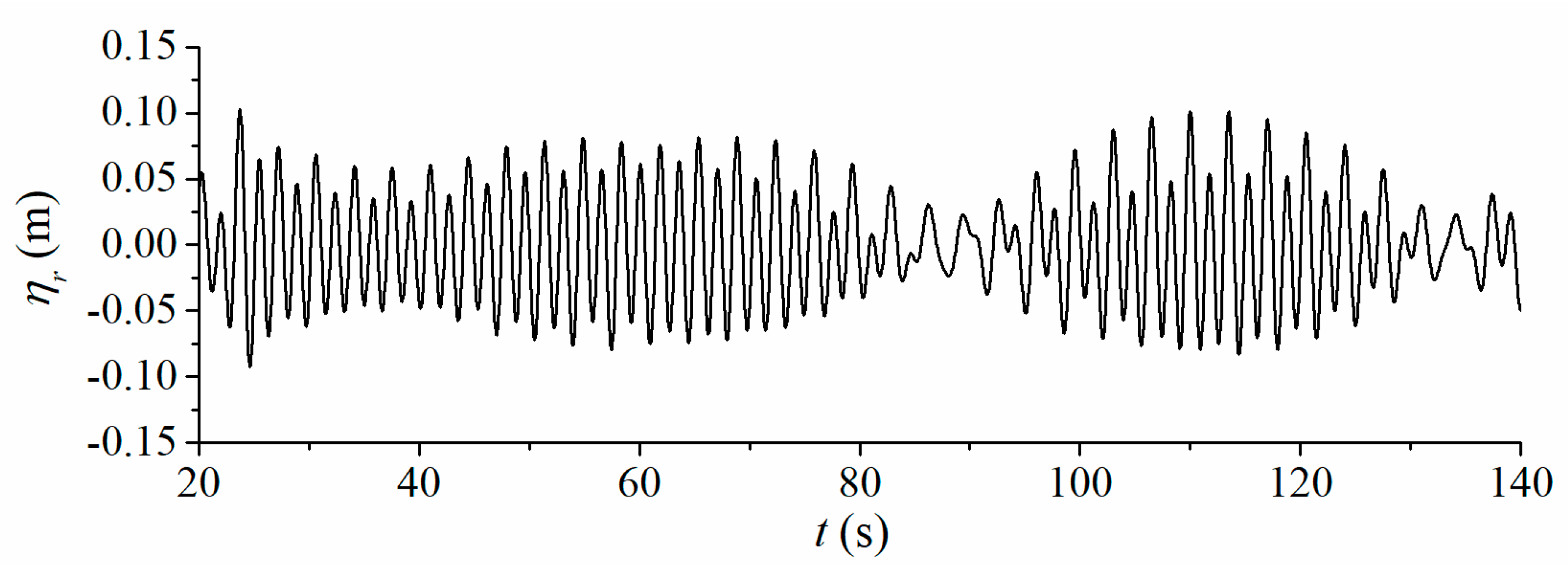
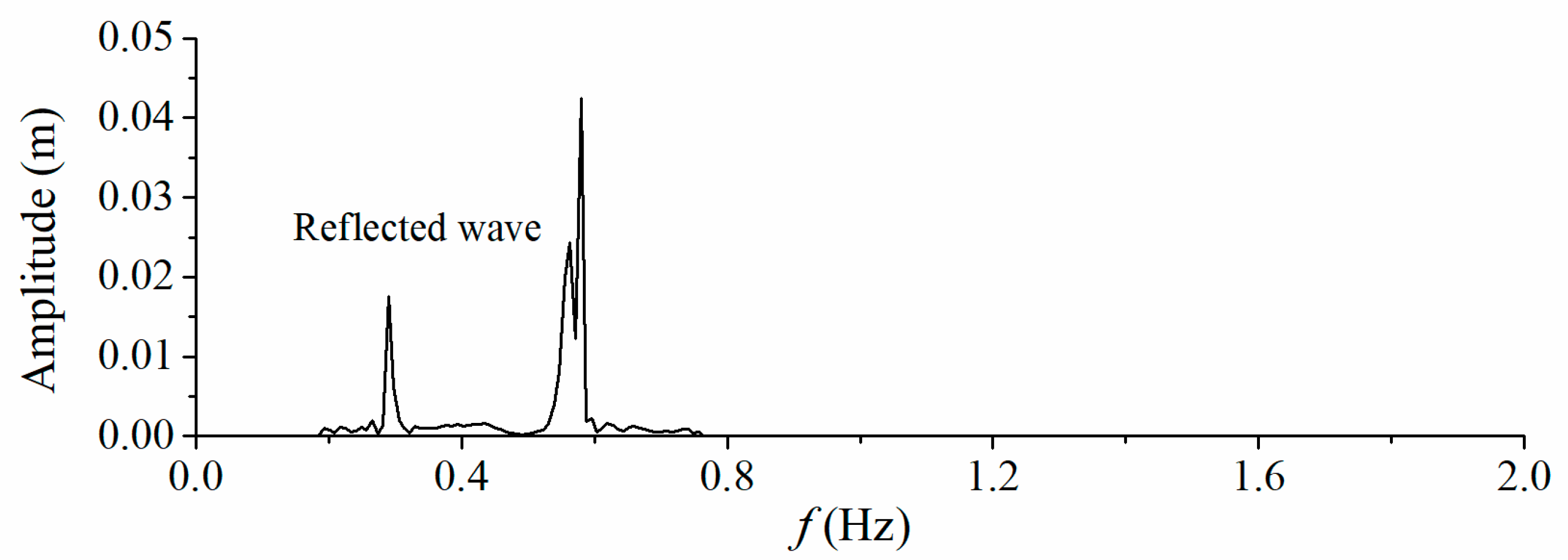
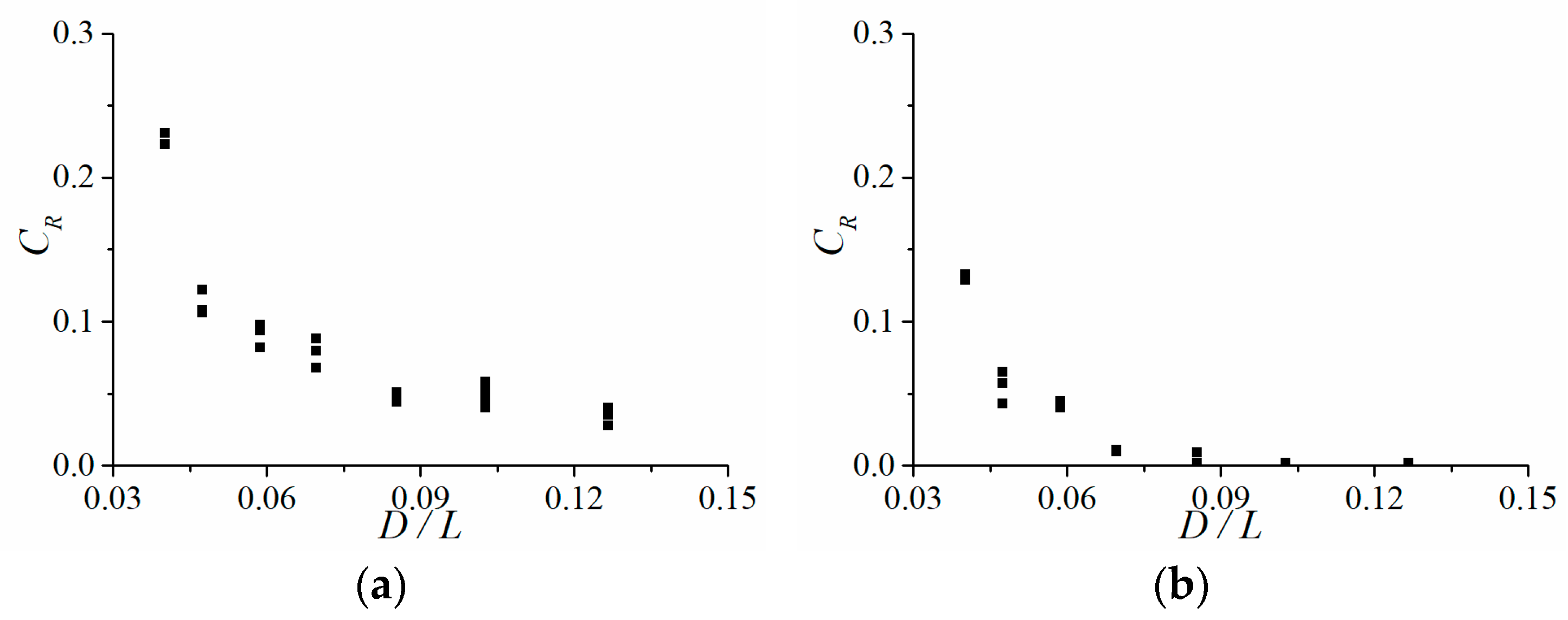
3.4. Influence on the Wave Field of the Cylinder
4. Conclusions
- (1)
- When the wave period is small, the dynamic pressure on the surface of the submerged cylinder shows a higher dynamic pressure in the upper part and a lower dynamic pressure in the lower part. The reflection effect of the cylinder on the wave field is enhanced with increases in wave periods and wave heights. Waves would break when passing through the cylinder, and the maximum stress position of the cylinder changed to an area 45° from the vertical axis of the cylinder on the up-wave and back-wave sides.
- (2)
- The horizontal and vertical forces acting on the submerged cylinder per linear meter are similar, with the horizontal force slightly greater than the vertical force. The first-order force on the structure is directly proportional to the wave height, while the second-order force is directly proportional to the square of the wave height; the overall force on the structure increases with an increase in wave height. However, as the wave height continues to increase, the cylinder has a significant impact on the wave field. After the wave passes through the submerged cylinder, it will break. Through calculation, the inertia force coefficient of the cylinder is between 1.2 and 2.0, indicating that the Morison formula can also be used for calculating the force on large-scale cylinders. The coefficient can be selected according to these specifications.
- (3)
- The deeper the submergence depth of the submerged cylinder, the less obvious the reflection effect. When the submergence depth is constant, the submerged cylinder has a certain reflection effect on long-period waves. The main reason is that the long-period wave action is deeper in water depth, which means that water quality points still exhibit obvious motion characteristics in deeper situations. Therefore, the submerged cylinder has a certain reflection effect on long-period waves.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Dean, W.R. On the reflexion of surface waves by a submerged circular cylinder. Math. Proc. Camb. Philos. Soc. 1948, 4, 483–491. [Google Scholar] [CrossRef]
- Ursell, F. Surface waves on deep water in the presence of a submerged circular cylinder, I and II. Math. Proc. Camb. Philos. Soc. 1950, 1, 141–158. [Google Scholar] [CrossRef]
- Ogilvie, T.F. First- and second-order forces on a cylinder submerged under a free surface. J. Fluid Mech. 1963, 3, 451–472. [Google Scholar] [CrossRef]
- Guerber, E.; Benoit, M.; Grilli, S.T.; Buvat, C. A fully nonlinear implicit model for wave interactions with submerged structures in forced or free motion. Eng. Anal. Bound. Elem. 2012, 7, 1151–1163. [Google Scholar] [CrossRef]
- Hannan, M.A.; Bai, W.; Ang, K.K. Modeling of fully nonlinear wave radiation by submerged moving structures using the higher order boundary element method. J. Mar. Sci. Appl. 2014, 1, 1–10. [Google Scholar] [CrossRef]
- Bai, W.; Hannan, M.A.; Ang, K.K. Numerical simulation of fully nonlinear wave interaction with submerged structures: Fixed or subjected to constrained motion. J. Fluids Struct. 2014, 49, 534–553. [Google Scholar] [CrossRef]
- Jin, R.J.; Liu, Y.; Geng, B.L.; Zhang, H.Q. Hydrodynamic characteristics of circular tube structure with wave action. J. Waterw. Harb. 2019, 3, 249–258. (In Chinese) [Google Scholar]
- Yan, B.; Lin, H.X.; Ning, D.Z. Nonlinear numerical simulation of wave action on submerged horizontal cylinder. Adv. Mar. Sci. 2013, 3, 319–325. (In Chinese) [Google Scholar]
- Mao, H.F. Numerical Examination of Wave Forces on Horizontal Circular Cylinder Based on Viscous Fluid Theory. Ph.D. Thesis, Dalian University of Technology, Dalian, China, 2018. (In Chinese). [Google Scholar]
- Liu, C.G.; Tao, J.H. Solitary waves interaction with horizontal cylinder submerged in different depth. J. Hydrodyn. 2006, 2, 155–160. (In Chinese) [Google Scholar]
- Liu, M.M.; Lu, L.; Teng, B.; Zhao, M.; Tang, G.Q. Numerical modeling of local scour and forces for submarine pipeline under surface waves. Coast. Eng. 2016, 116, 275–288. [Google Scholar] [CrossRef]
- Teng, B.; Mao, H.F.; Lu, L. Viscous Effects on Wave Forces on A Submerged Horizontal Circular Cylinder. China Ocean Eng. 2018, 3, 245–255. [Google Scholar] [CrossRef]
- Teng, B.; Mao, H.F.; Ning, D.Z.; Zhang, C.W. Viscous numerical examination of hydrodynamic forces on a submerged horizontal circular cylinder undergoing forced oscillation. J. Hydrodyn. 2018, 5, 887–899. [Google Scholar] [CrossRef]
- Bai, J.L.; Ma, N.; Gu, X.C. Wave-current loads on the horizontal cylinder with varying submergence depth. J. Shanghai Jiaotong Univ. 2018, 8, 938–945. (In Chinese) [Google Scholar]
- Moballa, B.; Chern, M.J.; Borthwick, A.G.L. Incompressible SPH simulation of flow past horizontal cylinder between plane wall and free surface. J. Fluids Struct. 2020, 97, 103091. [Google Scholar] [CrossRef]
- Gao, J.L.; Zang, J.; Chen, L.F.; Chen, Q.; Ding, H.Y.; Liu, Y.Y. On hydrodynamic characteristics of gap resonance between two fixed bodies in close proximity. Ocean Eng. 2019, 173, 28–44. [Google Scholar] [CrossRef]
- Gao, J.L.; He, Z.W.; Zang, J.; Chen, Q.; Ding, H.Y.; Wang, G. Numerical investigations of wave loads on fixed box in front of vertical wall with a narrow gap under wave actions. Ocean Eng. 2020, 206, 107323. [Google Scholar] [CrossRef]
- Gao, J.L.; Jing, L.Y.U.J.; Wang, J.H.; Zhang, J.; Liu, Q.; Zang, J.; Zou, T. Study on transient gap resonance with consideration of the motion of floating body. China Ocean Eng. 2022, 36, 994–1006. [Google Scholar] [CrossRef]
- Chaplin, J.R. On the irrotational flow around a horizontal cylinder in waves. J. Appl. Mech. 1981, 4, 689–694. [Google Scholar] [CrossRef]
- Chaplin, J.R. Mass transport around a horizontal cylinder beneath waves. J. Fluid Mech. 1984, 140, 175–187. [Google Scholar] [CrossRef]
- Chaplin, J.R. Non-linear forces on a horizontal cylinder beneath waves. J. Fluid Mech. 1984, 147, 449–464. [Google Scholar] [CrossRef]
- Chaplin, J.R. Non-linear wave interactions with a submerged horizontal cylinder. In Proceedings of the ISOPE International Ocean and Polar Engineering Conference, Stavanger, Norway, 17 June 2001; ISOPE: Stavanger, Norway, 2001. [Google Scholar]
- Contento, G.; Codiglia, R. Non-linear free surface induced pressure on a submerged horizontal circular cylinder at low Keulegan-Carpenters numbers. Appl. Ocean Res. 2001, 3, 175–185. [Google Scholar] [CrossRef]
- Fu, Y.Y. Analytical Solution for Wave Action on Submerged Horizontal Circular Cylinder. Master’s Thesis, Dalian University of Technology, Dalian, China, 2012. (In Chinese). [Google Scholar]
- LI, Y.C. Aspect of the normalization of hydrodynamic coefficients in Morison equation. J. Hydrodyn. 1998, 3, 329–337. (In Chinese) [Google Scholar]
- Chen, J.; Zhu, K.Q. Fitting of inertial coefficient and drag coefficient curve due to the changes in Reynolds and Keulegan-Carpecter. Ship Sci. Technol. 2014, 2, 40–43. (In Chinese) [Google Scholar]
- Hu, K.; Fu, S.X.; Xu, Y.W.; Ma, L.X. Experimental research about hydrodynamic characteristics of horizontal cylinder under different draft. J. Ship Mech. 2017, 10, 1190–1198. (In Chinese) [Google Scholar]
- Mao, H.F.; He, Y.L.; Yuan, J.P.; Pan, X.X.; Jia, B.Z. Hydrodynamic coefficients of a horizontal circular cylinder near free surface under wave action. J. Guangdong Ocean Univ. 2020, 4, 109–115. (In Chinese) [Google Scholar]
- Mansard, E.P.D.; Funke, E.R. The measurement of incident and reflected spectra using a least squares method. In Proceedings of the 17th International Conference on Coastal Engineering, Sydney, Australia, 23 March 1980; pp. 154–172. [Google Scholar]
















| Parameter | Meaning | Parameter | Meaning |
|---|---|---|---|
| D | Diameter of submerged cylinder | KC | Keulegan–Carpenter number |
| ds | Submerged depth | L | Wave length |
| d | Water depth | ηr | Reflected wave elevation |
| WP | Wave probe | CR | Reflection coefficient |
| H | Wave height | CM | Inertia force coefficient |
| T | Wave period | F(1)/F(2) | First-/Second-order wave force |
| k | Wave number | ρ | Water density |
| Re | Reynolds number | g | Gravity acceleration |
| No. | d/m | H/m | T/s | kA | H/gT2 | d/gT2 | Re (ds/d = 0.25) | Re (ds/d = 0.50) | KC (ds/d = 0.25) | KC (ds/d = 0.50) |
|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 4.2 | 0.123 | 2.25 | 0.0489 | 2.47 × 10−3 | 8.43 × 10−2 | 1.11 × 105 | 5.07 × 104 | 0.169 | 0.075 |
| 2 | 4.2 | 0.236 | 2.25 | 0.0938 | 4.74 × 10−3 | 8.43 × 10−2 | 2.13 × 105 | 9.20 × 104 | 0.323 | 0.144 |
| 3 | 4.2 | 0.301 | 2.25 | 0.1196 | 6.04 × 10−3 | 8.43 × 10−2 | 2.72 × 105 | 1.21 × 105 | 0.412 | 0.184 |
| 4 | 4.2 | 0.417 | 2.25 | 0.1658 | 8.37 × 10−3 | 8.43 × 10−2 | 3.77 × 105 | 1.65 × 105 | 0.572 | 0.255 |
| 5 | 4.2 | 0.16 | 2.51 | 0.0516 | 2.59 × 10−3 | 6.80 × 10−2 | 1.42 × 105 | 7.42 × 104 | 0.261 | 0.139 |
| 6 | 4.2 | 0.27 | 2.51 | 0.0871 | 4.37 × 10−3 | 6.80 × 10−2 | 2.40 × 105 | 1.25 × 105 | 0.441 | 0.235 |
| 7 | 4.2 | 0.37 | 2.51 | 0.1193 | 5.99 × 10−3 | 6.80 × 10−2 | 3.29 × 105 | 1.72 × 105 | 0.604 | 0.322 |
| 8 | 4.2 | 0.528 | 2.51 | 0.1703 | 8.55 × 10−3 | 6.80 × 10−2 | 4.70 × 105 | 2.45 × 105 | 0.861 | 0.459 |
| 9 | 4.2 | 0.174 | 2.77 | 0.0466 | 2.32 × 10−3 | 5.59 × 10−2 | 1.52 × 105 | 8.66 × 104 | 0.325 | 0.198 |
| 10 | 4.2 | 0.313 | 2.77 | 0.0839 | 4.17 × 10−3 | 5.59 × 10−2 | 2.73 × 105 | 1.69 × 105 | 0.585 | 0.356 |
| 11 | 4.2 | 0.499 | 2.77 | 0.1337 | 6.64 × 10−3 | 5.59 × 10−2 | 4.35 × 105 | 2.68 × 105 | 0.933 | 0.568 |
| 12 | 4.2 | 0.65 | 2.77 | 0.1742 | 8.65 × 10−3 | 5.59 × 10−2 | 5.67 × 105 | 3.30 × 105 | 1.215 | 0.740 |
| 13 | 4.2 | 0.202 | 3.11 | 0.0442 | 2.13 × 10−03 | 4.43 × 10−2 | 1.71 × 105 | 1.15 × 105 | 0.437 | 0.301 |
| 14 | 4.2 | 0.432 | 3.11 | 0.0946 | 4.55 × 10−3 | 4.43 × 10−2 | 3.66 × 105 | 2.45 × 105 | 0.935 | 0.644 |
| 15 | 4.2 | 0.64 | 3.11 | 0.1402 | 6.75 × 10−3 | 4.43 × 10−2 | 5.43 × 105 | 3.63 × 105 | 1.386 | 0.953 |
| 16 | 4.2 | 0.237 | 3.45 | 0.0437 | 2.03 × 10−3 | 3.59 × 10−2 | 1.97 × 105 | 1.42 × 105 | 0.579 | 0.434 |
| 17 | 4.2 | 0.503 | 3.45 | 0.0928 | 4.30 × 10−3 | 3.59 × 10−2 | 4.18 × 105 | 3.19 × 105 | 1.229 | 0.921 |
| 18 | 4.2 | 0.738 | 3.45 | 0.1362 | 6.31 × 10−3 | 3.59 × 10−2 | 6.13 × 105 | 4.57 × 105 | 1.804 | 1.352 |
| 19 | 4.2 | 0.296 | 3.98 | 0.0441 | 1.91 × 10−3 | 2.70 × 10−2 | 2.41 × 105 | 1.91 × 105 | 0.850 | 0.693 |
| 20 | 4.2 | 0.49 | 3.98 | 0.0730 | 3.15 × 10−3 | 2.70 × 10−2 | 3.98 × 105 | 3.16 × 105 | 1.407 | 1.148 |
| 21 | 4.2 | 0.699 | 3.98 | 0.1042 | 4.50 × 10−3 | 2.70 × 10−2 | 5.68 × 105 | 4.51 × 105 | 2.008 | 1.637 |
| 22 | 4.2 | 0.351 | 4.51 | 0.0442 | 1.76 × 10−3 | 2.11 × 10−2 | 2.81 × 105 | 2.30 × 105 | 1.158 | 0.994 |
| 23 | 4.2 | 0.745 | 4.51 | 0.0939 | 3.74 × 10−3 | 2.11 × 10−2 | 5.96 × 105 | 4.78 × 105 | 2.459 | 2.111 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jin, R.; Zhao, X.; Liu, Y.; Geng, B. Large Scale Experimental Study on Waves and Submerged Horizontal Cylinders. Water 2024, 16, 28. https://doi.org/10.3390/w16010028
Jin R, Zhao X, Liu Y, Geng B. Large Scale Experimental Study on Waves and Submerged Horizontal Cylinders. Water. 2024; 16(1):28. https://doi.org/10.3390/w16010028
Chicago/Turabian StyleJin, Ruijia, Xu Zhao, Ye Liu, and Baolei Geng. 2024. "Large Scale Experimental Study on Waves and Submerged Horizontal Cylinders" Water 16, no. 1: 28. https://doi.org/10.3390/w16010028
APA StyleJin, R., Zhao, X., Liu, Y., & Geng, B. (2024). Large Scale Experimental Study on Waves and Submerged Horizontal Cylinders. Water, 16(1), 28. https://doi.org/10.3390/w16010028






