An Explicit Solution for Characterizing Non-Fickian Solute Transport in Natural Streams
Abstract
:1. Introduction
2. Materials and Methods
2.1. Model Description
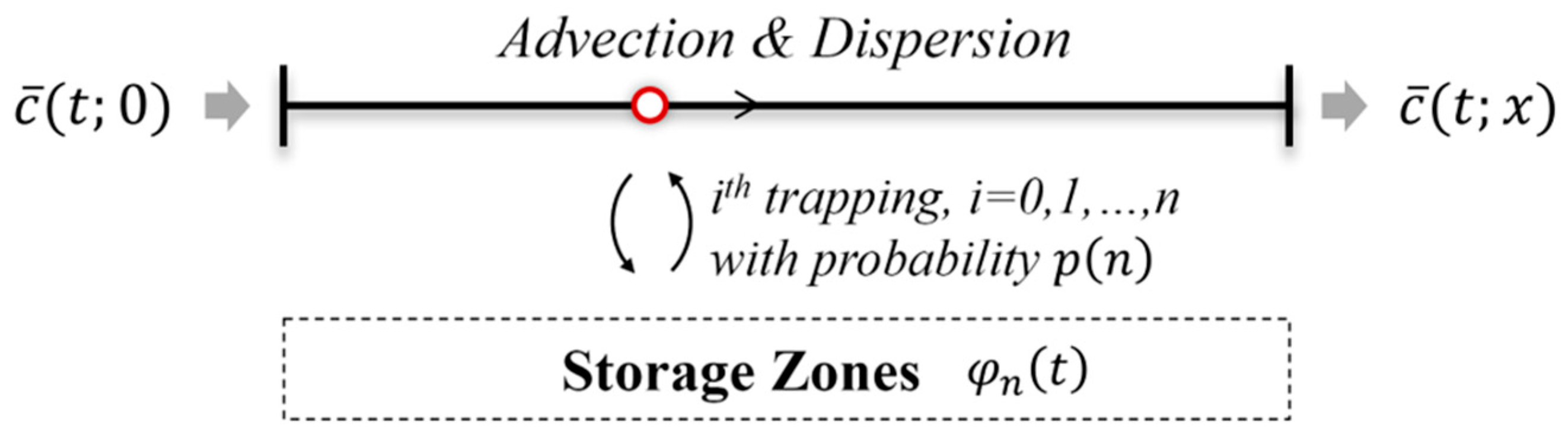
2.1.1. Formulation for Non-Fickian Tracer Transport
2.1.2. Parameter Determination
2.2. Field Experiment
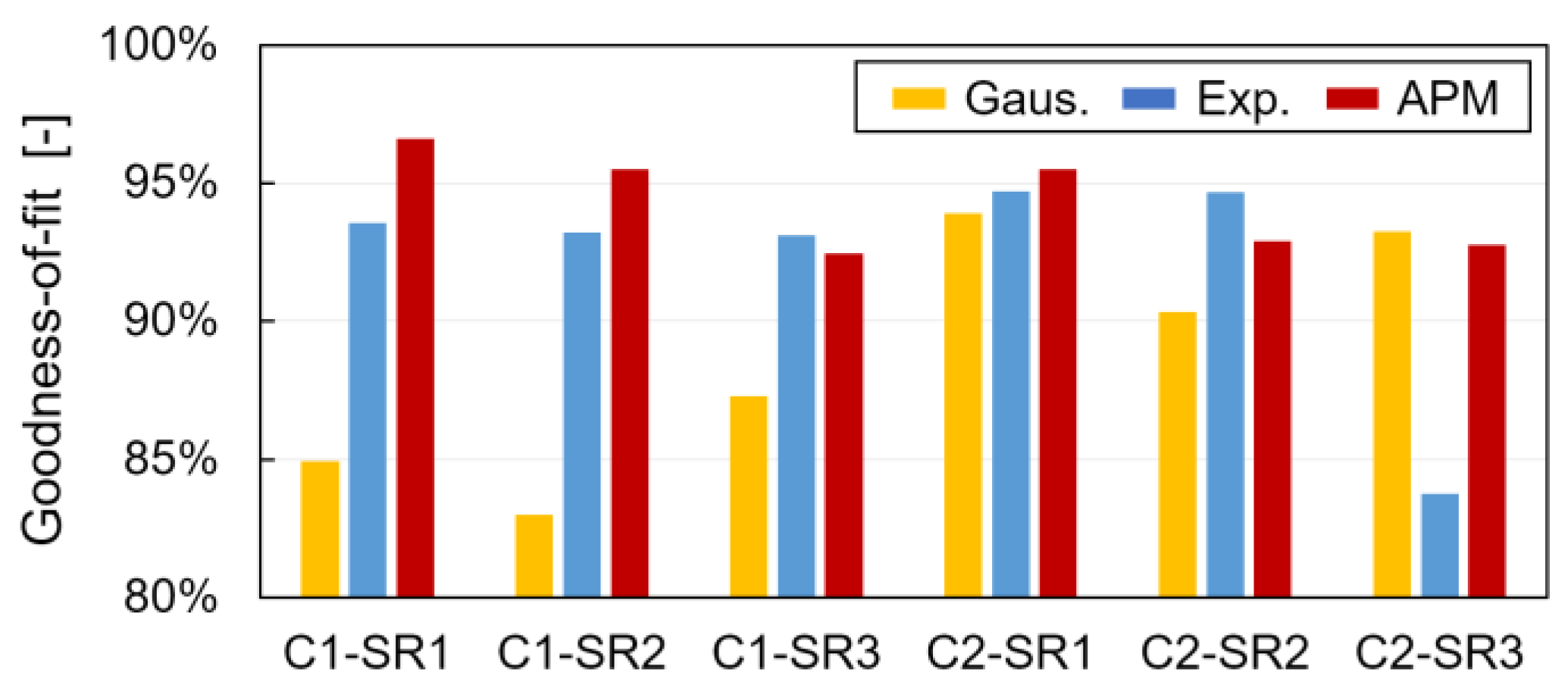
3. Results and Discussion
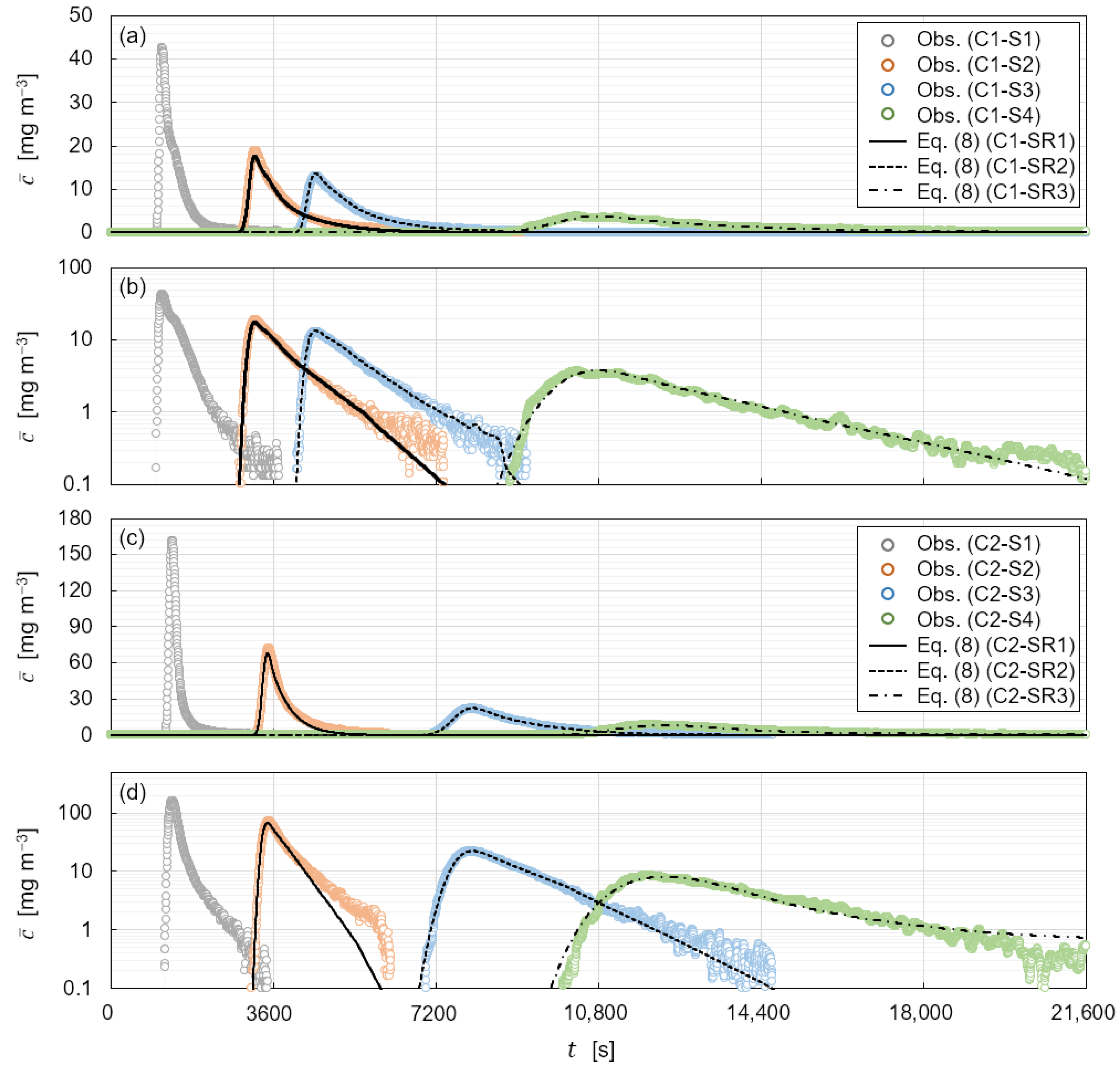
3.1. Verification by Synthetic Data
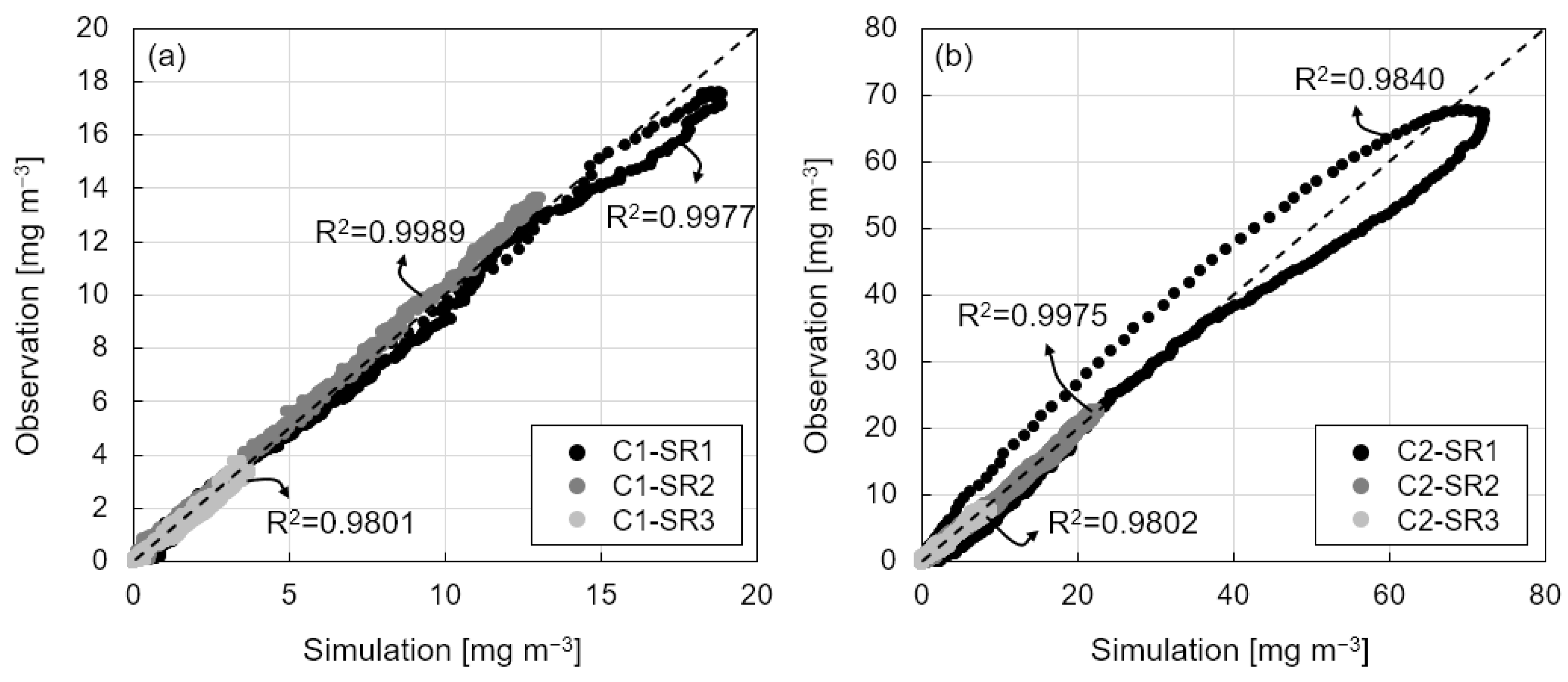
3.2. Validation by Field Data
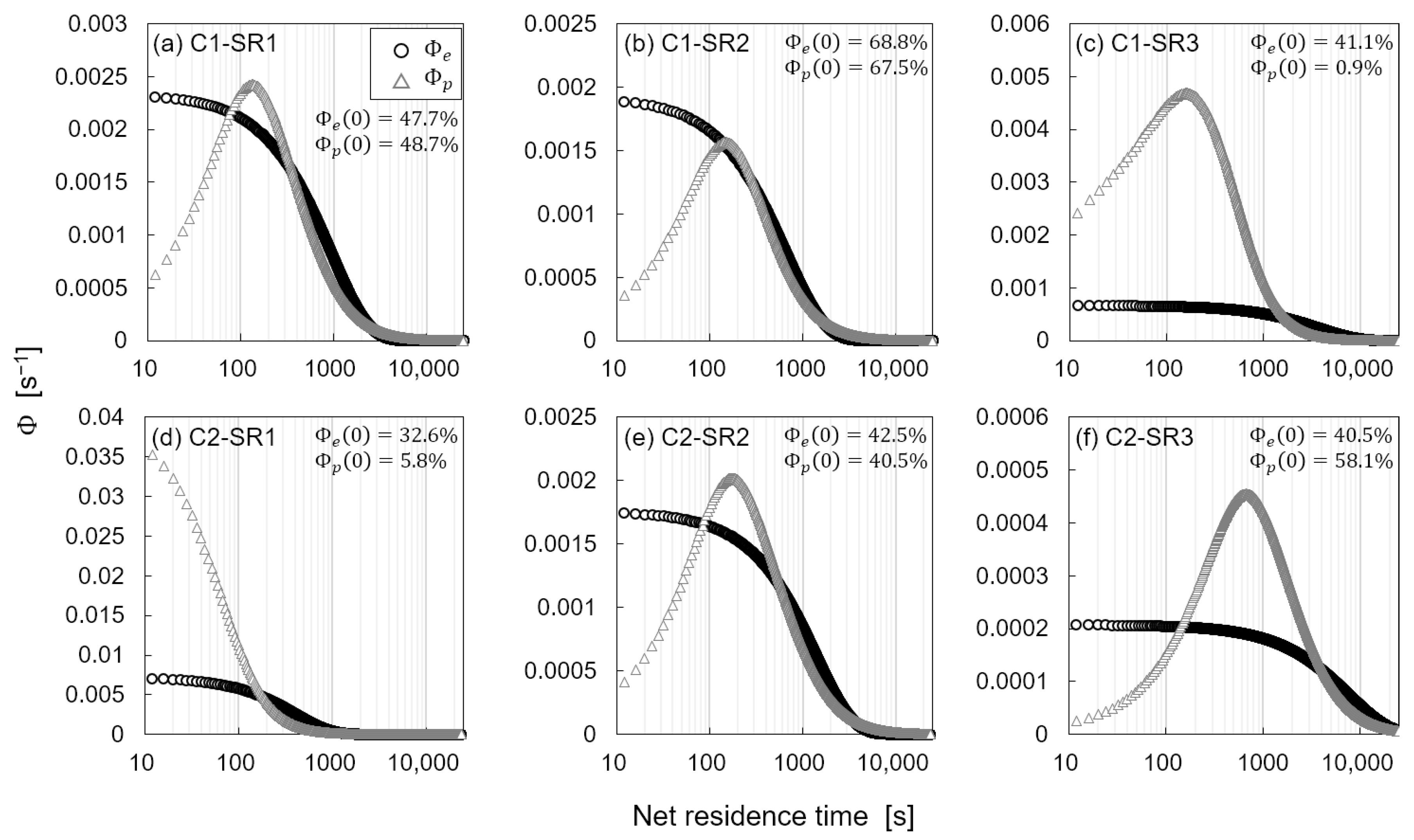
3.3. Late-Time Behavior of the Breakthrough Curve
4. Conclusions
- The proposed formula was validated by comparison with analytical and numerical solutions, and the results were exact. Its performance in simulating non-Fickian transport in streams was also validatesd using field tracer data, and good agreement was achieved.
- Despite the accurate results of reproducing the overall breakthrough curves, significant differences in their late-time behaviors were found according to the memory function modeling. This indicates that the best-fit breakthrough curve does not imply the accurate storage effect modeling.
- The tailing effect was discussed by comparing the optimal memory functions and net residence time functions. The key role of residence time-dependent functions is to enable us to quantitatively identify the storage effect, which is fundamental for a deeper understanding of non-Fickian tracer transport in streams. Hence, insight in characterizing non-Fickian mixing in can be provided from the memory function modeling.
- According to the data and optimal parameters, the proper formula for the memory function was inconsistent. The exponential memory function had a structural limit in generating a heavy-tailed breakthrough curve.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Park, I.; Seo, I.W. Modeling Non-Fickian Pollutant Mixing in Open Channel Flows Using Two-Dimensional Particle Dispersion Model. Adv. Water Resour. 2018, 111, 105–120. [Google Scholar] [CrossRef]
- Seo, I.W.; Choi, H.J.; Do Kim, Y.; Han, E.J. Analysis of Two-Dimensional Mixing in Natural Streams Based on Transient Tracer Tests. J. Hydraul. Eng. 2016, 142, 04016020. [Google Scholar] [CrossRef]
- Kim, B.; Seo, I.W.; Kwon, S.; Jung, S.H.; Choi, Y. Modelling One-Dimensional Reactive Transport of Toxic Contaminants in Natural Rivers. Environ. Model. Softw. 2021, 137, 104971. [Google Scholar] [CrossRef]
- Wörman, A.; Wachniew, P. Reach Scale and Evaluation Methods as Limitations for Transient Storage Properties in Streams and Rivers. Water Resour. Res. 2007, 43. [Google Scholar] [CrossRef]
- Fischer, H.B. Dispersion Predictions in Natural Streams. J. Sanit. Eng. Div. 1968, 94, 927–943. [Google Scholar] [CrossRef]
- Kwon, S.; Noh, H.; Seo, I.W.; Jung, S.H.; Baek, D. Identification Framework of Contaminant Spill in Rivers Using Machine Learning with Breakthrough Curve Analysisremote Sensing. Int. J. Environ. Res. Public Health 2021, 18, 1023. [Google Scholar] [CrossRef]
- Kim, B.; Kwon, S.; Noh, H.; Seo, I.W. Surrogate Prediction of the Breakthrough Curve of Solute Transport in Rivers Using Its Reach Length Dependence. J. Contam. Hydrol. 2022, 249, 104024. [Google Scholar] [CrossRef]
- Bencala, K.E.; Walters, R.A. Simulation of Solute Transport in a Mountain Pool-and-Riffle Stream: A Transient Storage Model. Water Resour. Res. 1983, 19, 718–724. [Google Scholar] [CrossRef]
- Szeftel, P.; Dan Moore, R.D.; Weiler, M. Influence of Distributed Flow Losses and Gains on the Estimation of Transient Storage Parameters from Stream Tracer Experiments. J. Hydrol. 2011, 396, 277–291. [Google Scholar] [CrossRef]
- Noh, H.; Kwon, S.; Seo, I.W.; Baek, D.; Jung, S.H. Multi-Gene Genetic Programming Regression Model for Prediction of Transient Storage Model Parameters in Natural Rivers. Water 2021, 13, 76. [Google Scholar] [CrossRef]
- Marion, A.; Bellinello, M.; Guymer, I.; Packman, A. Effect of Bed Form Geometry on the Penetration of Nonreactive Solutes into a Streambed. Water Resour. Res. 2002, 38, 1209. [Google Scholar] [CrossRef]
- Kim, J.S.; Seo, I.W.; Baek, D.; Kang, P.K. Recirculating Flow-Induced Anomalous Transport in Meandering Open-Channel Flows. Adv. Water Resour. 2020, 141, 103603. [Google Scholar] [CrossRef]
- Thackston, E.L.; Schnelle, K.B.J. Predicting Effects of Dead Zones on Stream Mixing. J. Sanit. Eng. Div. 1970, 96, 319–331. [Google Scholar] [CrossRef]
- Choi, S.Y.; Seo, I.W.; Kim, Y.O. Parameter Uncertainty Estimation of Transient Storage Model Using Bayesian Inference with Formal Likelihood Based on Breakthrough Curve Segmentation. Environ. Model. Softw. 2020, 123, 104558. [Google Scholar] [CrossRef]
- Haggerty, R.; Wondzell, S.M.; Johnson, M.A. Power-Law Residence Time Distribution in the Hyporheic Zone of a 2nd-Order Mountain Stream. Geophys. Res. Lett. 2002, 29, 1640. [Google Scholar] [CrossRef] [Green Version]
- Deng, Z.; Singh, V.P.; Bengtsson, L. Numerical Solution of Fractional Order Advection-Reaction-Diffusion Equation. J. Hydraul. Eng. 2004, 130, 422–431. [Google Scholar] [CrossRef] [Green Version]
- Boano, F.; Packman, A.I.; Cortis, A.; Revelli, R.; Ridolfi, L. A Continuous Time Random Walk Approach to the Stream Transport of Solutes. Water Resour. Res. 2007, 43, 1–12. [Google Scholar] [CrossRef]
- Marion, A.; Zaramella, M.; Bottacin-Busolin, A. Solute Transport in Rivers with Multiple Storage Zones: The STIR Model. Water Resour. Res. 2008, 44. [Google Scholar] [CrossRef] [Green Version]
- Marion, A.; Zaramella, M. A Residence Time Model for Stream-Subsurface Exchange of Contaminants. Acta Geophys. Pol. 2005, 53, 527–538. [Google Scholar]
- Deng, Z.Q.; Jung, H.S. Variable Residence Time-Based Model for Solute Transport in Streams. Water Resour. Res. 2009, 45. [Google Scholar] [CrossRef]
- Singh, S.K. Treatment of Stagnant Zones in Riverine Advection-Dispersion. J. Hydraul. Eng. 2003, 129, 470–473. [Google Scholar] [CrossRef]
- Runkel, R.L.; Broshears, R.E. One-Dimensional Transport with Inflow and Storage (OTIS): A Solute Transport Model for Small Streams; Center for Advanced Decision Support for Water and Environmental Systems, Department of Civil Engineering, University of Colorado: Boulder, CO, USA, 1991. [Google Scholar]
- Absi, R. Reinvestigating the Parabolic-shaped Eddy Viscosity Profile for Free Surface Flows. Hydrology 2021, 8, 126. [Google Scholar] [CrossRef]
- Fischer, H.B.; List, E.J.; Koh, R.C.Y.; Imberger, J.; Brooks, N.H. Mixing in Inland and Coastal Waters; Academic Press: Cambridge, MA, USA, 1979. [Google Scholar]
- Baek, K.O.; Seo, I.W. Routing Procedures for Observed Dispersion Coefficients in Two-Dimensional River Mixing. Adv. Water Resour. 2010, 33, 1551–1559. [Google Scholar] [CrossRef]
- Kim, B.; Seo, I.W. Net Retention Time Distribution Inducing Non-Fickian Solute Transport in Streams. In Proceedings of the 39th IAHR World Congress, Granada, Spain, 19–24 June 2022. [Google Scholar]
- Dekking, F.M.; Kraaikamp, C.; Lopuhaa, H.P.; Meester, L.E. A Modern Introduction to Probability and Statistics; Springer: London, UK, 2005; Volume 157, ISBN 9781852338961. [Google Scholar]
- Runkel, R.L. One-Dimensional Transport with Inflow and Storage (OTIS): A Solute Transport Model for Streams and Rivers; US Department of the Interior, US Geological Survey: Reston, VA, USA, 1998; Volume 98.
- Bencala, K.E. Simulation of Solute Transport in a Mountain Pool-and-Riffle Stream With a Kinetic Mass Transfer Model for Sorption. Water Resour. Res. 1983, 19, 732–738. [Google Scholar] [CrossRef]
- Cardenas, M.B. Potential Contribution of Topography-Driven Regional Groundwater Flow to Fractal Stream Chemistry: Residence Time Distribution Analysis of Tóth Flow. Geophys. Res. Lett. 2007, 34. [Google Scholar] [CrossRef]
- Elliott, A.H.; Brooks, N.H. Transfer of Nonsorbing Solutes to a Streambed with Bed Forms: Theory. Water Resour. Res. 1997, 33, 123–136. [Google Scholar] [CrossRef]
- Kazezyilmaz-Alhan, C.M. Analytical Solutions for Contaminant Transport in Streams. J. Hydrol. 2008, 348, 524–534. [Google Scholar] [CrossRef]
- Polyanin, A.; Manzhirov, A. Handbook of Integral Equations; CRC Press: Boca Raton, FL, USA, 2008; pp. 961–968. [Google Scholar]
- Valsa, J.; Brančik, L. Approximate Formulae for Numerical Inversion of Laplace Transforms. Int. J. Numer. Model. Electron. Netw. Devices Fields 1998, 11, 153–166. [Google Scholar] [CrossRef]
- Kraft, D. A Software Package for Sequential Quadratic Programming; DFVLR: San Bruno, CA, USA, 1988. [Google Scholar]
- Yuill, B.; Wang, Y.; Allison, M.; Meselhe, E.; Esposito, C. Sand Settling through Bedform-Generated Turbulence in Rivers. Earth Surf. Process. Landf. 2020, 45, 3231–3249. [Google Scholar] [CrossRef]
- Kim, J.S.; Kang, P.K. Anomalous Transport through Free-Flow-Porous Media Interface: Pore-Scale Simulation and Predictive Modeling. Adv. Water Resour. 2020, 135, 103467. [Google Scholar] [CrossRef]
- Kim, J.S.; Kang, P.K.; He, S.; Shen, L.; Kumar, S.S.; Hong, J.; Seo, I.W. Pore-Scale Flow Effects on Solute Transport in Turbulent Channel Flows Over Porous Media. Transp. Porous Media 2023, 146, 223–248. [Google Scholar] [CrossRef]
- SonTek, SonTek FlowTracker2 Handheld-ADV Specifications. Available online: https://www.ysi.com/flowtracker2 (accessed on 28 March 2023).
- Kilpatrick, F.A.; Wilson, J.F. Measurement of Time of Travel in Streams by Dye Tracing; US Government Printing Office: Washington, DC, USA, 1989.
- Baek, D.; Seo, I.W.; Kim, J.S.; Nelson, J.M. UAV-Based Measurements of Spatio-Temporal Concentration Distributions of Fluorescent Tracers in Open Channel Flows. Adv. Water Resour. 2019, 127, 76–88. [Google Scholar] [CrossRef]
- Bayani Cardenas, M.; Wilson, J.L.; Haggerty, R. Residence Time of Bedform-Driven Hyporheic Exchange. Adv. Water Resour. 2008, 31, 1382–1386. [Google Scholar] [CrossRef]
- Aquino, T.; Aubeneau, A.; Bolster, D. Peak and Tail Scaling of Breakthrough Curves in Hydrologic Tracer Tests. Adv. Water Resour. 2015, 78. [Google Scholar] [CrossRef]
- Haggerty, R.; McKenna, S.A.; Meigs, L.C. On the Late-Time Behavior of Tracer Test Breakthrough Curves. Water Resour. Res. 2000, 36, 3467–3479. [Google Scholar] [CrossRef] [Green Version]
- Scott, D.T.; Gooseff, M.N.; Bencala, K.E.; Runkel, R.L. Automated Calibration of a Stream Solute Transport Model: Implications for Interpretation of Biogeochemical Parameters. J. N. Am. Benthol. Soc. 2003, 22, 492–510. [Google Scholar] [CrossRef] [Green Version]
- Kim, B.; Seo, I.W.; Kwon, S.; Jung, S.H.; Yun, S.H. Analysis of Solute Transport in Rivers Using a Stochastic Storage Model. J. Korea Water Resour. Assoc. 2021, 54, 335–345. [Google Scholar] [CrossRef]
- Drummond, J.D.; Covino, T.P.; Aubeneau, A.F.; Leong, D.; Patil, S.; Schumer, R.; Packman, A.I. Effects of Solute Breakthrough Curve Tail Truncation on Residence Time Estimates: A Synthesis of Solute Tracer Injection Studies. J. Geophys. Res. Biogeosci. 2012, 117. [Google Scholar] [CrossRef] [Green Version]
- Gooseff, M.N.; Benson, D.A.; Briggs, M.A.; Weaver, M.; Wollheim, W.; Peterson, B.; Hopkinson, C.S. Residence Time Distributions in Surface Transient Storage Zones in Streams: Estimation via Signal Deconvolution. Water Resour. Res. 2011, 47. [Google Scholar] [CrossRef] [Green Version]
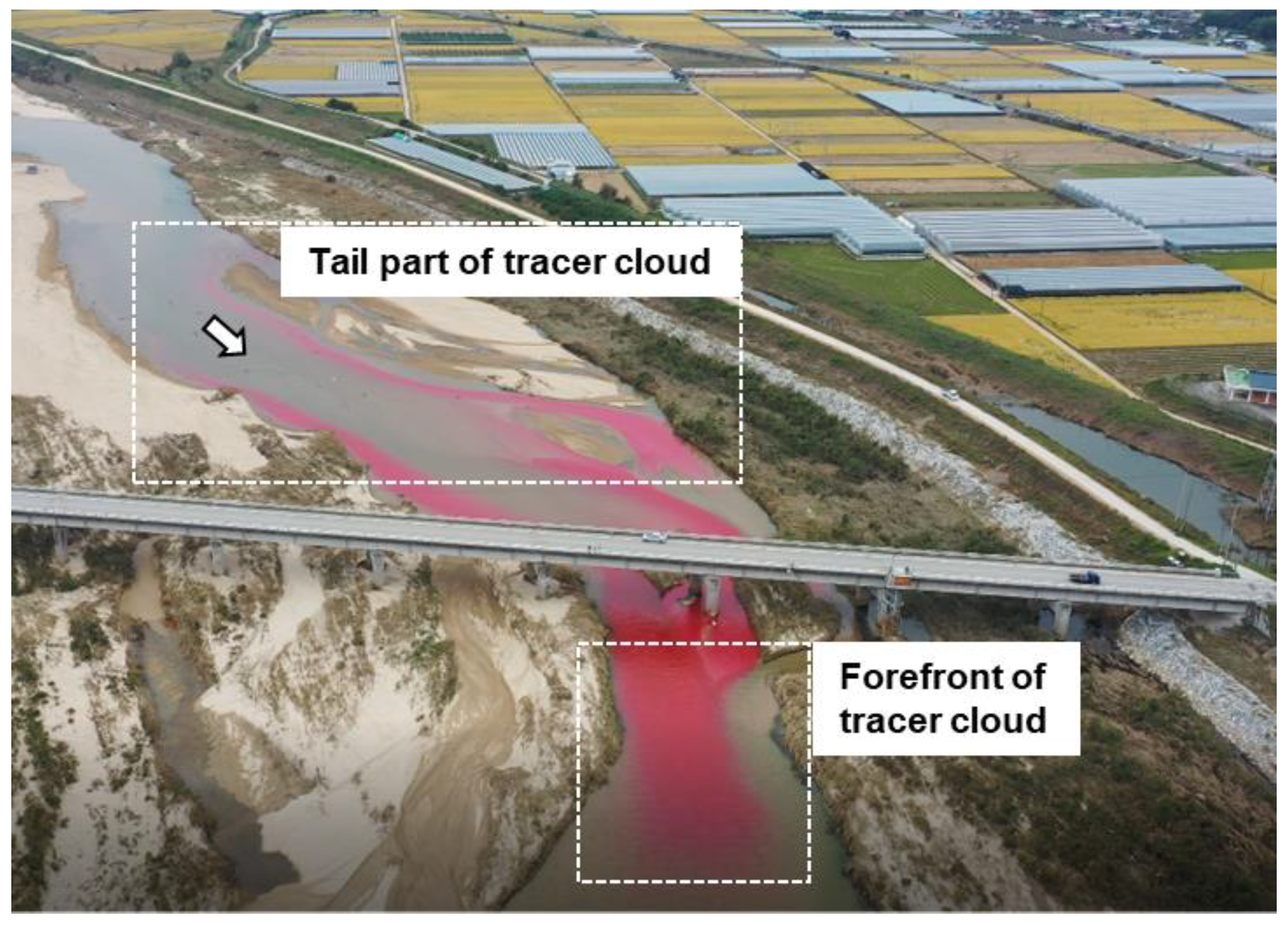





| Case | Sub-Reach | Length (m) | Discharge (m3/s) | Velocity (m2/s) | Area (m2) | Width (m) | Depth (m) |
|---|---|---|---|---|---|---|---|
| C1 | SR1 (C1-S1–C1-S2) | 1200 | 12.63 | 0.610 | 20.69 | 57.36 | 0.361 |
| SR2 (C1-S2–C1-S3) | 830 | 0.598 | 21.10 | 58.86 | 0.358 | ||
| SR3 (C1-S3–C1-S4) | 2000 | 0.553 | 22.83 | 53.00 | 0.431 | ||
| C2 | SR1 (C2-S1–C1-S2) | 954 | 2.17 | 0.322 | 6.17 | 20.75 | 0.305 |
| SR2 (C2-S2–C1-S3) | 1798 | 0.317 | 6.06 | 15.45 | 0.388 | ||
| SR3 (C2-S3–C1-S4) | 1105 | 0.315 | 6.74 | 16.75 | 0.395 |
| Simulation Case | (m) | (m2·s−1) | (s) | (10−4·s−1) |
|---|---|---|---|---|
| C1-SR1 | 0.605 | 0.56 | 604.94 | 3.76 |
| C1-SR2 | 0.644 | 0.59 | 536.39 | 2.92 |
| C1-SR3 | 0.355 | 4.89 | 2187.74 | 1.54 |
| C2-SR1 | 0.465 | 0.17 | 201.39 | 5.57 |
| C2-SR2 | 0.420 | 1.73 | 827.86 | 2.01 |
| C2-SR3 | 0.315 | 6.94 | 5767.09 | 1.35 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kim, B.; Kwon, S.; Seo, I.W. An Explicit Solution for Characterizing Non-Fickian Solute Transport in Natural Streams. Water 2023, 15, 1702. https://doi.org/10.3390/w15091702
Kim B, Kwon S, Seo IW. An Explicit Solution for Characterizing Non-Fickian Solute Transport in Natural Streams. Water. 2023; 15(9):1702. https://doi.org/10.3390/w15091702
Chicago/Turabian StyleKim, Byunguk, Siyoon Kwon, and Il Won Seo. 2023. "An Explicit Solution for Characterizing Non-Fickian Solute Transport in Natural Streams" Water 15, no. 9: 1702. https://doi.org/10.3390/w15091702
APA StyleKim, B., Kwon, S., & Seo, I. W. (2023). An Explicit Solution for Characterizing Non-Fickian Solute Transport in Natural Streams. Water, 15(9), 1702. https://doi.org/10.3390/w15091702






