Mitigating Scour at Bridge Abutments: An Experimental Investigation of Waste Material as an Eco-Friendly Solution
Abstract
:1. Introduction
2. Material and Methods
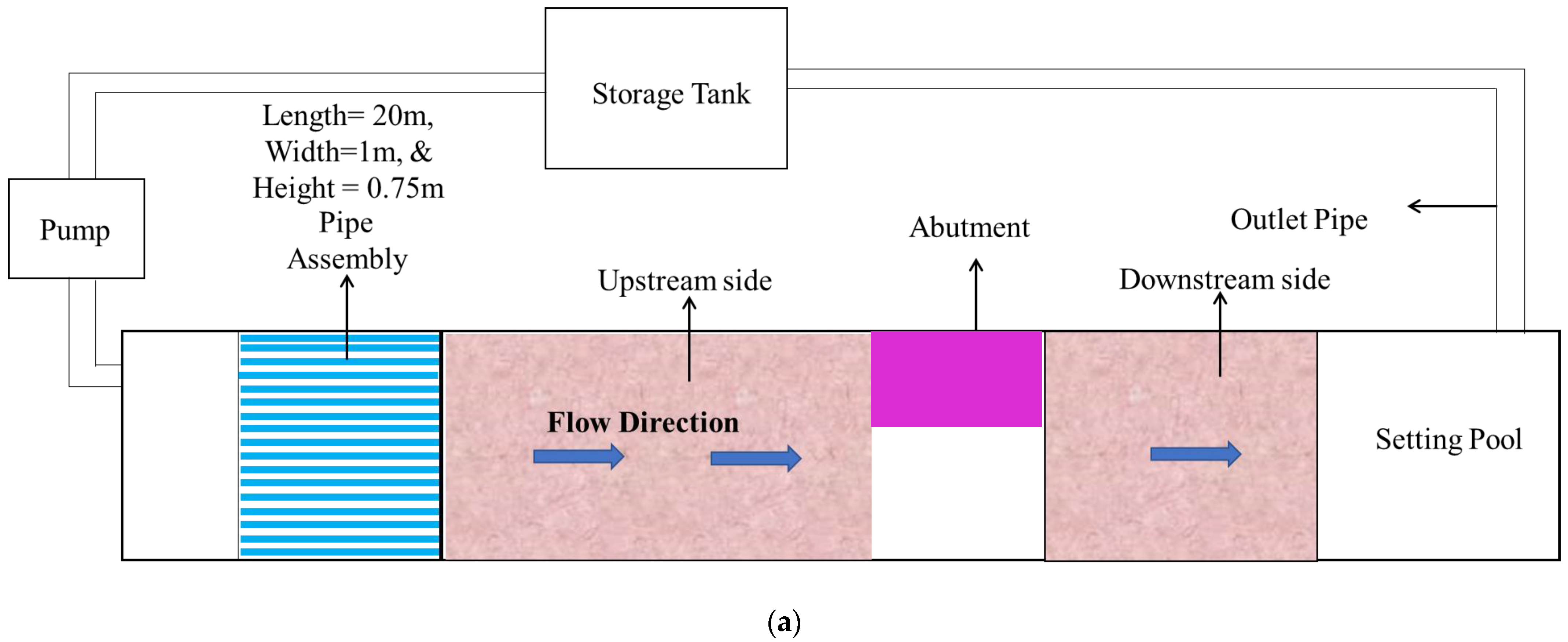
2.1. Flume Characteristic
2.2. Sediment Size and Flow Condition
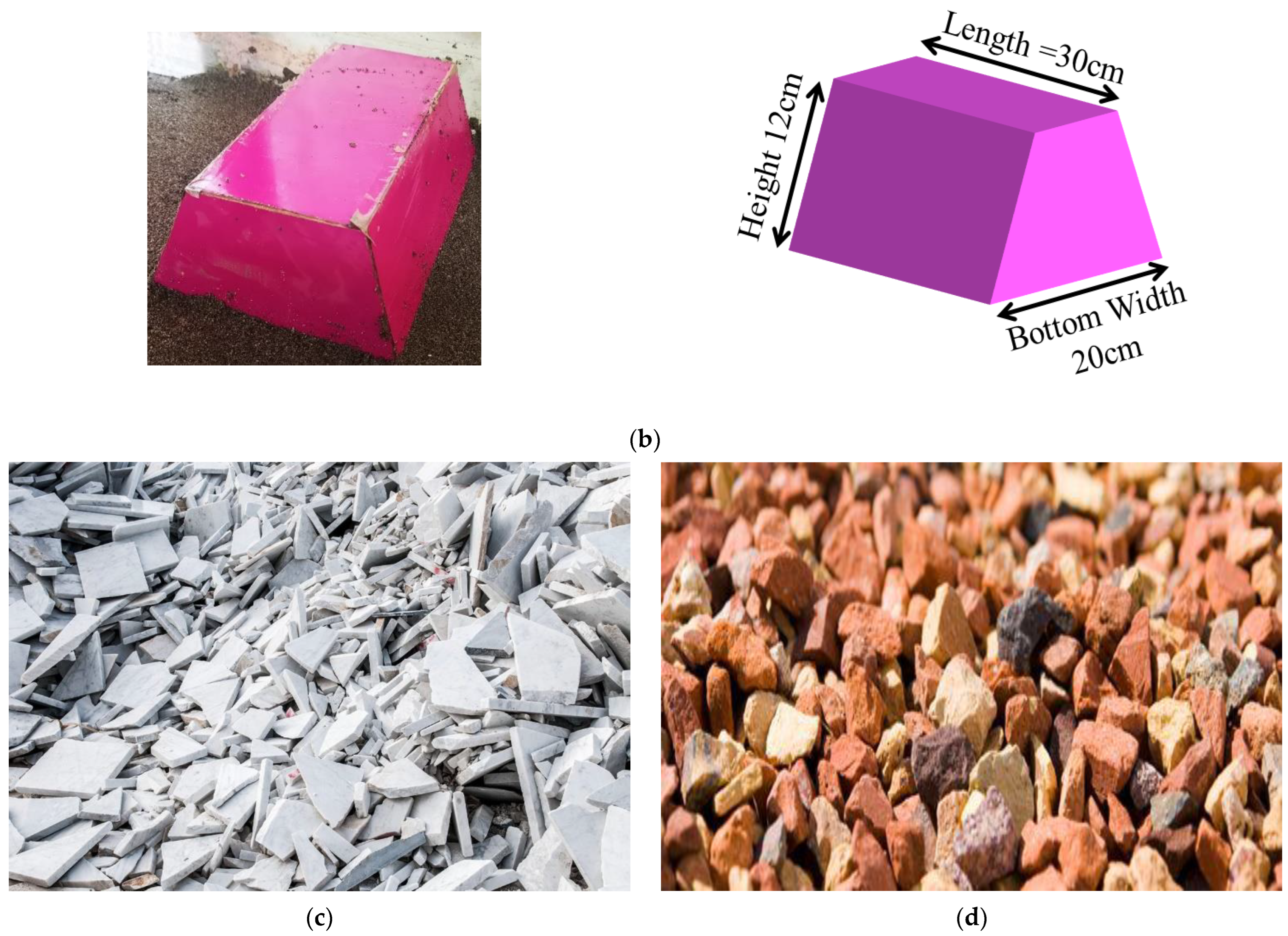
2.3. Abutment Specification
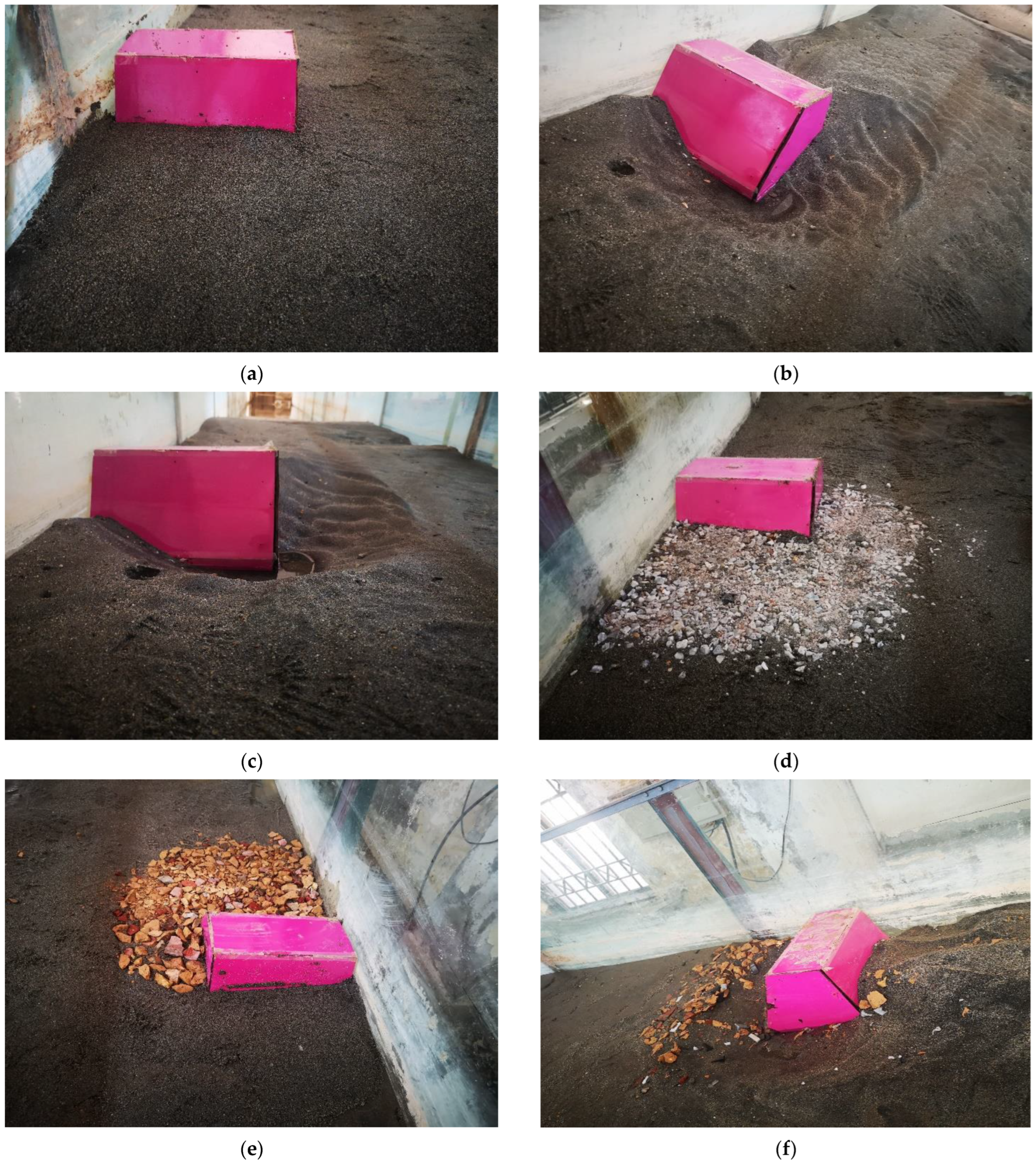
2.4. Marble and Brick Condition
2.5. Laboratory Work Procedure
- Crd = discharge coefficient of the rectangular, sharp-crested weir,
- Ctd = discharge coefficient for triangular, sharp-crested weir
- g = gravitational acceleration; h = water head on the weir crest; he = effective head
2.6. Dimensional Analysis
3. Results
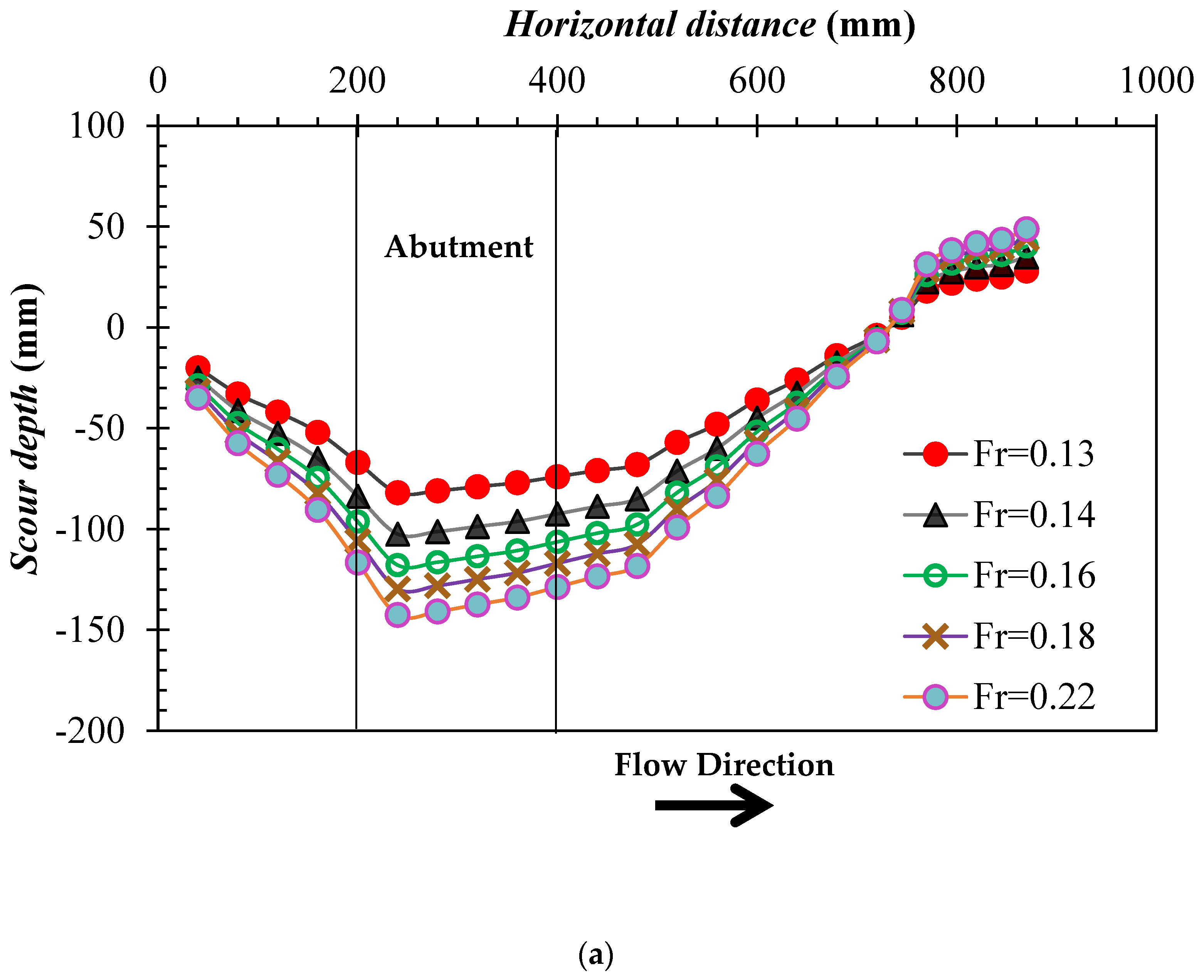
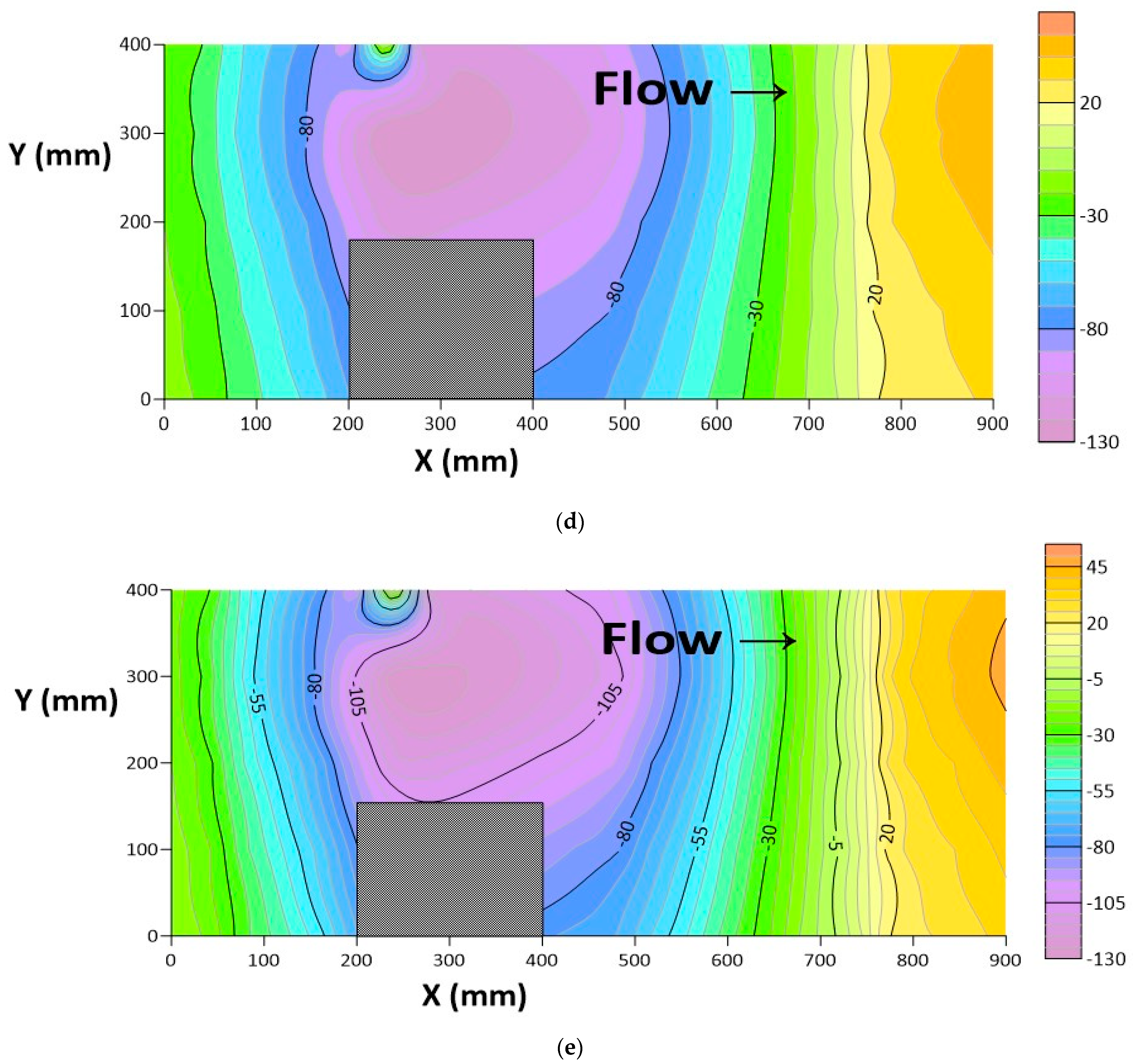
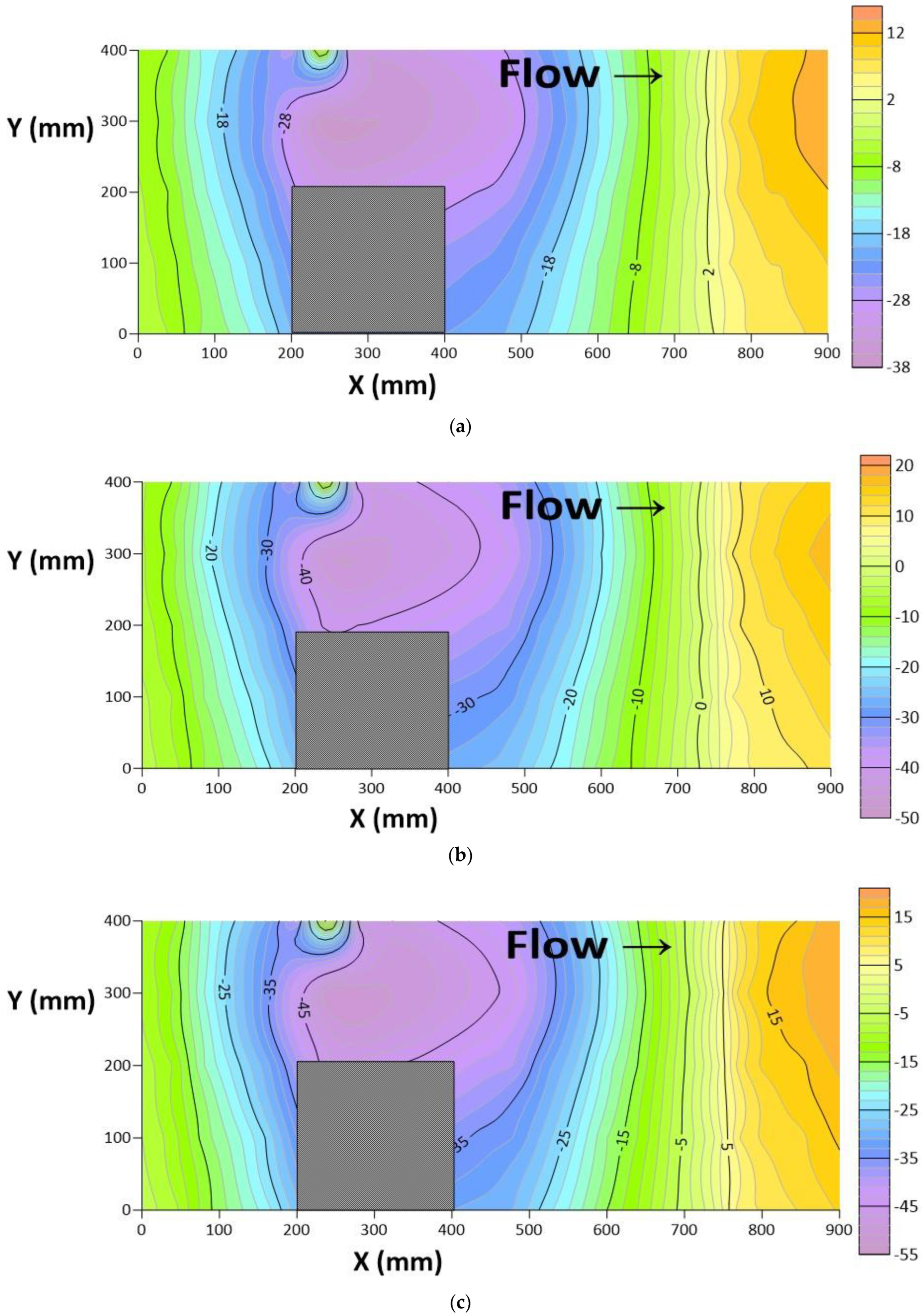
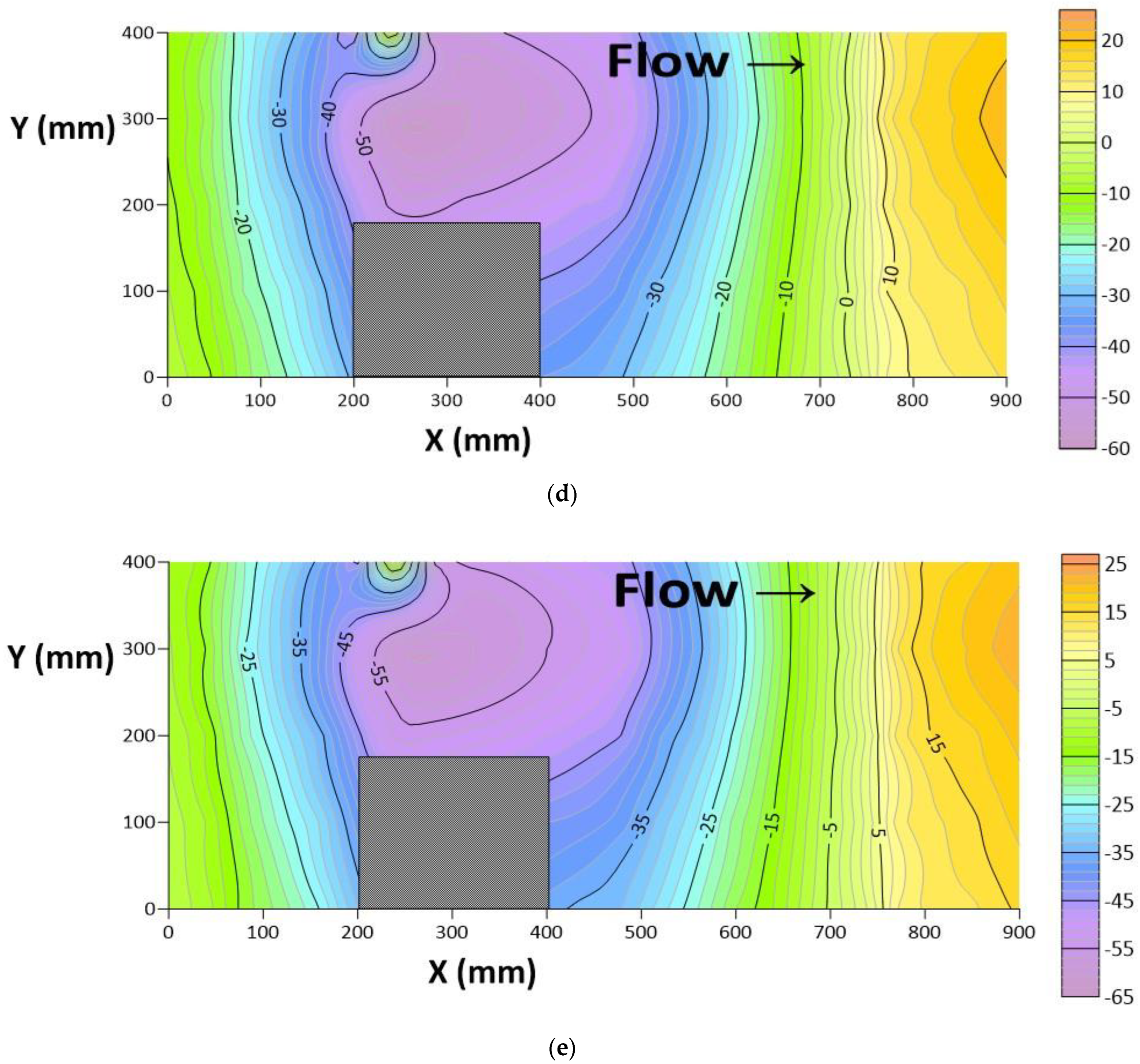
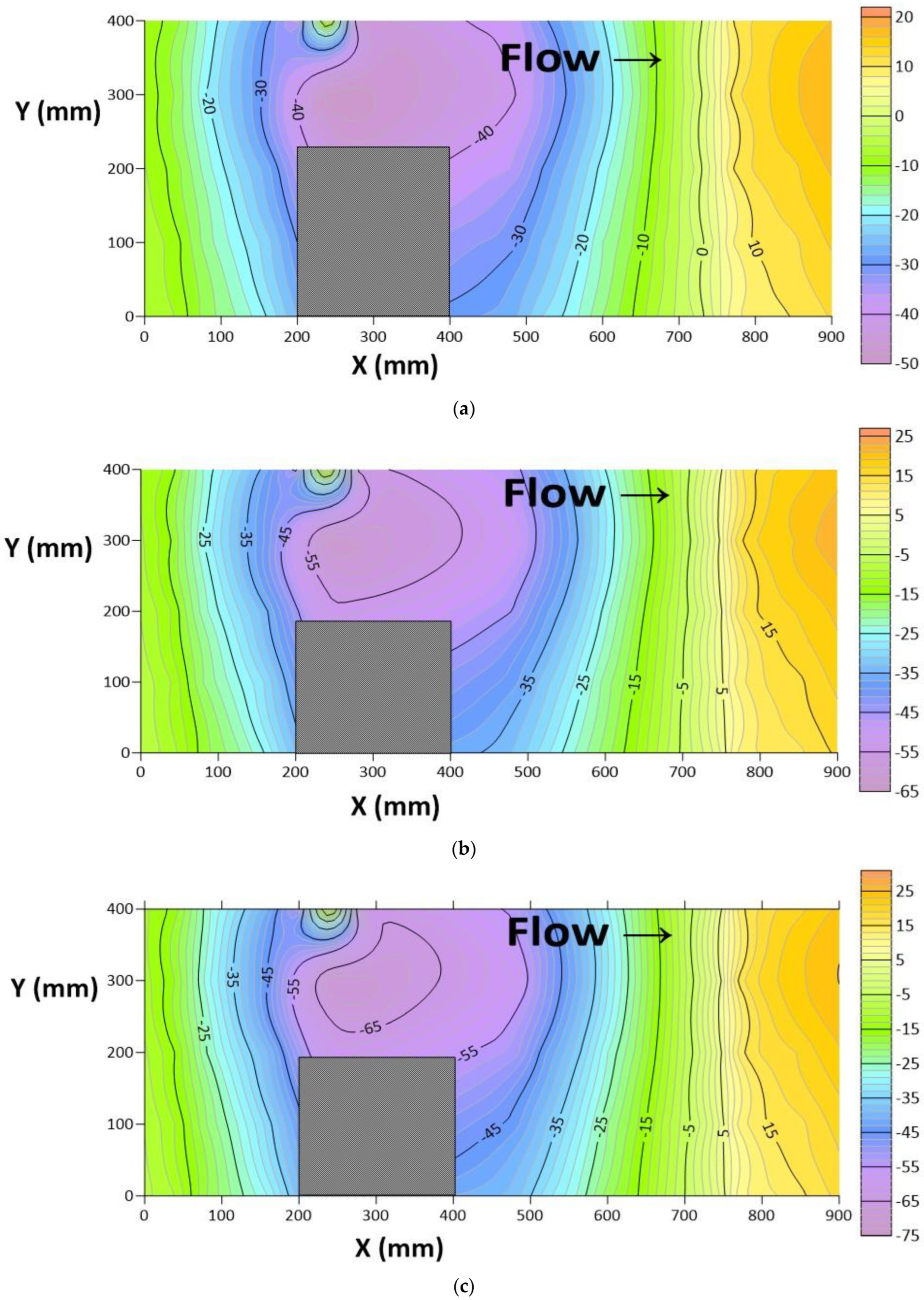
3.1. Scour Depth around the Bridge Abutment without Countermeasures
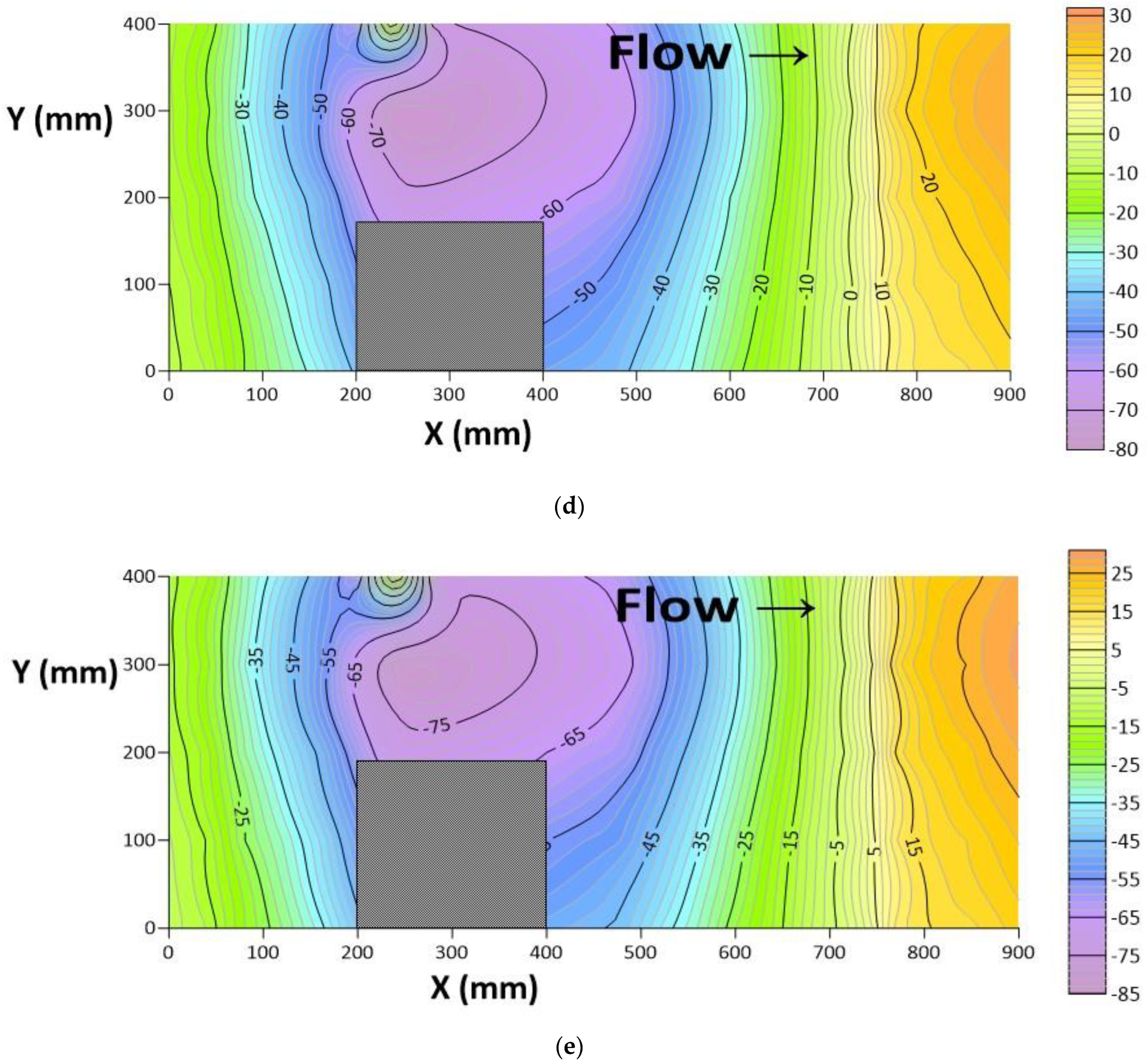
3.2. Scour Depth around Bridge Abutments with Marble Waste
3.3. Scour Depth around the Bridge Abutment with Brick Waste
3.4. Effect of Abutment Length and Water Depth on Scour
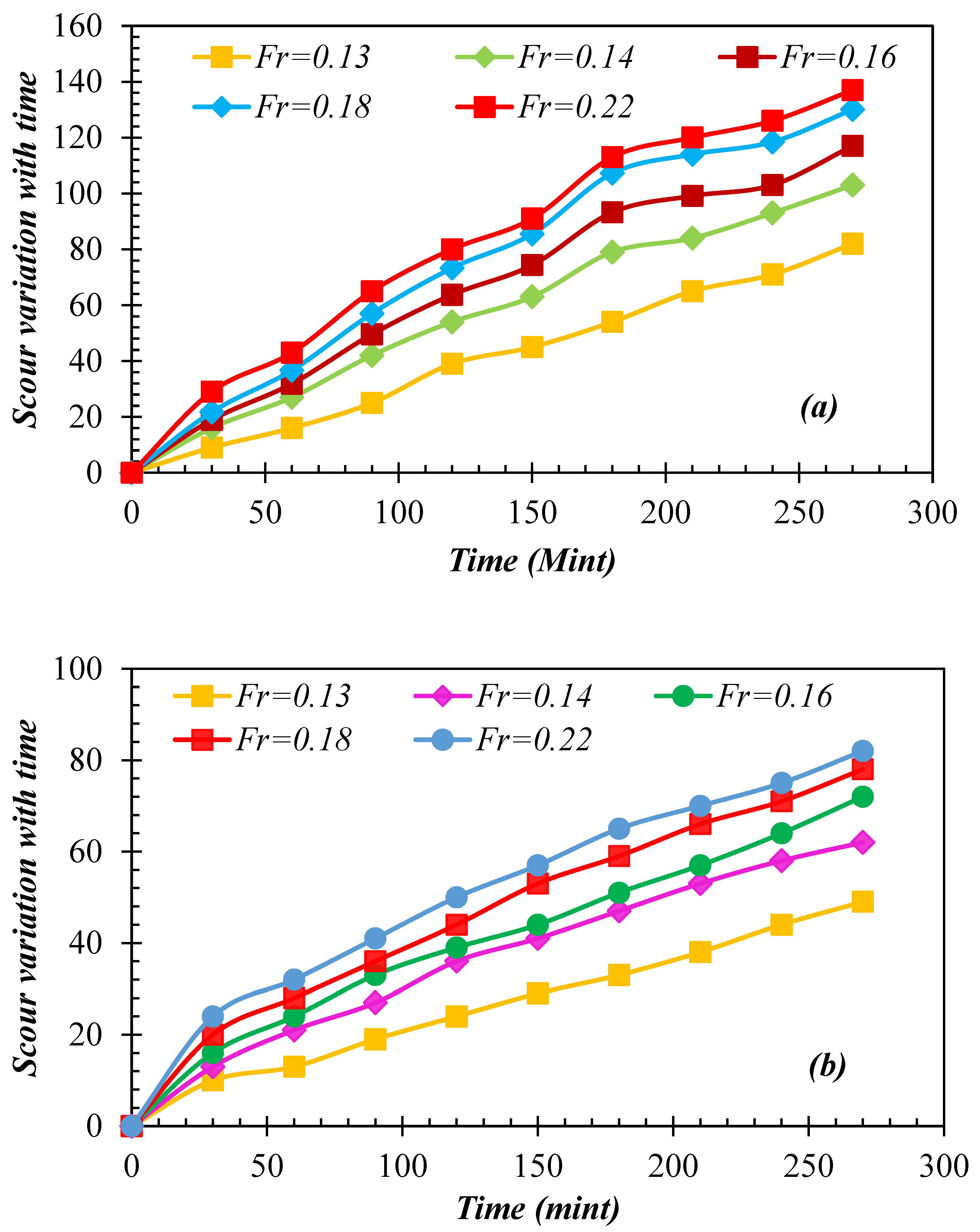
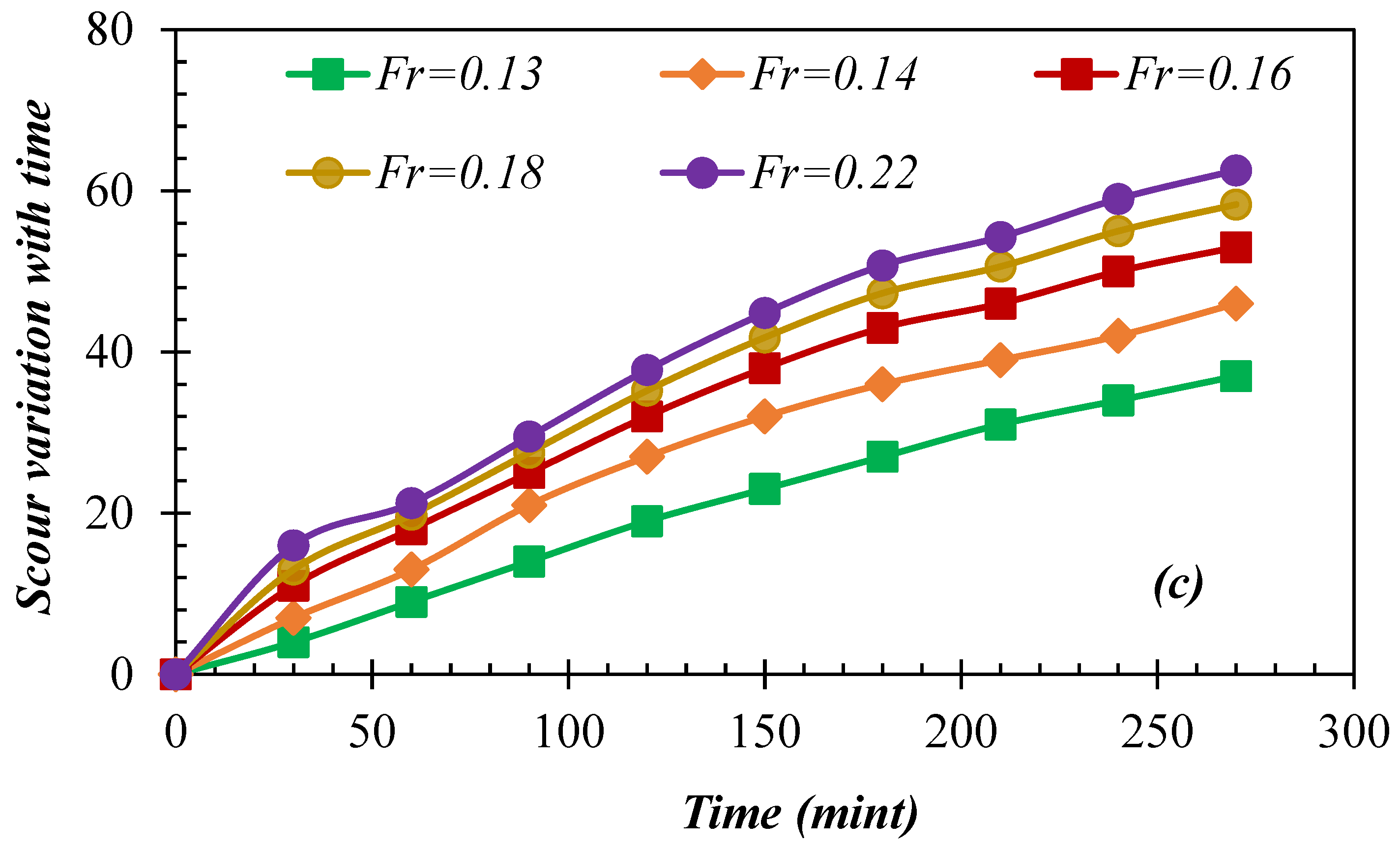
3.5. Temporal Variation of Scour
4. Discussion
5. Conclusions
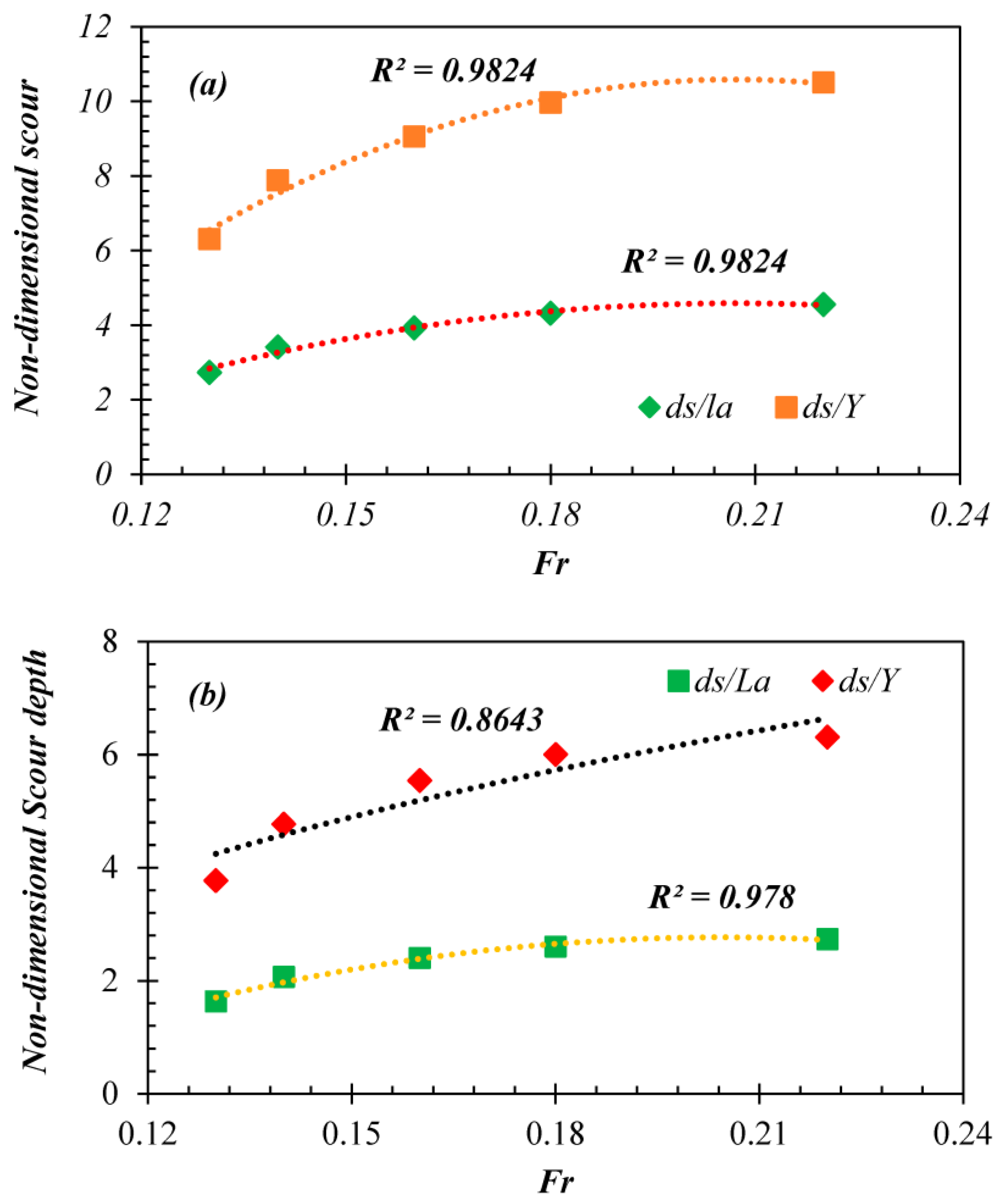
- The scour depth around the bridge abutment increased by increasing the initial Froude’s number. The maximum increment in a scour depth without countermeasure for the range of Froude’s number from 0.13 to 0.22 was observed to be 40%. This was because increasing Froude’s number implies that an increase in a flow velocity that interacts with the exterior face of abutments directly results in significant scouring.
- When marble waste was used as a countermeasure, it was noticed that some particles (marble waste) flowed with water and some were deposited near the exterior face of an abutment, which caused resistance to the flow against scouring. Therefore, the maximum reduction in scouring around the abutment was noticed compared to without any countermeasure. The maximum reduction in scour depth was observed to be 55% when marble was used as a countermeasure. But scour depth increases with increasing flow discharge, and the minimum and maximum scour depths were 0.037 m and 0.062 m, respectively.
- The maximum reduction in scour depth was observed to be 40% when brick waste was used as a countermeasure. The scour depth increases with increasing flow discharge, and the minimum and maximum scour depths were 0.049 m and 0.083 m, respectively.
- In the case when marble waste was used as a countermeasure, it was noticed that scour reduction was 15% higher compared to the case when brick waste was used as a countermeasure. This difference in scour reduction was because of the sample size in both scenarios. The size of the marble waste was larger than the brick waste, which was retained near the exterior face of the abutment, which caused a greater reduction in scour depth, whereas most of the brick waste used to flow with water and exposed the exterior face of the abutment after some time, hence causing a smaller reduction in scour depth.
- For considering the case without countermeasure, the maximum ds/La (4.55) and ds/Y (10.55) were observed for the Froude’s number of 0.22. The maximum ds/La and ds/Y for marble and brick waste were observed to be 2.30, 4.71, and 2.73, 6.3, respectively. The maximum reduction in ds/La and ds/Y when marble waste was used as a countermeasure was 55%, and for brick waste, it was reduced up to 40% and 57%, respectively.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Melville, B.W. Pier and abutment scour: Integrated approach. J. Hydraul. Eng. 1997, 123, 125–136. [Google Scholar] [CrossRef]
- Dey, S.; Sarkar, A. Scour downstream of an apron due to submerged horizontal jets. J. Hydraul. Eng. 2006, 132, 246–257. [Google Scholar] [CrossRef]
- Farooq, R.; Ghumman, A.R. Impact Assessment of Pier Shape and Modifications on Scouring around Bridge Pier. Water 2019, 11, 1761. [Google Scholar] [CrossRef]
- Kumar, V.; Raju, K.G.R.; Vittal, N. Reduction of local scour around bridge piers using slots and collars. J. Hydraul. Eng. 1999, 125, 1302–1305. [Google Scholar] [CrossRef]
- Breusers, H.; Nicollet, G.; Shen, H. Local scour around cylindrical piers. J. Hydraul. Res. 1977, 15, 211–252. [Google Scholar] [CrossRef]
- Kayatürk, Ş.Y. Scour and Scour Protection at Bridge Abutments. Ph.D. Thesis, Middle East Technical University, Ankara, Turkey, 2005. [Google Scholar]
- Al-Shukur, A.H.K.; Obeid, Z.H. Experimental study of bridge pier shape to minimize local scour. Int. J. Civ. Eng. Technol. 2016, 7, 162–171. [Google Scholar]
- Melville, B.W. Local scour at bridge abutments. J. Hydraul. Eng. 1992, 118, 615–631. [Google Scholar] [CrossRef]
- Kw, R.T.; Melville, B.W. Local scour and flow measurements at bridge abutments. J. Hydraul. Res. 1994, 32, 661–673. [Google Scholar]
- Dey, S.; Bose, S.K.; Sastry, G.L. Clear water scour at circular piers: A model. J. Hydraul. Eng. 1995, 121, 869–876. [Google Scholar] [CrossRef]
- Vittal, N.; Kothyari, U.; Haghighat, M. Clear water scour around bridge pier group. J. Hydraul. Eng. 1994, 120, 1309–1318. [Google Scholar] [CrossRef]
- Tafarojnoruz, A.; Gaudio, R.; Dey, S. Flow-altering countermeasures against scour at bridge piers: A review. J. Hydraul. Res. 2010, 48, 441–452. [Google Scholar] [CrossRef]
- Farooq, R.; Ghumman, A.R.; Tariq, M.A.U.R.; Ahmed, A.; Jadoon, K.Z. Optimal Octagonal Hooked Collar Countermeasure to Reduce Scour Around a Single Bridge Pier. Period. Polytech. Civ. Eng. 2020, 64, 1026–1037. [Google Scholar] [CrossRef]
- Hosseinjanzadeh, H.; Khozani, Z.S.; Ardeshir, A.; Singh, V.P. Experimental investigation into the use of collar for reducing scouring around short abutments. ISH J. Hydraul. Eng. 2019, 27, 616–632. [Google Scholar] [CrossRef]
- Chiew, Y.-M. Scour protection at bridge piers. J. Hydraul. Eng. 1992, 118, 1260–1269. [Google Scholar] [CrossRef]
- Chiew, Y.-M. Scour and scour countermeasures at bridge sites. Trans. Tianjin Univ. 2008, 14, 289–295. [Google Scholar] [CrossRef]
- Dey, S.; Raikar, R.V. Clear water scour at piers in sand beds with an armor layer of gravels. J. Hydraul. Eng. 2007, 133, 703–711. [Google Scholar] [CrossRef]
- Melville, B.W.; Coleman, S.E. Bridge Scour; Water Resources Publication: Littleton, CO, USA, 2000. [Google Scholar]
- Whitehouse, R. Scour at Marine Structures: A Manual for Practical Applications; Thomas Telford: London, UK, 1998. [Google Scholar]
- Ettema, R. Scour at Bridge Piers; No. 216 Monograph; The National Academies of Sciences, Engineering, and Medicine: Washington, DC, USA, 1980. [Google Scholar]
- Alabi, P.D. Time Development of Local Scour at a Bridge Pier Fitted with a Collar. Ph.D. Thesis, University of Saskatchewan, Saskatoon, SK, Canada, 2006. [Google Scholar]
- Kumcu, Ş.Y.; Gögüş, M.; Kökpinar, M.A. Temporal scour development at bridge abutments with a collar. Can. J. Civ. Eng. 2007, 34, 549–556. [Google Scholar] [CrossRef]
- Kumcu, S.Y.; Kokpinar, M.A.; Gogus, M. Scour protection around vertical-wall bridge abutments with collars. KSCE J. Civ. Eng. 2014, 18, 1884–1895. [Google Scholar] [CrossRef]
- Li, H.; Kuhnle, R.; Barkdoll, B. Countermeasures against Scour at Abutments; Research Report No. 49; National Sedimentation Laboratory: Oxford, MS, USA, 2006.
- Ballio, F.; Teruzzi, A.; Radice, A. Constriction effects in clear-water scour at abutments. J. Hydraul. Eng. 2009, 135, 140–145. [Google Scholar] [CrossRef]
- Fathi, A.; Zomorodian, S.M.A. Effect of Submerged Vanes on Scour around a Bridge Abutment. KSCE J. Civ. Eng. 2018, 22, 2281–2289. [Google Scholar] [CrossRef]
- Alhaddad, S.; Helmons, R. Sediment Erosion Generated by a Coandă-Effect-Based Polymetallic-Nodule Collector. J. Mar. Sci. Eng. 2023, 11, 349. [Google Scholar] [CrossRef]
- Fathi, A.; Zomorodian, S.M.A.; Zolghadr, M.; Chadee, A.; Chiew, Y.-M.; Kumar, B.; Martin, H. Combination of Riprap and Submerged Vane as an Abutment Scour Countermeasure. Fluids 2023, 8, 41. [Google Scholar] [CrossRef]
- Osroush, M.; Hosseini, S.A.; Kamanbedast, A.A.; Khosrojerdi, A. The effects of height and vertical position of slot on the reduction of scour hole depth around bridge abutments. Ain Shams Eng. J. 2019, 10, 651–659. [Google Scholar] [CrossRef]
- Wang, S.; Wei, K.; Shen, Z.; Xiang, Q. Experimental investigation of local scour protection for cylindrical bridge piers using anti-scour collars. Water 2019, 11, 1515. [Google Scholar] [CrossRef]
- Lauchlan, C.S.; Melville, B.W. Riprap protection at bridge piers. J. Hydraul. Eng. 2001, 127, 412–418. [Google Scholar] [CrossRef]
- Cardoso, A.H.; Fael, C.M. Protecting vertical-wall abutments with riprap mattresses. J. Hydraul. Eng. 2009, 135, 457–465. [Google Scholar] [CrossRef]
- Gaudio, R.; Tafarojnoruz, A.; Calomino, F. Combined flow-altering countermeasures against bridge pier scour. J. Hydraul. Res. 2012, 50, 35–43. [Google Scholar] [CrossRef]
- Dey, S.; Raikar, R.V. Characteristics of horseshoe vortex in developing scour holes at piers. J. Hydraul. Eng. 2007, 133, 399–413. [Google Scholar] [CrossRef]
- Akib, S.; Mamat, N.L.; Basser, H.; Jahangirzadeh, A. Reducing local scouring at bridge piles using collars and geobags. Sci. World J. 2014, 2014, 128635. [Google Scholar] [CrossRef]
- Gupta, L.K.; Pandey, M.; Raj, P.A.; Pu, J.H. Scour Reduction around Bridge Pier Using the Airfoil-Shaped Collar. Hydrology 2023, 10, 77. [Google Scholar] [CrossRef]
- Gupta, L.K.; Pandey, M.; Raj, P.A. Numerical simulation of local scour around the pier with and without airfoil collar (AFC) using FLOW-3D. Environ. Fluid Mech 2023, 1–19. [Google Scholar] [CrossRef]
- Farooq, R.; Azimi, A.H.; Tariq, M.A.U.R.; Ahmed, A. Effects of hooked-collar on the local scour around a lenticular bridge pier. Int. J. Sediment Res. 2023, 38, 1–11. [Google Scholar] [CrossRef]
- Hakim, M.; Yarahmadi, M.B.; Kashefipour, S.M. Use of spur dikes with different permeability levels for protecting bridge abutment against local scour under unsteady flow conditions. Can. J. Civ. Eng. 2022, 49, 1842–1854. [Google Scholar] [CrossRef]
- Bejestan, M.S.; Khademi, K.; Kozeymehnezhad, H. Submerged vane-attached to the abutment as scour countermeasure. Ain Shams Eng. J. 2015, 6, 775–783. [Google Scholar] [CrossRef]
- Mohamed, Y.A.; Nasr-Allah, T.H.; Abdel-Aal, G.M.; Awad, A.S. Investigating the effect of curved shape of bridge abutment provided with collar on local scour, experimentally and numerically. Ain Shams Eng. J. 2015, 6, 403–411. [Google Scholar] [CrossRef]
- Barkdoll, B.; Kuhnle, R. Bridge abutment collar as a scour countermeasure. In Impacts of Global Climate Change; ASCE Publishing: Reston, VA, USA, 2005; pp. 1–12. [Google Scholar]
- Gogus, M.; Dogan, A.E. Effects of collars on scour reduction at bridge abutments. In Scour and Erosion; ASCE Publishing: Reston, VA, USA, 2010; pp. 997–1007. [Google Scholar]
- Karami, H.; Hosseinjanzadeh, H.; Hosseini, K.; Ardeshir, A. Scour and three-dimensional flow field measurement around short vertical-wall abutment protected by collar. KSCE J. Civ. Eng. 2018, 22, 141–152. [Google Scholar] [CrossRef]
- Fahad, M.; Iqbal, Y.; Riaz, M.; Ubic, R.; Redfern, S.A.T. Metamorphic temperature investigation of coexisting calcite and dolomite marble––Examples from Nikani Ghar marble and Nowshera Formation, Peshawar Basin, Pakistan. J. Earth Sci. 2016, 27, 989–997. [Google Scholar] [CrossRef]
- Azhar, M.N.; Qureshi, L.A. Experimental study of structural behavior of Chinese bond brick Masonry: A new trend of durable & economical construction in Pakistan. J. King Saud Univ.-Eng. Sci. 2022, 34, 155–162. [Google Scholar] [CrossRef]
- Vaghefi, M.; Akbari, M.; Fiouz, A.R. An experimental study of mean and turbulent flow in a 180-degree sharp open channel bend: Secondary flow and bed shear stress. KSCE J. Civ. Eng. 2016, 20, 1582–1593. [Google Scholar] [CrossRef]
- Martinez, J.; Reca, J.; Morillas, M.T.; Lopez, J.G. Design and calibration of a compound sharp crested weir. J. Hydraul. Engg. ASCE 2005, 131, 112–116. [Google Scholar] [CrossRef]
- Sharafati, A.; Tafarojnoruz, A.; Shourian, M.; Yaseen, Z.M. Simulation of the depth scouring downstream sluice gate: The validation of newly developed data-intelligent models. J. Hydro-Environ. Res. 2020, 29, 20–30. [Google Scholar] [CrossRef]
- Sharafati, A. New stochastic modeling strategy on the prediction enhancement of pier scour depth in cohesive bed materials. J. Hydroinform. 2020, 22, 457–472. [Google Scholar] [CrossRef]
- Pagliara, S.; Kurdistani, S.M.; Cammarata, L. Scour of clear water rock W-weirs in straight rivers. J. Hydraul. Eng. 2014, 140, 06014002. [Google Scholar] [CrossRef]
- Hosseini, S.A.; Osroush, M.; Kamanbedast, A.A.; Khosrojerrdi, A. The effect of slot dimensions and its vertical and horizontal position on the scour around bridge abutments with vertical walls. Sādhanā 2020, 45, 157. [Google Scholar] [CrossRef]
- Osroush, M.; Hosseini, S.A.; Kamanbedast, A.A. Countermeasures against local scouring around bridge abutments: Combined system of collar and slot. Iran. J. Sci. Technol. Trans. Civ. Eng. 2021, 45, 11–25. [Google Scholar] [CrossRef]
- Saad, N.Y.; Fattouh, E.M.; Mokhtar, M. Effect of L-shaped slots on scour around a bridge abutment. Water Pract. Technol. 2021, 16, 935–945. [Google Scholar] [CrossRef]
- Melville, B.; van Ballegooy, S.; Coleman, S.; Barkdoll, B. Countermeasure toe protection at spill-through abutments. J. Hydraul. Eng. 2006, 132, 235–245. [Google Scholar] [CrossRef]
- Melville, B.; van Ballegooy, S.; Coleman, S.; Barkdoll, B. Scour countermeasures for wing-wall abutments. J. Hydraul. Eng. 2006, 132, 563–574. [Google Scholar] [CrossRef]
- Melville, B.W.; van Ballegooy, S.; Coleman, S.E.; Barkdoll, B. Riprap size selection at wing-wall abutments. J. Hydraul. Eng. 2007, 133, 1265–1269. [Google Scholar] [CrossRef]
- Morales, R.; Ettema, R.; Barkdoll, B. Large-scale flume tests of riprap-apron performance at a bridge abutment on a floodplain. J. Hydraul. Eng. 2008, 134, 800–809. [Google Scholar] [CrossRef]
- Oben-Nyarko, K.; Ettema, R. Pier and abutment scour interaction. J. Hydraul. Eng. 2011, 137, 1598–1605. [Google Scholar] [CrossRef]
- Yorozuya, A.; Ettema, R. Three abutment scour conditions at bridge waterways. J. Hydraul. 2015, 141, 04015028. [Google Scholar] [CrossRef]
- Sturm, T.W. Scour around Bankline and setback abutments in compound channels. J. Hydraul. Eng. 2006, 132, 21–32. [Google Scholar] [CrossRef]
- Sturm, T.W.; Melville, B.; Stoesser, T.; Abt, S.; Hong, S.; Abid, I.; Xiong, X.; Bugallo, B.F.; Chua, K.V. Combining Individual Scour Components to Determine Total Scour; Final Report, National Cooperative Highway Research Program (NCHRP) Project 24-37, Web-Only Document 249, Transportation Research Board; National Academy: Washington, DC, USA, 2018; Available online: http://nap.edu/25046 (accessed on 5 October 2023).
- Abid, I. Interaction of Pier, Contraction, and Abutment Scour in Clear Water Scour Conditions. Ph.D. Thesis, School of Civil and Environmental Engineering, Georgia Institute of Technology, Atlanta, GA, USA, 2017. [Google Scholar]
- Ettema, R.; Bergendahl, B.S.; Yorozuya, A.; Idil-Bektur, P. Breaching of bridge abutments and scour at exposed abutment columns. J. Hydraul. Eng. 2016, 142, 06016010. [Google Scholar] [CrossRef]
- Hong, S.H.; Sturm, T.W.; Stoesser, T. Clear water abutment scours in a compound channel for extreme hydrologic events. J. Hydraul. Eng. 2015, 141, 04015005. [Google Scholar] [CrossRef]
- Ballio, F.; Radice, A.; Dey, S. Temporal scales for live-bed scour at abutments. J. Hydraul. Eng. 2010, 136, 395–402. [Google Scholar] [CrossRef]
- Hong, S.H.; Abid, I. Scour around an erodible abutment with riprap apron over time. J. Hydraul. Eng. 2019, 145, 06019007. [Google Scholar] [CrossRef]
- Proske, D. Bridge Collapse Frequencies versus Failure Probabilities; Springer International Publishing: Cham, Switzerland, 2018. [Google Scholar]
- Pizarro, A.; Manfreda, S.; Tubaldi, E. The science behind scour at bridge foundations: A review. Water 2020, 12, 374. [Google Scholar] [CrossRef]
- Ettema, R.; Constantinescu, G.; Melville, B.W. Flow-field complexity and design estimation of pier-scour depth: Sixty years since Laursen and Toch. J. Hydraul. Eng. 2017, 143, 03117006. [Google Scholar] [CrossRef]
- Odgaard, A.J.; Wang, Y. Sediment management with submerged vanes. I: Theory. J. Hydraul. Eng. 1991, 117, 267–283. [Google Scholar]
- Chiew, Y.M. Mechanics of riprap failure at bridge piers. J. Hydraul. Eng. 1995, 121, 635–643. [Google Scholar] [CrossRef]
- Valela, C.; Nistor, I.; Rennie, C.D.; Lara, J.L.; Maza, M. Hybrid Modeling for Design of a Novel Bridge Pier Collar for Reducing Scour. J. Hydraul. Eng. 2021, 147, 04021012. [Google Scholar] [CrossRef]
- Simarro, G.; Civeira, S.; Cardoso, A.H. Influence of riprap apron shape on spill-through abutments. J. Hydraul. Res. 2012, 50, 138–141. [Google Scholar] [CrossRef]
- Ghorbani, B.; Kells, J.A. Effect of submerged vanes on the scour occurring at a cylindrical pier. J. Hydraul. Res. 2008, 46, 610–619. [Google Scholar] [CrossRef]
- Zolghadr, M.; Bejestan, M.S. Six-legged concrete (SLC) elements as scour countermeasures at wing wall bridge abutments. Int. J. River Basin Manag. 2021, 19, 319–325. [Google Scholar] [CrossRef]
- Valela, C.; Whittaker, C.N.; Rennie, C.D.; Nistor, I.; Melville, B.W. Novel Riprap Structure for Improved Bridge Pier Scour Protection. J. Hydraul. Eng. 2022, 148, 04022002. [Google Scholar] [CrossRef]
- Cope, E.D. Environmentally Friendly and Sustainable Stream Stability in the Vicinity of Bridges. Master’s Thesis, Brigham Young University, Provo, UT, USA, 2022. [Google Scholar]
- Bhuiyan, F.; Hey, R.D.; Wormleaton, P.R. Hydraulic evaluation of W-weir for river restoration. J. Hydraul. Eng. 2007, 133, 596–609. [Google Scholar] [CrossRef]






| Scour Countermeasures | Bridge Structure | Maximum % Age of Scour Reduction | References |
|---|---|---|---|
| Steel Collar and Geobags | Pier | 96 | [35] |
| Combination of collar and bed sill | Pier | 68 | [13] |
| Airfoil collar (Experimental) | Pier | 100 | [36] |
| Airfoil collar (Numerical) | Pier | 11–100 | [37] |
| Hooked collar | Lenticular Pier | 58 | [38] |
| Octagonal Pier | 73 | [14] | |
| Spur dike | Abutment | 47 | [39] |
| Submerged vane | Abutment | 95 | [40] |
| Riprap and submerged vane | Abutment | 54 | [28] |
| Collar and slot | Abutment | 100 | [41] |
| Collar | Abutment | 77 | [23] |
| Collar | Abutment | 96 | [42] |
| Collar | Abutment | 87 | [43] |
| Collar | Abutment | 100 | [44] |
| Collar | Abutment | 100 | [45] |
| Collar | Abutment | 88 | [15,46] |
| Q (m3/s) | d50 | U (m/s) | Y (m) | Fr | Uc (m/s) | La (m) |
|---|---|---|---|---|---|---|
| 0.019 | 0.00051 | 0.14 | 0.13 | 0.13 | 0.008 | 0.3 |
| 0.021 | 0.00051 | 0.16 | 0.13 | 0.14 | 0.009 | 0.3 |
| 0.023 | 0.00051 | 0.18 | 0.13 | 0.16 | 0.010 | 0.3 |
| 0.027 | 0.00051 | 0.21 | 0.13 | 0.18 | 0.012 | 0.3 |
| 0.033 | 0.00051 | 0.25 | 0.13 | 0.22 | 0.014 | 0.3 |
| Froude’s Number (Fr) | ds without Countermeasure (mm) | ds with Bricks Waste (mm) | ds with Marble Waste (mm) | ds/Y & ds/La (without Countermeasure) | ds/Y & ds/La (with Bricks Waste) | ds/Y & ds/La (with Marble Waste) |
|---|---|---|---|---|---|---|
| 0.13 | 82 | 49 | 37 | 6.3 & 2.7 | 3.7 & 1.6 | 2.85 & 1.23 |
| 0.14 | 103 | 62 | 46 | 7.8 & 3.4 | 4.7 & 2 | 3.56 & 1.53 |
| 0.16 | 118 | 71 | 53 | 9.06 & 3.9 | 5.5 & 2.4 | 4.08 & 1.77 |
| 0.18 | 130 | 78 | 58 | 9.9 & 4.32 | 6 & 2.6 | 4.49 & 1.94 |
| 0.22 | 137 | 82 | 61 | 10.5 & 4.55 | 6.3 & 2.7 | 4.71 & 2.3 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Murtaza, N.; Khan, Z.U.; Khedher, K.M.; Amir, R.A.; Khan, D.; Salem, M.A.; Alsulamy, S. Mitigating Scour at Bridge Abutments: An Experimental Investigation of Waste Material as an Eco-Friendly Solution. Water 2023, 15, 3798. https://doi.org/10.3390/w15213798
Murtaza N, Khan ZU, Khedher KM, Amir RA, Khan D, Salem MA, Alsulamy S. Mitigating Scour at Bridge Abutments: An Experimental Investigation of Waste Material as an Eco-Friendly Solution. Water. 2023; 15(21):3798. https://doi.org/10.3390/w15213798
Chicago/Turabian StyleMurtaza, Nadir, Zaka Ullah Khan, Khaled Mohamed Khedher, Rana Adnan Amir, Diyar Khan, Mohamed Abdelaziz Salem, and Saleh Alsulamy. 2023. "Mitigating Scour at Bridge Abutments: An Experimental Investigation of Waste Material as an Eco-Friendly Solution" Water 15, no. 21: 3798. https://doi.org/10.3390/w15213798
APA StyleMurtaza, N., Khan, Z. U., Khedher, K. M., Amir, R. A., Khan, D., Salem, M. A., & Alsulamy, S. (2023). Mitigating Scour at Bridge Abutments: An Experimental Investigation of Waste Material as an Eco-Friendly Solution. Water, 15(21), 3798. https://doi.org/10.3390/w15213798







