An Algebraic Non-Equilibrium Turbulence Model of the High Reynolds Number Transition Region
Abstract
:1. Introduction
2. The High Reynolds Number Transition Region
3. Model Overview
3.1. Basic Model
3.2. Turbulent Mixing Length Scales
3.3. Turbulence Intensity
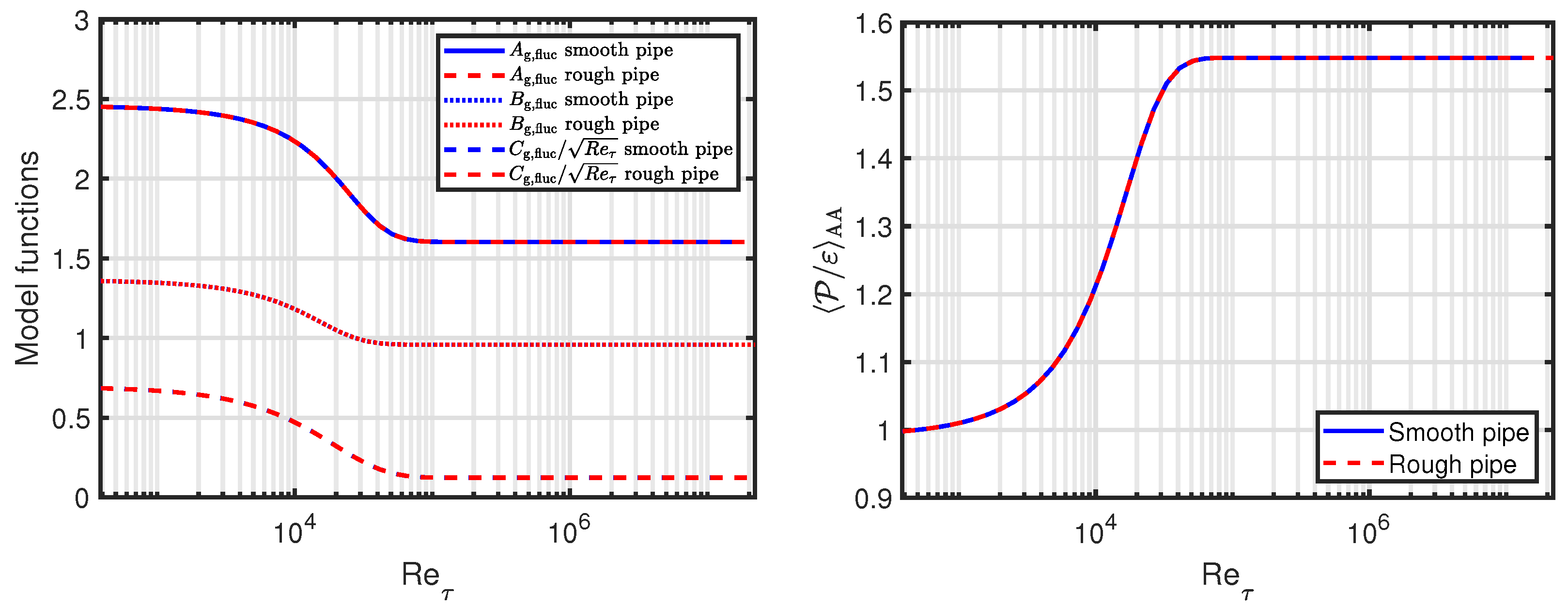
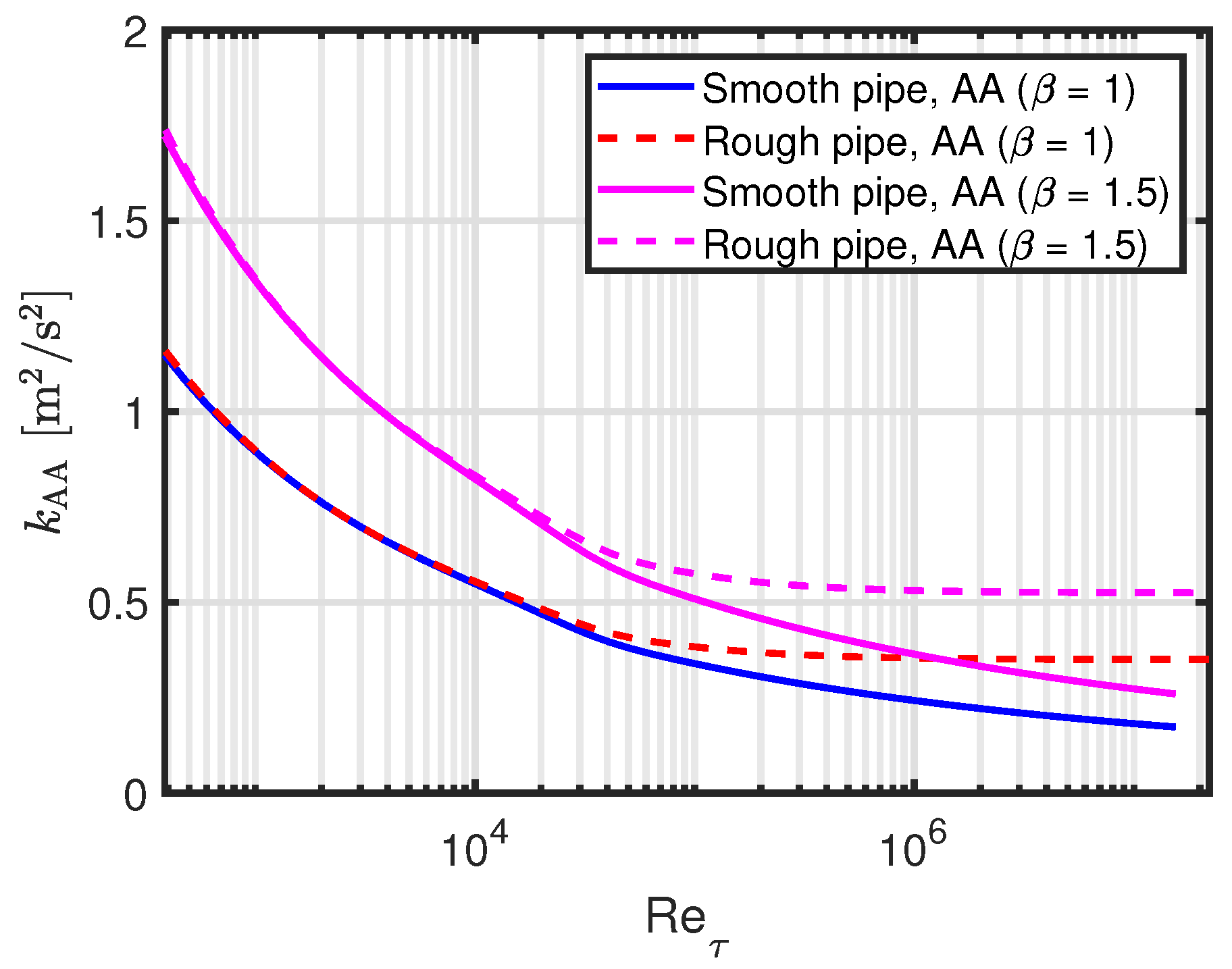
4. Model Results
4.1. Model Output
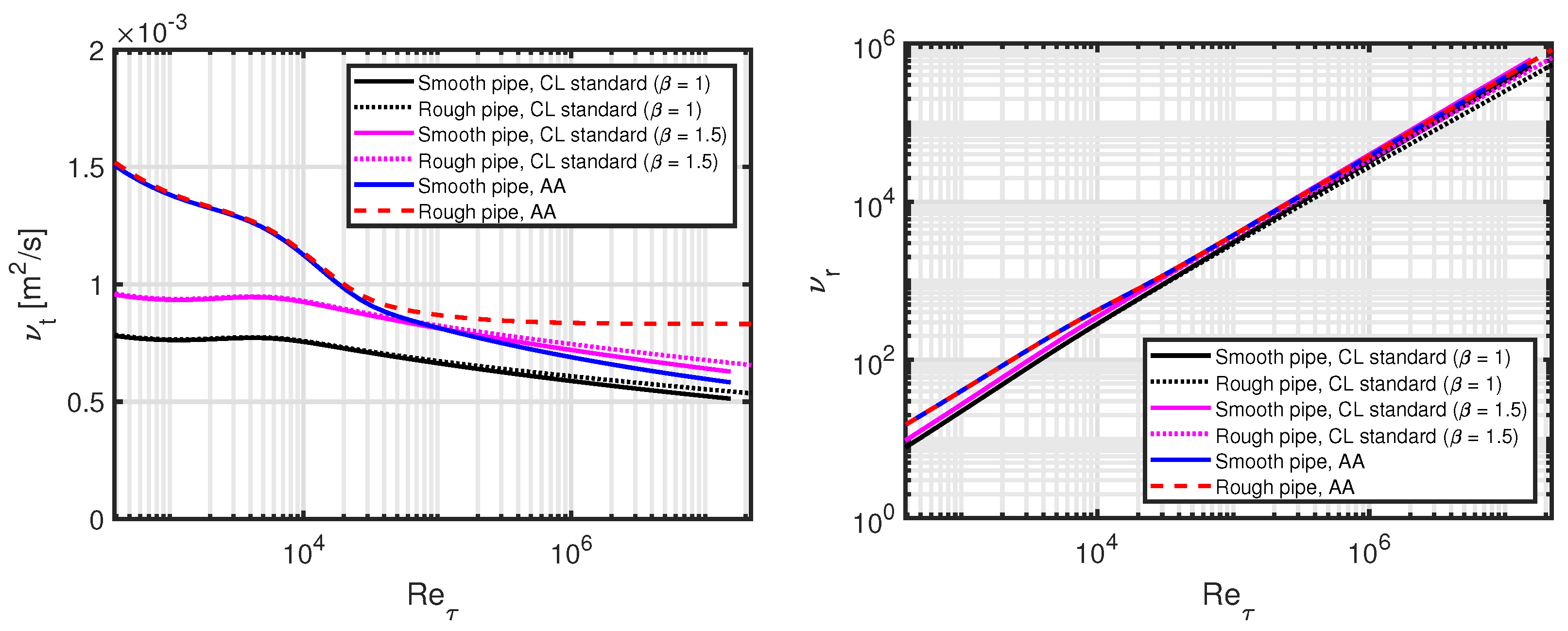
4.1.1. Quantities Depending on TKE, but Not Mixing Length
4.1.2. Quantities Depending on Mixing Length, but Not TKE
5. Discussion
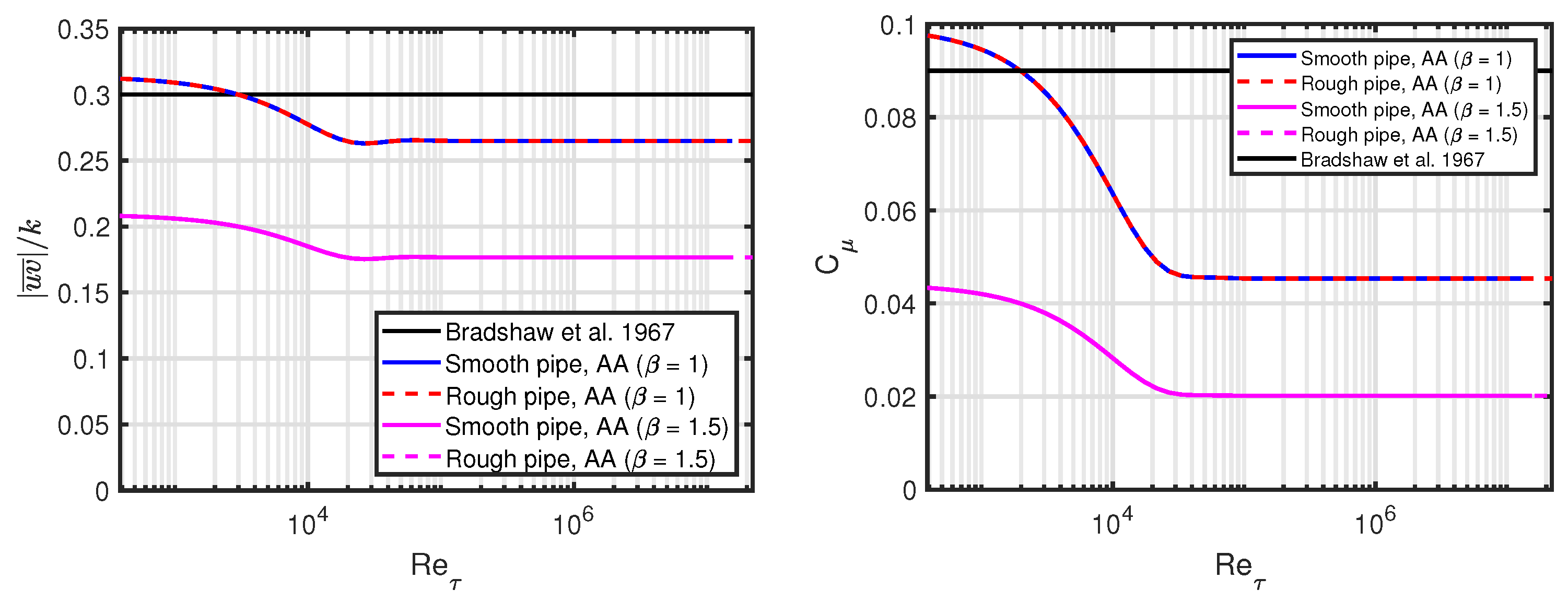
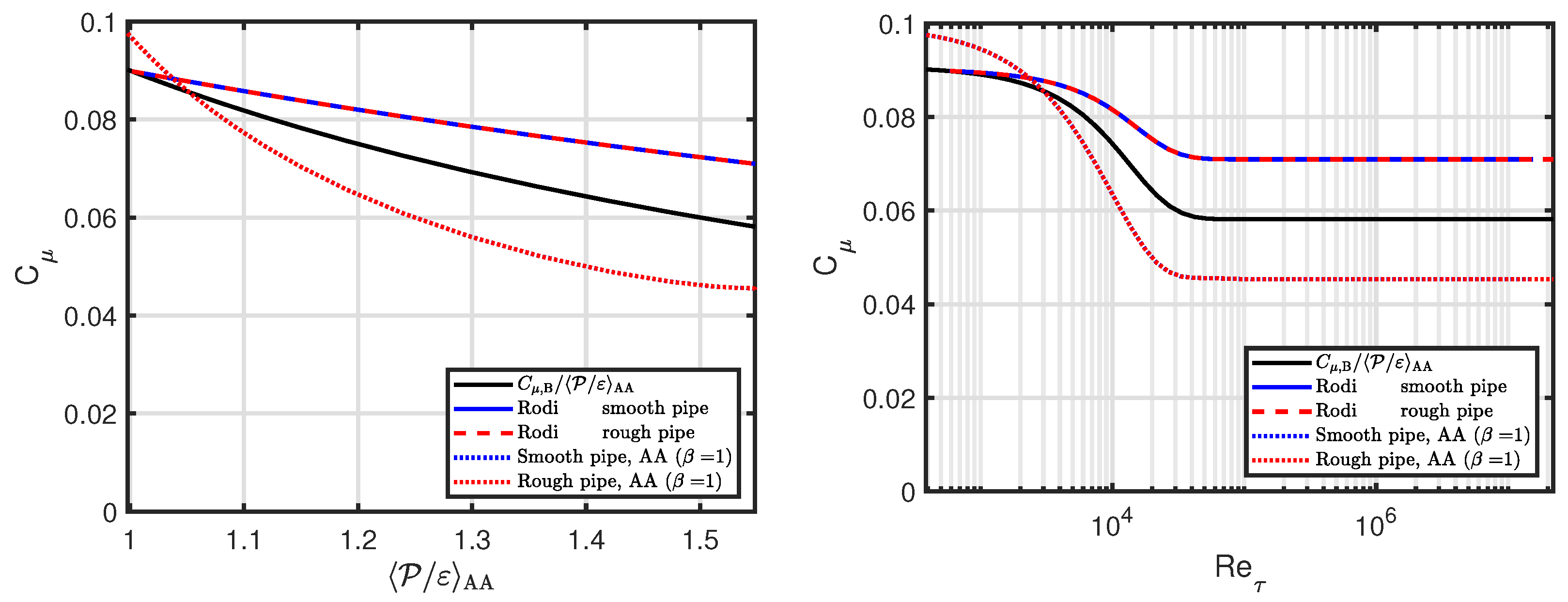
5.1. Turbulence Isotropy
5.2. Physical Mechanism
5.3. Scaling of
5.4. Scaling of and I
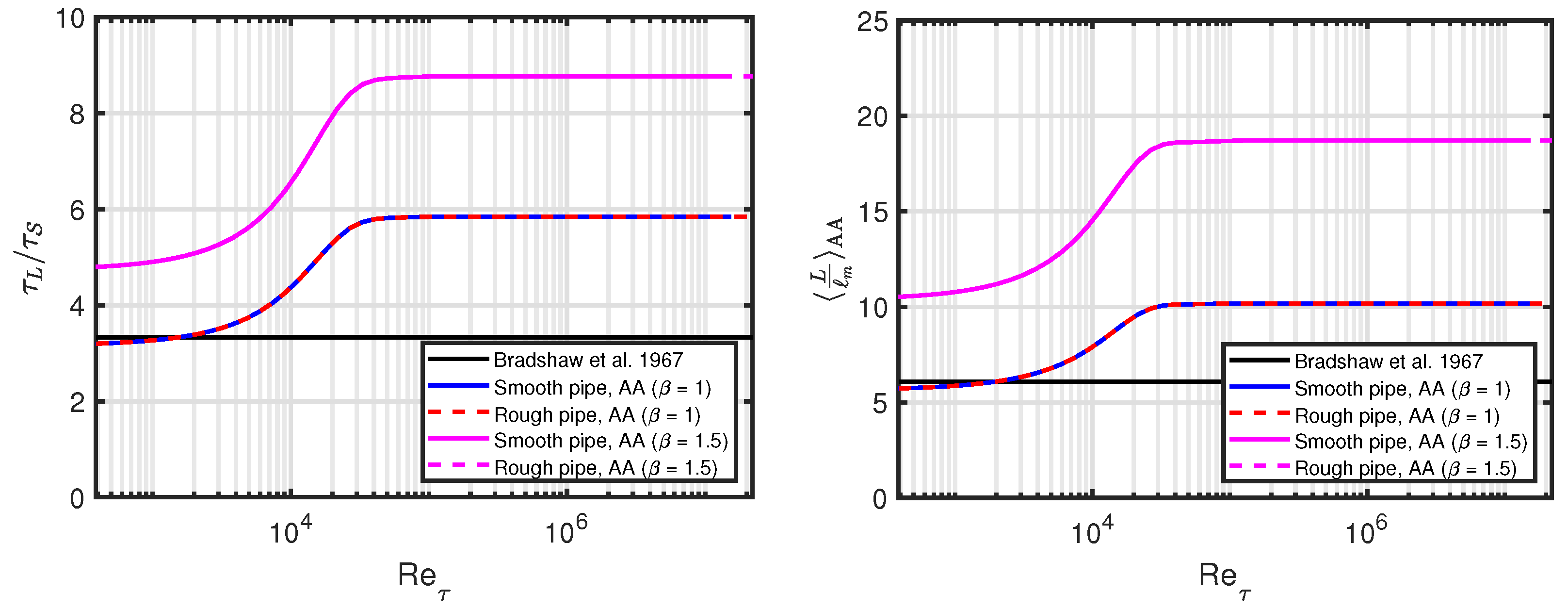
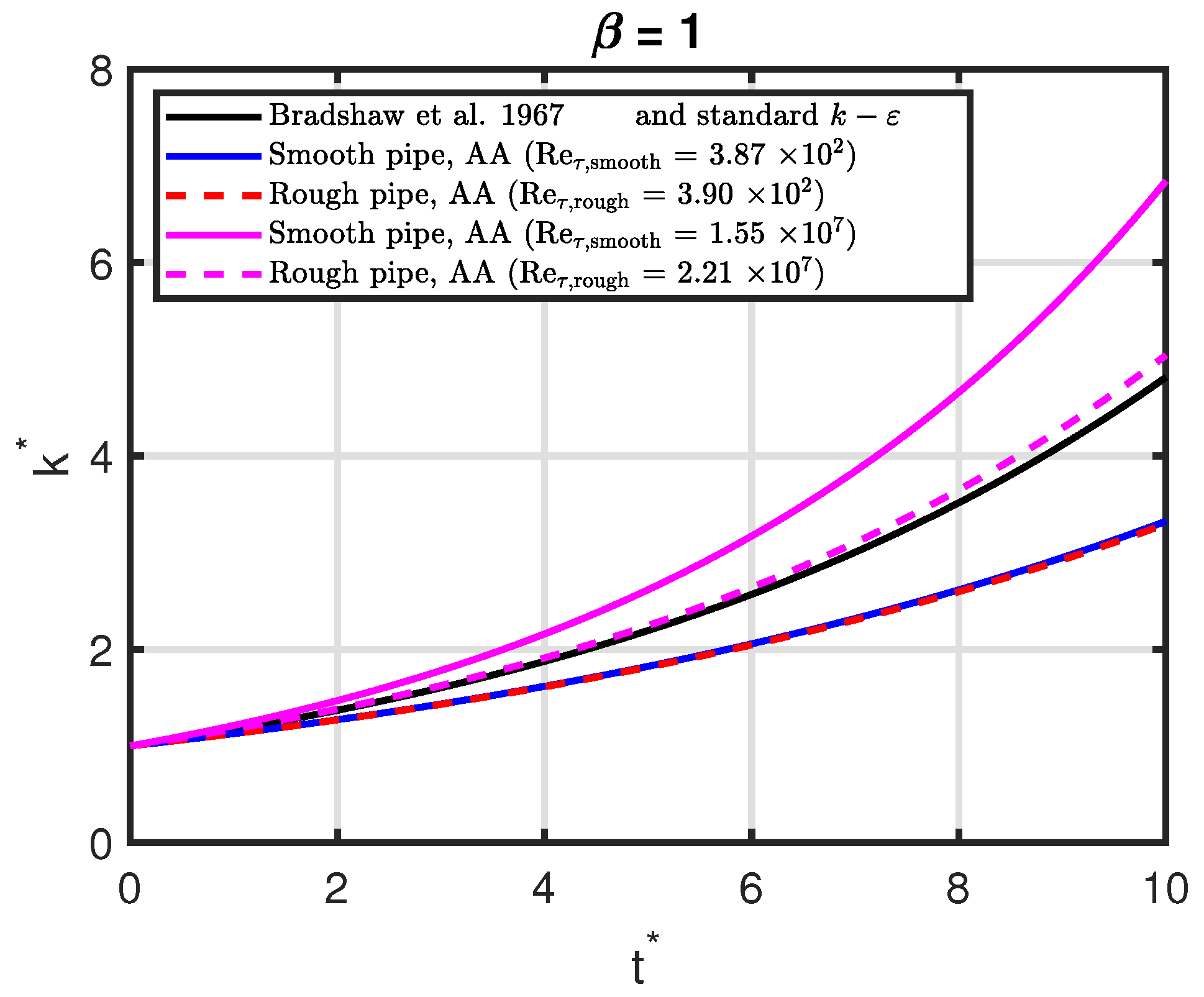
5.5. Time Scales
5.5.1. Turbulence Model with Two Time Scales
5.5.2. Eddy-Turnover Time Scales
5.5.3. Time Evolution of TKE
- Decaying Turbulence
5.6. A Plasma Physics Analogy
5.7. Recommendations for CFD Practitioners
5.7.1. Equilibrium Usage as Inlet Boundary Conditions for CFD Simulations
- L: The expressions are taken from [11]. Note that .
5.7.2. Non-Equilibrium Usage as a Standalone Model
5.8. Known Model Issues
6. Conclusions
Supplementary Materials
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Basse, N.T. Mind the Gap: Boundary Conditions for Turbulence Modelling. Available online: https://www.researchgate.net/publication/359218404_Mind_the_Gap_Boundary_Conditions_for_Turbulence_Modelling (accessed on 6 September 2023).
- Hultmark, M.; Vallikivi, M.; Bailey, S.C.C.; Smits, A.J. Logarithmic scaling of turbulence in smooth- and rough-wall pipe flow. J. Fluid Mech. 2013, 728, 376–395. [Google Scholar] [CrossRef]
- Smits, A.J. Princeton Superpipe Measurements. Available online: https://smits.princeton.edu/superpipe-turbulence-data (accessed on 6 September 2023).
- Basse, N.T. Scaling of global properties of fluctuating and mean streamwise velocities in pipe flow: Characterization of a high Reynolds number transition region. Phys. Fluids 2021, 33, 065127. [Google Scholar] [CrossRef]
- Basse, N.T. Scaling of global properties of fluctuating streamwise velocities in pipe flow: Impact of the viscous term. Phys. Fluids 2021, 33, 125109. [Google Scholar] [CrossRef]
- Prandtl, L. Bericht über Untersuchungen zur ausgebildeten Turbulenz. Z. Angew. Math. Mech. 1925, 5, 136–139. [Google Scholar] [CrossRef]
- Prandtl, L. Bericht über neuere Turbulenzforschung. In Hydraulische Probleme; VDI-Verlag: Berlin, Germany, 1926. [Google Scholar]
- Versteeg, H.K.; Malalasekera, W. An Introduction to Computational Fluid Dynamics, 2nd ed.; Pearson: London, UK, 2007. [Google Scholar]
- Greenshields, C.J.; Weller, H.G. Notes on Computational Fluid Dynamics: General Principles; CFD Direct Ltd.: Reading, UK, 2022. [Google Scholar]
- Rodriguez, S. Applied Computational Fluid Dynamics and Turbulence Modeling; Springer: Cham, Switzerland, 2019. [Google Scholar]
- Basse, N.T. Supplementary Information: An Algebraic Non-Equilibrium Turbulence Model of the High Reynolds Number Transition Region. Available online: https://www.researchgate.net/publication/373108195_Supplementary_Information_An_algebraic_non-equilibrium_turbulence_model_of_the_high_Reynolds_number_transition_region (accessed on 6 September 2023).
- von Kármán, T.H. Nachrichten von der Gesellschaft der Wissenschaften zu Göttingen, Mathematisch-Physikalische Klasse. Mech. Aenlichkeit Turbul. 1930, 1930, 58–76. [Google Scholar]
- Perry, A.E.; Henbest, S.; Chong, M.S. A theoretical and experimental study of wall turbulence. J. Fluid Mech. 1986, 165, 163–199. [Google Scholar] [CrossRef]
- Davidson, P.A.; Krogstad, P.-Å. A simple model for the streamwise fluctuations in the log-law region of a boundary layer. Phys. Fluids 2009, 21, 055105. [Google Scholar] [CrossRef]
- Basse, N.T. Turbulence intensity scaling: A fugue. Fluids 2019, 4, 180. [Google Scholar] [CrossRef]
- Bradshaw, P.; Ferriss, D.H.; Atwell, N.P. Calculation of boundary-layer development using the turbulent energy equation. J. Fluid Mech. 1967, 28, 593–616. [Google Scholar] [CrossRef]
- Gersten, K.; Herwig, H. Strömungsmechanik; Vieweg: Wiesbaden, Germany, 1992. [Google Scholar]
- Schlichting, H.; Gersten, K. Boundary-Layer Theory, 8th ed.; Springer: Berlin/Heidelberg, Germany, 2000. [Google Scholar]
- Prandtl, L. Der Luftwiderstand von Kugeln. Nachrichten der Gesellschaft der Wissenschaften zu Göttingen; Springer: Berlin/Heidelberg, Germany, 1914. [Google Scholar]
- Cogo, M.; Salvadore, F.; Picano, F.; Bernardini, M. Direct numerical simulation of supersonic and hypersonic turbulent boundary layers at moderate-high Reynolds numbers and isothermal wall condition. J. Fluid Mech. 2022, 945, A30. [Google Scholar] [CrossRef]
- Pirozzoli, S.; Romero, J.; Fatica, M.; Verzicco, R.; Orlandi, P. One-point statistics for turbulent pipe flow up to Reτ≈6000. J. Fluid Mech. 2021, 926, A28. [Google Scholar] [CrossRef]
- Chi, C.; Thévenin, D.; Smits, A.J.; Wolligandt, S.; Theisel, H. Identification and analysis of very-large-scale turbulent motions using multiscale proper orthogonal decomposition. Phys. Rev. Fluids 2022, 7, 084603. [Google Scholar] [CrossRef]
- Deshpande, R.; de Silva, C.M.; Marusic, I. Evidence that superstructures comprise self-similar coherent motions in high Reynolds number boundary layers. J. Fluid Mech. 2023, 969, A10. [Google Scholar] [CrossRef]
- Smits, A.J. Batchelor Prize Lecture: Measurements in wall-bounded turbulence. J. Fluid Mech. 2022, 940, A1. [Google Scholar] [CrossRef]
- Rodi, W. The Prediction of Free Turbulent Boundary Layers by Use of a Two-Equation Model of Turbulence. Ph.D. Thesis, Univerity of London, London, UK, 1972. [Google Scholar]
- Russo, F.; Basse, N.T. Scaling of turbulence intensity for low-speed flow in smooth pipes. Flow Meas. Instrum. 2016, 52, 101–114. [Google Scholar] [CrossRef]
- Hanjalić, K.; Launder, B.E.; Schiestel, R. Multiple-time-scale concepts in turbulent transport modelling. In Turbulent Shear Flows, 2nd ed.; Bradbury, L.J.S., Durst, F., Launder, B.E., Schmidt, F.W., Whitelaw, J.H., Eds.; Springer: Berlin/Heidelberg, Germany, 1980. [Google Scholar]
- Klein, T.S.; Craft, T.J.; Iacovides, H. The development and application of two-time-scale turbulence models for non-equilibrium flows. Int. J. Heat Fluid Flow 2018, 71, 334–352. [Google Scholar] [CrossRef]
- Hamlington, P.E.; Ihme, M. Modeling of non-equilibrium homogeneous turbulence in rapidly compressed flows. Flow Turbul. Combust. 2014, 93, 93–124. [Google Scholar] [CrossRef]
- Launder, B.E.; Spalding, D.B. The numerical computation of turbulent flows. Comput. Meth. Appl. Mech. Eng. 1974, 3, 269–289. [Google Scholar] [CrossRef]
- Jones, W.P.; Launder, B.E. The prediction of laminarization with a two-equation model of turbulence. Int. J. Heat Mass Transf. 1972, 15, 301–314. [Google Scholar] [CrossRef]
- Pope, S.B. Turbulent Flows; Cambridge University Press: Cambridge, UK, 2000. [Google Scholar]
- Speziale, C.G. Modeling non-equilibrium turbulent flows. In Modeling Complex Turbulent Flows; Salas, M.D., Hefner, J.N., Sakell, L., Eds.; Springer: Dordrecht, The Netherlands, 1999. [Google Scholar]
- Zoletnik, S.; Basse, N.P.; Saffman, M.; Svendsen, W.; Endler, M.; Hirsch, M.; Werner, A.; Fuchs, C.; The W7-AS Team. Changes in density fluctuations associated with confinement transitions close to a rational edge rotational transform in the W7-AS stellarator. Plasma Phys. Control. Fusion 2002, 44, 1581–1607. [Google Scholar] [CrossRef]
- Basse, N.P.; Michelsen, P.K.; Zoletnik, S.; Saffman, M.; Endler, M.; Hirsch, M. Spatial distribution of turbulence in the Wendelstein 7-AS stellarator. Plasma Sources Sci. Technol. 2002, 11, A138–A142. [Google Scholar] [CrossRef]
- Ansys Fluent User’s Guide, Release 2022 R2 (2022). Available online: https://support.ansys.com (accessed on 6 September 2023).
- Launder, B.E.; Sharma, B.I. Application of the energy-dissipation model of turbulence to the calculation of flow near a spinning disc. Lett. Heat Mass Transf. 1947, I, 131–138. [Google Scholar]
- Hoyas, S.; Oberlack, M.; Alcántara-Ávila, F.; Kraheberger, S.V.; Laux, J. Wall turbulence at high friction Reynolds numbers. Phys. Rev. Fluids 2022, 7, 014602. [Google Scholar] [CrossRef]
- Yao, J.; Chen, X.; Hussain, F. Direct numerical simulation of turbulent open channel flows at moderately high Reynolds numbers. J. Fluid Mech. 2022, 953, A19. [Google Scholar] [CrossRef]
- Pope, S.B. A more general effective-viscosity hypothesis. J. Fluid Mech. 1975, 72, 331–340. [Google Scholar] [CrossRef]
- Apsley, D.D. Turbulence Modelling. Available online: https://personalpages.manchester.ac.uk/staff/david.d.apsley/lectures/comphydr/index.htm (accessed on 6 September 2023).
- Boussinesq, J. Essai sur la théorie des eaux courantes. Mém. Présent Par Divers Savants l’Acad. Sci. 1877, 23, 1–680. [Google Scholar]
- Kolmogorov, A.N. The equations of turbulent motion in an incompressible fluid. Izv. Acad. Sci. USSR Phys. 1942, 6, 56–58. (In Russian) [Google Scholar]
- Prandtl, L. Über ein neues Formelsystem für die ausgebildete Turbulenz. Nachr. Akad. Wiss. Göttingen Math.-Phys. Klasse 1945, 6–19. [Google Scholar]
- Taylor, G.I. Statistical theory of turbulence, Parts I-IV. Proc. R. Soc. Lond. A 1935, 151, 421–478. [Google Scholar] [CrossRef]
- Wilcox, D.C. Turbulence Modeling for CFD, 3rd ed.; DCW Industries: La Canada Flintridge, CA, USA, 2006. [Google Scholar]
- Tennekes, H.; Lumley, J.L. A First Course in Turbulence; MIT Press: Cambridge, MA, USA, 1972. [Google Scholar]
- Nikuradse, J. Gesetzmäßigkeiten der turbulenten Strömung in Glatten Rohren. Vdi Forschungsheft 1932, 356, 1–36. [Google Scholar]
- Kármán, T.v. Mechanische Ähnlichkeit und Turbulenz. Nachrichten Ges. Wiss. Göttingen-Math.-Phys. Kl. 1930, 1930, 58–76. [Google Scholar]
- Cantwell, B.J. A universal velocity profile for smooth wall pipe flow. J. Fluid Mech. 2019, 878, 834–874. [Google Scholar] [CrossRef]
- Cantwell, B.J.; Bilgin, E.; Needels, J.T. A new boundary layer integral method based on the universal velocity profile. Phys. Fluids 2022, 34, 075130. [Google Scholar] [CrossRef]
- Greenshields, C. Private Communication, 2021.
- Basse, N.T. Extrapolation of turbulence intensity scaling to Reτ >> 105. Phys. Fluids 2022, 34, 075128. [Google Scholar] [CrossRef]



| Source | LIKE Algorithm | Equilibrium Model |
|---|---|---|
| L | ||
| I | ||
| K | ||
| E |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Basse, N.T. An Algebraic Non-Equilibrium Turbulence Model of the High Reynolds Number Transition Region. Water 2023, 15, 3234. https://doi.org/10.3390/w15183234
Basse NT. An Algebraic Non-Equilibrium Turbulence Model of the High Reynolds Number Transition Region. Water. 2023; 15(18):3234. https://doi.org/10.3390/w15183234
Chicago/Turabian StyleBasse, Nils T. 2023. "An Algebraic Non-Equilibrium Turbulence Model of the High Reynolds Number Transition Region" Water 15, no. 18: 3234. https://doi.org/10.3390/w15183234






