An Optimal Maintenance and Replacement Strategy for Deteriorating Water Mains
Abstract
1. Introduction
2. Degradation Model
2.1. Modelling Water Main Breaks as a Point Process
2.2. The Two-Time-Scale (TTS) Intensity Function
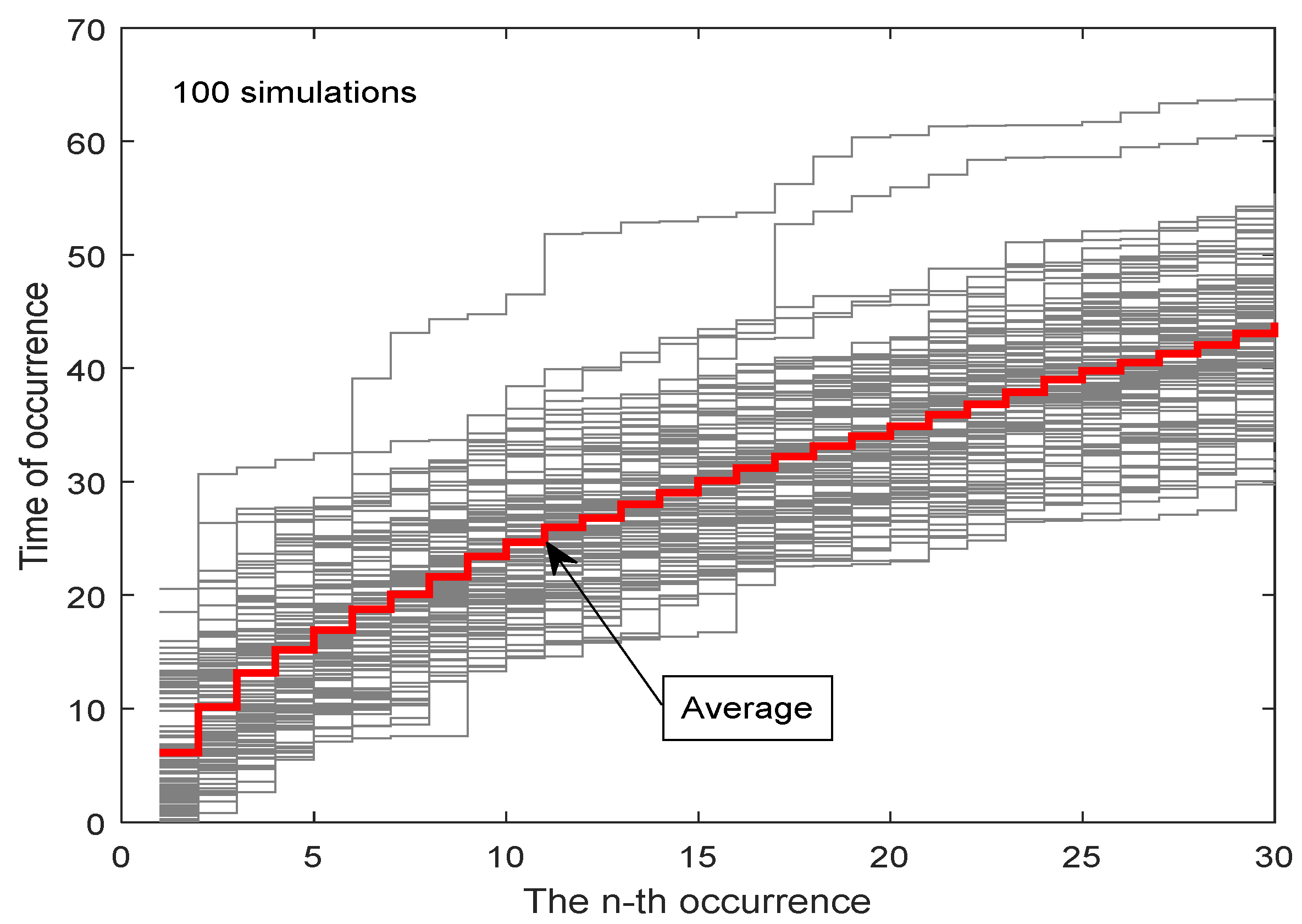
2.3. Simulating a TTS Point Process
3. Definition of Life Cycle Cost
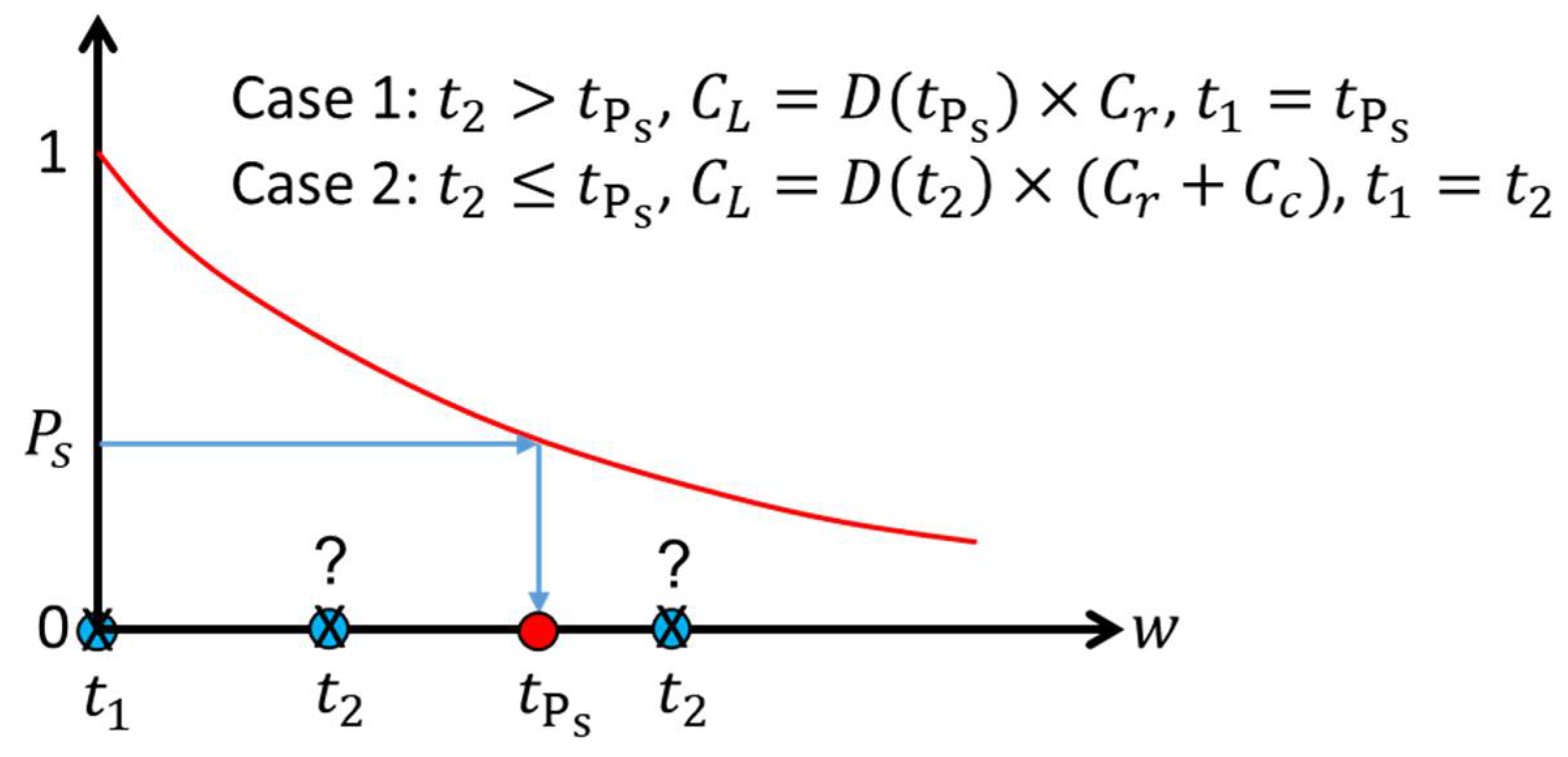
3.1. Corrective Strategy
3.2. Preventive Strategy
4. Results of Strategy Optimization
4.1. Corrective Strategy
4.2. Preventive Strategy
5. Case Study
6. Conclusions
- (1)
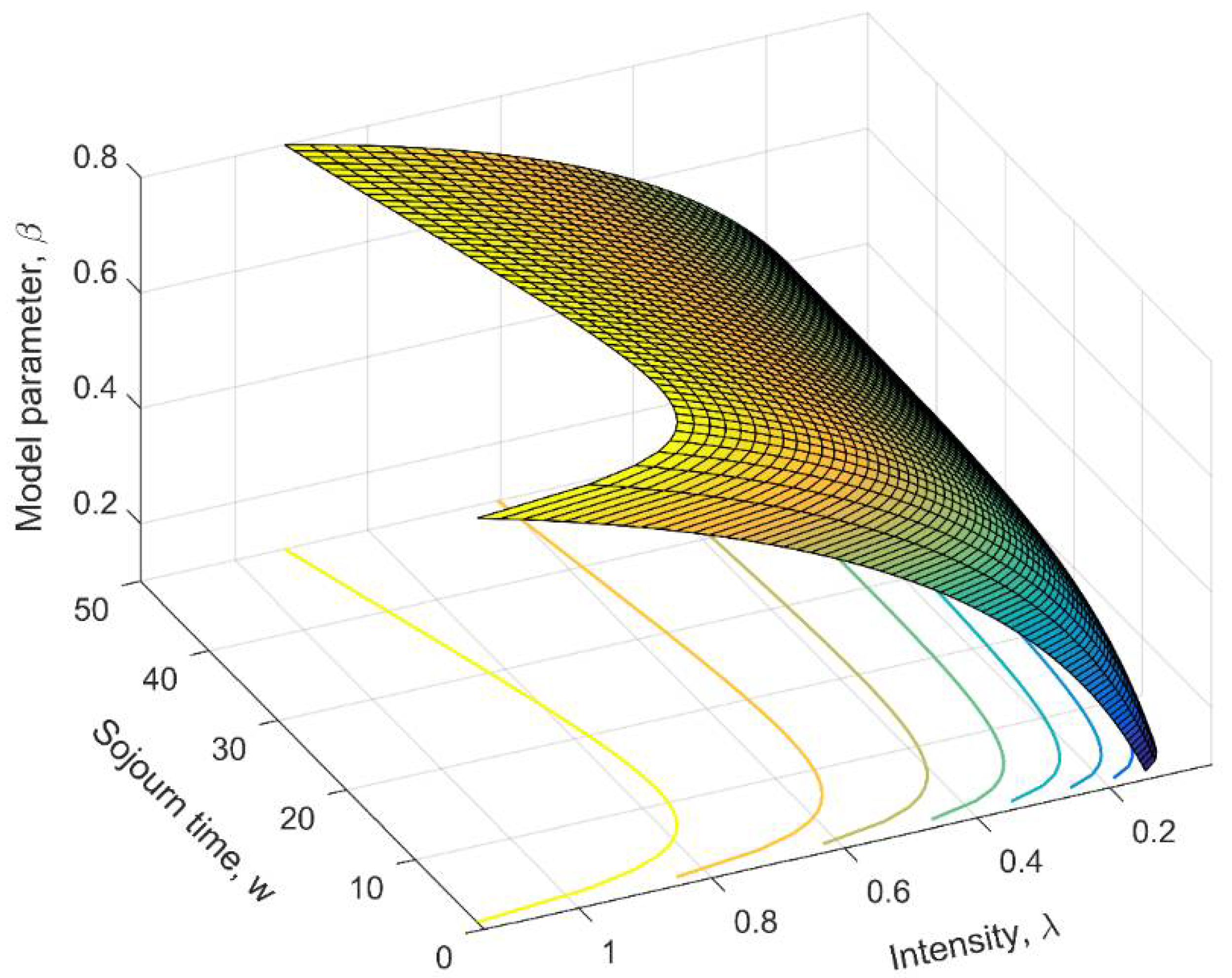
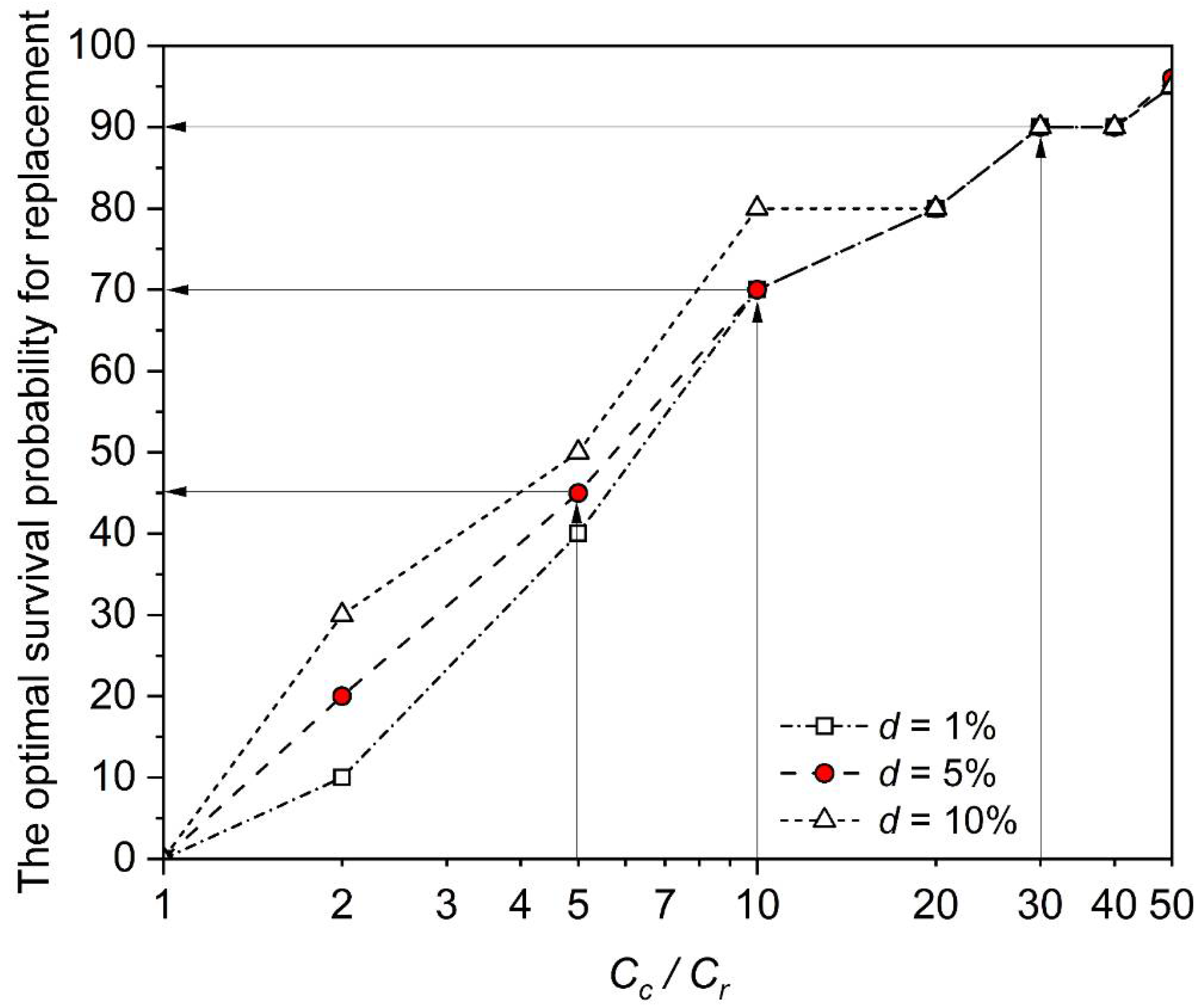
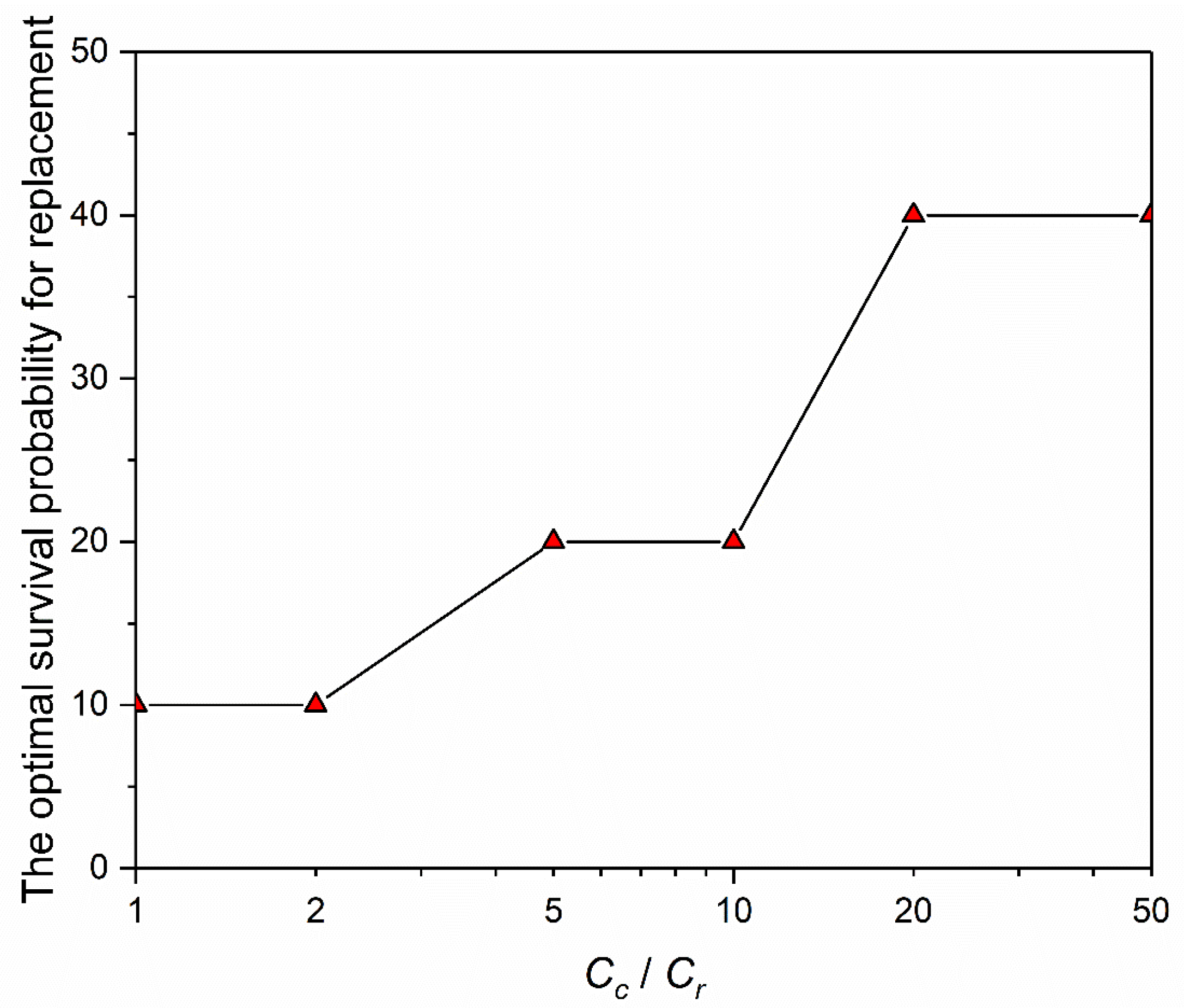
- Both of the optimal corrective and preventive strategies, optimal and , depend highly on the degradation intensity of water mains, as well as the ratios of maintenance, replacement, and failure consequence costs. For corrective strategy, the higher the breaking intensity, the higher the failure consequence loss, and the lower the replacement cost, the lower the optimal value. For preventive strategy, the higher the ratio of consequence loss to replacement cost, the higher the optimal . In addition, the effect of discounting rate diminishes when the ratio of consequence loss to replacement cost becomes large.
- (2)
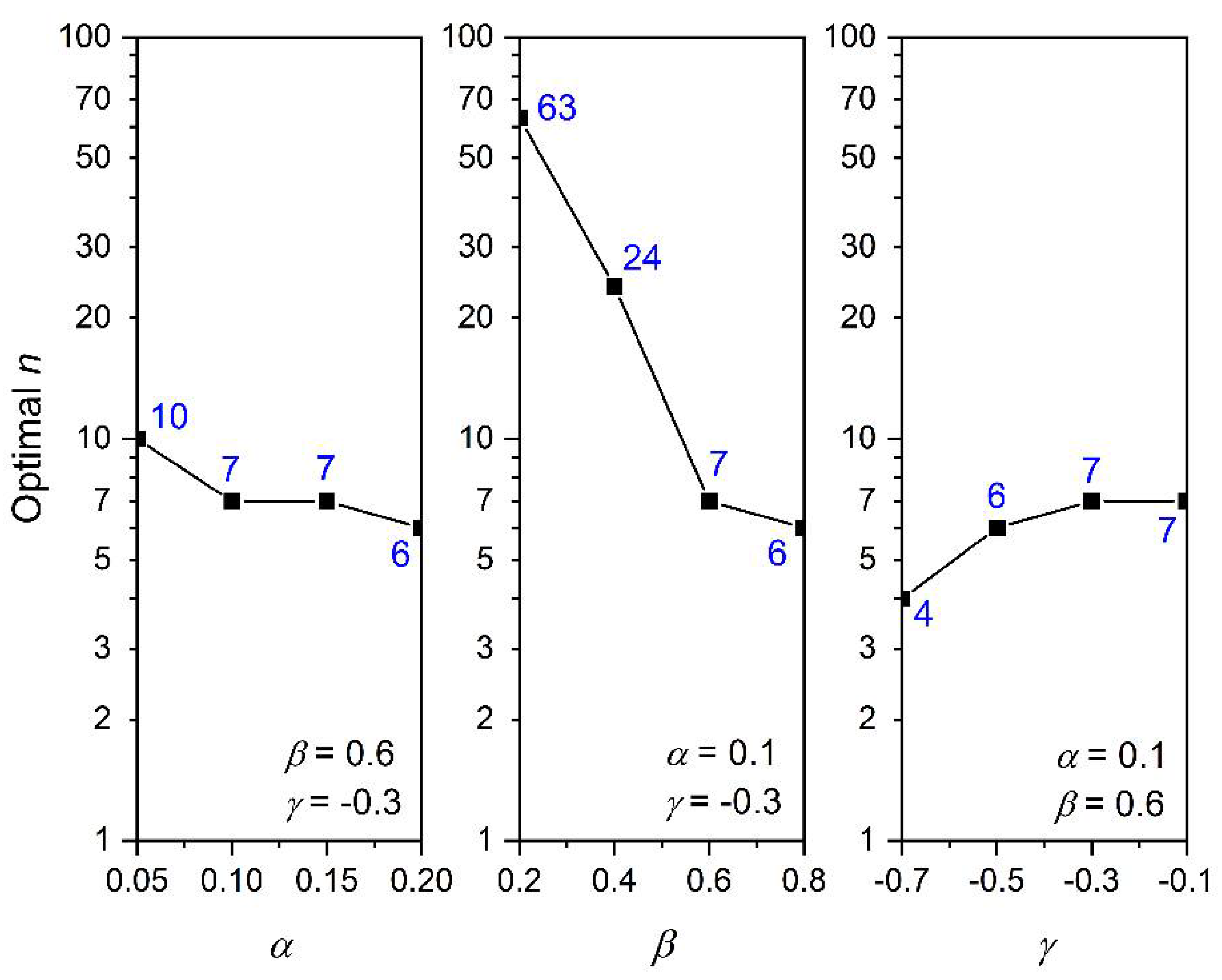
- The optimal maintenance and replacement strategies are sensitive to the overall shape parameter of the service age in the TTS intensity function, whereas the effects of the scale parameter and the local shape parameter of the sojourn time since the last break on the optimal and are comparatively secondary.
- (3)
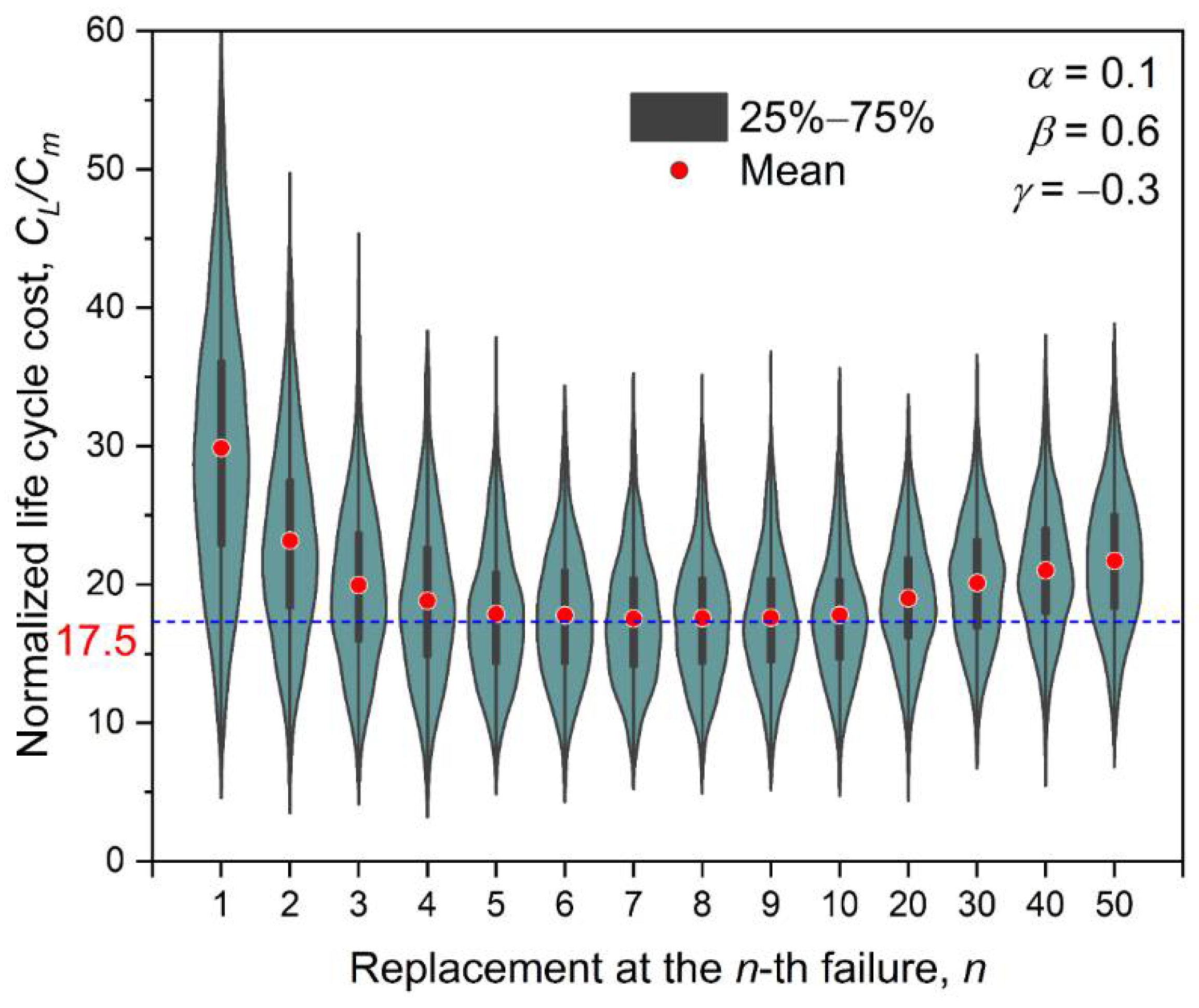
- A case study using a typical pipe diameter of 400 mm and length of 200 m shows that the optimal value is typically under 5, and the optimal lies below 50%.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Sköld, N.-P.; Bergion, V.; Lindhe, A.; Keucken, A.; Rosén, L. Risk-Based Evaluation of Improvements in Drinking Water Treatment Using Cost-Benefit Analysis. Water 2022, 14, 782. [Google Scholar] [CrossRef]
- Liu, Z.; Kleiner, Y.; Rajani, B.; Wang, L.; Condit, W. Condition Assessment Technologies for Water Transmission and Distribution Systems; United States Environmental Protection Agency (EPA): Washington, DC, USA, 2012; EPA/600/R-12/017; p. 108. [Google Scholar]
- Bergion, V.; Lindhe, A.; Sokolova, E.; Rosén, L. Accounting for Unexpected Risk Events in Drinking Water Systems. Expo. Health 2021, 13, 15–31. [Google Scholar] [CrossRef]
- Sjöstrand, K.; Klingberg, J.; Zadeh, N.; Haraldsson, M.; Rosén, L.; Lindhe, A. The Value of Water—Estimating Water-Disruption Impacts on Businesses. Water 2021, 13, 1565. [Google Scholar] [CrossRef]
- Sjöstrand, K.; Lindhe, A.; Söderqvist, T.; Rosén, L. Water Supply Delivery Failures—A Scenario-Based Approach to Assess Economic Losses and Risk Reduction Options. Water 2020, 12, 1746. [Google Scholar] [CrossRef]
- Scholten, L.; Scheidegger, A.; Reichert, P.; Mauer, M.; Lienert, J. Strategic rehabilitation planning of piped water networks using multi-criteria decision analysis. Water Res. 2014, 49, 124–143. [Google Scholar] [CrossRef] [PubMed]
- Sjöstrand, K.; Lindhe, A.; Söderqvist, T.; Rosén, L. Sustainability assessments of regional water supply interventions—Com-bining cost-benefit and multi-criteria decision analyses. J. Environ. Manag. 2018, 225, 313–324. [Google Scholar] [CrossRef]
- van Noortwijk, J.M. Explicit formulas for the variance of discounted life-cycle cost. Reliab. Eng. Syst. Saf. 2003, 80, 185–195. [Google Scholar] [CrossRef]
- van Noortwijk, J.M.; Frangopol, D.M. Two probabilistic life-cycle maintenance models for deteriorating civil infrastructures. Probabilistic Eng. Mech. 2003, 19, 345–359. [Google Scholar] [CrossRef]
- Jardine, A.K.; Tsang, A.H. Maintenance, Replacement, and Reliability: Theory and Applications; CRC Press: Boca Raton, FL, USA, 2005. [Google Scholar]
- Bull, J.W. Life Cycle Costing for the Analysis, Management and Maintenance of Civil Engineering Infrastructure; Whittles Publishing: Dunbeath, UK, 2015. [Google Scholar]
- Campbell, J.D.; Jardine, A.K.; McGlynn, J. Asset Management Excellence: Optimizing Equipment Life-Cycle Decisions; CRC Press: Boca Raton, FL, USA, 2016. [Google Scholar]
- Constantine, A.; Darroch, J. Pipeline Reliability. Stochastic Models in Engineering Technology & Management; Osaki, S., Murthy, D.N.P., Eds.; World Scientific Publishing: Singapore, 1993. [Google Scholar]
- Constantine, G.; Darroch, J.; Miller, R. Predicting underground pipeline failure. Water-Melb. Artarmon. 1996, 23, 9–10. [Google Scholar]
- Economou, T.; Kapelan, Z.; Bailey, T.C. On the prediction of underground water pipe failures: Zero inflation and pipe-specific effects. J. Hydroinform. 2012, 14, 872–883. [Google Scholar] [CrossRef][Green Version]
- Kleiner, Y.; Rajani, B. Using limited data to assess future needs. J. Am. Water Work. Assoc. 1999, 91, 47–61. [Google Scholar] [CrossRef]
- Kleiner, Y.; Rajani, B. I-WARP: Individual Water mAin Renewal Planner. Drink. Water Eng. Sci. 2010, 3, 71–77. [Google Scholar] [CrossRef]
- Le Gat, Y. Extending the Yule process to model recurrent pipe failures in water supply networks. Urban Water J. 2014, 11, 617–630. [Google Scholar] [CrossRef]
- Røstum, J. Statistical Modelling of Pipe Failures in Water Networks. Ph.D. Thesis, Norwegian University of Science and Technology, Trondheim, Norway, February 2000. [Google Scholar]
- Watson, T.G.; Christian, C.D.; Mason, A.J.; Smith, M.H.; Meyer, R. Bayesian-based pipe failure model. J. Hydroinform. 2004, 6, 259–264. [Google Scholar] [CrossRef][Green Version]
- Mailhot, A.; Pelletier, G.; Noël, J.-F.; Villeneuve, J.-P. Modeling the evolution of the structural state of water pipe networks with brief recorded pipe break histories: Methodology and application. Water Resour. Res. 2000, 36, 3053–3062. [Google Scholar] [CrossRef]
- Pelletier, G.; Mailhot, A.; Villeneuve, J.-P. Modeling water pipe breaks—Three case studies. J. Water Resour. Plan. Manag. 2003, 129, 115–123. [Google Scholar] [CrossRef]
- Scheidegger, A.; Scholten, L.; Maurer, M.; Reichert, P. Extension of pipe failure models to consider the absence of data from replaced pipes. Water Res. 2013, 47, 3696–3705. [Google Scholar] [CrossRef]
- Clair, A.M.S.; Sinha, S. State-of-the-technology review on water pipe condition, deterioration and failure rate prediction models. Urban Water J. 2012, 9, 85–112. [Google Scholar] [CrossRef]
- Kleiner, Y.; Rajani, B. Comprehensive review of structural deterioration of water mains: Statistical models. Urban Water 2001, 3, 131–150. [Google Scholar] [CrossRef]
- Nishiyama, M.; Filion, Y. Review of statistical water main break prediction models. Can. J. Civ. Eng. 2013, 40, 972–979. [Google Scholar] [CrossRef]
- Rajani, B.; Kleiner, Y. Comprehensive review of structural deterioration of water mains: Physically based models. Urban Water 2001, 3, 151–164. [Google Scholar] [CrossRef]
- Scheidegger, A.; Leitão, J.P.; Scholten, L. Statistical failure models for water distribution pipes—A review from a unified perspective. Water Res. 2015, 83, 237–247. [Google Scholar] [CrossRef] [PubMed]
- Termes-Rifé, M.; Molinos-Senante, M.; Hernández-Sancho, F.; Sala-Garrido, R. Life Cycle Costing: A tool to manage the urban water cycle. J. Water Supply Res. Technol. 2013, 62, 468–476. [Google Scholar] [CrossRef]
- Roshani, E.; Filion, Y.R. Event-Based Approach to Optimize the Timing of Water Main Rehabilitation with Asset Management Strategies. J. Water Resour. Plan. Manag. 2014, 140, 04014004. [Google Scholar] [CrossRef]
- Naoum-Sawaya, J.; Ghaddar, B.; Arandia, E.; Eck, B. Simulation-optimization approaches for water pump scheduling and pipe replacement problems. Eur. J. Oper. Res. 2015, 246, 293–306. [Google Scholar] [CrossRef]
- Lee, H.; Shin, H.; Rasheed, U.; Kong, M. Establishment of an Inventory for the Life Cycle Cost (LCC) Analysis of a Water Supply System. Water 2017, 9, 592. [Google Scholar] [CrossRef]
- Ghobadi, F.; Jeong, G.; Kang, D. Water Pipe Replacement Scheduling Based on Life Cycle Cost Assessment and Optimization Algorithm. Water 2021, 13, 605. [Google Scholar] [CrossRef]
- Lin, P.; Yuan, X.-X. A two-time-scale point process model of water main breaks for infrastructure asset management. Water Res. 2018, 150, 296–309. [Google Scholar] [CrossRef]
- Lin, P.Y.; Yuan, X.X.; Tovilla, E. Integrative modeling of performance deterioration and maintenance effectiveness for infrastructure assets with missing condition data. Comput. Aided Civ. Infrastruct. Eng. 2019, 34, 677–695. [Google Scholar] [CrossRef]
- Yuan, X.X. Principles and guidelines of deterioration modelling for water and waste water assets. Infrastruct. Asset. Manag. 2017, 4, 19–35. [Google Scholar] [CrossRef]
- Cox, D.R.; Isham, V. Point Processes; Taylor & Francis: Abingdon, UK, 1980. [Google Scholar]
- Cook, R.J.; Lawless, J.F. The Statistical Analysis of Recurrent Events; Springer: Berlin/Heidelberg, Germany, 2007. [Google Scholar] [CrossRef]
- Jacobsen, M. Point Process Theory and Applications: Marked Point and Piecewise Deterministic Processes; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2006. [Google Scholar]
- Daley, D.J.; Vere-Jones, D. An Introduction to the Theory of Point Processes: Volume II: General Theory and Structure; Springer: New York, NY, USA, 2007. [Google Scholar]
- Lin, P.; Yuan, X.-X. An Advanced Statistical Method for Point Process Modelling with Missing Event Histories. IEEE Access 2020, 8, 210081–210098. [Google Scholar] [CrossRef]
- Pietrucha-Urbanik, K. Failure Prediction in Water Supply System—Current Issues; Springer: Berlin/Heidelberg, Germany, 2015; pp. 351–358. [Google Scholar] [CrossRef]
- Pietrucha-Urbanik, K. Assessing the Costs of Losses Incurred as a Result of Failure; Springer: Berlin/Heidelberg, Germany, 2016; pp. 355–362. [Google Scholar] [CrossRef]
- Pietrucha-Urbanik, K.; Tchórzewska-Cieślak, B. Cost Analysis of Water Pipe Failure; Springer: Berlin/Heidelberg, Germany, 2020; pp. 411–424. [Google Scholar]
- Hashemi, B.; Najafi, M.; Mohamed, R. Cost of Underground Infrastructure Renewal: A Comparison of Open-Cut and Trenchless Methods. In Proceedings of the 12th International Pipelines Conference 2008, Atlanta, GA, USA, 22–27 July 2008. [Google Scholar]
- Najafi, M.; Gokhale, S.B. Trenchless Technology: Pipeline and Utility Design, Construction, and Renewal; McGraw-Hill Education: New York, NY, USA, 2005. [Google Scholar]
- Wu, Y.; Kang, C.; Nojumi, M.M.; Bayat, A.; Bontus, G. Current water main rehabilitation practice using trenchless technology. Water Pract. Technol. 2021, 16, 707–723. [Google Scholar] [CrossRef]
- Yang, M.-D.; Su, T.-C.; Wu, T.-Y.; Huang, K.-S. No-Dig Inspection Technologies for Underground Pipelines. J. GeoEng. 2010, 5, 99–104. [Google Scholar]
- Zhao, Y.; Ma, B.; Ariaratnam, S.T.; Zeng, C.; Yan, X.; Wang, F.; Wang, T.; Zhu, Z.; He, C.; Shi, G.; et al. Structural performance of damaged rigid pipe rehabilitated by centrifugal spray on mortar liner. Tunn. Undergr. Space Technol. 2021, 116, 104117. [Google Scholar] [CrossRef]
- Lemer, A. Life Cycle Costing: For the Analysis, Management and Maintenance of Civil Engineering Infrastructure. Constr. Manag. Econ. 2015, 33, 689–691. [Google Scholar] [CrossRef]
- van den Boomen, M.; Schoenmaker, R.; Wolfert, A.R.M. A life cycle costing approach for discounting in age and interval replacement optimisation models for civil infrastructure assets. Struct. Infrastruct. E 2018, 14, 1–13. [Google Scholar] [CrossRef]
- Mittal, A.; Scholten, L.; Kapelan, Z. A review of serious games for urban water management decisions: Current gaps and future research directions. Water Res. 2022, 215, 118217. [Google Scholar] [CrossRef]




| Case # | Model Parameter | Conditional Intensity, (Equation (2)) | |
|---|---|---|---|
| 1 | Monotonically increasing | ||
| 2 | Monotonically increasing | ||
| 3 | First decreasing, then increasing | ||
| 4 | Monotonically increasing | ||
| 5 | Constant | ||
| 6 | Monotonically decreasing | ||
| 7 | First increasing, then decreasing | ||
| 8 | Monotonically decreasing | ||
| 9 | Monotonically decreasing | ||
| Para. | Replacement at the -th Failure | |||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 20 | 30 | 40 | 50 | |
| Mean | 29.9 | 23.2 | 20.0 | 18.8 | 17.9 | 17.8 | 17.5 | 17.6 | 17.6 | 17.8 | 19.0 | 20.1 | 21.0 | 21.7 |
| Median | 29.3 | 22.6 | 19.5 | 18.4 | 17.6 | 17.6 | 17.1 | 17.5 | 17.2 | 17.5 | 18.8 | 20.1 | 20.8 | 21.7 |
| COV | 0.32 | 0.30 | 0.30 | 0.30 | 0.28 | 0.27 | 0.27 | 0.26 | 0.26 | 0.26 | 0.23 | 0.22 | 0.23 | 0.23 |
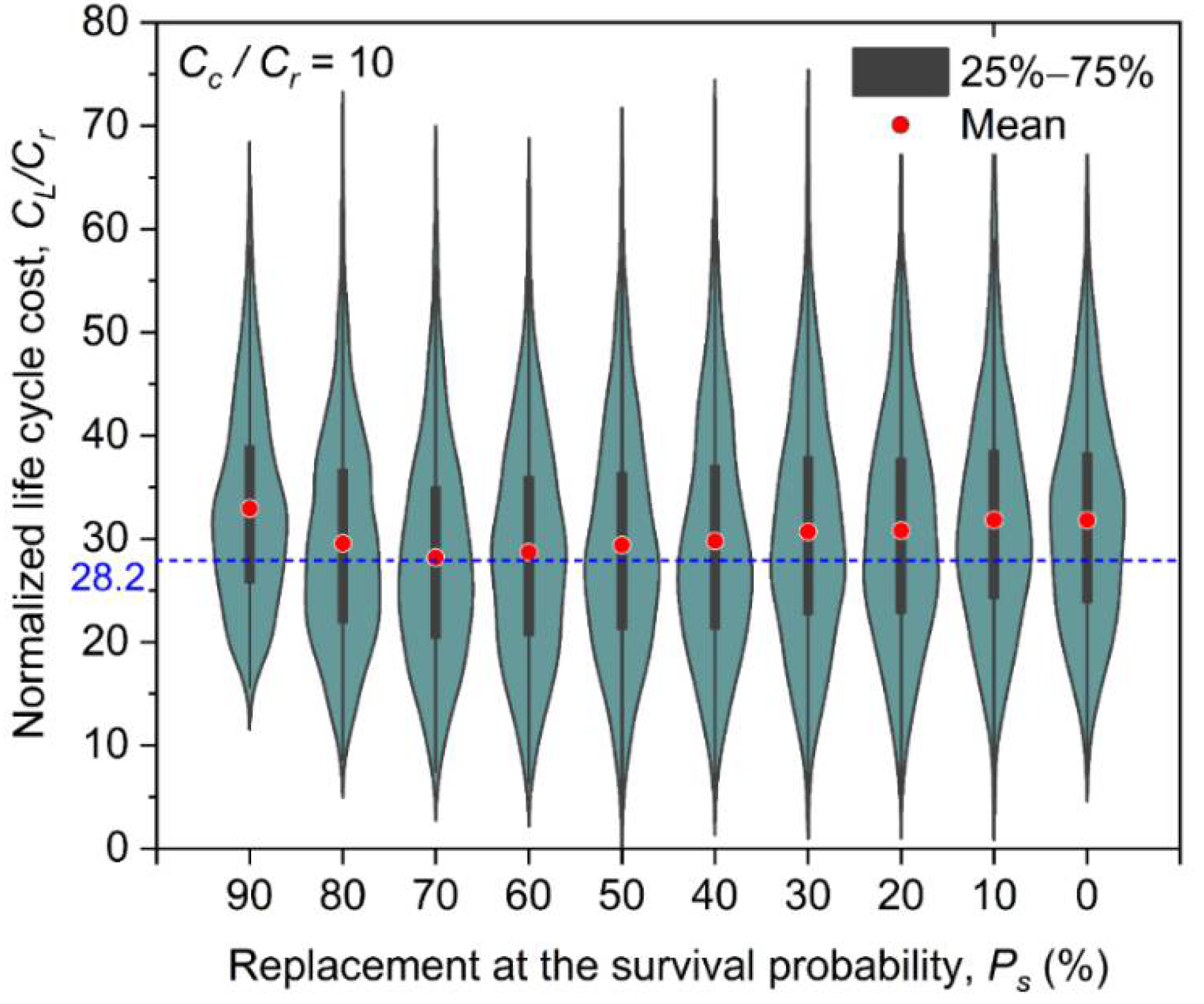
| Parameter | Replacement at the Survival Probability, (%) | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| 90 | 80 | 70 | 60 | 50 | 40 | 30 | 20 | 10 | 0 | |
| Mean | 32.9 | 29.6 | 28.2 | 28.7 | 29.4 | 29.8 | 30.7 | 30.8 | 31.8 | 31.8 |
| Median | 31.8 | 28.6 | 27.2 | 28.2 | 28.3 | 28.2 | 29.7 | 29.9 | 30.7 | 31.1 |
| COV | 0.29 | 0.35 | 0.38 | 0.37 | 0.38 | 0.38 | 0.37 | 0.36 | 0.35 | 0.33 |
| Parameter | Optimal | Optimal | Influence |
|---|---|---|---|
| Negative | Positive | Medium | |
| Negative | Positive | High | |
| Positive | Negative | Medium | |
| Positive | N/A | High | |
| Negative | N/A | High | |
| Negative | Positive | High | |
| Negative | Positive | Medium |
| Parameter | 1st | 2nd | 3rd | 4th | 5th |
|---|---|---|---|---|---|
| Mean (Year) | 103.0 | 148.5 | 185.3 | 217.0 | 241.4 |
| Median (Year) | 64.4 | 118.0 | 154.9 | 194.6 | 218.1 |
| COV | 1.055 | 0.796 | 0.678 | 0.605 | 0.546 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lin, P.; Chen, X.; Huang, S.; Ma, B. An Optimal Maintenance and Replacement Strategy for Deteriorating Water Mains. Water 2022, 14, 2097. https://doi.org/10.3390/w14132097
Lin P, Chen X, Huang S, Ma B. An Optimal Maintenance and Replacement Strategy for Deteriorating Water Mains. Water. 2022; 14(13):2097. https://doi.org/10.3390/w14132097
Chicago/Turabian StyleLin, Peiyuan, Xianying Chen, Sheng Huang, and Baosong Ma. 2022. "An Optimal Maintenance and Replacement Strategy for Deteriorating Water Mains" Water 14, no. 13: 2097. https://doi.org/10.3390/w14132097
APA StyleLin, P., Chen, X., Huang, S., & Ma, B. (2022). An Optimal Maintenance and Replacement Strategy for Deteriorating Water Mains. Water, 14(13), 2097. https://doi.org/10.3390/w14132097







