Reactive Transport Simulation of Cavern Formation along Fractures in Carbonate Rocks
Abstract
1. Introduction
2. Mathematical Models
2.1. Fluid Flow
2.2. Solute Transport
2.3. Chemical Reaction
3. Numerical Models
3.1. Scenarios
3.2. Model Setup
4. Results
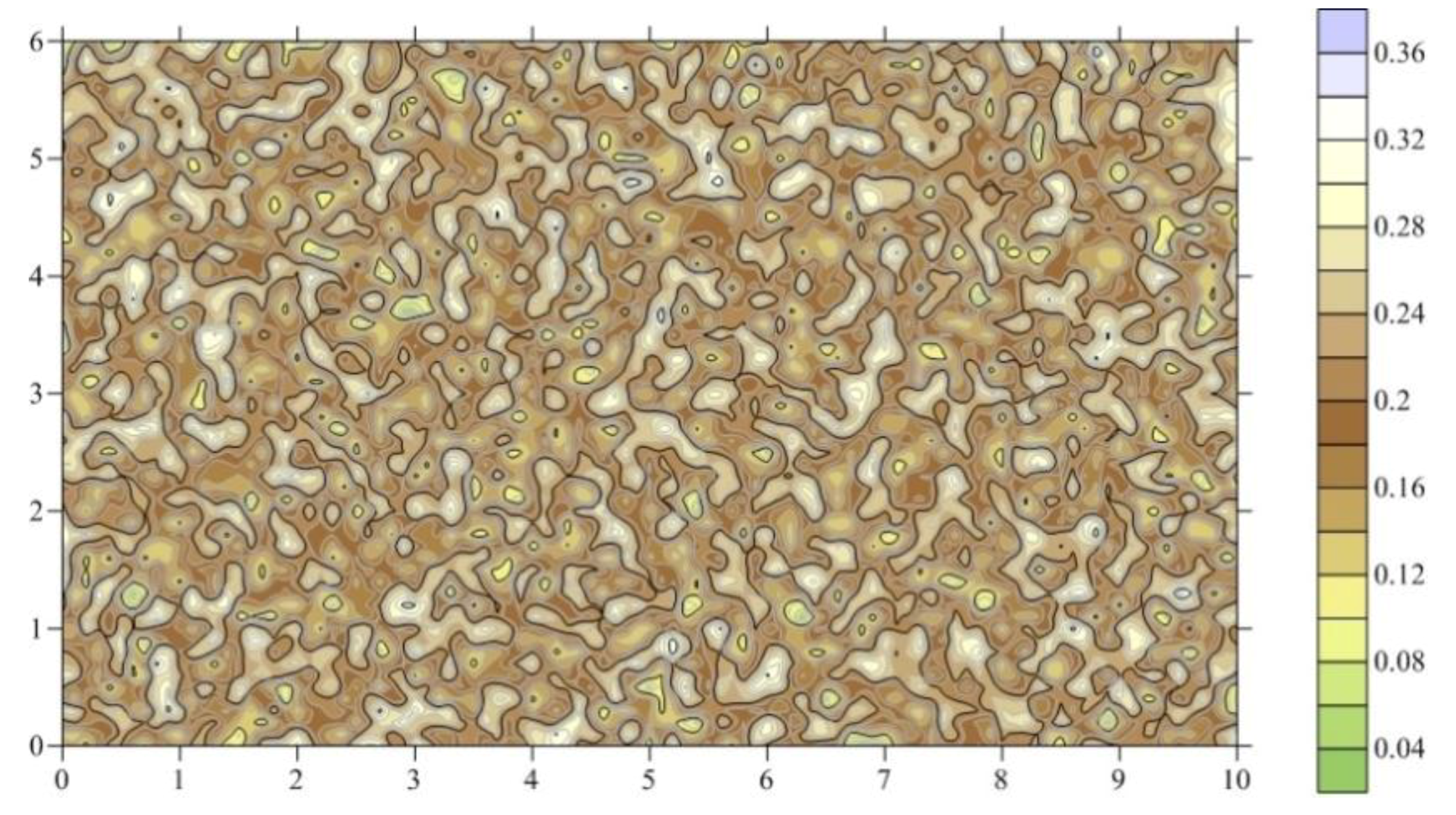
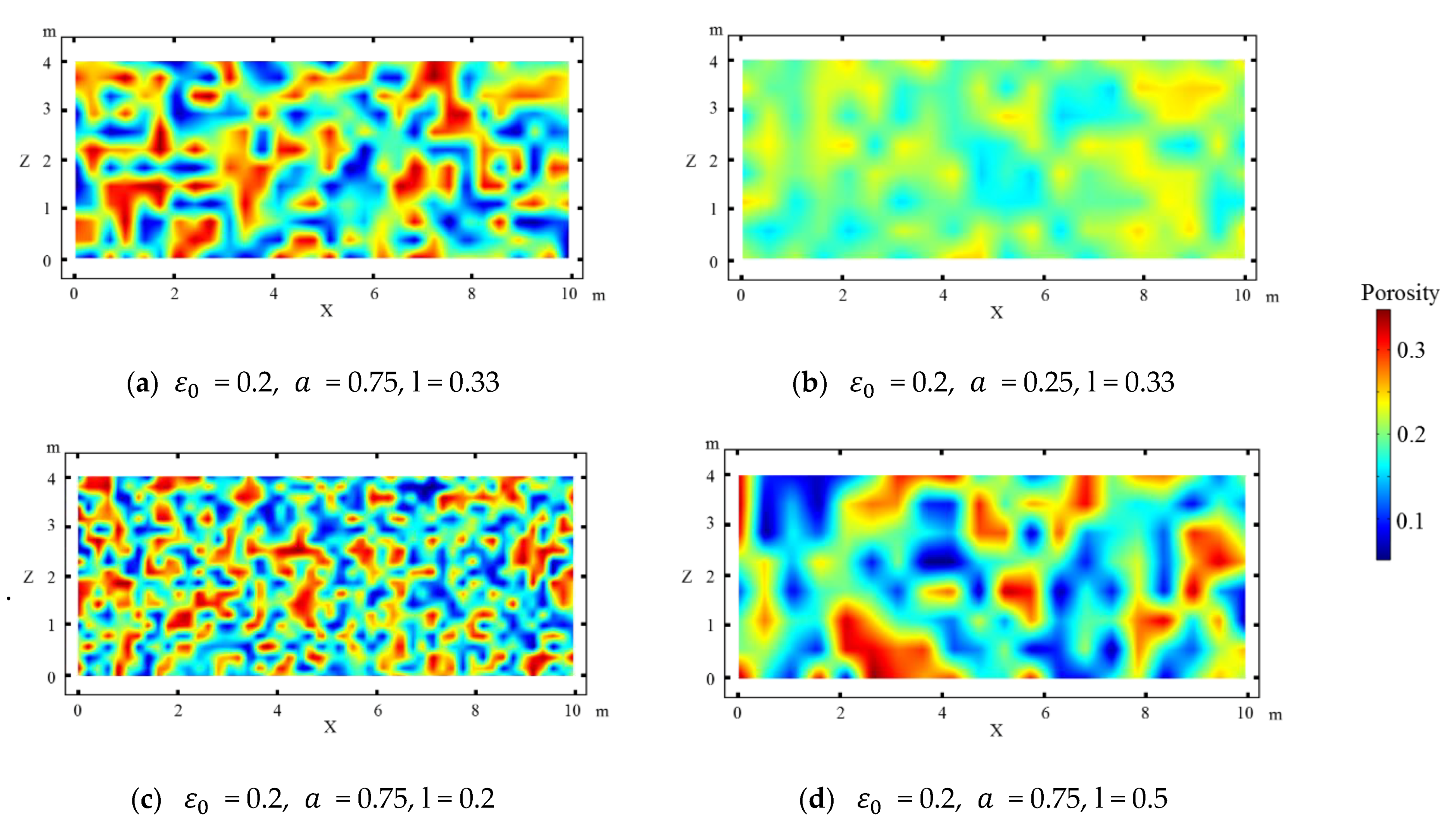
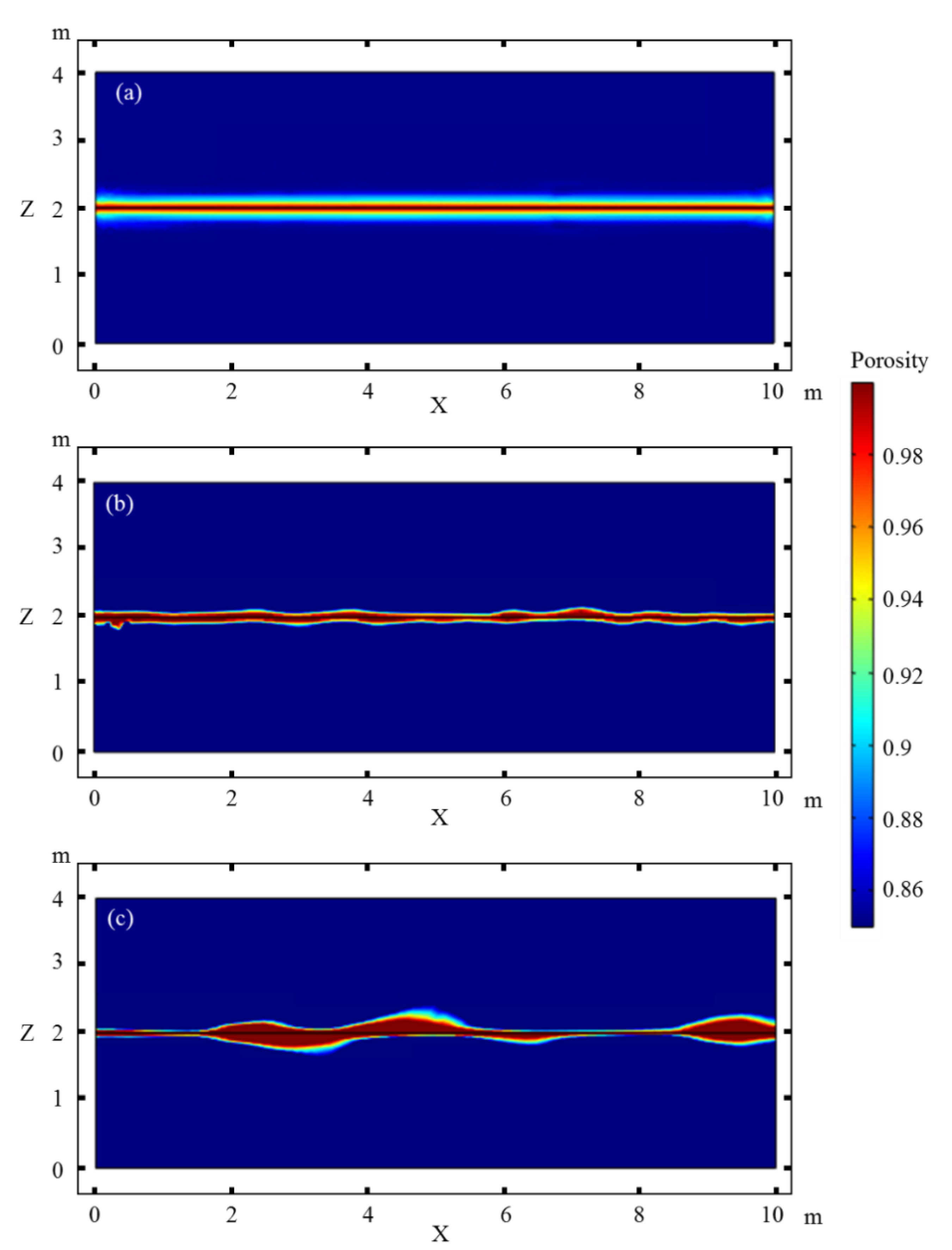
4.1. Porosity Evolution under Different Matrix Heterogeneity Magnitudes
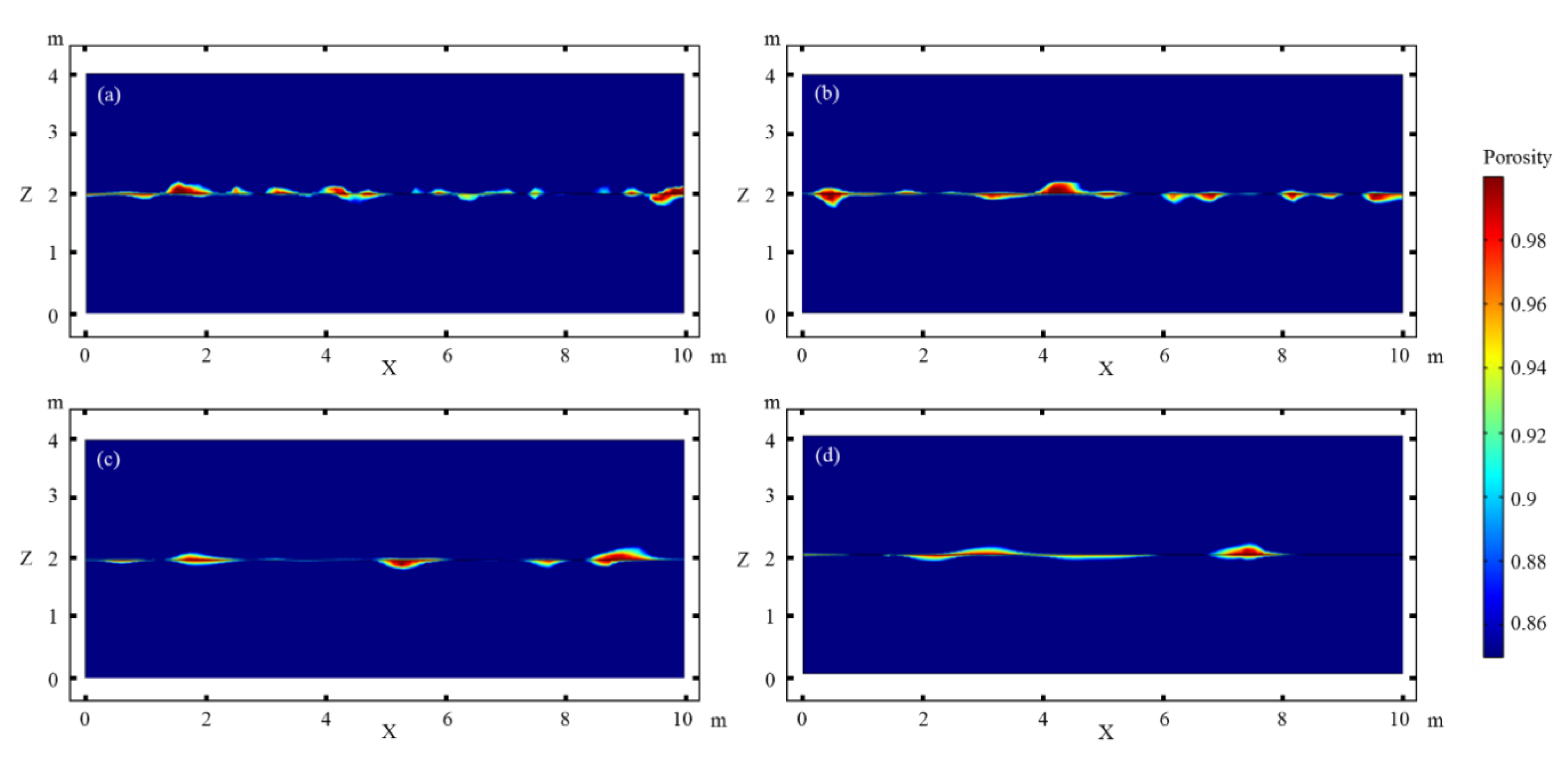
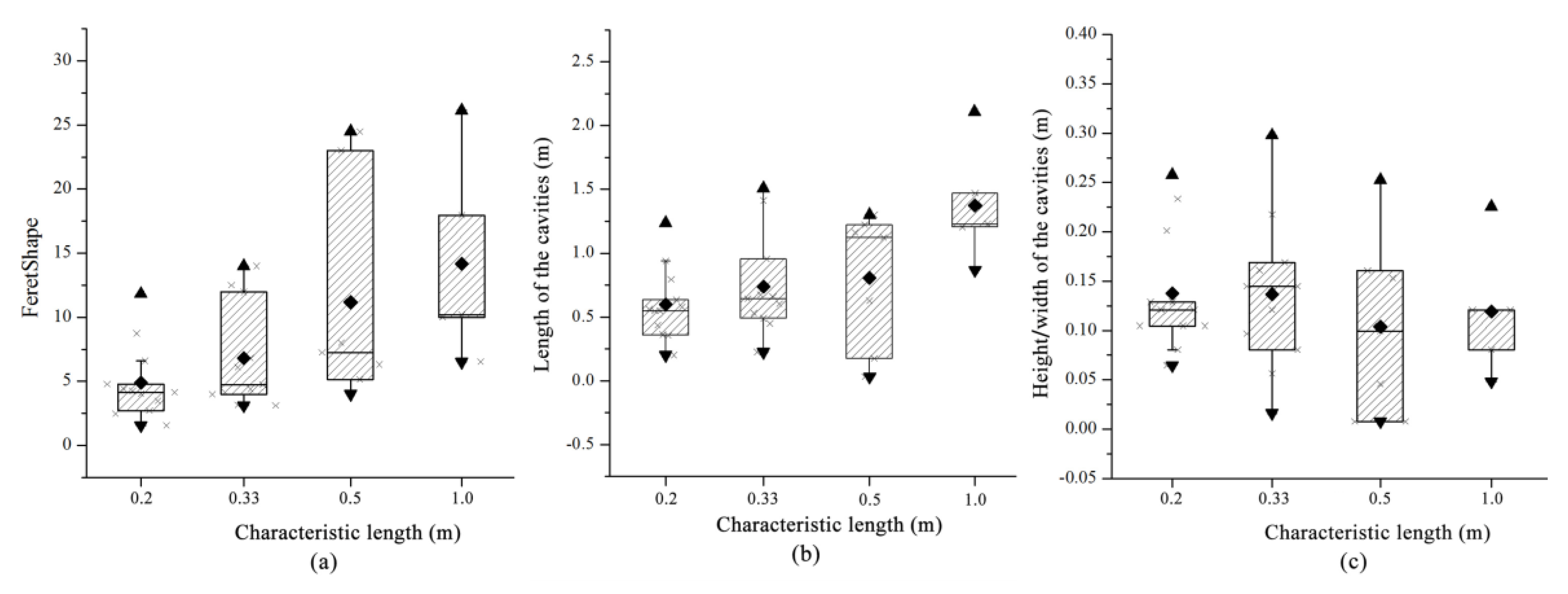
4.2. Porosity Evolution for Different Characteristic Lengths of the Matrix Porosity Heterogeneity
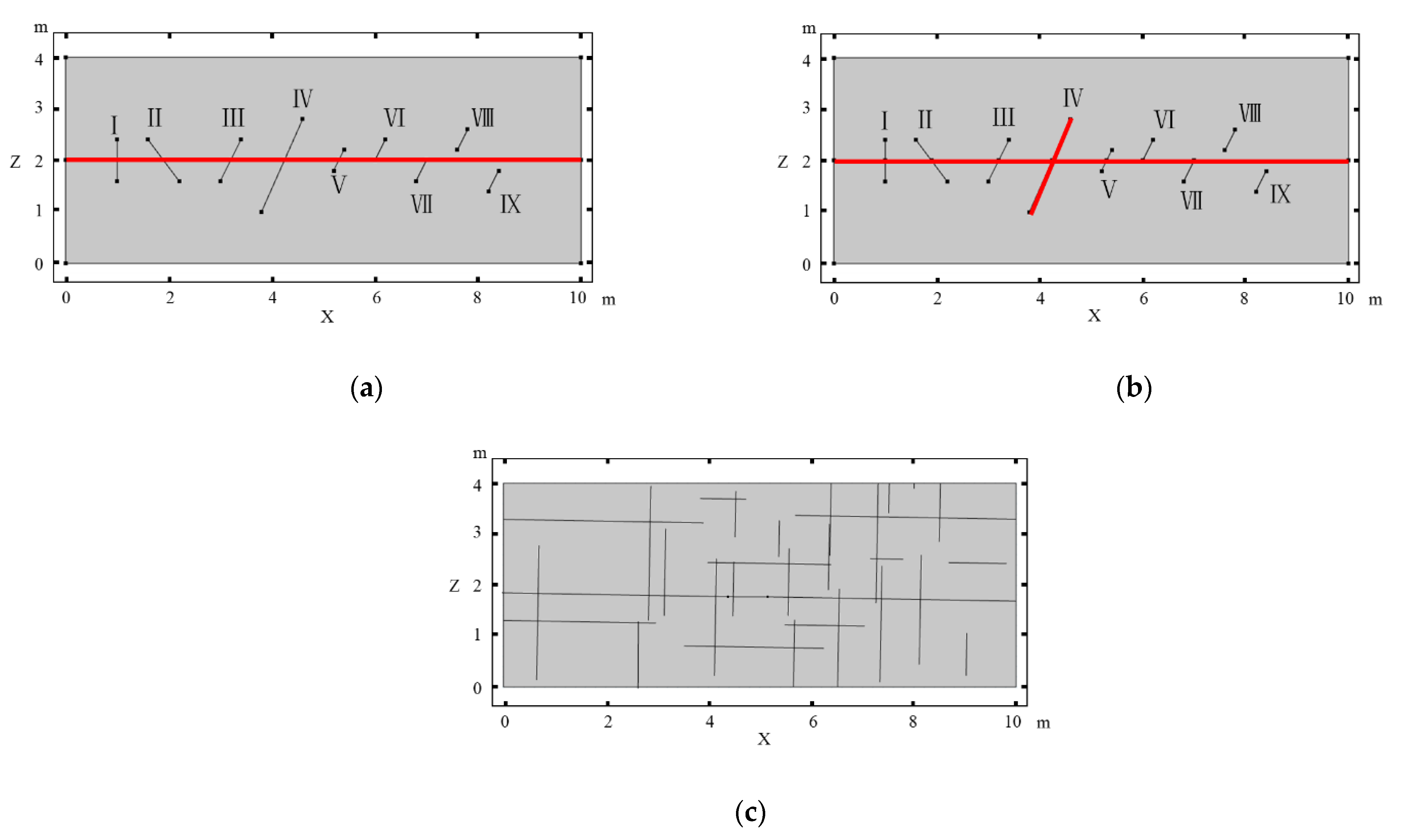
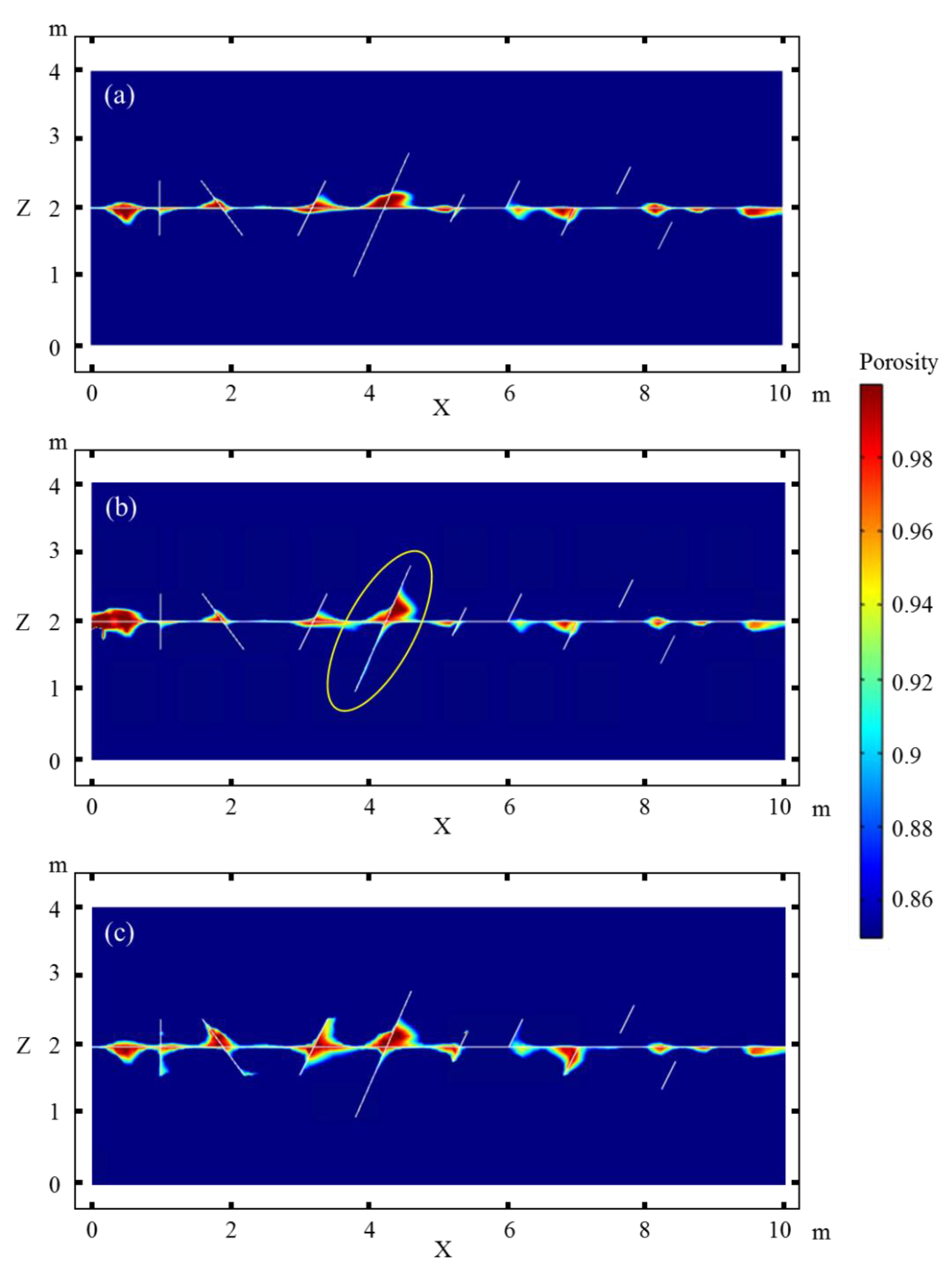
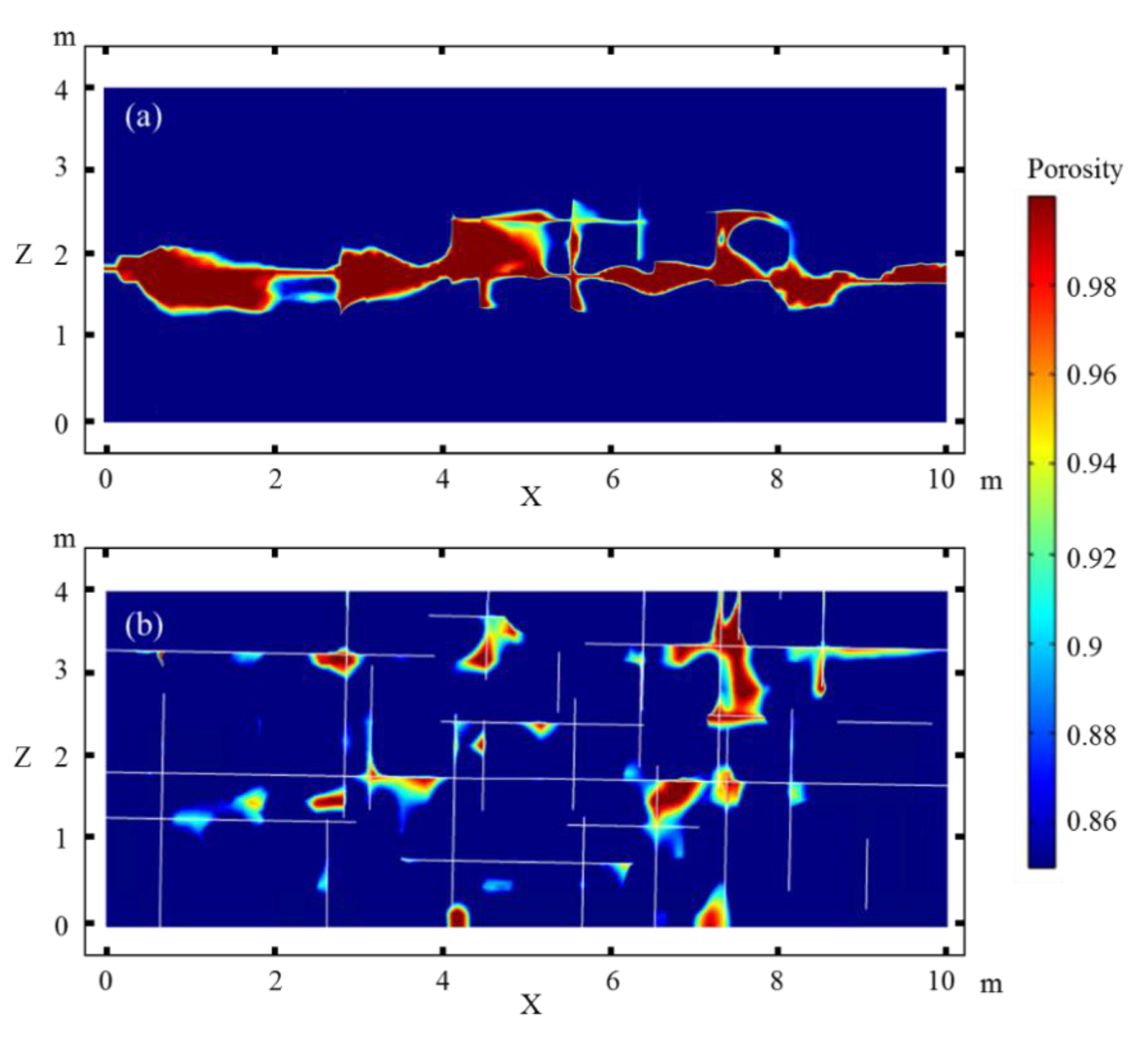
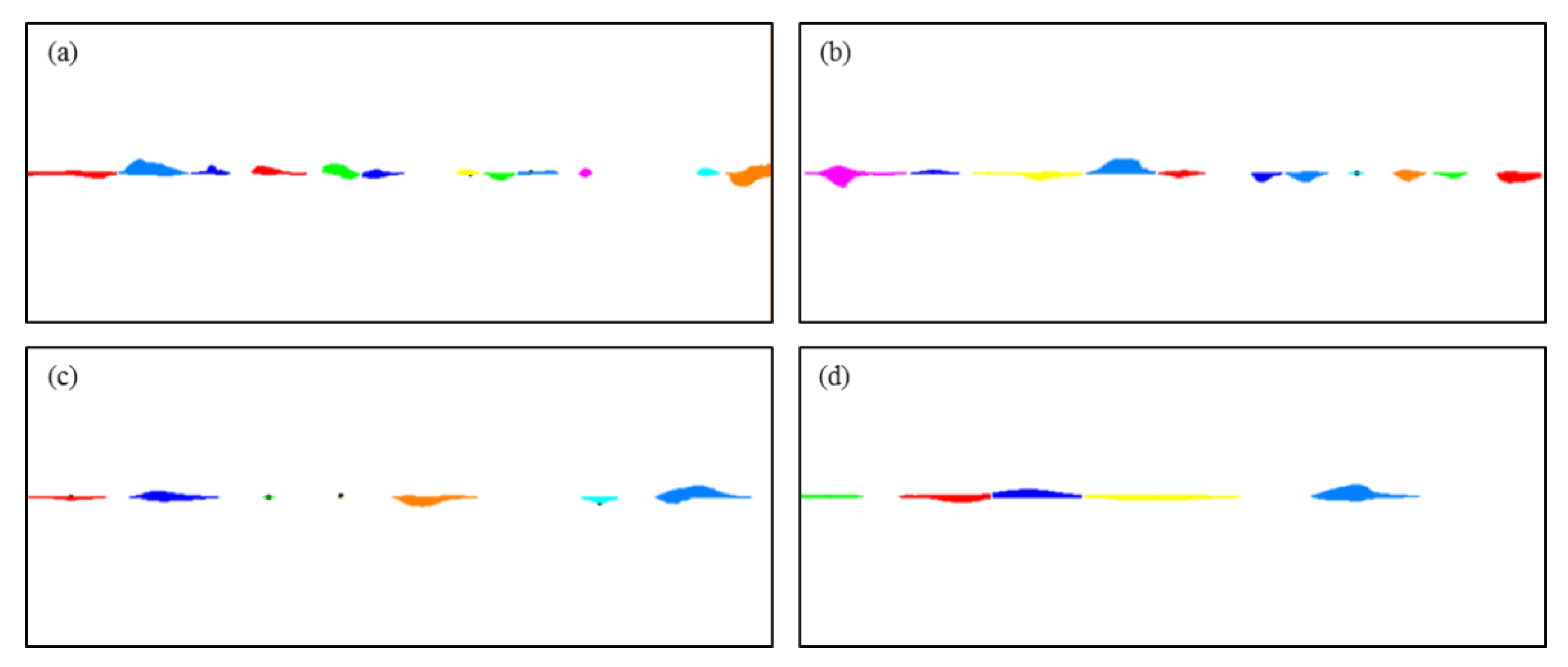
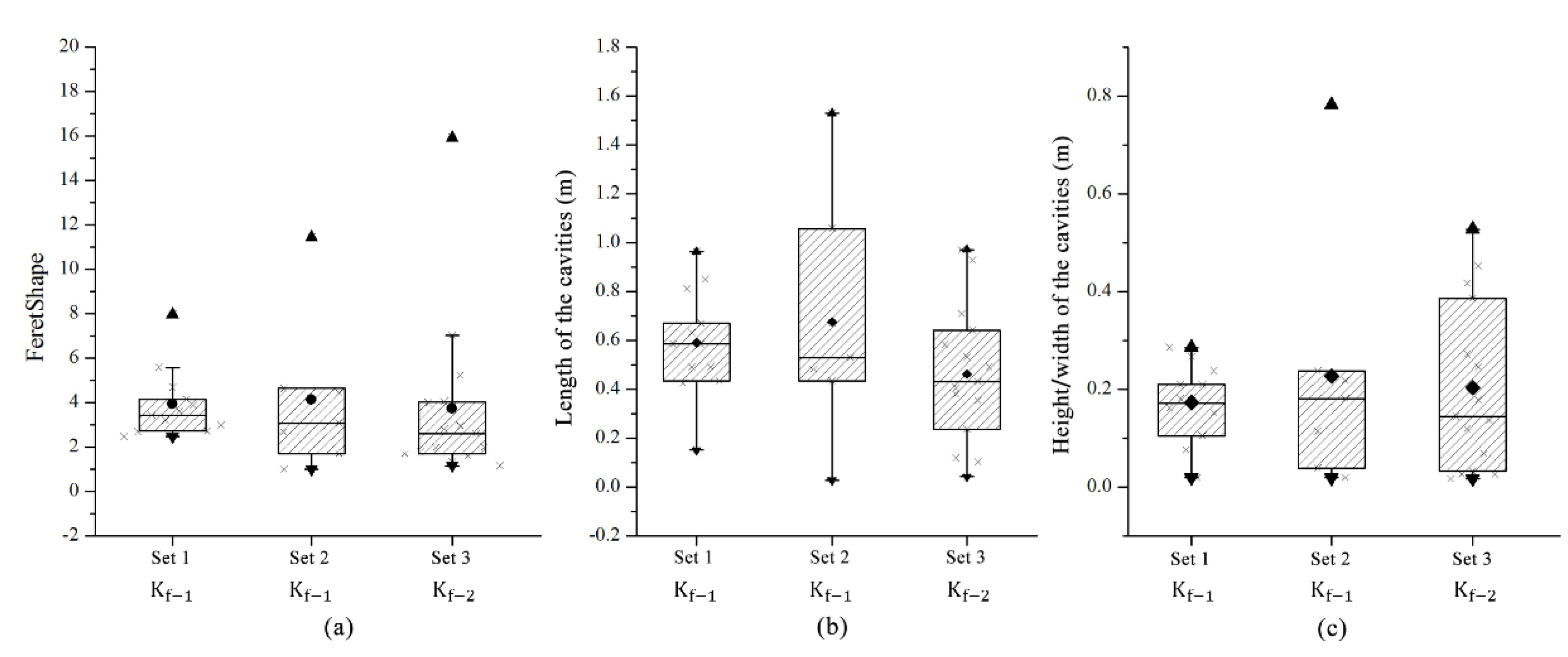
4.3. Porosity Evolution with Intersecting Fractures
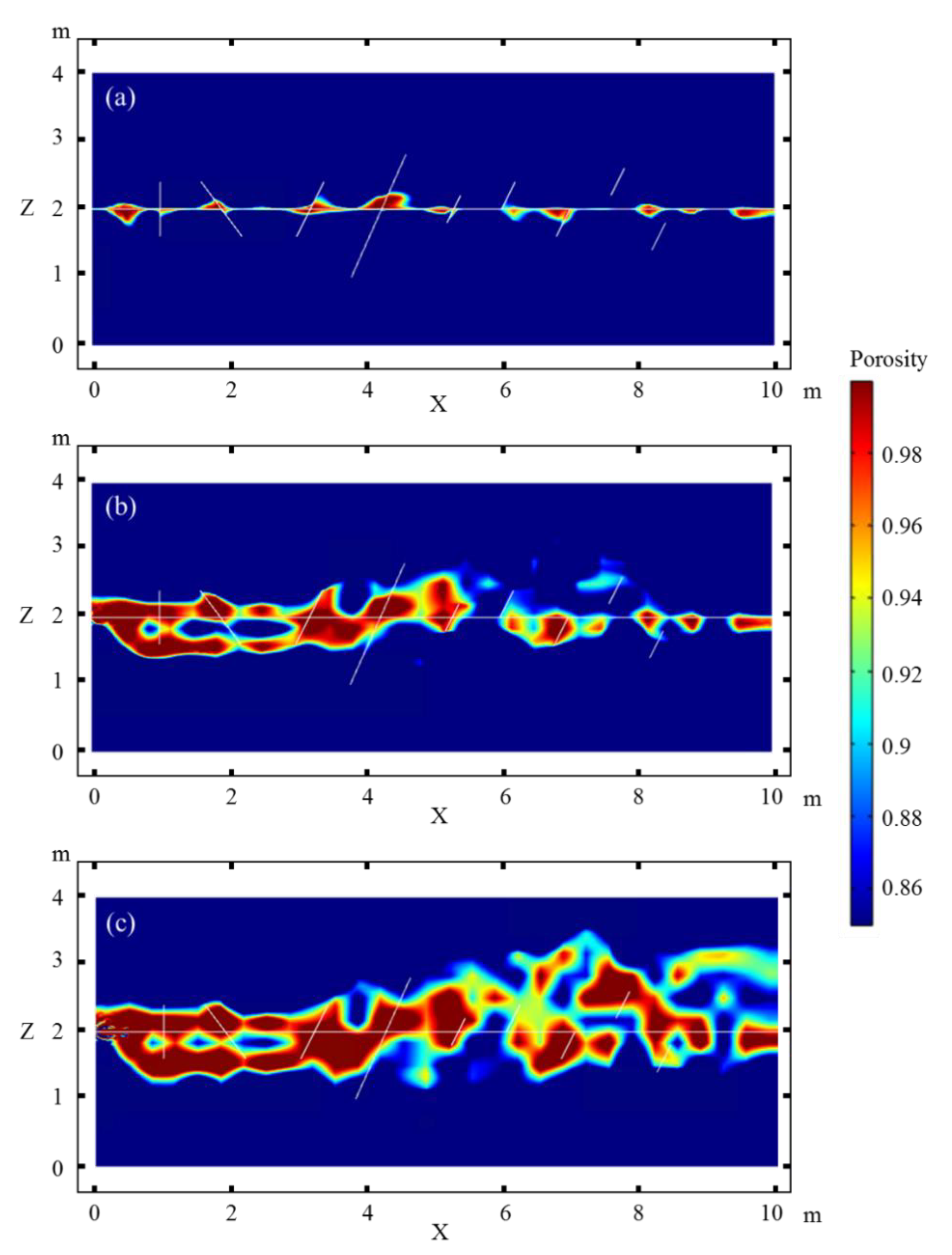
4.4. Porosity Evolution in Complex Fracture Networks
5. Discussion
5.1. Effect of the Magnitude of the Porosity Heterogeneity of the Matrix
5.2. Effect of the Characteristic Length of the Matrix Porosity Heterogeneity
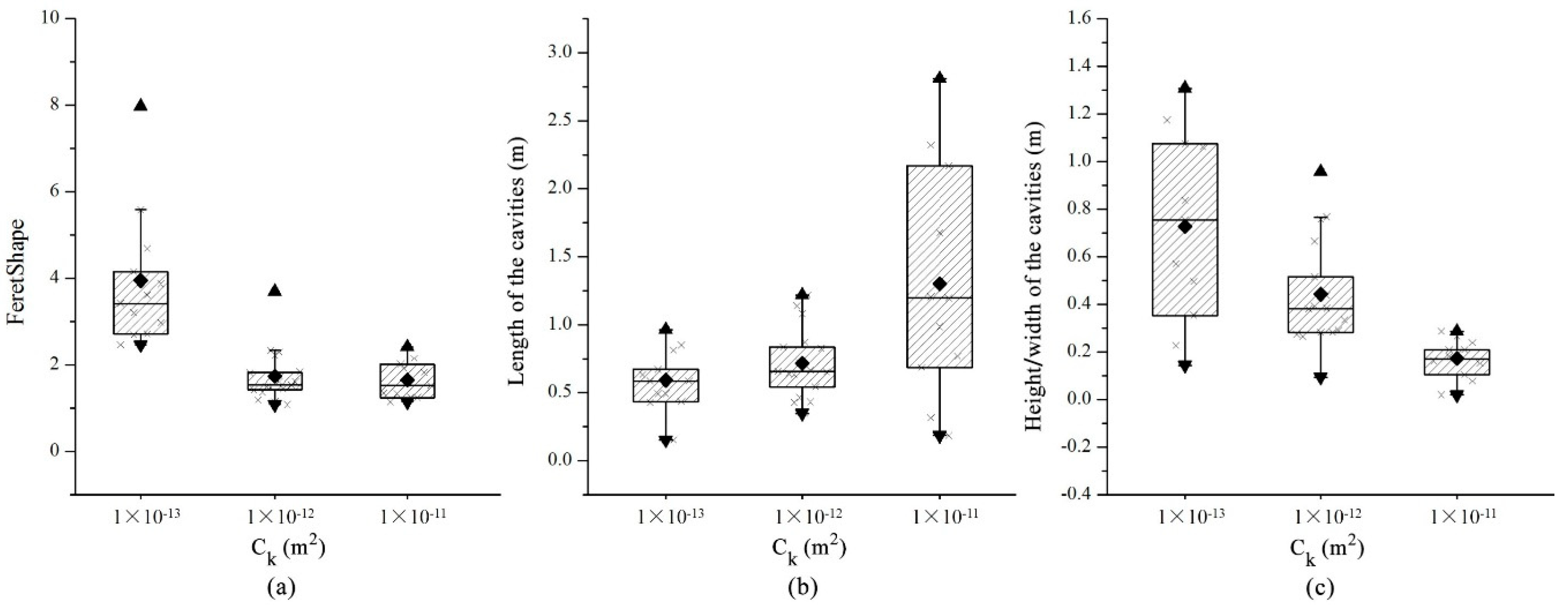
5.3. Effect of Cross Fractures
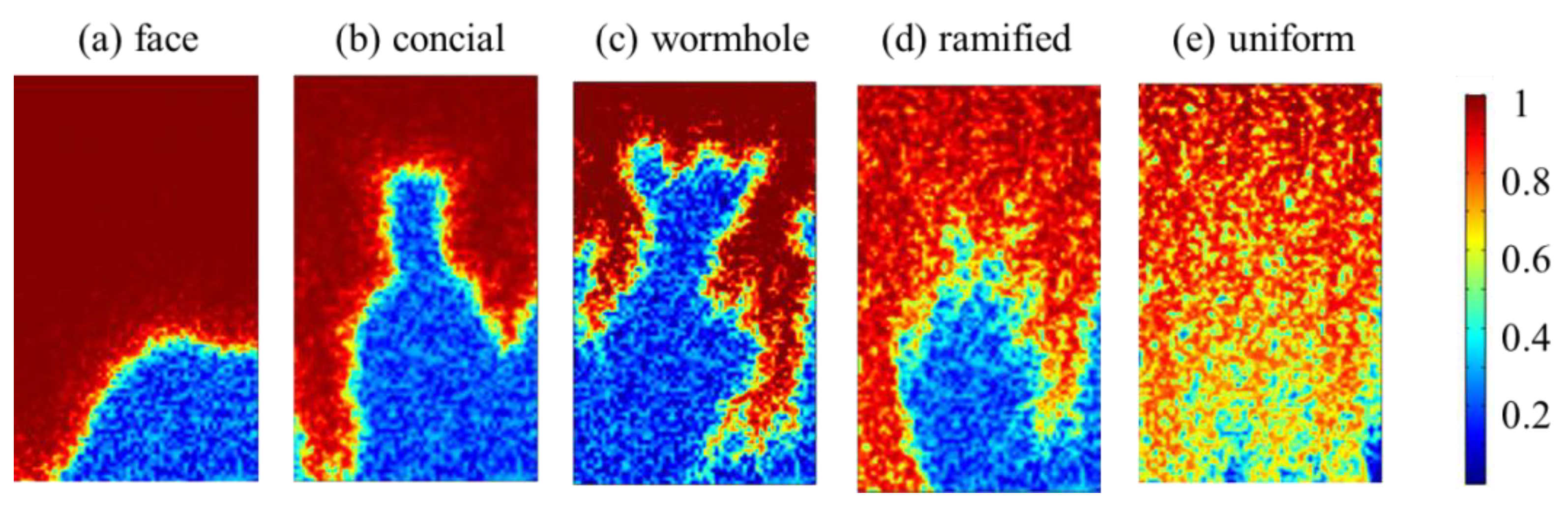
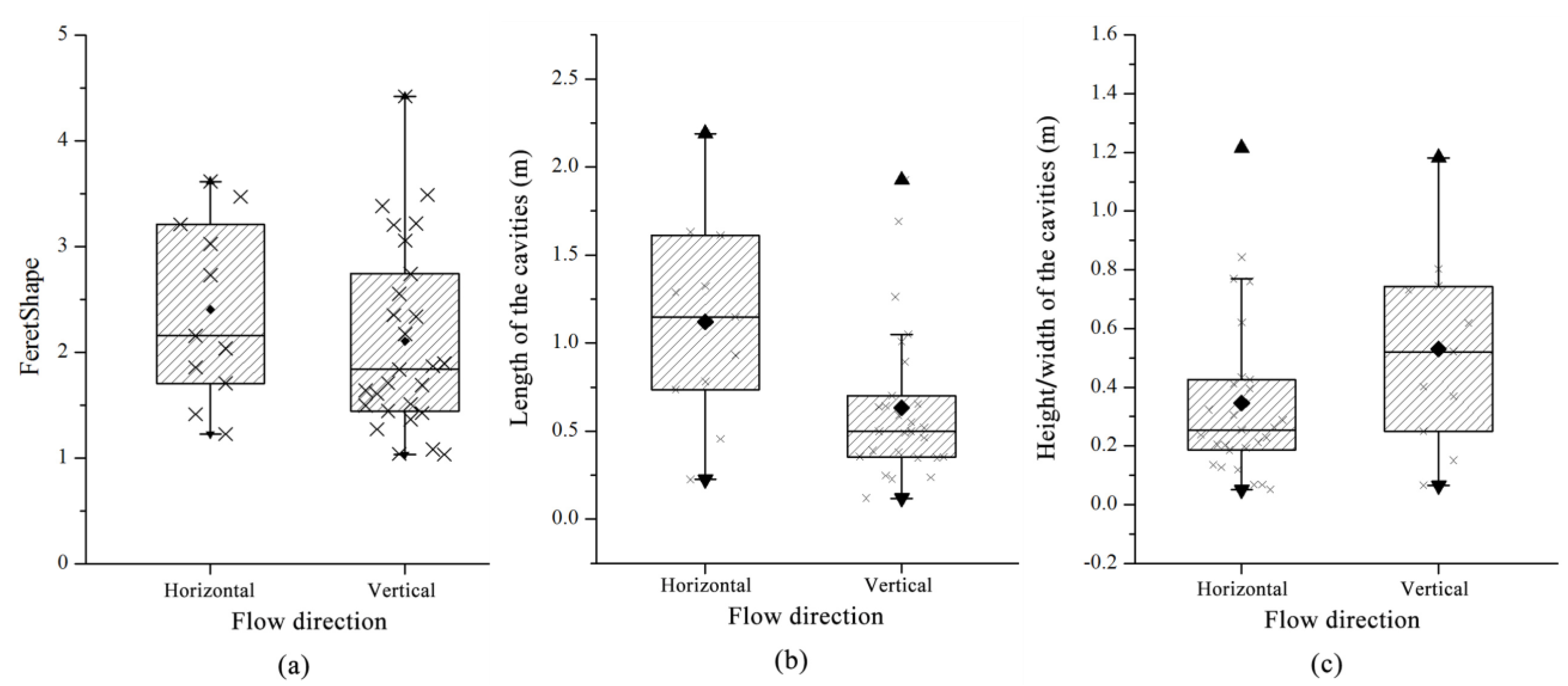
5.4. Cavity Formation in a Complex Fracture Network
6. Conclusions
- (1)
- The magnitude of the matrix heterogeneity has a notable impact on the dissolution distribution along the fracture. Stronger heterogeneity results in a more significant difference in the cavity shape.
- (2)
- The characteristic length affects the size, shape, and roundness of beam-shaped wormholes. When the characteristic length is larger, the caves are larger and flatter. Although the caves are smaller when the characteristic length is smaller, the number of the holes is larger.
- (3)
- The existence of cross fractures enhances the dissolution area. Increasing the permeability of the cross fractures or matrix greatly improves the size of the beam-shaped wormholes, leading to the formation of an underground river in the karst system. The sizes of the cavities increase and the roundness (FeretShape) decreases with increasing permeability of the cross fractures.
- (4)
- A more complex fracture network leads to the development of more karst dissolution pores and caves. The topology of the fracture network and preferential flow dominate the distribution of caves but alleviate the effect of the matrix heterogeneity. The seepage along multiple sets of short and straight fractures leads to the formation of cavities with smaller sizes and FeretShape values, whereas the seepage along the long and straight horizontal fracture results in the formation of large caves and underground river systems.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A
References
- Ford, D.; Williams, P. Karst Geomorphology and Hydrology; The Academic Division of Unwin Hyman Ltd.: London, UK, 1989; Volume 346. [Google Scholar]
- Al-Mumen, A.; Al-Habib, N.; Al-Anzi, E.; Al-Mutawa, M. Positive reactions in carbonate reservoir stimulation. Oilfield Rev. 2003, 15, 28–45. [Google Scholar]
- Burberry, C.M.; Jackson, C.A.-L.; Chandler, S.R. Seismic reflection imaging of karst in the Persian Gulf: Implications for the characterization of carbonate reservoirs. AAPG Bull. 2016, 100, 1561–1584. [Google Scholar] [CrossRef]
- Chen, Z.; Wang, T.-G.; Li, M.; Yang, F.; Cheng, B. Biomarker geochemistry of crude oils and Lower Paleozoic source rocks in the Tarim Basin, western China: An oil-source rock correlation study. Mar. Pet. Geol. 2018, 96, 94–112. [Google Scholar] [CrossRef]
- Russel-Houston, J.; Gray, K. Paleokarst in the Grosmont Formation and reservoir implications, Saleski, Alberta, Canada. Interpretation 2014, 2, SF29–SF50. [Google Scholar] [CrossRef]
- Jiang, L.; Cai, C.; Worden, R.H.; Crowley, S.F.; Jia, L.; Zhang, K.; Duncan, I.J. Multiphase dolomitization of deeply buried Cambrian petroleum reservoirs, Tarim Basin, north-west China. Sedimentology 2016, 63, 2130–2157. [Google Scholar] [CrossRef]
- Jiang, L.; Worden, R.H.; Cai, C.F.; Shen, A.J.; He, X.Y.; Pan, L.Y. Contrasting diagenetic evolution patterns of platform margin limestones and dolostones in the Lower Triassic Feixianguan Formation, Sichuan Basin, China. Mar. Pet. Geol. 2018, 92, 332–351. [Google Scholar] [CrossRef]
- Tian, F.; Di, Q.; Jin, Q.; Cheng, F.; Zhang, W.; Lin, L.; Wang, Y.; Yang, D.; Niu, C.; Li, Y. Multiscale geological-geophysical characterization of the epigenic origin and deeply buried paleokarst system in Tahe Oilfield, Tarim Basin. Mar. Pet. Geol. 2019, 102, 16–32. [Google Scholar] [CrossRef]
- Lu, X.; Wang, Y.; Tian, F.; Li, X.; Yang, D.; Li, T.; Lv, Y.; He, X. New insights into the carbonate karstic fault system and reservoir formation in the Southern Tahe area of the Tarim Basin. Mar. Pet. Geol. 2017, 86, 587–605. [Google Scholar] [CrossRef]
- Wang, D.; Li, Y.; Zhang, J.; Wei, C.; Jiao, Y.; Wang, Q. Improved CRM Model for Inter-Well Connectivity Estimation and Production Optimization: Case Study for Karst Reservoirs. Energies 2019, 12, 816. [Google Scholar] [CrossRef]
- Tian, F.; Wang, Z.; Cheng, F.; Xin, W.; Fayemi, O.; Zhang, W.; Shan, X. Three-Dimensional Geophysical Characterization of Deeply Buried Paleokarst System in the Tahe Oilfield, Tarim Basin, China. Water 2019, 11, 1045. [Google Scholar] [CrossRef]
- Li, Y.; Yu, Q.; Jia, C.; Liu, P.; Wang, Q.; Wang, D. Rate transient analysis for coupling Darcy flow and free flow in bead-string fracture-caved carbonate reservoirs. J. Pet. Sci. Eng. 2020, 195, 107809. [Google Scholar] [CrossRef]
- Zhu, H.; Zhu, X.; Chen, H. Seismic Characterization of Hypogenic Karst Systems Associated with Deep Hydrothermal Fluids in the Middle-Lower Ordovician Yingshan Formation of the Shunnan Area, Tarim Basin, NW China. Geofluids 2017, 2017, 1–13. [Google Scholar] [CrossRef]
- Mylroie, J.E.; Mylroie, J.R. Telogenetic limestones and island karst. In Coastal Karst Landforms; Lace, M.J., Mylroie, J.E., Eds.; Springer: Dordrecht, The Netherlands, 2013; pp. 375–393. [Google Scholar]
- Garcia-Rios, M.; Luquot, L.; Soler, J.M.; Cama, J. Influence of the flow rate on dissolution and precipitation features during percolation of CO2-rich sulfate solutions through fractured limestone samples. Chem. Geol. 2015, 414, 95–108. [Google Scholar] [CrossRef]
- Deng, H.; Voltolini, M.; Molins, S.; Steefel, C.; DePaolo, D.; Ajo-Franklin, J.; Yang, L. Alteration and Erosion of Rock Matrix Bordering a Carbonate-Rich Shale Fracture. Environ. Sci. Technol. 2017, 51, 8861–8868. [Google Scholar] [CrossRef] [PubMed]
- Gray, F.; Anabaraonye, B.; Shah, S.; Boek, E.; Crawshaw, J. Chemical mechanisms of dissolution of calcite by HCl in porous media: Simulations and experiment. Adv. Water Resour. 2018, 121, 369–387. [Google Scholar] [CrossRef]
- Liu, P.; Yao, J.; Couples, G.D.; Ma, J.; Iliev, O. 3-D Modelling and Experimental Comparison of Reactive Flow in Carbonates under Radial Flow Conditions. Sci. Rep. 2017, 7, 1–10. [Google Scholar] [CrossRef]
- Szymczak, P.; Ladd, A.J.C. Wormhole formation in dissolving fractures. J. Geophys. Res. Solid Earth 2009, 114. [Google Scholar] [CrossRef]
- Deng, H.; Steefel, C.; Molins, S.; DePaolo, D. Fracture Evolution in Multimineral Systems: The Role of Mineral Composition, Flow Rate, and Fracture Aperture Heterogeneity. ACS Earth Space Chem. 2018, 2, 112–124. [Google Scholar] [CrossRef]
- Roded, R.; Aharonov, E.; Holtzman, R.; Szymczak, P. Reactive flow and homogenization in anisotropic media. Water Resour. Res. 2020, 56, e2020WR027518. [Google Scholar] [CrossRef]
- Tansey, J.; Balhoff, M.T. Pore Network Modeling of Reactive Transport and Dissolution in Porous Media. Transp. Porous Media 2016, 113, 303–327. [Google Scholar] [CrossRef]
- Xiao, T.; Kweon, H.; McPherson, B.; Deo, M. Wormhole Generations in Indiana Limestone with CO2 Intrusion: Numerical Simulations Based on Core Flooding Experiments. Energy Fuels 2017, 31, 12487–12499. [Google Scholar] [CrossRef]
- Dávila, G.; Luquot, L.; Soler, J.M.; Cama, J. Interaction between a fractured marl caprock and CO2-rich sulfate solution under supercritical CO2 conditions. Int. J. Greenh. Gas Control 2016, 48, 105–119. [Google Scholar] [CrossRef]
- Fredd, C.N.; Fogler, H.S. Influence of transport and reaction on wormhole formation in porous media. AIChE J. 1998, 44, 1933–1949. [Google Scholar] [CrossRef]
- Soulaine, C.; Roman, S.; Kovscek, A.; Tchelepi, H.A. Mineral dissolution and wormholing from a pore-scale perspective. J. Fluid Mech. 2017, 827, 457–483. [Google Scholar] [CrossRef]
- Gong, X.; Hou, W.; Feng, D.; Luo, Q.; Yang, X. Modelling early karstification in future limestone geothermal reservoirs by mixing of meteoric water with cross-formational warm water. Geothermics 2019, 77, 313–326. [Google Scholar] [CrossRef]
- Gong, X.; Tóth, J.; Yang, X.; Yuan, B.; Feng, D. A numerical model in predicting the initial karst development in porous limestone. Environ. Earth Sci. 2018, 77, 295. [Google Scholar] [CrossRef]
- Romanov, D.; Dreybrodt, W. Evolution of porosity in the saltwater–freshwater mixing zone of coastal carbonate aquifers: An alternative modelling approach. J. Hydrol. 2006, 329, 661–673. [Google Scholar] [CrossRef]
- Deng, H.; Molins, S.; Steefel, C.; DePaolo, D.; Voltolini, M.; Yang, L.; Ajo-Franklin, J. A 2.5D Reactive Transport Model for Fracture Alteration Simulation. Environ. Sci. Technol. 2016, 50, 7564–7571. [Google Scholar] [CrossRef]
- Hill, A.D.; Zhu, D.; Dong, C.; Luna-Garcia, A.L. Computer Model Predicts Matrix Acidizing of Naturally Fractured Carbonate. J. Pet. Technol. 2001, 53, 20–25. [Google Scholar] [CrossRef]
- Dong, C.; Zhu, D.; Hill, A.D. Acid Penetration in Natural Fracture Networks. SPE Prod. Facil. 2002, 17, 160–170. [Google Scholar] [CrossRef]
- Dreybrodt, W.; Gabrovšek, F. Dynamics of wormhole formation in fractured limestones. Hydrol. Earth Syst. Sci. 2019, 23, 1995–2014. [Google Scholar] [CrossRef]
- Dreybrodt, W.; Gabrovšek, F. Basic processes and mechanisms governing the evolution of karst. Speleogenesis Evol. Karst Aquifers 2003, 1, 115–154. [Google Scholar]
- Gabrovšek, F.; Menne, B.; Dreybrodt, W. A model of early evolution of karst conduits affected by subterranean CO2 sources. Environ. Geol. 2000, 39, 531–543. [Google Scholar] [CrossRef]
- Gabrovšek, F.; Dreybrodt, W. Karstification in unconfined limestone aquifers by mixing of phreatic water with surface water from a local input: A model. J. Hydrol. 2010, 386, 130–141. [Google Scholar] [CrossRef]
- Liu, R.; Li, B.; Jiang, Y.; Huang, N. Review: Mathematical expressions for estimating equivalent permeability of rock fracture networks. Hydrogeol. J. 2016, 24, 1623–1649. [Google Scholar] [CrossRef]
- Liu, R.; Li, B.; Jiang, Y. Critical hydraulic gradient for nonlinear flow through rock fracture networks: The roles of aperture, surface roughness, and number of intersections. Adv. Water Resour. 2016, 88, 53–65. [Google Scholar] [CrossRef]
- Kaufmann, G.; Romanov, D.; Hiller, T. Modeling three-dimensional karst aquifer evolution using different matrix-flow contributions. J. Hydrol. 2010, 388, 241–250. [Google Scholar] [CrossRef]
- Kaufmann, G. Modelling karst geomorphology on different time scales. Geomorphology 2009, 106, 62–77. [Google Scholar] [CrossRef]
- Kaufmann, G. Numerical models for mixing corrosion in natural and artificial karst environments. Water Resour. Res. 2003, 39. [Google Scholar] [CrossRef]
- Kaufmann, G. A model comparison of karst aquifer evolution for different matrix-flow formulations. J. Hydrol. 2003, 283, 281–289. [Google Scholar] [CrossRef]
- Kaufmann, G.; Braun, J. Karst Aquifer evolution in fractured, porous rocks. Water Resour. Res. 2000, 36, 1381–1391. [Google Scholar] [CrossRef]
- Liu, P.; Yao, J.; Couples, G.D.; Huang, Z.; Sun, H.; Ma, J. Numerical modelling and analysis of reactive flow and wormhole formation in fractured carbonate rocks. Chem. Eng. Sci. 2017, 172, 143–157. [Google Scholar] [CrossRef]
- Groves, C.G.; Howard, A.D. Early development of karst systems: 1. Preferential flow path enlargement under laminar flow. Water Resour. Res. 1994, 30, 2837–2846. [Google Scholar] [CrossRef]
- Dreybrodt, W.; Bühmann, D. A mass transfer model for dissolution and precipitation of calcite from solutions in turbulent motion. Chem. Geol. 1991, 90, 107–122. [Google Scholar] [CrossRef]
- Plummer, L.N.; Busenberg, E. The solubilities of calcite, aragonite and vaterite in CO2-H2O solutions between 0 and 90 °C, and an evaluation of the aqueous model for the systemCaCO3-CO2-H2O. Geochim. Cosmochim. Acta 1982, 46, 1011–1040. [Google Scholar] [CrossRef]
- Tao, J.; Wu, Y.; Elsworth, D.; Li, P.; Hao, Y. Coupled Thermo-Hydro-Mechanical-Chemical Modeling of Permeability Evolution in a CO2-Circulated Geothermal Reservoir. Geofluids 2019, 2019, 1–15. [Google Scholar] [CrossRef]
- Panga, M.K.R.; Ziauddin, M.; Balakotaiah, V. Two-scale continuum model for simulation of wormholes in carbonate acidization. AIChE J. 2005, 51, 3231–3248. [Google Scholar] [CrossRef]
- Aliouache, M.; Wang, X.; Jourde, H.; Huang, Z.; Yao, J. Incipient karst formation in carbonate rocks: Influence of fracture network topology. J. Hydrol. 2019, 575, 824–837. [Google Scholar] [CrossRef]
- Palmer, A.N. Origin and morphology of limestone caves. Geol. Soc. Am. Bull. 1991, 103, 1–21. [Google Scholar] [CrossRef]
- Ford, D.C.; Ewers, R.O. The development of limestone cave systems in the dimensions of length and depth. Can. J. Earth Sci. 1978, 15, 1783–1798. [Google Scholar] [CrossRef]




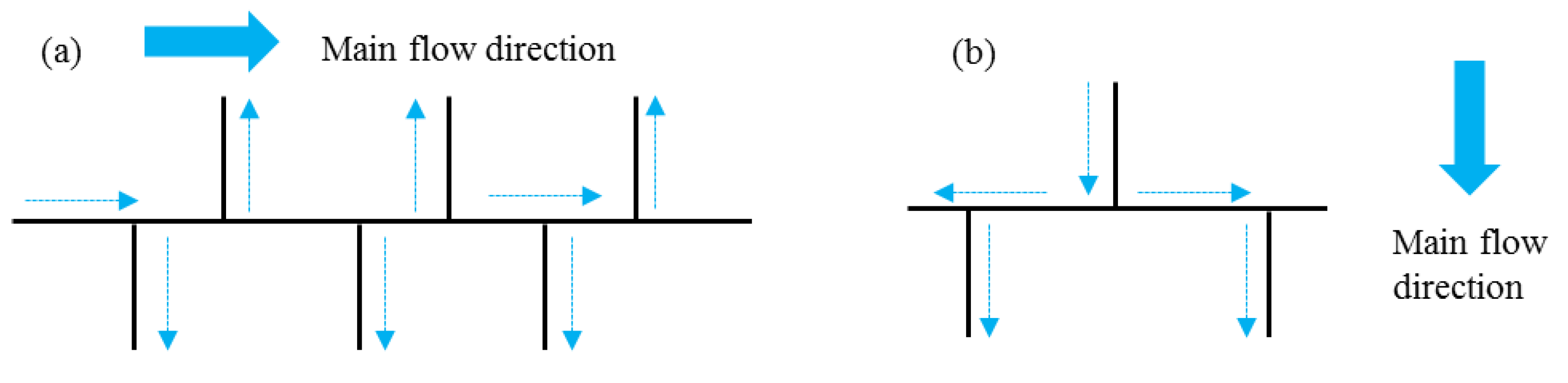
| Flow Direction | ||||||
|---|---|---|---|---|---|---|
| Case 1 | Homo = 0.2 = 0 | 1 × 10−13 | NON | 1.1 × 10−5 | NON | horizontal |
| Case 2 | = 0.2 = 0.25 | 1 × 10−13 | 0.33 | 1.1 × 10−5 | NON | horizontal |
| Case 3 | = 0.2 = 0.75 | 1 × 10−13 | 0.33 | 1.1 × 10−5 | NON | horizontal |
| Case 4 | = 0.2 = 0.75 | 1 × 10−13 | 0.2 | 1.1 × 10−5 | NON | horizontal |
| Case 5 | = 0.2 = 0.75 | 1 × 10−13 | 0.5 | 1.1 × 10−5 | NON | horizontal |
| Case 6 | = 0.2 = 0.75 | 1 × 10−13 | 0.33 | 1.1 × 10−5 | 1.1 × 10−6 | horizontal |
| Case 7 | = 0.2 = 0.75 | 1 × 10−12 | 0.33 | 1.1 × 10−5 | 1.1 × 10−6 | horizontal |
| Case 8 | = 0.2 = 0.75 | 1 × 10−11 | 0.33 | 1.1 × 10−5 | 1.1 × 10−6 | horizontal |
| Case 9 | = 0.2 = 0.75 | 1 × 10−13 | 0.33 | 1.1 × 10−5 | 2.45 × 10−6 | horizontal |
| Case 10 | = 0.2 = 0.75 | 1 × 10−13 | 0.33 | 1.1 × 10−5 | 1.1 × 10−6 | horizontal |
| Case 11 | = 0.2 = 0.75 | 1 × 10−13 | 0.33 | 1.1 × 10−5 | 1.1 × 10−6 | vertical |
| Scenario | Case 1 | Case 2 | Case 3 |
|---|---|---|---|
| Accumulated pore volume (PV) | 4.96 | 12.36 | 2.90 |
| Volume fraction of the cavities (%) | 2.54 | 3.43 | 4.63 |
| Height/width ratio of the cavities (m) | 0.20 | 0.19–0.28 | 0.01–0.41 |
| Characteristic Length (m) | 0.2 | 0.33 | 0.5 | 1 |
| Accumulated pore volume (PV) | 0.35 | 0.24 | 0.18 | 0.46 |
| Volume fraction of the cavities (%) | 1.38 | 1.51 | 0.97 | 1.17 |
| Height/width of the cavities (m) | 0.064–0.23 | 0.016–0.29 | 0.0076–0.25 | 0.08–0.23 |
| Length of the cavities (m) | 0.20–1.24 | 0.22–1.51 | 0.03–1.30 | 0.87–2.10 |
| FeretShape (D/d) | 1.56–11.82 | 3.10–13.98 | 4.00–24.48 | 6.52–26.13 |
| Cross Fracture Set | Set 1 | Set 2 | Set 3 |
|---|---|---|---|
| Accumulated pore volume (PV) | 0.23 | 0.32 | 0.25 |
| Volume fraction of the cavities (%) | 1.69 | 2.12 | 2.03 |
| Height/width of the cavities (m) | 0.019–0.29 | 0.019–0.78 | 0.017–0.53 |
| Length of the cavities (m) | 0.15–0.85 | 0.02–1.53 | 0.04–0.97 |
| FeretShape (D/d) | 2.46–7.97 | 1–11.45 | 1.13–15.92 |
| 1 × 10−11 | 1 × 10−12 | 1 × 10−13 | |
| Accumulated pore volume (PV) | 403.86 | 11.528 | 0.23 |
| Volume fraction of the cavities (%) | 20.17 | 10.05 | 1.69 |
| Height/width of the cavities (m) | 0.14–1.31 | 0.094–0.77 | 0.019–0.29 |
| Length of the cavities (m) | 0.31–2.81 | 0.35–1.22 | 0.15–0.85 |
| FeretShape (D/d) | 1.14–2.41 | 1.08–3.69 | 2.46–7.97 |
| Seepage Direction | Horizontal | Vertical |
|---|---|---|
| Accumulated pore volume (PV) | 7.11 | 7.10 |
| Volume fraction of the cavities (%) | 9.86 | 8.50 |
| Height/width of the cavities (m) | 0.065–1.18 | 0.051–1.21 |
| Length of the cavities (m) | 0.23–2.19 | 0.12–1.93 |
| FeretShape (D/d) | 1.23–3.62 | 1.03–4.42 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Duan, R.; Shang, G.; Yu, C.; Wang, Q.; Zhang, H.; Wang, L.; Xu, Z.; Dong, Y. Reactive Transport Simulation of Cavern Formation along Fractures in Carbonate Rocks. Water 2021, 13, 38. https://doi.org/10.3390/w13010038
Duan R, Shang G, Yu C, Wang Q, Zhang H, Wang L, Xu Z, Dong Y. Reactive Transport Simulation of Cavern Formation along Fractures in Carbonate Rocks. Water. 2021; 13(1):38. https://doi.org/10.3390/w13010038
Chicago/Turabian StyleDuan, Ruiqi, Genhua Shang, Chen Yu, Qiang Wang, Hong Zhang, Liheng Wang, Zhifang Xu, and Yanhui Dong. 2021. "Reactive Transport Simulation of Cavern Formation along Fractures in Carbonate Rocks" Water 13, no. 1: 38. https://doi.org/10.3390/w13010038
APA StyleDuan, R., Shang, G., Yu, C., Wang, Q., Zhang, H., Wang, L., Xu, Z., & Dong, Y. (2021). Reactive Transport Simulation of Cavern Formation along Fractures in Carbonate Rocks. Water, 13(1), 38. https://doi.org/10.3390/w13010038





