Sea-Level Rise and Shoreline Changes Along an Open Sandy Coast: Case Study of Gulf of Taranto, Italy
Abstract
:1. Introduction
1.1. Local Sea-Level Changes
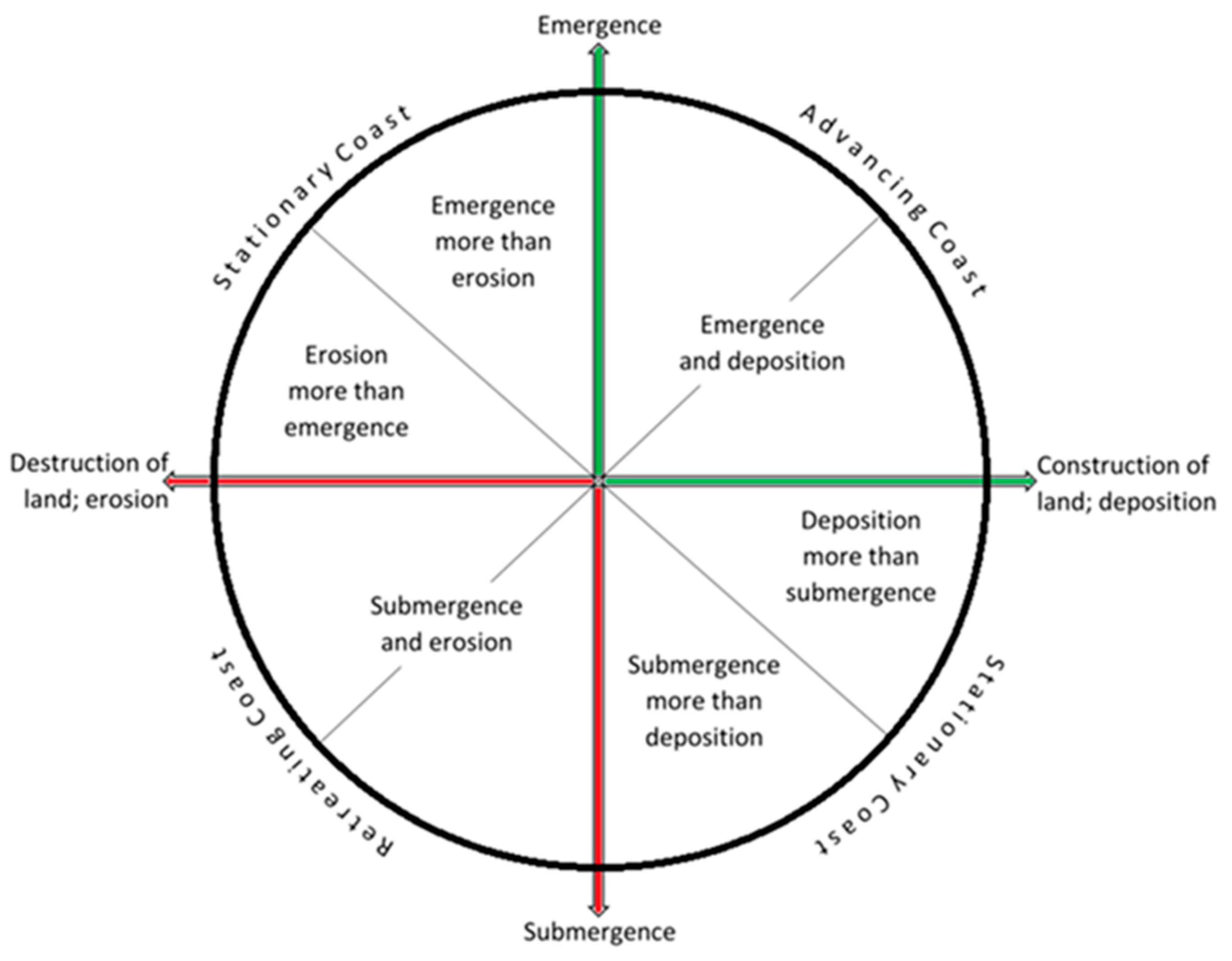
1.2. Coastal Behavior in Function of Sea-Level Rise
1.3. Objective
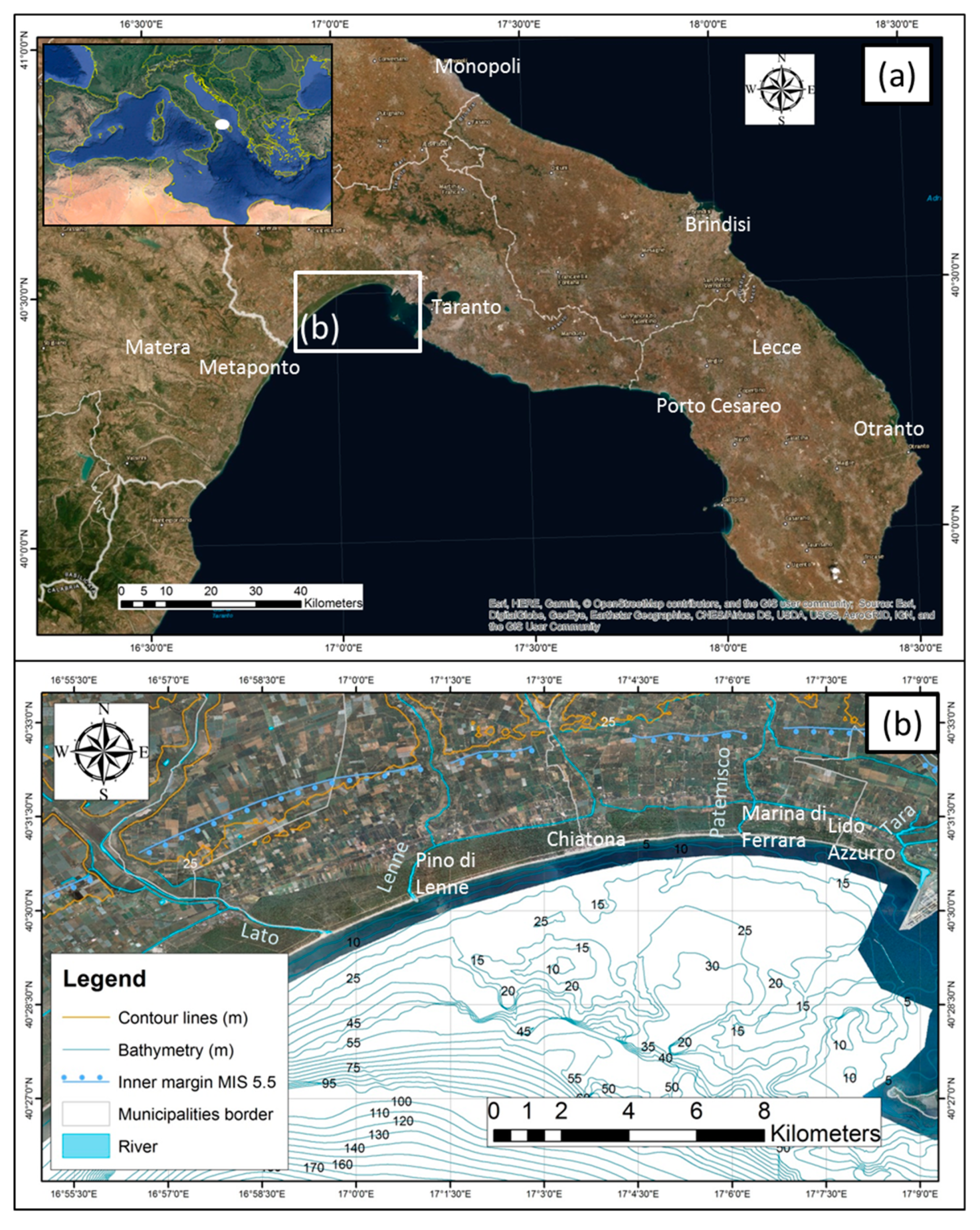
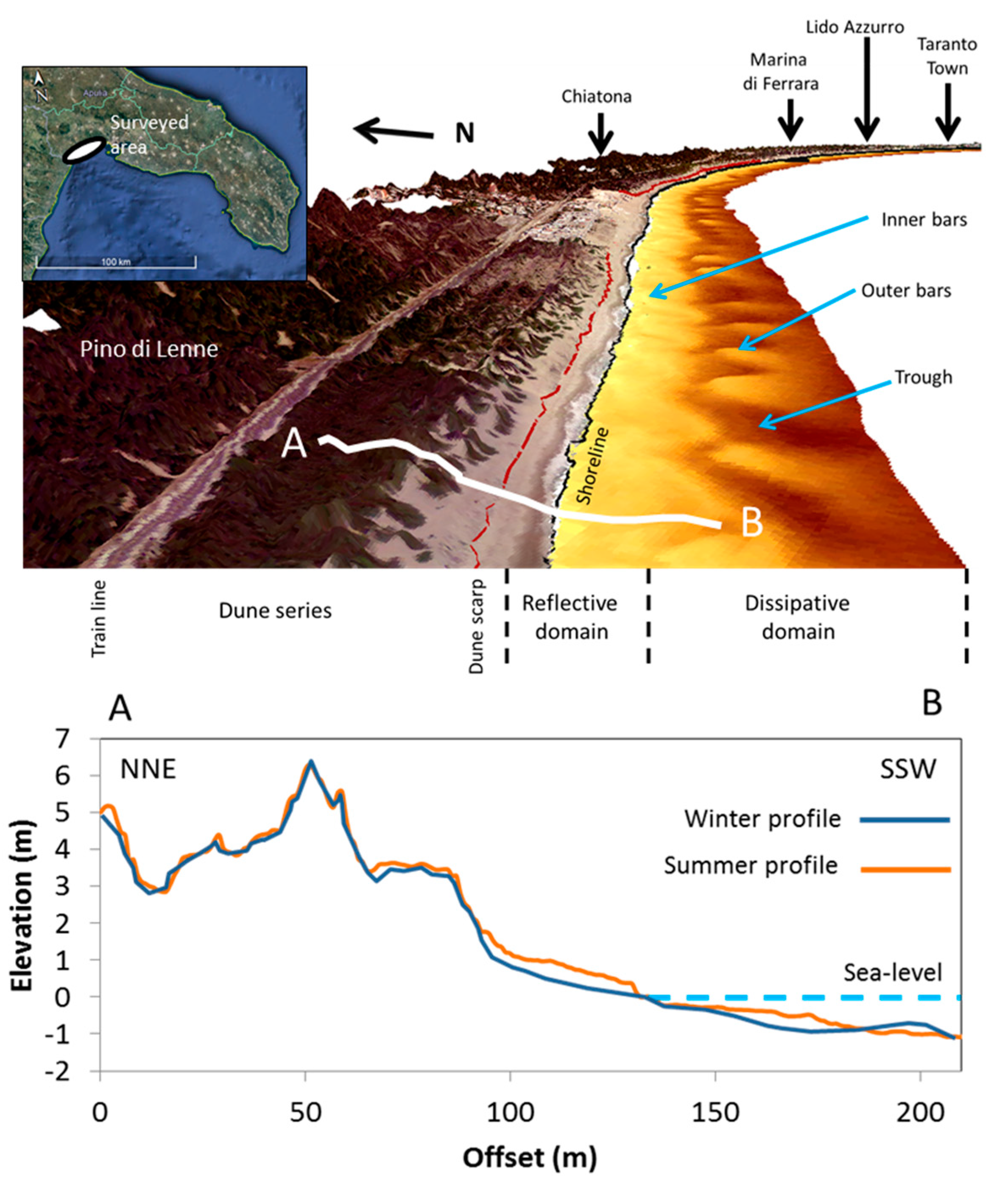
2. Geomorphological Setting
- Marine Isotope Stage (MIS) 2—Last Glacial Maximum (LGM): sea-level stand at about 120 m below the present sea level and deep engravings on the coastal wedge up to the shelf break
- 20-6/7 kyear: sea-level transgression with a rate of 8 mm/year and re-shaping of incised valleys along the Gulf of Taranto
- from 6/7 kyear to the second half of twentieth century: sea-level rise allowing the growth of the coastal plain and beach-dune system progradation conditioned by NE longshore drift.
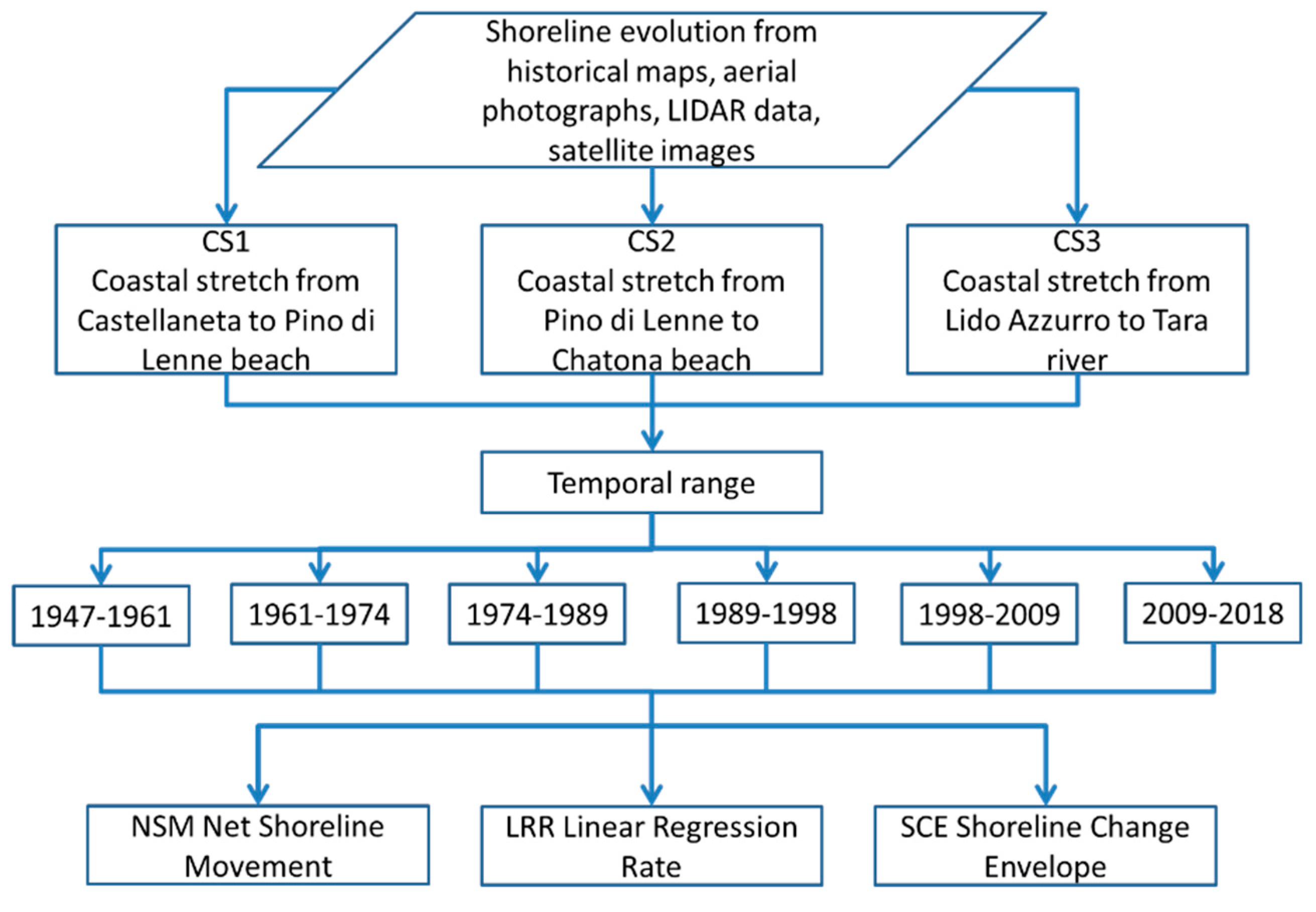
3. Methodology
3.1. Data
3.1.1. Tectonics and Isostasy
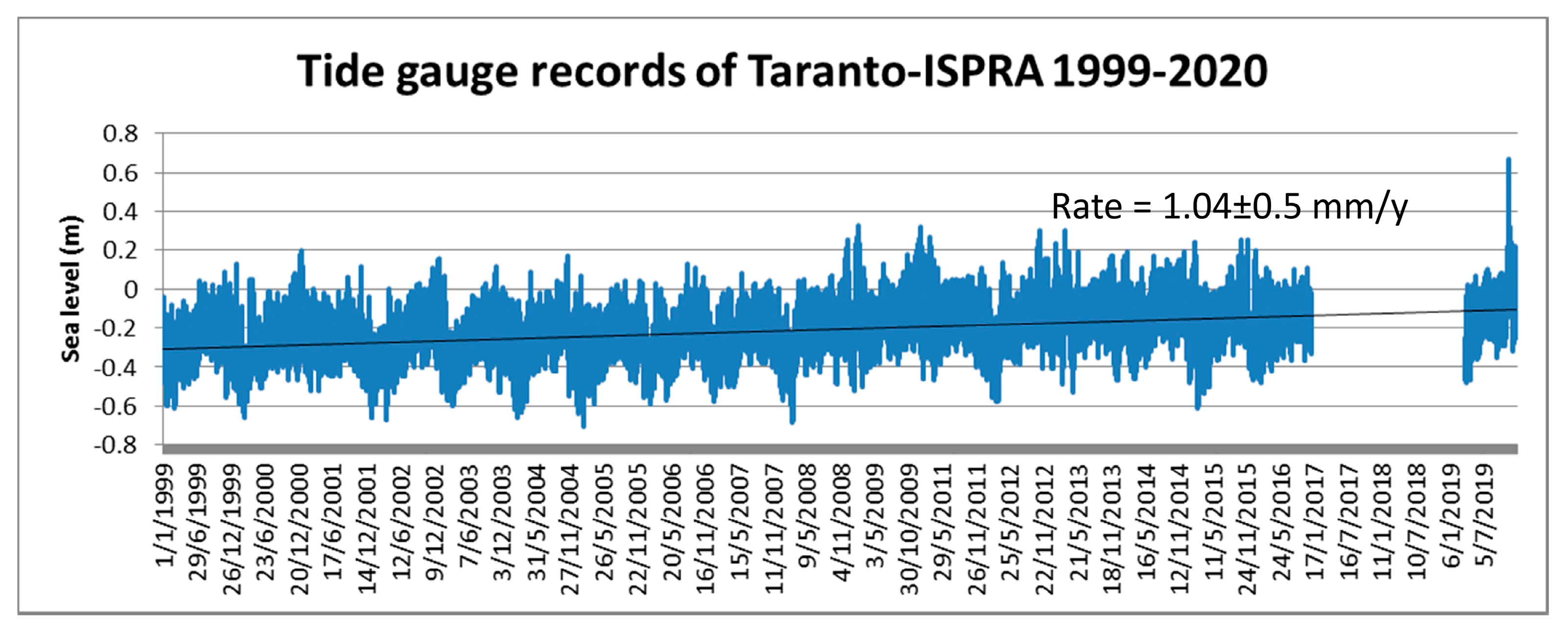
3.1.2. Tide and Sea-Level Trend
3.1.3. Orthophoto and Satellite Images
3.1.4. LIDAR and TLS
3.2. Data Processing
- Line drawing of the operator on the swash zone with an error range of ±3 m
- Weather conditions (tide excursion, atmospheric pressure, temperature, sea level, wind) at time of image acquisition with an error range of ±0.4 m
- Instrumental accuracy with an error range of 0.31–0.5 m
- Computer errors with an error range of ±0.1 m
3.3. Predictive Model of Submersion Surfaces Connected to Sea-Level Rise for 2100
- —relative sea-level rise (m)
- —relative sea-level rate (mm/year)
- —prediction time span (year)
- —tide amplitude (m)
- —longitude shoreline displacement (m)
- —latitude shoreline displacement (m)
- —shoreline rate changes (m/year)
- —normal shoreline angle (degrees).
4. Results
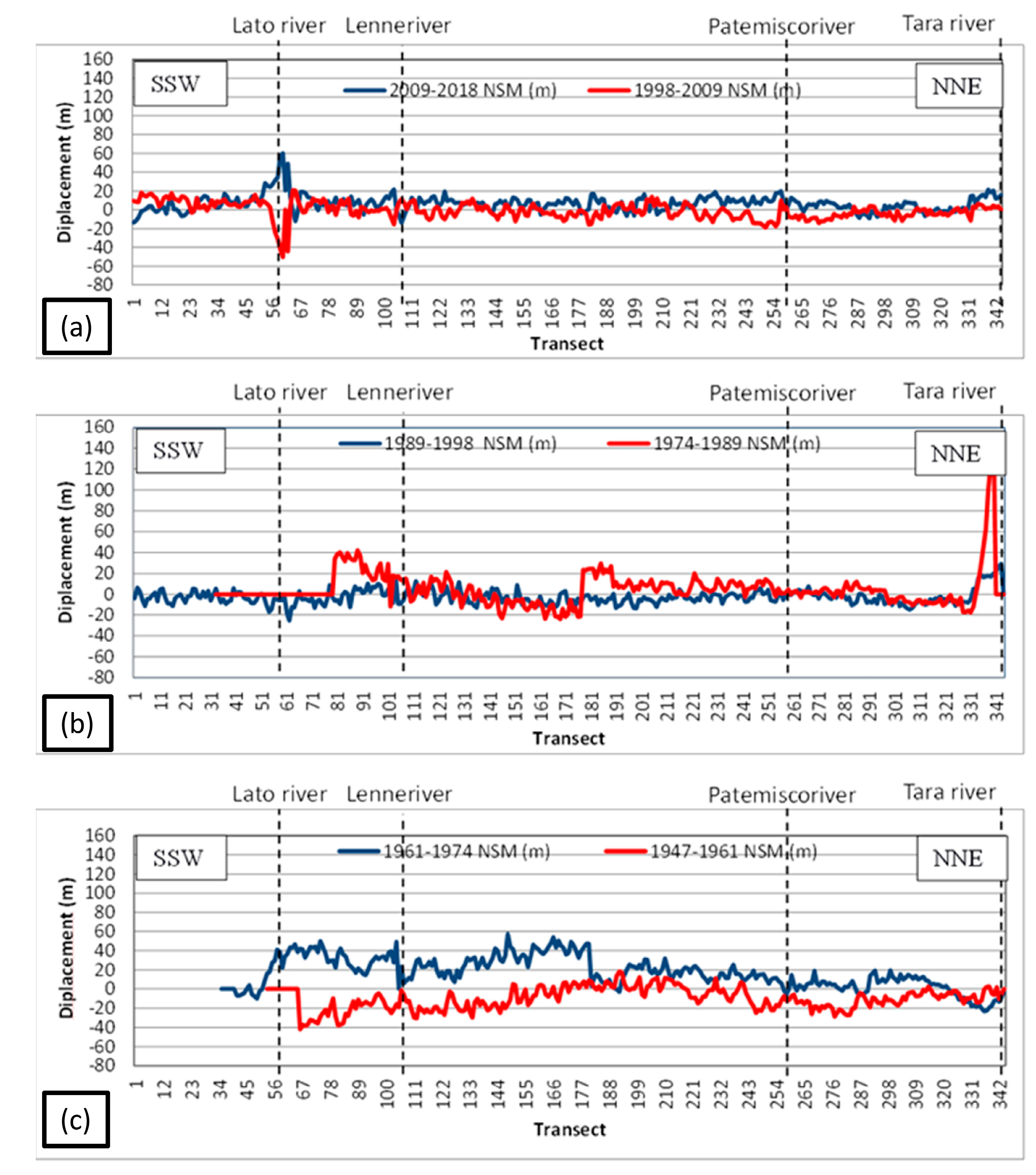
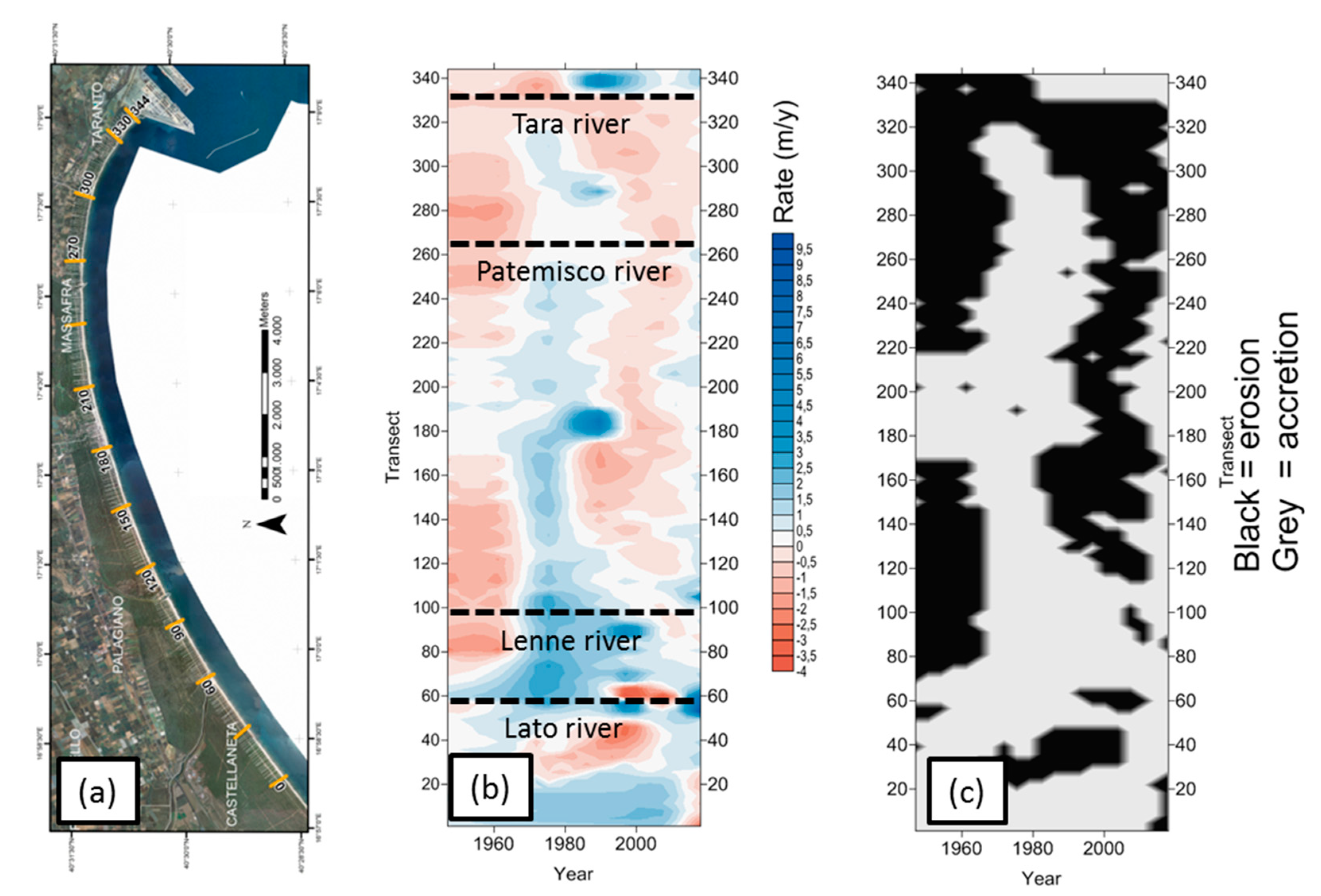
4.1. Shoreline Changes
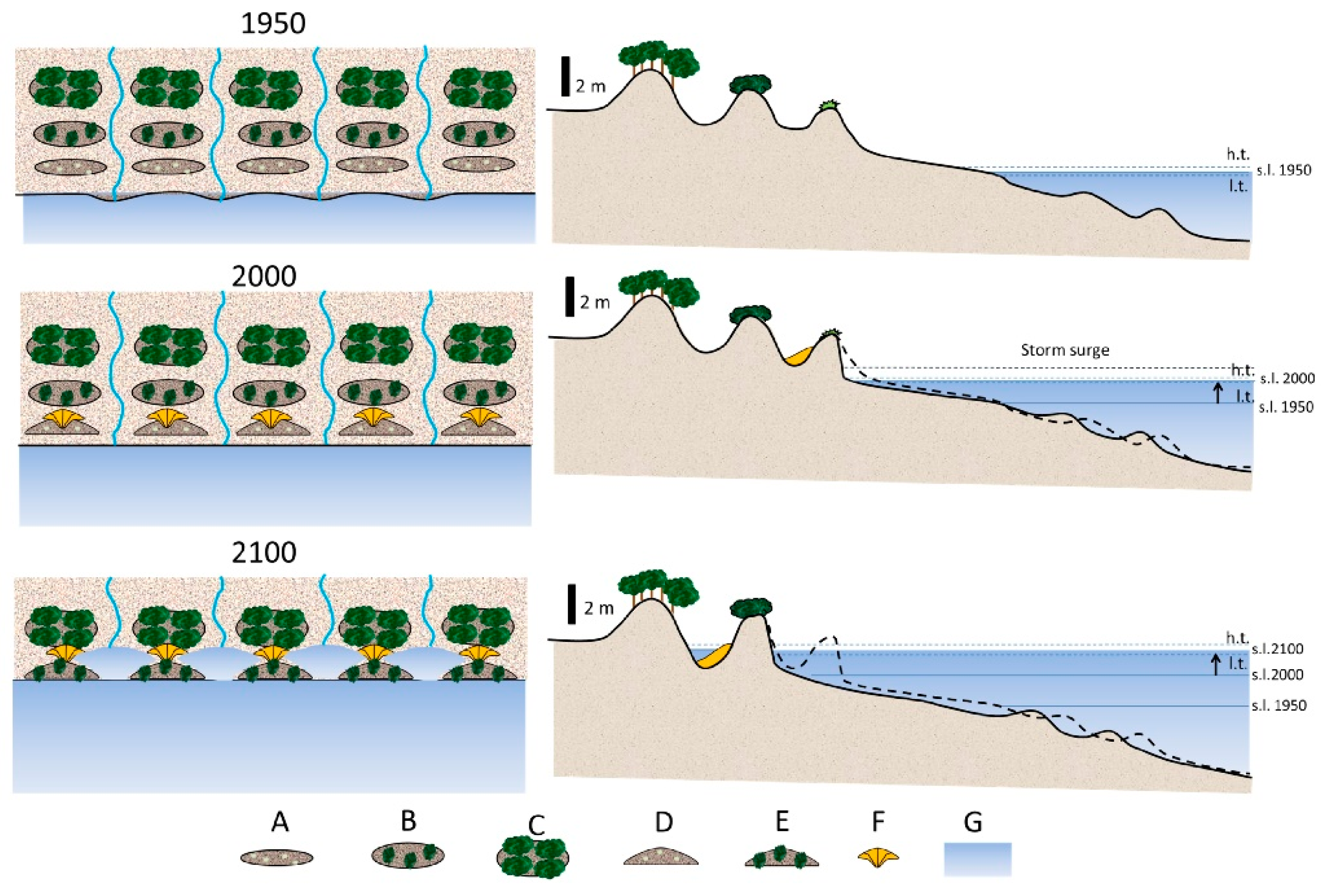
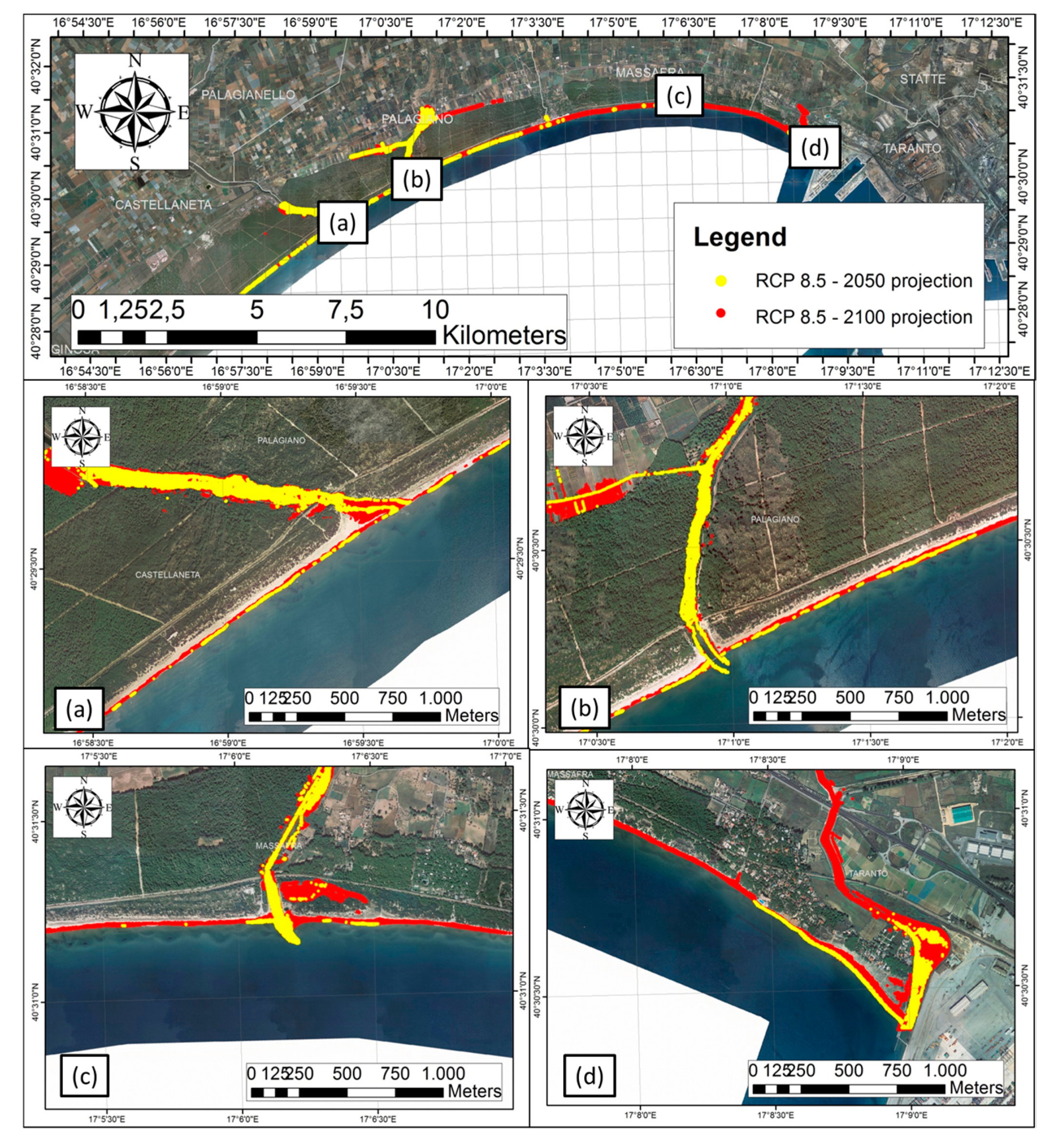
4.2. Geomorphological Scenario for the Future
5. Discussion
5.1. Simulating the Impact of a Rising Sea Level—A Difficult Methodology
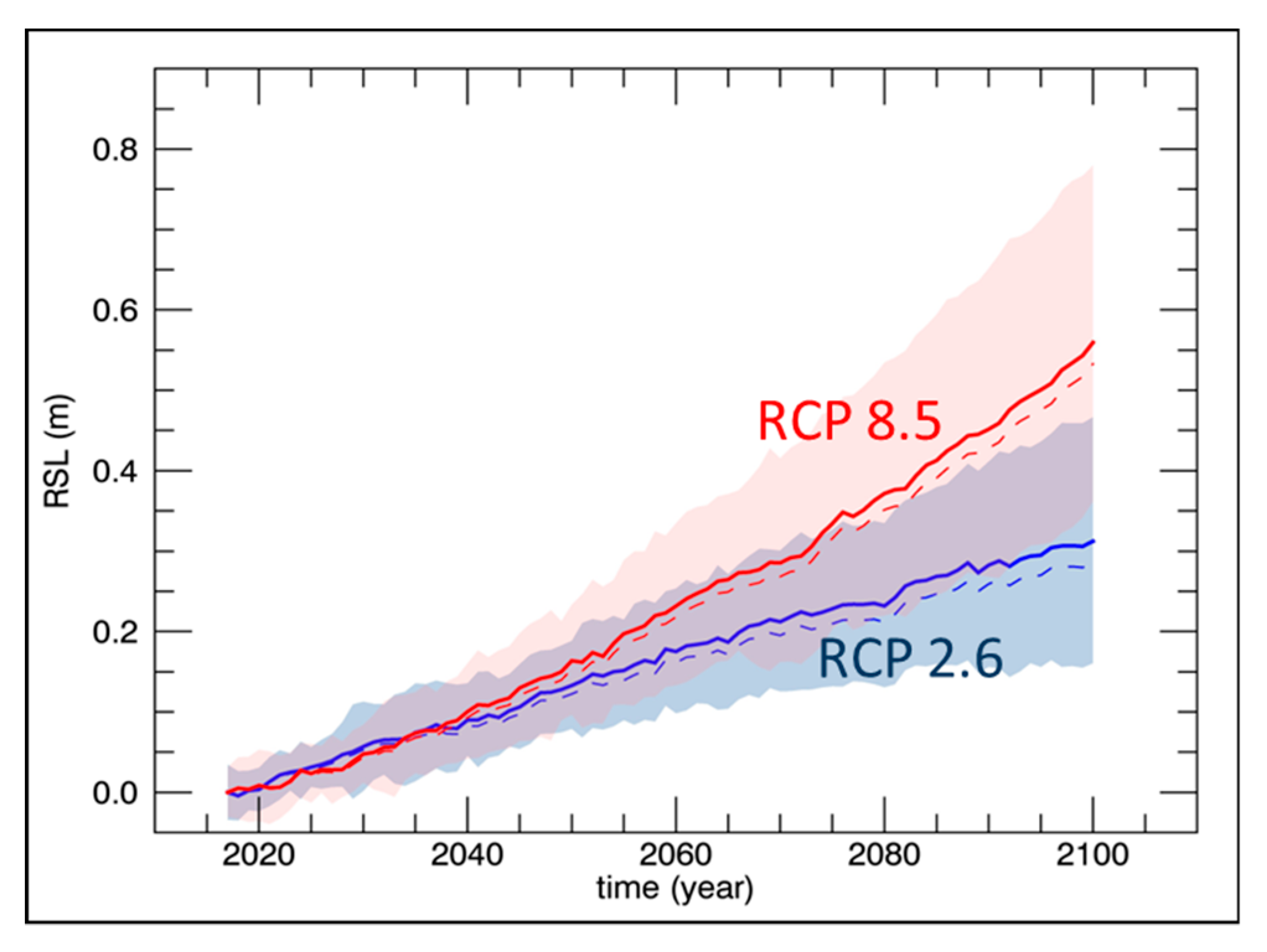
5.2. Sea-Level Rise and Predicted Shoreline Positions for Two IPCC Scenarios
6. Conclusions
- First half of the twentieth Century—progradation of the coastal system stretching from Castellaneta to Taranto, plantations of Pino d’Aleppo along the entire coast stabilized the dune system;
- 1950s–1970s—shoreline erosion highlighted along the entire littoral stretch, the building of holiday resorts in proximity to railway stations (Castellaneta Marina, Chiatona, Lido Azzurro);
- 1970s—construction of the Taranto Harbor outside the Mar Grande inlet with a substantial modification of the shoreline, and significant changes caused by the Tara, Patemisco, Lato and Lenne river mouths;
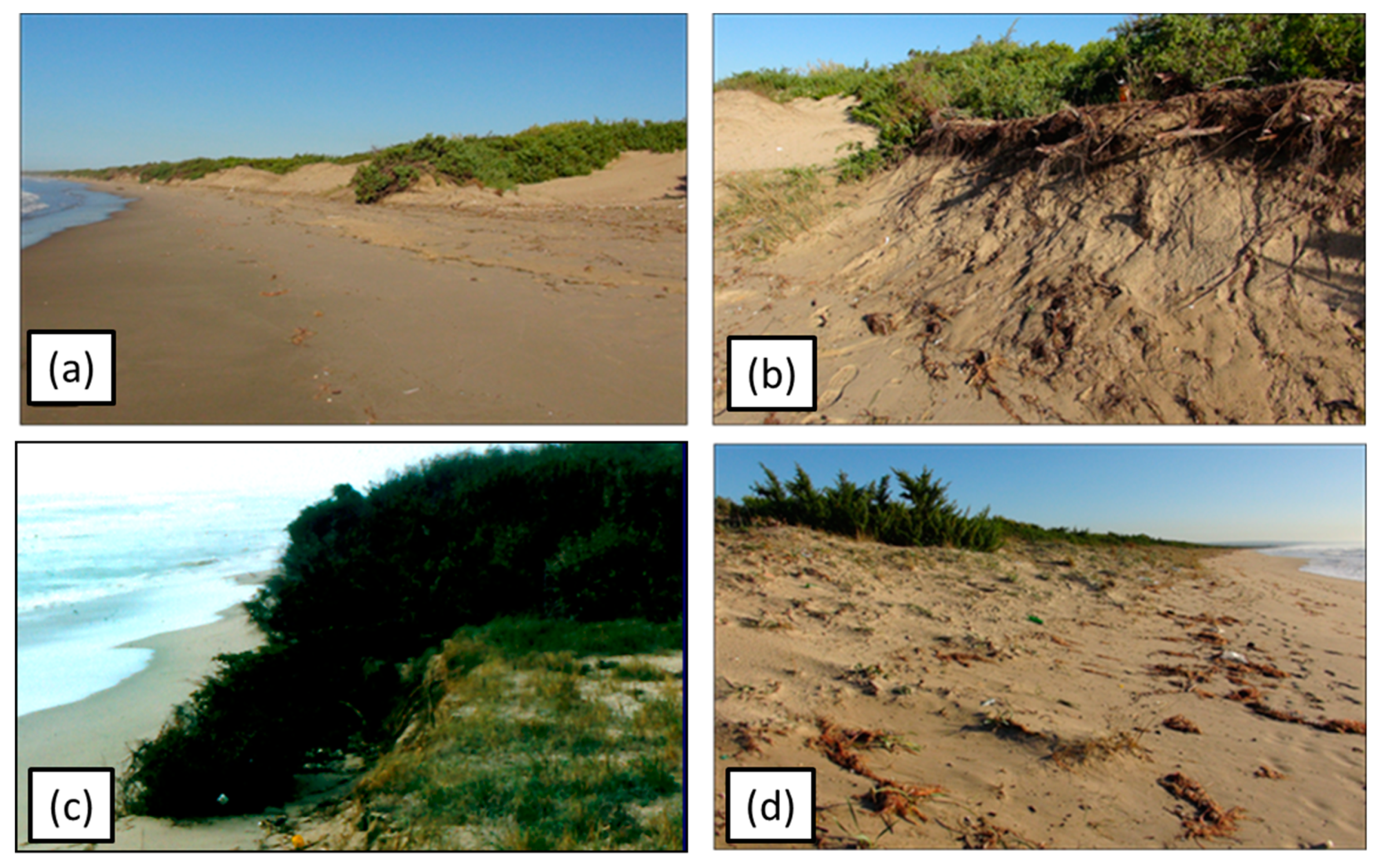
- 1970s–1990s—shoreline accretion for most of the littoral area, mainly for the beaches in proximity to the Tara river mouth, except for the Lido Azzurro and Marina di Ferrara coastal stretches, where a significant shoreline erosion and dune retreat were observed;
- 1999–2016—sea-level rise, recorded at the tide gauge in Taranto, at a rate of 1.04 ± 0.5 mm/year, and shoreline erosion recorded throughout the entire littoral area, with the exception of the area near the Tara mouth where accretion had continued;
- 2016–2018—shoreline stabilization and slow accretion in some coastal stretches, such as the Pino di Lenne beach, Chiatona beach, and Tara river mouth and an exception was made for Castellaneta, Marina di Ferrara and Lido Azzurro where a slow erosion was observed.
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Aucelli, P.P.C.; Iannantuono, E.; Rosskopf, C.M. Evoluzione recente e rischio di erosione della costa molisana (Italia meridionale). Ital. J. Geosci. 2009, 128, 759–771. [Google Scholar] [CrossRef]
- Aucelli, P.P.C.; Di Paola, G.; Incontri, P.; Rizzo, A.; Vilardo, G.; Benassai, G.; Buonocore, B.; Pappone, G. Coastal inundation risk assessment due to subsidence and sea level rise in a Mediterranean alluvial plain (Volturno coastal plain—Southern Italy). Estuar. Coast. Shelf Sci. 2017, 198, 597–609. [Google Scholar] [CrossRef]
- Sabatier, F.; Samat, O.; Brunel, C.; Heurtefeux, H.; Delanghe-Sabatier, D. Determination of set-back lines on eroding coasts. Example of the beaches of the Gulf of Lions (French Mediterranean Coast). J. Coast. Conserv. 2009, 13, 57. [Google Scholar] [CrossRef]
- Sabato, L.; Longhitano, S.G.; Gioia, D.; Cilumbriello, A.; Spalluto, L. Sedimentological and morpho-evolution maps of the ‘Bosco Pantano di Policoro’ coastal system (Gulf of Taranto, Southern Italy). J. Maps 2012, 8, 304–311. [Google Scholar] [CrossRef] [Green Version]
- Ayadi, K.; Boutiba, M.; Sabatier, F.; Guettouche, M.S. Detection and analysis of historical variations in the shoreline, using digital aerial photos, satellite images, and topographic surveys DGPS: Case of the Bejaia bay (East Algeria). Arab. J. Geosci. 2015, 9, 26. [Google Scholar] [CrossRef]
- Paskoff, R.P. Potential Implications of Sea-Level Rise for France. J. Coast. Res. 2004, 20, 424–434. [Google Scholar] [CrossRef]
- Antonioli, F.; Ferranti, L.; Fontana, A.; Amorosi, A.; Bondesan, A.; Braitengerg, C.; Dutton, A.; Fontolan, G.; Furlani, S.; Lambeck, K.; et al. Holocene relative sea-level changes and vertical movements along the Italian and Istrian coastlines. Quat. Int. 2009, 206, 102–133. [Google Scholar] [CrossRef]
- Mastronuzzi, G.; Antonioli, F.; Anzidei, M.; Auriemma, R.; Alfonso, C.; Scarano, T. Evidence of relative sea level rise along the coasts of central Apulia (Italy) during the late Holocene via maritime archaeological indicators. Quat. Int. 2017, 439, 65–78. [Google Scholar] [CrossRef]
- Brunel, C.; Sabatier, F. Potential influence of sea-level rise in controlling shoreline position on the French Mediterranean Coast. Geomorphology 2009, 107, 47–57. [Google Scholar] [CrossRef]
- Le Cozannet, G.; Garcin, M.; Yates, M.; Idier, D.; Meyssignac, B. Approaches to evaluate the recent impacts of sea-level rise on shoreline changes. Earth-Sci. Rev. 2014, 138, 47–60. [Google Scholar] [CrossRef] [Green Version]
- Lambeck, K.; Anzidei, M.; Antonioli, F.; Benini, A.; Esposito, A. Sea level in Roman time in the Central Mediterranean and implications for recent change. Earth Planet. Sci. Lett. 2004, 224, 563–575. [Google Scholar] [CrossRef]
- Rovere, A.; Stocchi, P.; Vacchi, M. Eustatic and Relative Sea Level Changes. Curr. Clim. Chang. Rep. 2016, 2, 221–231. [Google Scholar] [CrossRef] [Green Version]
- Lambeck, K.; Antonioli, F.; Anzidei, M.; Ferranti, L.; Leoni, G.; Scicchitano, G.; Silenzi, S. Sea level change along the Italian coast during the Holocene and projections for the future. Quat. Int. 2011, 232, 250–257. [Google Scholar] [CrossRef]
- Spampinato, C.R.; Costa, B.; Di Stefano, A.; Monaco, C.; Scicchitano, G. The contribution of tectonics to relative sea-level change during the Holocene in coastal south-eastern Sicily: New data from boreholes. Quat. Int. 2011, 232, 214–227. [Google Scholar] [CrossRef]
- Spampinato, C.R.; Scicchitano, G.; Ferranti, L.; Monaco, C. Raised Holocene paleo-shorelines along the Capo Schisò coast, Taormina: New evidence of recent co-seismic deformation in northeastern Sicily (Italy). J. Geodyn. 2012, 55, 18–31. [Google Scholar] [CrossRef]
- Anzidei, M.; Bosman, A.; Carluccio, R.; Casalbore, D.; Caracciolo, F.D.; Esposito, A.; Nicolosi, I.; Pietrantonio, G.; Vecchio, A.; Carmisciano, C.; et al. Flooding scenarios due to land subsidence and sea-level rise: A case study for Lipari Island (Italy). Terra Nova 2017, 29, 44–51. [Google Scholar] [CrossRef]
- Anzidei, M.; Scicchitano, G.; Tarascio, S.; De Guidi, G.; Monaco, C.; Barreca, G.; Mazza, G.; Serpelloni, E.; Vecchio, A. Coastal retreat and marine flooding scenario for 2100: A case study along the coast of Maddalena peninsula (Southeastern Sicily). Geogr. Fis. Din. Quat. 2018, 41, 5–16. [Google Scholar] [CrossRef]
- Anzidei, M.; Doumaz, F.; Vecchio, A.; Serpelloni, E.; Pizzimenti, L.; Civico, R.; Greco, M.; Martino, G.; Enei, F. Sea Level Rise Scenario for 2100 A.D. in the Heritage Site of Pyrgi (Santa Severa, Italy). J. Mar. Sci. Eng. 2020, 8, 64. [Google Scholar] [CrossRef] [Green Version]
- Marsico, A.; Lisco, S.; Presti, V.L.; Antonioli, F.; Amorosi, A.; Anzidei, M.; Deiana, G.; Falco, G.D.; Fontana, A.; Fontolan, G.; et al. Flooding scenario for four Italian coastal plains using three relative sea level rise models. J. Maps 2017, 13, 961–967. [Google Scholar] [CrossRef] [Green Version]
- Bonaldo, D.; Antonioli, F.; Archetti, R.; Bezzi, A.; Correggiari, A.; Davolio, S.; De Falco, G.; Fantini, M.; Fontolan, G.; Furlani, S.; et al. Integrating multidisciplinary instruments for assessing coastal vulnerability to erosion and sea level rise: Lessons and challenges from the Adriatic Sea, Italy. J. Coast. Conserv. 2019, 23, 19–37. [Google Scholar] [CrossRef]
- Rahmstorf, S. A Semi-Empirical Approach to Projecting Future Sea-Level Rise. Science 2007, 315, 368–370. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Rahmstorf, S.; Perrette, M.; Vermeer, M. Testing the robustness of semi-empirical sea level projections. Clim. Dyn. 2012, 39, 861–875. [Google Scholar] [CrossRef]
- Church, J.A.; Clark, P.U.; Cazenave, A.; Gregory, J.M.; Jevrejeva, S.; Levermann, A.; Merrifield, M.A.; Milne, G.A.; Nerem, R.S.; Nunn, P.D.; et al. Sea-Level Rise by 2100. Science 2013, 342, 1445. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Church, J.A.; Clark, P.U.; Cazenave, A.; Gregory, J.M.; Jevrejeva, S.; Levermann, A.; Merrifield, M.A.; Milne, G.A.; Nerem, R.S.; Nunn, P.D.; et al. Chapter 13: Sea-level change. In Climate Change 2013: The Physical Science Basis; Contribution of Working Group I to the Fifth Assessment Report of the Intergovernmental Panel on Climate Change; Stocker, T.F., Qin, D., Plattner, G.-K., Tignor, M., Allen, S.K., Boschung, J., Nauels, A., Xia, Y., Bex, V., Midgley, P.M., Eds.; Cambridge University Press: Cambridge, UK; New York, NY, USA, 2013; pp. 1137–1216. [Google Scholar]
- Stocker, T.F.; Qin, D.; Plattner, G.-K.; Tignor, M.; Allen, S.K.; Boschung, J.; Nauels, A.; Xia, Y.; Bex, V.; Midgley, P.M. (Eds.) IPCC Climate Change 2013: The Physical Science Basis; Contribution of Working Group I to the Fifth Assessment Report of the Intergovernmental Panel on Climate Change; Cambridge University Press: Cambridge, UK; New York, NY, USA, 2013; p. 1535. [Google Scholar]
- Core Writing Team; Pachauri, R.K.; Meyer, L.A. (Eds.) IPCC Climate Change 2014: Synthesis Report; Contribution of Working Groups I, II and III to the Fifth Assessment Report of the Intergovernmental Panel on Climate Change; IPCC: Geneva, Switzerland, 2014; p. 151. [Google Scholar]
- IPCC Special Report on the Ocean and Cryosphere in a Changing Climate, Pörtner, H.-O.; Roberts, D.C.; Masson-Delmotte, V.; Zhai, P.; Tignor, M.; Poloczanska, E.; Mintenbeck, K.; Alegría, A.; Nicolai, M.; Okem, A.; et al. (Eds.) 2019; in press.
- Wöppelmann, G.; Marcos, M. Coastal sea level rise in southern Europe and the nonclimate contribution of vertical land motion. J. Geophys. Res. Ocean. 2012, 117. [Google Scholar] [CrossRef] [Green Version]
- Anzidei, M.; Lambeck, K.; Antonioli, F.; Furlani, S.; Mastronuzzi, G.; Serpelloni, E.; Vannucci, G. Coastal structure, sea-level changes and vertical motion of the land in the Mediterranean. Geol. Soc. Lond. Spec. Publ. 2014, 388, 453–479. [Google Scholar] [CrossRef]
- Vermeer, M.; Rahmstorf, S. Global sea level linked to global temperature. Proc. Natl. Acad. Sci. USA 2009, 106, 21527–21532. [Google Scholar] [CrossRef] [Green Version]
- Vecchio, A.; Anzidei, M.; Serpelloni, E.; Florindo, F. Natural Variability and Vertical Land Motion Contributions in the Mediterranean Sea-Level Records over the Last Two Centuries and Projections for 2100. Water 2019, 11, 1480. [Google Scholar] [CrossRef] [Green Version]
- Lionello, P.; Conte, D.; Marzo, L.; Scarascia, L. The contrasting effect of increasing mean sea level and decreasing storminess on the maximum water level during storms along the coast of the Mediterranean Sea in the mid 21st century. Glob. Planet. Chang. 2017, 151, 80–91. [Google Scholar] [CrossRef]
- Antonioli, F.; Anzidei, M.; Amorosi, A.; Lo Presti, V.; Mastronuzzi, G.; Deiana, G.; De Falco, G.; Fontana, A.; Fontolan, G.; Lisco, S.; et al. Sea-level rise and potential drowning of the Italian coastal plains: Flooding risk scenarios for 2100. Quat. Sci. Rev. 2017, 158, 29–43. [Google Scholar] [CrossRef] [Green Version]
- Bamber, J.L.; Oppenheimer, M.; Kopp, R.E.; Aspinall, W.P.; Cooke, R.M. Ice sheet contributions to future sea-level rise from structured expert judgment. Proc. Natl. Acad. Sci. USA 2019, 116, 11195–11200. [Google Scholar] [CrossRef] [Green Version]
- Kulp, S.A.; Strauss, B.H. New elevation data triple estimates of global vulnerability to sea-level rise and coastal flooding. Nat. Commun. 2019, 10, 1–12. [Google Scholar] [CrossRef] [Green Version]
- Mastronuzzi, G.; Aringoli, D.; Aucelli, P.P.C.; Baldassarre, M.A.; Bellotti, P.; Bini, M.; Biolchi, S.; Bontempi, S.; Brandolini, P.; Chelli, A.; et al. Geomorphological map of the Italian Coast: From a descriptive to a morphodynamic approach. Geogr. Fis. Din. Quat. 2017, 40, 161–195. [Google Scholar] [CrossRef]
- Carbognin, L.; Teatini, P.; Tosi, L. Eustacy and land subsidence in the Venice Lagoon at the beginning of the new millennium. J. Mar. Syst. 2004, 51, 345–353. [Google Scholar] [CrossRef]
- Caldara, M.; Capolongo, D.; Damato, B.; Pennetta, L. Can the ground laser scanning technology be useful for coastal defenses monitoring? Ital. J. Eng. Geol. Environ. 2006, 1, 35–49. [Google Scholar]
- Syvitski, J.P.M.; Kettner, A.J.; Overeem, I.; Hutton, E.W.H.; Hannon, M.T.; Brakenridge, G.R.; Day, J.; Vörösmarty, C.; Saito, Y.; Giosan, L.; et al. Sinking deltas due to human activities. Nat. Geosci. 2009, 2, 681–686. [Google Scholar] [CrossRef]
- De Santis, V.; Caldara, M.; Torres, T.; Ortiz, J.E.; Sánchez-Palencia, Y. A review of MIS 7 and MIS 5 terrace deposits along the Gulf of Taranto based on new stratigraphic and chronological data. Ital. J. Geosci. 2018, 137, 349–368. [Google Scholar] [CrossRef]
- Bruun, P. Sea-Level Rise as a Cause of Shore Erosion. J. Waterw. Harb. Div. 1962, 88, 117–132. [Google Scholar]
- Dubois, R.N. How does a Barrier Shoreface Respond to a Sea-Level Rise? J. Coast. Res. 2002, 18, 3–5. [Google Scholar]
- Lorenzo-Trueba, J.; Ashton, A.D. Rollover, drowning, and discontinuous retreat: Distinct modes of barrier response to sea-level rise arising from a simple morphodynamic model. J. Geophys. Res. Earth Surf. 2014, 119, 779–801. [Google Scholar] [CrossRef] [Green Version]
- Valentin, H. Die Küsten der Erde. Petermanns Geogr. Mitt. Ergänzsungheft 1952, 246, 118. [Google Scholar]
- Wong, T.E.; Bakker, A.M.; Keller, K. Impacts of Antarctic fast dynamics on sea level projections and coastal flood defense. Clim. Change 2017, 144, 347–364. [Google Scholar] [CrossRef]
- Bruun, P. Coast Erosion and the Development of Beach Profiles; U.S. Beach Erosion Board: Washington, DC, USA, 1954. [Google Scholar]
- Davidson-Arnott, R.G.D. Conceptual Model of the Effects of Sea Level Rise on Sandy Coasts. J. Coast. Res. 2005, 21, 1166–1172. [Google Scholar] [CrossRef] [Green Version]
- Cilumbriello, A.; Sabato, L.; Tropeano, M.; Gallicchio, S.; Grippa, A.; Maiorano, P.; Mateu-Vicens, G.; Rossi, C.A.; Spilotro, G.; Calcagnile, L.; et al. Sedimentology, stratigraphic architecture and preliminary hydrostratigraphy of the Metaponto coastal-plain subsurface (Southern Italy). Mem. Descr. Carta Geol. d’Ital. 2010, XC, 67–84. [Google Scholar]
- Tropeano, M.; Cilumbriello, A.; Sabato, L.; Gallicchio, S.; Grippa, A.; Longhitano, S.G.; Bianca, M.; Gallipoli, M.R.; Mucciarelli, M.; Spilotro, G. Surface and subsurface of the Metaponto Coastal Plain (Gulf of Taranto—Southern Italy): Present-day- vs. LGM-landscape. Geomorphology 2013, 203, 115–131. [Google Scholar] [CrossRef]
- Nakada, M.; Lambeck, K. The melting history of the late Pleistocene Antarctic ice sheet. Nature 1988, 333, 36–40. [Google Scholar] [CrossRef]
- Mastronuzzi, G.; Sansò, P. Morfologia e genesi delle Isole Chéradi e del Mar Grande (Taranto, Puglia, Italia). Geogr. Fis. Din. Quat. 1998, 21, 131–138. [Google Scholar]
- Mastronuzzi, G.; Sansò, P. Quaternary coastal morphology and sea level changes. In Proceedings of the Puglia 2003, Final Conference—Project IGCP 437 UNESCO—IUGS, Otranto/Taranto, Puglia, Italy, 22–28 September 2003; GIS Coast Coast—Gruppo Informale di Studi Costieri, Research Publication: Taranto, Italy, 2003; Volume 5, p. 184. [Google Scholar]
- Valenzano, E.; Scardino, G.; Cipriano, G.; Fago, P.; Capolongo, D.; De Giosa, F.; Lisco, S.; Mele, D.; Moretti, M.; Mastronuzzi, G. Holocene Morpho-Sedimentary Evolution of the Mar Piccolo Basin (Taranto, Southern Italy). Geogr. Fis. Din. Quat. 2018, 41, 119–135. [Google Scholar] [CrossRef]
- Zander, A.; Fulling, A.; Brückner, H.; Mastronuzzi, G. OSL dating of upper pleistocene littoral sediments: A contribution to the chronostratigraphy of raised marine terraces bordering the Gulf of Taranto, South Italy. Geogr. Fis. Din. Quat. 2006, 29, 33–50. [Google Scholar]
- Carter, R.W.G. Coastal Evolution: Late Quaternary Shoreline Morphodynamics; Reprint Edizione; Cambridge University Press: Cambridge, UK, 1997; ISBN 978-0-521-59890-3. [Google Scholar]
- Mastronuzzi, G.; Sansò, P. Pleistocene sea-level changes, sapping processes and development of valley networks in the Apulia region (Southern Italy). Geomorphology 2002, 46, 19–34. [Google Scholar] [CrossRef]
- Mastronuzzi, G.; Romaniello, L. Holocene aeolian morphogenetic phases in Southern Italy: Problems in 14C age determinations using terrestrial gastropods. Quat. Int. 2008, 183, 123–134. [Google Scholar] [CrossRef]
- Caldara, M.; Centenaro, E.; Mastronuzzi, G.; Sansò, P.; Sergio, A. Features and present evolution of Apulian Coast (Southern Italy). J. Coast. Res. 1998, SI, 55–64. [Google Scholar]
- Lisi, I.; Bruschi, A.; Del Gizzo, M.; Archina, M.; Barbano, A.; Corsini, S. Le unità fisiografiche e le profondità di chiusura della costa italiana. L’ACQUA 2010, 2, 35–52. [Google Scholar]
- Di Bucci, D.; Caputo, R.; Mastronuzzi, G.; Fracassi, U.; Selleri, G.; Sansò, P. Quantitative analysis of extensional joints in the Southern Adriatic foreland (Italy), and the active tectonics of the Apulia region. J. Geodyn. 2011, 51, 141–155. [Google Scholar] [CrossRef] [Green Version]
- Amorosi, A.; Antonioli, F.; Bertini, A.; Marabini, S.; Mastronuzzi, G.; Montagna, P.; Negri, A.; Rossi, V.; Scarponi, D.; Taviani, M.; et al. The Middle–Upper Pleistocene Fronte Section (Taranto, Italy): An exceptionally preserved marine record of the Last Interglacial. Glob. Planet. Chang. 2014, 119, 23–38. [Google Scholar] [CrossRef]
- Lisco, S.; Corselli, C.; De Giosa, F.; Mastronuzzi, G.; Moretti, M.; Siniscalchi, A.; Marchese, F.; Bracchi, V.; Tessarolo, C.; Tursi, A. Geology of Mar Piccolo, Taranto (Southern Italy): The physical basis for remediation of a polluted marine area. J. Maps 2016, 12, 173–180. [Google Scholar] [CrossRef] [Green Version]
- Polcari, M.; Albano, M.; Montuori, A.; Bignami, C.; Tolomei, C.; Pezzo, G.; Falcone, S.; La Piana, C.; Doumaz, F.; Salvi, S.; et al. InSAR Monitoring of Italian Coastline Revealing Natural and Anthropogenic Ground Deformation Phenomena and Future Perspectives. Sustainability 2018, 10, 3152. [Google Scholar] [CrossRef] [Green Version]
- Savemedcoasts. Available online: http://www.savemedcoasts.eu/ (accessed on 4 April 2020).
- RING—Rete Integrata Nazionale GPS. Available online: http://ring.gm.ingv.it/ (accessed on 25 April 2020).
- Altamimi, Z.; Collilieux, X.; Métivier, L. ITRF2008: An improved solution of the international terrestrial reference frame. J. Geod. 2011, 85, 457–473. [Google Scholar] [CrossRef] [Green Version]
- Rete Mareografica Nazionale—Homepage. Available online: https://mareografico.it/ (accessed on 4 April 2020).
- Attivazione Del Nuovo Sistema Informativo Meteo Oceanografico Delle Coste Pugliesi (Simop). Available online: https://www.adb.puglia.it/public/news.php?extend.282 (accessed on 4 April 2020).
- Theiler, E.; Himmelstoss, E.; Zichichi, J.; Ergul, A. Digital Shoreline Analysis System (DSAS) Version 4.0-An ArcGIS Extension for Calculating Shoreline Change (Ver. 4.4, July 2017); U.S. Geological Survey Open-File Report; USGS, U.S.: Reston, VA, USA, 2017; p. 1278.
- Bonora, N.; Immordino, F.; Schiavi, C.; Simeoni, U.; Valpreda, E. Interaction between Catchment Basin Management and Coastal Evolution (Southern Italy). J. Coast. Res. 2002, 81–88. [Google Scholar] [CrossRef]
- AR5 Sea Level Rise. Available online: http://icdc.cen.uni-hamburg.de/1/daten/ocean/ar5-slr.html (accessed on 25 April 2020).
- Sabatier, F.; Provansal, M.; Fleury, T.-J. Discussion of: PASKOFF, R., 2004. Potential Implications of Sea-Level Rise for France. J. Coast. Res. 2004, 20, 424–434. [Google Scholar] [CrossRef]


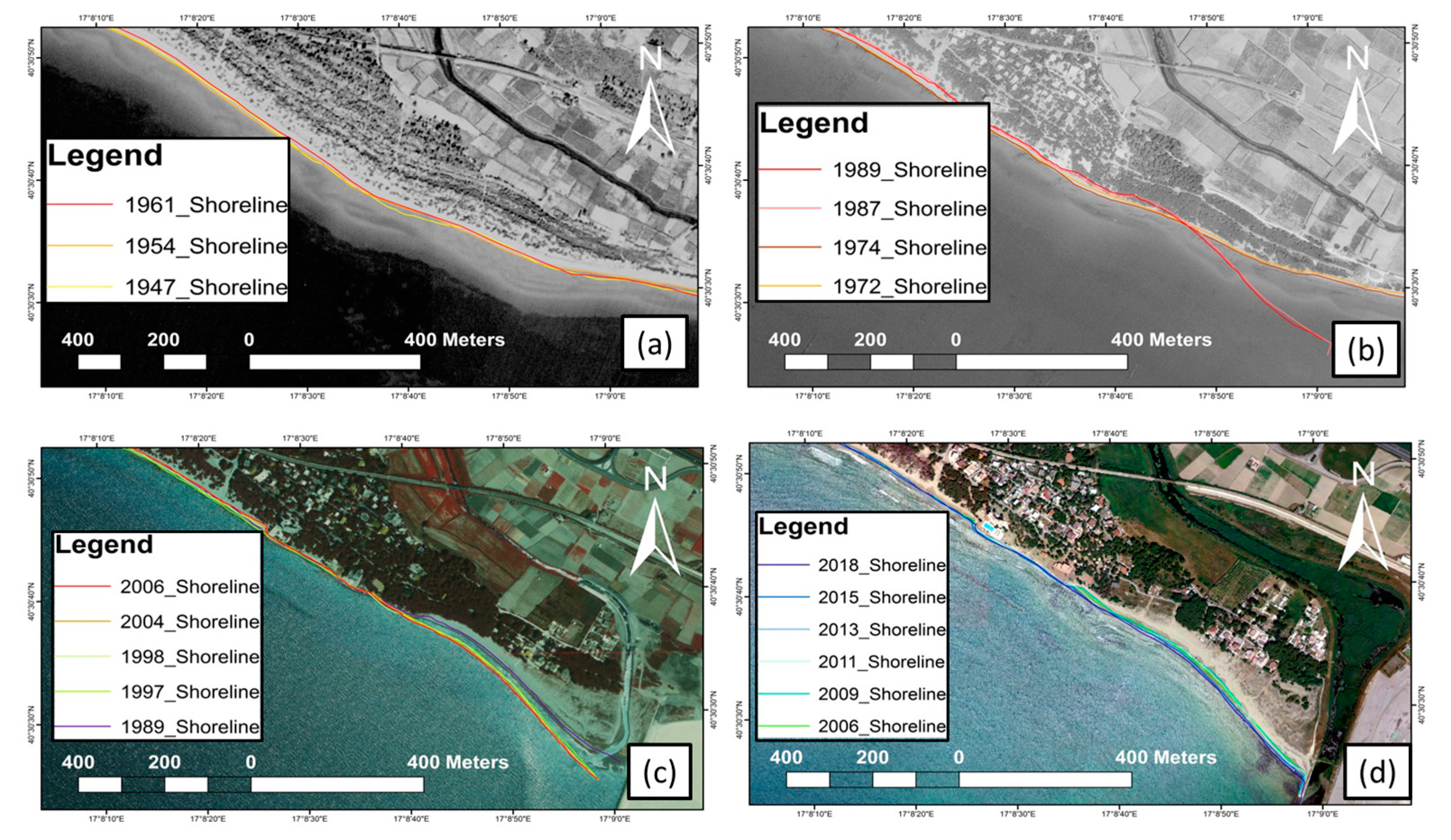
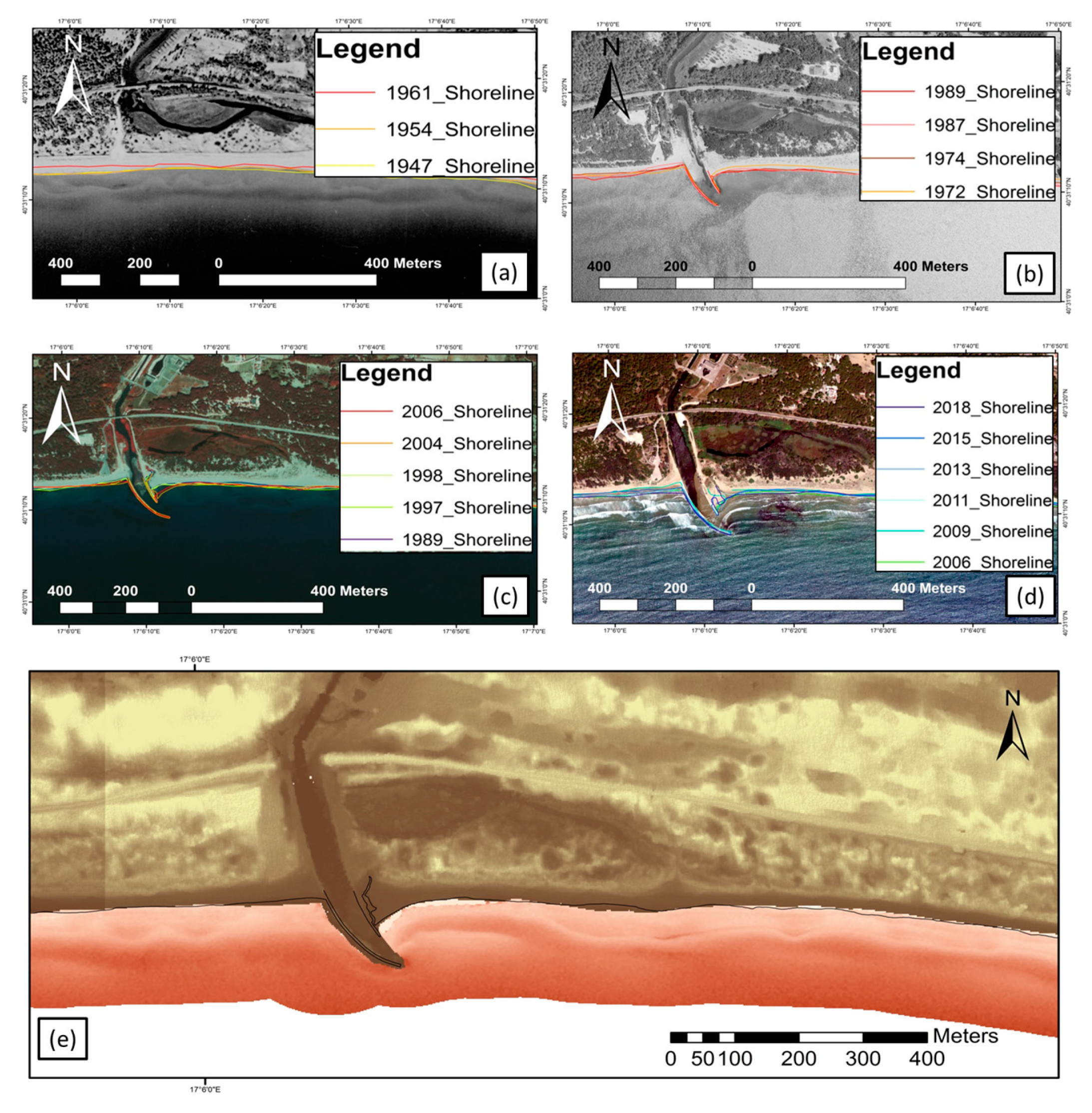
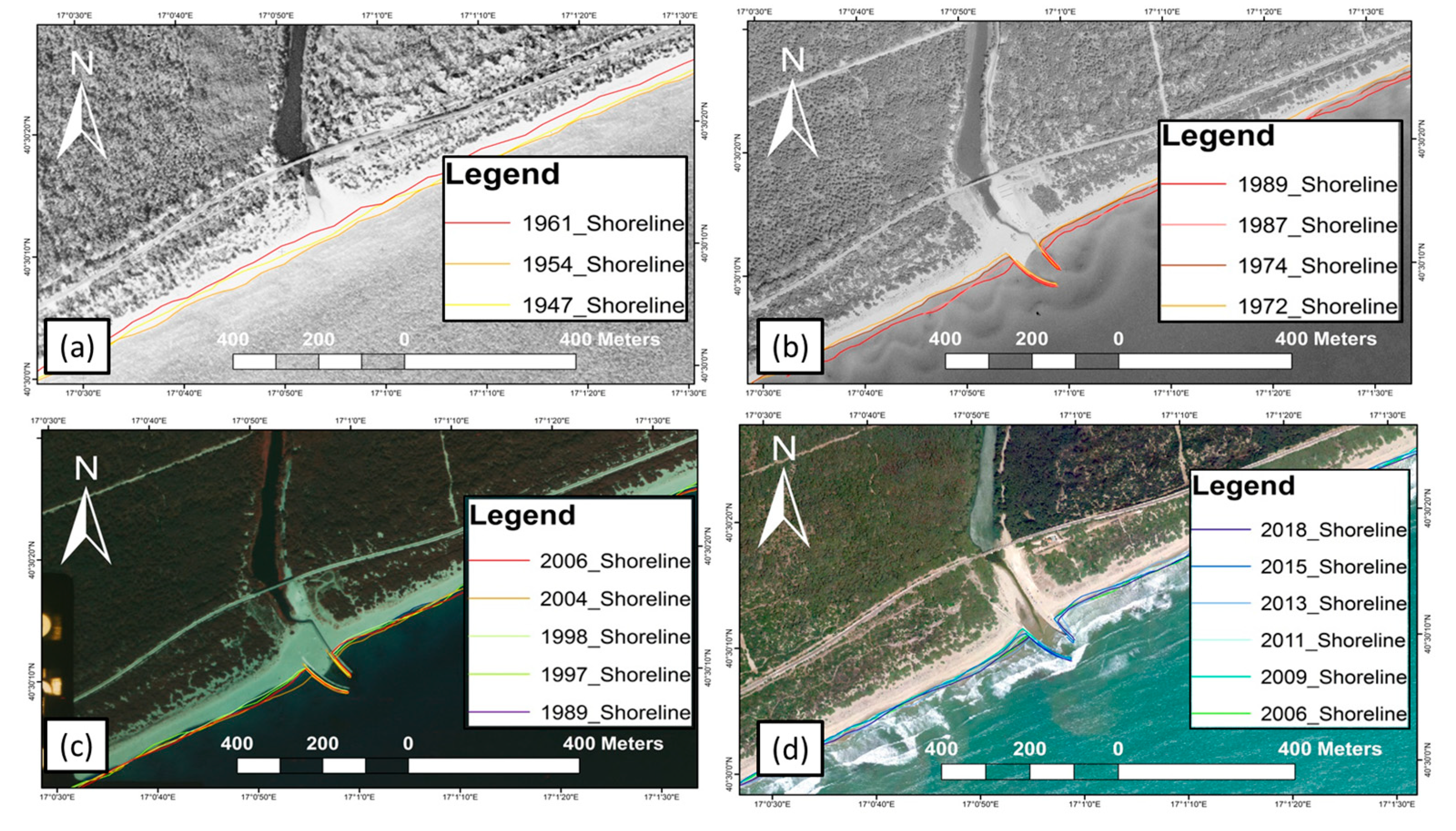
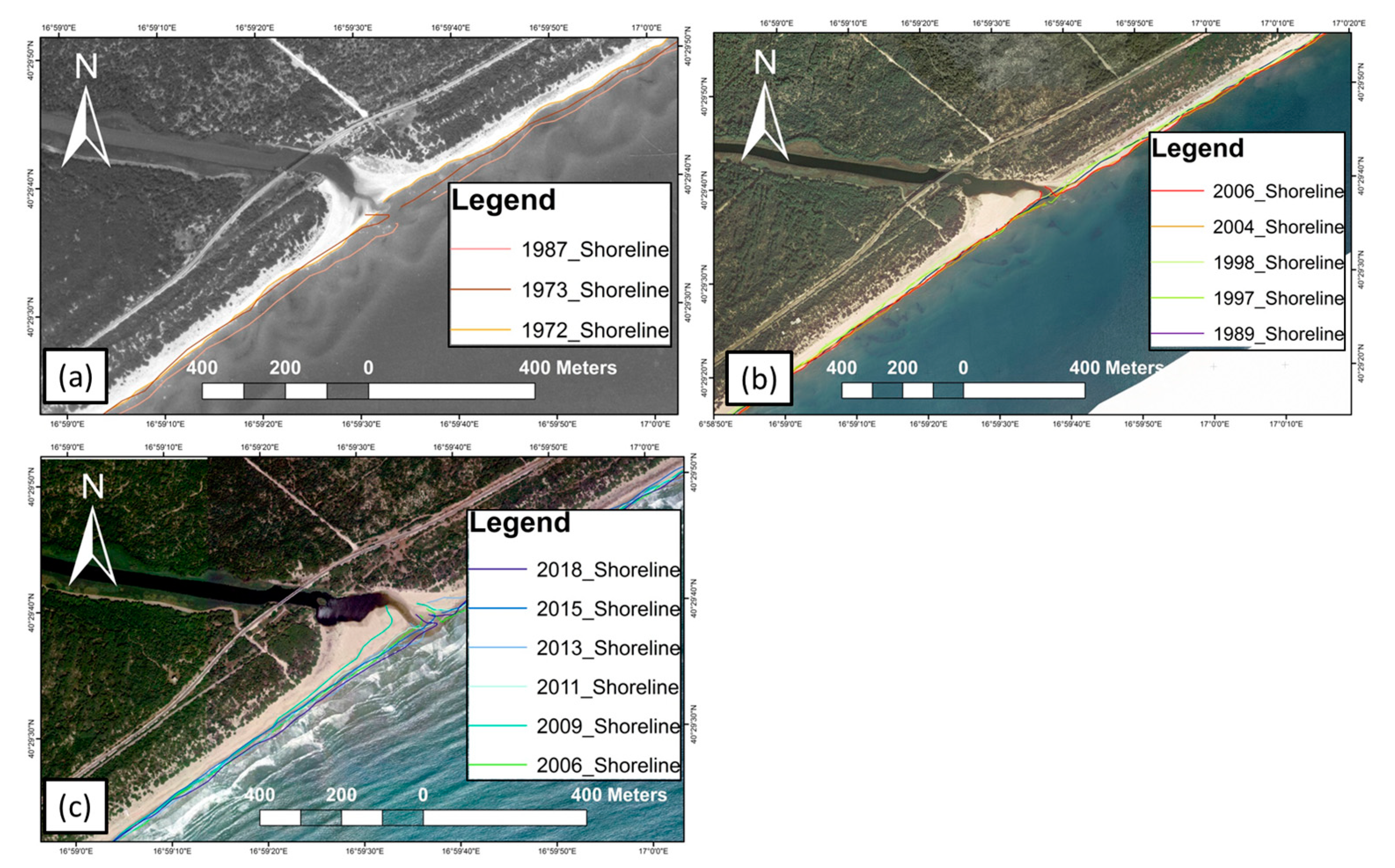
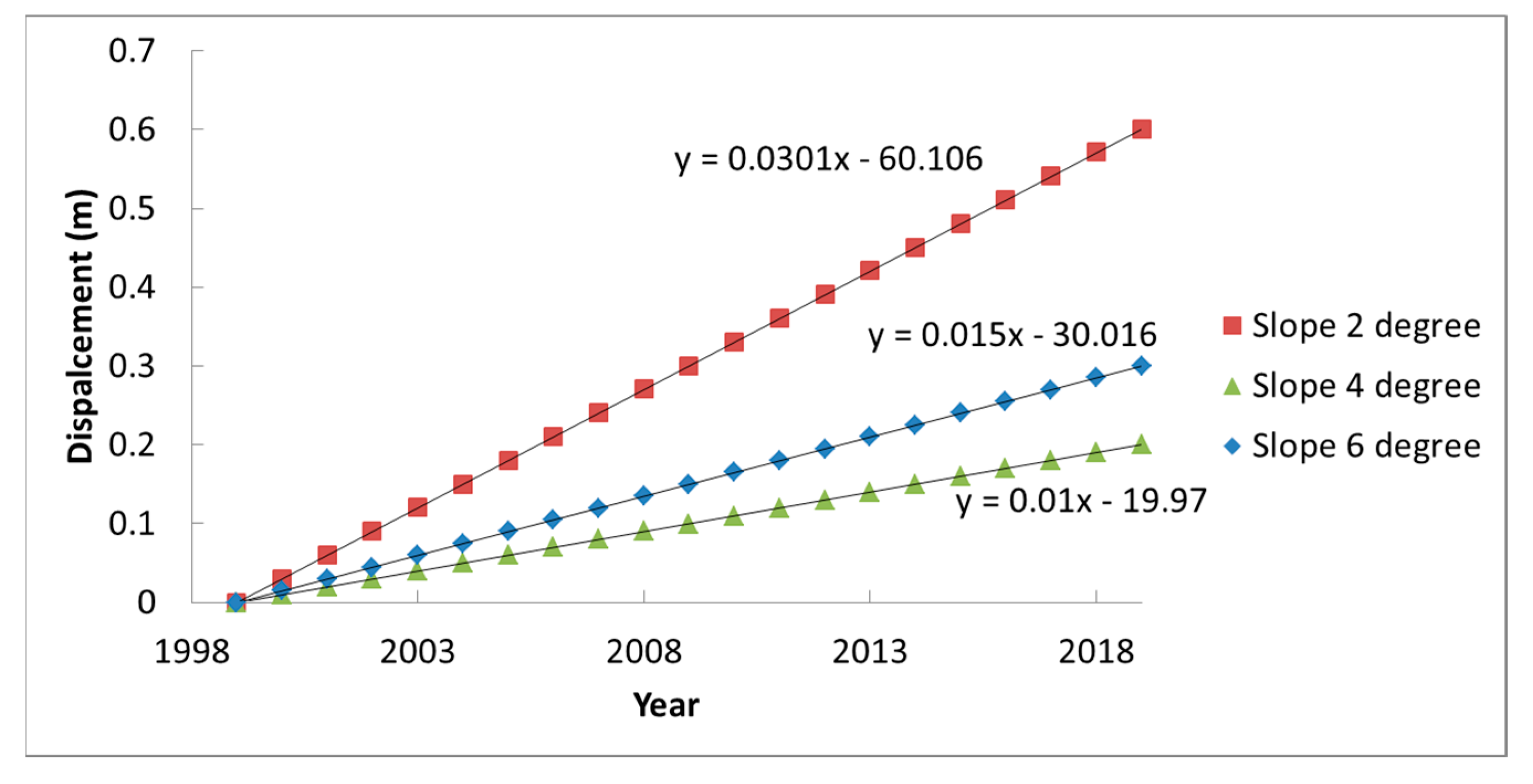
| ID | Coastal Slope (degrees) | Observed Shoreline Changes (m/year) | Geometric Horizontal Migration (m/year) | Effective Shoreline Changes (m/year) |
|---|---|---|---|---|
| CS1 | 6 | −0.40 | −0.01 | −0.39 |
| CS2 | 2 | −0.36 | −0.03 | −0.33 |
| CS3 | 4 | −0.38 | −0.02 | −0.36 |
| Relative Sea-Level Rise (m) | ||
|---|---|---|
| 2050 | 2100 | |
| RCP 2.6 | 0.133 ± 0.055 | 0.313 ± 0.153 |
| RCP 8.5 | 0.166 ± 0.068 | 0.559 ± 0.209 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Scardino, G.; Sabatier, F.; Scicchitano, G.; Piscitelli, A.; Milella, M.; Vecchio, A.; Anzidei, M.; Mastronuzzi, G. Sea-Level Rise and Shoreline Changes Along an Open Sandy Coast: Case Study of Gulf of Taranto, Italy. Water 2020, 12, 1414. https://doi.org/10.3390/w12051414
Scardino G, Sabatier F, Scicchitano G, Piscitelli A, Milella M, Vecchio A, Anzidei M, Mastronuzzi G. Sea-Level Rise and Shoreline Changes Along an Open Sandy Coast: Case Study of Gulf of Taranto, Italy. Water. 2020; 12(5):1414. https://doi.org/10.3390/w12051414
Chicago/Turabian StyleScardino, Giovanni, François Sabatier, Giovanni Scicchitano, Arcangelo Piscitelli, Maurilio Milella, Antonio Vecchio, Marco Anzidei, and Giuseppe Mastronuzzi. 2020. "Sea-Level Rise and Shoreline Changes Along an Open Sandy Coast: Case Study of Gulf of Taranto, Italy" Water 12, no. 5: 1414. https://doi.org/10.3390/w12051414
APA StyleScardino, G., Sabatier, F., Scicchitano, G., Piscitelli, A., Milella, M., Vecchio, A., Anzidei, M., & Mastronuzzi, G. (2020). Sea-Level Rise and Shoreline Changes Along an Open Sandy Coast: Case Study of Gulf of Taranto, Italy. Water, 12(5), 1414. https://doi.org/10.3390/w12051414









