Numerical Assessment of Shear Boundary Layer Formation in Sewer Systems with Fluid-Sediment Phases
Abstract
1. Introduction
2. Materials and Methods
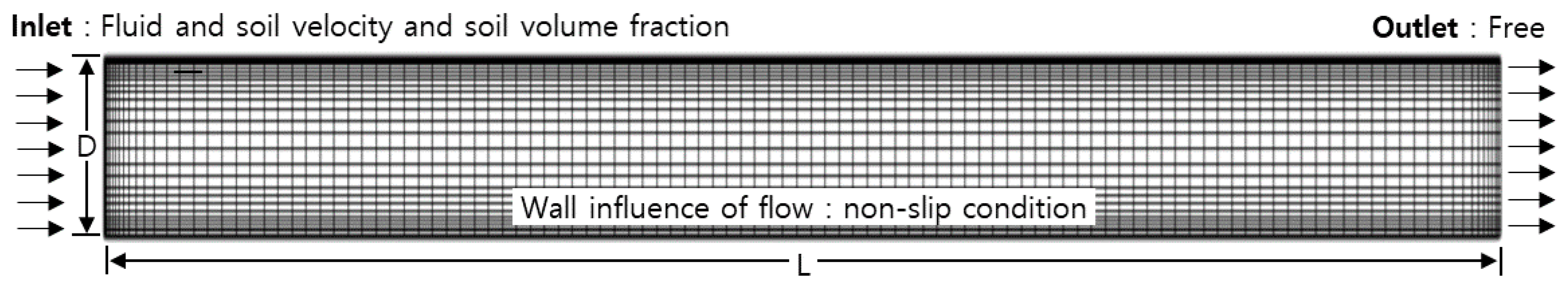
2.1. Numerical Analysis
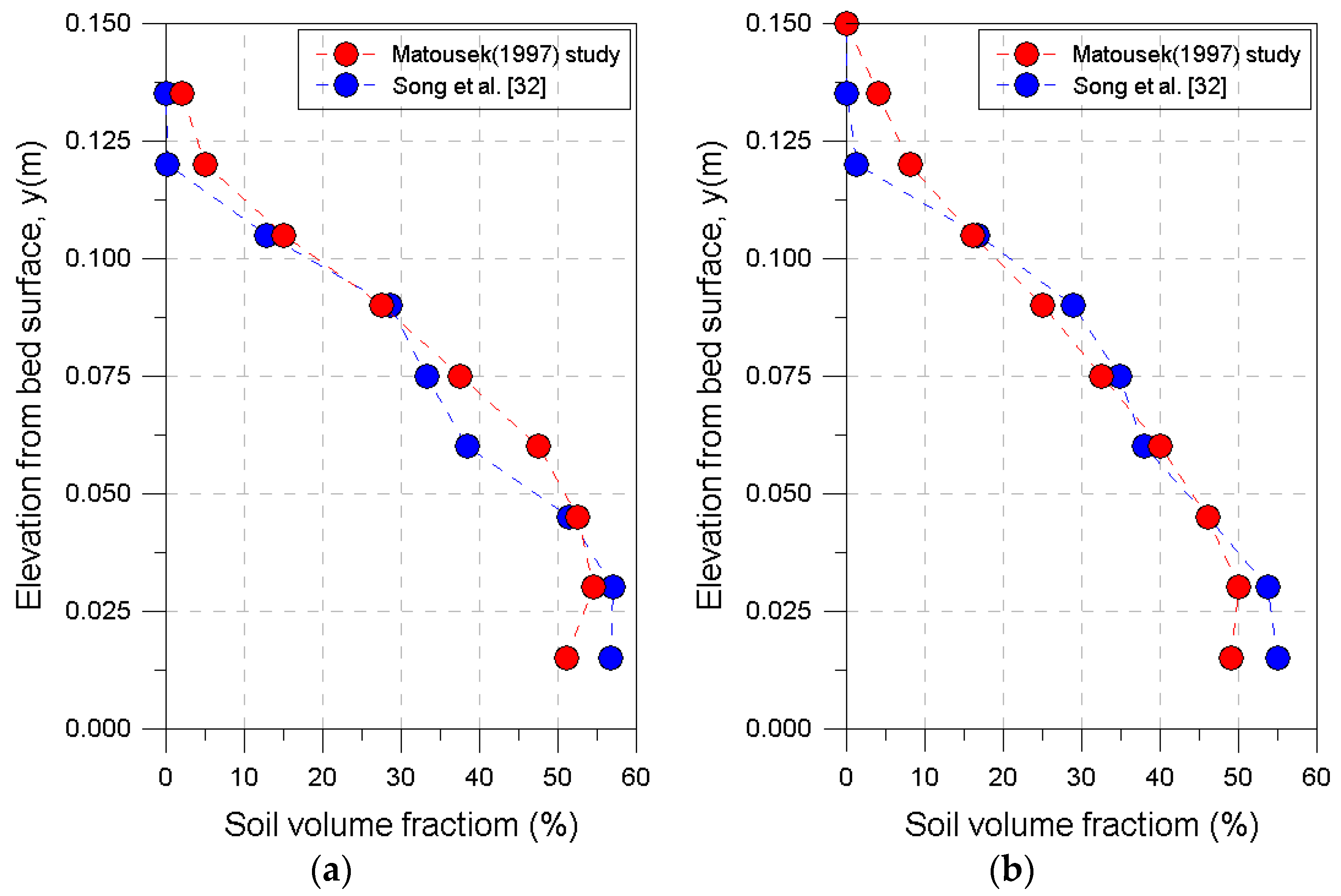
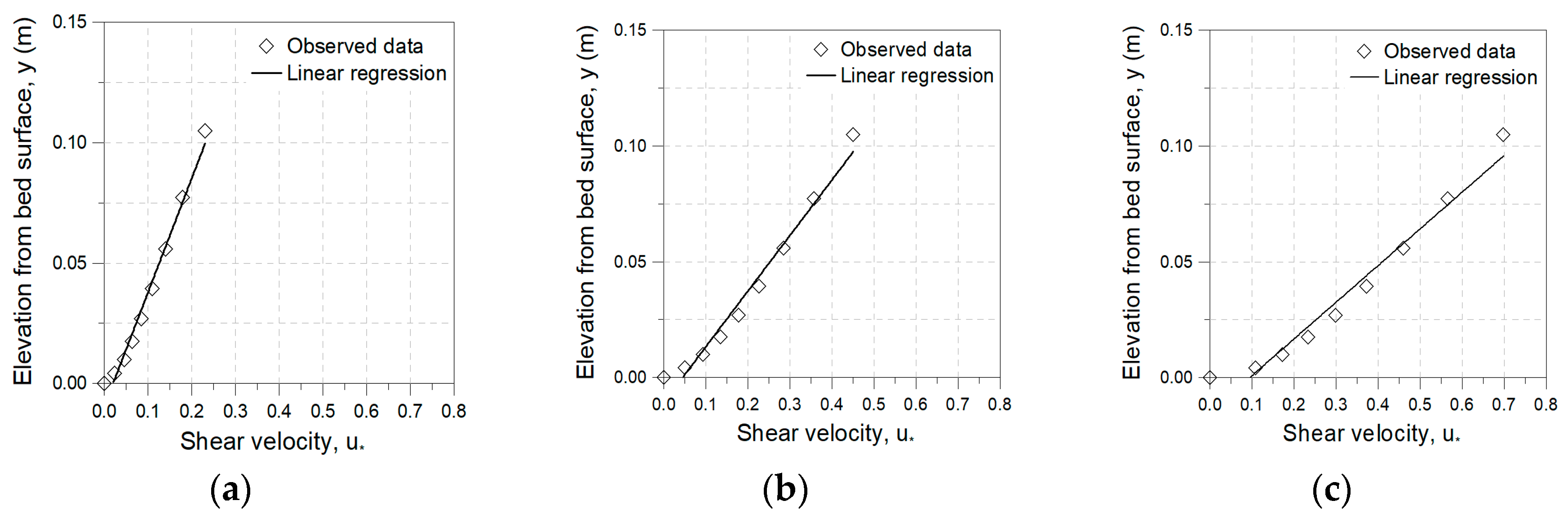
2.2. Analysis Model Validation
2.3. Numerical Analysis Conditions
3. Results
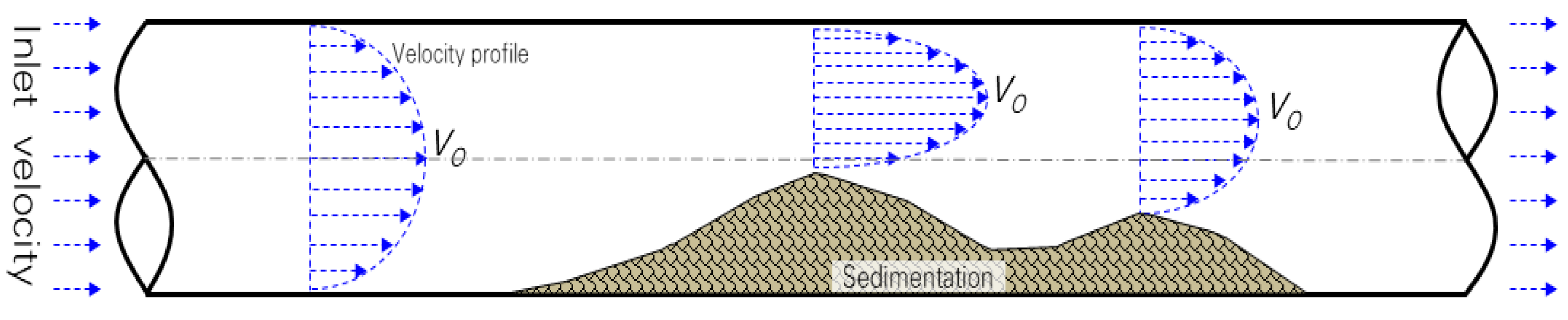
3.1. Flow Characteristics of a Soil Slurry Mixture in the Stormwater Sewer System
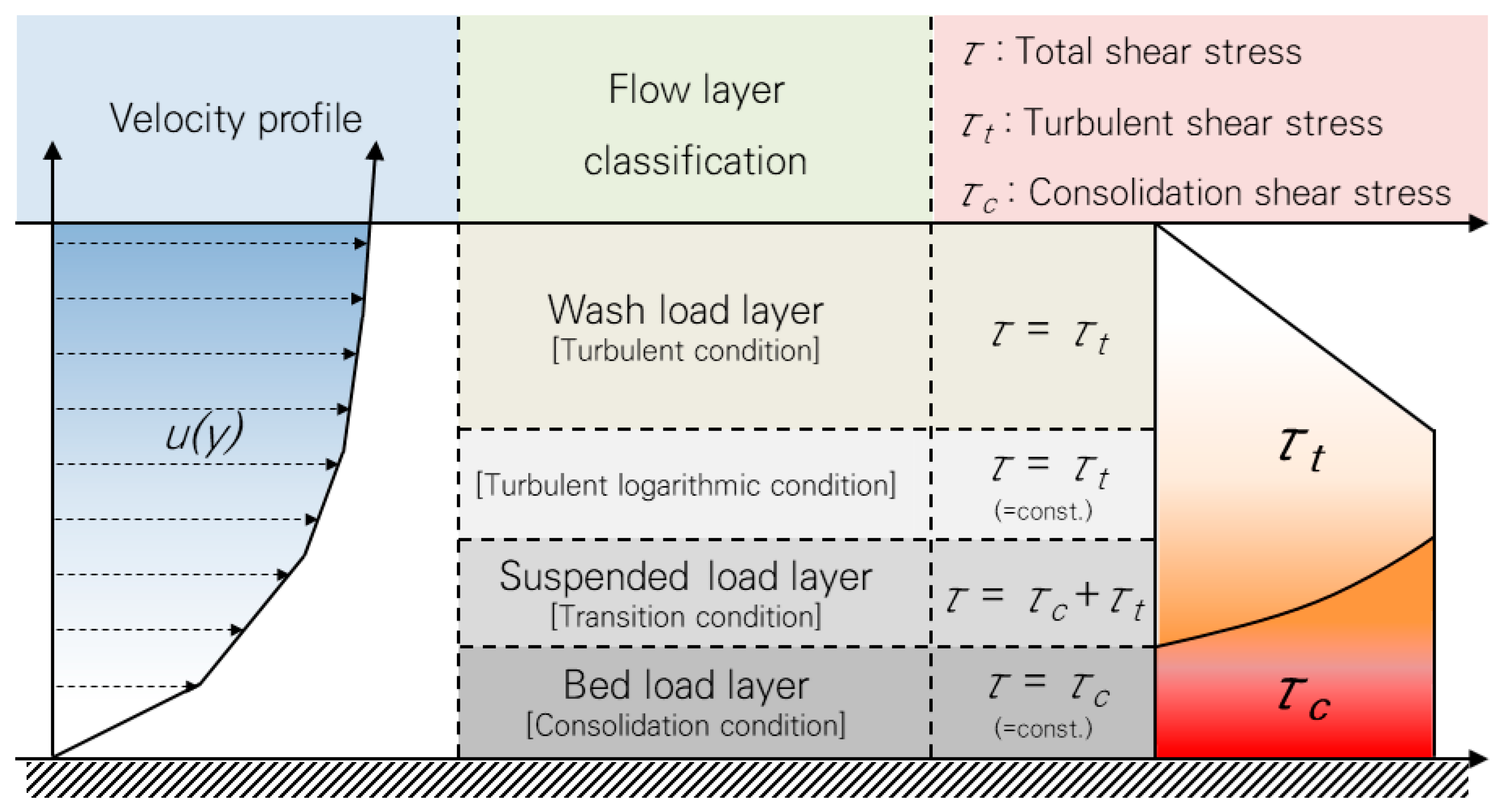
3.2. Analysis of the Flow Boundary Layer in Stormwater Pipes
4. Discussion
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Na, W.; Yoo, C. Optimize short-term rainfall forecast with combination of ensemble precipitation nowcasts by Lagrangian extrapolation. Water 2019, 111, 1752. [Google Scholar] [CrossRef]
- Song, Y.; Park, Y.; Lee, J.; Park, M.; Song, Y. Flood forecasting and warning system structures: Procedure and application to a small urban stream in South Korea. Water 2019, 11, 1571. [Google Scholar] [CrossRef]
- Lee, E.H. Advanced operating technique for centralized and decentralized reservoirs based on flood forecasting to increase system resilience in urban watersheds. Water 2019, 11, 1533. [Google Scholar] [CrossRef]
- Najafzadeh, M.; Rezaie Balf, M.; Rashedi, E. Prediction of maximum scour depth around piers with debris accumulation using EPR, MT, and GEP models. J. Hydroinform. 2016, 18, 867–884. [Google Scholar] [CrossRef]
- Panici, D.; de Almeida, G.A. A theoretical analysis of the fluid–solid interactions governing the removal of woody debris jams from cylindrical bridge piers. J. Fluid Mech. 2020, 886. [Google Scholar] [CrossRef]
- Kang, C.; Chan, D. Modeling of entrainment in debris flow analysis for dry granular material. Int. J. Geomech. 2017, 17, 04017087. [Google Scholar] [CrossRef]
- De Campos, T.M.P.; Galindo, M.S.V. Evaluation of the viscosity of tropical soils for debris flow analysis: A new approach. Géotechnique 2016, 66, 533–545. [Google Scholar] [CrossRef]
- Kwan, J.S.; Sze, E.H.; Lam, C. Finite element analysis for rockfall and debris flow mitigation work. Can. Geotech. J. 2019, 56, 1225–1250. [Google Scholar] [CrossRef]
- Xiong, K.; Adhikari, B.R.; Stamatopoulos, C.A.; Zhan, Y.; Wu, S.; Dong, Z.; Di, B. Comparison of different machine learning methods for debris flow susceptibility mapping: A case study in the Sichuan Province, China. Remote Sens. 2020, 12, 295. [Google Scholar] [CrossRef]
- Regueiro-Picallo, M.; Suárez, J.; Sañudo, E.; Puertas, J.; Anta, J. New insights to study the accumulation and erosion processes of fine-grained organic sediments in combined sewer systems from a laboratory scale model. Sci. Total Environ. 2020, 716, 136923. [Google Scholar] [CrossRef]
- Danandeh Mehr, A.; Safari, M.J.S. Application of soft computing techniques for particle Froude Number estimation in sewer Pipes. J. Pipeline Syst. Eng. Pract. 2020, 11, 04020002. [Google Scholar] [CrossRef]
- Ebtehaj, I.; Bonakdari, H.; Safari, M.J.S.; Gharabaghi, B.; Zaji, A.H.; Madavar, H.R.; Khozani, Z.S.; Es-haghi, M.S.; Shoshegaran, A.; Mehr, A.D. Combination of sensitivity and uncertainty analyses for sediment transport modeling in sewer pipes. Int. J. Sediment. Res. 2020, 35, 157–170. [Google Scholar] [CrossRef]
- Rinas, M.; Fricke, A.; Tränckner, J.; Frischmuth, K.; Koegst, T. Sediment transport in sewage pressure pipes, part II: 1D numerical simulation. Water 2020, 12, 282. [Google Scholar] [CrossRef]
- Zuo, Z.; Zheng, M.; Chang, J.; Ren, D.; Huang, X.; Yuan, Z.; Liu, Y. Free nitrous acid-based suppression of sulfide production in sewer sediments: In-situ effect mechanism. Sci. Total Environ. 2020, 715, 136871. [Google Scholar] [CrossRef] [PubMed]
- Archibong-Eso, A.; Aliyu, A.M.; Yan, W.; Okeke, N.E.; Baba, Y.D.; Fajemidupe, O.; Yeung, H. Experimental study on sand transport characteristics in horizontal and inclined two-phase solid-liquid pipe flow. J. Pipeline Syst. Eng. Pract. 2020, 11, 04019050. [Google Scholar] [CrossRef]
- Xu, Z.; Wu, J.; Li, H.; Chen, Y.; Xu, J.; Xiong, L.; Zhang, J. Characterizing heavy metals in combined sewer overflows and its influence on microbial diversity. Sci. Total Environ. 2018, 625, 1272–1282. [Google Scholar] [CrossRef]
- Ashley, R.M.; Arthur, S.; Coghlan, B.P.; McGregor, I. Fluid sediment in combined sewers. Water Sci. Technol. 1994, 29, 113–123. [Google Scholar] [CrossRef]
- Skipworth, P.J.; Tait, S.J.; Saul, A.J. Erosion of sediment beds in sewers: Model development. J. Environ. Eng. 1999, 125, 566–573. [Google Scholar] [CrossRef]
- Schertzinger, G.; Itzel, F.; Kerstein, J.; Tuerk, J.; Schmidt, T.C.; Sures, B. Accumulation pattern and possible adverse effects of organic pollutants in sediments downstream of combined sewer overflows. Sci. Total Environ. 2019, 675, 295–304. [Google Scholar] [CrossRef]
- Caviedes-Voullième, D.; Morales-Hernández, M.; Juez, C.; Lacasta, A.; García-Navarro, P. Two-dimensional numerical simulation of bed-load transport of a finite-depth sediment layer: Applications to channel flushing. J. Hydraul. Eng. 2017, 143, 04017034. [Google Scholar] [CrossRef]
- Rinas, M.; Tränckner, J.; Koegst, T. Sediment Transport in Sewage Pressure Pipes, Part I: Continuous Determination of Settling and Erosion Characteristics by In-Situ TSS Monitoring Inside a Pressure Pipe in Northern Germany. Water 2019, 11, 2125. [Google Scholar] [CrossRef]
- Einstein, H.A. Formula for the transportation of bed load. Transp. ASCE 1942, 107, 561–573. [Google Scholar]
- Meyer-Peter, E.; Müller, R. Formulas for bed-load transport. In IAHSR 2nd Meeting; IAHR: Stockholm, Sweden, 1948; Appendix 2; pp. 39–64. [Google Scholar]
- Brown, C.B. Sediment transportation. In Engineering Hydraulics; Rouse, H., Ed.; John Wiley & Sons: New York, NY, USA, 1950; pp. 769–857. [Google Scholar]
- Engelund, F.; Hansen, E. A Monograph on Sediment Transport in Alluvial Streams; Technical University of Denmark: Copenhagen, Denmark, 1967. [Google Scholar]
- Ackers, P.; White, W.R. Sediment transport: New approach and analysis. J. Hydraul. Div. 1973, 99, 204–254. [Google Scholar]
- van Rijn, L.C. Principles of Sedimen Transport in Rivers, Estuaries and Coastal Seas; Aqua Publications: Amsterdam, The Netherlands, 1993; Volume 1006. [Google Scholar]
- van Rijn, L.C.; Strypsteen, G. A fully predictive model for aeolian sand transport. Coast. Eng. 2020, 156, 103600. [Google Scholar] [CrossRef]
- van Rijn, L.C. Erodibility of mud-sand bed mixtures. J. Hydraul. Eng. 2020, 146, 04019050. [Google Scholar] [CrossRef]
- Juez, C.; Murillo, J.; García-Navarro, P. Numerical assessment of bed-load discharge formulations for transient flow in 1D and 2D situations. J. Hydroinform. 2013, 15, 1234–1257. [Google Scholar] [CrossRef]
- Song, Y.H.; Yun, R.; Lee, E.H.; Lee, J.H. Predicting sedimentation in urban sewer conduits. Water 2018, 10, 462. [Google Scholar] [CrossRef]
- Song, Y.H.; Lee, E.H.; Lee, J.H. Functional relationship between soil slurry transfer and deposition in urban sewer conduits. Water 2018, 10, 825. [Google Scholar] [CrossRef]
- Launder, B.E.; Spalding, D.B. The numerical computation of turbulent flows. In Numerical Prediction of Flow, Heat Transfer, Turbulence and Combustion; Pergamon Press: New York, NY, USA, 1983; pp. 96–116. [Google Scholar]
- Versteeg, H.K.; Malalasekera, W. An Introduction to Computational Fluid Dynamics: The Finite Volume Method; Pearson Education: London, UK, 2007. [Google Scholar]
- Zhu, X.; Chatain, V.; Gautier, M.; Blanc-Biscarat, D.; Delolme, C.; Dumont, N.; Aubin, J.-B.; Kouyi, G.L. Combination of Lagrangian Discrete Phase Model and sediment physico-chemical characteristics for the prediction of the distribution of trace metal contamination in a stormwater detention basin. Sci. Total Environ. 2020, 698, 134263. [Google Scholar] [CrossRef]
- Baalisampang, T.; Abbassi, R.; Garaniya, V.; Khan, F.; Dadashzadeh, M. Fire impact assessment in FLNG processing facilities using Computational Fluid Dynamics (CFD). Fire Saf. J. 2017, 92, 42–52. [Google Scholar] [CrossRef]
- Campisano, A.; Modica, C.; Creaco, E.; Shahsavari, G. A model for non-uniform sediment transport induced by flushing in sewer channels. Water Res. 2019, 163, 114903. [Google Scholar] [CrossRef] [PubMed]
- Heinl, E.; Bohnet, M. Calculation of particle–wall adhesion in horizontal gas–solids flow using CFD. Powder Technol. 2015, 159, 95–104. [Google Scholar] [CrossRef]
- Kaushal, D.R.; Thinglas, T.; Tomita, Y.; Kuchii, S.; Tsukamoto, H. CFD modeling for pipeline flow of fine particles at high concentration. Int. J. Multiph. Flow 2012, 43, 85–100. [Google Scholar] [CrossRef]
- ANSYS. ANSYS Fluent 12.1 Theory Guide; ANSYS Inc.: Canonsburg, PA, USA, 2010. [Google Scholar]
- Park, C.W.; Hong, C.H. User Guide of ANSYS Workbench; Intervision: Seoul, Korea, 2008. [Google Scholar]
- Everitt, B.S.; Hand, D. Finite Mixture Distributions; Wiley & Sons, Inc.: London, UK, 1985. [Google Scholar]
- Nabil, T.; EL-Sawaf, I.; EL-Nahhas, K. Sand-water slurry flow modelling in a horizontal pipeline by computational fluid dynamics technique. Int. Water Technol. J. 2014, 4, 1–17. [Google Scholar]
- Ota, J.J.; Perrusquia, G. Particle velocity and sediment transport at limit deposition in sewers. In Proceedings of the 12th International Conference on Urban Drainage, Porto Alegre/RS, Brazil, 11–16 September 2011; pp. 1–8. [Google Scholar]
- Kim, C.H.; Han, C.H. Numerical simulation of hydraulic transport of soil–water mixtures in pipelines. Open J. Fluid Dyn. 2013, 3, 266–270. [Google Scholar] [CrossRef]
- Anjum, N.; Ghani, U.; Ahmed Pasha, G.; Latif, A.; Sultan, T.; Ali, S. To investigate the flow structure of discontinuous vegetation patches of two vertically different layers in an open channel. Water 2018, 10, 75. [Google Scholar] [CrossRef]
- Török, G.T.; Baranya, S.; Rüther, N. 3D CFD modeling of local scouring, bed armoring and sediment deposition. Water 2017, 9, 56. [Google Scholar] [CrossRef]
- Fan, F.; Liang, B.; Li, Y.; Bai, Y.; Zhu, Y.; Zhu, Z. Numerical investigation of the influence of water jumping on the local scour beneath a pipeline under steady flow. Water 2017, 9, 642. [Google Scholar] [CrossRef]
- Hanjalić, K.; Launder, B.E. A Reynolds stress model of turbulence and its application to thin shear flows. J. Fluid Mech. 1972, 52, 609–638. [Google Scholar] [CrossRef]
- Matousek, V. Flow Mechanism of Soil–Water Mixtures in Pipelines. Ph.D. Thesis, Delft University, Delft, The Netherlands, 15 December 1997. [Google Scholar]
- Liu, Z. Sediment Transport; Aalborg Universitet: Aalborg, Denmark, 2001. [Google Scholar]
- Butler, D.; May, R.; Ackers, J. Self-cleansing sewer design based on sediment transport principles. J. Hydraul. Eng. 2004, 130, 723. [Google Scholar] [CrossRef]
- Raudkivi, A.J. Loose Boundary Hydraulics; CRC Press: Boca Raton, FL, USA, 1998. [Google Scholar]
- Nezu, I. Experimental Study on Secondary Currents in Open Channel Flows. In Proceedings of the 21st IAHR Congress, Melbourne, Australia, 13–18 August 1985. [Google Scholar]
- Nezu, I.; Nakagawa, H.; Jirka, G.H. Turbulence in open-channel flows. J. Hydraul. Eng. 1994, 120, 1235–1237. [Google Scholar] [CrossRef]
- Martinuzzi, R.; Pollard, A. Comparative study of turbulence models in predicting turbulent pipe flow. I-Algebraic stress and k-epsilon models. AIAA J. 1989, 27, 29–36. [Google Scholar] [CrossRef]
- Pollard, A.; Martinuzzi, R. Comparative study of turbulence models in predicting turbulent pipe flow. II-Reynolds stress and k-epsilon models. AIAA J. 1989, 27, 1714–1721. [Google Scholar] [CrossRef]
- Wilcox, D.C. Turbulence Modeling for CFD; DCW Industries: La Canada, CA, USA, 1998; Volume 2, pp. 172–180. [Google Scholar]
- Bjørlykke, K.; Middleton, G.V. Compaction (consolidation) of sediments. In Encyclopedia of Sediments and Sedimentary Rocks; Kluwer Academic Publishers: Dordrecht, The Netherlands, 2003; pp. 161–168. [Google Scholar]
- Gisselbrecht, M.; Plaut, E. High Reynolds number K-epsilon model of turbulent pipe flows with standard wall laws: First quantitative results. In 22ème Congrès Français de Mécanique 2015; HAL: Lyon, France, 2015. [Google Scholar]
- Singh, K.P.; Kumar, A.; Kaushal, D.R. CFD modelling and experimental investigation of bimodal slurry flow in horizontal pipeline and bends. In Advances in Fluid and Thermal Engineering; Springer: Singapore, 2019; pp. 337–345. [Google Scholar]
- Zhiyao, S.; Tingting, W.; Fumin, X.; Ruijie, L. A simple formula for predicting settling velocity of sediment particles. Water Sci. Eng. 2008, 1, 37–43. [Google Scholar] [CrossRef]
- Guo, S.J.; Zhang, F.H.; Song, X.G.; Wang, B.T. Deposited sediment settlement and consolidation mechanisms. Water Sci. Eng. 2015, 8, 335–344. [Google Scholar] [CrossRef]


| Classification | Fluid-Soil Interaction Modelling in Pipe |
|---|---|
| Pipe specification | 0.6 m (D) × 10 m (L) |
| Mesh specification | Generate 140,000 grid |
| Applied models and flow condition | Euler-Euler Model Standard k-ε model Turbulent flow |
| Pipe inlet condition | Inlet velocity condition (m/s): 1.0, 2.0, 3.0 Inlet volume fraction condition (%): 10, 30, 50 |
| Fluid property | Density (kg/m3): 998.2 Kinematic viscosity: 0.001003 Pa·s |
| Soil slurry property | Density (kg/m3): 2650 Soil particle diameter (mm): 0.5, 1.0, 3.0, 5.0, 7.0, 15.0, 20.0 |
| Condition | Sediment Transport Status |
|---|---|
| ∎ Particle travel in suspension | |
| ∎ Particle travel in saltation | |
| ∎ Particle travel as bedload |
| Soil Diameter (), mm | ||
|---|---|---|
| 0.5 | 0.0111 | 0.1148 |
| 1.0 | 0.0223 | 0.1623 |
| 3.0 | 0.0669 | 0.2811 |
| 5.0 | 0.1115 | 0.3629 |
| 7.0 | 0.1561 | 0.4294 |
| 15.0 | 0.3344 | 0.6286 |
| 20.0 | 0.4459 | 0.7259 |
| 1.0 m/s | 2.0 m/s | 3.0 m/s | |||||||
|---|---|---|---|---|---|---|---|---|---|
| 10% v/f | 30% v/f | 50% v/f | 10% v/f | 30% v/f | 50% v/f | 10% v/f | 30% v/f | 50% v/f | |
| 0.5 | 0.016 | 0.022 | 0.021 | 0.017 | 0.018 | 0.018 | 0.048 | 0.050 | 0.050 |
| 1.0 | 0.024 | 0.024 | 0.022 | 0.035 | 0.109 | 0.033 | 0.060 | 0.065 | 0.063 |
| 3.0 | 0.054 | 0.042 | 0.045 | 0.040 | 0.038 | 0.037 | 0.065 | 0.069 | 0.070 |
| 5.0 | 0.061 | 0.064 | 0.067 | 0.067 | 0.051 | 0.067 | 0.047 | 0.054 | 0.053 |
| 7.0 | 0.073 | 0.073 | 0.064 | 0.071 | 0.073 | 0.068 | 0.063 | 0.079 | 0.080 |
| 15.0 | 0.112 | 0.112 | 0.121 | 0.096 | 0.105 | 0.108 | 0.101 | 0.101 | 0.111 |
| 20.0 | 0.125 | 0.139 | 0.132 | 0.131 | 0.125 | 0.129 | 0.126 | 0.133 | 0.135 |
| Velocity v/f Load | 1.0 m/s Condition | 2.0 m/s Condition | 3.0 m/s Condition | |||||||
|---|---|---|---|---|---|---|---|---|---|---|
| 10% | 30% | 50% | 10% | 30% | 50% | 10% | 30% | 50% | ||
| 0.5 mm | Bed | 1.141 | 1.321 | 1.227 | 0.916 | 0.857 | 0.841 | 0.809 | 0.767 | 0.761 |
| Suspended | 0.120 | 0.106 | 0.109 | 0.154 | 0.154 | 0.169 | 0.193 | 0.205 | 0.203 | |
| Wash | 0.048 | 0.047 | 0.044 | 0.043 | 0.043 | 0.044 | 0.042 | 0.042 | 0.042 | |
| 1.0 mm | Bed | 1.219 | 1.219 | 1.171 | 1.023 | 1.029 | 1.034 | 0.906 | 0.872 | 0.879 |
| Suspended | 0.122 | 0.122 | 0.115 | 0.100 | 0.098 | 0.099 | 0.184 | 0.185 | 0.186 | |
| Wash | 0.049 | 0.049 | 0.053 | 0.046 | 0.049 | 0.044 | 0.047 | 0.042 | 0.042 | |
| 3.0 mm | Bed | 1.272 | 1.314 | 1.277 | 1.054 | 1.073 | 1.072 | 1.004 | 1.049 | 1.035 |
| Suspended | 0.137 | 0.149 | 0.164 | 0.121 | 0.118 | 0.117 | 0.101 | 0.100 | 0.101 | |
| Wash | 0.043 | 0.043 | 0.040 | 0.046 | 0.046 | 0.050 | 0.046 | 0.046 | 0.046 | |
| 5.0 mm | Bed | 1.199 | 1.055 | 1.202 | 0.985 | 0.978 | 0.894 | 0.907 | 1.086 | 0.988 |
| Suspended | 0.139 | 0.152 | 0.177 | 0.122 | 0.120 | 0.117 | 0.112 | 0.113 | 0.113 | |
| Wash | 0.036 | 0.030 | 0.029 | 0.046 | 0.047 | 0.052 | 0.046 | 0.047 | 0.046 | |
| 7.0 mm | Bed | 0.776 | 1.274 | 1.192 | 1.048 | 0.978 | 0.904 | 0.865 | 1.021 | 0.780 |
| Suspended | 0.114 | 0.127 | 0.126 | 0.115 | 0.127 | 0.125 | 0.116 | 0.119 | 0.115 | |
| Wash | 0.032 | 0.032 | 0.032 | 0.042 | 0.043 | 0.042 | 0.048 | 0.045 | 0.049 | |
| 15.0 mm | Bed | 0.705 | 0.617 | 0.614 | 0.682 | 0.688 | 0.664 | 0.650 | 0.704 | 0.731 |
| Suspended | 0.109 | 0.118 | 0.116 | 0.121 | 0.129 | 0.130 | 0.117 | 0.118 | 0.127 | |
| Wash | 0.026 | 0.033 | 0.032 | 0.038 | 0.042 | 0.042 | 0.043 | 0.044 | 0.044 | |
| 20.0 mm | Bed | 0.662 | 0.603 | 0.616 | 0.479 | 0.520 | 0.487 | 0.592 | 0.578 | 0.609 |
| Suspended | 0.115 | 0.105 | 0.110 | 0.122 | 0.121 | 0.141 | 0.115 | 0.111 | 0.121 | |
| Wash | 0.023 | 0.033 | 0.036 | 0.039 | 0.039 | 0.047 | 0.042 | 0.047 | 0.047 | |
| Condition | Sediment Transport Status |
|---|---|
| ∎ Particles travel in wash load. | |
| ∎ Particles travel in suspension. ∎ Particles dispersed within the flow travel in suspended load. | |
| ∎ Particles travel in saltation. ∎ Partial transport on the pipe bed or sediment boundary layer. | |
| ∎ Particles travel as bed load. ∎ Adhesion and consolidation of particles deposited on the bottom. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Song, Y.H.; Joo, J.G.; Lee, J.H.; Yoo, D.G. Numerical Assessment of Shear Boundary Layer Formation in Sewer Systems with Fluid-Sediment Phases. Water 2020, 12, 1332. https://doi.org/10.3390/w12051332
Song YH, Joo JG, Lee JH, Yoo DG. Numerical Assessment of Shear Boundary Layer Formation in Sewer Systems with Fluid-Sediment Phases. Water. 2020; 12(5):1332. https://doi.org/10.3390/w12051332
Chicago/Turabian StyleSong, Yang Ho, Jin Gul Joo, Jung Ho Lee, and Do Guen Yoo. 2020. "Numerical Assessment of Shear Boundary Layer Formation in Sewer Systems with Fluid-Sediment Phases" Water 12, no. 5: 1332. https://doi.org/10.3390/w12051332
APA StyleSong, Y. H., Joo, J. G., Lee, J. H., & Yoo, D. G. (2020). Numerical Assessment of Shear Boundary Layer Formation in Sewer Systems with Fluid-Sediment Phases. Water, 12(5), 1332. https://doi.org/10.3390/w12051332