Velocity Fields at Horizontal Bar Racks as Fish Guidance Structures
Abstract
1. Introduction
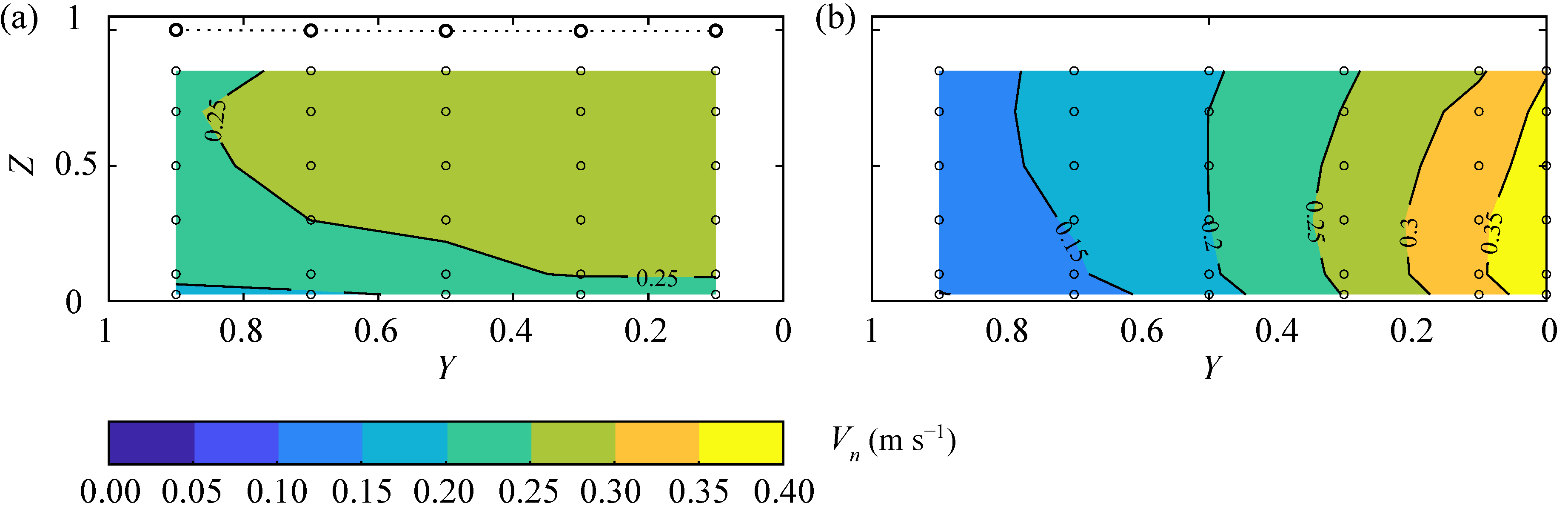
- Turnpenny and O’Keeffe [4] suggest designing fish guidance structures (FGSs) such that Vn does not exceed the 90th percentile of the maximum sustained swimming speed, related to the smallest fish and the lowest water temperatures during the migration period. Consequently, 90% of the downstream migrating fish should be able to swim against Vn for up to 200 min, without getting impinged at the rack. For salmonids with a total length of TL > 10 cm, Turnpenny and O’Keeffe [4] recommended Vn ≤ 0.75 m s−1, whereas Vn ≤ 0.22 m s−1 for cyprinids (except breams) with TL > 5 cm. They suggest designing racks with an over-capacity of at least 20%, since partial clogging eventually increases Vn during operation. Based on literature data, Ebel [1] proposed empirical equations to estimate the maximum sustained swimming speed of rheophile and non-rheophile fish species (Table 1). For weak swimmers such as eels, sturgeons, and lampreys, species-specific equations are recommended.
- For Vp > Vn (VpVn−1 > 1), it is assumed that fish follow the main flow direction and are therefore guided towards the downstream rack end, instead of getting impinged at the rack [5,6]. The ratio VpVn−1 is introduced as the fish guidance capacity (FGC). When comparing velocity fields at FGSs from literature, distinction should be made between sectional models, where only a section of the rack is investigated, and physical models where the bypass and/or the weir fields are also modelled in addition to the FGS. In the following, different laboratory studies, focusing on the velocity fields of FGSs, are presented. They are summarized in Table 2. Maager [7] and Albayrak et al. [8] experimentally investigated HBRs in a sectional 1:2 Froude-scaled physical model. The parameter matrix contained two bar shapes (rectangular and one-side rounded bars), two approach flow angles (α = 30° and 45°), three blocking ratios (BR = 0.33, 0.39, 0.55), and various overlay configurations. Maager [7] found that the blocking ratio in the range of BR = 0.39–0.55 was hardly affecting the velocity field. Szabo-Meszaros et al. [9] investigated HBRs with α = 30°, sb = 15 mm, and a bar thickness of tb = 8 mm with rectangular and hydrodynamic bars. Neglecting the effect of the vertical tie-bars, the blockage ratio was BR ≈ tb (sb + tb)−1 = 0.35. The model included a bypass with a relative width of 10% of the model flume and a fixed, 30°-angled bypass-ramp. The relative bypass discharge was QbyQo−1 = 4.0% for rectangular and QbyQo−1 = 3.1% for hydrodynamic bars, where Qby and Qo denote the bypass and total approach flow discharges, respectively. However, the authors did not find any significant differences in the velocity patterns between the bar shapes. Berger [10] conducted a laboratory study on HBRs with rectangular bars. A lateral opening next to the rack with a relative width of 12.5% represented a bypass. She focused on live-fish tests and measured flow fields for different approach flow velocities. According to her results, HBRs without overlays had no effect on the vertical velocity profile. The transversal velocity components upstream of the rack were small, and the flow slightly accelerated along the rack. De Bie et al. [11] investigated racks with α = 30° made of horizontally and vertically aligned wedge-wire bars (sb = 6 mm, tb = 3 mm, BR ≈ 0.33 without accounting for the vertical tie-bars) in a laboratory flume for two different discharges. The bypass was unregulated and extended over 10% of the channel width. However, the bypass discharge was not quantified. The authors found that the streamwise velocity component at racks with horizontal bars was continuously increasing from the rack head to the downstream rack end (measurements 5 cm above bottom; U ≈ 0.14 m s−1 to U ≈ 0.21 m s−1 for an average approach flow velocity from continuity of Uo = 0.17 m s−1 and U ≈ 0.32 m s−1 to U ≈ 0.47 m s−1 for Uo = 0.40 m s−1). Raynal et al. [12] studied a sectional model of vertically inclined racks with inclination angles to the horizontal channel bed ranging from γ = 15° to γ = 90° (angle definition in [13]). Cylindrical spacers were used to realize different bar spacings. They reported locally reduced flow velocities downstream of the horizontal tie-bars/spacers. This effect was most pronounced for the most downstream tie-bar/spacer and for shorter racks (large inclination angles). A number of recent laboratory studies involved angled racks with vertical bars with larger bar spacing (e.g., [14,15,16,17]). These racks are theoretically permeable for most fish, depending on their dimensions, but generate hydraulic cues, which guide fish to the bypass. These mechanical behavioral barriers include: (A) Louvers with the bars aligned orthogonally to the main flow [14], (B) angled bar racks (ABRs) with a 90° angle between the individual vertical bars and the rack axis [15,18], and (C) modified bar racks (MBRs), for which the bar angle is independent of the rack angle [16,17,19]. These FGSs induce strong flow deflections, leading to large velocity accelerations along the rack and therefore asymmetric turbine approach flows [19]. Beck et al. [20,21] developed curved-bar racks (CBRs), which significantly reduced velocity gradients along the rack and create quasi-symmetric turbine approach flow due to the straightening effect of the curved bars. Table 2 summarizes the studies describe above. The total relative overlay height HOv is defined in Section 2.2.
2. Experimentation
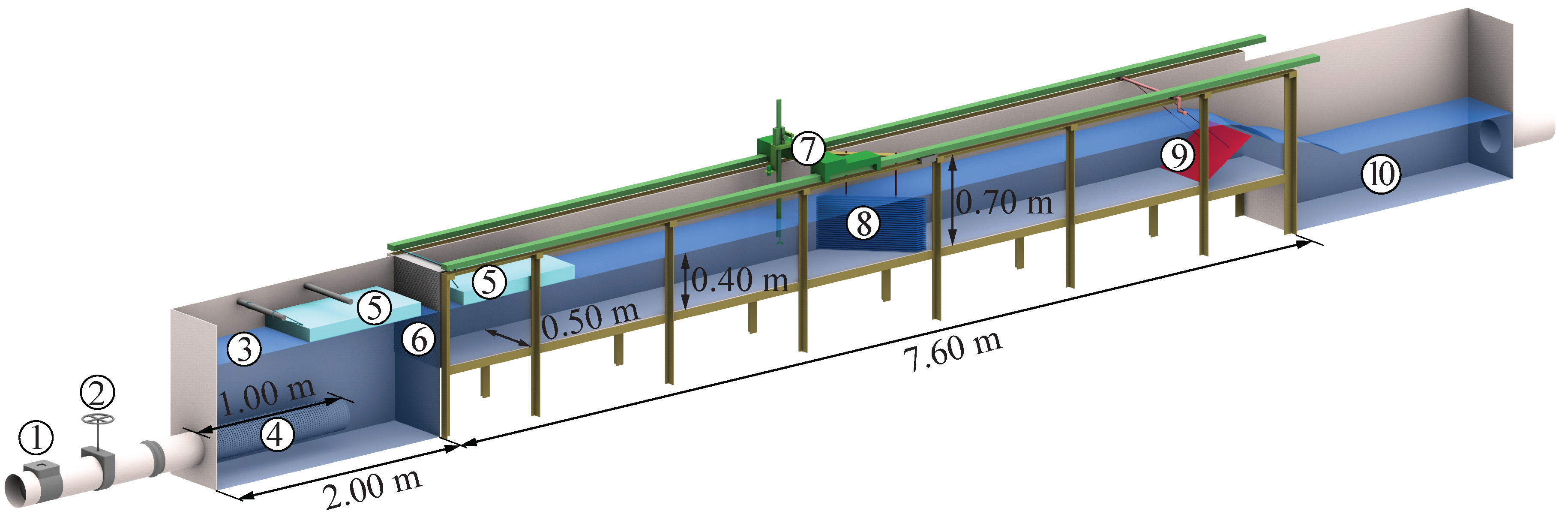
2.1. Test Setup
2.2. Parameter Range and Test Program
2.3. Experimental Procedure
3. Results
3.1. Parameter Effect on the Velocity Fields
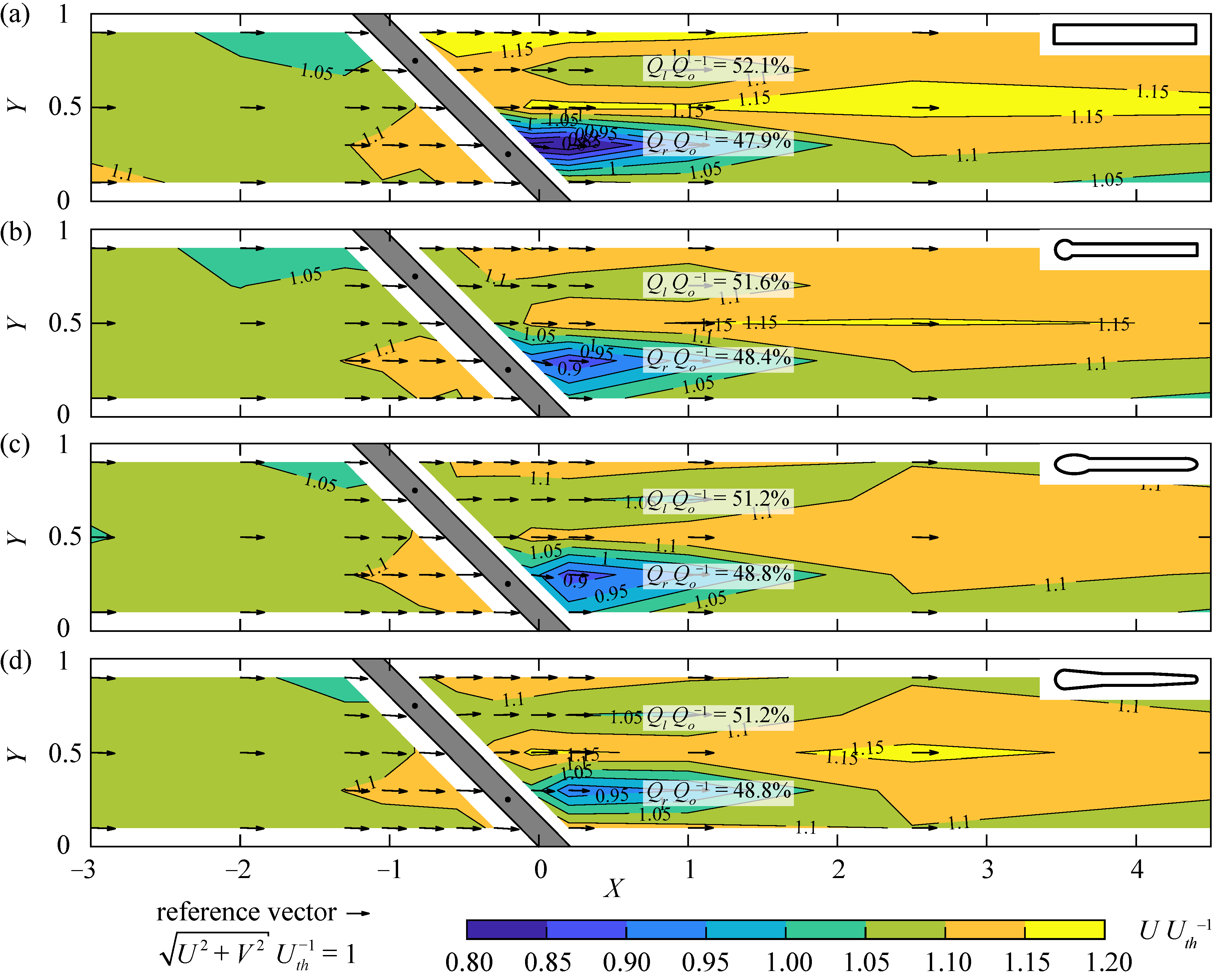
3.1.1. Bar Shape
3.1.2. Rack Angle
3.1.3. Bar Spacing
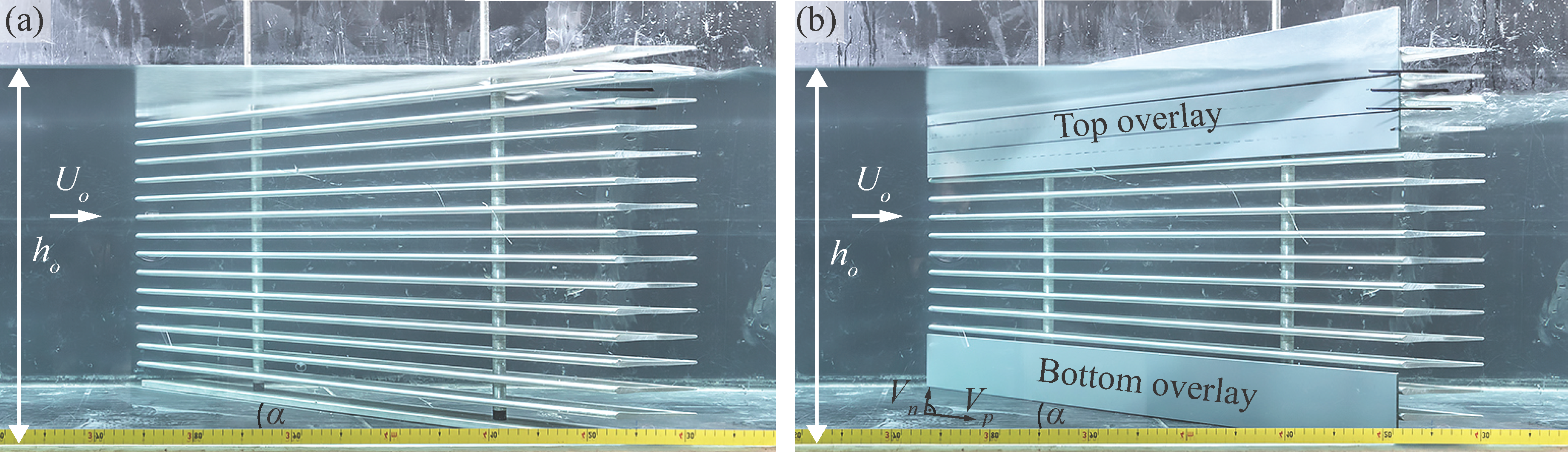
3.1.4. Overlays
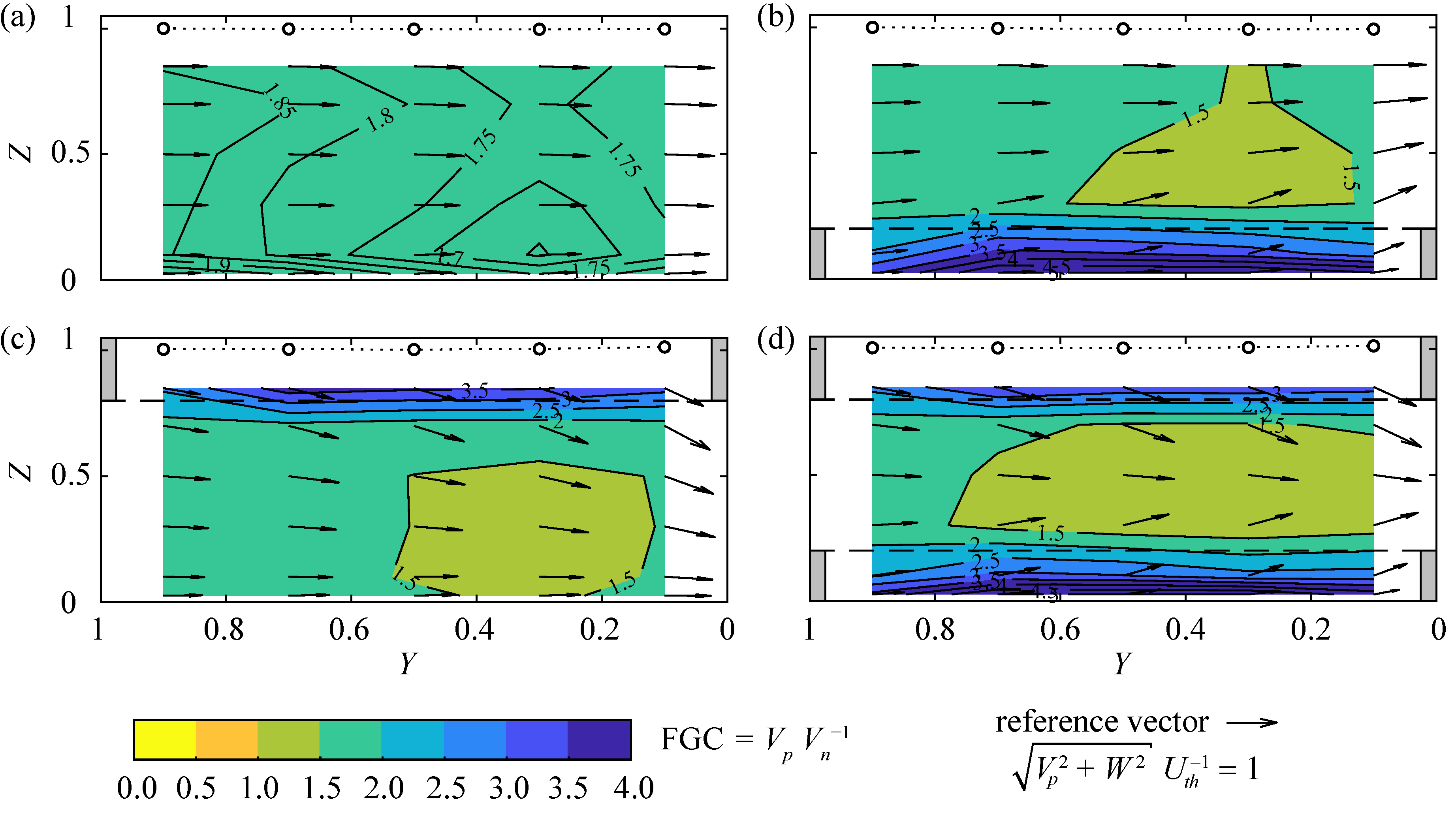
3.1.5. Fish Guidance Capacity
3.1.6. Turbine Admission Flow
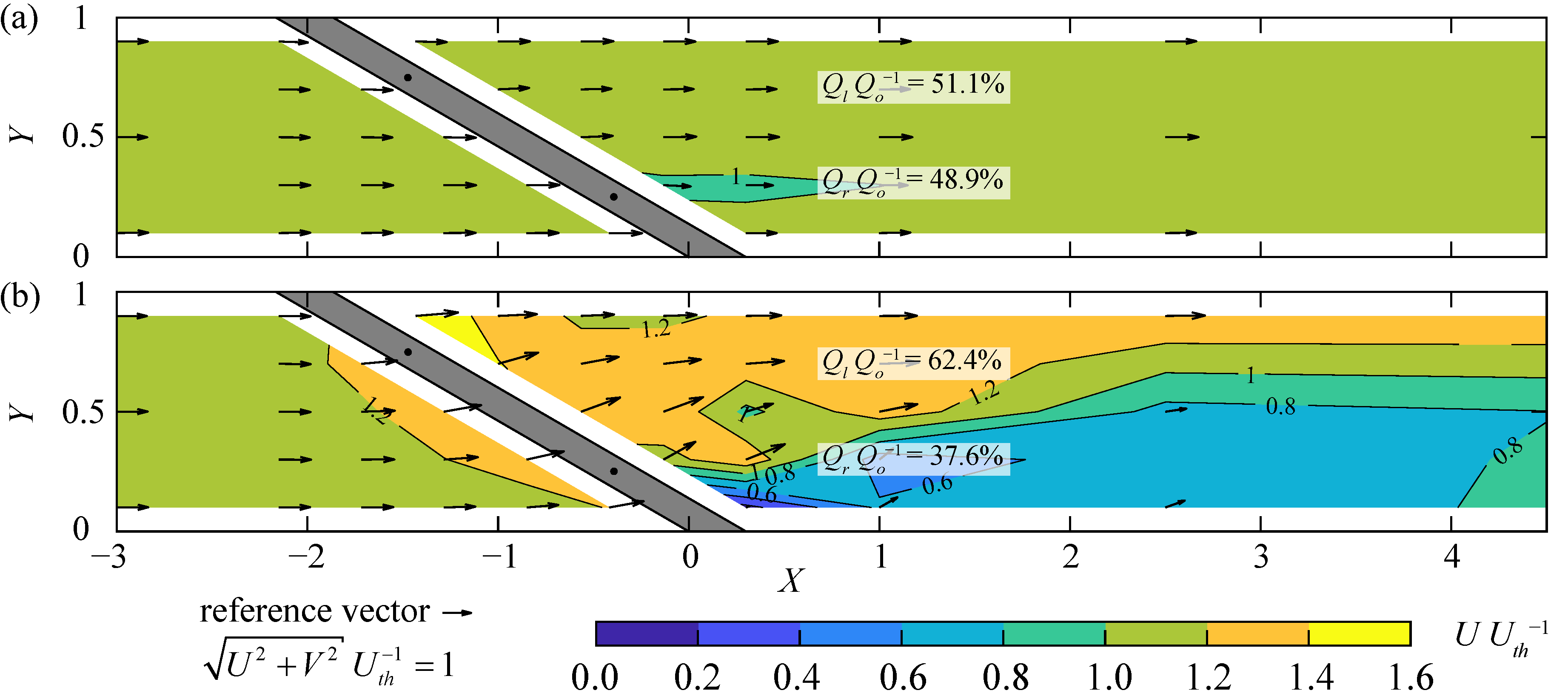
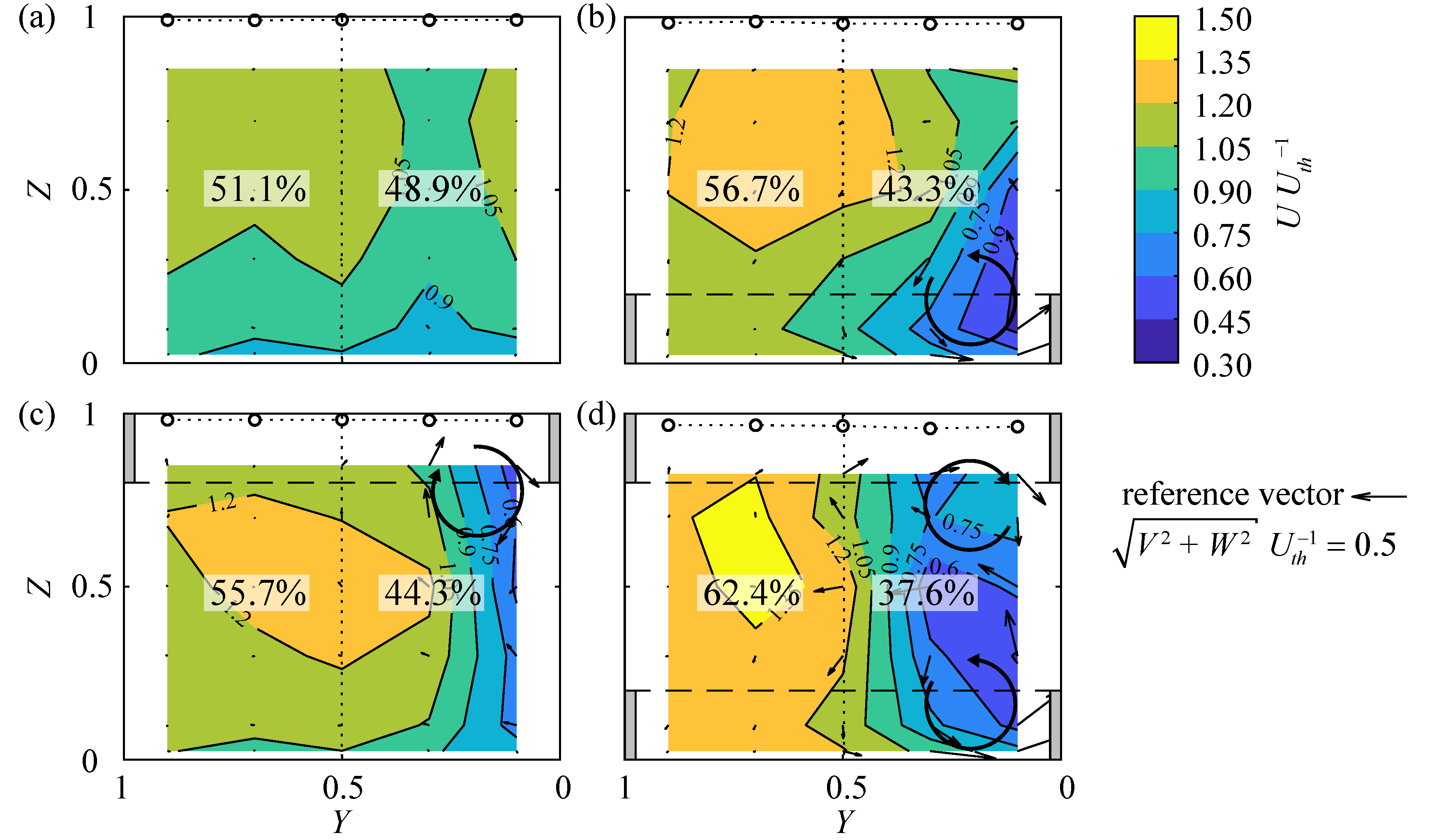
3.1.7. Effect of the HPP Layout on the Flow Field
4. Discussion
4.1. Comparison with Literature
4.2. Vertical Tie-Bars
4.3. Engineering Application Considerations
5. Conclusions and Outlook
- The bar shape, approach flow angle α, and the clear bar spacing sb have a minor effect on the velocity field. The discharge difference between the left and right channel halves downstream of the horizontal bar rack is smaller than 4.2% for all configurations without overlays. The effect of horizontal bar racks without overlays on the velocity field is largest for rectangular bars, large approach flow angles α, and small clear bar spacing sb.
- Bottom and top overlays at horizontal bar racks have a governing effect on the velocity fields upstream and downstream of the rack. The discharge difference between the left and right channel halves downstream of the horizontal bar rack is up to 24.8%. Overlays potentially improve guidance of fish and floating debris, but they can negatively affect the turbine admission flow and thus reduce the turbine efficiency. This negative effect is particularly important if the rack is located close to the turbines.
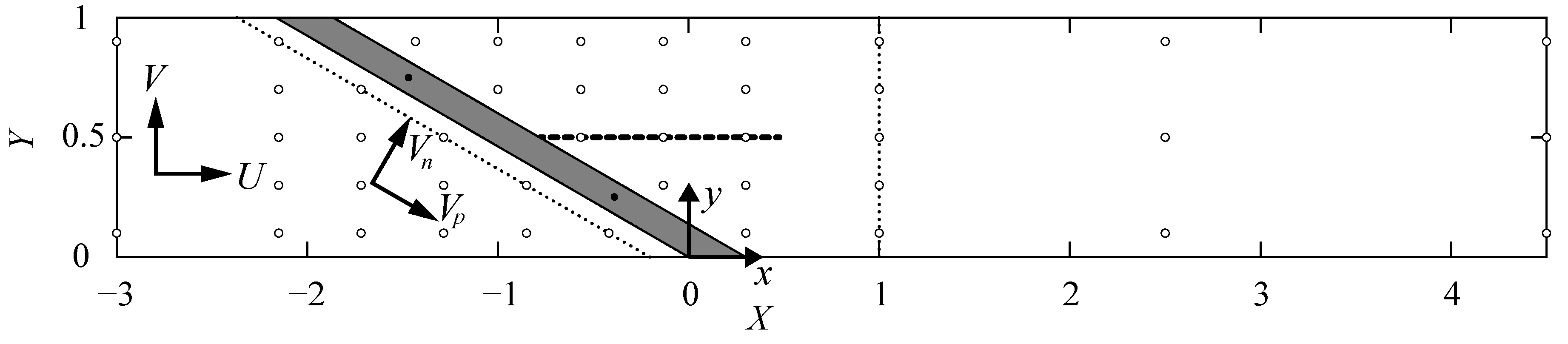
- For unclogged horizontal bar racks without overlays at a diversion hydropower plant, the ratio of the parallel to the normal flow velocity component (VpVn−1) mainly depends on the horizontal approach flow angle α and can be calculated in good approximation as a cross-sectional average to VpVn−1 = tan(α)−1. For a horizontal approach flow angle of α = 30° this ratio is VpVn−1 = 1.73, which is considered favorable for fish guidance.
- The hydropower plant layout strongly affects the approach flow field to horizontal bar racks. For a block-type hydropower plant with an abrupt contraction and without weir discharge, the ratio VpVn−1 continuously decreases from 1.8 at the rack head to 0 at the bypass inlet, resulting in unfavorable flow conditions for fish guidance.
- To optimize the fish guidance at horizontal bar racks at block-type hydropower plants, the installation of a dividing pier or a partial spillway operation are suitable measures. To maintain symmetrical turbine approach flow, the installation of a pier (block-type) or flow-straightening walls downstream of the rack (diversion HPP) are effective measures.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Abbreviations
| ABR | Angled bar rack |
| ADV | Acoustic Doppler velocimeter |
| CBR | Curved-bar rack |
| FGC | Fish guidance capacity, FGC = VpVn−1 |
| FGS | Fish guidance structure |
| HBR | Horizontal bar rack |
| HPP | Hydropower plant |
| MBR | Modified bar rack |
| MID | Magnetic-inductive flow meter |
| UDS | Ultrasonic distance sensor |
Notation
| BR | total blocking ratio (−) |
| db | bar depth (m) |
| F | Froude number (−), F = Uo(gho)−0.5 |
| g | gravity acceleration constant (m s−2), g = 9.81 m s−2 |
| hBo | bottom overlay height (m) |
| HBo | relative bottom overlay height (−), HBo = hBoho−1 |
| hds | downstream flow depth (m) |
| hTo | top overlay height (m) |
| HTo | relative top overlay height (−), HTo = hToho−1 |
| ho | approach flow depth (m) |
| HOv | total relative overlay height (−), HOv = HBo + HTo |
| lR | rack length (m), lR = wds sin(α)−1 |
| Qby | bypass discharge (m3 s−1) |
| Qd | design discharge (m3 s−1) |
| Ql | discharge through the left channel half (m3 s−1) |
| Qo | total approach flow discharge (m3 s−1), Uo = Qoho−1wo−1 |
| Qr | discharge through the right channel half (m3 s−1) |
| Qt | turbine discharge (m3 s−1) |
| Qw | weir discharge (m3 s−1) |
| R | Reynolds number based on hydraulic radius (−), R = 4RhUoν−1 |
| Rb | bar Reynolds number (−), Rb = tbUoν−1 |
| Rh | hydraulic radius (m), Rh = hwch(2h+wch) −1 |
| sb | clear bar spacing (m) |
| tb | bar thickness at thickest point (m) |
| TL | total fish length (m) |
| U,V,W | time-averaged velocities in streamwise, transversal, and vertical direction (m s−1) |
| Uds | mean downstream flow velocity (m s−1) |
| Uth | theoretical average flow velocity (m s−1) |
| Uo | mean upstream approach flow velocity from continuity (m s−1) |
| Vn | velocity component normal to the rack (m s−1) |
| Vp | velocity component parallel to the rack (m s−1) |
| wch | constant channel width (diversion HPP) (m) |
| wds | downstream channel width (m) |
| wo | upstream channel width (m) |
| x,y,z | coordinates in streamwise, transversal, and vertical direction (m) |
| X | normalized streamwise coordinate (−), X = xho−1 |
| Y | normalized transversal coordinate (−), Y = ywds−1 |
| Z | normalized vertical coordinate (−), Z = zho−1 |
| α | horizontal approach flow angle (°) |
| γ | rack inclination angle (°) |
| ∆hR | rack head loss (m) |
| ΔQ | discharge difference between left and right channel halves, ΔQ = |Ql − Qr| (m3 s−1) |
| ν | kinematic viscosity (m2 s−1) |
| ξc | measured contraction head loss coefficient (−) |
| ξR | measured rack head loss coefficient (−) |
References
- Ebel, G. Fischschutz und Fischabstieg an Wasserkraftanlagen—Handbuch Rechen- und Bypasssysteme. Ingenieurbiologische Grundlagen, Modellierung und Prognose, Bemessung und Gestaltung, 2nd ed.; Büro für Gewässerökologie und Fischereibiologie Dr. Ebel: Halle, Germany, 2016. [Google Scholar]
- Larinier, M.; Travade, F. Downstream migration: Problems and facilities. Bull. Fr. Pêche Piscic. 2002, 364, 181–202. [Google Scholar] [CrossRef]
- Gruber, R. Traun-Kraftwerk Danzermühl nimmt planmässig den Betrieb auf (The hydropower plant Danzermühl at Traun River starts operation on schedule). zekHydro 2019, 17, 22–27. [Google Scholar]
- Turnpenny, A.W.H.; O’Keeffe, N. Screening for Intake and Outfalls: A Best Practice Guide; Environment Agency: Bristol, UK, 2005.
- Courret, D.; Larinier, M. Guide pour la conception de prises d’eau "ichtyo-compatibles" pour les petites centrales hydroélectriques [Design guidelines of fish-friendly water intakes for small hydropower plants]; Agence de L’Environnement et de la Maîtrise de l’Energie (ADEME): Clermont-Ferrand, France, 2008. [Google Scholar]
- NMFS. Fish Screening Criteria for Anadromous Salmonids; National Marine Fisheries Service Southwest Region (NMFS): Santa Rosa, CA, USA, 1997.
- Maager, F. Fischleitrechen mit Horizontalen Stabelementen (Fish Guidance Structures with Horizontal Bars). Master’s Thesis, ETH Zurich, Zurich, Switzerland, 2016. [Google Scholar]
- Albayrak, I.; Maager, F.; Boes, R.M. An experimental investigation on fish guidance structures with horizontal bars. J. Hydraul. Res. 2019. [Google Scholar] [CrossRef]
- Szabo-Meszaros, M.; Navaratnam, C.U.; Aberle, J.; Silva, A.T.; Forseth, T.; Calles, O.; Fjeldstad, H.-P.; Alfredsen, K. Experimental hydraulics on fish-friendly trash-racks: an ecological approach. Ecol. Eng. 2018, 113, 11–20. [Google Scholar] [CrossRef]
- Berger, C. Rechenverluste und Auslegung von (elektrifizierten) Schrägrechen anhand ethohydraulischer Studien (Screen Losses and Design of Inclined (and Electrified) Screens with Horizontal Bars on the Basis of Ethohydraulic Studies). Ph.D. Thesis, Technische Universität, Darmstadt, Germany, 2018. [Google Scholar]
- de Bie, J.; Peirson, G.; Kemp, P.S. Effectiveness of horizontally and vertically oriented wedge-wire screens to guide downstream moving juvenile chub (Squalius cephalus). Ecol. Eng. 2018, 123, 127–134. [Google Scholar] [CrossRef]
- Raynal, S.; Courret, D.; Chatellier, L.; Larinier, M.; David, L. An experimental study on fish-friendly trashracks—Part 1. Inclined trashracks. J. Hydraul. Res. 2013, 51, 56–66. [Google Scholar] [CrossRef]
- Meister, J.; Fuchs, H.; Beck, C.; Albayrak, I.; Boes, R.M. Head losses of horizontal bar racks as fish guidance structures. Water 2020. under review. [Google Scholar]
- Shepherd, D.; Katopodis, C.; Rajaratnam, N. An experimental study of louvers for fish diversion. Can. J. Civ. Eng. 2007, 34, 770–776. [Google Scholar] [CrossRef]
- Raynal, S.; Chatellier, L.; Courret, D.; Larinier, M.; David, L. An experimental study on fish-friendly trashracks—Part 2. Angled trashracks. J. Hydraul. Res. 2013, 51, 67–75. [Google Scholar] [CrossRef]
- Kriewitz, C.R. Leitrechen an Fischabstiegsanlagen: Hydraulik und fischbiologische Effizienz [Guidance screens at fish protection facilities—Hydraulics and fish-biological efficiency]. In VAW-Mitteilungen 230; Boes, R.M., Ed.; Laboratory of Hydraulics, Hydrology and Glaciology (VAW), ETH Zurich: Zurich, Switzerland, 2015. [Google Scholar]
- Albayrak, I.; Kriewitz, C.R.; Hager, W.H.; Boes, R.M. An experimental investigation on louvres and angled bar racks. J. Hydraul. Res. 2018, 56, 59–75. [Google Scholar] [CrossRef]
- Chatellier, L.; Wang, R.-W.; David, L.; Courret, D.; Larinier, M. Experimental characterization of the flow across fish-friendly angled trashrack models. In Proceedings of the 34th IAHR World Congress 2011: Balance and Uncertainty: Water in a Changing World, Brisbane, Australia, 26 June–1 July 2011. [Google Scholar]
- Albayrak, I.; Boes, R.M.; Kriewitz, C.R.; Peter, A.; Tullis, B.P. Fish guidance structures: hydraulic performance and fish guidance efficiencies. J. Ecohydraul. 2019. [Google Scholar] [CrossRef]
- Beck, C.; Albayrak, I.; Meister, J.; Boes, R.M. Hydraulic performance of fish guidance structures with curved bars—Part 1: Head loss assessment. J. Hydraul. Res. 2019. [Google Scholar] [CrossRef]
- Beck, C.; Albayrak, I.; Meister, J.; Boes, R.M. Hydraulic performance of fish guidance structures with curved bars—Part 2: Flow fields. J. Hydraul. Res. 2019. [Google Scholar] [CrossRef]
- Whitney, R.R.; Calvin, L.D.; Erho, M.W.; Coutant, C.C. Downstream Passage for Salmon at Hydroelectric Projects in the Columbia River Basin: Development, Installation, and Evaluation; Northwest Power Planning Council: Portland, OR, USA, 1997. [Google Scholar]
- Heisey, P.G.; Mathur, D.; Skalski, J.R.; McDonald, R.D.; Velazquez, G. Effects of Spillway Structural Modifications on Fish Condition and Survival. In Advances in Fisheries Bioengineering; Amaral, S.V., Mathur, D., Taft, E.P., Eds.; American Fisheries Society: Bethesda, MD, USA, 2008; pp. 165–179. [Google Scholar]
- Watene, E.M.; Boubée, J.A.T. Selective opening of hydroelectric dam spillway gates for downstream migrant eels in New Zealand. Fish. Manage. Ecol. 2005, 12, 69–75. [Google Scholar] [CrossRef]
- Rouvé, G. Der Krafthaustrennpfeiler—Strömungsverhältnisse an gekrümmten Wänden [The dividing pier between powerhouse and weir—Flow conditions at curved walls]. In Mitteilung Nr. 145; Wittmann, H., Ed.; Theodor-Rehbock-Laboratorium, Karlsruhe Institute of Technology: Karlsruhe, Germany, 1958. [Google Scholar]
- Goring, D.G.; Nikora, V.I. Despiking acoustic doppler velocimeter data. J. Hydraul. Eng. 2002, 128, 117–126. [Google Scholar] [CrossRef]
- Mori, N.; Suzuki, T.; Kakuno, S. Noise of acoustic doppler velocimeter data in bubbly flows. J. Eng. Mech. 2007, 122, 122–125. [Google Scholar] [CrossRef]
- Godde, D. Experimentelle Untersuchungen zur Anströmung von Rohrturbinen [Experimental investigation of bulb turbine approach flow]. In TUM-Mitteilung Nr. 75; Strobl, T., Ed.; Chair of Hydraulic and Water Resources Engineering, TU Munich: Munich, Germany, 1994. [Google Scholar]
- Feigenwinter, L.; Vetsch, D.F.; Kammerer, S.; Kriewitz, C.R.; Boes, R.M. Conceptual Approach for Positioning of Fish Guidance Structures Using CF.D and expert knowledge. Sustainability 2019, 11, 1646. [Google Scholar] [CrossRef]







| Guild | TL = 0.1 m | TL = 0.2 m |
|---|---|---|
| rheophile | 0.38 | 0.66 |
| non-rheophile | 0.28 | 0.48 |
| Study | Rack | Angle α, γ | BR | HOv | Layout |
|---|---|---|---|---|---|
| Maager [7], Albayrak et al. [8] | HBR | α = 30°, 45° | 0.33, 0.39, 0.55 | 0–0.30 | S |
| Szabo-Meszaros et al. [9] | HBR 1 | α = 30° | ≈0.35 2 | 0 | B |
| Berger [10] | HBR | α = 30°, 45°, 55°, 70° | 0.32–0.57 | 0 | B |
| De Bie et al. [11] | HBR 1 | α = 30° | ≈0.33 2 | 0 | B |
| Raynal et al. [12] | inclined | γ = 15–90° | ≈0.25–0.50 2 | 0 | S |
| Raynal et al. [15] | ABR | α = 30°, 45°, 60°, 90° | ≈0.25–0.50 2 | 0 | S |
| Chatellier et al. [18] | ABR | α = 30°, 45°, 60° | ≈0.25–0.50 2 | 0 | S |
| Kriewitz [16], Albayrak et al. [17,19] | MBR, Louver | α = 15°, 30°, 45° | 0.04, 0.08, 0.17 | 0.11 | S, B, PW |
| Beck et al. [20,21] | CBR | α = 15°, 30°, 45° | 0.04, 0.08, 0.17 | 0–0.30 | S |
| Present study | HBR | α = 30°, 45°, 90° | 0.28, 0.35, 0.49 | 0–0.40 | S, W |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Meister, J.; Fuchs, H.; Beck, C.; Albayrak, I.; Boes, R.M. Velocity Fields at Horizontal Bar Racks as Fish Guidance Structures. Water 2020, 12, 280. https://doi.org/10.3390/w12010280
Meister J, Fuchs H, Beck C, Albayrak I, Boes RM. Velocity Fields at Horizontal Bar Racks as Fish Guidance Structures. Water. 2020; 12(1):280. https://doi.org/10.3390/w12010280
Chicago/Turabian StyleMeister, Julian, Helge Fuchs, Claudia Beck, Ismail Albayrak, and Robert M. Boes. 2020. "Velocity Fields at Horizontal Bar Racks as Fish Guidance Structures" Water 12, no. 1: 280. https://doi.org/10.3390/w12010280
APA StyleMeister, J., Fuchs, H., Beck, C., Albayrak, I., & Boes, R. M. (2020). Velocity Fields at Horizontal Bar Racks as Fish Guidance Structures. Water, 12(1), 280. https://doi.org/10.3390/w12010280







