Experimental and Numerical Investigation of River Closure Project
Abstract
1. Introduction
2. Numerical Method
2.1. Saint-Venant Equations
2.2. Shallow Water Equations
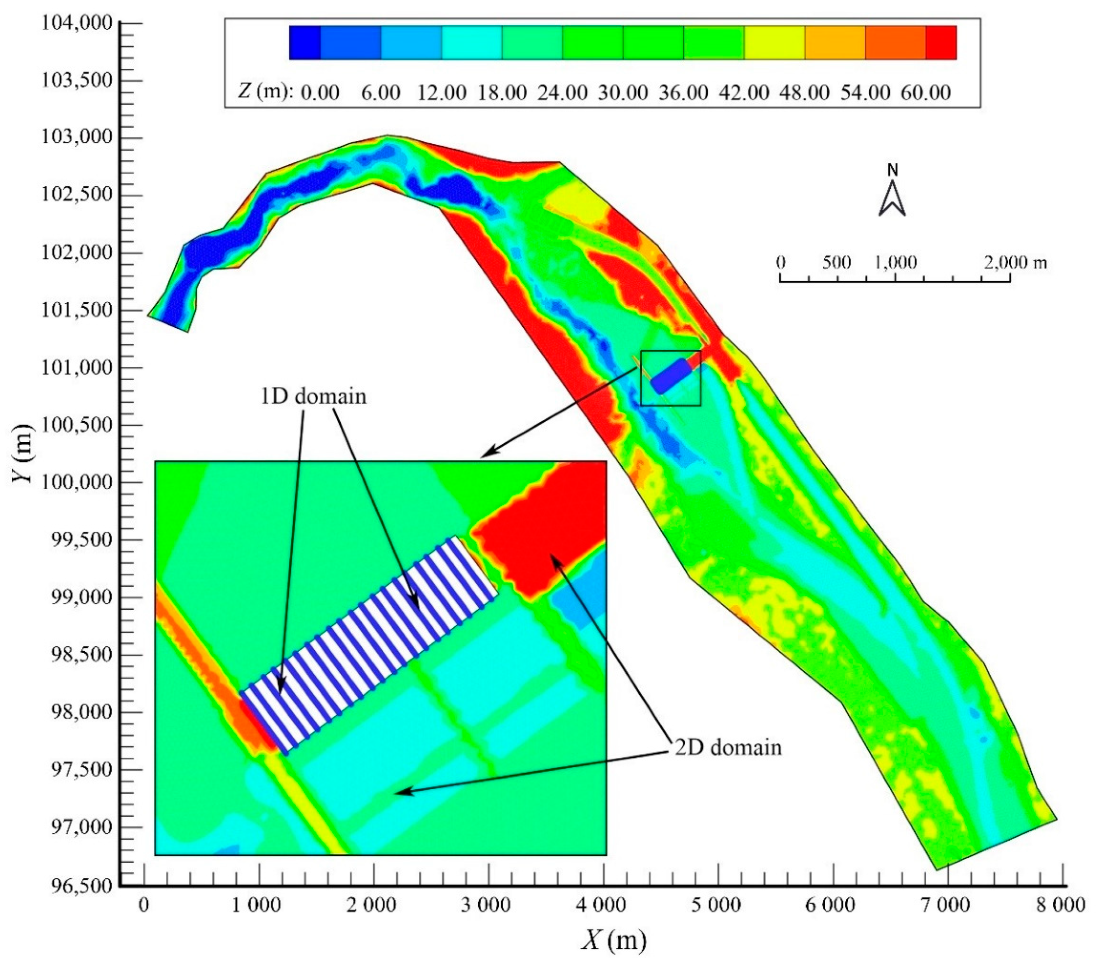
3. Model Coupling
4. Physical Experiment Setup
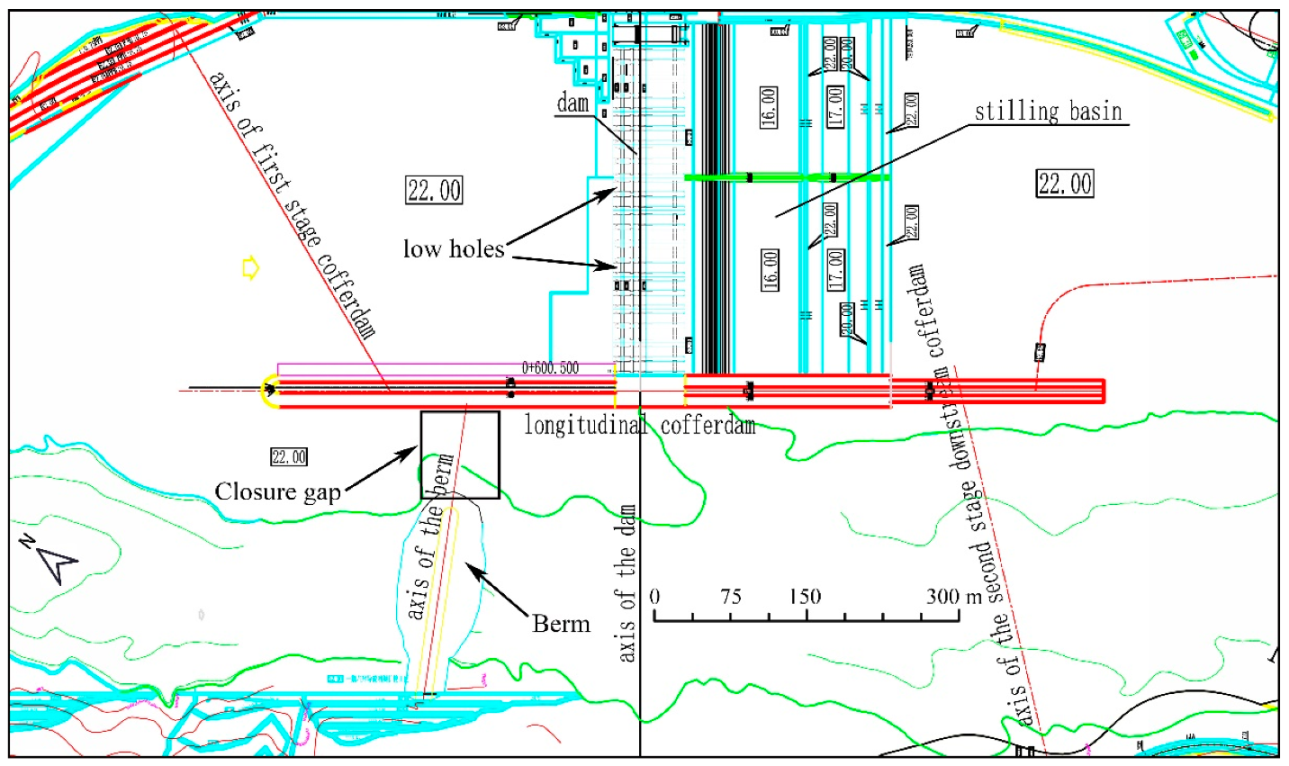
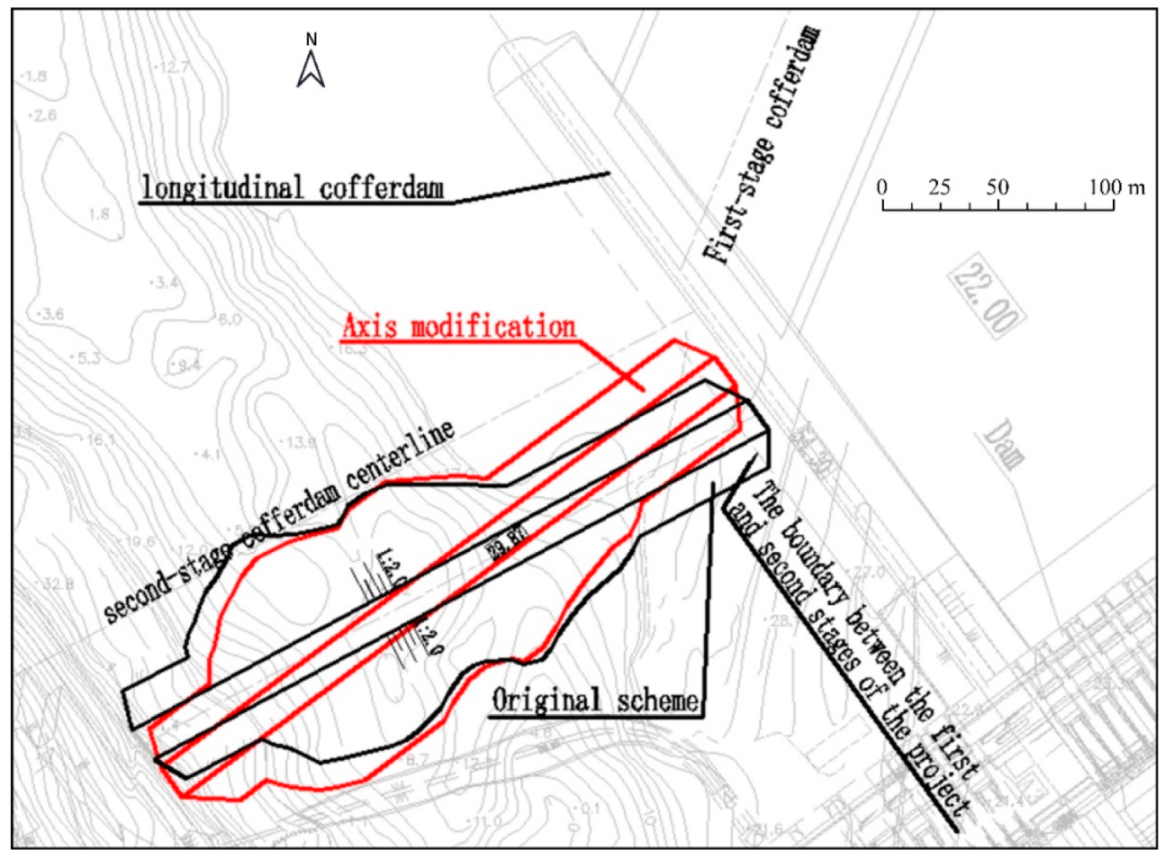
4.1. Project Overview
4.2. Experimental Set-Up and Instrumentation
4.3. Experimental Calibration
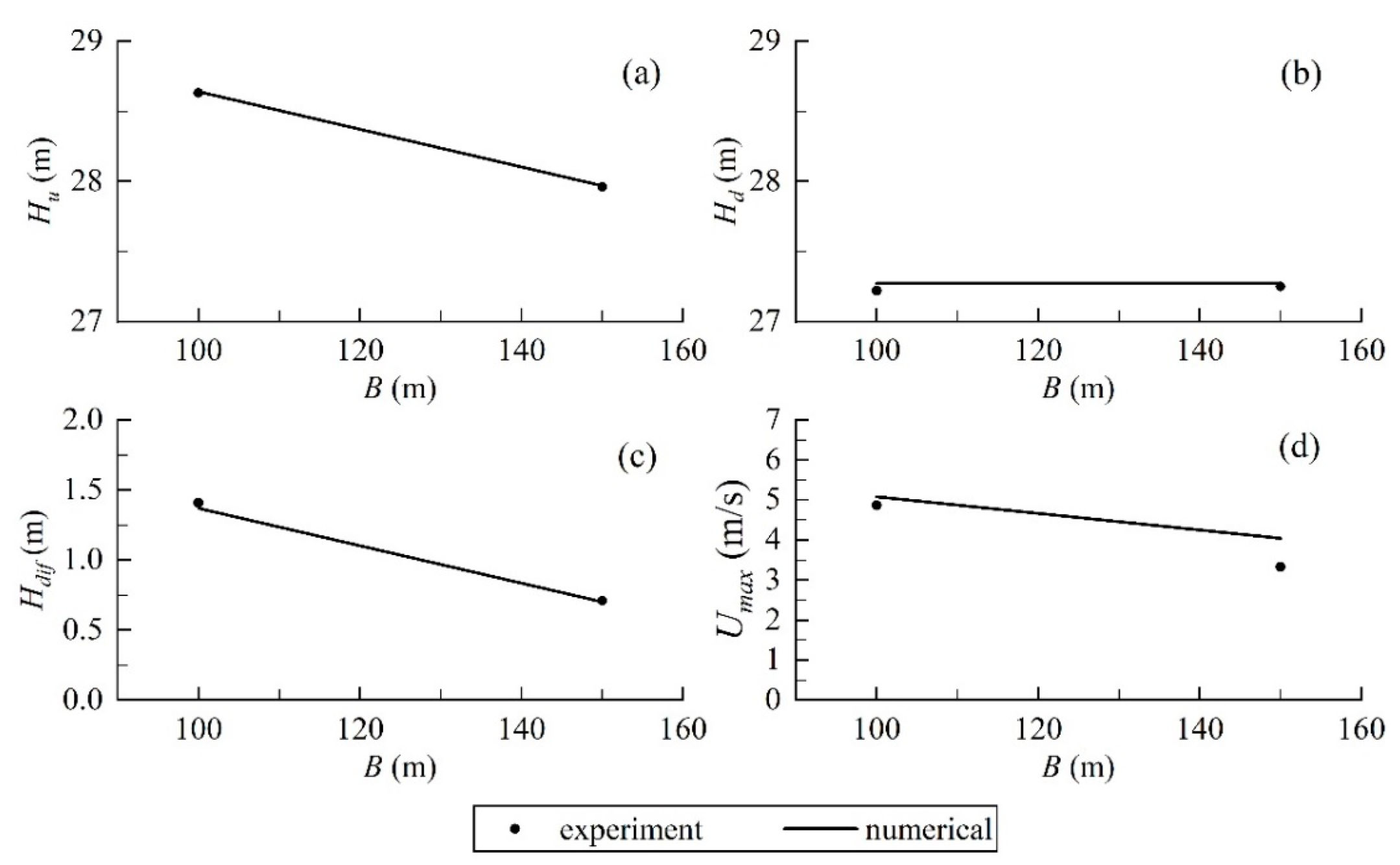
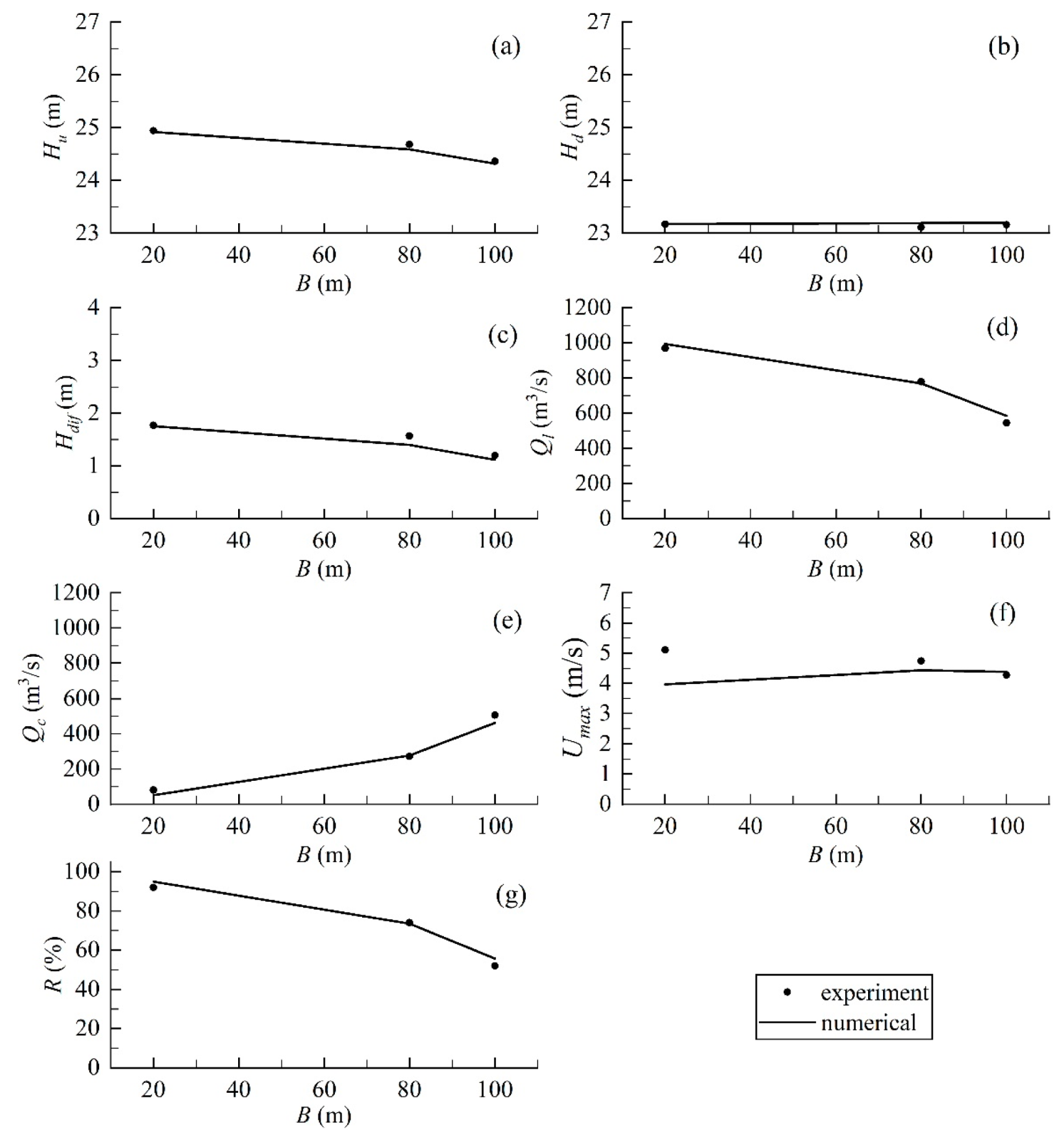
5. Model Validation
6. Application to the Real River Closure Project
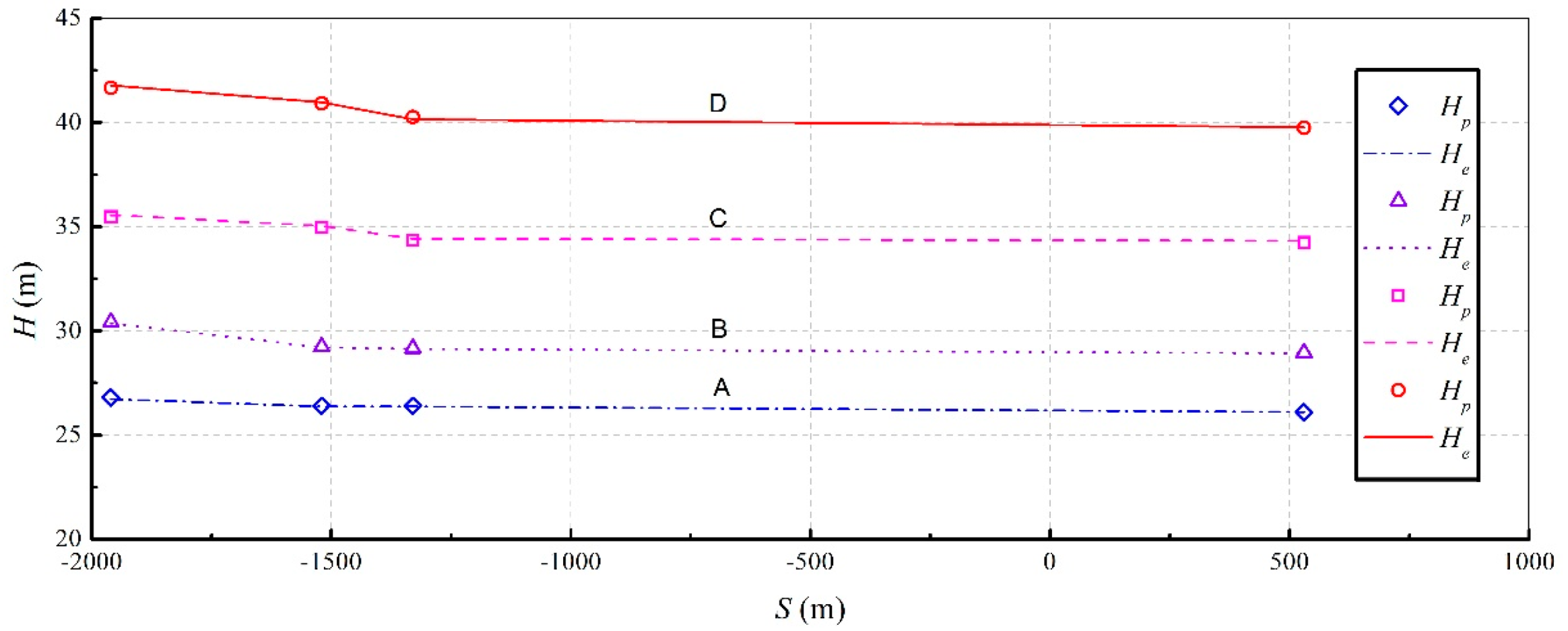
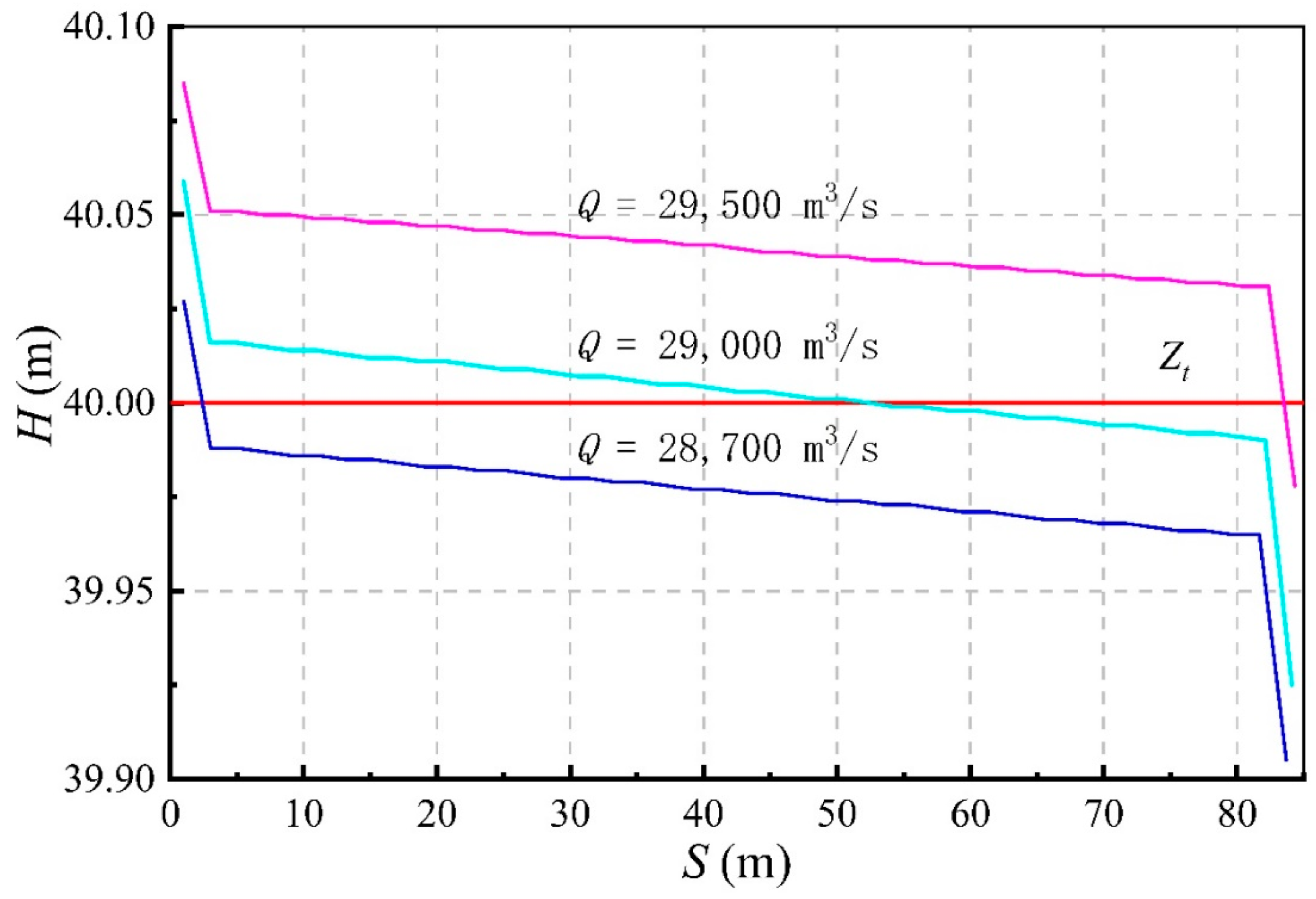
6.1. Transition Flow Rate
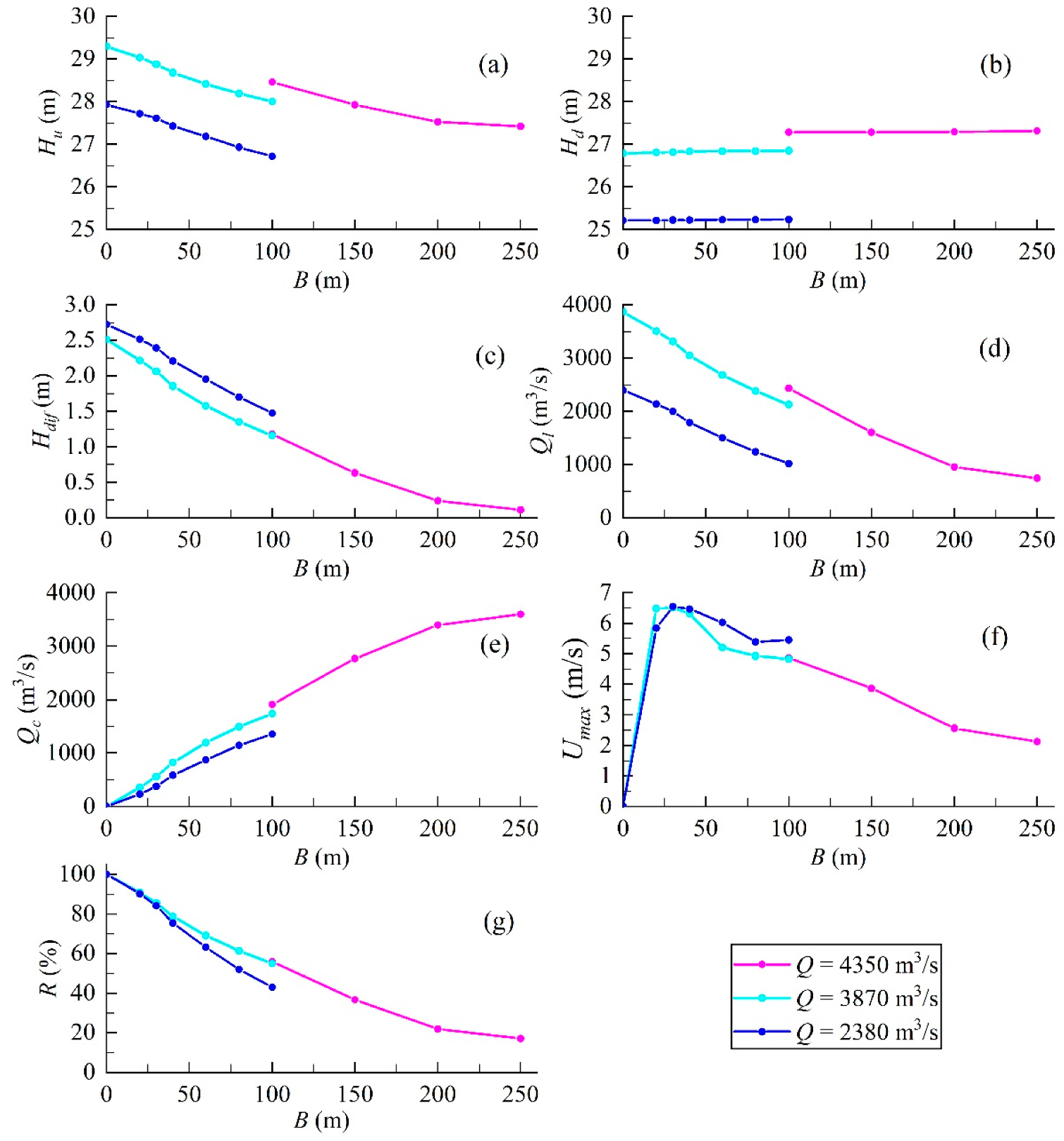
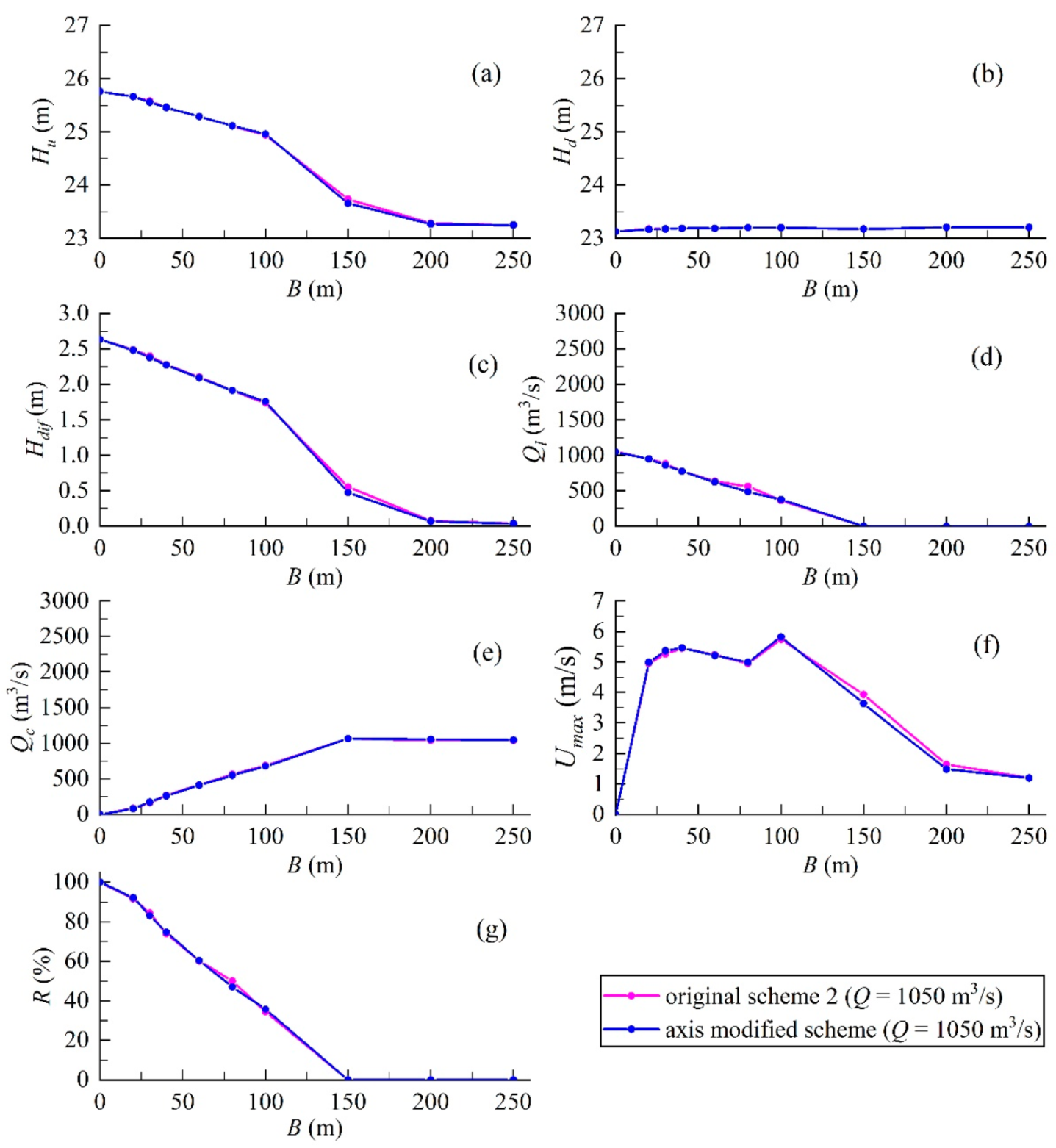
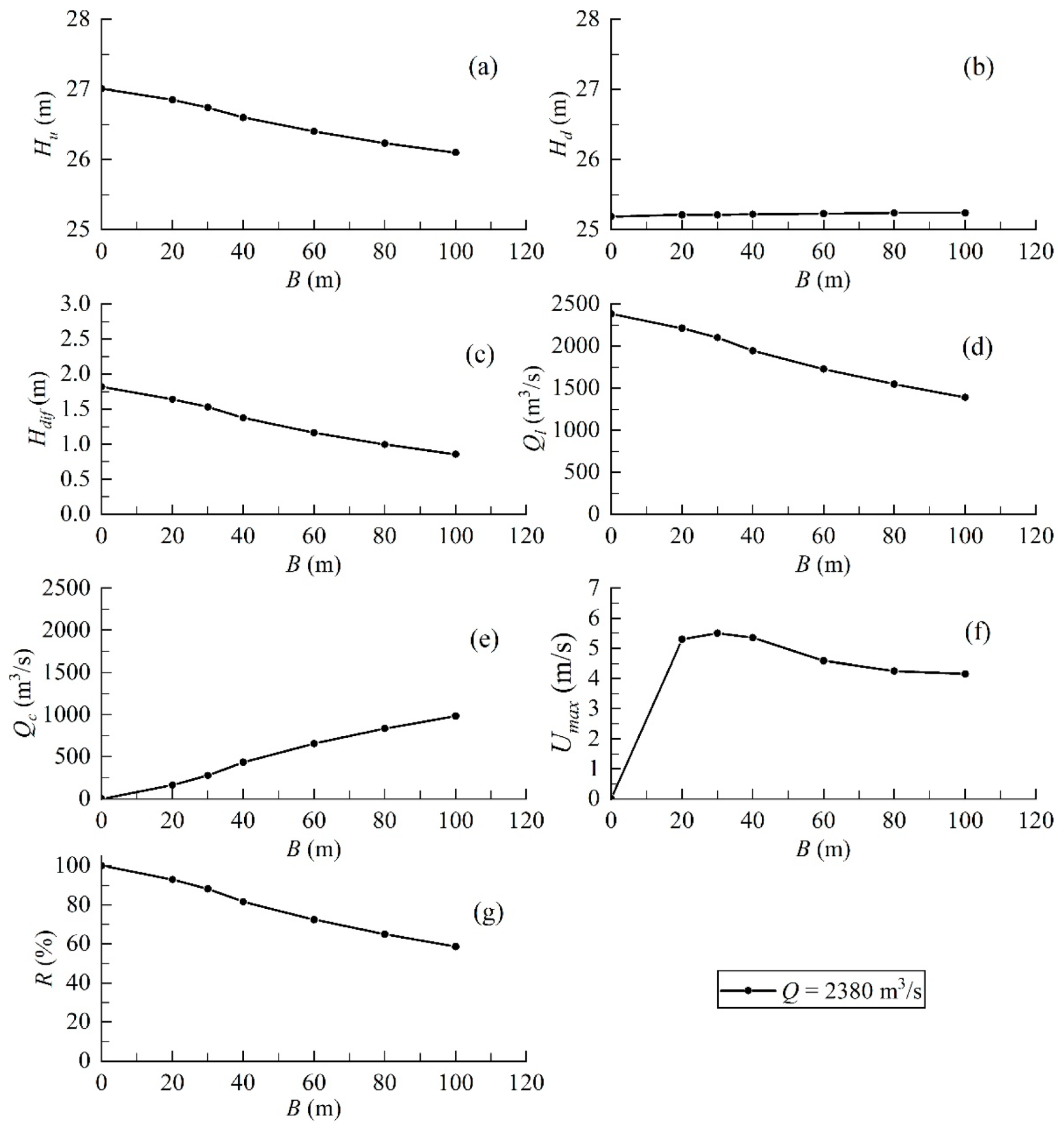
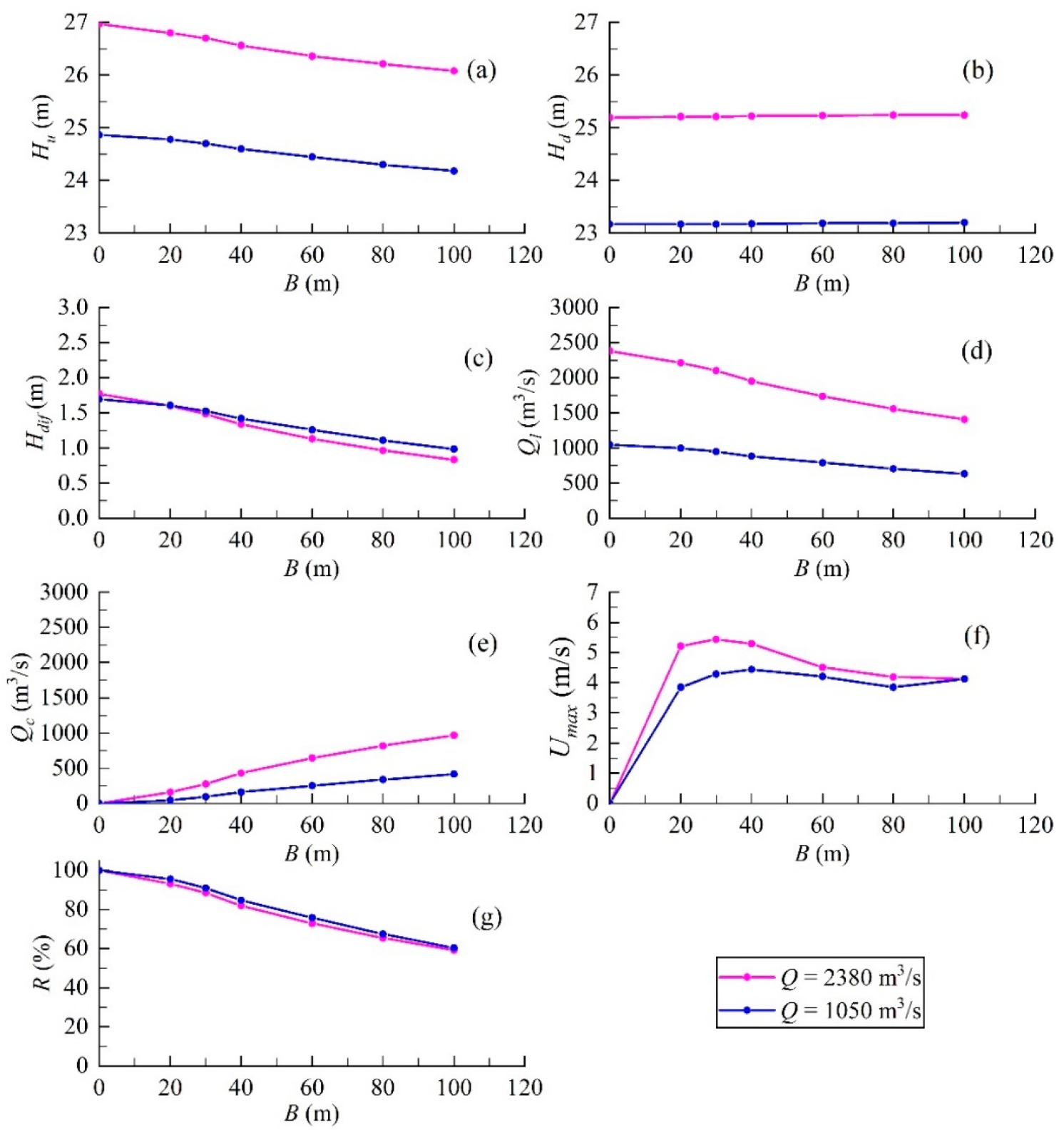
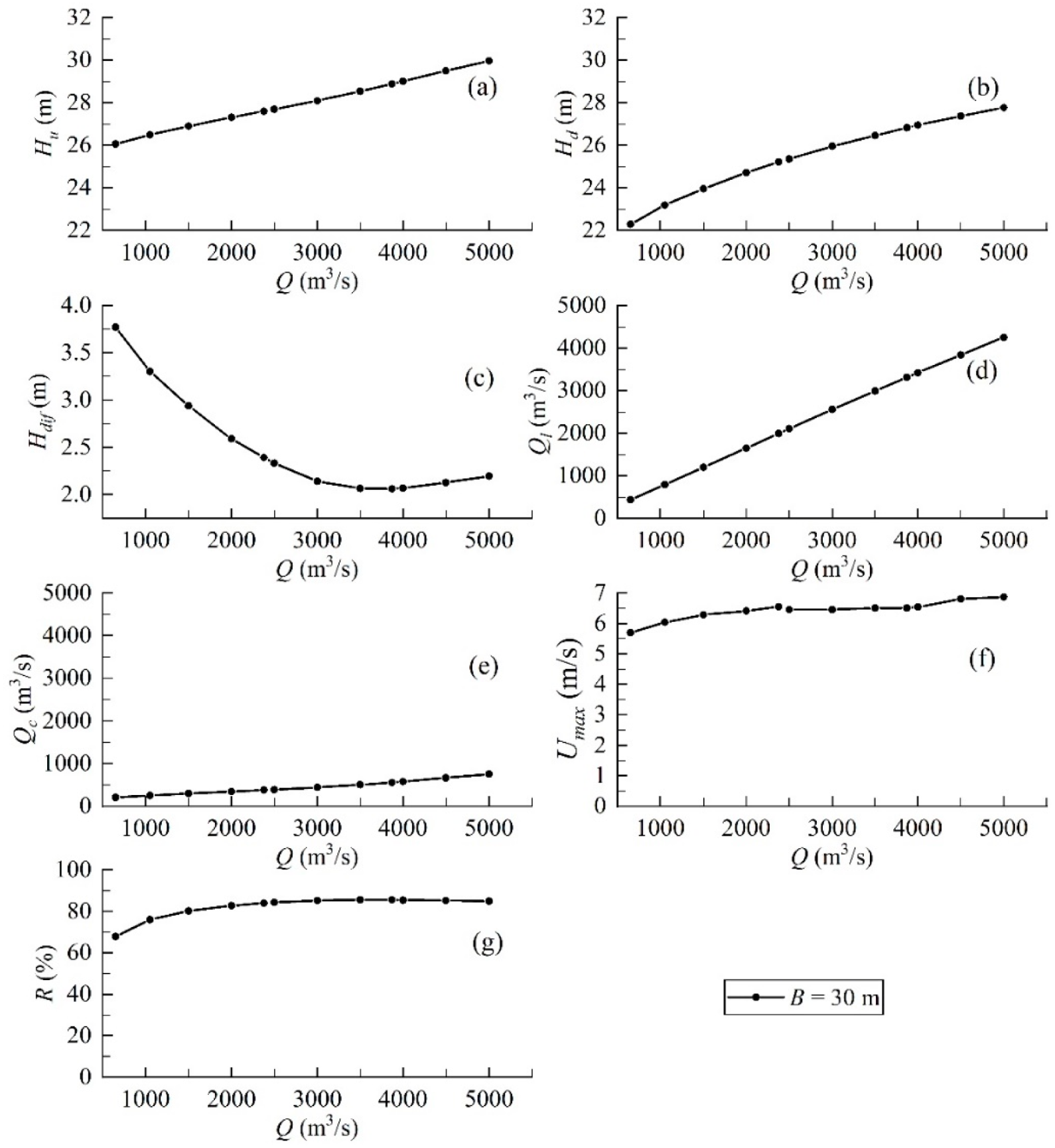
6.2. Numerical Results Under Different Working Conditions
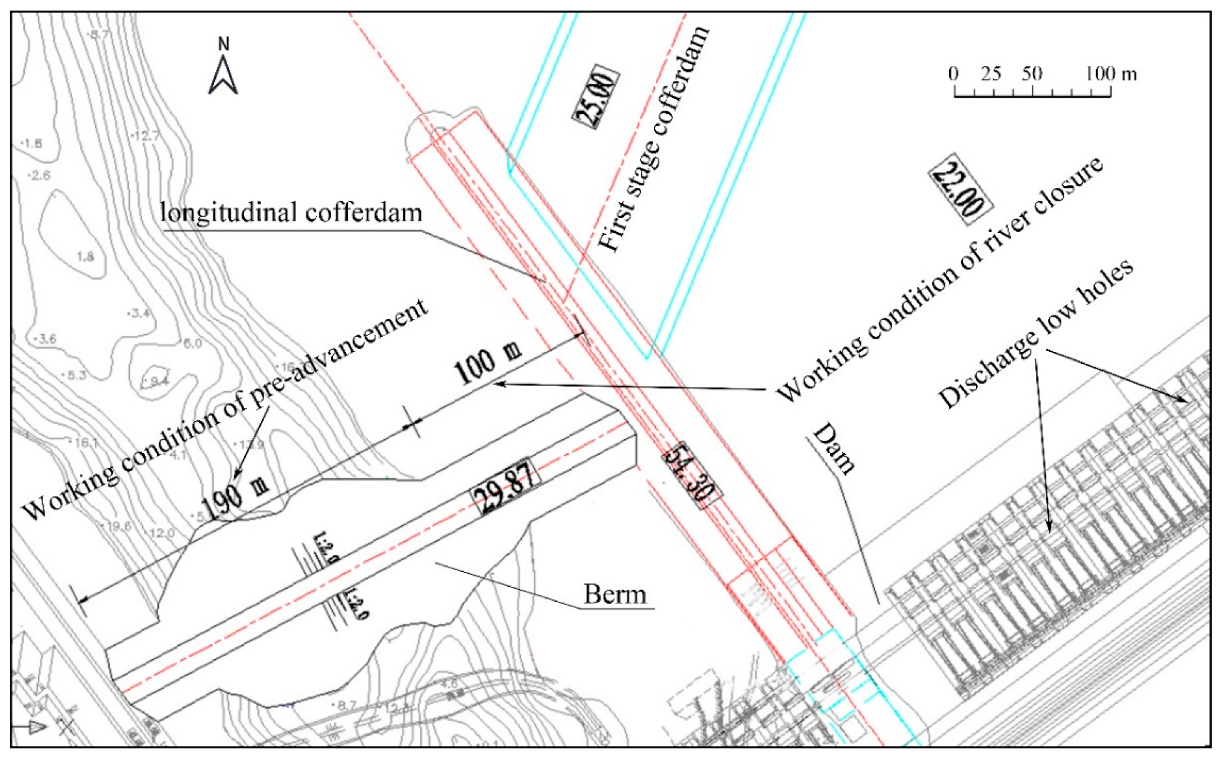
6.3. Process of River Closure
7. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Li, W.; Chen, Q.; Mao, J. Development of 1D and 2D coupled model to simulate urban inundation: An application to Beijing Olympic Village. Chin. Sci. Bull. 2009, 54, 1613–1621. [Google Scholar] [CrossRef]
- Marin, J.; Monnier, J. Superposition of local zoom models and simultaneous calibration for 1D–2D shallow water flows. Math. Comput. Simul. 2009, 80, 547–560. [Google Scholar] [CrossRef]
- Chen, Y.; Wang, Z.; Liu, Z.; Zhu, D. 1D–2D Coupled Numerical Model for Shallow-Water Flows. J. Hydraul. Eng. 2011, 138, 122–132. [Google Scholar] [CrossRef]
- Morales-Hernández, M.; García-Navarro, P.; Burguete, J.; Brufau, P. A conservative strategy to couple 1D and 2D models for shallow water flow simulation. Comput. Fluids 2013, 81, 26–44. [Google Scholar] [CrossRef]
- Timbadiya, P.V.; Patel, P.L.; Porey, P.D. A 1D–2D Coupled Hydrodynamic Model for River Flood Prediction in a Coastal Urban Floodplain. J. Hydrol. Eng. 2014, 20, 05014017. [Google Scholar] [CrossRef]
- Martins, R.; Leandro, J.; Djordjević, S. Influence of sewer network models on urban flood damage assessment based on coupled 1D/2D models. J. Flood Risk Manag. 2018, 11, S717–S728. [Google Scholar] [CrossRef]
- Vozinaki, A.E.K.; Morianou, G.G.; Alexakis, D.D.; Tsanis, I.K. Comparing 1D and combined 1D/2D hydraulic simulations using high-resolution topographic data: a case study of the Koiliaris basin. Greece. Hydrol. Sci. J. 2017, 62, 642–656. [Google Scholar] [CrossRef]
- Han, D.; Fang, H.W.; Bai, J.; He, G.J. A coupled 1-D and 2-D channel network mathematical model used for flow calculations in the middle reaches of the yangtze river. J. Hydrodyn. 2011, 23, 521–526. [Google Scholar] [CrossRef]
- Finaud-Guyot, P.; Delenne, C.; Guinot, V.; Llovel, C. 1D–2D coupling for river flow modeling. Comptes Rendus Mec. 2011, 339, 226–234. [Google Scholar] [CrossRef]
- Bladé, E.; Gómez-Valentín, M.; Dolz, J.; Aragón-Hernández, J.L.; Corestein, G.; Sánchez-Juny, M. Integration of 1D and 2D finite volume schemes for computations of water flow in natural channels. Adv. Water Resour. 2012, 42, 17–29. [Google Scholar] [CrossRef]
- Kuiry, S.N.; Sen, D.; Bates, P.D. Coupled 1D–Quasi-2D Flood Inundation Model with Unstructured Grids. J. Hydraul. Eng. 2010, 136, 493–506. [Google Scholar] [CrossRef]
- Fernández-Nieto, E.D.; Marin, J.; Monnier, J. Coupling superposed 1D and 2D shallow-water models: Source terms in finite volume schemes. Comput. Fluids 2010, 39, 1070–1082. [Google Scholar] [CrossRef]
- Liu, Q.; Qin, Y.; Zhang, Y.; Li, Z. A coupled 1D–2D hydrodynamic model for flood simulation in flood detention basin. Nat. Hazards 2015, 75, 1303–1325. [Google Scholar] [CrossRef]
- Morales-Hernández, M.; Petaccia, G.; Brufau, P.; García-Navarro, P. Conservative 1D–2D coupled numerical strategies applied to river flooding: The Tiber (Rome). Appl. Math. Model. 2016, 40, 2087–2105. [Google Scholar] [CrossRef]
- Vojinovic, Z.; Tutulic, D. On the use of 1d and coupled 1D–2D modelling approaches for assessment of flood damage in urban areas. Urban Water J. 2009, 6, 183–199. [Google Scholar] [CrossRef]
- Fan, Y.; Ao, T.; Yu, H.; Huang, G.; Li, X. A coupled 1D–2D hydrodynamic model for urban flood inundation. Adv. Meteorol. 2017, 2017, 2819308. [Google Scholar] [CrossRef]
- Kerger, F.; Archambeau, P.; Erpicum, S.; Dewals, B.J.; Pirotton, M. An exact Riemann solver and a Godunov scheme for simulating highly transient mixed flows. J. Comput. Appl. Math. 2011, 235, 2030–2040. [Google Scholar] [CrossRef]
- Kerger, F.; Archambeau, P.; Erpicum, S.; Dewals, B.J.; Pirotton, M. A fast universal solver for 1D continuous and discontinuous steady flows in rivers and pipes. Int. J. Numer. Methods Fluids 2011, 66, 38–48. [Google Scholar] [CrossRef]
- Ferńdez-Pato, J.; García-Navarro, P. A pipe network simulation model with dynamic transition between free surface and pressurized flow. Procedia Eng. 2014, 70, 641–650. [Google Scholar] [CrossRef]
- Fernández-Pato, J.; García-Navarro, P. Finite volume simulation of unsteady water pipe flow. Drink. Water Eng. Sci. 2014, 7, 83–92. [Google Scholar] [CrossRef]
- Malekpour, A.; Karney, B.W. Spurious Numerical Oscillations in the Preissmann Slot Method: Origin and Suppression. J. Hydraul. Eng. 2015, 142, 04015060. [Google Scholar] [CrossRef]
- Ji, Z. General hydrodynamic model for sewer/channel network systems. lournal Hydraul. Eng. 1998, 124, 307–315. [Google Scholar] [CrossRef]
- Wang, Z.; Geng, Y. An unstructured finite volume algorithm for nonlinear two dimensional shallow water equation. J. Hydrodyn. Ser. 2005, 17, 306–312. [Google Scholar]




| Cases | A | B | C | D |
|---|---|---|---|---|
| (m3/s) | 2420 | 5800 | 15,659 | 36,600 |
| Serial Number | Original Scheme 1 | Original Scheme 2 | Axis Modified | Original Scheme 3 | Original Scheme 4 | |||||
|---|---|---|---|---|---|---|---|---|---|---|
| Zf = 25 | Zf = 24 | Zf = 24 | Zf = 23 | Zf = 22 | ||||||
| Q (m3/s) | B (m) | Q (m3/s) | B (m) | Q (m3/s) | B (m) | Q (m3/s) | B (m) | Q (m3/s) | B (m) | |
| 1 | 4350 | 250 | 1050 | 250 | 1050 | 250 | 2380 | 100 | 2380 | 100 |
| 2 | 4350 | 200 | 1050 | 200 | 1050 | 200 | 2380 | 80 | 2380 | 80 |
| 3 | 4350 | 150 | 1050 | 150 | 1050 | 150 | 2380 | 60 | 2380 | 60 |
| 4 | 4350 | 100 | 1050 | 100 | 1050 | 100 | 2380 | 40 | 2380 | 40 |
| 5 | 3870 | 100 | 1050 | 80 | 1050 | 80 | 2380 | 30 | 2380 | 30 |
| 6 | 3870 | 80 | 1050 | 60 | 1050 | 60 | 2380 | 20 | 2380 | 20 |
| 7 | 3870 | 60 | 1050 | 40 | 1050 | 40 | 2380 | 0 | 2380 | 0 |
| 8 | 2380 | 40 | 1050 | 30 | 1050 | 30 | - | - | 1050 | 100 |
| 9 | 2380 | 30 | 1050 | 20 | 1050 | 20 | - | - | 1050 | 80 |
| 10 | 2380 | 20 | 1050 | 0 | 1050 | 0 | - | - | 1050 | 60 |
| 11 | 2380 | 0 | - | - | - | - | - | - | 1050 | 40 |
| 12 | 2380 | 100 | - | - | - | - | - | - | 1050 | 30 |
| 13 | 2380 | 80 | - | - | - | - | - | - | 1050 | 20 |
| 14 | 2380 | 60 | - | - | - | - | - | - | 1050 | 0 |
| 15 | 2380 | 40 | - | - | - | - | - | - | - | - |
| 16 | 2380 | 30 | - | - | - | - | - | - | - | - |
| 17 | 2380 | 20 | - | - | - | - | - | - | - | - |
| 18 | 2380 | 0 | - | - | - | - | - | - | - | - |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lin, J.; Jin, S.; Ai, C.; Ding, W. Experimental and Numerical Investigation of River Closure Project. Water 2020, 12, 241. https://doi.org/10.3390/w12010241
Lin J, Jin S, Ai C, Ding W. Experimental and Numerical Investigation of River Closure Project. Water. 2020; 12(1):241. https://doi.org/10.3390/w12010241
Chicago/Turabian StyleLin, Jinbo, Sheng Jin, Congfang Ai, and Weiye Ding. 2020. "Experimental and Numerical Investigation of River Closure Project" Water 12, no. 1: 241. https://doi.org/10.3390/w12010241
APA StyleLin, J., Jin, S., Ai, C., & Ding, W. (2020). Experimental and Numerical Investigation of River Closure Project. Water, 12(1), 241. https://doi.org/10.3390/w12010241





