3D Numerical Investigation of Forces and Flow Field around the Semi-Submersible Platform in An Internal Solitary Wave
Abstract
1. Introduction
2. Numerical Model
2.1. Governing Equations
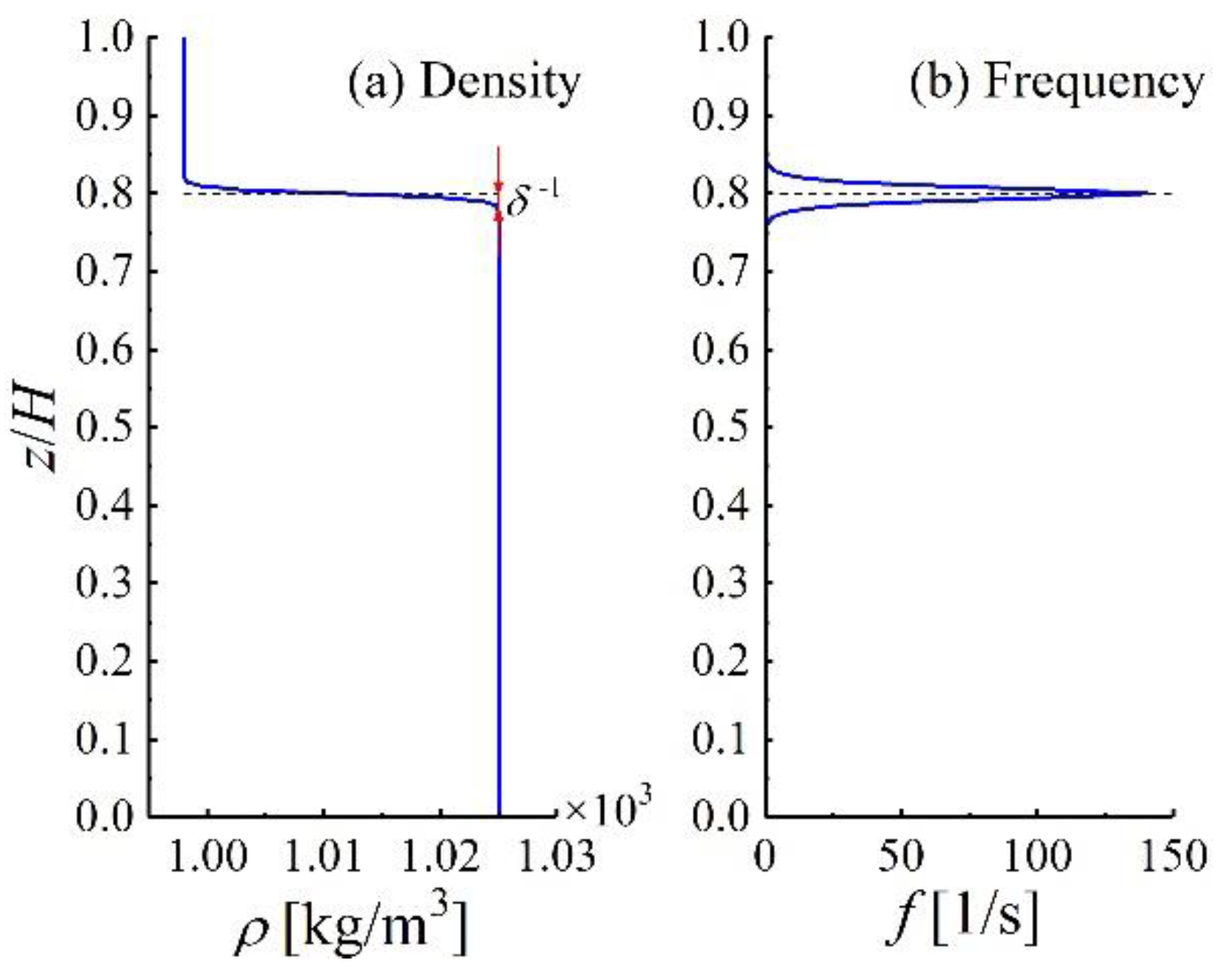
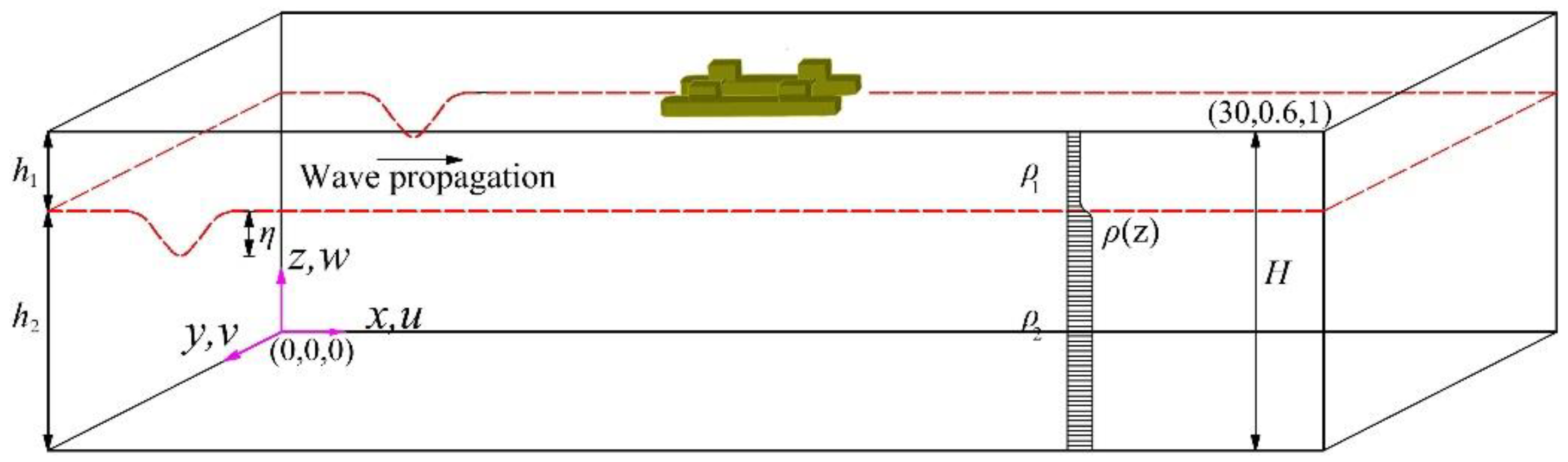
2.2. ISW Generation
2.3. Numerical Method
3. Flow around the Semi-Submersible Platform Induced by the ISW Propagation
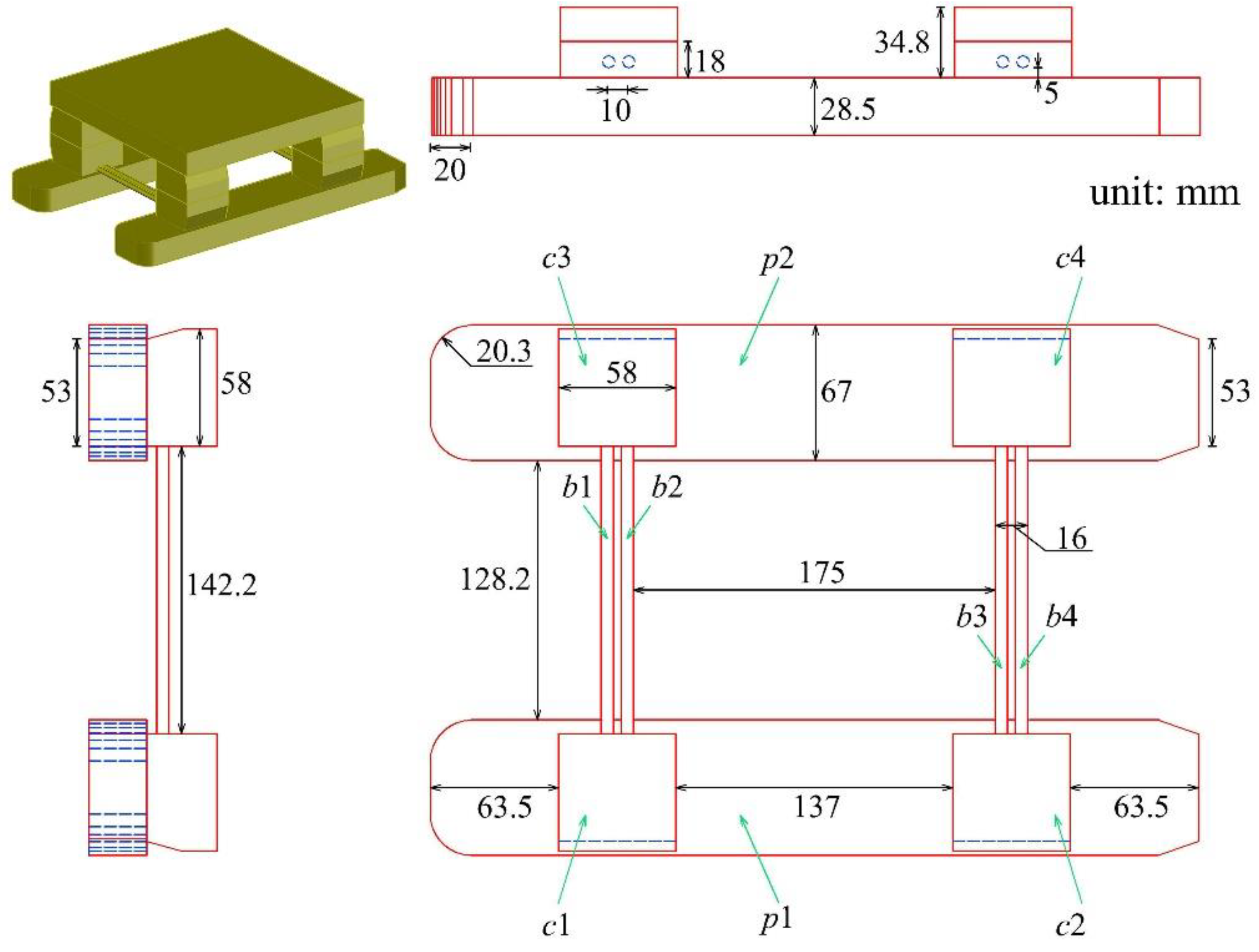
3.1. Computational Setup
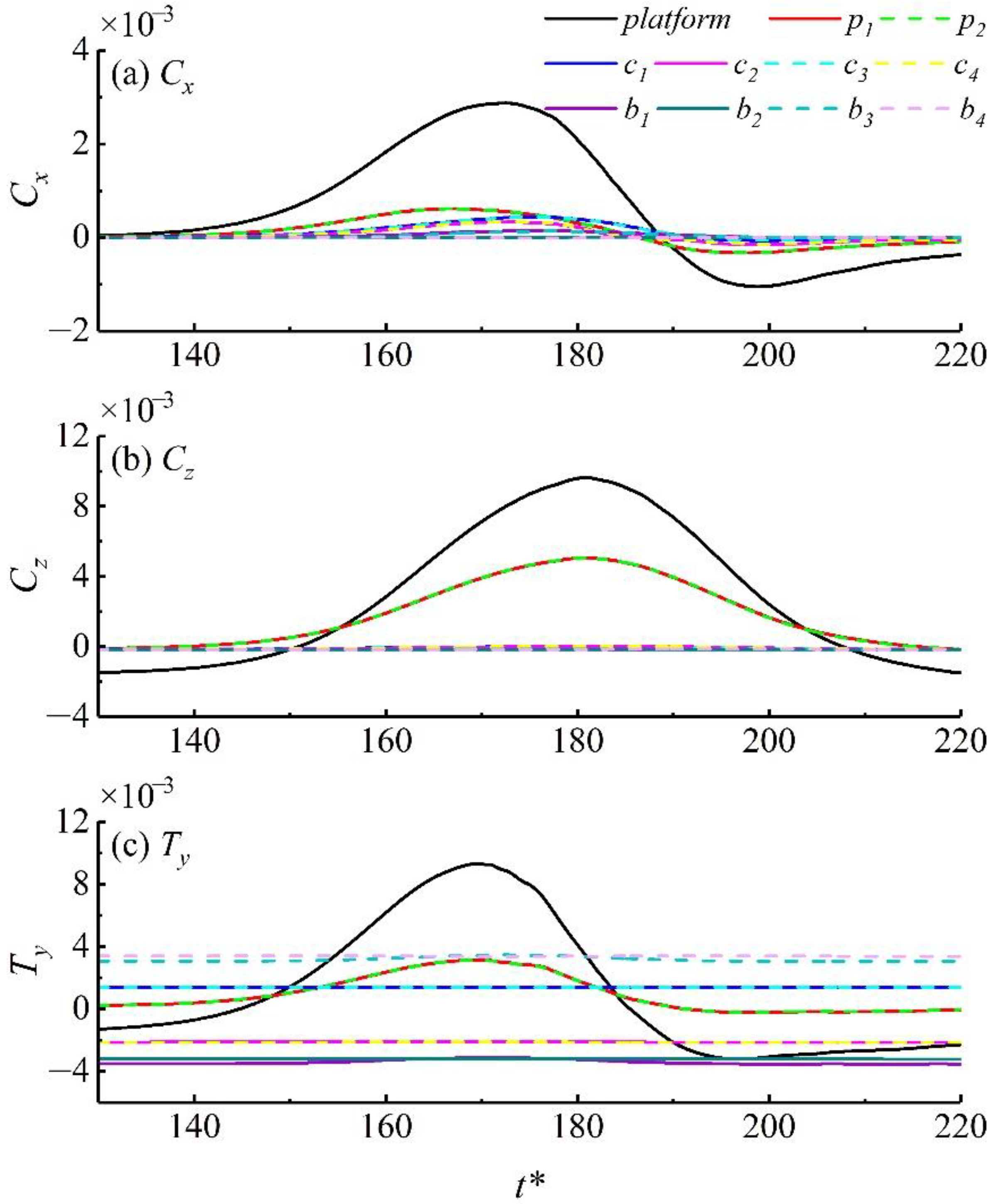
3.2. Analysis of Forces on the Platform
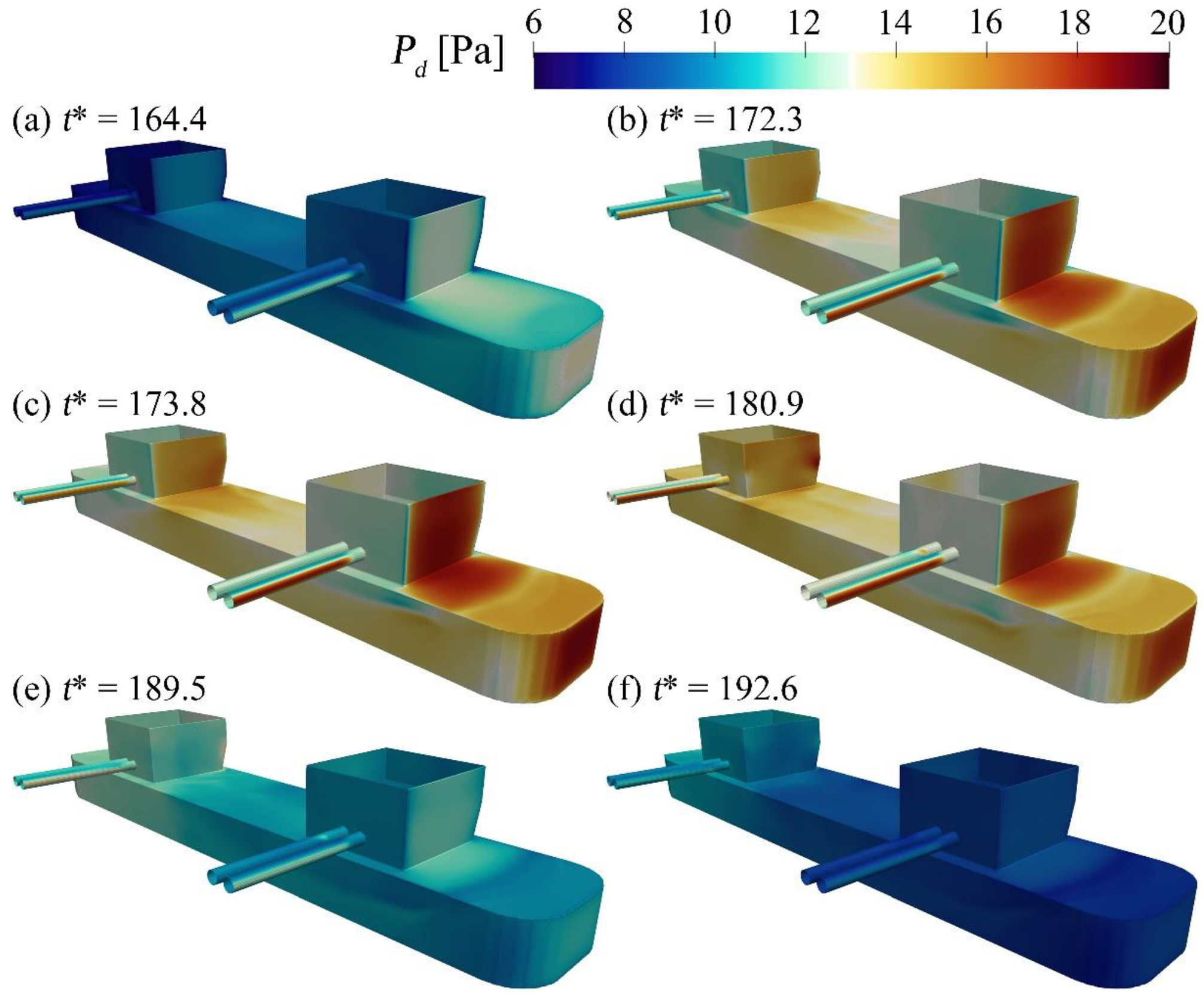
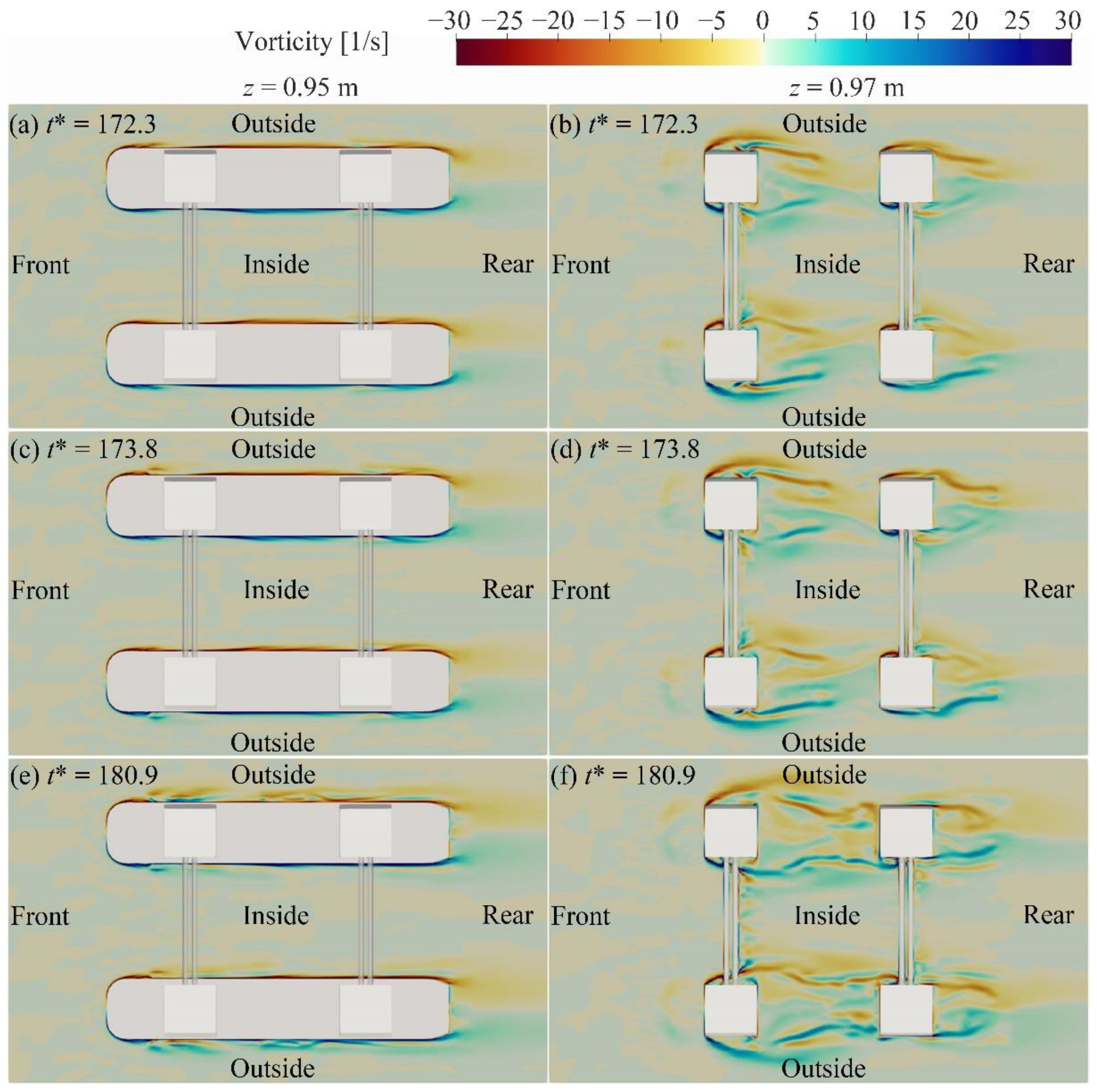
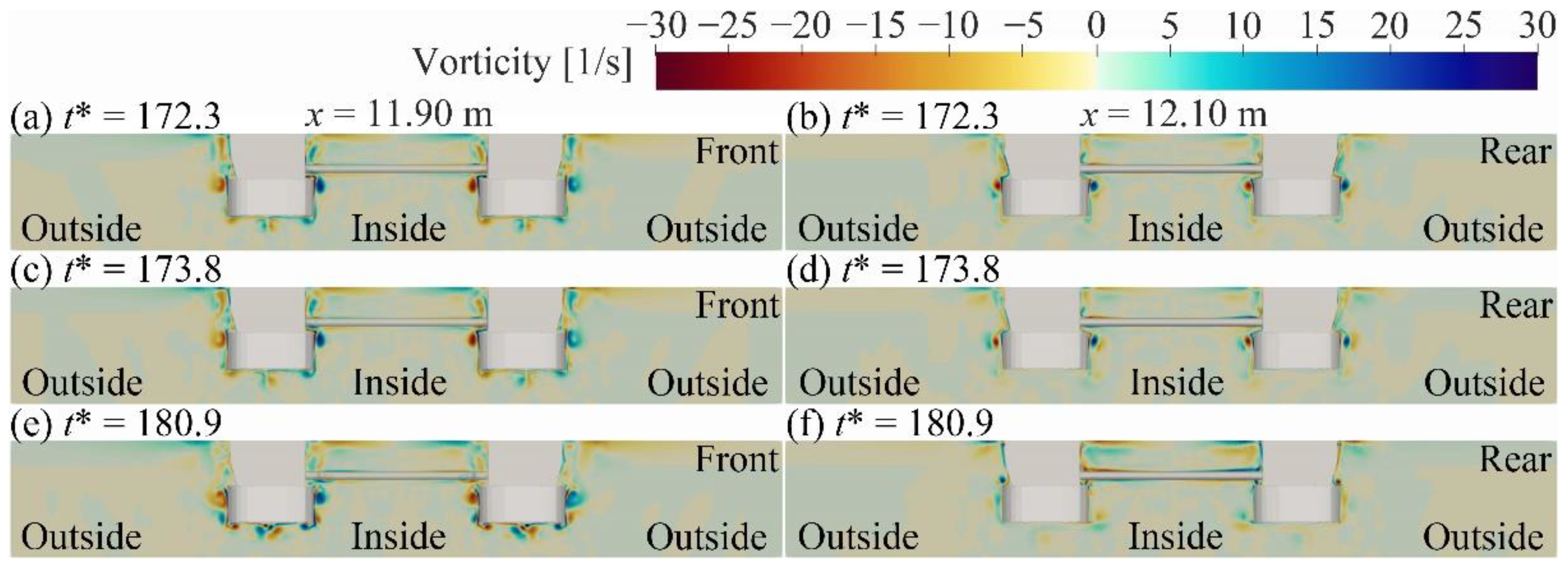
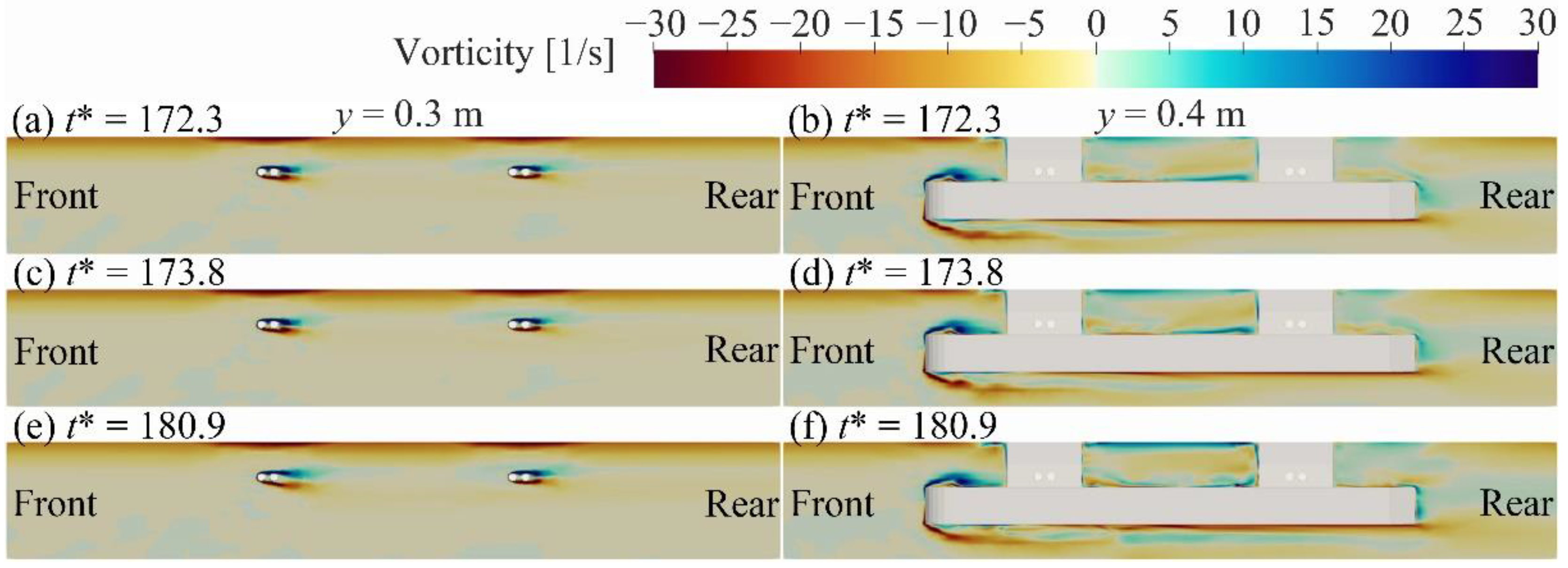
3.3. Dynamic Pressure and Flow Field around the Platform
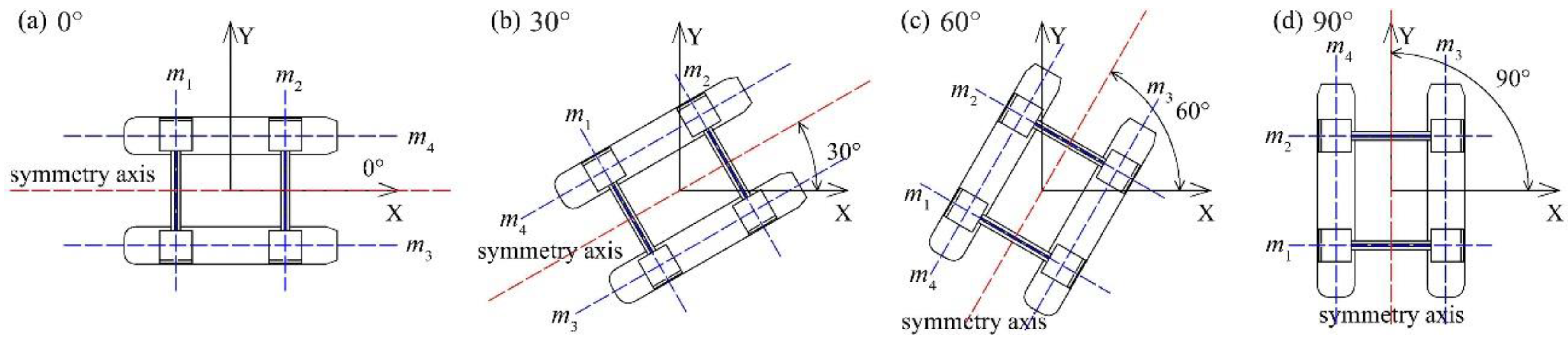
4. ISW Propagation over the Semi-Submersible Platform Located at Different Angles
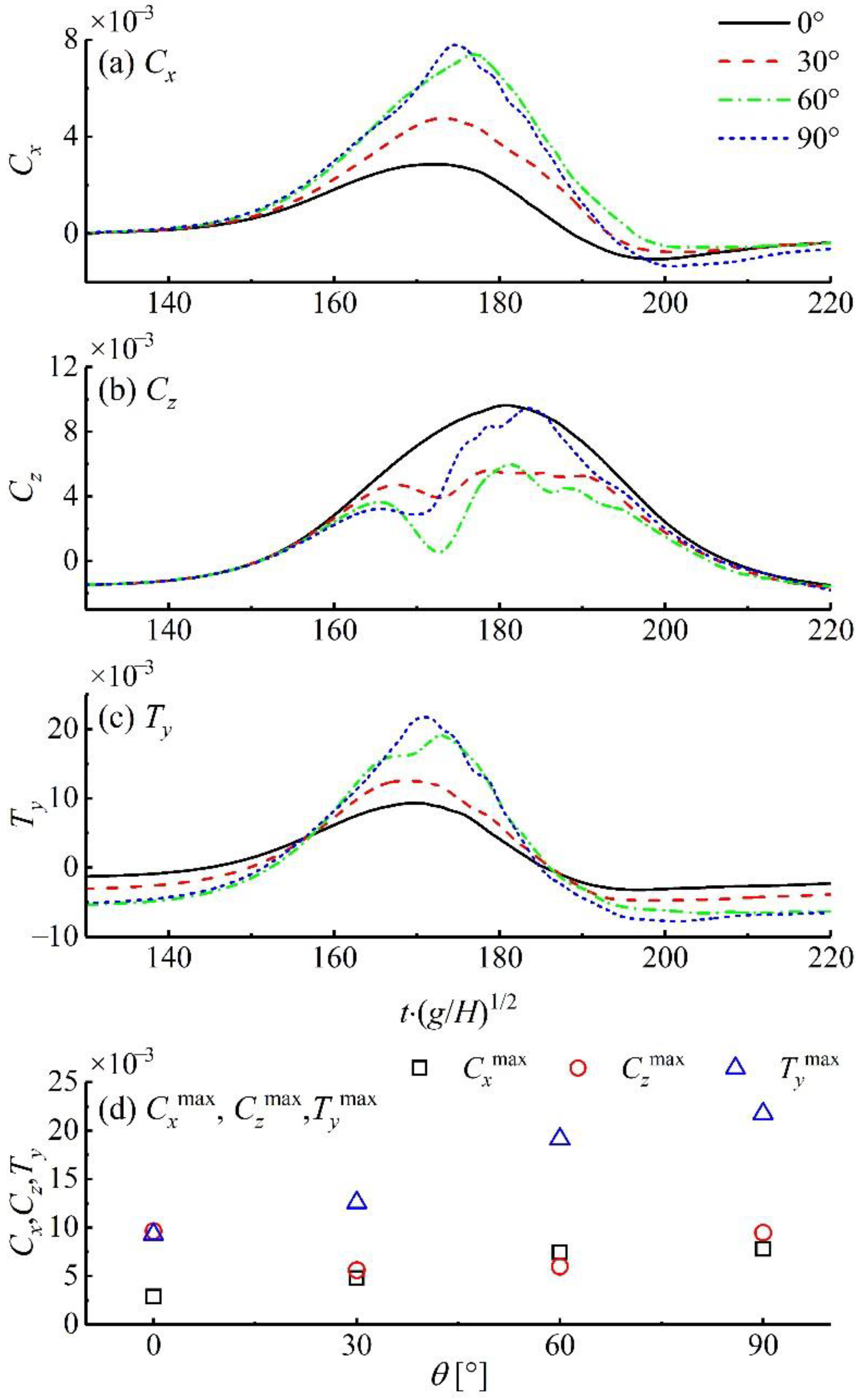
4.1. Analysis of Forces on the Platform Located at Different Angles
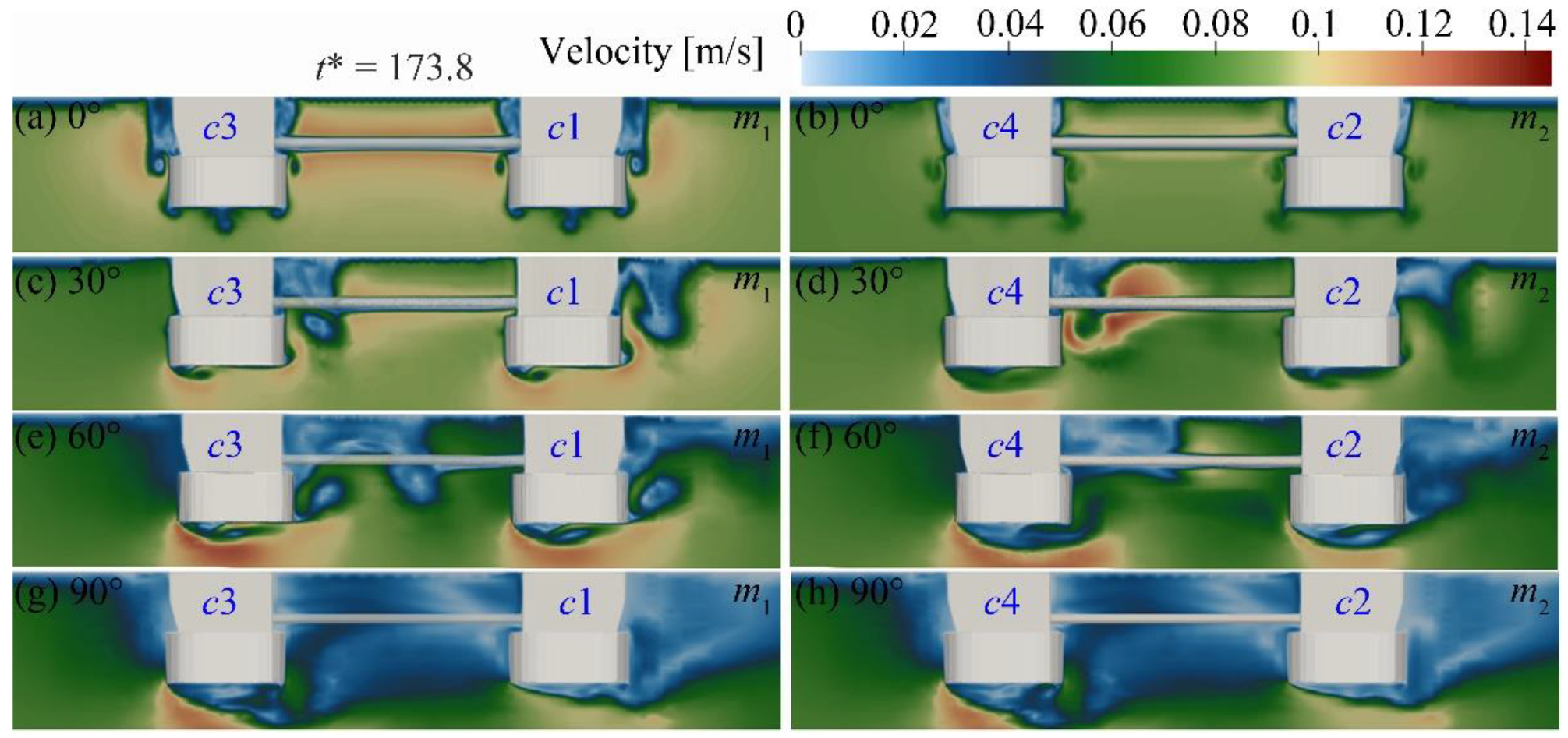
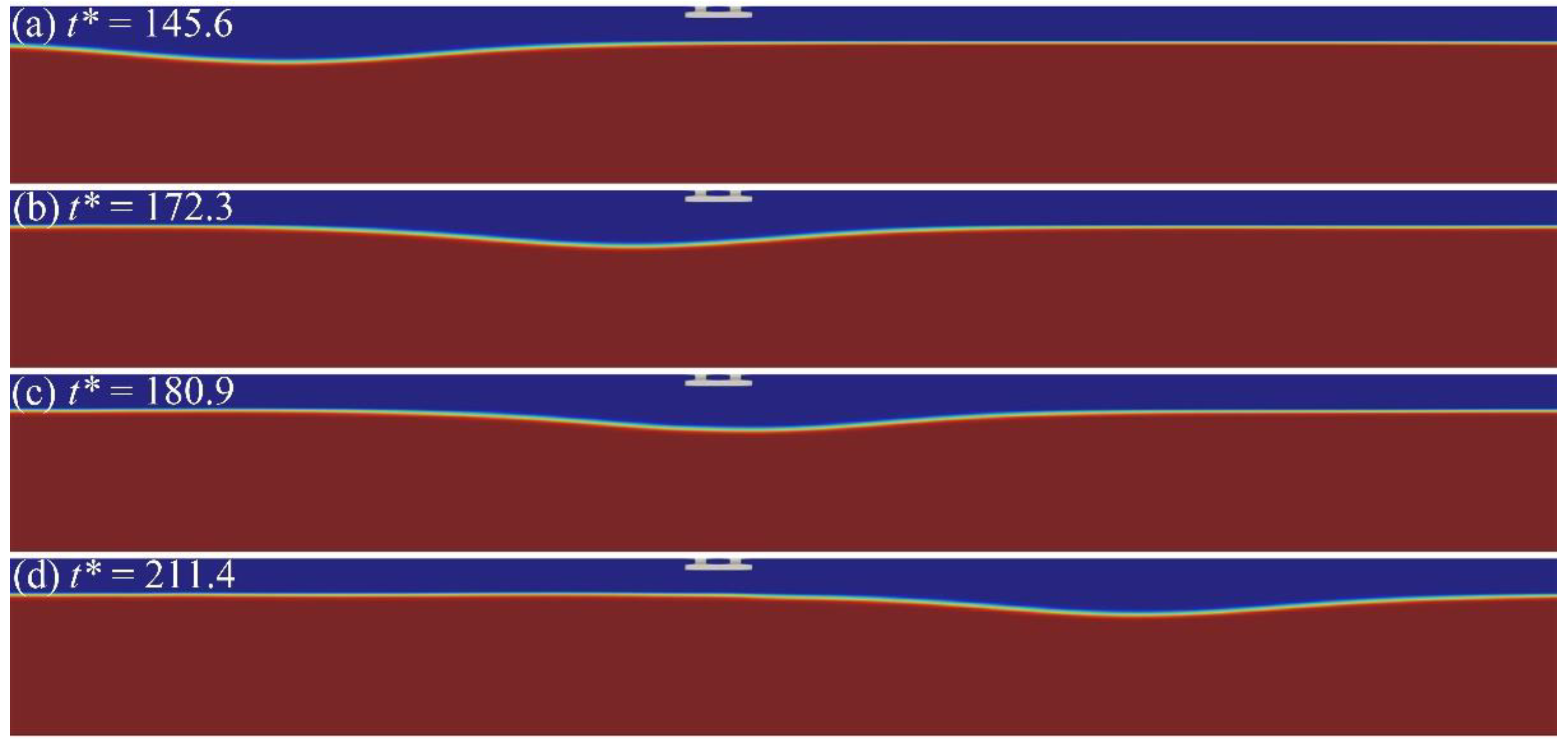
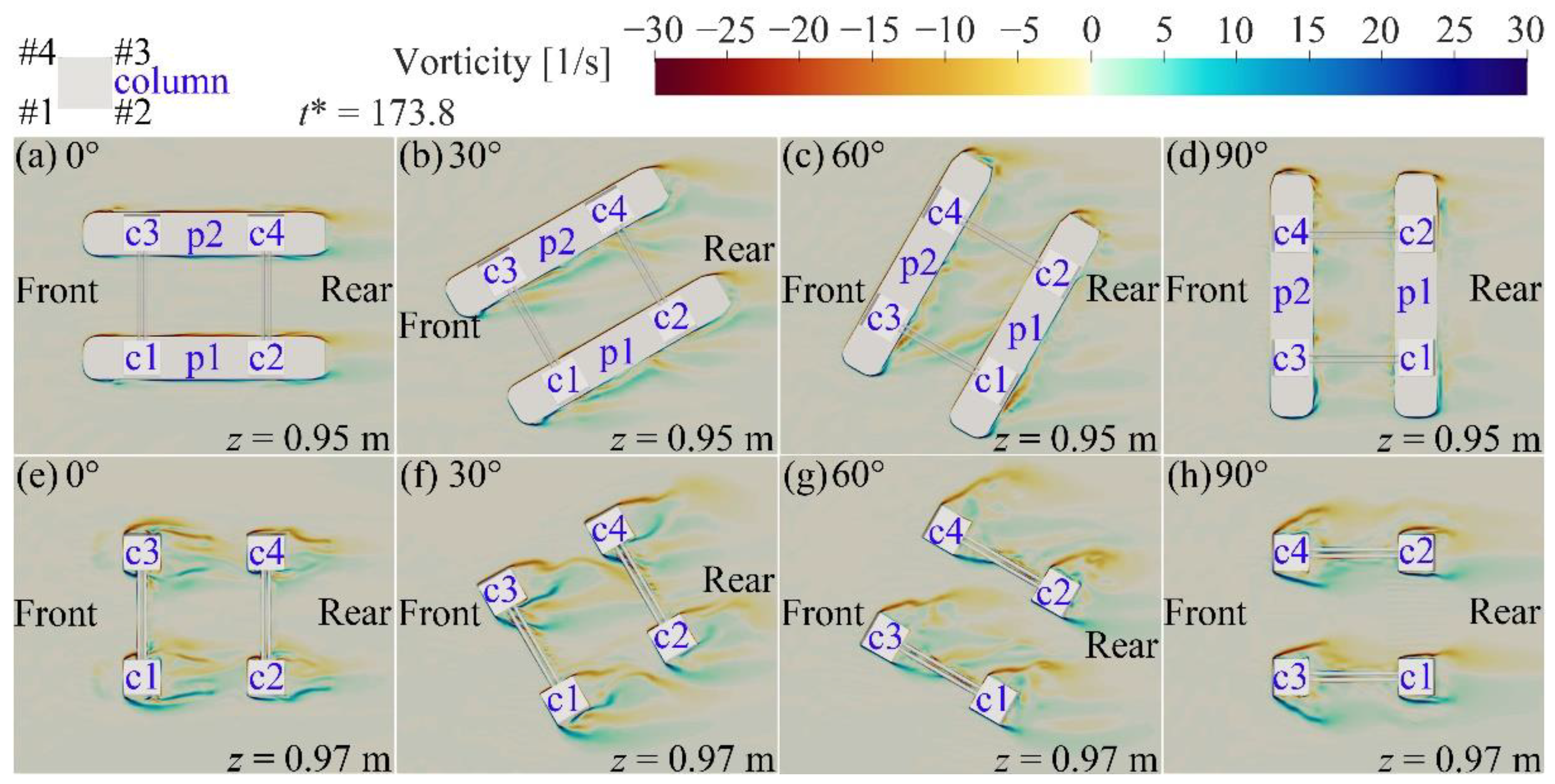
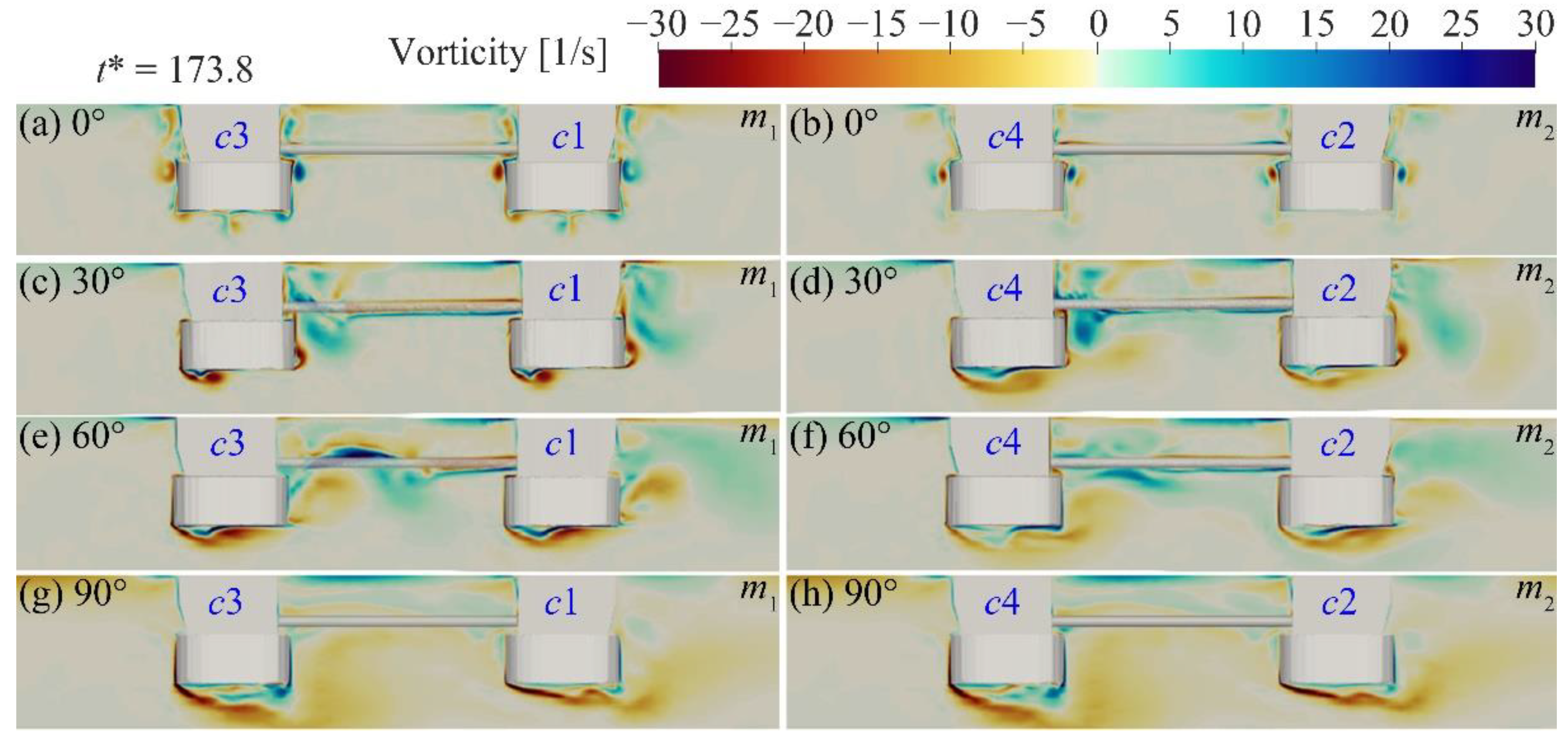
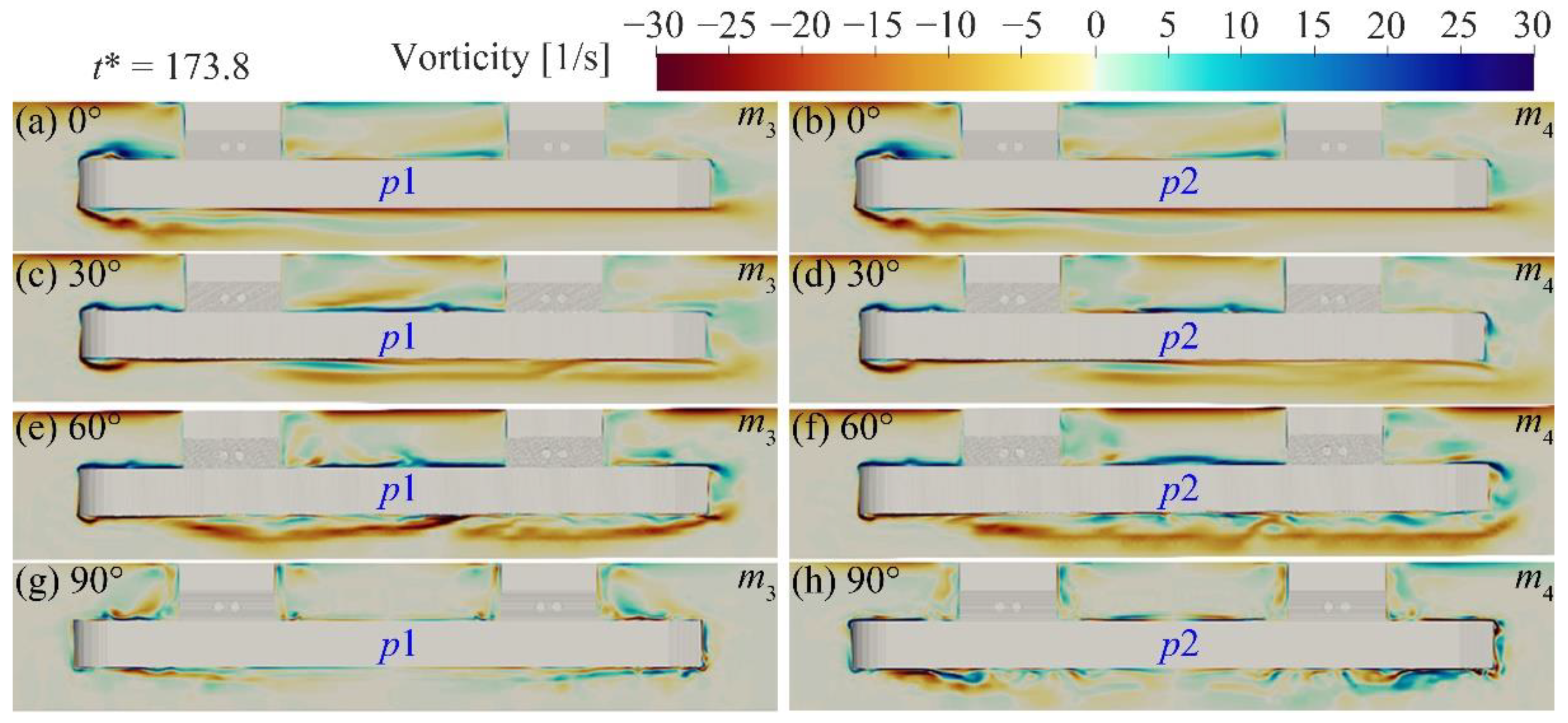
4.2. Flow Field around the Platform Located at Different Angles
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
Appendix A. The Velocity Field around the Platform Located at 0°
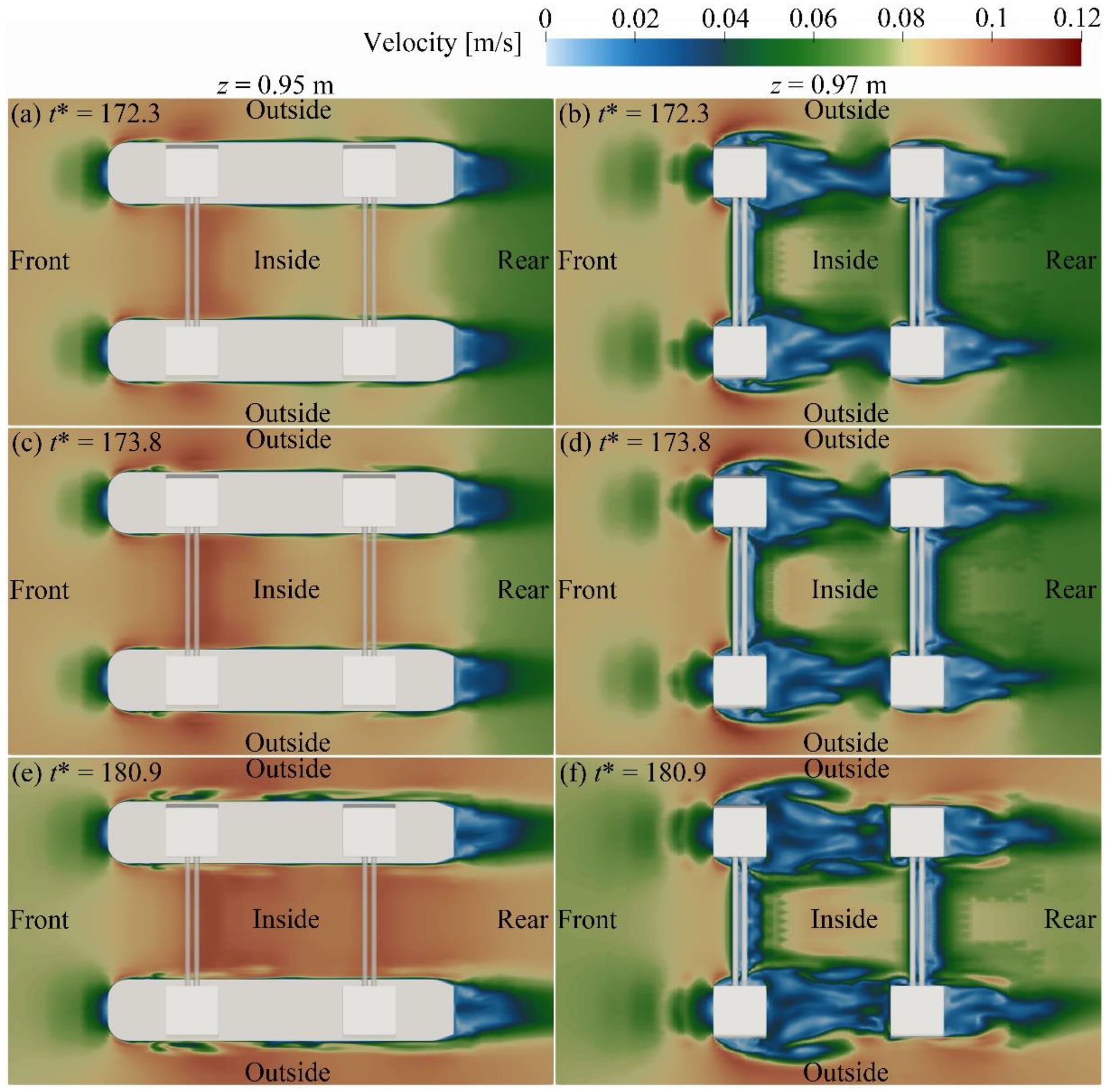
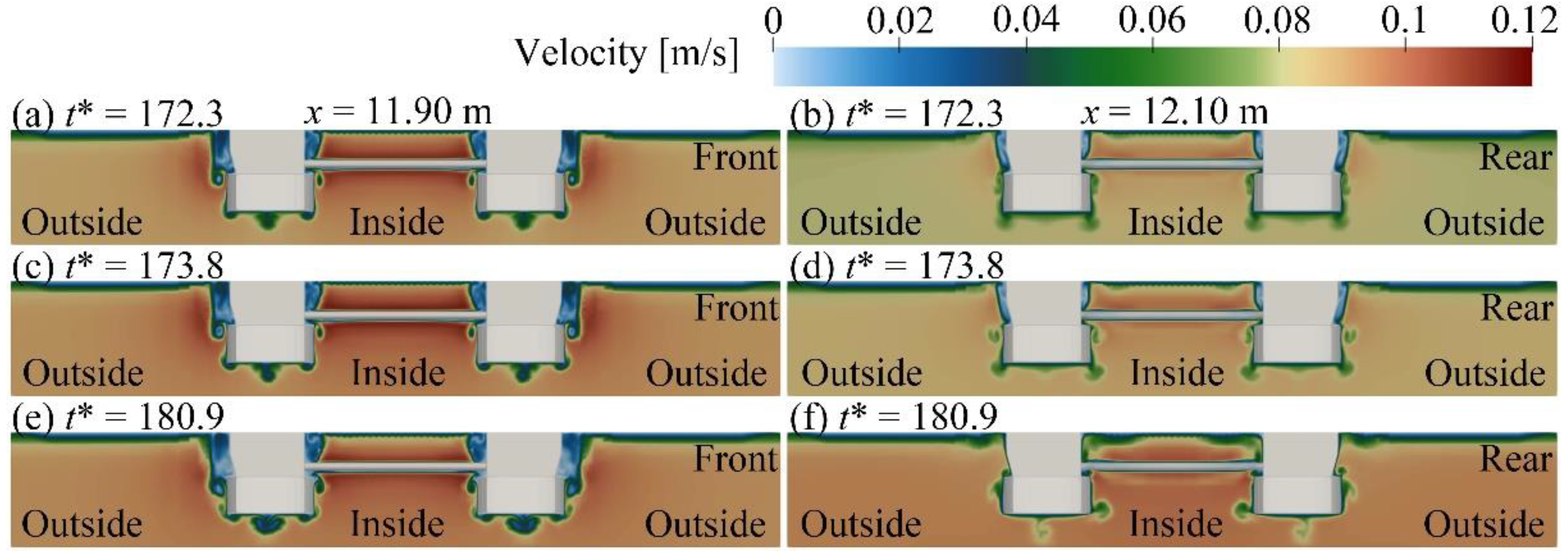
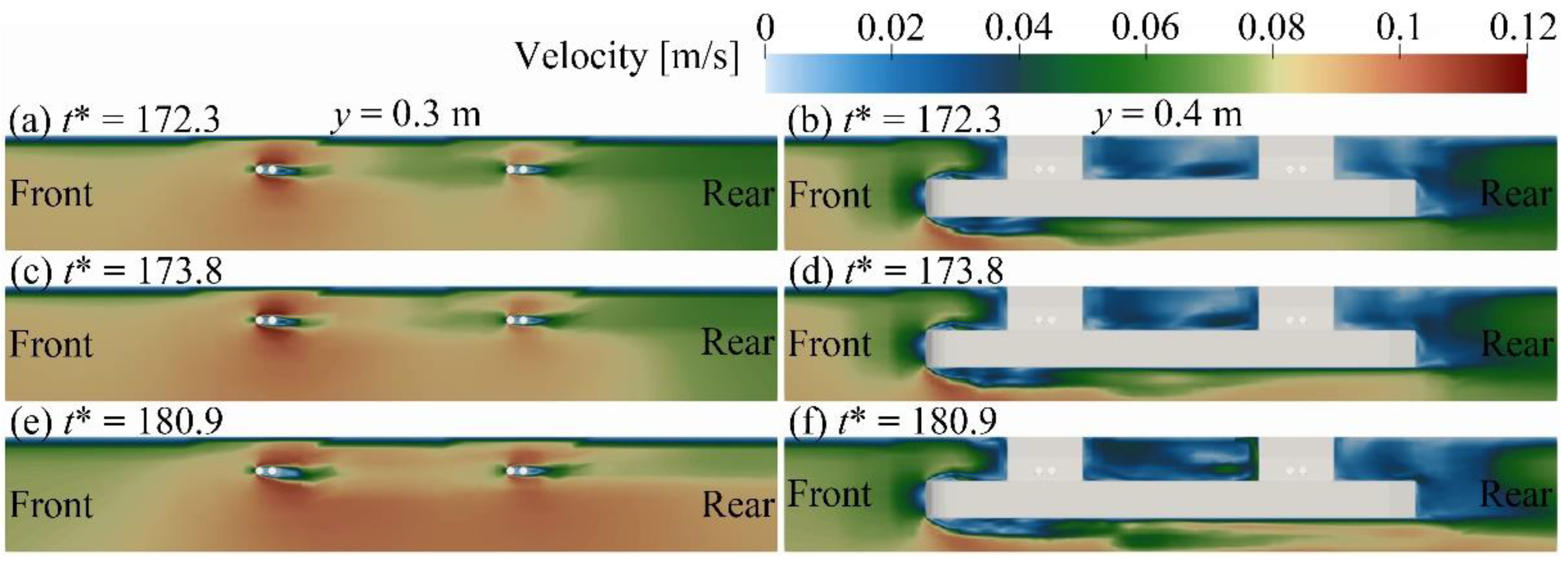
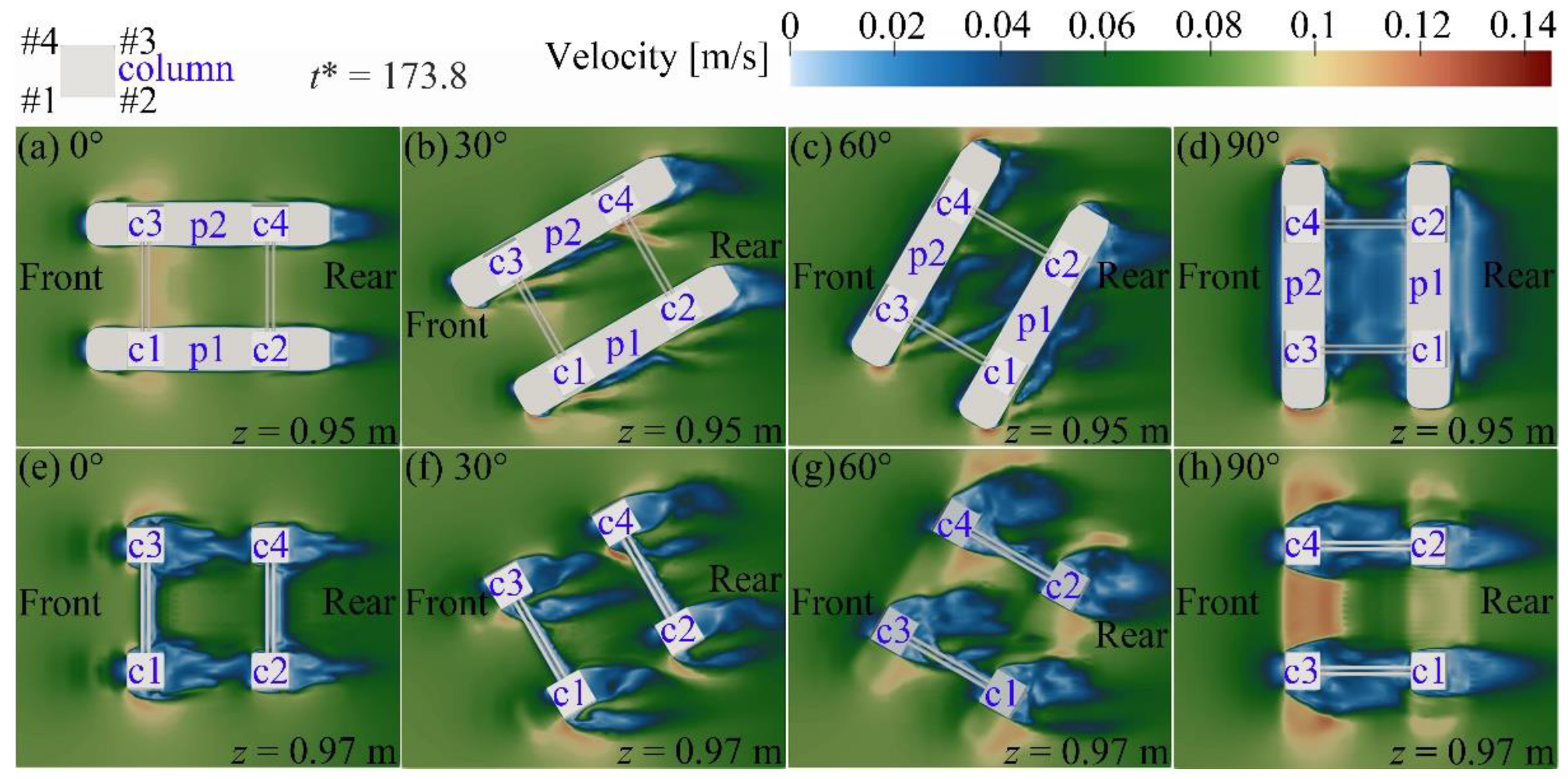
Appendix B. The Velocity Field around the Platform Located at Different Angles

References
- Bole, J.; Ebbesmeyer, C.; Romea, R. Soliton currents in the South China Sea: Measurements and theoretical modeling. In Proceedings of the Offshore Techn ology Conference: Offshore Technology Conference, Houston, TX, USA, 2–5 May 1994. [Google Scholar]
- Roberts, J. Internal Gravity Waves in the Ocean; Alaska Univ College Inst Of Marine Science: Fairbanks, AK, USA, 1975. [Google Scholar]
- Song, Z.; Teng, B.; Gou, Y.; Lu, L.; Shi, Z.; Xiao, Y.; Qu, Y. Comparisons of internal solitary wave and surface wave actions on marine structures and their responses. Appl. Ocean. Res. 2011, 33, 120–129. [Google Scholar] [CrossRef]
- Osborne, A.R.; Burch, T.L. Internal Solitons in the Andaman Sea. Science 1980, 208, 451–460. [Google Scholar] [CrossRef] [PubMed]
- Ebbesmeyer, C.; Coomes, C.A.; Hamilton, R.; Kurrus, K.A.; Sullivan, T.C.; Salem, B.L.; Romea, R.D.; Bauer, R.J. New observations on internal waves (solitons) in the South China Sea using an acoustic Doppler current profiler. Mar. Technol. Soc. 91 Proc 1991, 11, 165–175. [Google Scholar]
- Zhang, H.; Li, J. Wave loading on floating platforms by internal solitary waves. In New Trends in Fluid Mechanics Research; Springer: Berlin, Germany, 2007; pp. 304–307. [Google Scholar]
- Faltinsen, O. Sea Loads on Ships and Offshore Structures; Cambridge University Press: Cambridge, UK, 1993. [Google Scholar]
- Kvittem, M.I.; Bachynski, E.E.; Moan, T. Effects of hydrodynamic modelling in fully coupled simulations of a semi-submersible wind turbine. Energy Procedia 2012, 24, 351–362. [Google Scholar] [CrossRef]
- Li, Y.; Dong, G.; Liu, H.; Sun, D. The reflection of oblique incident waves by breakwaters with double-layered perforated wall. Coast. Eng. 2003, 50, 47–60. [Google Scholar] [CrossRef]
- Faraci, C.; Cammaroto, B.; Cavallaro, L.; Foti, E. Wave reflection generated by caissons with internal rubble mound of variable slope. Coast. Eng. Proc. 2012, 1, 51. [Google Scholar] [CrossRef]
- Liu, Y.; Faraci, C. Analysis of orthogonal wave reflection by a caisson with open front chamber filled with sloping rubble mound. Coast. Eng. 2014, 91, 151–163. [Google Scholar] [CrossRef]
- Faraci, C.; Scandura, P.; Foti, E. Reflection of sea waves by combined caissons. J. Waterw. Port Coast. Ocean Eng. 2014, 141, 04014036. [Google Scholar] [CrossRef]
- Cai, S.; Long, X.; Gan, Z. A method to estimate the forces exerted by internal solitons on cylindrical piles. Ocean Eng. 2003, 30, 673–689. [Google Scholar] [CrossRef]
- Cai, S.; Long, X.; Wang, S. Forces and torques exerted by internal solitons in shear flows on cylindrical piles. Appl. Ocean Res. 2008, 30, 72–77. [Google Scholar] [CrossRef]
- Cai, S.; Wang, S.; Long, X. A simple estimation of the force exerted by internal solitons on cylindrical piles. Ocean Eng. 2006, 33, 974–980. [Google Scholar] [CrossRef]
- Xie, J.; Jian, Y.; Yang, L. Strongly nonlinear internal soliton load on a small vertical circular cylinder in two-layer fluids. Appl. Math. Model. 2010, 34, 2089–2101. [Google Scholar] [CrossRef]
- Si, Z.; Zhang, Y.; Fan, Z. A numerical simulation of shear forces and torques exerted by large-amplitude internal solitary waves on a rigid pile in South China Sea. Appl. Ocean Res. 2012, 37, 127–132. [Google Scholar] [CrossRef]
- Xu, Z.; Yin, B.; Yang, H.; Qi, J. Depression and elevation internal solitary waves in a two-layer fluid and their forces on cylindrical piles. Chin. J. Oceanol. Limnol. 2012, 30, 703–712. [Google Scholar] [CrossRef]
- Chen, M.; Chen, K.; You, Y.-X. Experimental investigation of internal solitary wave forces on a semi-submersible. Ocean Eng. 2017, 141, 205–214. [Google Scholar] [CrossRef]
- Chen, M.; Chen, K.; You, Y.-X.; Yu, H.-T. Experimental study of forces on a multi-column floating platform in internal solitary waves. Appl. Ocean Res. 2018, 7, 192–200. [Google Scholar] [CrossRef]
- Wang, X.; Zhou, J.-F.; Wang, Z.; You, Y.-X. A numerical and experimental study of internal solitary wave loads on semi-submersible platforms. Ocean Eng. 2018, 150, 298–308. [Google Scholar] [CrossRef]
- Shih, T.-H.; Zhu, J.; Lumley, J.L. A realizable Reynolds stress algebraic equation model. In Proceedings of the A Realizable Reynolds Stress Algebraic Equation Model, Koyoto, Japan, 10–18 August 1993. [Google Scholar]
- Brown, S.A.; Magar, V.; Greaves, D.M.; Conley, D.C. An evaluation of rans turbulence clousure models for spilling breakers. Coast. Eng. Proc. 2014, 1, 5. [Google Scholar] [CrossRef][Green Version]
- Yakhot, V.; Orszag, S.; Thangam, S.; Gatski, T.; Speziale, C. Development of turbulence models for shear flows by a double expansion technique. Phys. Fluids A Fluid Dyn. 1992, 4, 1510–1520. [Google Scholar] [CrossRef]
- Michallet, H.; Barthelemy, E. Experimental study of interfacial solitary waves. J. Fluid Mech. 1998, 366, 159–177. [Google Scholar] [CrossRef]
- Tyvand, J.D. Computational Fluid Dynamics Simulations of Gravity Wave Flows; University of Oslo: Oslo, Norway, 2017. [Google Scholar]
- Meng, Q.; Zhang, C. A third-order KdV solution for internal solitary waves and its application in the numerical wave tank. J. Ocean Eng. Sci. 2016, 1, 93–108. [Google Scholar] [CrossRef]
- Jasak, H. Error Analysis and Estimation for the Finite Volume Method with Applications to Fluid Flows; Imperial College London: London, UK, 1996. [Google Scholar]
- Harten, A. High resolution schemes for hyperbolic conservation laws. J. Comput. Phys. 1983, 49, 357–393. [Google Scholar] [CrossRef]
- Ai, C.; Ding, W. A 3D unstructured non-hydrostatic ocean model for internal waves. Ocean Dyn. 2016, 66, 1253–1270. [Google Scholar] [CrossRef]
- Koop, C.G.; Butler, G. An investigation of internal solitary waves in a two-fluid system. J. Fluid Mech. 1981, 112, 225–251. [Google Scholar] [CrossRef]
- Jacobsen, N.G.; Fuhrman, D.R.; Fredsøe, J. A wave generation toolbox for the open-source CFD library: OpenFoam®. Int. J. Numer. Methods Fluids. 2012, 70, 1073–1088. [Google Scholar] [CrossRef]
- Chen, C.-Y.; Hsu, J.R.-C.; Cheng, M.-H.; Chen, H.-H.; Kuo, C.-F. An investigation on internal solitary waves in a two-layer fluid: Propagation and reflection from steep slopes. Ocean Eng. 2007, 34, 171–184. [Google Scholar] [CrossRef]
- Wessels, F.; Hutter, K. Interaction of internal waves with a topographic sill in a two-layered fluid. J. Phys. Oceanogr. 1996, 26, 5–20. [Google Scholar] [CrossRef][Green Version]
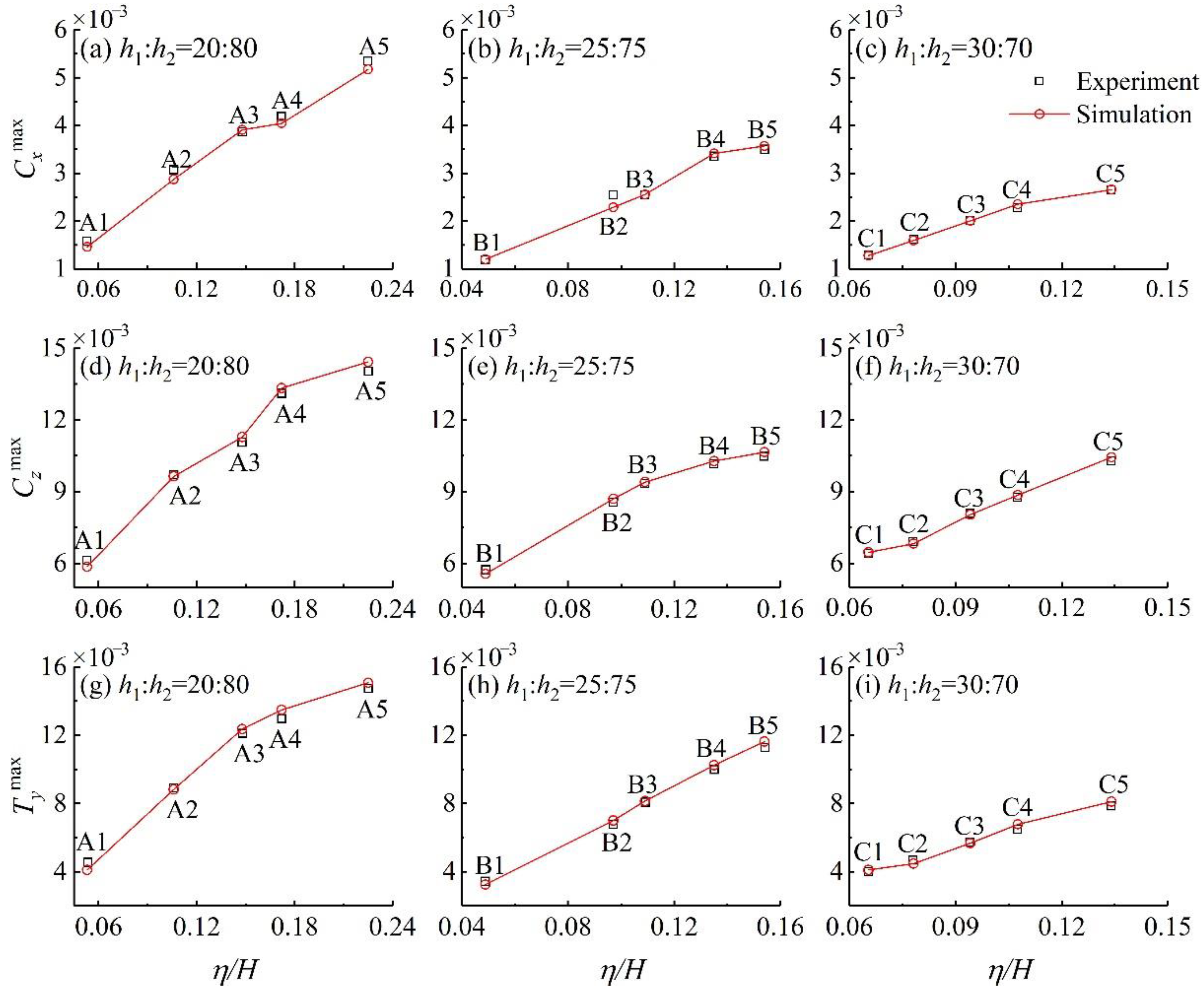

| Case No. | h1:h2 | η [m] | Case No. | h1:h2 | η [m] | Case No. | h1:h2 | η [m] |
|---|---|---|---|---|---|---|---|---|
| A1 | 20:80 | 0.053 | B1 | 25:75 | 0.049 | C1 | 30:70 | 0.065 |
| A2 | 0.106 | B2 | 0.097 | C2 | 0.078 | |||
| A3 | 0.148 | B3 | 0.109 | C3 | 0.094 | |||
| A4 | 0.172 | B4 | 0.135 | C4 | 0.108 | |||
| A5 | 0.225 | B5 | 0.154 | C5 | 0.134 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ding, W.; Ai, C.; Jin, S.; Lin, J. 3D Numerical Investigation of Forces and Flow Field around the Semi-Submersible Platform in An Internal Solitary Wave. Water 2020, 12, 208. https://doi.org/10.3390/w12010208
Ding W, Ai C, Jin S, Lin J. 3D Numerical Investigation of Forces and Flow Field around the Semi-Submersible Platform in An Internal Solitary Wave. Water. 2020; 12(1):208. https://doi.org/10.3390/w12010208
Chicago/Turabian StyleDing, Weiye, Congfang Ai, Sheng Jin, and Jinbo Lin. 2020. "3D Numerical Investigation of Forces and Flow Field around the Semi-Submersible Platform in An Internal Solitary Wave" Water 12, no. 1: 208. https://doi.org/10.3390/w12010208
APA StyleDing, W., Ai, C., Jin, S., & Lin, J. (2020). 3D Numerical Investigation of Forces and Flow Field around the Semi-Submersible Platform in An Internal Solitary Wave. Water, 12(1), 208. https://doi.org/10.3390/w12010208





