Hydrogeochemical Characteristics and Genesis of Geothermal Water from the Ganzi Geothermal Field, Eastern Tibetan Plateau
Abstract
:1. Introduction
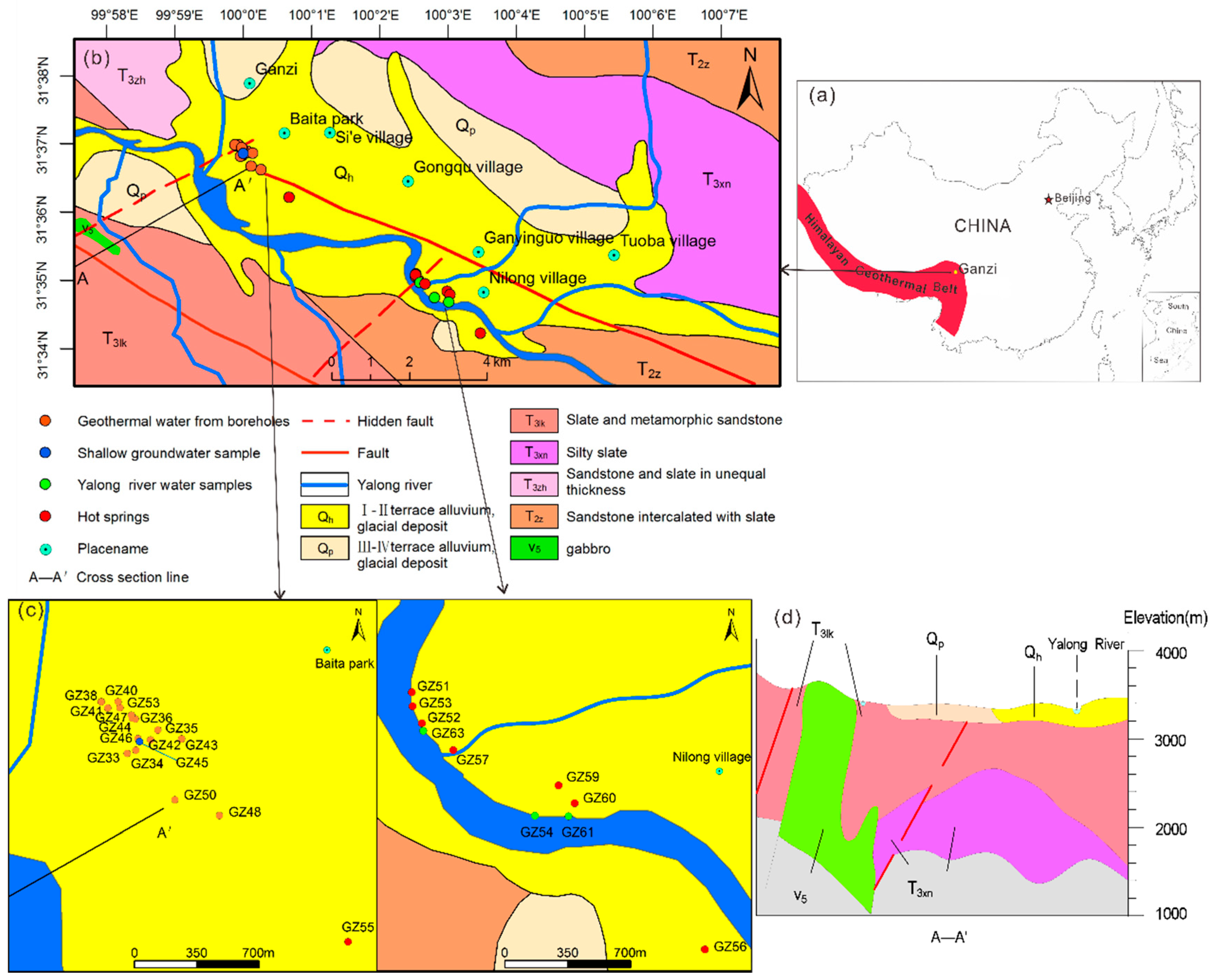
2. Geological Setting of the Study Area
3. Sampling and Analysis
4. Results and Discussion
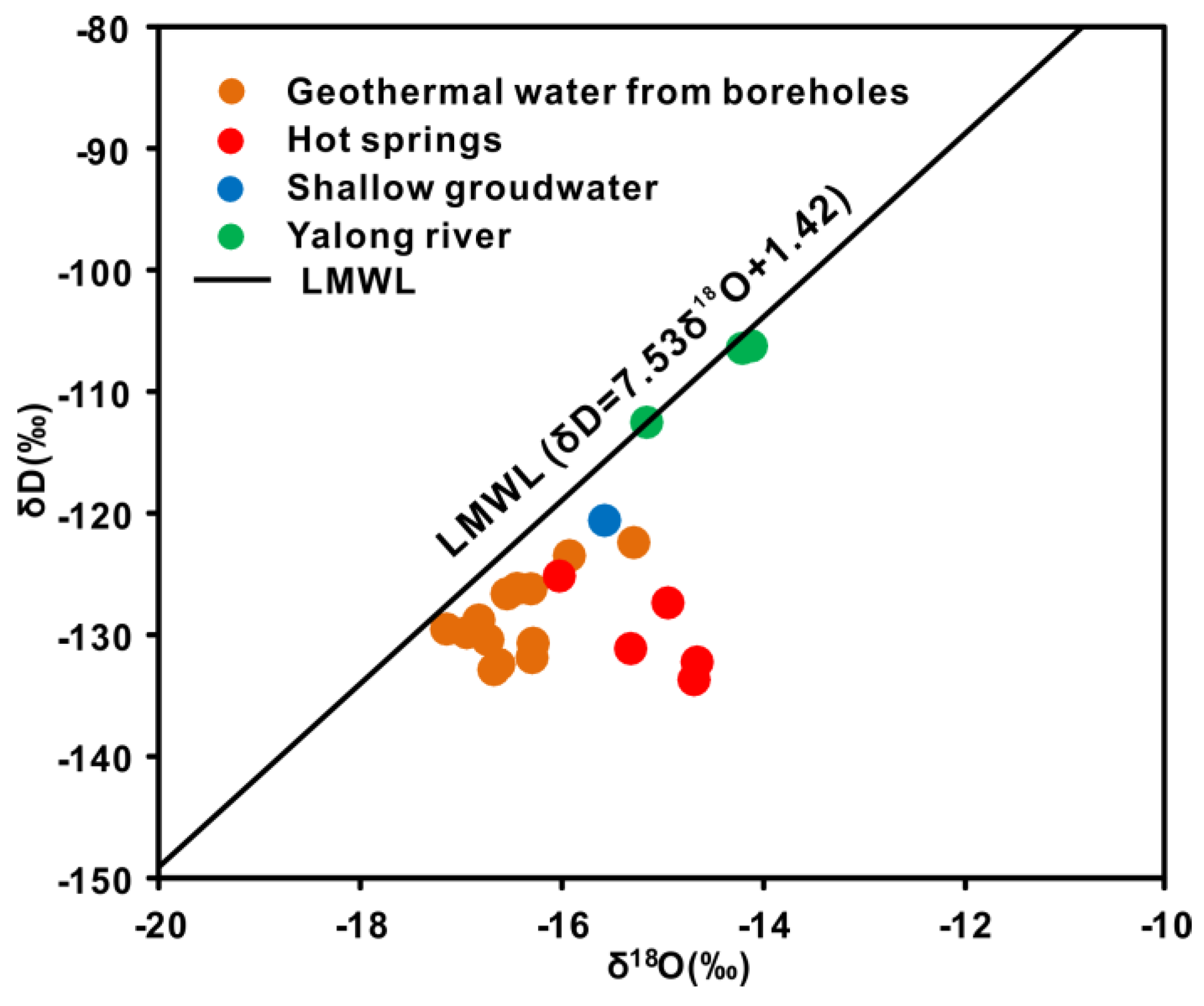
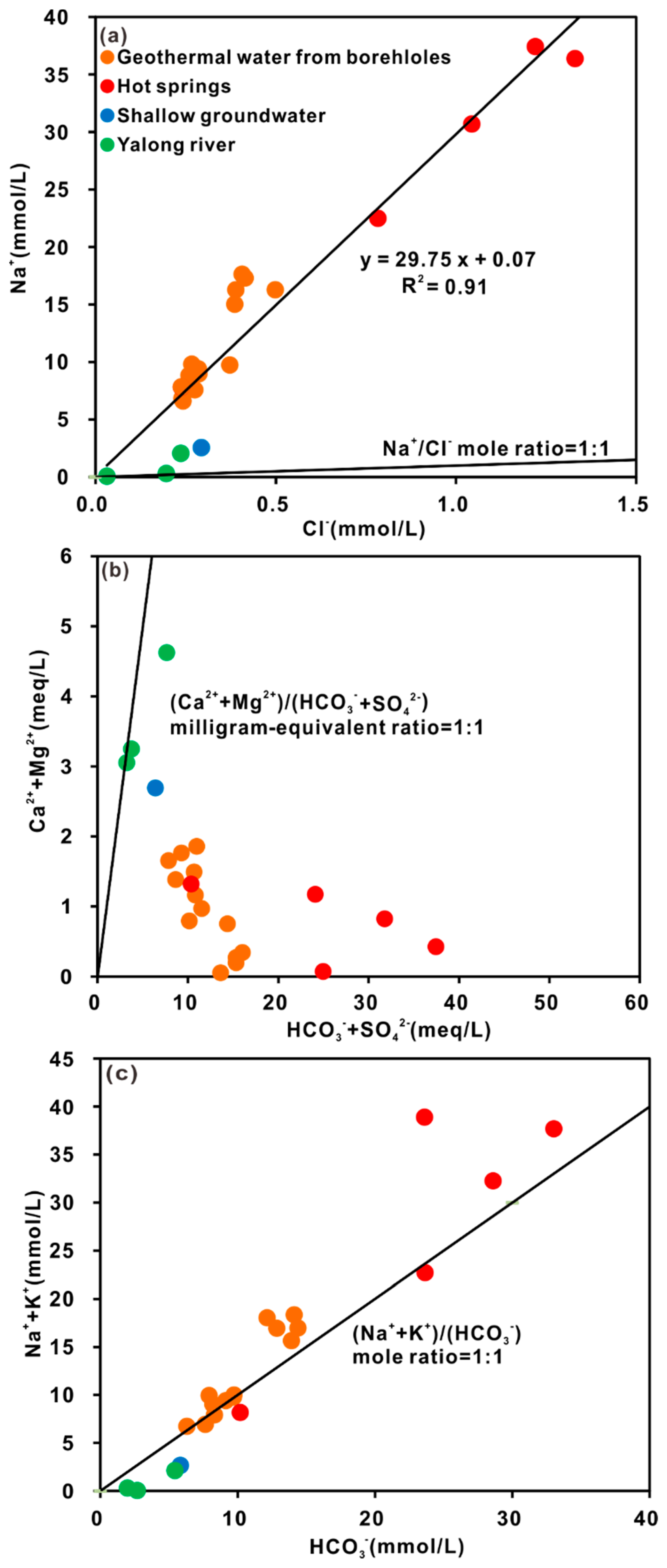
4.1. Origin and Recharge Source of Geothermal Water
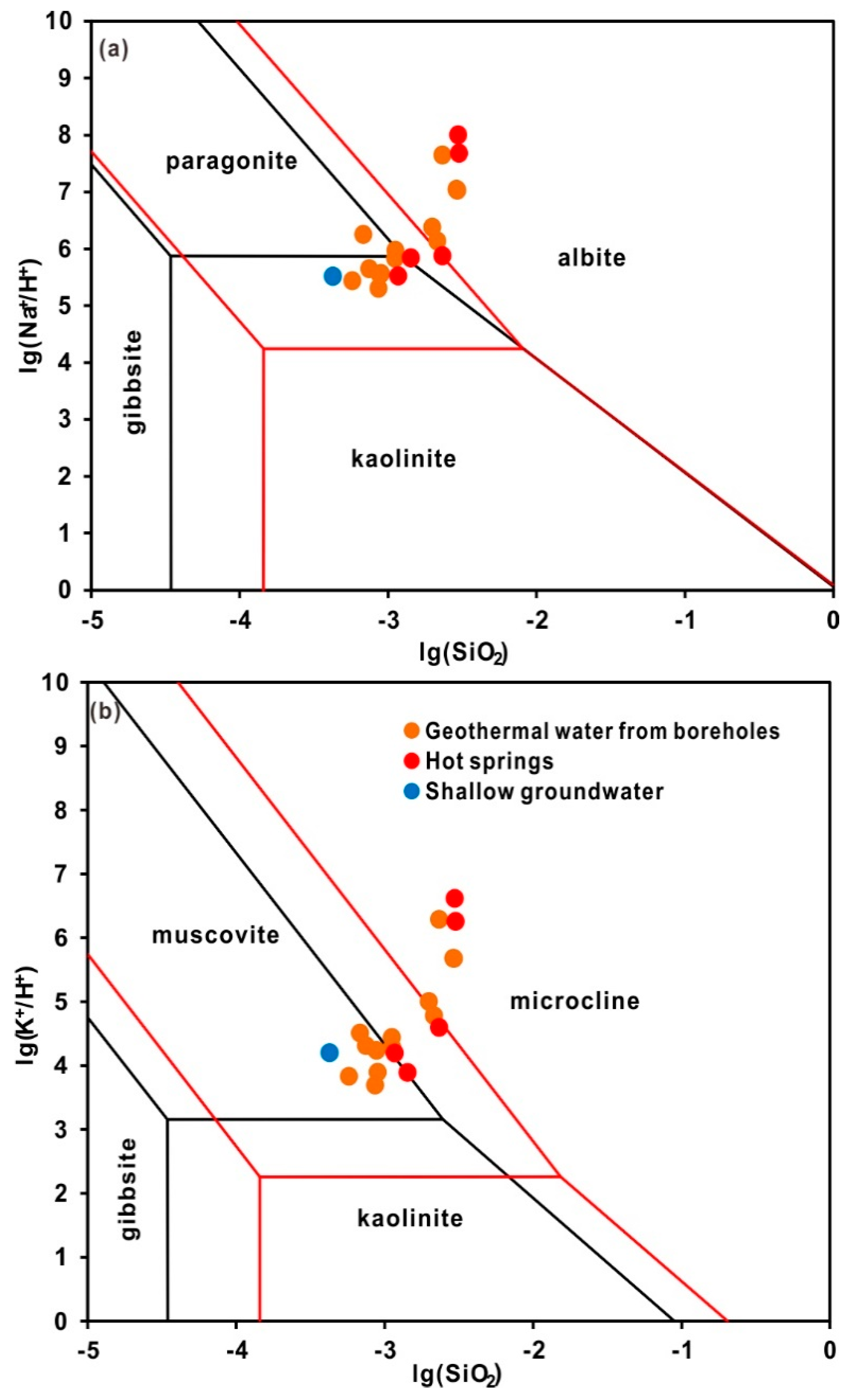
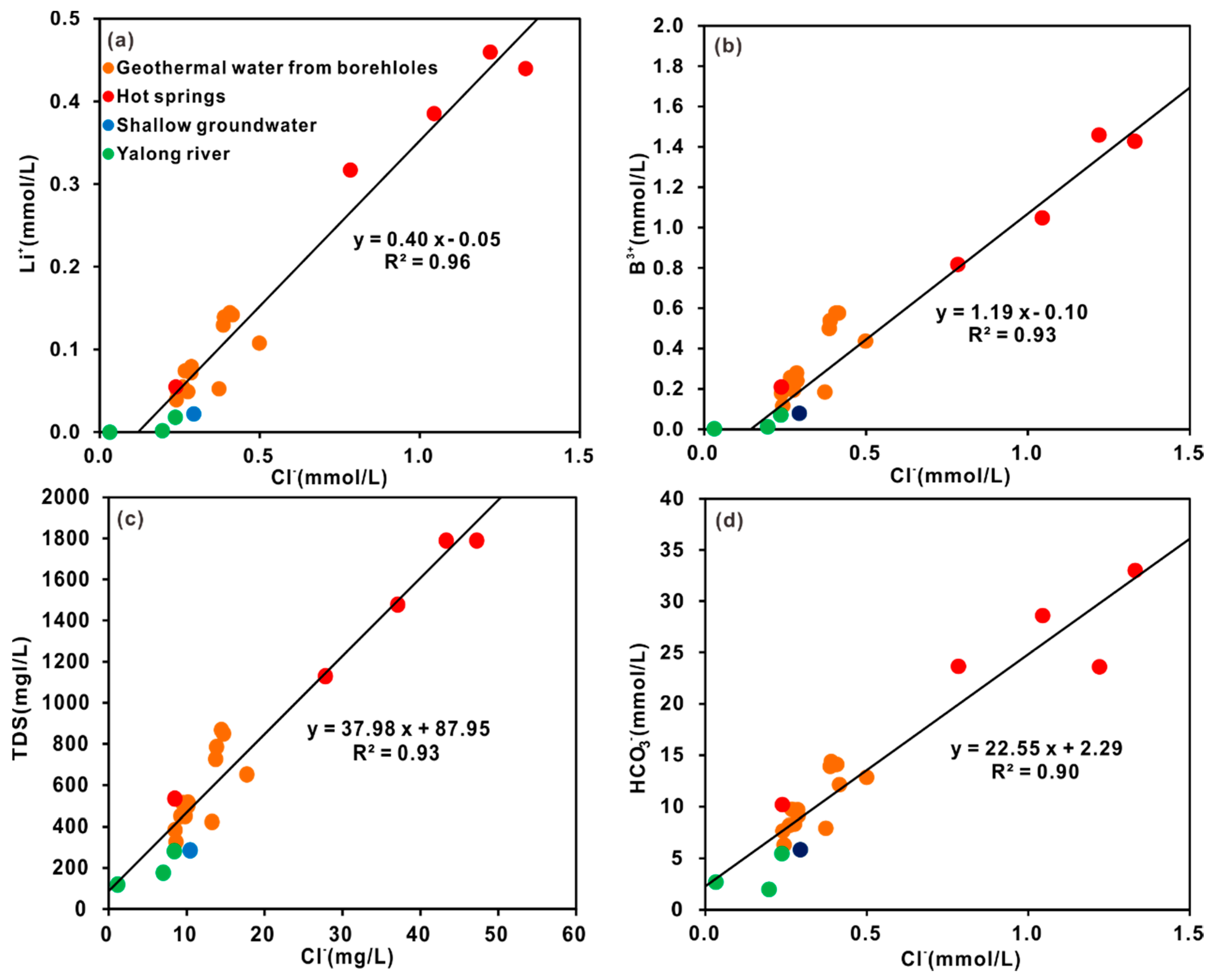
4.2. Genesis of Geothermal Water
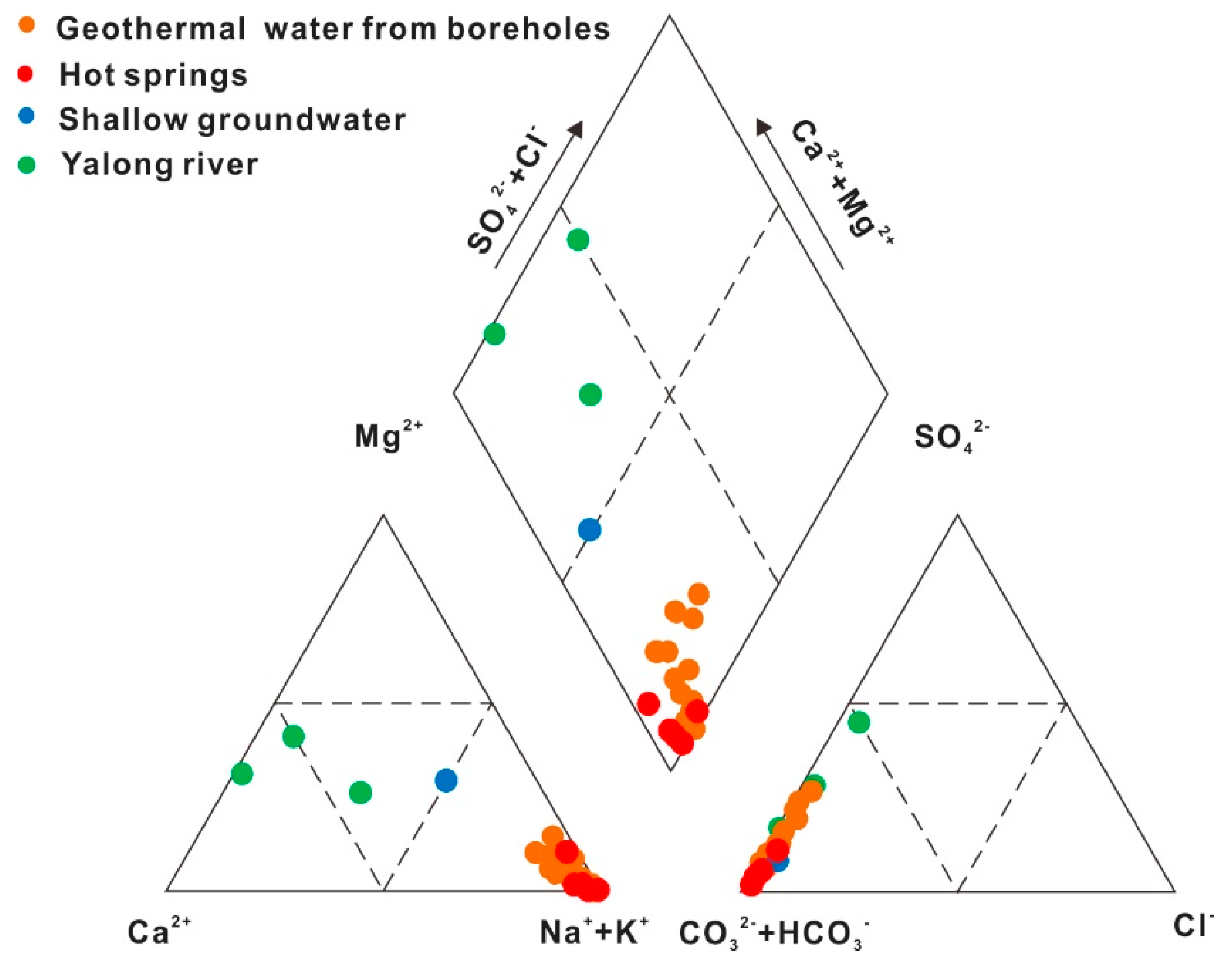
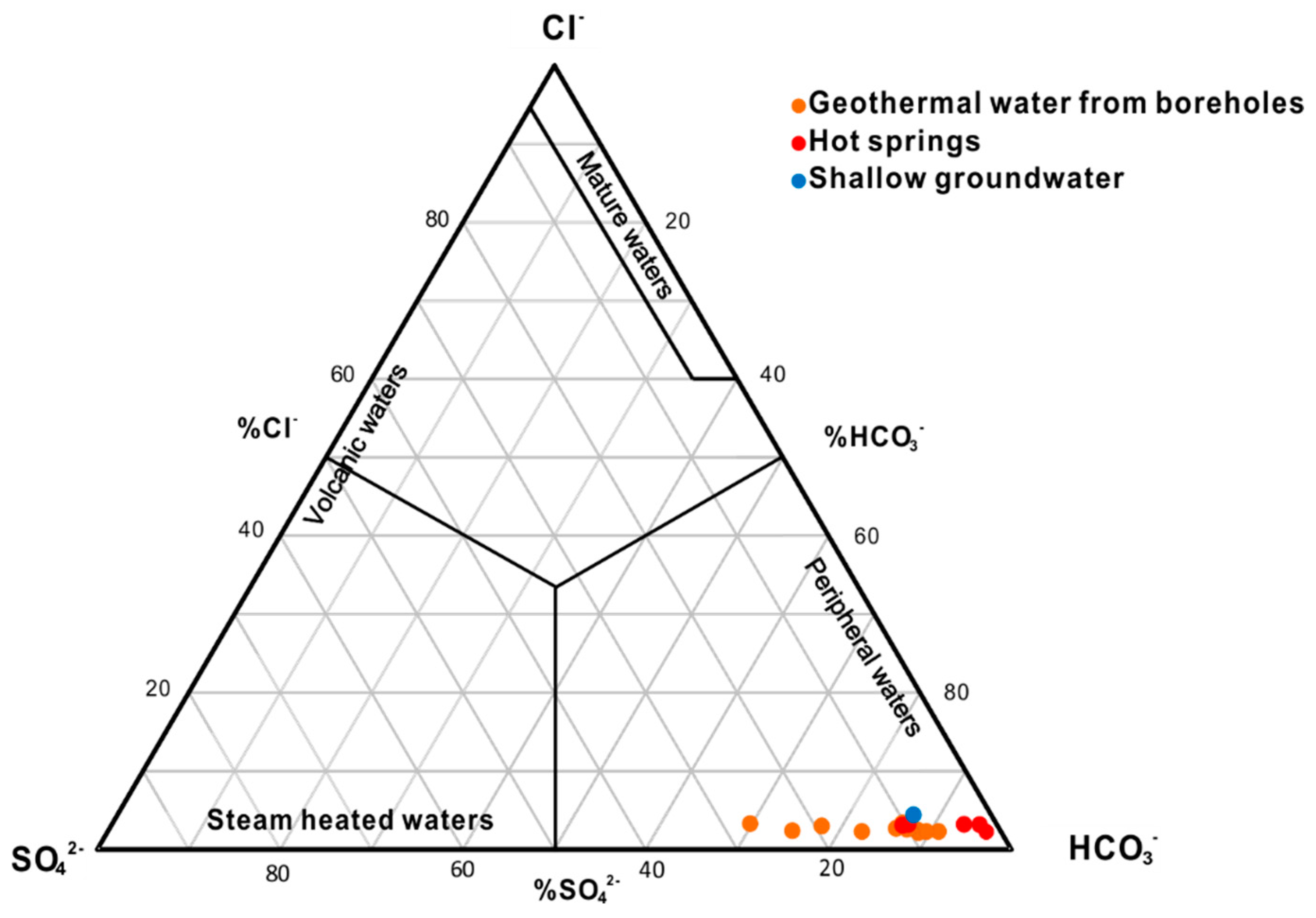
4.2.1. Hydrogeochemical Characteristics
4.2.2. Water-Rock Interaction and Mixing Process
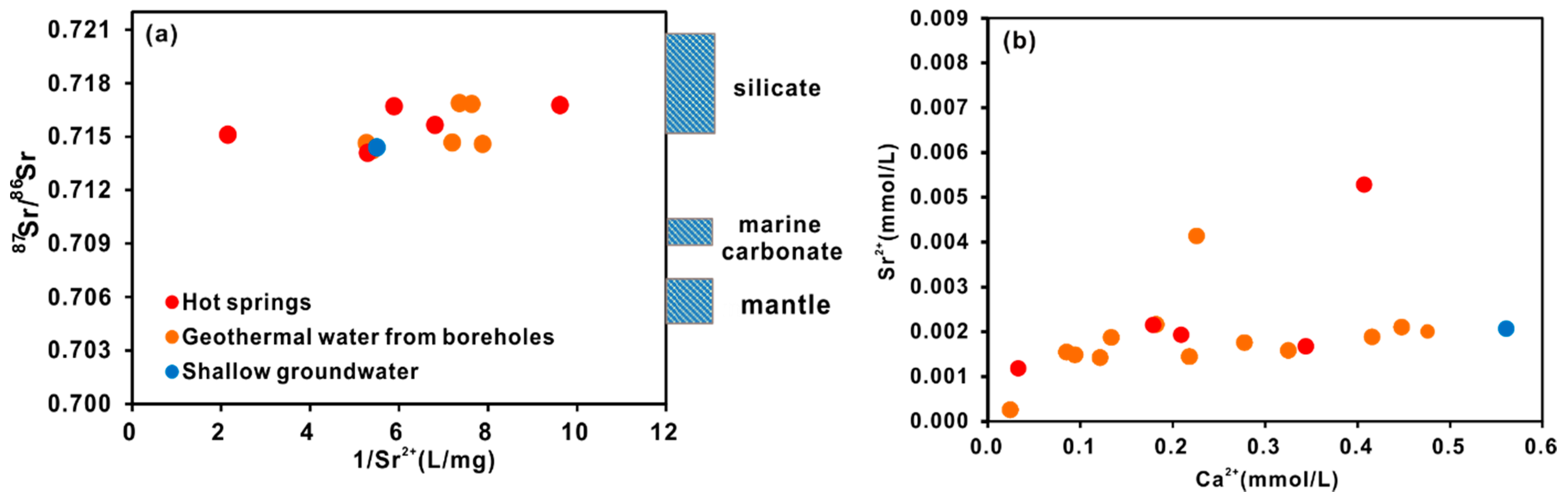
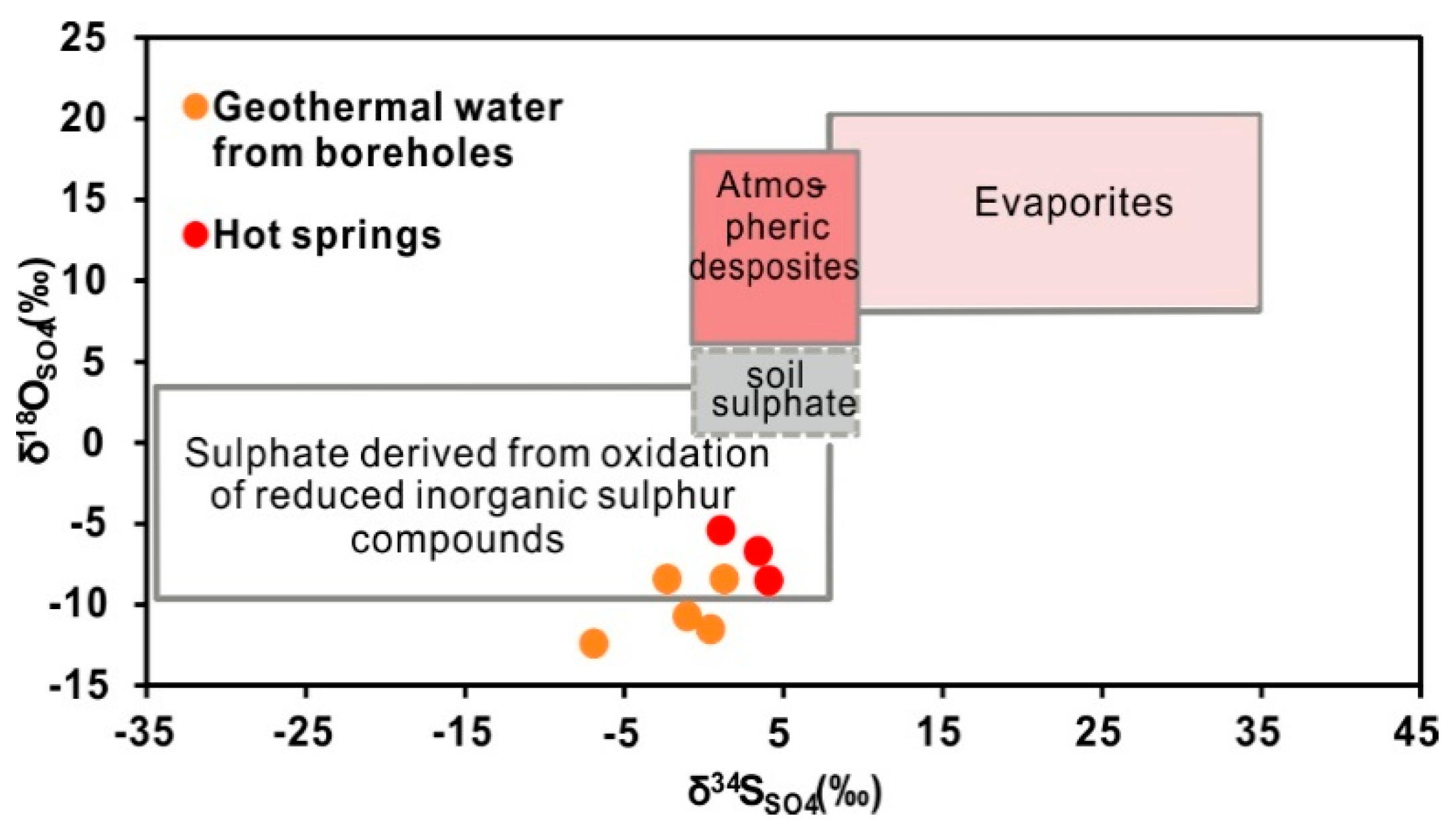
4.2.3. Constraints from 87Sr/86Sr and δ34SSO4
4.3. Origin of Geothermal Gases
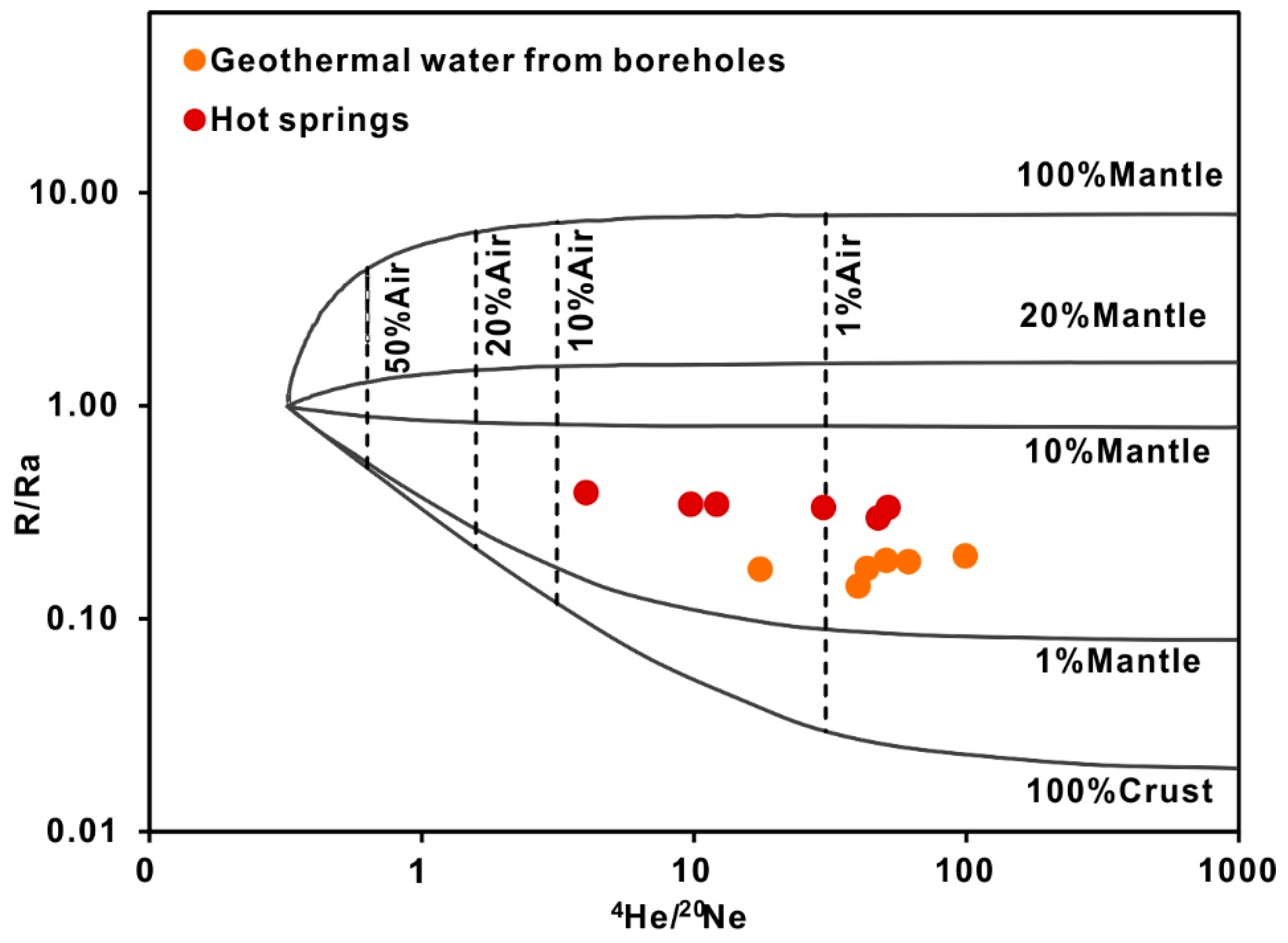
4.3.1. Helium Isotope
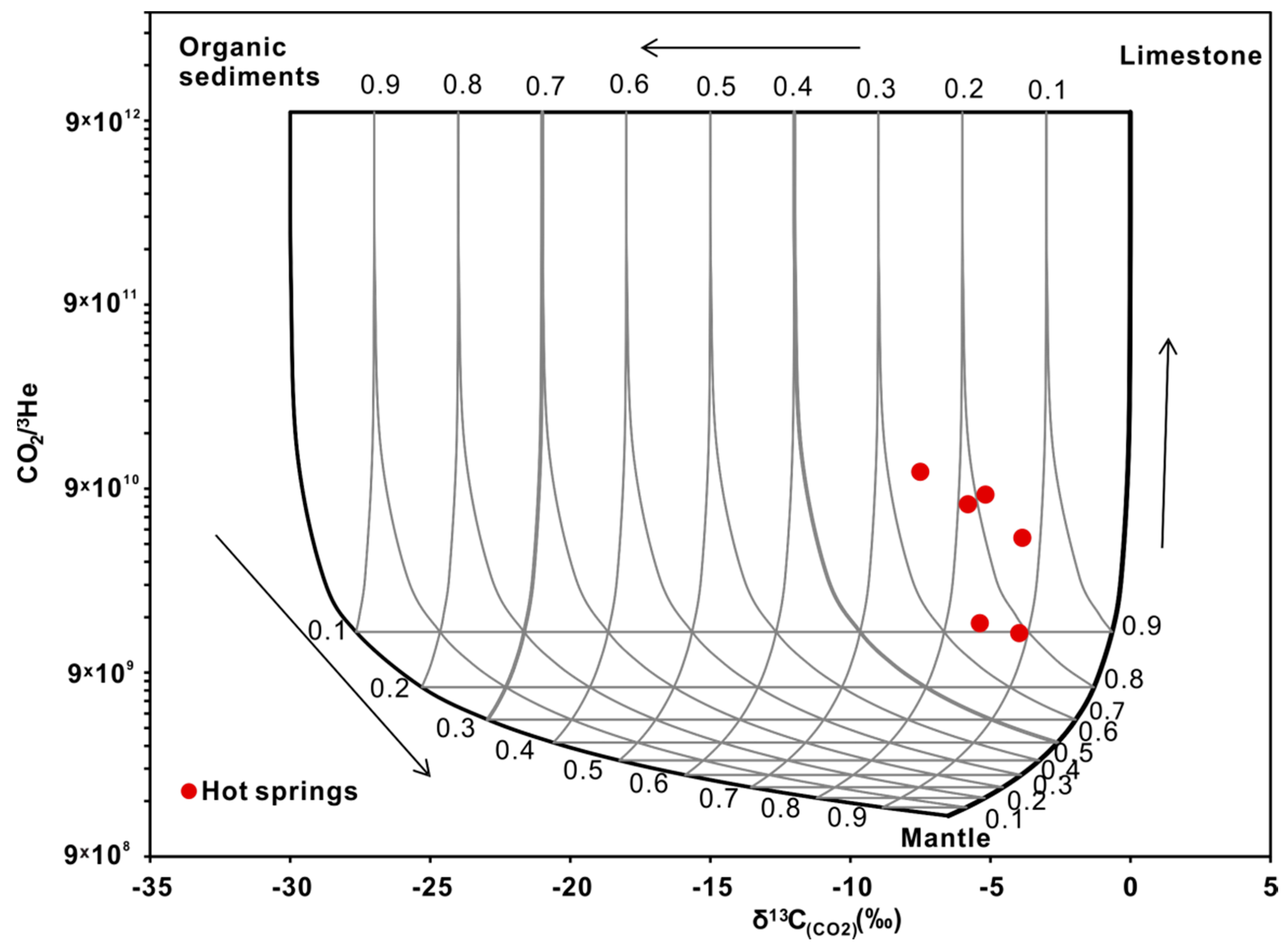
4.3.2. Carbon Isotopes
4.4. Geothermal Reservoir Temperature
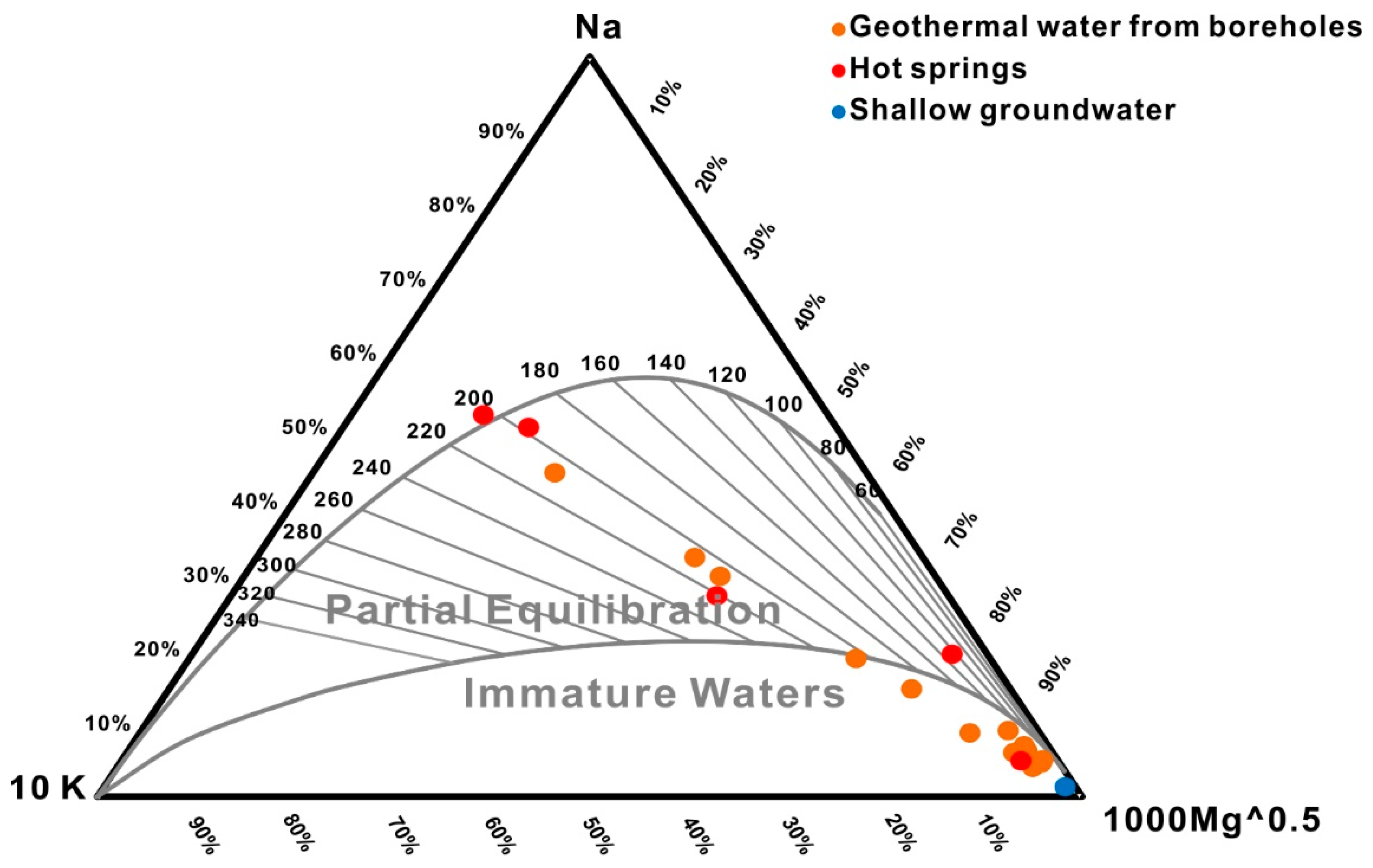
4.4.1. Chemical Geothermometers
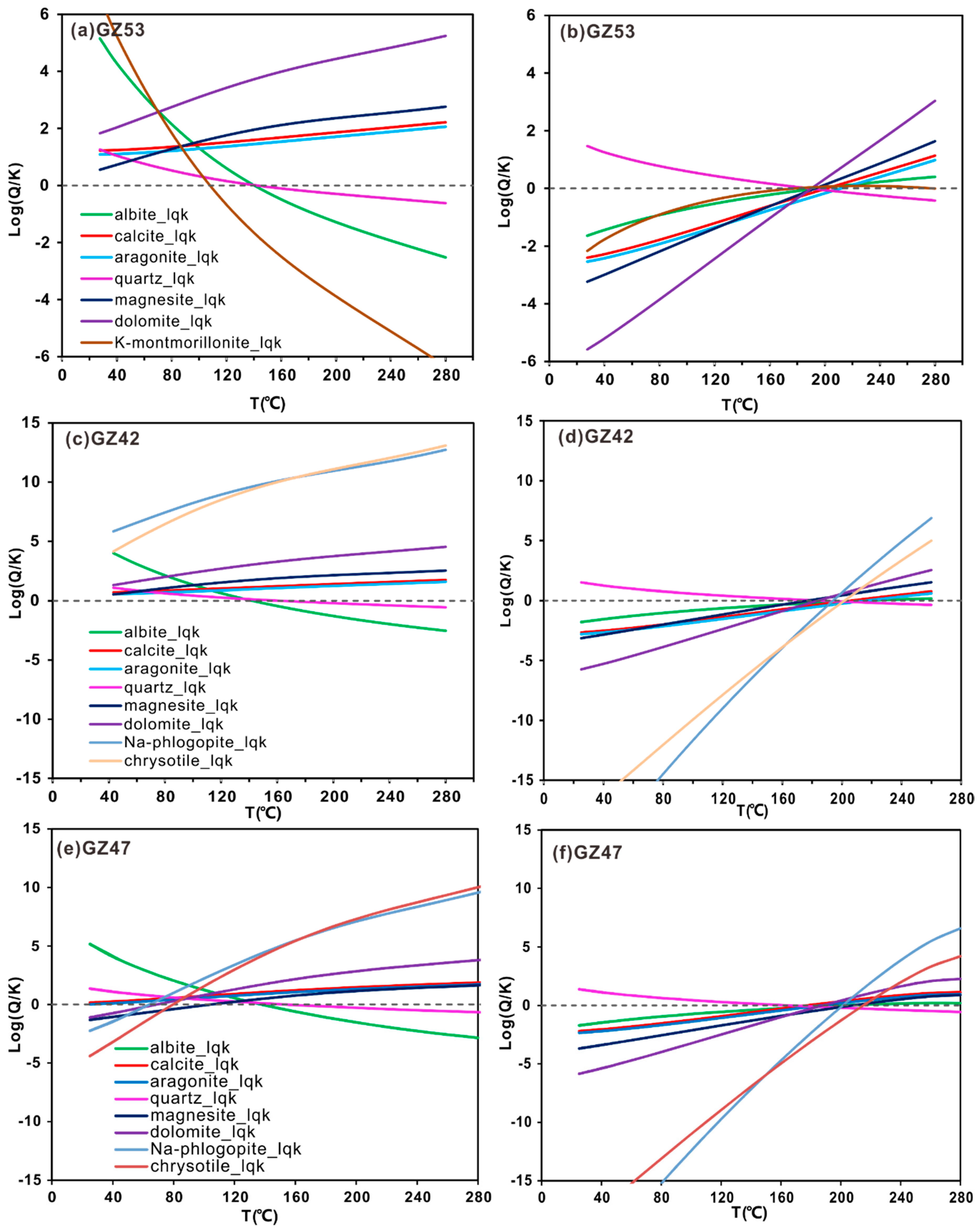
4.4.2. Geothermometrical Modelling
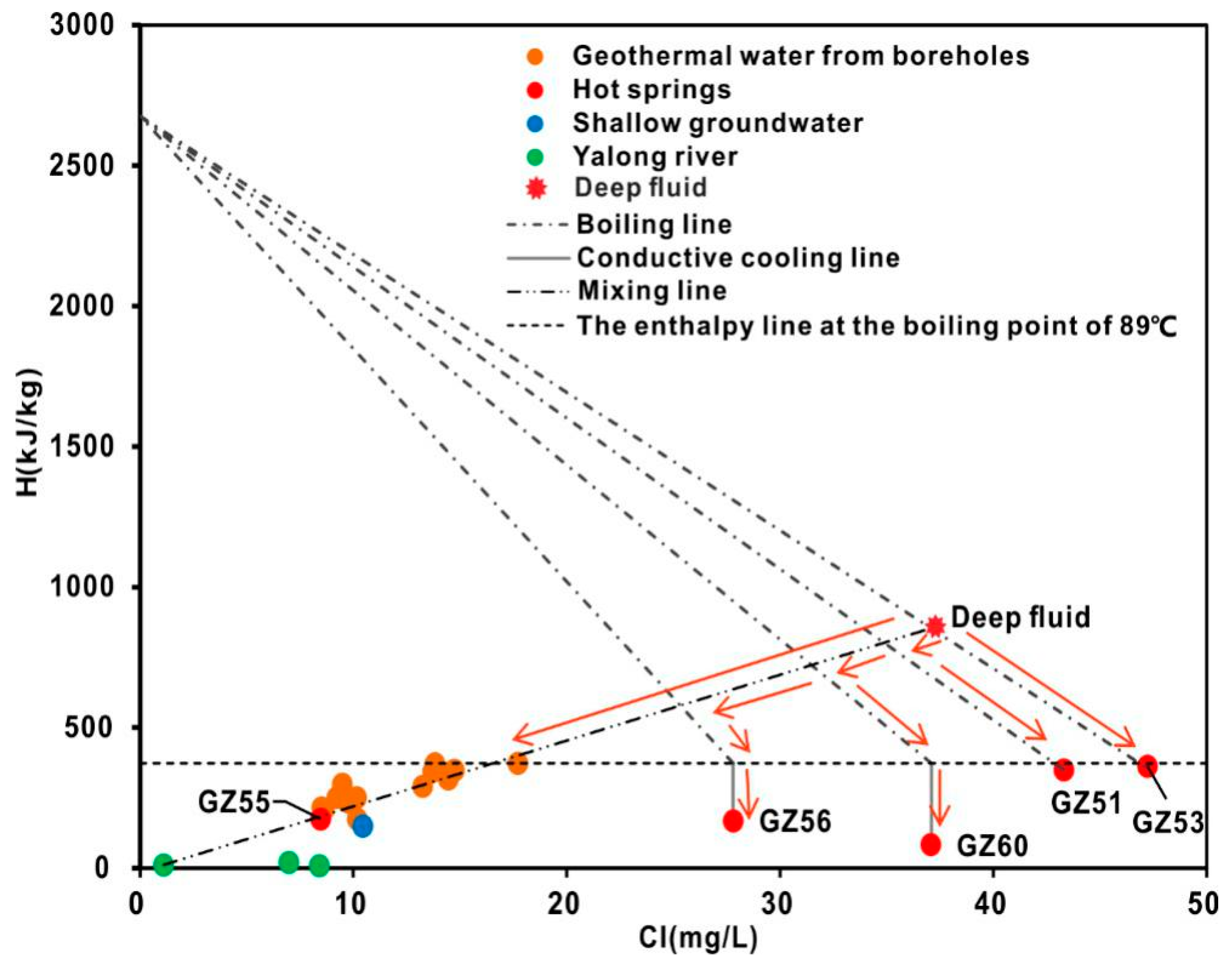
4.4.3. Cl-Enthalpy Mixing Model
4.5. Geothermal Water Circulation Depth
4.6. Heat Source
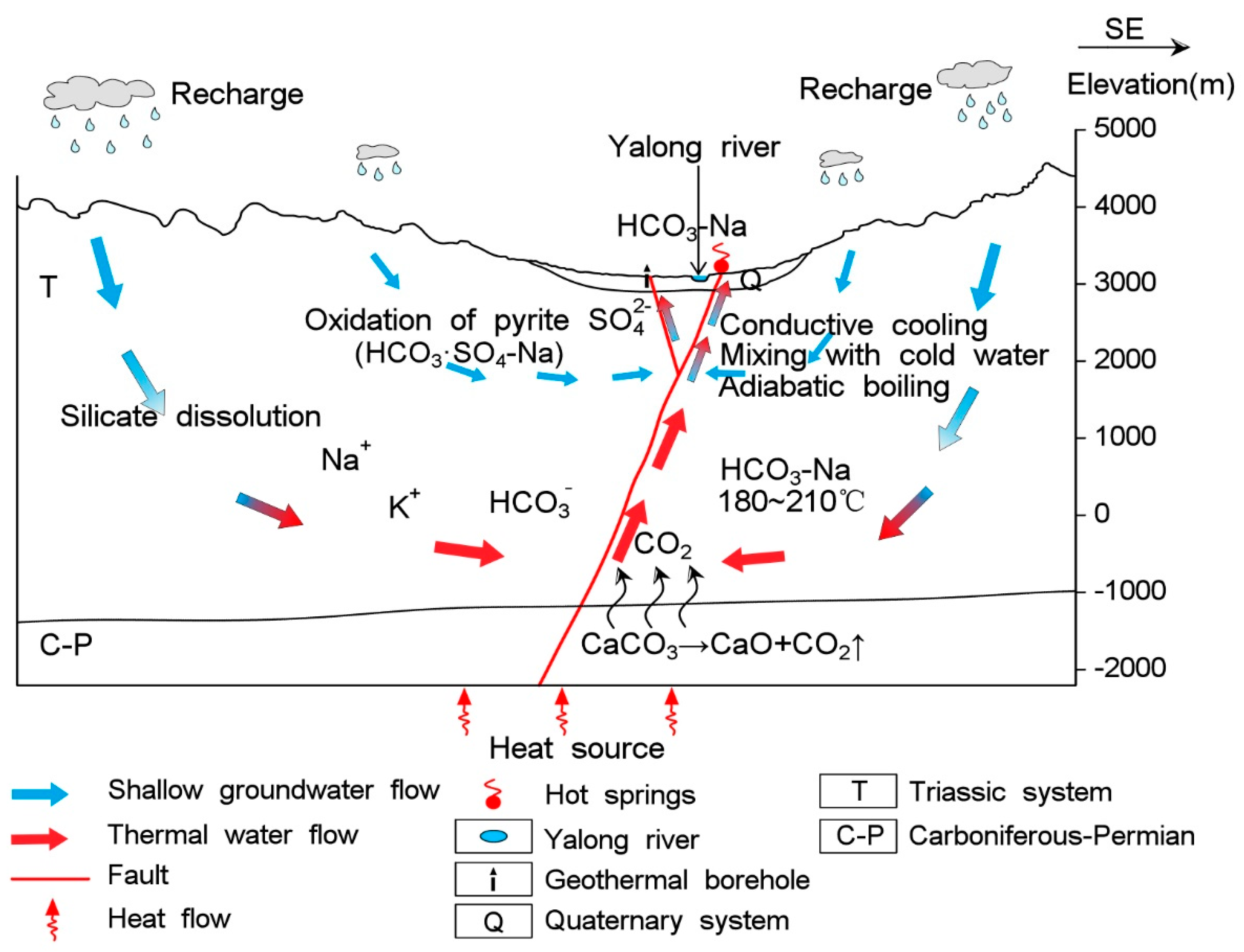
4.7. Conceptual Genetic Model
5. Conclusions
- The hydrochemical type is mainly HCO3-Na in geothermal water. The fluids have passed through the Triassic silicate reservoir, and its characteristics are mainly derived from water-CO2-silicate interactions. During ascent, the deep geothermal water experienced different cooling processes, including mixing with cool water, adiabatic boiling and conductive cooling, either individually or in combination.
- The stable oxygen and hydrogen isotopic data indicate that the geothermal water originates as snow and glacier melt water from the surrounding mountains, and the obvious shift in the δ18O values of the thermal waters is due to water-rock interactions at high temperatures. The isotopic data indicate that the CO2 is mainly derived from marine limestone decomposition, accounting for 74.3–85.6% of the CO2 in the system. The helium isotope ratios range from 0.17–0.39 Ra, indicating that the He is mainly a binary mixture between atmospheric and crustal sources.
- Both cationic geothermometers, including the Na-K-Mg ternary diagram and the Na-K and Na-Li ratios, and the Cl-enthalpy mixing model yield reliable temperature estimates of approximately 197 °C and 200 °C, respectively. The convergent reservoir equilibrium temperature of 180–210 °C is obtained via geothermometrical modelling.
- All data strongly indicate the absence of a magmatic heat source below the Ganzi geothermal field.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Pang, Z.; Hu, S.; Wang, J. A roadmap to geothermal energy development in China. Keji Daobao Sci. Technol. Rev. 2012, 30, 18–24. [Google Scholar]
- Vonsée, B.; Crijns-Graus, W.; Liu, W. Energy technology dependence—A value chain analysis of geothermal power in the EU. Energy 2019, 178, 419–435. [Google Scholar] [CrossRef]
- Aksoy, N. Power generation from geothermal resources in Turkey. Renew. Energy 2014, 68, 595–601. [Google Scholar] [CrossRef]
- Carlino, S.; Troiano, A.; Di Giuseppe, M.G.; Tramelli, A.; Troise, C.; Somma, R.; De Natale, G. Exploitation of geothermal energy in active volcanic areas: A numerical modelling applied to high temperature Mofete geothermal field, at Campi Flegrei caldera (Southern Italy). Renew. Energy 2016, 87, 54–66. [Google Scholar] [CrossRef]
- Guo, Q.; Pang, Z.; Wang, Y.; Tian, J. Fluid geochemistry and geothermometry applications of the Kangding high-temperature geothermal system in eastern Himalayas. Appl. Geochem. 2017, 81, 63–75. [Google Scholar] [CrossRef]
- Nicholson, K. Geothermal Fluids: Chemistry and Exploration Techniques, 1st ed.; Springer: Berlin/Heidelberg, Germany, 1993; pp. 3–7. [Google Scholar]
- Bernal, N.F.; Gleeson, S.A.; Dean, A.S.; Liu, X.-M.; Hoskin, P. The source of halogens in geothermal fluids from the Taupo Volcanic Zone, North Island, New Zealand. Geochim. Cosmochim. Acta. 2014, 126, 265–283. [Google Scholar] [CrossRef]
- Giggenbach, W.F. Variations in the chemical and isotopic composition of fluids discharged from the Taupo Volcanic Zone, New Zealand. J. Volcanol. Geotherm. Res. 1995, 68, 89–116. [Google Scholar] [CrossRef]
- Zierenberg, R.A.; Schiffman, P.; Barfod, G.H.; Lesher, C.E.; Marks, N.E.; Lowenstern, J.B.; Mortensen, A.K.; Pope, E.C.; Bird, D.K.; Reed, M.H.; et al. Composition and origin of rhyolite melt intersected by drilling in the Krafla geothermal field, Iceland. Contrib. Mineral. Petrol. 2013, 165, 327–347. [Google Scholar] [CrossRef]
- Hochstein, M.P.; Regenauer-Lieb, K. Heat generation associated with collision of two plates: The Himalayan geothermal belt. J. Volcanol. Geotherm. Res. 1998, 83, 75–92. [Google Scholar] [CrossRef]
- Liao, Z.; Zhao, P. Yunnan-Tibet Geothermal Belt-Geothermal Resources and Case Histories, 1st ed.; Science Press: Beijing, China, 1999; pp. 1–8. (In Chinese) [Google Scholar]
- Guo, Q. Hydrogeochemistry of high-temperature geothermal systems in China: A review. Appl. Geochem. 2012, 27, 1887–1898. [Google Scholar] [CrossRef]
- Wang, X.; Wang, G.; Lu, C.; Gan, H.; Liu, Z. Evolution of deep parent fluids of geothermal fields in the Nimu—Nagchu geothermal belt, Tibet, China. Geothermics 2018, 71, 118–131. [Google Scholar] [CrossRef]
- Tian, J.; Pang, Z.; Guo, Q.; Wang, Y.; Li, J.; Huang, T.; Kong, Y. Geochemistry of geothermal fluids with implications on the sources of water and heat recharge to the Rekeng high-temperature geothermal system in the Eastern Himalayan Syntax. Geothermics 2018, 74, 92–105. [Google Scholar] [CrossRef]
- Li, J.; Sagoe, G.; Yang, G.; Liu, D.; Li, Y. The application of geochemistry to bicarbonate thermal springs with high reservoir temperature: A case study of the Batang geothermal field, western Sichuan Province, China. J. Volcanol. Geotherm. Res. 2019, 371, 20–31. [Google Scholar] [CrossRef]
- Zhang, X.; Hu, Q. Development of Geothermal Resources in China: A Review. J. Earth Sci. 2018, 29, 452–467. [Google Scholar] [CrossRef]
- Zhao, Q. Characteristics of steam-type hydrothermal system in Ganzi geothermal field. Groundwater 1989, 3, 182–187. (In Chinese) [Google Scholar]
- Spycher, N.; Peiffer, L.; Sonnenthal, E.L.; Saldi, G.; Reed, M.H.; Kennedy, B.M. Integrated multicomponent solute geothermometry. Geothermics 2014, 51, 113–123. [Google Scholar] [CrossRef]
- Ding, L.; Zhong, D.; Yin, A.; Kapp, P.; Harrison, T.M. Cenozoic structural and metamorphic evolution of the eastern Himalayan syntaxis (Namche Barwa). Earth Planet. Sci. Lett. 2001, 192, 423–438. [Google Scholar] [CrossRef]
- Wang, E.; Meng, K.; Su, Z.; Meng, Q.; Chu, J.J.; Chen, Z.; Wang, G.; Shi, X.; Liang, X. Block rotation: Tectonic response of the Sichuan basin to the southeastward growth of the Tibetan Plateau along the Xianshuihe-Xiaojiang fault. Tectonics 2014, 33, 686–718. [Google Scholar] [CrossRef]
- Qu, W.; Lu, Z.; Zhang, Q.; Hao, M.; Wang, Q.; Qu, F.; Zhu, W. Present-day crustal deformation characteristics of the southeastern Tibetan Plateau and surrounding areas by using GPS analysis. J. Asian Earth Sci. 2018, 163, 22–31. [Google Scholar] [CrossRef]
- Shifeng, W.; Erchie, W.; Xiaomin, F.; Bihong, F. Late Cenozoic systematic left-lateral stream deflections along the Ganzi-Yushu fault, Xianshuihe fault system, Eastern Tibet. Int. Geol. Rev. 2008, 50, 624–635. [Google Scholar] [CrossRef]
- Lanxiang, W.X.B. Study on the deformation composition and the motion feature of the Xianshuihe active fault zone. Earthq. Res. China 1985, 1, 53–59. (In Chinese) [Google Scholar]
- Han, L.; Huang, J.; Fan, M.; Liu, Z.; Yang, X.; Li, T.; Jiang, H.; Peng, B. Remote Sensing Image Interpretation of Geological Environment in Garze, Sichuan. Acta Geol. Sichuan 2011, 31, 470–473. (In Chinese) [Google Scholar]
- Completion Report of Zhuode Geothermal Well in Ganzi; Ganzi Kangsheng Geothermal Limited Company: Sichuan, China, 2012. (In Chinese)
- The Geological Survey Team of the Sichuan Bureau of Geology. Regional Geological Survey Report of the Ganziregion; Sichuan provincial bureau of geology and mineral resources: Sichuan, China, 1980. (In Chinese) [Google Scholar]
- Arnórsson, S. Isotopic and Chemical Techniques in Geothermal Exploration, Development and Use; International Atomic Energy Agency: Venna, Austria, 2000; pp. 89–95. [Google Scholar]
- Shestakova, A.; Guseva, N.; Kopylova, Y.; Khvaschevskaya, A.; Polya, D.; Tokarev, I. Geothermometry and Isotope Geochemistry of CO2-Rich Thermal Waters in Choygan, East Tuva, Russia. Water 2018, 10, 729. [Google Scholar] [CrossRef]
- Chandrajith, R.; Barth, J.A.C.; Subasinghe, N.D.; Merten, D.; Dissanayake, C.B. Geochemical and isotope characterization of geothermal spring waters in Sri Lanka: Evidence for steeper than expected geothermal gradients. J. Hydrol. 2013, 476, 360–369. [Google Scholar] [CrossRef]
- Fusari, A.; Carroll, M.R.; Ferraro, S.; Giovannetti, R.; Giudetti, G.; Invernizzi, C.; Mussi, M.; Pennisi, M. Circulation path of thermal waters within the Laga foredeep basin inferred from chemical and isotopic (δ18O, δD, 3H, 87Sr/86Sr) data. Appl. Geochem. 2017, 78, 23–34. [Google Scholar] [CrossRef]
- Chen, L.; Ma, T.; Du, Y.; Xiao, C.; Chen, X.; Liu, C.; Wang, Y. Hydrochemical and isotopic (2H, 18O and 37Cl) constraints on evolution of geothermal water in coastal plain of Southwestern Guangdong Province, China. J. Volcanol. Geotherm. Res. 2016, 318, 45–54. [Google Scholar] [CrossRef]
- Zhang, Y.; Xu, M.; Li, X.; Qi, J.; Zhang, Q.; Guo, J.; Yu, L.; Zhao, R. Hydrochemical characteristics and multivariate statistical analysis of natural water system: A case study in Kangding County, Southwestern China. Water 2018, 10, 80. [Google Scholar] [CrossRef]
- Xudong, W. Stable isotope compositions for meteoric water from Chengdu and their implication of climate. Acta Geol. Sichuan 2009, 1, 52–54. (In Chinese) [Google Scholar]
- Li, J.; Pang, Z.; Kong, Y.; Huang, T.M.; Zhou, M.Z. Contrasting seasonal distribution of stable isotopes and deuterium excess in precipitation over China. Fresenius Environ. Bull. 2014, 23, 2074–2085. [Google Scholar]
- Clark, I.D.; Fritz, P. Environmental Isotopes in Hydrogeology, 2nd ed.; CRC press: Boca Raton, FL, USA, 1997; pp. 55, 117, 210–212. [Google Scholar]
- Jia, G.; Wei, K.; Chen, F.; Peng, P. Soil n-alkane δD vs. altitude gradients along Mount Gongga, China. Geochim. Cosmochim. Acta 2008, 72, 5165–5174. [Google Scholar] [CrossRef]
- Gao, J.; Masson-Delmotte, V.; Yao, T.; Tian, L.; Risi, C.; Hoffmann, G. Precipitation water stable isotopes in the south Tibetan Plateau: Observations and modeling. J. Clim. 2011, 24, 3161–3178. [Google Scholar] [CrossRef]
- Giggenbach, W.F. Chemical techniques in geothermal exploration. In Application of Geochemistry of Geothermal Reservoir Development, 1st ed.; D’Amore, F., Ed.; UNITAR/UNDP: Rome, Italy, 1991; pp. 119–144. [Google Scholar]
- Meybeck, M. Global chemical weathering of surficial rocks estimated from river dissolved loads. Am. J. Sci. 1987, 287, 401–428. [Google Scholar] [CrossRef]
- Helgeson, H.C.; Garrels, R.M.; MacKenzie, F.T. Evaluation of irreversible reactions in geochemical processes involving minerals and aqueous solutions—II. Applications. Geochim. Cosmochim. Acta 1969, 33, 455–481. [Google Scholar] [CrossRef]
- Shvartsev, S.L.; Sun, Z.; Borzenko, S.V.; Gao, B.; Tokarenko, O.G.; Zippa, E. V Geochemistry of the thermal waters in Jiangxi Province, China. Appl. Geochem. 2018, 96, 113–130. [Google Scholar] [CrossRef]
- Pasvanoğlu, S. Hydrogeochemical study of the thermal and mineralized waters of the Banaz (Hamamboğazi) area, western Anatolia, Turkey. Environ. Earth Sci. 2012, 65, 741–752. [Google Scholar] [CrossRef]
- Vengosh, A.; Helvaci, C.; Karamanderesi, I.H. Geochemical constraints for the origin of thermal waters from western Turkey. Appl. Geochem. 2002, 17, 163–183. [Google Scholar] [CrossRef]
- Tarcan, G.; Gemici, Ü. Water geochemistry of the Seferihisar geothermal area, Izmir, Turkey. J. Volcanol. Geotherm. Res. 2003, 126, 225–242. [Google Scholar] [CrossRef]
- Dotsika, E.; Leontiadis, I.; Poutoukis, D.; Cioni, R.; Raco, B. Fluid geochemistry of the Chios geothermal area, Chios Island, Greece. J. Volcanol. Geotherm. Res. 2006, 154, 237–250. [Google Scholar] [CrossRef]
- Faure, G.; Powell, J.L. Strontium Isotope Geology, 1st ed.; Springer: Berlin/Heidelberg, Germany, 1972; pp. 10–33. [Google Scholar]
- Palmer, M.R.; Edmond, J.M. The strontium isotope budget of the modern ocean. Earth Planet. Sci. Lett. 1989, 92, 11–26. [Google Scholar] [CrossRef]
- Palmer, M.R.; Edmond, J.M. Controls over the strontium isotope composition of river water. Geochim. Cosmochim. Acta 1992, 56, 2099–2111. [Google Scholar] [CrossRef]
- Capo, R.C.; Stewart, B.W.; Chadwick, O.A. Strontium isotopes as tracers of ecosystem processes: Theory and methods. Geoderma 1998, 82, 197–225. [Google Scholar] [CrossRef]
- Han, G.; Liu, C.-Q. Strontium isotope and major ion chemistry of the rainwaters from Guiyang, Guizhou Province, China. Sci. Total Environ. 2006, 364, 165–174. [Google Scholar] [CrossRef] [PubMed]
- Han, G.; Liu, C.-Q. Water geochemistry controlled by carbonate dissolution: A study of the river waters draining karst-dominated terrain, Guizhou Province, China. Chem. Geol. 2004, 204, 1–21. [Google Scholar] [CrossRef]
- Wang, Z.-L.; Zhang, J.; Liu, C.-Q. Strontium isotopic compositions of dissolved and suspended loads from the main channel of the Yangtze River. Chemosphere 2007, 69, 1081–1088. [Google Scholar] [CrossRef] [PubMed]
- Samborska, K.; Halas, S.; Bottrell, S.H. Sources and impact of sulphate on groundwaters of Triassic carbonate aquifers, Upper Silesia, Poland. J. Hydrol. 2013, 486, 136–150. [Google Scholar] [CrossRef]
- Hosono, T.; Delinom, R.; Nakano, T.; Kagabu, M.; Shimada, J. Evolution model of δ34S and δ18O in dissolved sulfate in volcanic fan aquifers from recharge to coastal zone and through the Jakarta urban area, Indonesia. Sci. Total Environ. 2011, 409, 2541–2554. [Google Scholar] [CrossRef] [PubMed]
- Du, F. Inorganic Sulfur and Nitrogen Isotope Variation in Atmospheric Precipitation at Chengdu, China. Master’s Thesis, Chengdu University of Technology, Chengdu, China, 2012. (In Chinese). [Google Scholar]
- Raab, M.; Spiro, B. Sulfur isotopic variations during seawater evaporation with fractional crystallization. Chem. Geol. Isot. Geosci. Sect. 1991, 86, 323–333. [Google Scholar] [CrossRef]
- Möller, P.; Geyer, S.; Salameh, E.; Dulski, P. Sources of mineralization and salinization of thermal groundwater of Jordan. Acta Hydrochim. Hydrobiol. 2006, 34, 86–100. [Google Scholar] [CrossRef]
- Taylor, B.E.; Wheeler, M.C.; Nordstrom, D.K. Isotope composition of sulphate in acid mine drainage as measure of bacterial oxidation. Nature 1984, 308, 538–541. [Google Scholar] [CrossRef]
- Zhu, G.; Zhang, S.; Liang, Y.; Dai, J.; Li, J. Isotopic evidence of TSR origin for natural gas bearing high H2S contents within the Feixianguan Formation of the northeastern Sichuan Basin, southwestern China. Sci. China Ser. D Earth Sci. 2005, 48, 1960. [Google Scholar] [CrossRef]
- Cai, C.; Li, K.; Zhu, Y.; Xiang, L.; Jiang, L.; Cai, X.; Cai, L. TSR origin of sulfur in Permian and Triassic reservoir bitumen, East Sichuan Basin, China. Organ. Geochem. 2010, 41, 871–878. [Google Scholar] [CrossRef]
- Toran, L.; Harris, R.F. Interpretation of sulfur and oxygen isotopes in biological and abiological sulfide oxidation. Geochim. Cosmochim. Acta 1989, 53, 2341–2348. [Google Scholar] [CrossRef]
- Marques, J.M.; Graça, H.; Eggenkamp, H.G.M.; Neves, O.; Carreira, P.M.; Matias, M.J.; Mayer, B.; Nunes, D.; Trancoso, V.N. Isotopic and hydrochemical data as indicators of recharge areas, flow paths and water—Rock interaction in the Caldas da Rainha—Quinta das Janelas thermomineral carbonate rock aquifer (Central Portugal). J. Hydrol. 2013, 476, 302–313. [Google Scholar] [CrossRef]
- Sano, Y.; Wakita, H. Geographical distribution of 3He/4He ratios in Japan: Implications for arc tectonics and incipient magmatism. J. Geophys. Res. Solid Earth 1985, 90, 8729–8741. [Google Scholar] [CrossRef]
- Hilton, D.R.; Hammerschmidt, K.; Teufel, S.; Friedrichsen, H. Helium isotope characteristics of Andean geothermal fluids and lavas. Earth Planet. Sci. Lett. 1993, 120, 265–282. [Google Scholar] [CrossRef]
- Umeda, K.; Ninomiya, A.; McCrank, G.F. High 3He emanations from the source regions of recent large earthquakes, central Japan. Geochem. Geophys. Geosyst. 2008, 9. [Google Scholar] [CrossRef]
- Oxburgh, E.R.; O’nions, R.K.; Hill, R.I. Helium isotopes in sedimentary basins. Nature 1986, 324, 632–635. [Google Scholar] [CrossRef]
- Gautheron, C.; Moreira, M.; Allègre, C. He, Ne and Ar composition of the European lithospheric mantle. Chem. Geol. 2005, 217, 97–112. [Google Scholar] [CrossRef]
- Hoke, L.; Lamb, S.; Hilton, D.R.; Poreda, R.J. Southern limit of mantle-derived geothermal helium emissions in Tibet: Implications for lithospheric structure. Earth Planet. Sci. Lett. 2000, 180, 297–308. [Google Scholar] [CrossRef]
- Ballentine, C.J.; Burnard, P.G. Production, release and transport of noble gases in the continental crust. Rev. Mineral. Geochem. 2002, 47, 481–538. [Google Scholar] [CrossRef]
- Ozima, M.; Podosek, F.A. Noble Gas Geochemistry, 2nd ed.; Cambridge University Press: Cambridge, UK, 2002; pp. 12–15. [Google Scholar]
- Lyon, G.L.; Hulston, J.R. Carbon and hydrogen isotopic compositions of New Zealand geothermal gases. Geochim. Cosmochim. Acta 1984, 48, 1161–1171. [Google Scholar] [CrossRef]
- Mutlu, H.; Güleç, N.; Hilton, D.R. Helium—Carbon relationships in geothermal fluids of western Anatolia, Turkey. Chem. Geol. 2008, 247, 305–321. [Google Scholar] [CrossRef]
- Veizer, J.; Ala, D.; Azmy, K.; Bruckschen, P.; Buhl, D.; Bruhn, F.; Carden, G.A.F.; Diener, A.; Ebneth, S.; Godderis, Y.; et al. 87Sr/86Sr, δ13C and δ18O evolution of Phanerozoic seawater. Chem. Geol. 1999, 161, 59–88. [Google Scholar] [CrossRef]
- Sano, Y.; Marty, B. Origin of carbon in fumarolic gas from island arcs. Chem. Geol. 1995, 119, 265–274. [Google Scholar] [CrossRef]
- Giggenbach, W.F. Geothermal solute equilibria. derivation of Na-K-Mg-Ca geoindicators. Geochim. Cosmochim. Acta 1988, 52, 2749–2765. [Google Scholar] [CrossRef]
- Kharaka, Y.K.; Lico, M.S.; Law, L.M. Chemical geothermometers applied to formation waters, Gulf of Mexico and California basins. AAPG Bull. 1982, 66, 588. [Google Scholar]
- Sanjuan, B.; Millot, R.; Asmundsson, R.; Brach, M.; Giroud, N. Use of two new Na/Li geothermometric relationships for geothermal fluids in volcanic environments. Chem. Geol. 2014, 389, 60–81. [Google Scholar] [CrossRef]
- Reed, M.; Spycher, N. Calculation of pH and mineral equilibria in hydrothermal waters with application to geothermometry and studies of boiling and dilution. Geochim. Cosmochim. Acta 1984, 48, 1479–1492. [Google Scholar] [CrossRef]
- Pang, Z.-H.; Reed, M. Theoretical chemical thermometry on geothermal waters: Problems and methods. Geochim. Cosmochim. Acta 1998, 62, 1083–1091. [Google Scholar] [CrossRef]
- Asta, M.P.; Gimeno, M.J.; Auqué, L.F.; Gómez, J.; Acero, P.; Lapuente, P. Secondary processes determining the pH of alkaline waters in crystalline rock systems. Chem. Geol. 2010, 276, 41–52. [Google Scholar] [CrossRef]
- Fournier, R.O. Chemical geothermometers and mixing models for geothermal systems. Geothermics 1977, 5, 41–50. [Google Scholar] [CrossRef]
- Li, J.; Yang, G.; Sagoe, G.; Li, Y. Major hydrogeochemical processes controlling the composition of geothermal waters in the Kangding geothermal field, western Sichuan Province. Geothermics 2018, 75, 154–163. [Google Scholar] [CrossRef]
- Xu, M. Study on the Present Geothermal Field and Lithospheric Thermal Structure in the Sichuan Basin. Ph.D. Thesis, University of Chinese Academy of Sciences, Beijing, China, 2011. (In Chinese). [Google Scholar]
- Jiang, G.Z.; Gao, P.; Rao, S.; Zhang, L.Y.; Tang, X.Y.; Huang, F.; Zhao, P.; Pang, Z.H.; He, L.J.; Hu, S.B.; et al. Compilation of heat flow data in the continental area of China (4th edition). Chin. J. Geophys.-Chin. Ed. 2016, 59, 2892–2910. [Google Scholar]
- Li, G.; Li, F. The Circulation Law, Sustainable Development and Utilization of Geothermal Water in Guanzhong Basin; Science Press: Beijing, China, 2010; p. 100. [Google Scholar]
- Shangguan, Z.; Zhao, C.; Li, H.; Gao, Q.; Sun, M. Evolution of hydrothermal explosions at Rehai geothermal field, Tengchong volcanic region, China. Geothermics 2005, 34, 518–526. [Google Scholar] [CrossRef]
| Sample ID | Type | Altitude (m) | Well Depth (m) | T (°C) | pH | Ec (μs/cm) | TDS (mg/L) | Ca2+ (mg/L) | Mg2+ (mg/L) | Na+ (mg/L) | K+ (mg/L) | CO32− (mg/L) | HCO3− (mg/L) | Cl− (mg/L) | SO42− (mg/L) | F− (mg/L) | NO3− (mg/L) | |||
| GZ33 | Geothermal water from boreholes | 3362 | 180 | 89 | 8.2 | 1344 | 651 | 8.7 | 3.8 | 374.7 | 25.9 | 0 | 783 | 17.7 | 72.9 | 35.2 | 1.8 | |||
| GZ36 | 3354 | 30 | 69 | 7.3 | 1205 | 422 | 19.0 | 10.9 | 223.9 | 9.0 | 0 | 483 | 13.2 | 146.6 | 14.9 | 1.1 | ||||
| GZ37 | 3331 | 40 | 61 | 7.6 | 1027 | 450 | 17.9 | 7.2 | 203.3 | 7.2 | 0 | 500 | 9.2 | 119.6 | 12.9 | 0.9 | ||||
| GZ39 | 3364 | 80 | 57 | 7.8 | 1169 | 450 | 7.3 | 16.8 | 174.4 | 13.3 | 0 | 507 | 9.8 | 45.8 | 4.6 | 3.2 | ||||
| GZ40 | 3334 | 40 | 50 | 7.6 | 706 | 326 | 16.6 | 9.9 | 151.8 | 6.2 | 0 | 384 | 8.6 | 74.9 | 11.9 | 1.0 | ||||
| GZ41 | 3335 | 50 | 62 | 7.9 | 1137 | 499 | 5.3 | 10.7 | 216.4 | 14.5 | 0 | 591 | 10.1 | 54.1 | 6.2 | 0.0 | ||||
| GZ42 | 3349 | 40 | 78 | 8.8 | 2430 | 868 | 3.4 | 0.3 | 405.3 | 29.0 | 66 | 860 | 14.4 | 58.8 | 24.3 | 1.0 | ||||
| GZ43 | 3359 | 180 | 87 | 8.8 | 1531 | 785 | 3.8 | 1.9 | 374.5 | 27.4 | 51 | 877 | 13.8 | 79.0 | 27.0 | 0.9 | ||||
| GZ44 | 3346 | 60 | 73 | 8.0 | 1186 | 513 | 11.1 | 5.0 | 225.6 | 7.7 | 0 | 593 | 9.5 | 86.2 | 14.8 | 0.8 | ||||
| GZ46 | 3301 | 170 | 53 | 8.4 | 750 | 383 | 13.0 | 8.8 | 157.1 | 4.7 | 0 | 466 | 8.5 | 48.1 | 9.7 | 1.0 | ||||
| GZ47 | 3292 | 40 | 83 | 8.0 | 2130 | 727 | 4.9 | 0.3 | 345.3 | 25.3 | 0 | 848 | 13.7 | 67.9 | 25.4 | 1.1 | ||||
| GZ48 | 3342 | 90 | 84 | 9.4 | 1867 | 850 | 1.0 | 0.1 | 397.9 | 28.8 | 128 | 740 | 14.7 | 71.7 | 24.8 | 0.8 | ||||
| GZ50 | 3306 | 80 | 80 | 7.6 | 1404 | 515 | 9.0 | 4.1 | 206.7 | 17.0 | 0 | 557 | 10.2 | 48.8 | 17.7 | 0.8 | ||||
| GZ55 | Hot springs | 3272 | 43 | 7.6 | 1076 | 534 | 7.1 | 11.5 | 180.0 | 14.0 | 0 | 621 | 8.5 | 8.3 | 6.5 | 0.0 | ||||
| GZ51 | 3315 | 83 | 9.4 | 3890 | 1789 | 1.3 | 0.1 | 860.8 | 58.4 | 357 | 1439 | 43.3 | 65.3 | 17.7 | 0.0 | |||||
| GZ53 | 3334 | 85 | 9.1 | 3490 | 1789 | 8.4 | 0.1 | 836.5 | 52.4 | 123 | 2013 | 47.2 | 213.3 | 16.5 | 0.9 | |||||
| GZ56 | 3284 | 40 | 7.5 | 2330 | 1128 | 16.3 | 4.3 | 517.1 | 9.6 | 0 | 1441 | 27.8 | 22.5 | 6.9 | 1.0 | |||||
| GZ60 | 3333 | 63 | 7.4 | 2630 | 1477 | 13.7 | 1.6 | 706.0 | 61.2 | 0 | 1744 | 37.1 | 152.5 | 14.2 | 0.9 | |||||
| GZ45 | Shallow groundwater | 3346 | 20 | 37 | 8.1 | 568 | 285 | 22.4 | 18.8 | 58.6 | 4.7 | 0 | 356 | 10.4 | 27.3 | 2.6 | 0.0 | |||
| GZ54 | Yalong river | 3315 | 5 | 8.4 | 235 | 176 | 35.5 | 17.7 | 6.9 | 1.0 | 0 | 120 | 7.0 | 84.8 | 0.0 | 1.4 | ||||
| GZ61 | 3336 | 3 | 7.3 | 339 | 281 | 56.5 | 21.5 | 47.3 | 3.6 | 0 | 332 | 8.4 | 106.0 | 1.2 | 1.9 | |||||
| GZ63 | 3342 | 2 | 8.0 | 148 | 119 | 41.7 | 11.6 | 1.3 | 0.4 | 0 | 163 | 1.1 | 25.7 | 0.2 | 0.5 | |||||
| Sample ID | Type | SiO2 (mg/L) | Li+ (mg/L) | B3+ (mg/L) | Sr2+ (μg/) | δ18O ‰ | δD ‰ | 87Sr/86Sr | δ34SSO4 ‰ | δ18OSO4 ‰ | Charge Balance (%) | Water Type | ||||||||
| GZ33 | Geothermal water from boreholes | 118.9 | 0.8 | 4.8 | 127 | −16.8 | −130.2 | 0.714594 | −2.3 | −8.4 | 2.8 | HCO3-Na | ||||||||
| GZ36 | 51.6 | 0.4 | 2.0 | 177 | −16.3 | −126.1 | n.m. | n.m. | n.m. | −1.3 | HCO3·SO4-Na | |||||||||
| GZ37 | 53.8 | 0.4 | 2.3 | 185 | −17.2 | −129.4 | 0.714235 | −6.9 | −12.4 | −50 | HCO3-Na | |||||||||
| GZ39 | 44.8 | 0.3 | 2.1 | 190 | −16.6 | −126.5 | 0.714644 | −1.0 | −10.7 | −0.7 | HCO3-Na | |||||||||
| GZ40 | 34.4 | 0.3 | 1.3 | 166 | −15.9 | −123.3 | n.m. | n.m. | n.m. | −1.9 | HCO3-Na | |||||||||
| GZ41 | 66.9 | 0.5 | 3.1 | 165 | −17.0 | −129.7 | n.m. | n.m. | n.m. | −2.2 | HCO3-Na | |||||||||
| GZ42 | 173.6 | 1.0 | 6.3 | 136 | −16.3 | −130.5 | 0.716888 | n.m. | n.m. | −1.8 | HCO3-Na | |||||||||
| GZ43 | 175.7 | 1.0 | 5.9 | 131 | −16.3 | −131.7 | 0.716831 | 1.3 | −8.4 | −6.0 | HCO3-Na | |||||||||
| GZ44 | 67.2 | 0.5 | 2.8 | 155 | −16.8 | −128.6 | n.m. | n.m. | n.m. | −6.8 | HCO3-Na | |||||||||
| GZ46 | 40.8 | 0.3 | 2.0 | 139 | −16.5 | −126.0 | 0.714664 | 0.4 | −11.5 | −6.1 | HCO3-Na | |||||||||
| GZ47 | 128.6 | 0.9 | 5.5 | 125 | −16.7 | −132.7 | n.m. | n.m. | n.m. | −3.4 | HCO3-Na | |||||||||
| GZ48 | 139.3 | 1.0 | 6.3 | 23 | −16.6 | −132.3 | n.m. | n.m. | n.m. | −4.0 | HCO3-Na | |||||||||
| GZ50 | 52.7 | 0.6 | 2.6 | 364 | −15.3 | −122.2 | n.m. | n.m. | n.m. | −5.4 | HCO3-Na | |||||||||
| GZ55 | Hot springs | 70.2 | 0.4 | 2.3 | 189 | −16.0 | −125.0 | 0.714098 | n.m. | n.m. | −7.0 | HCO3-Na | ||||||||
| GZ51 | 177.9 | 3.2 | 16.0 | 104 | −14.7 | −132.1 | 0.716767 | 1.1 | −5.4 | 0.0 | HCO3·CO3-Na | |||||||||
| GZ53 | 180.0 | 3.1 | 15.7 | 170 | −14.7 | −133.5 | 0.716706 | n.m. | n.m. | −6.9 | HCO3-Na | |||||||||
| GZ56 | 85.3 | 2.2 | 9.0 | 465 | −15.0 | −127.2 | 0.715115 | 3.4 | −6.7 | −2.8 | HCO3-Na | |||||||||
| GZ60 | 139.3 | 2.7 | 11.5 | 147 | −15.3 | −131.0 | 0.715660 | 4.1 | −8.5 | −0.7 | HCO3-Na | |||||||||
| GZ45 | Shallow groundwater | 25.5 | 0.2 | 0.9 | 182 | −15.6 | −120.4 | 0.714394 | n.m. | n.m. | −12.1 | HCO3-Na·Mg | ||||||||
| GZ54 | Yalong river | 8.2 | 0.0 | 0.1 | 245 | −14.1 | −106.1 | n.m. | n.m. | n.m. | −5.1 | HCO3·SO4-Ca·Mg | ||||||||
| GZ61 | 14.2 | 0.1 | 0.8 | 299 | −14.2 | −106.3 | n.m. | n.m. | n.m. | −8.2 | HCO3·SO4-Ca·Na·Mg | |||||||||
| GZ63 | 8.2 | 0.0 | 0.0 | 174 | −15.2 | −112.3 | n.m. | n.m. | n.m. | −2.2 | HCO3-Ca·Mg | |||||||||
| Sample ID | Type | He (%.vol) | H2 (%.vol) | O2 (%.vol) | Ar (%.vol) | N2 (%.vol) | CH4 (%.vol) | CO2 (%.vol) | 3He/4He | R/Ra | 4He/20Ne | δ13CCO2 (‰) | Mantle (%) | Organic Sedimentary (%) | Limestone (%) |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| GZ34 | Geothermal water from boreholes | 0.062 | 0.000 | 0.67 | 1.73 | 70.73 | 11.37 | 15.45 | 2.42 × 10−7 | 0.17 | 43.08 | ||||
| GZ35 | 0.060 | 0.009 | 1.17 | 1.57 | 71.99 | 8.95 | 16.25 | 2.63 × 10−7 | 0.19 | 50.59 | |||||
| GZ38 | 0.076 | 0.004 | 1.06 | 1.33 | 82.93 | 8.52 | 6.08 | 2.60 × 10−7 | 0.19 | 61.26 | |||||
| GZ39 | 0.030 | 0.002 | 1.79 | 1.27 | 75.52 | 5.94 | 15.46 | 2.40 × 10−7 | 0.17 | 17.48 | |||||
| GZ47 | 0.050 | 1.439 | 0.67 | 0.40 | 29.82 | 7.64 | 59.99 | 2.76 × 10−7 | 0.20 | 98.98 | |||||
| GZ50 | 0.060 | 0.099 | 3.31 | 1.09 | 58.10 | 9.60 | 27.74 | 2.00 × 10−7 | 0.14 | 39.97 | |||||
| GZ52 | hot springs | 0.012 | 0.005 | 1.57 | 0.18 | 12.57 | 2.55 | 83.12 | 4.17 × 10−7 | 0.30 | 47.34 | −5.4 | 6 | 17 | 77 |
| GZ53 | 0.001 | 0.005 | 5.25 | 0.25 | 13.83 | 0.37 | 80.30 | 5.49 × 10−7 | 0.39 | 4.01 | −7.5 | 1 | 25 | 74 | |
| GZ56 | 0.004 | 0.003 | 0.93 | 0.05 | 6.36 | 1.62 | 91.05 | 4.67 × 10−7 | 0.33 | 29.84 | −3.9 | 2 | 12 | 86 | |
| GZ57 | 0.012 | 0.132 | 1.02 | 0.09 | 12.03 | 3.54 | 83.17 | 4.67 × 10−7 | 0.33 | 51.52 | −4.0 | 7 | 12 | 81 | |
| GZ59 | 0.002 | 0.719 | 3.08 | 0.15 | 16.88 | 0.66 | 78.51 | 4.84 × 10−7 | 0.35 | 12.10 | −5.2 | 1 | 17 | 82 | |
| GZ60 | 0.002 | 0.003 | 0.93 | 0.05 | 11.33 | 1.34 | 86.35 | 4.85 × 10−7 | 0.35 | 9.71 | −5.8 | 1 | 19 | 80 |
| Sample ID | Na-K(°C) Giggenbach (1988) [75] | Na-Li(°C) Kharaka et al. (1982) [76] |
|---|---|---|
| GZ33 | 205 | 184 |
| GZ36 | 168 | 173 |
| GZ37 | 161 | 181 |
| GZ39 | 212 | 183 |
| GZ40 | 170 | 191 |
| GZ41 | 202 | 193 |
| GZ42 | 207 | 197 |
| GZ43 | 209 | 200 |
| GZ44 | 159 | 192 |
| GZ46 | 151 | 176 |
| GZ47 | 209 | 200 |
| GZ48 | 208 | 197 |
| GZ50 | 217 | 201 |
| GZ55 | 213 | 187 |
| GZ51 | 203 | 223 |
| GZ53 | 198 | 222 |
| GZ56 | 126 | 232 |
| GZ60 | 221 | 224 |
| GZ45 | 215 | 200 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fan, Y.; Pang, Z.; Liao, D.; Tian, J.; Hao, Y.; Huang, T.; Li, Y. Hydrogeochemical Characteristics and Genesis of Geothermal Water from the Ganzi Geothermal Field, Eastern Tibetan Plateau. Water 2019, 11, 1631. https://doi.org/10.3390/w11081631
Fan Y, Pang Z, Liao D, Tian J, Hao Y, Huang T, Li Y. Hydrogeochemical Characteristics and Genesis of Geothermal Water from the Ganzi Geothermal Field, Eastern Tibetan Plateau. Water. 2019; 11(8):1631. https://doi.org/10.3390/w11081631
Chicago/Turabian StyleFan, Yifan, Zhonghe Pang, Dawei Liao, Jiao Tian, Yinlei Hao, Tianming Huang, and Yiman Li. 2019. "Hydrogeochemical Characteristics and Genesis of Geothermal Water from the Ganzi Geothermal Field, Eastern Tibetan Plateau" Water 11, no. 8: 1631. https://doi.org/10.3390/w11081631
APA StyleFan, Y., Pang, Z., Liao, D., Tian, J., Hao, Y., Huang, T., & Li, Y. (2019). Hydrogeochemical Characteristics and Genesis of Geothermal Water from the Ganzi Geothermal Field, Eastern Tibetan Plateau. Water, 11(8), 1631. https://doi.org/10.3390/w11081631







