Comparison between 2D Shallow-Water Simulations and Energy-Momentum Computations for Transcritical Flow Past Channel Contractions
Abstract
1. Introduction
2. Materials and Methods
2.1. Steady-State Water Surface Profiles for Gradually Varied Open Channel Flow
2.2. Solution of the 2D Depth-Integrated Shallow-Water Equations (SWE)
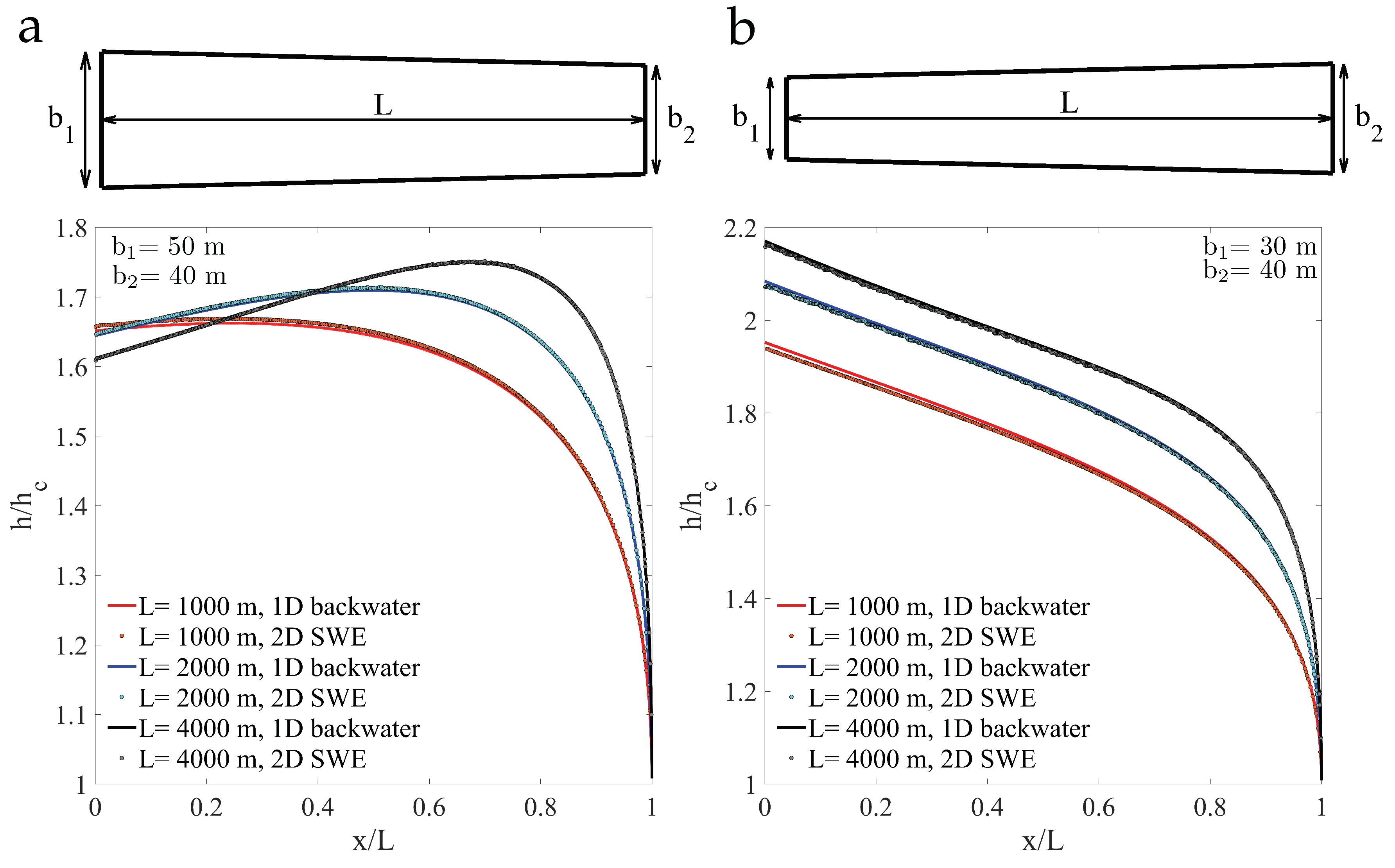
2.3. Model Verification Using Subcritical Flow: Simple Backwater Curves in Expanding/Contracting Channels
2.4. Construction of Water Surface Profiles for Transcritical Flow past a Contraction
3. Results: Model Validation Using Supercritical and Transcritical Flow in Laboratory-Scale Flumes
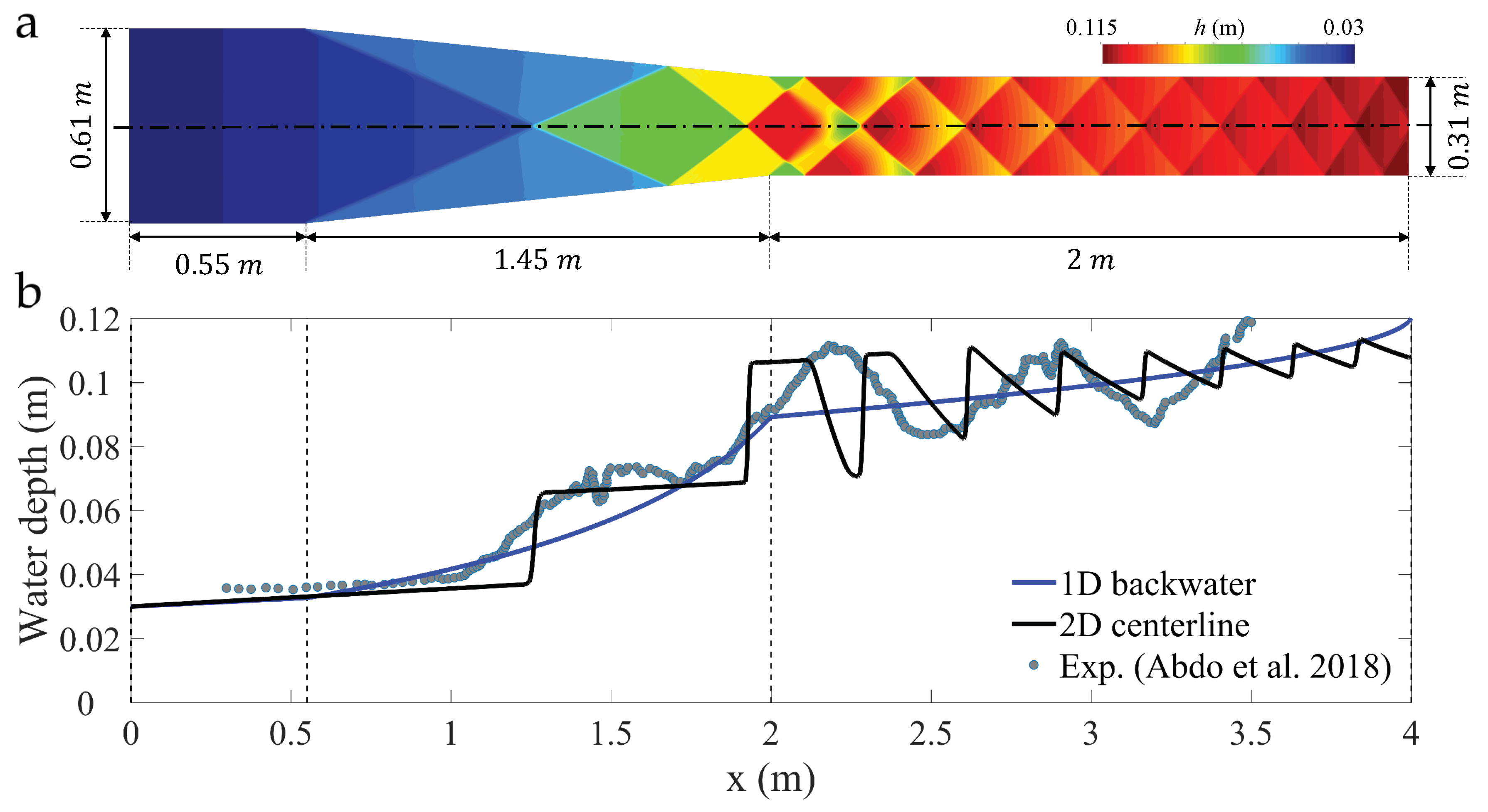
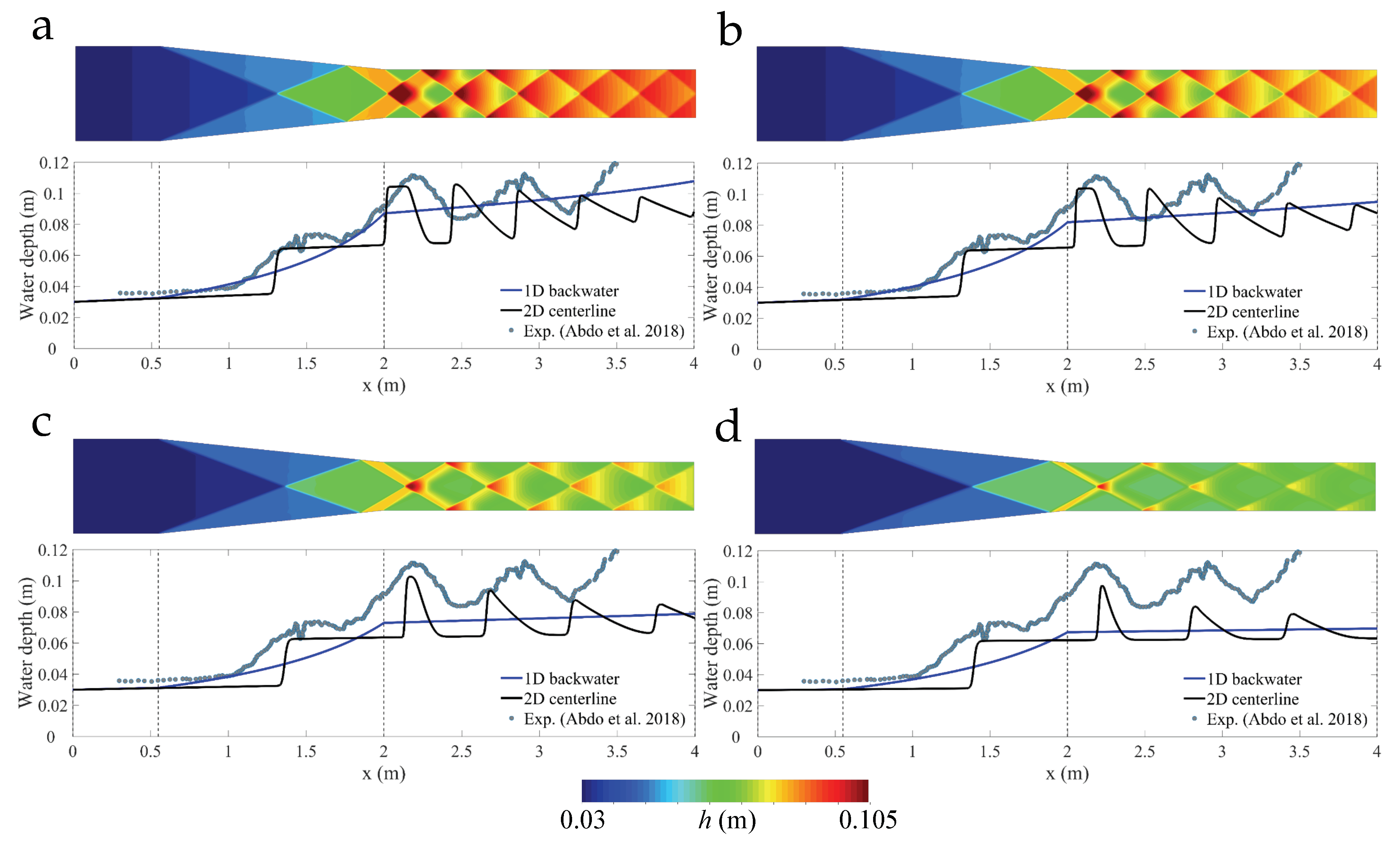
3.1. Supercritical Flow Past a Channel Contraction
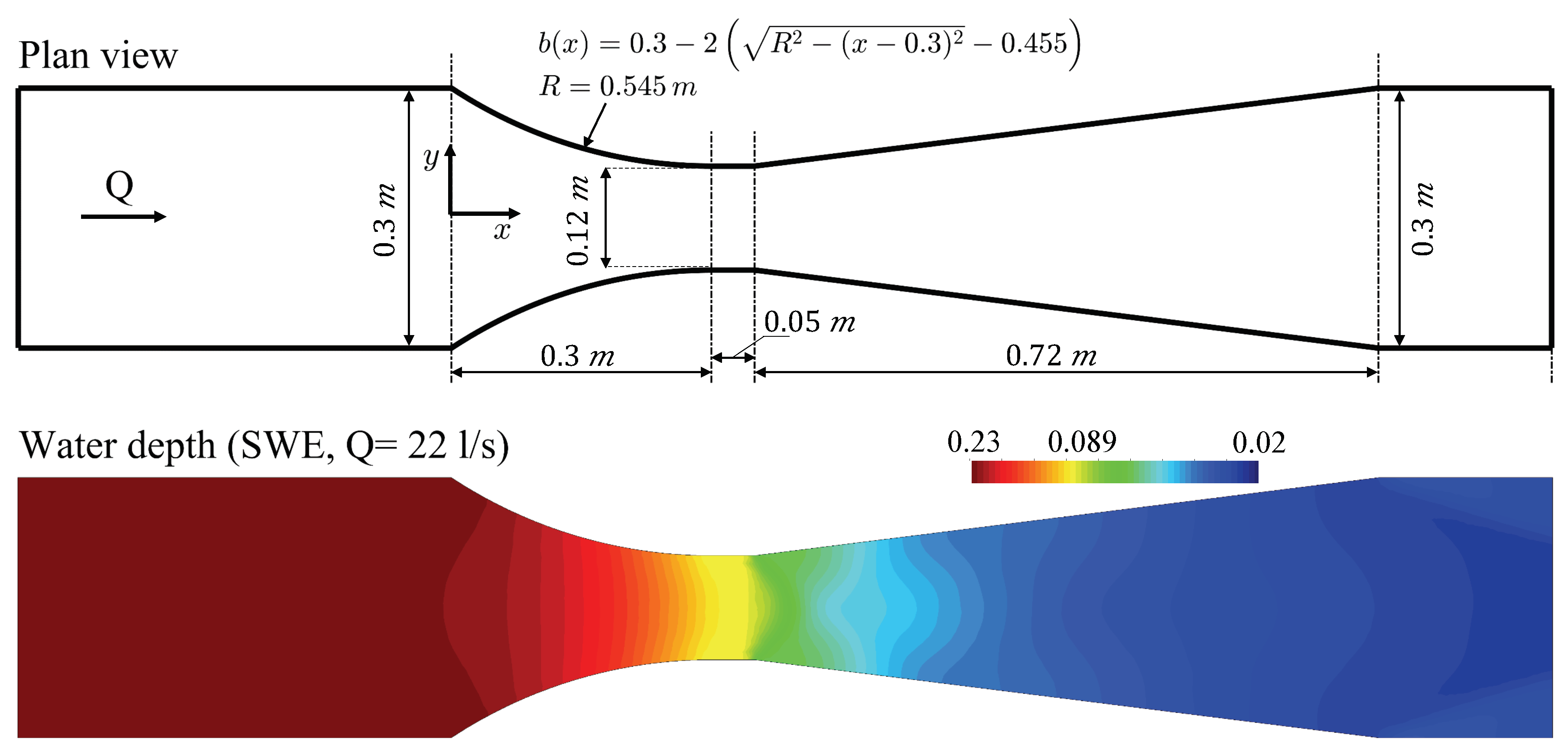
3.2. Gradually Varied Transcritical Flow in a Parshall Flume
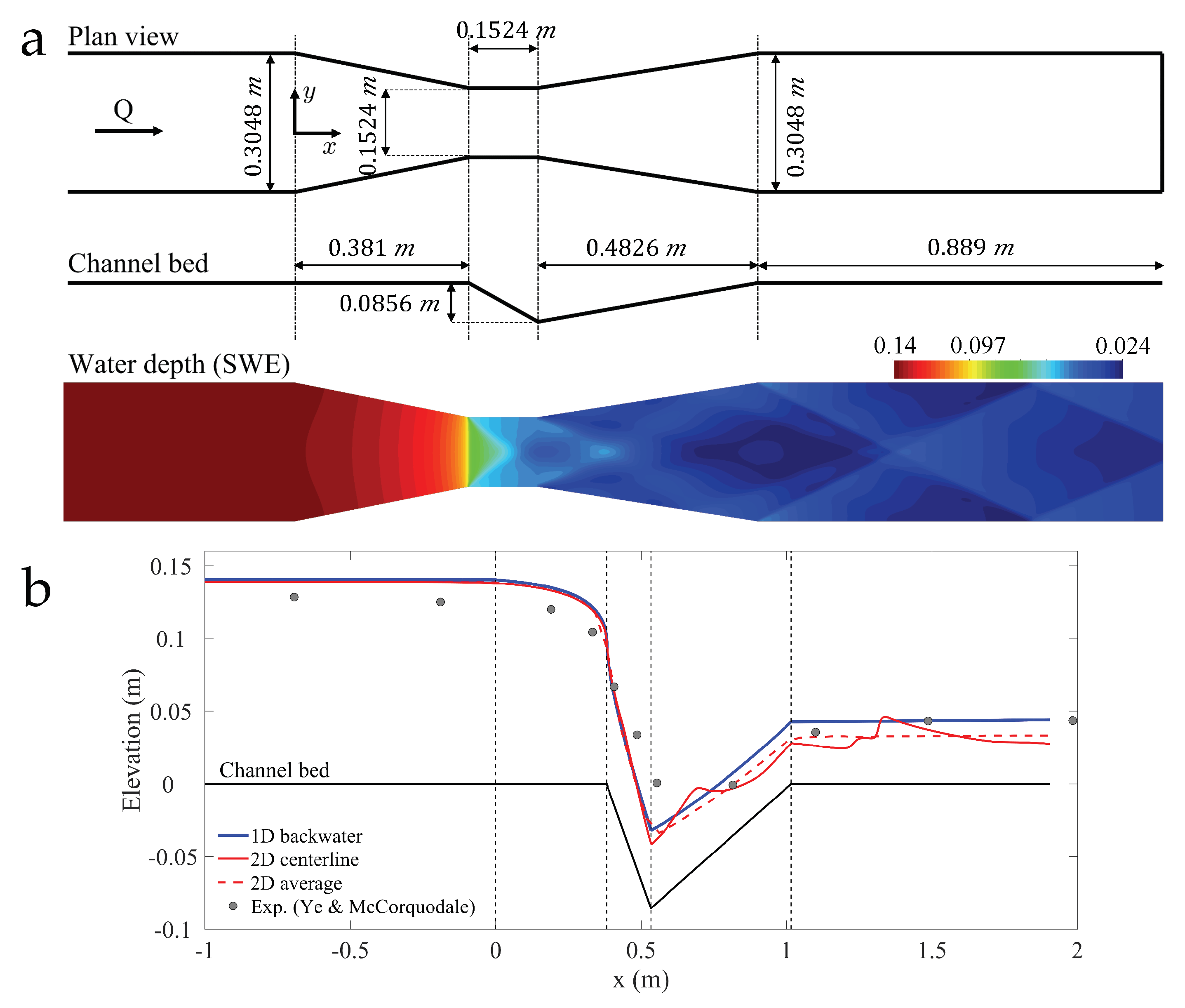
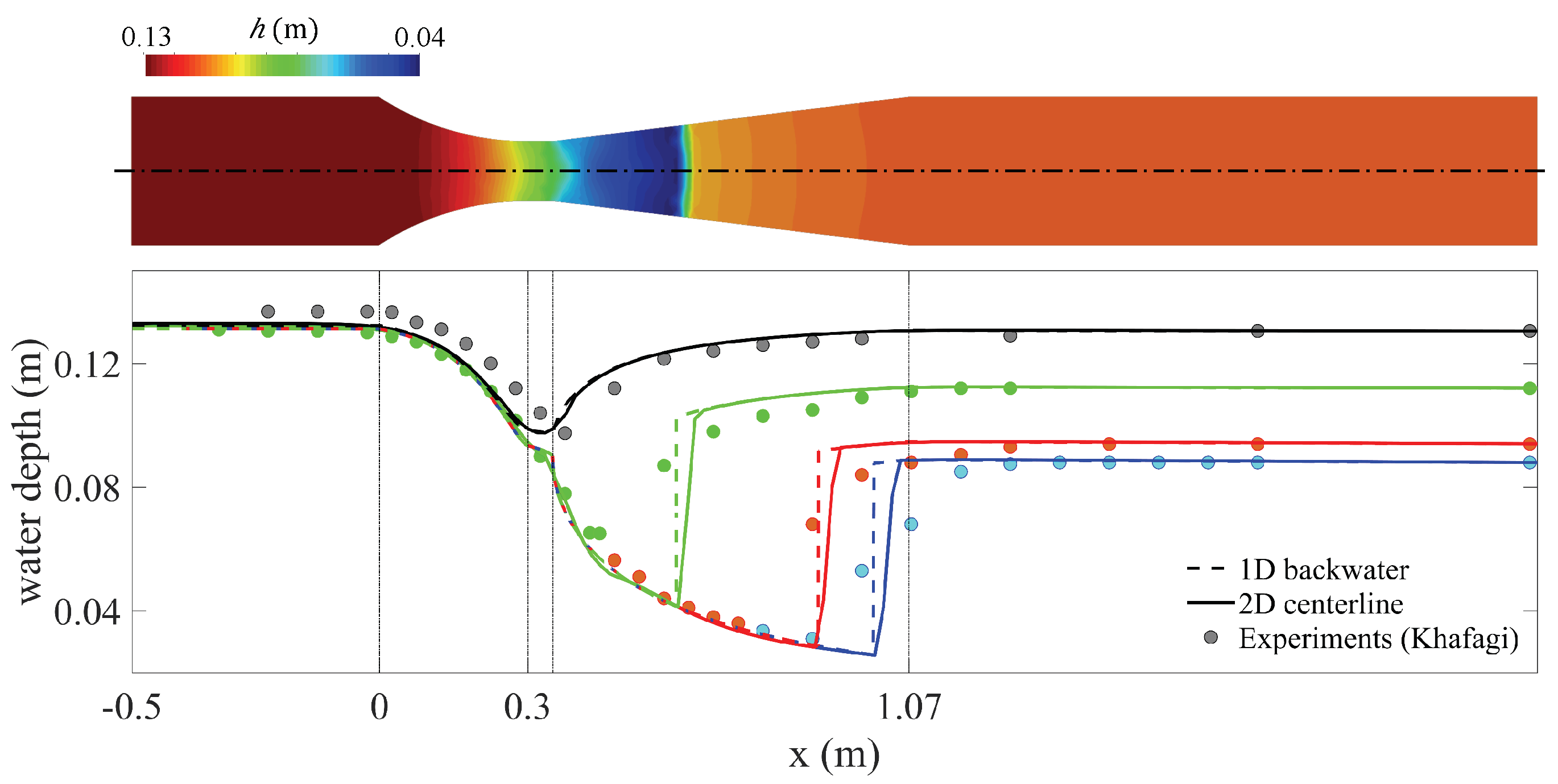
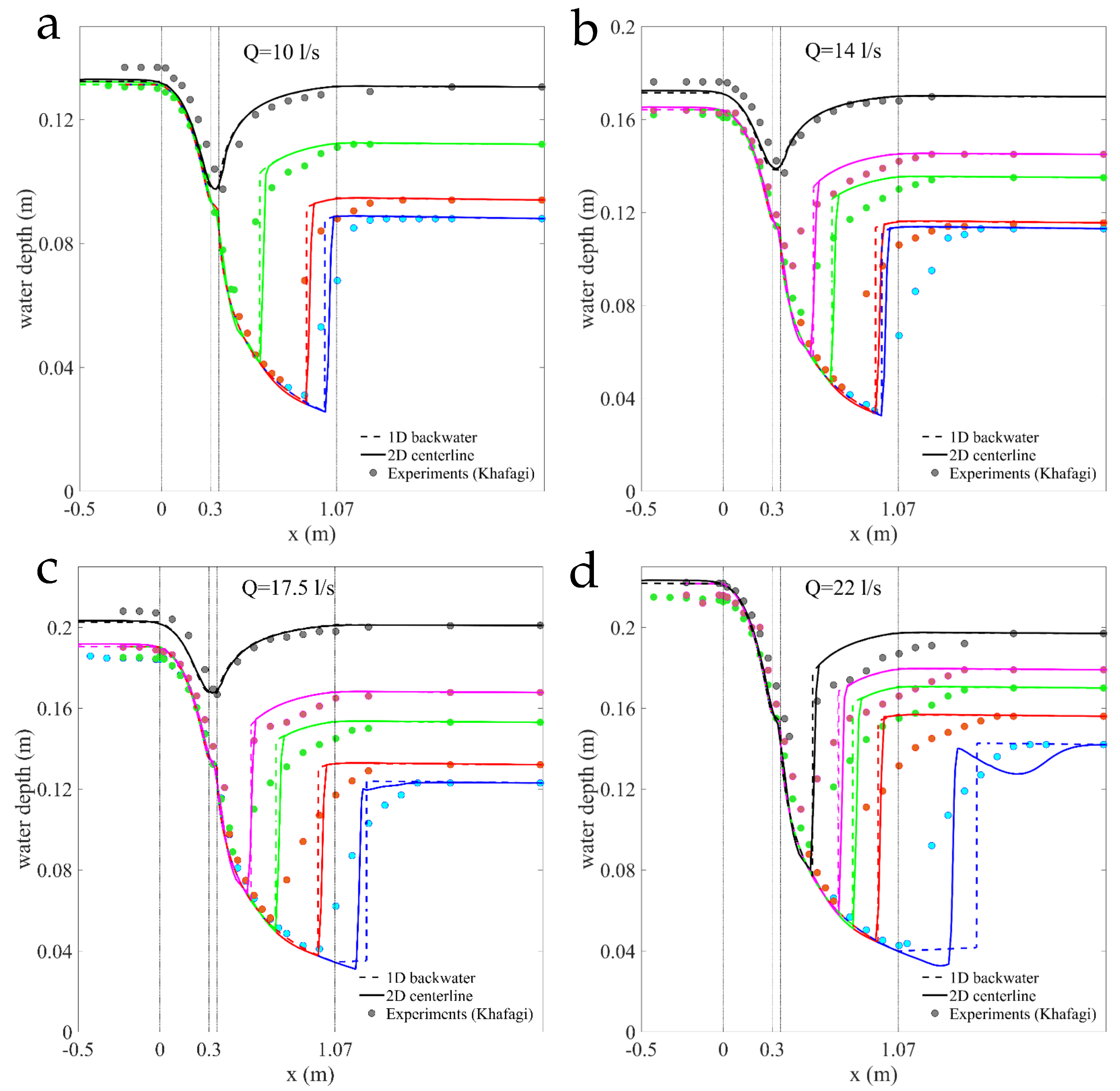
3.3. Gradually Varied Transcritical Flow in a Khafagi Flume
3.4. Predicting the Hydraulic Jump Position in the Khafagi’s Venturi Flume Experiments
4. Results: Transcritical Flow in Long Channels
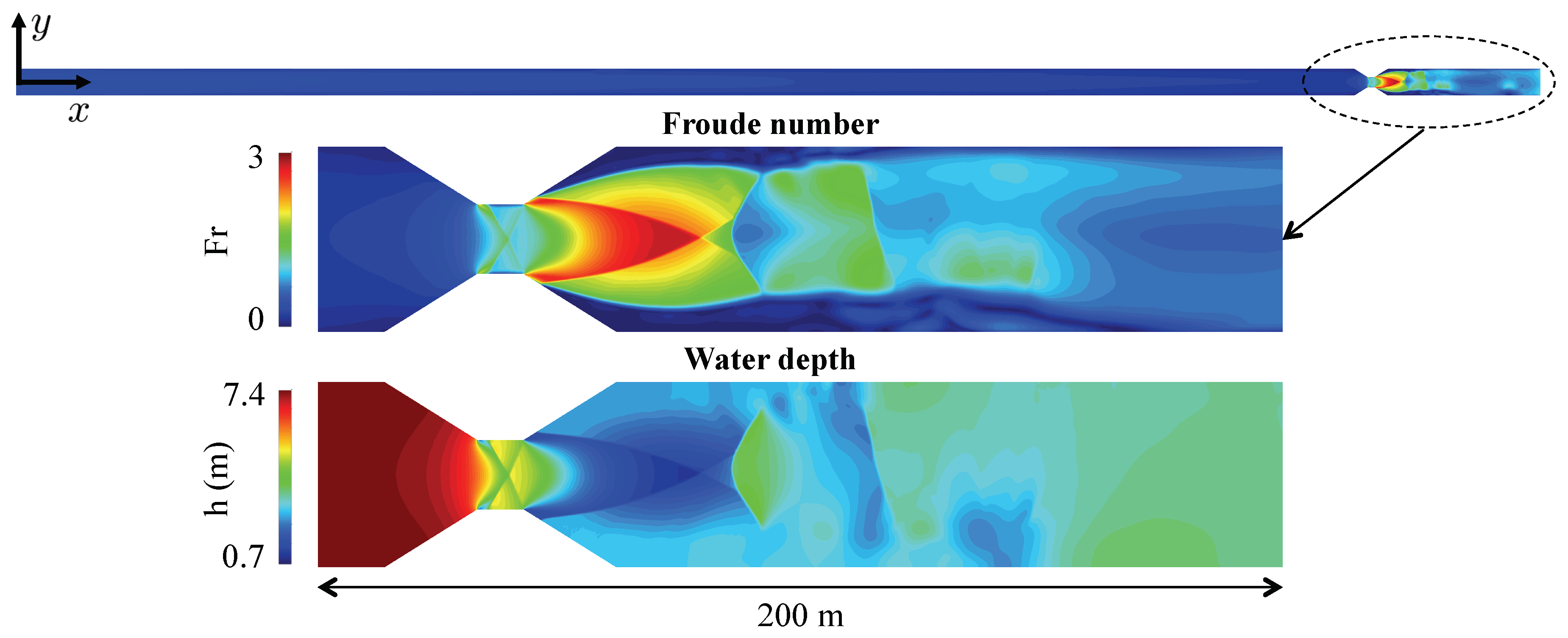
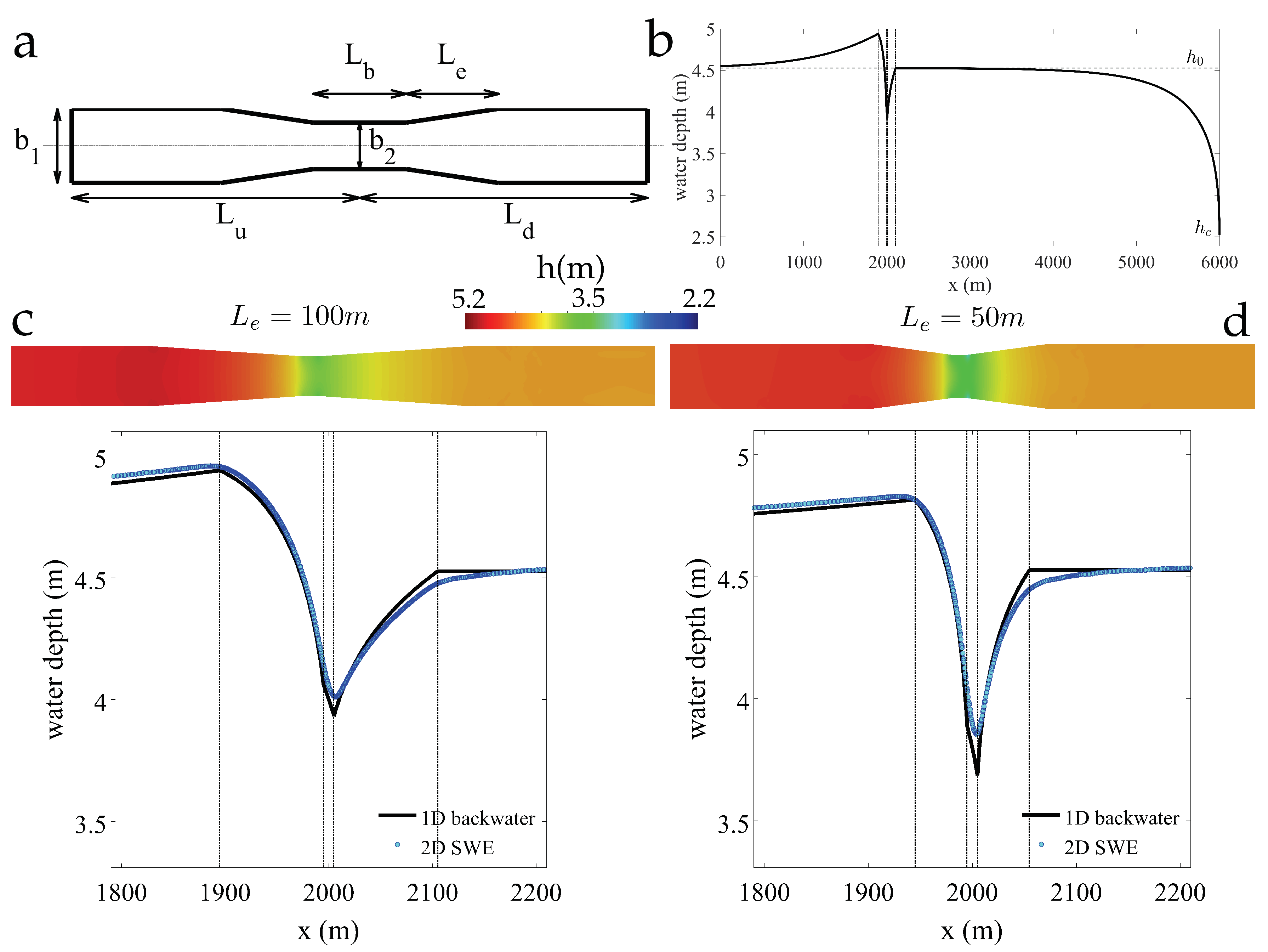
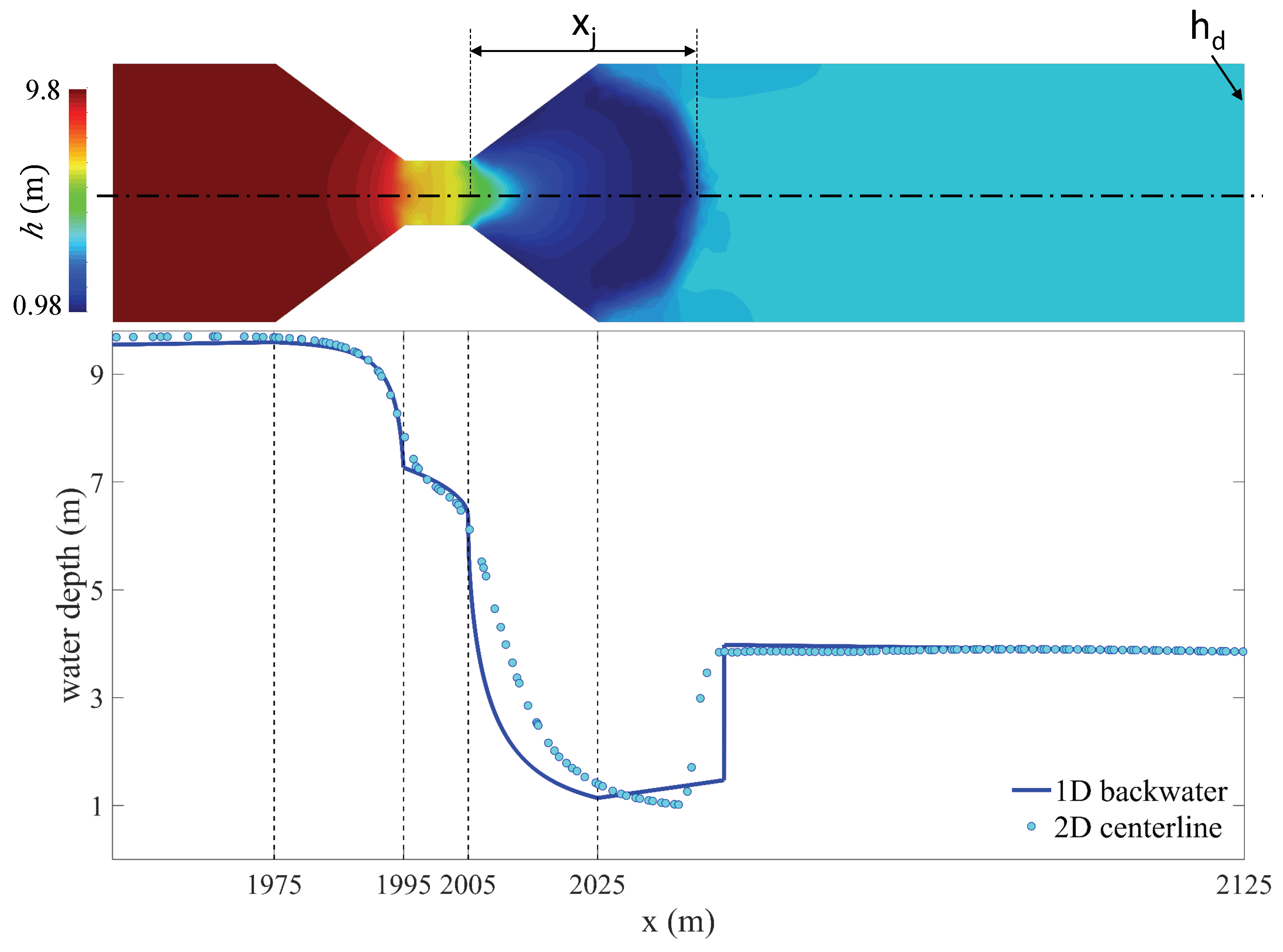
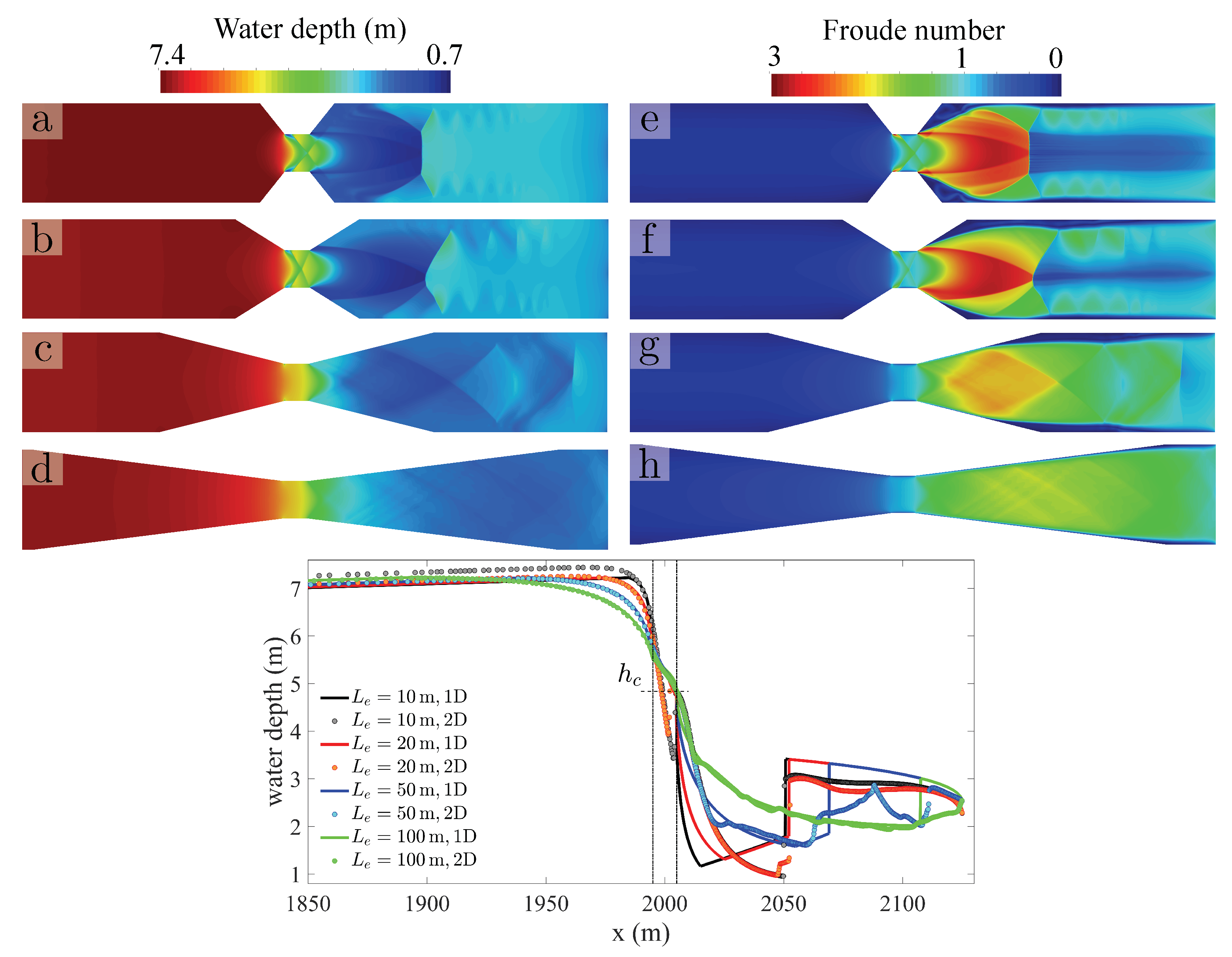
4.1. Predicting the Onset of Transcritical Flow and Jump Position in a Long Channel with a Linear Contraction
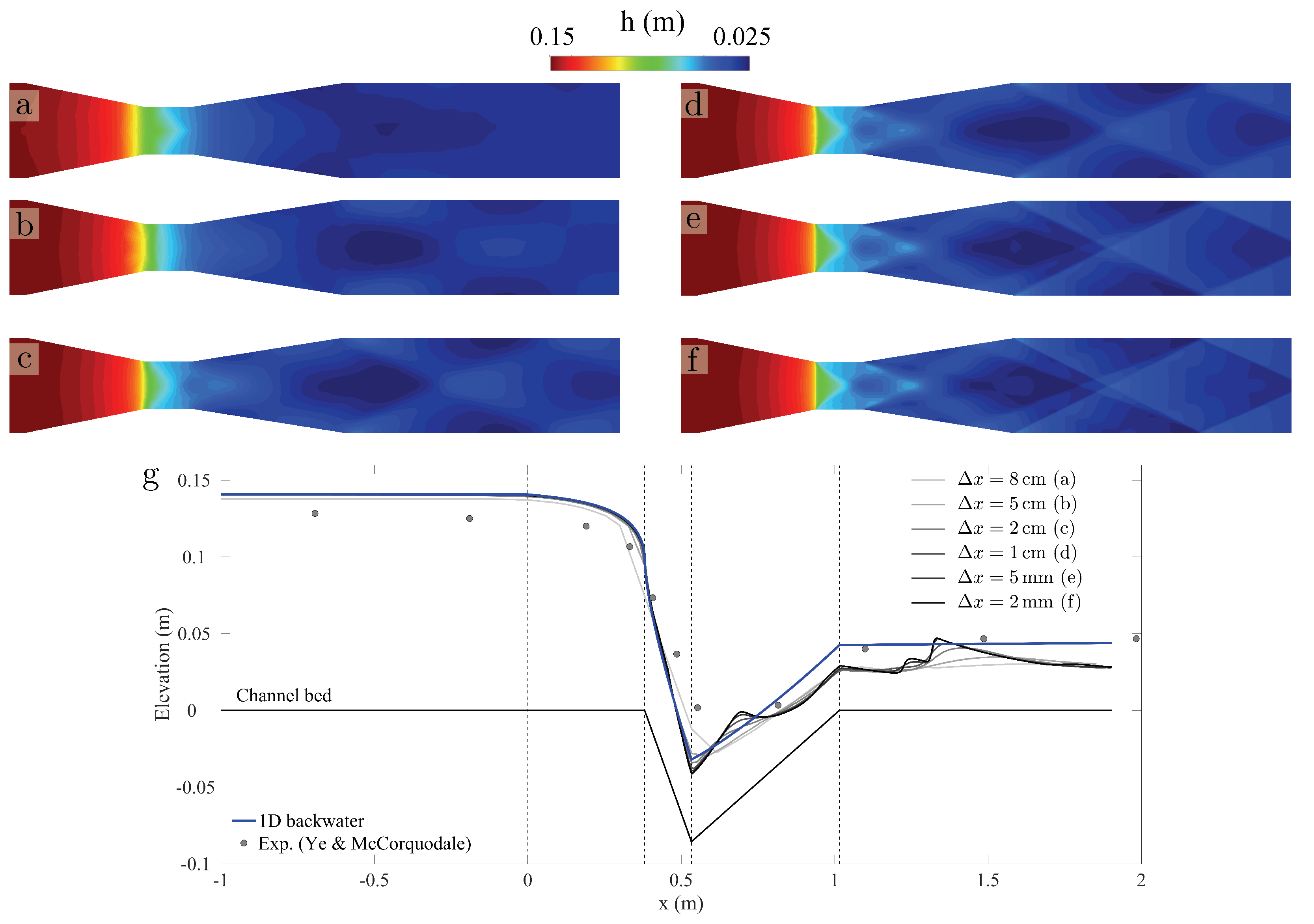
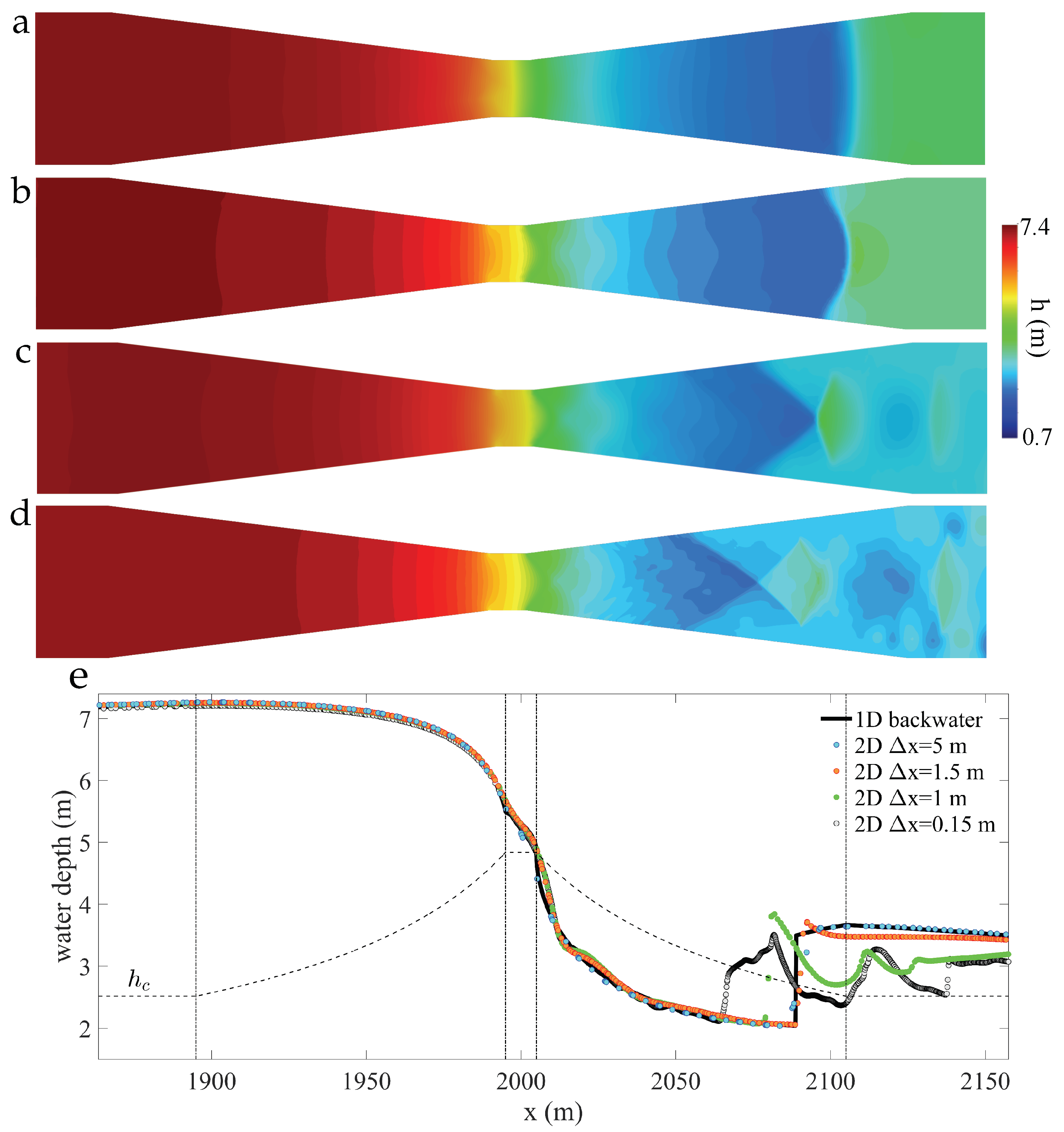
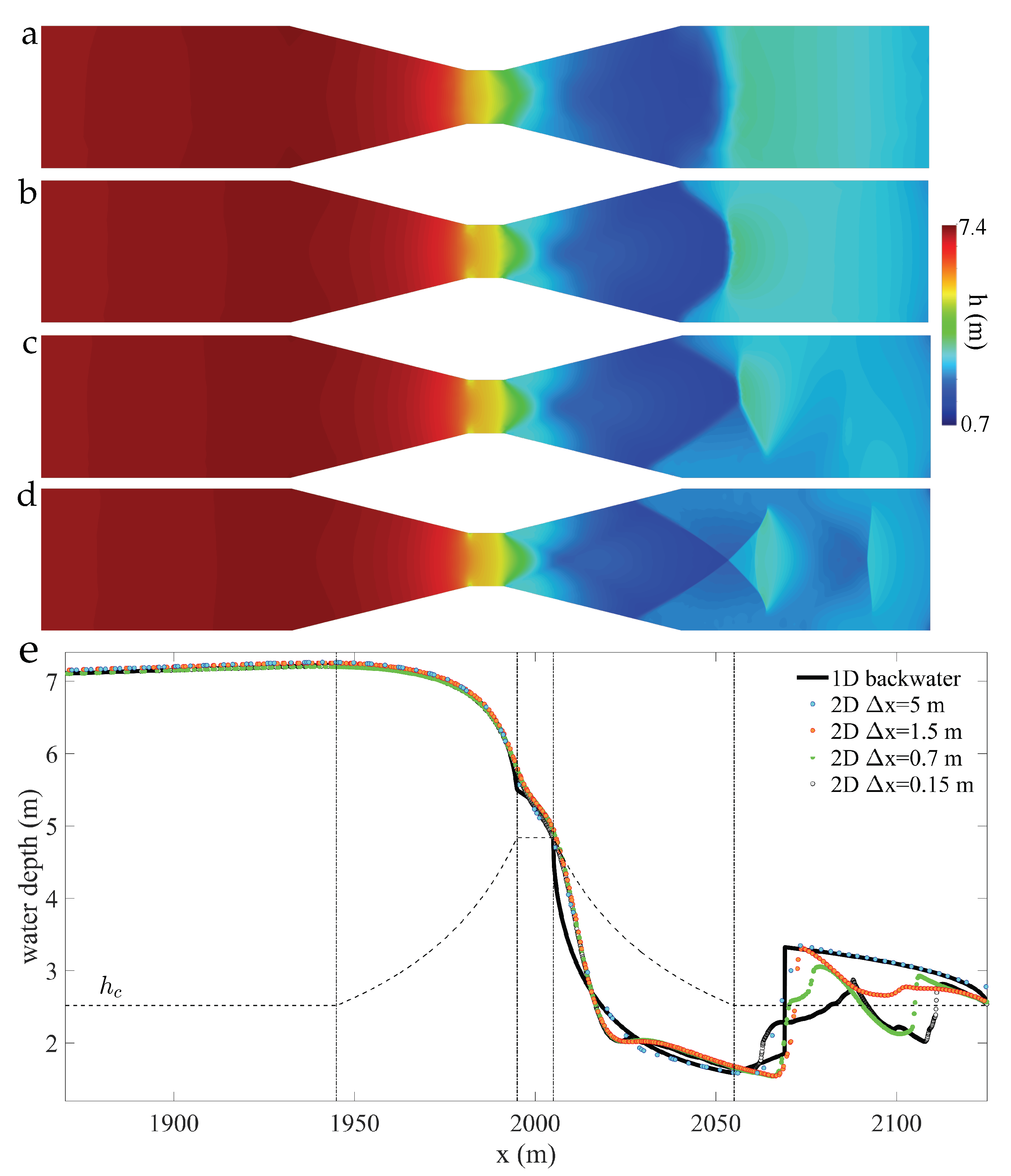
4.2. The Role of Grid Refinement on Capturing 2D Flow Features
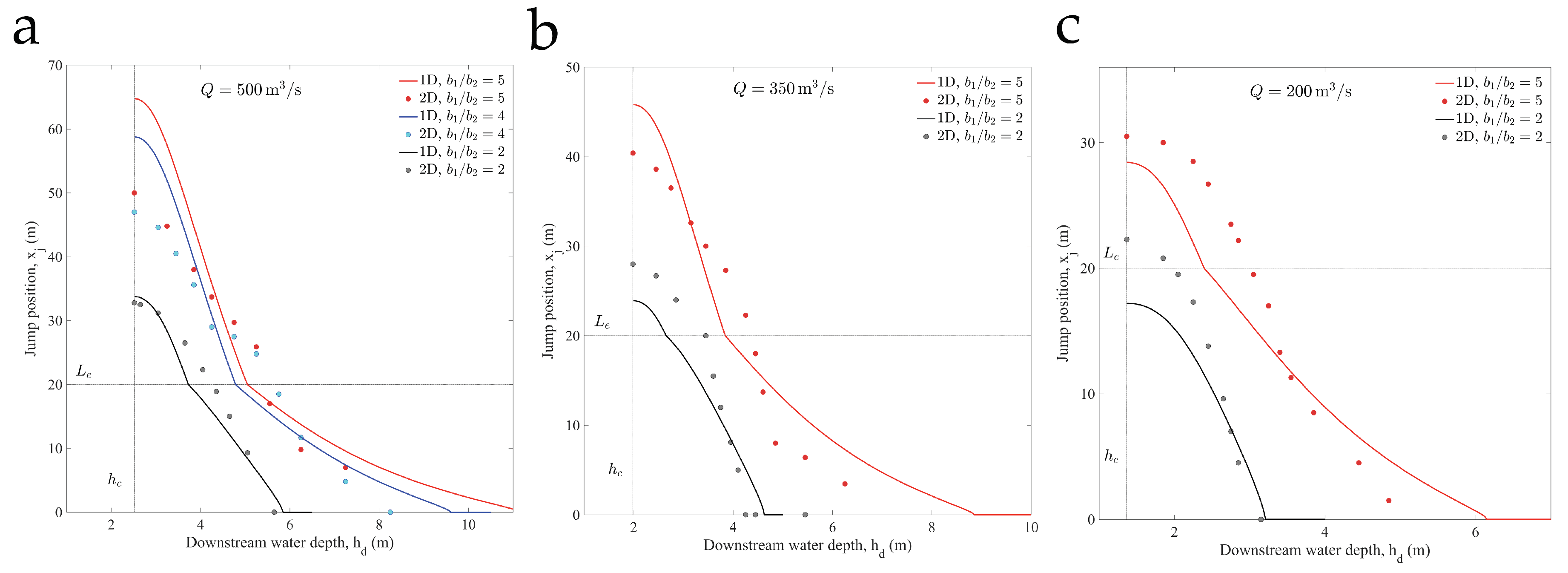
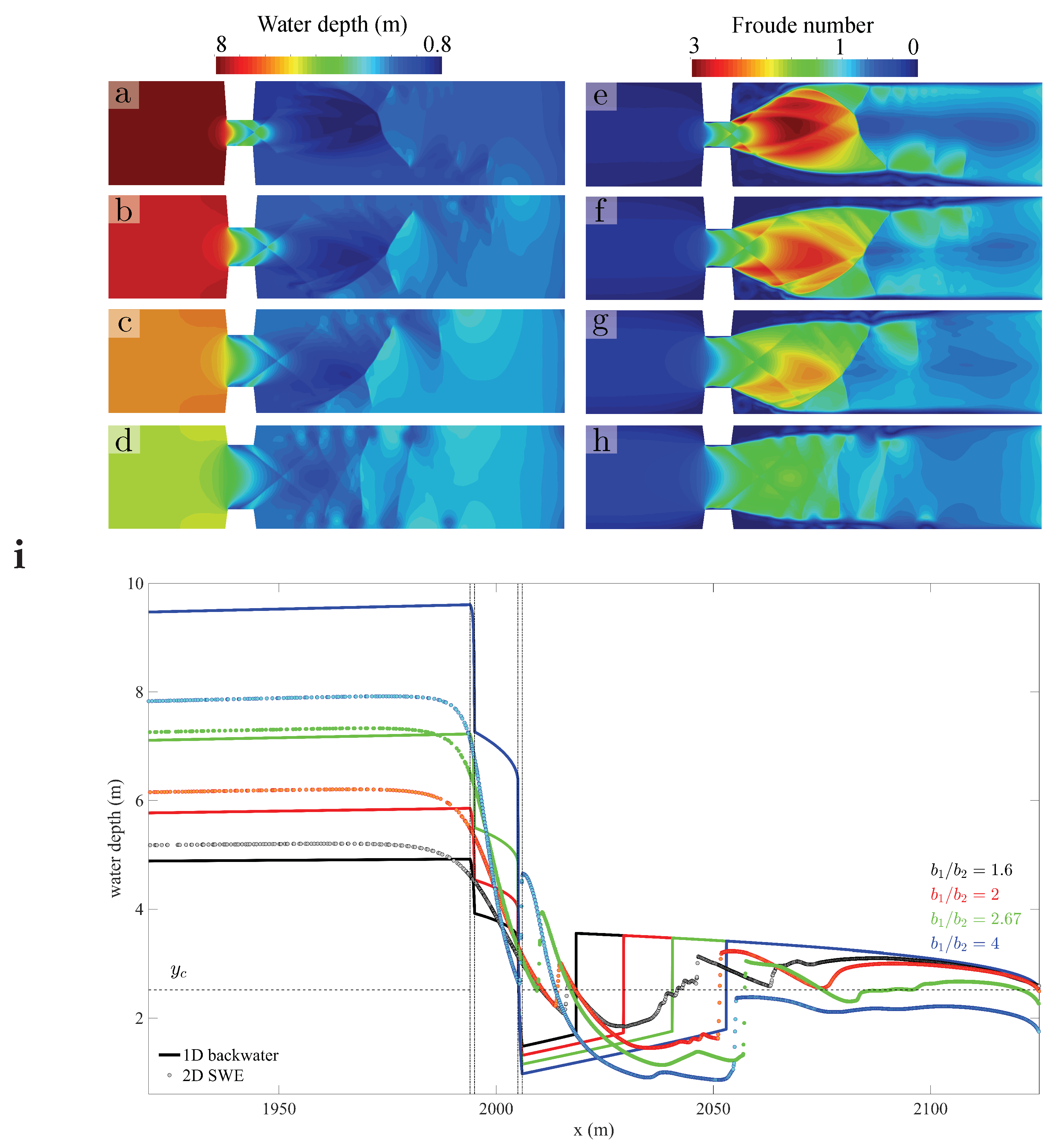
4.3. Influence of the Contraction Geometry on the Discrepancy between 1D and 2D Models
5. Discussion and Conclusions
- For transcritical flow past short, horizontal channels with relatively smooth contractions and negligible flow separation (e.g., for the experimental cases considered in the validation section), the deviations between models and experiments seem larger than among models. The discrepancy between models and experimental data is consistent with the well known limitations of the depth-averaged shallow-water model, in particular the impact of non-hydrostatic pressures and streamline curvature on the flow. The standard 1D theory shares this limitation.
- Considering its simplicity and negligible computational cost, the classical 1D theory performs remarkably well for a wide range of flow conditions and relatively smooth channel contractions. In particular, the 1D model yields a good prediction of the transition to supercritical flow at the contraction. Perhaps more importantly, the 1D model is more conservative, in the sense that it predicts an earlier onset of critical flow at the contraction as the tailwater depth decreases.
- The grid resolution used in the 2D SWE simulations plays an important role in capturing the spatial flow patterns, so that coarse grid 2D simulations provide essentially the same information as 1D ones. The implication of this observation is that the discrepancies among various 2D models with different spatial grid resolution may be as large as those between the 2D models and a classical 1D energy-momentum calculation. The impact of grid resolution on the agreement between 1D and 2D models is relevant in practice, as field-scale hydrodynamic models in fluvial dynamics rely on the available topography, whose spatial resolution is often limited to the meter scale.
Author Contributions
Funding
Conflicts of Interest
References
- Bakhmeteff, B.A. Hydraulics of Open Channels; McGraw-Hill: New York, NY, USA, 1932. [Google Scholar]
- Castro-Orgaz, O.; Sturm, T.W.; Boris, A. Bakhmeteff and the Development of Specific Energy and Momentum Concepts. J. Hydraul. Eng. 2018, 144, 02518004. [Google Scholar] [CrossRef]
- Chow, V.T. Open-Channel Hydraulics; McGraw-Hill: New York, NY, USA, 1959. [Google Scholar]
- Henderson, F.M. Open Channel Flow; Mcmillan Publishing Co., Inc.: New York, NY, USA, 1966. [Google Scholar]
- Chanson, H. The Hydraulics of Open Channel Flow: An Introduction, 2nd ed.; Elsevier Butterworth-Heinemann: Burlington, MA, USA, 2004. [Google Scholar]
- Sturm, T.W. Open Channel Hydraulics; McGraw-Hill: New York, NY, USA, 2001. [Google Scholar]
- Deltares. Simulation of Multidimensional Hydrodynamic Flows and Transport Phenomena, Including Sediments; User Manual; Deltares: Delft, The Netherlands, 2011. [Google Scholar]
- Bladé, E.; Cea, L.; Corestein, G.; Escolano, E.; Puertas, J.; Vázquez-Cendón, E.; Dolz, J.; Coll, A. Iber: Herramienta de simulación numérica del flujo en ríos. Rev. Int. Métodos Numér. Cálc. Diseño Ing. 2014, 30, 1–10. [Google Scholar] [CrossRef]
- Hager, W.H. Transitional Flow in Channel Junctions. J. Hydraul. Eng. 1989, 115, 243–259. [Google Scholar] [CrossRef]
- Hager, W.H.; Castro-Orgaz, O. Transcritical Flow in Open Channel Hydraulics: From Boss to De Marchi. J. Hydraul. Eng. 2016, 142, 02515003. [Google Scholar] [CrossRef]
- Chanson, H.; Montes, J.S. Characteristics of undular hydraulic jumps: Experimental apparatus and flow patterns. J. Hydraul. Eng. 1995, 121, 129–144. [Google Scholar] [CrossRef]
- Montes, J.S.; Chanson, H. Characteristics of Undular Hydraulic Jumps: Experiments and Analysis. J. Hydraul. Eng. 1998, 124, 192–205. [Google Scholar] [CrossRef]
- Ohtsu, I.; Yasuda, Y.; Gotoh, H. Flow Conditions of Undular Hydraulic Jumps in Horizontal Rectangular Channels. J. Hydraul. Eng. 2003, 129, 948–955. [Google Scholar] [CrossRef]
- Bose, S.K.; Castro-Orgaz, O.; Dey, S. Free Surface Profiles of Undular Hydraulic Jumps. J. Hydraul. Eng. 2012, 138, 362–366. [Google Scholar] [CrossRef]
- Ippen, A.T. High-Velocity Flow in Open Channels: A Symposium: Mechanics of Supercritical Flow. ASCE Trans. 1951, 116, 268–295. [Google Scholar]
- Ippen, A.T.; Dawson, J.H. Design of channel contractions. ASCE Trans. 1951, 116, 326–346. [Google Scholar]
- Ippen, A.T.; Harleman, D.R.F. Verification of Theory for Oblique Standing Waves. ASCE Trans. 1956, 121, 678–694. [Google Scholar]
- Behlke, C.E.; Pritchett, H.D. The Design of Supercritical Channel Junctions; Highway Res. Record, Nr. 123, Publication 1365, 17-35; Highway Research Board, National Research Council: Washington, DC, USA, 1966. [Google Scholar]
- Hager, W.H. Supercritical Flow in Channel Junctions. J. Hydraul. Eng. 1989, 115, 595–616. [Google Scholar] [CrossRef]
- Rouse, H.; Bhoota, B.V.; Hsu, E.Y. Design of channel expansions. ASCE Trans. 1951, 116, 347–363. [Google Scholar]
- Akers, B.; Bokhove, O. Hydraulic flow through a channel contraction: Multiple steady states. Phys. Fluids 2008, 20, 056601. [Google Scholar] [CrossRef]
- Defina, A.; Viero, D.P. Open channel flow through a linear contraction. Phys. Fluids 2010, 22, 036602. [Google Scholar] [CrossRef]
- Viero, D.P.; Defina, A. Extended Theory of Hydraulic Hysteresis in Open-Channel Flow. J. Hydraul. Eng. 2017, 143, 06017014. [Google Scholar] [CrossRef]
- Khafagi, A. Der Venturikanal: Theorie und Anwendung; Eidgenössiche Technishe Hochschule Zürich, Mitteilungen der Versuchsanstalt für Wasserbau und Erdbau: Zürich, Switzerland, 1942. [Google Scholar]
- Rajaratnam, N.; Subramanya, K. Hydraulic jumps below abrupt symmetrical expansions. J. Hydraul. Div. ASCE 1968, 94, 481–503. [Google Scholar]
- Bremen, R.; Hager, W.H. T-jump in abruptly expanding channel. J. Hydraul. Res. 1993, 31, 61–78. [Google Scholar] [CrossRef]
- Hager, W.H. Hydraulic jump in non-prismatic rectangular channels. J. Hydraul. Res. 1985, 23, 21–35. [Google Scholar] [CrossRef]
- Ferreri, G.B.; Nasello, C. Hydraulic jumps at drop and abrupt enlargement in rectangular channel. J. Hydraul. Res. 2002, 40, 491–505. [Google Scholar] [CrossRef]
- Toro, E.F. Shock-Capturing Methods for Free-Surface Shallow Flows; John Wiley & Sons, Ltd.: Chichester, UK, 2001. [Google Scholar]
- Zhou, J.G.; Causon, D.M.; Mingham, C.G.; Ingram, D.M. The Surface Gradient Method for the Treatment of Source Terms in the Shallow-Water Equations. J. Comput. Phys. 2001, 168, 1–25. [Google Scholar] [CrossRef]
- Fernández-Pato, J.; Morales-Hernández, M.; García-Navarro, P. Implicit finite volume simulation of 2D shallow water flows in flexible meshes. Comput. Methods Appl. Mech. Eng. 2018, 328, 1–25. [Google Scholar] [CrossRef]
- Jiménez, O.F.; Chaudhry, M.H. Computation of Supercritical Free-Surface Flows. J. Hydraul. Eng. 1988, 114, 377–395. [Google Scholar] [CrossRef]
- Gharangik, A.M.; Chaudhry, M.H. Numerical Simulation of Hydraulic Jump. J. Hydraul. Eng. 1991, 117, 1195–1211. [Google Scholar] [CrossRef]
- Zhou, J.G.; Stansby, P.K. 2D Shallow water flow model for the hydraulic jump. Int. J. Numer. Meth. Fluids 1999, 29, 375–387. [Google Scholar] [CrossRef]
- Cea, L.; Garrido, M.; Puertas, J. Experimental validation of two-dimensional depth-averaged models for forecasting rainfall–runoff from precipitation data in urban areas. J. Hydrol. 2010, 382, 88–102. [Google Scholar] [CrossRef]
- Cea, L.; Legout, C.; Darboux, F.; Esteves, M.; Nord, G. Experimental validation of a 2D overland flow model using high resolution water depth and velocity data. J. Hydrol. 2014, 513, 142–153. [Google Scholar] [CrossRef]
- Yoshioka, H.; Unami, K.; Fujihara, M. A finite element/volume method model of the depth-averaged horizontally 2D shallow water equations. Int. J. Numer. Meth. Fluids 2014, 75, 23–41. [Google Scholar] [CrossRef]
- Caviedes-Voulliéme, D.; García-Navarro, P.; Murillo, J. Influence of mesh structure on 2D full shallow water equations and SCS Curve Number simulation of rainfall/runoff events. J. Hydrol. 2012, 448, 39–59. [Google Scholar] [CrossRef]
- Hou, J.; Liang, Q.; Zhang, H.; Hinkelmann, R. An efficient unstructured MUSCL scheme for solving the 2D shallow water equations. Environ. Model. Softw. 2015, 66, 131–152. [Google Scholar] [CrossRef]
- Fernández-Pato, J.; Caviedes-Voulliéme, D.; García-Navarro, P. Rainfall/runoff simulation with 2D full shallow water equations: Sensitivity analysis and calibration of infiltration parameters. J. Hydrol. 2016, 536, 496–513. [Google Scholar] [CrossRef]
- Molls, T.; Chaudhry, M.H. Depth-Averaged Open-Channel Flow Model. J. Hydraul. Eng. 1995, 121, 453–465. [Google Scholar] [CrossRef]
- Rahman, M.; Chaudhry, M.H. Computation of flow in open-channel transitions. J. Hydraul. Res. 1997, 35, 243–256. [Google Scholar] [CrossRef]
- Ye, J.; McCorquodale, J.A. Depth-Averaged Hydrodynamic Model in Curvilinear Collocated Grid. J. Hydraul. Eng. 1997, 123, 380–388. [Google Scholar] [CrossRef]
- Abdo, K.; Riahi-Nezhad, C.K.; Imran, J. Steady supercritical flow in a straight-wall open-channel contraction. J. Hydraul. Res. 2018. [Google Scholar] [CrossRef]
- Zerihun, Y.T. A Numerical Study on Curvilinear Free Surface Flows in Venturi Flumes. Fluids 2016, 1, 21. [Google Scholar] [CrossRef]
- Berger, R.C.; Carey, G.F. Free-surface flow over curved surfaces Part II: Computational model. Int. J. Numer. Meth. Fluids 1998, 28, 201–213. [Google Scholar] [CrossRef]
- Shimozono, T.; Sato, S. Coastal vulnerability analysis during tsunami-induced levee overflow and breaching by a high-resolution flood model. Coast. Eng. 2016, 107, 116–126. [Google Scholar] [CrossRef]
- Zerihun, Y.T.; Fenton, J.D. One-dimensional simulation model for steady transcritical free surface flows at short length transitions. Adv. Water Resour. 2006, 29, 1598–1607. [Google Scholar] [CrossRef]
- Matthew, G.D. Higher order one-dimensional equations of potential flow in open channels. Proc. ICE 1991, 91, 187–201. [Google Scholar] [CrossRef]
- Castro-Orgaz, O.; Hager, W.H. One-dimensional modelling of curvilinear free surface flow: Generalized Matthew theory. J. Hydraul. Res. 2014, 52, 14–23. [Google Scholar] [CrossRef]
- Castro-Orgaz, O. Hydraulic design of Khafagi flumes. J. Hydraul. Res. 2008, 46, 691–698. [Google Scholar] [CrossRef]
- Castro-Orgaz, O.; Giráldez, J.V.; Ayuso, J.L. Transcritical Flow due to Channel Contraction. J. Hydraul. Eng. 2008, 134, 492–496. [Google Scholar] [CrossRef]
- Castro-Orgaz, O.; Hager, W.H. Critical Flow: A Historical Perspective. J. Hydraul. Eng. 2010, 136, 3–11. [Google Scholar] [CrossRef]
- Hager, W.H. Critical Flow Condition in Open Channel Hydraulics. Acta Mech. 1985, 54, 157–179. [Google Scholar] [CrossRef]
- Cea, L.; Puertas, J.; Vázquez-Cendón, M.E. Depth Averaged Modelling of Turbulent Shallow Water Flow with Wet-Dry Fronts. Arch. Comput. Methods Eng. 2007, 14, 303–341. [Google Scholar] [CrossRef]
- Van Leer, B. Towards the Ultimate Conservative Difference Scheme, V. A Second Order Sequel to Godunov’s Method. J. Comput. Phys. 1979, 32, 101–136. [Google Scholar] [CrossRef]
- Alcrudo, A.; García-Navarro, P. A High Resolution Godunov–Type Scheme in Finite Volumes for the 2D Shallow Water Equations. Int. J. Numer. Meth. Eng. 1993, 16, 489–505. [Google Scholar] [CrossRef]
- García-Navarro, P.; Hubbard, M.E.; Priestley, A. Genuinely Multidimensional Upwinding for the 2D Shallow Water Equations. J. Comput. Phys. 1995, 121, 79–93. [Google Scholar] [CrossRef]
- Toro, E.F.; García-Navarro, P. Godunov–Type Methods for Free–Surface Shallow Flows: A Review. J. Hydraul. Res. 2007, 45, 736–751. [Google Scholar] [CrossRef]
- Cueto-Felgueroso, L.; Colominas, I.; Fe, J.; Navarrina, F.; Casteleiro, M. High-order finite volume schemes on unstructured grids using moving least-squares reconstruction. Application to shallow water dynamics. Int. J. Numer. Meth. Engng. 2006, 65, 295–331. [Google Scholar] [CrossRef]
- Cueto-Felgueroso, L.; Colominas, I. High-order finite volume methods and multiresolution reproducing kernels. Arch. Comput. Meth. Eng. 2008, 15, 185–228. [Google Scholar] [CrossRef]
- García-Navarro, P.; Vázquez-Cendón, M.E. On numerical treatment of the source terms in the shallow water equations. Comput. Fluids 2000, 29, 951–979. [Google Scholar] [CrossRef]
- Canestrelli, A.; Dumbser, M.; Siviglia, A.; Toro, E.F. Well-balanced high-order centered schemes on unstructured meshes for shallow water equations with fixed and mobile bed. Adv. Water Resour. 2010, 33, 291–303. [Google Scholar] [CrossRef]
- Chanson, H. Current knowledge in hydraulic jumps and related phenomena. A survey of experimental results. Eur. J. Mech. B/Fluids 2009, 28, 191–210. [Google Scholar] [CrossRef]
- Hager, W.H.; Hutter, K. Approximate treatment of the plane hydraulic jump with separation zone above the flow zone. J. Hydraul. Res. 1983, 21, 195–204. [Google Scholar] [CrossRef]



© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cueto-Felgueroso, L.; Santillán, D.; García-Palacios, J.H.; Garrote, L. Comparison between 2D Shallow-Water Simulations and Energy-Momentum Computations for Transcritical Flow Past Channel Contractions. Water 2019, 11, 1476. https://doi.org/10.3390/w11071476
Cueto-Felgueroso L, Santillán D, García-Palacios JH, Garrote L. Comparison between 2D Shallow-Water Simulations and Energy-Momentum Computations for Transcritical Flow Past Channel Contractions. Water. 2019; 11(7):1476. https://doi.org/10.3390/w11071476
Chicago/Turabian StyleCueto-Felgueroso, Luis, David Santillán, Jaime H. García-Palacios, and Luis Garrote. 2019. "Comparison between 2D Shallow-Water Simulations and Energy-Momentum Computations for Transcritical Flow Past Channel Contractions" Water 11, no. 7: 1476. https://doi.org/10.3390/w11071476
APA StyleCueto-Felgueroso, L., Santillán, D., García-Palacios, J. H., & Garrote, L. (2019). Comparison between 2D Shallow-Water Simulations and Energy-Momentum Computations for Transcritical Flow Past Channel Contractions. Water, 11(7), 1476. https://doi.org/10.3390/w11071476







