Physics-Based Simulation of Hydrologic Response and Sediment Transport in a Hilly-Gully Catchment with a Check Dam System on the Loess Plateau, China
Abstract
1. Introduction
2. Materials and Methods
2.1. Study Site and Data
2.2. The Integrated Hydrology Model (InHM)
2.3. Scenario Setting and Modelling Procedure
2.3.1. Calibration and Validation Simulations in WMG Catchment
2.3.2. Annual Continuous Simulations in GDG Catchment
2.4. Model Settings and Parameters
2.4.1. Boundary Conditions and Initial Conditions
2.4.2. Soil Parameters
3. Results and Discussion
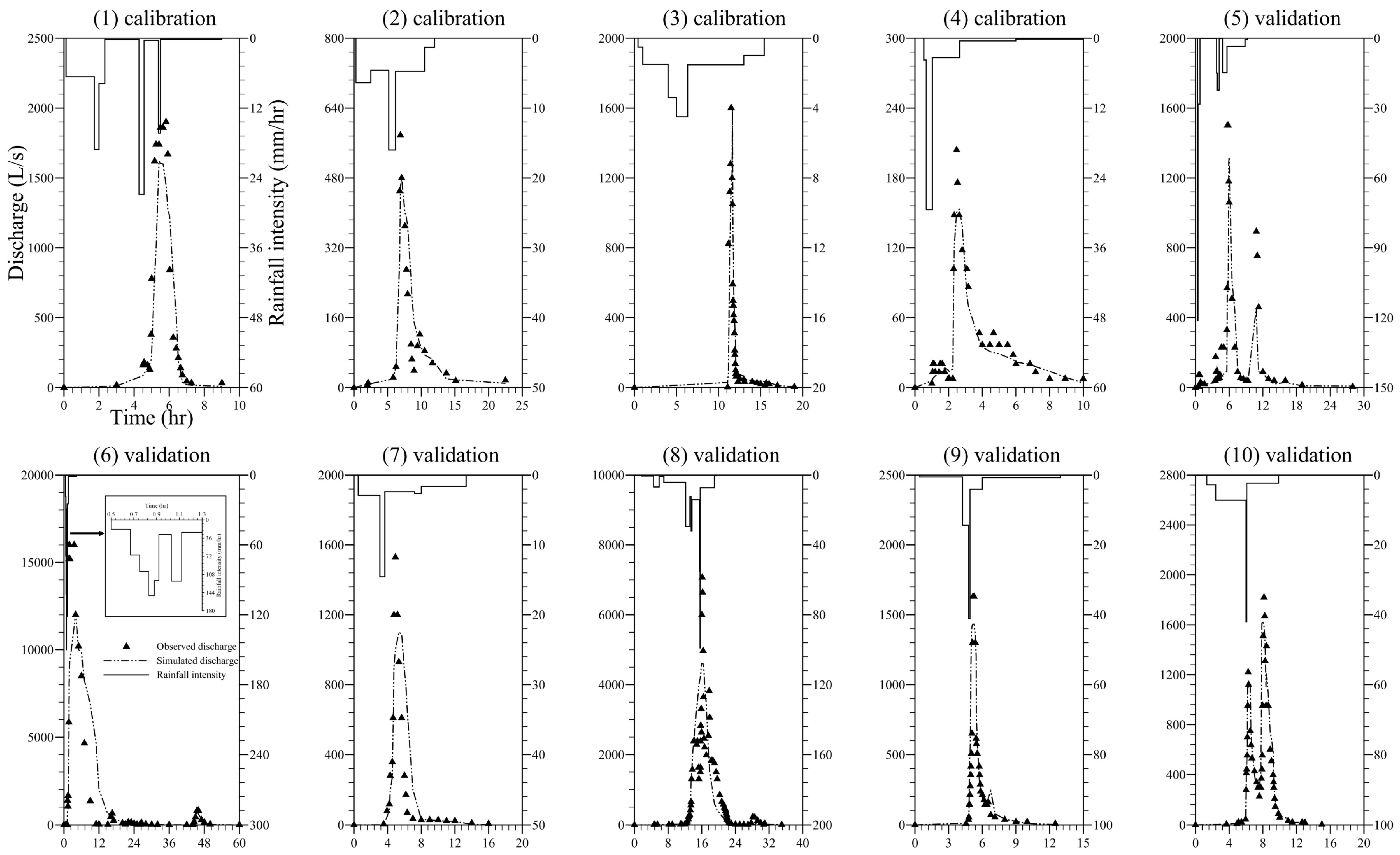
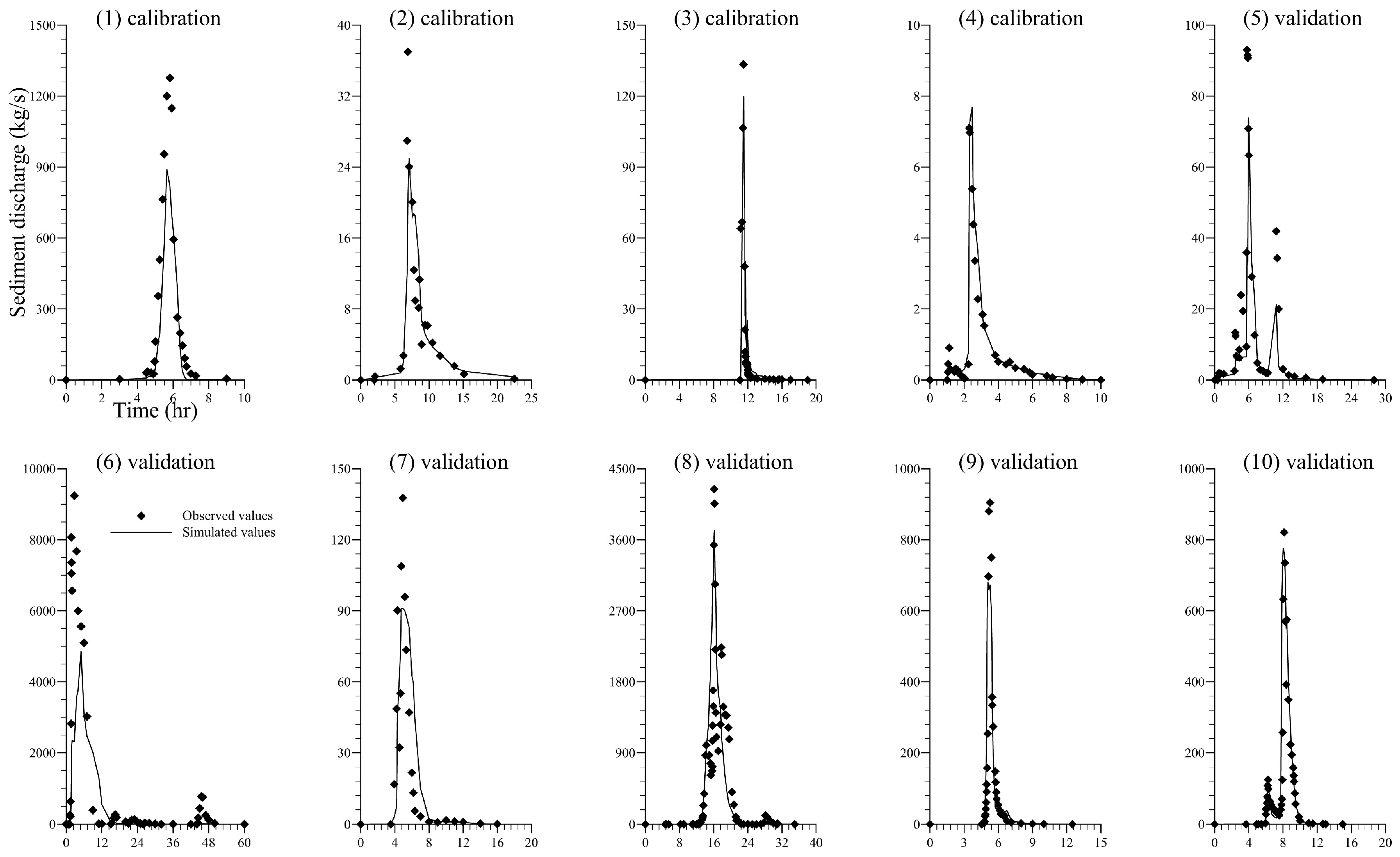
3.1. Results of Calibration and Validation in WMG Catchment
3.2. Water Redistribution in Long-Term Simulations of GDG Catchment
- Surface runoff decreased significantly after the construction of GDG1 dam (i.e., averagely reduced by 12.74%) and remained a low percentage in the following 12 simulation years.
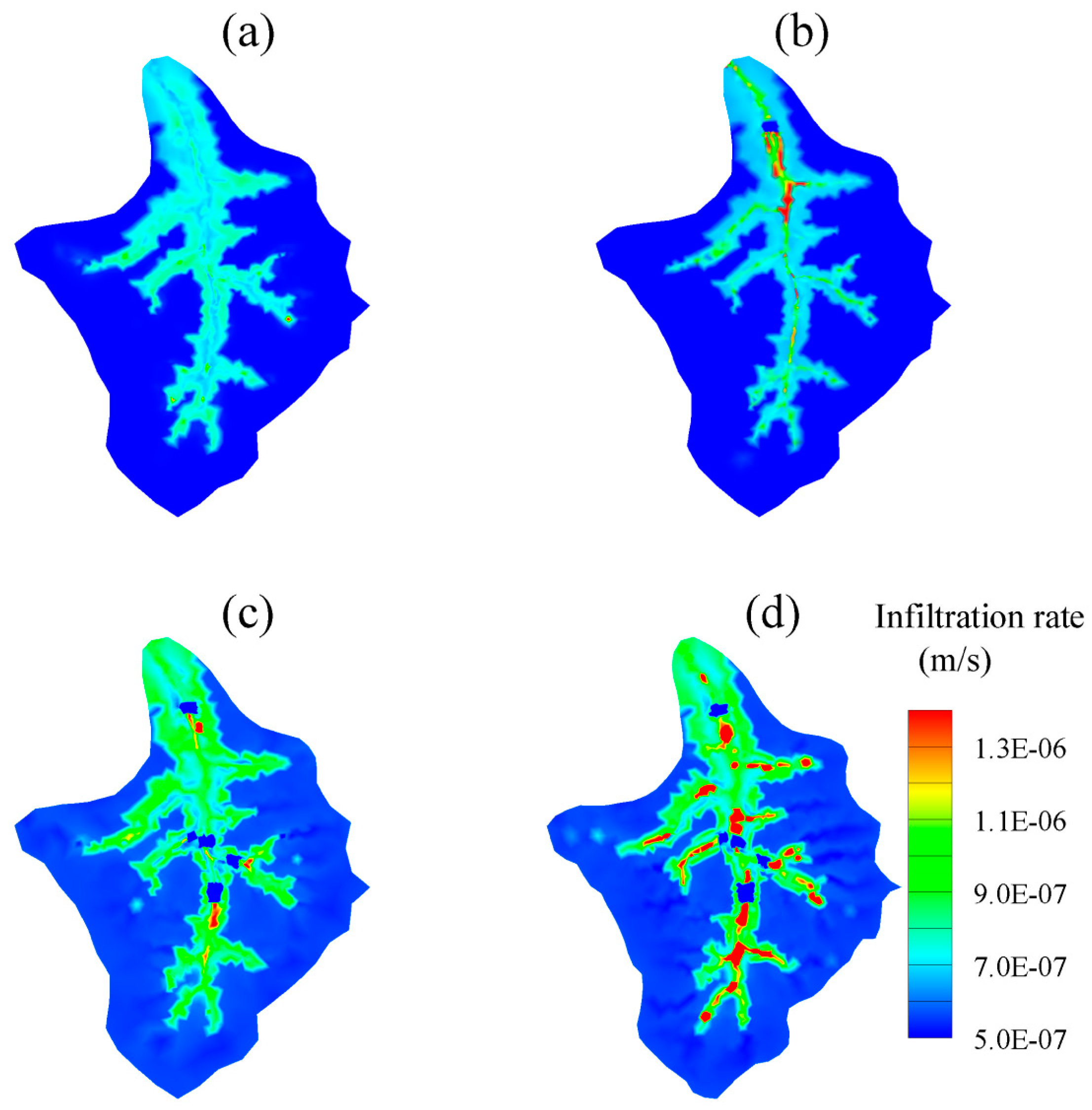
- Surface storage responded in two different ways in the four scenarios. Surface storage dramatically increased by 8.94% and 18.37% immediately at the end of the first year in SD and EDS scenarios, indicating a large amount of stormwater during the rainy seasons of 1955 and 1959 was retained behind check dams. Comparing to the large growth in 1955 and 1959, small increases of surface storage occurred in the following years of SD and EDS scenarios (i.e., 1956, 1957, 1958, 1960, and 1962). PD and CDS scenarios had similarly slight surface storage increases (i.e., average changes were +0.90% and +1.53%, respectively) for different reasons: (1) surface runoff left the gully without the interception of dams in PD scenario; (2) surface water was retained behind dams and infiltrated into subsurface quickly in CDS scenario. (See the expanding permeable sedimentary layers with relatively high infiltration rate in Figure 7)
- Subsurface outflow increased to 7.17% after the construction of GDG1 dam. The mean annual subsurface outflow was 33.5 mm (7.17%) and 54.0 mm (11.49%) for SD and EDS scenarios, increasing by 4.32% after the construction of four more dams. The subsurface outflow remained stable in CDS scenario, fluctuating slightly between wet years and dry years.
- Subsurface storage started increasing significantly in 1956, and fluctuating slightly between dry years and wet years. The check dams’ role in transforming from a surface reservoir to subsurface reservoir was obvious when comparing the storage-change tendencies of the surface and subsurface.
- Evapotranspiration, the largest term in GDG water balance, showed a slight decline from PD to CDS scenarios. It could be explained by the enhancement of infiltration in the sedimentary areas, which reduced the residence time of rainfall as surface water. In SD and EDS scenarios, dry years showed higher ET proportions than wet years.
3.3. Impacts of Check Dams on Groundwater in Long-Term Simulations of GDG Catchment
- The water table rose sharply at 200 m upstream from the dam heel of GDG1 and dropped sharply at the dam toe at the end of 1955 (see the green dash line with square symbols in Figure 8), indicating the formation a “subsurface reservoir”.
- Surface ponding was found in the first year of SD scenario (also see the green line in Figure 8) and disappeared in the following years.
- The position where water table started rising moved upstream to the location 320 m away from the dam heel of GDG1 at the end of 1958 (see the orange dash line with diamond symbols in Figure 8) and remained there in EDS scenario (Figure 9), indicating the expansion of “subsurface reservoir” referred above.
- The water table downstream the dam averagely increased by 2.0 m and 0.6 m from the 1st year to the 4th year in SD and EDS scenarios, respectively. However, the water table in the upstream reach remained stable in the SD scenario but averagely rose by 1.0 m in the EDS scenario (see Figure 8 and Figure 9).
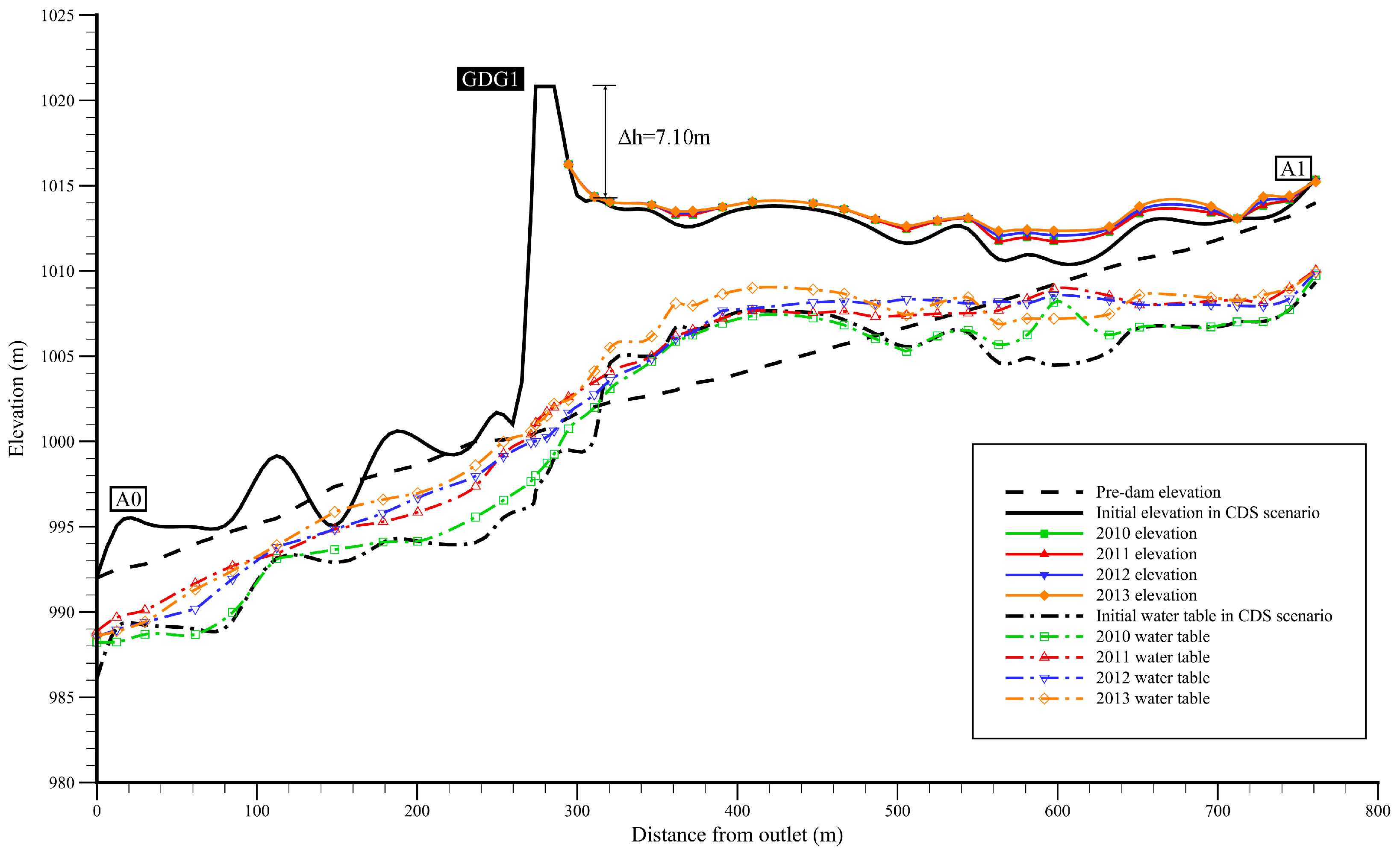
- The water table profiles in the CDS scenario (Figure 10) were different from those in the former two scenarios. First, the dam’s impact that caused the water table rising sharply disappeared in CDS scenario. Second, the water table along the gully had risen by 3~5 m both in the upstream reach and in the downstream reach. Third, the water table at the dam toe was relatively high and might expose as springs, which was actually observed during the field survey in April 2014.
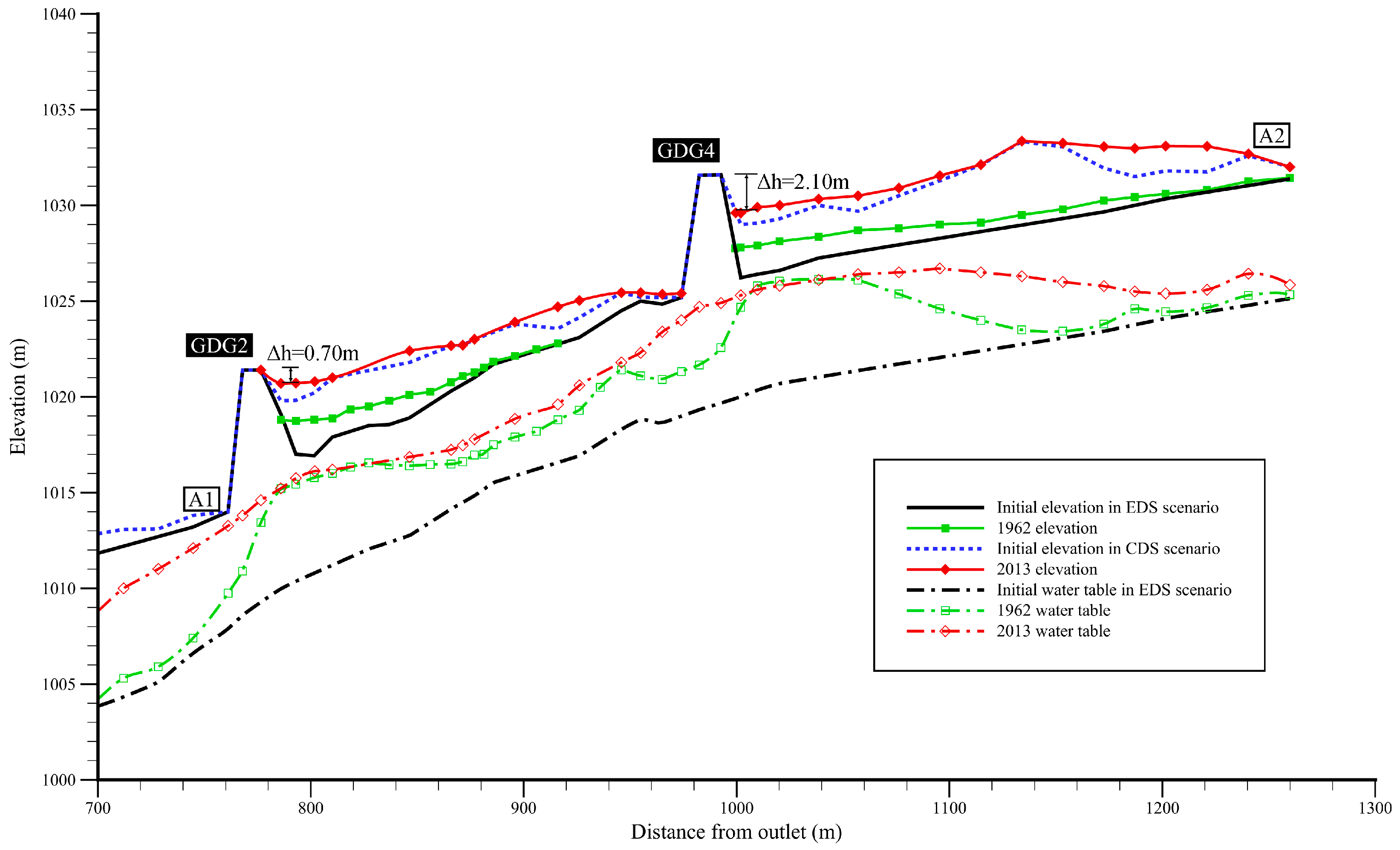
- The water table in front of GDG2 and GDG4 in EDS scenario showed similar profile to the water table in front of GDG1 in SD scenario (see the green dash lines with square symbols in Figure 8 and Figure 11). However, the intermediate reach of the two dams (i.e., the reach ranging from 880 m to 980 m away from the outlet) experienced a higher water table lifting (3.0 m averagely), indicating a promotion effect of water table rising by two adjacent check dams (Figure 11).
3.4. Impacts of Check Dams on Sediment Transport in Long-Term Simulations of GDG Catchment
- Deposition in front of GDG1 occurred quickly in SD scenario (1955–1958). The 10 m high check dam was nearly fully deposited and facing the risk of dam-break at the end of 1958 (Figure 8).
- Being heightened by 13 m before the rainy season of 1959, GDG1 was prevented from being over-deposited during the rainy season of 1959 (Figure 9). Less sediment silted in front of GDG1 in EDS scenario due to the existence of the four newly-built dams.
- Comparing to PD scenario, a sedimentary layer which has an average thickness of 8 m formed in front of GDG1 dam at the beginning of CDS scenario (see the black solid line in Figure 10). The flat sedimentary area with relatively high soil water content was turned into productive croplands. Long-term alternative erosion and sedimentary formed the undulating terrain in the downstream reach of GDG1. More sediment deposited in the upper reach 600 m away from the outlet rather than at the dam heel for GDG1.
- GDG1, which formed productive croplands, had a residual deposition height (Δh) of 7.10 m at the end of CDS scenario. However, the other four check dams all had been almost fully-filled and were vulnerable to floods.
- Table 9 also shows that most of the simulated Δh values were higher than the observed values (except for GDG3 in 1962, GDG2 and GDG4 in 2013), indicating that the sediment volumes deposited in front of dams were underestimated in most simulations.
3.5. Model Performance Evaluation: Influence of Gravity Erosion
4. Conclusions
- (1)
- Check dams do change the water redistribution in catchment-scale. GDG1 check dam near the gully outlet can effectively reduce surface outflow and increase subsurface outflow. The four other check dams located in the upstream can protect GDG1 from floods risk and promote the redistribution of water.
- (2)
- The construction of check dam can significantly change the water table profile along the gully. A surface reservoir behind the dam will be formed in the first few years and gradually transformed into a subsurface reservoir, resulting in relatively high soil water content in the sedimentary areas. After more than 50 years of operation, the water table along the gully has been averagely elevated by 3–5 m. Adjacent check dams have a promoting effect on water table rising.
- (3)
- Check dams intercept surface water and force sediment in the water to deposit. Gully erosion can be alleviated indirectly due to the formation of expanding sedimentary areas.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A. The Integrated Hydrologic Model (InHM)
Appendix A.1. Hydrologic-Response Module
Appendix A.2. Sediment-Transport Module
References
- Shi, H.; Shao, M. Soil and water loss from the Loess Plateau in China. J. Arid Environ. 2000, 45, 9–20. [Google Scholar] [CrossRef]
- Xin, Z.; Xu, J.; Zheng, W. Spatiotemporal variations of vegetation cover on the Chinese Loess Plateau (1981–2006): Impacts of climate changes and human activities. Sci. China Ser. D Earth Sci. 2008, 51, 67–78. [Google Scholar] [CrossRef]
- Xu, X.; Zhang, H.; Zhang, O. Development of check dam systems in gullies on the Loess Plateau, China. Environ. Sci. Policy 2004, 7, 79–86. [Google Scholar]
- Jin, Z.; Cui, B.; Song, Y.; Shi, W.; Wang, K.; Wang, Y.; Liang, J. How Many Check Dams Do We Need To Build on the Loess Plateau? Environ. Sci. Technol. 2012, 46, 8527–8528. [Google Scholar] [CrossRef] [PubMed]
- Wang, Y.F.; Fu, B.J.; Chen, L.D.; Lu, Y.H.; Gao, Y. Check Dam in the Loess Plateau of China: Engineering for Environmental Services and Food Security. Environ. Sci. Technol. 2011, 45, 10298–10299. [Google Scholar] [CrossRef] [PubMed]
- Xu, Y.; Fu, B.; He, C. Assessing the hydrological effect of the check dams in the Loess Plateau, China, by model simulations. Hydrol. Earth Syst. Sci. 2013, 17, 2185–2193. [Google Scholar] [CrossRef]
- Shi, H.; Li, T.; Wang, K.; Zhang, A.; Wang, G.; Fu, X. Physically-based simulation of the streamflow decrease caused by sediment-trapping dams in the middle Yellow River. Hydrol. Process. 2015, 30, 783–794. [Google Scholar] [CrossRef]
- Boix-Fayos, C.; de Vente, J.; Martínez-Mena, M.; Barberá, G.G.; Castillo, V. The impact of land use change and check dams on catchment sediment yield. Hydrol. Process. 2008, 22, 4922–4935. [Google Scholar] [CrossRef]
- Ran, D.-C.; Luo, Q.-H.; Zhou, Z.-H.; Wang, G.-Q.; Zhang, X.-H. Sediment retention by check dams in the Hekouzhen-Longmen Section of the Yellow River. Int. J. Sediment Res. 2008, 23, 159–166. [Google Scholar] [CrossRef]
- Shi, H.; Wang, G. Impacts of climate change and hydraulic structures on runoff and sediment discharge in the middle Yellow River. Hydrol. Process. 2015, 29, 3236–3246. [Google Scholar] [CrossRef]
- Zhao, P.; Shao, M.; Wang, T. Spatial distributions of soil surface-layer saturated hydraulic conductivity and controlling factors on dam farmlands. Water Resour. Manag. 2010, 24, 2247–2266. [Google Scholar] [CrossRef]
- Callow, J.N.; Smettem, K.R.J. The effect of farm dams and constructed banks on hydrologic connectivity and runoff estimation in agricultural landscapes. Environ. Model. Softw. 2009, 24, 959–968. [Google Scholar] [CrossRef]
- Parimalarenganayaki, S.; Elango, L. Assessment of effect of recharge from a check dam as a method of Managed Aquifer Recharge by hydrogeological investigations. Environ. Earth. Sci. 2015, 73, 5349–5361. [Google Scholar] [CrossRef]
- Huang, J.; Hinokidani, O.; Yasuda, H.; Ojha, C.P.; Kajikawa, Y.; Li, S. Effects of the check dam system on water redistribution in the Chinese Loess Plateau. J. Hydrol. Eng. 2013, 18, 929–940. [Google Scholar] [CrossRef]
- Gao, H. Hydro-Ecological Impact of the Gully Erosion Control Works in Loess Hilly-Gully Region. Ph.D. Thesis, The University of Chinese Academy of Sciences, Beijing, China, 2013. [Google Scholar]
- Shaanxi Administration of Surveying, Mapping and Geoinformation. Available online: http://www.shasm.gov.cn/ (accessed on 4 April 2014).
- Freeze, R.A.; Harlan, R.L. Blueprint for a physically-based, digitally-simulated hydrologic response model. J. Hydrol. 1969, 9, 237–258. [Google Scholar] [CrossRef]
- Heppner, C.S.; Ran, Q.; VanderKwaak, J.E.; Loague, K. Adding sediment transport to the integrated hydrology model (InHM): Development and testing. Adv. Water Resour. 2006, 29, 930–943. [Google Scholar] [CrossRef]
- Ran, Q.; Heppner, C.S.; VanderKwaak, J.E.; Loague, K. Further testing of the integrated hydrology model (InHM): Multiple-species sediment transport. Hydrol. Process. 2007, 21, 1522–1531. [Google Scholar] [CrossRef]
- VanderKwaak, J.E. Numerical Simulation of Flow and Chemical Transport in Integrated Surface-Subsurface Hydrologic Systems. Ph.D. Thesis, University of Waterloo, Waterloo, ON, Canada, 1999. [Google Scholar]
- VanderKwaak, J.E.; Loague, K. Hydrologic-response simulations for the R-5 catchment with a comprehensive physics-based model. Water Resour. Res. 2001, 37, 999–1013. [Google Scholar] [CrossRef]
- Carr, A.E.; Loague, K.; VanderKwaak, J.E. Hydrologic-response simulations for the North Fork of Caspar Creek: Second-growth, clear-cut, new-growth, and cumulative watershed effect scenarios. Hydrol. Process. 2014, 28, 1476–1494. [Google Scholar] [CrossRef]
- Mirus, B.B.; Ebel, B.A.; Loague, K.; Wemple, B.C. Simulated effect of a forest road on near-surface hydrologic response: Redux. Earth Surf. Process. Landf. 2007, 32, 126–142. [Google Scholar] [CrossRef]
- Heppner, C.S.; Loague, K. A dam problem: Simulated upstream impacts for a Searsville-like watershed. Ecohydrology 2008, 1, 408–424. [Google Scholar] [CrossRef]
- Ran, Q.; Loague, K.; VanderKwaak, J.E. Hydrologic-response-driven sediment transport at a regional scale, process-based simulation. Hydrol. Process. 2012, 26, 159–167. [Google Scholar] [CrossRef]
- Nash, J.E.; Sutcliffe, J.V. River flow forecasting through conceptual models part 1-a discussion of principles. J. Hydrol. 1970, 10, 282–290. [Google Scholar] [CrossRef]
- Ran, Q. Regional Scale Landscape Evolution: Physics-Based Simulation of Hydrologically-Driven Surface Erosion. Ph.D. Thesis, Stanford University, Stanford, CA, USA, 2006. [Google Scholar]
- Gabet, E.J.; Dunne, T. Sediment detachment by rain power. Water Resour. Res. 2003, 39, 1002. [Google Scholar] [CrossRef]
- Van Genuchten, M.T. A closed-form equation for predicting the hydraulic conductivity of unsaturated soils. Soil Sci. Soc. Am. Proc. 1980, 44, 892–898. [Google Scholar] [CrossRef]
- Mirus, B.B.; Ebel, B.A.; Heppner, C.S.; Loague, K. Assessing the detail needed to capture rainfall-runoff dynamics with physics-based hydrologic response simulation. Water Resour. Res. 2011, 47, W00H10. [Google Scholar] [CrossRef]
- BeVille, S.H.; Mirus, B.B.; Ebel, B.A.; Mader, G.G.; Loague, K. Using simulated hydrologic response to revisit the 1973 Lerida Court landslide. Environ. Earth Sci. 2010, 61, 1249–1257. [Google Scholar] [CrossRef]
- Thomas, M.A.; Loague, K. Hydrogeologic insights for a Devil’s Slide-like system. Water Resour. Res. 2014, 50, 6628–6645. [Google Scholar] [CrossRef]
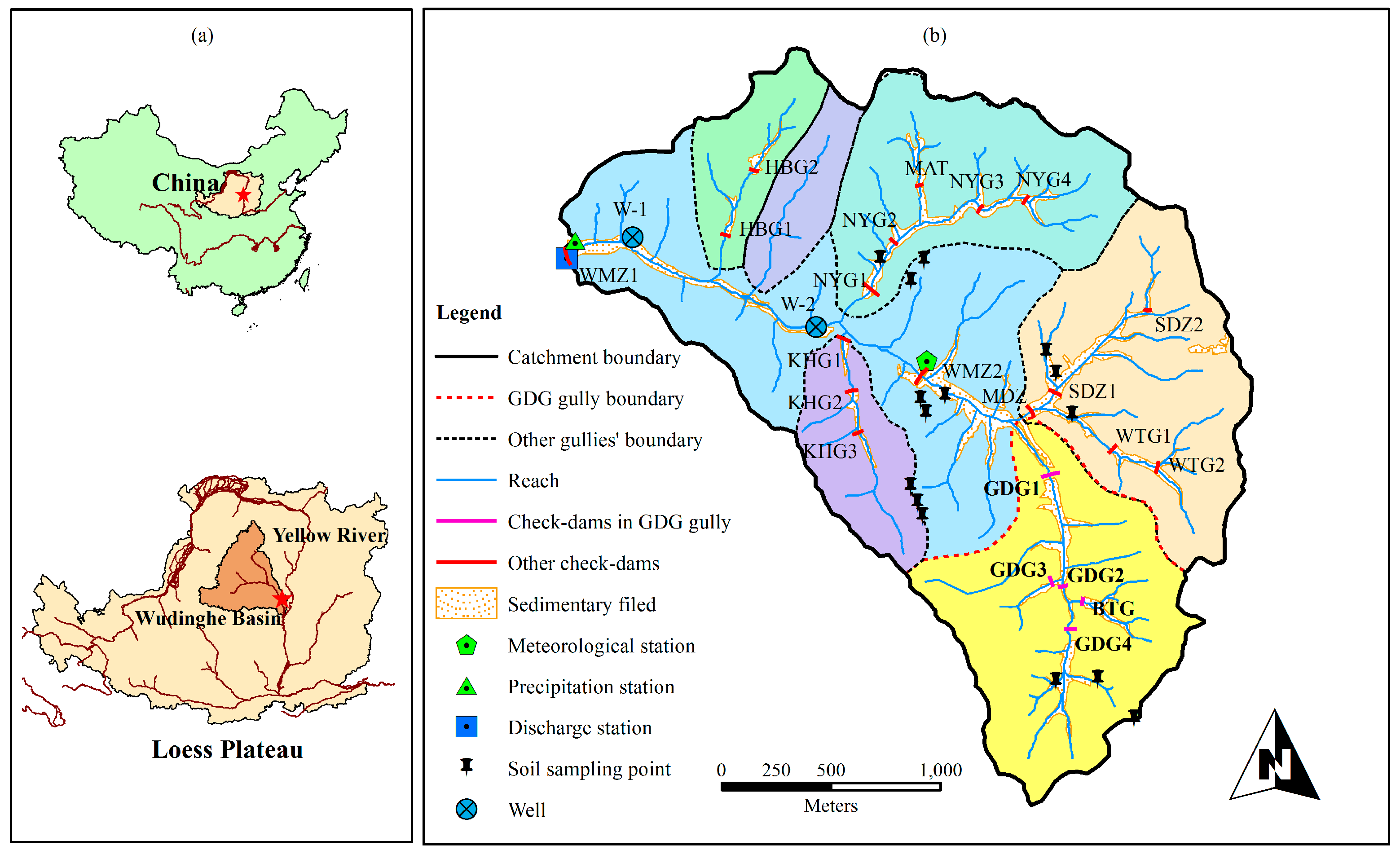
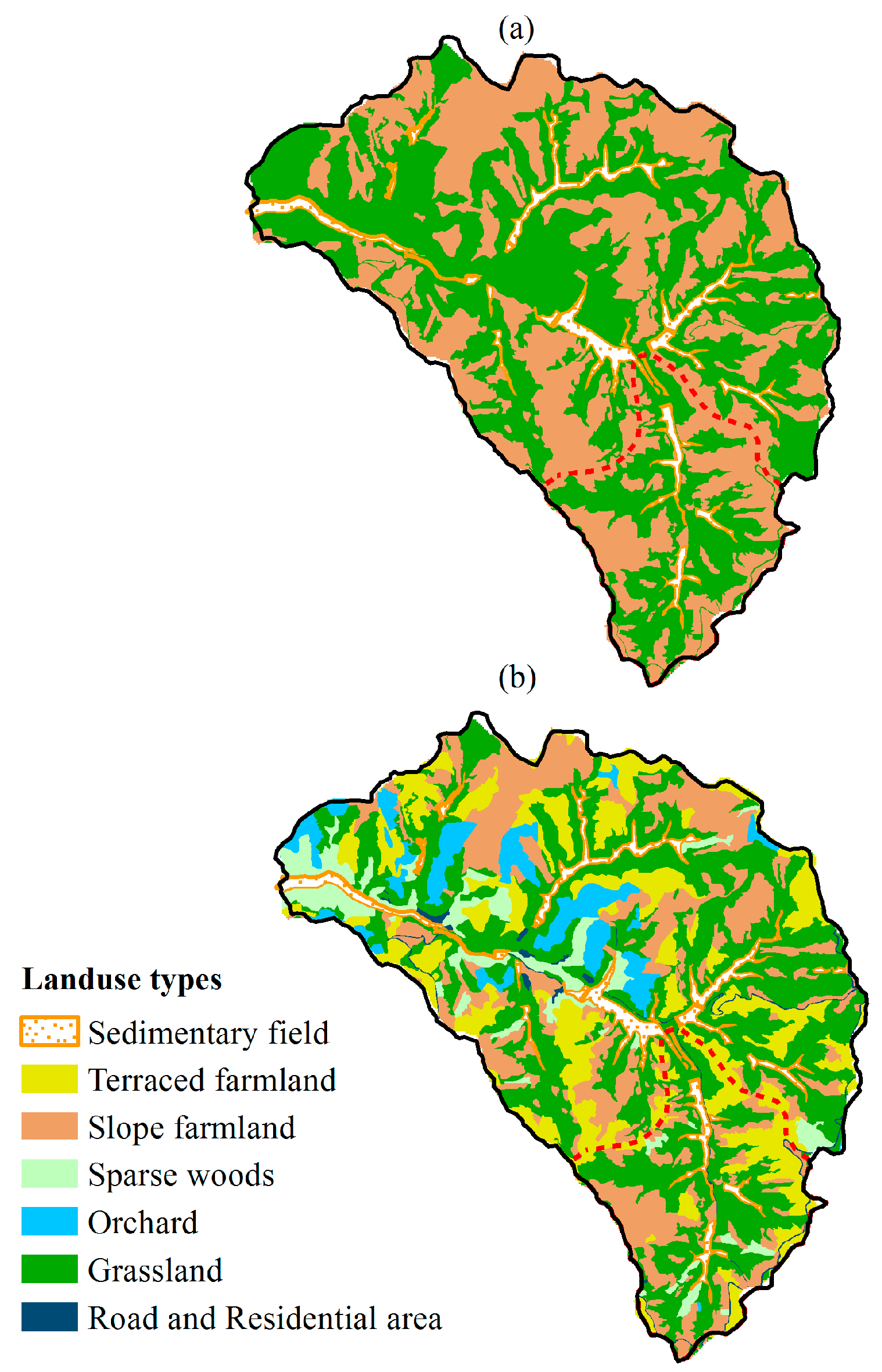
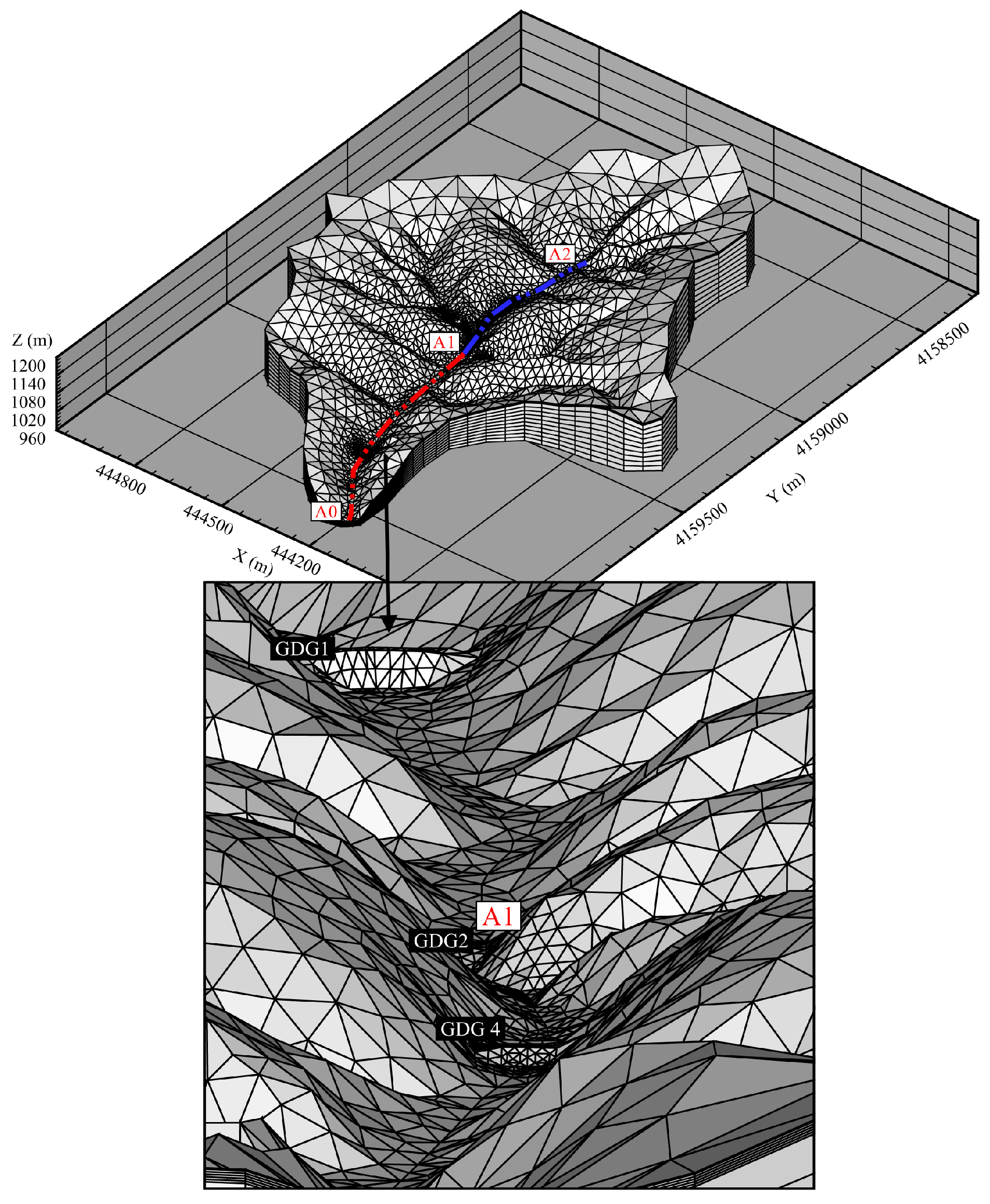
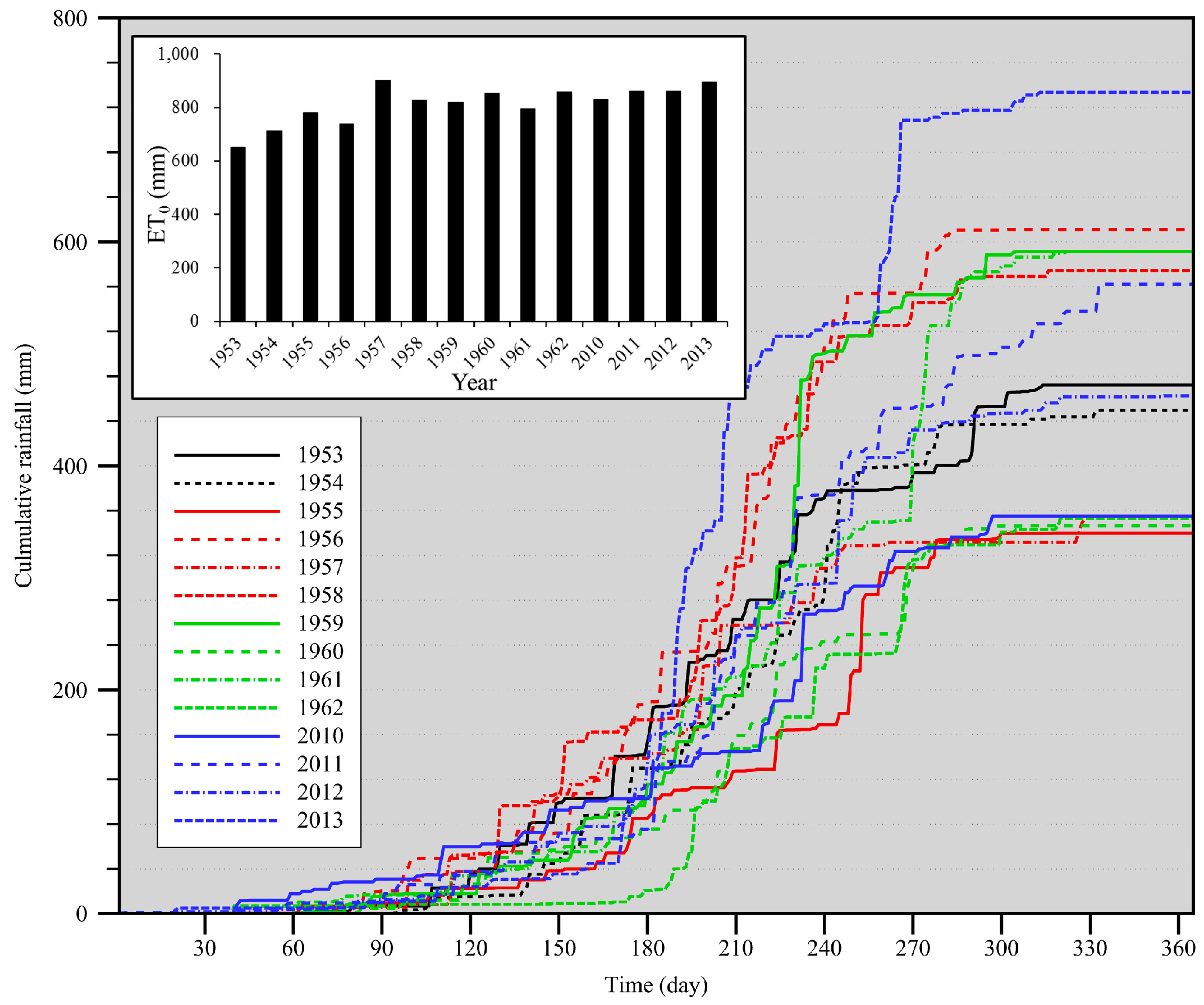


| Data Type | Year | Resolution | Data Source | Use in This Study |
|---|---|---|---|---|
| Land use type | 1962, 2010 | 20-m | Literature [15] | Model construction |
| Soil data | 2014 | Field survey | Model construction | |
| Groundwater data | 2014 | Semi-monthly | Field survey | Model construction |
| Rainfall-runoff event | 10 events during 1962–1964 | 5-min to 1-hour | Suide Soil and Water Conservation Station | Model calibration |
| Topography data | 1953, 2010 | 25-m for 1953 map; 5-m for 2010 map | Shaanxi Administration of Surveying [16] | Model construction |
| Check dam information | 1953–1964, 2014 | Suide Soil and Water Conservation Station; field survey | Model construction |
| Name | Year 1 | Height (m) | Length (m) | Control Area (km2) | Sedimentary Area (× 104 m2) | △h 2 (m) |
|---|---|---|---|---|---|---|
| GDG1 | 1955 (1959) 3 | 10 (+13) 3 | 66 | 1.14 | 2.81 | 7.00 |
| GDG2 | 1959 | 8 | 28 | 0.10 | 0.24 | 1.00 |
| GDG3 | 1959 | 12 | 36 | 0.05 | 0.24 | 1.10 |
| GDG4 | 1959 | 8 | 46 | 0.40 | 1.00 | 2.90 |
| BTG | 1959 | 10 | 45 | 0.20 | 0.94 | 0.80 |
| Scenario Name | Simulation Time Range | Check Dam Involved |
|---|---|---|
| Pre-dam (PD) | 1953–1954 | none |
| Single-dam (SD) | 1955–1958 | GDG1 |
| Early dam-system (EDS) | 1959–1962 | GDG1–4, BTG |
| Current dam-system (CDS) | 2010–2013 | GDG1–4, BTG |
| Scenario | Surface Zone a | Hydrologic Response | Sediment Transport | |||||||
|---|---|---|---|---|---|---|---|---|---|---|
| n b (-) | ψim c (m) | Ssr d (-) | Ht e (m) | cf f (s m−1)0.6 | cd g (1/m) | b h (-) | ξ i (-) | (m−1) | ||
| PD | Slope_G | 0.10 | 0.0005 | 0.01 | 0.001 | 4.000 | 600 | 1.6 | 0.25 | 0.050 |
| Slope_F | 0.12 | 8.000 | 0.050 | |||||||
| Gully_G | 0.05 | 4.000 | 0.050 | |||||||
| SD & EDS | Slope_G | 0.10 | 4.000 | 0.050 | ||||||
| Slope_F | 0.12 | 8.000 | 0.050 | |||||||
| Gully_G | 0.05 | 4.000 | 0.050 | |||||||
| Gully_D | 0.01 | 0.001 | 0.050 | |||||||
| Gully_S | 0.05~0.12 k | 0.100 | 0.050 | |||||||
| CDS | Slope_G | 0.10 | 4.000 | 0.038 | ||||||
| Slope_F | 0.12 | 8.000 | 0.037 | |||||||
| Slope_T | 0.50 | 0.500 | 0.038 | |||||||
| Gully_G | 0.05 | 4.000 | 0.040 | |||||||
| Gully_D | 0.01 | 0.001 | 0.010 | |||||||
| Gully_S | 0.05~0.12 k | 0.100 | 0.040 | |||||||
| Scenario | Subsurface Zone | Ksat (m s−1) | Porosity (-) | Sr a (-) | α b (m−1) | n c (-) |
|---|---|---|---|---|---|---|
| PD | Slope_G d | 6.20 × 10−7 | 0.4800 | 0.0900 | 1.29 | 1.630 |
| Slope_F d | 3.40 × 10−6 | 0.4500 | 0.1000 | 1.27 | 1.710 | |
| Gully_G d | 3.50 × 10−6 | 0.4800 | 0.0900 | 1.40 | 1.650 | |
| 20~50 cm e | 5.00 × 10−6 | 0.4500 | 0.0976 | 1.20 | 1.503 | |
| Below 50 cm e | 3.00 × 10−6 | 0.4000 | 0.1000 | 0.92 | 1.542 | |
| SD & EDS | Slope_G d | 6.20 × 10−7 | 0.4800 | 0.0900 | 1.29 | 1.630 |
| Slope_F d | 3.40 × 10−6 | 0.4500 | 0.1000 | 1.27 | 1.710 | |
| Gully_G d | 3.50 × 10−6 | 0.4800 | 0.0900 | 1.40 | 1.650 | |
| Gully_D e | 3.23 × 10−8 | 0.2500 | 0.0599 | 1.01 | 1.374 | |
| Gully_S e | 3.19 × 10−5 | 0.5000 | 0.0931 | 3.05 | 1.667 | |
| 20~50 cm e | 5.00 × 10−6 | 0.4500 | 0.0976 | 1.20 | 1.503 | |
| Below 50 cm e | 3.00 × 10−6 | 0.4000 | 0.1000 | 0.92 | 1.542 | |
| CDS | Slope_G e | 8.45 × 10−7 | 0.5068 | 0.1176 | 1.74 | 1.579 |
| Slope_F e | 2.18 × 10−6 | 0.4268 | 0.0929 | 1.50 | 1.601 | |
| Slope_T e | 7.81 × 10−6 | 0.4819 | 0.0833 | 2.19 | 1.735 | |
| Gully_G e | 3.96 × 10−6 | 0.4640 | 0.0888 | 1.63 | 1.704 | |
| Gully_D e | 3.23 × 10−8 | 0.2500 | 0.0599 | 1.01 | 1.374 | |
| Gully_S e | 3.19 × 10−5 | 0.5221 | 0.0931 | 3.05 | 1.667 | |
| 20~50 cm e | 5.00 × 10−6 | 0.4500 | 0.0976 | 1.20 | 1.503 | |
| Below 50 cm e | 3.00 × 10−6 | 0.4000 | 0.1000 | 0.92 | 1.542 |
| N a | Date | P (mm) | Qpk (m3/s) b | Tpk (h) c | EFh d | Qs, pk(Kg/s) b | Ts, pk(h) c | EFs d | ||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| O | S | O | S | O | S | O | S | |||||
| 1 | 1962/7/15 | 29.3 | 1.90 | 1.62 | 5.8 | 5.4 | 0.78 | 1276 | 890 | 5.8 | 5.7 | 0.71 |
| 2 | 1963/7/05 | 64.8 | 0.58 | 0.48 | 6.9 | 7.1 | 0.79 | 37 | 25 | 6.9 | 7.3 | 0.72 |
| 3 | 1964/9/07 | 26.8 | 1.60 | 1.59 | 11.5 | 11.6 | 0.71 | 133 | 120 | 11.5 | 11.6 | 0.72 |
| 4 | 1965/7/07 | 19.0 | 0.20 | 0.15 | 2.5 | 2.6 | 0.93 | 7 | 8 | 2.3 | 2.5 | 0.88 |
| 5 | 1961/7/05 | 46.3 | 1.50 | 1.32 | 5.7 | 6.0 | 0.59 | 93 | 74 | 5.7 | 6.0 | 0.60 |
| 6 | 1961/8/01 | 54.7 | 21.00 | 12.12 | 2.8 | 4.0 | 0.71 | 9240 | 4863 | 2.8 | 5.0 | 0.41 |
| 7 | 1961/9/27 | 35.2 | 1.53 | 1.10 | 4.9 | 5.3 | 0.68 | 137 | 91 | 4.9 | 4.9 | 0.62 |
| 8 | 1964/7/05 | 133.1 | 7.07 | 4.61 | 16.1 | 16.0 | 0.60 | 4242 | 3726 | 16.1 | 16.3 | 0.50 |
| 9 | 1964/7/12 | 24.6 | 1.63 | 1.43 | 5.2 | 5.3 | 0.56 | 904 | 681 | 5.2 | 5.1 | 0.69 |
| 10 | 1964/9/11 | 51.4 | 1.82 | 1.63 | 8.1 | 8.0 | 0.65 | 820 | 777 | 8.1 | 8.0 | 0.45 |
| Scenario | Year | Precipitation (mm) | Outflow (%) | Storage Change (%) | ET (%) | ||
|---|---|---|---|---|---|---|---|
| Surface | Subsurface | Surface | Subsurface | ||||
| PD | 1953 | 476.50 | 15.00 | 5.00 | 0.80 | 1.70 | 77.50 |
| 1954 | 449.30 | 14.32 | 3.94 | 1.00 | −0.63 | 81.37 | |
| Average | 462.90 | 14.66 | 4.47 | 0.90 | 0.54 | 79.43 | |
| SD | 1955 | 326.90 | 1.01 | 4.51 | 8.94 | 4.93 | 80.61 |
| 1956 | 610.88 | 2.11 | 7.35 | 3.20 | 11.01 | 76.33 | |
| 1957 | 354.70 | 1.36 | 7.54 | 3.18 | 8.69 | 79.23 | |
| 1958 | 574.50 | 3.20 | 9.27 | 2.24 | 15.10 | 70.19 | |
| Average | 466.75 | 1.92 | 7.17 | 4.39 | 9.93 | 76.59 | |
| EDS | 1959 | 590.80 | 4.39 | 8.74 | 18.37 | 11.09 | 57.41 |
| 1960 | 346.70 | 1.03 | 12.31 | 4.87 | 14.99 | 66.80 | |
| 1961 | 591.11 | 2.57 | 10.74 | 8.96 | 12.38 | 65.35 | |
| 1962 | 353.00 | 0.97 | 14.17 | 3.01 | 6.74 | 75.11 | |
| Average | 470.40 | 2.24 | 11.49 | 8.80 | 11.30 | 66.17 | |
| CDS | 2010 | 355.00 | 1.12 | 9.79 | 1.64 | 10.24 | 77.21 |
| 2011 | 562.30 | 3.71 | 11.62 | 1.02 | 12.12 | 71.53 | |
| 2012 | 462.70 | 3.58 | 11.69 | 0.67 | 9.10 | 74.96 | |
| 2013 | 733.70 | 3.08 | 13.15 | 2.79 | 8.88 | 72.10 | |
| Average | 646.03 | 2.90 | 11.56 | 1.53 | 10.09 | 73.92 | |
| Scenario | Year | Eroded Mass (× 104 t) | Eroded Mass of Different Zones (× 104 t) (%) | ||||
|---|---|---|---|---|---|---|---|
| Slope_G | Slope_F | Slope_T | Gully_G | Gully_S | |||
| PD | 1953 | 3.26 | 0.85 (26) | 1.08 (33) | 0.00 (0) | 1.34 (41) | 0.00 (0) |
| 1954 | 3.37 | 0.94 (28) | 1.25 (37) | 0.00 (0) | 1.18 (35) | 0.00 (0) | |
| SD | 1955 | 2.13 | 0.60 (28) | 0.75 (35) | 0.00 (0) | 0.77 (36) | 0.02 (1) |
| 1956 | 4.75 | 1.19 (25) | 1.95 (41) | 0.00 (0) | 1.57 (33) | 0.05 (1) | |
| 1957 | 3.01 | 0.69 (23) | 1.17 (39) | 0.00 (0) | 1.08 (36) | 0.06 (2) | |
| 1958 | 2.93 | 1.23 (42) | 0.88 (30) | 0.00 (0) | 0.79 (27) | 0.03 (1) | |
| EDS | 1959 | 3.27 | 0.98 (30) | 1.21 (37) | 0.00 (0) | 0.98 (30) | 0.10 (3) |
| 1960 | 3.36 | 0.97 (29) | 1.34 (40) | 0.00 (0) | 0.84 (25) | 0.20 (6) | |
| 1961 | 3.12 | 0.94 (30) | 1.12 (36) | 0.00 (0) | 0.81 (26) | 0.25 (8) | |
| 1962 | 1.97 | 0.59 (30) | 0.81 (41) | 0.00 (0) | 0.47 (24) | 0.10 (5) | |
| CDS | 2010 | 1.18 | 0.46 (39) | 0.28 (24) | 0.12 (10) | 0.24 (20) | 0.08 (7) |
| 2011 | 1.37 | 0.60 (44) | 0.29 (21) | 0.16 (12) | 0.26 (19) | 0.05 (4) | |
| 2012 | 1.42 | 0.58 (41) | 0.23 (16) | 0.26 (18) | 0.31 (22) | 0.04 (3) | |
| 2013 | 2.93 | 1.00 (34) | 0.67 (23) | 0.41 (14) | 0.59 (20) | 0.26 (9) | |
| Name | 1958 | 1962 | 2013 | ||||||
|---|---|---|---|---|---|---|---|---|---|
| O a (m) | S b (m) | D c (%) | O a (m) | S b (m) | D c (%) | O a (m) | S b (m) | D c (%) | |
| GDG1 | 0.50 | 1.96 | 292.0 | 7.90 | 8.50 | 7.6 | 7.00 | 7.10 | 1.4 |
| GDG2 | - | - | - | 3.60 | 3.90 | 7.7 | 1.00 | 0.70 | −30.0 |
| GDG3 | - | - | - | 6.93 | 6.20 | −10.5 | 1.10 | 2.10 | 90.9 |
| GDG4 | - | - | - | 4.20 | 5.10 | 21.4 | 2.90 | 2.10 | −27.6 |
| BTG | - | - | - | 4.70 | 5.65 | 20.2 | 0.80 | 1.05 | 31.3 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tang, H.; Ran, Q.; Gao, J. Physics-Based Simulation of Hydrologic Response and Sediment Transport in a Hilly-Gully Catchment with a Check Dam System on the Loess Plateau, China. Water 2019, 11, 1161. https://doi.org/10.3390/w11061161
Tang H, Ran Q, Gao J. Physics-Based Simulation of Hydrologic Response and Sediment Transport in a Hilly-Gully Catchment with a Check Dam System on the Loess Plateau, China. Water. 2019; 11(6):1161. https://doi.org/10.3390/w11061161
Chicago/Turabian StyleTang, Honglei, Qihua Ran, and Jihui Gao. 2019. "Physics-Based Simulation of Hydrologic Response and Sediment Transport in a Hilly-Gully Catchment with a Check Dam System on the Loess Plateau, China" Water 11, no. 6: 1161. https://doi.org/10.3390/w11061161
APA StyleTang, H., Ran, Q., & Gao, J. (2019). Physics-Based Simulation of Hydrologic Response and Sediment Transport in a Hilly-Gully Catchment with a Check Dam System on the Loess Plateau, China. Water, 11(6), 1161. https://doi.org/10.3390/w11061161




