Hydrodynamic Interactions between Bracket and Propeller of Podded Propulsor Based on Particle Image Velocimetry Test
Abstract
1. Introduction
2. Materials and Methods
2.1. Experimental Model
2.2. PIV Measurement Design
3. Results and Discussion
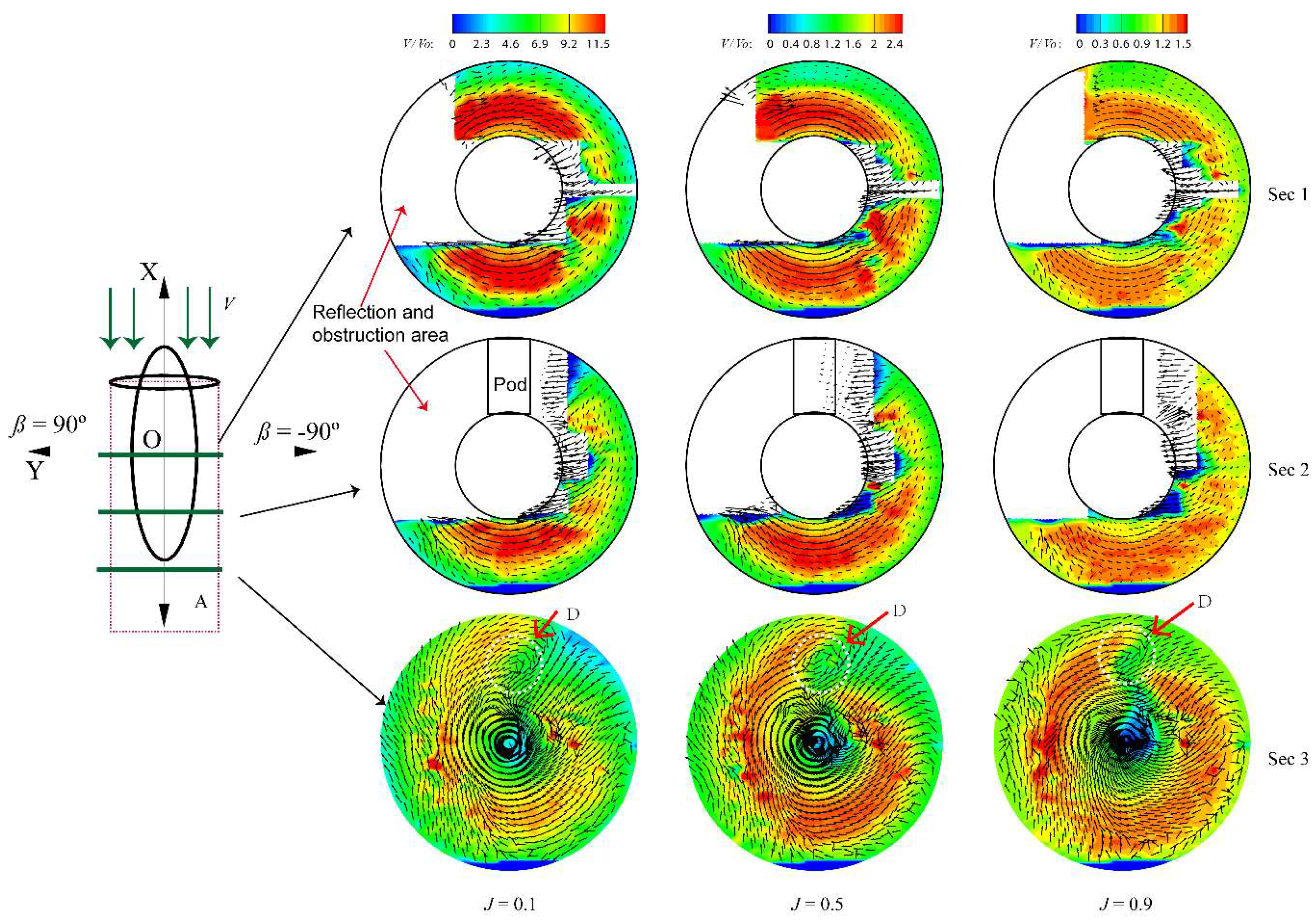
3.1. PIV Measurements in Straight Drive
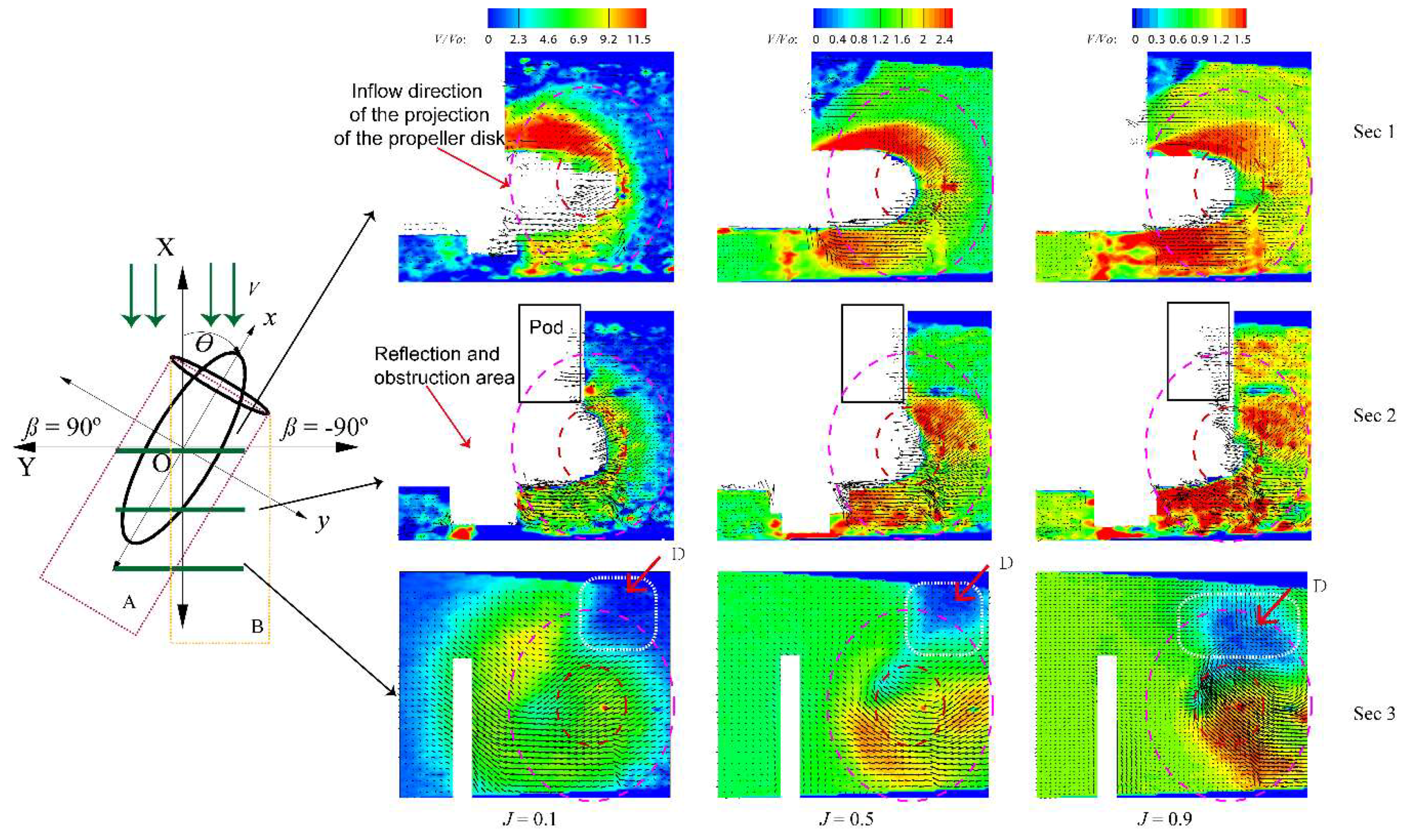
3.2. PIV Measurements in −30° Oblique Drive
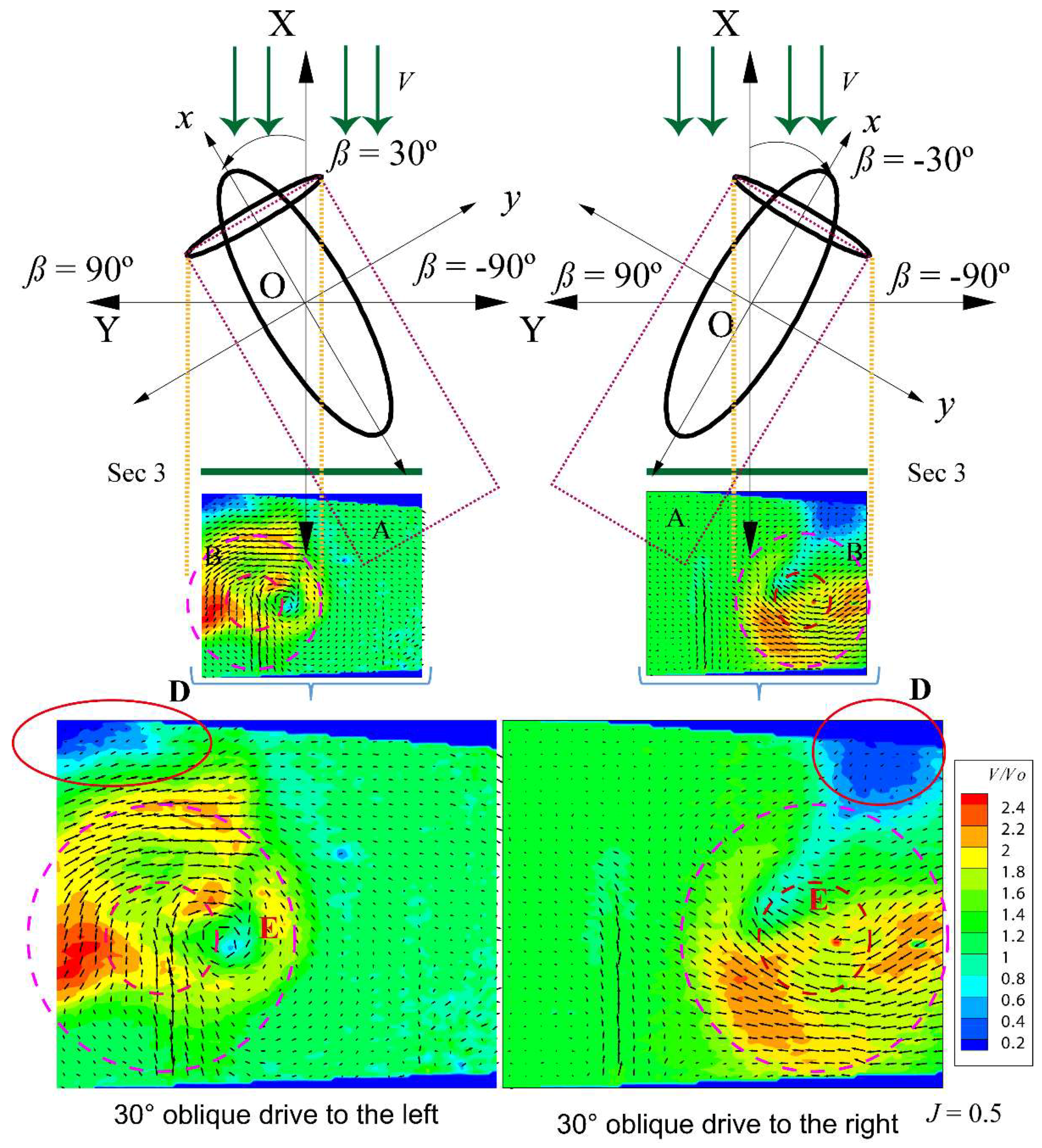
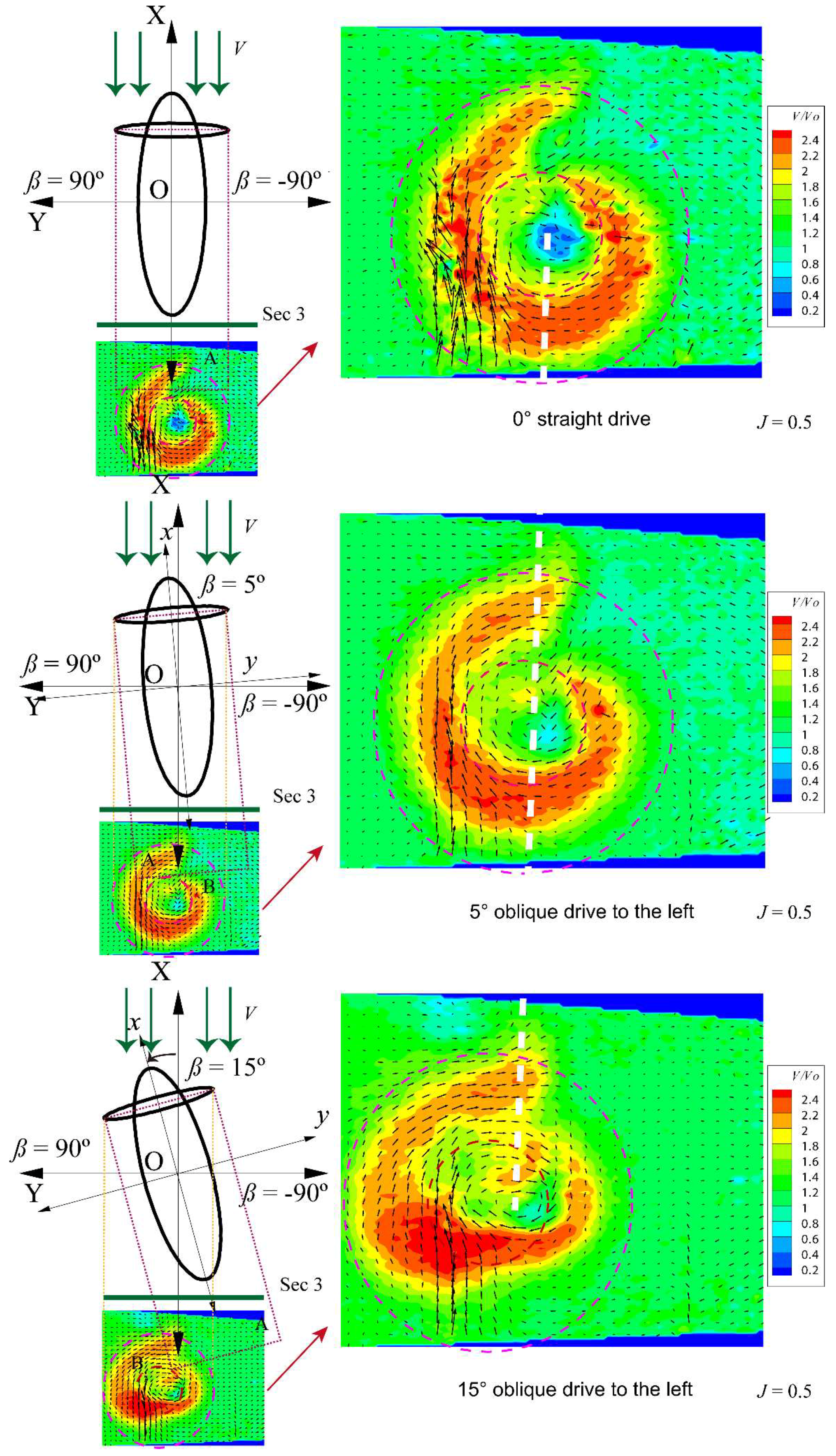
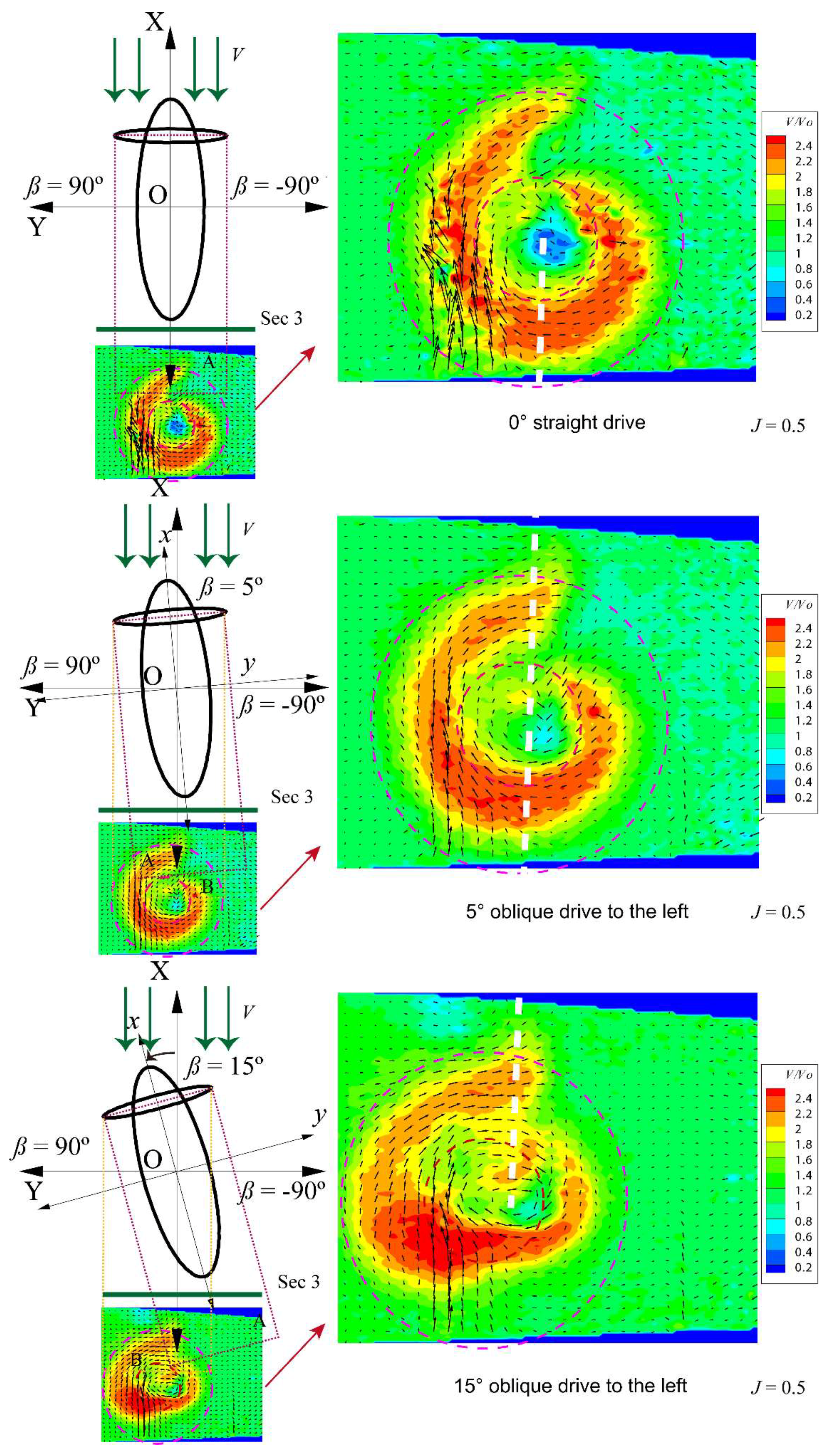
3.3. PIV Measurements at Identical Rudder Angles and Different Oblique Drive Directions
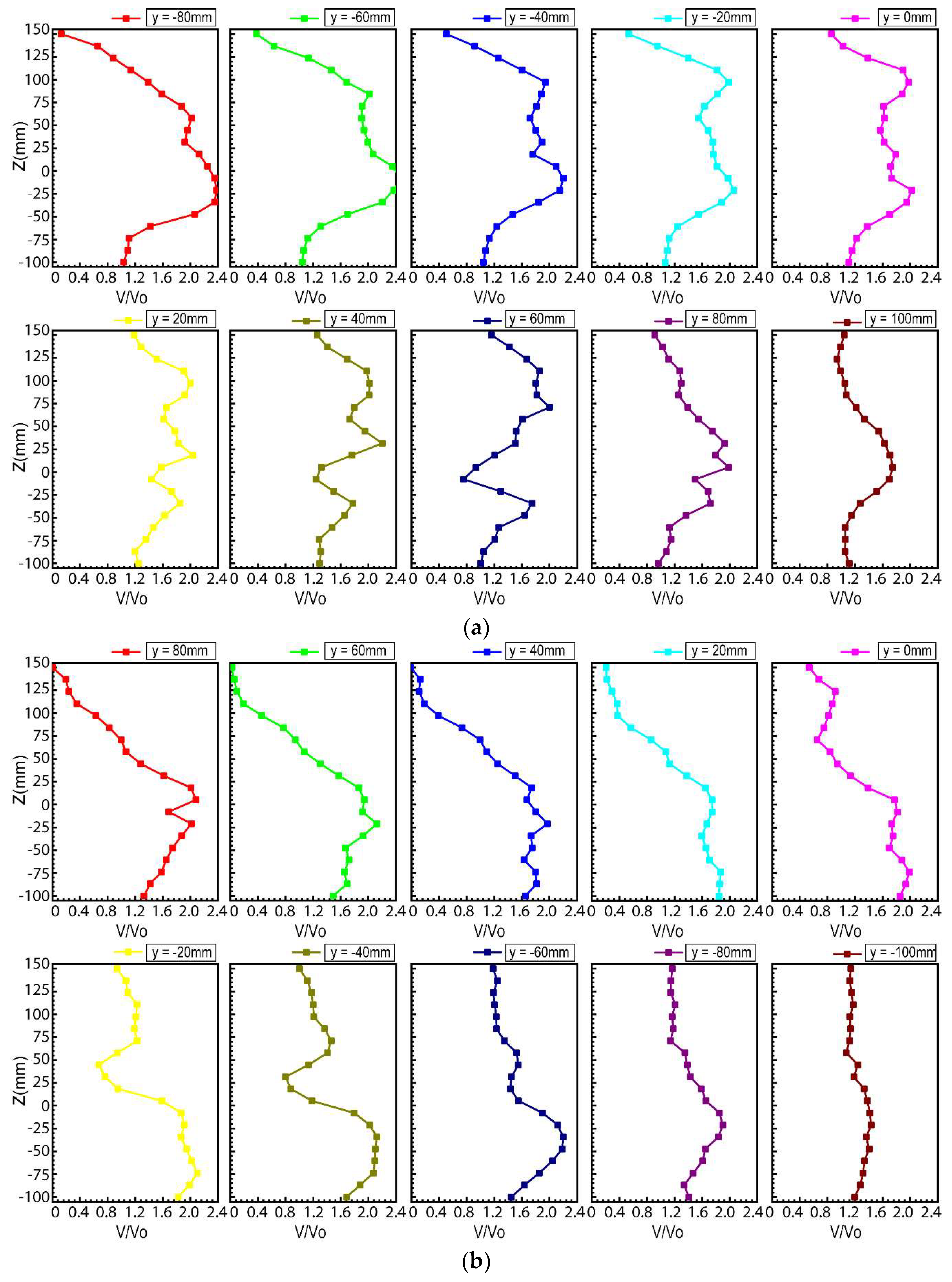
3.4. PIV Measurements at Different Rudder Angles and Identical Advance Coefficients
4. Conclusions
- In the straight drive conditions with different advance coefficients, the velocity difference for the propeller wake flow indicated the magnitude of the interaction intensity between the propeller and inflow. Distinct asymmetry near the pod tail characterizes the propeller wake flow.
- Owing to pod interference in the Y-direction, the right side of the pod interfered with the flow field, which created an obvious area of velocity interference. The interference area also had an obvious area of vortex recirculation.
- In the oblique drive conditions, the existence of an oblique drive angle results in a flow field deflection angle in both the propeller rotating wake flow and inflow areas. This deflection angle was equivalent to the oblique drive angle. The magnitude of the deflection angle determined the shape of the interference between the propeller rotating wake and inflow.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Propulsion Committee of 22nd ITTC. Final report and recommendations to the 23rd ITTC. In Proceedings of the 23rd ITTC, Hamburg, Germany, 8–14 September 2002.
- Szantyr, J.A. Hydrodynamic model experiments with pod drives. Ocean. Eng. Int. 2001, 5, 95–103. [Google Scholar]
- Heinke, H.J. Investigation about the Forces and Moment at Podded Drives. In Proceedings of the 1st International Conference on Technological Advances in Podded Propulsion; Atlar, M., Clarke, D., Glover, E.J., Eds.; University of Newcastle: Newcastle, UK, 2004; pp. 305–320. [Google Scholar]
- Grygorowicz, M.; Szantyr, J.A. Open water experiment with two pod propulsor models. In Proceedings of the 1st International Conference on Technological Advances in Podded Propulsion; Atlar, M., Clarke, D., Glover, E.J., Eds.; University of Newcastle: Newcastle, UK, 2004; pp. 357–370. [Google Scholar]
- Reichel, M. Manoeuvring forces on azimuthing podded propulsor model. Pol. Marit. Res. 2007, 14, 3–8. [Google Scholar] [CrossRef]
- Islam, M.F.; Veitch, B.; Liu, P.F. Experimental research on marine podded propulsors. J. Nav. Archit. Mar. Eng. 2007, 4, 57–71. [Google Scholar] [CrossRef]
- Islam, M.F.; Akinturk, A.; Veitch, B.; Liu, P.F. Performance characteristics of static and dynamic azimuthing podded propulsor. In Proceedings of the 1st International Symposium on Marine Propulsors-smp’09, Trondheim, Norway, 22–24 June 2009; pp. 482–492. [Google Scholar]
- Xin, G.Z.; Liu, D.C.; Shi, X.Y.; Wei, X.H. Numerical prediction of hydrodynamic performance and model test for contra-rotating POD propulsion. In Proceedings of the 23rd National Symposium on Hydraulics and 10th National Academic Conference on Hydraulics, Xian, China, 1 September 2011. [Google Scholar]
- Shen, X.R.; Cai, Y.J.; Cai, R.Q.; Feng, X.M. Summarization of hydrodynamics performance study of podded propulsor. J. Ship Mech. 2011, 15, 189–197. [Google Scholar]
- Shen, X.; Fan, S.; Cai, Y.; Sun, Q.; Yang, C. Experimental investigation on cavitation performance of podded propulsor under small helm-angle condition. Shipbuild. China 2012, 53, 1–8. [Google Scholar]
- Shen, X.; Sun, Q.; Wei, Y.; Wu, Y.; Wang, H. Experimental investigation on hydrodynamic performance of podded propulsor under static azimuthing conditions. Shipbuild. China 2016, 57, 9–18. [Google Scholar]
- He, W.; Chen, K.; Li, Z. Experimental research on hydrodynamics of tandem podded propulsor in azimuthing conditions. J. Huazhong Univ. Sci. Technol. (Nat. Sci. Ed.) 2015, 1, 107–111. [Google Scholar]
- Felice, F.D.; Florio, D.D.; Felli, M.; Romano, G.P. Experimental investigation of the propeller wake at different loading conditions by particle image velocimetry. J. Ship Res. 2004, 48, 168–190. [Google Scholar]
- Stettler, J.W.; Hover, F.S.; Triantafylyidejilou, M.S. Preliminary results of testing on the dynamics of an azimuthing podded propulsor relating to vehicle maneuvering. In Proceedings of the 1st International Conference on Technological Advances in Podded Propulsion; Atlar, M., Clarke, D., Glover, E.J., Eds.; University of Newcastle: Newcastle, UK, 2004; pp. 321–337. [Google Scholar]
- Paik, B.G.; Kim, J.; Park, Y.H.; Kim, K.S.; Yu, K.K. Analysis of wake behind a rotating propeller using PIV technique in a cavitation tunnel. Ocean Eng. 2007, 34, 594–604. [Google Scholar] [CrossRef]
- Guo, C.Y.; Wu, T.C.; Luo, W.Z.; Chang, X.; Gong, J.; She, W.X. Experimental study on the wake fields of a Panamax Bulker based on stereo particle image velocimetry. Ocean Eng. 2018, 165, 91–106. [Google Scholar] [CrossRef]
- Gong, J.; Guo, C.Y.; Song, K.W.; Wu, T.C. SPIV measurements and URANS simulations on the inlet velocity distribution for a waterjet-propelled ship with stabiliser fins. Ocean Eng. 2019, 171, 120–130. [Google Scholar] [CrossRef]
- Falchi, M.; Felli, M.; Grizzi, S.; Aloisio, G.; Broglia, R.; Stern, F. SPIV measurements around the DELFT 372 catamaran in steady drift. Exp. Fluids 2014, 55, 1844. [Google Scholar] [CrossRef]
- Felli, M.; Falchi, M. Propeller wake evolution mechanisms in oblique flow conditions. J. Fluid Mech. 2018, 845, 520–559. [Google Scholar] [CrossRef]
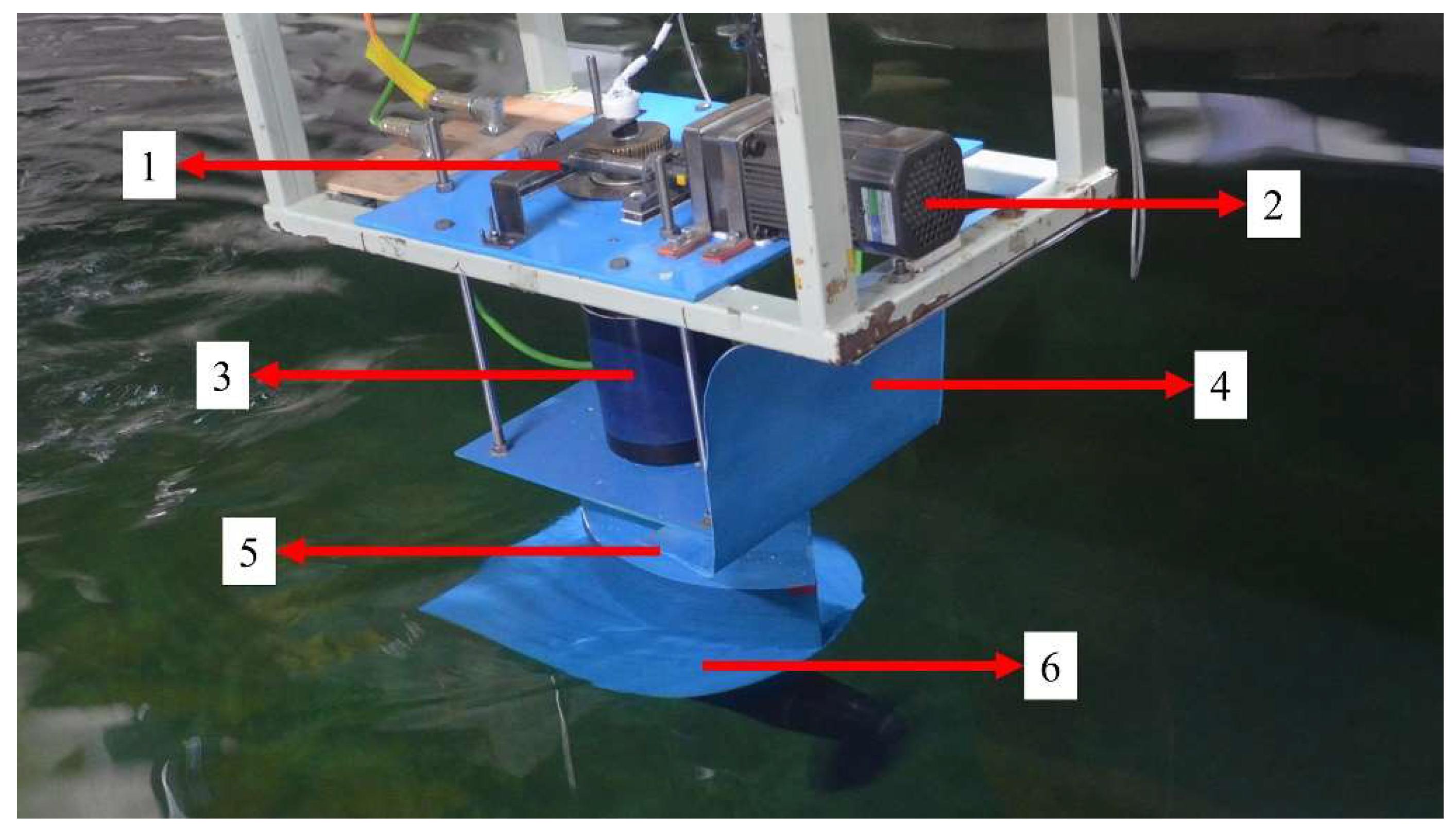
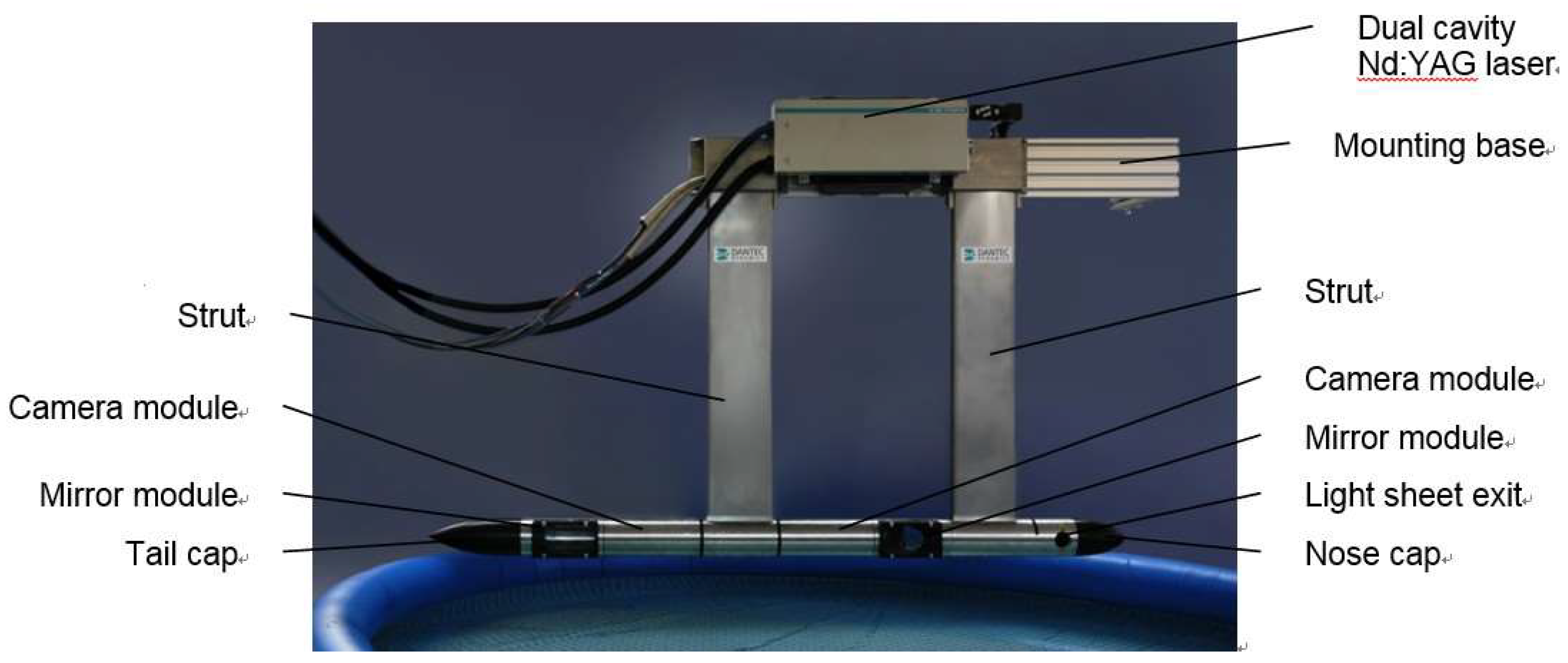
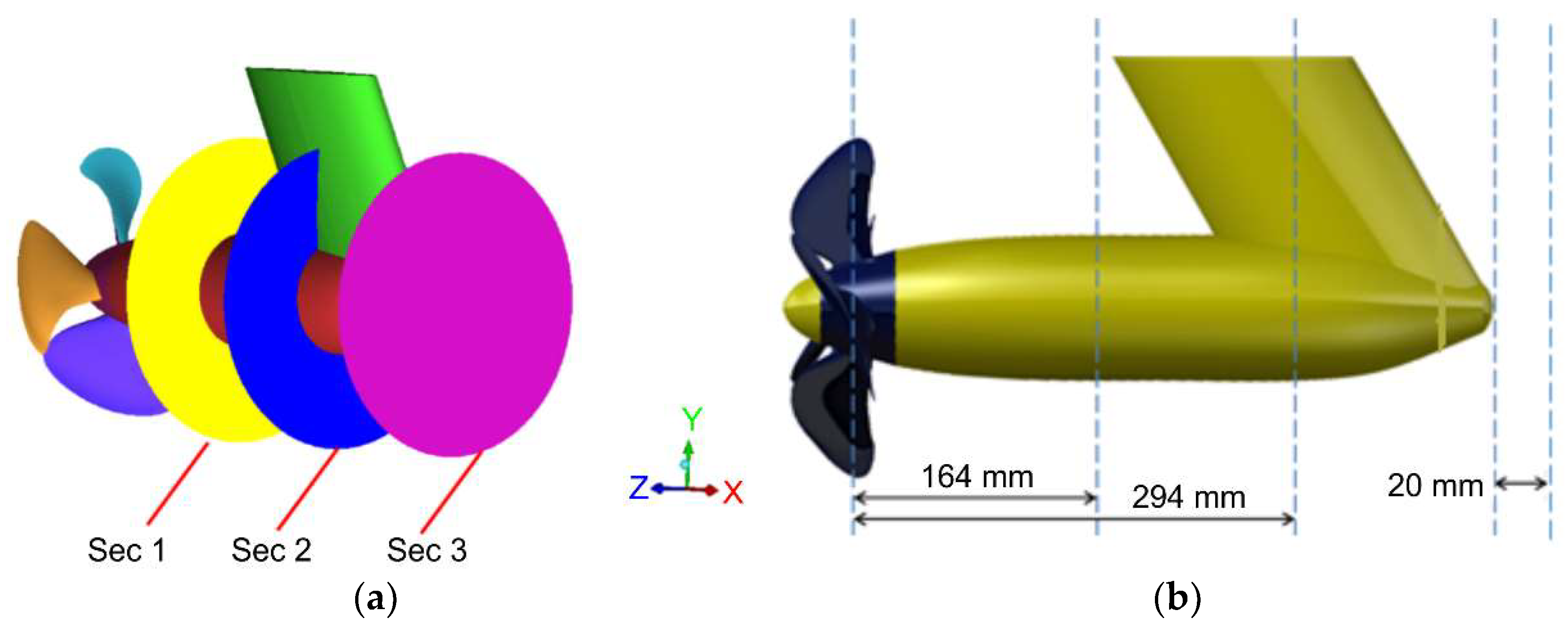
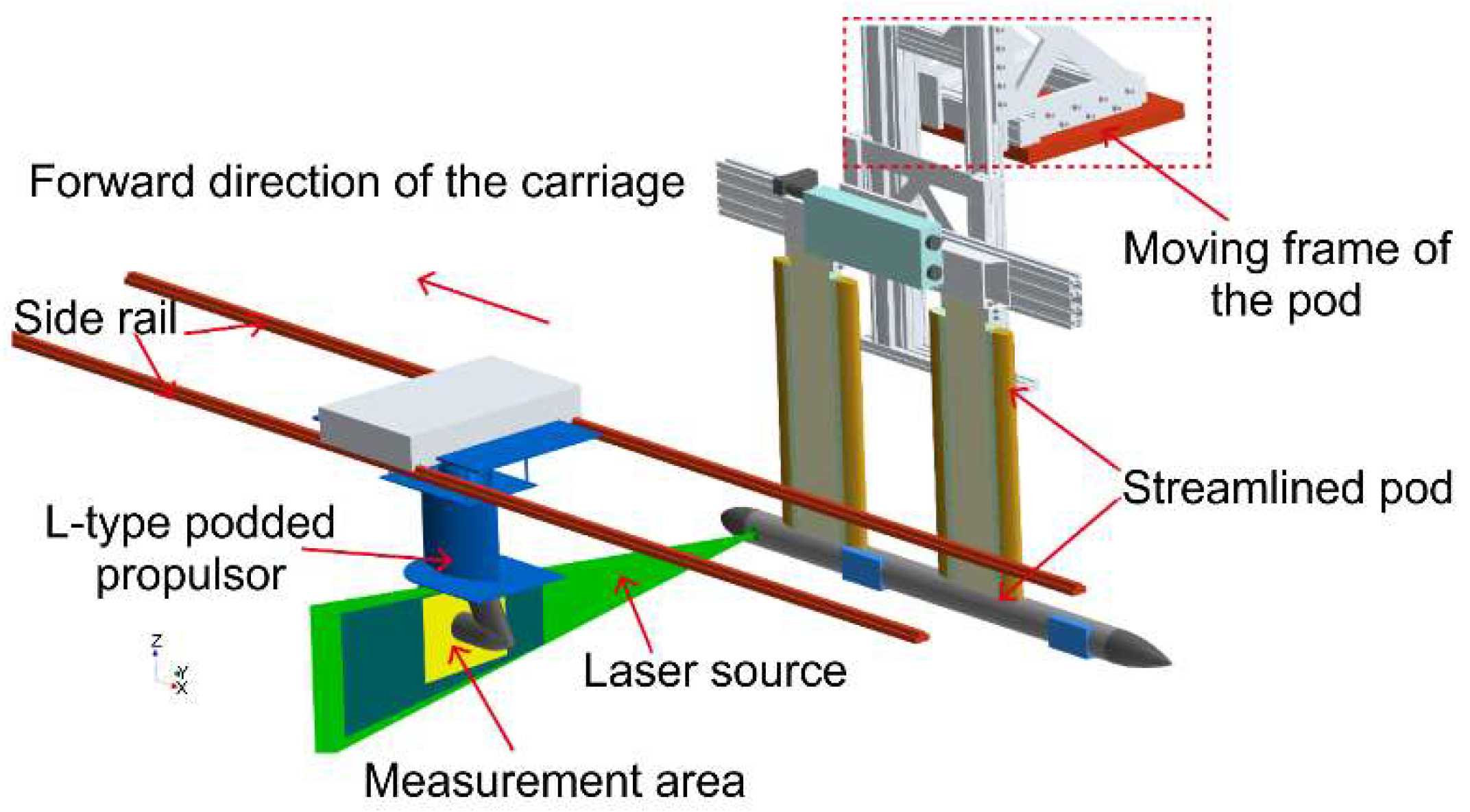

| Parameter | Value |
|---|---|
| Inclined angle of the bracket (°) | 60 |
| Total length of the pod (m) | 0.473 |
| Hub length (m) | 0.075 |
| Maximum radius of the pod (m) | 0.049 |
| Number of propeller blades | 4 |
| Propeller diameter (m) | 0.24 |
| Propeller pitch ratio (0.7R) | 1.284 |
| Parameter | Value |
|---|---|
| Resolution of CCD Camera | 2048 × 2048 pixels |
| Maximum Pulse Frequency of Laser | 15 Hz |
| Maximum Pulse Laser Energy | 1200 mJ |
| Conventional working energy of laser | 200 mJ |
| Laser beam duration | 4 ns |
| Laser wavelength | 532 nm |
| Thickness of Pulsed Laser Sheet | 0.6 mm |
| Maximum measurement range | 400 × 400 mm |
| Tracer particle | polyamide (PSP-50μm) |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, D.; Guo, C.; Wu, T.; Wang, W.; Yin, X. Hydrodynamic Interactions between Bracket and Propeller of Podded Propulsor Based on Particle Image Velocimetry Test. Water 2019, 11, 1142. https://doi.org/10.3390/w11061142
Zhao D, Guo C, Wu T, Wang W, Yin X. Hydrodynamic Interactions between Bracket and Propeller of Podded Propulsor Based on Particle Image Velocimetry Test. Water. 2019; 11(6):1142. https://doi.org/10.3390/w11061142
Chicago/Turabian StyleZhao, Dagang, Chunyu Guo, Tiecheng Wu, Wei Wang, and Xunbin Yin. 2019. "Hydrodynamic Interactions between Bracket and Propeller of Podded Propulsor Based on Particle Image Velocimetry Test" Water 11, no. 6: 1142. https://doi.org/10.3390/w11061142
APA StyleZhao, D., Guo, C., Wu, T., Wang, W., & Yin, X. (2019). Hydrodynamic Interactions between Bracket and Propeller of Podded Propulsor Based on Particle Image Velocimetry Test. Water, 11(6), 1142. https://doi.org/10.3390/w11061142





