Wave Overtopping of Stepped Revetments
Abstract
1. Introduction
1.1. Surface Roughness of Stepped Revetments
2. Experimental Set-Up and Test Conditions
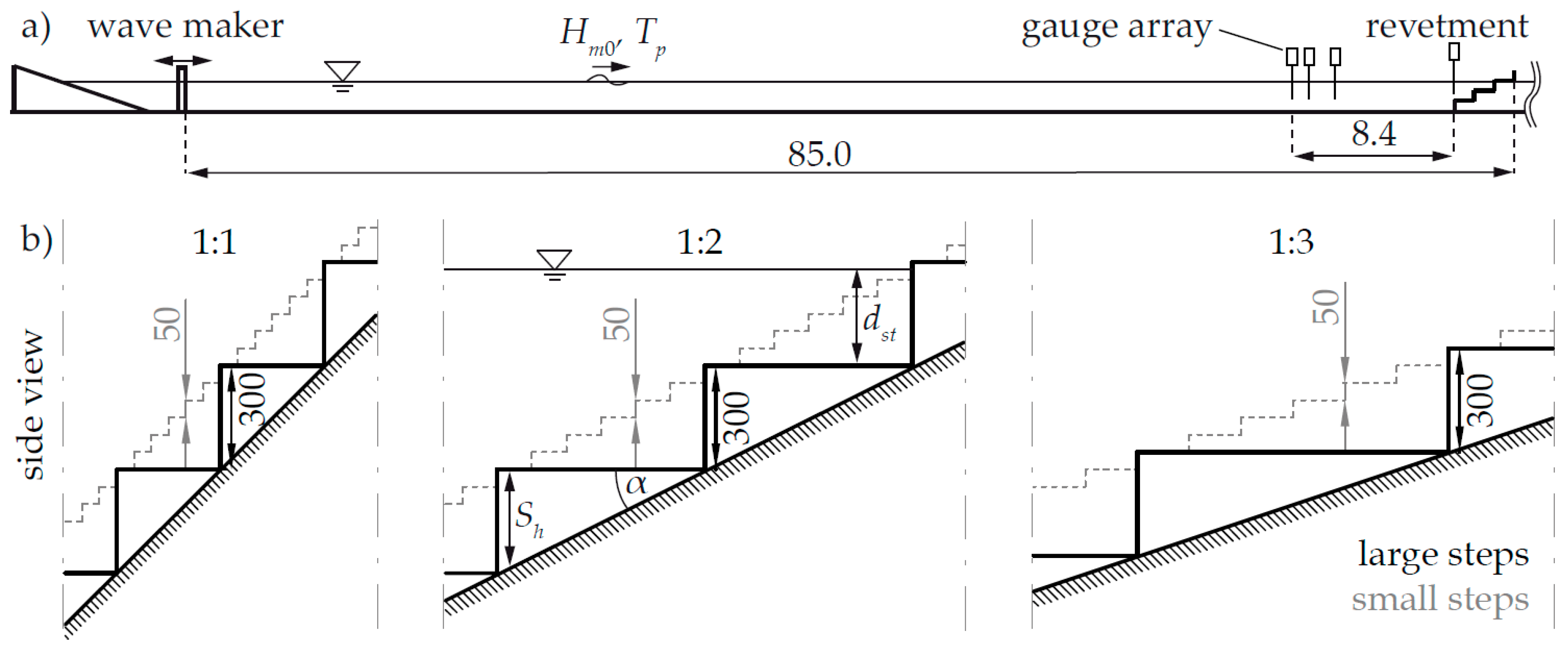
2.1. Model Set-Up
2.2. Test Conditions
3. Results
3.1. Calibration
3.2. Measured and Determined Values
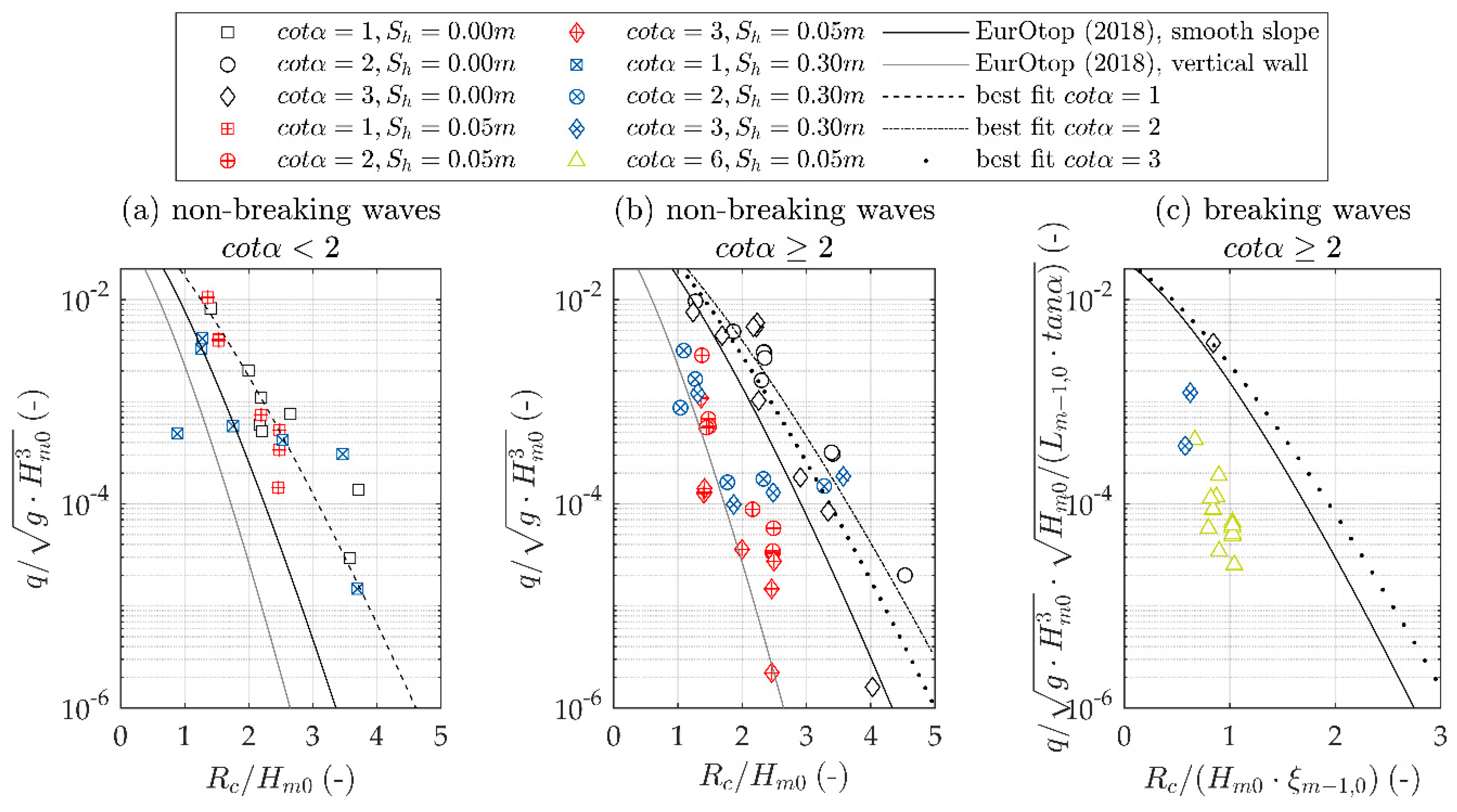
4. Analysis
5. Discussion
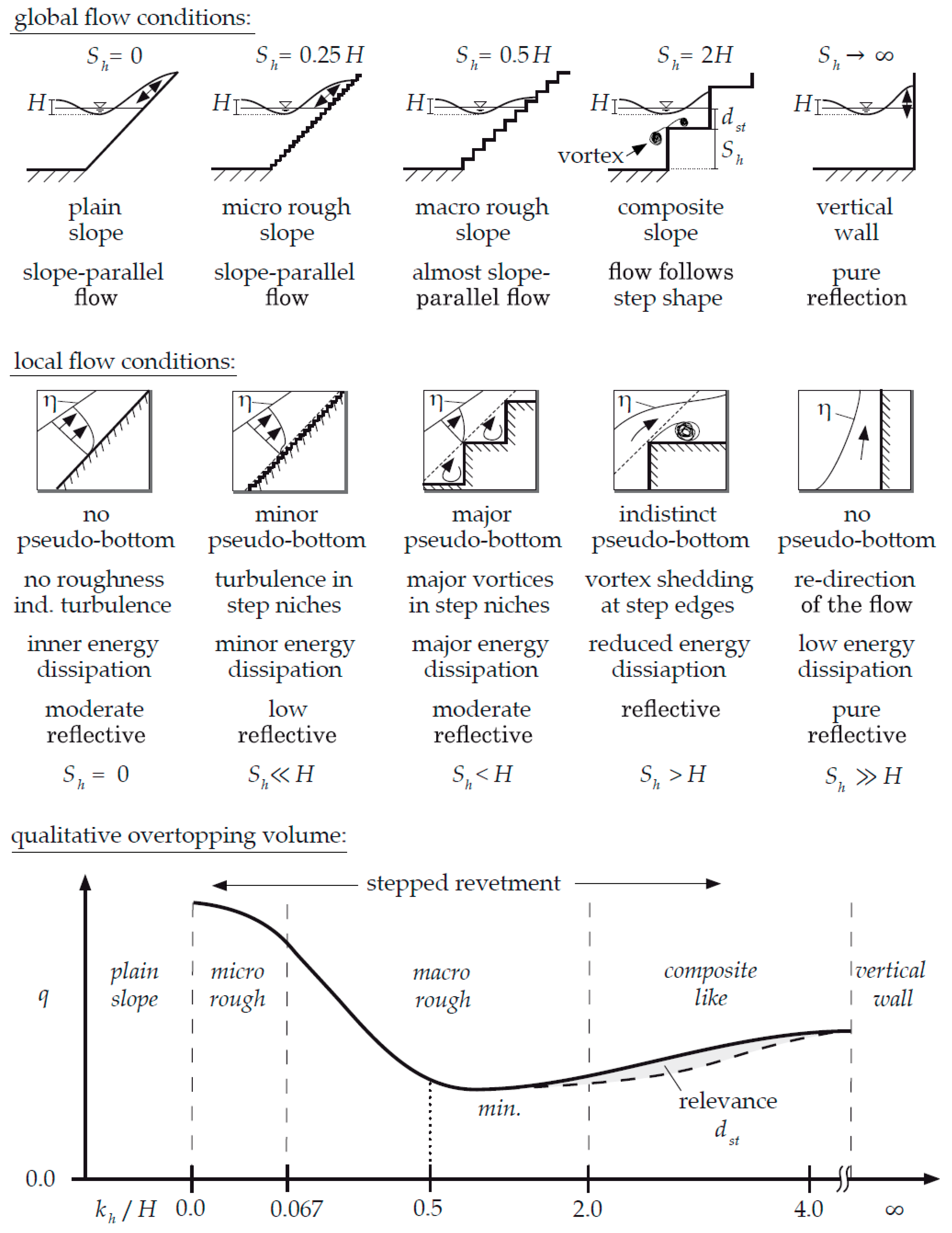
5.1. Governing Process Description
5.2. Model and Scale Effects
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A. Technical Background
Appendix A.1. Harmonization of Data Sets
Appendix A.2. Wave Overtopping Prediction
Appendix A.3. The Influence of Roughness
References
- Pranzini, E.; Wetzel, L.; Williams, A.T. Aspects of coastal erosion and protection in Europe. J. Coast. Conserv. 2015, 19, 445–459. [Google Scholar] [CrossRef]
- Schoonees, T.; Gijón Mancheño, A.; Scheres, B.; Bouma, T.J.; Silva, R.; Schlurmann, T.; Schüttrumpf, H. Hard Structures for Coastal Protection, Towards Greener Designs. Estuar. Coasts 2019, 1–22. [Google Scholar] [CrossRef]
- Saville, T. Wave Run-Up on Shore Structures. Proc. Am. Soc. Civ. Eng. 1956, 82, 1–14. [Google Scholar]
- Wassing, F. Model Investigation on Wave Run-Up Carried out in the Netherlands during the Past Twenty Years. In Proceedings of the 6th Conference on Coastal Engineering, Hydraulics Laboratory Delft, Gainesville, FL, USA, 1957; Volume 6, pp. 700–714. Available online: https://journals.tdl.org/icce/index.php/icce/issue/view/128 (accessed on 31 January 2019).
- Jachowski, R.A. Interlocking Precast Concrete Block Seawall. In Proceedings of the 9th International Conference on Coastal Engineering, Lisbon, Portugal, 1964; pp. 504–517. Available online: https://ascelibrary.org/doi/10.1061/9780872620056.032 (accessed on 31 January 2019).
- Goda, Y.; Kishira, X. Experiments on Irregular Wave Overtopping Characteristics of Seawall of Low Crest Types; Technical Note of PARI; Port and Airport Research Institute: Yokosuka, Japan, 1976; pp. 1–29. [Google Scholar]
- Heimbaugh, M.S. Coastal Engineering Studies in Support of Virginia Beach, Virginia, Beach Erosion Control and Hurricane Protection Project: Report 1, Physical Model Tests of Irregular Wave Overtopping and Pressure Measurements; CERC U.S. Army Corps of Engineers: Springfield, VA, USA, 1988; Volume 88-1. [Google Scholar]
- Kerpen, N.B. Wave-Induced Responses of Stepped Revetments. Ph.D. Thesis, Gottfried Wilhelm Leibniz University, Hannover, Germany, 2017. [Google Scholar]
- Van Steeg, P.; Joosten, R.A.; Steendam, G.J. Physical model tests to determine the roughness of stair shaped revetments. In Proceedings of the 3rd International Conference on Protection against Overtopping, Grange-over-Sands, UK, 6–8 June 2018; p. 8. [Google Scholar]
- Gallach-Sánchez, D. Experimental Study of Wave Overtopping Performance of Steep Low-Crested Structures. Ph.D. Thesis, Ghent University, Zwijnaarde, Belgium, 2018. [Google Scholar]
- Ward, D.L. Overtopping Studies of a Stepped Revetment for City of Chicago, Illinois; Final Report ERDC/CHLTR-03-14; Defense Technical Information Center: Fort Belvoir, VA, USA, 2003; p. 48.
- Schoonees, T.; Kerpen, N.B.; Liebisch, S.; Schlurmann, T. Wave overtopping prediction of a gentle sloped stepped revetment. Coast. Eng. Proc. 2018, 1, 99. [Google Scholar] [CrossRef]
- EurOtop. Manual on Wave Overtopping of Sea Defences and Related Structures. An Overtopping Manual Largely Based on European Research, but for Worldwide Application, 2nd ed.; Van der Meer, J.W., Allsop, N.W.H., Bruce, T., De Rouck, J., Kortenhaus, A., Pullen, T., Schüttrumpf, H.F.R., Troch, P., Zanuttigh, B., Eds.; 2018; Available online: www.overtopping-manual.com (accessed on 31 January 2019).
- Kerpen, N.B.; Schlurmann, T. Stepped revetments–Revisited. In Proceedings of the 6th International Conference on the Application of Physical Modelling in Coastal and Port Engineering and Science, Ottawa, ON, Canada, 10–13 May 2016; p. 10. [Google Scholar]
- Van Steeg, P.; Wolters, G.; Van Gent, M.R.A. Invloedsfactor voor Ruwheid van een Getrapt talud bij Golfoverslag bij Dijken: Verslag Fysieke Modeltesten en Analyse; Project Report 1206984-000-HYE-0006; Deltares: Delft, The Netherlands, 2012. (In Dutch) [Google Scholar]
- Kerpen, N.B.; Goseberg, N.; Schlurmann, T. Experimental Investigations on Wave Overtopping on Stepped Embankments. In Proceedings of the 5th International Conference on Application of Physical Modelling to Port and Coastal Protection (Coastlab14), Varna, Bulgaria, 29 September–2 October 2014; Volume 1, pp. 226–269. [Google Scholar]
- Ward, D.L.; Ahrens, J.P. Overtopping Rates for Seawalls; Miscellaneous Paper CERC-92-3; Coastal Engineering Research Center: Washington, DC, USA, 1992; p. 64. [Google Scholar]
- Melby, J.A.; Burg, E.C.; McVan, D.; Henderson, W. South Florida Reservoir Embankment Study; ERDC/CHL-TR-09-3; Engineer Research and Development Center Vicksburg MS Coastal and Hydraulic Lab: Vicksburg, MS, USA, 2009; p. 46.
- Schüttrumpf, H.F.R. Wellenüberlaufströmung bei Seedeichen: Experimentelle und theoretische Untersuchungen. Ph.D. Thesis, Mitteilungen aus dem Leichtweiss Institut für Wasserbau, Technischen Universität Carlo-Wilhelmina zu Braunschweig, Braunschweig, Germany, 2001; p. 173. (In German). [Google Scholar]
- TAW. Technisch Rapport Golfoploop en Golfoverslag Bij Dijken; Technical Report; Technische Adviescommissie voor de Waterkeringen (TAW): Delft, The Netherlands, 2002; p. 42. (In Dutch) [Google Scholar]
- Kerpen, N.B.; Schoonees, T.; Schlurmann, T. Wave Impact Pressures on Stepped Revetments. J. Mar. Sci. Eng. 2018, 6, 156. [Google Scholar] [CrossRef]
- Daemrich, K.-F.; Mai, S.; Kerpen, N.B. On the reasons of scatter in data for design formula evolution. In Proceedings of the 6th CG Joint Symposium on Hydraulic and Ocean Engineering, Keelung, Taiwan, 23–29 September 2012; p. 6. [Google Scholar]
- Mansard, E.P.D.; Funke, E.R. The Measurement of Incident and Reflected Spectra Using a Least Squares Method; Coastal Engineering; ASCE—Texas Digital Library: Sydney, Australia, 1980; pp. 154–172. [Google Scholar]
- Klopman, G.; Van der Meer, J.W. Random Wave Measurements in Front of Reflective Structures. J. Water Port Coast. Ocean Eng. 1999, 125, 39–45. [Google Scholar] [CrossRef]
- Brodtkorb, P.A.; Johannesson, P.; Lindgren, G.; Rychlik, I.; Rydén, J.; Sjö, E. WAFO—A Matlab Toolbox for the Analysis of Random Waves and Loads. In Proceedings of the 10th ISOPE 2000, Seattle, WA, USA, 28 May–2 June 2000; Volume 3, pp. 343–350. [Google Scholar]
- Buckingham, E. On Physically Similar Systems; Illustrations of the Use of Dimensional Equations. Phys. Rev. 1914, 4, 345–376. [Google Scholar] [CrossRef]
- Chanson, H. Stepped spillway flows and air entrainment. Can. J. Civ. Eng. 1993, 20, 422–435. [Google Scholar]
- Von Kármán, T. Mechanical Similitude and Turbulence. Nachrichten von der Gesellschaft der Wissenschaften zu Göttingen 1930, 5, 58–76. [Google Scholar]
- Kerpen, N.B.; Bung, D.B.; Valero, D.; Schlurmann, T. Energy dissipation within the wave run-up at stepped revetments. J. Ocean Univ. China 2017, 16, 649–654. [Google Scholar] [CrossRef]
- Troch, P.; De Rouck, J.; Van Damme, L. Instrumentation and prototype measurements at the Zeebrugge rubble mound breakwater. Coast. Eng. 1998, 35, 141–166. [Google Scholar] [CrossRef]
- Führböter, A. Über die Bedeutung des Lufteinschlages für die Energieumwandlung in Brandungszonen; Die Küste; Boyens: Heide, Germany, 1971; Volume 21, pp. 34–42. (In German) [Google Scholar]
- Chanson, H.; Murzyn, F. Froude Similitude and Scale Effects Affecting Air Entrainment in Hydraulic Jumps. In Proceedings of the World Environmental and Water Resources Congress 2008, Honolulu, HI, USA, 12–16 May 2008; Babcock, R.W., Jr., Walton, R., Eds.; pp. 1–10. [Google Scholar]
- De Rouck, J.; Geeraerts, J.; Troch, P.; Kortenhaus, A.; Pullen, T.; Franco, L. New results on scale effects for wave overtopping at coastal structures. In International Conference on Coastlines, structures and breakwaters 2005; Allsop, N.W.H., Ed.; Thomas Telford: London, UK, 2005; pp. 29–43. [Google Scholar]
- Heineke, D.; Verhagen, H.J. On the use of the fictitious wave steepness and related surf-similarity parameter in methods that describe the hydraulic and structural response to waves. In Proceedings of the Coastal Structures 2007, Venice, Italy, 2–4 June 2007; World Scientific Publishing Company: Venice, Italy, 2009; pp. 1057–1066. [Google Scholar]
- Goda, Y. Random Seas and Design of Maritime Structures, 3rd ed.; Advanced Series on Ocean Engineering; World Scientific Publ.: Singapore, 2010; Volume 33, ISBN 978-981-4282-40-6. [Google Scholar]
- Bretschneider, C.L. Revisions in Wave Forecasting: Deep and Shallow Water. Coast. Eng. Proc. 1957, 1, 3. [Google Scholar] [CrossRef]
- Victor, L. Optimization of the Hydrodynamic Performance of Overtopping Wave Energy Converters: Experimental Study of Optimal Geometry and Probability Distribution of Overtopping Volumes. Ph.D. Thesis, Ghent University, Zwijnaarde, Belgium, 2012. [Google Scholar]
- Van der Meer, J.W.; Bruce, T.P. New Physical Insights and Design Formulas on Wave Overtopping at Sloping and Vertical Structures. J. Waterw. Port Coast. Ocean Eng. 2014, 140, 04014025. [Google Scholar] [CrossRef]


| Author | Step Height | Dimensionless Step Height | Iribarren Number | Slope | Roughness Factor |
|---|---|---|---|---|---|
| (m) | (-) | (-) | (-) | (-) | |
| [6] | 0.006; 0.009 | 19.4; 29.1 | 2 | 0.8–0.9 | |
| [18] | 0.015 | 5.0–8.0 | 3 | 0.6 | |
| [8] | 0.05; 0.3 | 0.5–2.5 | 1; 2; 3 | 0.35–0.9 () 0.5–0.95 () | |
| [9] | 0.023; 0.046 | 1.6–6.7 | 2; 3 | 0.8–0.9 () 0.6–0.7 () | |
| [10] | 0.053, 0.106 | 0.7–2.45 | 0.58; 1 | 0.5–0.91 0.91 () | |
| [12] | 0.05 | 3.1–4.6 | 6 | 0.74 |
| a (-) | b (-) | ||
|---|---|---|---|
| 1 | 0.08 | 1.4 | |
| 2 | 0.09 | 1.2 | |
| 3 | 0.09 | 1.3 | |
| 3 | 0.023 | 2.3 | |
| 6 | 0.023 | 2.3 |
| (-) | (m) | (m) | (m) | (m) | (s) | (-) | (l/(sm)) | (-) | (-) | (-) | (m) | (m) | (m) | (m) | (s) | (-) | (l/(sm)) | (-) | (-) |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 0.0 | 0.921 | 0.300 | 0.081 | 1.43 | 6.27 | 0.010 | 1.0 | 1.0 | 2 | 0.05 | 1.10 | 0.121 | 0.056 | 1.43 | 3.77 | 0.004 | 0.49 | 0.37 |
| 1.01 | 0.211 | 0.059 | 1.46 | 7.52 | 0.001 | 1.0 | 1.0 | 0.082 | 1.38 | 3.01 | 0.042 | 0.46 | 0.38 | ||||||
| 0.080 | 1.41 | 6.25 | 0.053 | 1.0 | 1.0 | 0.082 | 1.38 | 3.00 | 0.050 | 0.47 | 0.39 | ||||||||
| 0.096 | 2.13 | 8.61 | 0.048 | 1.0 | 1.0 | 0.084 | 1.38 | 2.97 | 0.042 | 0.45 | 0.37 | ||||||||
| 0.096 | 2.13 | 8.58 | 0.103 | 1.0 | 1.0 | 0.088 | 2.11 | 4.45 | 0.234 | 0.53 | 0.37 | ||||||||
| 0.097 | 2.13 | 8.55 | 0.056 | 1.0 | 1.0 | 0.3 | 0.921 | 0.300 | 0.170 | 2.11 | 3.19 | 0.036 | 0.47 | 0.38 | |||||
| 1.10 | 0.121 | 0.061 | 1.46 | 7.42 | 0.095 | 1.0 | 1.0 | 1.01 | 0.211 | 0.064 | 1.36 | 3.34 | 0.008 | 0.73 | 0.68 | ||||
| 0.086 | 1.39 | 5.93 | 0.654 | 1.0 | 1.0 | 0.091 | 1.34 | 2.79 | 0.015 | 0.56 | 0.51 | ||||||||
| 0.05 | 1.01 | 0.211 | 0.085 | 2.18 | 9.36 | 0.041 | 0.76 | 0.54 | 0.166 | 2.01 | 3.08 | 0.354 | 0.49 | 0.41 | |||||
| 0.085 | 2.14 | 9.18 | 0.026 | 0.73 | 0.48 | 1.10 | 0.121 | 0.111 | 1.37 | 2.57 | 0.367 | 0.48 | 0.43 | ||||||
| 0.086 | 2.15 | 9.16 | 0.011 | 0.67 | 0.37 | 0.116 | 1.38 | 2.53 | 0.109 | 0.40 | 0.35 | ||||||||
| 1.10 | 0.121 | 0.055 | 1.46 | 7.79 | 0.030 | 0.69 | 0.46 | 3 | 0.0 | 0.921 | 0.300 | 0.074 | 1.42 | 2.17 | 0.000 | 1.0 | 1.0 | ||
| 0.079 | 1.40 | 6.23 | 0.276 | 0.65 | 0.47 | 0.090 | 1.38 | 1.92 | 0.007 | 1.0 | 1.0 | ||||||||
| 0.079 | 1.40 | 6.20 | 0.284 | 0.65 | 0.47 | 1.01 | 0.211 | 0.073 | 1.44 | 2.22 | 0.011 | 1.0 | 1.0 | ||||||
| 0.079 | 1.39 | 6.18 | 0.286 | 0.65 | 0.47 | 0.094 | 1.39 | 1.89 | 0.092 | 1.0 | 1.0 | ||||||||
| 0.089 | 2.18 | 9.12 | 0.877 | 0.70 | 0.42 | 0.095 | 2.09 | 2.83 | 0.540 | 1.0 | 1.0 | ||||||||
| 0.3 | 0.921 | 0.300 | 0.081 | 1.38 | 6.07 | 0.001 | 0.81 | 0.71 | 0.095 | 2.08 | 2.82 | 0.493 | 1.0 | 1.0 | |||||
| 0.171 | 2.18 | 6.59 | 0.127 | 0.61 | 0.39 | 0.097 | 2.10 | 2.81 | 0.514 | 1.0 | 1.0 | ||||||||
| 1.01 | 0.211 | 0.061 | 1.31 | 6.64 | 0.014 | 0.95 | 0.92 | 1.10 | 0.121 | 0.072 | 1.48 | 2.30 | 0.266 | 1.0 | 1.0 | ||||
| 0.084 | 1.30 | 5.63 | 0.032 | 0.75 | 0.64 | 0.098 | 1.40 | 1.87 | 0.723 | 1.0 | 1.0 | ||||||||
| 0.168 | 2.13 | 6.48 | 0.715 | 0.60 | 0.37 | 0.05 | 1.01 | 0.211 | 0.085 | 2.11 | 3.02 | 0.002 | 0.54 | 0.48 | |||||
| 1.10 | 0.121 | 0.095 | 1.38 | 5.58 | 0.383 | 0.60 | 0.41 | 0.086 | 2.10 | 2.98 | 0.000 | 0.45 | 0.37 | ||||||
| 0.137 | 1.40 | 4.72 | 0.077 | 0.39 | 0.17 | 0.086 | 2.07 | 2.94 | 0.001 | 0.51 | 0.45 | ||||||||
| 2 | 0.0 | 0.921 | 0.300 | 0.066 | 1.45 | 3.52 | 0.001 | 1.0 | 1.0 | 1.10 | 0.121 | 0.061 | 1.40 | 2.37 | 0.002 | 0.46 | 0.42 | ||
| 0.088 | 1.41 | 2.97 | 0.025 | 1.0 | 1.0 | 0.086 | 1.36 | 1.93 | 0.010 | 0.41 | 0.40 | ||||||||
| 1.01 | 0.211 | 0.062 | 1.46 | 3.65 | 0.016 | 1.0 | 1.0 | 0.086 | 1.36 | 1.93 | 0.011 | 0.41 | 0.40 | ||||||
| 0.090 | 2.13 | 4.45 | 0.226 | 1.0 | 1.0 | 0.086 | 1.36 | 1.93 | 0.010 | 0.41 | 0.40 | ||||||||
| 0.090 | 2.12 | 4.42 | 0.261 | 1.0 | 1.0 | 0.089 | 2.07 | 2.89 | 0.090 | 0.49 | 0.42 | ||||||||
| 0.090 | 2.13 | 4.42 | 0.260 | 1.0 | 1.0 | 0.3 | 0.921 | 0.300 | 0.161 | 2.09 | 2.17 | 0.020 | 0.49 | 0.47 | |||||
| 0.092 | 1.41 | 2.91 | 0.141 | 1.0 | 1.0 | 1.01 | 0.211 | 0.059 | 1.35 | 2.32 | 0.008 | 0.86 | 0.85 | ||||||
| 1.10 | 0.121 | 0.065 | 1.46 | 3.58 | 0.252 | 1.0 | 1.0 | 0.085 | 1.35 | 1.94 | 0.010 | 0.62 | 0.61 | ||||||
| 0.095 | 1.41 | 2.85 | 0.884 | 1.0 | 1.0 | 0.161 | 2.05 | 2.12 | 0.245 | 0.50 | 0.48 | ||||||||
| 0.05 | 1.01 | 0.211 | 0.085 | 2.07 | 4.45 | 0.004 | 0.54 | 0.39 | 1.10 | 0.121 | 0.116 | 1.36 | 1.67 | 0.441 | 0.55 | 0.55 | |||
| 0.085 | 2.08 | 4.45 | 0.003 | 0.52 | 0.36 | 0.134 | 1.38 | 1.57 | 0.154 | 0.46 | 0.46 | ||||||||
| 0.085 | 2.09 | 4.48 | 0.003 | 0.51 | 0.35 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kerpen, N.B.; Schoonees, T.; Schlurmann, T. Wave Overtopping of Stepped Revetments. Water 2019, 11, 1035. https://doi.org/10.3390/w11051035
Kerpen NB, Schoonees T, Schlurmann T. Wave Overtopping of Stepped Revetments. Water. 2019; 11(5):1035. https://doi.org/10.3390/w11051035
Chicago/Turabian StyleKerpen, Nils B., Talia Schoonees, and Torsten Schlurmann. 2019. "Wave Overtopping of Stepped Revetments" Water 11, no. 5: 1035. https://doi.org/10.3390/w11051035
APA StyleKerpen, N. B., Schoonees, T., & Schlurmann, T. (2019). Wave Overtopping of Stepped Revetments. Water, 11(5), 1035. https://doi.org/10.3390/w11051035






