Non-Parametric Regression Analysis of Diuron and Gabapentin Degradation in Lake Constance Water by Ozonation and Their Toxicity Assessment
Abstract
:1. Introduction
2. Material and Methods
2.1. Material and Water Quality Measurement Methods
2.2. Sample Preparation
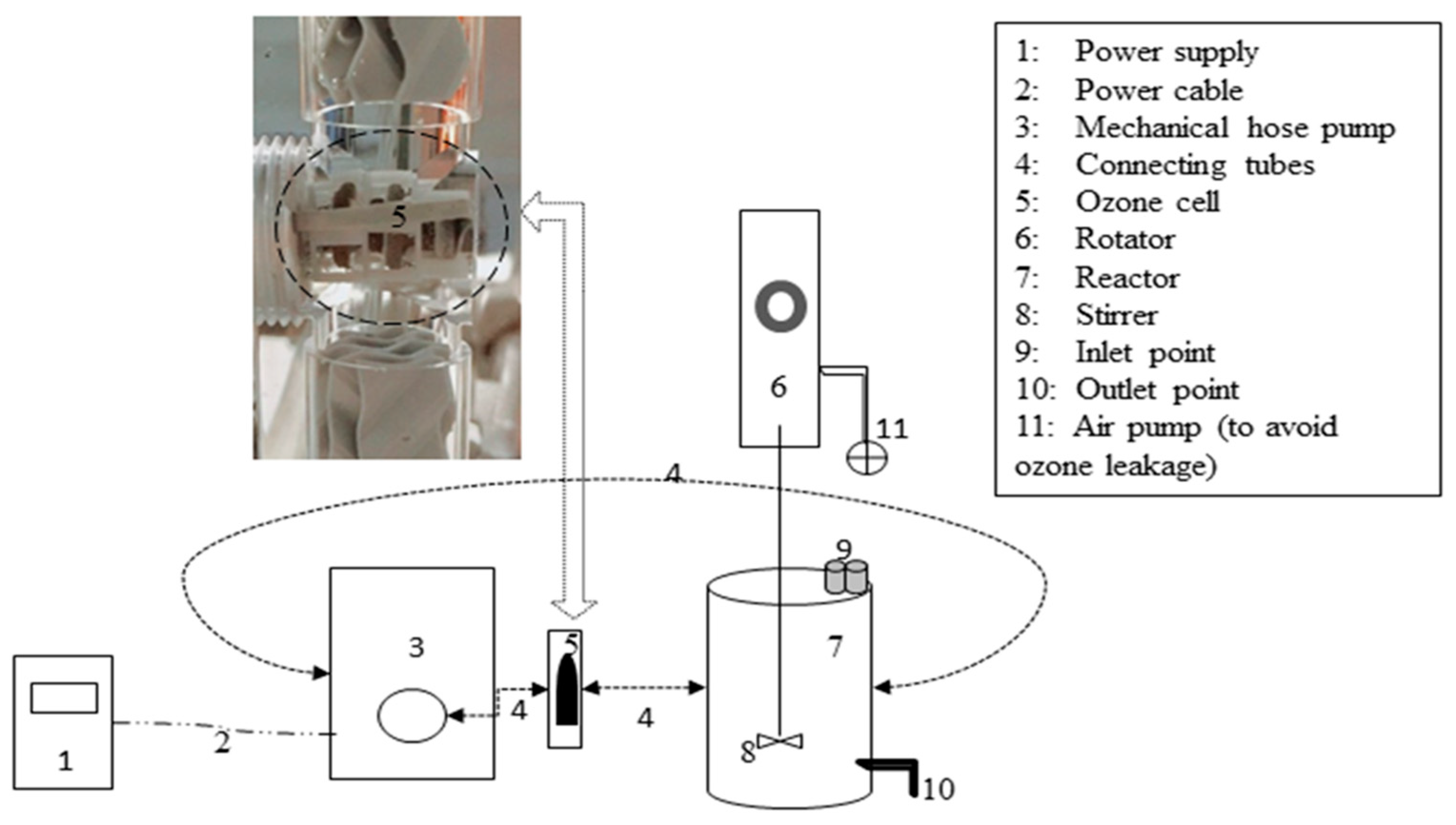
2.3. Experimental Process and Data Analysis
2.4. Ozone Measurement
2.5. Pollutants’ Concentration Measurement
2.6. Toxicity Assessment
3. Results and Discussion
3.1. Characterisation of Lake Constance Water and Inlet Ozone Concentration
3.2. Degradation and Mineralization of the Pollutants
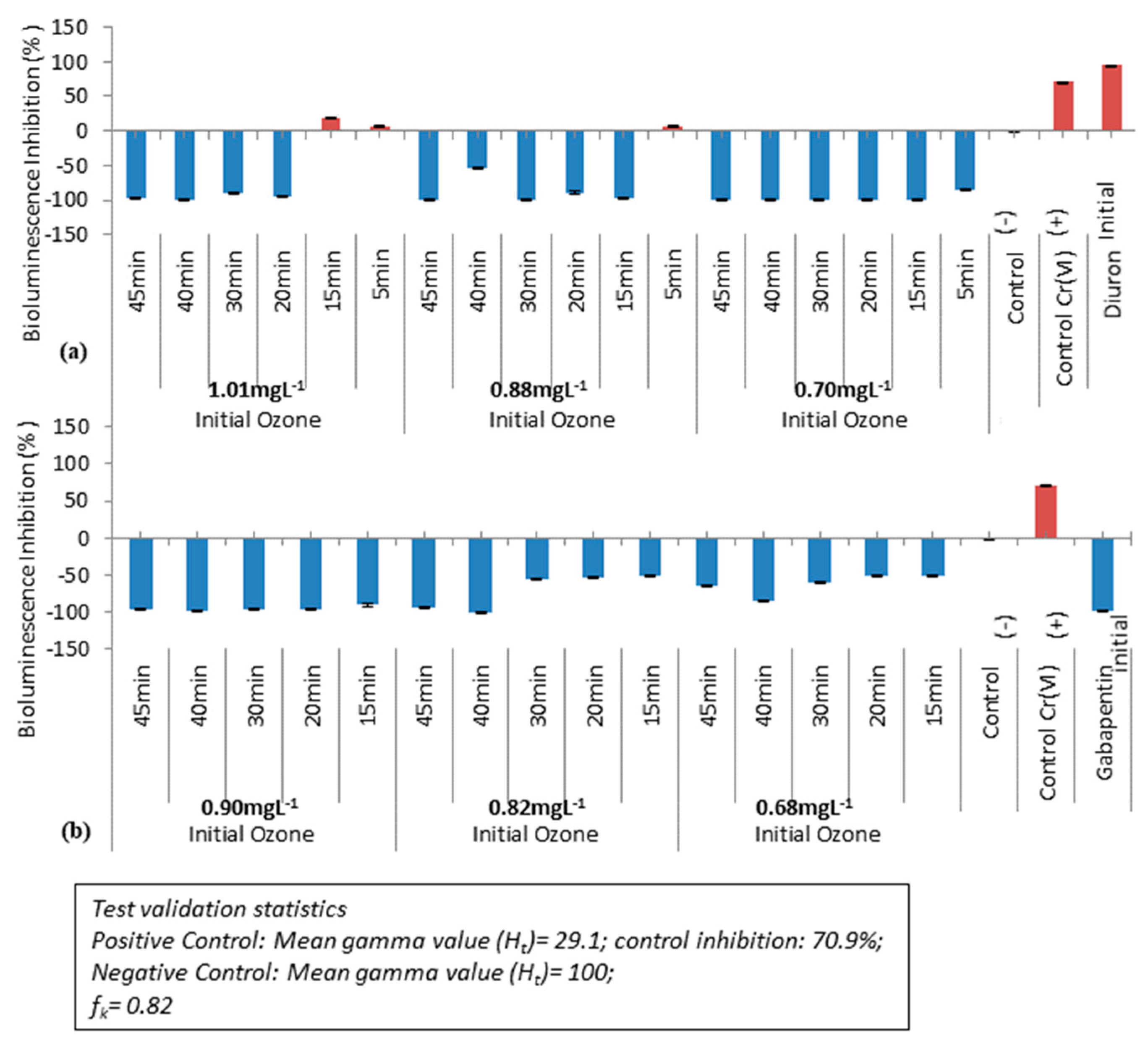
3.3. Toxicity Assessment
3.4. Kruskal–Wallis Test
3.5. Generalised Linear Model
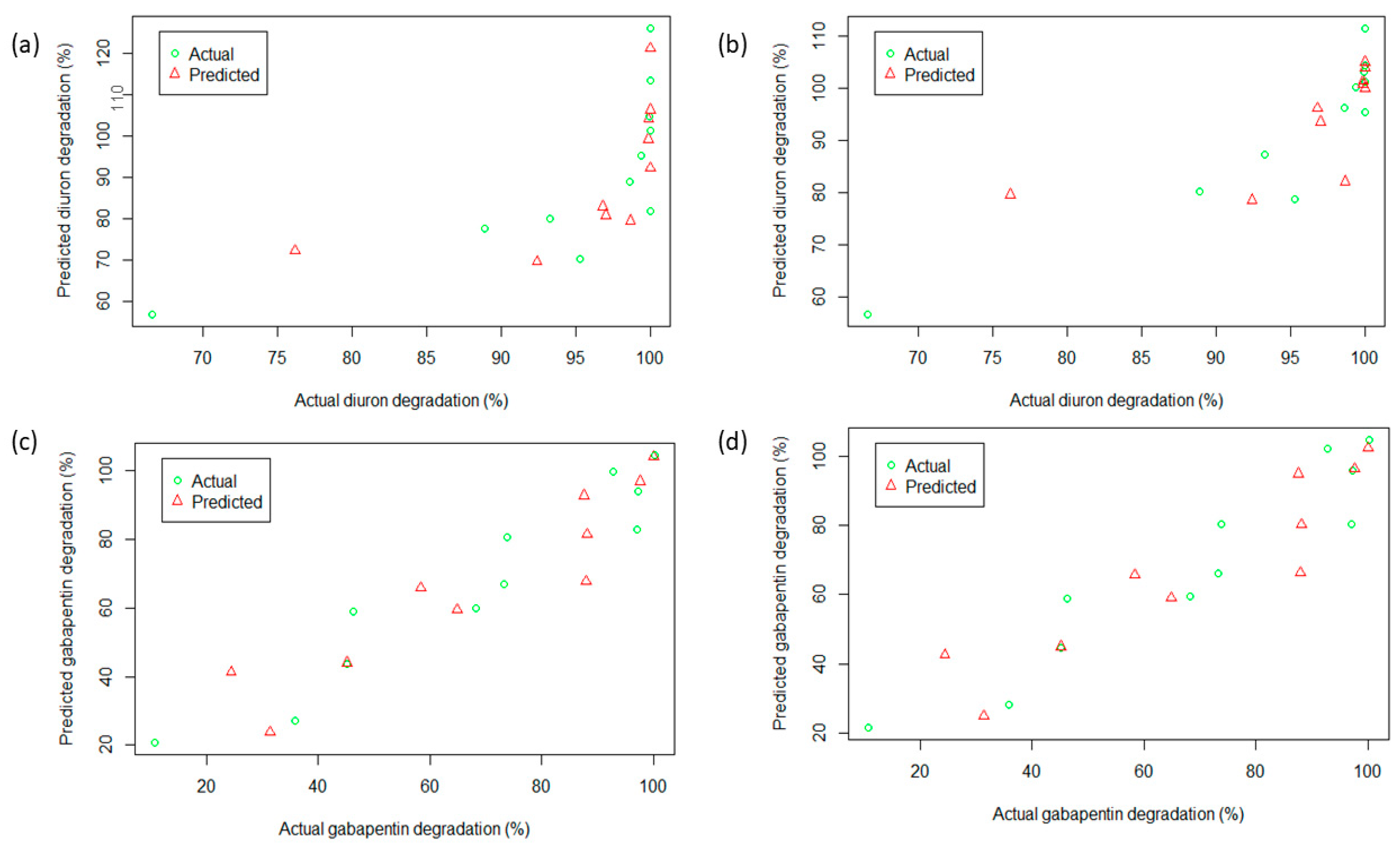
3.6. Gaussian Model Prediction
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Proposal for a Directive of the Europian Parliament of the Council Amending Directives 2000/60/EC and 2008/105/EC as Regards Priority Substances in the Field of Water Policy. 2012. Available online: https://europa.eu/european-union/eu-law/legal-acts_en (accessed on 27 February 2018).
- Thornton, I.; Butler, D.; Docx, P.; Hession, M.; Markropoulos, C.M.M. Pollutants in Urban Waste Water and Sewage Sludge; Office for Official Publications of the European Communities: Luxembourg, 2001. [Google Scholar]
- Benitez, F.J.; Real, F.J.; Acero, J.L.; Garcia, C. Kinetics of the transformation of phenyl-urea herbicides during ozonation of natural waters: Rate constants and model predictions. Water Res. 2007, 41, 4073–4084. [Google Scholar] [CrossRef] [PubMed]
- Herrmann, M.; Menz, J.; Olsson, O.; Kümmerer, K. Identification of phototransformation products of the antiepileptic drug gabapentin: Biodegradability and initial assessment of toxicity. Water Res. 2015, 85, 11–21. [Google Scholar] [CrossRef] [PubMed]
- Arslan, A.; Veli, S.; Bingöl, D. Use of response surface methodology for pretreatment of hospital wastewater by O3/UV and O3/UV/H2O2 processes. In Separation and Purification Technology; Elsevier: Amsterdam, The Netherlands, 2014; pp. 561–567. [Google Scholar]
- Paździor, K.; Wrebiak, J.; Smólka, A.K.; Gmurek, M.; Bilińska, L.; Kos, L.; Ledakowicz, J.S.; Ledakowicz, S. Influence of ozonation and biodegradation on toxicity of industrial textile wastewater. J. Environ. Manag. 2017, 195, 166–173. [Google Scholar] [CrossRef] [PubMed]
- Ziabari, S.S.H.; Khezri, S.M.; Kalantary, R.R. Ozonation optimization and modeling for treating diesel-contaminated water. Mar. Pollut. Bull. 2016, 104, 240–245. [Google Scholar] [CrossRef] [PubMed]
- Tizaoui, C.; Grima, N. Kinetics of the ozone oxidation of Reactive Orange 16 azo-dye in aqueous solution. Chem. Eng. J. 2011, 173, 463–473. [Google Scholar] [CrossRef]
- Robinson, T.J. Book review: Generalized linear models: With applications in engineering and the sciences. IIE Trans. 2003, 35, 917–918. [Google Scholar] [CrossRef]
- Snelson, E.L. Flexible and Efficient Gaussian Process Models for Machine Learning. Ph.D. Thesis, University London, London, UK, 2007; pp. 1–135. [Google Scholar]
- Gilbert, E.; Hoigné, J. Messung von ozon in wasserwerken: Vergleich der DPD- und indigo-methode. GWF-Wasser/Abwasser 1983, 124, 527–531. [Google Scholar]
- Jiang, J.-Q.; Durai, H.B.P.; Petri, M.; Grummt, T.; Winzenbacher, R. Drinking water treatment by ferrate(VI) and toxicity assessment of the treated water. Desalinat. Water Treat. 2016, 57, 26369–26375. [Google Scholar] [CrossRef]
- McCullagh, P.; Nelder, J.A. Generalized linear models. J. R. Stat. Soc. 1972, 135, 370–384. [Google Scholar] [CrossRef]
- Fox, J. Effect displays in R for generalised linear models. J. Stat. Softw. 2003, 8, 1–27. [Google Scholar] [CrossRef]
- McCullagh, P.; Nelder, J.A. Generalized Linear Models, 2nd ed.; CRC Press: Boca Raton, FL, USA, 1989. [Google Scholar]
- Burnham, K.P.; Anderson, D.R. Multimodel inference: Understanding AIC and BIC in model selection. Sociol. Methods Res. 2004, 33, 261–304. [Google Scholar] [CrossRef]

| Gradient Programme no. | Time (min) | Eluent A (%) | Eluent B (%) | Volume Flow (µL min−1) |
|---|---|---|---|---|
| 0 | 0 | 95 | 5 | 300 |
| 1 | 0.5 | 80 | 20 | 300 |
| 2 | 10 | 20 | 80 | 300 |
| 3 | 12 | 20 | 80 | 300 |
| 4 | 12.5 | 95 | 5 | 300 |
| 5 | 20 | 95 | 5 | 300 |
| Parameter | Value |
|---|---|
| pH | 8.0 ± 0.09 at 25 °C |
| Temperature | 7.0 ± 2.5 °C |
| Turbidity | 0.30 ± 0.03 NTU |
| Colour at 436 nm | 0.10 ± 0.02 m−1 |
| UV-abs at 254 nm | 3.1 ± 0.1 m−1 |
| TOC | 1.2 ± 0.1 mg L−1 |
| Diuron | <LOQ (10 ng L−1) |
| Gabapentin | 40 ng L−1 |
| Organic Compound | Low-Level Inlet mgO3 L−1 | Medium Level Inlet mgO3 L−1 | High-Level Inlet mgO3 L−1 | ||||||
|---|---|---|---|---|---|---|---|---|---|
| R1 | R2 | Average | R1 | R2 | Average | R1 | R2 | Average | |
| Diuron | 0.71 | 0.68 | 0.70 | 0.86 | 0.89 | 0.88 | 1.01 | 1.01 | 1.01 |
| Gabapentin | 0.68 | 0.68 | 0.68 | 0.81 | 0.83 | 0.82 | 0.91 | 0.90 | 0.90 |
| R1: replication 1; R2: replication 2 | |||||||||
| Gabapentin | Diuron | ||||||||
|---|---|---|---|---|---|---|---|---|---|
| min | (R1) mgO3 L−1 | (R2) mgO3 L−1 | Average | Variance | min | (R1) mgO3 L−1 | (R2) mgO3 L−1 | Average | Variance |
| 0 | 0.68 | 0.68 | 0.68 | 0 | 0 | 0.71 | 0.68 | 0.70 | 3.59 × 10−5 |
| 1 | 0.26 | 0.37 | 0.31 | 5.75 × 10−3 | 1 | 0.26 | 0.31 | 0.28 | 1.10 × 10−3 |
| 5 | 0.07 | 0.07 | 0.07 | 0 | 5 | 0.13 | 0.11 | 0.12 | 2.02 × 10−4 |
| 15 | 0.07 | 0.05 | 0.06 | 8.98 × 10−5 | 15 | 0.15 | 0.15 | 0.15 | 2.24 × 10−5 |
| 20 | 0.07 | 0.05 | 0.06 | 2.02 × 10−4 | 20 | 0.17 | 0.15 | 0.16 | 2.02 × 10−4 |
| 30 | 0.08 | 0.05 | 0.07 | 3.48 × 10−4 | 30 | 0.22 | 0.21 | 0.21 | 9.66 × 10−5 |
| 40 | 0.09 | 0.11 | 0.10 | 3.59 × 10−4 | 40 | 0.30 | 0.25 | 0.27 | 1.44 × 10−3 |
| 45 | 0.10 | 0.11 | 0.10 | 2.24 × 10−5 | 45 | 0.32 | 0.21 | 0.27 | 5.75 × 10−3 |
| 0 | 0.81 | 0.84 | 0.82 | 3.59 × 10−4 | 0 | 0.86 | 0.89 | 0.88 | 3.59 × 10−4 |
| 1 | 0.50 | 0.40 | 0.45 | 5.75 × 10−3 | 1 | 0.21 | 0.38 | 0.30 | 1.40 × 10−2 |
| 5 | 0.13 | 0.13 | 0.13 | 2.24 × 10−5 | 5 | 0.13 | 0.23 | 0.18 | 5.05 × 10−3 |
| 15 | 0.08 | 0.08 | 0.08 | 0 | 15 | 0.14 | 0.21 | 0.17 | 2.24 × 10−3 |
| 20 | 0.12 | 0.09 | 0.10 | 5.61 × 10−4 | 20 | 0.16 | 0.24 | 0.20 | 3.24 × 10−3 |
| 30 | 0.13 | 0.11 | 0.12 | 2.02 × 10−4 | 30 | 0.20 | 0.32 | 0.26 | 7.27 × 10−3 |
| 40 | 0.22 | 0.15 | 0.19 | 2.24 × 10−3 | 40 | 0.29 | 0.43 | 0.36 | 1.06 × 10−2 |
| 45 | 0.26 | 0.17 | 0.21 | 4.40 × 10−3 | 45 | 0.34 | 0.48 | 0.41 | 1.09 × 10−2 |
| 0 | 0.91 | 0.9 | 0.90 | 6.27 × 10−5 | 0 | 1.01 | 1.01 | 1.01 | 2.24 × 10−5 |
| 1 | 0.38 | 0.37 | 0.37 | 7.08 × 10−5 | 1 | 0.50 | 0.52 | 0.51 | 8.98 × 10−5 |
| 5 | 0.09 | 0.08 | 0.08 | 2.52 × 10−5 | 5 | 0.37 | 0.38 | 0.38 | 5.62 × 10−5 |
| 15 | 0.07 | 0.07 | 0.07 | 6.84 × 10−6 | 15 | 0.40 | 0.41 | 0.40 | 3.61 × 10−5 |
| 20 | 0.08 | 0.08 | 0.08 | 8.00 × 10−8 | 20 | 0.39 | 0.49 | 0.44 | 4.90 × 10−3 |
| 30 | 0.10 | 0.11 | 0.11 | 4.51 × 10−5 | 30 | 0.59 | 0.55 | 0.57 | 7.20 × 10−4 |
| 40 | 0.17 | 0.16 | 0.16 | 2.81 × 10−5 | 40 | 0.56 | 0.45 | 0.50 | 6.20 × 10−3 |
| 45 | 0.17 | 0.05 | 0.05 | 6.90 × 10−3 | 45 | 0.48 | 0.38 | 0.43 | 4.82 × 10−3 |
| Organic-Pollutant | Chi-Squared | Degree of Freedom | P-Value | Significance |
|---|---|---|---|---|
| Diuron | 35.70 | 2 | 1.7 × 10−8 | Significant |
| Gabapentin | 33.15 | 2 | 6.3 × 10−8 | Significant |
| Analyte | Model | Interaction Studied | Significant Interaction (Ozone × Reaction Time) | AIC | Model Significance |
|---|---|---|---|---|---|
| Diuron | |||||
| Poisson | No | - | Infinity | No | |
| Gaussian | No | - | 220.69 | Yes | |
| Poisson | Yes | Yes | Infinity | No | |
| Gaussian | Yes | Yes | 208.89 | Yes | |
| Gabapentin | |||||
| Poisson | No | - | Infinity | No | |
| Gaussian | No | - | 188.15 | Yes | |
| Poisson | Yes | Yes | Infinity | No | |
| Gaussian | Yes | No | 189.46 | Yes | |
| AIC: Akaike information criterion | |||||
| Analyte | Estimate | Coefficients | Std. Error | z Value | P | Significant |
|---|---|---|---|---|---|---|
| Diuron | ||||||
| Intercept | 88.37 | 9.76 | 9.06 | 1.06 × 10−8 | Yes | |
| Ozone | −64.01 | 16.33 | −3.92 | 0.000789 | Yes | |
| Reaction time | 0.98 | 0.27 | 3.58 | 0.001747 | Yes | |
| Gabapentin | ||||||
| Intercept | 39.42 | 5.44 | 7.25 | 3.84 × 10−7 | Yes | |
| Ozone | −45.08 | 10.96 | −4.11 | 0.000497 | Yes | |
| Reaction time | 1.55 | 0.16 | 9.56 | 4.27 × 10−9 | Yes |
| Analyte | Estimate | Coefficients | Std. Error | z Value | P | Significant | |
|---|---|---|---|---|---|---|---|
| Diuron | |||||||
| Intercept | 112.84 | 9.73 | 11.59 | 2.49 × 10−10 | Yes | ||
| Ozone | −112.60 | 0.39 | −0.82 | 0.424535 | No | ||
| Reaction time | −0.33 | 17.59 | −6.40 | 3.04 × 10−6 | Yes | ||
| Ozone × Reaction time | 2.86 | 0.73 | 3.94 | 0.000804 | Yes | ||
| Gabapentin | |||||||
| Intercept | 41.31 | 6.02 | 6.862 | 0.00000115 | Yes | ||
| Ozone | −48.50 | 11.94 | −4.06 | 0.0006 | Yes | ||
| Reaction time | 1.30 | 0.36 | 3.58 | 0.0019 | Yes | ||
| Ozone × Reaction time | 1.60 | 2.09 | 0.77 | 0.4530 | No | ||
| Pollutant | Interaction | Correlation |
|---|---|---|
| Diuron | ||
| No | 0.67 | |
| Yes | 0.84 | |
| Gabapentin | ||
| No | 0.94 | |
| Yes | 0.94 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Goswami, A.; Jiang, J.-Q.; Petri, M. Non-Parametric Regression Analysis of Diuron and Gabapentin Degradation in Lake Constance Water by Ozonation and Their Toxicity Assessment. Water 2019, 11, 852. https://doi.org/10.3390/w11040852
Goswami A, Jiang J-Q, Petri M. Non-Parametric Regression Analysis of Diuron and Gabapentin Degradation in Lake Constance Water by Ozonation and Their Toxicity Assessment. Water. 2019; 11(4):852. https://doi.org/10.3390/w11040852
Chicago/Turabian StyleGoswami, Anuradha, Jia-Qian Jiang, and Michael Petri. 2019. "Non-Parametric Regression Analysis of Diuron and Gabapentin Degradation in Lake Constance Water by Ozonation and Their Toxicity Assessment" Water 11, no. 4: 852. https://doi.org/10.3390/w11040852
APA StyleGoswami, A., Jiang, J.-Q., & Petri, M. (2019). Non-Parametric Regression Analysis of Diuron and Gabapentin Degradation in Lake Constance Water by Ozonation and Their Toxicity Assessment. Water, 11(4), 852. https://doi.org/10.3390/w11040852






