1. Introduction
This paper presents the problem of reservoir operation in the specific conditions of lowland areas. The paper focuses on special type of reservoir, whose construction has become rather popular. These are two-stage reservoirs, consisting of a preliminary sedimentation zone and a main zone. Only the main zone stores water for basic purposes of reservoir operation. The popularity of the two-stage reservoir is related to the fact that its construction reflects the nature of the reservoir sedimentation process. This feature simplifies removal of sediments without raising additional problems in the reservoir operation. Because in the past such solutions were not very popular and have become quite popular recently, the functioning of two-stage reservoirs is not understood at a satisfactory level.
The water resources in Poland compared to other European countries are relatively limited. Hence, it is important to build water reservoirs improving water balance, environmental water conditions, as well as microclimate. Typical reservoirs may be used for different purposes including drinking water supply, industrial water supply, and flood protection. Building reservoirs is economically profitable due to the production of electricity in water power plants. Reservoirs also may play an important role in tourism or as elements of the inland waterway.
The Wielkopolska (Great Poland) district is the region with the lowest rainfalls level in Poland. This is the reason why small retention is intensively developed in this area. There are 38 reservoirs classified as small retention objects. According to the Polish regulations, these are the reservoirs with storage smaller than 5 hm
3. In Wielkopolska, there are also two large reservoirs with storage exceeding 5 hm
3 [
1]. The number of reservoirs indicates the significance of the discussed problem. Additionally, it is worth noting the economic importance of the reservoirs.
Operation of the water reservoir may cause several problems. In general, processes which are difficult to control occur in the reservoir and around it. Two problems are common for small and large reservoirs. These are, (1) erosion downstream of the reservoir dam, and (2) sediment deposition in the backward part of the inflowing river [
2,
3,
4]. These processes occur relatively fast and cause a direct threat of reservoir catastrophe [
3,
5]. Although the processes occurring in the entire reservoir volume are also very important, they are slower and less visible in general [
4,
5,
6,
7]. The sediment transport in equilibrium conditions, occurring in the inflowing river, changes when water flows into the reservoir. The sediment transport potential rapidly decreases and the stream is not able to transport the same amount of sediments. Suspended and bed-loaded grains are deposited, creating alluvial fans and deltas in the inlet part of the reservoir. The density currents influence the water circulation in the reservoir. Finally, the fine sediments deposited in the deeper parts of the reservoir reduce the available volume and change the operational conditions in the longer time horizon [
8,
9,
10,
11,
12]. In the inlet part of the reservoir, the deposition causes a decrease in the local depths and expansion of the riparian vegetation [
6,
13]. In two-stage reservoirs, these processes occur in the preliminary sedimentation zone. Water pollutants are also inhibited there, which improves water quality in the main part of the reservoir. However, pollutants are frequently deposited in the sediments (e.g., heavy metals) [
14,
15,
16,
17].
The object analyzed is the Stare Miasto reservoir, located in the Powa river in central Poland. This is a lowland reservoir with specific construction of the bottom. There is a preliminary zone separated from the rest of the reservoir by an internal dam. The flow between the upper and lower parts of the reservoir is limited. The upper part is usually smaller and plays the role of the initial sediment container, whereas the lower part is greater and it is designed to provide water for the main purpose of the reservoir operation. Hence, the lower part is usually called the main reservoir. There are basic reservoir capacities (i.e., active conservation storage, flood storage capacity, flood surcharge capacity, as well as dead storage). The described solution is one of the methods applied for prevention of sedimentation and water quality degradation in reservoirs [
11,
18,
19]. Such an approach is not prohibitively expensive, though there are also some drawbacks. From the economic point of view, separation of the upper part means the loss of its conservation or flood storage. For this reason, broad research on such reservoirs, describing their operational conditions, seems worthwhile.
Taking into account the simplicity of the described concept, it is expected that such reservoirs will be built more frequently. In literature such objects have been rarely analyzed. Two other problems are discussed: (a) water and sediment quality [
15,
16,
17,
19,
20]; (b) reservoir sedimentation [
4,
5,
11,
21,
22,
23]. These two processes make the described reservoir construction so attractive. Today a number of two-stage reservoirs in Poland can be found, for example, the Poraj reservoir in the Warta river, the Rydzyny reservoir in the Sama river, Kowalskie Lake in the Główna river, or the new concept of the Wielowieś Klasztorna reservoir in the Prosna river.
The basic feature of lowland reservoirs is very good vertical mixing of heat and dissolved substances. It means the lack of temperature stratification and uniform distribution of solutes along the depth. Due to the small depths, density currents caused by temperature and salinity gradients should not be present in such reservoirs. As reported by Krenkel et al. [
24], the processes of heat and dissolved mass exchange in reservoirs without stratification vary in horizontal dimensions as in rivers.
Measurements and field surveys provide limited information on the spatial as well as temporal scale. The sampling techniques provide point information. Detailed description of the spatial distributions of investigated parameters (e.g., velocities, temperature, etc.) requires a huge number of such measurements and, obviously, it is time-consuming and expensive. A similar problem arises when the temporal variability of the selected parameters is formulated. The duration of the analyzed processes is important. The description of their dynamics requires continuous measurements or very frequent momentary measurements. Hence, the application of mathematical modeling in the analysis may help. Different types of models for description of river and reservoir dynamics have been applied for many years. One-dimensional (1D) unsteady flow models are the simplest models applied for reservoirs [
25,
26]. However, models of this kind are able to reconstruct properly only the variability of the depth in the reservoir. Other hydraulic parameters (e.g., velocities, shear stress, etc.) are determined approximately. Modeling of other processes in 1D mode for reservoirs is burdened with significant inaccuracies. Because of this, 2D and 3D modeling is more frequently used for analysis of reservoirs. In the lowland reservoir, 2D models are preferred due to relatively small depths [
25,
27,
28,
29,
30]. Such an approach enables determination of quite accurate spatial distributions of basic hydraulic variables (e.g., depth and velocity) and parameters dependent on their values (e.g., shear stress, stream power, and others) [
29,
30]. More accurate reconstruction of the hydraulic variables range is also the basis for modeling of other processes (e.g., transport of pollutants or sediment transport). The field measurements impact the construction of the model during the procedure of identification and verification. The final product is a model giving results (e.g., depth, velocities, etc.), consistent with observations in the field. It may be applied for test computations and forecasting. Such a model may support analysis of spatial and temporal variability of the investigated variables.
The purpose of the present research is to analyze two-stage reservoir operation, with its hydraulic and operational problems. The presented approach is based on the 2D simulations of a hydrodynamic model made for a flood scenario selected from historical data. The flood wave observed between 10 April and 19 July of 2014 is used. The attention is focused on three variables identified as the measures of potential hazards: (1) magnitude of flow velocity, (2) shear stress, and (3) stream power. The role of the flow velocity in morphodynamical changes in rivers and reservoirs is well known. Such processes as sediment deposition and erosion are observed in locations of significant velocity changes (e.g., reservoir inlets, contractions in channels, etc.). The shear stress describes these processes better with the so-called incipient motion criteria. In such objects as reservoirs, the shear stresses have specific distributions, and they may be concentrated near hydraulic obstacles (e.g., dams and bridge piers). The stream power is a combination of velocity and shear stress, with potential to link the features of both variables. Hence the stream power is a basis of many sediment transport formulae applied for modeling of sediment deposition and erosion in rivers and reservoirs. The spatial distributions of these variables are confronted with the known locations of threats (e.g., internal dam almost crashed in 2014). The main assumption is that the careful analysis of such spatial distributions may help to prevent undesirable threats in the stage of reservoir design. The analyses are made for three bathymetries reconstructed for the years 2006, 2013, and 2018. The comparison of velocity, shear stress, and stream power distributions in these three moments of time could help to understand better the nature of processes responsible for morphodynamic changes in the reservoir.
2. Case Study System
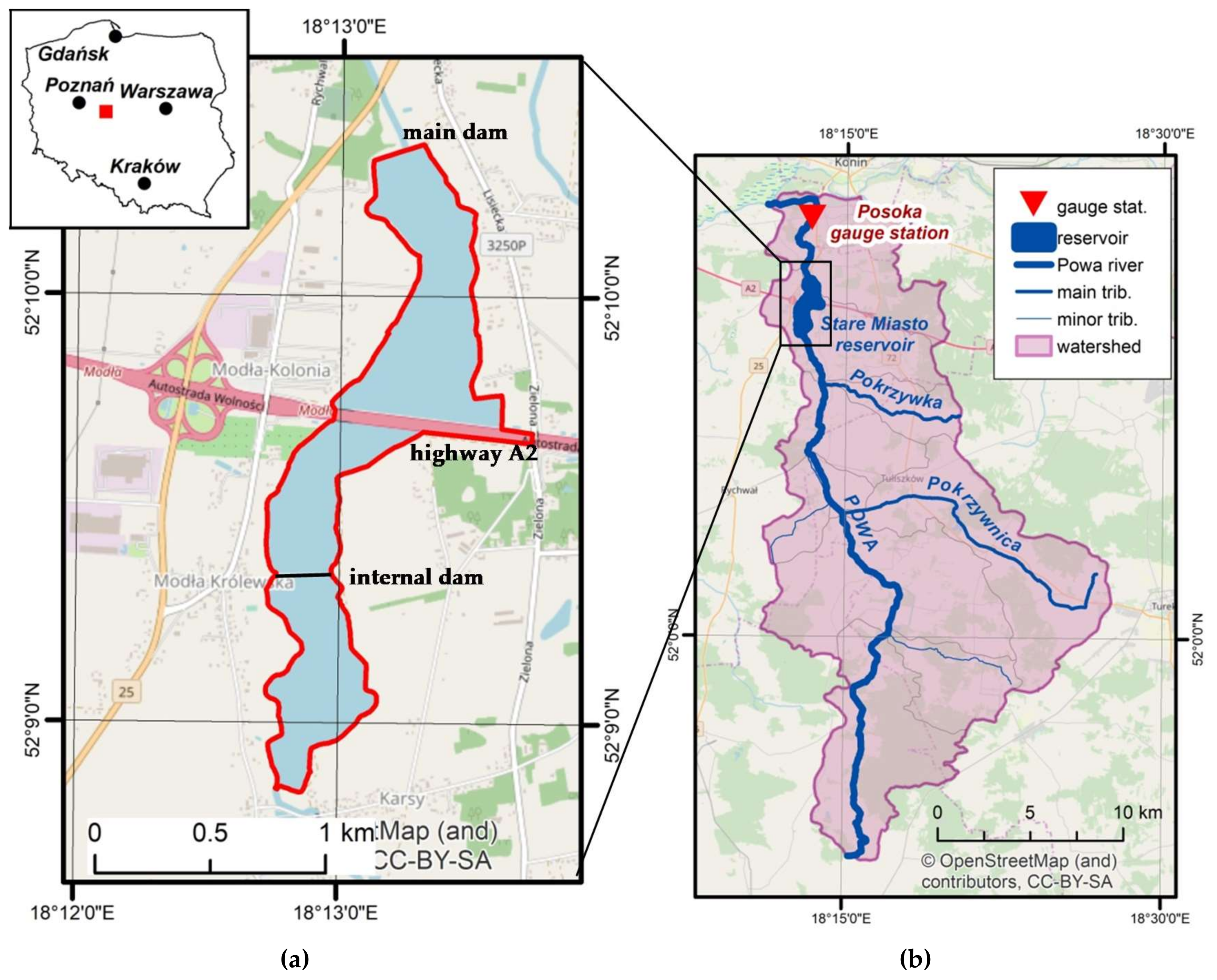
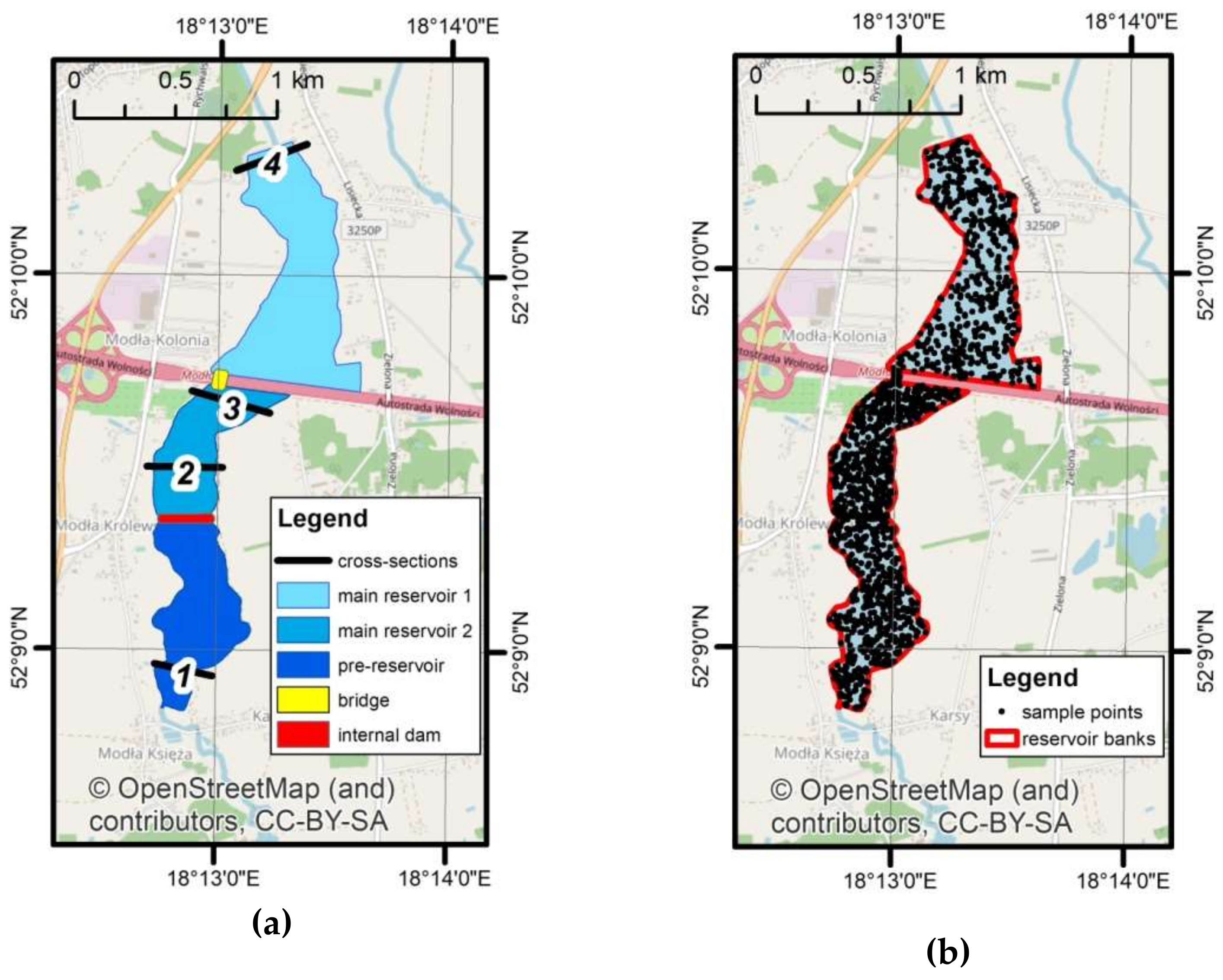
Taking into account the purpose of the research, quite a specific object, the Stare Miasto reservoir in the Powa river, was chosen as a case study. The Powa is a moderately sized river flowing in the lowland of Great Poland province in the central part of Poland. The Stare Miasto reservoir was built as an element of the small retention program [
31]. The purposes of this program are the improvement of watershed capacity, flood and drought protection, and prevention of a decrease in the groundwater table. The analyzed reservoir has been in operation since 2006. It is divided into two parts (
Figure 1), with an internal dam located at km 12 + 000 of the Powa river. There is a regulated sluice applied to control flow through the dam. The upper zone works as a sediment trap.
The inundation area of the upper part is 27 ha and its storage equals 0.294 hm
3. The lower part is the so-called main reservoir. This part is additionally split into two internal parts by highway A2 (
Figure 1), running from Poznań to Warsaw [
32]. The watershed area of the Stare Miasto reservoir in the cross-section of the main dam is 299.7 km
2. The average depth of the reservoir is 2.4 m. The estimated length of this object is 4.5 km. The inundation area for the minimum headwater level (MinPP = 92.0 m a.s.l.) is 75.77 ha. For the normal headwater level (NPP = 93.5 m a.s.l.) the inundation area is 90.68 ha. The maximum headwater level (MaxPP) is 94.0 m a.s.l. The total reservoir capacity is 2.159 hm
3. The active conservation storage is 1.216 hm
3. The inundation area of the upper zone is 13.62% of the total water surface area. The land use in the neighborhood of the reservoir agriculture. Because the usefulness of the terrain is limited, crop production has been stopped in this region [
32,
33]. The reservoir is working in an annual compensation cycle, which may cause annual variation in the water level, from MinPP to NPP. The operational water level is from 92.00 m a.s.l. to 94.00 m a.s.l. The reservoir is filled in March and the water surface is kept at the level of NPP until the end of October. After October, the main reservoir is emptied to the level of MinPP. To protect the inlet part from degradation and sediment accumulation, the water surface in the upper part is kept at the NPP level for the entire year.
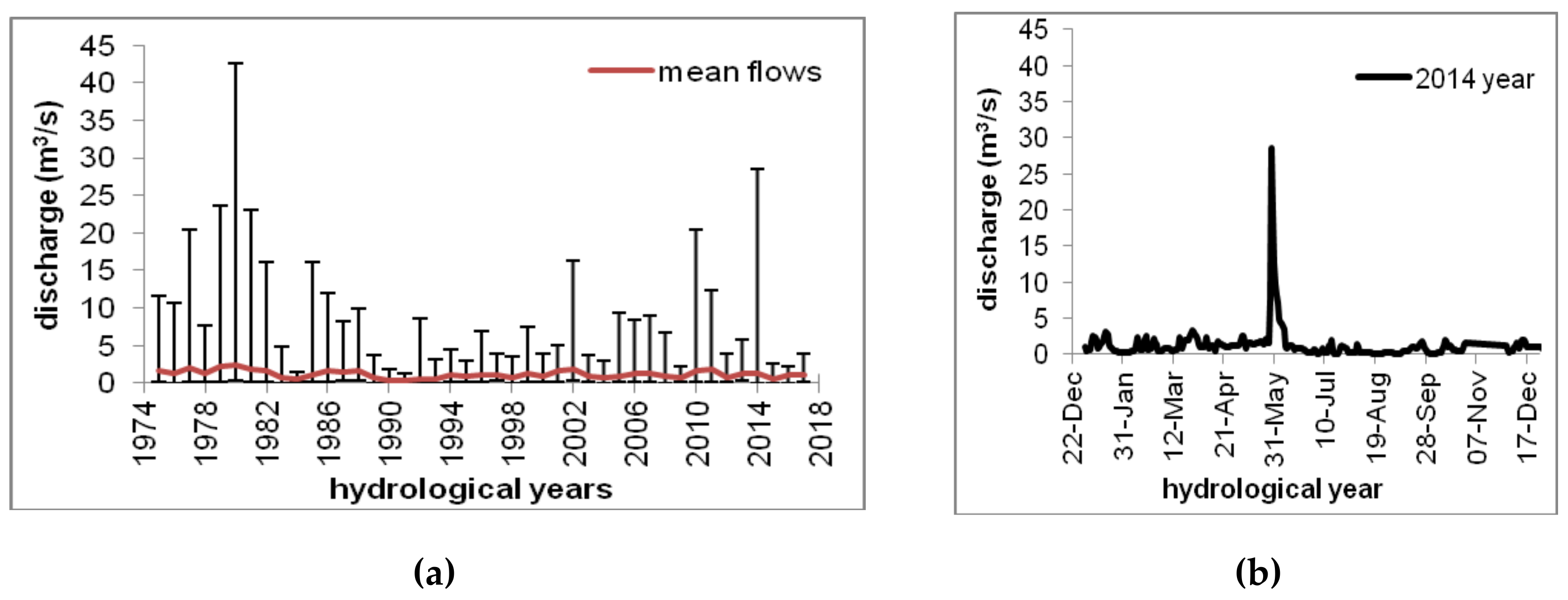
In the Powa river there is one gauge station called Posoka (
Figure 1b). This is located downstream of the reservoir at km 3 + 900 of the Powa course. The watershed area in the cross-section of the gauge is 332 km
2. The characteristic flows were determined on the basis of recorded observations at this gauge station during the period 1975–2017 [
34]. The results are presented in
Figure 2. The total minimum observed was 0.006 m
3/s, while the total maximum was 42.6 m
3/s. The mean flow was about 1.15 m
3/s. During the time of reservoir operation, from 2006 to 2017, the maximum flow of 28.5 m
3/s (
Figure 2b) occurred in 2014. The minimum and mean flows did not differ much from those estimated for the entire period. In the analyzed period, the average annual outflow from the reservoir was 36.9 hm
3.
Operational Problems in the Reservoir
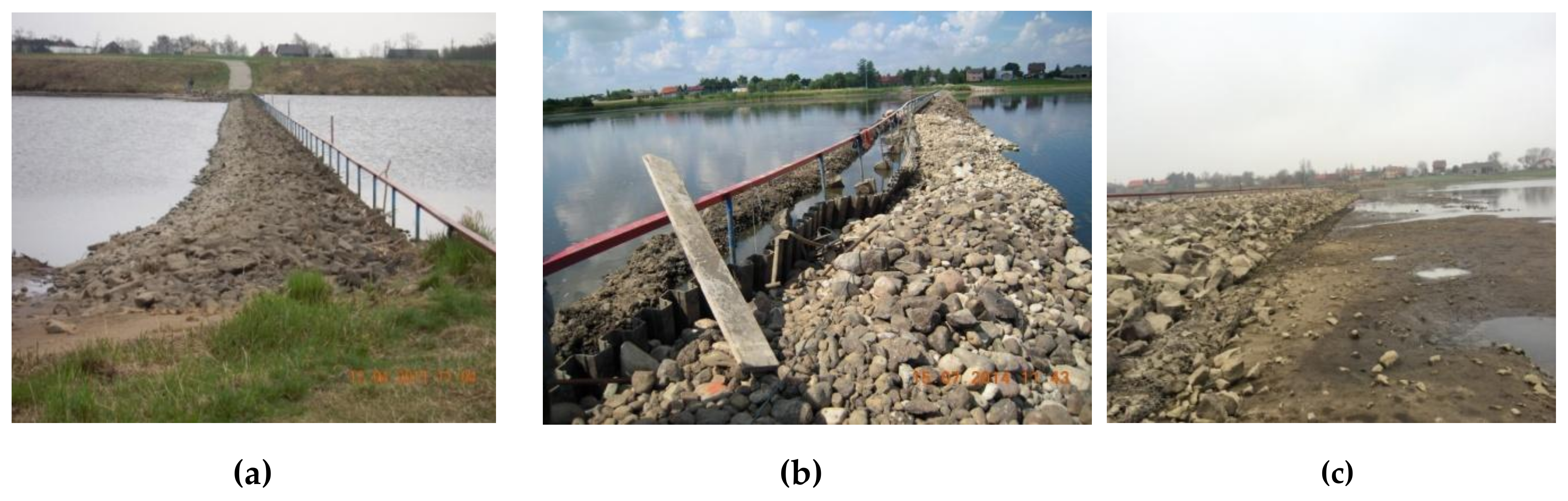
Operational problems have been present in the Stare Miasto reservoir since its construction. The first of them is caused by the internal dam splitting the reservoir into two parts. As mentioned above, the headwater level should be constant over the entire year (
Figure 3a–c). The flow through the internal dam from the preliminary sedimentation zone to the main part of the reservoir is regulated by the sluice installed in the internal dam (
Figure 4b,c). Due to the internal dam instability and the too small span of the sluice, equal to 3 m, the sluice was totally opened almost all the year. The problems with the internal dam increased in 2014, when huge flows in the Powa river occurred (
Figure 4b) after heavy rainfalls in the upper watershed, which caused local flooding.
High water stages and a fast-flowing flood wave reached the reservoir and met the internal dam. Due to the huge force acting on the dam during the flood wave propagation in 2014, the structure was damaged (
Figure 3b).
In 2014, it was decided that the internal dam would be reconstructed and the sediments would be removed from the upper part of the reservoir. The construction works were carried out in 2015. In the upper part the layer of sediments from the reservoir bottom was removed. The depth of the layer was 40 cm. The area of removal covered about 2 ha and the estimated volume of the removed sediments was 8000 m3. The soil removed was distributed more or less uniformly along the banks. The sluice gate was reconstructed in such a way that inverted elevation was decreased.
4. Results and Discussion
On the basis of 2D simulations, the depths, flow velocities, shear stresses, and stream power were determined for the entire reservoir with each of the three different bathymetries. Due to the huge amount of data, the results were kept in three groups: maps, graphs of selected cross-sections, and graphs with statistical analyses. In
Figure 8, the locations of the main elements applied to analyze the results is shown. The reservoir was divided into five parts as presented in
Figure 8a. They were, (1) the main reservoir 1, (2) the main reservoir 2, (3) pre-reservoir, (4) area under bridge, and (5) the internal dam. The four cross-sections were located in characteristic places of the greatest parts. There was 241,699 computational cells in the numerical grid. Because this number of cells was difficult for processing, the sample points (
Figure 8b) were used for monitoring the obtained results. The total number of sample points was 1670, which was about 0.67% of the available locations of computed values. Although this number was much smaller than the number of computational cells, the sample points covered the entire reservoir with sufficient density. Because the reservoir parts differed in size, their distribution over the parts was not uniform. In the main reservoir 1, the main reservoir 2, and pre-reservoir there were 500 points, while 20 points were generated in the area under the bridge and 150 points generated over the internal dam.
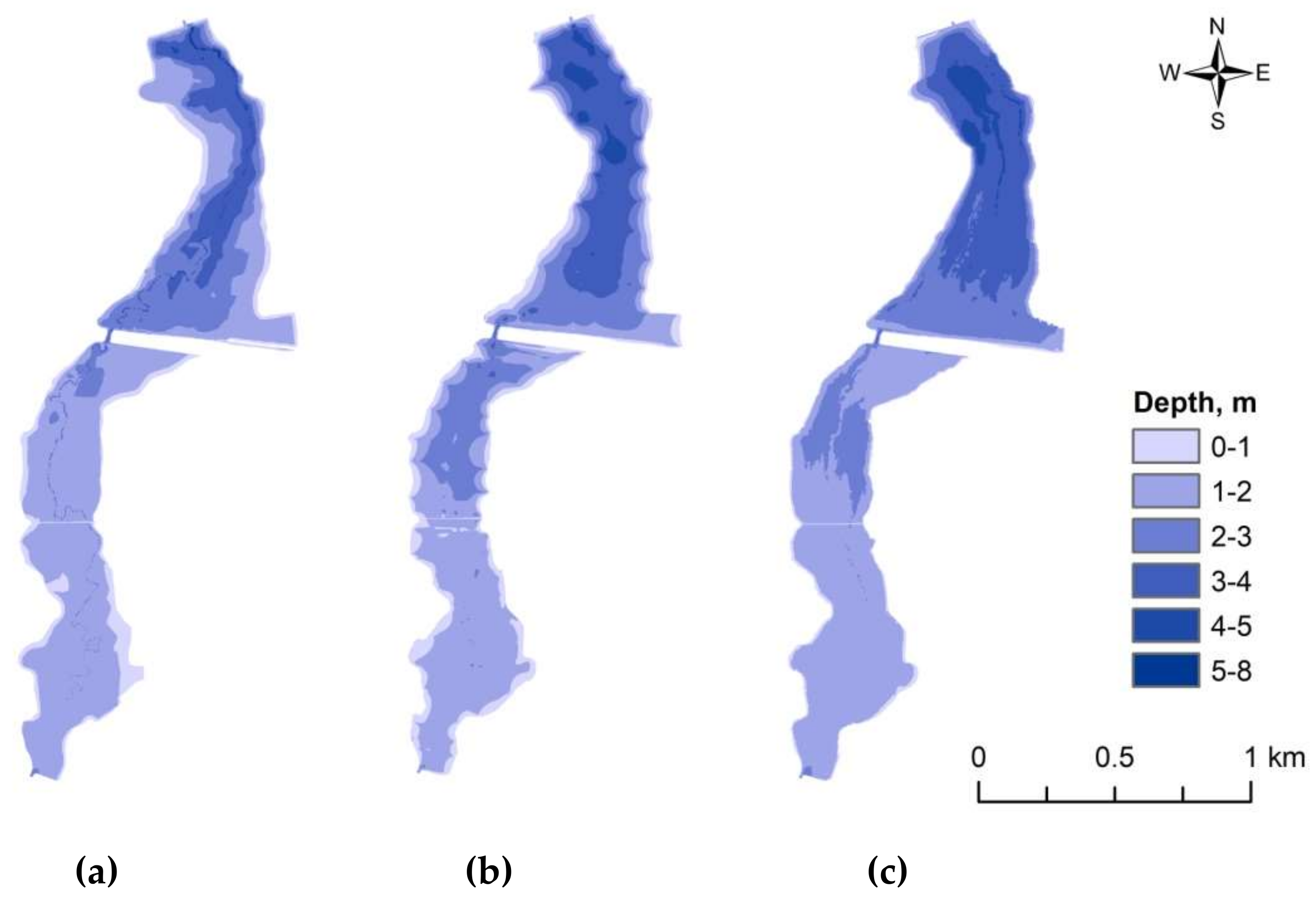
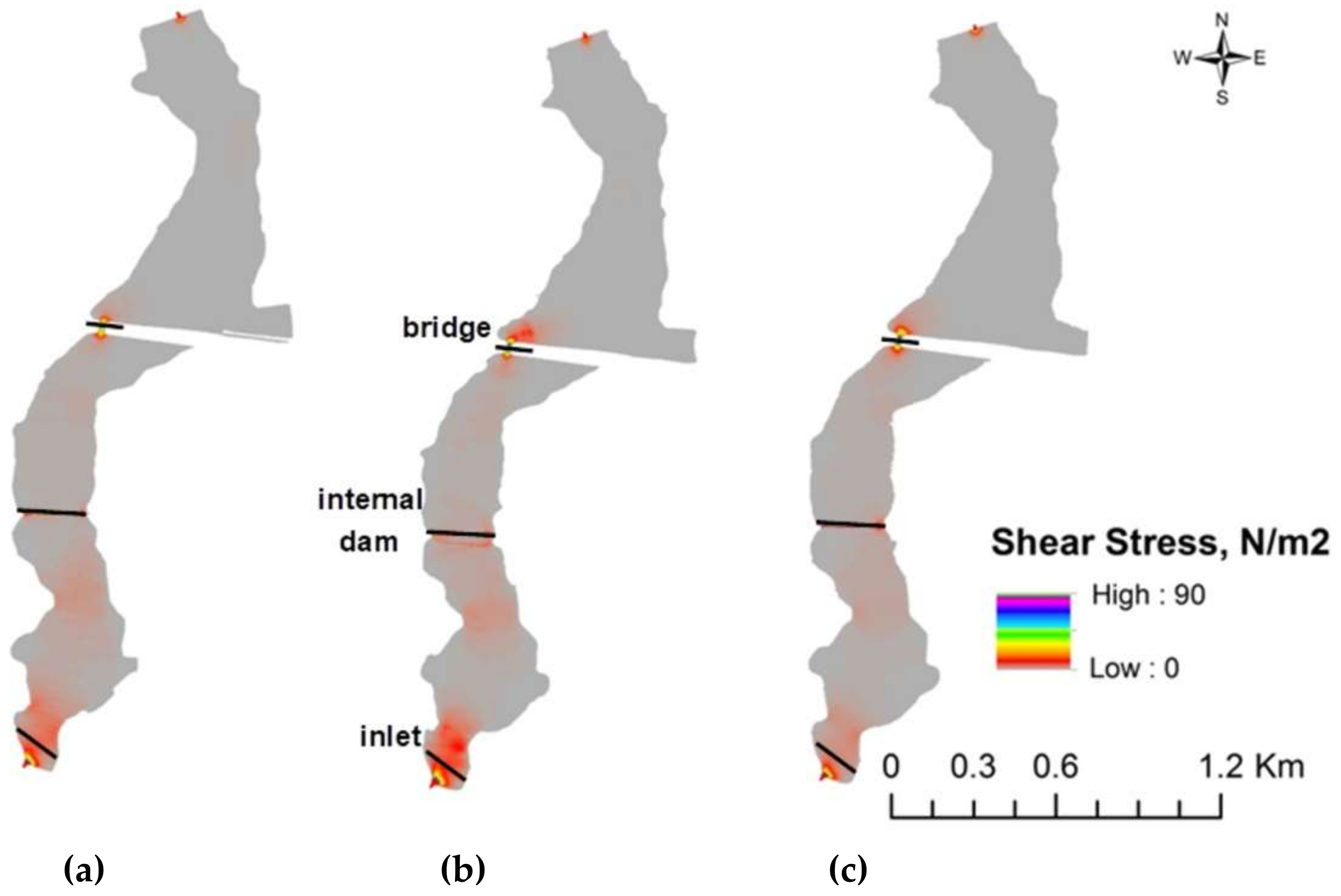
The maps presented in
Figure 9, Figure 11, and Figure 12 show the spatial distribution of depth, velocity, and shear stress over the three reservoir bathymetries, namely 2006, 2013, and 2018. As may be observed, the reservoir bottom changed during 12 years.
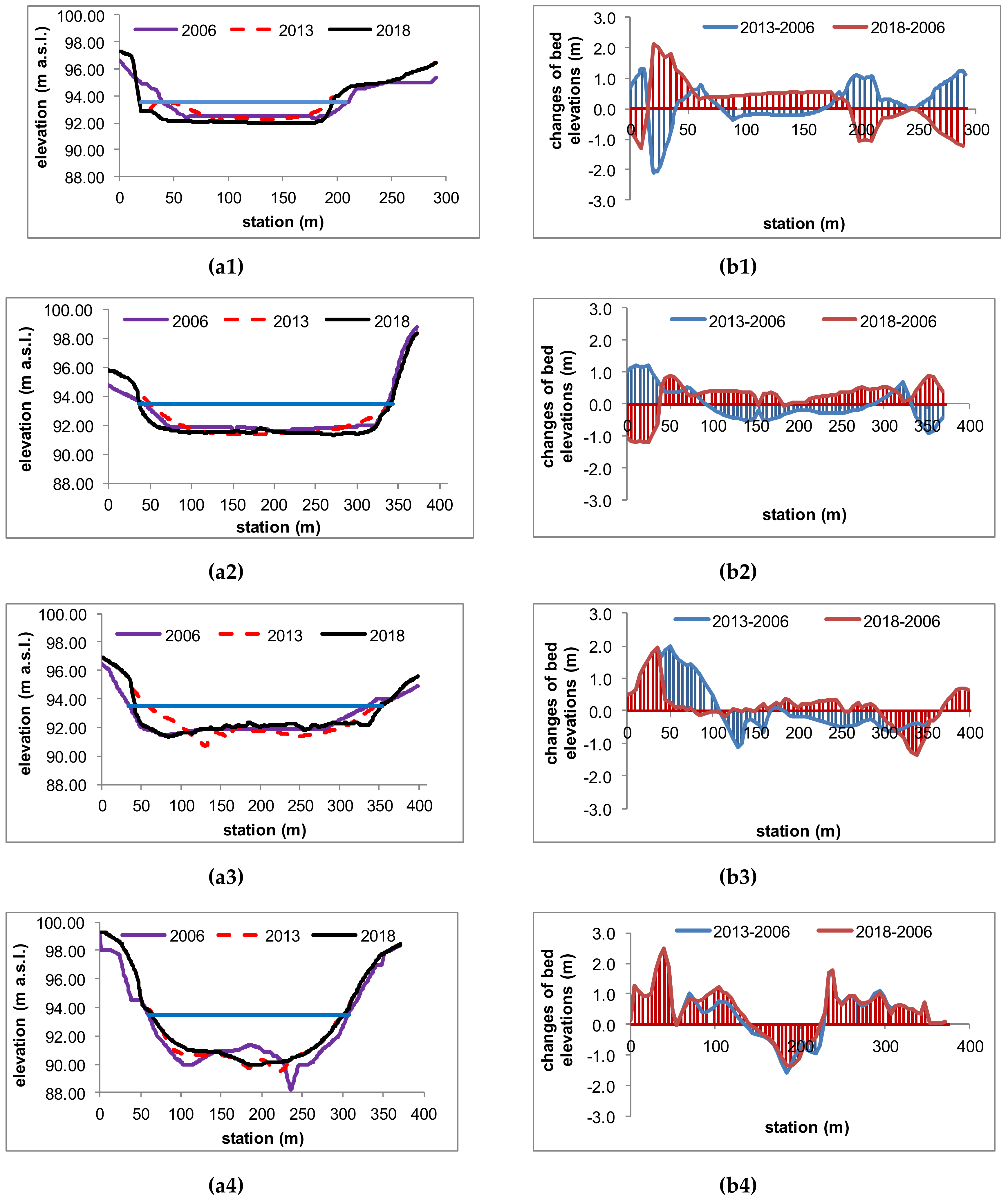
Figure 9 presents the maps of maximum depths obtained as the results of simulations. The following maps (
Figure 9a–c) show the results for bathymetries of 2006, 2013, and 2018, respectively. The comparison of bathymetries is made in
Figure 10—part A (
Figure 10(a1–a4)), where the geometry of cross-sections marked in
Figure 8 is shown. In part B of
Figure 10 (
Figure 10(b1–b4)), the differences of the bottom elevations related to the initial bathymetry 2006 were analyzed. The blue line denotes increase in the elevations, from the initial bathymetry to the bathymetry measurement in 2013. The red lines represent differences between measurements of 2018 and the initial data.
As it can be seen, during the life of the reservoir, since 2006, the reservoir bottom was considerably transformed.
Figure 10, graph b1 shows the cross-section located in the inlet of the Stare Miasto reservoir. The differences in the bottom elevations varied from 2 m of accumulated sediments to 2 m of removed sediments. The comparison with the bathymetry of 2006 shows that the greatest differences occurred in 2018 as a result of natural processes, as well as artificial removal of sediments in the end of 2014 and the beginning of 2015. At this time, a 0.4-m layer of sediments was removed from the bottom of the upper part.
Figure 10(b2,b3) show similar bottom increases for the bathymetries of 2018 and 2006. In the last cross-section located near the main dam, the sediments were accumulated during 12 years of reservoir life. The only place where erosion occurred was located near the valve tower and spillway.
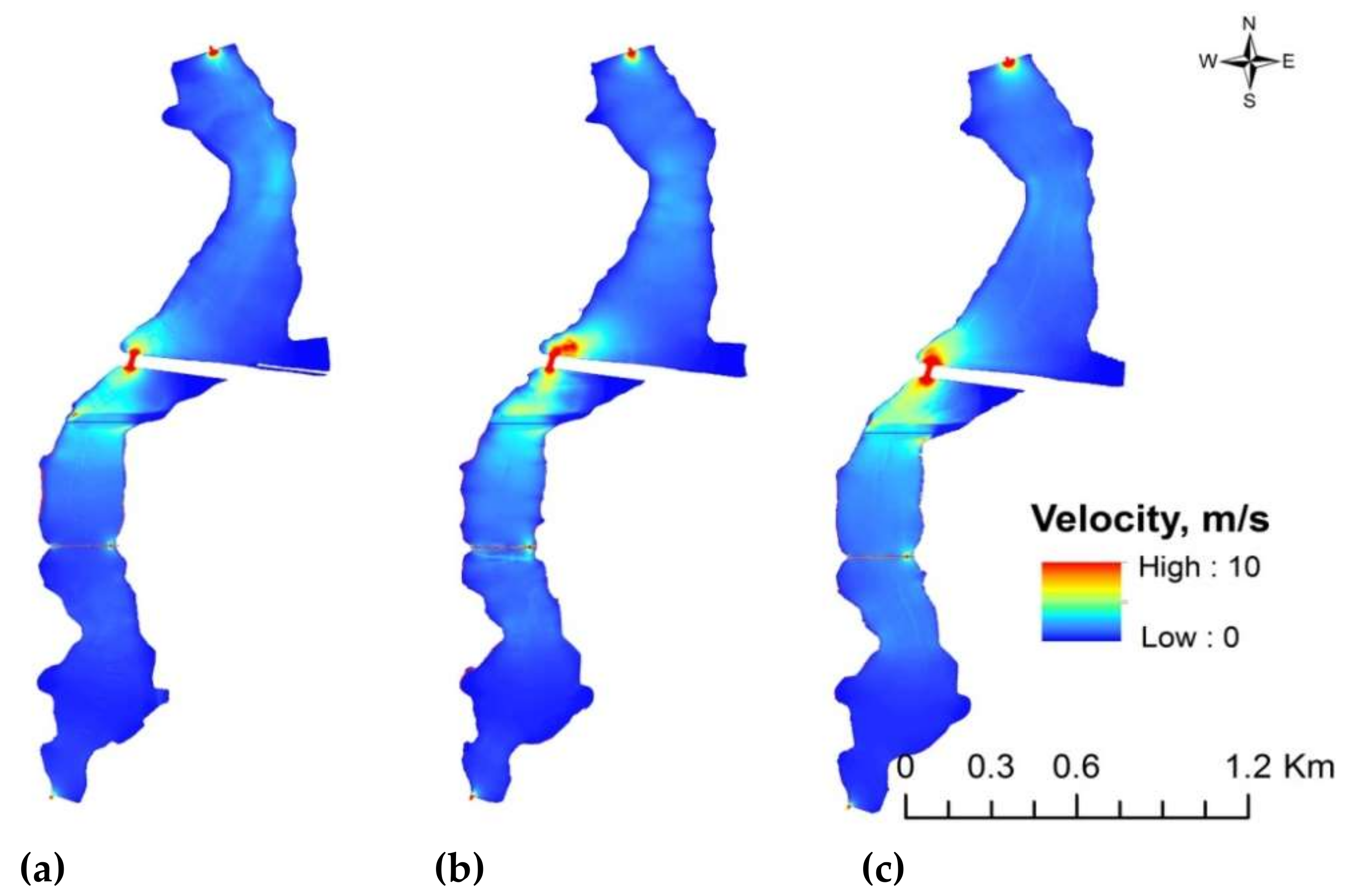
The graphs presented in
Figure 11 illustrate the spatial distribution of maximum magnitudes of velocity in the reservoir. According to the maps, the maximum velocities occurred near structures such as the internal dam, the sluice in the internal dam, the flow below the bridge, and the reservoir outflow. There were also greater velocities in the reservoir inlet, though the area is so small that it was not visible well in the scale of
Figure 11. The internal dam separates the preliminary part of the reservoir from its main part. The single and very small sluice caused local increase in the velocity magnitude and erosion of the alluvial bed. The maximum velocities over 3 m/s occurred also below the bridge. The reason was the too narrow bridge span.
The results presented ealier were confirmed by the results shown in
Figure 12a–c, illustrating that the distribution of the shear stresses in the reservoir was similar to that of velocity. The maximum values occurred at the reservoir inlet, near the internal dam, and in the bridge span. Additionally, the reservoir inlet was more prone to shear stress increase. The maximum shear stresses occurred for the bathymetry of 2013 (
Figure 12b). In the bathymetry of 2018, the corresponding values were lower, especially, in the pre-reservoir. Supposedly, the artificial change in the bottom configuration as a result of dredging in 2014/2015 was the reason for such a change. Additionally, the very specific cross-sections marked in
Figure 12 are cross-sections near the inlet, in the internal dam, and under the bridge. They were applied for analyses presented in
Figure 13.
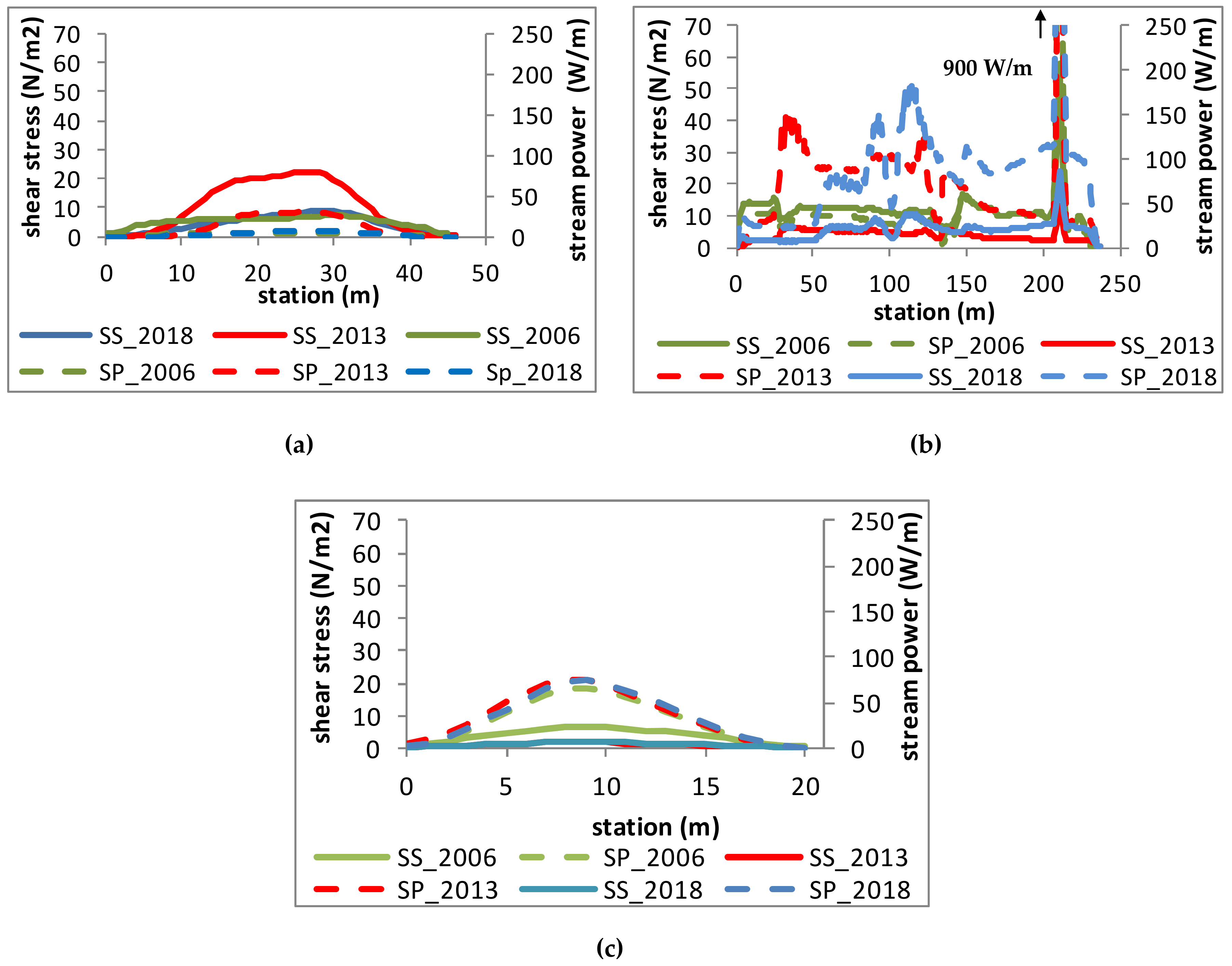
The next variable analyzed was the stream power [
41,
42,
43], but it is shown only in the graphs created with one of two methods: (1) as cross-sections with results extracted from results in raster format (
Figure 8a and
Figure 12) (2) as statistics of values in the random sample points (
Figure 8b). In
Figure 13a–c, the graphs of shear stresses (SS) and stream power (SP) in selected cross-sections are shown for comparison. The cross-sections applied were those shown in
Figure 12. In
Figure 13a, the inlet cross-section is presented. The shear stresses (SS) simulated there were over 20 N/m
2, while the stream power (SP) values reached about 100 W/m. The highest values of shear stress as well as stream power were observed for the reservoir in 2013. Supposedly, the accumulation of sediments in the period 2006–2013 changed the configuration of the bottom in such a way that there was an increase in these two factors. The values of shear stress and stream power were lower for the conditions in 2018. The removal of sediments in the pre-reservoir in 2014/2015 induced another kind of change. The next specific cross-section is shown in
Figure 13b, at the internal dam with its sluice gate. The shear stresses as well as stream power in the sluice were increasing rapidly, independently of the bottom configuration tested. However, the sluice gate is made of concrete and it should be resistant to such forces. The huge values of the analyzed variable are more dangerous for the rest of the internal dam. The maximum values of shear stress were about 40 N/m
2 there, while the stream power reached about 200 W/m. The values of these two factors were relatively small for the conditions in 2006, but in other configurations of the bottom, 2013 and 2018, high shear stresses and stream power were noticed. These values were quite high, especially in the lowland reservoirs where fine sand is deposited with grain sizes smaller than 0.5 mm. It means that, irrespective of the dredging and rebuild of the sluice gate, the internal dam is still exposed to huge forces and is prone to break. In the cross-section of the bridge, the shear stresses were smaller, about 7 N/m
2 and seem to be stable. The same was noticed about the stream power values obtained there. The hydraulic conditions under the bridge seemed to be less dependent on the bottom configuration.
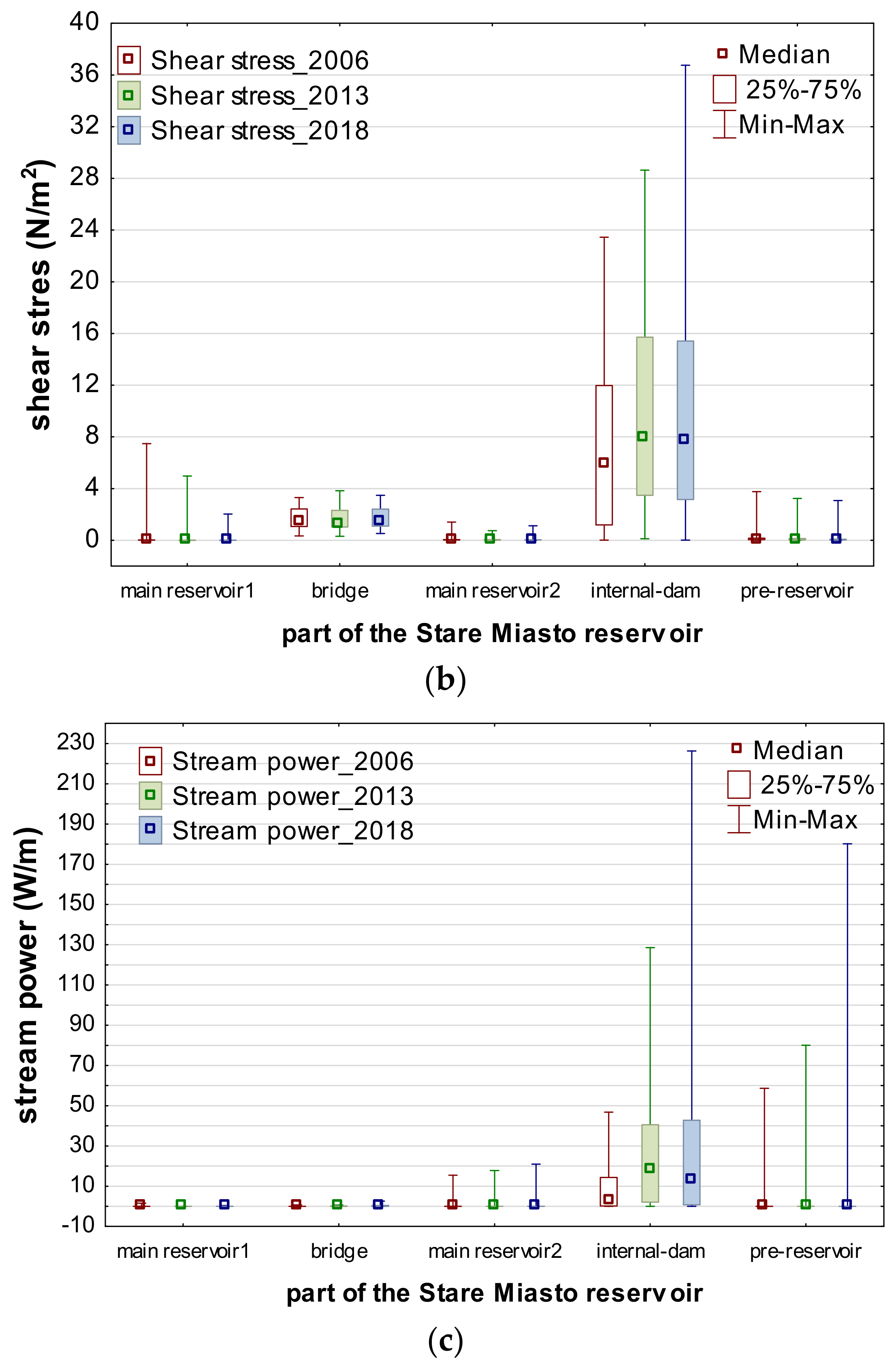
The next stage of the research was statistical analysis performed with Statistica 13. The values of maximum magnitudes of velocity, maximum shear stresses, and maximum stream power were read in the locations of the randomly generated points shown in
Figure 8b, and then processed over the set of points and subsets included in particular reservoir parts (
Figure 8a). Such results are presented as standard box-and-whisker plots in
Figure 14a–c. The graph in
Figure 14a shows the maximum flow velocities for different parts of the reservoir indicated in
Figure 8a. The total medians were 0.32, 0.41, and 0.44 for bathymetries 2006, 2013, and 2018, respectively, while the mean values were 0.53, 0.67, 0.73 m/s. The significant differences between median and mean values for all bathymetries were caused by greater velocities calculated for the bridge and the internal dam. In the first case, the medians were 4.69, 4.81, and 6.38 m/s for the same bathymetries as previously. In the internal dam these factors reach values 2.33, 3.65, and 4.11 m/s. Such great irregularities indicate the importance of these two locations. The greatest values for all bathymetries were noticed under the bridge and over the internal dam. The velocities simulated with the initial bathymetry were slightly smaller than the others. The similarity of the internal dam median velocities for 2013 and 2018 shows that the dredging in 2014/2015 did not improve the safety of this dam much. Fortunately, the maximum velocities decreased from about 10 m/s in 2013 to 6–7 m/s in 2018. In the other parts of the reservoir, the velocities obtained were much smaller irrespective of the bathymetry tested.
The trends observed in the spatial distributions of shear stresses (
Figure 14b) and stream power (
Figure 14c) were different. Definitely the highest values of these two variables were observed in the internal dam. The discrepancy of the velocity magnitudes with shear stress and stream power observed under the bridge may be explained by relatively great narrowing of the flow area but small hydraulic slope governed by the head in the main dam. The internal dam influenced from the upstream and downstream was in different conditions. Hence, the shear stress and stream power confirm the observations of velocities.
It is also important that there is a difference in trend between the median and maximum values. The median values of the shear stress as well as stream power more or less decreased between 2013 and 2018. The simulation over the bottom for 2013 provided median shear stress equal to 7.95 N/m2 and median stream power of 27.97 W/m over the internal dam. The median results obtained for bathymetry 2018 were 7.77 N/m2 and 19.27 W/m, respectively. Hence, the decreases were 2.2% and 31.1% for shear stress and stream power, respectively. The same results indicate significant increases of the maximum values in the case of these two factors. The maximum shear stress and stream power values for bottom for 2013 were 28.63 N/m2 and 653.78 W/m, respectively. The results for the bathymetry 2018 gave maximum values of the same variables equaling 36.75 N/m2 and 815.12 W/m. Hence, the increase of shear stress was 28.4% and the increase of the stream power was 24.7%. It confirms the previous conclusion that the rebuild of the internal dam and sluice gate did not improve the safety of this object.
The analysis of bed changes and shear stress or stream power in reservoirs is relatively difficult for modeling. In many papers there is a description of the shear stresses acting on the river bed in mountainous as well as lowland conditions [
30,
44,
45]. The majority of such concepts is based on the simulation in 1D or calculated with empirical formulae [
30,
45]. In results provided by Glock et al. [
30], who simulated flow in Austrian rivers and a laboratory flume, shear stresses varied from 0.4 to 93 N/m
2. The course of the channel, meandering or straight, influenced the obtained values greatly. The results were also dependent on the type of model applied. They compared results of 1D, 2D, and 3D models. It is obvious that shear stresses are impacted by the roughness of the channel bed and flow velocities. In the Stare Miasto reservoir maximum velocities up to 10 m/s (
Figure 14a) generated huge shear stresses, reaching values of 90 N/m
2 (
Figure 13b). Such magnitudes were noted in the internal dam and near the sluice installed there. Huge velocities and shear stresses caused huge values of stream power. In the sluice it was 900 W/m (
Figure 13b). During the flood wave propagation in the reservoir, the most critical location was the reservoir inlet. The velocities simulated there equaled 5 m/s (
Figure 11), which was related to huge shear stresses reaching values of 25 N/m
2 (
Figure 13a).
5. Conclusions
The impact of hydraulic parameters, such as velocity, shear stress, and stream power, on the operational conditions in a two-stage reservoir was analyzed. The basis for the investigations was simulations assuming the 2D hydrodynamic model. Such an approach enabled better illustration of the processes and changes in the reservoir for different moments of time. The method applied was different from the 1D models usually applied, as it enabled better recognition of the advantages and drawbacks of two-stage constructions. The shear stresses were calculated for the entire reservoir basin and especially for the structures located in it, and visualization revealed significant differences in response to impacts such as flood wave propagation. The simulations were made for the bathymetries of 2006, 2013, and 2018. The conclusions drawn from the results indicated really great stresses exerted on the internal dam, which may lead to breakage of the dam structure. In general, the analyses proved that structures in the reservoir are prone to profound stresses. The magnitudes of shear stresses in specific flood conditions are not observed frequently in lowland rivers and reservoirs. The contraction caused by the bridge span is also very dangerous. In flooding conditions, it may cause great acceleration of water flow. The highway bridge is a massive construction and no direct threat related to great stress has been detected in this case. However, it is not possible to exclude the probability of erosion below the bridge and collapse of the construction due to bridge scour in future. One thing is obvious—the velocities, the shear stress, and stream power values are not typical of lowland conditions if the flood propagates through the reservoir. The presented analyses confirm that two-stage reservoirs are a good solution of some reservoir problems, if the inflows are moderate. However, the increase in inflows up to flood magnitudes may generate new problems related to the stability of the structures located in the reservoir basin.
The applied 2D hydrodynamic modeling enabled more detailed analysis focused on spatial distributions of important factors: the velocity, the shear stress, and the stream power. The obtained results are satisfactory and they may be applied in several ways. The approach presented enabled identification of potential threats, which is well shown on the example of the internal dam. However, such an approach required greater computational costs than in other cases—more simulation time, faster processors, more effective models, more memory on disks, etc. Although, the use of three different bathymetries and comparisons between them increased the complexity of the problem, it also enabled simplified verification of the object vulnerability to flood hazard. As indicated, the rebuild of the internal dam did not improve the safety of this structure and future breakage could be expected. Some application areas of the results presented seem to be obvious—improvement of reservoir design, optimization of structure shapes, invention of protective elements reducing detected threats. Others are not so obvious (e.g., operational control of reservoir to safely propagate the flood wave). However, such an approach requires greater computational power than that applied in this research.
As can be seen, the two-stage reservoirs are quite an interesting alternative approach to prevention of reservoir sedimentation. However, their construction should be carefully analyzed and computationally tested before the main dam is built. The results of the present research could help in the design of crucial elements, such as the sluice in the internal dam, the span of the bridge, etc. All such elements could cause unexpected effects dangerous for the stability of the reservoir construction and safety of the water management in the reservoir.