Fixed-Bed and Mobile-Bed Resistance of Channels with Steep Gradients in Mountainous Areas
Abstract
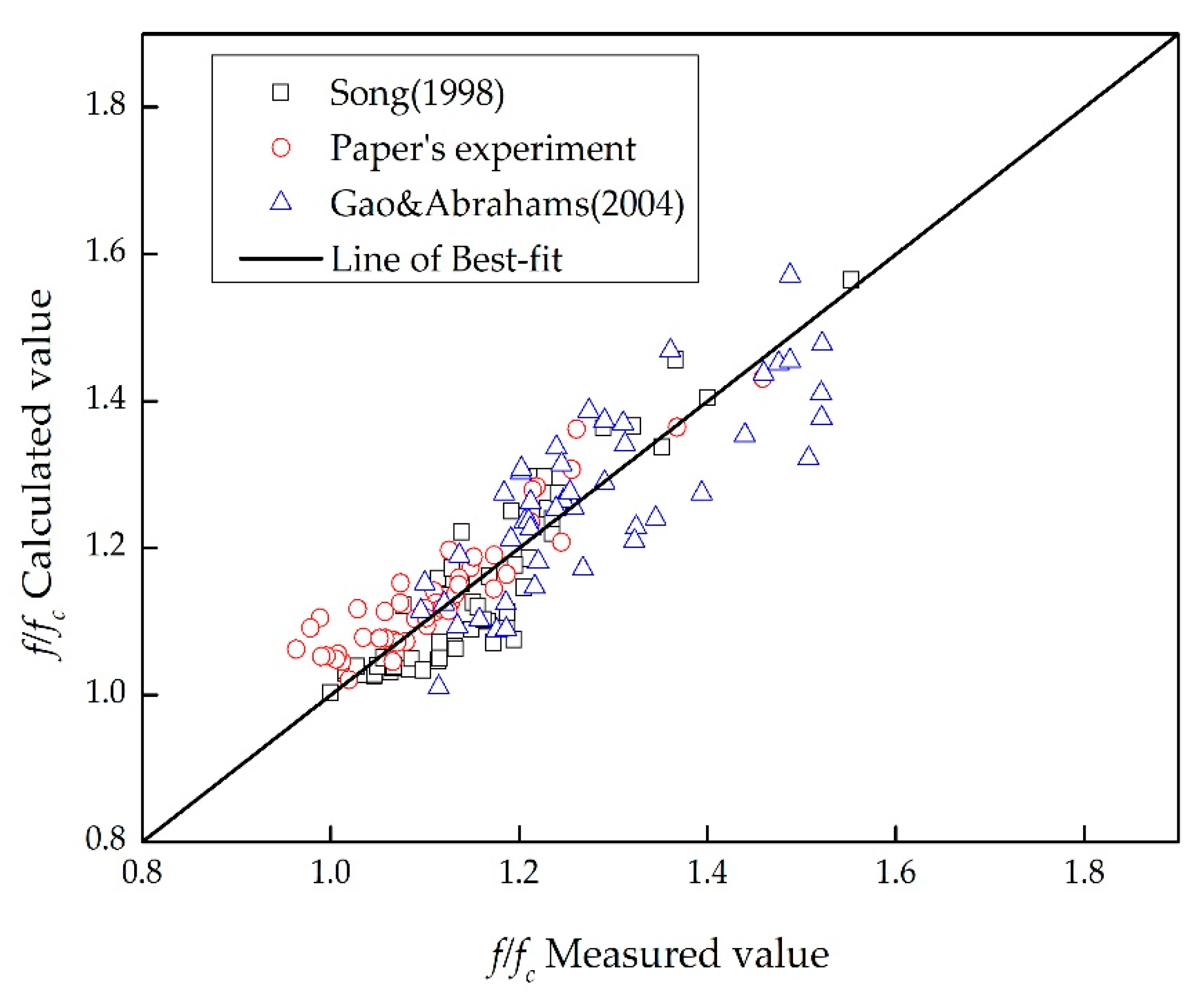
:1. Summary
2. Experiment Setup
3. Data Description and Method
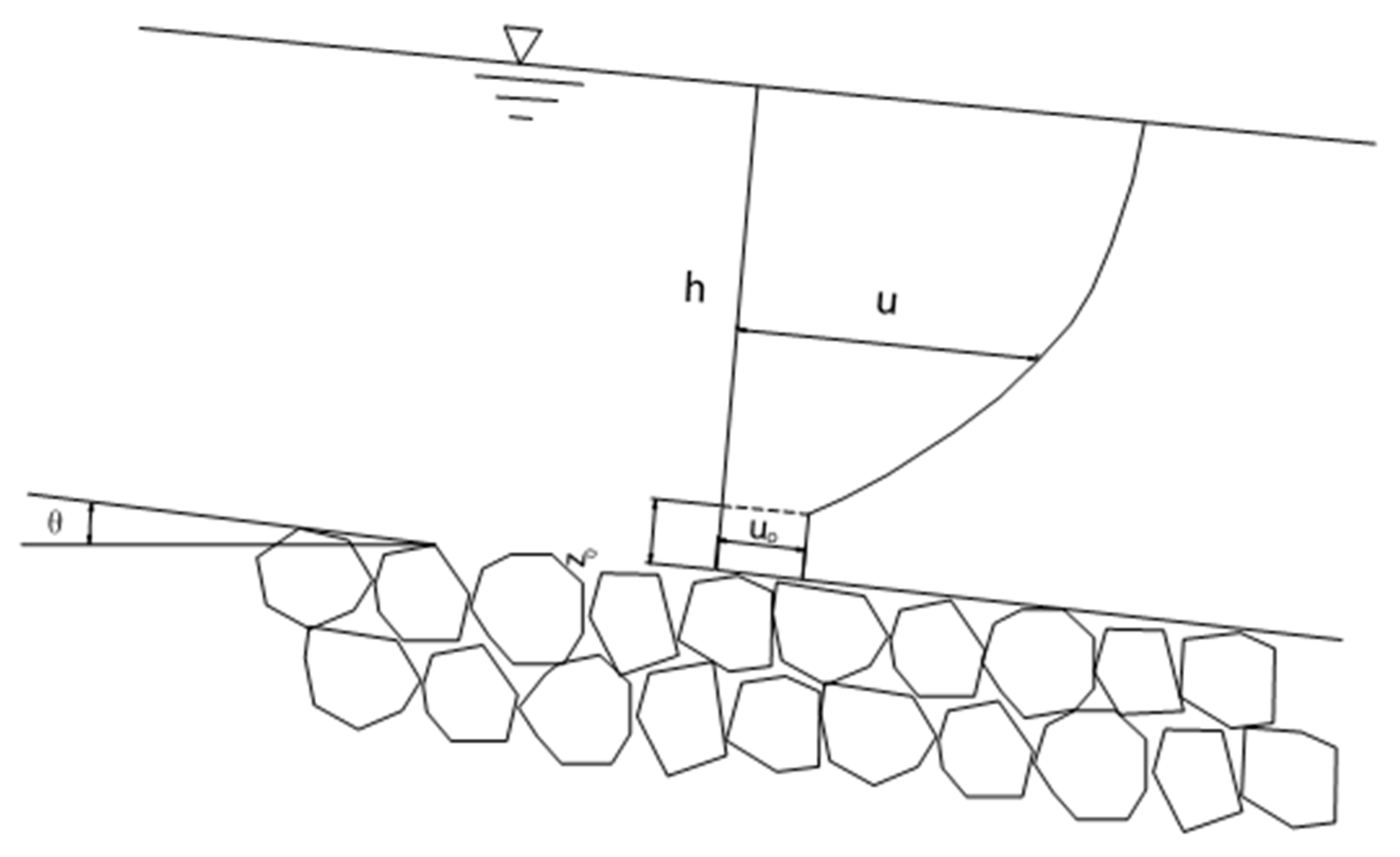
3.1. Experimental Study on the Fixed Bed with Uniform Sediment Resistance of Steep Gradient Channnel
- ; Rough region
- ; Smooth region
- ; Transition region
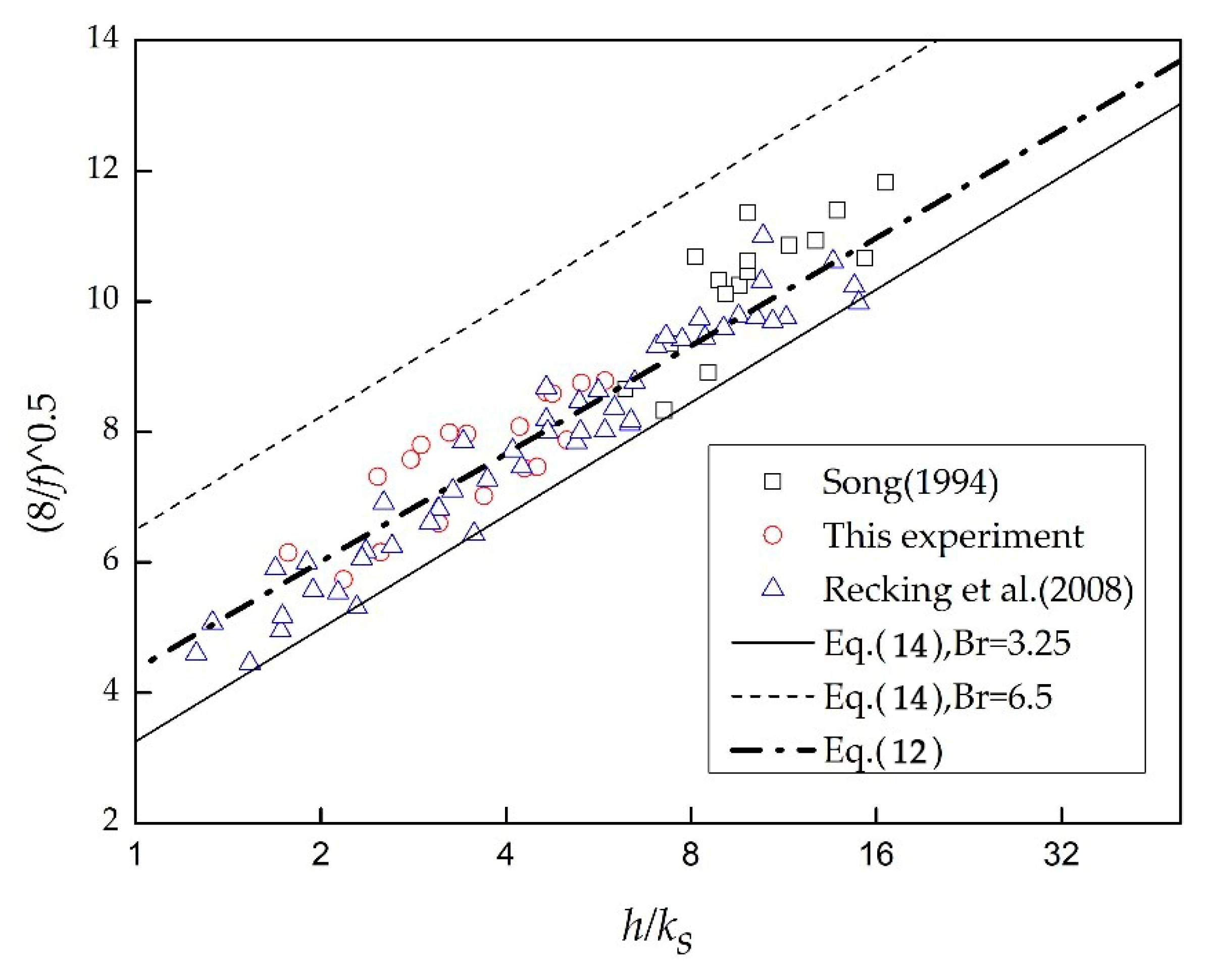
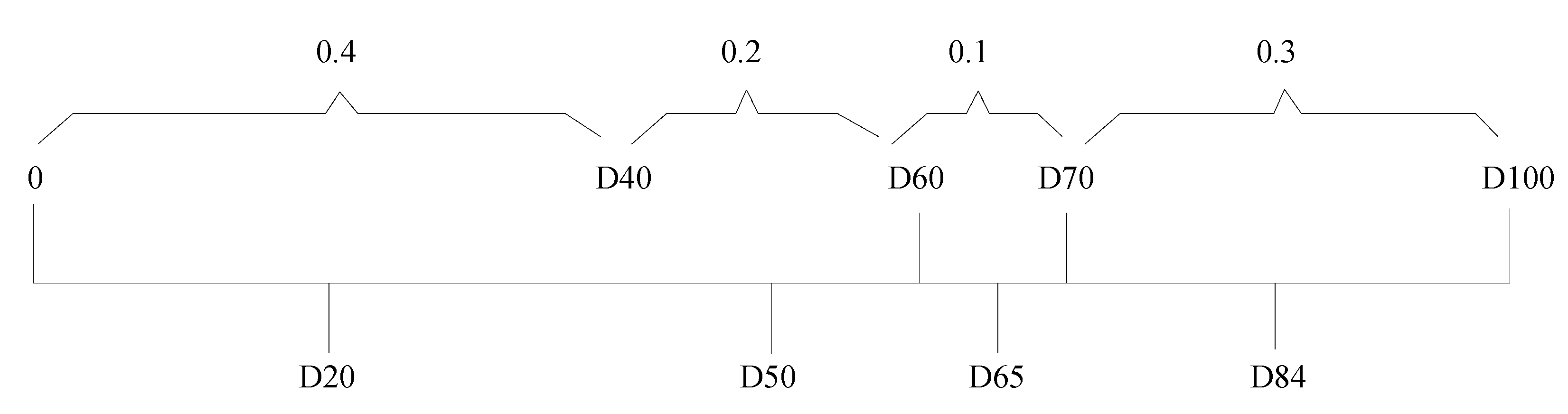
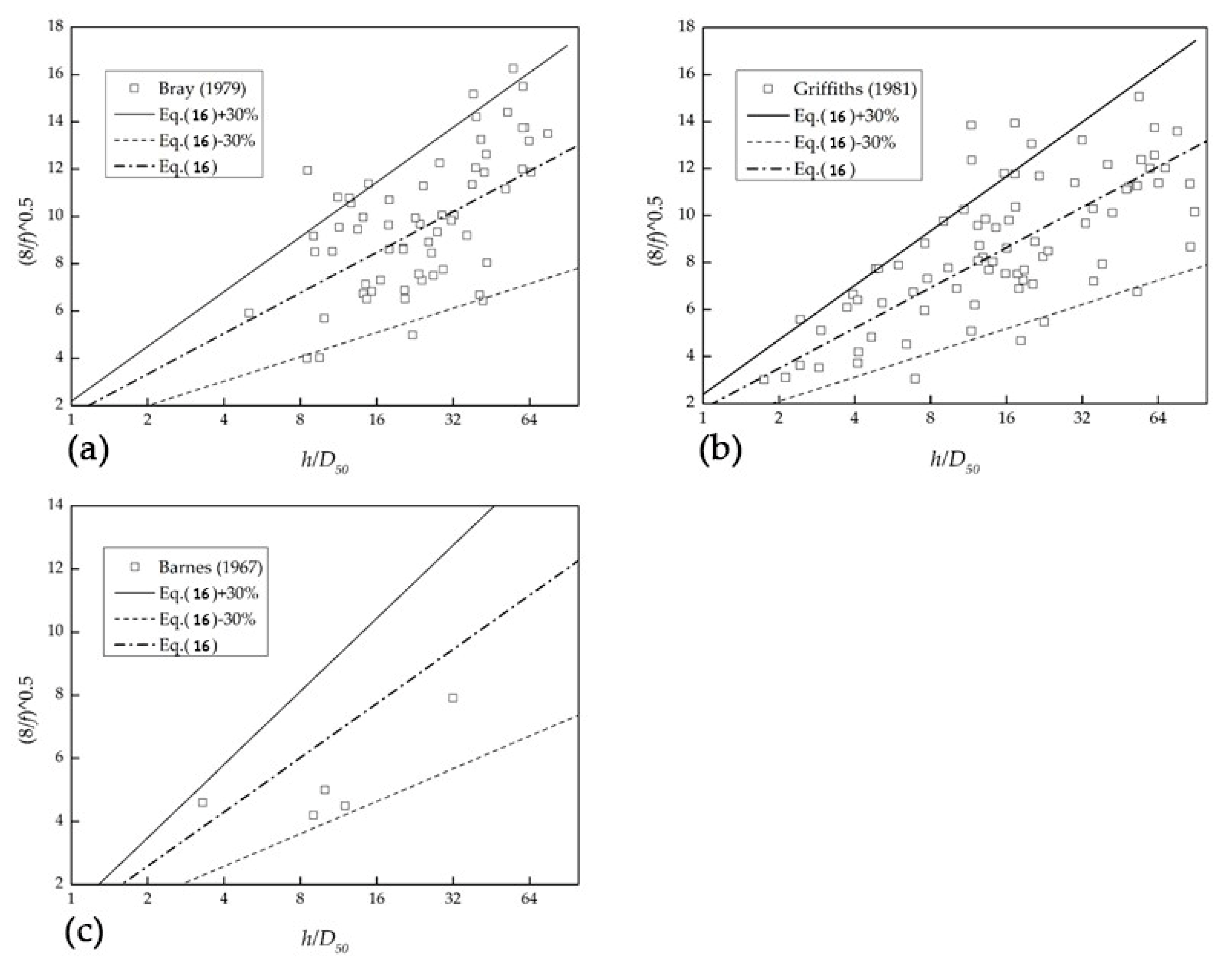
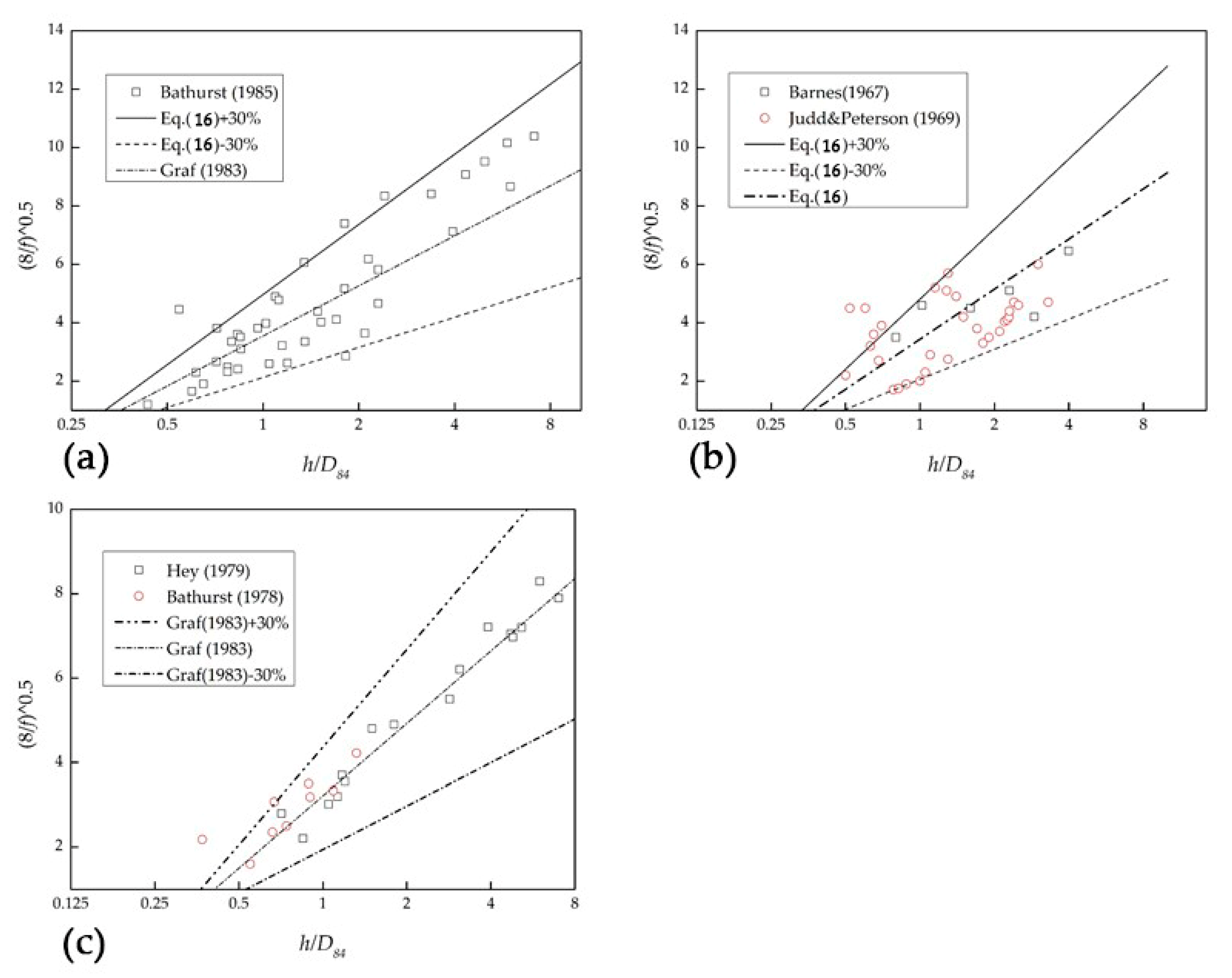
3.2. Investigation of the Resistance Characteristics of the Non-Uniform Sediment River Bed
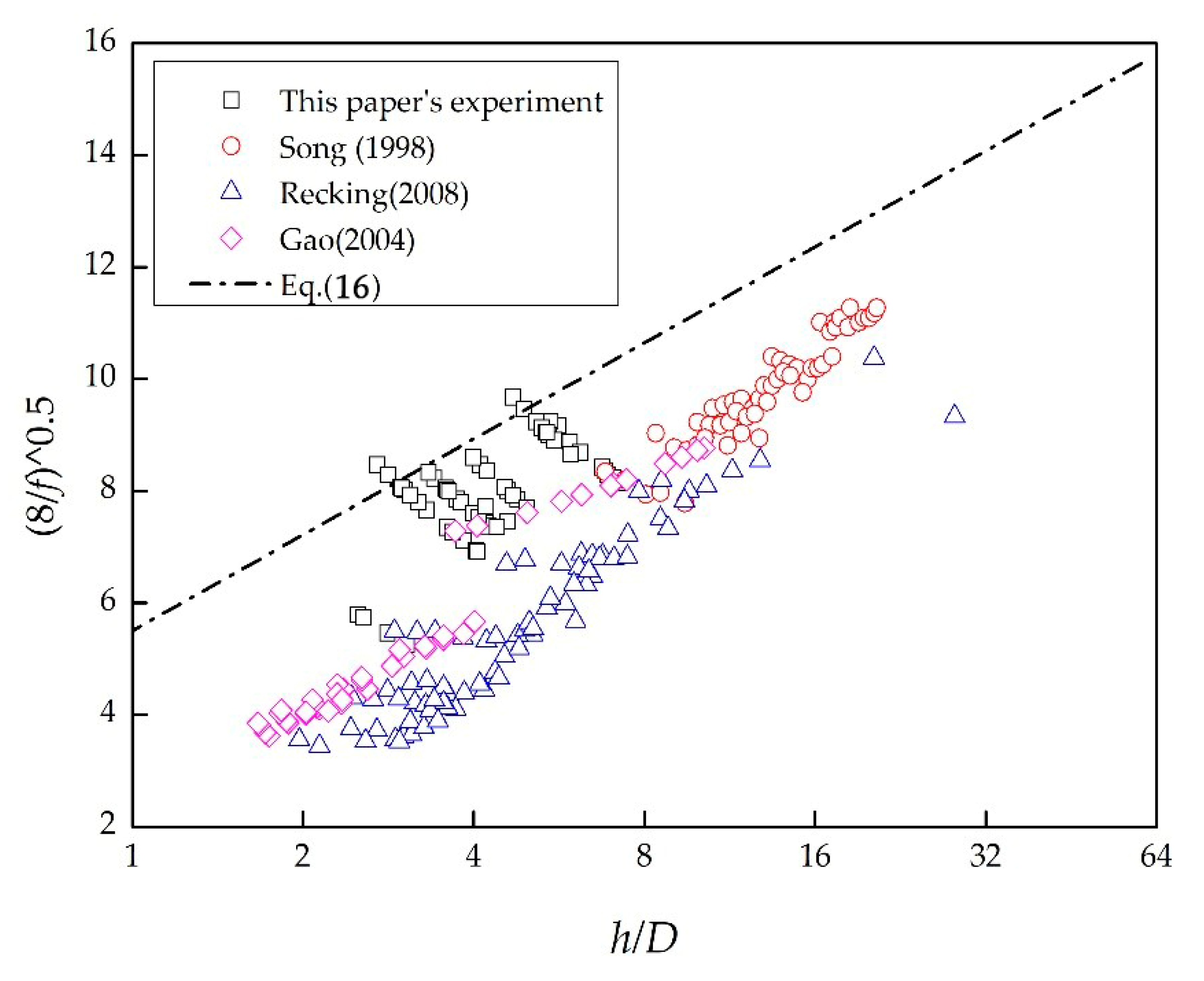
3.3. Law of Sediment Laden Flow Resistance in the Steep Gradient Channels
4. Conclusions
- Based on the existing results, a calculation scheme is established to simulate the velocity distribution of the uniform sediment bed on the steep gradient channels. Based on the test data, the proposed expressions are verified to have a reasonable performance;
- Through the existing research results, an expression is determined to calculate the resistance of the fixed bed with non-uniform sediment. Moreover, the selection of the roughness size is analyzed to put forward a new calculation method. The expression for the resistance of the non-uniform bed surface of the steep gradient channels is obtained through analyzing the measured field data;
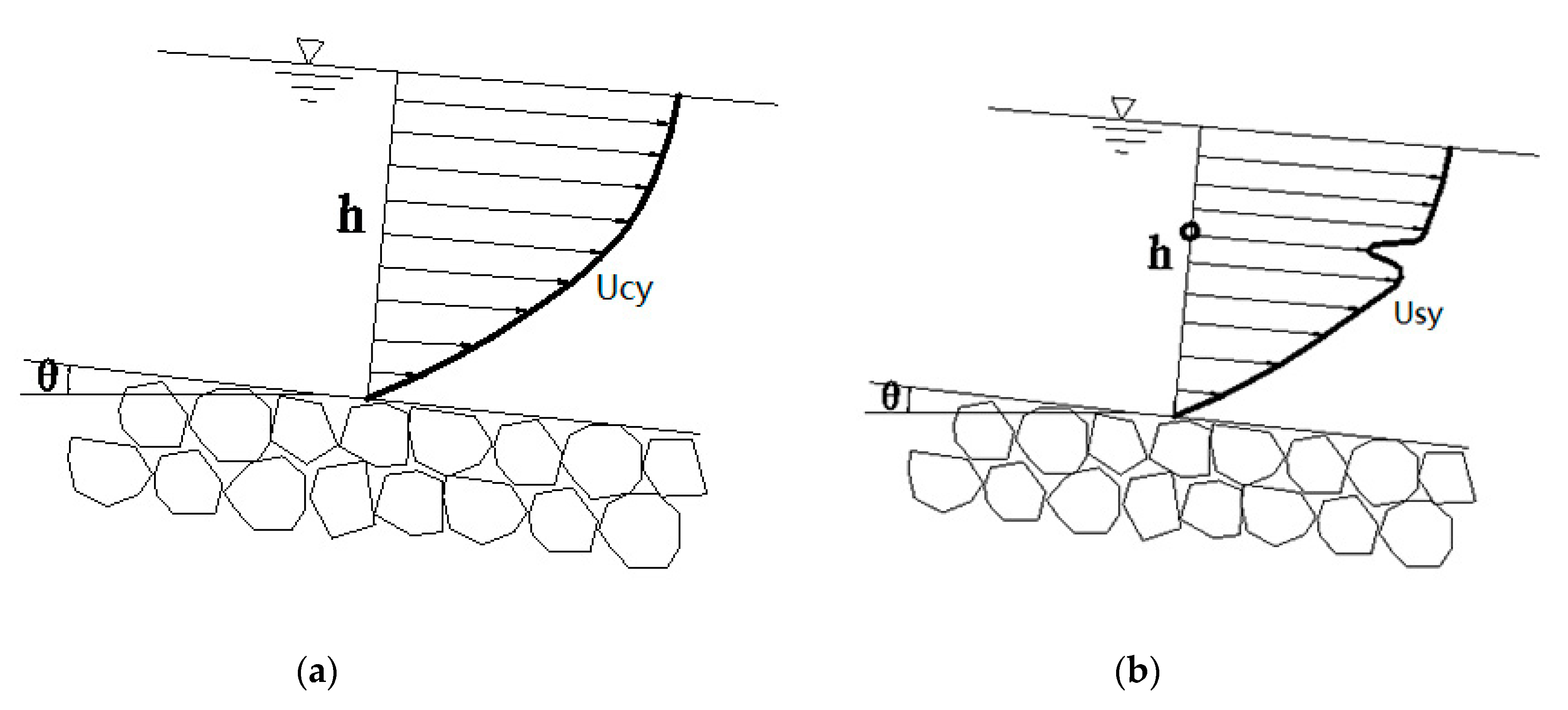
- When calculating the resistance of the mobile bed in mountainous rivers, the fixed bed resistance cannot be used. Experimental results show that, when the bed surface is stable and the bed load particle size is much smaller than that of the gravel, the bed surface is smooth and flat. At this point, there are several possibilities so the resistance may increase, may remain unchanged, or even may decrease;
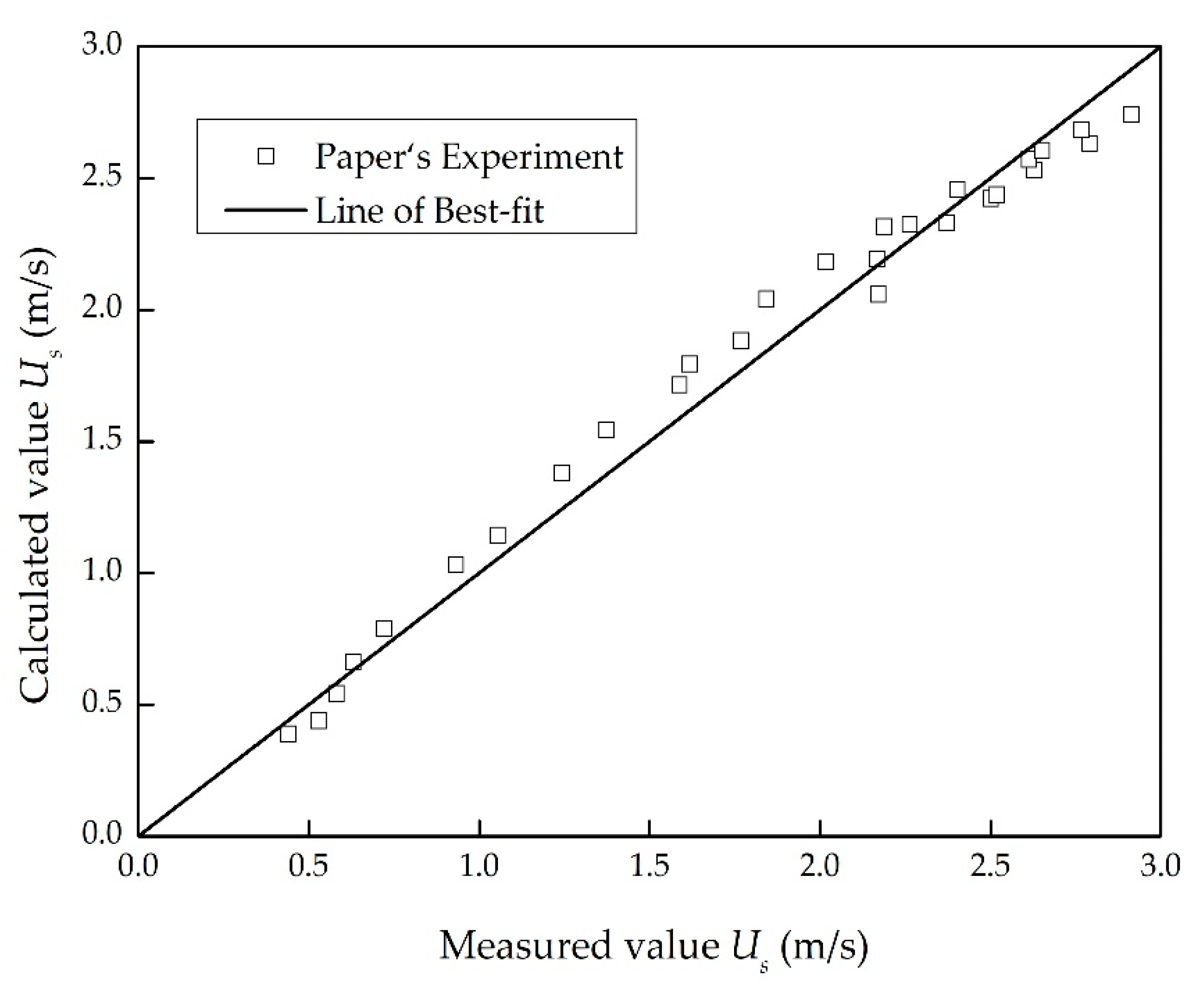
- By assuming the flow velocity distribution of clean water and sediment laden flows, the variation law for the resistance of sediment laden flow is derived. Moreover, the relationship between the velocity of the sediment movement and the average flow velocity is obtained. It is found that the proposed method can accurately predict the variation of the sediment carrying resistance.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Nitsche, M.; Rechenman, D.; Turoswski, J.M.; Badoux, A.; Kirchner, J.W. Evaluation of bedload transport predictions using flow resistance equations to account for macro-roughness instep-pool streams. Water Resour. Res. 2011, 47, W08513. [Google Scholar] [CrossRef]
- Bathurst, J.C. Flow resistance of large-scale roughness. J. Hydraul. Div. 1978, 104, 1587–1603. [Google Scholar]
- Bathurst, J.C. Flow resistance estimation in mountain rivers. J. Hydraul. Eng. 1985, 111, 625–643. [Google Scholar] [CrossRef]
- Maxwell, A.R.; Papanicolaou, A.N. Step-pool morphology in high-gradient streams. Int. J. Sediment Res. 2001, 16, 380–390. [Google Scholar]
- Yu, B.Y. Flow resistance of large-scale roughness in open channel. In Proceedings of the International Symposium on Hydraulic Research in Nature and Laboratory, Wuhan, China, 17–20 November 1992. [Google Scholar]
- Graf, W.H.; Altinakar, M. Hydraulique Fluviale; tome 1; Presses Polytechniques et Universitaires Romandes: Lausanne, Switzerland, 1993. [Google Scholar]
- Bathurst, J.C.; Thorne, C.R.; Hey, R.D. Secondary flow and shear stress at river bends. J. Hydraul. Div. 1979, 105, 1277–1295. [Google Scholar]
- Hey, R.D. Flow resistance in gravel-bed rivers. J. Hydraul. Div. 1979, 105, 365–379. [Google Scholar]
- Bathurst, J.C.; Li, R.M.; Simons, D.B. Resistance equation for large scale roughness. J. Hydraul. Div. 1981, 107, 1593–1613. [Google Scholar]
- Rice, C.E.; Kadavy, C.K.; Robinson, K.M. Roughness of loose rock riprap on steep slope. J. Hydraul. Eng. 1998, 124, 179–185. [Google Scholar] [CrossRef]
- Pagliara, S.; Chiavaccini, P. Flow resistance of rock chutes with protruding boulders. J. Hydraul. Eng. 2006, 132, 545–552. [Google Scholar] [CrossRef]
- Griffith, G.A. Flow resistance in coarse gravel bed rivers. J. Hydraul. Div. 1981, 107, 899–918. [Google Scholar]
- Aguirre-Pe, J.; Fuentes, R. Resistance to flow in steep rough streams. J. Hydraul. Eng. 1990, 116, 1374–1387. [Google Scholar] [CrossRef]
- Song, T.; Chiew, Y.M.; Chin, C.O. Effect of bed-load movement on flow friction factor. J. Hydraul. Eng. 1998, 124, 165–175. [Google Scholar] [CrossRef]
- Recking, A.; Frey, P.; Paquier, A.; Belleudy, P.; Champagne, J.Y. Feedback between bed load and flow resistance in gravel and cobble bed rivers. Water Resour. Res. 2008, 44, W05412. [Google Scholar]
- Ferguson, R. Flow resistance equations for gravel- and boulder-bed streams. Water Resour. Res. 2007, 43, W05427. [Google Scholar] [CrossRef]
- Aberle, J.; Smart, G.M. The influence of roughness structure on flow resistance on steep slopes. J. Hydraul. Res. 2003, 41, 259–269. [Google Scholar] [CrossRef]
- Gao, P.; Abrahams, A.D. Bedload transport resistance in rough open-channel flows. Earth Surf. Process. Landf. 2004, 29, 423–435. [Google Scholar] [CrossRef]
- Cassan, L.; Roux, H.; Garambois, P.A. A semi-analytical model for the hydraulic resistance due to macro-roughnesses of varying shapes and densities. Water 2017, 9, 637. [Google Scholar] [CrossRef]
- Chien, N.; Wan, C.H.; McNown, J.S. Mechanics of Sediment Transport; ASCE: Reston, VA, USA, 1999. [Google Scholar]
- Montes, S. A new theory about bidimensional flow over a rough boundary. In Proceedings of the 3rd Latin America Congress of the IAHR, La Plata, Agentia, 1968; pp. 100–118. (In Spanish). [Google Scholar]
- Bathurst, J.C.; Thorne, C.R.; Hey, R.D. Direct measurements of secondary currents in river bends. Nature 1977, 269, 504–506. [Google Scholar] [CrossRef]
- Thompson, S.M.; Campbell, P.L. Hydraulics of a large channel paved with boulders. J. Hydraul. Res. 1979, 17, 351–354. [Google Scholar] [CrossRef]
- Song, T.; Graf, W.H. Nonuniform open-channel flow over arough bed. J. Hydrosci. Hydr. Eng. 1994, 12, 1–25. [Google Scholar]
- Graf, W.H.; Cao, H.H.; Suszka, L. Hydraulics of steep, mobile bed channels. In Proceedings of the 20th Congress of the IAHR, Moscow, Russia, 5–9 September 1983; pp. 301–305. [Google Scholar]
- Kikkawa, H.; Hiroyoshi, S.; Fukuoka, S. On the effects of suspended sediments to the bed roughness. In Proceedings of the 12th Congress of IAHR, Fort Collins, CO, USA, 11–14 September 1967; pp. 49–56. [Google Scholar]
- Barnes, H.H., Jr. Roughness Characteristics of Natural Channels; U.S. Geological Survey Water Supply Paper 1849: Washington, DC, USA, 1967.
- Judd, H.E.; Peterson, D.F. Hydraulics of Large Bed Elements Channels; Report No. PRW17-6; Utah Water Research Laboratory, Utah State University: Logan, UT, USA, 1969. [Google Scholar]
- Abrahams, A.D.; Li, G. Effect of saltating sediment on flow resistance and bed roughness in overland flow. Earth Surf. Process. Landf. 1998, 23, 953–960. [Google Scholar] [CrossRef]
- Bagnold, R.A. The nature of saltation and of bed-load transport in water. Proc. R. Soc. A Math. Phys. Sci. 1973, 332, 473–504. [Google Scholar] [CrossRef]

| Type | Number of Test | S | D (cm) | h (cm) |
|---|---|---|---|---|
| Song | 16 | 0.5–1.5 | 1.23 | 7.7–20.4 |
| Recking | 62 | 1.0–9.0 | 0.23–0.9 | 1.07–8.33 |
| Paper’s experiment | 18 | 10.0 | 0.92, 1.23 | 1.63–6.18 |
| No. | Discharge Q (m3/s) | Particle Size D (cm) | Gradient Ratio | Water Depth h (cm) | Flow Velocity U (m/s) | R (cm) | R/d | (8/f)0.5 |
|---|---|---|---|---|---|---|---|---|
| 1 | 0.0051 | 0.92 | 0.1 | 1.63 | 0.777 | 1.507 | 1.638 | 6.146 |
| 2 | 0.0100 | 0.92 | 0.1 | 2.28 | 1.093 | 2.047 | 2.225 | 7.310 |
| 3 | 0.0124 | 0.92 | 0.1 | 2.58 | 1.205 | 2.285 | 2.484 | 7.577 |
| 4 | 0.0135 | 0.92 | 0.1 | 2.68 | 1.264 | 2.363 | 2.569 | 7.798 |
| 5 | 0.0163 | 0.92 | 0.1 | 2.98 | 1.365 | 2.594 | 2.819 | 7.985 |
| 6 | 0.0179 | 0.92 | 0.1 | 3.18 | 1.407 | 2.744 | 2.982 | 7.969 |
| 7 | 0.0244 | 0.92 | 0.1 | 3.88 | 1.575 | 3.250 | 3.532 | 8.078 |
| 8 | 0.0302 | 0.92 | 0.1 | 4.28 | 1.762 | 3.526 | 3.832 | 8.604 |
| 9 | 0.0312 | 0.92 | 0.1 | 4.38 | 1.779 | 3.593 | 3.906 | 8.585 |
| 10 | 0.0373 | 0.92 | 0.1 | 4.88 | 1.913 | 3.923 | 4.264 | 8.748 |
| 11 | 0.0428 | 0.92 | 0.1 | 5.33 | 2.006 | 4.208 | 4.574 | 8.779 |
| 12 | 0.0100 | 1.23 | 0.1 | 2.68 | 0.930 | 2.363 | 1.921 | 5.736 |
| 13 | 0.0132 | 1.23 | 0.1 | 3.08 | 1.069 | 2.669 | 2.170 | 6.154 |
| 14 | 0.0196 | 1.23 | 0.1 | 3.83 | 1.278 | 3.214 | 2.613 | 6.595 |
| 15 | 0.0268 | 1.23 | 0.1 | 4.53 | 1.478 | 3.693 | 3.003 | 7.015 |
| 16 | 0.0358 | 1.23 | 0.1 | 5.28 | 1.693 | 4.177 | 3.396 | 7.444 |
| 17 | 0.0384 | 1.23 | 0.1 | 5.53 | 1.736 | 4.332 | 3.522 | 7.459 |
| 18 | 0.0479 | 1.23 | 0.1 | 6.18 | 1.937 | 4.721 | 3.838 | 7.870 |
| Barnes (1967) | 2.35 | Barnes (1967) | 5.64 |
| Judd & Peterson (1969) | 2.34 | Bray (1979) | 4.82 |
| Bathurst (1978) | 2.52 | Griffiths (1978) | 4.51 |
| Hey (1979) | 2.51 | ||
| Bathurst (1985) | 2.25 |
| No. | Q (L/s) | DB (mm) | DT (mm) | hc (cm) | h (cm) | S | U (m/s) | Cv | f | fc | f/fc |
|---|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 17.89 | 9.20 | 3.80 | 3.10 | 3.14 | 0.10 | 1.44 | 0.0091 | 0.1181 | 0.1167 | 1.0123 |
| 2 | 17.89 | 9.20 | 3.80 | 3.10 | 3.07 | 0.10 | 1.44 | 0.0302 | 0.1154 | 0.1167 | 0.9890 |
| 3 | 17.89 | 9.20 | 3.80 | 3.10 | 3.44 | 0.10 | 1.44 | 0.0459 | 0.1295 | 0.1167 | 1.0700 |
| 4 | 17.89 | 9.20 | 3.80 | 3.10 | 3.28 | 0.10 | 1.44 | 0.0337 | 0.1235 | 0.1167 | 1.0581 |
| 5 | 24.45 | 9.20 | 3.80 | 3.75 | 3.78 | 0.10 | 1.63 | 0.0167 | 0.1116 | 0.1107 | 1.0080 |
| 6 | 24.45 | 9.20 | 3.80 | 3.75 | 3.88 | 0.10 | 1.63 | 0.0276 | 0.1145 | 0.1107 | 1.0347 |
| 7 | 24.45 | 9.20 | 3.80 | 3.75 | 3.67 | 0.10 | 1.63 | 0.0343 | 0.1083 | 0.1107 | 0.9789 |
| 8 | 35.76 | 9.20 | 3.80 | 4.49 | 4.33 | 0.10 | 1.99 | 0.0309 | 0.0855 | 0.0888 | 0.9635 |
| 9 | 35.76 | 9.20 | 3.80 | 4.49 | 4.52 | 0.10 | 1.99 | 0.0216 | 0.0893 | 0.0888 | 1.0058 |
| 10 | 42.22 | 9.20 | 3.80 | 5.10 | 5.20 | 0.10 | 2.07 | 0.0073 | 0.0952 | 0.0933 | 1.0196 |
| 11 | 40.03 | 9.20 | 3.80 | 5.00 | 4.98 | 0.10 | 2.00 | 0.0267 | 0.0975 | 0.0979 | 0.9960 |
| 12 | 41.67 | 9.20 | 3.80 | 5.08 | 5.03 | 0.10 | 2.05 | 0.0277 | 0.0938 | 0.0947 | 0.9902 |
| 13 | 12.80 | 9.20 | 3.80 | 2.42 | 2.49 | 0.10 | 1.32 | 0.0258 | 0.1116 | 0.1085 | 1.0289 |
| 14 | 12.80 | 9.20 | 3.80 | 2.42 | 2.60 | 0.10 | 1.32 | 0.0370 | 0.1166 | 0.1085 | 1.0744 |
| 15 | 12.80 | 9.20 | 3.80 | 2.42 | 2.78 | 0.10 | 1.32 | 0.0442 | 0.1246 | 0.1085 | 1.0400 |
| 16 | 17.89 | 9.20 | 6.70 | 3.10 | 3.86 | 0.10 | 1.44 | 0.0331 | 0.1453 | 0.1167 | 1.2452 |
| 17 | 17.89 | 9.20 | 6.70 | 3.10 | 3.49 | 0.10 | 1.44 | 0.0307 | 0.1314 | 0.1167 | 1.1258 |
| 18 | 17.89 | 9.20 | 6.70 | 3.10 | 3.31 | 0.10 | 1.44 | 0.0078 | 0.1246 | 0.1167 | 1.1123 |
| 19 | 17.89 | 9.20 | 6.70 | 3.10 | 3.33 | 0.10 | 1.44 | 0.0166 | 0.1254 | 0.1167 | 1.0742 |
| 20 | 24.45 | 9.20 | 6.70 | 3.75 | 4.23 | 0.10 | 1.63 | 0.0234 | 0.1248 | 0.1107 | 1.1280 |
| 21 | 24.45 | 9.20 | 6.70 | 3.75 | 4.40 | 0.10 | 1.63 | 0.0280 | 0.1299 | 0.1107 | 1.1733 |
| 22 | 24.45 | 9.20 | 6.70 | 3.75 | 4.17 | 0.10 | 1.63 | 0.0230 | 0.1231 | 0.1107 | 1.1120 |
| 23 | 35.76 | 9.20 | 6.70 | 4.49 | 4.75 | 0.10 | 1.99 | 0.0179 | 0.0939 | 0.0888 | 1.0579 |
| 24 | 35.76 | 9.20 | 6.70 | 4.49 | 4.85 | 0.10 | 1.99 | 0.0164 | 0.0959 | 0.0888 | 1.0802 |
| 25 | 35.76 | 9.20 | 6.70 | 4.49 | 4.99 | 0.10 | 1.99 | 0.0310 | 0.0987 | 0.0888 | 1.1114 |
| 26 | 41.67 | 9.20 | 6.70 | 5.08 | 5.44 | 0.10 | 2.05 | 0.0184 | 0.1014 | 0.0947 | 1.0987 |
| 27 | 41.67 | 9.20 | 6.70 | 5.08 | 5.68 | 0.10 | 2.05 | 0.0373 | 0.1059 | 0.0947 | 1.2100 |
| 28 | 40.03 | 9.20 | 6.70 | 5.00 | 5.45 | 0.10 | 2.00 | 0.0297 | 0.1067 | 0.0979 | 1.0900 |
| 29 | 12.80 | 9.20 | 6.70 | 2.42 | 3.31 | 0.10 | 1.32 | 0.0493 | 0.1484 | 0.1085 | 1.3678 |
| 30 | 12.80 | 9.20 | 6.70 | 2.42 | 2.74 | 0.10 | 1.32 | 0.0137 | 0.1228 | 0.1085 | 1.1322 |
| 31 | 12.80 | 9.20 | 6.70 | 2.42 | 3.04 | 0.10 | 1.32 | 0.0398 | 0.1363 | 0.1085 | 1.2562 |
| 32 | 12.80 | 9.20 | 6.70 | 2.42 | 2.75 | 0.10 | 1.32 | 0.0168 | 0.1233 | 0.1085 | 1.1100 |
| 33 | 17.89 | 9.20 | 9.20 | 3.10 | 3.91 | 0.10 | 1.44 | 0.0417 | 0.1472 | 0.1167 | 1.3600 |
| 34 | 17.89 | 9.20 | 9.20 | 3.10 | 3.68 | 0.10 | 1.44 | 0.0149 | 0.1385 | 0.1167 | 1.1600 |
| 35 | 17.89 | 9.20 | 9.20 | 3.10 | 3.76 | 0.10 | 1.44 | 0.0242 | 0.1416 | 0.1167 | 1.1800 |
| 36 | 24.45 | 9.20 | 9.20 | 3.75 | 4.32 | 0.10 | 1.63 | 0.0248 | 0.1275 | 0.1107 | 1.1520 |
| 37 | 24.45 | 9.20 | 9.20 | 3.75 | 4.57 | 0.10 | 1.63 | 0.0425 | 0.1349 | 0.1107 | 1.3200 |
| 38 | 35.76 | 9.20 | 9.20 | 4.49 | 5.10 | 0.10 | 1.99 | 0.0283 | 0.1009 | 0.0888 | 1.1359 |
| 39 | 35.76 | 9.20 | 9.20 | 4.49 | 4.94 | 0.10 | 1.99 | 0.0202 | 0.0977 | 0.0888 | 1.1002 |
| 40 | 35.76 | 9.20 | 9.20 | 4.49 | 4.95 | 0.10 | 1.99 | 0.0148 | 0.0979 | 0.0888 | 1.1024 |
| 41 | 49.62 | 9.20 | 9.20 | 6.00 | 6.35 | 0.10 | 2.07 | 0.0147 | 0.1165 | 0.1100 | 1.0980 |
| 42 | 49.04 | 9.20 | 9.20 | 5.90 | 6.50 | 0.10 | 2.08 | 0.0225 | 0.1180 | 0.1071 | 1.1017 |
| 43 | 49.04 | 9.20 | 9.20 | 5.90 | 6.64 | 0.10 | 2.08 | 0.0255 | 0.1206 | 0.1071 | 1.1254 |
| 44 | 12.80 | 9.20 | 9.20 | 2.42 | 3.53 | 0.10 | 1.32 | 0.0375 | 0.1583 | 0.1085 | 1.4587 |
| 45 | 12.80 | 9.20 | 9.20 | 2.42 | 2.94 | 0.10 | 1.32 | 0.0220 | 0.1318 | 0.1085 | 1.2149 |
| 46 | 12.80 | 9.20 | 9.20 | 2.42 | 2.84 | 0.10 | 1.32 | 0.0133 | 0.1273 | 0.1085 | 1.1736 |
| 47 | 49.04 | 9.20 | 12.30 | 5.90 | 6.29 | 0.10 | 2.08 | 0.0044 | 0.1142 | 0.1071 | 1.1600 |
| 48 | 49.04 | 9.20 | 12.30 | 5.90 | 6.21 | 0.10 | 2.08 | 0.0094 | 0.1128 | 0.1071 | 1.1900 |
| 49 | 12.07 | 12.30 | 3.80 | 3.00 | 3.08 | 0.10 | 1.01 | 0.0256 | 0.2387 | 0.2325 | 1.0267 |
| 50 | 12.07 | 12.30 | 3.80 | 3.00 | 3.14 | 0.10 | 1.01 | 0.0470 | 0.2433 | 0.2325 | 1.0467 |
| 51 | 12.07 | 12.30 | 6.70 | 3.00 | 3.47 | 0.10 | 1.01 | 0.0479 | 0.2689 | 0.2325 | 1.1567 |
| 52 | 26.31 | 12.30 | 6.70 | 4.30 | 4.52 | 0.10 | 1.53 | 0.0249 | 0.1515 | 0.1441 | 1.1100 |
| 53 | 34.72 | 12.30 | 6.70 | 5.00 | 5.16 | 0.10 | 1.74 | 0.0293 | 0.1342 | 0.1301 | 1.1230 |
| 54 | 12.07 | 12.30 | 9.20 | 3.00 | 3.75 | 0.10 | 1.01 | 0.0446 | 0.2906 | 0.2325 | 1.2500 |
| 55 | 26.31 | 12.30 | 9.20 | 4.30 | 4.97 | 0.10 | 1.53 | 0.0344 | 0.1665 | 0.1441 | 1.1558 |
| 56 | 35.76 | 12.30 | 9.20 | 5.10 | 5.64 | 0.10 | 1.75 | 0.0239 | 0.1439 | 0.1301 | 1.1059 |
| 57 | 26.31 | 12.30 | 12.30 | 4.30 | 5.00 | 0.10 | 1.53 | 0.0114 | 0.1675 | 0.1441 | 1.1628 |
| 58 | 33.18 | 12.30 | 12.30 | 4.90 | 5.41 | 0.10 | 1.69 | 0.0192 | 0.1480 | 0.1341 | 1.2000 |
| No. | h (m) | D (m) | J | w (u/s) | Uc (u/s) | Us (u/s) | |
|---|---|---|---|---|---|---|---|
| 1 | 0.01 | 0.005 | 0.1 | 0.489 | 1.260 | 1.063 | 0.099 |
| 2 | 0.01 | 0.01 | 0.1 | 0.692 | 1.260 | 0.941 | 0.099 |
| 3 | 0.01 | 0.02 | 0.1 | 0.978 | 1.260 | 0.723 | 0.099 |
| 4 | 0.01 | 0.025 | 0.1 | 1.094 | 1.260 | 0.638 | 0.099 |
| 5 | 0.01 | 0.03 | 0.1 | 1.198 | 1.260 | 0.581 | 0.099 |
| 6 | 0.01 | 0.04 | 0.1 | 1.383 | 1.260 | 0.529 | 0.099 |
| 7 | 0.01 | 0.05 | 0.1 | 1.547 | 1.260 | 0.439 | 0.099 |
| 8 | 0.02 | 0.01 | 0.1 | 0.692 | 2.223 | 2.185 | 0.140 |
| 9 | 0.02 | 0.02 | 0.1 | 0.978 | 2.223 | 1.769 | 0.140 |
| 10 | 0.02 | 0.025 | 0.1 | 1.094 | 2.223 | 1.625 | 0.140 |
| 11 | 0.02 | 0.03 | 0.1 | 1.198 | 2.223 | 1.589 | 0.140 |
| 12 | 0.02 | 0.04 | 0.1 | 1.383 | 2.223 | 1.381 | 0.140 |
| 13 | 0.02 | 0.05 | 0.1 | 1.547 | 2.223 | 1.246 | 0.140 |
| 14 | 0.03 | 0.01 | 0.1 | 0.692 | 2.751 | 2.644 | 0.171 |
| 15 | 0.03 | 0.02 | 0.1 | 0.978 | 2.751 | 2.401 | 0.171 |
| 16 | 0.03 | 0.03 | 0.1 | 1.198 | 2.751 | 2.196 | 0.171 |
| 17 | 0.03 | 0.04 | 0.1 | 1.383 | 2.751 | 2.022 | 0.171 |
| 18 | 0.03 | 0.05 | 0.1 | 1.547 | 2.751 | 1.852 | 0.171 |
| 19 | 0.04 | 0.01 | 0.1 | 0.692 | 2.814 | 2.772 | 0.198 |
| 20 | 0.04 | 0.02 | 0.1 | 0.978 | 2.814 | 2.624 | 0.198 |
| 21 | 0.04 | 0.03 | 0.1 | 1.198 | 2.814 | 2.527 | 0.198 |
| 22 | 0.04 | 0.04 | 0.1 | 1.383 | 2.814 | 2.381 | 0.198 |
| 23 | 0.04 | 0.05 | 0.1 | 1.547 | 2.814 | 2.178 | 0.198 |
| 24 | 0.05 | 0.01 | 0.1 | 0.692 | 2.864 | 2.912 | 0.221 |
| 25 | 0.05 | 0.02 | 0.1 | 0.978 | 2.864 | 2.784 | 0.221 |
| 26 | 0.05 | 0.03 | 0.1 | 1.198 | 2.864 | 2.622 | 0.221 |
| 27 | 0.05 | 0.04 | 0.1 | 1.383 | 2.864 | 2.511 | 0.221 |
| 28 | 0.05 | 0.05 | 0.1 | 1.547 | 2.864 | 2.269 | 0.221 |
| No. | h (cm) | S (%) | D (mm) | Cv | f | fc | f/fc (Exp) | f/fc (Auth) | Difference (%) |
|---|---|---|---|---|---|---|---|---|---|
| 1 | 20.10 | 0.50 | 12.3 | 3.58 × 10−7 | 0.066 | 0.066 | 1.0000 | 1.0054 | 0.54 |
| 2 | 20.90 | 0.50 | 12.3 | 1.15 × 10−6 | 0.068 | 0.065 | 1.0462 | 1.0381 | −0.77 |
| 3 | 21.30 | 0.50 | 12.3 | 1.72 × 10−6 | 0.066 | 0.064 | 1.0313 | 1.0498 | 1.80 |
| 4 | 21.40 | 0.50 | 12.3 | 1.76 × 10−6 | 0.067 | 0.064 | 1.0469 | 1.0506 | 0.36 |
| 5 | 21.80 | 0.50 | 12.3 | 2.37 × 10−6 | 0.065 | 0.064 | 1.0156 | 1.0596 | 4.33 |
| 6 | 22.50 | 0.50 | 12.3 | 2.91 × 10−6 | 0.067 | 0.063 | 1.0635 | 1.0666 | 0.30 |
| 7 | 22.70 | 0.50 | 12.3 | 3.93 × 10−6 | 0.063 | 0.062 | 1.0161 | 1.0754 | 5.83 |
| 8 | 23.50 | 0.50 | 12.3 | 5.74 × 10−6 | 0.066 | 0.061 | 1.0820 | 1.0876 | 0.52 |
| 9 | 24.00 | 0.50 | 12.3 | 6.06 × 10−6 | 0.065 | 0.061 | 1.0656 | 1.0901 | 2.30 |
| 10 | 24.50 | 0.50 | 12.3 | 5.66 × 10−6 | 0.065 | 0.060 | 1.0833 | 1.0890 | 0.53 |
| 11 | 25.00 | 0.50 | 12.3 | 8.19 × 10−6 | 0.064 | 0.060 | 1.0667 | 1.1005 | 3.17 |
| 12 | 25.30 | 0.50 | 12.3 | 8.57 × 10−6 | 0.063 | 0.060 | 1.0500 | 1.1023 | 4.98 |
| 13 | 16.50 | 0.75 | 12.3 | 7.30 × 10−6 | 0.074 | 0.072 | 1.0278 | 1.0656 | 3.68 |
| 14 | 17.10 | 0.75 | 12.3 | 1.57 × 10−5 | 0.075 | 0.071 | 1.0563 | 1.0886 | 3.06 |
| 15 | 17.70 | 0.75 | 12.3 | 1.56 × 10−5 | 0.076 | 0.070 | 1.0857 | 1.0900 | 0.39 |
| 16 | 18.30 | 0.75 | 12.3 | 3.55 × 10−5 | 0.077 | 0.069 | 1.1159 | 1.1151 | −0.08 |
| 17 | 19.10 | 0.75 | 12.3 | 4.17 × 10−5 | 0.080 | 0.067 | 1.1940 | 1.1217 | −6.06 |
| 18 | 19.40 | 0.75 | 12.3 | 5.98 × 10−5 | 0.077 | 0.067 | 1.1493 | 1.1330 | −1.41 |
| 19 | 19.90 | 0.75 | 12.3 | 7.69 × 10−5 | 0.077 | 0.066 | 1.1667 | 1.1416 | −2.15 |
| 20 | 20.30 | 0.75 | 12.3 | 1.19 × 10−4 | 0.076 | 0.066 | 1.1515 | 1.1556 | 0.35 |
| 21 | 21.10 | 0.75 | 12.3 | 1.17 × 10−4 | 0.074 | 0.064 | 1.1563 | 1.1569 | 0.06 |
| 22 | 13.00 | 0.90 | 12.3 | 3.86 × 10−6 | 0.089 | 0.081 | 1.0988 | 1.0322 | −6.05 |
| 23 | 13.60 | 0.90 | 12.3 | 1.08 × 10−5 | 0.088 | 0.079 | 1.1139 | 1.0622 | −4.64 |
| 24 | 14.10 | 0.90 | 12.3 | 1.39 × 10−5 | 0.087 | 0.078 | 1.1154 | 1.0708 | −4.00 |
| 25 | 14.60 | 0.90 | 12.3 | 2.70 × 10−5 | 0.086 | 0.076 | 1.1316 | 1.0910 | −3.59 |
| 26 | 15.30 | 0.90 | 12.3 | 6.00 × 10−5 | 0.089 | 0.075 | 1.1867 | 1.1160 | −5.96 |
| 27 | 15.70 | 0.90 | 12.3 | 6.98 × 10−5 | 0.086 | 0.074 | 1.1622 | 1.1216 | −3.49 |
| 28 | 16.00 | 0.90 | 12.3 | 9.16 × 10−5 | 0.082 | 0.073 | 1.1233 | 1.1304 | 0.63 |
| 29 | 16.50 | 0.90 | 12.3 | 1.50 × 10−4 | 0.082 | 0.072 | 1.1389 | 1.1464 | 0.66 |
| 30 | 16.90 | 0.90 | 12.3 | 1.66 × 10−4 | 0.080 | 0.071 | 1.1268 | 1.1506 | 2.12 |
| 31 | 17.30 | 0.90 | 12.3 | 1.78 × 10−4 | 0.078 | 0.070 | 1.1143 | 1.1538 | 3.55 |
| 32 | 17.80 | 0.90 | 12.3 | 2.10 × 10−4 | 0.079 | 0.070 | 1.1286 | 1.1601 | 2.80 |
| 33 | 18.70 | 0.90 | 12.3 | 3.11 × 10−4 | 0.084 | 0.068 | 1.2353 | 1.1744 | −4.93 |
| 34 | 12.20 | 1.00 | 12.3 | 2.10 × 10−5 | 0.094 | 0.083 | 1.1325 | 1.0725 | −5.30 |
| 35 | 12.80 | 1.00 | 12.3 | 2.90 × 10−5 | 0.095 | 0.081 | 1.1728 | 1.0837 | −7.60 |
| 36 | 13.40 | 1.00 | 12.3 | 7.26 × 10−5 | 0.095 | 0.080 | 1.1875 | 1.1119 | −6.36 |
| 37 | 13.90 | 1.00 | 12.3 | 1.19 × 10−4 | 0.094 | 0.078 | 1.2051 | 1.1280 | −6.40 |
| 38 | 14.30 | 1.00 | 12.3 | 1.48 × 10−4 | 0.090 | 0.077 | 1.1688 | 1.1357 | −2.83 |
| 39 | 14.90 | 1.00 | 12.3 | 1.93 × 10−4 | 0.092 | 0.076 | 1.2105 | 1.1455 | −5.37 |
| 40 | 15.40 | 1.00 | 12.3 | 3.20 × 10−4 | 0.091 | 0.074 | 1.2297 | 1.1623 | −5.49 |
| 41 | 16.20 | 1.00 | 12.3 | 3.50 × 10−4 | 0.087 | 0.073 | 1.1918 | 1.1674 | −2.05 |
| 42 | 10.30 | 1.25 | 12.3 | 7.67 × 10−5 | 0.098 | 0.091 | 1.0769 | 1.0942 | 1.61 |
| 43 | 11.10 | 1.25 | 12.3 | 1.50 × 10−4 | 0.104 | 0.087 | 1.1954 | 1.1169 | −6.57 |
| 44 | 11.70 | 1.25 | 12.3 | 2.54 × 10−4 | 0.105 | 0.085 | 1.2353 | 1.1347 | −8.14 |
| 45 | 12.20 | 1.25 | 12.3 | 3.26 × 10−4 | 0.103 | 0.083 | 1.2410 | 1.1441 | −7.81 |
| 46 | 12.60 | 1.25 | 12.3 | 3.95 × 10−4 | 0.100 | 0.082 | 1.2195 | 1.1513 | −5.59 |
| 47 | 13.80 | 1.25 | 12.3 | 5.83 × 10−4 | 0.103 | 0.078 | 1.3205 | 1.2674 | −4.02 |
| 48 | 14.60 | 1.25 | 12.3 | 6.45 × 10−4 | 0.098 | 0.076 | 1.2895 | 1.1732 | −9.02 |
| 49 | 15.70 | 1.25 | 12.3 | 6.42 × 10−4 | 0.100 | 0.074 | 1.3514 | 1.2765 | −5.54 |
| 50 | 8.40 | 1.50 | 12.3 | 1.73 × 10−4 | 0.115 | 0.101 | 1.1386 | 1.1023 | −3.19 |
| 51 | 9.10 | 1.50 | 12.3 | 4.16 × 10−4 | 0.119 | 0.097 | 1.2268 | 1.1314 | −7.78 |
| 52 | 9.90 | 1.50 | 12.3 | 5.88 × 10−4 | 0.127 | 0.093 | 1.3656 | 1.3455 | −1.47 |
| 53 | 10.50 | 1.50 | 12.3 | 8.05 × 10−4 | 0.126 | 0.090 | 1.4000 | 1.3577 | −3.02 |
| 54 | 11.60 | 1.50 | 12.3 | 9.16 × 10−4 | 0.132 | 0.085 | 1.5529 | 1.4620 | −5.86 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hou, J.; Zhang, C.; Wang, D.; Li, F.; Yu, Z.; Zhou, Q. Fixed-Bed and Mobile-Bed Resistance of Channels with Steep Gradients in Mountainous Areas. Water 2019, 11, 681. https://doi.org/10.3390/w11040681
Hou J, Zhang C, Wang D, Li F, Yu Z, Zhou Q. Fixed-Bed and Mobile-Bed Resistance of Channels with Steep Gradients in Mountainous Areas. Water. 2019; 11(4):681. https://doi.org/10.3390/w11040681
Chicago/Turabian StyleHou, Ji, Chunze Zhang, Dan Wang, Feng Li, Zijian Yu, and Qin Zhou. 2019. "Fixed-Bed and Mobile-Bed Resistance of Channels with Steep Gradients in Mountainous Areas" Water 11, no. 4: 681. https://doi.org/10.3390/w11040681
APA StyleHou, J., Zhang, C., Wang, D., Li, F., Yu, Z., & Zhou, Q. (2019). Fixed-Bed and Mobile-Bed Resistance of Channels with Steep Gradients in Mountainous Areas. Water, 11(4), 681. https://doi.org/10.3390/w11040681




