Design and Optimization of a Fully-Penetrating Riverbank Filtration Well Scheme at a Fully-Penetrating River Based on Analytical Methods

Abstract

In order to maintain the sustainable development of pumping wells in riverbank filtration (RBF) and simultaneously minimize the possible negative effects induced, it is vital to design and subsequently optimize the engineering parameters scientifically. An optimizing method named Five-Step Optimizing Method was established by using analytic methods (Mirror-Image Method, Dupuit Equation and the Interference Well Group Method, etc.) systematically in this study considering both the maximum allowable drawdown of the groundwater level and the water demand as the constraint conditions, followed by a case study along the Songhua River of northeast China. It contained three parameters (number of wells, distance between wells, and distance between well and river) for optimizing in the method, in which the well type, depth and radius were beforehand designed and fixed, without the need of optimizing. The interference between wells was found to be a decisive factor that significantly impacts the optimizing effort of all the three parameters. The distance between the well and the river was another decisive factor impacting the recharge from the river and subsequently, the well water yield. There would be more than one optional scheme sometimes in the optimized result, while it’s not yet difficult in practice to single out the optimal one considering both the field setting and the water demand. The established method proved to be applicable in the case study.

1. Introduction

Riverside water source (RWS) refers to the water source where the wells are arranged close to the riverbank and mainly recharged by the adjacent river water through riverbank filtration (RBF) [1]. As a very important method in the development and utilization of water resources, the RWS has been widely valued and applied worldwide for its advantage of water pre-treatment and the regulatory capacity of water quantity [2]. In order to give full play to the advantages such as more sufficient and stable water supply, better water quality and more beneficial to centralized exploitation, etc., it is the most critical step for RWS to design the well group layout and the exploitation plan scientifically [3]. Different from the general surface water source and the general groundwater source, a set of parameters of RWS should be considered systematically and skillfully, such as the hydrological and hydrogeological conditions, surface water and groundwater quality, the structure of the RBF and its physical and chemical properties, distance between wells, distance between well and river, well depth, location and length of the filter pipe, allowable drawdown of groundwater level and water yield [4]. Moreover, some parameters impact each other. Thus, the design of RWS is extraordinarily complex and difficult. In past decades, most of the studies on RWS and RBF focused on the surface water, groundwater interaction, pollutant migration and transformation, RBF clogging, numerical simulation, and an evaluation of the water resource, etc., while studies on the well layout optimizing are relatively insufficient considering its importance. How to design and optimize the layout of the pumping wells, and the exploitation schemes in RBF has become an urgent problem to be studied.

The well group layout and the exploitation schemes of the RWS play a decisive role in water yield, water quality and the impact on the geologic environment [5,6]. In addition to the surface water and groundwater level, the influence factors of the hydrodynamic process and water yield of the RWS include the integrity of the river (whether the river is disjointed is also included) [7], the topography [8] and silting [9] of the riverbed, the permeability of the riverbed and aquifer [10], and the river crossing seepage (partial penetrating river) [11], etc. Therefore, the above factors should be fully considered in the design of RWS. Moreover, the number of wells, well depth, distance between wells, and distance between well and river should be optimized in combination with the water demand and the allowable drawdown of the groundwater level [12]. At present, the study methods of RWS mainly include analytical methods [13] and numerical simulation methods [14]. As a means of obtaining hydrogeological parameters and verifying results, pumping tests are useful [15], and tracer tests have also been widely used [16].

The study on the RWS could be carried out in a number of ways, and the recharge rate of infiltration captured from the river water was usually determined through productive experiments in the early stage [17], which could provide a basis for the determination of the well location. Later, the iterative moving subdomain method [18] and fuzzy comprehensive evaluation model [19] based on the basic theory of fuzzy mathematics; were introduced to optimize the layout of pumping wells. The distance between well and river and the distance between wells could be determined by using the phreatic well equation of linear-arranged interferential well group [20], and the distance between well and river value could also be furtherly minimized by coupling riverbank filtration and reverse osmosis [21]. The optimal water yield can be determined by using the nonlinear optimizing method, evolutionary algorithm [22] and numerical simulation method using the Visual MODFLOW software. In addition, the sustainable water yield can be calculated by using the analytical method [23] while the optimization study of the water yield can be carried out by using analytical method [24], multi-objective optimizing model [25], and modelling method [26]. A large number of study cases showed that the distance between the well and river influenced the water yield profoundly. It could also pose an impact on the recharge from the river [27]. However, with a certain exploitation amount, the capture zone is less affected by the distance between well and river [28]. The construction and operation cost of an RBF scheme is also a factor impacting the selection of the types of pumping wells [29]. In addition, in terms of the study of the effectiveness of the RBF as a pre-treatment means of water, the methods of investigation and study are constantly being innovated and upgraded, and the joint application of multiple means is increasingly emphasized [30]. Although a lot of studies have been carried out on the design and optimization of well groups of RWS, critical issues that need to be coped with still exist. For example, it is worth further exploration to establish a popularizing method of optimizing the well layout from the perspective of river-groundwater dynamics.

In this study, both the maximum allowable drawdown of the groundwater level and the water demand are taken as the constraint conditions. On this basis, various well group layouts and exploitation schemes are firstly formed by combining some parameters with the well type, the number of wells, the distance between wells and the distance between well and river. Secondly, the interactions among engineering parameters are explored and the values of those parameters are compared and screened step-by-step, so as to form a modularized optimizing method for the well group layout and the exploitation plan of an RBF scheme. During this process, the sustainable water yield would be calculated by the analytical method. Further, a case study is carried out by using the established method and subsequently discussed.

2. Scenarios and Methods

2.1. Scenarios

In this study, the optimizing method was discussed by taking an RBF scheme with a condition of river fully penetrating the phreatic aquifer in the vertical dimension [31] as an example. The necessary parameters characterizing the geological and hydrogeological settings, such as hydraulic conductivity (K), aquifer thickness (H₀), etc., could be determined through hydrogeologic drilling, a pumping test, analogy and collecting previous data. After the hydrogeological conditions are identified, the characteristics of the aquifer, boundary conditions, initial conditions, hydraulic characteristics, and source sink term could be determined and subsequently generalized.

2.2. Argument Method of Water Supply Capacity of Rws

In order to reveal the differences in water supply capacity, the yields of single pumping wells under different conditions were calculated separately: an off-riverside well (known as non-riverside water source) and a riverside well (known as RWS). Besides, a pumping well group along a riverside was also considered here, as the third scenario considering the practice needs.

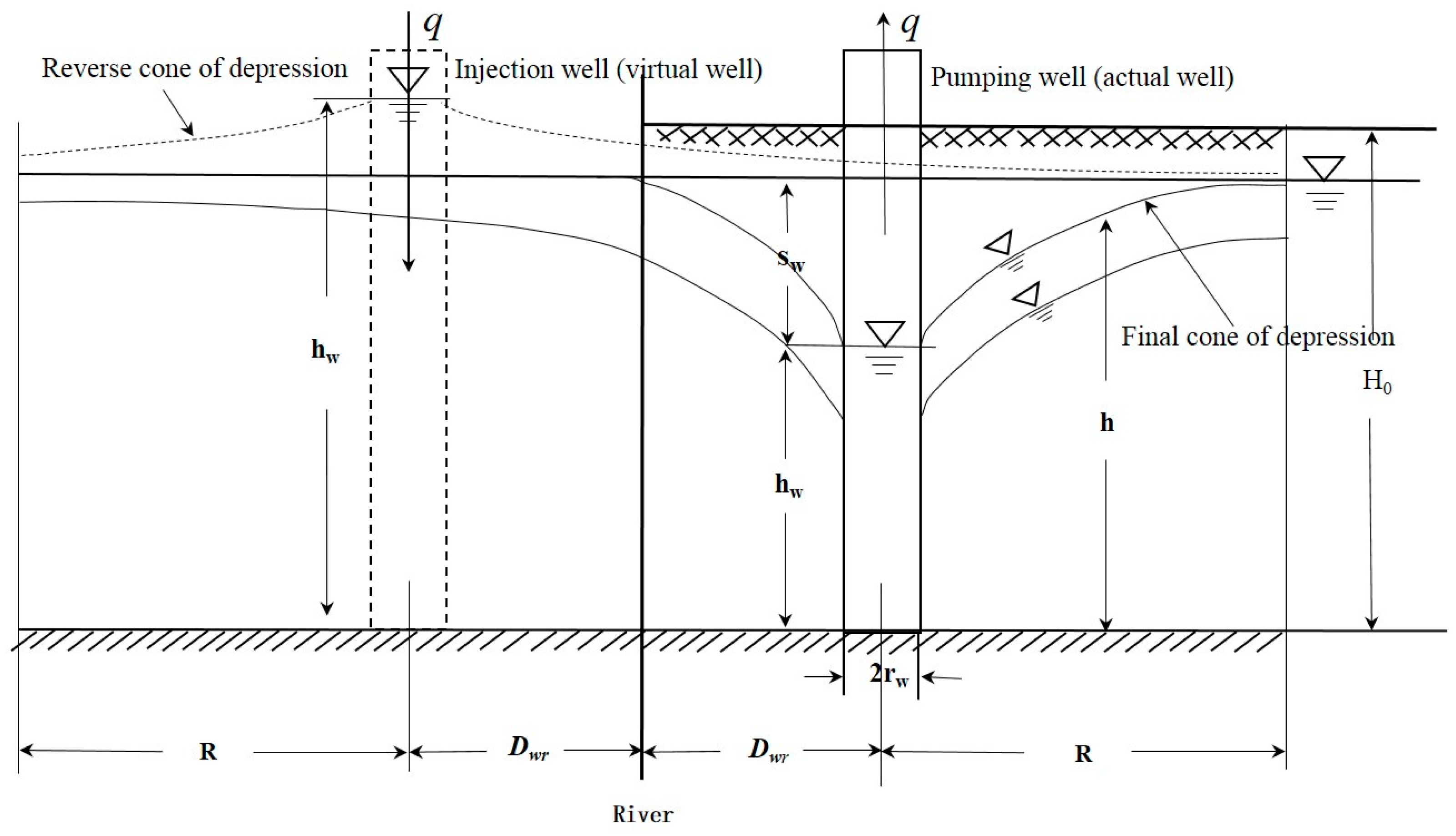
2.2.1. Scenario I: A Single Pumping Well Off-Riverside

Under the condition of a single pumping well off-riverside that was independent of the river, the well could be generalized as an incomplete well in an infinite phreatic aquifer in the horizontal dimension (excluding the upper sealing section). In order to facilitate the calculation, it was assumed that the well meets the Dupuit Hypothesis, that is, the flow line to the well is approximately horizontal, and the contour map of groundwater level was a coaxial cylinder, which was consistent with the passing water section. According to the Dupuit Equation, the water yield of the single well could be calculated as the following:

$$\mathrm{q}=\mathsf{\pi }\mathrm{K}\frac{\left(2{\mathrm{H}}_0-{\mathrm{s}}_{\mathrm{w}}\right){\mathrm{s}}_{\mathrm{w}}}{\mathrm{l}\mathrm{n}\frac{\mathrm{R}}{{\mathrm{r}}_{\mathrm{w}}}}$$

(1)

where q is the water yield (m³/d); K is the hydraulic conductivity (m/d); H₀ is the phreatic aquifer thickness (m); s_w is the drawdown of the groundwater level (m); R is the influence radius (m); and r_w is the well radius (m).

2.2.2. Scenario II: A Single Pumping Well along a Linear Riverside

Under the condition of a single pumping well along a linear riverside, the river could be determined as a recharge boundary with a specific water level, which could be coped with according to the Mirror-Image Method (Bear, 1979) [32]. To save space, the detailed description of the method was omitted here (the schematic diagram can be seen in Figure 1), which could be referenced to Bear (1979) [32], and the water yield of the single well could be calculated, as the following:

$$\mathrm{q}=\mathsf{\pi }\mathrm{K}\frac{\left(2{\mathrm{H}}_0-{\mathrm{s}}_{\mathrm{w}}\right){\mathrm{s}}_{\mathrm{w}}}{\mathrm{l}\mathrm{g}\frac{2{\mathrm{D}}_{\mathrm{w}\mathrm{r}}}{{\mathrm{r}}_{\mathrm{w}}}}$$

(2)

where D_wr is the distance between the well and the river (m); and the other symbols are the same as those in Equation (1).

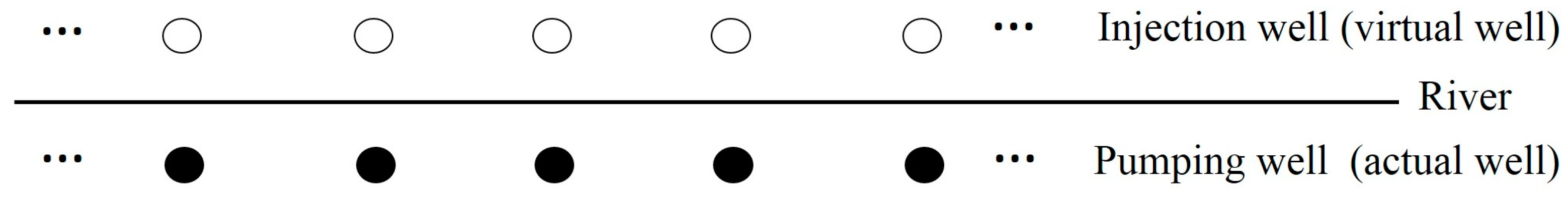
2.2.3. Scenario III: A Well Group along a Linear Riverside

In many practice cases, a set of pumping wells were arranged along a riverside replacing a single well due to the limited water supply capacity of the latter. Thus, the scenario where a well group along a linear riverside was considered in this study. The water supply capacity of this scenario was jointly impacted by the layout of the wells, and the exploitation plan. In this section, we deduced the calculating equations of the exploitation amount of a single well in the well group in addition to that of the well group. Those equations were convenient for the optimization of the layout of the wells and the exploitation plan in the following sections. The Mirror-Image Method was also applicable for this scenario, and the corresponding schematic diagram adapted from that of the scenario with a single well could be seen in Figure 2.

The equation describing the interference between pumping wells could be deduced from Dupuit Equation:

$${\mathrm{H}}_0^2-{\mathrm{h}}_{\mathrm{w}}^2=\frac{\mathrm{q}}{\mathsf{\pi }\mathrm{K}}\mathrm{l}\mathrm{n}\frac{\mathrm{R}}{{\mathrm{r}}_{\mathrm{w}}}$$

(3)

where h_w is the groundwater level of the pumping well compared with the bottom of the aquifer, which is valued as the difference value between the aquifer thickness (H₀) and the drawdown of the groundwater level (s_w) (m); and the other symbols are the same as those of the above equations. The water yield of a pumping well in the well group could be determined by this equation, based on which the total water yield of the well group could be calculated easily if the number of wells was specified.

Superposition calculation could be carried out based on Equation (3) when the total water yield of all the pumping wells were the same [33]:

$${\mathrm{H}}_0^2-{\mathrm{h}}_{\mathrm{i}}^2=\sum _{\mathrm{j}=1}^{\mathrm{n}}\frac{\mathrm{q}}{\mathsf{\pi }\mathrm{K}}\mathrm{l}\mathrm{n}\frac{\mathrm{R}}{{\mathrm{r}}_{\mathrm{j}}^{-}}-\sum _{\mathrm{j}=1}^{\mathrm{n}}\frac{\mathrm{q}}{\mathsf{\pi }\mathrm{K}}\mathrm{l}\mathrm{n}\frac{\mathrm{R}}{{\mathrm{r}}_{\mathrm{j}}^{+}}=\frac{\mathrm{q}}{\mathsf{\pi }\mathrm{K}}\mathrm{l}\mathrm{n}\frac{{{\displaystyle \prod }}_1^{\mathrm{n}}{\mathrm{r}}_{\mathrm{j}}^{+}}{{{\displaystyle \prod }}_1^{\mathrm{n}}{\mathrm{r}}_{\mathrm{j}}^{-}}$$

(4)

where r_j⁺ is the distance between the j injection well and the calculated pumping well (the i well) (m); r_j⁻ is the distance between two adjacent pumping wells (the i and j well) (m); r_j⁻ = r_w when the pumping well is the calculated pumping well; and the other symbols are the same as those of the above equations.

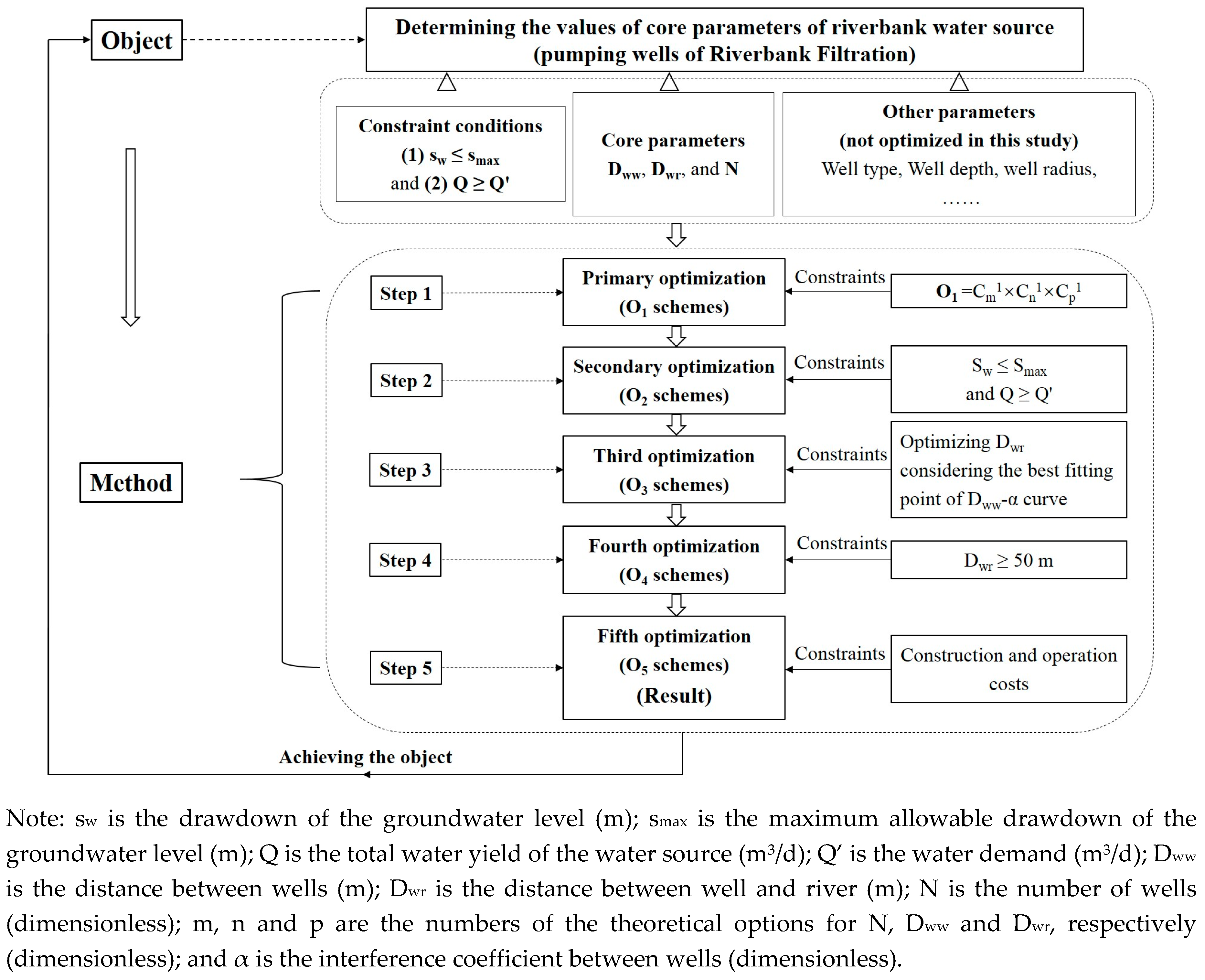
2.3. Method of Design and Optimization of Well Group of Rws

2.3.1. Constraint Conditions

Considering the sustainable exploitation and utilization of RWS, it was essential to avoid causing the persistent decline of the groundwater level and bring an unacceptable negative impact on the ecosystem at the site and around. In this regard, it is common to set one third of the aquifer thickness (H₀), as the maximum allowable drawdown of the groundwater level (s_max) [33] which should be the upper limit of the drawdown of the groundwater level (s_w). The planned water resource exploitation is usually determined jointly by the water demand (Q′), as well as the water supply capacity of the water source (Q) [34]. Sometimes, the construction and operational costs were also taken into account. Taking these factors into account, both s_w and Q were selected as the constraint conditions (objective function) as seen in Figure 3. In detail, the results of the design and optimization effort needed to satisfy the two conditions simultaneously: s_w ≤ s_max, and Q ≥ Q′.

2.3.2. Parameter Design

The layout of the well group directly determined the success of the establishment of the water source. The parameters involved included not only the well type, well depth and well radius (r_w), but also the number of wells (N), the distance between wells (D_ww) and the distance between well and river (D_wr). It is common to arrange tube wells in a straight line parallel to the river [33], considering it has better stimulation from the recharge from the river. In order to ensure the water intake efficiency, the pumping well was often designed as a completely penetrating well, which penetrated the whole phreatic aquifer in the vertical dimension. As to r_w, it is usually determined by the manufacturing technique, and 0.25 m was the most designed value in many regions.

Different from the fixed type and parameters discussed above, it is very complex and difficult to determine the values of N, D_ww, and D_wr due to their interactions with each other. Thus, N, D_ww, and D_wr were selected as the objective parameters for optimizing through a certain method in this study, and the numbers of the theoretical options for these three parameters were assumed to be m, n, and p, respectively.

2.3.3. Parameter Optimization

In order to facilitate the discussion, the method established in this study was named the “Five-Step Optimizing Method” (Figure 3), and the result schemes obtained by the latter step were the subset of those obtained by the former step (O₅ ⊂ O₄ ⊂ O₃ ⊂ O₂ ⊂ O₁).

(1) The First Step: The Establishment of all the Possible Schemes (O₁)

The first step was to establish the scheme set including all the possible schemes. Considering the numbers of the theoretical options for N, D_ww, and D_wr being m, n, and p, respectively, the number of all the possible schemes (O₁) equaled the result of ${\mathrm{C}}_{\mathrm{m}}^1\times {\mathrm{C}}_{\mathrm{n}}^1\times {\mathrm{C}}_{\mathrm{p}}^1$.

(2) The Second Step: Screening from N

The second step was to compare all the schemes established in O₁ and then screen the possible schemes satisfying s_w ≤ s_max and Q ≥ Q′ simultaneously, forming a scheme set named O₂. N depended on Q′ and the water yield of a single well, and the latter was determined by s_max. Thus, Q and s_w of each scheme should be calculated, followed by the screening of the favorable schemes.

(3) The Third Step: Screening from D_ww

The third step was to screen those schemes in O₂ with the favorable D_ww, which was carried out by establishing the relationship between D_ww and α (the interference coefficient between wells) and subsequently, finding the inflection point at the curve. The corresponding D_ww value of the inflection point or around was considered to be the favorable D_ww [35]. A strong relationship between D_ww and α existed, and the greater the D_ww was, the smaller α was, which could facilitate obtaining more water yield. However, the greater D_ww would increase, the cost including waterline, power transmission system and the corresponding management cost [33]. Thus, it is the designer’s responsibility to minimize D_ww as much as possible under the premise of ensuring meeting the water demand [36]. After screening the favorable D_ww from O₂, the possible schemes further decreased temporarily to form O₃.

α referred to the percentage change of the water yield of a single well with disturbance relative to that of a single well without disturbance [35], which could be described as:

α = (q′ − q)/q′

(5)

where q is the water yield of a single well with disturbance calculated by Equation (4) (m³/d); and q′ is the water yield of a single well without disturbance calculated by Equation (2) (m³/d).

(4) The Fourth Step: Screening from D_wr

The fourth step was to screen those schemes in O₃ with the favorable D_wr. Generally speaking, the smaller the D_wr was, the greater the water yield was, which could increase the efficiency of the water intake engineering. Inversely, the water yield would decrease with the D_wr increasing because the recharge from the river would decrease. Under certain conditions, the impact of river water quality on the well water quality should also be considered, especially in a circumstance of surface water pollution, as pollutants in the RBF usually decreased with the D_wr increasing [37]. After screening the favorable D_wr from O₃, the possible schemes further decreased temporarily to form O₄, which usually only included two or three schemes or less.

(5) The Fifth (Last) Step: Obtaining the Optimal Option (O₅) through Appropriate Consideration of Construction and Operation Costs

More than one scheme was often obtained after the accomplishment of the fourth optimizing step (O₄). If that happened, the optimal scheme usually could be screened by appropriately considering the construction and operation cost of the water intake engineering, which was usually not hard to accomplish. Besides, it is also common to encounter other factors that require consideration, such as the available land issue. Thus, it’s occasionally necessary to go further to the fifth (last) step, while more details was omitted here considering the step being relatively strong subjective and maneuverable.

3. Case Study

3.1. Study Area and Generalization

The case study referred to an experimental riverside water source established by the Songhua River, which was located at Harbin City of Heilongjiang Province, northeastern China. The hydrological and hydrogeological investigation, including pumping tests had been carried out during 2017–2018, through which the study area setting had been identified in detail. Limited to the length of this paper, more details were omitted, which could be referenced to Zhu et al. (2019) [2]. Generally speaking, the conditions in the study area met the assumptions of the method with the main parameter values such as K, H₀, R, and r_w being 50 m/d, 42 m, 200 m and 0.5 m, respectively. As the designed requirement in the method, s_max equaled 14 m (one third of H₀).

3.2. Water Supply Capacity of the RWS

If a single pumping well was established at the study area without considering the impact of the river, the q (23,056 m³/d ≈ 23,000 m³/d) could be easily determined by the Equation (1). If a single pumping well was established at the study area with simultaneously considering the actual impact of the river, three q values (69,800 m³/d, 61,400 m³/d and 57,400 m³/d) could be easily determined by Equation (2) separately with three designed D_wr values (20 m, 40 m and 60 m). These results showed that the water yields of the single pumping well in the riverside water source were 2.5–3 times as much as that of the single pumping well without the recharge from the river. The variation in multiples was caused by the differences of D_wr, and the smaller the D_wr, the greater both q and the corresponding multiple were. The results showed that D_wr had a great impact on q. As to the scenario of a well group along the riverside, the total water yield was impacted by many parameters complicatedly, which could be seen in the following section.

3.3. The Design and Optimization of the Well Group

3.3.1. Constraint Conditions

As discussed at the beginning of Section 3.1, s_max equaled 14 m. Q′ was designed as 2 × 10⁵ m³/d considering the water demand of Harbin City. Thus, the constraint conditions in this case study were: s_w ≤ s_max = 14 m, and Q ≥ Q′ = 2 × 10⁵ m³/d. The subsequent design and optimization effort was carried out around this goal.

3.3.2. Parameter Design

In this case study, all the pumping wells of the well group of the RWS were designed with the same specifications, considering the convenience of construction and management. The well type (tube well), depth (50 m from the ground to the bottom) and radius (r_w, 0.25 m) were directly designed without optimizing. As for those parameters needing optimization (N, D_ww and D_wr), the alternative options of the parameter values for optimizing needed to be fixed, considering both the study area setting and the water demand.

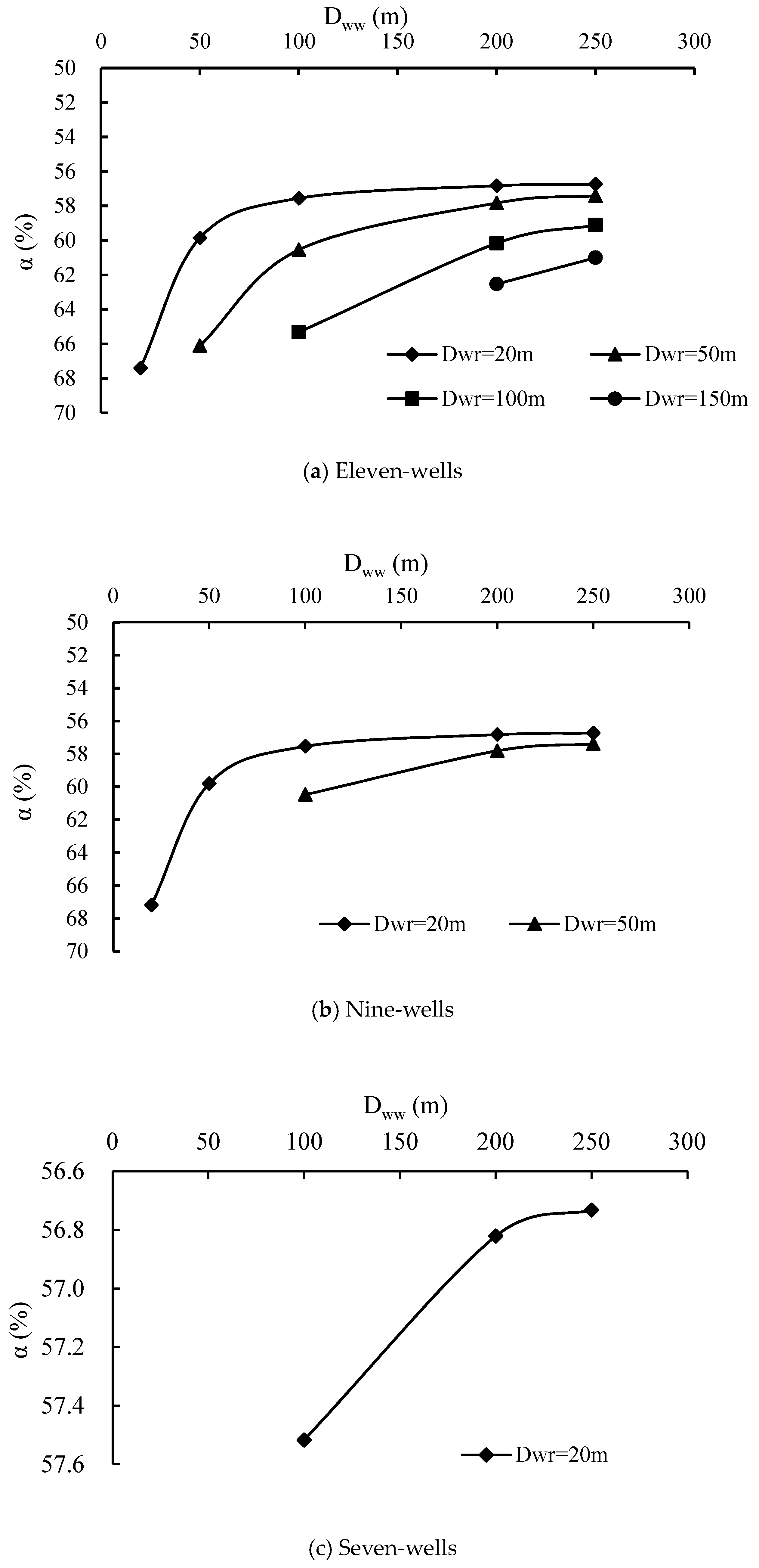
3.3.3. Parameter Optimization

(1) The First Step: The Establishment of all the Possible Schemes (O₁)

The alternative values of N were designed as 7, 9, and 11 (m = 3) considering the ratio of Q′/q (8.7) and the possible interaction between wells. The alternative values of D_ww were designed as 20 m, 50 m, 100 m, 150 m, and 200 m (n = 5) considering the influence radius and the impact from the river. The alternative values of D_wr were designed as 20 m, 50 m, 100 m, 200 m and 250 m (p = 5) considering the influence radius and the impact from the river. That’s to say, the optimizing effort was to screen the most favorable values set (scheme) of the three parameters from the 75 (${\mathrm{C}}_3^1\times {\mathrm{C}}_5^1\times {\mathrm{C}}_5^1$) established schemes (O₁) (

Table 1. All possible schemes and the corresponding results of each optimizing step.

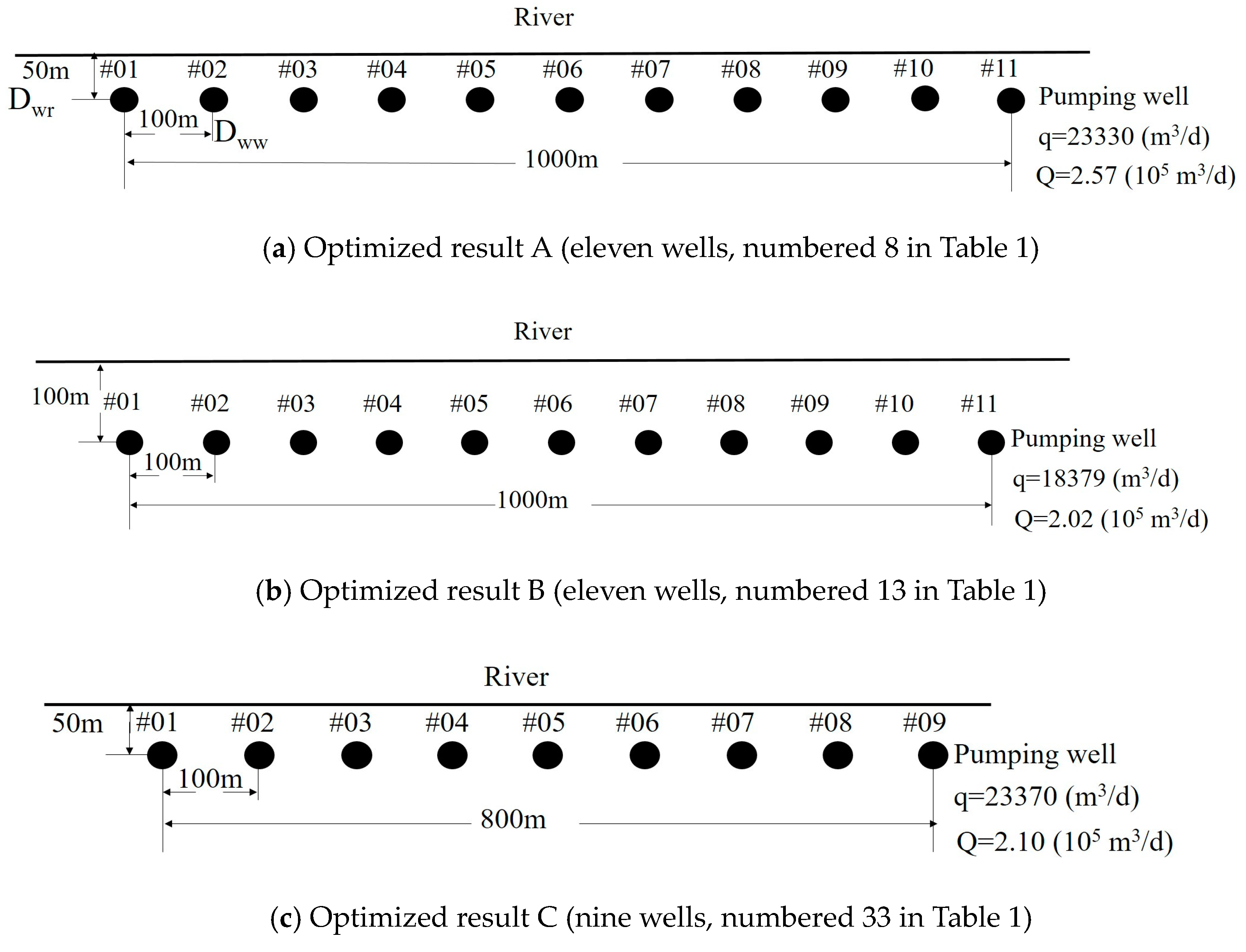
No.	N	Dww (m)	Dwr (m)	q (m3/d)	Q (×105 m3/d)	Result *
1	11	20	20	22,754	2.50	AB
2	11	50	20	28,022	3.08	AB
3	11	100	20	29,629	3.26	ABC
4	11	200	20	30,135	3.32	AB
5	11	250	20	30,199	3.32	AB
6	11	20	50	14,021	1.54	A
7	11	50	50	20,038	2.20	AB
8	11	100	50	23,330	2.57	ABCD
9	11	200	50	24,935	2.74	AB
10	11	250	50	25,185	2.77	AB
11	11	20	100	9555	1.05	A
12	11	50	100	14,499	1.60	A
13	11	100	100	18,379	2.02	ABCDE
14	11	200	100	21,111	2.32	AB
15	11	250	100	21,670	2.38	AB
16	11	20	150	7763	0.85	A
17	11	50	150	11,772	1.30	A
18	11	100	150	15,523	1.71	A
19	11	200	150	18,723	2.06	AB
20	11	250	150	19,485	2.14	AB
21	11	20	200	6785	0.75	A
22	11	50	200	10,135	1.12	A
23	11	100	200	13,610	14.97	A
24	11	200	200	16,974	1.87	A
25	11	250	200	17,858	1.96	A
26	9	20	20	22,902	2.06	AB
27	9	50	20	28,058	2.53	AB
28	9	100	20	29,639	2.67	ABC
29	9	200	20	30,137	2.71	AB
30	9	250	20	30,201	2.72	AB
31	9	20	50	14,343	1.29	A
32	9	50	50	20,153	1.81	A
33	9	100	50	23,370	2.10	ABCDE
34	9	200	50	24,946	2.25	AB
35	9	250	50	25,193	2.27	AB
36	9	20	100	10,020	0.90	A
37	9	50	100	14,727	1.33	A
38	9	100	100	18,476	1.66	A
39	9	200	100	21,144	1.90	A
40	9	250	100	21,692	1.95	A
41	9	20	150	8287	0.75	A
42	9	50	150	12,085	1.09	A
43	9	100	150	15,675	1.41	A
44	9	200	150	18,779	1.70	A
45	9	250	150	19,525	1.76	A
46	9	20	200	7333	0.66	A
47	9	50	200	10,507	0.95	A
48	9	100	200	13,811	1.24	A
49	9	200	200	17,056	1.54	A
50	9	250	200	17,917	1.61	A
51	7	20	20	23,141	1.62	A
52	7	50	20	28,117	1.97	A
53	7	100	20	29,656	2.08	AB
54	7	200	20	30,142	2.11	ABC
55	7	250	20	30,204	2.11	AB
56	7	20	50	14,857	1.04	A
57	7	50	50	20,338	1.42	A
58	7	100	50	23,433	1.64	A
59	7	200	50	24,964	1.75	A
60	7	250	50	25,204	1.76	A
61	7	20	100	10,738	0.75	A
62	7	50	100	15,092	1.06	A
63	7	100	100	18,631	1.30	A
64	7	200	100	21,196	1.48	A
65	7	250	100	21,727	1.52	A
66	7	20	150	9077	0.64	A
67	7	50	150	12,577	0.88	A
68	7	100	150	15,918	1.11	A
69	7	200	150	18,871	1.32	A
70	7	250	150	19,588	1.37	A
71	7	20	200	8148	0.57	A
72	7	50	200	11,086	0.78	A
73	7	100	200	14,132	0.99	A
74	7	200	200	17,188	1.20	A
75	7	250	200	18,011	1.26	A

* A: the result of the first optimizing step; AB: the result of the second optimizing step; ABC: the result of the third optimizing step; ABCD: the result of the fourth optimizing step; and ABCDE: the result of the fifth (last) optimizing step.

) by using the established method. N, D_ww, D_wr, q, and Q in Table 1 refer to number of wells, distance between wells, distance between well and river, single well yield, and total yield, respectively, and q was calculated by Equation (4).

(2) The Second Step: Screening from N

The results (Table 1) showed that the favorable schemes (Q ≥ Q′ = 2 × 10⁵ m³/d) for N of 11, 9, and 7 were 14, 8, and 3, respectively, which constituted a new scheme set with 25 possible schemes (O₂) (Table 1).

(3) The Third Step: Screening from D_ww

All the interference coefficients (α) between wells of schemes in O₂ were calculated (

Table 2. Interference coefficient of wells.

No.	N	Dww (m)	Dwr (m)	q (m3/d)	q′ (m3/d)	α (%)
1	11	20	20	22,753.5	69,805.6	67.40
2	11	50	20	28,021.8	69,805.6	59.86
3	11	100	20	29,629.2	69,805.6	57.55
4	11	200	20	30,134.7	69,805.6	56.83
5	11	250	20	30,199.2	69,805.6	56.74
6	11	50	50	20,038.2	59,130.1	66.11
7	11	100	50	23,330.2	59,130.1	60.54
8	11	200	50	24,934.7	59,130.1	57.83
9	11	250	50	25,185.2	59,130.1	57.41
10	11	100	100	18,379.0	52,998.7	65.32
11	11	200	100	21,111.3	52,998.7	60.17
12	11	250	100	21,669.9	52,998.7	59.11
13	11	200	150	18,722.6	49,967.8	62.53
14	11	250	150	19,485.1	49,967.8	61.00
15	9	20	20	22,901.5	69,805.6	67.19
16	9	50	20	28,058.2	69,805.6	59.81
17	9	100	20	29,639.4	69,805.6	57.54
18	9	200	20	30,137.3	69,805.6	56.83
19	9	250	20	30,200.9	69,805.6	56.74
20	9	100	50	23,369.6	59,130.1	60.48
21	9	200	50	24,946.0	59,130.1	57.81
22	9	250	50	25,192.6	59,130.1	57.39
23	7	100	20	29,655.8	69,805.6	57.52
24	7	200	20	30,141.6	69,805.6	56.82
25	7	250	20	30,203.6	69,805.6	56.73

), and correlation diagrams (Figure 4) were drawn to illustrate the relationships between D_ww and α with different N and different D_wr. The results showed that α decreased with D_ww increasing at the beginning, while decreased almost no longer after the inflection points appeared at the top left corners of all the curves. It suggested that the favorable value of D_ww should be around the inflection point, because smaller value would bring more disturbance between wells while greater value would bring more construction, and operation costs considering the occupying space of the wells. Thus, the favorable value of D_ww should be 100 m for both eleven-wells and nine-wells, while the same value should be 200 m for seven-wells. The favorable schemes decreased to six (O₃) after this optimizing step (Table 2). N, D_ww, D_wr, q, q′ and α in Table 2 refer to number of wells, distance between wells, distance between well and river, single well yield, single well yield without interference, and interference coefficient, respectively.

(4) The Fourth Step: Screening from D_wr

In O₃ with six schemes (Table 1), the three schemes with D_wr = 20 m were not a wise choice after considering the study area setting carefully, because the distance between the pumping wells and the river was too small to protect the wells from occasional flooding happening in the river. In addition, the safety of the river levee and the possible clogging of the RBF should be also considered. Thus, the schemes numbered 3, 28 and 54 in Table 1 had to be abandoned. So far, only three schemes left in Table 1 constituting O₄, which left very little scope to decision maker to select. That is to say, the optimal scheme in fact could be easily singled out from the three options (Figure 5). The drawdown of the groundwater level of the middle pumping well of each scheme is 14.0 m, while the drawdowns of the well at each edge of the three schemes are 12.7 m, 11.7 m, and 12.8 m respectively satisfy the constraint condition. Thus, sometimes the optimizing process could be terminated after this step.

(5) The Fifth (Last) Step: Obtaining the Optimal Option through the Appropriate Consideration of Construction and Operation Costs

In fact, the need to go on to the fifth step is obvious, on occasion, especially when the budget is not adequate, or the riverbed clogging occurs. Out of the three left schemes (Figure 5), the scheme (a) would have to be abandoned considering saving water resource and energy, as the total water yield (Q = 2.57 × 10⁵ m³/d) of it is much more than needed (Q′ = 2 × 10⁵ m³/d). Nevertheless, scheme (a) could also become a choice if the water demand increases. And the scheme (b) would also have to be abandoned considering saving investment, as it has two more pumping wells compared with the scheme (c). Thus, the scheme (c) could be singled out as the final result of the optimizing effort, if other factors are not considered.

However, the scheme (b) is the best choice if 50 m as the distance between the well and the river is considered to be too small to protect against floods and water pollution incidents, or 23,370 m³/d as the pumping yield of each well is considered to be too large to avoid the possible riverbed clogging in practice. That is to say, the final result may switch among the three scenarios considering the establishing investment, the energy consumption, the disaster risk, and the riverbed clogging, etc. Thus, all the three optimized schemes are valuable for the decision maker to choose.

4. Discussion

In this study, we tentatively established a method of design and optimization of pumping wells in riverbank filtration (RBF) by using analytical methods, in which the traditional methods such as the Mirror-Image Method, Dupuit Equation and Interference Well Group Method were jointly adopted. The method assumed that the phreatic aquifer was fully penetrated by the linear river in the vertical dimension, and there was a close hydraulic relation between the surface water and groundwater, both of which had nearly the same natural hydrological curve. The aquifer extended indefinitely in the other three directions and was bounded by an impervious rock at the bottom. The RBF was a homogeneous and isotropic medium, and there was no aquitard or aquiclude around the river. Thus, the model assumed was generally the same as that established by Theis (1941) [38], which was usually considered the simplest mode of surface water-groundwater interactions and rare in practice [31]. The main purpose of this simplification was to facilitate calculation, so we used the Mirror-Image Method in this study, after we proved the applicability [2]. This method was established by Jacob (1979) [32] to calculate the extending of the groundwater level, which is induced by pumping groundwater near the river and the corresponding water supply capacity of the pumping wells. Considering their simplicity, the mode and the corresponding method were often adopted in order to obtain the approximate solutions.

Similar to many other optimizing efforts, the parameters are not independent [39], which makes the optimizing efforts more difficult, especially in the use of analytical methods [40]. The basic hydrogeology tells us that the cone of depression of the groundwater level will continually extend both in the vertical and horizontal dimensions when pumping, unless the aquifer could capture as much as water recharge relative to the water yield [41]. Thus, the cones of depression of the different pumping wells will interact with each other if the distance between wells is not far enough [42], which will inevitably affect the efficiency of the pumping wells through decreasing the water capacity of a single well [43]. However, the cost will increase considering the water supply network and the power supply line if the distance is too far, especially when the number of the pumping wells are great [44]. Thus, it is an art to design the distance between wells, especially when there are many wells [45]. More than that, the recharge of the pumping well of RBF is usually much more than that of off-riverside because the former could capture a portion of river water [2]. Thus, the interaction of cones of depression of different wells will be more complex, which further complicates the design art of the distance of wells. As to the river-groundwater interaction, the river water captured by the pumping well is directly determined by the distance of the well and the river if the other parameters are fixed [46]. It is better to design a smaller value to the distance between well and river, only if the water quantity is considered in the engineering. However, it is not favorable for the effectiveness of the RBF as a pre-treatment measure of water [47]. Furthermore, it is also not favorable for protecting the wells from possible river flooding and water pollution, which will pose significant impact on the safety of the social water supply. The water quality is obviously also an important issue considering both the pre-treatment function of the RBF and the subsequent design of the post-treatment of the plant. The water quality issue was not considered quantitatively in this study, mainly because we considered that, the optimal scheme considering both the water quantity and quality of the pumping wells should only be singled out from the optional schemes only considering the water quantity, which was obtained from the optimizing method established in this study. That is to say, the design and optimization effort made in this study is the basis of considering the water quality issue, based on which the optimal scheme can be determined for both water quantity and quality.

River-groundwater interactions are at the core of a wide range of major contemporary challenges [48], out of which the provision of high-quality drinking water in sufficient quantities is without doubt the most important because it involves water demand of human society. Riverbank filtration (RBF) has been used for many decades widely worldwide especially in Europe, the United States and some Asian countries (China, India, Japan, South Korea, etc.) to provide drinking water [1,49]. However, the existing RBF comprehension mainly depends on practical understandings, and no standards have been developed to guide the optimization of the RBF design [50]. Thus, we have good reason to believe that more design and optimization efforts on RBF considering water quantity and quality or both will be carried out in the following periods.

5. Conclusions

An optimizing method named Five-Step Optimizing Method was established systematically by us aiming to improve the design effort of engineering parameters of water pumping wells in riverbank filtration. The maximum allowable drawdown of groundwater level (s_max) and the water demand of society (Q′) jointly constituted the constraint conditions. Three parameters including the number of wells (N), the distance between wells (D_ww) and the distance between well and river (D_wr) could be optimized through the established method step-by-step by screening the alternative values beforehand designed for the parameters. The interference between wells was found to be a decisive factor, which had a significant impact on the design and optimization effort of all the three parameters. D_wr was another decisive factor impacting the recharge from the river, and subsequently the well water yield. The optimized result would sometimes supply the decision maker with more than one optional scheme for selecting, while it is not yet difficult in practice to single out the optimal one considering both the field setting and the water demand.

A case study was carried out along the Songhua River in Harbin City of Heilongjiang Province of northeast China, whose setting generally met the assumptions of the method, aiming to illustrate the application and simultaneously verify the applicability of the method. Three schemes with different parameter values obtained from the optimizing method could meet the constraint conditions (s_max = 14 m; and Q′ = 2 × 10⁵ m³/d), out of which the optimal option had the parameters: N = 9; D_ww = 100 m; and D_wr = 50 m. Meanwhile, the other two schemes were valuable in certain circumstances, which should also be referred to the decision maker. This case study proved that the established method was applicable.

Admittedly, the method established in this study has some limitations, especially in the limitations of the assumptions. At the very least, however, this effort provides a relatively simple and operational approach to relevant practices and decisions.