Stormwater Reservoir Sizing in Respect of Uncertainty
Abstract
1. Introduction
2. Materials and Methods
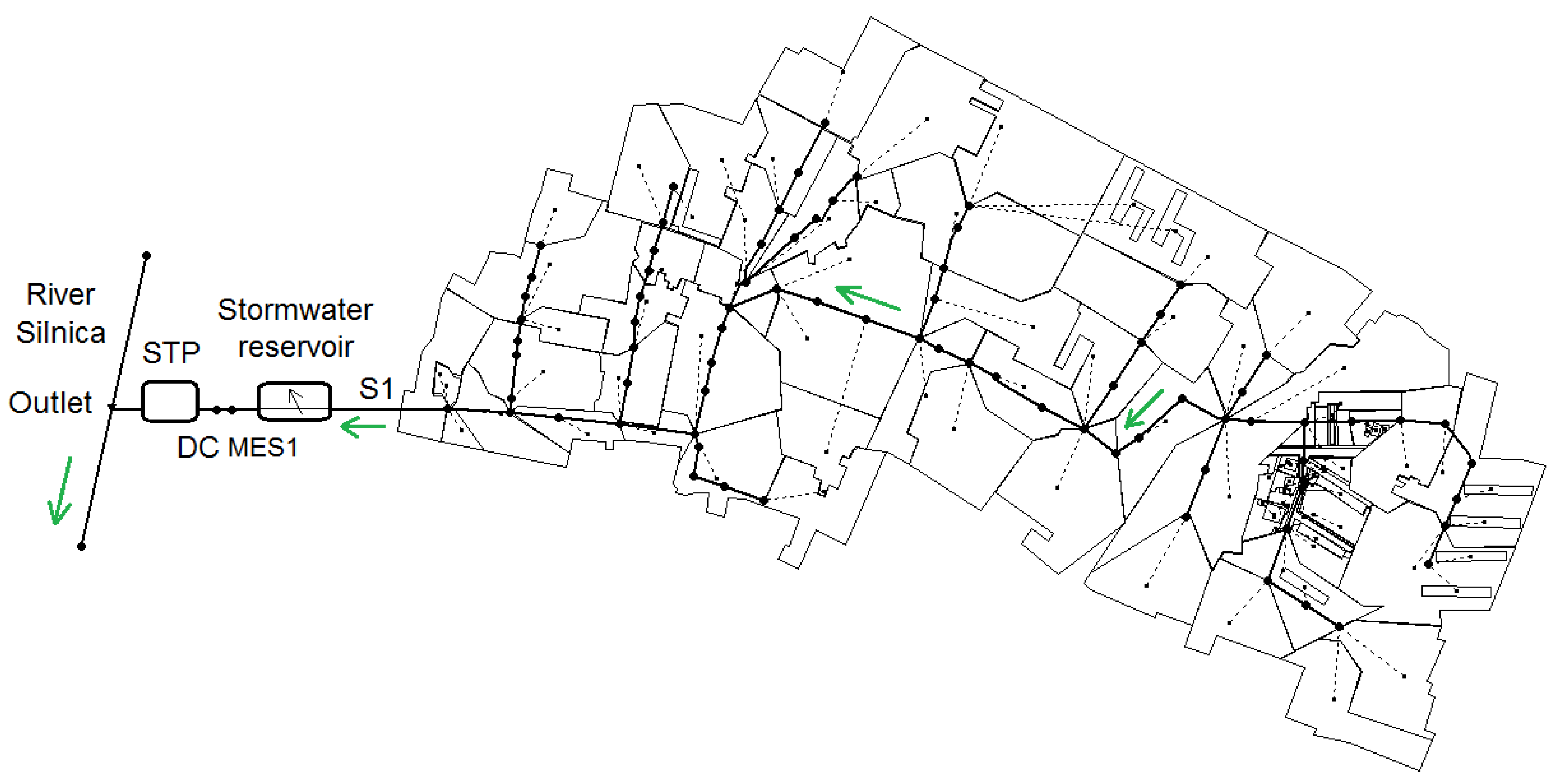
2.1. Object of Study
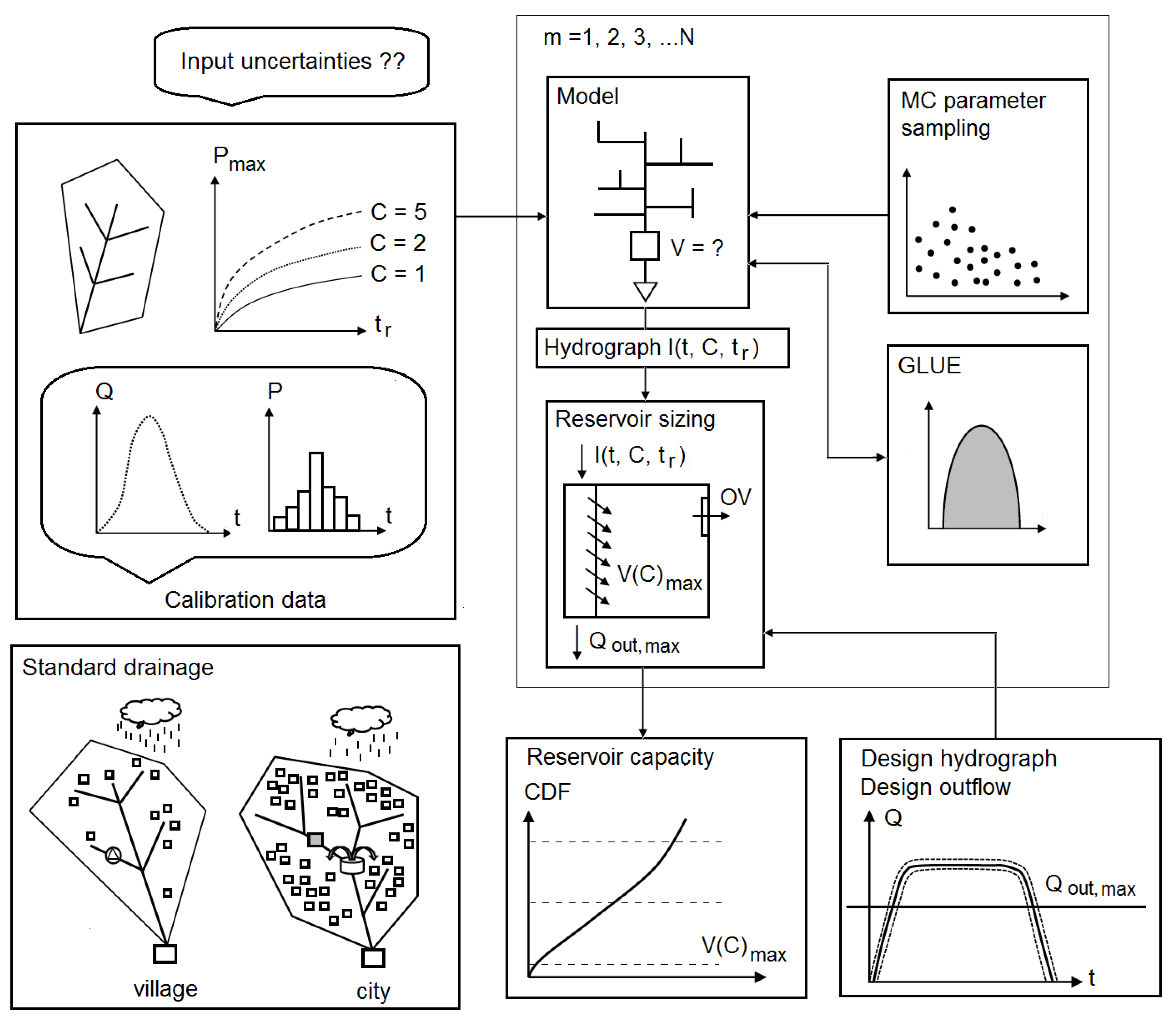
2.2. Methodology
2.3. Dimensioning the Retention Reservoir
2.4. Uncertainty Analysis by the GLUE Method
2.5. Rainfall Depth
2.6. Surface Runoff Modelling
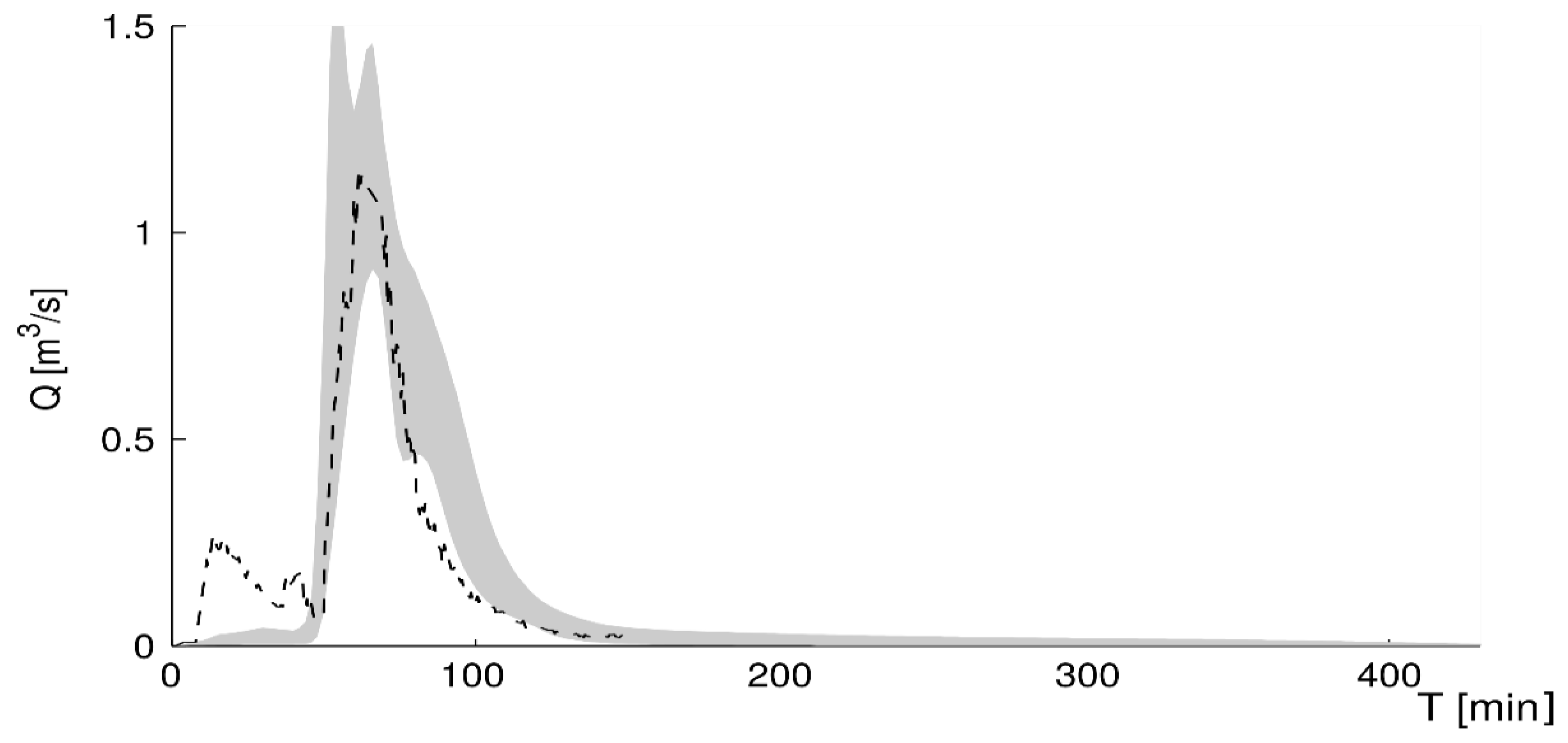
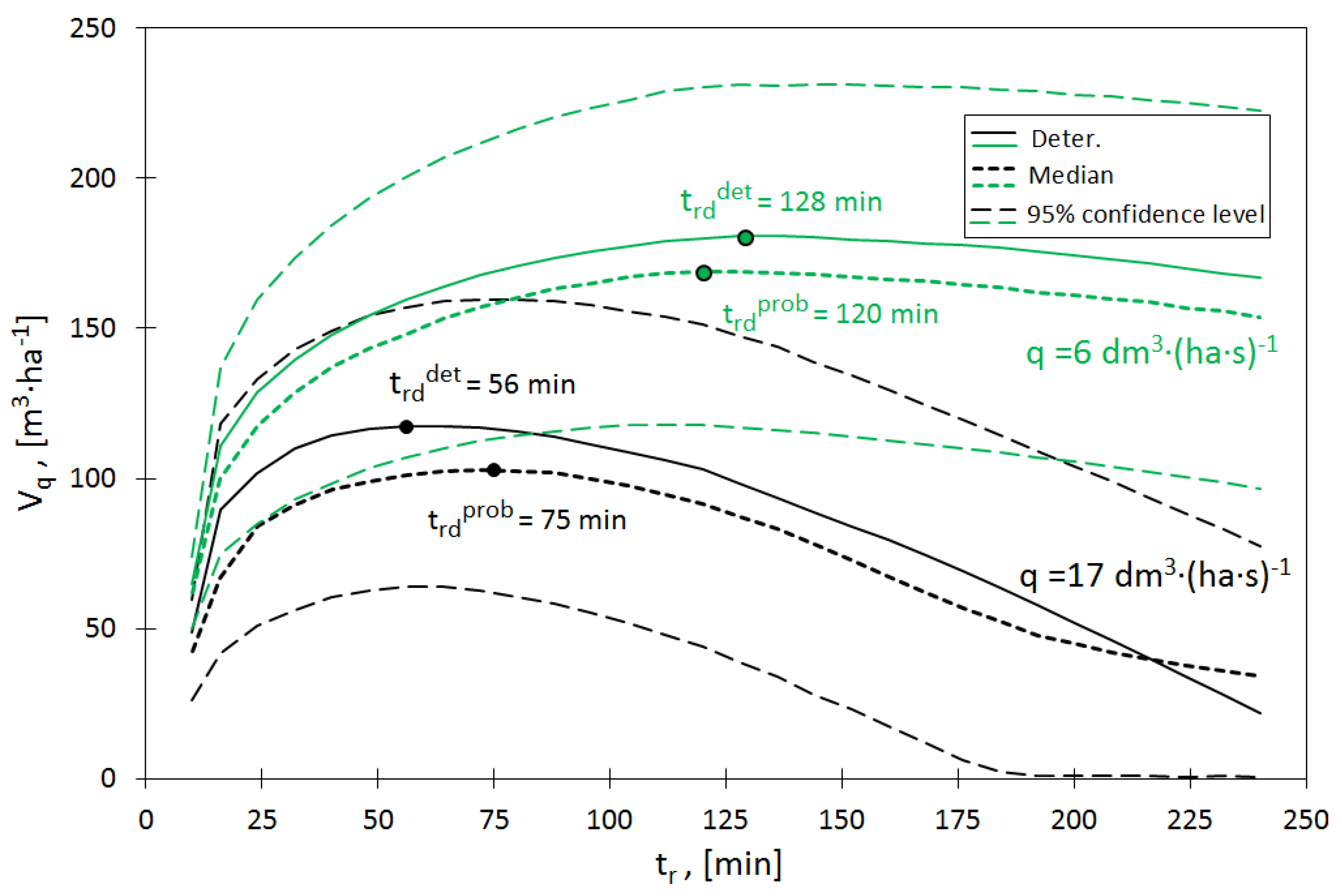
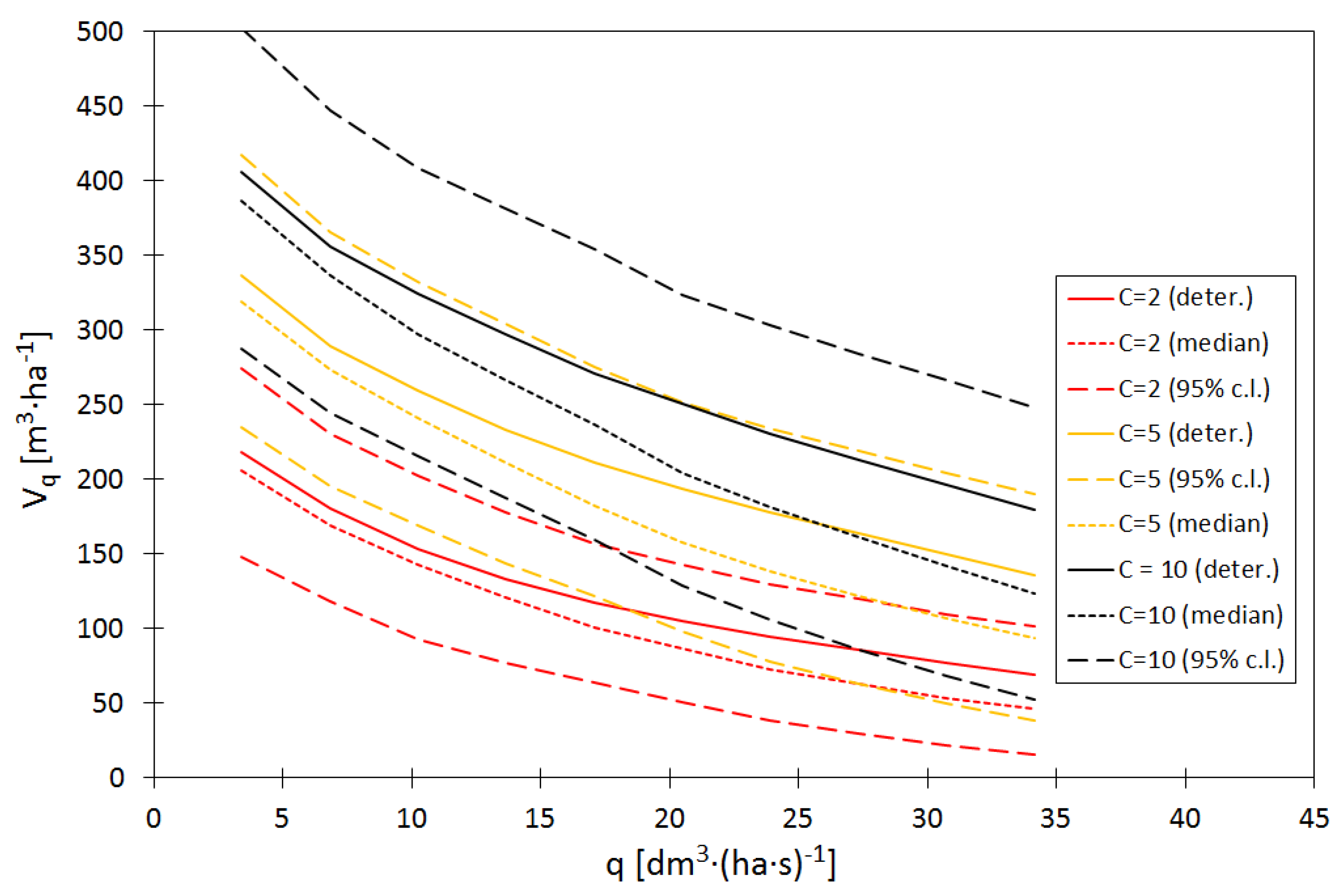
3. Results
4. Summary and Conclusions
Author Contributions
Funding
Conflicts of Interest
Abbreviations
| subcatchment area in the runoff model, ha; | |
| rainfall frequency; | |
| retention depth of impervious areas in the runoff model, mm; | |
| retention depth of pervious areas in the runoff model, mm; | |
| impermeable of the catchment, ha; | |
| reservoir depth, m; | |
| maximum reservoir depth, m; | |
| inflow to reservoir, m3·s−1; | |
| subcatchments slope in the runoff model; | |
| percentage impervious areas in the runoff model; | |
| likelihood function; | |
| roughness coefficient for impervious areas in the runoff model, m−1/3·s; | |
| roughness coefficient for sewer channels in the runoff model, m−1/3·s; | |
| roughness coefficient for pervious areas in the runoff model, m−1/3·s; | |
| denotes a priori parameter distribution; | |
| the a posteriori distribution; | |
| maximum rainfall depth, mm; | |
| rainfall depth in an episode, mm; | |
| probability of rainfall exceeding; | |
| outflow from stormwater reservoir, m3·s−1; | |
| maximum outflow from stormwater reservoir, m3·s−1 | |
| unit outflow from reservoir; dm3·(ha·s)−1 | |
| surface area of the reservoir in the projection, m2; | |
| rainfall duration, min; | |
| design rainfall event—deterministic solution, min; | |
| design rainfall event—probabilistic solution, min; | |
| unit capacity index, m3·ha−1; | |
| unit capacity index—deterministic solution, m3·ha−1; | |
| unit capacity index—probabilistic solution, m3·ha−1; | |
| volume of runoff, m3; | |
| flow path width in the runoff model, m; | |
| coefficient for flow path width in the runoff model; | |
| correction coefficient for the percentage of impervious areas; | |
| correction coefficient for subcatchments slope in the runoff model; | |
| the factor used to control the variance of the a posteriori distribution. |
References
- Paola, D.P.; Martino, D.F. Stormwater tank performance: Design and management criteria for capture tanks using a continuous simulation and a semi—probabilistic analytical approach. Water 2013, 5, 1699–1711. [Google Scholar] [CrossRef]
- Andrés-Doménech, I.; Montanari, A.; Marco, J.B. Stochastic rainfall analysis for storm tank performance evaluation. Hydrol. Earth Syst. Sci. 2010, 14, 1221–1232. [Google Scholar] [CrossRef]
- Water Framework Directive, European Union (WFD EU). Establishing a Framework for Community Action in the Field of Water Policy; Direcive 2000/60/EC of the European Parliament and the Council of 23 October 2000; EU: Brussels, Belgium, 2000. [Google Scholar]
- Kotowski, A.; Wartalska, K.; Nowakowska, M. Uogólniona metoda analityczna wymiarowania przelewowych zbiorników retencyjnych ścieków deszczowych [Generalized analytical methods of overfall storm water retention reservoir sizing]. Ochr. Srod. 2016, 38, 45–52. (In Polish) [Google Scholar]
- Dziopak, J.; Słyś, D. Modelowanie Zbiorników Klasycznych i Grawitacyjno—Pompowych w Kanalizacji [Classical and Pump—Gravitational Storage Reservoirs Modeling in Sewage Systems]; Oficyna Wydawnicza Politechniki Rzeszowskiej: Rzeszów, Poland, 2007. (In Polish) [Google Scholar]
- Hong, Y.M. Graphical estimation of detention pond volume for rainfall of short duration. J. Hydrol. Environ. Res. 2008, 2, 109–117. [Google Scholar] [CrossRef]
- DWA A-117. Bemessung von Regenrückhalteräumen; Deutsche Vereinigug für Wässerwirschaft, Abwasser und Abfalle e.V.: Hennef, Germany, 2006. (In German) [Google Scholar]
- Guo, J.C.Y. Hydrology-based approach to storm water detention design using new routing schemes. J. Hydrol. Eng. 2004, 9, 1–13. [Google Scholar] [CrossRef]
- Mrowiec, M. Efektywne Wymiarowanie i Dynamiczna Regulacja Kanalizacyjnych Zbiorników Retencyjnych [The Effective and Dimensioning and Dynamic Regulation Sewage Reservoirs]; Wydawnictwo Politechniki Częstochowskiej: Czętochowa, Poland, 2008; ISBN 978-83-7193-424-7. (In Polish) [Google Scholar]
- Wang, J.; Guo, J.C.Y. An analytical stochastic approach for evaluating the performance of combined sewer overflow tanks. Water Resour. Res. 2018, 54, 3357–3375. [Google Scholar] [CrossRef]
- DWA A-118E. Hydraulic Dimensioning and Verification of Drain and Sewer Systems; DWA German Association for Water, Wastewater and Waste: Hennef, Germany, 2006. (In German) [Google Scholar]
- Licznar, P. Wymiarowanie zbiorników retencyjnych ścieków deszczowych na podstawie syntetycznych szeregów czasowych opadów deszczów [Stormwater Reservoir Dimensioning Based on Synthetic Rainfall Time Series]. Ochr. Srod. 2013, 35, 27–32. (In Polish) [Google Scholar]
- Xie, J.; Wu, H.; Li, H.; Chen, G.A. Study on storm-water management of grassed swales and permeable pavement based on SWMM. Water 2017, 9, 840. [Google Scholar] [CrossRef]
- Strzec, M.; Dziopak, J.; Słyś, D.; Pochwat, K.; Kordana, S. Dimensioning of required volumes of interconnected detention tanks taking into account the direcion and speed of rain movement. Water 2018, 10, 1826. [Google Scholar] [CrossRef]
- Fu, G.; Butler, D. Copula-based frequency analysis of overflow and flooding in urban drainage systems. J. Hydrol. 2014, 510, 49–58. [Google Scholar] [CrossRef]
- Osorio, F.; Muhaisen, O.; García, P.A. Copula based simulation for the estimation of optimal volume for detention basin. J. Hydrol. Eng. 2009, 14, 1378–1382. [Google Scholar] [CrossRef]
- Vandenberghe, S.; Verhoest, N.; Buyse, E.; Baets, B. A stochastic design rainfall generator based on copulas and mass curves. Hydrol. Earth Syst. Sci. 2010, 14, 2429–2442. [Google Scholar] [CrossRef]
- Vernieuwe, H.; Vandenberghe, S.; Baets, B.; Verhoest, N. A continuous rainfall model based on vine copulas. Hydrol. Earth Syst. Sci. 2015, 19, 2685–2699. [Google Scholar] [CrossRef]
- Szeląg, B.; Kiczko, A.; Studziński, J.; Dąbek, L. Hydrodynamic and probabilistic modeling of storm overflow discharges. J. Hydroinform. 2018, 20, 1100–1110. [Google Scholar] [CrossRef]
- Burszta-Adamiak, E.; Licznar, P.; Zalewski, J. Criteria for identifying maximum rainfall rainfalls determined by peak-over-threshold (POT) method under the Polish Atlas of Rainfalls Intensities (PANDa) project. Meteorol. Hydrol. Water Manag. Res. Oper. Appl. 2018, 7, 3–13. [Google Scholar] [CrossRef]
- Tfwala, W.M.; Rensburg, L.D.; Schall, R.; Mosia, S.M.; Dlamini, P. Precipitation intensity-duration-fequency curves and their uncertainties for Ghaap plateau. Clim. Risk Manag. 2017, 16, 1–9. [Google Scholar] [CrossRef]
- Goncalves, M.L.R.; Zischg, J.; Rau, S.; Sitzmann, M.; Rauch, W.; Kleidorfer, M. Modelling the efect itroducting low impact development in a tropical city: A case study from Joinville, Brazil. Sustainability 2018, 10, 728. [Google Scholar] [CrossRef]
- Knighton, J.; White, E.; Lennon, E.; Rajan, R. Development of probability distributions for urban hydrologic model parameters and a Monte Carlo analysis of model sensitivity. Hydrol. Process. 2014, 28, 5131–5139. [Google Scholar] [CrossRef]
- Knighton, J.; Lennon, E.; Bastidas, L.; White, E. Stormwater detention system parameter sensitivity and uncertainty analysis using SWMM. J. Hydrol. Eng. 2016, 21, 05016014. [Google Scholar] [CrossRef]
- Sun, N.; Hong, B.; Hall, M. Assessment of the SWMM model uncertainties with generalized likelihood uncertainty estimation (GLUE) framework for a high-resolution urban sewershed. Hydrol. Process. 2014, 28, 3018–3034. [Google Scholar] [CrossRef]
- Zheng, Y.; Keller, A.A. Understaning parameter sensitivity and its management implications in watershed-scale water quality modeling. Water Resour. Res. 2006, 42, 1–14. [Google Scholar] [CrossRef]
- Kiczko, A.; Szeląg, B.; Kozioł, A.; Krukowski, M.; Kubrak, E.; Kubrak, J.; Romanowicz, J. Optimal capacity of a stormwater reservoir for flood peak reduction. J. Hydrol. Eng. 2018, 23, 1–9. [Google Scholar] [CrossRef]
- Beven, K.; Binley, A. The future distributed models: Model calibration and uncertainty prediction. Hydrol. Process. 1992, 6, 279–298. [Google Scholar] [CrossRef]
- Dąbkowki, S.L.; Górska, K.; Górski, J.; Szeląg, B. Wstępne wyniki badań ścieków deszczowych w jednym z kanałów w Kielcach [Introductory results of examining precipitation sewage in one Kielce channels]. Gaz Woda i Technika Sanitarna 2010, 34, 20–24. (In Polish) [Google Scholar]
- EN-752. Drain and Sewer Systems Outside Building—Part 4: Hydraulics Design and Environmental Cosideration; British Standards Institution (BSI): London, UK, 2017. [Google Scholar]
- Beven, K.; Binley, A. GLUE: 20 year on. Hydrol. Process. 2014, 28, 5897–5911. [Google Scholar] [CrossRef]
- Romanowicz, J.; Beven, K. Comments on generalized likelihood uncertainty estimation. Reliab. Eng. Syst. Safe 2006, 91, 1315–1321. [Google Scholar] [CrossRef]
- Bogdanowicz, E.; Stachy, J. Maksymalne Opady w Polsce—Charakterystyki Projektowe [Maximum Rainfall in Poland—Design Characteristics]; Oficyna Wydawnicza Instytu Meteorologii i Gospodarki Wodnej: Warszawa, Poland, 1998. (In Polish) [Google Scholar]
- Licznar, P.; Łomotowski, J. Analiza chwilowych natężeń deszczów miarodajnych we Wrocławiu [Analysis of the time frequncy distribution on maximal intensities of storms in the city of Wrocław]. Ochr. Srod. 2005, 27, 25–28. (In Polish) [Google Scholar]
- Licznar, P.; Siekanowicz-Grochowina, K.; Oktawiec, M.; Kotowski, A.; Burszta-Adamiak, E. Empiryczna weryfikacja formuły Błaszczyka do obliczania wartości natężenia deszczu miarodajnego [Empirical verfication of Błaszczyk’s formula for design rainfall intensity calculation]. Ochr. Srod. 2018, 40, 17–22. (In Polish) [Google Scholar]
- Rossmann, L.A. Storm Water Management Model User’s Manual; Version 5.0; National Risk Management Research Laboratory, Office of Research and Development, U.S. Environmental Protection Agency: Cincinatti, OH, USA, 2010.
- Szeląg, B.; Kiczko, A.; Dąbek, L. Analiza wrażliwości i niepewności modelu hydrodynamicznego (SWMM) do prognozowania odpływu wód opadowych ze zlewni zurbanizowanej—Studium przypadku [Sensitivity and uncertainty analysis of hydrodynamic model (SWMM) for storm water runoff forecating in an urban basin—A case study]. Ochr. Srod. 2016, 38, 15–22. (In Polish) [Google Scholar]
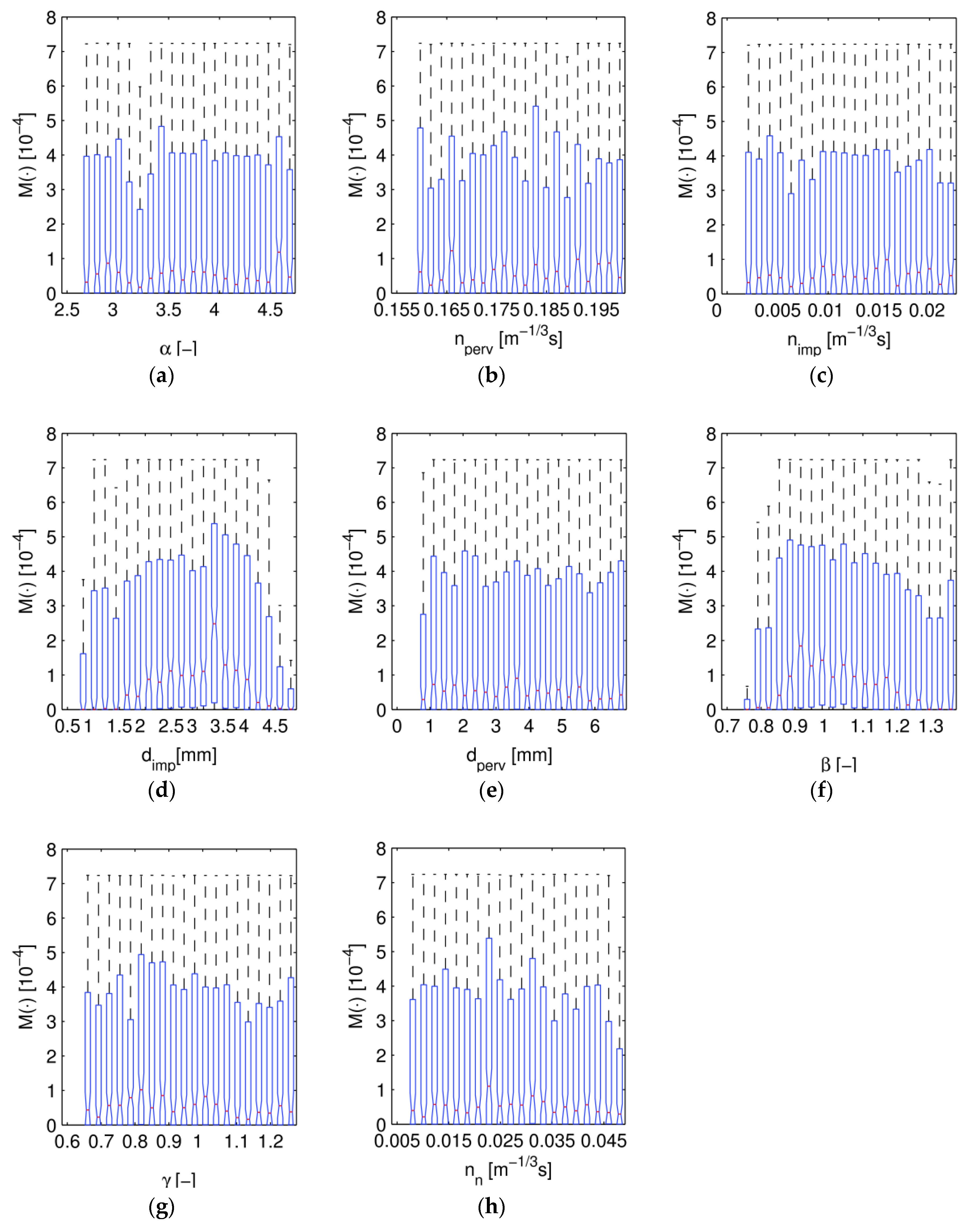


| Parameters | Unit | Range |
|---|---|---|
| Coefficient for flow path width (α) | - | 2.7–4.7 |
| Retention of impervious areas (dimp) | mm | 0.8–4.8 |
| Retention of pervious areas (dperv) | mm | 0.8–6.8 |
| Roughness coefficient for impervious areas (nimp) | m−1/3·s | 0.01–0.022 |
| Roughness coefficient for pervious areas (nperv) | m−1/3·s | 0.16–0.20 |
| Roughness coefficient of sewer channels (nn) | m−1/3·s | 0.01–0.048 |
| Correction coefficient for percentage of impervious areas (γ) | - | 0.7–1.275 |
| Correction coefficient for sub-catchments slope (β) | - | 0.8–1.375 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Szeląg, B.; Kiczko, A.; Dąbek, L. Stormwater Reservoir Sizing in Respect of Uncertainty. Water 2019, 11, 321. https://doi.org/10.3390/w11020321
Szeląg B, Kiczko A, Dąbek L. Stormwater Reservoir Sizing in Respect of Uncertainty. Water. 2019; 11(2):321. https://doi.org/10.3390/w11020321
Chicago/Turabian StyleSzeląg, Bartosz, Adam Kiczko, and Lidia Dąbek. 2019. "Stormwater Reservoir Sizing in Respect of Uncertainty" Water 11, no. 2: 321. https://doi.org/10.3390/w11020321
APA StyleSzeląg, B., Kiczko, A., & Dąbek, L. (2019). Stormwater Reservoir Sizing in Respect of Uncertainty. Water, 11(2), 321. https://doi.org/10.3390/w11020321






