Assessing Managed Aquifer Recharge Processes under Three Physical Model Concepts
Abstract
1. Introduction
2. Materials and Methods
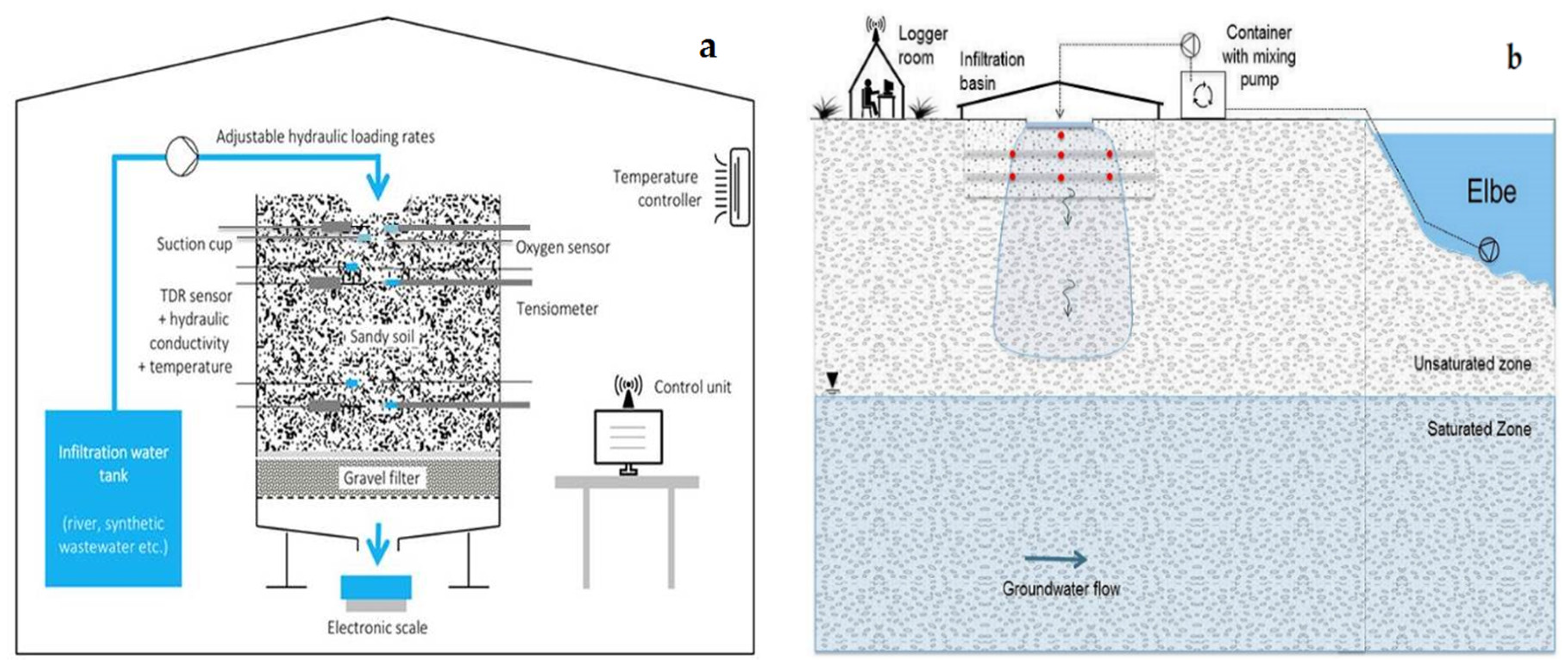
2.1. Experimental Setup
- -
- a cylindrical plastic 1D-column in the laboratory (Length (L) 1 m, Diameter (D) 0.15 m),
- -
- a rectangular-shaped, stainless steel 3D-infiltration tank in the laboratory (Length (L) 1.5 × Width (W) 1.0 × Hight (H) 1.0 m) and
- -
- a rectangular-shaped 3D-infiltration unit in the field without confinement (L 4.5 × W 3.0 × H 1.0 m)
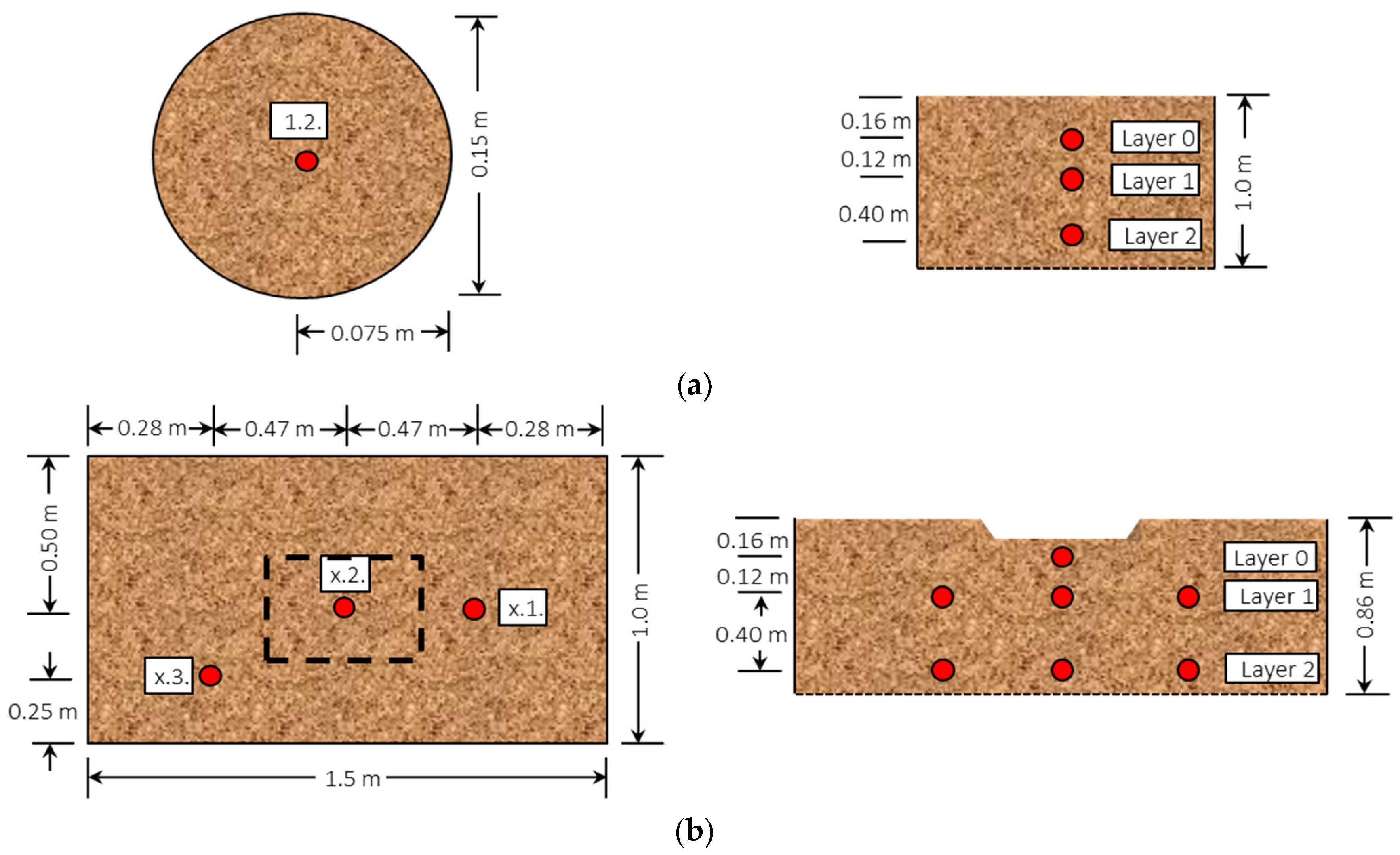
2.1.1. Installation of the Soil Material
2.1.2. Infiltration Area
2.1.3. Measurement Devices
2.1.4. Placement of Sensors
2.2. Operation System
2.3. Determination of Infiltration Capacity Reduction
3. Results and Discussion
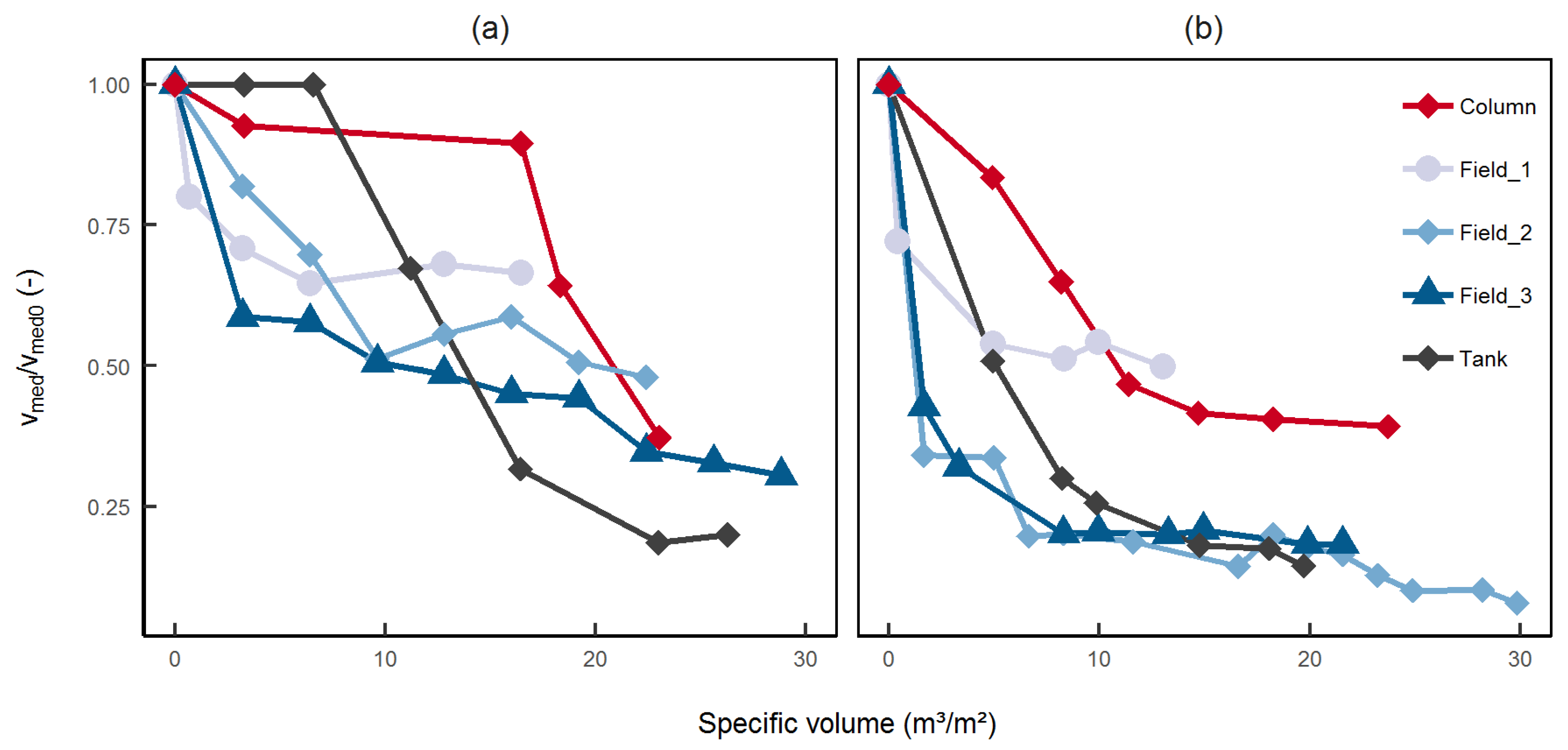
3.1. Infiltration Capacity Reduction
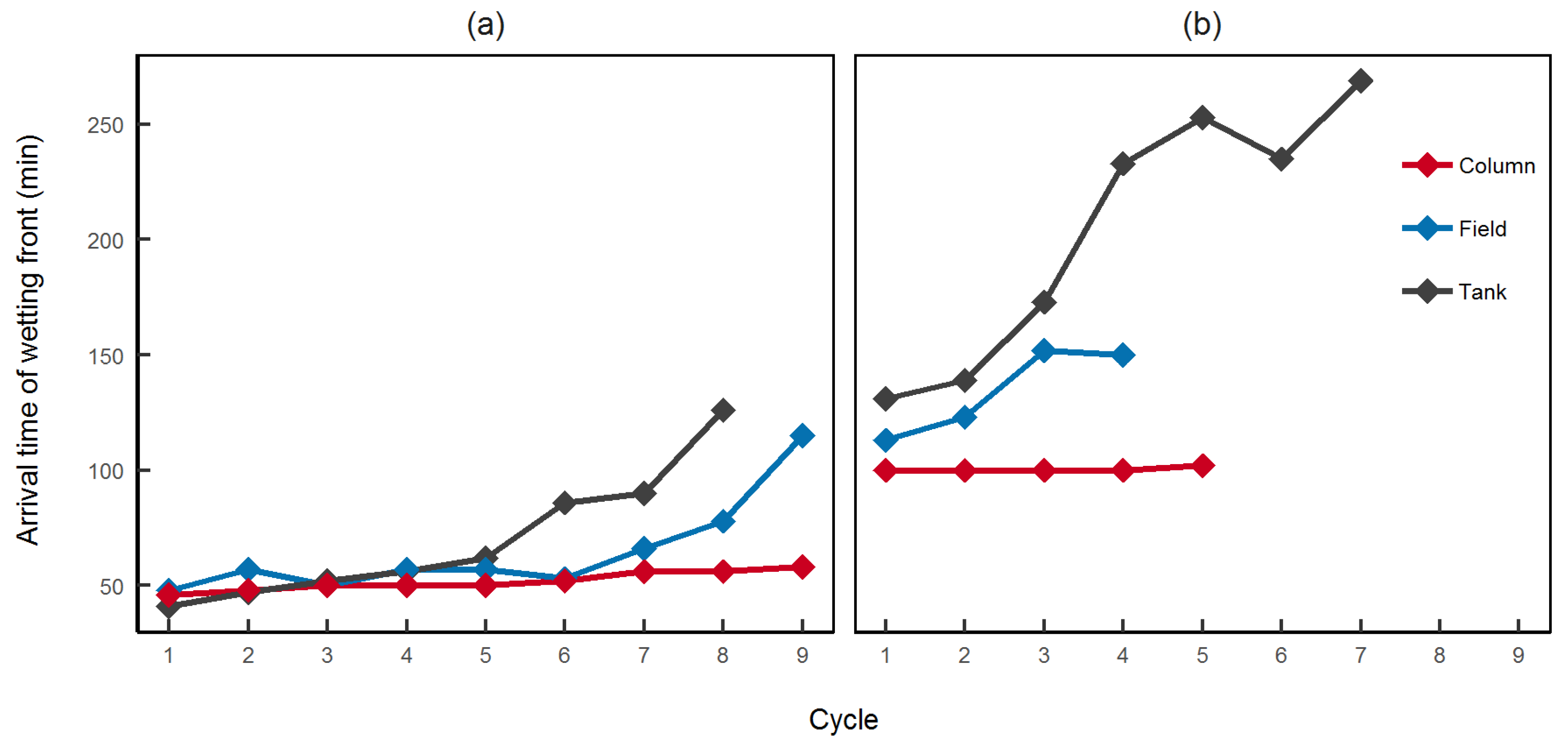
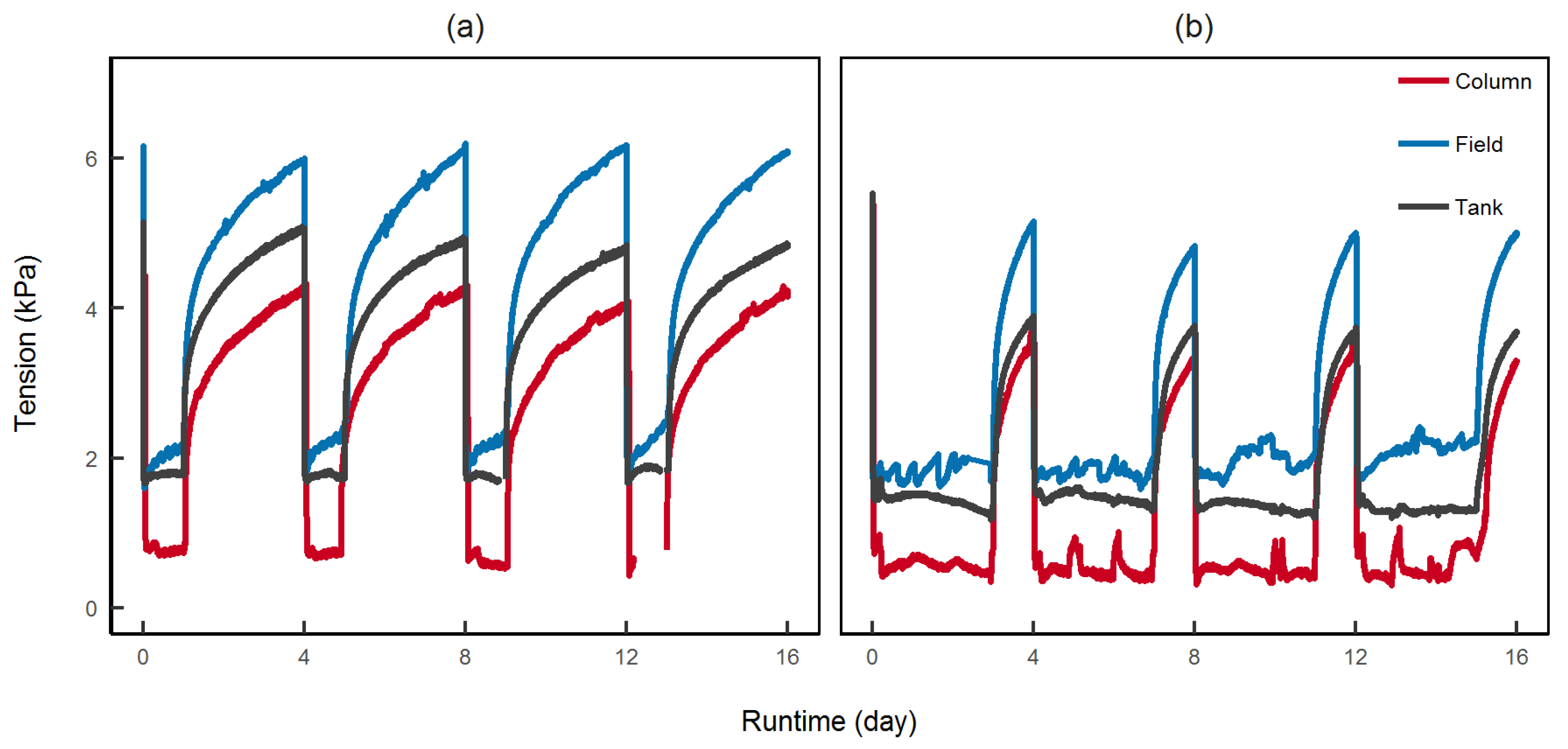
3.2. Water Flow
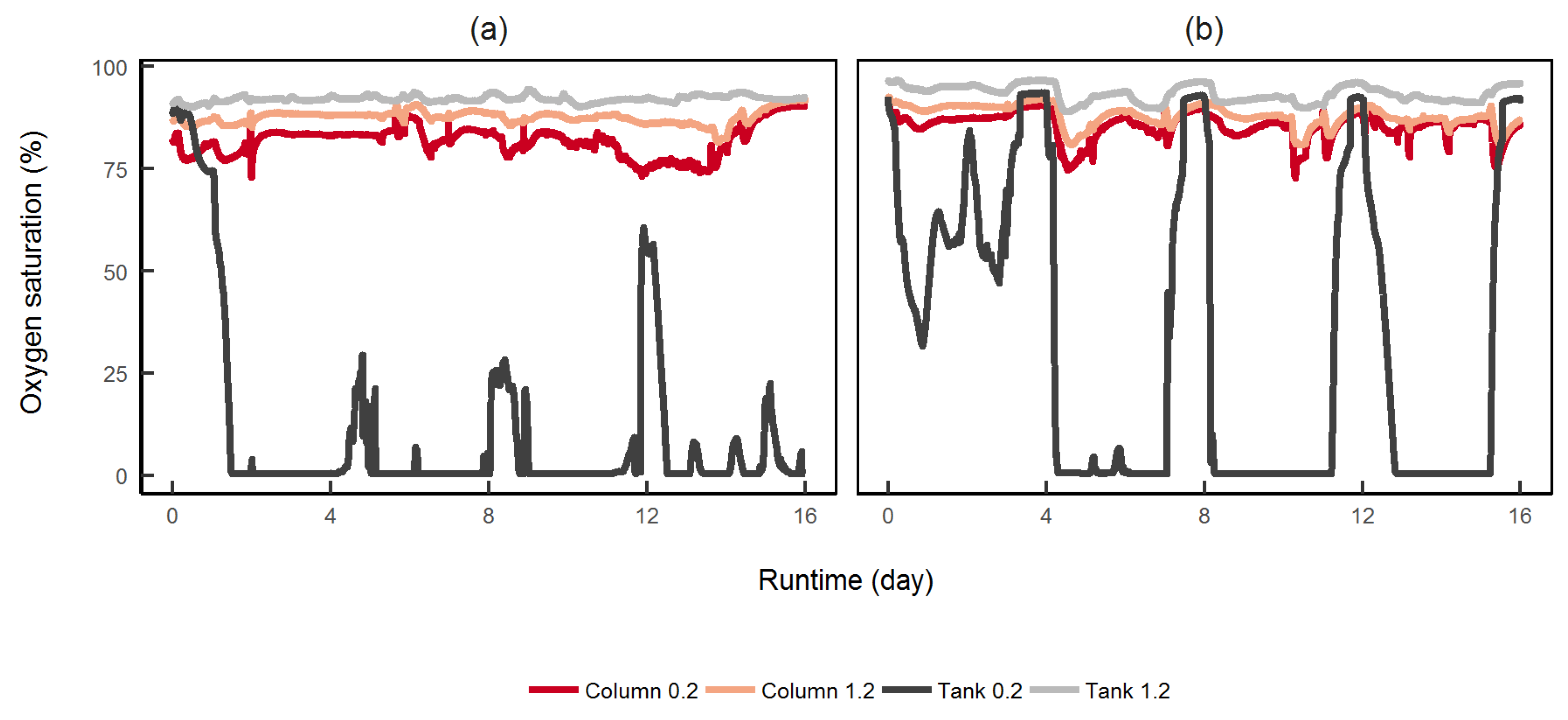
3.3. Oxygen Consumption
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Gleeson, T.; Wada, Y.; Bierkens, M.F.P.; van Beek, L.P.H. Water balance of global aquifers revealed by groundwater footprint. Nature 2012, 488, 197–200. [Google Scholar] [CrossRef]
- Esteller, M.V.; Diaz-Delgado, C. Environmental Effects of Aquifer Overexploitation: A Case Study in the Highlands of Mexico. Environ. Manag. 2002, 29, 266–278. [Google Scholar] [CrossRef]
- Malki, M.; Bouchaou, L.; Hirich, A.; Ait Brahim, Y.; Choukr-Allah, R. Impact of agricultural practices on groundwater quality in intensive irrigated area of Chtouka-Massa, Morocco. Sci. Total Environ. 2017, 574, 760–770. [Google Scholar] [CrossRef] [PubMed]
- Marston, L.; Konar, M.; Cai, X.; Troy, T.J. Virtual groundwater transfers from overexploited aquifers in the United States. PNAS 2015, 112, 8561–8566. [Google Scholar] [CrossRef] [PubMed]
- Pophare, A.M.; Lamsoge, B.R.; Katpatal, Y.B.; Nawale, V.P. Impact of over-exploitation on groundwater quality: A case study from WR-2 Watershed, India. J. Earth Syst. Sci. 2014, 123, 1541–1566. [Google Scholar] [CrossRef]
- Salameh, E. Over-exploitation of groundwater resources and their environmental and socio-economic implications: the case of Jordan. Water Int. 2008, 33, 55–68. [Google Scholar] [CrossRef]
- Caballero, Y.; Ladouche, B. Impact of climate change on groundwater in a confined Mediterranean aquifer. Hydrol. Earth Syst. Sci. Discuss. 2015, 12, 10109–10156. [Google Scholar] [CrossRef]
- Kahsay, K.D.; Pingale, S.M.; Hatiye, S.D. Impact of climate change on groundwater recharge and base flow in the sub-catchment of Tekeze basin, Ethiopia. Groundwater Sustain. Dev. 2018, 6, 121–133. [Google Scholar] [CrossRef]
- Kumar, C.P. GRIN-Impact of Climate Change on Groundwater Resources; GRIN: Munich, Germany, 2014. [Google Scholar]
- Salem, G.S.A.; Kazama, S.; Shahid, S.; Dey, N.C. Impacts of climate change on groundwater level and irrigation cost in a groundwater dependent irrigated region. Agric. Water Manag. 2018, 208, 33–42. [Google Scholar] [CrossRef]
- Bagher, R.M.; Rasoul, M. Effect of Groundwater Table Decline on Groundwater Quality in Sirjan Watershed. Arab. J. Sci. Eng. 2010, 35, 197–212. [Google Scholar]
- Lashkaripour, G.R.; Ghafoori, M. The Effects of Water Table Decline on the Groundwater Quality in Aquifer of Torbat Jam Plain, Northeast Iran. Int. J. Emerg. Sci. 2011, 1, 153–163. [Google Scholar]
- Pettenati, M.; Perrin, J.; Pauwels, H.; Ahmed, S. Simulating fluoride evolution in groundwater using a reactive multicomponent transient transport model: Application to a crystalline aquifer of Southern India. Appl. Geochem. 2013, 29, 102–116. [Google Scholar] [CrossRef]
- Aeschbach-Hertig, W.; Gleeson, T. Regional strategies for the accelerating global problem of groundwater depletion. Nat. Geosci. 2012, 5, 853–861. [Google Scholar] [CrossRef]
- Hoekstra, A.Y.; Mekonnen, M.M.; Chapagain, A.K.; Mathews, R.E.; Richter, B.D. Global Monthly Water Scarcity: Blue Water Footprints versus Blue Water Availability. PLOS ONE 2012, 7, e32688. [Google Scholar] [CrossRef]
- Kinzelbach, W.; Aeschbach, W.; Alberich, C.; Goni, I.B.; Beyerle, U.; Brunner, P.; Chiang, W.H.; Rueedi, J.; Zoellmann, K. A Survey of Methods for Groundwater Recharge in Arid and Semi-Arid Regions; United Nations Environment Programme: Nairobi, Kenya, 2002; p. 107. [Google Scholar]
- Dillon, P.; Pavelic, P.; Page, D.; Behringen, H.; Ward, J. Managed Aquifer Recharge: An Introduction; Waterlines Report Series; National Water Commission: Canberra, Australia, 2009; p. 77. [Google Scholar]
- Hannappel, S.; Scheibler, F.; Huber, A.; Sprenger, C. Characterization of European Managed Aquifer Recharge (MAR) Sites—Analysis; Demeau research project: Dübendorf, Switzerland, 2014; p. 141. [Google Scholar]
- Bouwer, H. Issues in artificial recharge. Water Sci. Technol. 1996, 33, 381–390. [Google Scholar] [CrossRef]
- Russel, M. (Ed.) Clogging Issues Associated with Managed Aquifer Recharge Methods; IAH Commission on Managing Aquifer Recharge: Mount Gambier, Australia, 2013; ISBN 978-0-646-90852-6. [Google Scholar]
- Sprenger, C.; Hartog, N.; Hernández, M.; Vilanova, E.; Grützmacher, G.; Scheibler, F.; Hannappel, S. Inventory of managed aquifer recharge sites in Europe: historical development, current situation and perspectives. Hydrogeol. J. 2017, 25, 1909–1922. [Google Scholar] [CrossRef]
- Dillon, P.; Vanderzalm, J.; Page, D.; Barry, K.; Gonzalez, D.; Muthukaruppan, M.; Hudson, M. Analysis of ASR Clogging Investigations at Three Australian ASR Sites in a Bayesian Context. Water 2016, 8, 442. [Google Scholar] [CrossRef]
- Bekele, E.B.; Donn, M.J.; Barry, K.E.; Vanderzalm, J.L.; Kaksonen, A.H.; Puzon, G.J.; Wylie, J.; Miotlinski, K.; Cahill, K.; Walsh, T.; et al. Managed Aquifer Recharge and RecyclingOptions: Understanding Clogging Processes and Water Quality Impacts; Australian Water recycling center of Excellence: Brisbane, Australia, 2015. [Google Scholar]
- Siriwardene, N.; Deletic, A.; Fletcher, T. Clogging of stormwater gravel infiltration systems and filters: Insights from a laboratory study. Water Res. 2007, 41, 1433–1440. [Google Scholar] [CrossRef]
- Azaroual, M.; Thiéry, D.; Amraoui, N.; Pettenati, M.; Croiset, N.; Casanova, J.; Besnard, K.; Rampnoux, N. Physical, geochemical and microbial processes induced during the aquifer recharge using treated wastewaters: laboratory and pilot experiments and numerical simulations. In Achieving Groundwater Supply Sustainability & Reliability through Managed Aquifer Recharge—Proceedings of the Symosium ISMAR 7, 9–13 October 2009, Abu Dhabi, UAE; Draeger, M., Ed.; Managed Aquifer Recharge: Abu Dhabi, UAE, 2012; pp. 170–177. [Google Scholar]
- Hudson, M.; Muthukaruppan, M. Meeting Melbourne’s future demand for water using aquifer storage and recovery. In Proceedings of the 9th International Symposium on Managed Aquifer Recharge, Mexico City, Mexico, 20–24 June 2016. [Google Scholar]
- Pavelic, P.; Dillon, P.J.; Mucha, M.; Nakai, T.; Barry, K.E.; Bestland, E. Laboratory assessment of factors affecting soil clogging of soil aquifer treatment systems. Water Res. 2011, 45, 3153–3163. [Google Scholar] [CrossRef]
- Rinck-Pfeiffer, S.; Ragusa, S.; Sztajnbok, P.; Vandevelde, T. Interrelationships between biological, chemical, and physical processes as an analog to clogging in aquifer storage and recovery (ASR) wells. Water Res. 2000, 34, 2110–2118. [Google Scholar] [CrossRef]
- Taylor, S.W.; Jaffé, P.R. Biofilm growth and the related changes in the physical properties of a porous medium: 1. Experimental investigation. Water Resour. Res. 1990, 26, 2153–2159. [Google Scholar]
- Awedat, A.M. Effect of Dispersed Clay and Soil Pore Size on the Hydraulic Conductivity of Soils Irrigated with Saline-Sodic Water. Ph.D. Thesis, University of Southern Queensland, Toowoomba, Australia, 2014; p. 119. [Google Scholar]
- Can, Ö.; Balköse, D.; Ülkü, S. Batch and column studies on heavy metal removal using a local zeolitic tuff. Desalination 2010, 259, 17–21. [Google Scholar] [CrossRef]
- Fakhreddine, S.; Dittmar, J.; Phipps, D.; Dadakis, J.; Fendorf, S. Geochemical Triggers of Arsenic Mobilization during Managed Aquifer Recharge. Environ. Sci. Technol. 2015, 49, 7802–7809. [Google Scholar] [CrossRef] [PubMed]
- Fey, M.V. Clay dispersion and hydraulic conductivity of clay-sand mixtures as affected by the addition of various anions. Clays Clay Miner. 1992, 40, 7. [Google Scholar]
- Schweich, D.; Sardin, M. Adsorption, partition, ion exchange and chemical reaction in batch reactors or in columns—A review. J. Hydrol. 1981, 50, 1–33. [Google Scholar] [CrossRef]
- Test No. 106: Adsorption—Desorption Using a Batch Equilibrium Method. Available online: https://www.oecd-ilibrary.org/environment/test-no-106-adsorption-desorption-using-a-batch-equilibrium-method_9789264069602-en (accessed on 21 December 2018).
- Page, D.; Bekele, E.; Vanderzalm, J.; Sidhu, J. Managed Aquifer Recharge (MAR) in Sustainable Urban Water Management. Water 2018, 10, 239. [Google Scholar] [CrossRef]
- Vanderzalm, J.L.; Dillon, P.J.; Barry, K.E.; Miotlinski, K.; Kirby, J.K.; Le Gal La Salle, C. Arsenic mobility and impact on recovered water quality during aquifer storage and recovery using reclaimed water in a carbonate aquifer. Appl. Geochem. 2011, 26, 1946–1955. [Google Scholar] [CrossRef]
- Vanderzalm, J.; Sidhu, J.; Bekele, E.; Ying, G.-G.; Pavelic, P.; Toze, S.; Dillon, P.; Kookana, R.; Hanna, J.; Barry, K.; et al. Water Quality Changes During Aquifer Storage and Recovery; Web Report #2974; Water Research Foundation: Denver, FL, USA, 2009. [Google Scholar]
- Bustos Medina, D.A.; van den Berg, G.A.; van Breukelen, B.M.; Juhasz-Holterman, M.; Stuyfzand, P.J. Iron-hydroxide clogging of public supply wells receiving artificial recharge: near-well and in-well hydrological and hydrochemical observations. Hydrogeol. J. 2013, 21, 1393–1412. [Google Scholar] [CrossRef]
- Thullner, M. Comparison of bioclogging effects in saturated porous media within one- and two-dimensional flow systems. Ecol. Eng. 2010, 36, 176–196. [Google Scholar] [CrossRef]
- Hijnen, W.A.M.; Van der Koou, D. The effect of low concentrations of assimilable organic carbon (AOC) in water on biological clogging of sand beds. Water Res. 1992, 26, 963–972. [Google Scholar] [CrossRef]
- Seki, K.; Thullner, M.; Hanada, J.; Miyazaki, T. Moderate Bioclogging Leading to Preferential Flow Paths in Biobarriers. Ground Water Monit. Remediat. 2006, 26, 68–76. [Google Scholar]
- Sentenac, P.; Lynch, R.J.; Bolton, M.D. Measurement of a side-wall boundary effect in soil columns using fibre-optics sensing. Int. J. Phys. Model. Geotech. 2015. [Google Scholar] [CrossRef]
- Saffigna, P.G.; Keeney, D.R. Nitrate and chloride in groundwater under irrigated agriculture in Central Wisconsin. Groundwater 1977, 15. [Google Scholar] [CrossRef]
- Till, A.R.; McCabe, T.P. Sulfur leaching and lysimeter characterization. Soil Sci. 1976, 122, 44–47. [Google Scholar] [CrossRef]
- Pavelic, P.; Dillon, P.J.; Barry, K.E.; Vanderzalm, J.L.; Correll, R.L.; Rinck-Pfeiffer, S.M. Water quality effects on clogging rates during reclaimed water ASR in a carbonate aquifer. J. Hydrol. 2007, 334, 1–16. [Google Scholar] [CrossRef]
- Xia, L.; Gao, Z.; Zheng, X.; Wei, J. Impact of recharge water temperature on bioclogging during managed aquifer recharge: a laboratory study. Hydrogeol. J. 2018, 26, 2173–2187. [Google Scholar] [CrossRef]
- Schuh, W.M. Seasonal variation of clogging of an artificial recharge basin in a northern climate. J. Hydrol. 1990, 121, 193–215. [Google Scholar] [CrossRef]
- Jaynes, D.B. Temperature Variations Effect on Field-Measured Infiltration. Soil Sci. Soc. Am. J. 1990, 54, 305–312. [Google Scholar] [CrossRef]
- Vandenbohede, A.; Van Houtte, E. Heat transport and temperature distribution during managed artificial recharge with surface ponds. J. Hydrol. 2012, 472–473, 77–89. [Google Scholar] [CrossRef]
- Heilweil, V.M.; Solomon, D.K.; Ortiz, G. Silt and gas accumulation beneath an artificial recharge spreading basin, Southwestern Utah, U.S.A. Boletin Geologico y Minero 2009, 120, 12. [Google Scholar]
- Lin, C.; Greenwald, D.; Banin, A. Temperature dependence of infiltration rate during large scale water recharge into soils. Soil Sc. Soc. Am. J. 2003, 67, 487. [Google Scholar] [CrossRef]
- German institute for standardization. DIN 4220 Pedologic Site Assessment-Designation, Classification and Deduction of Soil Parameters (Normative and Nominal Scaling); German Institute for Standardization: Berlin, Germany, 2017; p. 50. [Google Scholar]
- Šimůnek, J.; van Genuchten, M.T.; Šejna, M. Recent Developments and Applications of the HYDRUS Computer Software Packages. Vadose Zone J. 2016, 15. [Google Scholar] [CrossRef]
- Sallwey, J.; Glass, J.; Stefan, C. Utilizing unsaturated soil zone models for assessing managed aquifer recharge. Sustain. Water Resour. Manag. 2018, 4, 383–397. [Google Scholar] [CrossRef]
- Bagarello, V.; Castellini, M.; Di Prima, S.; Giordano, G.; Iovino, M. Testing a Simplified Approach to Determine Field Saturated Soil Hydraulic Conductivity. Procedia Environ. Sci. 2013, 19, 599–608. [Google Scholar] [CrossRef]
- Fodor, N.; Sándor, R.; Orfanus, T.; Lichner, L.; Rajkai, K. Evaluation method dependency of measured saturated hydraulic conductivity. Geoderma 2011, 165, 60–68. [Google Scholar] [CrossRef]
- Angulo-Jaramillo, R.; Bagarello, V.; Iovino, M.; Lassabatere, L. Infiltration Measurements for Soil Hydraulic Characterization; Springer: Berlin, Germany, 2016; ISBN 978-3-319-31786-1. [Google Scholar]
- Reynolds, W.D.; Elrick, D.E. Ponded Infiltration From a Single Ring: I. Analysis of Steady Flow. Soil Sci. Soc. Am. J. 1990, 54, 1233–1241. [Google Scholar] [CrossRef]
- Nimmo, J.R.; Schmidt, K.M.; Perkins, K.S.; Stock, J.D. Rapid measurement of field-saturated hydraulic conductivity for areal characterization. Vadose Zone J. 2009, 8, 8. [Google Scholar] [CrossRef]
- Irving, J.; Singha, K. Stochastic inversion of tracer test and electrical geophysical data to estimate hydraulic conductivities. Water Resour. Res. 2010, 46. [Google Scholar] [CrossRef]
- Pollock, D.; Cirpka, O.A. Fully coupled hydrogeophysical inversion of synthetic salt tracer experiments. Water Resour. Res. 2010, 46. [Google Scholar] [CrossRef]


| Scenario | Wet/Dry Ratio = HLC (-) | Climate | |||
|---|---|---|---|---|---|
| Lab Scale Infiltration Units | Field Scale Infiltration Unit | ||||
| 1 2 3 4 | 1:3 (24 h/72 h) 1:1 (168 h/168 h) 1:- (648 h:0 h) 3:1 (72 h/24 h) | 2 (mild) Temp. 17 °C Humidity 70% | 1 (cold) Temp. < 10 °C Humidity 85% Solar irradiance < 34 W/h | 2 (mild) Temp. 10 to 17 °C Humidity 83% Solar irradiance 34 to 230 W/h | 3 (warm) Temp. > 18 °C Humidity 79% Solar irradiance > 230 W/h |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fichtner, T.; Barquero, F.; Sallwey, J.; Stefan, C. Assessing Managed Aquifer Recharge Processes under Three Physical Model Concepts. Water 2019, 11, 107. https://doi.org/10.3390/w11010107
Fichtner T, Barquero F, Sallwey J, Stefan C. Assessing Managed Aquifer Recharge Processes under Three Physical Model Concepts. Water. 2019; 11(1):107. https://doi.org/10.3390/w11010107
Chicago/Turabian StyleFichtner, Thomas, Felix Barquero, Jana Sallwey, and Catalin Stefan. 2019. "Assessing Managed Aquifer Recharge Processes under Three Physical Model Concepts" Water 11, no. 1: 107. https://doi.org/10.3390/w11010107
APA StyleFichtner, T., Barquero, F., Sallwey, J., & Stefan, C. (2019). Assessing Managed Aquifer Recharge Processes under Three Physical Model Concepts. Water, 11(1), 107. https://doi.org/10.3390/w11010107






