Calculation of Comprehensive Ecological Flow with Weighted Multiple Methods Considering Hydrological Alteration
Abstract
:1. Introduction
2. Materials and Methods
2.1. Study Area
2.2. Methodology
2.2.1. Evaluation of the Intra-Annual Runoff Distribution Degrees of Evenness
- (1)
- Group and sort historical monthly flow data. Divide the 12 monthly flows in the ith year into a group, and sort the monthly flows into a group in ascending order. The ascending runoff series of the ith year is , where i represents the ith year, .
- (2)
- Accumulate the ascending runoff data in each group:where k is the ranking order.
- (3)
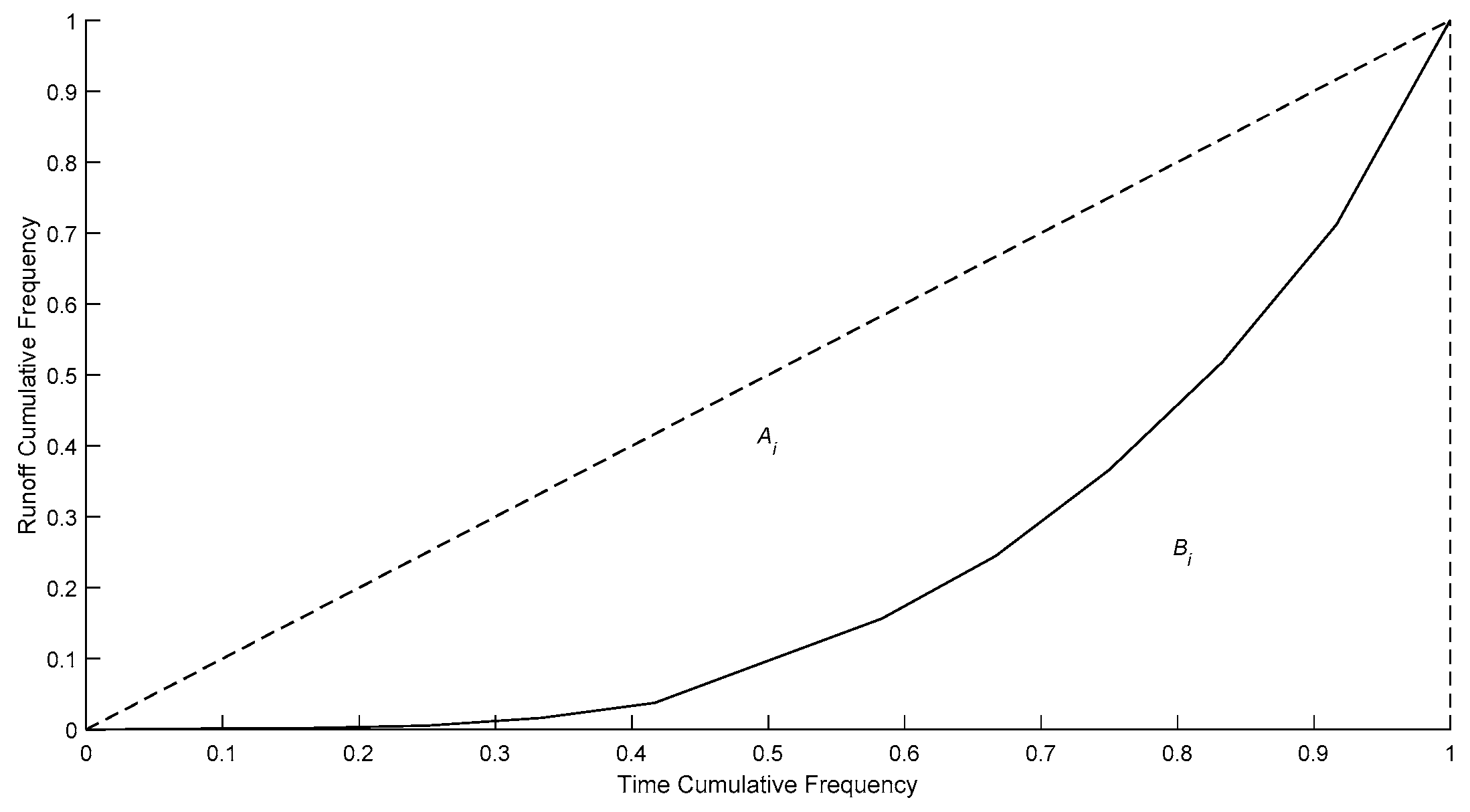
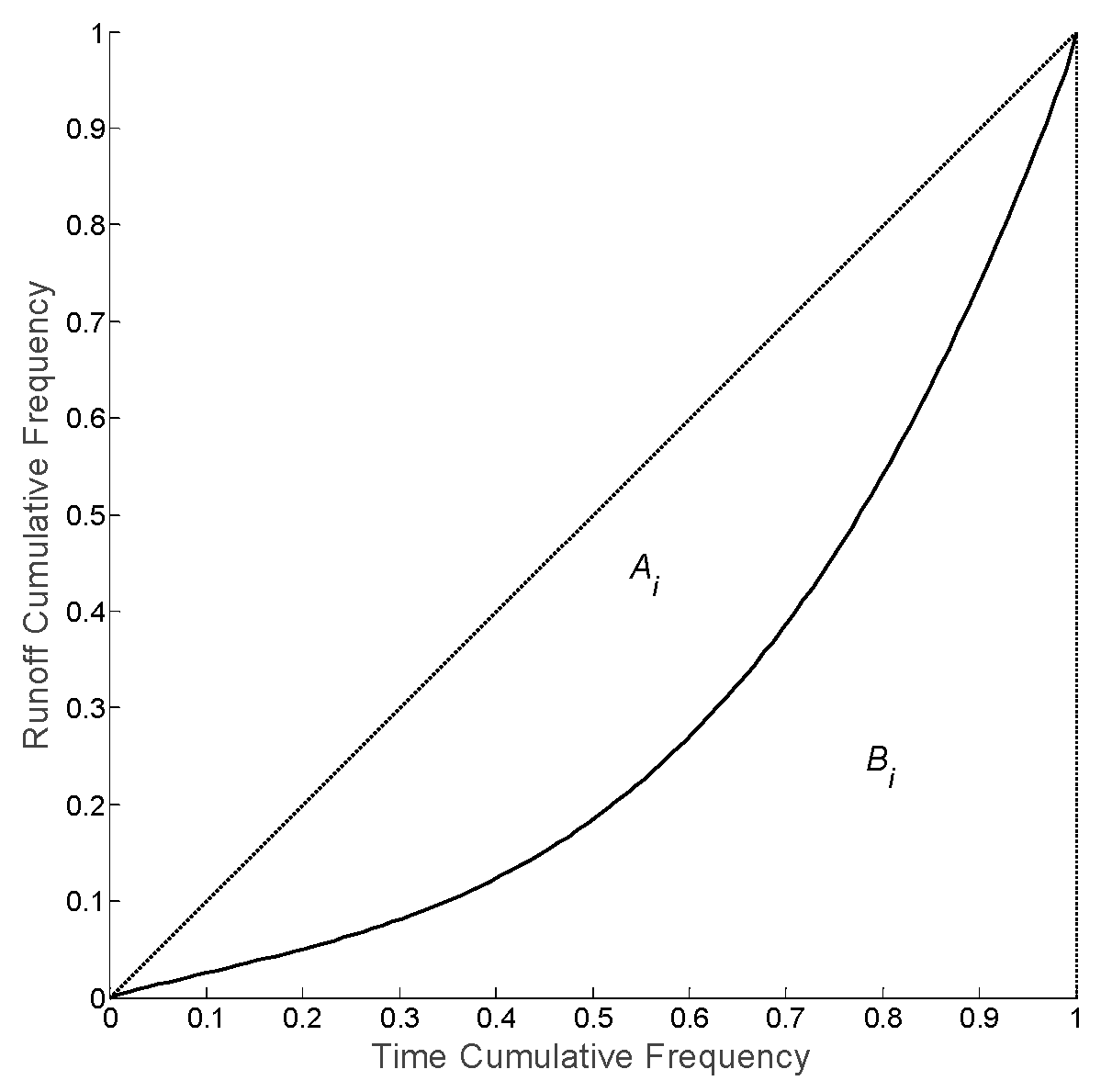
- Draw a Lorenz curve of the annual distribution for monthly flow. Take k/12 as the abscissa, as the ordinate, and draw a Lorenz curve of the annual distribution for monthly flow. The Lorenz curve is shown in Figure 3.
- (4)
- The ith year runoff Gini coefficient GIi is computed as:where SAi and SBi are the area acreages of Ai and Bi, respectively.
2.2.2. Hydrological Alteration Diagnosis System
2.2.3. Ecological Flow Calculation Method
- In the range of the variability approach (RVA) method, ecological flow calculations should consider the hydrological regime. Parameters of the indicators of the hydrologic alteration (IHA) method are all closely related to runoff, and monthly flow influences river-living organisms, soil, etc. Therefore, the RVA analyses the monthly flow frequency distribution of each month and selects the flow corresponding to 25% and 75% in the frequency distribution as the upper and lower limits of the monthly flow, respectively [26]. Ecological flow is calculated as:where is the ecological flow of the jth month, is monthly mean flow of the jth month, is the upper limit of RVA, and is the lower limit of RVA.
- The steps for ecological flow calculation using the monthly frequency method (MFM) are as follows:
- (1)
- Calculate the monthly flow distribution empirical frequency of the jth month.
- (2)
- Select the probability distribution function and draw the monthly flow distribution theoretical frequency curve of the jth month. The Pearson III distribution curve and generalized extreme value (GEV) distribution curve are commonly used. The GEV distribution curve is better fitting with the runoff data [27].
- (3)
- Select the flow corresponding to the maximum frequency in the GEV distribution curve as the ecological flow of the jth month [28].
- The annual daily mean flow frequency method (ADMFFM) assumes that daily flow may appear in a month with a certain probability. The frequency of daily flow in the jth month of the historical runoff series is calculated and the daily flow corresponding to 60% is selected as the ecological flow [24] of the jth month, . The ecological flow calculated using this method meets the ecological water requirements in different cases.
- In the comprehensive ecological flow calculation method, ecological flow maintains riverine ecological function. Too much or too little will affect the riverine ecosystem. Therefore, the weights of each ecological flow calculation method are used to comprehensively calculate ecological flow. The steps for this method are as follows:
- (1)
- Evaluate ecological flows from RVA, MFM, and ADMFFM, and the comprehensive evaluation values of RVA, MFM, and ADMFFM of the jth month are , and , respectively.
- (2)
- Calculate the comprehensive ecological flow of the jth month as follows:
2.2.4. Weight Calculation of Comprehensive Ecological Flow
- We first determined the deviation rate of monthly flow. The median of the natural daily mean flow series in the jth month of N years was called the standard value. This index is a ratio of ecological flow in the jth month to the standard value. It reflects the deviation degree between the ecological flow and the natural flow in the same period [28,29]. It is computed as follows:
- (1)
- Calculate the natural daily mean flow in the jth month of N years , where i is the year, i = 1, 2, …, N; N is equal to the length of the runoff series; and j is the month, j = 1, 2, …, 12;
- (2)
- Sort in the ascending order , (, n is the serial order), where the standard value of the jth month is ;
- (3)
- The calculation formula of the deviation rate of the monthly flow is:where is the deviation rate of the monthly flow of the jth month and is the ecological flow of the jth month, in m3/s. If the value is close to 1, the calculated flow is approaching the natural flow and the riverine ecosystem is healthy.
- Satisfaction degree of the monthly ecological flow. This a ratio of the days where the natural flow is equal to or greater than the ecological flow to the total number of days in the same month. The formula to calculate the satisfaction of the monthly ecological water requirement is:where is the satisfaction degree of the monthly ecological flow of the jth month, is the number of days where the natural flow was equal to or greater than the ecological flow in the jth month, is the total number of days in the jth month in N years, is the number of days in the jth month, is the natural daily flow on the kth day of the jth month in the ith year in m3/s, and is the ecological flow of the jth month in m3/s. The greater the satisfaction degree of the monthly ecological flow, the healthier the riverine ecosystem.
- Suitability degree of the monthly ecological flow. The monthly ecological flow discrete coefficient is the sum of the discrete degree between the median and the characteristic extreme value of the ecological flow and natural flow. It reflects the suitability degree between the ecological flow and natural flow in a month.where is the discrete coefficient of the monthly ecological flow in the jth month, is the median of the jth month’s ecological flow of over N years, is the median of the jth month’s natural flow of N years, is the maximum of the jth month’s ecological flow of N years, and is the maximum of the jth month’s natural flow of N years. In the case of being greater than 10, it is considered as completely discrete and the value of is taken as 10.The suitability degree of the monthly ecological flow is computed as:When equals 1, a complete suitableness exists between the ecological flow and natural flow in the jth month; when it equals 0, absolutely no suitableness exists between the ecological flow and natural flow in the jth month.This index reflects the degree of suitability between the total ecological flow requirement and the natural flow in a month. When is 1, the total ecological flow requirement and the natural flow completely match. When is 0, the flow is completely unsuitable. A discrete coefficient greater than 10 can be considered completely discrete and the value of the discrete coefficient is equal to 10.
- Ecological flow comprehensive evaluation. The above indexes compare the ecological flow and natural flow from different aspects. The deviation rate of the monthly flow evaluates the degree and magnitude of deviation between the ecological flow and natural flow. The satisfaction degree of the monthly ecological flow temporally analyses the degree of satisfaction between the ecological flow and natural flow. The suitability degree of the monthly ecological flow evaluates the degree of suitability between the ecological flow and natural flow in discrete degrees. The above three indexes were synthesized into a comprehensive index to evaluate ecological flow in the jth month. The formula for calculating is:where and a value of close to 1 means the calculated ecological flow is approaching the natural flow and the riverine ecosystem is healthy.
- Weight calculation of the comprehensive ecological flow. The geometric mean method was used to calculate the weights for each ecological flow calculation method result.
3. Results
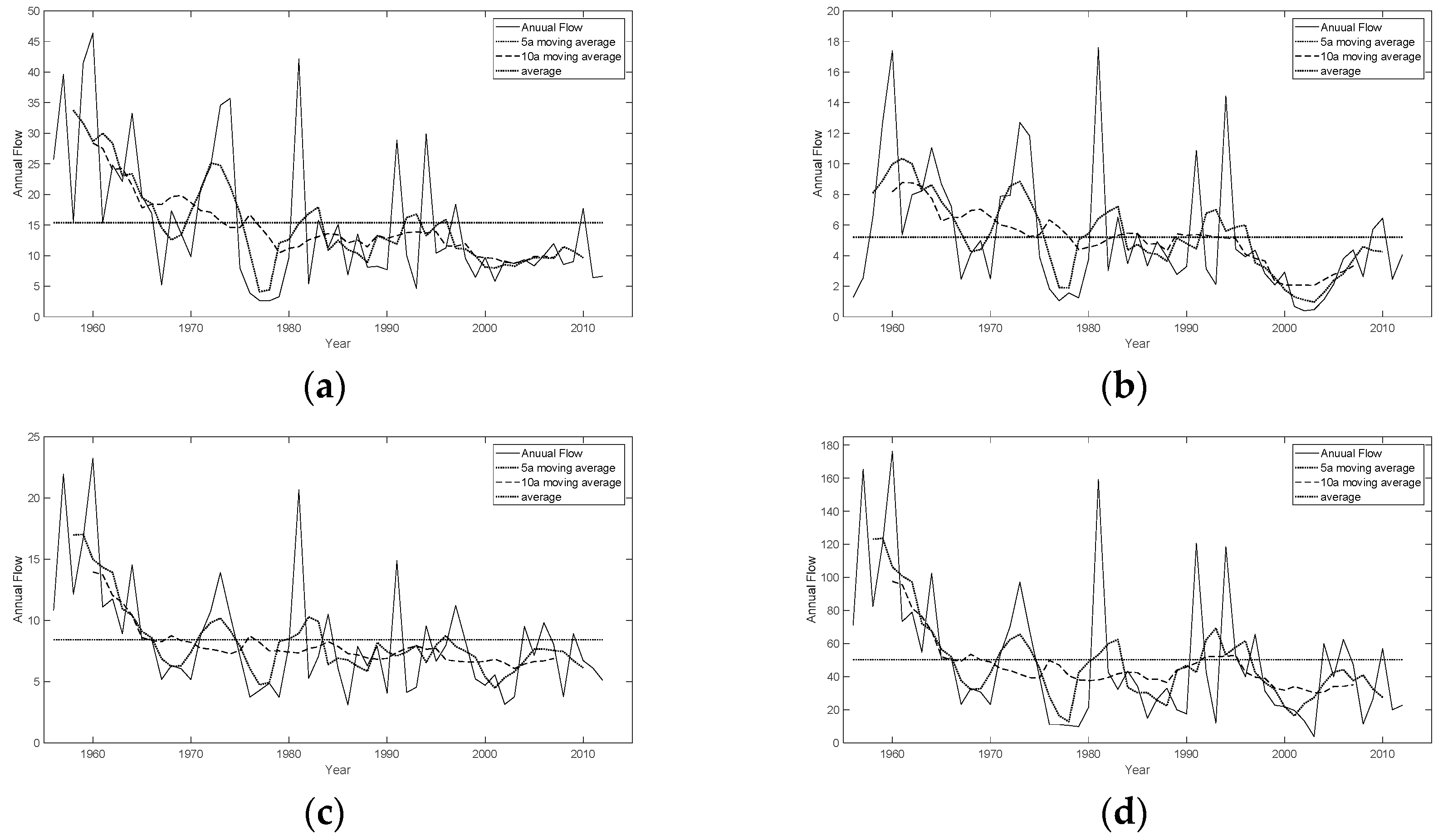
3.1. Hydrological Alteration Diagnosis System
3.2. Results of Ecological Flow Calculation
4. Discussion
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A. An Example for GI Value Calculation
| Month | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Monthly mean flow | 0.08 | 0.009 | 0.027 | 18.4 | 37.7 | 27.4 | 89.5 | 60.1 | 47.5 | 18.6 | 6.6 | 0.57 |
| Month | Order | Monthly Flow | Accumulated Value | Runoff Cumulative Frequency | Time Cumulative Frequency |
|---|---|---|---|---|---|
| (1) | (2) | (3) | (4) | (5) | (6) |
| 2 | 1 | 0.009 | 0.009 | 0.000002965 | 0.083333 |
| 3 | 2 | 0.027 | 0.036 | 0.000117461 | 0.166667 |
| 1 | 3 | 0.08 | 0.116 | 0.000378484 | 0.25 |
| 12 | 4 | 0.57 | 0.686 | 0.002238275 | 0.333333 |
| 11 | 5 | 6.6 | 7.286 | 0.023772701 | 0.416667 |
| 4 | 6 | 18.4 | 25.686 | 0.08380807 | 0.5 |
| 10 | 7 | 18.6 | 44.286 | 0.144495997 | 0.583333 |
| 6 | 8 | 27.4 | 71.686 | 0.233896491 | 0.666667 |
| 5 | 9 | 37.7 | 109.386 | 0.356903741 | 0.75 |
| 9 | 10 | 47.5 | 156.886 | 0.51188635 | 0.833333 |
| 8 | 11 | 60.1 | 216.986 | 0.707980136 | 0.916667 |
| 7 | 12 | 89.5 | 306.486 | 1 | 1 |
| Baoqing | Baoan | Caizuizi | Hongqiling | |
|---|---|---|---|---|
| 1956 | 0.575167 | 0.542804 | 0.465529 | 0.428152 |
| 1957 | 0.663721 | 0.599417 | 0.562583 | 0.477699 |
| 1958 | 0.654811 | 0.621435 | 0.5955 | 0.494503 |
| 1959 | 0.553547 | 0.530033 | 0.65446 | 0.524602 |
| 1960 | 0.572132 | 0.553499 | 0.437941 | 0.414069 |
| 1961 | 0.665426 | 0.646424 | 0.43319 | 0.411643 |
| 1962 | 0.559209 | 0.553148 | 0.496531 | 0.443979 |
| 1963 | 0.616581 | 0.515054 | 0.352364 | 0.370382 |
| 1964 | 0.6856 | 0.65623 | 0.630919 | 0.512584 |
| 1965 | 0.603178 | 0.580838 | 0.377696 | 0.383314 |
| 1966 | 0.650194 | 0.545597 | 0.43394 | 0.412027 |
| 1967 | 0.610227 | 0.606012 | 0.713139 | 0.554558 |
| 1968 | 0.634882 | 0.469468 | 0.54839 | 0.470453 |
| 1969 | 0.42967 | 0.441901 | 0.348785 | 0.368555 |
| 1970 | 0.738535 | 0.615852 | 0.686196 | 0.540803 |
| 1971 | 0.607347 | 0.588313 | 0.469413 | 0.430135 |
| 1972 | 0.617986 | 0.543292 | 0.564369 | 0.47861 |
| 1973 | 0.607321 | 0.607421 | 0.375552 | 0.382219 |
| 1974 | 0.555022 | 0.582396 | 0.287487 | 0.337262 |
| 1975 | 0.713496 | 0.661335 | 0.71216 | 0.554058 |
| 1976 | 0.684477 | 0.570556 | 0.713834 | 0.554912 |
| 1977 | 0.595298 | 0.538128 | 0.83657 | 0.617569 |
| 1978 | 0.520268 | 0.545812 | 0.636622 | 0.515496 |
| 1979 | 0.611183 | 0.632446 | 0.61084 | 0.470432 |
| 1980 | 0.515341 | 0.534083 | 0.627068 | 0.44741 |
| 1981 | 0.613004 | 0.581639 | 0.597752 | 0.603207 |
| 1982 | 0.600386 | 0.556539 | 0.759367 | 0.617718 |
| 1983 | 0.697738 | 0.628078 | 0.655349 | 0.477994 |
| 1984 | 0.524324 | 0.410819 | 0.480368 | 0.389041 |
| 1985 | 0.605347 | 0.513835 | 0.584753 | 0.416934 |
| 1986 | 0.547696 | 0.493103 | 0.681788 | 0.551018 |
| 1987 | 0.599018 | 0.558052 | 0.476933 | 0.445092 |
| 1988 | 0.632303 | 0.533406 | 0.635709 | 0.492935 |
| 1989 | 0.630692 | 0.412365 | 0.714885 | 0.527368 |
| 1990 | 0.537402 | 0.507299 | 0.587728 | 0.455828 |
| 1991 | 0.705846 | 0.692314 | 0.561183 | 0.567586 |
| 1992 | 0.708555 | 0.63015 | 0.744296 | 0.472671 |
| 1993 | 0.577032 | 0.445792 | 0.624847 | 0.508258 |
| 1994 | 0.631919 | 0.582762 | 0.600289 | 0.546941 |
| 1995 | 0.487417 | 0.452052 | 0.668352 | 0.472467 |
| 1996 | 0.717055 | 0.590542 | 0.719361 | 0.550217 |
| 1997 | 0.626837 | 0.558618 | 0.6547 | 0.407772 |
| 1998 | 0.712293 | 0.465754 | 0.721465 | 0.589609 |
| 1999 | 0.654239 | 0.651277 | 0.697595 | 0.650854 |
| 2000 | 0.723293 | 0.637565 | 0.667018 | 0.640227 |
| 2001 | 0.710492 | 0.629317 | 0.704367 | 0.595746 |
| 2002 | 0.564298 | 0.535856 | 0.554664 | 0.500066 |
| 2003 | 0.581597 | 0.546915 | 0.630194 | 0.512214 |
| 2004 | 0.585974 | 0.549713 | 0.630597 | 0.51242 |
| 2005 | 0.585971 | 0.549178 | 0.736604 | 0.566536 |
| 2006 | 0.631846 | 0.558946 | 0.637788 | 0.516091 |
| 2007 | 0.621954 | 0.623716 | 0.722809 | 0.559494 |
| 2008 | 0.586923 | 0.597498 | 0.738638 | 0.567575 |
| 2009 | 0.604515 | 0.639023 | 0.71146 | 0.622316 |
| 2010 | 0.680025 | 0.651626 | 0.793393 | 0.595616 |
| 2011 | 0.652022 | 0.546681 | 0.692261 | 0.555792 |
| 2012 | 0.545863 | 0.567307 | 0.683058 | 0.539201 |
References
- Zhang, Q.; Zhang, Z.J.; Shi, P.J.; Singh, V.P.; Gu, X.H. Evaluation of ecological instream flow considering hydrological alterations in the Yellow River basin, China. Glob. Planet. Chang. 2018, 160, 61–74. [Google Scholar] [CrossRef]
- Xia, Z.Q.; Li, Q.F.; Guo, L.D.; Li, J.; Zhang, W.; Wang, H.Q. Computation of minimum and optimal instream ecological flow for the Yiluohe River. IAHS-AISH Publ. 2007, 315, 142–148. [Google Scholar]
- Yang, F.C.; Xia, Z.Q.; Yu, L.L.; Guo, L.D. Calculation and analysis of the instream ecological flow for the Irtysh River. Procedia Eng. 2012, 28, 438–441. [Google Scholar] [CrossRef]
- Xu, Z.X.; Wu, W.; Yu, S.Y. Ecological baseflow: Progress and challenge. J. Hydroelectr. Eng. 2016, 35, 1–11. [Google Scholar] [CrossRef]
- Yang, Z.F.; Cui, B.S.; Liu, J.L. Estimation methods of eco-environmental water requirements: Case study. Sci. China Ser. D Earth Sci. 2005, 48, 1280–1292. [Google Scholar] [CrossRef]
- Chen, M.J.; Feng, H.L.; Wang, L.Q.; Chen, Q.Y. Calculation methods for appropriate ecological flow. Adv. Water Sci. 2007, 18, 745–750. [Google Scholar] [CrossRef]
- Wang, X.Q.; Liu, C.M.; Yang, Z.F. Method of resolving lowest environmental water demands in river course (I)—Theory. Acta Sci. Circumstantiae 2001, 21, 544–547. [Google Scholar] [CrossRef]
- Geoffrey, E.P. Instream flow science for sustainable river management. J. Am. Water Resour. Assoc. 2009, 45, 1071–1086. [Google Scholar] [CrossRef]
- Northern Great Plains Resource Program (NGPRP). Instream Needs Sub-Group Report; Work Group C, Water; US Fish and Wildlife Service, Office of Biological Service: Washington, DC, USA, 1974. [Google Scholar]
- Li, L.J.; Zheng, H.X. Environmental and ecological water consumption of river systems in Haihe-Luanhe basins. Acta Geogr. Sin. 2000, 55, 495–500. [Google Scholar] [CrossRef]
- Yu, L.J.; Xia, Z.Q.; Du, X.S. Connotation of minimum ecological runoff and its calculation method. J. Hehai Univ. (Nat. Sci.) 2004, 32, 18–22. [Google Scholar]
- Ravindra, K.V.; Shankar, M.; Sangeeta, V.; Surendra, K.M. Design flow duration curves for environmental flows estimation in Damodar River Basin, India. Appl. Water Sci. 2017, 7, 1283–1293. [Google Scholar] [CrossRef]
- Danial, C.; Nassir, E.J. Comparison and regionalization of hydrologically based instream flow. Can. J. Civ. Eng. 1995, 5, 235–246. [Google Scholar] [CrossRef]
- Donald, L.T. Instream flow regimens for fish, wildlife, recreation and related environmental resources. Fish. Mag. 1976, 1, 6–10. [Google Scholar] [CrossRef]
- Brian, D.R.; Jeffrey, B.; Robert, W.; David, P.B. How much water does a river need? Freshw. Biol. 1997, 37, 231–249. [Google Scholar] [CrossRef]
- Li, J.; Xia, Z.Q.; Ma, G.H. A new monthly frequency computation method for instream ecological flow. Acta Ecol. Sin. 2007, 27, 2916–2921. [Google Scholar]
- Zhan, C.S.; Wang, H.X.; Xu, Z.X.; Li, L. Quantitative study on the climate variability and human activities to hydrological response in the Weihe River Basin. In Proceedings of the China Academy of Natural Resources Academic Annual Meeting 2011, Wulumuqi, China, 25 July 2011. [Google Scholar]
- Poff, N.L.; Bledsoe, B.P.; Cuhaciyan, C.O. Hydrologic variation with land use across the contiguous United States: Geomorphic and ecological consequences for stream ecosystems. Geomorphology 2006, 79, 264–285. [Google Scholar] [CrossRef]
- Huang, S.Z.; Chang, J.X.; Huang, Q.; Wang, Y.M.; Chen, Y.T. Calculation of the instream ecological flow of the Wei River based on Hydrological Variation. J. Appl. Math. 2014, 11, 1–9. [Google Scholar] [CrossRef]
- Cai, S.Y.; Lei, X.H.; Meng, X.Y. Ecological flow analysis method based on the comprehensive variation diagnosis of Gini coefficient. J. Intell. Fuzzy Syst. 2018, 34, 1025–1031. [Google Scholar] [CrossRef]
- Xie, P.; Chen, G.C.; Lei, H.F.; Wu, F.Y. Hydrological alteration diagnosis system. J. Hydroelectr. Eng. 2010, 29, 85–91. [Google Scholar]
- Hu, C.X.; Xie, P.; Xu, B.; Chen, G.C.; Liu, X.Y.; Tang, Y.S. Variation analysis method for hydrologic annual distribution homogeneity based on Gini coefficient. A case study of runoff series at Longchun station in Dongjiang river basin. J. Hydroelectr. Eng. 2012, 31, 7–13. [Google Scholar]
- Yang, T.; Zhang, Q.; Chen, Y.D. A spatial assessment of hydrologic alteration caused by dam construction in the middle and lower Yellow River, China. Hydrol. Process. 2008, 24, 262–267. [Google Scholar] [CrossRef]
- Li, Z.Y.; Liu, D.F.; Huang, Q.; Zhang, S.A. Study on the ecological flux of the Ziwu River in Hanjiang River based on several hydrological methods. J. North China Univ. Water Resour. Electr. Power (Nat. Sci. Ed.) 2017, 38, 8–12. [Google Scholar] [CrossRef]
- Xie, P.; Chen, G.C.; Lei, H.F. Hydrological alteration analysis method based on hurst coefficient. J. Basic Sci. Eng. 2009, 17, 32–39. [Google Scholar]
- Liu, G.H.; Zhu, J.X.; Xiong, M.Y.; Wang, D.; Qi, S.H. Assessment of hydrological regime alteration and ecological flow at Meigang station of Xinjiang River. J. China Hydrol. 2016, 26, 51–57. [Google Scholar]
- Li, J.F.; Zhang, Q.; Chen, X.H.; Jiang, T. Study of ecological instream flow in Yellow River, considering the hydrological change. Acta Geogr. Sin. 2011, 66, 99–110. [Google Scholar]
- Zhang, Q.; Cui, Y.; Chen, Y.Q. Evaluation of ecological instream flow of the Pearl River basin. Ecol. Environ. Sci. 2010, 19, 1828–1837. [Google Scholar]
- Halwatura, D.; Najim, M.M.M. Environmental flow assessment—A analysis. J. Environ. Proffessionals Sri Lanka 2014, 3, 1–11. [Google Scholar] [CrossRef]


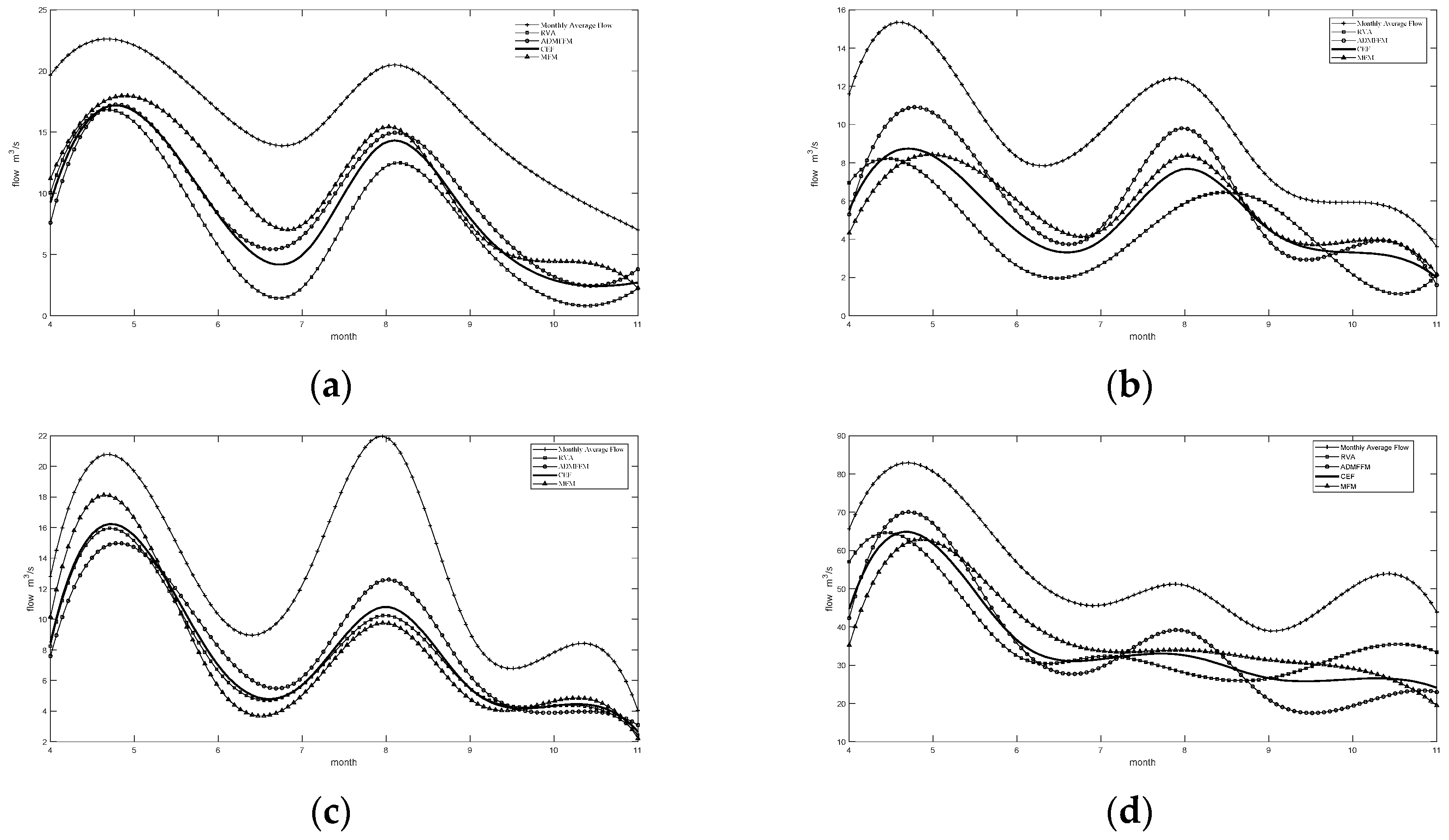
| Diagnosis Method | Intra-Annual Runoff Distribution of GI (1956–2012) | |||||
|---|---|---|---|---|---|---|
| Station | ||||||
| Baoqing | Baoan | Hongqiling | Caizuizi | |||
| Primary diagnosis | Hurst coefficient | 0.741 | 0.896 | 0.727 | 0.615 | |
| Alteration existence | Yes | Yes | Yes | Yes | ||
| Detailed diagnosis | Jump diagnosis | Sliding F test | 1966 (+) | 1990 (+) | 1987 (−) | 1990 (−) |
| Sliding T test | 1966 (−) | 1998 (−) | 2009 (−) | 1981 (+) | ||
| Lee–Heghinan method | 1966 (+) | 1990 (+) | 1989 (+) | 1990 (+) | ||
| Orderly cluster method | 1966 (+) | 1974 (−) | 1987 (+) | 1990 (−) | ||
| R/S | 1989 (+) | 1990 (−) | 1979 (−) | 1990 (+) | ||
| Brown–Forsythe | 1964(−) | 1990(+) | 1987(+) | 1992 (−) | ||
| Sliding run test method | 1992 (+) | 1990 (−) | 1992 (+) | 1985(+) | ||
| Sliding rank test method | 1985 (+) | 1992 (+) | 1987 (+) | 1991 (+) | ||
| Optimum information dichotomy | 1966 (−) | 1989 (+) | 1989 (−) | 1990 (+) | ||
| Mann–Kendall | 1992 (+) | 1992 (−) | 1987 (−) | 1991 (−) | ||
| BSYES | 1966 (+) | 1989 (−) | 1987 (+) | 1989 (+) | ||
| Trend diagnosis | Trend alteration degree | Significant alteration | Significant alteration | Significant alteration | Significant alteration | |
| Correlation coefficient method | (+) | (+) | (+) | (+) | ||
| Spearman | (+) | (+) | (−) | (+) | ||
| Kendall | (+) | (+) | (+) | (+) | ||
| Jump point | 1966 | 1990 | 1987 | 1990 | ||
| Jump | Comprehensive weight | 0.55 | 0.67 | 0.42 | 0.34 | |
| Comprehensive significance | 4 (+) | 5 (+) | 4 (+) | 4 (+) | ||
| Trend | Comprehensive significance | 3 (+) | 3 (+) | 2 (−) | 3 (+) | |
| Comprehensive diagnosis | Alteration form selection | Jump efficiency coefficient/% | 45.3 | 35.1 | 52.5 | 50.3 |
| Trend efficiency coefficient/% | 38.3 | 46.2 | 39.1 | 37.5 | ||
| Diagnosis result | 1966 | 1990 | 1987 | 1990 | ||
| Station | Method | April | May | June | July | ||||
| (1) | (2) | (1) | (2) | (1) | (2) | (1) | (2) | ||
| Baoqing | RVA | 10.05 | 6.89 | 15.81 | 11.48 | 5.67 | 6.80 | 2.29 | 1.28 |
| MFM | 11.19 | 6.85 | 17.99 | 12.85 | 11.95 | 7.72 | 7.39 | 4.95 | |
| ADMFFM | 7.64 | 5.90 | 16.84 | 12.55 | 8.35 | 6.55 | 6.52 | 4.35 | |
| Baoan | RVA | 6.95 | 4.92 | 6.98 | 4.94 | 2.61 | 1.12 | 2.68 | 1.15 |
| MFM | 4.32 | 3.63 | 8.43 | 6.02 | 6.03 | 4.44 | 4.42 | 3.36 | |
| ADMFFM | 5.32 | 4.92 | 6.98 | 5.44 | 2.61 | 2.94 | 2.68 | 2.54 | |
| Hongqiling | RVA | 8.26 | 8.61 | 15.11 | 12.53 | 6.61 | 3.35 | 5.69 | 0.75 |
| MFM | 10.17 | 9.18 | 16.59 | 12.94 | 5.62 | 5.39 | 5.04 | 3.82 | |
| ADMFFM | 7.61 | 7.60 | 14.72 | 11.7 | 8.21 | 6.35 | 6.21 | 4.70 | |
| Caizuizi | RVA | 57.08 | 37.18 | 30.13 | 38.18 | 30.10 | 32.47 | 17.44 | 29.62 |
| MFM | 35.28 | 34.94 | 62.39 | 55.30 | 43.69 | 39.44 | 33.76 | 30.13 | |
| ADMFFM | 42.31 | 38.65 | 67.89 | 56 | 35.74 | 31.85 | 29.32 | 28.3 | |
| Station | Method | August | September | October | November | ||||
| (1) | (2) | (1) | (2) | (1) | (2) | (1) | (2) | ||
| Baoqing | RVA | 12.21 | 5.14 | 7.02 | 3.25 | 1.32 | 0.49 | 2.22 | 0.98 |
| MFM | 15.42 | 11.66 | 7.44 | 5.09 | 4.44 | 3.01 | 2.26 | 1.63 | |
| ADMFFM | 14.82 | 11.65 | 9.42 | 5.5 | 3.22 | 2.03 | 3.82 | 2.5 | |
| Baoan | RVA | 5.89 | 2.97 | 5.81 | 8.3 | 2.16 | 1.46 | 2.13 | 1.45 |
| MFM | 8.37 | 6.51 | 4.56 | 4.22 | 3.91 | 3.51 | 2.16 | 2.08 | |
| ADMFFM | 5.89 | 5.04 | 5.81 | 5.60 | 2.16 | 2.12 | 2.13 | 1.86 | |
| Hongqiling | RVA | 10.26 | 5.27 | 5.49 | 1.37 | 4.35 | 2.67 | 2.46 | 1.03 |
| MFM | 9.76 | 9.33 | 4.78 | 5.49 | 4.66 | 4.08 | 2.22 | 2.41 | |
| ADMFFM | 12.63 | 11.75 | 6.34 | 5.75 | 3.92 | 3.90 | 3.12 | 2.55 | |
| Caizuizi | RVA | 17.36 | 35.66 | 15.91 | 26.41 | 15.18 | 19.09 | 33.42 | 19.10 |
| MFM | 33.99 | 33.05 | 31.41 | 28.60 | 29.11 | 26.61 | 19.49 | 20.74 | |
| ADMFFM | 39.45 | 37.8 | 21.22 | 22.05 | 19.41 | 22.35 | 23.33 | 22 | |
| Station | Method | April | May | June | July | ||||
| (1) | (2) | (1) | (2) | (1) | (2) | (1) | (2) | ||
| Baoqing | RVA | 0.31 | 0.29 | 0.44 | 0.32 | 0.44 | 0.31 | 0.42 | 0.32 |
| MFM | 0.25 | 0.35 | 0.32 | 0.43 | 0.30 | 0.40 | 0.24 | 0.42 | |
| ADMFFM | 0.44 | 0.29 | 0.24 | 0.32 | 0.26 | 0.31 | 0.34 | 0.31 | |
| Baoan | RVA | 0.32 | 0.34 | 0.41 | 0.38 | 0.39 | 0.41 | 0.31 | 0.36 |
| MFM | 0.29 | 0.34 | 0.35 | 0.39 | 0.17 | 0.43 | 0.41 | 0.39 | |
| ADMFFM | 0.39 | 0.35 | 0.24 | 0.39 | 0.44 | 0.43 | 0.28 | 0.35 | |
| Hongqiling | RVA | 0.31 | 0.34 | 0.34 | 0.42 | 0.29 | 0.39 | 0.31 | 0.36 |
| MFM | 0.27 | 0.33 | 0.33 | 0.42 | 0.29 | 0.42 | 0.29 | 0.38 | |
| ADMFFM | 0.42 | 0.29 | 0.33 | 0.32 | 0.42 | 0.31 | 0.40 | 0.30 | |
| Caizuizi | RVA | 0.34 | 0.34 | 0.42 | 0.39 | 0.31 | 0.46 | 0.38 | 0.38 |
| MFM | 0.37 | 0.35 | 0.22 | 0.34 | 0.24 | 0.44 | 0.24 | 0.37 | |
| ADMFFM | 0.29 | 0.36 | 0.36 | 0.35 | 0.45 | 0.46 | 0.38 | 0.40 | |
| Station | Method | August | September | October | November | ||||
| (1) | (2) | (1) | (2) | (1) | (2) | (1) | (2) | ||
| Baoqing | RVA | 0.34 | 0.29 | 0.41 | 0.30 | 0.32 | 0.31 | 0.43 | 0.32 |
| MFM | 0.42 | 0.40 | 0.23 | 0.39 | 0.25 | 0.42 | 0.27 | 0.43 | |
| ADMFFM | 0.24 | 0.30 | 0.36 | 0.30 | 0.43 | 0.31 | 0.30 | 0.33 | |
| Baoan | RVA | 0.43 | 0.41 | 0.18 | 0.40 | 0.29 | 0.37 | 0.29 | 0.41 |
| MFM | 0.31 | 0.37 | 0.41 | 0.42 | 0.41 | 0.41 | 0.42 | 0.43 | |
| ADMFFM | 0.26 | 0.38 | 0.41 | 0.41 | 0.30 | 0.39 | 0.29 | 0.43 | |
| Hongqiling | RVA | 0.25 | 0.42 | 0.20 | 0.39 | 0.30 | 0.42 | 0.25 | 0.33 |
| MFM | 0.42 | 0.42 | 0.43 | 0.42 | 0.41 | 0.43 | 0.33 | 0.34 | |
| ADMFFM | 0.33 | 0.31 | 0.37 | 0.31 | 0.29 | 0.31 | 0.42 | 0.30 | |
| Caizuizi | RVA | 0.40 | 0.39 | 0.24 | 0.33 | 0.29 | 0.38 | 0.23 | 0.31 |
| MFM | 0.40 | 0.36 | 0.41 | 0.37 | 0.30 | 0.37 | 0.37 | 0.33 | |
| ADMFFM | 0.20 | 0.37 | 0.35 | 0.36 | 0.41 | 0.40 | 0.40 | 0.38 | |
| Month | Baoqing | Baoan | Hongqiling | Caizuizi | ||||
|---|---|---|---|---|---|---|---|---|
| (1) | (2) | (1) | (2) | (1) | (2) | (1) | (2) | |
| 4 | 27.26 | 16.89 | 16.14 | 14.67 | 25.86 | 14.57 | 18.12 | 14.61 |
| 5 | 14.63 | 19.35 | 12.23 | 16.57 | 32.43 | 28.07 | 21.86 | 12.87 |
| 6 | 16.15 | 17.29 | 14.97 | 16.12 | 24.79 | 34.65 | 23.44 | 35.32 |
| 7 | 15.89 | 16.23 | 14.03 | 23.87 | 24.68 | 33.74 | 24.34 | 33.47 |
| 8 | 19.55 | 24.56 | 18.10 | 22.48 | 27.65 | 32.80 | 22.80 | 23.49 |
| 9 | 12.56 | 18.96 | 19.57 | 23.63 | 30.08 | 36.36 | 21.42 | 26.31 |
| 10 | 28.65 | 16.23 | 12.18 | 14.35 | 26.79 | 13.57 | 22.62 | 14.06 |
| 11 | 28.63 | 15.99 | 12.70 | 14.30 | 16.36 | 14.73 | 15.98 | 13.04 |
| Average | 20.42 | 18.19 | 14.99 | 18.25 | 26.08 | 26.06 | 21.32 | 21.65 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xing, Z.; Wang, Y.; Gong, X.; Wu, J.; Ji, Y.; Fu, Q. Calculation of Comprehensive Ecological Flow with Weighted Multiple Methods Considering Hydrological Alteration. Water 2018, 10, 1212. https://doi.org/10.3390/w10091212
Xing Z, Wang Y, Gong X, Wu J, Ji Y, Fu Q. Calculation of Comprehensive Ecological Flow with Weighted Multiple Methods Considering Hydrological Alteration. Water. 2018; 10(9):1212. https://doi.org/10.3390/w10091212
Chicago/Turabian StyleXing, Zhenxiang, Yinan Wang, Xinglong Gong, Jingyan Wu, Yi Ji, and Qiang Fu. 2018. "Calculation of Comprehensive Ecological Flow with Weighted Multiple Methods Considering Hydrological Alteration" Water 10, no. 9: 1212. https://doi.org/10.3390/w10091212
APA StyleXing, Z., Wang, Y., Gong, X., Wu, J., Ji, Y., & Fu, Q. (2018). Calculation of Comprehensive Ecological Flow with Weighted Multiple Methods Considering Hydrological Alteration. Water, 10(9), 1212. https://doi.org/10.3390/w10091212





