Interaction between Perched Epikarst Aquifer and Unsaturated Soil Cover in the Initiation of Shallow Landslides in Pyroclastic Soils
Abstract
1. Introduction
2. Materials and Methods
2.1. The Hydrometeorological Monitoring Station
2.2. The Mathematical Model of the Slope
3. Results and Discussion
3.1. Slope Water Balance
3.2. Estimation of the Parameters of the Slope Model
3.3. Long-Term Simulations
3.4. Simulation of Year 1999
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Crozier, M.J. Prediction of rainfall-triggered landslides: A test of the antecedent water status model. Earth Surf. Process. Landf. 1999, 24, 825–833. [Google Scholar] [CrossRef]
- Rahimi, A.; Rahardjo, H.; Leong, E.C. Effect of Antecedent Rainfall Patterns on Rainfall-Induced Slope Failure. J. Geotech. Geoenviron. Eng. 2011, 137. [Google Scholar] [CrossRef]
- Von Ruette, J.; Lehmann, P.; Or, D. Effects of rainfall spatial variability and intermittency on shallow landslide triggering patterns at a catchment scale. Water Resour. Res. 2014, 50, 7780–7799. [Google Scholar] [CrossRef]
- Napolitano, E.; Fusco, F.; Baum, R.L.; Godt, J.W.; De Vita, P. Effect of antecedent-hydrological conditions on rainfall triggering of debris flows in ash-fall pyroclastic mantled slopes of Campania (southern Italy). Landslides 2016, 13, 967–983. [Google Scholar] [CrossRef]
- Bogaard, T.A.; Greco, R. Landslide hydrology: From hydrology to pore pressure. Wiley Interdiscip. Rev. Water 2016, 3, 439–459. [Google Scholar] [CrossRef]
- Tromp-van Meerveld, H.J.; McDonnell, J.J. Threshold relations in subsurface stormflow: 2. The fill and spill hypothesis. Water Resour. Res. 2006, 42, W02411. [Google Scholar] [CrossRef]
- Nieber, J.L.; Sidle, R.C. How do disconnected macropores in sloping soils facilitate preferential flow? Hydrol. Process. 2010, 24, 1582–1594. [Google Scholar] [CrossRef]
- Damiano, E.; Greco, R.; Guida, A.; Olivares, L.; Picarelli, L. Investigation on rainwater infiltration into layered shallow covers in pyroclastic soils and its effect on slope stability. Eng. Geol. 2017, 220, 208–218. [Google Scholar] [CrossRef]
- Cascini, L.; Sorbino, G.; Cuom, O.S.; Ferlisi, S. Seasonal effects of rainfall on the shallow pyroclastic deposits of the Campania region (southern Italy). Landslides 2014, 11, 779–792. [Google Scholar] [CrossRef]
- Comegna, L.; Damiano, E.; Greco, R.; Guida, A.; Olivares, L.; Picarelli, L. Field hydrological monitoring of a sloping shallow pyroclastic deposit. Can. Geotech. J. 2016, 53, 1125–1137. [Google Scholar] [CrossRef]
- Glade, T.; Crozier, M.; Smith, P. Applying probability determination to refine landslide-triggering rainfall thresholds using an empirical “antecedent daily rainfall model”. Pure Appl. Geophys. 2000, 157, 1059–1079. [Google Scholar] [CrossRef]
- Chleborad, A.F.; Baum, R.L.; Godt, J.W.; Powers, P.S. A prototype for forecasting landslides in the Seattle, Washington, Area. Rev. Eng. Geol. 2008, 20, 103–120. [Google Scholar] [CrossRef]
- Bogaard, T.A.; Greco, R. Invited perspectives: Hydrological perspectives on precipitation intensity-duration thresholds for landslide initiation: Proposing hydro-meteorological thresholds. Nat. Hazards Earth Syst. Sci. 2018, 18, 31–39. [Google Scholar] [CrossRef]
- Picarelli, L.; Damiano, E.; Greco, R.; Minardo, A.; Olivares, L.; Zeni, L. Performance of slope beahviour indicators in unsaturated pyroclastic soils. J. Mt. Sci. 2015, 12, 1434–1447. [Google Scholar] [CrossRef]
- Lu, N.; Likos, W.J. Suction stress characteristic curve for unsaturated soil. J. Geotech. Geoenviron. Eng. 2006, 132, 131–142. [Google Scholar] [CrossRef]
- Greco, R.; Gargano, R. A novel equation for determining the suction stress of unsaturated soils from the water retention curve based on wetted surface area in pores. Water Resour. Res. 2015, 51, 6143–6155. [Google Scholar] [CrossRef]
- Olivares, L.; Picarelli, L. Shallow flowslides triggered by intense rainfalls on natural slopes covered by loose unsaturated pyroclastic soils. Geotechnique 2003, 53, 283–288. [Google Scholar] [CrossRef]
- Damiano, E.; Olivares, L.; Picarelli, L. Steep-slope monitoring in unsaturated pyroclastic soils. Eng. Geol. 2012, 137–138, 1–12. [Google Scholar] [CrossRef]
- Reder, A.; Pagano, L.; Picarelli, L.; Rianna, G. The role of the lowermost boundary conditions in the hydrological response of shallow sloping covers. Landslides 2017, 14, 861–873. [Google Scholar] [CrossRef]
- Greco, R.; Comegna, L.; Damiano, E.; Guida, A.; Olivares, L.; Picarelli, L. Conceptual hydrological modeling of the soil-bedrock interface at the bottom of the pyroclastic cover of Cervinara (Italy). Procedia Earth Plan. Sci. 2014, 9, 122–131. [Google Scholar] [CrossRef]
- Cascini, L.; Cuomo, S.; Guida, D. Typical source areas of May 1998 flow-like mass movements in the Campania region, Southern Italy. Eng. Geol. 2008, 96, 107–125. [Google Scholar] [CrossRef]
- Allocca, V.; Manna, F.; De Vita, P. Estimating annual groundwater recharge coefficient for karst aquifers of the southern Apennines (Italy). Hydrol. Earth Syst. Sci. 2014, 18, 803–817. [Google Scholar] [CrossRef]
- Bakalowicz, M. Epikarst. In Encyclopedia of Caves, 2nd ed.; White, W.B., Culver, D.C., Eds.; Academic Press: London, UK, 2012; pp. 284–288. ISBN 9780123838339. [Google Scholar]
- Perrin, J.; Jeannin, P.-Y.; Zwahlen, F. Epikarst storage in a karst aquifer: A conceptual model based on isotopic data, Milandre test site, Switzerland. J. Hydrol. 2003, 279, 106–124. [Google Scholar] [CrossRef]
- Hartmann, A.; Goldscheider, N.; Wagener, T.; Lange, J.; Weiler, M. Karst water resources in a changing world: Review of hydrological modeling approaches. Rev. Geophys. 2014, 52, 218–242. [Google Scholar] [CrossRef]
- Celico, F.; Naclerio, G.; Bucci, A.; Nerone, V.; Capuano, P.; Carcione, M.; Allocca, V.; Celico, P. Influence of pyroclastic soil on epikarst formation: A test study in southern Italy. Terra Nova 2010, 22, 110–115. [Google Scholar] [CrossRef]
- Petrella, E.; Capuano, P.; Carcione, M.; Celico, F. A high altitude temporary spring in a compartmentalized carbonate aquifer: The role of low-permeability faults and karst conduits. Hydrol. Process. 2009, 23, 3354–3364. [Google Scholar] [CrossRef]
- Jukic, D.; Denic-Jukic, V. Groundwater balance estimation in karst by using a conceptual rainfall–runoff model. J. Hydrol. 2009, 373, 302–315. [Google Scholar] [CrossRef]
- Fu, Z.; Chen, H.; Xu, Q.; Jia, J.; Wang, S.; Wang, K. Role of epikarst in near-surface hydrological processes in a soil mantled subtropical dolomite karst slope: Implications of field rainfall simulation experiments. Hydrol. Process. 2016, 30, 795–811. [Google Scholar] [CrossRef]
- Xu, X.; Huang, G.; Zhan, H.; Qu, Z.; Huang, Q. Integration of SWAP and MODFLOW-2000 for modeling groundwater dynamics in shallow water table areas. J. Hydrol. 2012, 412, 170–181. [Google Scholar] [CrossRef]
- Szymkiewicz, A.; Gumuła-Kawęcka, A.; Šimůnek, J.; Leterme, B.; Beegum, S.; Jaworska-Szulc, B.; Pruszkowska-Cacers, M.; Gorczewska-Langner, W.; Angulo-Jaramillo, R.; Jacques, D. Simulations of freshwater lens recharge and salt/freshwater interfaces using the HYDRUS and SWI2 packages for MODFLOW. J. Hydrol. Hydromech. 2018, 66, 246–256. [Google Scholar] [CrossRef]
- Shuttleworth, W.J. Evaporation. In Handbook of Hydrology; Maidment, D.R., Ed.; McGraw-Hill: New York, NY, USA, 1993; pp. 4.1–4.53. ISBN 0070397325. [Google Scholar]
- Greco, R.; Comegna, L.; Damiano, E.; Guida, A.; Olivares, L.; Picarelli, L. Hydrological modelling of a slope covered with shallow pyroclastic deposits from field monitoring data. Hydrol. Earth Syst. Sci. 2013, 17, 4001–4013. [Google Scholar] [CrossRef]
- Van Genuchten, M.T. A closed-form equation for predicting the hydraulic conductivity of unsaturated soils. Soil Sci. Soc. Am. J. 1980, 44, 892–898. [Google Scholar] [CrossRef]
- Feddes, R.A.; Kowalik, P.; Kolinska-Malinka, K.; Zaradny, H. Simulation of field water uptake by plants using a soil water dependent root extraction function. J. Hydrol. 1976, 31, 13–26. [Google Scholar] [CrossRef]
- Nyambayo, V.P.; Potts, D.M. Numerical simulation of evapotranspiration using a root water uptake model. Comput. Geotech. 2010, 37, 175–186. [Google Scholar] [CrossRef]
- Peyret, R.; Taylor, T.D. Computational Methods for Fluid Flow; Springer: New York, NY, USA, 1983; p. 358. ISBN 978-3-642-85952-6. [Google Scholar]
- Al-Fares, W.; Bakalowicz, M.; Guérin, R.; Dukhan, M. Analysis of the karst aquifer structure by means of a Ground Penetrating Radar (GPR). Example of the Lamalou area (Hérault, France). J. Appl. Geophys. 2002, 51, 97–106. [Google Scholar] [CrossRef]
- Freeze, R.A.; Cherry, J.A. Groundwater; Prentice-Hall: Englewood Cliffs, NJ, USA, 1979; p. 604. [Google Scholar]
- White, W.B. A brief history of karst hydrogeology: Contributions of the NSS. J. Cave Karst. Stud. 2007, 69, 13–26. [Google Scholar]
- Worthington, S.R.H. Diagnostic hydrogeologic characteristics of a karst aquifer Kentucky, (USA). Hydrogeol. J. 2009, 17, 1665–1678. [Google Scholar] [CrossRef]
- Allocca, V.; De Vita, P.; Manna, F.; Nimmo, J.R. Groundwater recharge assessment at local and episodic scale in a soil mantled perched karst aquifer in southern Italy. J. Hydrol. 2015, 529, 843–853. [Google Scholar] [CrossRef]
- Binet, S.; Joigneaux, E.; Pauwels, H.; Albéric, P.; Fléhoc Ch Bruand, A. Water exchange, mixing and transient storage between a saturated karstic conduit and the surrounding aquifer: Groundwater flow modeling and inputs from stable water isotopes. J. Hydrol. 2017, 544, 278–289. [Google Scholar] [CrossRef]
- Ladouche, B.; Marechal, J.C.; Dorfliger, N. Semi-distributed lumped model of a karst system under active management. J. Hydrol. 2014, 509, 215–230. [Google Scholar] [CrossRef]
- Zhang, Z.; Chen, X.; Chen, X.; Shi, P. Quantifying time lag of epikarst-spring hydrograph response to rainfall using correlation and spectral analyses. Hydrogeol. J. 2013, 21, 1619–1631. [Google Scholar] [CrossRef]
- Lu, N.; Godt, J.W.; Wu, D.T. A closed-form equation for effective stress in unsaturated soil. Water Resour. Res. 2010, 46, W05515. [Google Scholar] [CrossRef]
- Fiorillo, F.; Guadagno, F.M.; Equino, S.; De Blasio, A. The December 1999 Cervinara landslides: Further debris flows in the pyroclastic deposits of Campania (southern Italy). Bull. Eng. Geol. Environ. 2001, 60, 171–184. [Google Scholar] [CrossRef]

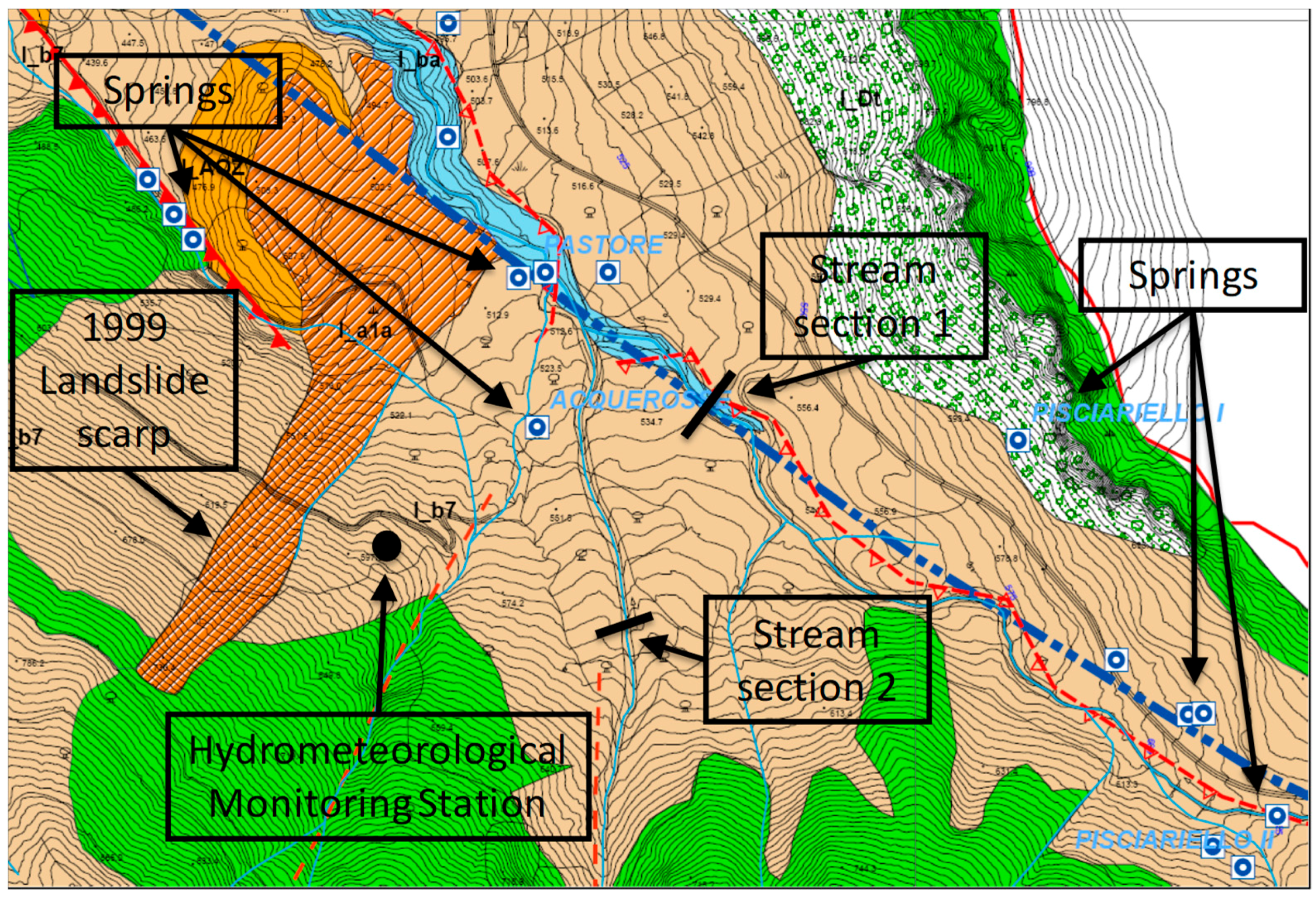
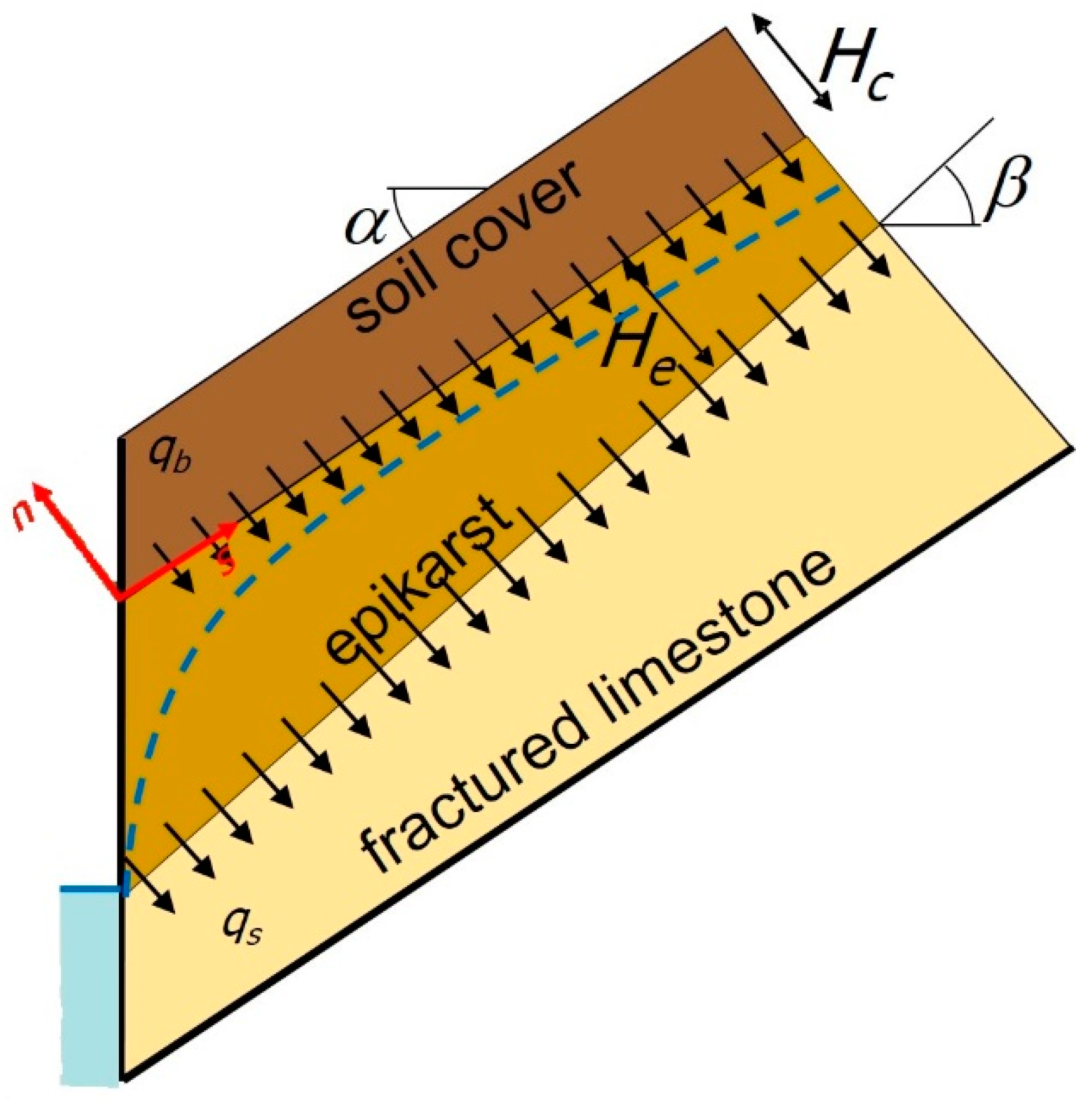
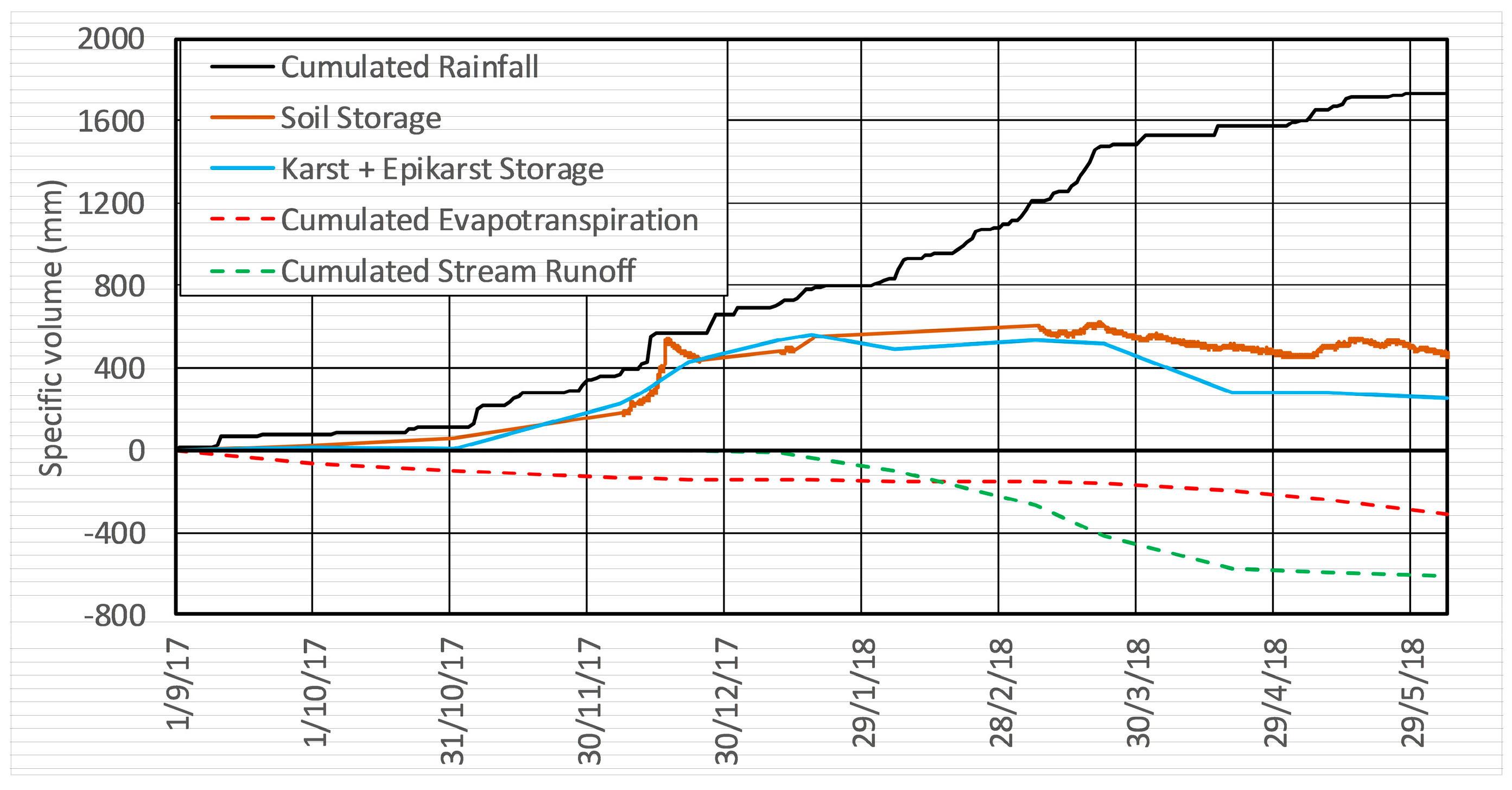

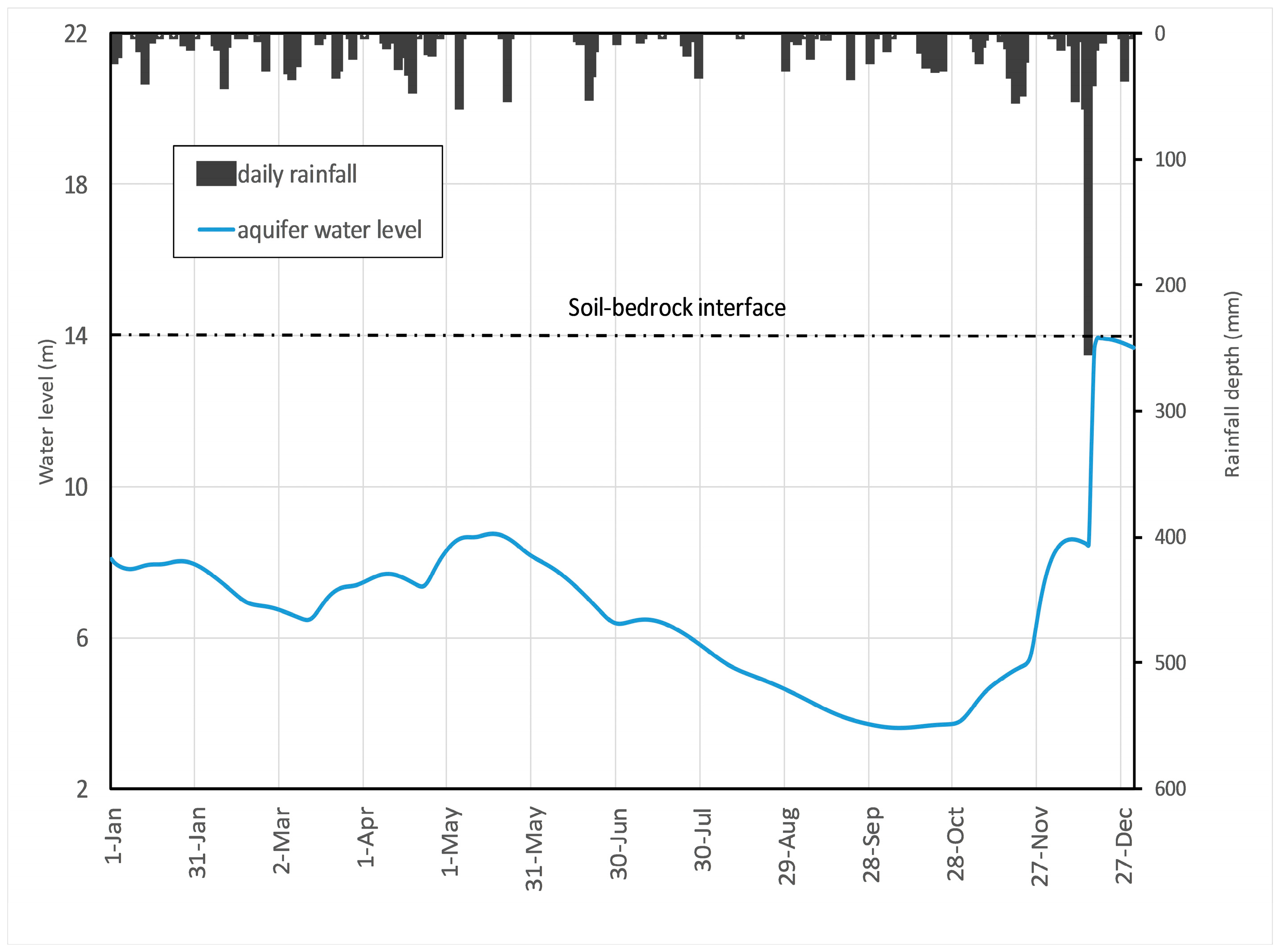
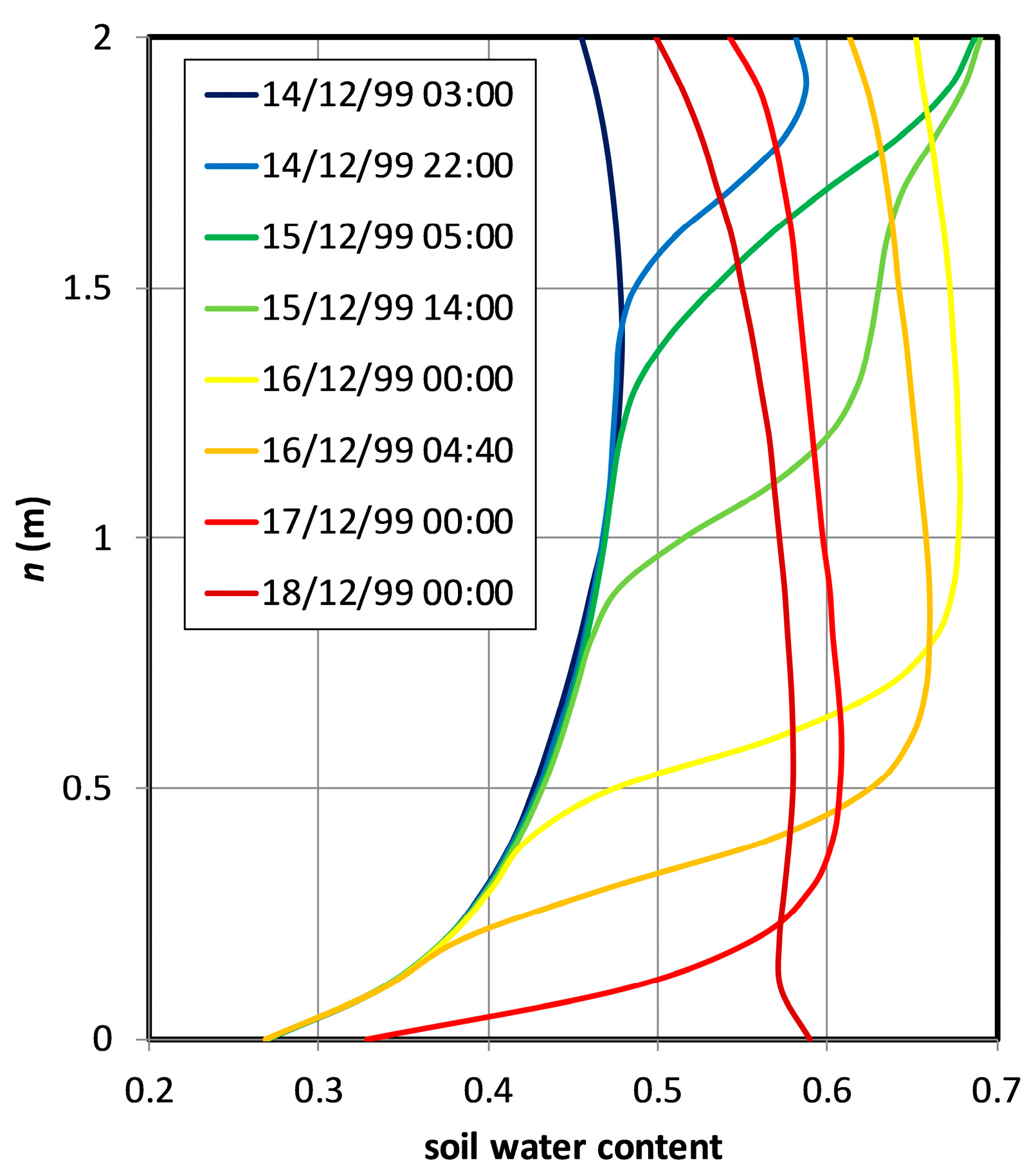
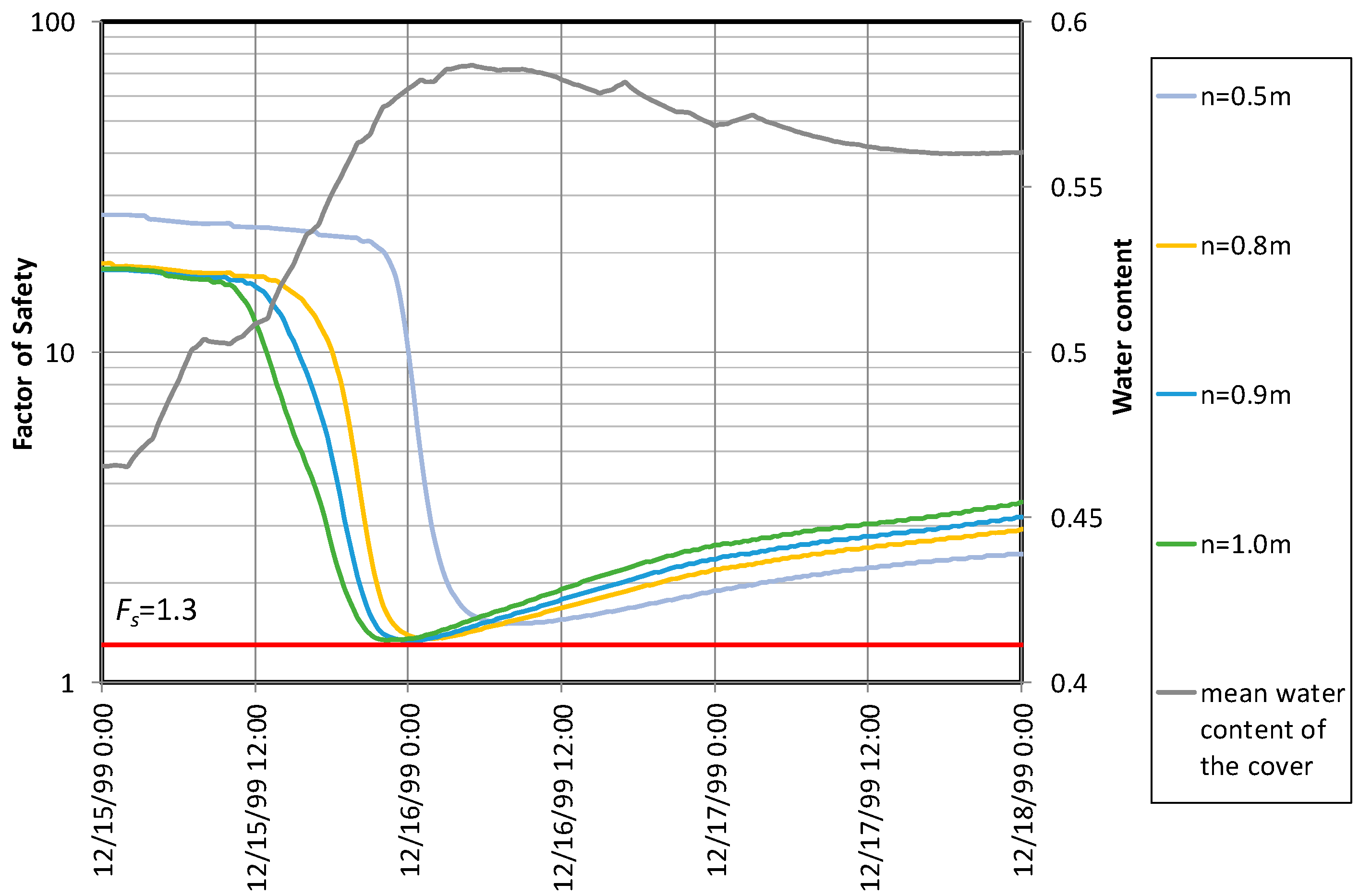
| Soil | Clay % | Silt % | Sand % | Gravel % | n (%) | γdry (kN/m3) | Ksat (m/s) | ф’ (°) | c’ (kPa) |
|---|---|---|---|---|---|---|---|---|---|
| coarse pumices | 0 | 0–4 | 48–60 | 38–56 | 50–55 | 1.2–1.3 | 5 × 10−6–1 × 10−5 | - | - |
| ashes | 2–5 | 4–14 | 75–85 | 0–8 | 70–75 | 0.7–0.8 | 1 × 10−6–6 × 10−6 | 38 | 0 |
| fine pumices | 0–4 | 2–15 | 55–60 | 25–40 | 50–55 | 1.2–1.3 | - | - | - |
| weathered ashes | 2–10 | 10–40 | 40–80 | 2–8 | 60–65 | 0.9–1.0 | 8 × 10−7–1 × 10−6 | 31 | 11 |
| Period | Mean Precipitation Depth (mm) | ||
|---|---|---|---|
| January | 14 | 10 | 226 |
| February | 16 | 12 | 168 |
| March | 31 | 26 | 201 |
| April | 51 | 45 | 127 |
| May | 85 | 76 | 87 |
| June | 121 | 107 | 71 |
| July | 145 | 127 | 54 |
| August | 135 | 118 | 43 |
| September | 98 | 86 | 94 |
| October | 64 | 57 | 124 |
| November | 30 | 26 | 214 |
| December | 17 | 14 | 193 |
| Year | 808 | 703 | 1602 |
| Measurement Date | Section 1 | Section 2 | |||
|---|---|---|---|---|---|
| 7 December 2017 | 0.0 | 0.0 | 0.0 | 0.0 | 0.0 |
| 12 December 2017 | 2.0 | 0.2 | 0.0 | 0.0 | 0.0 |
| 22 December 2017 | 11.0 | 13.8 | 0.0 | 0.0 | 1.7 |
| 11 January 2018 | 14.0 | 25.3 | 0.0 | 0.0 | 3.1 |
| 18 January 2018 | 28.0 | 143.1 | 7.0 | 7.4 | 18.7 |
| 5 February 2018 | 18.0 | 47.4 | 0.0 | 0.0 | 5.9 |
| 8 March 2018 | 35.0 | 250.0 | 10.0 | 18.1 | 33.4 |
| 23 March 2018 | 35.0 | 250.0 | 15.0 | 50.0 | 37.3 |
| 20 April 2018 | 15.0 | 30.1 | 0.0 | 0.0 | 3.7 |
| 11 May 2018 | 14.0 | 25.3 | 0.0 | 0.0 | 3.1 |
| 6 June 2018 | 12.5 | 19.1 | 0.0 | 0.0 | 2.4 |
| Soil cover | Cover thickness, (m) | 2.0 |
| Slope length (m) | 200 | |
| Saturated water content, (−) | 0.75 | |
| Residual water content, (−) | 0.01 | |
| Air entry point, (m−1) | 6.0 | |
| Van Genuchten retention curve shape parameter, (−) | 1.1 | |
| Saturated hydraulic conductivity, (m/s) | 3.0 × 10−5 | |
| Epikarst | Epikarst thickness, (m) | 14 |
| Effective porosity, (−) | 0.015 | |
| Epikarst hydraulic conductivity, (m/s) | 1.1 × 10−6 |
| Year | Annual Rainfall Height (mm) |
|---|---|
| 2006–2007 | 1235 |
| 2007–2008 | 1431 |
| 2008–2009 | 2119 |
| 2009–2010 | 1739 |
| 2010–2011 | 1928 |
| 2011–2012 | 1122 |
| 2012–2013 | 2129 |
| 2013–2014 | 2000 |
| 2014–2015 | 1427 |
| 2015–2016 | 1524 |
| 2016–2017 | 1090 |
| Mean | 1613 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Greco, R.; Marino, P.; Santonastaso, G.F.; Damiano, E. Interaction between Perched Epikarst Aquifer and Unsaturated Soil Cover in the Initiation of Shallow Landslides in Pyroclastic Soils. Water 2018, 10, 948. https://doi.org/10.3390/w10070948
Greco R, Marino P, Santonastaso GF, Damiano E. Interaction between Perched Epikarst Aquifer and Unsaturated Soil Cover in the Initiation of Shallow Landslides in Pyroclastic Soils. Water. 2018; 10(7):948. https://doi.org/10.3390/w10070948
Chicago/Turabian StyleGreco, Roberto, Pasquale Marino, Giovanni Francesco Santonastaso, and Emilia Damiano. 2018. "Interaction between Perched Epikarst Aquifer and Unsaturated Soil Cover in the Initiation of Shallow Landslides in Pyroclastic Soils" Water 10, no. 7: 948. https://doi.org/10.3390/w10070948
APA StyleGreco, R., Marino, P., Santonastaso, G. F., & Damiano, E. (2018). Interaction between Perched Epikarst Aquifer and Unsaturated Soil Cover in the Initiation of Shallow Landslides in Pyroclastic Soils. Water, 10(7), 948. https://doi.org/10.3390/w10070948








