Temperature Rise of Seawater Simulation under the Influence of Sediment-Water Heat Exchange
Abstract
:1. Introduction
2. Methods
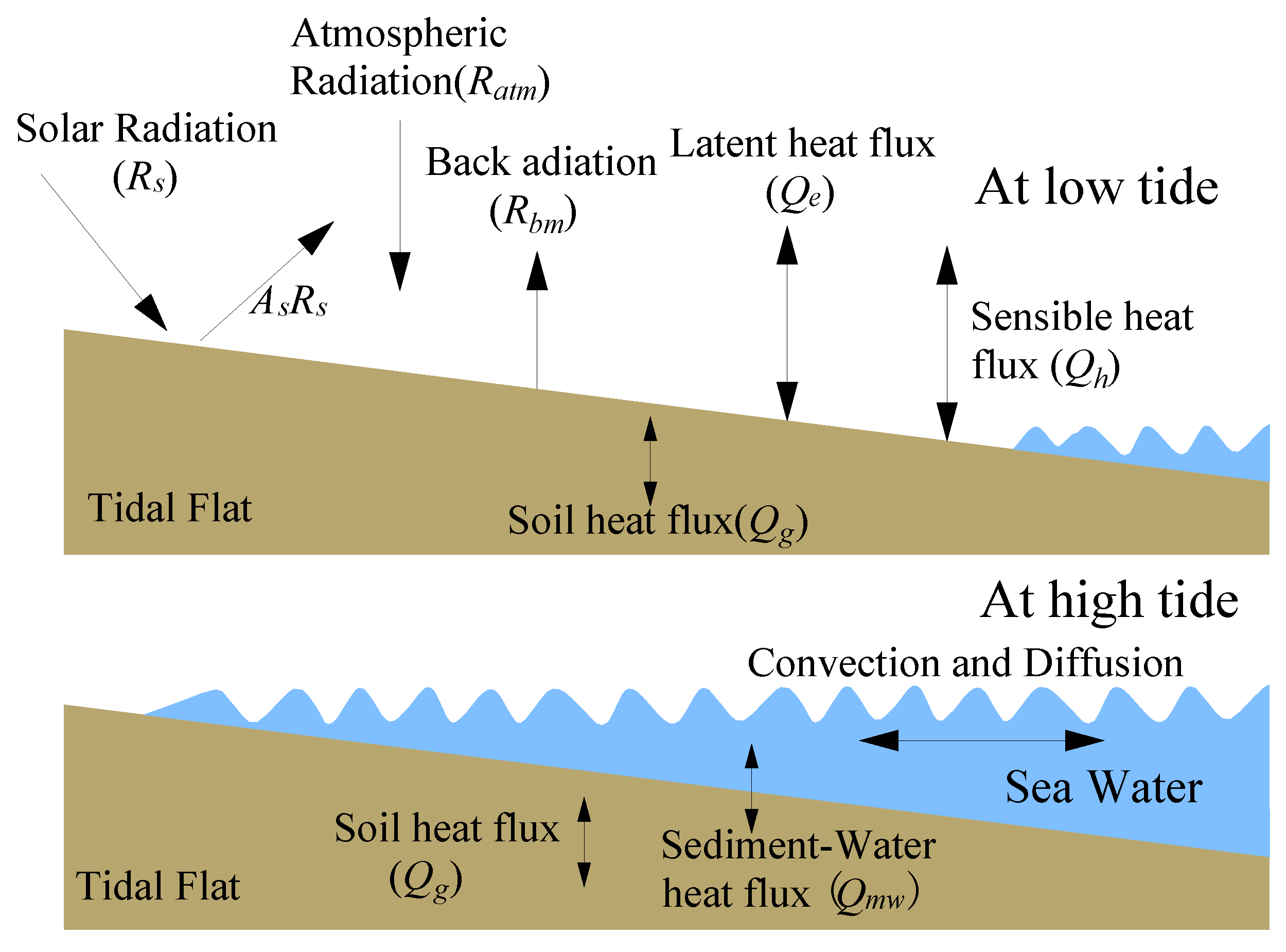
2.1. General Factors
2.2. Tidal Sediment Temperature Model
- (1)
- z ≤ 0.4 m,
- (2)
- 0.4 m < z ≤ 1.0 m,
2.3. Sea Water Temperature Rise Model
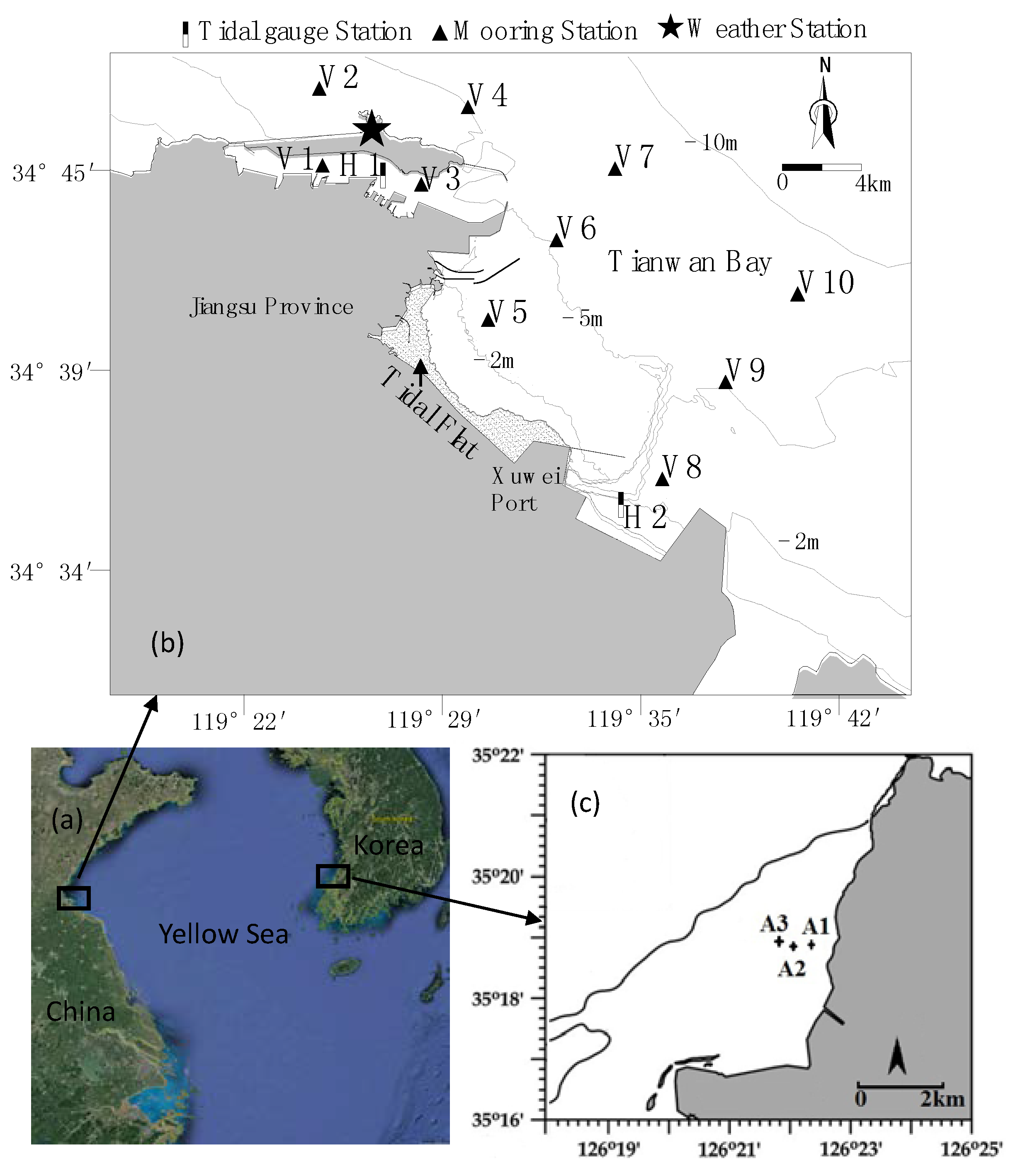
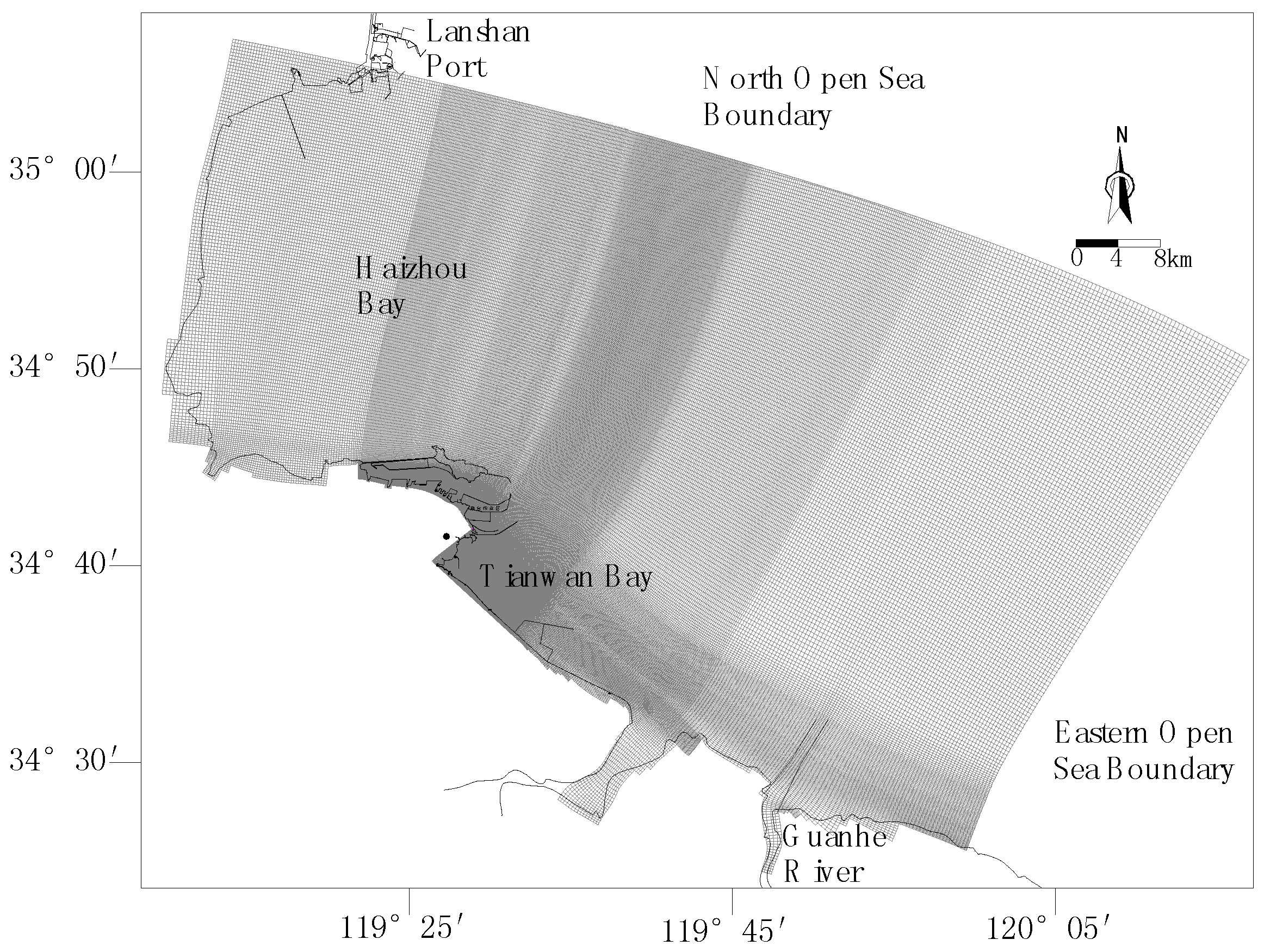
2.4. Study Area
3. Model Calibration
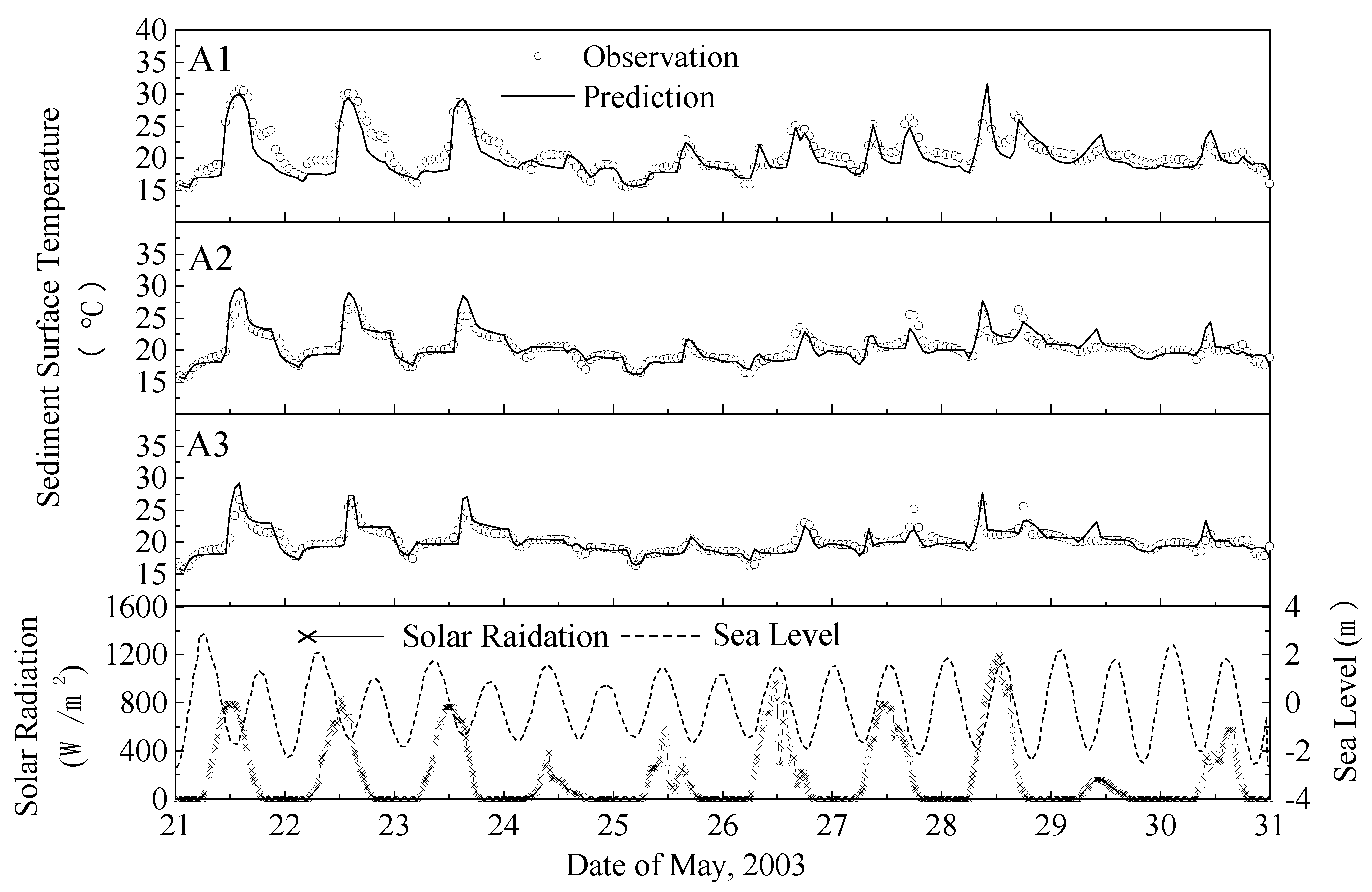
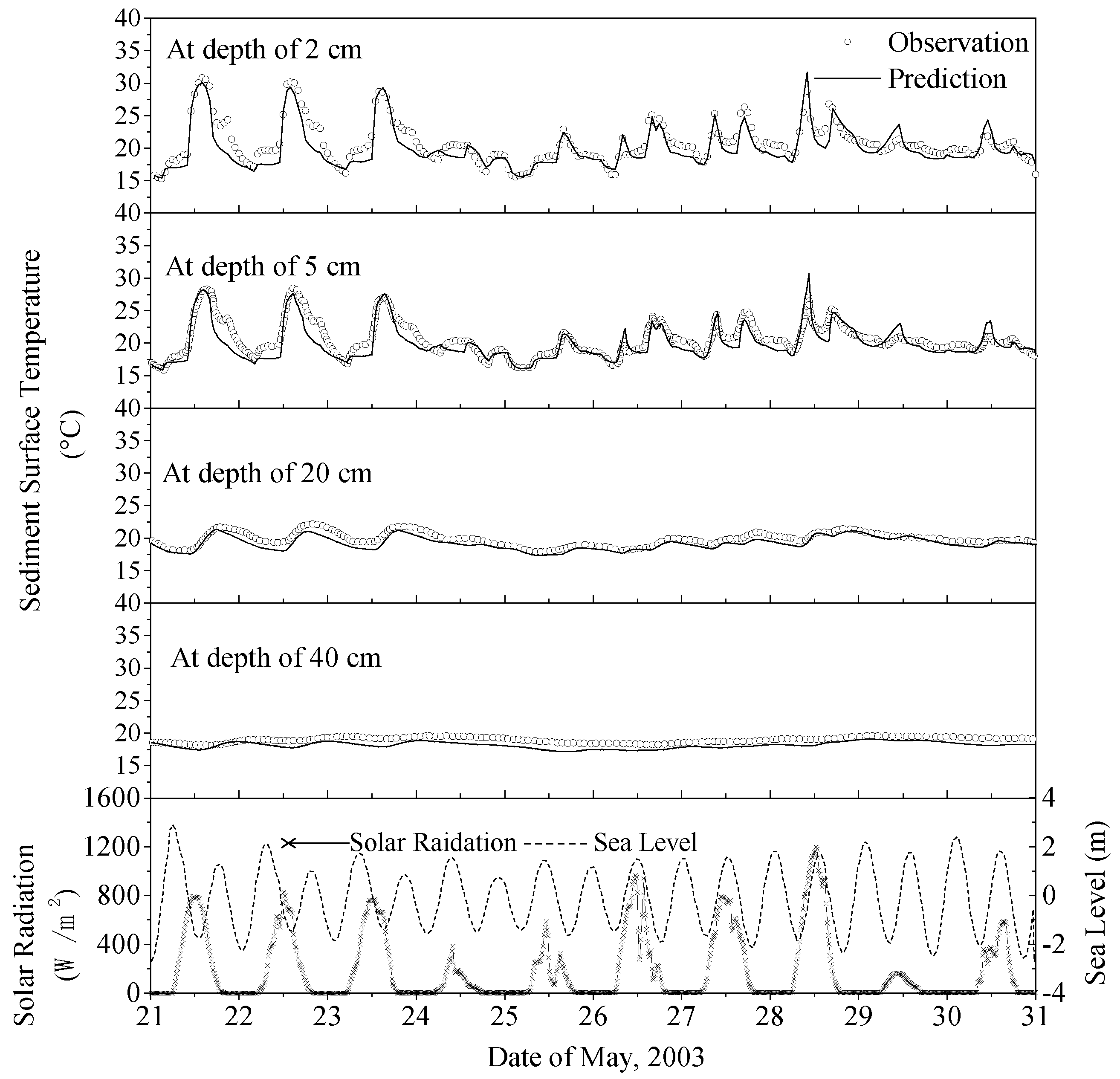
3.1. The Calibration of Temperature Rise Model
3.2. The Calibration of Sediment Temperature Model
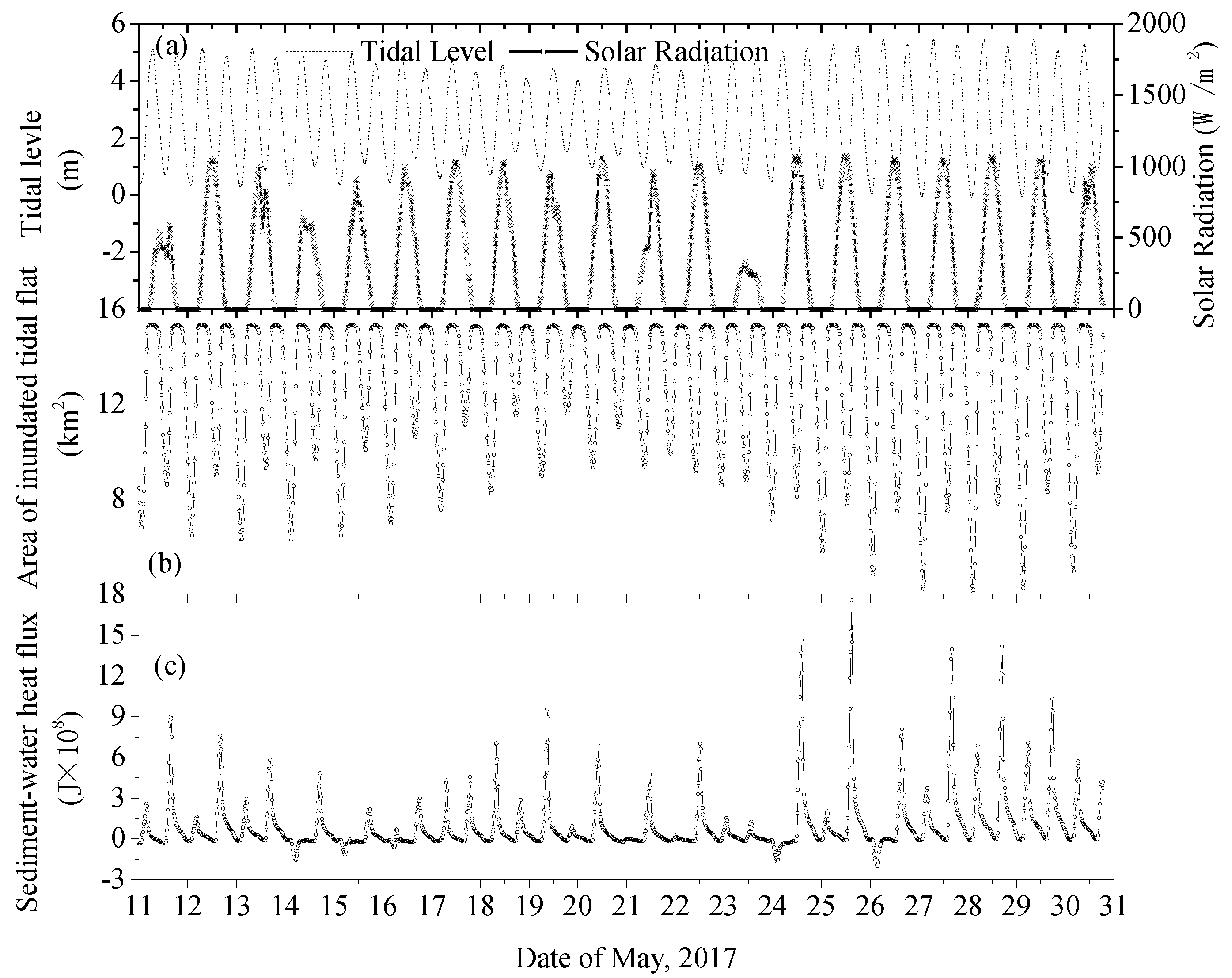
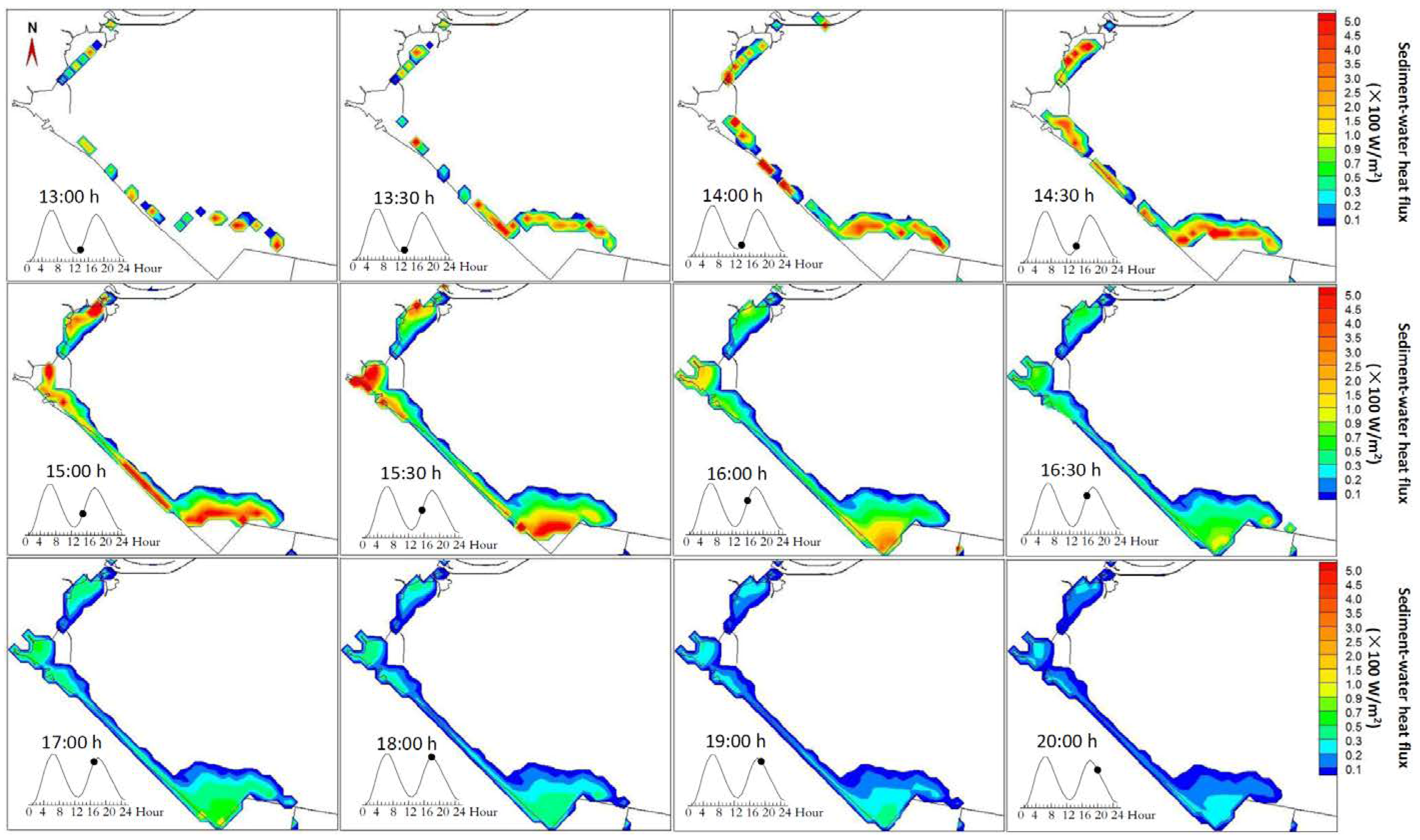
4. Case Study
- (1)
- Scenario 1—from 0.33 × 10−6 to 1.06 × 10−6 m2·s−1
- (2)
- Scenario 2—from 0.40 × 10−6 to 1.27 × 10−6 m2·s−1 (positive deviation 20%)
- (3)
- Scenario 3—from 0.26 × 10−6 to 0.85 × 10−6 m2·s−1 (negative deviation 20%)
5. Discussion
5.1. The Sediment Diffusivity
5.2. Coefficient of Heat Loss from the Water Surface
6. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Cech, J.J.; Mitchell, S.J.; Castleberry, D.T.; Mcenroe, M. Distribution of California stream fishes: Influence of environmental temperature and hypoxia. Environ. Biol. Fishes 1990, 29, 95–105. [Google Scholar] [CrossRef]
- Verones, F.; Hanafiah, M.M.; Pfister, S.; Huijbregts, M.A.; Pelletier, G.J.; Koehler, A. Characterization factors for thermal pollution in freshwater aquatic environments. Environ. Sci. Technol. 2010, 44, 9364–9368. [Google Scholar] [CrossRef] [PubMed]
- Wither, A.; Bamber, R.; Colclough, S.; Dyer, K.; Elliott, M.; Holmes, P.; Jenner, H.; Taylor, C.; Turnpenny, A. Setting new thermal standards for transitional and coastal (TraC) waters. Mar. Pollut. Bull. 2012, 68, 46–50. [Google Scholar] [CrossRef] [PubMed]
- Kim, T.W.; Cho, Y.K. Calculation of heat flux in a macrotidal flat using FVCOM. J. Geophys. Res. Oceans 2011, 116, 869–881. [Google Scholar] [CrossRef]
- Masahiro, S.; Masumi, K.; Katumi, Y.; Fumiya, T. Heat and temperature environments at the muddy tidal flat in the interior parts of the Ariake Sea and its effects on bioturbation. J. Coast. Eng. 2009, 65, 1136–1140. [Google Scholar]
- Vugts, H.F.; Zimmerman, J.T.F. Heat balance of a tidal flat area. Neth. J. Sea Res. 1985, 19, 1–14. [Google Scholar] [CrossRef]
- Rinehimer, J.P.; Thomson, J.T. Observations and modeling of heat fluxes on tidal flats. J. Geophys. Res. Oceans 2014, 119, 133–146. [Google Scholar] [CrossRef]
- Harrison, S.J.; Phizacklea, A.P. Vertical temperature gradients in muddy intertidal sediments in the Forth estuary, Scotland. Limnol. Oceanogr. 1987, 32, 954–963. [Google Scholar] [CrossRef]
- Kim, T.W.; Cho, Y.K. Heat flux across the surface of a macrotidal flat in southwest Korea. J. Geophys. Res. 2009, 114, 1748–1755. [Google Scholar] [CrossRef]
- Kim, T.W.; Cho, Y.K.; You, K.W.; Jung, K.T. Effect of tidal flat on seawater temperature variation in the southwest coast of Korea. J. Geophys. Res. 2010, 115, 526–534. [Google Scholar] [CrossRef]
- Guarini, J.M.; Blanchard, G.F.; Gros, P.; Harrison, S.J. Modelling the mud surface temperature on intertidal flats to investigate the spatio-temporal dynamics of the benthic microalgal photosynthetic capacity. Mar. Ecol. Prog. Ser. 1997, 153, 25–36. [Google Scholar] [CrossRef]
- Wyrtki, K. The average annual heat balance of the North Pacific Ocean and its relation to ocean circulation. J. Geophys. Res. 1965, 70, 4547–4559. [Google Scholar] [CrossRef]
- Cuceloglu, G.; Abbaspour, K.; Ozturk, I. Assessing the Water-Resources Potential of Istanbul by Using a Soil and Water Assessment Tool (SWAT) Hydrological Model. Water 2017, 9, 814. [Google Scholar] [CrossRef]
- Tuller, S.E. Energy balance microclimatic variations on a coastal beach. Tellus 1972, 24, 260–270. [Google Scholar] [CrossRef]
- Piccolo, M.C.; Perillo, G.M.E.; Daborn, G.R. Soil Temperature Variations on a Tidal Flat in Minas Basin, Bay of Fundy, Canada. Estuar. Coast. Shelf Sci. 1993, 36, 345–357. [Google Scholar] [CrossRef]
- Miao, Y.C.; Liu, S.H.; Lu, S.H.; Zhang, Y. A comparative study of computing methods of soil thermal diffusivity, temperature and heat flux. Chin. J. Geophys. 2012, 55, 441–451. [Google Scholar] [CrossRef]
- Thomson, J.M. Observations of thermal diffusivity and a relation to the porosity of tidal flat sediments. Agric. Meteorol. 2010, 115, C05016. [Google Scholar] [CrossRef]
- Ma, J.R.; Zhang, X.Y.; Zhang, X.N. Thermal buoyancy effect in two-dimensional cooling water mathematical models. Hydro-Sci. Eng. 2005, 3, 37–40. [Google Scholar]
- Ma, J.R.; Guo, Y.Q.; Zou, G.L. Analysis on changes of flow and sediment characteristic caused by Jiaojiang River control works. Yangtze River 2013, 44, 85–89. [Google Scholar]
- Ma, J.R.; Guo, Y.Q.; Yao, L. Flow condition and engineering measures of lanqiao jetty and oil dock area in Lanshan harbor of Rizhao port. Ocean Eng. 2010, 28, 127–131. [Google Scholar]
- Durán-Colmenares, A.; Barrios-Piña, H.; Ramírez-León, H. Numerical Modeling of Water Thermal Plumes Emitted by Thermal Power Plants. Water 2016, 8, 482. [Google Scholar] [CrossRef]
- Song, Z.Y.; Yan, Y.X.; Mao, L.H. A tidal harmonious analysis method. Ocean Eng. 1997, 15, 40–45. [Google Scholar]
- Gunneberg, F. Probleme bei der Berechnung des Warmeaustauches Gewasser/Atmoshpare. Warmeeinleitungen in Gewasser und Deren Auswirkungen; Die Abwarme Kommission Geschaftsstelle beim Umweltbundesamt: Berlin, Germany, 1987. [Google Scholar]
- Zhang, S.N.; Tong, L. Experimental study on the heat loss coefficient from water surface in laboratory. Water Resour. Prot. 1986, 2, 1059–1067. [Google Scholar]
- Losordo, T.M.; Piedrahita, R.H. Modelling temperature variation and thermal stratification in shallow aquaculture ponds. Ecol. Model. 1991, 54, 189–226. [Google Scholar] [CrossRef]
- China Meteorological Data Service Center. Available online: http://data.cma.cn/en/?r=site/index (accessed on 7 June 2017).




| Source | Study Site | Composition of Sediment | Water Content % | Thermal Diffusivity α × 10−6 m2·s−1 | Heat Conductivity kz W·m−1·K−1 |
|---|---|---|---|---|---|
| Vugst and Zimmerman [6] | Dutch Wadden Sea, Mok bay | Muddy sand | 40 | 1.06 | 3.11 |
| Harrison and Phizacklea [8] | Forth estuary, Scotland | 4% organic matter, 16% sand, 80% silt and clay. | 70 | 0.47 | 0.88 |
| Guarini et al. [11] | Marennes-Oleron Bay | low clay content muddy flat | 55 | 0.48 | 0.80 |
| Kim et al. [4,10] | The western coast of Korea | 7% sand, 67% silt and 26% clay | 70 | 0.33–1.0 | 1.26–2.50 |
| Rinehimer and Thomson [7] | Skagit Bay in Washington State | Sand | - | 1.00 | 5–8 |
| Willapa Bay in Washington State | Mud | - | 0.50 | 8 | |
| Loaordo and Piedrahita [25] | In shallow aquaculture ponds | - | - | - | 0.70 |
| Time on 25 May | Area of Seawater Temperature Rise (in km2) | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| 0.1 °C | 0.2 °C | 0.3 °C | 0.4 °C | 0.5 °C | 0.6 °C | 0.7 °C | 0.8 °C | 0.9 °C | 1.0 °C | |
| 13:00 | 3.83 | 0.51 | 0.37 | 0.29 | 0.22 | 0.18 | 0.14 | 0.11 | 0.08 | 0.06 |
| 13:15 | 4.55 | 1.26 | 0.94 | 0.75 | 0.61 | 0.49 | 0.38 | 0.30 | 0.23 | 0.17 |
| 13:30 | 5.10 | 1.95 | 1.50 | 1.18 | 0.95 | 0.77 | 0.61 | 0.48 | 0.36 | 0.26 |
| 13:45 | 5.24 | 2.37 | 1.85 | 1.47 | 1.17 | 0.93 | 0.73 | 0.56 | 0.43 | 0.33 |
| 14:00 | 5.25 | 2.60 | 2.00 | 1.59 | 1.26 | 1.00 | 0.80 | 0.65 | 0.51 | 0.38 |
| 14:15 | 5.21 | 2.76 | 2.06 | 1.58 | 1.25 | 0.98 | 0.77 | 0.57 | 0.42 | 0.31 |
| 14:30 | 5.25 | 3.07 | 2.28 | 1.77 | 1.38 | 1.06 | 0.81 | 0.62 | 0.46 | 0.34 |
| 14:45 | 5.61 | 3.22 | 2.39 | 1.82 | 1.38 | 1.05 | 0.74 | 0.49 | 0.32 | 0.21 |
| 15:00 | 6.13 | 3.43 | 2.49 | 1.84 | 1.38 | 1.00 | 0.69 | 0.43 | 0.23 | 0.09 |
| 15:15 | 6.43 | 3.44 | 2.39 | 1.74 | 1.27 | 0.87 | 0.55 | 0.33 | 0.16 | 0.05 |
| 15:30 | 6.43 | 3.30 | 2.16 | 1.46 | 0.97 | 0.58 | 0.29 | 0.14 | 0.06 | 0.02 |
| 15:45 | 6.19 | 3.04 | 1.81 | 1.08 | 0.56 | 0.24 | 0.11 | 0.07 | 0.03 | 0.01 |
| 16:00 | 6.10 | 2.92 | 1.60 | 0.83 | 0.36 | 0.15 | 0.08 | 0.05 | 0.02 | 0.01 |
| 17:00 | 6.12 | 2.65 | 1.10 | 0.44 | 0.21 | 0.10 | 0.06 | 0.03 | 0.01 | 0.00 |
| 18:00 | 6.44 | 2.59 | 0.96 | 0.40 | 0.17 | 0.09 | 0.05 | 0.03 | 0.01 | 0.00 |
| 19:00 | 8.19 | 3.33 | 1.24 | 0.46 | 0.18 | 0.10 | 0.04 | 0.03 | 0.01 | 0.00 |
| 20:00 | 11.31 | 4.98 | 2.01 | 0.58 | 0.26 | 0.15 | 0.03 | 0.02 | 0.00 | 0.00 |
| 21:00 | 15.77 | 7.25 | 3.00 | 1.00 | 0.49 | 0.25 | 0.00 | 0.00 | 0.00 | 0.00 |
| 22:00 | 20.07 | 8.23 | 2.52 | 0.73 | 0.35 | 0.07 | 0.00 | 0.00 | 0.00 | 0.00 |
| 23:00 | 24.38 | 8.95 | 1.16 | 0.22 | 0.00 | 0.00 | 0.00 | 0.00 | 0.00 | 0.00 |
| The Envelope Area 1 | 36.27 | 20.46 | 10.63 | 8.99 | 8.17 | 7.07 | 5.91 | 4.83 | 3.76 | 2.76 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Guo, Y.; Ma, J. Temperature Rise of Seawater Simulation under the Influence of Sediment-Water Heat Exchange. Water 2018, 10, 656. https://doi.org/10.3390/w10050656
Guo Y, Ma J. Temperature Rise of Seawater Simulation under the Influence of Sediment-Water Heat Exchange. Water. 2018; 10(5):656. https://doi.org/10.3390/w10050656
Chicago/Turabian StyleGuo, Yaqiong, and Jinrong Ma. 2018. "Temperature Rise of Seawater Simulation under the Influence of Sediment-Water Heat Exchange" Water 10, no. 5: 656. https://doi.org/10.3390/w10050656
APA StyleGuo, Y., & Ma, J. (2018). Temperature Rise of Seawater Simulation under the Influence of Sediment-Water Heat Exchange. Water, 10(5), 656. https://doi.org/10.3390/w10050656




