Removal of Pb2+ from Water by Synthesized Tannin Resins from Invasive South African Trees
Abstract
1. Introduction
2. Experimental
2.1. Collection, Authentication and Pre-Extraction Treatment
2.2. Sequential Extraction of Tannin
2.3. Estimation of Tannin in the Aqueous Fraction
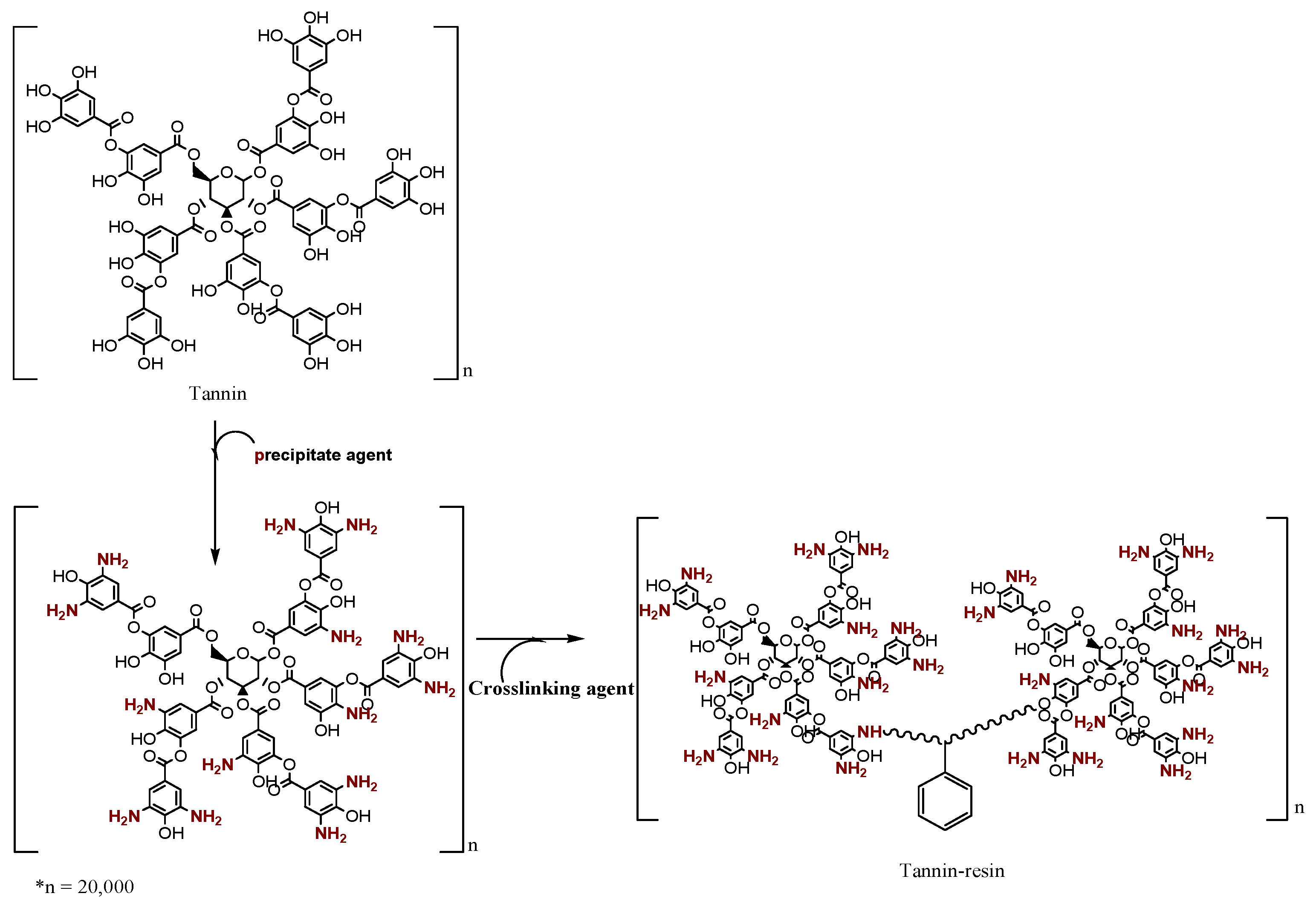
2.4. Synthesis of Tannin-Resin
2.5. Characterisation of Tannins and Resins
2.6. Batch Adsorption Study
2.7. Kinetic Models
2.7.1. Pseudo-First-Order Kinetic Equation
2.7.2. Pseudo-Second-Order Kinetic Model
2.7.3. Elovich Equation
2.7.4. Intra-Particle Diffusion Equation
2.8. Adsorption Isotherms
2.8.1. Langmuir Adsorption Isotherm
2.8.2. Dubinin-Radushkevich (D-R) Adsorption Isotherm
2.8.3. Temkin Adsorption Isotherm
2.8.4. Freundlich Adsorption Isotherm
3. Results and Discussion
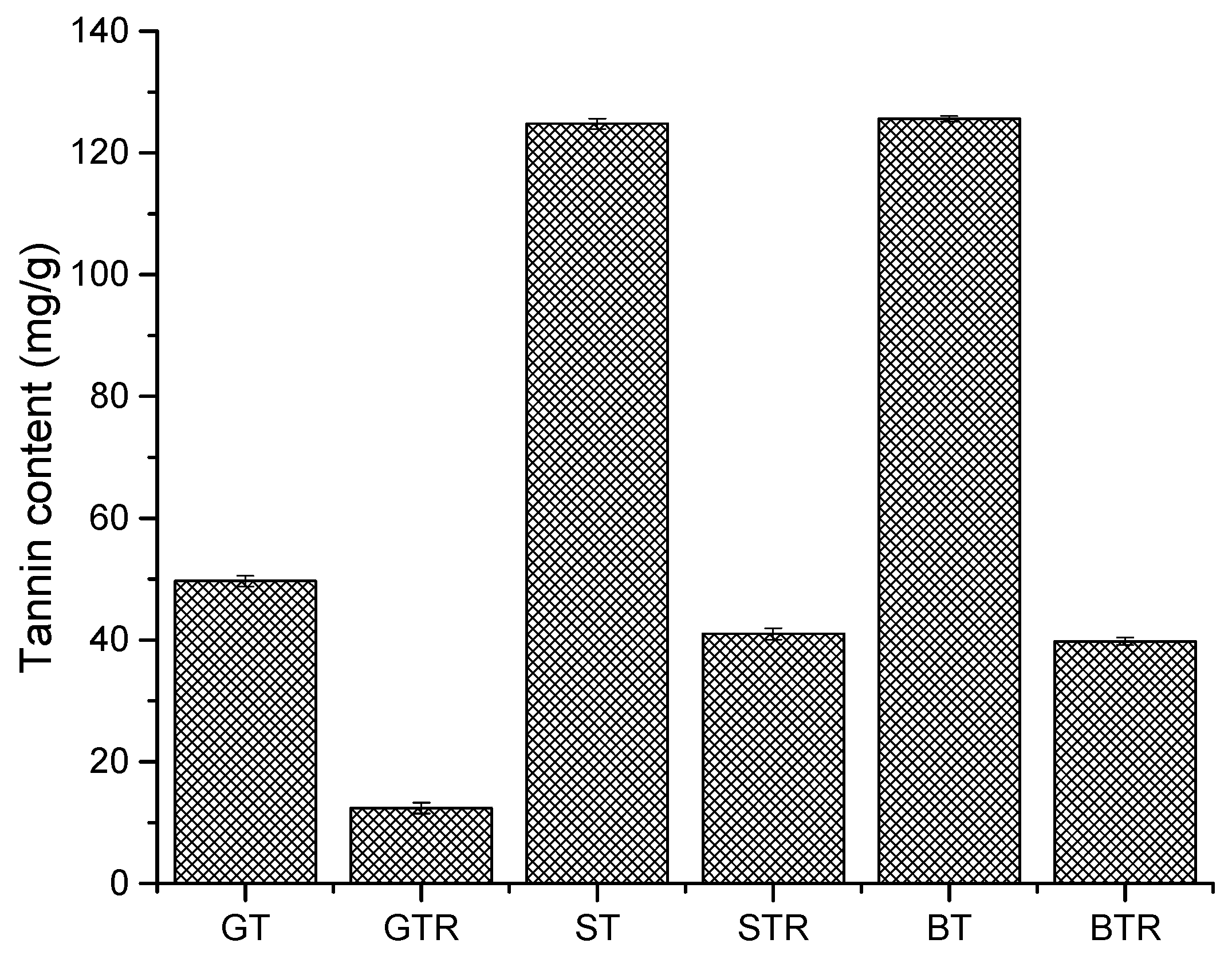
3.1. Yield of Extracted Tannins and Resins per Gram of the Aqueous Fraction
3.2. Thermal and Functional Group Properties of the Tannins and Resins
3.3. Crystalline and Amorphous Profiling of the Tannins and Resins
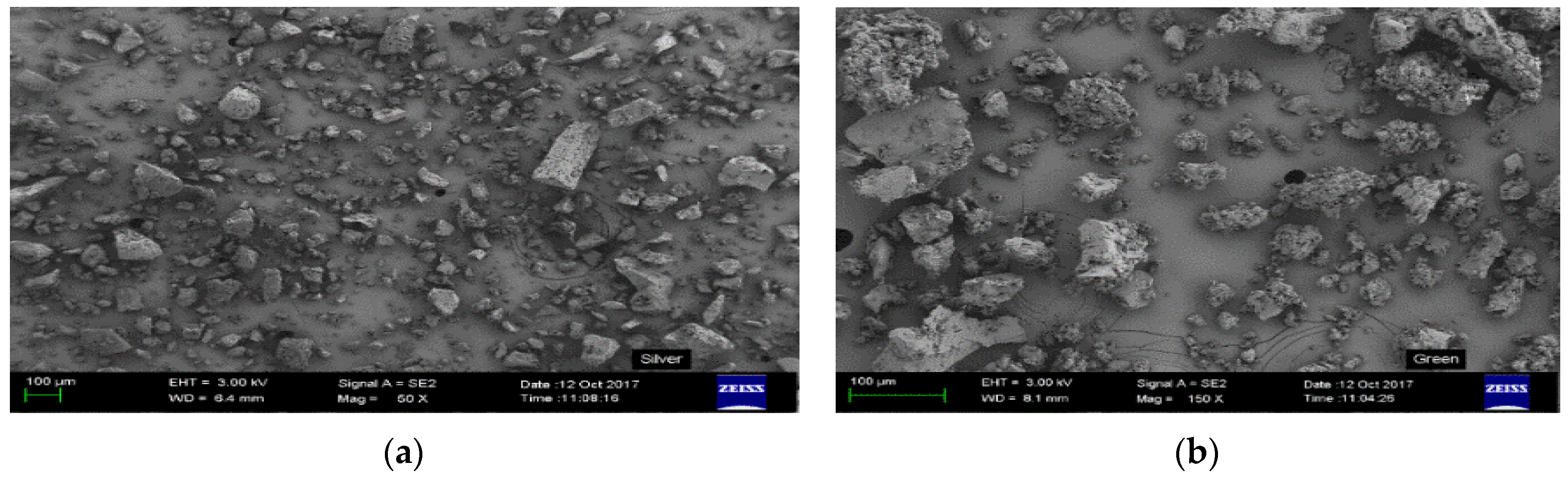
3.4. Surface Morphology, Area and Pore Volume Analysis of the Resins
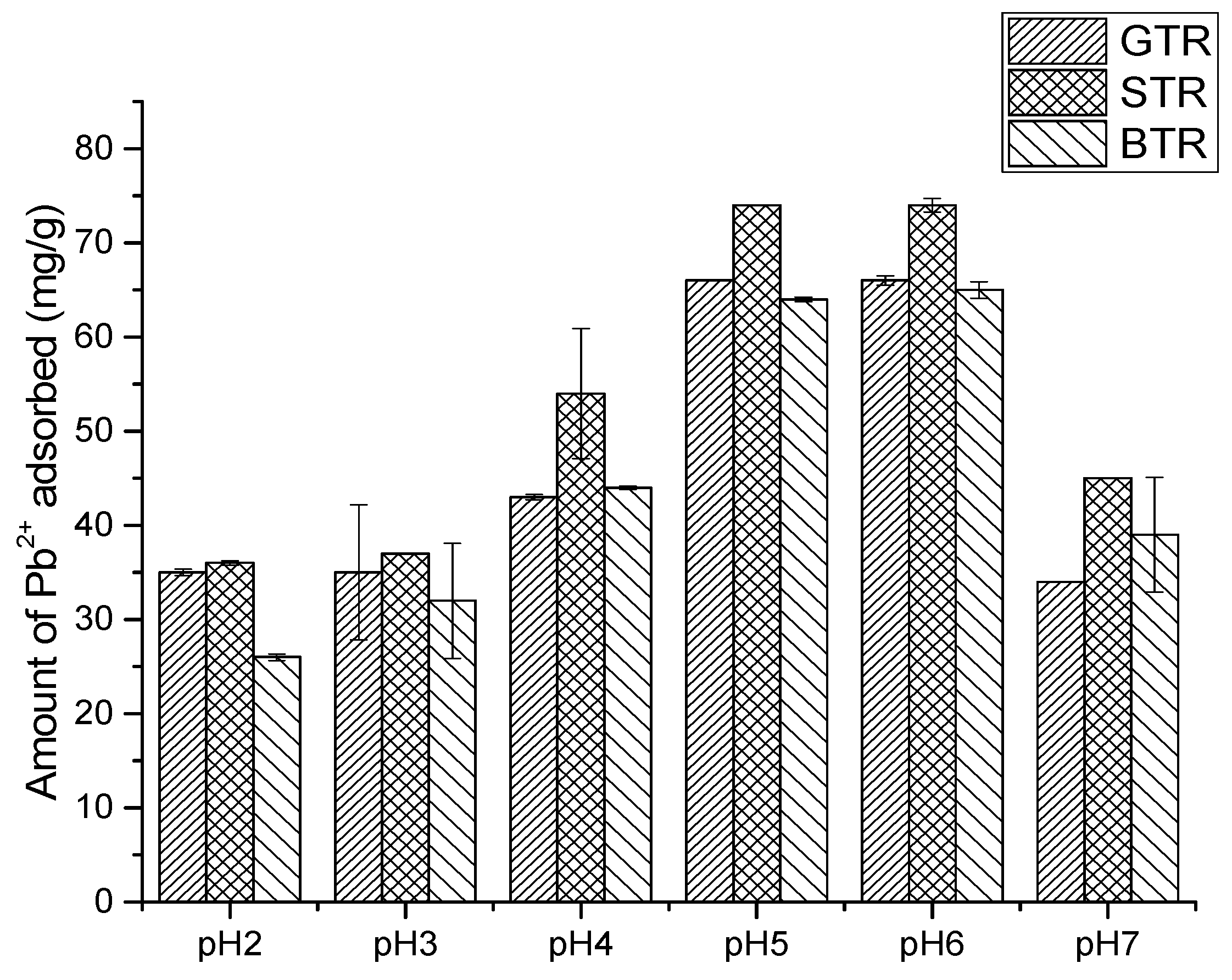
3.5. pH and Temperature Dependence of the Resins on Pb2+ Uptake
3.6. Evaluation of Kinetics Results
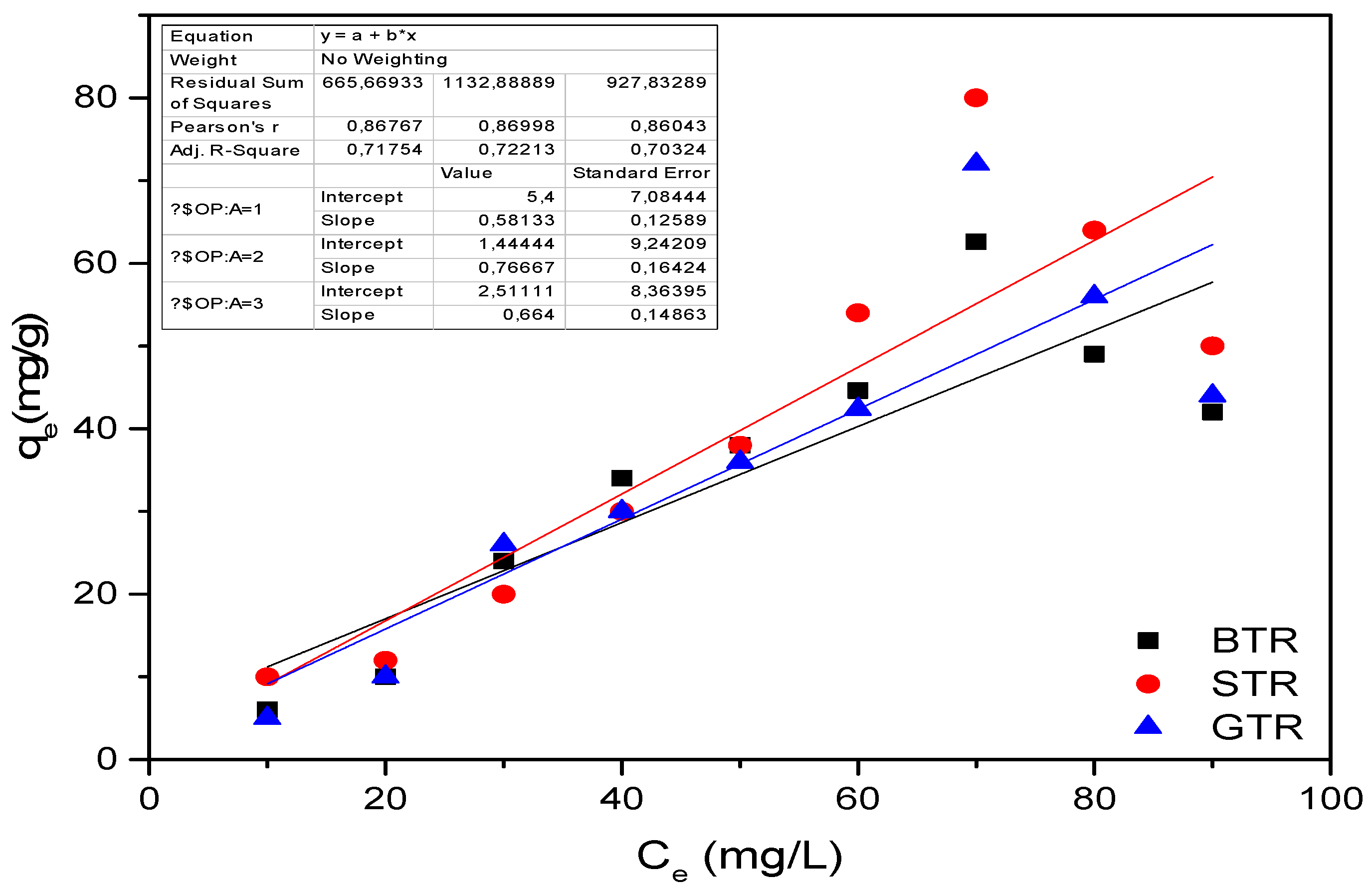
3.7. Evaluation of the Isotherm Results
4. Conclusions
Supporting Information
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Abbreviations
| XRD | X-ray Diffractometer |
| BET | Brunauer–Emmett–Teller |
| TGA | Thermogravimetric analyzer |
| SEM | Scanning electron microscopy |
| FT-IR | Fourier transform infrared spectroscopy |
| ST | Silver Wattle Tannin |
| BT | Black Wattle Tannin |
| GT | Green Wattle Tannin |
| STR | Silver Wattle Tannin Resin |
| GTR | Green Wattle Tannin Resin |
| BTR | Black Wattle Tannin Resin |
| ICP-OES | Inductively coupled plasma optical emission spectrometer |
| * | After adsorption |
References
- Bharti, N.; Katyal, D. Water quality indices used for surface water vulnerability assessment. J. Environ. Sci. 2011, 2, 154–173. [Google Scholar] [CrossRef]
- Manara, A. Plants and heavy metals. Signal Transduct. 2012, 27–54. [Google Scholar] [CrossRef]
- Agência Nacional das Águas (ANA). Cuidando das águas: Soluções para melhorar a qualidade dos recursos hídricos; Agência Nacional das Águas (ANA): Brasília, Brazil, 2011; ISBN 978-85-8210-018-9. (In Portuguese) [Google Scholar]
- Duse, A.G.; Da Silva, M.P.; Zietsman, I. Coping with hygiene in South Africa, a water scarce country. Int. J. Environ. Health Res. 2003, 13 (Suppl. 1), S95–S105. [Google Scholar] [CrossRef] [PubMed]
- Chamier, J.; Schachtschneider, K.; le Maitre, D.C.; Ashton, P.J.; van Wilgen, B.W. Impacts of invasive alien plants on water quality, with particular emphasis on South Africa. Water SA 2012, 38, 345–356. [Google Scholar] [CrossRef]
- Calder, I.R.; Dye, P. Hydrological impacts of invasive alien plants. Land Use Water Resour. Res. 2001, 1, 1–8. [Google Scholar]
- Görgens, A.H.M.; Van Wilgen, B.W. Invasive alien plants and water resources in South Africa: Current understanding, predictive ability and research challenges. S. Afr. J. Sci. 2004, 100, 27–33. [Google Scholar]
- Wells, M.J.; Balsinhas, A.A.; Joffe, H.; Engelbrecht, V.M.; Harding, G.; Stirton, C.H. Acatalogue of problem plants in Southern Africa. In Catalogue of Problem Plants in Southern Africa; Botanical Research Institute: Lucknow, India, 1986. [Google Scholar]
- Dogra, K.; Sood, S.; Dobhal, P.; Seema, S. Alien plant invasion and their impact on indigenous species diversity at global scale: A review. J. Ecol. Nat. Environ. 2010, 2, 175–186. [Google Scholar]
- Anoop Krishnan, A.; Anirudhan, T.S. Removal of lead(II) in the presence of organic ligand from aqueous solution using activated carbon. Indian J. Environ. Prot. 2002, 22, 52–59. [Google Scholar]
- Issabayeva, G.; Aroua, M.K.; Sulaiman, N.M.N. Removal of lead from aqueous solutions on palm shell activated carbon. Bioresour. Technol. 2006, 97, 2350–2355. [Google Scholar] [CrossRef] [PubMed]
- Nriagu, J.O. Lead orthophosphates. I. Solubility and hydrolysis of secondary lead orthophosphate. Inorg. Chem. 1972, 11, 2499–2503. [Google Scholar] [CrossRef]
- Can, M.; Bulut, E.; örnek, A.; özacar, M. Synthesis and characterization of Valonea tannin resin and its interaction with palladium (II), rhodium (III) chloro complexes. Chem. Eng. J. 2013, 221, 146–158. [Google Scholar] [CrossRef]
- Bin, Z.; Shawon, Z. Synthesis and characterization of janus magnetic nanoparticles and its application as an adsorbent. J. Chem. Eng. 2012, 27, 64–68. [Google Scholar]
- Peres, R.S.; Armelin, E.; Alemán, C.; Ferreira, C.A. Modified tannin extracted from black wattle tree as an environmentally friendly antifouling pigment. Ind. Crops Prod. 2015, 65, 506–514. [Google Scholar] [CrossRef]
- Zhao, C.; Zheng, H.; Sun, Y.; Liu, B.; Zhou, Y.; Liu, Y.; Zheng, X. Fabrication of tannin-based dithiocarbamate biosorbent and its application for Ni(II) Ion removal. Water. Air Soil Pollut. 2016, 228. [Google Scholar] [CrossRef]
- Binaeian, E.; Seghatoleslami, N.; Chaichi, M.J. Synthesis of oak gall tannin-immobilized hexagonal mesoporous silicate (OGT-HMS) as a new super adsorbent for the removal of anionic dye from aqueous solution. Desalin. Water Treat. 2016, 57, 8420–8436. [Google Scholar] [CrossRef]
- Karamać, M. Fe(II), Cu(II) and Zn(II) chelating activity of buckwheat and buckwheat tannin groats tannin fractions. Polish J. Food Nutr. Sci. 2007, 57, 357–362. [Google Scholar]
- Karamać, M. Chelation of Cu(II), Zn(II), and Fe(II) by tannin constituents of selected edible nuts. Int. J. Mol. Sci. 2009, 10, 5485–5497. [Google Scholar] [CrossRef] [PubMed]
- Santana Romero, J.L.; Martínez Luzardo, F.; Codorniú Hernández, E.; Vargas Guerra, L.; Melo Cala, P.; García Guillén, M.; Isaac Olivé, K.; Estevez, P.; Roque Córdoba, A.; Benítez, M. Radioisotope method for characterization of vegetable tannins, extracted from waste of forestry production in Cuba. J. Radioanal. Nucl. Chem. 2002, 253, 101–106. [Google Scholar] [CrossRef]
- Liao, X.; Zhang, M.; Shi, B. Collagen-fiber-immobilized tannins and their adsorption of Au(III). Ind. Eng. Chem. Res. 2004, 43, 2222–2227. [Google Scholar] [CrossRef]
- Chibata, I.; Tosa, T.; Mori, T.; Watanabe, T.; Sakata, N. Immobilized tannin—A novel adsorbent for protein and metal ion. Enzym. Microb. Technol. 1986, 8, 130–136. [Google Scholar] [CrossRef]
- Bamidele, O.; Nnana, M.; Imelda, L.; Sekomeng, M. Acacia decurrens (wild) an invasive South Africa tree: Chemical profile, antibacterial and antioxidant activities. Org. Med. Chem IJ 2017, 3. [Google Scholar] [CrossRef]
- Guillemaud, T.; Ciosi, M.; Lombaert, É.; Estoup, A. Biological invasions in agricultural settings: Insights from evolutionary biology and population genetics. C.R. Biol. 2011, 334, 237–246. [Google Scholar] [CrossRef] [PubMed]
- Kaur, C.; Kapoor, H.C. Anti-oxidant activity and total phenolic content of some Asian vegetables. Int. J. Food Sci. Technol. 2002, 37, 153–161. [Google Scholar] [CrossRef]
- Shirato, W. Method of Preparing Insoluble Hydrolysable Tannin and Method of Treating Waste Liquid with the Tannin. U.S. Patent 5,274,169, 28 September 1993. [Google Scholar]
- Ho, Y.S.; Mckay, G. A Comparison of chemisorption kinetic models applied to pollutant removal on various sorbents. Process Saf. Environ. Prot. 1998, 76, 332–340. [Google Scholar] [CrossRef]
- Ho, Y.-S. Removal of copper ions from aqueous solution by tree fern. Water Res. 2003, 37, 2323–2330. [Google Scholar] [CrossRef]
- Cheung, C.W.; Porter, J.F.; Mckay, G. Sorption kinetic analysis for the removal of cadmium ions from effluents using bone char. Water Res. 2001, 35, 605–612. [Google Scholar] [CrossRef]
- Weber, W.J.; Morris, J.C. Kinetics of adsorption on carbon from solution. J. Sanit. Eng. Div. 1963, 89, 31–60. [Google Scholar]
- Langmuir, I. Adsorption of gases on plain surface of glass mica platinum. J. Am. Chem. Soc. 1918, 40, 1361–1403. [Google Scholar] [CrossRef]
- Dubinin, M.M.; Radushkevich, L.V. The equation of the characteristic curve of activated charcoal. Proc. Acad. Sci. Phys. Chem. Sect. 1947, 55, 331. [Google Scholar] [CrossRef][Green Version]
- Namasivayam, C.; Sureshkumar, M.V. Removal of chromium(VI) from water and wastewater using surfactant modified coconut coir pith as a biosorbent. Bioresour. Technol. 2008, 99, 2218–2225. [Google Scholar] [CrossRef] [PubMed]
- Ho, Y.S.; Ng, J.C.Y.; McKay, G. Removal of lead(II) from effluents by sorption on peat using second-order kinetics. Sep. Sci. Technol. 2001, 36, 241–261. [Google Scholar] [CrossRef]
- Freundlich, H. Über die adsorption in Lösungen. Z. Phys. Chem. 1907, 57, 385–470. [Google Scholar] [CrossRef]
- Sieniawska, E.; Baj, T. Tannins. In Pharmacognosy: Fundamentals, Applications and Strategy; Elsevier: New York, NY, USA, 2016; pp. 199–232. ISBN 9780128020999. [Google Scholar]
- Swanson, B.G. Tannins and polyphenols. In Encyclopedia of Food Sciences and Nutrition; Elsevier: New York, NY, USA, 2003; pp. 5729–5733. ISBN 9780122270550. [Google Scholar]
- Sepe, M. Why (And What) You Need to Dry. Plast. Technol. 2014. Available online: https://www.ptonline.com/articles/why-and-what-you-need-to-dry (accessed on 23 April 2018).
- Baschek, G.; Hartwig, G.; Zahradnik, F. Effect of water absorption in polymers at low and high temperatures. Polymer 1999, 40, 3433–3441. [Google Scholar] [CrossRef]
- Ye, Z.B.; Cheng, L.; Xiang, W.T.; Liu, Y.G.; Li, J.H. Characterization of resins and the adsorption of resins on asphaltene particles. Xinan Shiyou Daxue Xuebao/J. Southwest Pet. Univ. 2010, 32, 147–154. [Google Scholar] [CrossRef]
- Grabowska, B.; Holtzer, M. Structural examination of the cross-linking reaction mechanism of polyacrylate binding agents. Arch. Metall. Mater. 2009, 54, 427–437. [Google Scholar]
- González-González, M.; Cabanelas, J.C.; Baselga, J. Applications of FTIR on epoxy resins – identification, monitoring the curing process, phase separation and water uptake. In Infrared Spectroscopy-Materials Science, Engineering and Technology; Theophile, T., Ed.; InTech: Rijeka, Croatia, 2012; Volume 2, pp. 261–284. [Google Scholar]
- Almeida, E. Surface treatments and coatings for metals. A general overview. 1. Surface treatments, surface preparation, and the nature of coatings. Ind. Eng. Chem. Res. 2001, 40, 3–14. [Google Scholar] [CrossRef]
- Narayanan, T.S.N. Surface pretreatment by phospahte conversion coatings—A review. History 2005, 9, 130–177. [Google Scholar]
- Peres, R.S.; Cassel, E.; Ferreira, C.A.; Azambuja, D.S. Grain refiner effect of black wattle tannin in iron and zinc phosphate coatings. Ind. Eng. Chem. Res. 2014, 53, 2706–2712. [Google Scholar] [CrossRef]
- Mangun, C.L.; Daley, M.A.; Braatz, R.D.; Economy, J. Effect of pore size on adsorption of hydrocarbons in phenolic-based activated carbon fibers. Carbon 1998, 36, 123–129. [Google Scholar] [CrossRef]
- Liao, X.; Li, L.; Shi, B. Adsorption recovery of thorium(IV) by Myrica rubra tannin and larch tannin immobilized onto collagen fibres. J. Radioanal. Nucl. Chem. 2004, 260, 619–625. [Google Scholar] [CrossRef]
- Dho, N.Y.; Lee, S.R. Effect of temperature on single and competitive adsorptions of {Cu} ({II}) and {Zn} ({II}) onto natural clays. Environ. Monit. Assess. 2003, 83, 177–203. [Google Scholar] [CrossRef] [PubMed]
- Seward, T.M. The formation of lead (II) chloride complexes to 300 C: A spectrophotometric study. Geochim. Cosmochim. Acta 1984, 48, 121–134. [Google Scholar] [CrossRef]
- Luo, Y.; Millero, F.J. Stability constants for the formation of lead chloride complexes as a function of temperature and ionic strength. Geochim. Cosmochim. Acta 2007, 71, 326–334. [Google Scholar] [CrossRef] [PubMed]
- Luzardo, F.H.M.; Velasco, F.G.; Correia, I.K.S.; Silva, P.M.S.; Salay, L.C. Removal of lead ions from water using a resin of Mimosa tannin and carbon nanotubes. Environ. Technol. Innov. 2017, 7, 219–228. [Google Scholar] [CrossRef]
- Fabbricino, M.; Ferraro, A.; Luongo, V.; Pontoni, L.; Race, M. Soil washing optimization, recycling of the solution, and ecotoxicity assessment for the remediation of Pb-contaminated sites using EDDS. Sustainability 2018, 10, 636. [Google Scholar] [CrossRef]
- Aharoni, C.; Ungarish, M. Kinetics of activated chemisorptions. Part I: The non-elovichian part of the isotherm. J. Chem. Soc. Faraday Trans. 1976, 72, 265–268. [Google Scholar] [CrossRef]
- Onyang, M.S.; Kojima, Y.; Aoyi, O.; Bernardo, E.C.; Matsuda, H. Adsorption equilibrium modeling and solution chemistry dependence of fluoride removal from water by trivalent-cation-exchanged zeolite F-9. J. Interface Sci. 2004, 279, 341–350. [Google Scholar] [CrossRef] [PubMed]
- Hameed, B.H. Equilibrium and kinetic studies of metil violet sorption by agricultural waste. J. Hazard. Mater. 2008, 154, 204–212. [Google Scholar] [CrossRef] [PubMed]
- Hameed, B.H.; Mahmoud, D.K.; Ahmad, A.L. Equilibrium modeling and kinetic studies on the adsorption of basic dye by a low-cost adsorbent: Coconut (Cocos nucifera) bunch waste. J. Hazard. Mater. 2008, 158, 65–72. [Google Scholar] [CrossRef] [PubMed]
- Sekar, M.; Sakthi, V.; Rengaraj, S. Kinetics and equilibrium adsorption study of lead(II) onto activated carbon prepared from coconut shell. J. Colloid Interface Sci. 2004, 279, 307–313. [Google Scholar] [CrossRef] [PubMed]



| Synthesized Resin | Surface Area (m²/g) | Pore Volume (cc/g) |
|---|---|---|
| GTR | 2.38 | 9 × 10−3 |
| STR | 8.65 | 7 × 10−3 |
| BTR | 2.31 | 9 × 10−3 |
| Resins | Optimal Temperature (K) | Amount of Pb2+ Absorbed (mg/g) |
|---|---|---|
| STR | 328 | 81.65 |
| BTR | 294 | 69.96 |
| GTR | 294 | 66.18 |
| Resins | (mg/g) | Pseudo-First-Order Equation | Pseudo-Second-Order Equation | ||||
| K1 () | (mg/g) | r2 | K2 (g/mg min) | (mg/g) | r2 | ||
| STR | 93.37 | 0.067 | 10.63 | 0.966 | 0.055 | 93.87 | 0.953 |
| BTR | 83.43 | 0.073 | 68.36 | 0.853 | 0.042 | 84.64 | 0.958 |
| GTR | 63.74 | 0.063 | 52.27 | 0.912 | 0.074 | 64.83 | 0.983 |
| Resins | (mg/g) | Elovich Equation | Intraparticle-Diffusion Equation | ||||
| α (mg/g min) | β (g/min) | r2 | Kint (mg/g min1/2) | r2 | |||
| STR | 73.37 | 5.363 | 6.323 | 0.993 | 0.367 | 0.983 | |
| BTR | 65.34 | 6473 | 3.345 | 0.835 | 0.647 | 0.783 | |
| GTR | 53.62 | 356.7 | 2.566 | 0.543 | 0.326 | 0.823 | |
| Resins | Langmuir | Dubinin–Radushkevich | ||||||
| (L/g) | (L/mg) | (mg/g) | (mmol/g) | (mmol2/J2) | (KJ/mol) | |||
| STR | 37.84 | 0.326 | 189.30 | 0.9966 | 465.40 | 1 10-4 | 2.24 | 0.9744 |
| BTR | 13.98 | 0.363 | 105.70 | 0.9937 | 746.50 | 1 10-4 | 2.24 | 0.9367 |
| GTR | 11.83 | 0.464 | 98.82 | 0.9832 | 582.30 | 1 10-5 | 7.07 | 0.9543 |
| Resins | Temkin | Freundlich | ||||||
| (L/g) | ||||||||
| STR | 14.74 | 127.60 | 0.9655 | 87.38 | 7.773 | 0.7865 | ||
| BTR | 10.73 | 32.32 | 0.9561 | 64.88 | 6.237 | 0.8853 | ||
| GTR | 2.83 | 45.37 | 0.9533 | 53.73 | 7.467 | 0.8335 | ||
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Okoli, B.J.; Shilowa, P.M.; Anyanwu, G.O.; Modise, J.S. Removal of Pb2+ from Water by Synthesized Tannin Resins from Invasive South African Trees. Water 2018, 10, 648. https://doi.org/10.3390/w10050648
Okoli BJ, Shilowa PM, Anyanwu GO, Modise JS. Removal of Pb2+ from Water by Synthesized Tannin Resins from Invasive South African Trees. Water. 2018; 10(5):648. https://doi.org/10.3390/w10050648
Chicago/Turabian StyleOkoli, Bamidele J., Patience M. Shilowa, Gabriel O. Anyanwu, and Johannes S. Modise. 2018. "Removal of Pb2+ from Water by Synthesized Tannin Resins from Invasive South African Trees" Water 10, no. 5: 648. https://doi.org/10.3390/w10050648
APA StyleOkoli, B. J., Shilowa, P. M., Anyanwu, G. O., & Modise, J. S. (2018). Removal of Pb2+ from Water by Synthesized Tannin Resins from Invasive South African Trees. Water, 10(5), 648. https://doi.org/10.3390/w10050648






