The Impact of Shrubby Floodplain Vegetation Growth on the Discharge Capacity of River Valleys
Abstract
1. Introduction
2. Materials and Methods
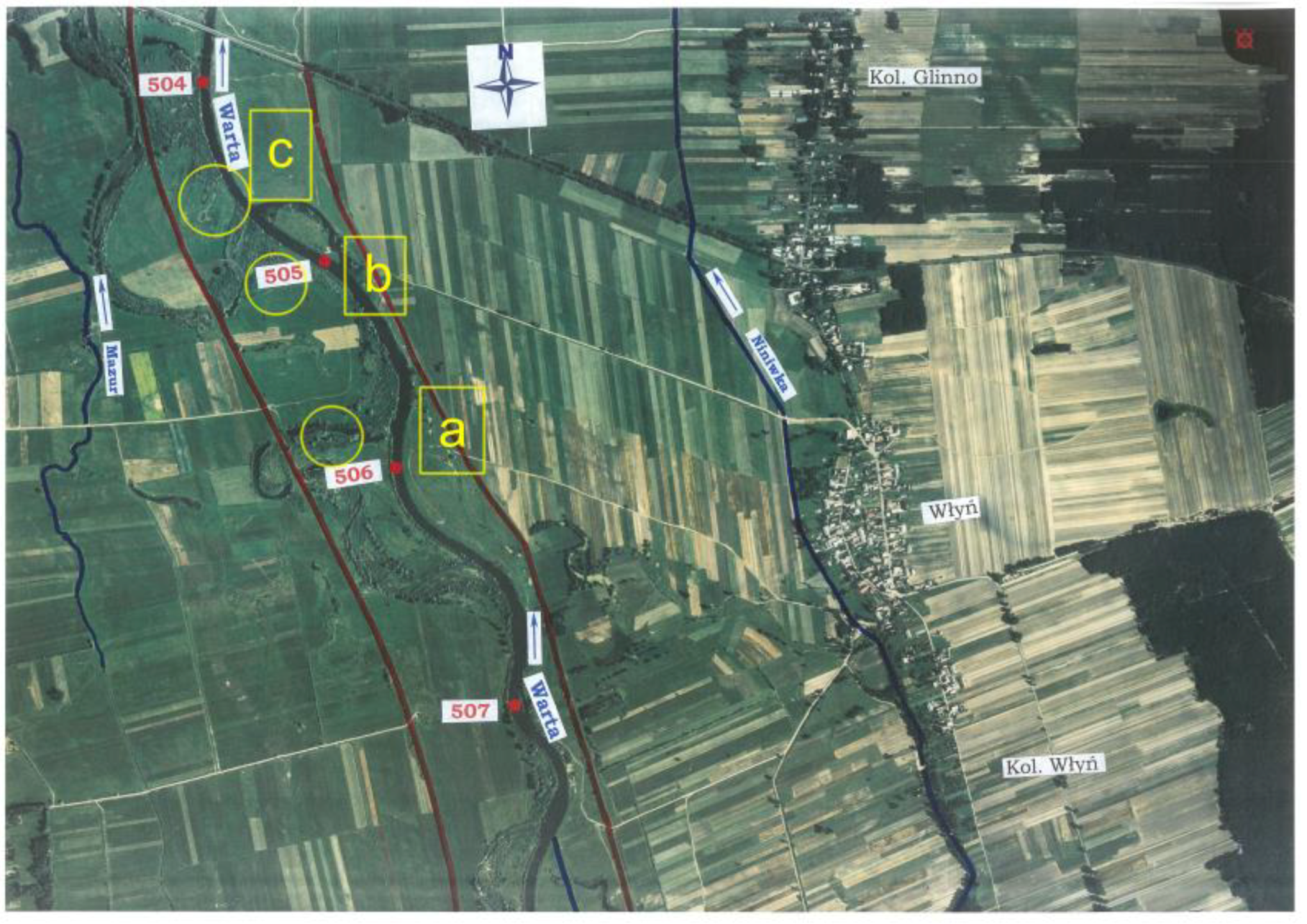
2.1. General Characteristics of the Research Area and Field Measurement
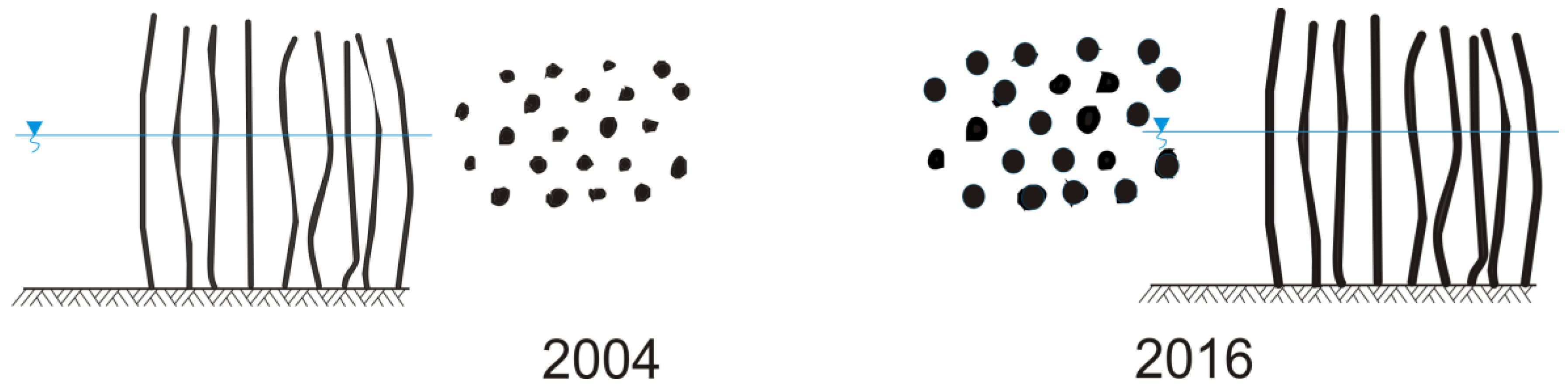
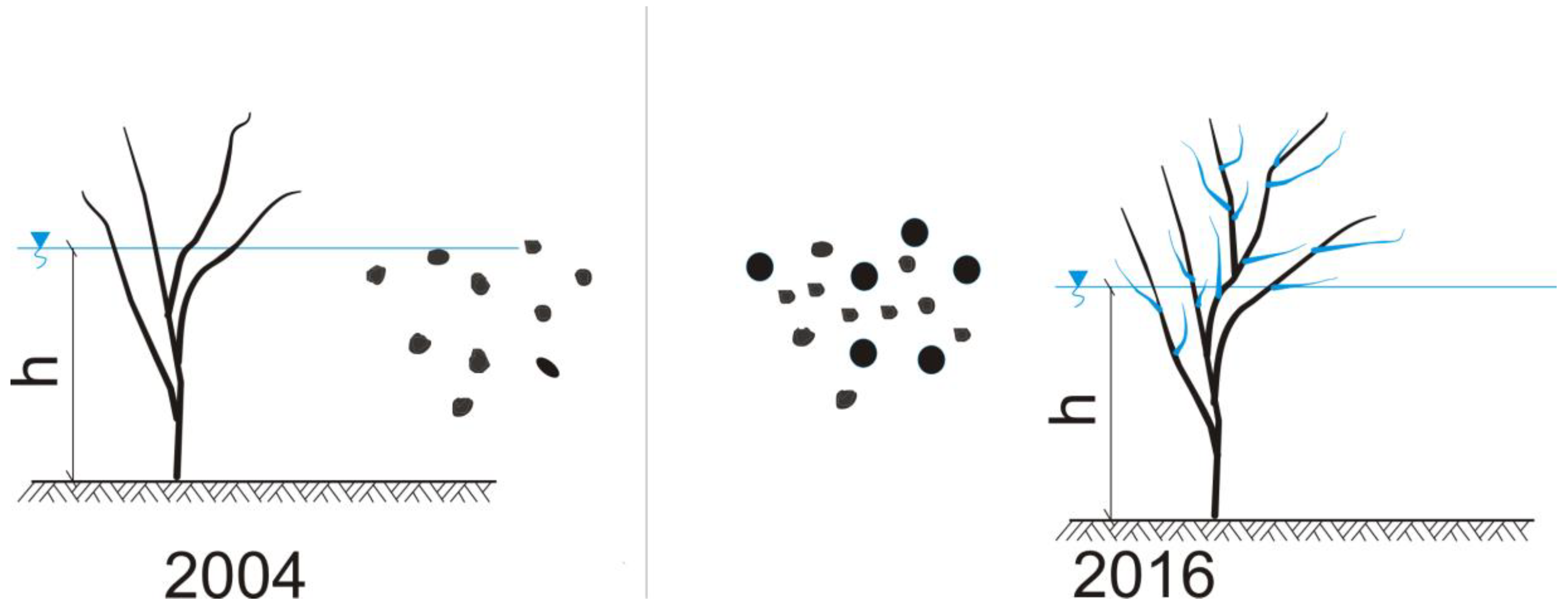
2.2. Methodology
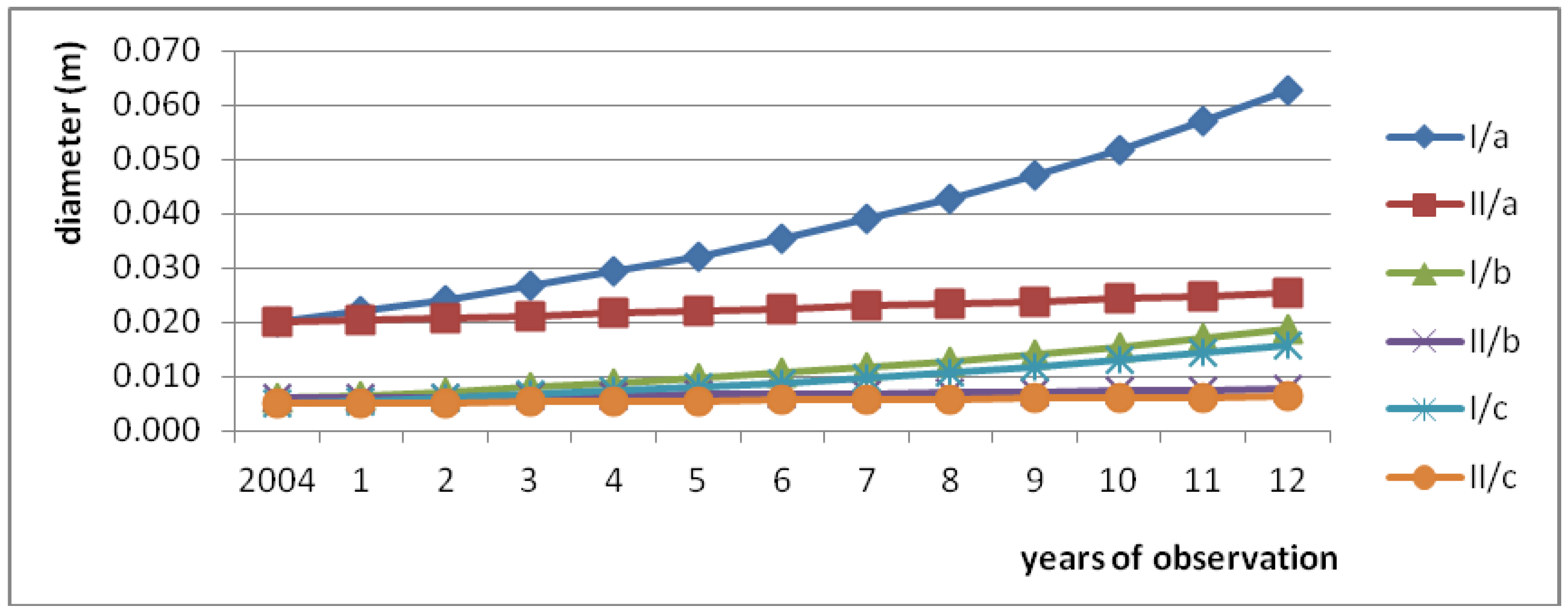
3. Results and Discussion
4. Conclusions
Supplementary Materials
Author Contributions
Conflicts of Interest
References
- Gurnell, A.M.; Bertoldi, W.; Corenblit, D. Changing river channels: The roles of hydrological processes, plants and pioneer fluvial landforms in humid temperate, mixed load, gravel bed rivers. Earth-Sci. Rev. 2012, 111, 129–141. [Google Scholar] [CrossRef]
- Crosato, A.; Saleh, M.S. Numerical study on the effects of floodplain vegetation on river planform style. Earth Surf. Process. Landf. 2011, 36, 711–720. [Google Scholar] [CrossRef]
- Tabacchi, E.; Correll, D.L.; Hauer, R.; Pinay, G.; Planty-Tabacchi, A.-M.; Wissmar, R.C. Development, maintenance and role of riparian vegetation in the river landscape. Freshw. Biol. 1998, 40, 497–516. [Google Scholar] [CrossRef]
- Murray, A.B.; Chris, P. Modelling the effect of vegetation on channel pattern in bedload rivers. Earth Surf. Process. Landf. 2003, 28, 131–143. [Google Scholar] [CrossRef]
- Schiechtl, H.M.; Stern, R. Naturnaher Wasserbau: Anleitung für Ingenieurbiologische Bauweisen; John Wiley & Sons: Berlin, Germany, 2002. [Google Scholar]
- Chen, S.-C.; Kuo, Y.-M.; Li, Y.-H. Flow characteristics within different configurations of submerged flexible vegetation. J. Hydrol. 2011, 398, 124–134. [Google Scholar] [CrossRef]
- Meire, D.W.; Kondziolka, J.M.; Nepf, H.M. Interaction between neighboring vegetation patches: Impact on flow and deposition. Water Resour. Res. 2014, 50, 3809–3825. [Google Scholar] [CrossRef]
- Bertoldi, W.; Welber, M.; Gurnell, A.M.; Mao, L.; Comiti, F.; Tal, M. Physical modelling of the combined effect of vegetation and wood on river morphology. Geomorphology 2015, 246, 178–187. [Google Scholar] [CrossRef]
- Nepf, H.M. Flow and transport in regions with aquatic vegetation. Ann. Rev. Fluid Mech. 2012, 44, 123–142. [Google Scholar] [CrossRef]
- Mazur, R.; Kałuża, T.; Chmist, J.; Walczak, N.; Laks, I.; Strzeliński, P. Influence of deposition of fine plant debris in river floodplain shrubs on flood flow conditions–The Warta River case study. Phys. Chem. Earth Parts A/B/C 2016, 94, 106–113. [Google Scholar] [CrossRef]
- Yang, P.-P.; Zhang, H.-L.; Ma, C. Effects of simulated submerged and rigid vegetation and grain roughness on hydraulic resistance to simulated overland flow. J. Mt. Sci. 2017, 14, 2042–2052. [Google Scholar] [CrossRef]
- Walczak, N.; Walczak, Z.; Hämmerling, M.; Przedwojski, B. Analytical model for vertical velocity distribution and hydraulic roughness at the flow through river bed and valley with vegetation. Rocznik Ochrona Środowiska 2013, 15, 405–419. [Google Scholar]
- Lee, J.K.; Roig, L.C.; Jenter, H.L.; Visser, H.M. Drag coefficients for modeling flow through emergent vegetation in the Florida Everglades. Ecol. Eng. 2004, 22, 237–248. [Google Scholar] [CrossRef]
- Zhao, M.; Fan, Z. Hydrodynamic characteristics of submerged vegetation flow with non-constant vertical porosity. PLoS ONE 2017, 12, e0176712. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Zeng, Y.; Huai, W.; Zhao, M. Flow characteristics of rectangular open channels with compound vegetation roughness. Appl. Math. Mech. 2016, 37, 341–348. [Google Scholar] [CrossRef]
- Jordanova, A.A.; James, C.S.; Birkhead, A.L. Practical estimation of flow resistance through emergent vegetation. Proc. Inst. Civ. Eng.-Water Manag. 2006, 159, 173–181. [Google Scholar] [CrossRef]
- Hui, E.-Q.; Hu, X.-E.; Jiang, C.-B.; Zhu, Z.-D. A study of drag coefficient related with vegetation based on the flume experiment. J. Hydrodyn. Ser. B 2010, 22, 329–337. [Google Scholar] [CrossRef]
- Aberle, J.; Järvelä, J. Flow resistance of emergent rigid and flexible floodplain vegetation. J. Hydraul. Res. 2013, 51, 33–45. [Google Scholar] [CrossRef]
- Miyab, N.M.; Afzalimehr, H.; Singh, V.P.; Ghorbani, B. On Flow Resistance Due to Vegetation in a Gravel-Bed River. Int. J. Hydraul. Eng. 2014, 3, 85–92. [Google Scholar]
- Gurnell, A. Plants as river system engineers. Earth Surf. Process. Landf. 2014, 39, 4–25. [Google Scholar] [CrossRef]
- Uijttewaal, W.S.J. Hydrodynamics of shallow flows: Application to rivers. J. Hydraul. Res. 2014, 52, 157–172. [Google Scholar] [CrossRef]
- Verschoren, V.; Meire, D.; Schoelynck, J.; Buis, K.; Bal, K.D.; Troch, P.; Meire, P.; Temmerman, S. Resistance and reconfiguration of natural flexible submerged vegetation in hydrodynamic river modelling. Environ. Fluid Mech. 2016, 16, 245–265. [Google Scholar] [CrossRef]
- Meitzen, K.M.; Phillips, J.N.; Perkins, T.; Manning, A.; Julian, J.P. Catastrophic flood disturbance and a community’s response to plant resilience in the heart of the Texas Hill Country. Geomorphology 2018, 305, 20–32. [Google Scholar] [CrossRef]
- Ishikawa, Y.; Sakamoto, T.; Mizuhara, K. Effect of density of riparian vegetation on effective tractive force. J. For. Res. 2003, 8, 235–246. [Google Scholar] [CrossRef]
- Gu, F.-F.; Ni, H.-G.; Qi, D.-M. Roughness coefficient for unsubmerged and submerged reed. J. Hydrodyn. Ser. B 2007, 19, 421–428. [Google Scholar] [CrossRef]
- Zhang, M.; Li, C.W.; Shen, Y. Depth-averaged modeling of free surface flows in open channels with emerged and submerged vegetation. Appl. Math. Model. 2013, 37, 540–553. [Google Scholar] [CrossRef]
- Aberle, J.; Järvelä, J. Hydrodynamics of vegetated channels. In Rivers—Physical, Fluvial and Environmental Processes; Rowinski, P., Radecki-Pawlik, A., Eds.; Springer International Publishing: Cham, Switzerland, 2015; pp. 519–541. [Google Scholar]
- Gran, K.; Paola, C. Riparian vegetation controls on braided stream dynamics. Water Resour. Res. 2001, 37, 3275–3283. [Google Scholar] [CrossRef]
- Politti, E.; Bertoldi, W.; Gurnell, A.; Henshaw, A. Feedbacks between the riparian Salicaceae and hydrogeomorphic processes:A quantitative review. Earth-Sci. Rev. 2018, 176, 147–165. [Google Scholar] [CrossRef]
- Merritt, D.M.; Scott, M.L.; Leroy Poff, N.; Auble, G.T.; Lytle, D.A. Theory, methods and tools for determining environmental flows for riparian vegetation: Riparian vegetation-flow response guilds. Freshw. Biol. 2010, 55, 206–225. [Google Scholar] [CrossRef]
- Nilsson, C.; Berggren, K. Alterations of riparian ecosystems caused by river regulation: Dam operations have caused global-scale ecological changes in riparian ecosystems. How to protect river environments and human needs of rivers remains one of the most important questions of our time. BioScience 2000, 50, 783–792. [Google Scholar]
- Bätz, N.; Colombini, P.; Cherubini, P.; Lane, S.N. Groundwater controls on biogeomorphic succession and river channel morphodynamics. J. Geophys. Res. Earth Surf. 2016, 121, 1763–1785. [Google Scholar] [CrossRef]
- Gullberg, U. Towards making willows pilot species for coppicing production. For. Chron. 1993, 69, 721–726. [Google Scholar] [CrossRef]
- Haughton, A.J.; Bond, A.J.; Lovett, A.A.; Dockerty, T.; Sünnenberg, G.; Clark, S.J.; Bohan, D.A.; Sage, R.B.; Mallott, M.D.; Mallott, V.E.; et al. A novel, integrated approach to assessing social, economic and environmental implications of changing rural land-use: A case study of perennial biomass crops. J. Appl. Ecol. 2009, 46, 315–322. [Google Scholar] [CrossRef]
- Lisowski, A. Technologie Zbioru Roślin Energetycznych; Wydawnictwo SGGW: Warsaw, Poland, 2010. (In Polish) [Google Scholar]
- Dubas, J.W.; Grzybek, A.; Kotowski, W.; Tomczyk, A. Wierzba Energetyczna—Uprawa i Technologie Przetwarzania; Wyższa Szkoła Ekonomii i Administracji w Bytomiu: Bytom, Poland, 2004; Volume 35. [Google Scholar]
- Bullard, M.J.; Mustill, S.J.; McMillan, S.D.; Nixon, P.M.I.; Carver, P.; Britt, C.P. Yield improvements through modification of planting density and harvest frequency in short rotation coppice Salix spp.—1. Yield response in two morphologically diverse varieties. Biomass Bioenergy 2002, 22, 15–25. [Google Scholar] [CrossRef]
- Melin, G.; Larsson, S. Agrobränsle AB—world leading company on short rotation coppice willow. In Proceedings of the 14th European Biomass Conference, Paris, France, 17–21 October 2005; pp. 36–37. [Google Scholar]
- Hoffmann, D.; Weih, M. Limitations and improvement of the potential utilisation of woody biomass for energy derived from short rotation woody crops in Sweden and Germany. Biomass Bioenergy 2005, 28, 267–279. [Google Scholar] [CrossRef]
- Mola-Yudego, B.; Pelkonen, P. The effects of policy incentives in the adoption of willow short rotation coppice for bioenergy in Sweden. Energy Policy 2008, 36, 3062–3068. [Google Scholar] [CrossRef]
- Kopp, R.F.; Abrahamson, L.P.; White, E.H.; Volk, T.A.; Nowak, C.A.; Fillhart, R.C. Willow biomass production during ten successive annual harvests. Biomass Bioenergy 2001, 20, 1–7. [Google Scholar] [CrossRef]
- Liro, A.; Tederko, Z. Strategia Wdrażania Krajowej Sieci Ekologicznej ECONET-Polska: Praca Zbiorowa; Fundacja IUCN Poland: Warsaw, Poland, 1998. [Google Scholar]
- BirdLife Data Zone. Available online: http://datazone.birdlife.org/site/factsheet/926 (accessed on 10 April 2018).
- Straatsma, M.W.; Middelkoop, H. Airborne laser scanning as a tool for lowland floodplain vegetation monitoring. Hydrobiologia 2006, 565, 87–103. [Google Scholar] [CrossRef]
- Tymiński, T. Hydraulic Model Investigation of Flow Conditions for Floodplains with Coniferous and Deciduous Shrubs. Pol. J. Environ. Stud. 2012, 21, 1047–1052. [Google Scholar]
- Ballesteros, J.A.; Bodoque, J.M.; Díez-Herrero, A.; Sanchez-Silva, M.; Stoffel, M. Calibration of floodplain roughness and estimation of flood discharge based on tree-ring evidence and hydraulic modelling. J. Hydrol. 2011, 403, 103–115. [Google Scholar] [CrossRef]
- Anderson, B.G.; Rutherfurd, I.D.; Western, A.W. An analysis of the influence of riparian vegetation on the propagation of flood waves. Environ. Model. Softw. 2006, 21, 1290–1296. [Google Scholar] [CrossRef]
- Zong, L.; Nepf, H. Flow and deposition in and around a finite patch of vegetation. Geomorphology 2010, 116, 363–372. [Google Scholar] [CrossRef]
- Ortiz, A.C.; Ashton, A.; Nepf, H. Mean and turbulent velocity fields near rigid and flexible plants and the implications for deposition. J. Geophys. Res. Earth Surf. 2013, 118, 2585–2599. [Google Scholar] [CrossRef]
- Vargas-Luna, A.; Crosato, A.; Uijttewaal, W.S.J. Effects of vegetation on flow and sediment transport: Comparative analyses and validation of predicting models. Earth Surf. Process. Landf. 2015, 40, 157–176. [Google Scholar] [CrossRef]
- Wu, F.-S. Characteristics of Flow Resistance in Open Channels with Non-Submerged Rigid Vegetation. J. Hydrodyn. Ser. B 2008, 20, 239–245. [Google Scholar] [CrossRef]
- Thorne, C.R. Effects of vegetation on riverbank erosion and stability. In Vegetation and Erosion: Processes and Environments; John Wiley: Chichester, UK, 1990; pp. 125–144. [Google Scholar]
- Florsheim, J.L.; Mount, J.F.; Chin, A. Bank erosion as a desirable attribute of rivers. AIBS Bull. 2008, 58, 519–529. [Google Scholar] [CrossRef]
- Wang, H.; Tang, H.-W.; Zhao, H.-Q.; Zhao, X.-Y.; Lü, S.-Q. Incipient motion of sediment in presence of submerged flexible vegetation. Water Sci. Eng. 2015, 8, 63–67. [Google Scholar] [CrossRef]
- Västilä, K.; Järvelä, J. Characterizing natural riparian vegetation for modeling of flow and suspended sediment transport. J. Soils Sediments 2017, 1–17. [Google Scholar] [CrossRef]
- Walczak, N.; Walczak, Z.; Hämmerling, M.; Spychala, M.; Niec, J. Head Losses in Small Hydropower Plant Trash Racks (SHP). Acta Sci. Pol. Form. Circumiectus 2016, 15, 369–382. [Google Scholar]
- Vries, P.G. Sampling Theory for Forest Inventory: A Teach-Yourself Course; Springer: Berlin/Heidelberg, Germany, 1986. [Google Scholar]
- Kałuża, T. Einfluss der Bewuchsentwicklung auf das Abflussverhalten in Fliessgewassern. Wasserwirtschaft 2009, 99, 29–32. [Google Scholar]
- Juliszewski, T.; Kwaśniewski, D.; Baran, D. Comparison of planting of energetic willow (salix viminalis) in the spring and autumn time. Inżynieria Rolnicza 2005, 9, 251–258. (In Polish) [Google Scholar]
- Rickert, K. Der Einfluß von Gehölzen auf die Lichtverhältnisse und das Abflußverhalten in Fließgewässern; Institut für Wasserwirtschaft, Hydrologie und Landwirtschaftlichen Wasserbau: Hannover, Germany, 1986. (In German) [Google Scholar]
- Kubrak, J.; Kozioł, A.; Kubrak, E.; Wasilewicz, M.; Kiczko, A. Analiza wpływu roślinności na warunki przepływu wody w międzywalu. In Określenie Kryteriów Ustalania Miejsc Przeprowadzania Wycinek i Usuwania Nadmiaru Roślinności. Szkoła Główna Gospodarstwa Wiejskiego w Warszawie; Wydział Budownictwa i Inżynierii Środowiska: Warsaw, Poland, 2012. [Google Scholar]
- Tworkowski, J.; Szczukowski, S.; Stolarski, M. Yielding and morphological characteristics of willow grown in eco-salix system. Fragm. Agron. 2010, 27, 135–146. [Google Scholar]
- Kubrak, J.; Nachlik, E. Hydrauliczne Podstawy Obliczania Przepustowości Koryt Rzecznych; Wydawnictwo SGGW: Warsaw, Poland, 2003. (In Polish) [Google Scholar]
- Lindner, K. Der Strömungswiderstand von Pflanzenbeständen. Mitteilungen 75; Leichtweiss-Institut für Wasserbau, Technische Universität Braunschweig: Braunschweig, Germany, 1982. (In German) [Google Scholar]
- Pasche, E. Turbulenzmechanismen in Naturnahen Fließgewässern und Die Möglichkeit Ihrer Mathematischen Erfassung; Lehrstuhl und Institut für Wasserbau und Wasserwirtschaft: Aachen, Germany, 1984. (In German) [Google Scholar]
- Jovanovic, M.; Pasche, E.; Töppel, M.; Donner, M. 1D-Hydraulic; Technische Universität: Hamburg, Germany, 2006. [Google Scholar]
- Deutscher Verband für Wasserwirtschaft und Kulturbau (DVWK). Hydraulische Berechnung von Fließgewässern; DVWK Merkblätter zur Wasserwirtschaft; Paul Parey: Berlin, Germany, 1991. (In German) [Google Scholar]
- Rickert, K.; Nickel, A. Naturnahe Regelung von Fließgewässern; Unterlagen zum Kurs WH06 des Weiterbildungsstudiums “Wasser und Umwelt” der; Universität Hannover: Hanover, Germany, 2003. (In German) [Google Scholar]
- Stolarski, M.; Kisiel, R.; Szczukowski, S.; Tworkowski, J. Costs of liquidation of short-rotation willow plantation. Roczniki Nauk Rolniczych Seria G 2008, 94, 172–177. (In Polish) [Google Scholar]
- Wrócił Czas Wierzby Energetycznej!—Agrofakt.pl. Available online: https://www.agrofakt.pl/czas-wierzby-energetycznej/ (accessed on 12 April 2018).
- Szczukowski, S.; Budny, J. Wierzba Krzewiasta-Roślina Energetyczna; Wojewódzki Fundusz Ochrony Środowiska i Gospodarki Wodnej: Olsztyn, Poland, 2003. [Google Scholar]
- Stolarski, M.J.; Szczukowski, S.; Tworkowski, J.; Klasa, A. Willow biomass production under conditions of low-input agriculture on marginal soils. For. Ecol. Manag. 2011, 262, 1558–1566. [Google Scholar] [CrossRef]
- Zaliwski, A.S. Technological and organizational aspects of combining agricultural production for food and energy. Agric. Eng. 2013, 4, 399–407. (In Polish) [Google Scholar]
- Szczukowski, S.; Tworkowski, J.; Wiwart, M.; Przyborowski, J. Wiklina (Salix sp.) Uprawa i Możliwości Wykorzystania; Wydawnictwo Uniwersytetu Warmińsko-Mazurskiego: Olsztyn, Poland, 2002. [Google Scholar]
- Swiatek, D.; Wej, A. The computer program RIVER for calculation of the flow capacity of the vegetated river valley. Infrastruktura i Ekologia Terenów Wiejskich 2006, 4, 173–182. (In Polish) [Google Scholar]
- Miroslaw-Swiatek, D.; Amatya, D.M. Effects of cypress knee roughness on flow resistance and discharge estimates of the Turkey Creek watershed. Ann. Wars. Univ. Life Sci. SGGW. Land Reclam. 2017, 49, 179–199. [Google Scholar] [CrossRef]
- Da Silva, Y.J.A.B.; Cantalice, J.R.B.; Singh, V.P.; Cruz, C.M.C.A.; Silva Souza, W.L.D. Sediment transport under the presence and absence of emergent vegetation in a natural alluvial channel from Brazil. Int. J. Sediment Res. 2016, 31, 360–367. [Google Scholar] [CrossRef]
- Romero, M.; Revollo, N.; Molina, J. Flow resistance in steep mountain rivers in Bolivia. J. Hydrodyn. Ser. B 2010, 22, 702–707. [Google Scholar] [CrossRef]
- Gilley, J.E.; Kottwitz, E.R.; Wieman, G.A. Darcy-Weisbach roughness coefficients for gravel and cobble surfaces. J. Irrig. Drain. Eng. 1992, 118, 104–112. [Google Scholar] [CrossRef]
- Han, L.; Zeng, Y.; Chen, L.; Huai, W. Lateral velocity distribution in open channels with partially flexible submerged vegetation. Environ. Fluid Mech. 2016, 16, 1267–1282. [Google Scholar] [CrossRef]
- Thomas, H.; Nisbet, T.R. An assessment of the impact of floodplain woodland on flood flows. Water Environ. J. 2007, 21, 114–126. [Google Scholar] [CrossRef]
- Billi, P.; Fazzini, M. Global change and river flow in Italy. Glob. Planet. Chang. 2017, 155, 234–246. [Google Scholar] [CrossRef]
- Marchese, E.; Scorpio, V.; Fuller, I.; McColl, S.; Comiti, F. Morphological changes in Alpine rivers following the end of the Little Ice Age. Geomorphology 2017, 295, 811–826. [Google Scholar] [CrossRef]
- Arnaud-Fassetta, G. River channel changes in the Rhone Delta (France) since the end of the Little Ice Age: Geomorphological adjustment to hydroclimatic change and natural resource management. Catena 2003, 51, 141–172. [Google Scholar] [CrossRef]
- Comiti, F.; Da Canal, M.; Surian, N.; Mao, L.; Picco, L.; Lenzi, M.A. Channel adjustments and vegetation cover dynamics in a large gravel bed river over the last 200 years. Geomorphology 2011, 125, 147–159. [Google Scholar] [CrossRef]
- Chin, A. Urban transformation of river landscapes in a global context. Geomorphology 2006, 79, 460–487. [Google Scholar] [CrossRef]
- Magilligan, F.J.; Nislow, K.H. Changes in hydrologic regime by dams. Geomorphology 2005, 71, 61–78. [Google Scholar] [CrossRef]
- Gregory, K.J. The human role in changing river channels. Geomorphology 2006, 79, 172–191. [Google Scholar] [CrossRef]
- Benke, K.K.; Lowell, K.E.; Hamilton, A.J. Parameter uncertainty, sensitivity analysis and prediction error in a water-balance hydrological model. Math. Comput. Model. 2008, 47, 1134–1149. [Google Scholar] [CrossRef]
- Zhan, C.-S.; Song, X.-M.; Xia, J.; Tong, C. An efficient integrated approach for global sensitivity analysis of hydrological model parameters. Environ. Model. Softw. 2013, 41, 39–52. [Google Scholar] [CrossRef]
- Song, X.; Zhang, J.; Zhan, C.; Xuan, Y.; Ye, M.; Xu, C. Global sensitivity analysis in hydrological modeling: Review of concepts, methods, theoretical framework, and applications. J. Hydrol. 2015, 523, 739–757. [Google Scholar] [CrossRef]
- Castaings, W.; Dartus, D.; Le Dimet, F.-X.; Saulnier, G.-M. Sensitivity analysis and parameter estimation for distributed hydrological modeling: Potential of variational methods. Hydrol. Earth Syst. Sci. 2009, 13, 503–517. [Google Scholar] [CrossRef]
- Armanini, A.; Righetti, M.; Grisenti, P. Direct measurement of vegetation resistance in prototype scale. J. Hydraul. Res. 2005, 43, 481–487. [Google Scholar] [CrossRef]
- Västilä, K.; Järvelä, J.; Aberle, J. Characteristic reference areas for estimating flow resistance of natural foliated vegetation. J. Hydrol. 2013, 492, 49–60. [Google Scholar] [CrossRef]
- Wilson, C.A.; Hoyt, J.; Schnauder, I. Impact of foliage on the drag force of vegetation in aquatic flows. J. Hydraul. Eng. 2008, 134, 885–891. [Google Scholar] [CrossRef]
- Järvelä, J. Flow resistance of flexible and stiff vegetation: A flume study with natural plants. J. Hydrol. 2002, 269, 44–54. [Google Scholar] [CrossRef]
- Searle, S.Y.; Malins, C.J. Will energy crop yields meet expectations? Biomass Bioenergy 2014, 65, 3–12. [Google Scholar] [CrossRef]
- Bilyeu, D.M.; Cooper, D.J.; Hobbs, N.T. Water tables constrain height recovery of willow on Yellowstone’s northern range. Ecol. Appl. 2008, 18, 80–92. [Google Scholar] [CrossRef] [PubMed]
- Toivonen, R.M.; Tahvanainen, L.J. Profitability of willow cultivation for energy production in Finland. Biomass Bioenergy 1998, 15, 27–37. [Google Scholar] [CrossRef]
- Ericsson, K.; Rosenqvist, H.; Ganko, E.; Pisarek, M.; Nilsson, L. An agro-economic analysis of willow cultivation in Poland. Biomass Bioenergy 2006, 30, 16–27. [Google Scholar] [CrossRef]
- Posthumus, H.; Rouquette, J.R.; Morris, J.; Gowing, D.J.G.; Hess, T.M. A framework for the assessment of ecosystem goods and services; a case study on lowland floodplains in England. Ecol. Econ. 2010, 69, 1510–1523. [Google Scholar] [CrossRef]
- Tabacchi, E.; Lambs, L.; Guilloy, H.; Planty-Tabacchi, A.-M.; Muller, E.; Dcamps, H. Impacts of riparian vegetation on hydrological processes. Hydrol. Process. 2000, 14, 2959–2976. [Google Scholar] [CrossRef]



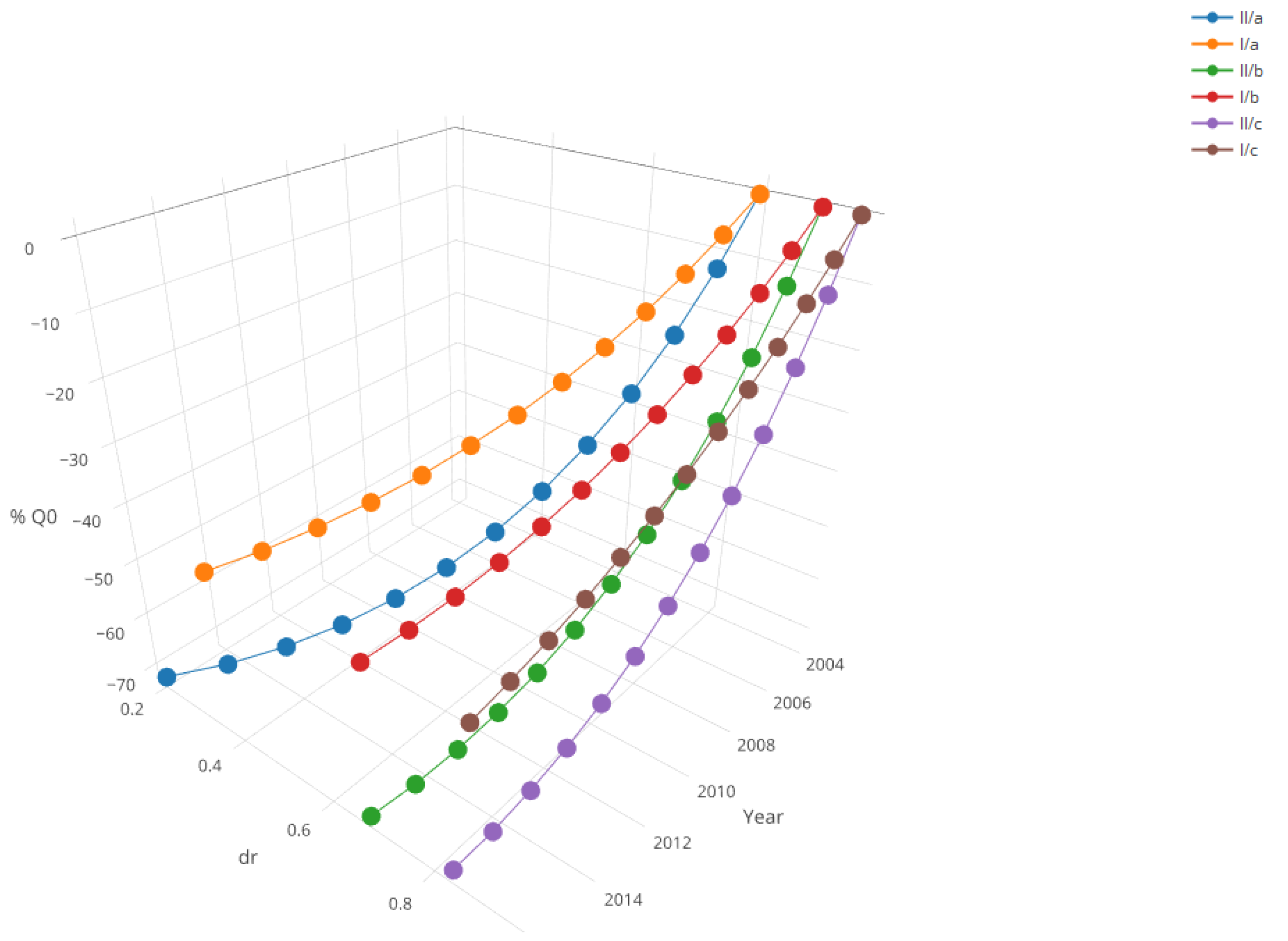
| Parameter | Diameter | Distance | ||
|---|---|---|---|---|
| zone | SD | Cv | SD | Cv |
| I/a | 0.014 | 0.366 | 0.001 | 0.008 |
| II/a | 0.002 | 0.077 | 0.022 | 0.366 |
| I/b | 0.004 | 0.366 | 0.000 | 0.008 |
| II/b | 0.001 | 0.077 | 0.013 | 0.366 |
| I/c | 0.005 | 0.366 | 0.001 | 0.008 |
| II/c | 0.010 | 0.530 | 0.026 | 0.366 |
| Option/Zone | Years of Obsrevation | ||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | |
| Resistance Coefficient (-) | |||||||||||||
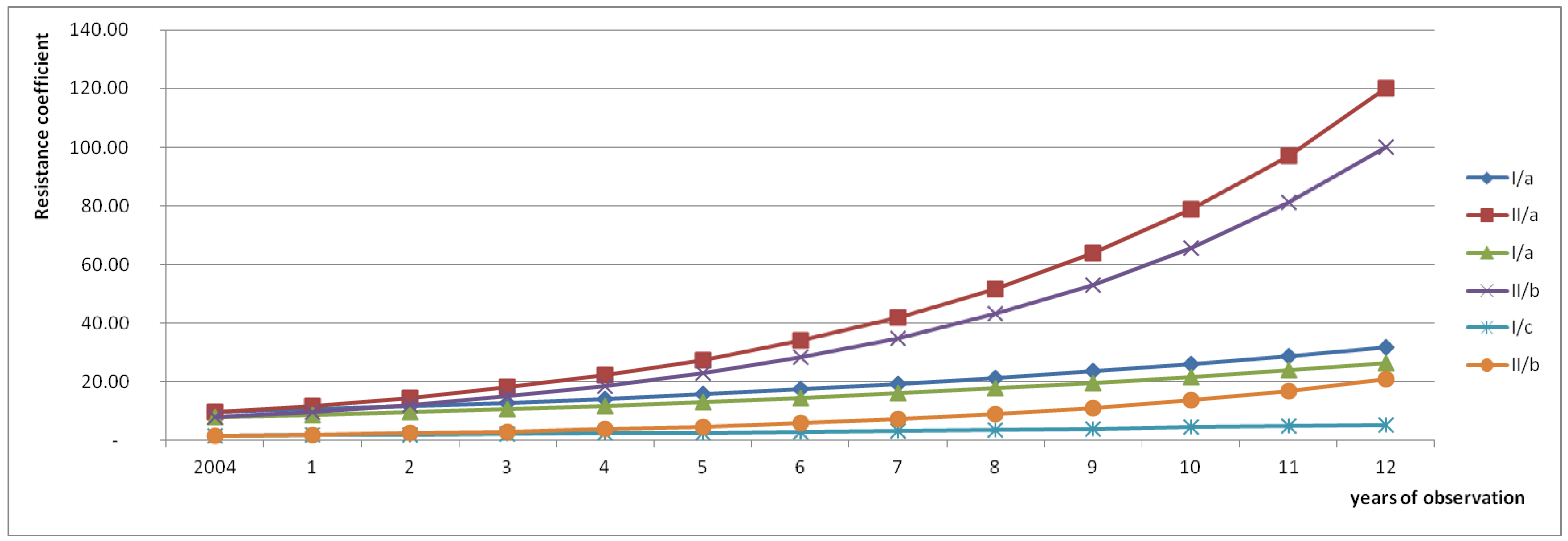
| I/a | 9.66 | 10.66 | 11.77 | 12.99 | 14.34 | 15.83 | 17.48 | 19.30 | 21.31 | 23.53 | 25.98 | 28.68 | 31.67 |
| II/a | 9.66 | 11.91 | 14.68 | 18.11 | 22.33 | 27.55 | 33.99 | 41.94 | 51.74 | 63.85 | 78.79 | 97.23 | 119.98 |
| I/b | 8.06 | 8.90 | 9.82 | 10.84 | 11.96 | 13.20 | 14.58 | 16.09 | 17.77 | 19.61 | 21.66 | 23.91 | 26.40 |
| II/b | 8.06 | 9.93 | 12.25 | 15.10 | 18.62 | 22.97 | 28.34 | 34.96 | 43.13 | 53.22 | 65.67 | 81.03 | 99.99 |
| I/c | 1.73 | 1.90 | 2.09 | 2.31 | 2.54 | 2.80 | 3.08 | 3.40 | 3.75 | 4.13 | 4.56 | 5.03 | 5.55 |
| II/c | 1.73 | 2.12 | 2.60 | 3.19 | 3.93 | 4.83 | 5.95 | 7.33 | 9.03 | 11.13 | 13.73 | 16.93 | 20.88 |
| Author | The Scope of the Study | The Range of Dimensionless Resistance Coefficient |
|---|---|---|
| Kałuża [58] | Ems-field measurements | 0.08–0.175 |
| Swiatek, D., and Wej, A. [75] | Biebrza-field measurements | 0.04–1.424 |
| Miroslaw-Swiatek, D., and Amatya, D.M. [76] | Turcja-field measurements | 0.15–0.47 |
| da Silva, et al. [77] | Brazil-field measurements | 0.38–47.67 |
| Mauricio Romero et al. [78] | Bolivia-field measurements, Riverbed slope | 0.18–7.0 |
| Gilley et al. [79] | Laboratory | 0.1–8.0 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Walczak, N.; Walczak, Z.; Kałuża, T.; Hämmerling, M.; Stachowski, P. The Impact of Shrubby Floodplain Vegetation Growth on the Discharge Capacity of River Valleys. Water 2018, 10, 556. https://doi.org/10.3390/w10050556
Walczak N, Walczak Z, Kałuża T, Hämmerling M, Stachowski P. The Impact of Shrubby Floodplain Vegetation Growth on the Discharge Capacity of River Valleys. Water. 2018; 10(5):556. https://doi.org/10.3390/w10050556
Chicago/Turabian StyleWalczak, Natalia, Zbigniew Walczak, Tomasz Kałuża, Mateusz Hämmerling, and Piotr Stachowski. 2018. "The Impact of Shrubby Floodplain Vegetation Growth on the Discharge Capacity of River Valleys" Water 10, no. 5: 556. https://doi.org/10.3390/w10050556
APA StyleWalczak, N., Walczak, Z., Kałuża, T., Hämmerling, M., & Stachowski, P. (2018). The Impact of Shrubby Floodplain Vegetation Growth on the Discharge Capacity of River Valleys. Water, 10(5), 556. https://doi.org/10.3390/w10050556





