Rainfall Detection and Rainfall Rate Estimation Using Microwave Attenuation
Abstract
1. Introduction
2. Materials
2.1. Experimental Site
2.2. Rain Gauges and Rain Detectors
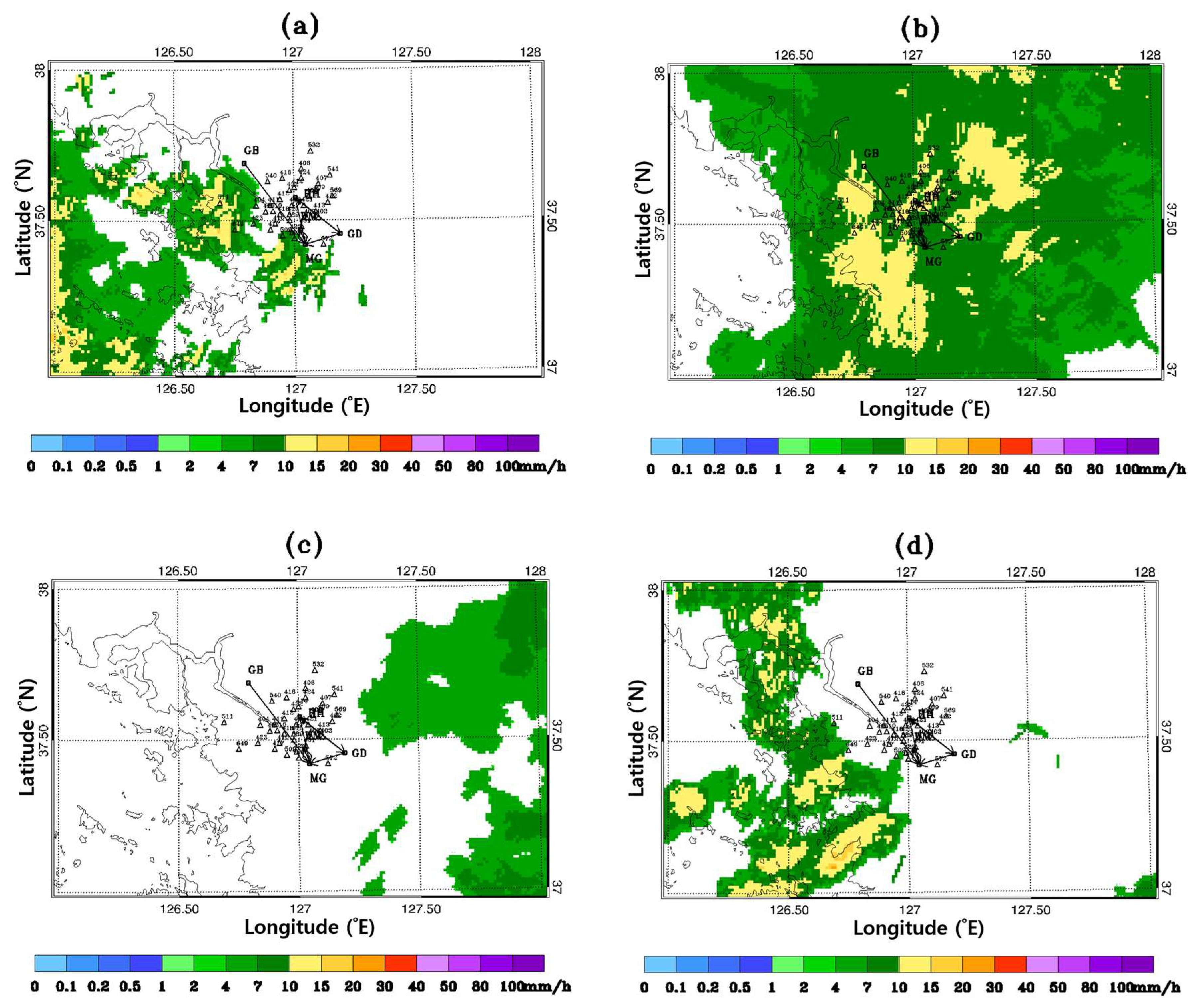
2.3. Weather Radar
2.4. Microwave Links
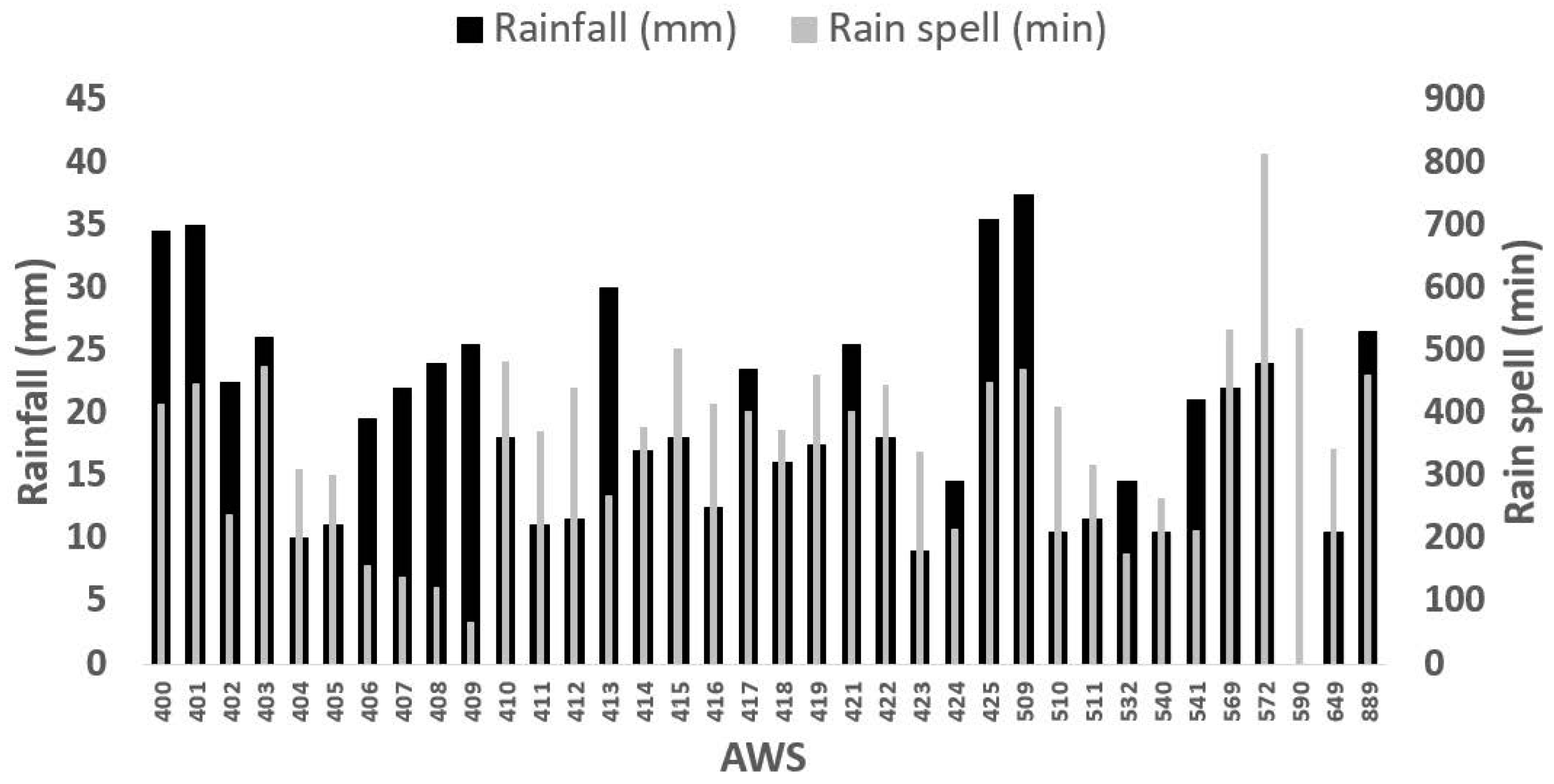
2.5. Rainfall Cases
3. Methods
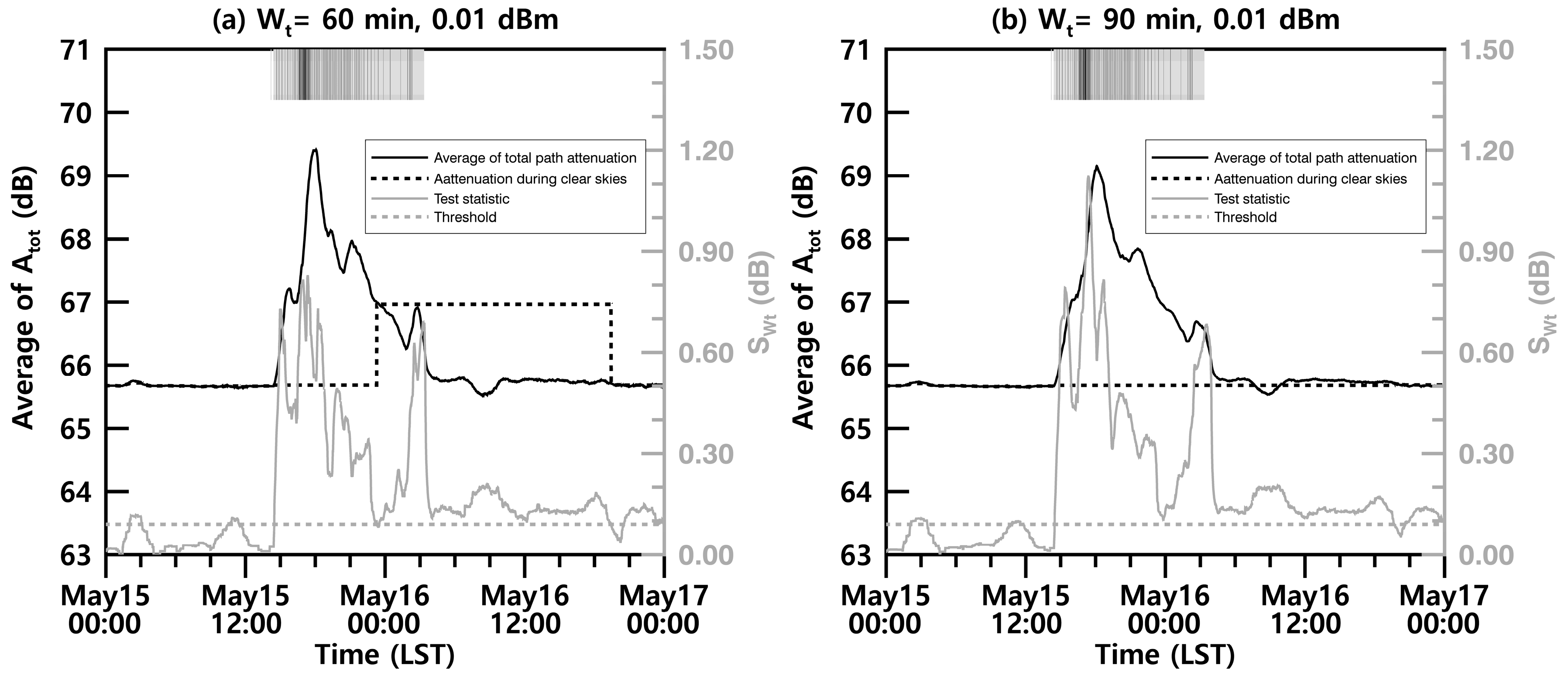
3.1. Classification of Rain and Dry Spells
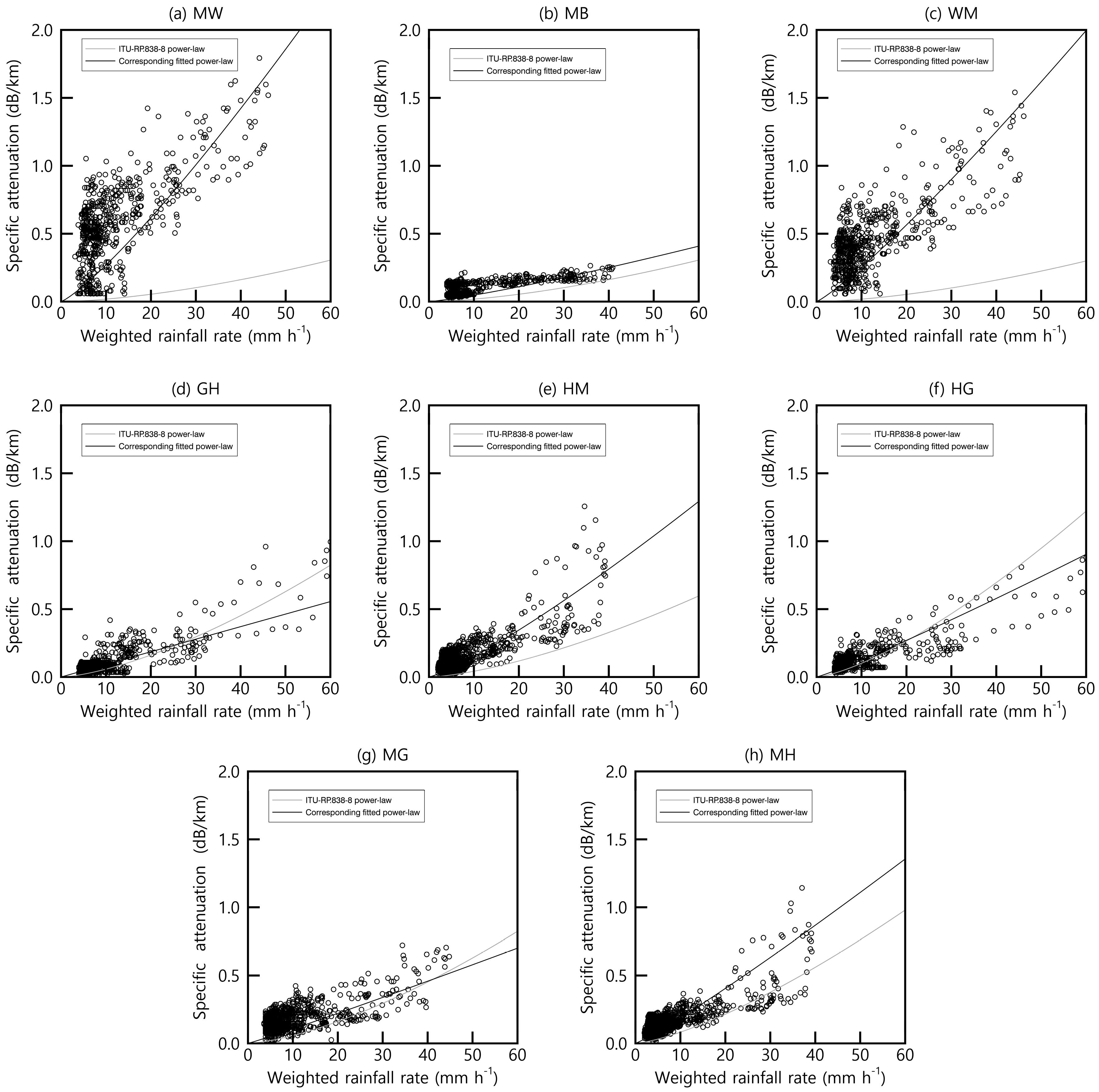
3.2. Weighted Rainfall Rate
4. Results and Discussion
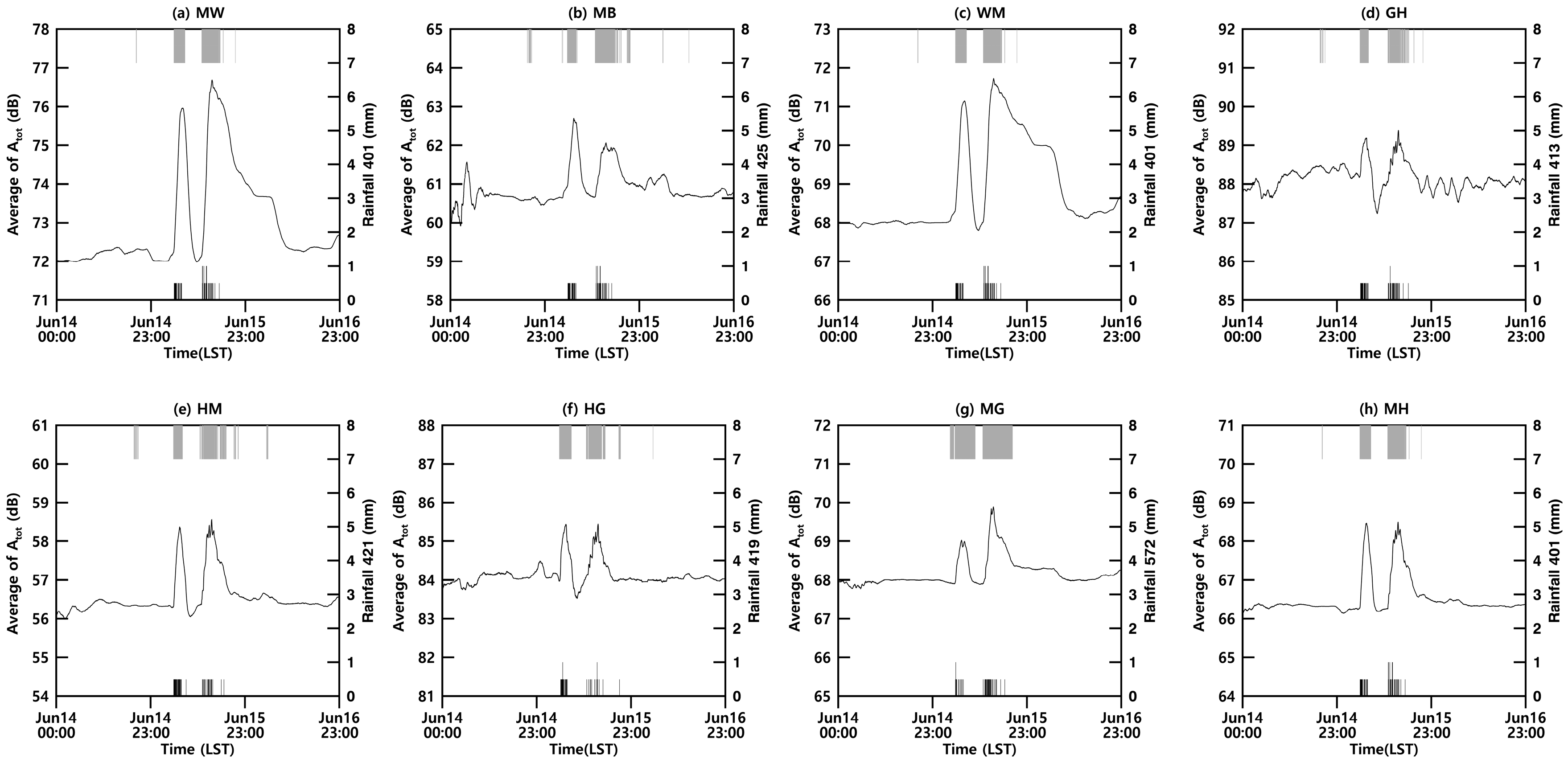
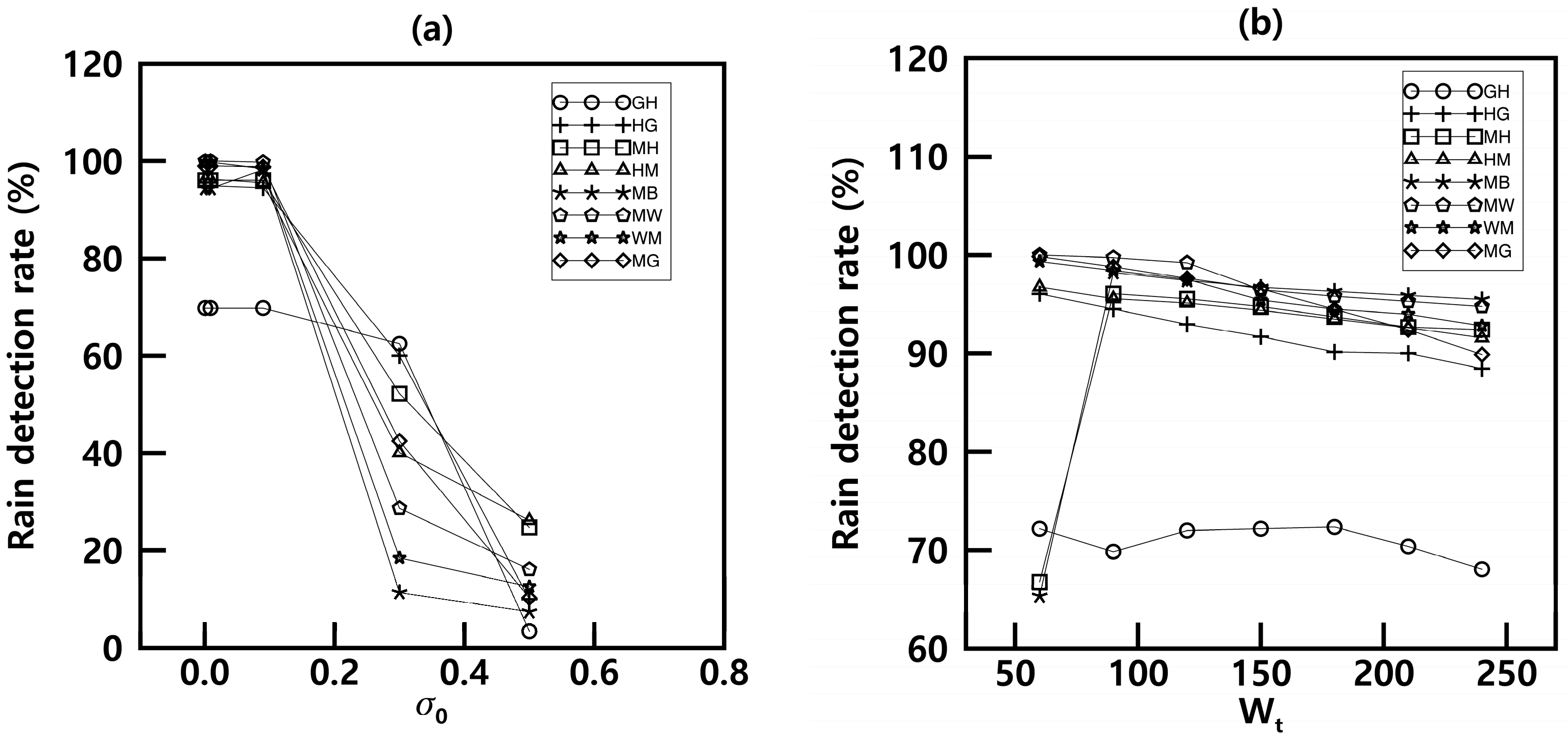
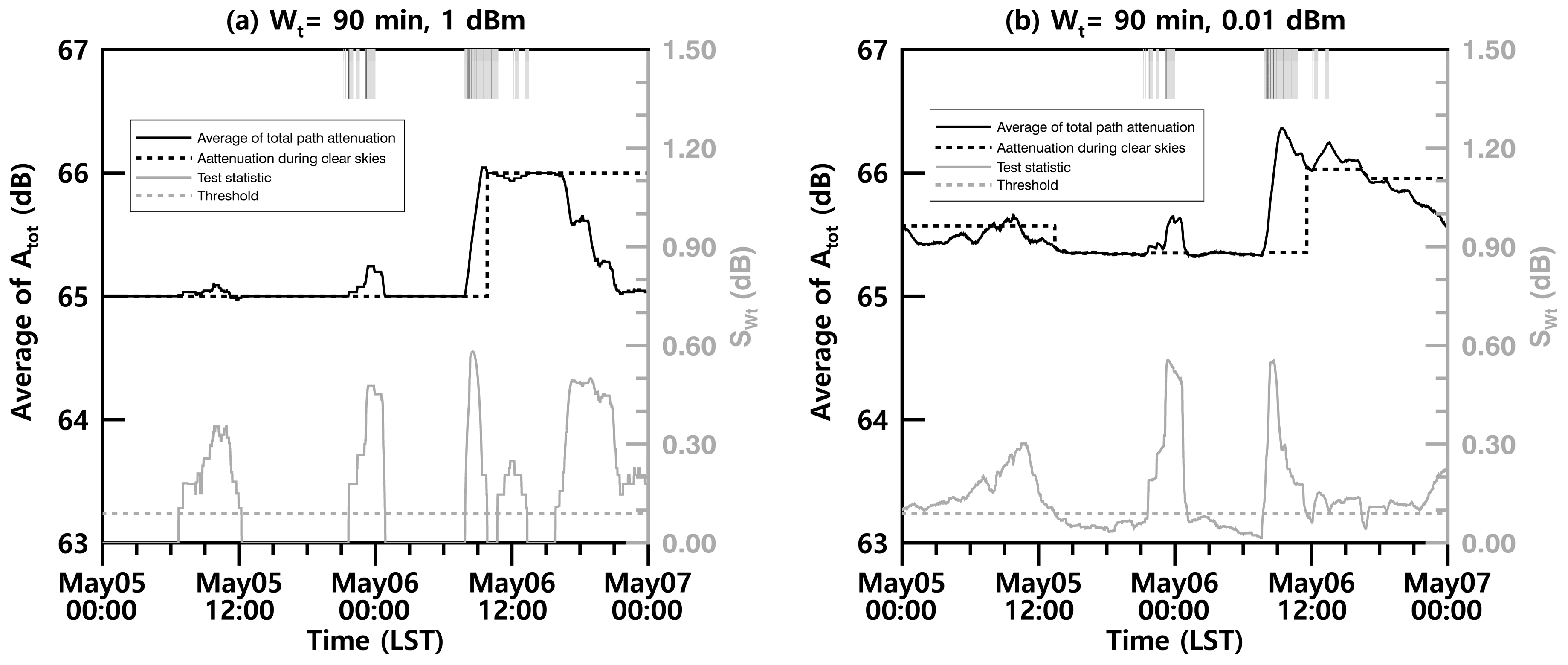
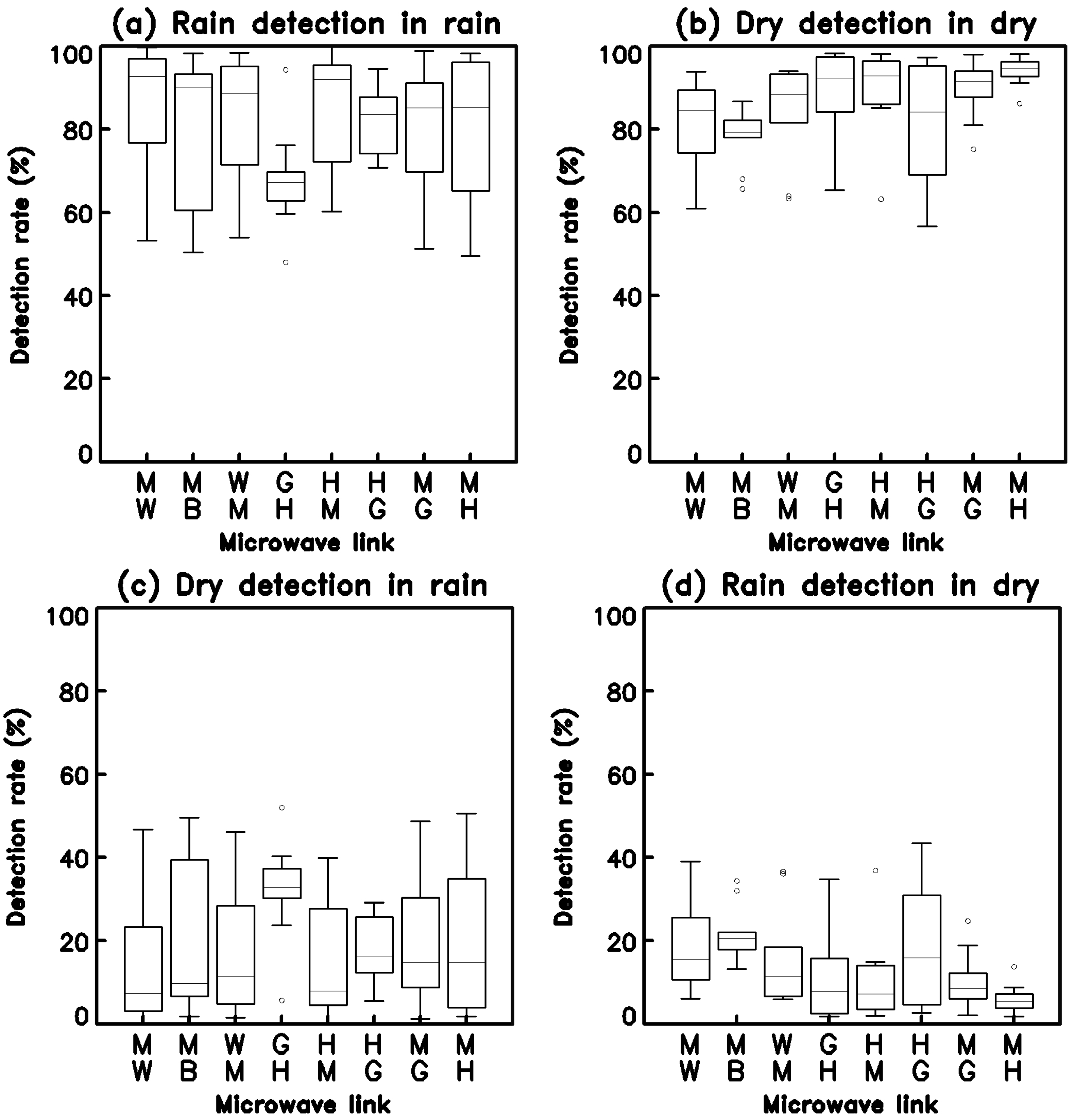
4.1. Detection of Rain and Dry Spells
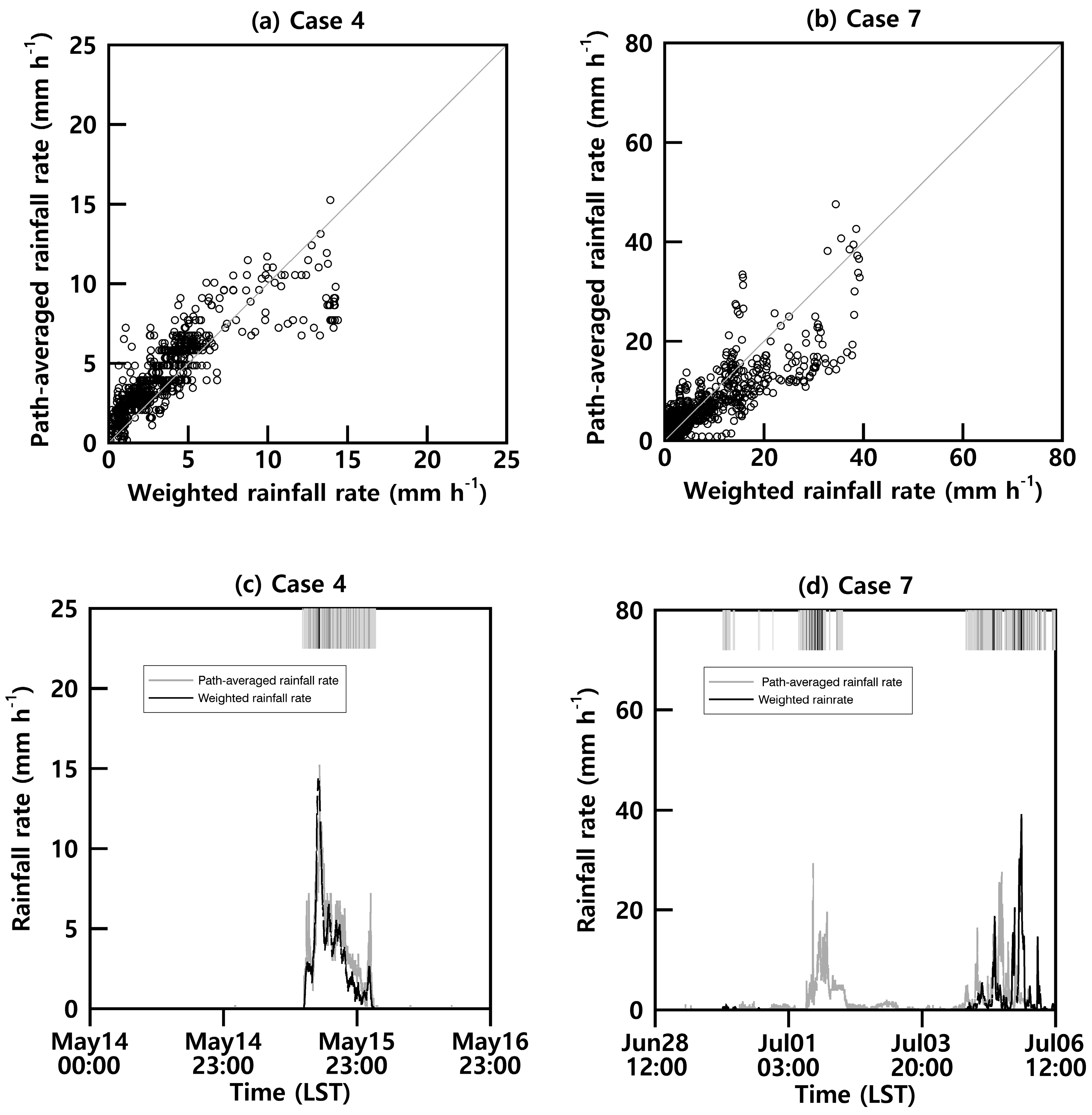
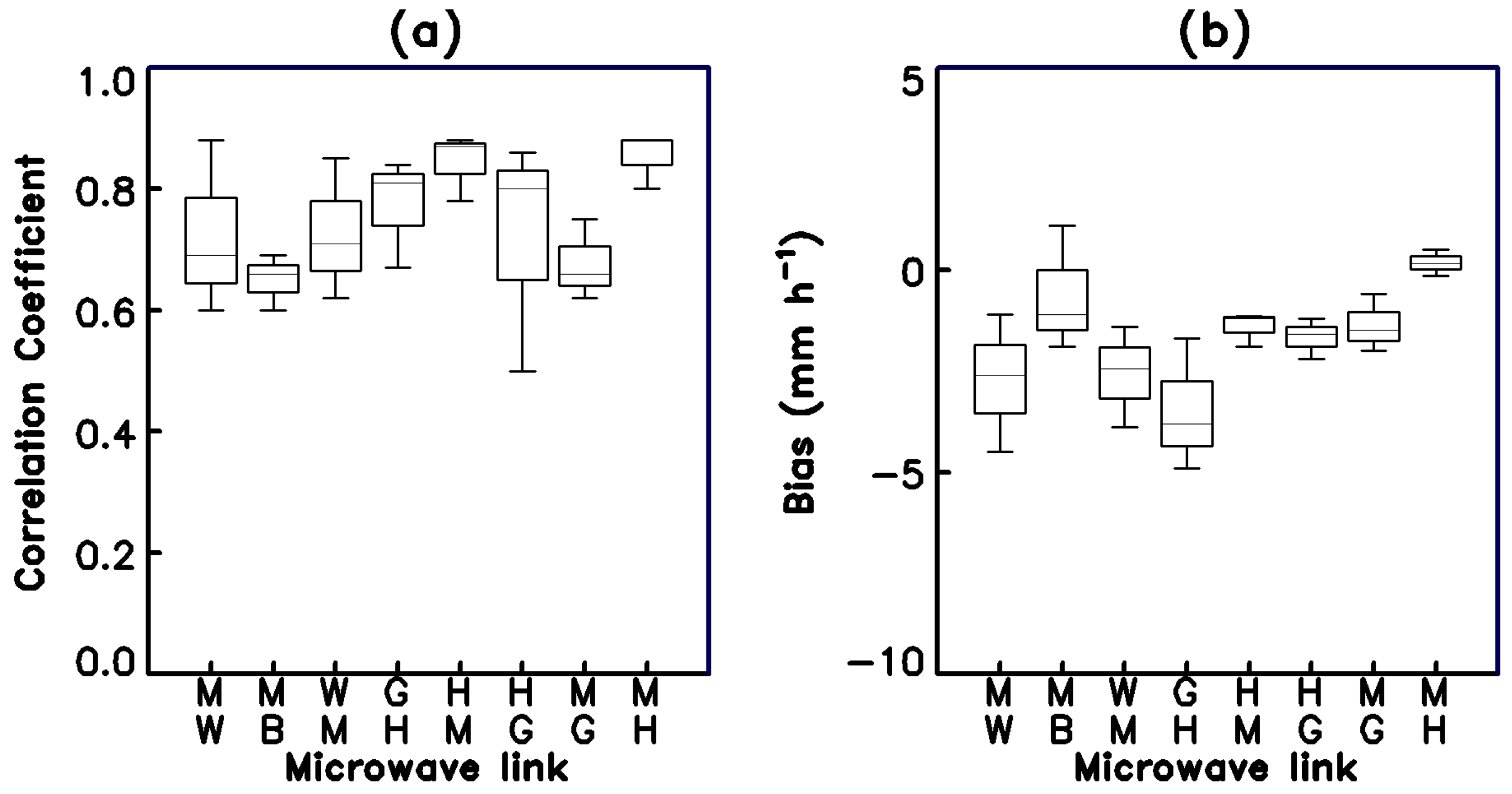
4.2. Path-Averaged Rainfall Rate
5. Conclusions
Author Contributions
Acknowledgments
Conflicts of Interest
References
- Fencl, M.; Dohnal, M.; Rieckermann, J.; Bares, V. Gauge-Adjusted Rainfall Estimates from Commercial Microwave Links. Hydrol. Earth Syst. Sci. 2017, 21, 617–634. [Google Scholar] [CrossRef]
- Rahimi, A.; Holt, A.; Upton, G.; Cummings, R. Use of Dual-Frequency Microwave Links for Measuring Path-Averaged Rainfall. J. Geophys. Res. Atmos. 2003, 108. [Google Scholar] [CrossRef]
- Fenicia, F.; Pfister, L.; Kavetski, D.; Matgen, P.; Iffly, J.; Hoffmann, L.; Uijlenhoet, R. Microwave Links for Rainfall Estimation in an Urban Environment: Insights from an Experimental Setup in Luxembourg-City. J. Hydrol. 2012, 464, 69–78. [Google Scholar] [CrossRef]
- Uijlenhoet, R.; Steiner, M.; Smith, J.A. Variability of Raindrop Size Distributions in a Squall Line and Implications for Radar Rainfall Estimation. J. Hydrometeorol. 2003, 4, 43–61. [Google Scholar] [CrossRef]
- Verrier, S.; Barthès, L.; Mallet, C. Theoretical and Empirical Scale Dependency of Z-R Relationships: Evidence, Impacts, and Correction. J. Geophys. Res. Atmos. 2013, 118, 7435–7449. [Google Scholar] [CrossRef]
- Humphrey, M.; Istok, J.; Lee, J.; Hevesi, J.; Flint, A. A New Method for Automated Dynamic Calibration of Tipping-Bucket Rain Gauges. J. Atmos. Ocean. Technol. 1997, 14, 1513–1519. [Google Scholar] [CrossRef]
- Molini, A.; Lanza, L.; La Barbera, P. The Impact of Tipping-bucket Raingauge Measurement Errors on Design Rainfall for Urban-Scale Applications. Hydrol. Process. 2005, 19, 1073–1088. [Google Scholar] [CrossRef]
- Berne, A.; Uijlenhoet, R. Path-averaged Rainfall Estimation Using Microwave Links: Uncertainty Due to Spatial Rainfall Variability. Geophys. Res. Lett. 2007, 34. [Google Scholar] [CrossRef]
- Atlas, D.; Ulbrich, C.W. Path-and Area-Integrated Rainfall Measurement by Microwave Attenuation in the 1–3 cm Band. J. Appl. Meteorol. 1977, 16, 1322–1331. [Google Scholar] [CrossRef]
- Olsen, R.; Rogers, D.V.; Hodge, D. The aR B Relation in the Calculation of Rain Attenuation. IEEE Trans. Antennas Propag. 1978, 26, 318–329. [Google Scholar] [CrossRef]
- Krämer, S.; Verworn, H.; Redder, A. Improvement of X-Band Radar Rainfall Estimates Using a Microwave Link. Atmos. Res. 2005, 77, 278–299. [Google Scholar] [CrossRef]
- Messer, H.; Zinevich, A.; Alpert, P. Environmental Monitoring by Wireless Communication Networks. Science 2006, 312, 713. [Google Scholar] [CrossRef] [PubMed]
- Overeem, A.; Leijnse, H.; Uijlenhoet, R. Measuring Urban Rainfall Using Microwave Links from Commercial Cellular Communication Networks. Water Resour. Res. 2011, 47. [Google Scholar] [CrossRef]
- Mercier, F.; Barthès, L.; Mallet, C. Estimation of Finescale Rainfall Fields using Broadcast TV Satellite Links and a 4DVAR Assimilation Method. J. Atmos. Ocean. Technol. 2015, 32, 1709–1728. [Google Scholar] [CrossRef]
- Zinevich, A.; Messer, H.; Alpert, P. Frontal Rainfall Observation by a Commercial Microwave Communication Network. J. Appl. Meteorol. Climatol. 2009, 48, 1317–1334. [Google Scholar] [CrossRef]
- Schleiss, M.; Berne, A. Identification of Dry and Rainy Periods using Telecommunication Microwave Links. IEEE Geosci. Remote Sens. Lett. 2010, 7, 611–615. [Google Scholar] [CrossRef]
- Rayitsfeld, A.; Samuels, R.; Zinevich, A.; Hadar, U.; Alpert, P. Comparison of Two Methodologies for Long Term Rainfall Monitoring Using a Commercial Microwave Communication System. Atmos. Res. 2012, 104, 119–127. [Google Scholar] [CrossRef]
- Ostrometzky, J.; Cherkassky, D.; Messer, H. Accumulated Mixed Precipitation Estimation Using Measurements from Multiple Microwave Links. Adv. Meteorol. 2015, 2015. [Google Scholar] [CrossRef]
- Minda, H.; Nakamura, K. High Temporal Resolution Path-Average Rain Gauge with 50-GHz Band Microwave. J. Atmos. Ocean. Technol. 2005, 22, 165–179. [Google Scholar] [CrossRef]
- Upton, G.; Holt, A.; Cummings, R.; Rahimi, A.; Goddard, J. Microwave Links: The Future for Urban Rainfall Measurement? Atmos. Res. 2005, 77, 300–312. [Google Scholar] [CrossRef]
- Goldshtein, O.; Messer, H.; Zinevich, A. Rain Rate Estimation Using Measurements from Commercial Telecommunications Links. IEEE Trans. Signal Process. 2009, 57, 1616–1625. [Google Scholar] [CrossRef]
- Zhang, P.; Liu, X.; Li, Z.; Zhou, Z.; Song, K.; Yang, P. Attenuation Correction of Weather Radar Reflectivity with Arbitrary Oriented Microwave Link. Adv. Meteorol. 2017, 2017. [Google Scholar] [CrossRef]
- Leijnse, H.; Uijlenhoet, R.; Stricker, J. Microwave Link Rainfall Estimation: Effects of Link Length and Frequency, Temporal Sampling, Power Resolution, and Wet Antenna Attenuation. Adv. Water Resour. 2008, 31, 1481–1493. [Google Scholar] [CrossRef]
- Overeem, A.; Leijnse, H.; Uijlenhoet, R. Country-Wide Rainfall Maps from Cellular Communication Networks. Proc. Natl. Acad. Sci. USA 2013, 110, 2741–2745. [Google Scholar] [CrossRef] [PubMed]
- Fencl, M.; Rieckermann, J.; Sýkora, P.; Stránský, D.; Bareš, V. Commercial Microwave Links Instead of Rain Gauges: Fiction or Reality? Water Sci. Technol. 2015, 71, 31–37. [Google Scholar] [CrossRef] [PubMed]
- Barthès, L.; Mallet, C. Rainfall Measurement from the Opportunistic Use of an Earth–Space Link in the Ku Band. Atmos. Meas. Tech. 2013, 6, 2181–2193. [Google Scholar] [CrossRef]
- Doumounia, A.; Gosset, M.; Cazenave, F.; Kacou, M.; Zougmore, F. Rainfall Monitoring Based on Microwave Links from Cellular Telecommunication Networks: First Results from a West African Test Bed. Geophys. Res. Lett. 2014, 41, 6016–6022. [Google Scholar] [CrossRef]
- Ramos, B.; Cordero, M.; Hurtado, K.; Núñez, A.; D’Amico, M. Rain Rate Estimation using a Microwave Link in Guayaquil City. In Proceedings of the 2017 IEEE Second Ecuador Technical Chapters Meeting (ETCM), Salinas, Ecuador, 16–20 October 2017; pp. 1–6. [Google Scholar]
- Wang, B.; Ding, Q.; Jhun, J. Trends in Seoul (1778–2004) Summer Precipitation. Geophys. Res. Lett. 2006, 33. [Google Scholar] [CrossRef]
- Jeong, S.; Kim, Y.; Lee, J.K.; Kim, J. The 27 July 2011 Debris Flows at Umyeonsan, Seoul, Korea. Landslides 2015, 12, 799–813. [Google Scholar] [CrossRef]
- Jarraud, M. Guide to Meteorological Instruments and Methods of Observation (WMO-no. 8); World Meteorological Organisation: Geneva, Switzerland, 2008. [Google Scholar]
- Park, S.; Lee, G. Calibration of Radar Reflectivity Measurements from the KMA Operational Radar Network. Asia-Pac. J. Atmos. Sci. 2010, 46, 243–259. [Google Scholar] [CrossRef]
- Leijnse, H.; Uijlenhoet, R.; Stricker, J. Rainfall Measurement Using Radio Links from Cellular Communication Networks. Water Resour. Res. 2007, 43. [Google Scholar] [CrossRef]
- Jameson, A. A Comparison of Microwave Techniques for Measuring Rainfall. J. Appl. Meteorol. 1991, 30, 32–54. [Google Scholar] [CrossRef]
- Leijnse, H.; Uijlenhoet, R.; Berne, A. Errors and Uncertainties in Microwave Link Rainfall Estimation Explored Using Drop Size Measurements and High-Resolution Radar Data. J. Hydrometeorol. 2010, 11, 1330–1344. [Google Scholar] [CrossRef]
- Kaufmann, M.; Rieckermann, J. Identification of Dry and Rainy Periods Using Telecommunication Microwave Links. In Proceedings of the 12nd International Conference on Urban Drainage, Porto Alegre, RS, Brazil, 11–16 September 2011; pp. 10–15. [Google Scholar]
- Verrier, S.; De Montera, L.; Barthès, L.; Mallet, C. Multifractal Analysis of African Monsoon Rain Fields, Taking into Account the Zero Rain-Rate Problem. J. Hydrol. 2010, 389, 111–120. [Google Scholar] [CrossRef]
- Verrier, S.; Mallet, C.; Barthès, L. Multiscaling Properties of Rain in the Time Domain, Taking into Account Rain Support Biases. J. Geophys. Res. Atmos. 2011, 116. [Google Scholar] [CrossRef]
- Szolgay, J.; Parajka, J.; Kohnová, S.; Hlavčová, K. Comparison of Mapping Approaches of Design Annual Maximum Daily Precipitation. Atmos. Res. 2009, 92, 289–307. [Google Scholar] [CrossRef]
- Ahrens, B. Distance in Spatial Interpolation of Daily Rain Gauge Data. Hydrol. Earth Syst. Sci. Discuss. 2006, 10, 197–208. [Google Scholar] [CrossRef]
- Gaona, M.R.; Overeem, A.; Leijnse, H.; Bierkens, M.; Uijlenhoet, R. Rainfall Field Estimation Using Simulated Microwave Link Information. In Proceedings of the 11th International Precipitation Conference, Ede, The Netherlands, 30 June–3 July 2013; p. 73. [Google Scholar]
- ITU-R Recommendations. 838-3. Specific Attenuation Model for Rain for Use in Prediction Methods; P Series Fasicle; ITU: Geneva, Switzerland, 2005. [Google Scholar]
- Frey, T.L. The Effects of the Atmosphere and Weather on the Performance of a mm-Wave Communication Link. Appl. Microw. Wirel. 1999, 11, 76–81. [Google Scholar]
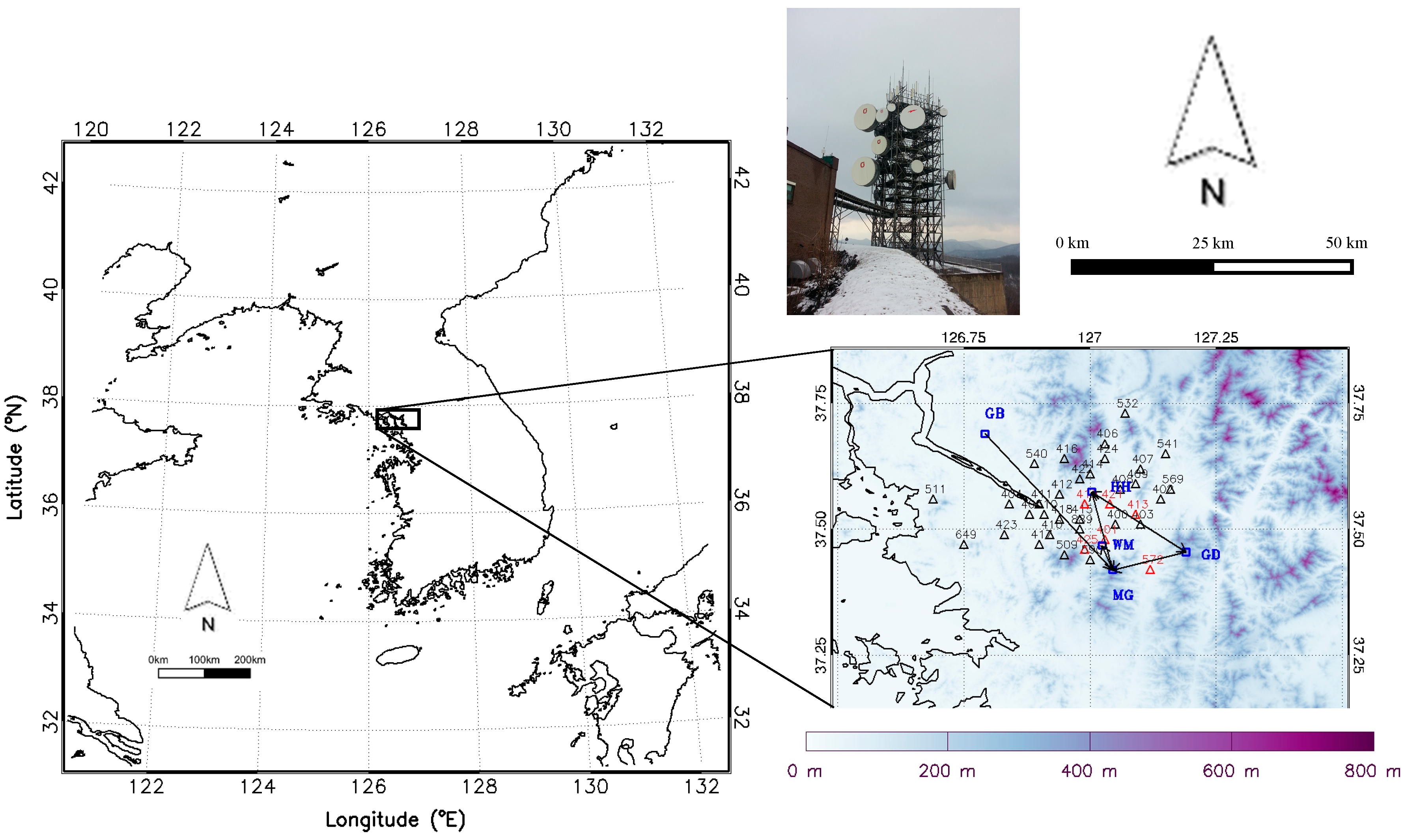
| Link Name | Receiving Antenna | Transmitting Antenna | Frequency (GHz) | Nearest AWS | Power (dBm) | Link Length (km) |
|---|---|---|---|---|---|---|
| HG | Heyhwa | Geomdan | 8.06 | 419 | 29 | 21.1 |
| GH | Geomdan | Heyhwa | 7.75 | 413 | 29 | 21.1 |
| WM | Woomyeon | Manggyeong | 6.32 | 401 | 29 | 5.7 |
| MW | Manggyeong | Woomyeon | 6.06 | 401 | 29 | 5.7 |
| MH | Manggyeong | Heyhwa | 8.26 | 401 | 30 | 17.6 |
| HM | Heyhwa | Manggyeong | 7.95 | 421 | 30 | 17.6 |
| MB | Manggyeong | Gobong | 6.23 | 425 | 30 | 37.4 |
| MG | Manggyeong | Geomdan | 8.1 | 572 | 29 | 13.4 |
| Case | Type of Rainfall Rate | Maximum Radar Rainfall Rate (mm h−1) | Total Rainfall (mm) | Rain spell from Rain Gauge (min) | Rain spell from Rain Detector (min) | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 401 | 413 | 419 | 425 | 401 | 413 | 419 | 425 | 401 | 413 | 419 | 425 | |||
| 1 | convective | 25 | 53 | 56 | 44 | 73 | 106 | 111 | 88 | 145 | 1383 | 966 | 1213 | 1372 |
| 2 | stratiform | 9 | 9 | 10 | 13 | 8 | 17 | 19 | 26 | 16 | 305 | 214 | 565 | 395 |
| 3 | stratiform | 5 | 14 | 14 | 6 | 16 | 28 | 27 | 12 | 32 | 621 | 456 | 318 | 666 |
| 4 | convective | 20 | 45 | 42 | 42 | 55 | 89 | 84 | 83 | 110 | 764 | 557 | 676 | 730 |
| 5 | stratiform | 8 | 31 | 31 | 36 | 42 | 61 | 62 | 71 | 83 | 644 | 525 | 635 | 626 |
| 6 | convective | 20 | 35 | 30 | 18 | 36 | 63 | 59 | 33 | 67 | 447 | 268 | 461 | 450 |
| 7 | convective | 50 | 115 | 119 | 57 | 134 | 180 | 238 | 109 | 206 | 1006 | 554 | 1076 | 967 |
| 8 | convective | 50 | 138 | 155 | 124 | 154 | 244 | 255 | 196 | 254 | 1764 | 820 | 1033 | 1509 |
| 9 | stratiform | 7 | 47 | 48 | 31 | 64 | 93 | 95 | 62 | 126 | 1092 | 472 | 843 | 1371 |
| 10 | convective | 80 | 23 | 38 | 33 | 33 | 39 | 44 | 50 | 56 | 332 | 137 | 254 | 386 |
| Case | Data Resolution | Rain Detector | RR (%) | DD (%) | RD (%) | DR (%) | |||
|---|---|---|---|---|---|---|---|---|---|
| 4 | 60 min | 0.01 dB | 0.09 | 764 | 526 | 68.8 | 97.8 | 28.9 | 0.4 |
| 90 min | 725 | 97.9 | 93.6 | 2.1 | 4.6 |
| Case | Data Resolution | Rain Detector | RR (%) | DD (%) | RD (%) | DR (%) | |||
|---|---|---|---|---|---|---|---|---|---|
| 2 | 1 dB | 90 min | 0.09 | 305 | 134 | 43.9 | 97.7 | 56.1 | 0.8 |
| 0.01 dB | 248 | 81.3 | 88.3 | 18.7 | 10.2 |
| Link Name | Frequency (GHz) | Polarization | Path Length (km) | R-k Relationships | ITU-R P.838-8 R-k Relationships |
|---|---|---|---|---|---|
| GH | 7.75 | H | 21.1 | ||
| HG | 8.06 | H | 21.1 | ||
| MG | 8.1 | H | 13.4 | ||
| WM | 6.32 | V | 5.7 | ||
| MW | 6.06 | V | 5.7 | ||
| MB | 6.23 | V | 37.4 | ||
| MH | 8.26 | V | 17.6 | ||
| HM | 7.95 | V | 17.6 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kim, M.-S.; Kwon, B.H. Rainfall Detection and Rainfall Rate Estimation Using Microwave Attenuation. Atmosphere 2018, 9, 287. https://doi.org/10.3390/atmos9080287
Kim M-S, Kwon BH. Rainfall Detection and Rainfall Rate Estimation Using Microwave Attenuation. Atmosphere. 2018; 9(8):287. https://doi.org/10.3390/atmos9080287
Chicago/Turabian StyleKim, Min-Seong, and Byung Hyuk Kwon. 2018. "Rainfall Detection and Rainfall Rate Estimation Using Microwave Attenuation" Atmosphere 9, no. 8: 287. https://doi.org/10.3390/atmos9080287
APA StyleKim, M.-S., & Kwon, B. H. (2018). Rainfall Detection and Rainfall Rate Estimation Using Microwave Attenuation. Atmosphere, 9(8), 287. https://doi.org/10.3390/atmos9080287





